Significance of the Coriolis Force on the Dynamics of Carreau–Yasuda Rotating Nanofluid Subject to Darcy–Forchheimer and Gyrotactic Microorganisms
Abstract
:1. Introduction
2. Mathematical Model
3. Numerical Solution and Validation of Results
Validation of Results
4. Analysis and Discussion of Results
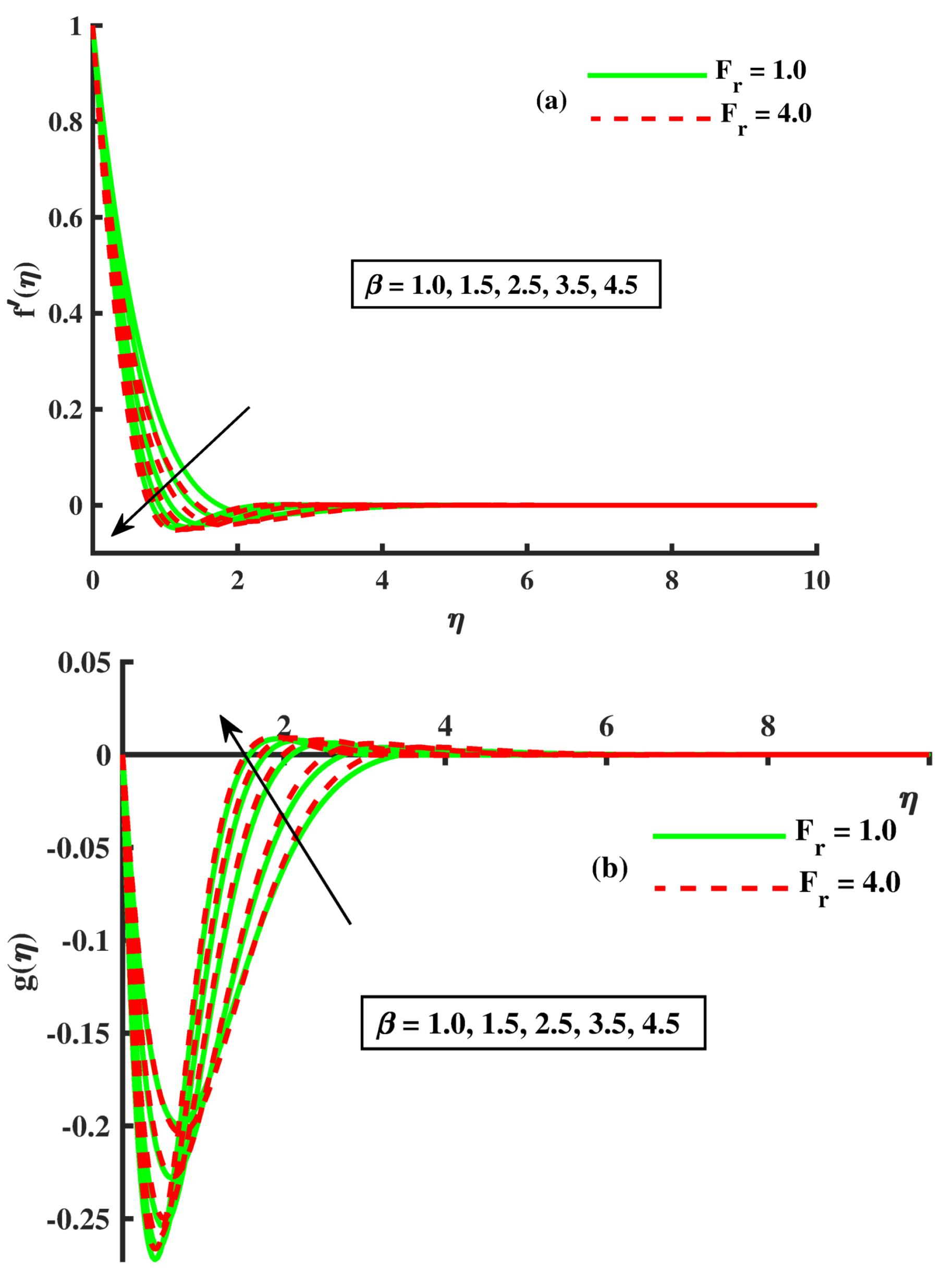
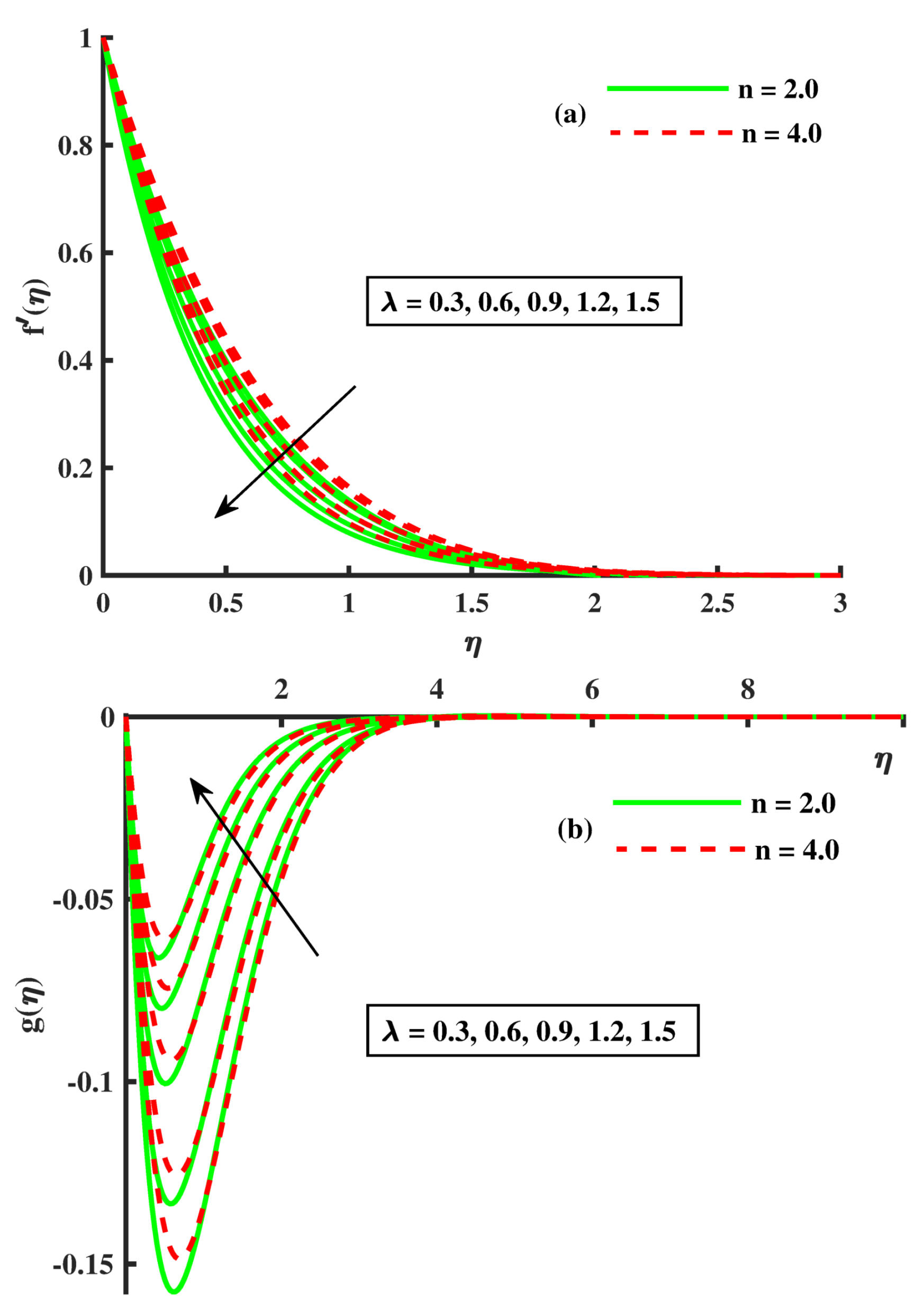
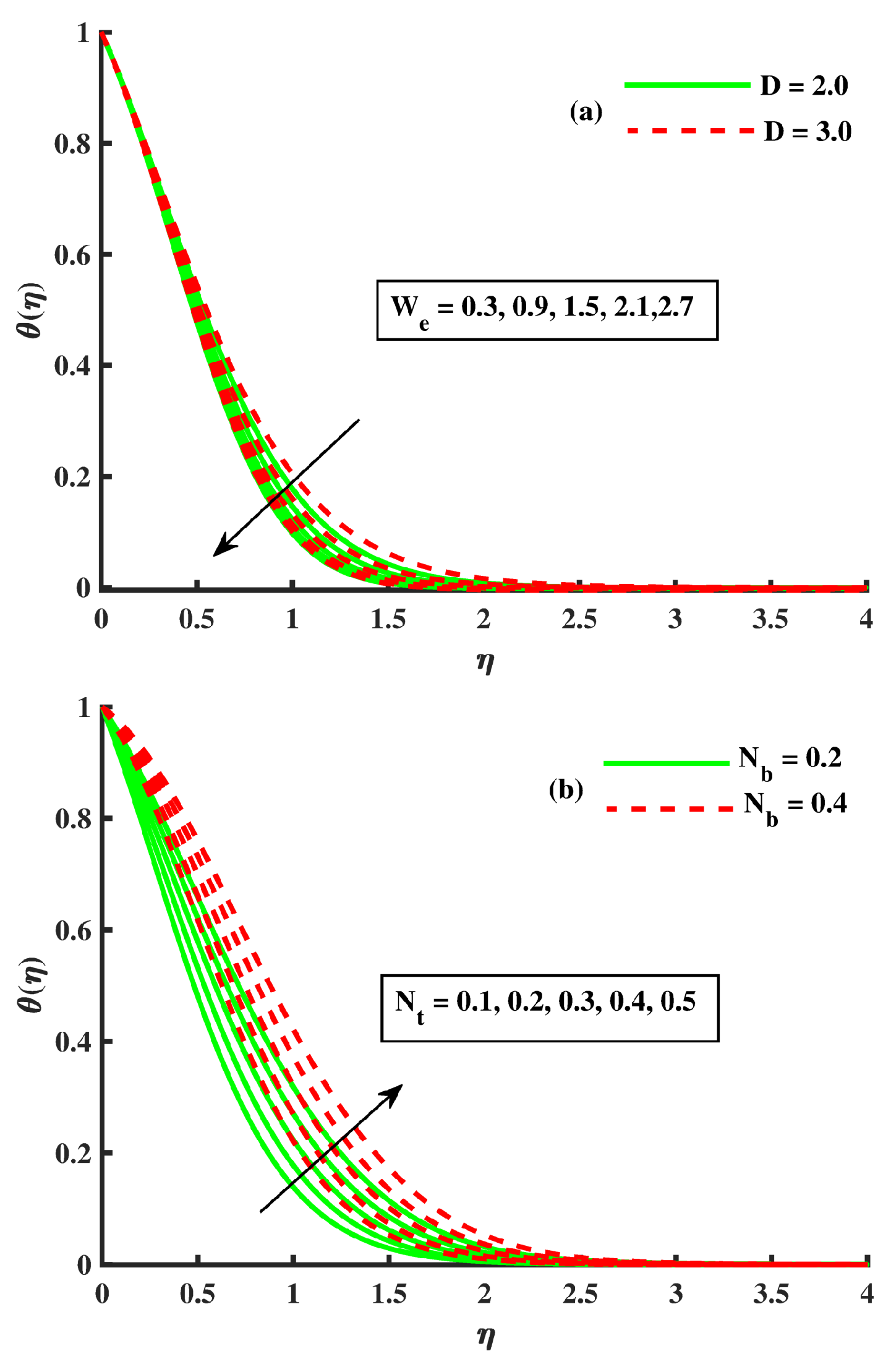
4.1. Analysis of the Results
4.2. Discussion of the Results
5. Conclusions
- The velocity profile along the x-axis has negative behavior for the boosted values of , , D, and
- The velocity profile along the y-axis has negative behavior for the boosted values of , , and
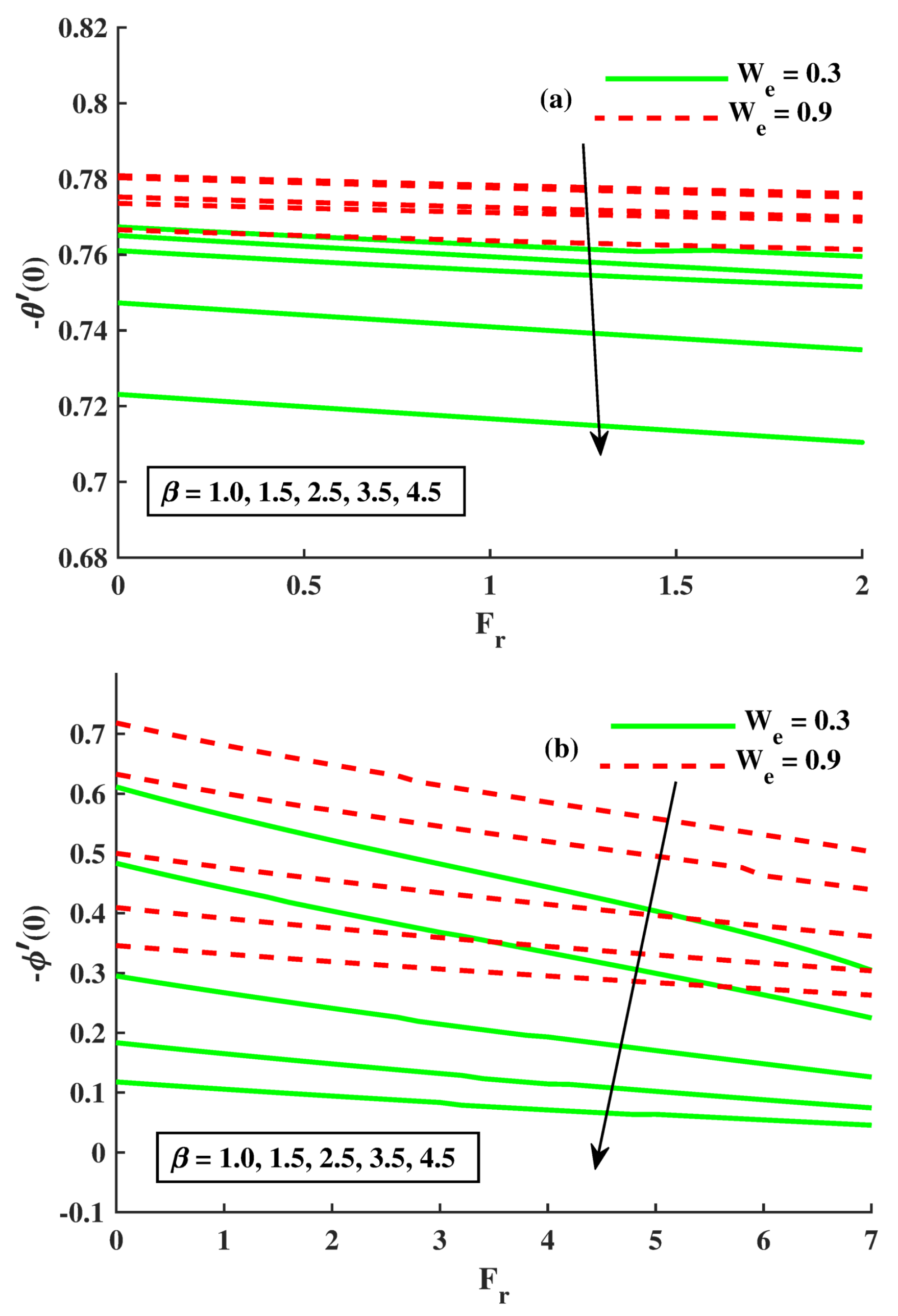
- The temperature profile of the nanofluid was enhanced for the boosted value of and , with the decreasing behavior nature for the large value of
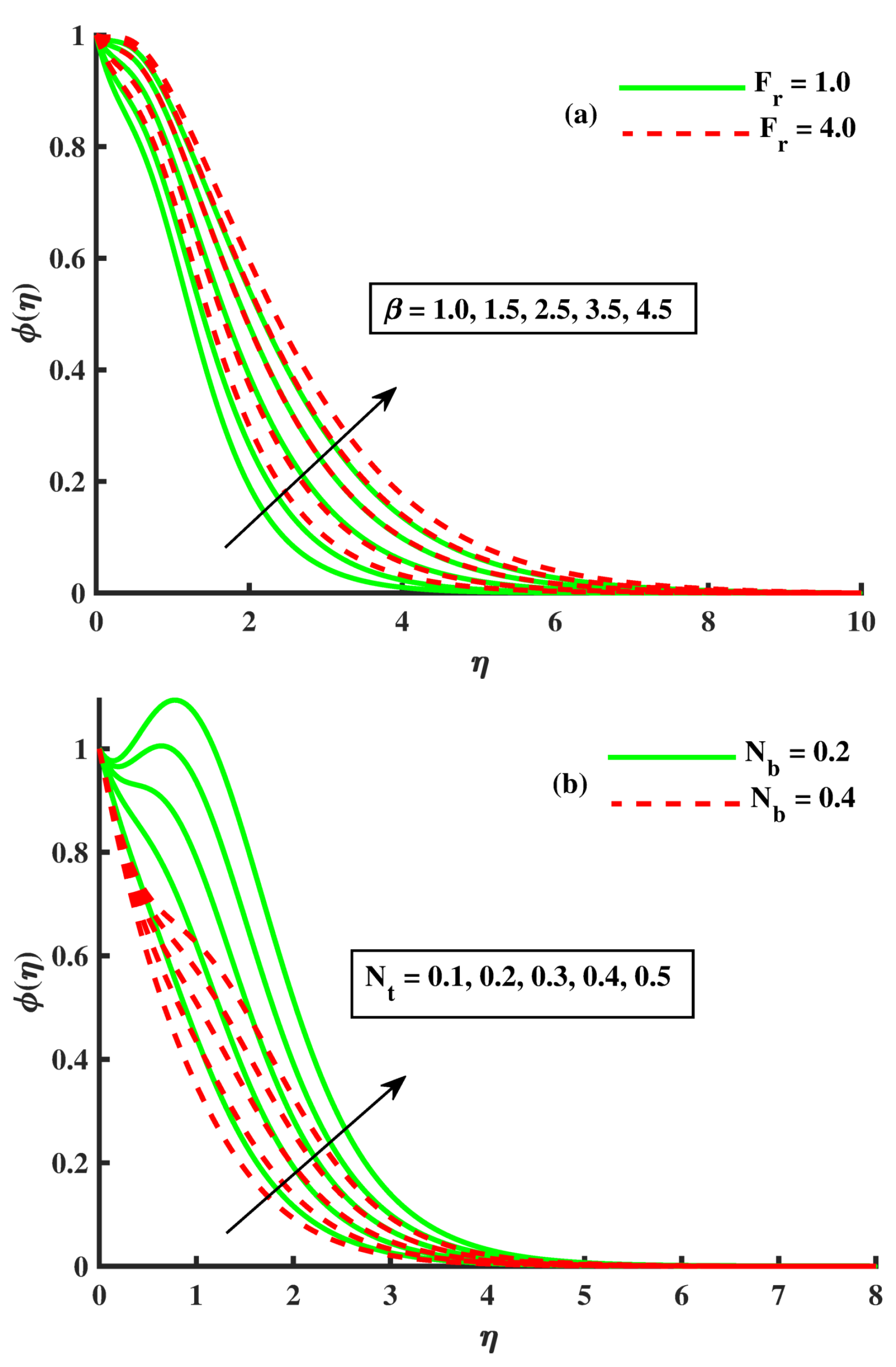
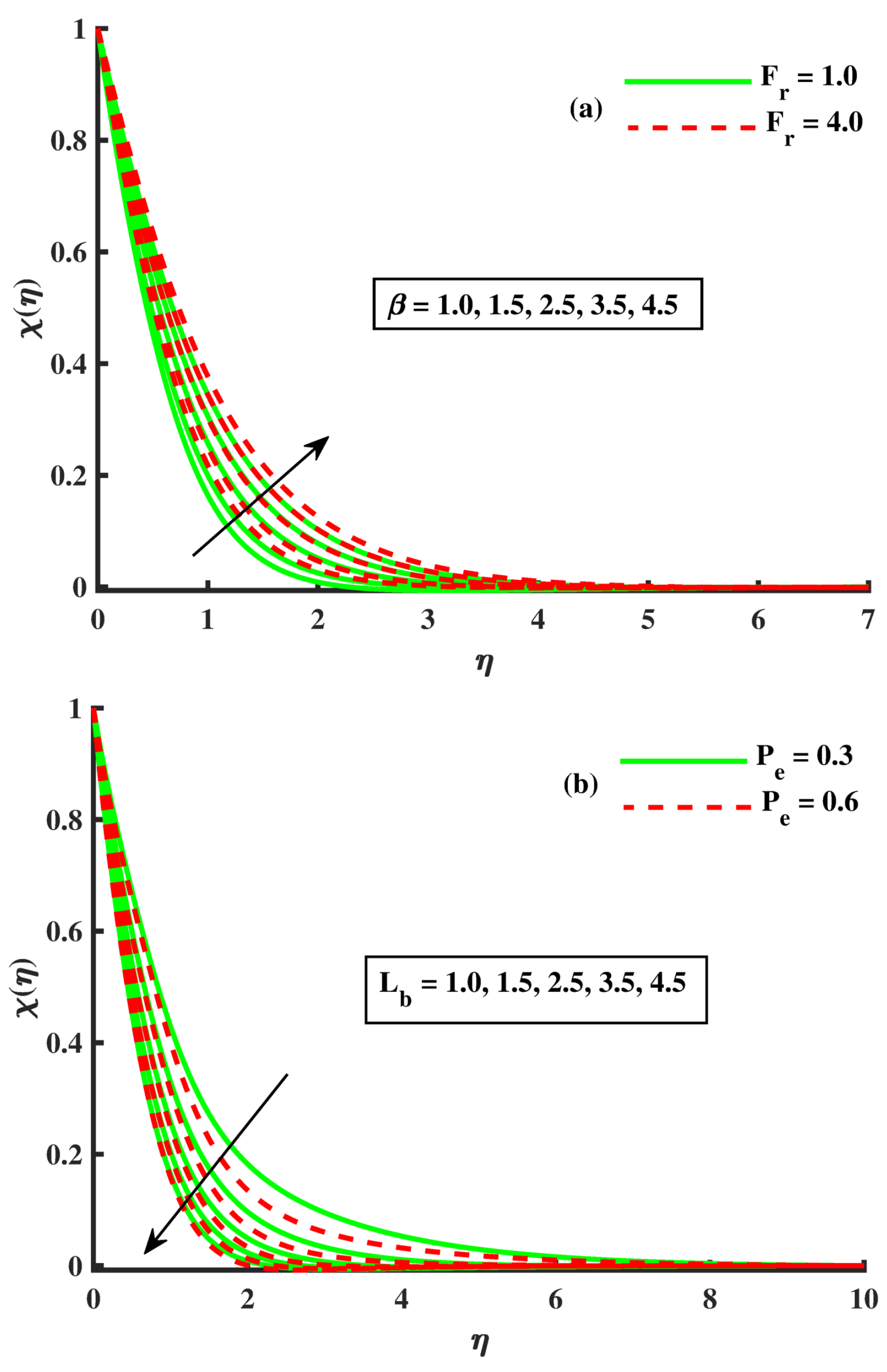
- The concentration of the nanofluid increased for the boosted value of , , , and we observed opposite behavior for .
- Regarding the profile of the micro-motile organism, there was an ’increasing nature’ for the enhanced value of , and we observed ’decreasing behavior’ for the boosted value of &
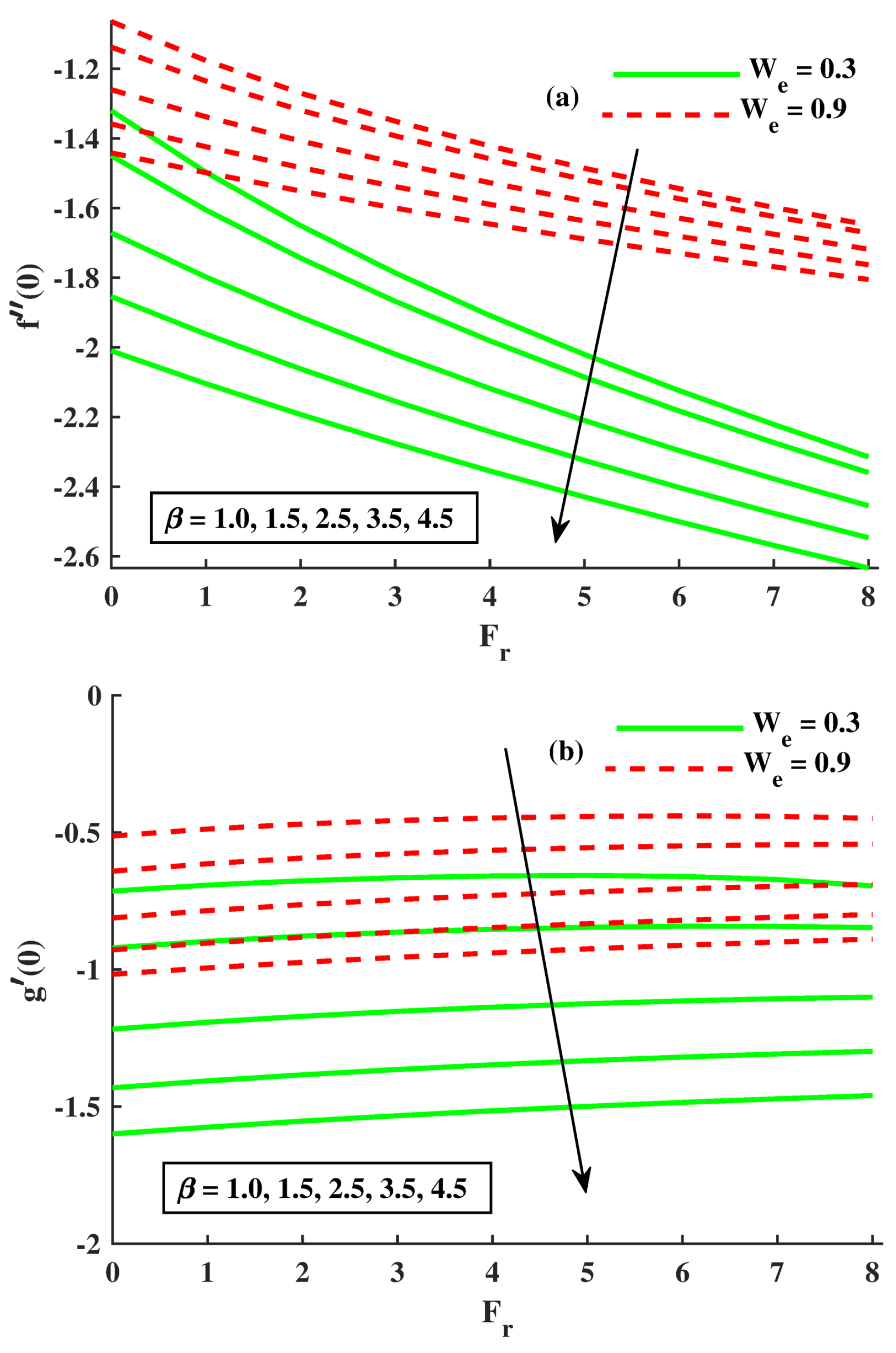
- The values of the skin friction coefficients increased with the increase of and the decrease of & .
- The Sherwood number increment was observed for the boosted values of , and was opposite to & .
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| T | non-dimensional temperature |
| C | non-dimensional nanoparticles concentration |
| temperature away from the surface | |
| concentration away from the surface | |
| skin friction at x-direction | |
| skin friction at y-direction | |
| Nusselt number | |
| Brownian motion parameter | |
| thermophoresis parameter | |
| thermophoretic diffusion coefficient | |
| Base fluid heat capacity | |
| p | pressure |
| Cartesian coordinates | |
| angle of inclination | |
| Lewis number parameter | |
| temperature at surface | |
| concentration at surface | |
| E | activation energy |
| Schmidt number | |
| velocity components | |
| velocity of stretching sheet | |
| Sherwood number | |
| kinematic viscosity | |
| density of fluid | |
| Brownian diffusion coefficient | |
| M | uniform magnetic field |
| rate of chemical reaction | |
| rotational parameter parameter | |
| Prandtl number |
References
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; Technical Report; Argonne National Laboratory: Lemont, IL, USA, 1995. [Google Scholar]
- Xuan, Y.; Li, Q. Heat transfer enhancement of nanofluids. Int. J. Heat Fluid Flow 2000, 21, 58–64. [Google Scholar] [CrossRef]
- Niranjana, N.; Vidhya, M.; Govindarajan, A.; Priyadarshini, E. Heat and mass transfer effect between vertical plates through porous medium and chemical reaction effect on free convective MHD flow with soret effect. J. Phys. Conf. Ser. 2021, 1770, 12054. [Google Scholar] [CrossRef]
- Mahian, O.; Kianifar, A.; Kleinstreuer, C.; Moh’d A, A.N.; Pop, I.; Sahin, A.Z.; Wongwises, S. A review of entropy generation in nanofluid flow. Int. J. Heat Mass Transf. 2013, 65, 514–532. [Google Scholar] [CrossRef]
- Jothia, E. Performance of Dufour Effect on Unsteady MHD Flow past through Porous Medium an Exponentially Accelerated Inclined Vertical plate with Variable Temperature and Mass Diffusion. Turk. J. Comput. Math. Educ. (TURCOMAT) 2021, 12, 3457–3463. [Google Scholar]
- Dawar, A.; Wakif, A.; Thumma, T.; Shah, N.A. Towards a new MHD non-homogeneous convective nanofluid flow model for simulating a rotating inclined thin layer of sodium alginate-based Iron oxide exposed to incident solar energy. Int. Commun. Heat Mass Transf. 2022, 130, 105800. [Google Scholar] [CrossRef]
- Ashraf, M.Z.; Rehman, S.U.; Farid, S.; Hussein, A.K.; Ali, B.; Shah, N.A.; Weera, W. Insight into Significance of Bioconvection on MHD Tangent Hyperbolic Nanofluid Flow of Irregular Thickness across a Slender Elastic Surface. Mathematics 2022, 10, 2592. [Google Scholar] [CrossRef]
- Shah, S.A.A.; Ahammad, N.A.; Din, E.M.T.E.; Gamaoun, F.; Awan, A.U.; Ali, B. Bio-Convection Effects on Prandtl Hybrid Nanofluid Flow with Chemical Reaction and Motile Microorganism over a Stretching Sheet. Nanomaterials 2022, 12, 2174. [Google Scholar] [CrossRef]
- Awan, A.U.; Ahammad, N.A.; Majeed, S.; Gamaoun, F.; Ali, B. Significance of hybrid nanoparticles, Lorentz and Coriolis forces on the dynamics of water based flow. Int. Commun. Heat Mass Transf. 2022, 135, 106084. [Google Scholar] [CrossRef]
- Wu, B.; Chen, S. CFD simulation of non-Newtonian fluid flow in anaerobic digesters. Biotechnol. Bioeng. 2008, 99, 700–711. [Google Scholar] [CrossRef]
- Sankar, D.; Hemalatha, K. A non-Newtonian fluid flow model for blood flow through a catheterized artery—Steady flow. Appl. Math. Model. 2007, 31, 1847–1864. [Google Scholar] [CrossRef]
- Kumar, D.; Sahu, A.K. Non-Newtonian fluid flow over a rotating elliptic cylinder in laminar flow regime. Eur. J. Mech.-B/Fluids 2022, 93, 117–136. [Google Scholar] [CrossRef]
- Abbas Khan, A.; Naveed Khan, M.; Irfan Shah, S.; Ashraf, M.; Matoog, R. Heat and mass transfer exploration of non-Newtonian fluid flow induced by the exponentially stretching Riga surface with the application of Generalized Fourier’s and Fick’s law. In Waves Random Complex Media; Taylor & Francis: Abingdon, UK, 2022; pp. 1–16. [Google Scholar]
- Kumar, A.; Ridha, S.; Narahari, M.; Ilyas, S.U. Physics-guided deep neural network to characterize non-Newtonian fluid flow for optimal use of energy resources. Expert Syst. Appl. 2021, 183, 115409. [Google Scholar] [CrossRef]
- Sarada, K.; Gowda, R.J.P.; Sarris, I.E.; Kumar, R.N.; Prasannakumara, B.C. Effect of magnetohydrodynamics on heat transfer behaviour of a non-Newtonian fluid flow over a stretching sheet under local thermal non-equilibrium condition. Fluids 2021, 6, 264. [Google Scholar] [CrossRef]
- Bilal, M.; Urva, Y. Analysis of non-Newtonian fluid flow over fine rotating thin needle for variable viscosity and activation energy. Arch. Appl. Mech. 2021, 91, 1079–1095. [Google Scholar] [CrossRef]
- Khan, Z.H.; Khan, W.A.; Hamid, M. Non-Newtonian fluid flow around a Y-shaped fin embedded in a square cavity. J. Therm. Anal. Calorim. 2021, 143, 573–585. [Google Scholar] [CrossRef]
- Xia, W.F.; Animasaun, I.; Wakif, A.; Shah, N.A.; Yook, S.J. Gear-generalized differential quadrature analysis of oscillatory convective Taylor-Couette flows of second-grade fluids subject to Lorentz and Darcy–Forchheimer quadratic drag forces. Int. Commun. Heat Mass Transf. 2021, 126, 105395. [Google Scholar] [CrossRef]
- Habib, D.; Salamat, N.; Abdal, S.H.S.; Ali, B. Numerical investigation for MHD Prandtl nanofluid transportation due to a moving wedge: Keller box approach. Int. Commun. Heat Mass Transf. 2022, 135, 106141. [Google Scholar] [CrossRef]
- Ali, L.; Ali, B.; Liu, X.; Iqbal, T.; Zulqarnain, R.M.; Javid, M. A comparative study of unsteady MHD Falkner–Skan wedge flow for non-Newtonian nanofluids considering thermal radiation and activation energy. Chin. J. Phys. 2022, 77, 1625–1638. [Google Scholar] [CrossRef]
- Borré, G.; Maier, G. On linear versus nonlinear flow rules in strain localization analysis. Meccanica 1989, 24, 36–41. [Google Scholar] [CrossRef]
- Rasool, G.; Shafiq, A.; Khalique, C.M.; Zhang, T. Magnetohydrodynamic Darcy-Forchheimer nanofluid flow over a nonlinear stretching sheet. Phys. Scr. 2019, 94, 105221. [Google Scholar] [CrossRef]
- Singh, T.; Atieh, M.A.; Al-Ansari, T.; Mohammad, A.W.; McKay, G. The role of nanofluids and renewable energy in the development of sustainable desalination systems: A review. Water 2020, 12, 2002. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Khan, T.A.; Khan, M.I.; Ahmad, S.; Alsaedi, A. Entropy generation in Darcy-Forchheimer bidirectional flow of water-based carbon nanotubes with convective boundary conditions. J. Mol. Liq. 2018, 265, 629–638. [Google Scholar] [CrossRef]
- Shaw, S.; Dogonchi, A.; Nayak, M.; Makinde, O. Impact of entropy generation and nonlinear thermal radiation on Darcy–Forchheimer flow of MnFe2 O4 -Casson/water nanofluid due to a rotating disk: Application to brain dynamics. Arab. J. Sci. Eng. 2020, 45, 5471–5490. [Google Scholar] [CrossRef]
- Shafiq, A.; Rasool, G.; Khalique, C.M. Significance of thermal slip and convective boundary conditions in three dimensional rotating Darcy-Forchheimer nanofluid flow. Symmetry 2020, 12, 741. [Google Scholar] [CrossRef]
- Waqas, H.; Yasmin, S.; Althobaiti, N.; Bonyah, E.; Alshehri, A.; Shah, Z. Evaluating the Higher-Order Slip Consequence in Bioconvection Nanofluid Flow Configured by a Variable Thick Surface of Disk. J. Nanomater. 2022, 2022, 2766317. [Google Scholar] [CrossRef]
- Farooq, U.; Waqas, H.; Shah, Z.; Kumam, P.; Deebani, W. On unsteady 3D bio-convection flow of viscoelastic nanofluid with radiative heat transfer inside a solar collector plate. Sci. Rep. 2022, 12, 2952. [Google Scholar] [CrossRef]
- Ali, B.; Shafiq, A.; Manan, A.; Wakif, A.; Hussain, S. Bioconvection: Significance of mixed convection and mhd on dynamics of Casson nanofluid in the stagnation point of rotating sphere via finite element simulation. Math. Comput. Simul. 2022, 194, 254–268. [Google Scholar] [CrossRef]
- Yin, J.; Zhang, X.; Rehman, M.I.U.; Hamid, A. Thermal radiation aspect of bioconvection flow of magnetized Sisko nanofluid along a stretching cylinder with swimming microorganisms. Case Stud. Therm. Eng. 2022, 30, 101771. [Google Scholar] [CrossRef]
- Nabwey, H.A.; Alshber, S.I.; Rashad, A.M.; Mahdy, A.E.N. Influence of Bioconvection and Chemical Reaction on Magneto-Carreau Nanofluid Flow through an Inclined Cylinder. Mathematics 2022, 10, 504. [Google Scholar] [CrossRef]
- Ali, B.; Siddique, I.; Shafiq, A.; Abdal, S.; Khan, I. and Khan, A. Magnetohydrodynamic mass and heat transport over a stretching sheet in a rotating nanofluid with binary chemical reaction, non-fourier heat flux, and swimming microorganisms. Case Stud. Therm. Eng. 2021, 28, 101367. [Google Scholar] [CrossRef]
- Tayebi, T. Analysis of the local non-equilibria on the heat transfer and entropy generation during thermal natural convection in a non-Darcy porous medium. Int. Commun. Heat Mass Transf. 2022, 135, 106133. [Google Scholar] [CrossRef]
- Nima, N.I.; Salawu, S.; Ferdows, M.; Shamshuddin, M.; Alsenafi, A.; Nakayama, A. Melting effect on non-Newtonian fluid flow in gyrotactic microorganism saturated non-darcy porous media with variable fluid properties. Appl. Nanosci. 2020, 10, 3911–3924. [Google Scholar] [CrossRef]
- Rashid, S.; Hayat, T.; Qayyum, S.; Ayub, M.; Alsaedi, A. Three-dimensional rotating Darcy-Forchheimer flow with activation energy. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 935–948. [Google Scholar] [CrossRef]
- Rana, S.; Nawaz, M.; Alaoui, M. Three dimensional heat transfer in the Carreau-Yasuda hybrid nanofluid with Hall and ion slip effects. Phys. Scr. 2021, 96, 125215. [Google Scholar] [CrossRef]
- Abbas, Z.; Javed, T.; Sajid, M.; Ali, N. Unsteady MHD flow and heat transfer on a stretching sheet in a rotating fluid. J. Taiwan Inst. Chem. Eng. 2010, 41, 644–650. [Google Scholar] [CrossRef]
- Kuznetsov, A. Nanofluid bio-thermal convection: Simultaneous effects of gyrotactic and oxytactic micro-organisms. Fluid Dyn. Res. 2011, 43, 55505. [Google Scholar] [CrossRef]
- Tayyab, M.; Siddique, I.; Jarad, F.; Ashraf, M.K.; Ali, B. Numerical solution of 3D rotating nanofluid flow subject to Darcy-Forchheimer law, bio-convection and activation energy. S. Afr. J. Chem. Eng. 2022, 40, 48–56. [Google Scholar] [CrossRef]
- Hayat, T.; Aziz, A.; Muhammad, T.; Alsaedi, A. Effects of binary chemical reaction and Arrhenius activation energy in Darcy-Forchheimer three-dimensional flow of nanofluid subject to rotating frame. J. Therm. Anal. Calorim. 2019, 136, 1769–1779. [Google Scholar] [CrossRef]
- Ali, B.; Pattnaik, P.K.; Naqvi, R.A.; Waqas, H. Brownian motion and thermophoresis effects on bioconvection of rotating Maxwell nanofluid over a Riga plate with Arrhenius activation energy and Cattaneo-Christov heat flux theory. Therm. Sci. Eng. Prog. 2021, 23, 100863. [Google Scholar] [CrossRef]
- Nazar, R.; Amin, N.; Pop, I. Unsteady boundary layer flow due to a stretching surface in a rotating fluid. Mech. Res. Commun. 2004, 31, 121–128. [Google Scholar] [CrossRef]
- Zaimi, K.; Ishak, A.; Pop, I. Stretching surface in rotating viscoelastic fluid. Appl. Math. Mech. 2013, 34, 945–952. [Google Scholar] [CrossRef]
- Ali, B.; Nie, Y.; Hussain, S.; Manan, A.; Sadiq, M.T. Unsteady magneto-hydrodynamic transport of rotating Maxwell nanofluid flow on a stretching sheet with Cattaneo–Christov double diffusion and activation energy. Therm. Sci. Eng. Prog. 2020, 20, 100720. [Google Scholar] [CrossRef]
- Ali, B.; Rasool, G.; Hussain, S.; Baleanu, D.; Bano, S. Finite element study of magnetohydrodynamics (MHD) and activation energy in Darcy-Forchheimer rotating flow of Casson Carreau nanofluid. Processes 2020, 8, 1185. [Google Scholar] [CrossRef]


| Nazar et al. [42] | Zaimi et al. [43] | Current Findings | ||||
|---|---|---|---|---|---|---|
| 0 | 1.0000 | 0.0000 | 1.0000 | 0.0000 | 1.00001 | 0.00000 |
| 0.5 | 1.1384 | 0.5128 | 1.1384 | 0.5128 | 1.13839 | 0.51277 |
| 1 | 1.3250 | 0.8371 | 1.3250 | 0.8371 | 1.32503 | 0.83710 |
| 2 | 1.6523 | 1.2873 | 1.6523 | 1.2873 | 1.65232 | 1.28725 |
| 3 | 1.6523 | 1.2870 | 1.9289 | 1.6248 | 1.92894 | 1.62474 |
| Ali et al. [44] | Bagh et al. [45] | Current Findings | ||||
|---|---|---|---|---|---|---|
| 0 | 1.0000 | 0.0000 | 1.0000 | 0.0000 | 1.00001 | 0.00000 |
| 0.5 | 1.1384 | 0.5128 | 1.1384 | 0.5128 | 1.13839 | 0.51277 |
| 1 | 1.3250 | 0.8371 | 1.3250 | 0.8371 | 1.32503 | 0.83710 |
| 2 | 1.6523 | 1.2873 | 1.6523 | 1.2873 | 1.6523 5 | 1.28725 |
| 3 | 1.6523 | 1.2870 | 1.9289 | 1.6248 | 1.92894 | 1.62474 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ahmad, B.; Ahmad, M.O.; Ali, L.; Ali, B.; Hussein, A.K.; Shah, N.A.; Chung, J.D. Significance of the Coriolis Force on the Dynamics of Carreau–Yasuda Rotating Nanofluid Subject to Darcy–Forchheimer and Gyrotactic Microorganisms. Mathematics 2022, 10, 2855. https://doi.org/10.3390/math10162855
Ahmad B, Ahmad MO, Ali L, Ali B, Hussein AK, Shah NA, Chung JD. Significance of the Coriolis Force on the Dynamics of Carreau–Yasuda Rotating Nanofluid Subject to Darcy–Forchheimer and Gyrotactic Microorganisms. Mathematics. 2022; 10(16):2855. https://doi.org/10.3390/math10162855
Chicago/Turabian StyleAhmad, Bilal, Muhammad Ozair Ahmad, Liaqat Ali, Bagh Ali, Ahmed Kadhim Hussein, Nehad Ali Shah, and Jae Dong Chung. 2022. "Significance of the Coriolis Force on the Dynamics of Carreau–Yasuda Rotating Nanofluid Subject to Darcy–Forchheimer and Gyrotactic Microorganisms" Mathematics 10, no. 16: 2855. https://doi.org/10.3390/math10162855
APA StyleAhmad, B., Ahmad, M. O., Ali, L., Ali, B., Hussein, A. K., Shah, N. A., & Chung, J. D. (2022). Significance of the Coriolis Force on the Dynamics of Carreau–Yasuda Rotating Nanofluid Subject to Darcy–Forchheimer and Gyrotactic Microorganisms. Mathematics, 10(16), 2855. https://doi.org/10.3390/math10162855








