Stability and Optimal Control of Tree-Insect Model under Forest Fire Disturbance
Abstract
:1. Introduction
2. Deterministic Model for Tree–Beetle–Fire System
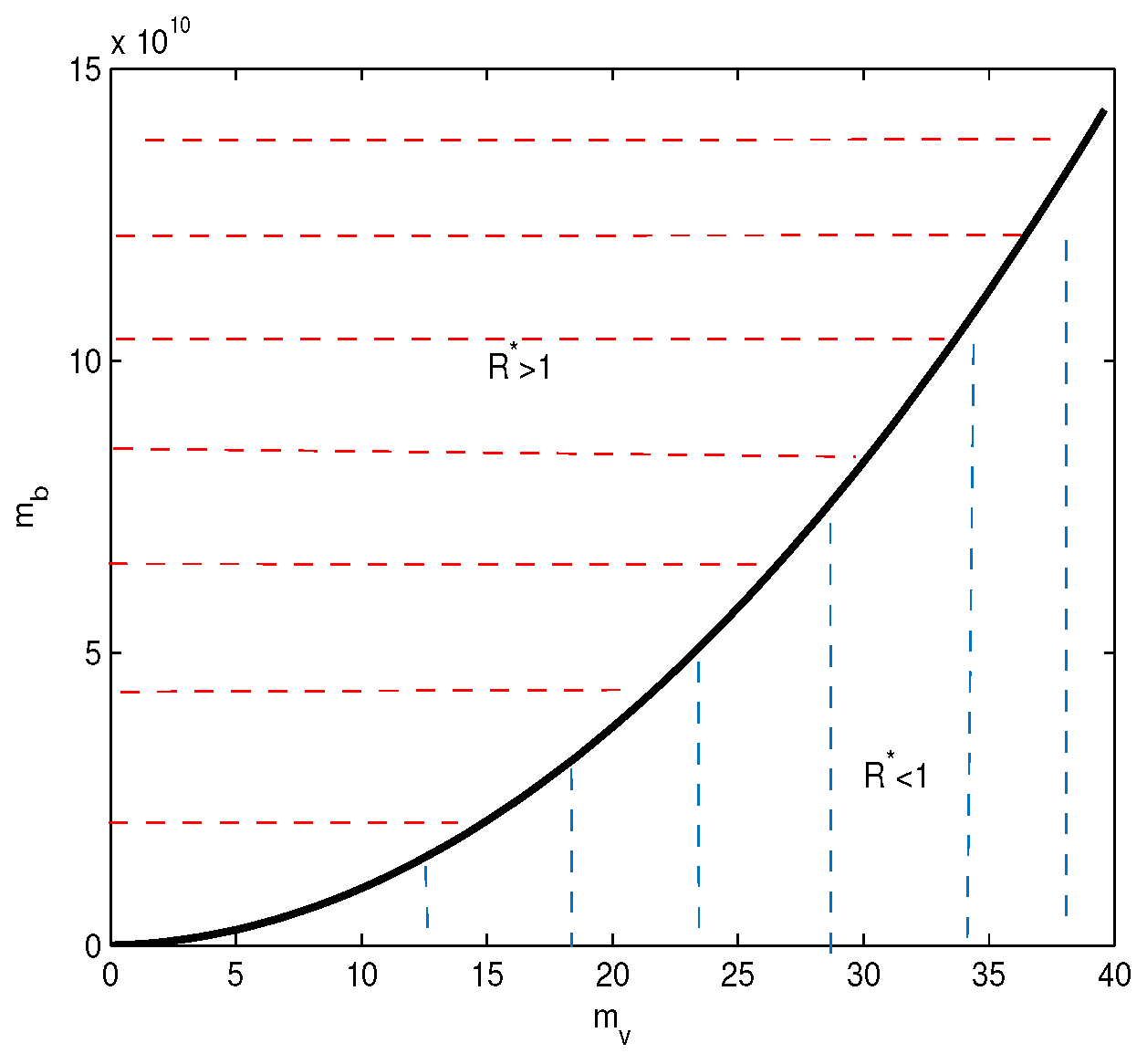
Existence and Stability of Equilibrium
- (1)
- If , boundary equilibrium of system (3) is local asymptotically stable.
- (2)
- If , boundary equilibrium of system (3) is unstable.
- (1)
- If , coexistence equilibrium of system (3) is local asymptotically stable.
- (2)
- If , coexistence equilibrium of system (3) is unstable.
3. Optimal Control Strategy
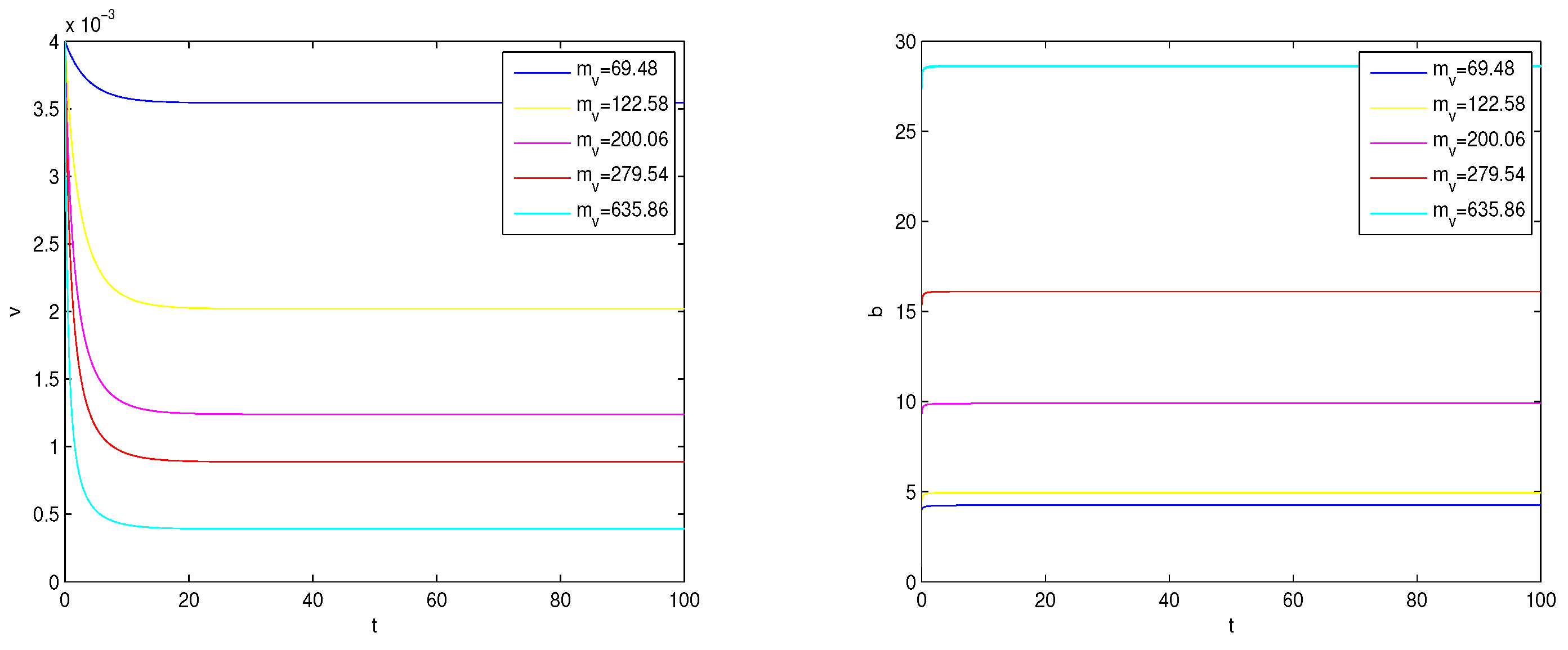
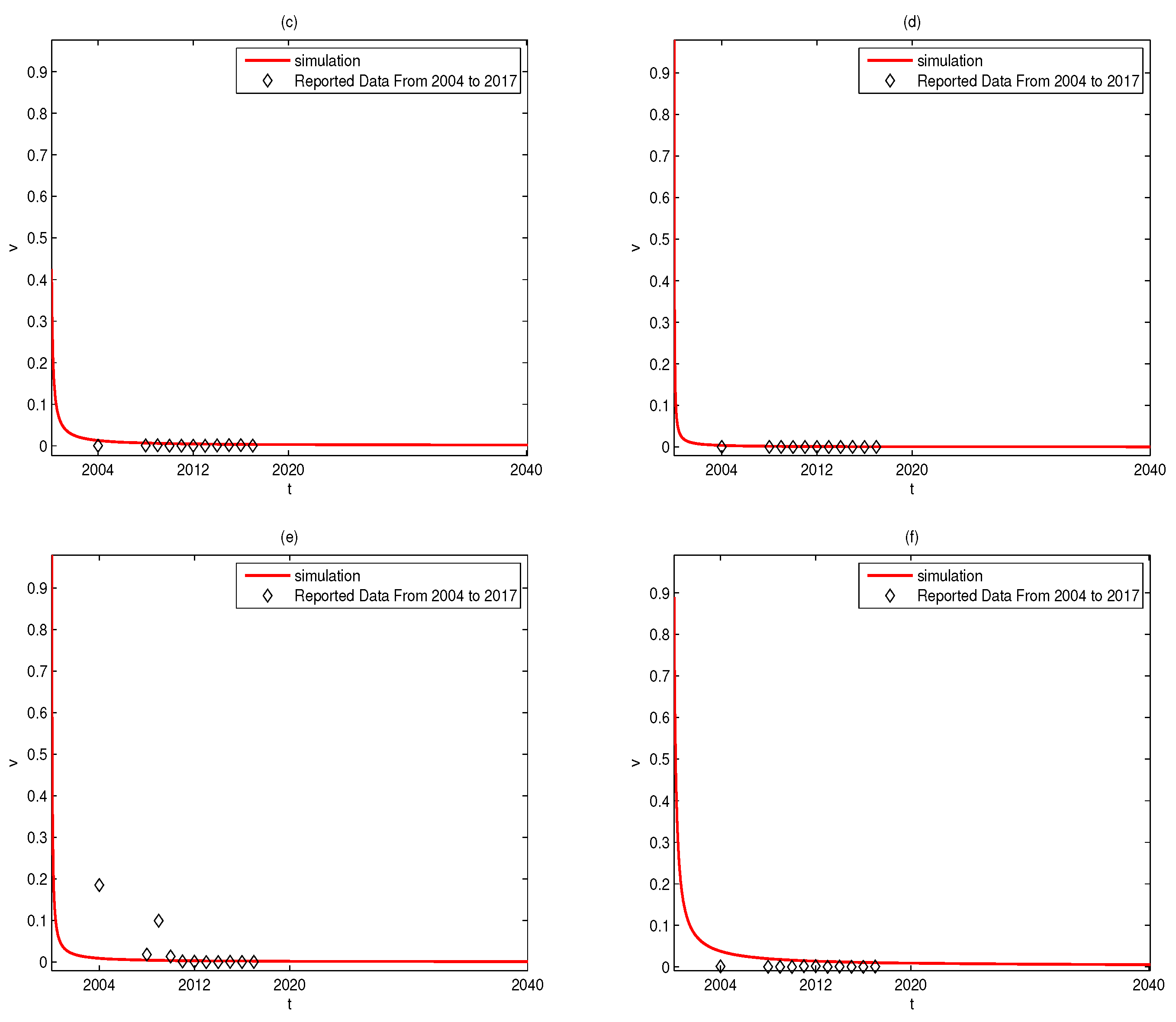
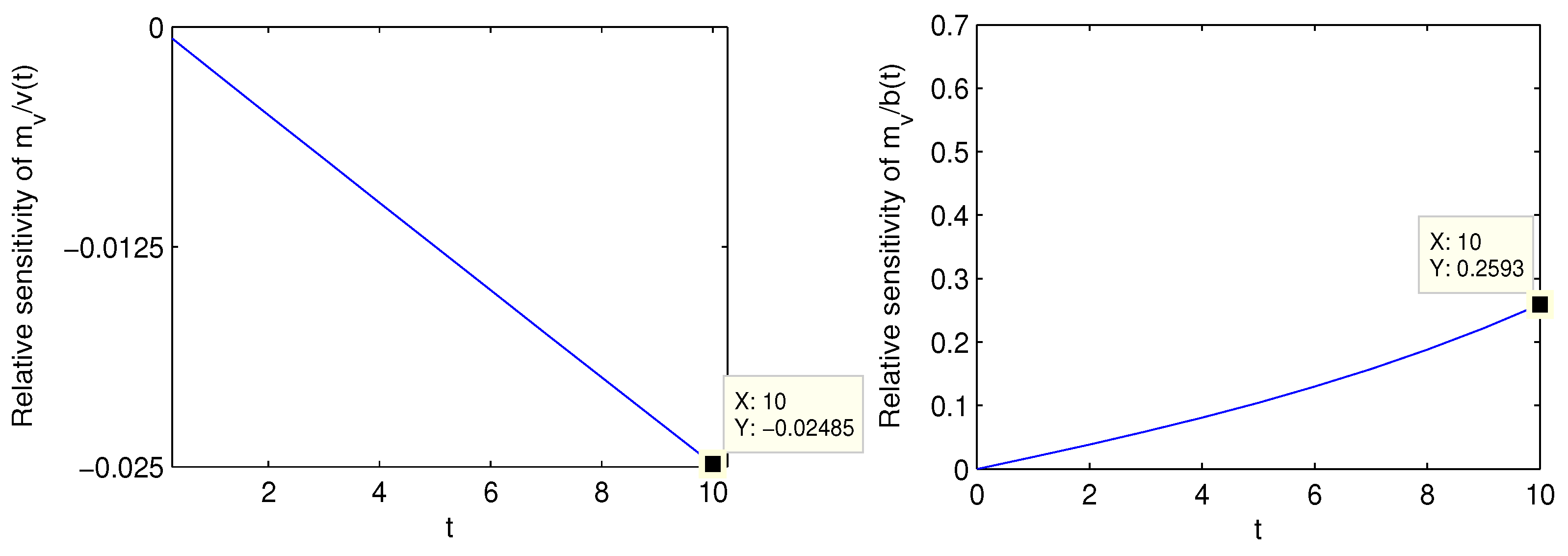
Sensitivity Analysis and Numerical Simulations
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Amman, G.D.; Schmitz, R.F. Mountain Pine Beetle: Lodgepole Pine Interactions and Strategies for Reducing Tree Losses. Ambio 1988, 17, 62–68. [Google Scholar]
- Green, P.W.; James, J.M. Mountain Pine Beetle-Induced Changes to Selected Lodgepole Pine Fuel Complexes within the Intermountain Region. For. Sci. 2007, 4, 507–518. [Google Scholar]
- Bigler, C.; Kulakoswki, D.; Veblen, T.T. Multiple disturbance interactions and drought influence fire severity in Rocky Mountain subalpine forests. Ecology 2005, 86, 3018–3029. [Google Scholar]
- Geiszler, D.R.; Gara, R.I.; Driver, C.H.; Gallucci, V.F.; Martin, R.E. Fire, Fungi, and Beetle Influences on a Lodgepole Pine Ecosystem of South-Central Oregon. Oecologia 1980, 46, 239–243. [Google Scholar] [PubMed]
- Mccullough, D.G. Fire and Insects in Northern and Boreal Forest Ecosystems of North Americal. Annu. Rev. Entomol. 1998, 43, 107–127. [Google Scholar] [PubMed] [Green Version]
- Forthofer, J.M.; Butler, B.W.; McHugh, C.W.; Finney, M.A. A comparison of three approaches for simulating fine-scale surface winds in support of wildland fire management. Part II. An exploratory study of the effect of simulated winds on fire growth simulations. Int. J. Wildland Fire 2014, 23, 969–981. [Google Scholar]
- Barclay, H.J.; Li, C.; Benson, L.; Taylor, S.; Shore, T. Effects of fire return rates on traversability of lodgepole pine forests for mountain pine beetle (Coleoptera: Scolytidae) and the use of patch metrics to estimate traversability. Can. Entomol. 2005, 137, 566–583. [Google Scholar]
- Bradley, T.; Tueller, P. Effects of fire on bark beetle presence on Jeffrey pine in the Lake Tahoe Basin. For. Ecol. Manag. 2001, 142, 205–214. [Google Scholar]
- Elkin, C.M.; Reid, M.L. Attack and Reproductive Success of Mountain Pine Beetles (Coleoptera: Scolytidae) in Fire-Damaged Lodgepole Pines. Environ. Entomol. 2004, 4, 1070–1080. [Google Scholar]
- Mart, G.S.; Wagner, M.R. Bark beetle community structure under four ponderosa pine forest stand conditions in northern Arizona. For. Ecol. Manag. 2002, 170, 145–160. [Google Scholar]
- Fleming, R.A.; Candau, J.N.; Mcalpine, R.S. Landscape-Scale Analysis of Interactions between Insect Defoliation and Forest Fire in Central Canada. Clim. Chang. 2002, 55, 251–272. [Google Scholar]
- Mchugh, C.W.; Kolb, T.E.; Wilson, J.L. Bark Beetle Attacks on Ponderosa Pine Following Fire in Northern Arizona. Environ. Entomol. 2003, 32, 510–522. [Google Scholar]
- Santoro, A.E.; Lombardero, M.J.; Ayres, M.P.; Ruel, J.J. Interactions between fire and bark beetles in an old growth pine forest. For. Ecol. Manag. 2001, 144, 245–254. [Google Scholar]
- Bebi, P.; Kulakowski, D.; Veblen, T. Interactions between fire and spruce beetles in a subalpine Rocky Mountain forest landscape Ecology. For. Ecol. Manag. 2003, 84, 362–371. [Google Scholar]
- Heimann, B.; Fleming, W.H.; Rishel, R.W. Deterministic and Stochastic Optimal Control. J. Appl. Math. Mech. 1979, 59, 494. [Google Scholar]
- Safranyik, L.; Wilson, W.R. The Mountain Pine Beetle: A Synthesis of Biology, Management and Impacts on Lodgepole Pine; Safranyik, L., Wilson, B., Eds.; Canadian Forest Service Publications: Ottawa, ON, Canada, 2007; p. 299. [Google Scholar]
- Charpentier, B.C.; Leite, M. A model for coupling fire and insect outbreak in forests. Ecol. Model. 2014, 286, 26–36. [Google Scholar] [CrossRef]
- Zhou, L.; Fan, M.; Hou, Q.; Jin, Z.; Sun, X. Transmission dynamics and optimal control of brucellosis in Inner Mongolia of China. Math. Biosci. Eng. 2018, 15, 543–567. [Google Scholar] [CrossRef] [Green Version]
- Martin, R.H. Logarithmic norms and projections applied to linear differential systems. J. Math. Anal. Appl. 1974, 45, 432–454. [Google Scholar]
- Zhang, J.L. China Forestry Statisitical Yearbook; China Forestry Publishing House: Beijing, China, 2017; pp. 5–396. [Google Scholar]
- Bonnans, J.F.; Shapiro, A. Perturbation Analysis of Optimization Problems Stability and Sensitivity Analysis; Springer: New York, NY, USA, 2000; Volume 4, pp. 260–400. [Google Scholar]




| Parameter | |||||
|---|---|---|---|---|---|
| Beijing | 0.764572 | 0.048204 | 158.611171 | 1657.993 | 828.9964 |
| Tianjin | 0.445343 | 0.021843 | 203.882824 | 3658.925 | 1829.463 |
| Hebei | 0.154946 | 0.023128 | 66.995922 | 3455.703 | 1727.852 |
| Shanxi | 0.252996 | 0.036412 | 69.4821260 | 2194.957 | 1097.479 |
| Inner Mongoria | 0.561737 | 0.045826 | 122.5812760 | 1744.046 | 872.0231 |
| Liaoning | 0.337569 | 0.016873 | 200.0673550 | 4736.759 | 2368.379 |
| Jilin | 0.38393 | 0.006038 | 635.8630730 | 13236.66 | 6618.332 |
| Heilongjiang | 0.239552 | 0.008569 | 279.546910 | 9326.556 | 4663.278 |
| Shandong | 0.524125 | 0.024039 | 218.031458 | 3324.691 | 1662.345 |
| Henan | 0.258396 | 0.046559 | 55.498005 | 1716.561 | 858.2804 |
| Year | 2004 | 2008 | 2009 | 2010 | 2011 | 2012 | 2013 | 2014 | 2015 | 2016 | 2017 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Shanxi | 0.18412 | 0.1013 | 0.3149 | 0.0499 | 0.6372 | 0.048 | 0.2145 | 0.076 | 0.0814 | 0.0272 | 0.0299 |
| Inner Mongoria | 0.51265 | 1.5358 | 1.7764 | 0.9129 | 0.1741 | 0.4464 | 0.0826 | 0.595 | 0.3847 | 0.1622 | 2.4805 |
| Liaoning | 0.04303 | 0.1249 | 0.1424 | 0.0404 | 0.0577 | 0.0374 | 0.0143 | 0.1045 | 0.1922 | 0.1706 | 0.0802 |
| Jilin | 0.03272 | 0.0445 | 0.0351 | 0.0084 | 0.024 | 0.0178 | 0.0063 | 0.0134 | 0.0541 | 0.0123 | 0.045 |
| Heilong jiang | 18.55472 | 1.8402 | 9.9819 | 1.3779 | 0.1741 | 0.1046 | 0.0106 | 0.0301 | 0.0787 | 0.064 | 0.0883 |
| Hebei | 0.13467 | 0.033 | 0.0443 | 0.0221 | 0.2169 | 0.2112 | 0.0422 | 0.1168 | 0.0471 | 0.0573 | 0.1157 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, X.; Zhang, C. Stability and Optimal Control of Tree-Insect Model under Forest Fire Disturbance. Mathematics 2022, 10, 2563. https://doi.org/10.3390/math10152563
Liu X, Zhang C. Stability and Optimal Control of Tree-Insect Model under Forest Fire Disturbance. Mathematics. 2022; 10(15):2563. https://doi.org/10.3390/math10152563
Chicago/Turabian StyleLiu, Xiaoxiao, and Chunrui Zhang. 2022. "Stability and Optimal Control of Tree-Insect Model under Forest Fire Disturbance" Mathematics 10, no. 15: 2563. https://doi.org/10.3390/math10152563
APA StyleLiu, X., & Zhang, C. (2022). Stability and Optimal Control of Tree-Insect Model under Forest Fire Disturbance. Mathematics, 10(15), 2563. https://doi.org/10.3390/math10152563







