A New Parameter-Uniform Discretization of Semilinear Singularly Perturbed Problems
Abstract
:1. Introduction
2. Quasilinearization
3. Some Properties of the Linear Problem
4. Construction of the FOFDM
5. Convergence Analysis
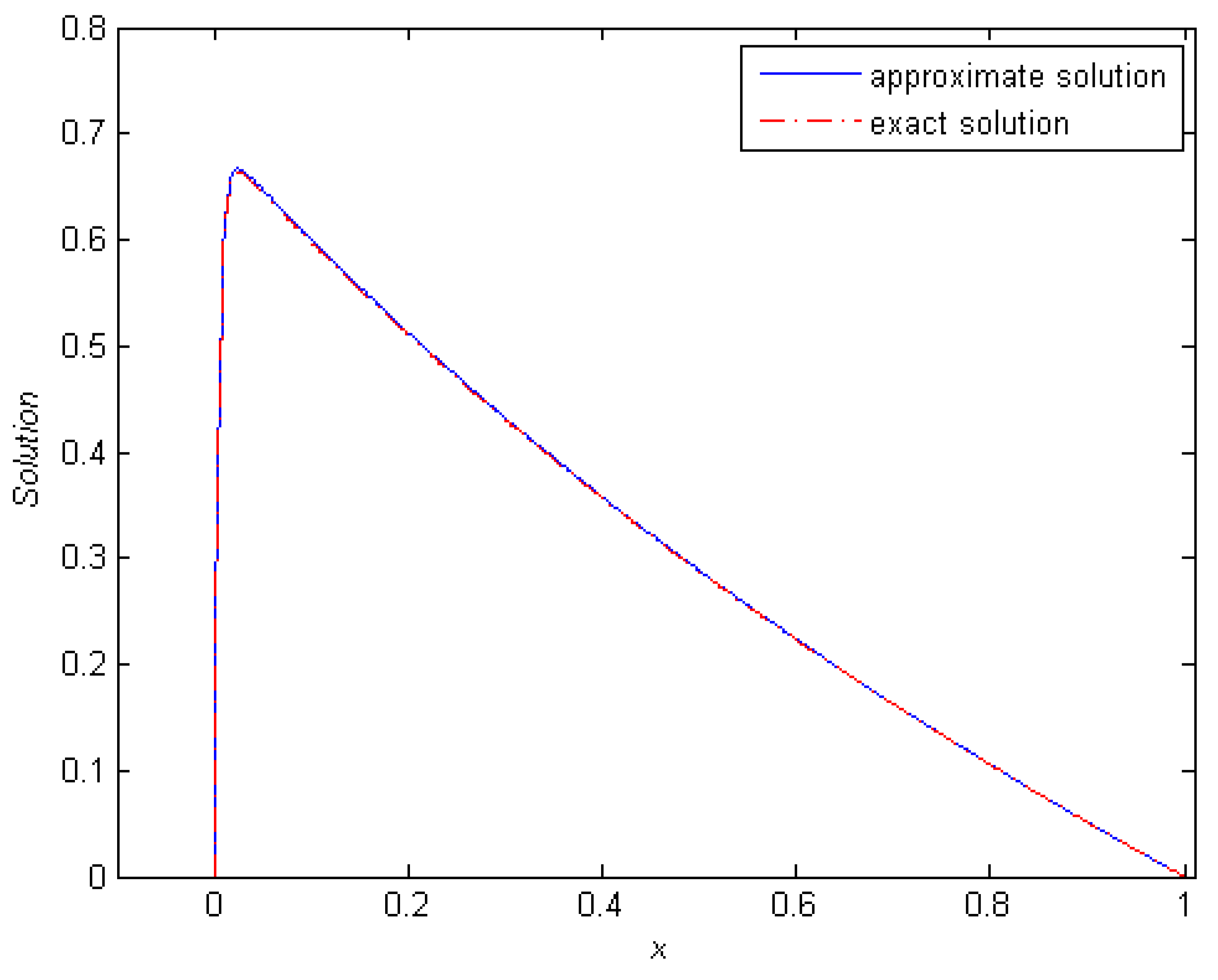
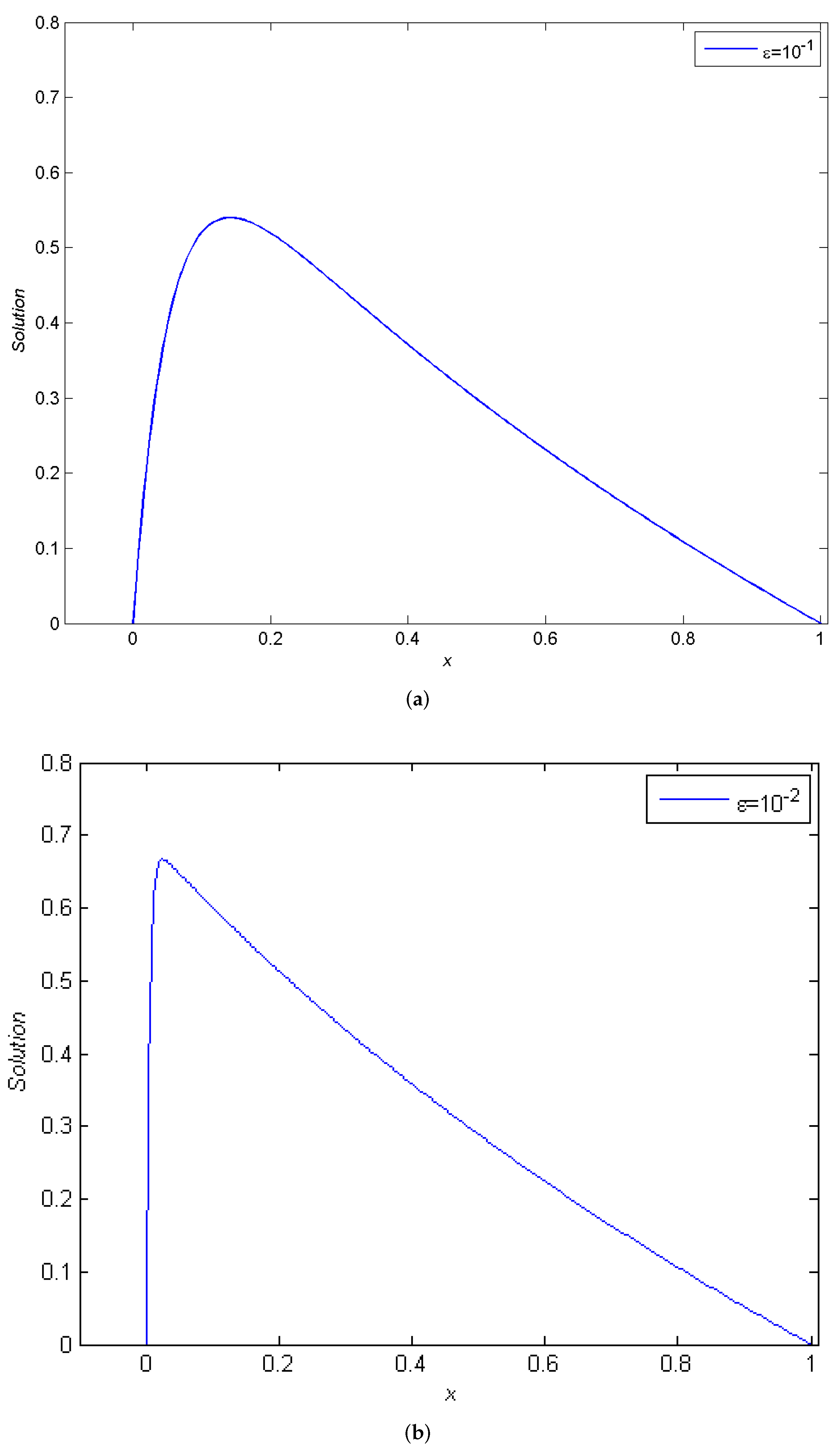
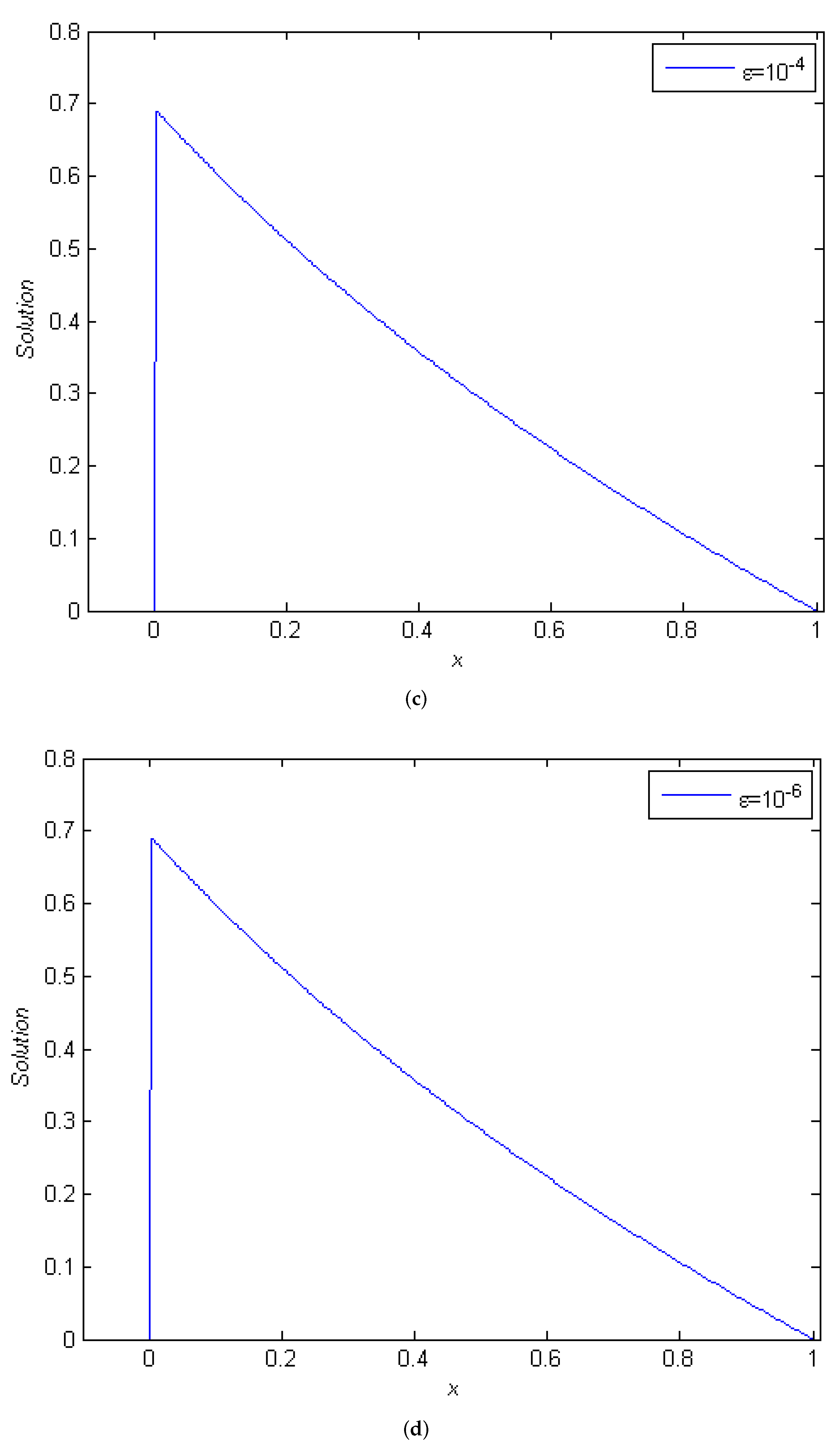
6. Numerical Results
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Carusotto, I.; Ciuti, C. Quantum fluids of light. Rev. Mod. Phys. 2013, 85, 299. [Google Scholar] [CrossRef] [Green Version]
- Malchow, A.K.; Azhand, A.; Knoll, P.; Engel, H.; Steinbock, O. From nonlinear reaction-diffusion processes to permanent microscale structures. Chaos. Interdiscip. J. Nonlinear Sci. 2019, 29, 53–129. [Google Scholar]
- Shyy, W.; Udaykumar, H.S.; Rao, M.M. Computational Fluid Dynamics with Moving Boundaries; CRC Press: Boca Raton, FL, USA, 1995. [Google Scholar]
- Nagy, A.; Saleh, M.; Omle, I.; Kareem, H.; Kovács, E. New Stable, Explicit, Shifted-Hopscotch Algorithms for the Heat Equation. Math. Comput. Appl. 2021, 26, 61. [Google Scholar] [CrossRef]
- Farrell, P.; Hegarty, A.; Miller, J.M.; O’Riordan, E.; Shishkin, G.I. Robust Computational Techniques for Boundary Layers; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Linß, T. Layer-adapted meshes for convection-diffusion problems. Comput. Methods Appl. Mech. Eng. 2003, 192, 1061–1105. [Google Scholar] [CrossRef] [Green Version]
- Miller, J.J.; O’riordan, E.; Shishkin, G.I. Fitted Numerical Methods for Singular Perturbation Problems: Error Estimates in the Maximum Norm for Linear Problems in One and Two Dimensions; World Scientific: Singapore, 1996. [Google Scholar]
- O’Malley, R.E. Historical Developments in Singular Perturbations; Springer International Publishing: Cham, Switzerland, 2014. [Google Scholar]
- Roos, H.G.; Stynes, M.; Tobiska, L.; Kellogg, R.B. Numerical methods for singularly perturbed differential equations. SIAM Rev. 1997, 39, 535. [Google Scholar]
- Roos, H.G.; Stynes, M.; Tobiska, L. Robust Numerical Methods for Singularly Perturbed Differential Equations: Convection-Diffusion-Reaction and Flow Problems; Springer Science & Business Media: Cham, Switzerland, 2008; p. 24. [Google Scholar]
- Gracia, J.L.; O’Riordan, E. A defect-correction parameter-uniform numerical method for ia singularly perturbed convection-diffusion problem in one dimension. Numer. Algorithms 2006, 41, 359–385. [Google Scholar] [CrossRef]
- Kopteva, N.; O’Riordan, E. Shishkin meshes in the numerical solution of singularly perturbed differential equations. Int. J. Numer. Anal. Modeling 2010, 7, 393–415. [Google Scholar]
- Mo, J.Q. Quasilinear singularly perturbed problem with boundary perturbation. J. Zhejiang Univ.-Sci. A 2004, 5, 1144–1147. [Google Scholar] [CrossRef]
- Vulanovic, R.; Teofanov, L. On the quasilinear boundary-layer problem and its numerical solution. J. Comput. Appl. Math. 2014, 268, 56–67. [Google Scholar] [CrossRef]
- Cimen, E.; Amiraliyev, G.M. A uniform convergent method for singularly perturbed nonlinear differential-difference equation. J. Inform. Math. Sci. 2017, 9, 191–199. [Google Scholar]
- Niijima, K. A uniformly convergent difference scheme for a semilinear singular perturbation problem. Numer. Math. 1984, 43, 175–198. [Google Scholar] [CrossRef]
- Stynes, M. An adaptive uniformly convergent numerical method for a semilinear singular perturbation problem. SIAM J. Numer. Anal. 1989, 26, 442–455. [Google Scholar] [CrossRef] [Green Version]
- Cakir, M.; Arslan, D. Finite difference method for nonlocal singularly perturbed problem. Int. J. Mod. Res. Eng. Technol. 2016, 1, 25–39. [Google Scholar]
- Cakir, M.; Amiraliyev, G.M. Numerical solution of a singularly perturbed three-point boundary value problem. Int. J. Comput. Math. 2007, 84, 1465–1481. [Google Scholar] [CrossRef]
- Boglaev, I.; Pack, S. A uniformly convergent method on arbitrary meshes for a semilinear convection-diffusion problem with discontinuous data. Int. J. Numer. Anal. Model 2008, 5, 24–39. [Google Scholar]
- Linß, T. Robustness of an upwind finite difference scheme for semilinear convection-diffusion problems with boundary turning points. J. Comput. Math. 2003, 21, 401–410. [Google Scholar]
- Shishkin, G.I.; Shishkina, L.P. A higher order richardson scheme for a singularly perturbed semilinear elliptic convection-diffusion equation. Comput. Math. Math. Phys. 2010, 50, 437–456. [Google Scholar] [CrossRef]
- Linss, T.; Vulanović, R. Uniform methods for semilinear problems with attractive boundary turning points. PAMM Proc. Appl. Math. Mech. 2002, 1, 518–519. [Google Scholar] [CrossRef]
- Yamac, K.; Erdogan, F. A Numerical scheme for semilinear singularly perturbed reaction-diffusion problems. Appl. Math. Nonlinear Sci. 2020, 5, 405–412. [Google Scholar] [CrossRef]
- Kabeto, M.J.; Duressa, G.F. Robust numerical method for singularly perturbed semilinear parabolic differential difference equations. Math. Comput. Simul. 2021, 188, 537–547. [Google Scholar] [CrossRef]
- Clavero, C.; Jorge, J.C. An efficient and uniformly convergent scheme for one-dimensional parabolic singularly perturbed semilinear systems of reaction-diffusion type. Numer. Algorithms 2020, 85, 1005–1027. [Google Scholar] [CrossRef]
- Kumar, S.; Rao, S.C.S. A robust domain decomposition algorithm for singularly perturbed semilinear systems. Int. J. Comput. Math. 2017, 94, 1108–1122. [Google Scholar] [CrossRef]
- Mariappan, M.; Tamilselvan, A. Higher order numerical method for a semilinear system of singularly perturbed differential equations. Math. Commun. 2021, 26, 41–52. [Google Scholar]
- Rao, S.C.S.; Chaturvedi, A.K. Analysis of an almost fourth-order parameter-uniformly convergent numerical method for singularly perturbed semilinear reaction-diffusion system with non-smooth source term. Appl. Math. Comput. 2022, 421, 126944. [Google Scholar]
- Munyakazi, J.B. A robust finite difference method for two-parameter parabolic convection-diffusion problems. Appl. Math. Inf. Sci. 2015, 9, 2877–2883. [Google Scholar]
- Munyakazi, J.B. A uniformly convergent nonstandard finite difference scheme for a system of convection-diffusion equations. Comput. Appl. Math. 2015, 34, 1153–1165. [Google Scholar] [CrossRef]
- Patidar, K.C. High order fitted operator numerical method for self-adjoint singular perturbation problems. Appl. Math. Comput. 2005, 171, 547–566. [Google Scholar] [CrossRef]
- Munyakazi, J.B.; Patidar, K.C. A fitted numerical method for singularly perturbed parabolic reaction-diffusion problems. Comput. Appl. Math. 2013, 32, 509–519. [Google Scholar] [CrossRef] [Green Version]
- Mickens, R.E. Nonstandard Finite Difference Models of Differential Equations; World Scientific: Singapore, 1994. [Google Scholar]
- Micken, R.E. Applications of Nonstandard Finite Difference Schemes; World Scientific: Singapore, 2000. [Google Scholar]
- Kadalbajoo, M.K.; Patidar, K.C. ε-uniformly convergent fitted mesh finite difference methods for general singular perturbation problems. Appl. Math. Comput. 2006, 179, 248–266. [Google Scholar] [CrossRef]
- Doolan, E.P.; Miller, J.J.; Schilders, W.H.A. Uniform Numerical Methods for Problems with Initial and Boundary Layers; Boole Press: Dublin, Ireland, 1980. [Google Scholar]
- Bender, C.M.; Orszag, S.A. Advanced Mathematical Methods for Scientists and Engineers I: Asymptotic Methods and Perturbation Theory; Springer Science and Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Cash, J.R.; Wright, M.H. A deferred correction method for nonlinear two-point boundary value problems: Implementation and numerical evaluation. SIAM J. Sci. Stat. Comput. 1991, 12, 971–989. [Google Scholar] [CrossRef]
- Kadalbajoo, M.K.; Patidar, K.C. Numerical solution of singularly perturbed non-linear two point boundary value problems by spline in compression. Int. J. Comput. Math. 2002, 79, 271–288. [Google Scholar] [CrossRef]
- Tikhovskaya, S.V.; Zadorin, A.I. A two-grid method with Richardson extrapolation for a semilinear convection-diffusion problem. AIP Conf. Proc. 2015, 1684, 090007. [Google Scholar]
| 1.60 × 10 | 9.36 × 10 | 5.37 × 10 | 3.82 × 10 | 3.37 × 10 | 3.25 × 10 | 3.22 × 10 | |
| 0.77 | 0.80 | 0.49 | 0.18 | 0.05 | 0.01 | ||
| 1.60 × 10 | 9.33 × 10 | 5.03 × 10 | 2.61 × 10 | 1.33 × 10 | 6.95 × 10 | 4.45 × 10 | |
| 0.78 | 0.89 | 0.95 | 0.97 | 0.94 | 0.64 | ||
| 1.60 × 10 | 9.33 × 10 | 5.03 × 10 | 2.61 × 10 | 1.33 × 10 | 6.71 × 10 | 3.37 × 10 | |
| 0.78 | 0.89 | 0.95 | 0.97 | 0.99 | 0.99 | ||
| 1.60 × 10 | 9.33 × 10 | 5.03 × 10 | 2.61 × 10 | 1.33 × 10 | 6.71 × 10 | 3.37 × 10 | |
| ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ |
| 1.60 × 10 | 9.33 × 10 | 5.03 × 10 | 2.61 × 10 | 1.33 × 10 | 6.71 × 10 | 3.37 × 10 | |
| 0.78 | 0.89 | 0.95 | 0.97 | 0.99 | 0.99 | ||
| 1.60 × 10 | 9.33 × 10 | 5.03 × 10 | 2.61 × 10 | 1.33 × 10 | 6.71 × 10 | 3.37 × 10 | |
| 0.78 | 0.89 | 0.95 | 0.97 | 0.99 | 0.99 |
| 4.03 × 10 | 1.85 × 10 | 5.58 × 10 | 1.10 × 10 | 1.71 × 10 | 3.52 × 10 | 7.68 × 10 | |
| 1.13 | 1.72 | 2.35 | 2.68 | 2.28 | 2.20 | ||
| 4.01 × 10 | 2.03 × 10 | 1.02 × 10 | 5.12 × 10 | 2.48 × 10 | 8.71 × 10 | 1.93 × 10 | |
| 0.98 | 0.99 | 0.99 | 1.05 | 1.51 | 2.18 | ||
| 4.01 × 10 | 2.03 × 10 | 1.02 × 10 | 5.10 × 10 | 2.55 × 10 | 1.28 × 10 | 6.39 × 10 | |
| 0.98 | 0.99 | 1.00 | 1.00 | 1.00 | 1.00 | ||
| 4.01 × 10 | 2.03 × 10 | 1.02 × 10 | 5.10 × 10 | 2.55 × 10 | 1.28 × 10 | 6.38 × 10 | |
| 0.98 | 0.99 | 1.00 | 1.00 | 1.00 | 1.00 | ||
| ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ |
| 4.01 × 10 | 2.03 × 10 | 1.02 × 10 | 5.10 × 10 | 2.55 × 10 | 1.28 × 10 | 6.38 × 10 | |
| 0.98 | 0.99 | 1.00 | 1.00 | 1.00 | 1.00 | ||
| 4.01 × 10 | 2.03 × 10 | 1.02 × 10 | 5.10 × 10 | 2.55 × 10 | 1.28 × 10 | 6.38 × 10 | |
| 0.98 | 0.99 | 1.00 | 1.00 | 1.00 | 1.00 |
| 2.39 × 10 | 1.16 × 10 | 6.45 × 10 | 1.24 × 10 | 1.14 × 10 | 7.52 × 10 | 4.29 × 10 | |
| 1.04 | −2.47 | −0.95 | 0.13 | 0.60 | 0.81 | ||
| 2.38 × 10 | 1.11 × 10 | 5.38 × 10 | 2.65 × 10 | 1.33 × 10 | 3.29 × 10 | 1.09 × 10 | |
| 1.10 | 1.05 | 1.02 | 0.99 | −4.62 | −1.72 | ||
| 2.38 × 10 | 1.11 × 10 | 5.38 × 10 | 2.65 × 10 | 1.31 × 10 | 6.54 × 10 | 3.26 × 10 | |
| 1.10 | 1.05 | 1.02 | 1.01 | 1.01 | 1.00 | ||
| 2.38 × 10 | 1.11 × 10 | 5.38 × 10 | 2.65 × 10 | 1.31 × 10 | 6.54 × 10 | 3.26 × 10 | |
| 1.10 | 1.05 | 1.02 | 1.01 | 1.01 | 1.00 | ||
| ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ |
| 2.38 × 10 | 1.11 × 10 | 5.38 × 10 | 2.65 × 10 | 1.31 × 10 | 6.54 × 10 | 3.26 × 10 | |
| 1.10 | 1.05 | 1.02 | 1.01 | 1.01 | 1.00 | ||
| 2.38 × 10 | 1.11 × 10 | 5.38 × 10 | 2.65 × 10 | 1.31 × 10 | 6.54 × 10 | 3.26 × 10 | |
| 1.10 | 1.05 | 1.02 | 1.01 | 1.01 | 1.00 |
| 2.36 × 10 | 7.94 × 10 | 2.04 × 10 | 3.67 × 10 | 9.17 × 10 | 3.31 × 10 | 1.43 × 10 | |
| 1.51 | 1.96 | 2.47 | 2.00 | 1.47 | 1.21 | ||
| 2.43 × 10 | 9.97 × 10 | 4.58 × 10 | 2.17 × 10 | 9.48 × 10 | 3.05 × 10 | 6.41 × 10 | |
| 1.28 | 1.12 | 1.08 | 1.20 | 1.63 | 2.25 | ||
| 2.43 × 10 | 9.97 × 10 | 4.58 × 10 | 2.20 × 10 | 1.08 × 10 | 5.36 × 10 | 2.65 × 10 | |
| 1.28 | 1.12 | 1.06 | 1.03 | 1.01 | 1.01 | ||
| 2.43 × 10 | 9.97 × 10 | 4.58 × 10 | 2.20 × 10 | 1.08 × 10 | 5.36 × 10 | 2.67 × 10 | |
| 1.28 | 1.12 | 1.06 | 1.03 | 1.01 | 1.01 | ||
| ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ |
| 2.43 × 10 | 9.97 × 10 | 4.58 × 10 | 2.20 × 10 | 1.08 × 10 | 5.36 × 10 | 2.67 × 10 | |
| 1.28 | 1.12 | 1.06 | 1.03 | 1.01 | 1.01 | ||
| 2.43 × 10 | 9.97 × 10 | 4.58 × 10 | 2.20 × 10 | 1.08 × 10 | 5.36 × 10 | 2.67 × 10 | |
| 1.28 | 1.12 | 1.06 | 1.03 | 1.01 | 1.01 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Munyakazi, J.B.; Kehinde, O.O. A New Parameter-Uniform Discretization of Semilinear Singularly Perturbed Problems. Mathematics 2022, 10, 2254. https://doi.org/10.3390/math10132254
Munyakazi JB, Kehinde OO. A New Parameter-Uniform Discretization of Semilinear Singularly Perturbed Problems. Mathematics. 2022; 10(13):2254. https://doi.org/10.3390/math10132254
Chicago/Turabian StyleMunyakazi, Justin B., and Olawale O. Kehinde. 2022. "A New Parameter-Uniform Discretization of Semilinear Singularly Perturbed Problems" Mathematics 10, no. 13: 2254. https://doi.org/10.3390/math10132254
APA StyleMunyakazi, J. B., & Kehinde, O. O. (2022). A New Parameter-Uniform Discretization of Semilinear Singularly Perturbed Problems. Mathematics, 10(13), 2254. https://doi.org/10.3390/math10132254






