On the Double Roman Domination in Generalized Petersen Graphs P(5k,k)

Abstract

A double Roman dominating function on a graph $G=(V,E)$ is a function $f:V\to \{0,1,2,3\}$ satisfying the condition that every vertex u for which $f\left(u\right)=0$ is adjacent to at least one vertex assigned 3 or at least two vertices assigned 2, and every vertex u with $f\left(u\right)=1$ is adjacent to at least one vertex assigned 2 or 3. The weight of f equals $w\left(f\right)={\sum }_{v\in V}f\left(v\right)$. The double Roman domination number ${\gamma }_{dR}\left(G\right)$ of a graph G equals the minimum weight of a double Roman dominating function of G. We obtain closed expressions for the double Roman domination number of generalized Petersen graphs $P(5k,k)$. It is proven that ${\gamma }_{dR}\left(P(5k,k)\right)=8k$ for $k\equiv 2,3mod5$ and $8k\le {\gamma }_{dR}\left(P(5k,k)\right)\le 8k+2$ for $k\equiv 0,1,4mod5$. We also improve the upper bounds for generalized Petersen graphs $P(20k,k)$.

1. Introduction

Double Roman domination of graphs was first studied in [1], motivated by a number of applications of Roman domination in present time and in history [2]. The initial studies of Roman domination [3,4] have been motivated by a historical application. In the 4th century, Emperor Constantine was faced with a difficult problem of how to defend the Roman Empire with limited resources. His decision was to allocate two types of armies to the provinces in such a way that all the provinces in the empire will be safe. Some military units were well trained and capable of moving rapidly from one city to another in order to respond to any attack. Other legions consisted of a local militia and they were permanently positioned in a given province. The Emperor decreed that no legion could ever leave a province to defend another if in this case they left the province undefended. Thus, at some provinces two units were stationed, a local militia units were stationed at others, and some provinces had no army. While the problem is still of interest in military operations research [5], it also has applications in cases where a time-critical service is to be provided with some backup. For example, a fire station should never send all emergency vehicles to answer a call.

Similar reasoning applies in any emergency service. Hence positioning the fire stations, first aid stations, etc. at optimal positions improves the public services without increasing the cost. A natural generalization, in particular in the case of emergency services, is the k-Roman domination [6], where in the district not one, but k emergency teams are expected to be quickly available in case of multiple emergency calls. Special case $k=2$, the double Roman domination, is considered in this work. It is well-known that the decision version of the double Roman domination problem (MIN-DOUBLE-RDF) is NP-complete, even when restricted to planar graphs, chordal graphs, bipartite graphs, undirected path graphs, chordal bipartite graphs and to circle graphs [7,8,9]. It is therefore of interest to study the complexity of the problem for other families of graphs. For example, linear time algorithms exist for interval graphs and block graphs [8], for trees [10], for proper interval graphs [11] and for unicyclic graphs [9]. Another avenue of research that is motivated by high complexity of the problem is to obtain closed expressions for the double Roman domination number of some families of graphs. In particular, generalized Petersen graphs and certain subfamilies of generalized Petersen graphs have been studied extensively in recent years. The results listed in subsection on related previous work include closed expressions for the double Roman domination number of some, and tight bounds for other subfamilies [12,13,14,15]. For more results on double Roman domination we refer to recent papers [16,17,18,19] and the references there.

The rest of the paper is organized as follows. In Section 2, we recall some basic definitions and known results that will be used in the following sections. In the last part of Section 2 our main results, Theorems 6 and 7, are presented. In Section 3, we present upper and lower bounds for double Roman domination number in generalized Petersen graphs $P(5k,k)$. Finally, in Section 4, we give an improved upper bounds for double Roman domination number of generalized Petersen graphs $P(20k,k)$, using the notion of covering graphs.

2. Preliminaries

2.1. Graphs and Double Roman Domination

Let $G=(V,E)$ be a graph without loops and multiple edges. As usual, we denote with $V=V\left(G\right)$ the vertex set of G and with $E=E\left(G\right)$ its edge set.

A set $D\subseteq V\left(G\right)$ is a dominating set if every vertex in $V\left(G\right)\setminus D$ has at least one neighbor in D. The domination number $\gamma \left(G\right)$ is the cardinality of a minimum dominating set of G. A double Roman dominating function (DRDF) on a graph $G=(V,E)$ is a function $f:V\to \{0,1,2,3\}$ with the following properties:

(1)

every vertex u with $f\left(u\right)=0$ is adjacent to at least one vertex assigned 3 or at least two vertices assigned 2, and

(2)

every vertex u with $f\left(u\right)=1$ is adjacent to at least one vertex assigned 2 or 3 under f.

Define $f\left(U\right)={\sum }_{u\in U}f\left(u\right)$ as the weight of f on an arbitrary subset $U\subseteq V\left(G\right)$. Then, the weight of f equals $w\left(f\right)=f\left(V\left(G\right)\right)={\sum }_{v\in V\left(G\right)}f\left(v\right)$. The double Roman domination number ${\gamma }_{dR}\left(G\right)$ of a graph G is the minimum weight of a double Roman dominating function of G. A DRD function f is called a ${\gamma }_{dR}$-function of G if $w\left(f\right)={\gamma }_{dR}\left(G\right)$.

For any double Roman dominating function f, defined on G we define a partition of the vertex set $V=V_0\cup V_1\cup V_2\cup V_3$, where $V_i=V_i^f=\left\{u\right|f\left(u\right)=i\}$.

The study of the double Roman domination in graphs was initiated by Beeler et al. [1]. It was proved that $2\gamma \left(G\right)\le {\gamma }_{dR}\left(G\right)\le 3\gamma \left(G\right)$. Furthermore, Beeler at al. defined a graph G to be double Roman if ${\gamma }_{dR}\left(G\right)=3\gamma \left(G\right)$, where $\gamma \left(G\right)$ is the domination number of G. For a later reference we recall the following result, also obtained by Beeler et al.

Proposition 1

([1]). In a double Roman dominating function f of weight ${\gamma }_{dR}\left(G\right)$, no vertex needs to be assigned the value 1.

Domination in graphs with its many varieties has been extensively studied in the past [20,21,22,23]. Roman domination and double Roman domination is a rather new variety of interest [1,2,7,24,25,26,27].

2.2. Generalized Petersen Graphs

The generalized Petersen graph $P(n,k)$ is a graph with vertex set $U\cup V$ and edge set $E_1\cup E_2\cup E_3$, where $U=\{u_0,u_1,\cdots ,u_{n-1}\}$, $V=\{v_0,v_1,\cdots ,v_{n-1}\}$, $E_1=\left\{u_i{u}_{i+1}\right|i=0,1,\dots ,n-1\}$, $E_2=\left\{u_i{v}_i\right|i=0,1,\dots ,n-1\}$, $E_3=\left\{v_i{v}_{i+k}\right|i=0,1,\dots ,n-1\}$, and subscripts are reduced modulo n, see Figure 1. Thus, we identify integers i and j iff $i\equiv jmodn$. (As usual, $m\equiv rmodn$ means that $m=kn+r$, or equivalently, $m-r=kn$ for some integer $k\in \mathbb{Z}$.

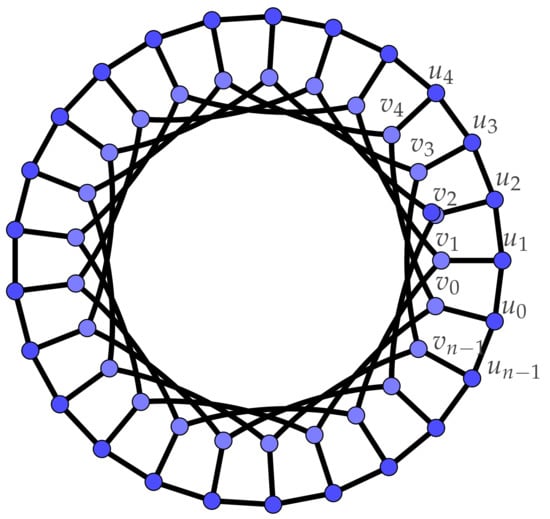
Figure 1. A generalized Petersen graph $P(n,k)$.

It is well known that the graphs $P(n,k)$ are 3-regular unless $k=\frac{n}{2}$ and that $P(n,k)$ are highly symmetric [28,29]. As $P(n,k)$ and $P(n,n-k)$ are isomorphic, it is natural to restrict attention to $P(n,k)$ with $n\ge 3$ and k, $1\le k<\frac{n}{2}$.

Petersen graphs are among the most interesting examples when considering nontrivial graph invariants. The domination and its variations (such as vertex domination, exact domination, rainbow domination, double Roman domination and other) of generalized Petersen graphs have been extensively studied in recent years, see for example [14,30,31,32,33,34,35,36].

2.3. Related Previous Work

The domination number for the generalized Petersen graphs $P(ck,k)$ for integer constants $c\ge 3$ was studied by Zhao et al. [37]. They obtained upper bound on $\gamma \left(P\right(ck,k\left)\right)$ for general c.

Theorem 1

([37]). For any $k\ge 1$ and $c\ge 3$

$$\gamma \left(P(ck,k)\right)\le \left\{\begin{array}{cc}\frac{c}{3}⌈\frac{5k}{3}⌉,\hfill & c\equiv 0mod3,\hfill \\ ⌈\frac{c}{3}⌉⌈\frac{5k}{3}⌉-⌈\frac{2k}{3}⌉,\hfill & c\equiv 1mod3,\hfill \\ ⌈\frac{c}{3}⌉⌈\frac{5k}{3}⌉-⌈\frac{2k}{3}⌉+⌈\frac{k}{3}⌉,\hfill & c\equiv 2mod3.\hfill \end{array}\right.$$

Shao et al. [14] determine the exact value of ${\gamma }_{dR}\left(P(n,1)\right)$, and Jiang et al. [13] determine ${\gamma }_{dR}\left(P(n,2)\right)$.

Theorem 2

([13,14]). Let $n\ge 3$. Then we have

$${\gamma }_{dR}\left(P(n,1)\right)=\left\{\begin{array}{cc}\frac{3n}{2},\hfill & n\equiv 0mod4,\hfill \\ \frac{3n+3}{2},\hfill & n\equiv 1,3mod4,\hfill \\ \frac{3n+4}{2},\hfill & n\equiv 2mod4\hfill \end{array}\right.$$

and for $n\ge 5$

$${\gamma }_{dR}\left(P(n,2)\right)=\left\{\begin{array}{cc}⌈\frac{8n}{5}⌉,\hfill & n\equiv 0mod5,\hfill \\ ⌈\frac{8n}{5}⌉+1,\hfill & n\equiv 1,2,3,4mod5.\hfill \end{array}\right.$$

Shao et al. in [14] obtained also a general lower bound on double Roman domination numbers for arbitrary graphs of a maximum degree greater or equal one.

Theorem 3

([14]). If G is a graph of maximum degree $▵\ge 1$, then

$${\gamma }_{dR}\left(G\right)\ge ⌈\frac{3V\left(G\right)}{▵+1}⌉.$$

Clearly, as the generalized Petersen graph $P(n,k)$ is 3-regular and has $2n$ vertices.

Corollary 1

([14]). In Petersen graphs $P(n,k)$, ${\gamma }_{dR}\left(P(n,k)\right)\ge \lceil \frac{3n}{2}\rceil .$

Gao et al. [12] determined the exact value of ${\gamma }_{dR}\left(P(n,k)\right)$ for $n\equiv 0mod4$ and $k\equiv 1mod2$, and presented an improved upper bound for ${\gamma }_{dR}\left(P(n,k)\right)$ in other cases. The results are summarized in the next theorem.

Theorem 4

([12]). For $k\ge 3$, ${\gamma }_{dR}\left(P(n,k)\right)=\frac{3n}{2}$, $k\equiv 1mod2,n\equiv 0mod4.$

$$⌈\frac{3n}{2}⌉\le {\gamma }_{dR}\left(P(n,k)\right)\le \left\{\begin{array}{cc}\frac{3n}{2}+\frac{5k+5}{4},\hfill & k\equiv 1mod4,n\cancel{\equiv }0mod4,\hfill \\ \frac{3n}{2}+\frac{5k+7}{4},\hfill & k\equiv 3mod4,n\cancel{\equiv }0mod4,\hfill \\ \frac{3n}{2}\frac{(3k+2)}{(3k+1)},\hfill & k\equiv 0mod4,n\equiv 0mod(3k+1),\hfill \\ \lceil \frac{3n}{2}\frac{(3k+2)}{(3k+1)}\rceil +\frac{5k+4}{4},\hfill & k\equiv 0mod4,n\cancel{\equiv }0mod(3k+1),\hfill \\ \frac{3n}{2}\frac{\left(3k\right)}{(3k-1)},\hfill & k\equiv 2mod4,n\equiv 0mod(3k-1),\hfill \\ \lceil \frac{3n}{2}\frac{\left(3k\right)}{(3k-1)}\rceil +\frac{5k+6}{4},\hfill & k\equiv 2mod4,n\cancel{\equiv }0mod(3k-1).\hfill \end{array}\right.$$

Double Roman domination of families $P(ck,k)$ has been studied recently for small k, including $c=3$, 4, and 5. Shao et al. [15] considered the double Roman domination number in generalized Petersen graphs $P(3k,k)$ and constructed solutions providing the upper bounds, which gives exact values for ${\gamma }_{dR}\left(P(3k,k)\right).$

Theorem 5

([15]).

$${\gamma }_{dR}\left(P(3k,k)\right)=\left\{\begin{array}{cc}5k+1,\hfill & k\in \{1,2,4\}\hfill \\ 5k,\hfill & otherwise\hfill \end{array}\right.$$

For small cases in the families $P(4k,k)$ and $P(5k,k)$, the known facts are summarized in the next proposition.

Proposition 2.

${\gamma }_{dR}\left(P(4,1)\right)=6$, ${\gamma }_{dR}\left(P(8,2)\right)=14$ [13], ${\gamma }_{dR}\left(P(12,3)\right)=18$ and

${\gamma }_{dR}\left(P(5,1)\right)=9$ [14], ${\gamma }_{dR}\left(P(10,2)\right)=16$ [13], $23\le {\gamma }_{dR}\left(P(15,3)\right)\le 26$ [12].

Wang et al. in [38] showed that $\gamma \left(P(4k,k)\right)=\left\{\begin{array}{cc}2k;\hfill & k\equiv 1mod2,\hfill \\ 2k+1;\hfill & k\equiv 0mod2\hfill \end{array}\right.$, and $\gamma \left(P\right(5k,k\left)\right)=3k$ for all $k\ge 1$. Furthermore, recall the lower bound given in Corollary 1 and recall that Theorem 4 implies ${\gamma }_{dR}\left(P(4k,k)\right)=6k$ for $k\equiv 1mod2.$ Thus, we can write the known facts regarding ${\gamma }_{dR}\left(P(4k,k)\right)$ and ${\gamma }_{dR}\left(P(5k,k)\right)$ in the next two propositions.

Proposition 3.

Let $k\ge 1$. If $k\equiv 1mod2$, then ${\gamma }_{dR}\left(P(4k,k)\right)=6k$, and if $k\equiv 0mod2$ then $6k\le {\gamma }_{dR}\left(P(4k,k)\right)\le 6k+3.$

Proposition 4.

Let $k\ge 3$. Then $7k+⌈\frac{k}{2}⌉\le {\gamma }_{dR}\left(P(5k,k)\right)\le 9k.$

2.4. Our Results

The main result of our paper are either exact values or narrow bounds for the double Roman domination numbers of all Petersen graphs $P(5k,k)$. More precisely, we will show that the following theorem holds.

Theorem 6.

Let $k\ge 2$.

$$8k\le {\gamma }_{dR}\left(P(5k,k)\right)\le \left\{\begin{array}{cc}8k,\hfill & k\equiv 2,3mod5\hfill \\ 8k+2,\hfill & \mathrm{otherwise}\hfill \end{array}\right.$$

As mentioned earlier, a graph G is double Roman if ${\gamma }_{dR}\left(G\right)=3\gamma \left(G\right)$. Using the known equality $\gamma \left(P\right(5k,k\left)\right)=3k$ for all $k\ge 1$ [38], we can conclude that the only double Roman graph in the set of generalized Petersen graphs $P(5k,k)$ is $P(5,1)$.

Corollary 2.

There is no double Roman graphs in the set of generalized Petersen graphs $P(5k,k)$ for $k\ge 2$. The graph $P(5,1)$ is a double Roman graph.

We also show that certain generalized Petersen graphs are covering graphs of other generalized Petersen graphs (Proposition 7). This provides a method for establishing new upper bounds, see Proposition 8. In particular, we elaborate the case $P(20k,k)$ to obtain exact values in some, and tight bounds in other cases.

Theorem 7.

${\gamma }_{dR}\left(P(20,1)\right)\le 40$, ${\gamma }_{dR}\left(P(40,2)\right)\le 64$, ${\gamma }_{dR}\left(P(60,3)\right)\le 96$.

Furthermore, let $k>3$. Then, for odd k, we have

$${\gamma }_{dR}\left(P(20k,k)\right)=30k$$

(1)

and for k even,

$$30k\le {\gamma }_{dR}\left(P(20k,k)\right)\le 30k+15.$$

(2)

By Proposition 1, we can only consider the DRDF of a graph G with no vertex assigned the value 1.

3. Constructions and Proofs

In this section, the constructions of double Roman dominating functions providing upper bounds for the double Roman dominating numbers are given. We start by introducing some convenient notation for representing the DRDFs and providing some basic constructions.

In order to present the double Roman dominating functions of generalized Petersen graphs as concise as possible we use two different notations. For smaller graphs, we use the notation in brackets, showing weights on outer and inner cycles in two lines:

$$\left(\begin{array}{cccc}f\left(u_0\right)& f\left(u_1\right)& \cdots & f\left(u_{n-1}\right)\\ f\left(v_0\right)& f\left(v_1\right)& \cdots & f\left(v_{n-1}\right)\end{array}\right).$$

For example, a DRD function showing ${\gamma }_{dR}\left(P(4,1)\right)=6$ is the following:

$$\left(\begin{array}{cccc}0& 0& 3& 0\\ 3& 0& 0& 0\end{array}\right).$$

For bigger graphs we use the notation that provides only the values on the outer cycle, see Table 1. (In this case, the assignment on the inner cycles is completed such that the weight is minimal, see Lemma 1 for details.)

Table 1. A DRD function of $U_i$ for $P(4k,k)$.

$f\left(u_0\right)$	$f\left(u_1\right)$	...	$f\left(u_i\right)$	...	$f\left(u_{k-1}\right)$	$f\left(u_k\right)$	$f\left(u_{k+1}\right)$	...
$f\left(u_k\right)$	$f\left(u_{k+1}\right)$	...	$f\left(u_{k+i}\right)$	...	$f\left(u_{2k-1}\right)$	$f\left(u_{2k}\right)$	$f\left(u_{2k+1}\right)$	...
$f\left(u_{2k}\right)$	$f\left(u_{2k+1}\right)$	...	$f\left(u_{2k+i}\right)$	...	$f\left(u_{3k-1}\right)$	$f\left(u_{3k}\right)$	$f\left(u_{3k+1}\right)$	...
$f\left(u_{3k}\right)$	$f\left(u_{3k+1}\right)$	...	$f\left(u_{3k+i}\right)$	...	$f\left(u_{4k-1}\right)$	$f\left(u_{4k}\right)=f\left(u_0\right)$	$f\left(u_1\right)$	...
0	1	...	i	...	$k-1$	k	$k+1$	...

The columns correspond to the sets $U_i=\{u_i,u_{i+k},u_{i+2k},\dots ,u_{i+(c-1)k}\}$, and we assume that the inner cycles, sets $V_i=\{v_i,v_{i+k},v_{i+2k},\dots ,v_{i+(c-1)k}\}$, are completed such that the whole assignment presents a DRD function. As we can see from Table 1 below, the first two and the last two columns provide the same information on DRD function, namely the values at $U_0=U_k$, and $U_1=U_{k+1}$. We will use this property observing certain patterns appearing in case of optimal assignment —it will hold exactly when columns 0 and k will match, taking into account the shift of rows as indicated in Table 1.

To better understand the notation in tables, consider the pattern in Table 2 that provides DRDF(double Roman dominating function) for $P(12,3)$ and $P(28,7)$.

Table 2. An optimal DRDF(double Roman dominating function) of $U_i$ for $P(4k,k)$. The first column provides a DRD function for $P(4,1)$, the first 3 columns provide a DRD function for $P(12,3)$, and the first 7 columns provide a DRD function for $P(28,7)$.

0	0	3	0	0	0	3	0	...
0	0	0	3	0	0	0	3	...
3	0	0	0	3	0	0	0	...
0	3	0	0	0	3	0	0	...
0	1	2	3	4	5	6	7	...

Considering closely the graph $P(12,3)$ and using the fact that the columns 0 and 4 correspond to the same set of vertices, $U_0=U_4$, and that the column 4 equals column 0 shifted one row downwards (see Table 1), we can see that the pattern is well defined on the outer cycle of $P(12,3)$. Obviously, the vertices on the inner cycles could be assigned with three more weights of 3, so we have a DRDF of $P(12,3)$ of weight $9+9=18$. Similarly, regarding $P(28,7)$, we have $U_0=U_7$, and the same reasoning applies. Recalling Theorem 4, the constructions are best possible (compare the bounds in Theorem 3.)

3.1. Basic Constructions for $P(5k,k)$

Recall that ${\gamma }_{dR}\left(P(5,1)\right)=9$ [14]. A simple DRD function showing ${\gamma }_{dR}\left(P(5,1)\right)\le 9$ is the following:

$$\left(\begin{array}{ccccc}3& 0& 0& 0& 3\\ 0& 0& 3& 0& 0\end{array}\right).$$

For larger graphs among $P(5k,k)$, we are going to use the notation introduced in Table 3.

Table 3. A DRD function of $U_i$ for $P(5k,k)$.

$f\left(u_0\right)$	$f\left(u_1\right)$	...	$f\left(u_i\right)$	...	$f\left(u_{k-1}\right)$	$f\left(u_k\right)$	$f\left(u_{k+1}\right)$	...
$f\left(u_k\right)$	$f\left(u_{k+1}\right)$	...	$f\left(u_{k+i}\right)$	...	$f\left(u_{2k-1}\right)$	$f\left(u_{2k}\right)$	$f\left(u_{2k+1}\right)$	...
$f\left(u_{2k}\right)$	$f\left(u_{2k+1}\right)$	...	$f\left(u_{2k+i}\right)$	...	$f\left(u_{3k-1}\right)$	$f\left(u_{3k}\right)$	$f\left(u_{3k+1}\right)$	...
$f\left(u_{3k}\right)$	$f\left(u_{3k+1}\right)$	...	$f\left(u_{3k+i}\right)$	...	$f\left(u_{4k-1}\right)$	$f\left(u_{4k}\right)$	$f\left(u_{4k+1}\right)$	...
$f\left(u_{4k}\right)$	$f\left(u_{4k+1}\right)$	...	$f\left(u_{4k+i}\right)$	...	$f\left(u_{5k-1}\right)$	$f\left(u_{5k}\right)=f\left(u_0\right)$	$f\left(u_1\right)$	...
0	1	...	i	...	$k-1$	k	$k+1$	...

The next two examples provide constructions of DRDF that show ${\gamma }_{dR}\left(P(10,2)\right)\le 16$ and ${\gamma }_{dR}\left(P(35,7)\right)\le 56$.

We proceed with two comments on Table 4.

• First, note that the columns in Table 4 have the following properties: each column has two consecutive vertices that are assigned two legions: say $u_i$ and $u_{i+k}$ for some i. Then, in the column i, vertices $u_{i+2k}$ and $u_{i+4k}$ have one neighbor and the vertex $u_{i+3k}$ has two neighbors in the outer cycle that are assigned 2. Clearly, the missing legions can be provided by assigning weight 2 to vertices $v_{i+2k}$ and $v_{i+4k}$ on the inner cycle. In this assignment, each of the vertices $u_{i+2k}$, $u_{i+3k}$ and $u_{i+4k}$ is adjacent to two vertices of weight 2. Hence we have weight 8 for each column.
• Second, observe that columns 2 and 7 coincide. Recalling the convention given in Table 3, note that for $k=2$, column 2 is column 0 shifted one row upwards (cyclicaly). Similarly, for $k=7$, and columns 0 and 7.

Table 4. A DRDF of $U_i$ that implies ${\gamma }_{dR}\left(P(10,2)\right)\le 16$ and ${\gamma }_{dR}\left(P(35,7)\right)\le 56$.

2	0	2	0	0	2	0	2	...
2	0	0	2	0	2	0	0	...
0	2	0	2	0	0	2	0	...
0	2	0	0	2	0	2	0	...
0	0	2	0	2	0	0	2	...
0	1	2	3	4	5	6	7	8	...

These constructions are optimal, which will follow from the lower bound that will be proved below. Recall that ${\gamma }_{dR}\left(P(10,2)\right)=16$ [13], therefore the RDF for ${\gamma }_{dR}\left(P(10,2)\right)$ given in Table 4 is best possible.

A symmetrical construction, given in Table 5 shows ${\gamma }_{dR}\left(P(15,3)\right)\le 24$ and ${\gamma }_{dR}\left(P(40,8)\right)\le 64$.

Table 5. A DRDF of $U_i$ that implies ${\gamma }_{dR}\left(P(15,3)\right)\le 24$ and ${\gamma }_{dR}\left(P(40,8)\right)\le 64$.

2	0	0	2	0	2	0	0	2	...
2	0	2	0	0	2	0	2	0	...
0	0	2	0	2	0	0	2	0	...
0	2	0	0	2	0	2	0	0	...
0	2	0	2	0	0	2	0	2	...
0	1	2	3	4	5	6	7	8	...

3.2. Double Roman Domination in $P(5k,k)$—Upper Bounds

Observe that the patterns used in Table 4 and Table 5 have period five (columns). This implies the next proposition.

Proposition 5.

Let $k\equiv 2mod5$ or $k\equiv 3mod5$. Then ${\gamma }_{dR}\left(P(5k,k)\right)\le 8k$.

Proof. 

Recall from previous considerations (Table 4) that ${\gamma }_{dR}\left(P(10,2)\right)\le 16$ and ${\gamma }_{dR}\left(P(35,7)\right)\le 56$. Observe that if we repeat the columns 2-6 in Table 4, we obtain a DRDF showing ${\gamma }_{dR}\left(P(60,12)\right)\le 96$. By induction, it follows that ${\gamma }_{dR}(P(5(5i+2),(5i+2))\le 8(5i+2)$ for all integers $i\ge 0$. Thus, ${\gamma }_{dR}\left(P(5k,k)\right)\le 8k$ for $k\equiv 2mod5$.

The statement for $k\equiv 3mod5$ follows from Table 5 by analogous argument. □

The next table provides a DRDF for $P(25,5)$. It is obtained from Table 4 by deleting columns 3 and 4 and altering only one entry in the original column 5, see Table 6.

Table 6. A DRDF of $U_i$ that implies ${\gamma }_{dR}\left(P(25,5)\right)\le 42$.

2	0	2	2	0	2	...
2	0	0	2	0	0	...
0	2	0	2	2	0	...
0	2	0	0	2	0	...
0	0	2	0	0	2	...
0	1	2	3,4,5	6	7	...	merged columns
0	1	2	3	4	5	...	columns renamed

It is straightforward to check that the table provides a DRDF. Observe that we can in this way delete two columns and alter one column to obtain a DRDF of ${\gamma }_{dR}(P(5\left(5i\right),5i)\le 8\left(5i\right)+2$ from ${\gamma }_{dR}(P(5(5i+2),5i+2)\le 8(5i+2)$ for all integers $i\ge 0$.

The same idea, applied to Table 5 gives a DRDF of $P(30,6)$ of weight 50. We omit the details. Using the periodicity of the basic pattern, we have a construction that gives RDF showing ${\gamma }_{dR}(P(5(5i+1),5i+1)\le 8(5i+1)+2$ from ${\gamma }_{dR}(P(5(5i+3),(5i+3))\le 8(5i+3)$ for all integers $i\ge 0$.

Similarly, inserting two columns in the pattern comes with additional cost of 8 + 8 + 2 = 18 legions, thus increasing the total weight by 18. For example, see Table 7.

Table 7. An alternative DRDF of $U_i$ that shows ${\gamma }_{dR}\left(P(25,5)\right)\le 42$ and ${\gamma }_{dR}\left(P(50,10)\right)\le 82$.

2	0	0	2	0	2	0	2	0	0	2	...
2	0	2	0	2	0	0	2	0	2	0	...
0	0	2	0	2	0	2	0	0	2	0	...
0	2	0	2	0	0	2	0	2	0	0	...
0	2	0	2	0	2	0	0	2	0	2	...
0	1	2	3’	2’	3	4	5	6	7	8	...
0	1	2	3	4	5	6	7	8	9	10	columns renamed

For completeness, in Table 8 we give a RDF of $P(20,4)$ proving that ${\gamma }_{dR}\left(P(20,4)\right)\le 34$. The construction starts with RDF of weight 16 for ${\gamma }_{dR}\left(P(10,2)\right)$, and inserts two columns as in Table 7. In more detail, note that column 4 is a copy of column 2 and column 3 is a copy of column 1. Then, additional two legions are assigned to vertex $u_{17}$ in column 1. It follows that ${\gamma }_{dR}(P(5(5i+4),(5i+4))\le 8(5i+4)+2$ for all integers $i\ge 0$.

Table 8. A DRDF of $U_i$ that implies ${\gamma }_{dR}\left(P(20,4)\right)\le 34$.

2	0	2	0	2
2	0	0	0	0
0	2	0	2	0
0	2	0	2	0
0	2	2	0	2
0	1’	2’	1	2
0	1	2	3	4	columns renamed

Summarizing the arguments, we have a proof of the next proposition.

Proposition 6.

Let $k\equiv 0,1,4mod5$. Then ${\gamma }_{dR}\left(P(5k,k)\right)\le 8k+2$.

3.3. Double Roman Domination in $P(5k,k)$—Lower Bound

The proof of lower bound in Theorem 8 is based on several technical lemmas. In all proofs below we assume that f is a DRDF and there are no vertices with $f\left(v\right)=1$. As before, let $U_i=\{u_i,u_{i+k},u_{i+2k},u_{i+3k},u_{i+4k}\}$ and $V_i=\{v_i,v_{i+k},v_{i+2k},$$v_{i+3k},$$v_{i+4k}\}$. Let us denote with $W_i$ the weight of $H_i=V_i\cup U_i$, $W_i=f\left(H_i\right)=f(V_i\cup U_i)$.

Lemma 1.

Let f be DRDF f. Then

if $f\left(U_i\right)=0$ then $f\left(V_i\right)\ge 6$ and $W_i\ge 6$,

if $f\left(U_i\right)=2$ then $f\left(V_i\right)\ge 5$ and $W_i\ge 7$,

if $f\left(U_i\right)=3$ then $f\left(V_i\right)\ge 5$ and $W_i\ge 8$,

if $f\left(U_i\right)=4$ then $f\left(V_i\right)\ge 4$ and $W_i\ge 8$,

if $f\left(U_i\right)=5$ then $f\left(V_i\right)\ge 4$ and $W_i\ge 9$,

if $f\left(U_i\right)=6$ then $f\left(V_i\right)\ge 3$ and $W_i\ge 9$,

if $f\left(U_i\right)=7$ then $f\left(V_i\right)\ge 4$ and $W_i\ge 11$,

if $f\left(U_i\right)=8$ then $f\left(V_i\right)\ge 3$ and $W_i\ge 11$, and

if $f\left(U_i\right)\ge 9$ then $W_i\ge 12$.

Proof. 

We will list all possible examples (up to the isomorphism), using the following notation:

$$\left(\begin{array}{ccccc}f\left(u_i\right)& f\left(u_{i+k}\right)& f\left(u_{i+2k}\right)& f\left(u_{i+3k}\right)& f\left(u_{i+4k}\right)\\ f\left(v_i\right)& f\left(v_{i+k}\right)& f\left(v_{i+2k}\right)& f\left(v_{i+3k}\right)& f\left(v_{i+4k}\right)\end{array}\right).$$

• Case $f\left(U_i\right)\ge 9$. First, assume $f\left(U_i\right)=9$. Excluding weights 1, the sum 9 can be achieved as $9=3+3+3$ or $9=3+2+2+2$. In the first case, three vertices among five can be chosen in two ways, either the two zeros are at adjacent columns or not. Similarly, in the second case, the 0 can either be next to 3, or not. Thus, we have 4 cases listed below. The values on $V_i$ are chosen so that the total weight is minimal.

  $$\begin{array}{cccc}\left(\begin{array}{ccccc}3& 3& 3& 0& 0\\ 0& 0& 0& 3& 0\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 3& 0& 3& 0\\ 0& 0& 0& 3& 0\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 2& 2& 2& 0\\ 0& 0& 2& 0& 2\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 2& 2& 0& 2\\ 0& 1& 0& 2& 0\end{array}\right).\hfill \end{array}$$
  In all cases we have $f\left(V_i\right)\ge 3$, thus $W_i\ge 9+3=12.$
  Furthermore, if $f\left(U_i\right)=10$ or $f\left(U_i\right)=11$ then observe that $f\left(V_i\right)\ge 2$, and hence $W_i\ge 12$.
• Case $f\left(U_i\right)=8$. Possible subcases with $8=3+3+2=2+2+2+2$ are

  $$\begin{array}{cccc}\left(\begin{array}{ccccc}3& 3& 2& 0& 0\\ 0& 0& 0& 3& 0\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 3& 0& 2& 0\\ 0& 0& 0& 3& 0\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 2& 3& 0& 0\\ 0& 0& 2& 0& 2\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 2& 0& 3& 0\\ 0& 0& 2& 0& 2\end{array}\right)\hfill \end{array}$$

  and

  $$\begin{array}{ccc}\left(\begin{array}{ccccc}2& 2& 2& 2& 0\\ 0& 2& 0& 0& 2\end{array}\right).\hfill & & \end{array}$$
  In all cases, $f\left(V_i\right)\ge 3$, thus $W_i\ge 8+3=11.$
• Case $f\left(U_i\right)=7$. There is only one possibility, $7=3+2+2$, and we have the following subcases:

  $$\begin{array}{cccc}\left(\begin{array}{ccccc}3& 2& 2& 0& 0\\ 0& 0& 2& 0& 2\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 2& 0& 2& 0\\ 0& 0& 2& 0& 2\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 2& 0& 0& 2\\ 0& 0& 2& 2& 0\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 0& 2& 2& 0\\ 0& 2& 0& 0& 2\end{array}\right).\hfill \end{array}$$
  It is obvious that $f\left(V_i\right)\ge 4$ and $W_i\ge 7+4=11.$
• Case $f\left(U_i\right)=6$. This sum can be achieved as $6=3+3=2+2+2.$ There are four subcases:

  $$\begin{array}{cccc}\left(\begin{array}{ccccc}3& 3& 0& 0& 0\\ 0& 0& 0& 3& 0\end{array}\right),\hfill & \left(\begin{array}{ccccc}3& 0& 3& 0& 0\\ 0& 2& 0& 0& 3\end{array}\right),\hfill & \left(\begin{array}{ccccc}2& 2& 2& 0& 0\\ 0& 0& 2& 0& 2\end{array}\right),\hfill & \left(\begin{array}{ccccc}2& 2& 0& 2& 0\\ 0& 0& 2& 0& 2\end{array}\right).\hfill \end{array}$$
  In all cases the value $f\left(V_i\right)$ is at least 3, which implies $W_i\ge 6+3=9.$
• Case $f\left(U_i\right)=5$. We have $5=3+2$, and two possibilities.

  $$\left(\begin{array}{ccccc}3& 2& 0& 0& 0\\ 0& 0& 2& 0& 2\end{array}\right),\left(\begin{array}{ccccc}3& 0& 2& 0& 0\\ 0& 2& 0& 3& 0\end{array}\right).$$
  Clearly, in both cases $f\left(V_i\right)$ must be at least 4, which implies $W_i\ge 5+4=9.$
• Case $f\left(U_i\right)=4$. As $4=2+2$, we have two cases:

  $$\left(\begin{array}{ccccc}2& 2& 0& 0& 0\\ 0& 0& 2& 0& 2\end{array}\right),\left(\begin{array}{ccccc}2& 0& 2& 0& 0\\ 0& 2& 0& 3& 0\end{array}\right).$$
  As $f\left(V_i\right)\ge 4$ in both cases, we have $W_i\ge 4+4=8.$
• Case $f\left(U_i\right)=3$. There is only one possible subcase $\left(\begin{array}{ccccc}3& 0& 0& 0& 0\\ 0& 0& 3& 0& 2\end{array}\right)$ with $f\left(V_i\right)=5$, thus $W_i\ge 3+5=8.$
• Case $f\left(U_i\right)=2$. The only possible subcase is $\left(\begin{array}{ccccc}2& 0& 0& 0& 0\\ 0& 2& 0& 3& 0\end{array}\right)$ with $f\left(V_i\right)=5$, thus $W_i\ge 2+5=7.$
• Case $f\left(U_i\right)=0$ has two possible subcases,

  $$\left(\begin{array}{ccccc}0& 0& 0& 0& 0\\ 0& 3& 0& 0& 3\end{array}\right),\left(\begin{array}{ccccc}0& 0& 0& 0& 0\\ 2& 2& 0& 2& 0\end{array}\right),$$

  with $f\left(V_i\right)=6$, thus $W_i\ge 0+6=6.$

This concludes the proof of lemma. □

In order to prove the lower bound in Theorem 8, we will need to consider the $H_i$ with $W_i<8$, thus by Lemma 1, the cases $W_i=7$ ($f\left(U_i\right)=2$, and $f\left(U_i\right)=0$) or $W_i=6$$(f\left(U_i\right)=0)$. In the Figure 2 below all cases (up to the isomorphism) with $W_i=6$ and $W_i=7$ are drawn.

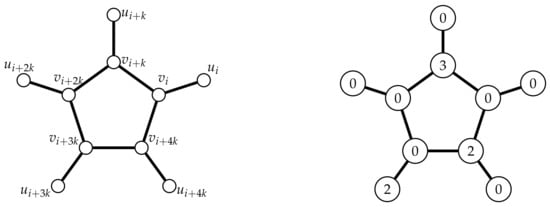
Figure 2. The standard drawing of $H_i$ (left) and the case $f\left(H_i\right)=W_i=7$ with $f\left(U_i\right)=2$ (right).

First we consider the cases where two adjacent $H_i$ have weights less than 8. Note that the proof of the next lemma also implies that it is not possible to have a DRDF with more that two consecutive $W_i<8$.

Lemma 2.

(a)

If $W_i=6$ and $W_{i+1}=6$ then $W_{i-1}\ge 12$ and $W_{i+2}\ge 12$.

(b)

If $W_i=7$ and $W_{i+1}=7$ then $W_{i-1}\ge 11$ and $W_{i+2}\ge 11$.

(c)

If $W_i=6$ and $W_{i+1}=7$ then $W_{i-1}\ge 11$, $W_{i+2}\ge 11$, and $W_{i-1}+W_{i+2}\ge 23$.

Proof. 

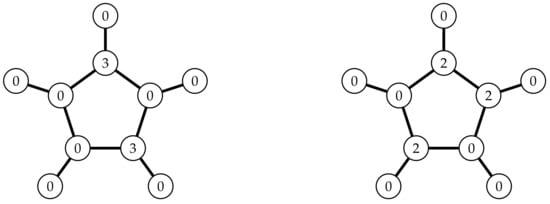
The proof will be derived in several steps using the notation introduced in Table 3. We only give the values on the outer cycle, and in addition, in some cases (for sets $H_i$ and $H_{i+1}$) the values on the inner cycles are provided in parenthesis, as $\mathbf{f}\left({\mathbf{u}}_{\mathbf{j}}\right)\left(f\left(v_j\right)\right)$. For other neighbor sets $H_{*}$, we will assume that the inner cycles $V_{*}$ are completed such that the whole assignment is a DRD function. The weights $W_i$ are estimated using the results of Lemma 1.

(a)

Case $W_i=6$ and $W_{i+1}=6$ obviously implies that $f\left(U_i\right)=0$ and $f\left(U_{i+1}\right)=0$. There are two cases (see Figure 3), for which Table 9 (columns A1 and A2) show the minimal demands that the two neighboring vertices in $U_{i-1}\cup U_{i+1}$ have to fulfil. Without loss of generality, consider first $H_i$. Since $f\left(U_{i+1}\right)=0$, we read from Table 9 that at least three vertices of $U_{i-1}$ must have weights 3, thus $f\left(U_{i-1}\right)\ge 9$ and $W_{i-1}\ge 9+3=12.$ By analogous reasoning, $W_{i+2}\ge 9+3=12.$

(b)

Case $W_i=7$ and $W_{i+1}=7$ (see Figure 4). First, consider the case when $f\left(U_{i+1}\right)=0$. Then, from Table 9 (columns A3 and A4) there are at least two vertices of $U_{i-1}$ which must have weights 3, and two more vertices with weights at least two, thus $f\left(U_{i-1}\right)\ge 10$ and $W_{i-1}\ge 12.$ By symmetry, $f\left(U_i\right)=0$ implies $W_{i+2}\ge 12.$

Therefore, we may assume that $f\left(U_i\right)=2$ and $f\left(U_{i+1}\right)=2$. The DRDF for $H_i$ is in Figure 2 (right). Considering neighbor sets $H_{i-1}$ and $H_{i+2}$ we have all subcases listed in Table 10 and Table 11.

In Table 10 and Table 11, we fix DRDF on $H_i$ (second column), and consider all possible DRDF with $W_{i+1}=7$ and $f\left(U_{i+1}\right)=2$ (third column). The first and fourth columns provide the minimal f values in $H_{i-1}$ and $H_{i+2}$, respectively. The labeled graph $H_{i+1}$ in this case has no symmetries, hence we have to consider five rotations, and in each case two cases due to reflexion. Thus, we have ten cases in total, b1 to b10, outlined in Table 10 and Table 11.

From Table 10 and Table 11 using the results of Lemma 1 we can estimate the weights $W_i$: (b1) $W_{i-1}\ge 8+4=12$, $W_{i+2}\ge 8+4=12$, (b2) $W_{i-1}\ge 6+5=11$, $W_{i+2}\ge 7+4=11$, (b3) $W_{i-1}\ge 7+4=11$, $W_{i+2}\ge 8+4=12$, (b4) $W_{i-1}\ge 8+4=12$, $W_{i+2}\ge 7+4=11$, (b5) $W_{i-1}\ge 8+4=12$, $W_{i+2}\ge 6+5=11$, (b6) $W_{i-1}\ge 8+4=12$, $W_{i+2}\ge 8+4=12$, (b7) $W_{i-1}\ge 6+5=11$, $W_{i+2}\ge 7+4=11$, (b8) $W_{i-1}\ge 7+4=11$, $W_{i+2}\ge 8+4=12$, (b9) $W_{i-1}\ge 8+4=12$, $W_{i+2}\ge 7+4=11$, (b10) $W_{i-1}\ge 8+4=12$, $W_{i+2}\ge 8+4=12$.

(c)

Case $W_i=6$ and $W_{i+1}=7$. First, observe that in the case when $f\left(U_{i+1}\right)=0$, the reasoning in case (a) and (b) implies that $W_{i-1}\ge 12$ and $W_{i+2}\ge 11$. So we can assume that $f\left(U_{i+1}\right)=2$. As seen in Figure 3, three vertices in $V_i$ could have weights 2 or two of them have weights 3. As we know, one vertex in $U_{i+1}$ has weight 3 and the other one (two steps further) has weight 2, see Figure 2. We thus fix the assignment in $H_i$ (second column), add all possible assignments in $H_{i+1}$ (third column) and write the minimal weights on $H_{i-1}$ and $H_{i+2}$ in the first and fourth column. As each of the two assignments of $H_i$ is reflexion symmetric, it is clear that there are exactly 10 different cases. All possible outcomes for sets $H_{i-1}\cup H_i\cup H_{i+1}$ are given below, Table 12 and Table 13.

In Table 12, we find all subcases (c1 to c5) when two vertices in $V_i$ have weights 3.

In Table 13, we have all subcases (c6 to c10) when three vertices in $V_i$ have weights 2.

Similarly, as in case (b), we can estimate the weights $W_i$ from Table 12 and Table 13 using the results of Lemma 1: (c1) $W_{i-1}\ge 8+3=11$, $W_{i+2}\ge 8+4=12$, (c2) $W_{i-1}\ge 9+3=12$, $W_{i+2}\ge 8+4=12$, (c3) $W_{i-1}\ge 8+4=12$, $W_{i+2}\ge 8+4=12$, (c4) $W_{i-1}\ge 8+4=12$, $W_{i+2}\ge 8+4=12$, (c5) $W_{i-1}\ge 9+3=12$, $W_{i+2}\ge 8+4=12$, (c6) $W_{i-1}\ge 10+4=14$, $W_{i+2}\ge 8+4=12$, (c7) $W_{i-1}\ge 10+4=14$, $W_{i+2}\ge 8+4=12$, (c8) $W_{i-1}\ge 11+4=15$, $W_{i+2}\ge 8+4=12$, (c9) $W_{i-1}\ge 10+4=14$, $W_{i+2}\ge 8+4=12$, (c10) $W_{i-1}\ge 11+4=15$, $W_{i+2}\ge 8+4=12$.

Figure 3. The two cases with $f\left(H_i\right)=W_i=6$.

Table 9. Demands for $U_{i-1}\cup U_{i+1}$ when $W_i=6$ or $W_i=7$ and $f\left(U_i\right)=0$.

(A1)		(A2)		(A3)		(A4)
0(0)	3+0/2+2	0(2)	0+2	0(0)	3+0/2+2	0(0)	3+0/2+2
0(3)	0	0(2)	0+2	0(3)	0	0(3)	0
0(0)	3+0/2+2	0(0)	3+0/2+2	0(0)	3+0/2+2	0(2)	2+0
0(0)	3+0/2+2	0(2)	0+2	0(2)	2+0	0(0)	3+0/2+2
0(3)	0	0(0)	3+0/2+2	0(2)	2+0	0(2)	2+ 0

Figure 4. The two cases with $f\left(H_i\right)=W_i=7$ and $f\left(U_i\right)=0$.

Table 10. Subcases of $f\left(U_{i-1}\right)$, $f\left(U_i\right)$, $f\left(U_{i+1}\right)$ and $f\left(U_{i+2}\right)$ with $W_i=7$, $W_{i+1}=7$ and $f\left(U_{i+1}\right)=2$ (first part).

(b1)				(b2)				(b3)				(b4)				(b5)
0	2(0)	2(0)	0	0	2(0)	0(0)	2	0	2(0)	0(3)	0	0	2(0)	0(0)	2	0	2(0)	0(2)	0
2	0(2)	0(2)	2	0	0(2)	2(0)	0	2	0(2)	0(0)	3	2	0(2)	0(3)	0	2	0(2)	0(0)	3
3	0(0)	0(0)	3	3	0(0)	0(2)	2	2	0(0)	2(0)	0	3	0(0)	0(0)	3	3	0(0)	0(3)	0
0	0(3)	0(3)	0	0	0(3)	0(0)	3	0	0(3)	0(2)	2	0	0(3)	2(0)	0	0	0(3)	0(0)	3
3	0(0)	0(0)	3	3	0(0)	0(3)	0	3	0(0)	0(0)	3	3	0(0)	0(2)	2	3	0(0)	2(0)	0

Table 11. Subcases of $f\left(U_{i-1}\right)$, $f\left(U_i\right)$, $f\left(U_{i+1}\right)$ and $f\left(U_{i+2}\right)$ with $W_i=7$, $W_{i+1}=7$ and $f\left(U_{i+1}\right)=2$ (second part).

(b6)				(b7)				(b8)				(b9)				(b10)
0	2(0)	0(2)	2	0	2(0)	0(0)	2	0	2(0)	0(3)	0	0	2(0)	0(0)	2	0	2(0)	2(0)	0
2	0(2)	2(0)	0	0	0(2)	0(2)	2	2	0(2)	0(0)	3	2	0(2)	0(3)	0	2	0(2)	0(0)	3
3	0(0)	0(0)	3	3	0(0)	2(0)	0	2	0(0)	0(2)	2	3	0(0)	0(0)	3	3	0(0)	0(3)	0
0	0(3)	0(3)	0	0	0(3)	0(0)	3	0	0(3)	2(0)	0	0	0(3)	0(2)	2	0	0(3)	0(0)	3
3	0(0)	0(0)	3	3	0(0)	0(3)	0	3	0(0)	0(0)	3	3	0(0)	2(0)	0	3	0(0)	0(2)	2

Table 12. First five subcases of $f\left(U_{i-1}\right)$, $f\left(U_i\right)$, $f\left(U_{i+1}\right)$ and $f\left(U_{i+2}\right)$ with $W_i=6$ and $W_{i+1}=7$.

(c1)				(c2)				(c3)				(c4)				(c5)
2	0(0)	2(0)	0	3	0(0)	0(2)	2	3	0(0)	0(0)	3	3	0(0)	0(3)	0	3	0(0)	0(0)	3
0	0(3)	0(0)	3	0	0(3)	2(0)	0	0	0(3)	0(2)	2	0	0(3)	0(0)	3	0	0(3)	0(3)	0
3	0(0)	0(3)	0	3	0(0)	0(0)	3	2	0(0)	2(0)	0	3	0(0)	0(2)	2	3	0(0)	0(0)	3
3	0(0)	0(0)	3	3	0(0)	0(3)	0	3	0(0)	0(0)	3	2	0(0)	2(0)	0	3	0(0)	0(2)	2
0	0(3)	0(2)	2	0	0(3)	0(0)	3	0	0(3)	0(3)	0	0	0(3)	0(0)	3	0	0(3)	2(0)	0

Table 13. Second five subcases of $f\left(U_{i-1}\right)$, $f\left(U_i\right)$, $f\left(U_{i+1}\right)$ and $f\left(U_{i+2}\right)$ with $W_i=6$ and $W_{i+1}=7$.

(c6)				(c7)				(c8)				(c9)				(c10)
0	0(2)	2(0)	0	2	0(2)	0(2)	2	2	0(2)	0(0)	3	2	0(2)	0(3)	0	2	0(2)	0(0)	3
2	0(2)	0(0)	3	0	0(2)	2(0)	0	2	0(2)	0(2)	2	2	0(2)	0(0)	3	2	0(2)	0(3)	0
3	0(0)	0(3)	0	3	0(0)	0(0)	3	2	0(0)	2(0)	0	3	0(0)	0(2)	2	3	0(0)	0(0)	3
2	0(2)	0(0)	3	2	0(2)	0(3)	0	2	0(2)	0(0)	3	0	0(2)	2(0)	0	2	0(2)	0(2)	2
3	0(0)	0(2)	2	3	0(0)	0(0)	3	3	0(0)	0(3)	0	3	0(0)	0(0)	3	2	0(0)	2(0)	0

 □

Lemma 3.

(a)

If $W_i=6$ and $W_{i-1}\ge 8,W_{i+1}\ge 8$ then either ($W_{i-1}+W_{i+1}\ge 20$)

or ($W_{i-2}+W_{i-1}\ge 19$, $W_{i+1}+W_{i+2}\ge 19$, and $W_{i-2}+W_{i-1}+W_{i+1}+W_{i+2}\ge 39$).

(b)

If $W_i=7$ and $W_{i-1}\ge 8,W_{i+1}\ge 8$ then either ($W_{i-1}+W_{i+1}\ge 18$)

or ($W_{i-2}+W_{i-1}\ge 18$ and $W_{i+1}+W_{i+2}\ge 18$).

Proof. 

As in the proof of Lemma 2, we will use the notation introduced in Table 3. We give only the values on the outer cycle, and in some cases the values on the inner cycles are provided in parenthesis, as $\mathbf{f}\left({\mathbf{u}}_{\mathbf{j}}\right)\left(f\left(v_j\right)\right)$. For other neighbor sets $H_{*}$ we will assume that the inner cycles $V_{*}$ are completed such that the whole assignment is a DRD function.

(a)

Case $W_i=6$ implies $f\left(U_i\right)=0$ (Figure 3). Recall that either three vertices in $V_i$ have weight 2 or two of them have weight 3, and that Table 9 gives the demands that need to be fulfilled by the neighboring $H_{*}$. As $W_{i-1}\ge 8$ and $W_{i+1}\ge 8$, according to lemma 1, we have $f\left(U_{i-1}\right)\ge 3$ and $f\left(U_{i+1}\right)\ge 3$. We may also assume that $f\left(U_{i-1}\right)\le f\left(U_{i+1}\right)$. Thus, we have $f\left(U_{i-1}\right)\in \{3,4,5,6\}$, and all cases are analyzed in Table 14, Table 15 and Table 16. Note that there is only one case for $f\left(U_{i-1}\right)=6=2+2+2$ (and $f\left(U_{i+1}\right)=6=2+2+2$), because if $f\left(U_{i-1}\right)=6=3+3$ then $f\left(U_{i+1}\right)=3<6$.

Reading Table 14 we observe that weights are (A1-1) ${}^{*}$ $W_{i-1}\ge 3+5=8$, $W_{i+1}\ge 6+3=9$, (A1-2)${}^{*}$ $W_{i-1}\ge 3+5=8$, $W_{i+1}\ge 6+5=11$, (A1-3)${}^{*}$ $W_{i-1}\ge 5+4=9$, $W_{i+1}\ge 5+5=10$, (A1-4)${}^{*}$ $W_{i-1}\ge 4+4=8$, $W_{i+1}\ge 7+4=11$, (A1-5)${}^{*}$ $W_{i-1}\ge 5+5=10$, $W_{i+1}\ge 5+4=9$, (A1-6) $W_{i-1}\ge 5+5=10$, $W_{i+1}\ge 5+5=10$, (A1-7) $W_{i-1}\ge 4+5=9$, $W_{i+1}\ge 7+4=11$, (A1-8) $W_{i-1}\ge 6+4=10$, $W_{i+1}\ge 6+4=10$, so in cases (A1-6), (A1-7), and (A1-8), $W_{i-1}+W_{i+1}\ge 20$. However, in the first five cases (labelled with asterisk), $W_{i-1}+W_{i+1}<20$, and therefore we need to consider $H_{i-2}$ and $H_{i+2}$ to conclude the proof of assertion (a) of Lemma 3, see Table 15.

From Table 15 we can estimate weights (A1-1)${}^{*}$ $W_{i-2}\ge 8+4=12$, $W_{i-1}\ge 3+5=8$, $W_{i+1}\ge 6+3=9$, $W_{i+2}\ge 6+5=11$, (A1-2)${}^{*}$ $W_{i-2}\ge 8+4=12$, $W_{i-1}\ge 3+5=8$, $W_{i+1}\ge 6+5=11$, $W_{i+2}\ge 5+5=10$, (A1-3)${}^{*}$ $W_{i-2}\ge 7+4=11$, $W_{i-1}\ge 5+4=9$, $W_{i+1}\ge 5+5=10$, $W_{i+2}\ge 5+5=10$, (A1-4)${}^{*}$ $W_{i-2}\ge 7+4=11$, $W_{i-1}\ge 4+4=8$, $W_{i+1}\ge 7+4=11$, $W_{i+2}\ge 4+5=9$, (A1-5)${}^{*}$ $W_{i-2}\ge 5+5=10$, $W_{i-1}\ge 5+5=10$, $W_{i+1}\ge 5+4=9$, $W_{i+2}\ge 7+4=11$. Here, in all cases, $W_{i-2}+W_{i-1}\ge 19$, $W_{i+1}+W_{i+2}\ge 19$ and $W_{i-2}+W_{i-1}+W_{i+1}+W_{i+2}\ge 39$.

Reading Table 16 we observe that (A2-1) $W_{i-1}\ge 6+5=11$, $W_{i+1}\ge 6+4=10$, (A2-2) $W_{i-1}\ge 6+4=10$, $W_{i+1}\ge 8+4=12$, (A2-3) $W_{i-1}\ge 6+4=10$, $W_{i+1}\ge 8+4=12$, (A2-4) $W_{i-1}\ge 4+5=9$, $W_{i+1}\ge 10+4=14$, (A2-5) $W_{i-1}\ge 5+5=10$, $W_{i+1}\ge 8+4=12$, (A2-6) $W_{i-1}\ge 5+4=9$, $W_{i+1}\ge 7+4=11$, (A2-7) $W_{i-1}\ge 5+5=10$, $W_{i+1}\ge 7+4=11$, (A2-8) $W_{i-1}\ge 3+5=8$, $W_{i+1}\ge 9+3=12$, (A2-9) $W_{i-1}\ge 6+4=10$, $W_{i+1}\ge 7+4=11$, (A2-10) $W_{i-1}\ge 6+4=10$, $W_{i+1}\ge 7+4=11$, (A2-11) $W_{i-1}\ge 4+4=8$, $W_{i+1}\ge 9+4=13$, (A2-12) $W_{i-1}\ge 4+5=9$, $W_{i+1}\ge 9+4=13$, (A2-13) $W_{i-1}\ge 6+4=10$, $W_{i+1}\ge 6+5=11$, (A2-14) $W_{i-1}\ge 4+5=9$, $W_{i+1}\ge 8+4=12$, (A2-15) $W_{i-1}\ge 4+4=8$, $W_{i+1}\ge 8+4=12$, so in all cases $W_{i-1}+W_{i+1}\ge 20$, which proves the assertion (a) of Lemma 3.

(b)

Case $W_i=7$. First, assume that $f\left(U_i\right)=2$ (see Figure 2), so one vertex in $U_i$ has weight 3 and the other one has weight 2. Possible (due to symmetry) solutions for the whole set $H_{i-1}\cup H_i\cup H_{i+1}$ are considered in the following Table 17.

Without loss of generality, assume that $f\left(U_{i-1}\right)\le f\left(U_{i+1}\right)$. As $W_{i-1}\ge 8$ and $W_{i+1}\ge 8$ we have the following subcases (see Table 18).

Reading Table 18 we observe that in all cases except one (B1-4) we have $W_{i-1}+W_{i+1}\ge 18$. Indeed, in (B1-1) $W_{i-1}\ge 5+5=10$, $W_{i+1}\ge 3+5=8$, (B1-2) $W_{i-1}\ge 4+5=9$, $W_{i+1}\ge 5+5=10$, (B1-3) $W_{i-1}\ge 4+4=8$, $W_{i+1}\ge 5+5=10$, (B1-4)${}^{*}$ $W_{i-1}\ge 3+5=8$, $W_{i+1}\ge 5+4=9$, (B1-5) $W_{i-1}\ge 3+5=8$, $W_{i+1}\ge 5+5=10$, (B1-6) $W_{i-1}\ge 4+5=9$, $W_{i+1}\ge 6+4=10$. In case (B1-4) we need to consider weights on $H_{i-2}$ and $H_{i+2}$ to conclude the proof of assertion (b) of Lemma 3.

As seen from Table 19, in case (B1-4) we can confirm $W_{i-2}+W_{i-1}\ge 18$ and $W_{i+1}+W_{i+2}\ge 18$.

Finally, if $f\left(U_i\right)=0$ (see Figure 4), then observe that in comparison to the case $f\left(U_i\right)=2$, there must be at least one more vertex of weight 2 in $U_{i-1}\cup U_{i+1}$. We omit detailed analysis of the cases that confirm $W_{i-1}+W_{i+1}\ge 18$.

Table 14. Subcases of $f\left(U_{i-1}\right)$, $f\left(U_i\right)$ and $f\left(U_{i+1}\right)$ with $W_i=6=3+3$.

(A1-1)${}^{*}$			(A1-2)${}^{*}$			(A1-3)${}^{*}$			(A1-4)${}^{*}$
3	0(0)	0	0	0(0)	3	0	0(0)	3	0	0(0)	3
0	0(3)	0	0	0(3)	0	0	0(3)	0	0	0(3)	0
0	0(0)	3	3	0(0)	0	3	0(0)	0	2	0(0)	2
0	0(0)	3	0	0(0)	3	2	0(0)	2	2	0(0)	2
0	0(3)	0	0	0(3)	0	0	0(3)	0	0	0(3)	0
(A1-5)${}^{*}$			(A1-6)			(A1-7)			(A1-8)
3	0(0)	0	2	0(0)	2	2	0(0)	2	2	0(0)	2
0	0(3)	0	0	0(3)	0	0	0(3)	0	0	0(3)	0
2	0(0)	2	3	0(0)	0	2	0(0)	2	2	0(0)	2
0	0(0)	3	0	0(0)	3	0	0(0)	3	2	0(0)	2
0	0(3)	0	0	0(3)	0	0	0(3)	0	0	0(3)	0

Table 15. Subcases${}^{*}$ of $f\left(U_{i-2}\right)$, $f\left(U_{i-1}\right)$, $f\left(U_i\right)$, $f\left(U_{i+1}\right)$ and $f\left(U_{i+2}\right)$ with $W_{i-1}+W_{i+1}<20$.

(A1-1)${}^{*}$					(A1-2)${}^{*}$					(A1-3)${}^{*}$
0	3(0)	0(0)	0(3)	0	3	0(0)	0(0)	3(0)	0	3	0(0)	0(0)	3(0)	0
3	0(0)	0(3)	0(0)	3	2	0(2)	0(3)	0(0)	3	2	0(2)	0(3)	0(0)	3
0	0(3)	0(0)	3(0)	0	0	3(0)	0(0)	0(3)	0	0	3(0)	0(0)	0(3)	0
3	0(0)	0(0)	3(0)	0	3	0(0)	0(0)	3(0)	0	0	2(0)	0(0)	2(0)	0
2	0(2)	0(3)	0(0)	3	0	0(3)	0(3)	0(2)	2	2	0(2)	0(3)	0(2)	2
(A1-4)${}^{*}$					(A1-5)${}^{*}$
3	0(0)	0(0)	3(0)	0	0	3(0)	0(0)	0(0)	3
2	0(2)	0(3)	0(2)	2	2	0(2)	0(3)	0(2)	2
0	2(0)	0(0)	2(0)	0	0	2(0)	0(0)	2(0)	0
0	2(0)	0(0)	2(0)	0	0	0(3)	0(0)	3(0)	0
2	0(2)	0(3)	0(2)	2	3	0(0)	0(3)	0(2)	2

Table 16. Subcases of $f\left(U_{i-1}\right)$, $f\left(U_i\right)$ and $f\left(U_{i+1}\right)$ with $W_i=6=2+2+2$.

(A2-1)			(A2-2)			(A2-3)			(A2-4)			(A2-5)
0	0(2)	2	0	0(2)	2	2	0(2)	0	0	0(2)	2	0	0(2)	2
0	0(2)	2	0	0(2)	2	0	0(2)	2	0	0(2)	2	0	0(2)	2
3	0(0)	0	2	0(0)	2	2	0(0)	2	2	0(0)	2	2	0(0)	2
0	0(2)	2	2	0(2)	0	0	0(2)	2	0	0(2)	2	0	0(2)	2
3	0(0)	0	2	0(0)	2	2	0(0)	2	2	0(0)	2	3	0(0)	0
(A2-6)			(A2-7)			(A2-8)			(A2-9)			(A2-10)
0	0(2)	2	2	0(2)	0	0	0(2)	2	2	0(2)	0	2	0(2)	0
0	0(2)	2	0	0(2)	2	0	0(2)	2	0	0(2)	2	2	0(2)	0
3	0(0)	0	3	0(0)	0	3	0(0)	0	2	0(0)	2	2	0(0)	2
2	0(2)	0	0	0(2)	2	0	0(2)	2	2	0(2)	0	0	0(2)	2
0	0(0)	3	0	0(0)	3	0	0(0)	3	0	0(0)	3	0	0(0)	3
(A2-11)			(A2-12)			(A2-13)			(A2-14)			(A2-15)
0	0(2)	2	2	0(2)	0	2	0(2)	0	2	0(2)	0	2	0(2)	0
0	0(2)	2	0	0(2)	2	2	0(2)	0	0	0(2)	2	2	0(2)	0
2	0(0)	2	2	0(0)	2	0	0(0)	3	0	0(0)	3	0	0(0)	3
2	0(2)	0	0	0(2)	2	2	0(2)	0	2	0(2)	0	0	0(2)	2
0	0(0)	3	0	0(0)	3	0	0(0)	3	0	0(0)	3	0	0(0)	3

Table 17. Possible values for $f\left(U_{i-1}\right)$, $f\left(U_i\right)$ and $f\left(U_{i+1}\right)$ with $W_i=7$ and $f\left(U_i\right)=2$.

(B1)
-	0	2(0)	0	-
-	2/0	0(2)	0/2	-
-	0/3/2	0(0)	3/0/2	-
-	0	0(3)	0	-
-	0/3/2	0(0)	3/0/2	-
$i-2$	$i-1$	i	$i+1$	$i+2$

Table 18. Subcases of $f\left(U_{i-1}\right)$, $f\left(U_i\right)$ and $f\left(U_{i+1}\right)$ with $W_i=7$, $f\left(U_i\right)=2$, $W_{i-1}\ge 8$ and $W_{i+1}\ge 8$.

(B1-1)			(B1-2)			(B1-3)			(B1-4)${}^{*}$
0	2(0)	0	0	2(0)	0	0	2(0)	0	0	2(0)	0
2	0(2)	0	2	0(2)	0	2	0(2)	0	0	0(2)	2
0	0(0)	3	0	0(0)	3	2	0(0)	2	0	0(0)	3
0	0(3)	0	0	0(3)	0	0	0(3)	0	0	0(3)	0
3	0(0)	0	2	0(0)	2	0	0(0)	3	3	0(0)	0
(B1-5)			(B1-6)
0	2(0)	0	0	2(0)	0
0	0(2)	2	0	0(2)	2
3	0(0)	0	2	0(0)	2
0	0(3)	0	0	0(3)	0
0	0(0)	3	2	0(0)	2

Table 19. Subcase (B1-4) with $W_{i-2}\ge 7+4=11$, $W_{i-1}\ge 3+5=8$, $W_{i+1}\ge 5+4=9$ and $W_{i+2}\ge 5+4=9$.

(B1-4)${}^{*}$
2	0(0)	2(0)	0(2)	0
0	0(3)	0(2)	2(0)	0
3	0(0)	0(0)	3(0)	0
2	0(2)	0(3)	0(2)	2
0	3(0)	0(0)	0(0)	3

 □

Theorem 8.

For all k we have ${\gamma }_{dR}\left(P(5k,k)\right)\ge 8k.$

Proof. 

We use the discharging method (see [14]). The basic idea is as follows. Assume that we have a DRDF. Consider certain subgraphs, in our case the subgraphs $H_i$, that are induced on $V_i\cup U_i$. Define some rules how the weights of heavy subgraphs are discharged to the neighbors such that the total weight does not change. Observe the weights of subgraphs after discharging.

In our case, the discharging rule is simple: If $f\left(H_i\right)=W_i>8$ then $H_i$ sends $\frac{1}{2}(W_i-8)$ to $H_{i-i}$ and to $H_{i-i}$. The new charge of $H_i$ is thus 8. We denote the charges after the first round by $W_i^{*}$.

Now we show that if $f\left(H_i\right)<8$ then, after at most four rounds of discharging, the new charge $W_i^{****}$ of $H_i$ is at least 8. Note that once the charge of $H_i$ is at least 8, discharging will never decrease its charge below 8.

First, let us consider the cases of Lemma 2.

(a)

If $W_i=6$ and $W_{i+1}=6$ then $W_{i-1}\ge 12$ and $W_{i+2}\ge 12$. After discharging, $W_i^{*}=8$ and $W_{i+1}^{*}=8$, as needed.

(b)

If $W_i=7$ and $W_{i+1}=7$ then $W_{i-1}\ge 11$ and $W_{i+2}\ge 11$. After discharging we have $W_i^{*}=7+\frac{3}{2}\ge 8$ and $W_{i+1}^{*}=7+\frac{3}{2}\ge 8$.

(c)

If $W_i=6$ and $W_{i+1}=7$ then $W_{i-1}\ge 11$, $W_{i+2}\ge 11$, and $W_{i-1}+W_{i+2}\ge 23$. Assume that $W_{i-1}\ge 11$, $W_{i+2}\ge 12$. After the first round of discharging, we get $W_i^{*}\ge 6+\frac{3}{2}=7+\frac{1}{2}$ and $W_{i+1}^{*}\ge 7+2=9$. However, after the second round of discharging, we have $W_i^{**}\ge 7+\frac{1}{2}+\frac{1}{2}=8$.

If $W_{i-1}\ge 12$, $W_{i+2}\ge 11$, then observe that already $W_i^{*}\ge 8$ and $W_{i+1}^{*}\ge 8$.

Next, we consider the cases of Lemma 3.

(a)

$W_i=6$ and $W_{i-1}\ge 8$, $W_{i+1}\ge 8$. If $W_{i-1}+W_{i+1}\ge 20$, then $W_i^{*}\ge 6+\frac{(20-16)}{2}=8$, and we are done. Otherwise, by Lemma 3, $W_{i-2}+W_{i-1}\ge 19$, $W_{i+1}+W_{i+2}\ge 19$, and $W_{i-2}+W_{i-1}+W_{i+1}+W_{i+2}\ge 39$. Assume that $W_{i-2}+W_{i-1}=19$ and distinguish two cases.

(a11)

$W_{i-1}=9$ and $W_{i-2}=10$. In the first round of discharging, $H_i$ receives $\frac{1}{2}$ from $H_{i-1}$, and $W_{i-1}^{*}=9-2·\frac{1}{2}+1=9.$ In the second round of discharging, $H_i$ again receives $\frac{1}{2}$ from $H_{i-1}$, and in total $H_i$ receives charge 1 from the left side.

(a12)

$W_{i-1}=8$ and $W_{i-2}=11$. After the first round of discharging, $W_{i-1}^{*}=8+\frac{3}{2}.$ In the second round of discharging, $H_{i-1}$ sends $\frac{3}{4}$ to its neighbors. Thus, $H_i$ receives $\frac{3}{4}$ from the left side.

Recall that by Lemma 3, $W_{i-2}+W_{i-1}=19$ implies $W_{i+1}+W_{i+2}\ge 20$, and distinguish two cases.

(a21)

$W_{i+1}=9$ and $W_{i+2}\ge 11$. In the first round of discharging, $H_i$ receives $\frac{1}{2}$ from $H_{i+1}$, and $W_{i+1}^{*}=9-2·\frac{1}{2}+\frac{3}{2}=8+\frac{3}{2}.$ In the second round of discharging, $H_i$ again receives $\frac{3}{4}$ from $H_{i+1}$, so in total $H_i$ receives charge $\frac{5}{4}$ from the right side.

(a22)

$W_{i+1}=8$ and $W_{i+2}\ge 12$. After the first round of discharging, $W_{i+1}^{*}\ge 8+2=10.$ In the second round of discharging, $H_i$ receives charge 1 from $H_{i+1}$, and also $W_{i+2}^{**}\ge 8+1=9.$ Thus, after the third round $W_{i+1}^{***}\ge 8+\frac{1}{2}$, and, in the fourth round $H_i$ receives charge $\frac{1}{4}$ from $H_{i+1}$. So, in total $H_i$ receives charge $\frac{5}{4}$ from the right side.

Summarizing, $H_i$ receives charge at least $\frac{3}{4}$ from the left side, and at least $\frac{5}{4}$ from the right side. Hence $W_i^{****}\ge 6+2=8$, as claimed.

(b)

$W_i=7$ and $W_{i-1}\ge 8,W_{i+1}\ge 8$. If $W_{i-1}+W_{i+1}\ge 18$ then $W_i^{*}\ge 7+(18-16)\frac{1}{2}=8$, as needed. Otherwise, $W_{i-2}+W_{i-1}\ge 18$ and $W_{i+1}+W_{i+2}\ge 18$. We now show that $W_{i-2}+W_{i-1}=18$ implies that $H_i$ will in two rounds receive at least $\frac{1}{2}$ charge from the left side. Consider two cases.

(b1)

$W_{i-1}=9$. In the first round of discharging, $H_i$ receives $\frac{1}{2}$ from the left side.

(b2)

If $W_{i-1}=8$, then $W_{i-2}\ge 10$. After the first round of discharging, $W_{i-1}^{*}\ge 8+1.$ In the second round of discharging, $H_{i-1}$ sends at least $\frac{1}{2}$ to its neighbors.

Thus, $H_i$ receives at least $\frac{1}{2}$ from the left side. By analogous reasoning, $W_{i+1}+W_{i+2}\ge 18$ implies that $H_i$ receives at least $\frac{1}{2}$ from the right side. Consequently, in total $H_i$ receives at least $\frac{1}{2}+\frac{1}{2}$, as claimed.

Therefore, after discharging, each subgraph $H_i$ has weight $W_i$ at least 8, and consequently, the total weight is at least $8k$, as claimed. □

4. Domination in Generalized Petersen Graphs $\mathit{P}(\mathbf{20}\mathit{k},\mathit{k})$

In this section, we discuss how the constructions, and the corresponding upper bounds can be extended from $P(c_{0}k,k)$ to $P(\left(h{c}_0\right)k,k)$, for $h=2,3,\cdots$. In particular, we obtain improved upper bounds for $P(20k,k)$ from upper bounds for $P(4k,k)$ and $P(5k,k)$.

First, we recall the notion of covering graph and h-lift to observe that $P(20k,k)$ is a covering graph of both $P(4k,k)$ and $P(5k,k)$. For basic information on covering graphs see [39]. Here we follow the approach used in [40]. Let $G=(V_1,E_1)$ and $H=(V_2,E_2)$ be two graphs, and let $p:V_2\to V_1$ be a surjection. We will call p a covering map from H to G if for each $v\in V_2$, the restriction of p to the neighborhood of $v\in V_2$ is a bijection onto the neighborhood of $p\left(v\right)$ in G. In other words, p maps edges incident to v one-to-one onto edges incident to $p\left(v\right)$. H is called a covering graph, or a lift, of G if there exists a covering map from H to G. Assuming H is a lift of G with a covering map p. If p has a property that for every vertex $v\in V\left(G\right)$, its fiber $p^{-1}\left(v\right)$ has exactly h elements, we call H a h-lift of G.

Obviously, a long cycle may be a covering graph of shorter cycles. For example, the cycle $C_{100}$ is a 2-lift of $C_{50}$, considering the surjection $p\left(v_i\right)=v_{imod50}$. Furthermore, $C_{100}$ is also a 25-lift of $C_4$, etc.

Proposition 7.

Let $k\ge 1$, $c_0\ge 3$, and $h\ge 2$. Petersen graph $P(\left(h{c}_0\right)k,k)$ is a h-lift of $P(c_{0}k,k)$.

Proof. 

Consider the surjection $p:V\left(P(\left(h{c}_0\right)k,k)\right)\to V\left(P(c_{0}k,k)\right)$ defined by $p\left(v_i\right)=v_{imodh}$, and $p\left(u_i\right)=u_{imodh}$. □

Proposition 8.

${\gamma }_{dR}\left(P(\left(h{c}_0\right)k,k)\right)\le h{\gamma }_{dR}\left(P(c_{0}k,k)\right)$.

Proof. 

Let f be a DRDF of $P(c_{0}k,k)$. Define $\tilde{f}$ as $\tilde{f}\left(v\right)=f\left(p\left(v\right)\right)$ and observe that $\tilde{f}$ is a DRDF of $P(\left(h{c}_0\right)k,k)$. Clearly, the weight $\tilde{f}\left(P(\left(h{c}_0\right)k,k)\right)$ is exactly $h\tilde{f}(P\left((c_{0}k,k)\right)$. We omit the details. □

Corollary 3.

${\gamma }_{dR}\left(P(20k,k)\right)\le 5{\gamma }_{dR}\left(P(4k,k)\right)\le 30k+15$.

As ${\gamma }_{dR}\left(P(20k,k)\right)\ge \frac{3}{2}20k=30k$ by Corollary 1, we also have

Corollary 4.

If $k\equiv 1mod2$, then ${\gamma }_{dR}\left(P(20k,k)\right)=30k$.

Applying Proposition 8 to the case $P(20k,k)$ and $P(5k,k)$, we obtain another Corollary.

Corollary 5.

${\gamma }_{dR}\left(P(20k,k)\right)\le 4{\gamma }_{dR}\left(P(5k,k)\right)=32k+8$.

Clearly, the upper bound in Corollary 5 is only better for $k=1$, 2, and 3. In these cases, we obtain

$${\gamma }_{dR}\left(P(20,1)\right)\le 40,{\gamma }_{dR}\left(P(40,2)\right)\le 64,{\gamma }_{dR}\left(P(60,3)\right)\le 96,$$

(3)

which, together with Corollary 3 and Theorem 3 implies Theorem 7.

Note that this bound is a considerable improvement over the general bounds given in Theorem 4 [12]. Indeed, the upper bound (2) grows as $\mathcal{O}\left(30k\right)$. The bounds in Theorem 4 are of the from $\frac{3}{2}\left(20k\right)F\left(k\right)+\frac{5k}{4}+C$, where ${lim}_{k\to \infty }F\left(k\right)=1$, so the asymptotic growth is $\mathcal{O}(30k+\frac{5k}{4})$.

5. Conclusions and Future Work

In this paper, we have extended the known results on double Roman domination of families $P(ck,k)$ of generalized Petersen graphs, by adding either exact values or bounds with gap at most 2 for the family $P(5k,k)$. This naturally continues previous work, where the families $P(3k,k)$ and $P(4k,k)$ were studied.

There are several interesting related questions that open avenues for future work. For example:

• Find closed expressions or good lower and upper bounds for ${\gamma }_{dR}\left(P(6k,k)\right)$. Which graphs among $P(6k,k)$ are double Roman?
• The method used here to improve bounds for ${\gamma }_{dR}\left(P(20k,k)\right)$ using ${\gamma }_{dR}\left(P(4k,k)\right)$ and ${\gamma }_{dR}\left(P(5k,k)\right)$ may be used to improve bounds for ${\gamma }_{dR}\left(P(ck,k)\right)$ for larger c.
• Can the small gaps between lower and upper bounds for ${\gamma }_{dR}\left(P(5k,k)\right)$ (and, also for ${\gamma }_{dR}\left(P(4k,k)\right)$) be resolved by finding and proving exact values?

The authors believe that this study has solved the problem on $P(5k,k)$ to the limits of the standard method. These methods may be sufficient to handle the problem, e.g., on $P(6k,k)$, but probably can not be applied to much larger c. Covering graphs, as indicated in Section 4, may provide a tool to provide improved bounds for larger c. On the other hand, the gaps between the lower and upper bounds in some cases may be solved by other methods, see for example [41] and the references there.

More generally, this work again shows the power of the discharging method. The discharging method is most well known for its central role in the proof of the four color theorem. This proof technique was extensively applied to study various graph coloring problems, in particular on planar graphs. In [14], it is shown that a suitably altered discharging technique can also be used on domination type problems and is illustrated on the double Roman domination on some generalized Petersen graphs. Here, we apply the method to another family of graphs and the same problem. This may encourage future applications to other domination type problems.