Interactive Learning: Unpacking the Influence of Computer Simulations on Students’ Mathematical Modeling Processes
Abstract
1. Introduction
2. Review of the Literature
2.1. Mathematical Modeling
2.2. Use of Technology in Developing Mathematical Models of Complex Systems
2.3. Use of Computer Simulations for Mathematical Modeling
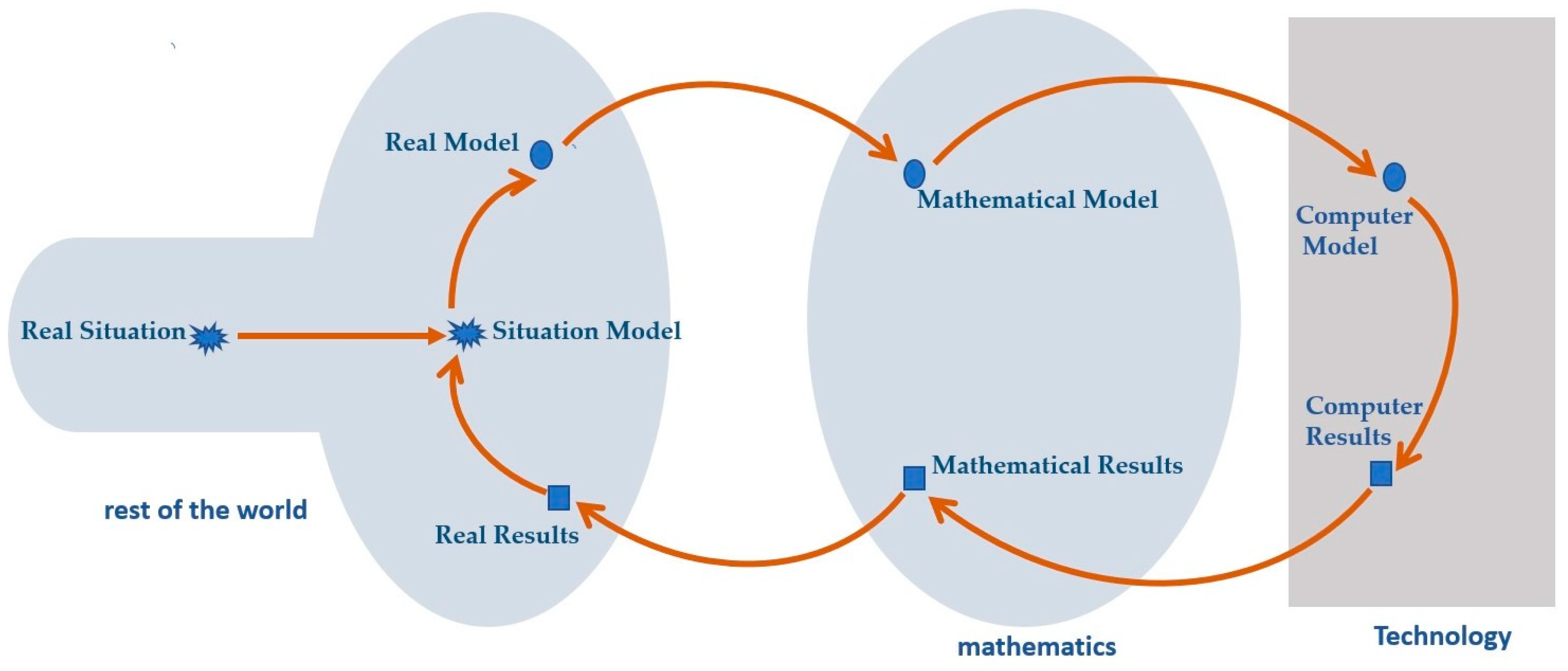
3. Theoretical Framework
4. Methodology
4.1. Research Design and Data Collection
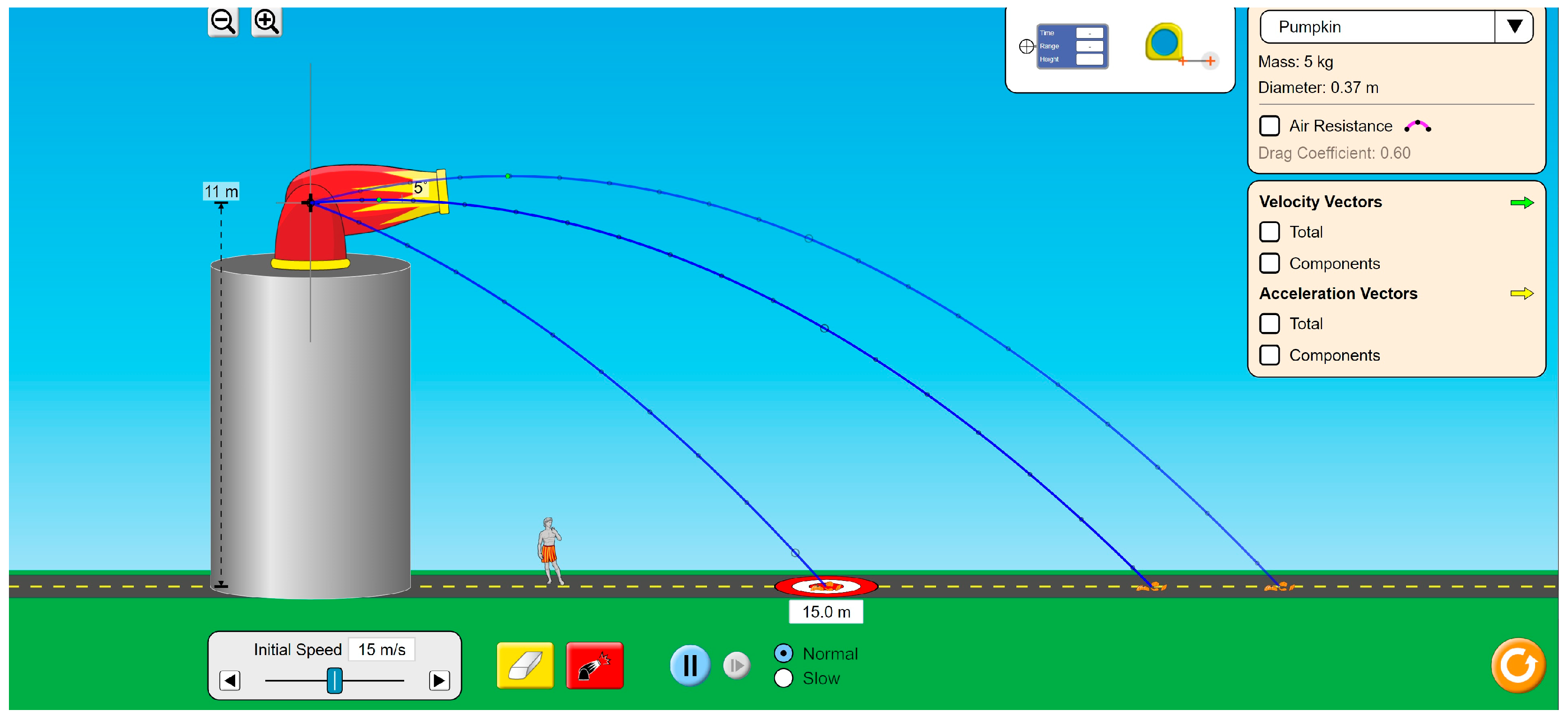
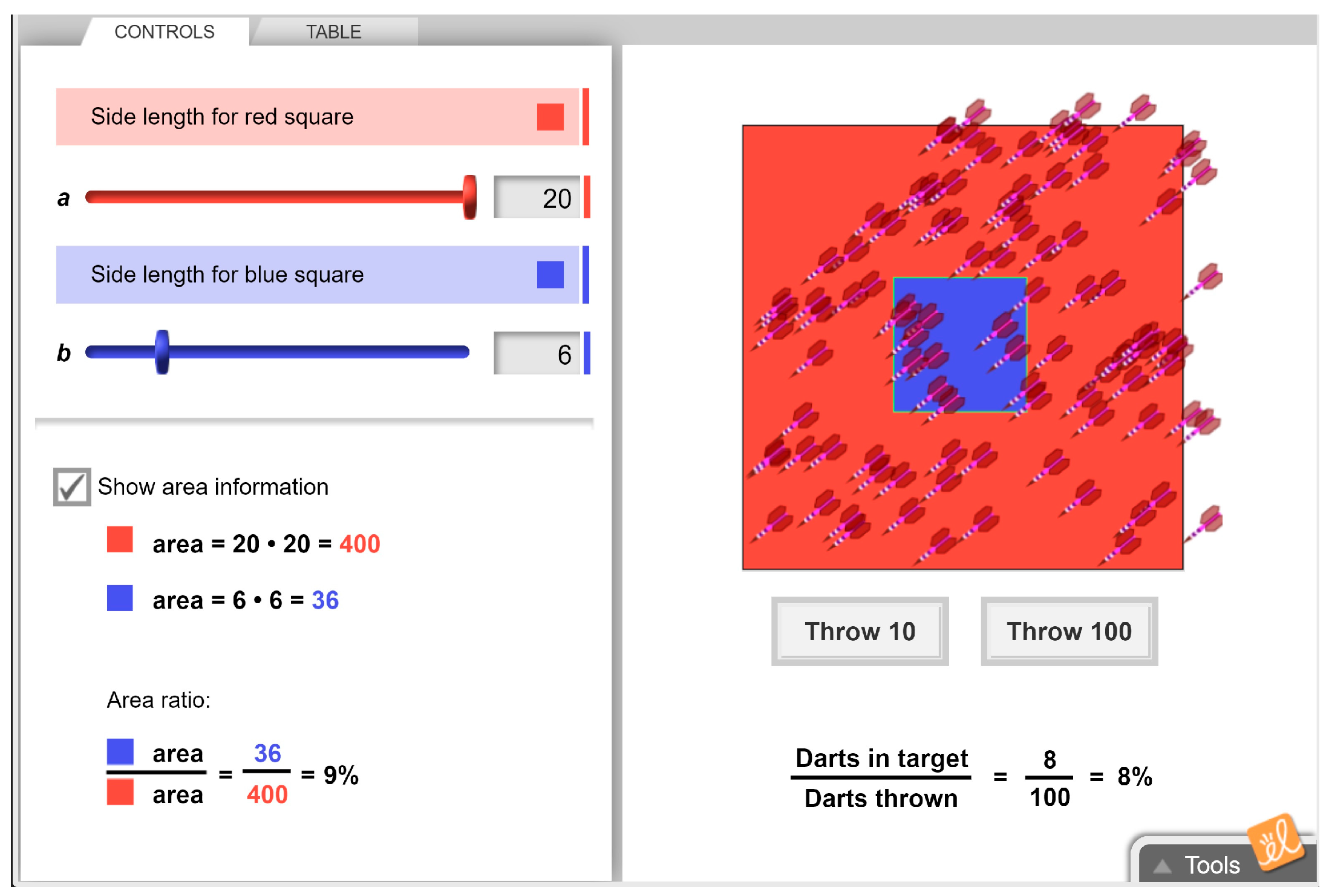
4.2. Task Selection
4.3. Data Analysis Process
5. Results
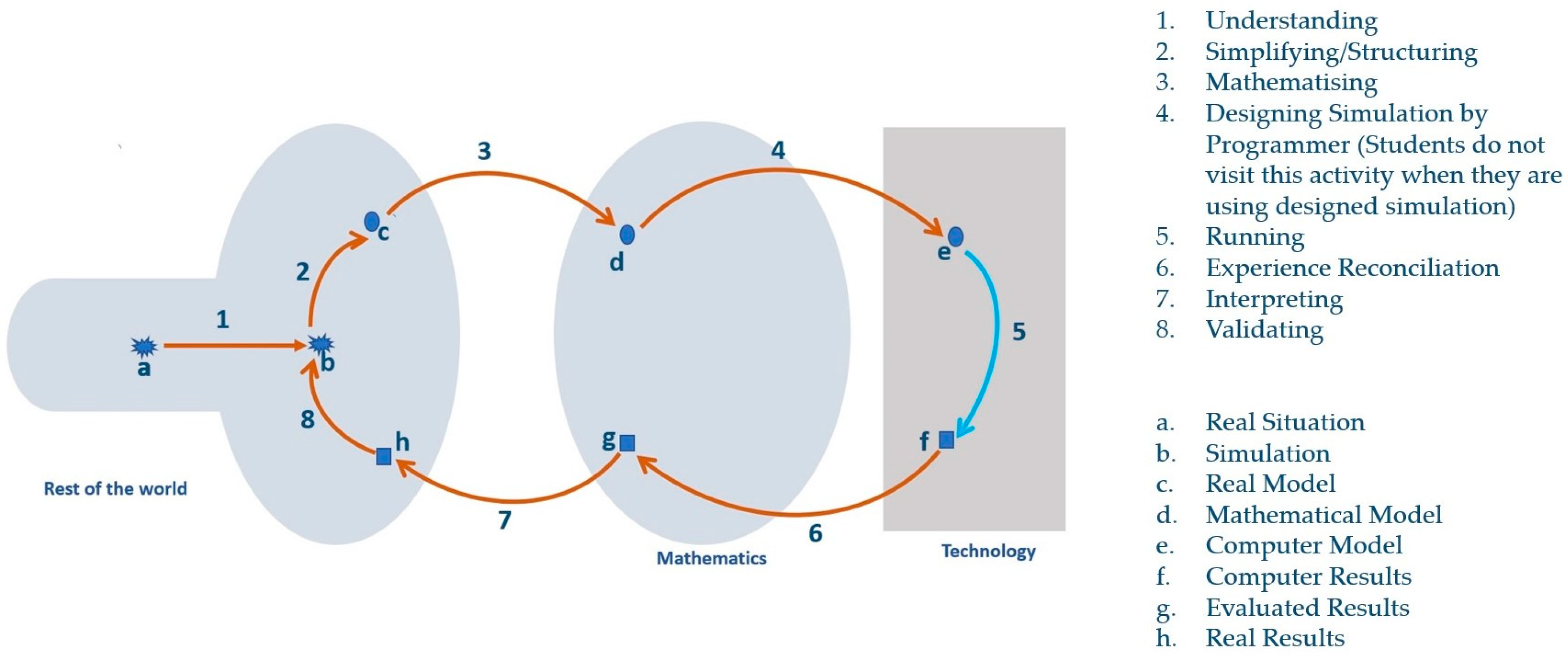
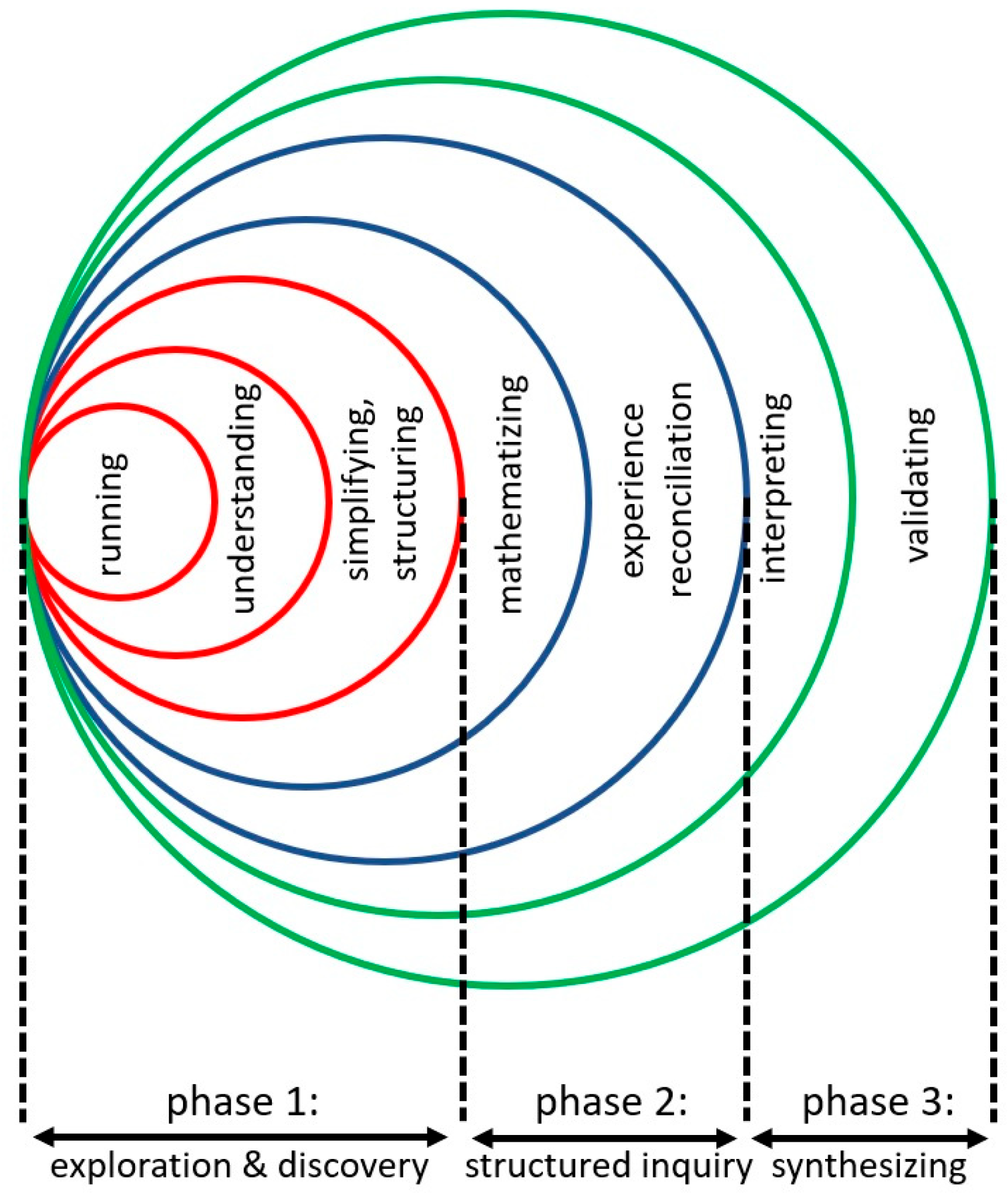
5.1. Adapting the Extended Blum Modeling Cycle to Student Activities
5.2. Generating Modeling Activity Diagram (MAD)
5.3. A Three-Stage Model of Learning
- Student A:
- … also I realized that it would double such as … the first square, the second square … like it would double the squares [showing the square units in the graph] and then I tripled it and I got … umm … 12, 4 and 12 [12 for head start, 4 for mouse speed and 12 for cat speed] and I simulated and it did triple there too [showing the point that the cat catches the mouse] and I realize that it doubled again in how many squares … because it was two squares high and now it’s four squares high and also it is 18 units [showing the y component of the intersection point] … So it doubled again
- Interviewer:
- So, now, I have a question. Your numbers are seven, 12 and 22, okay? So, if I increase … We just want to predict what will happen. So, if we increase the mouse’s speed, okay? Without using the simulation, I want you to predict, by increasing the mouse’s speed, will the cat still catch the mouse?
- Student B:
- Well, if the cat can catch the mouse now, if we make the speed higher, I’m guessing the cat can’t catch the mouse, because right now the speed is lower than the cat.
- Interviewer:
- Okay.
- Student B:
- If both … It was showing and, what, in comparison? … I was thinking, if we increase the speed, the mouse not only has a head start, but he has the advantage of more speed …That’s why I think that the mouse would win.
6. Discussion
7. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kaiser, G. Mathematical modelling and applications in education. In Encyclopedia of Mathematics Education; Springer: Berlin/Heidelberg, Germany, 2020; pp. 553–561. [Google Scholar]
- Blum, W.; Galbraith, P.L.; Henn, H.W.; Niss, M. (Eds.) Modelling and applications in mathematics education. In Modelling and Applications in Mathematics Education. The 14th ICMI Study; Springer: New York, NY, USA, 2007; pp. 1–14. [Google Scholar]
- Greer, B.; Verschaffel, L.; Mukhopadhyay, S. Modelling for life: Mathematics and children’s experience. In Applications and Modelling in Mathematics Education: ICMI Study 14; Blum, W., Henne, W., Niss, M., Eds.; Kluwer: Dordrecht, Germany, 2007; pp. 89–98. [Google Scholar]
- Stein, M.K.; Smith, M.S.; Henningsen, M.A.; Silver, E.A. Implementing Standards-Based Mathematics Instruction: A Casebook for Professional Development, 2nd ed.; National Council of Teachers of Mathematics: Reston, VA, USA; Teacher College Press: New York, NY, USA, 2009. [Google Scholar]
- Asempapa, R.S. Mathematical modeling: Essential for elementary and middle school students. J. Math. Educ. 2015, 8, 16–29. [Google Scholar]
- Flevares, L.M.; Schiff, J.R. Engaging young learners in integration through mathematical modeling: Asking big questions, finding answers, and doing big thinking. Adv. Early Educ. Day Care 2013, 17, 33–56. [Google Scholar]
- Doerr, H.M.; English, L.D. A modeling perspective on students’ mathematical reasoning about data. J. Res. Math. Educ. 2003, 34, 110–136. [Google Scholar] [CrossRef]
- Stohlmann, M.S.; Albarracín, L. What is known about elementary grades mathematical modelling. Educ. Res. Int. 2016, 20, 1–9. [Google Scholar] [CrossRef]
- Suh, J.M.; Matson, K.; Seshaiyer, P. Engaging Elementary Students in the Creative Process of Mathematizing Their World through Mathematical Modeling. Educ. Sci. 2017, 7, 62. [Google Scholar] [CrossRef]
- Borromeo Ferri, R. Modelling problems from a cognitive perspective. In Mathematical Modeling: Education, Engineering, and Economics; Haines, C., Galbraith, P., Blum, W., Khan, S., Eds.; Woodhead Publishing Limited: Cambridge, UK, 2007; pp. 260–270. [Google Scholar]
- National Council of Teachers of Mathematics. Principles and Standards for School Mathematics; National Council of Teachers of Mathematics: Reston, VA, USA, 2000. [Google Scholar]
- Niemiec, R.P.; Walberg, H.J. The effects of computers on learning. Int. J. Educ. Res. 1992, 17, 99–108. [Google Scholar] [CrossRef]
- Shwalb, D.; Shwalb, B.; Azuma, H. Educational technology in Japanese schools: A meta-analysis of findings. Educ. Technol. Res. 1986, 9, 13–30. [Google Scholar]
- Williams, C. Internet Access in Public Schools: 1994–1999 (NCES 2000-086); U.S. Department of Education, National Center for Educational Statistics: Washington, DC, USA, 2000.
- Geiger, V. Factors affecting teachers’ adoption of innovative practices with technology and mathematical modelling. In Trends in the Teaching and Learning of Mathematical Modelling; Kaiser, G., Blum, W., Ferri, R.B., Stillman, G., Eds.; Springer: New York, NY, USA, 2011; pp. 305–314. [Google Scholar]
- Greefrath, G.; Siller, H.S. Modelling and simulation with the help of digital tools. In Mathematical Modelling and Applications: Crossing and Researching Boundaries in Mathematics Education; Springer: Berlin/Heidelberg, Germany, 2017; pp. 529–539. [Google Scholar]
- English, L.D.; Fox, J.L.; Watters, J.J. Problem posing and solving with mathematical modeling. Teach. Child. Math. 2005, 12, 156–163. [Google Scholar] [CrossRef]
- Lesh, R.; Fennewald, T. Introduction to Part I Modeling: What Is It? Why Do It? In Modeling Students’ Mathematical Modeling Competencies; Springer: New York, NY, USA, 2010; pp. 5–10. [Google Scholar]
- Lesh, R.; Doerr, H.M. (Eds.) Beyond Constructivism: Models and Modeling Perspectives on Mathematics Problem Solving, Learning, and Teaching; Lawrence Erlbaum Associates: Mahwah, NJ, USA, 2003. [Google Scholar]
- Dym, C.L. Principles of Mathematical Modeling, 2nd ed.; Elsevier Academic Press: Amsterdam, The Netherlands; Boston, MA, USA, 2004. [Google Scholar]
- Blum, W.; Borromeo Ferri, R. Mathematical modelling: Can it be taught and learnt? J. Math. Model. Appl. 2009, 1, 45–58. [Google Scholar]
- Lingefjärd, T. Assessing Engineering Student’s Modeling Skills. 2004. Available online: http://wvvw.cdio.org/files/document/file/assess_model_skls.pdf (accessed on 15 December 2021).
- Blum, W.; Leiß, D. How do students and teachers deal with modelling problems? Math. Model. 2007, 12, 222–231. [Google Scholar] [CrossRef]
- Czocher, J.A. Mathematical Modeling Cycles as a Task Design Heuristic. Math. Enthus. 2017, 14, 129. [Google Scholar] [CrossRef]
- Hmelo-Silver, C.E.; Duncan, R.G.; Chinn, C.A. Scaffolding and achievement in problem-based and inquiry learning: A response to Kirschner, Sweller, and Clark. Educ. Psychol. 2007, 42, 99–107. [Google Scholar] [CrossRef]
- Lesh, R. Modeling students modeling abilities: The teaching and learning of complex systems in education. J. Learn. Sci. 2006, 15, 45–52. [Google Scholar] [CrossRef]
- English, L.D. Complex systems in the elementary and middle school mathematics curriculum: A focus on modeling. Festschrift in Honor of Gunter Torner. Mont. Math. Enthus. 2007, 13, 139–156. [Google Scholar]
- Klopfer, E.; Yoon, S. Developing games and simulations for today and tomorrow’s tech savvy youth. TechTrends 2004, 49, 33–34. [Google Scholar] [CrossRef]
- Lee, H.; Plass, J.L.; Homer, B. Optimizing cognitive load for learning from computer science simulations. J. Educ. Psychol. 2006, 98, 902–913. [Google Scholar] [CrossRef]
- Park, S.I.; Lee, G.; Kim, M. Do students benefit equally from interactive computer simulations regardless of prior knowledge levels? Comput. Educ. 2009, 52, 649–655. [Google Scholar] [CrossRef]
- Rieber, L.P.; Tzeng, S.; Tribble, K. Discovery learning, representation, and explanation within a computer-based simulation: Finding the right mix. Learn. Instr. 2004, 14, 307–323. [Google Scholar] [CrossRef]
- Chilcott, M.J.D. Effective Use of Simulations in the Classroom; CFSD System Dynamics Project: Tucson, AZ, USA, 1996. [Google Scholar]
- Greefrath, G. Using technologies: New possibilities of teaching and learning modelling–Overview. In Trends in Teaching and Learning of Mathematical Modelling; Springer: Amsterdam, The Netherlands, 2011; pp. 301–304. [Google Scholar]
- Czocher, J.A. Toward a Description of How Engineering Students Think Mathematically. Ph.D. Thesis, Ohio State University, Columbus, OH, USA, 2013. Available online: http://rave.ohiolink.edu/etdc/view?acc_num=osu1371873286 (accessed on 10 August 2018).
- Maher, C.A.; Sigley, R. Task-based interviews in mathematics education. In Encyclopedia of Mathematics Education; Springer: Amsterdam, The Netherlands, 2014; pp. 579–582. [Google Scholar]
- Ärlebäck, J.B. On the use of realistic Fermi problems for introducing mathematical modelling in school. Mont. Math. Enthus. 2009, 6, 331–364. [Google Scholar] [CrossRef]
- Greefrath, G.; Vorhölter, K. Teaching and Learning Mathematical Modelling: Approaches and Developments from German Speaking Countries; Springer: New York, NY, USA, 2016; pp. 1–42. [Google Scholar]
- Borromeo Ferri, R. Learning How to Teach Mathematical Modelling—In School and Teacher Education; Springer: New York, NY, USA, 2018. [Google Scholar]


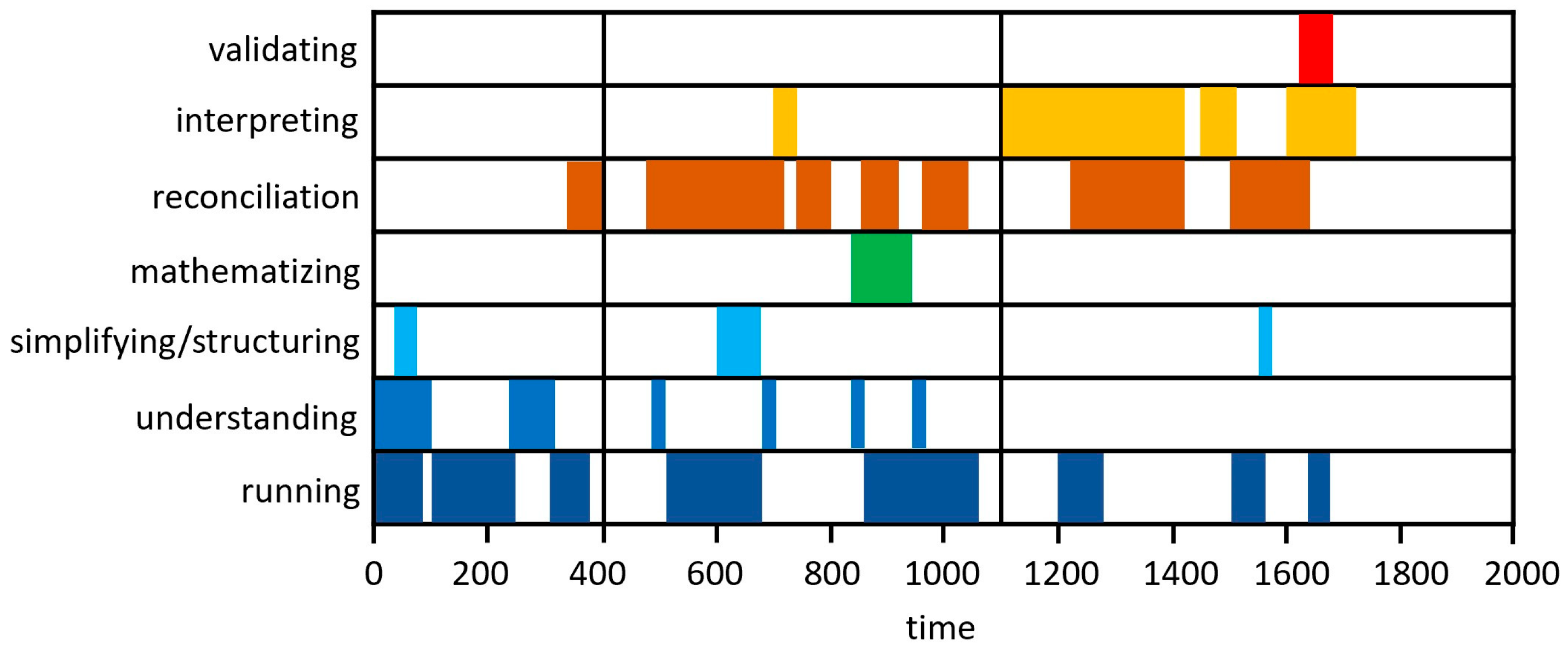
| Time (Seconds) | Modeling Activities (Code) | Evidence from Participants’ Interactions with Simulation (Indicator) | |
|---|---|---|---|
| 0:00:00 | 0:00:20 | Understanding | Student reads the problem |
| 0:00:20 | 0:00:40 | Understanding, Running | She tries to see how the simulation works, changing the angle (graphically), runs |
| 0:00:40 | 0:01:00 | Understanding, Running | Plays with height and angle (graphically) |
| 0:01:00 | 0:01:20 | Understanding, Running | Plays with height and angle (graphically) |
| 0:01:20 | 0:01:40 | Understanding, Running, Simplifying | Angle on 45 (middle), height on maximum |
| 0:01:40 | 0:02:00 | Running, Conditional | Runs and it does not hit, fixes all variables and reduces the speed |
| 0:02:00 | 0:02:20 | Understanding, Running, Simplifying | Fixes one variable and changes two others; runs each time to see the results |
| 0:02:20 | 0:02:40 | Running | Runs the new setting |
| 0:02:40 | 0:03:00 | Running, Simplifying | Fixes one variable and changes two others; runs each time to see the results |
| 0:03:00 | 0:03:20 | Understanding, Simplifying | Tries to understand the effect of different objects, fixing one variable at time |
| 0:03:20 | 0:03:40 | Understanding, Running, Simplifying | Maximizing and minimizing variable values, running each setting |
| 0:03:40 | 0:04:00 | Understanding, Running, Simplifying | Maximizing and minimizing variable values, running each setting |
| 0:04:00 | 0:04:20 | Understanding, Running, Simplifying | Maximizing and minimizing variable values, running each setting |
| 0:04:20 | 0:04:40 | Understanding, Running, Simplifying | Maximizing and minimizing variable values, running each setting |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sanjari, A.; Manouchehri, A. Interactive Learning: Unpacking the Influence of Computer Simulations on Students’ Mathematical Modeling Processes. Educ. Sci. 2024, 14, 397. https://doi.org/10.3390/educsci14040397
Sanjari A, Manouchehri A. Interactive Learning: Unpacking the Influence of Computer Simulations on Students’ Mathematical Modeling Processes. Education Sciences. 2024; 14(4):397. https://doi.org/10.3390/educsci14040397
Chicago/Turabian StyleSanjari, Azin, and Azita Manouchehri. 2024. "Interactive Learning: Unpacking the Influence of Computer Simulations on Students’ Mathematical Modeling Processes" Education Sciences 14, no. 4: 397. https://doi.org/10.3390/educsci14040397
APA StyleSanjari, A., & Manouchehri, A. (2024). Interactive Learning: Unpacking the Influence of Computer Simulations on Students’ Mathematical Modeling Processes. Education Sciences, 14(4), 397. https://doi.org/10.3390/educsci14040397





