Next Article in Journal
Assessing the Impact of AI Education on Hispanic Healthcare Professionals’ Perceptions and Knowledge
Previous Article in Journal
A Novel Influence Analysis-Based University Major Similarity Study
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Education Sciences
Volume 14
Issue 4
10.3390/educsci14040338
education-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Speech and Gesture Complementarity in a Preschooler’s Conceptualization of Mechanical Equilibrium

by
Maria-Eleni Chachlioutaki
1 and
Panagiotis Pantidos
2,*
1
School of Early Childhood Education, Aristotle University of Thessaloniki, 54124 Thessaloniki, Greece
2
Department of Early Childhood Education, National and Kapodistrian University of Athens, 10680 Athens, Greece
*
Author to whom correspondence should be addressed.
Educ. Sci. 2024, 14(4), 338; https://doi.org/10.3390/educsci14040338
Submission received: 16 February 2024 / Revised: 12 March 2024 / Accepted: 20 March 2024 / Published: 22 March 2024
(This article belongs to the Section Early Childhood Education)
Browse Figures
Versions Notes

Abstract

:
Considering learning to be situated and modally defined, the purpose of this paper was to identify and describe action structures as thought structures. We aimed to investigate the modal patterns that emerge during the learning process through a case study that examines how a 5-year-old preschool child conceptualizes the dimensions of mechanical equilibrium. Three identical tests were designed to elicit different modal responses from the student. These tests consisted of three tasks that differed semiotically from one another. The tests were conducted at different time points and were interspersed with two contextually distinct teaching interventions. The findings demonstrate that, while conceptualizing mechanical equilibrium, the student constructed meanings through semantic supplements of speech, bodily expression, and drawing, thus creating a form of multimodal syntax to express their thoughts. Complementarity evidently contributes to the concept of variability and serves as components of learning, which is perceived as a dynamic process.

1. Introduction

Certain contemporary approaches suggest that learning inherently links to multimodality, wherein students activate various modes of expression to create meanings [1,2,3,4,5]. In the realm of science teaching and learning, several researchers have demonstrated how different semiotic systems, employed to represent concepts in physical space, explicitly influence the construction of meanings [6,7,8]. The multitude of semiotic modes present within a learning environment does not merely serve as explicit representations of pre-existing mental constructs. Instead, the semiotic profile of the learning context creates a distinct conceptual foundation upon which students express their thoughts, influenced by their pre-existing mental representations. Vosniadou et al. [9] explored variations in questioning methods regarding the earth’s shape and the day/night cycle, aiming to understand how different inquiry approaches influence children’s reasoning. By comparing forced-choice questioning (FCQ) with open questioning (OQ), they revealed that the method of inquiry significantly impacts children’s responses and reasoning coherence, with FCQ eliciting more scientifically correct responses but less consistent reasoning compared to OQ. To the same trajectory, Ping and Goldin-Meadow [10] investigated the role of iconic gestures in enhancing learning, specifically regarding quantity conservation. Their study contrasted teaching methods involving gestures combined with speech versus speech alone, demonstrating how multimodal instruction can lead to more effective learning outcomes. Hadzigeorgiou et al. [11] investigated the influence of sensorimotor tasks on preschoolers’ understanding of mechanical equilibrium. They demonstrated, among other findings, that preschool children who engaged in kinesthetic activities, attempting to balance on a beam, retained their ability to apply the rules of balance compared to a control group that participated in preparatory activities using typical (plastic) mechanical balances. This highlights the importance of embodied cognition and active engagement in learning processes. Overall, these studies underscore the significance of instructional methods and contextual factors in shaping children’s cognitive development and learning outcomes.
In this framework, semiotic resources traditionally unrelated to meaning production come to the forefront, assuming an active role in learning. For instance, Goldin-Meadow [12] asserted that gestures do not solely reflect prior knowledge but are intrinsically linked to conceptual development. Numerous researchers have argued that gestures contribute to conceptual improvement rather than solely clarifying speech and can foster the evolution of reasoning skills [13]. In particular, action-based learning plays a pivotal role in the construction of scientific concepts. Kontra et al. [14] examined the learning of torque and angular momentum through hands-on experiences. Two groups of students participated in pre/posttest assessments, with one group engaging in the active manipulation of a wheel under various conditions (action group), while the other group observed the first group’s activities (observation group). Both groups received the same oral instructions. Despite comparable pretest performance, the group that was physically active during the intervention demonstrated greater improvement in the posttest compared to the observation group. Even for phenomena in the microworld, such as the particle nature of matter and the relationship between temperature and molecular motion, students’ active involvement contributes to enhanced learning outcomes [15]. This preference for bodily engagement over speech is also observed in laboratory settings, where students frequently resort to gestures for meaning-making rather than relying solely on speech. Over time, students initially explore materials and devices by manipulating them, utilizing iconic gestures to communicate effectively. Gradually, the predominance of gestures diminishes, allowing speech to overcome its initial limitations and participate in the conceptualization of scientific entities [2,16].
Particularly for preschoolers, embodied thinking represents a form of covert cognition, as children often rely on bodily engagement for thinking, a practice that thrives when the semiotic context encourages bodily expression. Generally, research has indicated that preschool-age children develop scientific concepts through diverse pathways. For instance, in a multimodal approach involving preschoolers’ understanding of earthquakes, Chachlioutaki et al. [3] demonstrated through a pre/posttest research design that as children develop their semiotic modes, they concurrently enhance their reasoning abilities regarding the conceptual aspects of earthquakes. Notably, bodily expressions and drawings conveyed meanings related to the origin of earthquakes and the movement of lithospheric plates that speech alone failed to capture. Ιn another instance, Herakleioti and Pantidos [17] demonstrated that preschool children used oral language to conceptualize the area of shadow formation while employing their body expression to convey the journey of light and obstruction. In preschool education, in addition to oral language, children’s drawings traditionally serve as a means of expressing and generating ideas and reasoning about concepts and phenomena in the natural sciences. Nevertheless, the analysis of drawings has been extended to include the examination of children’s gestures. Children’s thinking, as expressed through drawings, has two dimensions. It encompasses conceptualizations conveyed through the content of the drawing itself and those that arise from the verbal and gestural explanations accompanying the drawing [18,19].
It is worth noting that the field of early childhood science education has been shaped for decades, considering both the psychological and social aspects of learning at this age and various trends in science education. From this perspective, school science in early childhood education exhibits features of the Piagetian framework, socio-cognitive approaches, and socio-cultural approaches [20]. In the Piagetian framework, cognitive development is fostered through organized physical knowledge activities that enhance children’s ability to work with substances and objects. This documentation aids in assessing and predicting the reorganization of space and material choices to promote more effective interactions [21,22]. A framework influenced by post-Piagetian and Vygotskian theories emphasizes addressing the cognitive constraints of young children for significant progress in processing concepts in natural sciences. In this socio-cognitive approach, children participate in organized communication, argumentation, prediction, and exploration, utilizing multiple representation systems to reconstruct their reasoning [23,24]. Socio-cultural approaches, influenced by Vygotskian theories, emphasize the qualitative study of young children’s development of scientific thinking in relation to their cultural and social surroundings. Pedagogical materials and activities enable the simultaneous emergence of intellectual, affective, bodily, and social aspects in classroom situations, encouraging children’s creativity and diverse approaches to the physical world [25,26].
In all three theoretical currents of early childhood science education, multimodality is a significant concept. In the Piagetian framework, physical knowledge activities are action-based activities that essentially focus on children’s (embodied) interaction with the material context. Socio-cognitive approaches, allowing children to reconstruct their mental representations through multiple representation systems, refer to the ability of thinking to be shared in various semiotic modes. In the socio-cultural theoretical current, as a framework leading to the creation of multifactorial situations in the classroom, it is evident that multimodality is an inherent element. Overall, the role of multimodality holds particular value at the preschool age, as children find it challenging to fully express their thoughts [27], preferring to communicate through physicality and the manipulation of objects [28,29]. Multimodality contributes to the development of preschool children’s expression in different ways, enriching thinking with the ability to construct concepts as products of transition through various contexts [30].
Furthermore, given that the mental representations of scientific entities intertwine with the semiotic modes that students employ, we can assume the following conclusions, which relevant research in cognitive sciences and science education supports: (a) learning situates within an embodied context, where any expression in the environment constitutes thinking (action-based) [31,32], (b) human expression, i.e., thinking, is not merely a recollection of existing knowledge but a co-construction resulting from the interaction between the student’s cognitive world and the immediate environment (locality/contextuality) [33,34], and (c) any such co-construction, being context-dependent, may vary even when the same student interacts within the same environment at different points in time (time-induced variability) [30,35].
Therefore, considering learning as situated and modally defined, this research aimed to explore expressive regularities in terms of multimodality associated with learning. More specifically, it sought to expand research on multimodality in early childhood science education by examining the concept within the context of mechanical equilibrium. We referred to equilibrium in a balance beam, balance scale, or seesaw; that is, situations where someone needs to move along a beam or weights must be placed on a scale or seesaw to achieve balance. In studies focused on equilibrium as a learning subject, those leading to a kind of standardization of the learners’ reasoning evolution dominate. These range from intuitive choices to causal explanations and cover the entire lifespan/developmental range, such as the study by Inhelder and Piaget [36] for ages 3 to 14 years, Siegler [37] for ages 5 to 17 years, Hardiman et al. [38] for ages 17 to 36 years, or Roth [39] (average age 25.8 years).
However, despite having a long tradition for at least the last sixty years in the teaching and learning of natural sciences, mechanical equilibrium has not been studied based on multimodal thinking in early childhood education. Researchers investigating preschoolers’ conceptions about mechanical equilibrium have mostly relied on manipulating three-dimensional balances (i.e., pan balance and balance scales) [36,37,40], virtual manipulatives of them [29], or engaging in kinesthetic activities such as holding weights or balancing on a beam [11]. Specifically, as mentioned earlier, Hadzigeorgiou et al. [11] demonstrated that preschoolers engaged in kinesthetic activities, such as balancing on a beam, retained balance rules better than those using mechanical balances. Furthermore, for kindergarten children, Zacharia et al. [29] revealed that manipulating a mechanical balance during equilibrium tasks, particularly for children initially struggling with mechanical equilibrium, eventually led to greater improvements compared to children who prepared by engaging in simulated (virtual) equilibrium tasks. Both of the last two studies, focusing on preschool-aged children, explored learning about mechanical equilibrium, emphasizing the children’s bodies in a kinesthetic and physicality approach, respectively. However, none of these research endeavors was specifically designed to comprehensively delve into children’s cognition regarding mechanical equilibrium. In other words, there has not been an exploration of how the modalities of oral discourse, space, and body intersect harmoniously, constructing meanings pertaining to the conceptual dimensions of mechanical equilibrium. Consequently, this provides the opportunity to systematically investigate the significance of various modes of expression and thinking arising from semiotically distinct contexts.

2. Materials and Methods

2.1. Research Design

The research design focused on a case study involving a five-year-old preschool child from a public kindergarten in an urban setting. The researchers obtained parental consent as well as assent from the participant before implementing the project. The goal was to develop a plan that allowed for the emergence of as many action-based thoughts as possible from the child regarding the conceptual dimensions of mechanical equilibrium, specifically beam equilibrium with equal and unequal weights. Therefore, we sought a process that would enable the child to express different modalities of thinking.
The epistemological assumption is that knowledge is complex, multileveled, and intertwined with the environmental elements in various ways. Therefore, any context, including the assessment tests, uniquely impacts the learning process and does not objectively capture the entirety of reasoning. Instead, it records responses/expressions to specific stimuli presented to the student at a particular moment. Another important understanding is that learning is a continuous and non-linear process without clear beginnings or ends. It is not possible to claim that an initial test captures initial ideas or that subsequent tests measure what students have learned after experiencing certain effects. With these considerations in mind, we designed the methodology to create effects with clear semiotic content that activate all system-producing modalities. Furthermore, our methodology aimed to record the student’s expressions in space and time and attribute elements of thought to them.
As previously mentioned, research on multimodal thinking has shown that preschool-aged children, depending on the type of influence they receive from the question framework, may express different meanings through oral speech and different meanings through different semiotic systems [1,9,10,17]. Furthermore, even within the same framework, some dimensions may be expressed through oral speech while others may be expressed through drawing or the human body [3]. Therefore, a research design that would elicit multiple expressions/thoughts from the learner could be an influence/task consisting of different contexts. With this in mind, we designed a test consisting of three tasks, specifically differentiated, which were designed to activate different modalities from the child. The test comprised an oral speech task, a drawing expression task, and an object manipulation task. Task one primarily prompted oral speech but also gestures, task two led to the production of a drawing and oral speech and gestures during the explanation of the drawing, while task three primarily prompted gestures and oral speech. Additionally, we introduced the rationale for using pre/posttests and teaching interventions in the research design, as this increased the possibility that the three tasks would elicit even more and different modalities over time, as teaching interventions mediate with the aim of changing the child’s ideas. This was further reinforced by the use of two teaching interventions with differentiated semiotic frameworks. That is, teaching intervention one, which we developed around a seesaw simulation where the child interacted with the virtual environment formulating and testing hypotheses about balance. Moreover, teaching intervention two was employed, in which a physical 3 m seesaw played a central role, allowing the child to control their thinking by walking on it. The two different semiotic interventions, by activating different action thoughts in the child, aimed to increase the possibility of activating different modalities in the posttests (tests two and three) compared to test one, but also between them.
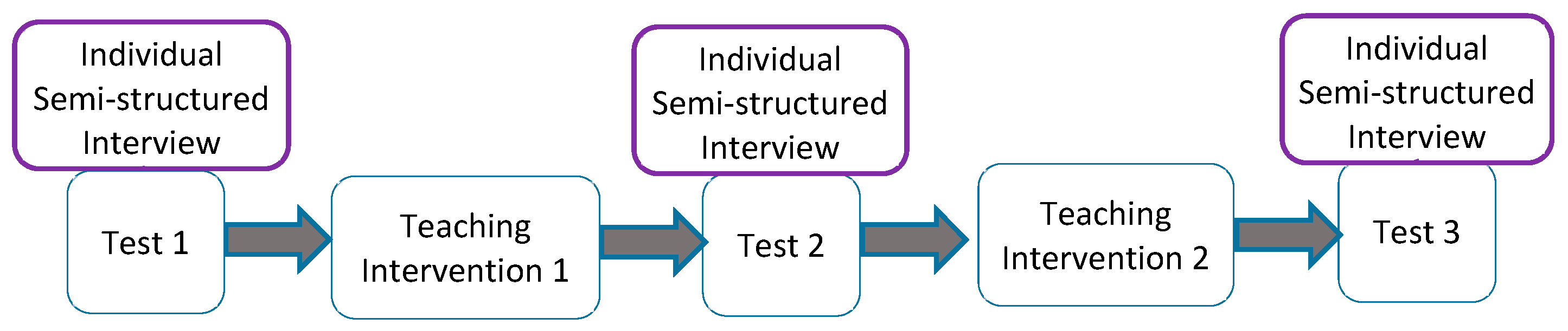
Overall, we employed two types of effects in the study. A repeated semi-structured individual interview/test consisting of three tasks, along with two different teaching interventions. We administered the test at three different time points (tests one, two, and three), while the teaching interventions were inserted between them, each with a distinct context (Figure 1).
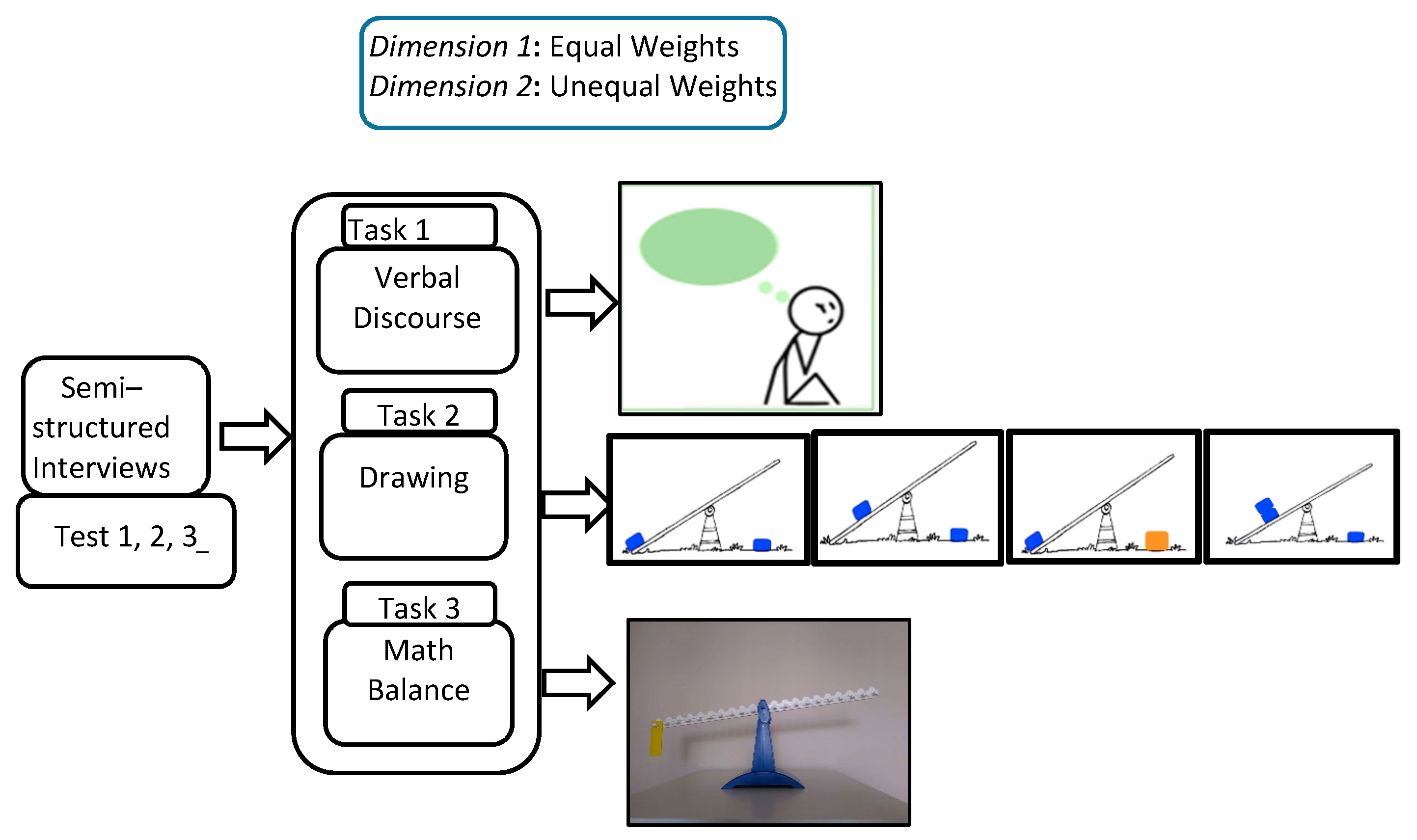
The semi-structured interview, lasting almost 20 min, included three equivalent tasks related to the same conceptual dimensions of mechanical equilibrium (Figure 2).
An example of questions posed in the first task regarding the balance of equal weights is as follows:
“Imagine you are on the seesaw and sitting on one end. How will the seesaw be? Will it be level? Show me.
And how could it be level if a friend of yours with the same weight sat on the other end?
Where should your friend sit on the other side?
Can you explain why?
And if you sat a little further in, what would happen to the seesaw?
Where should the other person sit?”
Similarly, another example of questions in the third task with the mathematical balance scale (Figure 3) for the equilibrium of unequal weights is as follows:
“If I hang a weight a little further in on one side, could I hang two of those weights somewhere on the other side to balance it out?
Can you show me where I could hang them?
Further in or further out?
Why?”
Despite the semiotic differences, all three tasks included equivalent questions about balancing equal and unequal weights. It is important to note that the purpose of the test was not to have the child repeat their earlier ideas precisely. The goal was to have an unambiguous effect on the student, with each task emphasizing one semiotic system at a time.
The second type of effect consisted of two teaching interventions that also differed semiotically. The first author conducted each intervention, which lasted approximately 45 min in a kindergarten classroom as part of the regular program involving all the children in the class. The interventions were based on a five-step design: (a) engaging children’s interest by recalling their experiences from playing on a seesaw, (b) eliciting their ideas through inquiry about predictions and explanations regarding where to place weights for balance, (c) encouraging children to experiment to verify or refute their predictions, with suggestions from the teacher to measure distances when children did not incorporate measurement into their reasoning, (d) facilitating the formulation of conclusions in the whole group, and (e) conducting an evaluation through questions to the children about achieving balance at various points, either on the physical or the virtual seesaw. The first intervention utilized a mechanical equilibrium simulation available at https://phet.colorado.edu/sims/html/balancing-act/latest/balancing-act_en.html (accessed on 3 March 2020). The second intervention, which occurred between the second and third tests, involved a floor-standing original three-meter wooden seesaw constructed specifically for the research. The semiotic context of these two teaching interventions was intentionally different to create unique effects. The simulation involved intangible human–entity interactions, while the seesaw involved material object–human interactions. However, both interventions revolved around the same referent, a seesaw, with the student interacting with it in different ways and experiencing different possibilities of bodily activation.
Furthermore, the teaching interventions and subsequent tests were spaced ten days apart, which was deliberately chosen to ascertain the potential sustainability of teaching interventions’ effect [41]. Hence, the researchers selected a rather intense research design to render the impact of multimodal frameworks sufficiently robust, implementing two teaching interventions and one test consisting of three tasks. This allowed for a total of five distinctively differentiated effects to be observed. Additionally, both the physical seesaw, measuring 3 m in length, and the simulation of the seesaw created a dominant framework of impact and sustainability, owing to their morphological characteristics and the children’s lack of prior experience with such artifacts.
It is worth mentioning that the case study method is recognized as a reliable approach in education and early science education, particularly when thoroughly and parametrically analyzing the case used and when the findings exhibit variability among the categories and diversity within a category [42,43,44,45,46]. Indeed, the researchers questioned the child under different semiotic contexts a total of nine times, each time focusing on the same conceptual dimension of mechanical equilibrium. Additionally, they applied a coding and analysis framework to all the modalities of the oral discourse, spatial entities, and gestures expressed by the child that conveyed the concept of mechanical equilibrium in some way. These two factors resulted in the documentation of at least seven different modalities. Furthermore, the findings support the selection of a single child as a case study since they revealed five different complements among the various modalities, referring to two broader structures of complementarity.

2.2. Data Collection

For each test, the three tasks were videotaped at the three different time points to gather the data. The position of the material object, which represented the conceptual dimensions of mechanical equilibrium, was presented to the student as a given during each test. In the first task, this information was conveyed verbally. In the second task, a predrawn object was placed on a seesaw, and in the third task, the object was placed on the mathematical balance. We asked the student questions about the possible placement of another material object on the opposite side of the seesaw. It is important to note that the questions used different data for each task. Specifically, the dimension of equal weights (D1) was divided into two sub-dimensions. In D1a, the given object was placed at one end of the seesaw or mathematical balance, and the researcher asked the student where an object of the same weight should be positioned to achieve balance, along with an explanation. In dimension D1b, the given object was positioned slightly further in, and the child was asked the same question. The dimension of unequal weights (D2) was also divided into two sub-dimensions. In D2a, the given object was placed at one end of the seesaw or balance, and we asked the child where a heavier object should be placed to restore equilibrium. In D2b, the given object was moved a little further in, and we asked the child the same question. Additionally, dimension D2 included questions where the given object placed at various positions had twice the weight, and the researcher asked the student to express and explain where a lighter object should be placed to restore equilibrium.
The data collection process varied depending on the task. In the first task, the student answered oral questions, resulting in data consisting of speech and occasional bodily expressions if the child gestured while speaking. In the second task, the child drew weights and placed them on the drawn seesaw at points where it would be balanced. The collected data included the student’s drawings, speech, and any gestures used to explain the drawings. In the third task, the child answered questions about balancing equal and unequal weights on the mathematical balance. The data collected for this task consisted of speech and bodily expressions, as the child used gestures to indicate the point at which each weight should hang. Although the tasks differed in form, equivalent questions about the equal and unequal weights were asked in each task. The researcher repeated the same questions about the equal and unequal weights three times, adapting them to the respective context.

2.3. Coding

The student’s responses were codified based on the “how” and “why” of balancing the seesaw with equal or unequal weights. The two authors employed a video data coding scheme that they had utilized in previous research involving the analysis of multimodal thinking [3,17,47]. For encoding the 20-min video, they used a key (Table 1) to specify certain significant concepts for conceptualizing mechanical balance at the preschool age. Specifically, these concepts are the “same weight” and “same position on the other side” for one dimension, and “heavy object + inner position” and “light object + outer position” for the other dimension of mechanical equilibrium. The encoding process involved assigning codes to words or phrases, elements of the drawing, and gestures that have an explicit semantic relationship with the aforementioned key concepts. Each modality was assigned a code name: speech (si), deictic gestures (dgi), iconic gestures (igi), ergotic gestures (egi), and drawing (di). The deictic gestures involved pointing in a specific direction, iconic gestures depicting human activities or animate/inanimate entities morphologically, and ergotic gestures, which were associated with interacting with material objects. Initially, we conducted a viewing of the video, and codes were assigned to each gesture based on the morphological characteristics, i.e., all deictic, iconic, and ergotic gestures were observed and documented without capturing their semantic content. The same process was applied to the elements of the drawing. Thus, the di was registered as the morphological element, e.g., a drawn box at the edge of the seesaw, etc. Finally, after the phonetic transcription, the words and phrases were coded, but according to the semantic criteria, i.e., elements of speech (si) which signify the key concepts of Table 1. That is, words such as “me”, “the other”, and “the small one’” which signify the “same weight” or “light object”, etc., or words like “there” and “same position”, which in some way signify the “same position on the other side”, “inner position”, etc. Finally, after assigning meaning to the elements of speech, meanings were attributed based on both the linguistic context and the elements of the drawing and gestures. For example, a deictic gesture pointing to a specific point on the balance scale clarifying the linguistic “there”, identified, for example, the “outer position”. In this way, all the grammatical elements si, di, dgi, igi, and egi, of the different semiotic systems, as well as their synergies, were semantically evaluated.
The codified data, including the student’s modalities, their relationship to scientific compatibility, and the referenced conceptual dimensions of mechanical equilibrium, were entered into the timeline of NVivo 12 qualitative research data analysis software (NVivo 12 Pro). The coding process was initially conducted by the two authors. The two coders independently encoded the data, and subsequently compared their coding one by one. In cases where an agreement was not reached, the instances were excluded from the analysis, although these cases accounted for less than 1% of the data. The validity of the coding process was ensured by the use of the specific key concepts outlined in Table 1, which are fundamental for conceptualizing mechanical equilibrium in terms of preschool knowledge [11,36]. Furthermore, the trustworthiness of the coding was grounded in the high agreement between the two coders, who underwent the same process independently.

2.4. Data Analysis

In the data analysis process, the authors assessed each modality produced by the child in the context of scientific compatibility. Table 1 provides a key that assigns meaning to each modality and records the semiotic system from which they derive.
Table 1 presents the conceptual dimensions of mechanical equilibrium (D1 and D2) that the student is referring to. The key concepts column indicates the essential concepts necessary for the student’s reasoning to be complete for each conceptual dimension. For D2, the “+” symbol distinguishes the two complementary concepts. The signifiers (modes) column contains the codification of the empirical data, including speech, gestures, and drawings. This allows the recording of distinct pieces of meaning conveyed by each modality and how the modalities from different semiotic systems collaborate. This data analysis method enables the identification and exploration of modalities derived from different semiotic systems that, together, contribute to reasoning (first axis of analysis).
Each modality was semantically evaluated in terms of its scientific compatibility. As a result, the timeline created using NVivo 12 facilitated the recording of sequences of correct/incorrect thought acts as responses to the “same” question (second axis of analysis).

3. Results

Τhe researcher posed questions to the student that assumed one aspect of the equilibrium condition and asked the student to determine the other aspect. For instance, in task three, when balancing equal weights, one of the questions already had a weight hanging from a position on the balance, and the student had to identify where another weight should be placed to achieve equilibrium. Similarly, in task one, for balancing unequal weights, the researcher asked the student to imagine someone sitting in the middle of one side of a seesaw on the playground and explore where someone else lighter than him/her should sit to balance the seesaw. It is important to mention that, specifically for unequal weights, each task provided either the heavy or the light object as a given, ensuring that the student’s responses would include the corresponding reasoning, depending on the scenario each time. The following results encompass all aspects expressed by the student, including where they were expressed, along with the given part of the reasoning presented in the question. The given parts of the reasoning, which were explicitly stated in the question itself, are highlighted in italics when the student utilized them.
Complementation appeared between the speech and gestures that arose for the two conceptual dimensions D1 and D2 of mechanical equilibrium (Table 2). The complementation concerned two types of synergies, i.e., between speech and an iconic gesture and between speech and deictic gestures. For the conceptual dimension of equal weights (D1), complementation was signified through the structures s1 + ig1, s1 + dg1 and s1 + dg2. In all three structures (“sentences”) the student chose speech to conceptualize the “same weight”, while communicating the “same position” with gestures. As regards speech, the child used the same words in all three “sentences”, i.e., the statement “same kilos”, while for the same position the student used different gestures: wide open arms (ig1), pointing to the right or left end of the balance (dg1), and pointing a little further in from the right or left end of the balance (dg2). It should be noted that the two deictic gestures were both correct answers although they referred to different points on the balance because the student was asked about the dimension of equal weights more than once for different positions.
Specifically, the complementation structure s1 + ig1 appeared as follows in the empirical data:
“Because if we weighed the same kilos (s1), the other could have sat on the other side (ig1: opens his/her arms wide, hands facing each other) and it would stay level.”
Gesture ig1 apparently conceptualizes the appearance of the position through visual symmetry. Each hand illustrates where (the position) each object must be placed to achieve equilibrium. This is something speech does not clearly convey, since the phrase “on the other side” creates an incomplete meaning, because it refers to a broader area rather than a specific position. Additionally, one might say that ig1 depicts the objects themselves, since each hand represents an object of equal size (and equal weight).
The remaining complementation structures related to the general structure si + dgi. In other words, the student used speech to express the concept “same kilos” and deictic gestures for the concept “same position on the other side”. For example, in task three, with the mathematical balance, the student created the multimodal “sentence”:
“Because where you put the one that weighs the same kilos (s1), it should go there (dg2: pointing a little further in from the left end of the balance).”
In this case, a weight was placed on the mathematical balance and the child was asked where they should place another equal weight to achieve equilibrium. It is obvious that modalities s1 and dg2 are the semantically strong ones, as the location adverb “there” is general and abstract compared to the deictic gesture (dg2), which precisely signifies the point where the second object must be placed for equilibrium to be achieved.
Similarly, for the conceptual dimension of unequal weights (D2), the following is an example of a “sentence” consisting of the synergy of distinct meanings that complement one another:
“Because the small ones (s4) always usually go here (dg1: pointing to the right end of the balance) and the large ones (s2) (dg4: pointing to the two bricks in the center of the one side of the balance) are always placed further in”.
In this case, the student produced this particular answer while two weights were hanging on one end of the balance and was asked where the single weight should be placed to achieve equilibrium and to defend its answer. The student signified the concepts “light object + outer position” with the synergy of the pronouncement “the small ones” (s4) and the “deictic gesture towards the right end of the balance” (dg1). As before, the deictic gesture communicates the exact position where the object must be placed to achieve equilibrium, as the pronouncement “here” is inaccurate and its meaning weak.
Table 3 presents the multimodal “sentences” put together by the student through speech, drawing, and gestures. Student’s multimodal activity is described by the following structures: x + y, x + y(z). The complementation structure x + y has been discussed previously and appears between speech and a deictic or iconic gesture. In a complementation structure, the elements x and y carry different information and need each other, complementing one another to create a semantic whole. In y(z) the y element is semantically stronger than z and does not need z to signify it, while viewed on its own, z creates ambiguities, and the meaning is incomplete. For example, with dg1(s8) the student points to the end of the balance and utters the adverb “out” (s8). While the gesture describes the position with absolute precision, the adverb “out” expresses a wider area.

4. Discussion

In a sense, the child’s expressions in the material environment constitute narratives of the conceptual dimensions of mechanical equilibrium [48]. The student displayed in its syntax complementation structures of the form si + igi and si + dgi, creating semantic sets. For the dimension of equal weights, both complementation structures were employed, while si + dgi was employed for the dimension of unequal weights. In other words, there appeared modalities of speech and of deictic and iconic gestures, which carry distinct information; as a result, when combined, they occasionally form semantic sets. The two types of gestures, deictic and iconic, exclusively communicate the concept of position/distance, while speech exclusively communicates the concept of weight, and they express themselves in the context of formulating a rule. When balancing equal weights, the rule refers to the relationship of equal weights and symmetrical position, while for balancing unequal weights, the rule refers to balancing greater weight and shorter distance with lighter weight and greater distance. According to Inhelder and Piaget [11], the formulation of these rules constitutes a stage before the formulation of the rule of quantitative proportionality in the process of conceptualizing mechanical equilibrium. More specifically, they categorize the following stages in the development of learners’ reasoning: (a) the recognition that weight is required on both sides of the beam, but with no acknowledgment of a weight-distance relationship, (b) the achievement of equilibrium with equal weights based on the symmetry of weight and distance, or achieving equilibrium through trial and error with greater weight and shorter distance and lighter weight and greater distance, without formulating a rule, (c) the formulation of a qualitative rule for equilibrium with greater weight and shorter distance compared to lighter weight and greater distance, (d) the formulation of a quantitative rule of proportionality, and (e) seeking causal explanations.
The iconic gesture appeared in the speech task, where the child did not use material objects as an expressive need; it thus appears to replace the missing material parameters of the environment. It is conceivable that this absence of material objects caused the child to produce a bodily depiction. This is associated with the views of Ping and Goldin-Meadow [10] and Roth [49] who reported that when material objects are absent, iconic gestures develop to replace them. This particular gesture (ig1) might be associated with the simulation the student engaged in during the first teaching intervention. There, during the teaching process, the student used their hands to count, interacting with the simulation projected onto the wall. Thus, although this gesture might have been activated by the current, “materialess” environment of task one, it might have been constructed in an early, different form at a previous time when the simulation was used to teach. The absence of material objects in task one—when the student was asked to imagine what would have to happen for a seesaw in the playground to balance—led to the “objects and their placement on the seesaw” being loaded onto the gesture. According to Ping et al. [50] and Trofatter et al. [51], the iconic gestures’ representational dynamic and a problem that must be solved (as in task one) might cause grounded thought in action, with the gestures remaining unaffected by the context of speech. The same researchers believed that iconic gestures might have a greater effect on learning when solving a problem than action on objects itself, even when an action is accompanied by speech. In the context of embodied cognition, actions are grounded in thought. Thus, gestures not only have a one-way relationship with thoughts, constituting outcomes of the latter, but actively fuel the cognitive processes [52]. Gestures are not merely carriers for expressing action information, but they add action information to the mental representations of the person who produced the gesture [53].
It seems that the morphology of each task led to the production of different complementation structures to speech. Task one resulted in the emergence of iconic gesture, while task three led to the use of deictic gestures. This point is linked to the perception of situated and embodied cognition, i.e., with the unique understandings of an agent through specific bodily interactions with a specific context [54]. In task three, naturally, there is no alternative to using deictic gestures when one is placed before a mathematical balance. In task one, the iconic gesture, as mentioned, was a morphological response to the lack of materials of the specific semiotic context [10]. The effect of the semiotic context on the activation of specific semiotic systems and modalities permits the creation of learning environments that educate students in multimodal thinking and “multilingualism”, through their initiation in every mode of expression.
Many researchers in science education emphasize the value of the multimodal production of meaning in the education process, something confirmed by the current study in the context of early childhood science education [55,56,57]. It was demonstrated that the student’s thinking was multimodal, assembling, as the situation required, a different syntax, using various modalities and semiotic systems. The student produced complementation [x + y] while expressing modalities semantically stronger than others [y(z)]. In the current research, to balance equal weights, the student used deictic gestures exclusively, along with one iconic gesture, to conceptualize the correct position, perhaps because gestures are more precise than the ambivalent local adverbs “here” or “there”. In many cases in preschool-aged children, it is the very nature of the entities that render them amenable to more precise conceptualizations via semiotic systems other than speech. Spatial entities, intangible entities (e.g., light), and entities from the microcosm and the megacosm (e.g., earth’s rotation), are apparently more clearly conceptualized through bodily expression or drawings than speech [3,58,59]. Frequently, in the case of preschool children—and not only them—the human body appears to be the exclusive signification provider in science activities [11,13,60].
The methodology developed in this particular study can capture the ideas of the learners, regardless of how they are expressed, thereby providing additional insights into research on students’ precursor models at the preschool age. The precursor model holds a significant role in examining how mental representations align with scientific models in educational settings. Introduced in the 1990s, the idea of the precursor model integrates elements from different theoretical perspectives, including science education, Piagetian genetic epistemology, and Vygotskian socio-cultural approaches [61,62]. Its purpose is to bridge the gap between children’s naive mental representations and science models, facilitating the development of intermediate mental schemes within an educational framework. These mental entities correspond with children’s developmental capabilities while remaining consistent with scientific models [63]. Recent studies in early childhood science education have shown the construction of precursor models across various subjects [64,65]. Therefore, the multifaceted exploration of children’s mental representations can reveal items of meaning related to precursor models that may not be detected by adopting a research discipline based solely on orality.

5. Conclusions

We have shown that during the process of understanding the concept of mechanical equilibrium, the student utilizes a multimodal syntax, combining speech and gestures in a complementary manner. The results indicated that the child employs complementation structures of the form “speech + iconic gesture” and “speech + deictic gesture”, signifying distinct dimensions of mechanical equilibrium. Specifically, for the concept of equal weights, both complementation structures were utilized, while for unequal weights, only the “speech + deictic gesture” structure was employed. The findings suggest a developmental progression in reasoning from the simple recognition of weight placement to the qualitative understanding of weight–distance relationships.
Furthermore, the presence or absence of material objects influenced the types of gestures produced. When material objects were absent, iconic gestures emerged to replace them, indicating a form of embodied cognition where gestures supplement missing environmental parameters. Additionally, the morphology of tasks influenced the production of different complementation structures, with iconic gestures appearing in tasks with absent material objects and deictic gestures in tasks with material presence.
The presence of complementarity and multimodality indicates their significance in the learning process, aligning with the concept of variability in thinking that has been recognized in cognitive sciences for several decades [50,66,67]. This brings the notion of variability into the realm of early childhood science education, emphasizing the need for the further exploration of semantically complementary modalities and their collaborative role in creating diverse syntactic structures.

6. Limitations and Extensions

This research constitutes a case study and does not aim to draw generalizations from a single student. Further research is needed with a larger sample size, encompassing children from schools with diverse socioeconomic characteristics.
The study focused on children’s expressions within a controlled educational setting, centered around three different tasks and two distinct teaching interventions. The relationship between oral speech and gestures in students’ conceptual development could also be explored in contexts involving technological artifacts such as robots or objects that children can use to construct something, such as educational engineering projects. In this regard, the methodology relying on the analysis of speech and gestures may not capture all aspects of children’s cognitive processes and requires adaptation to different contexts.
Analyzing children’s expressions across various modalities opens avenues for exploring their understanding in educational settings conducive to constructing precursor models, which are intermediate mental schemes consistent with scientific models.
Extensions of this work could involve longitudinal studies to monitor children’s conceptual development over time through the modalities they use, and cross-cultural comparisons to examine variations in children’s conceptualization across different cultural contexts. Additionally, integrating advanced technologies, such as eye-tracking or neuroimaging, could provide new avenues for investigating the underlying cognitive mechanisms involved in children’s scientific multimodal reasoning.

Author Contributions

Conceptualization, M.-E.C. and P.P.; methodology, M.-E.C. and P.P.; software, M.-E.C. and P.P.; validation, M.-E.C. and P.P.; formal analysis, M.-E.C. and P.P.; investigation, M.-E.C. and P.P.; resources, M.-E.C. and P.P.; data curation, M.-E.C. and P.P.; writing—original draft preparation, M.-E.C. and P.P.; writing—review and editing, M.-E.C. and P.P.; visualization, M.-E.C. and P.P.; supervision, M.-E.C. and P.P.; project administration, M.-E.C. and P.P. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

The study was conducted in accordance with the Declaration of Helsinki and approved by the Ethics Committee of the School of Early Childhood Education, Aristotle University of Thessaloniki.

Informed Consent Statement

Informed consent was obtained from all subjects involved in the study.

Data Availability Statement

The data presented in this study are available on request from the corresponding author. The data are not publicly available due to privacy and ethical restrictions.

Acknowledgments

The authors would like to thank all of the participants who participated in this research.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Tytler, R.; Prain, V.; Peterson, S. Representational issues in students learning about evaporation. Res. Sci. Educ. 2007, 37, 313–331. [Google Scholar] [CrossRef]
  2. Hostetter, A.; Alibali, M. Visible embodiment: Gestures as simulated action. Psychon. Bull. Rev. 2008, 15, 495–514. [Google Scholar] [CrossRef] [PubMed]
  3. Chachlioutaki, M.-E.; Pantidos, P.; Kampeza, M. Changing semiotic modes indicates the introduction of new elements in children’s reasoning: The case of earthquakes. Educ. J. Univ. Patras UNESCO Chair 2016, 3, 198–208. [Google Scholar] [CrossRef]
  4. Leung, C.B. Peirce’s concept of signs and kindergarten literacy. Int. J. Learn. Teach. Educ. Res. 2018, 17, 104–121. [Google Scholar] [CrossRef]
  5. Waldrip, B.; Prain, V.; Carolan, J. Using multi-modal representations to improve learning in junior secondary science. Res. Sci. Educ. 2010, 40, 65–80. [Google Scholar] [CrossRef]
  6. Hwang, S.; Roth, W.M. The (embodied) performance of physics concepts in lectures. Res. Sci. Educ. 2011, 41, 461–477. [Google Scholar] [CrossRef]
  7. Zohar, R.; Bagno, E.; Eylon, B.-S.; Abrahamson, D. Motor skills, creativity, and cognition in learning physics concepts. Funct. Neurol. Rehabil. Ergon. 2017, 7, 67–76. [Google Scholar]
  8. Givry, D.; Roth, W.M. Toward a new conception of conceptions: Interplay of talk, gestures, and structures in the setting. J. Res. Sci. Teach. 2006, 43, 1086–1109. [Google Scholar] [CrossRef]
  9. Vosniadou, S.; Skopeliti, I.; Ikospentaki, K. Modes of knowing and ways of reasoning in elementary astronomy. Cogn. Dev. 2004, 19, 203–222. [Google Scholar] [CrossRef]
  10. Ping, R.M.; Goldin-Meadow, S. Hands in the air: Using ungrounded iconic gestures to teach children conservation of quantity. Dev. Psychol. 2008, 44, 1277. [Google Scholar] [CrossRef]
  11. Hadzigeorgiou, Y.; Anastasiou, L.; Konsolas, M.; Prevezanou, B. A study of the effect of preschool children’s participation in sensorimotor activities on their understanding of the mechanical equilibrium of a balance beam. Res. Sci. Educ. 2009, 39, 39–55. [Google Scholar] [CrossRef]
  12. Goldin-Meadow, S. Taking a hands-on approach to learning. Policy Insights Behav. Brain Sci. 2018, 5, 163–170. [Google Scholar] [CrossRef]
  13. Novack, M.; Goldin-Meadow, S. Learning from gesture: How our hands change our minds. Educ. Psychol. Rev. 2015, 27, 405–412. [Google Scholar] [CrossRef] [PubMed]
  14. Kontra, C.; Goldin-Meadow, S.; Beilock, S.L. Embodied learning across the life span. Top. Cogn. Sci. 2012, 4, 731–739. [Google Scholar] [CrossRef] [PubMed]
  15. Hadzigeorgiou, Y. The utilization of sensori-motor experiences for introducing young pupils to molecular motion: A report of a pilot study. Phys. Educ. 2002, 37, 239. Available online: https://iopscience.iop.org/article/10.1088/0031-9120/37/3/308 (accessed on 5 May 2023). [CrossRef]
  16. Roth, W.-M.; Lawless, D. Signs, deixis, and the emergence of scientific explanation. Semiotica 2002, 138, 95–130. [Google Scholar] [CrossRef]
  17. Herakleioti, E.; Pantidos, P. The contribution of the human body in young children’s explanations about shadow formation. Res. Sci. Educ. 2016, 46, 21–42. [Google Scholar] [CrossRef]
  18. Einarsdottir, J.; Dockett, S.; Perry, B. Making meaning: Children’s perspectives expressed through drawings. Early Child Dev. Care 2009, 179, 217–232. [Google Scholar] [CrossRef]
  19. Chang, N. What are the roles that children’s drawings play in inquiry of science concepts? Early Child Dev. Care 2012, 182, 621–637. [Google Scholar] [CrossRef]
  20. Ravanis, K. Early Childhood Science Education: State of the art and perspectives. J. Balt. Sci. Educ. 2017, 16, 284–288. [Google Scholar] [CrossRef]
  21. Crahay, M.; Delhaxhe, A. La Compréhension du Fonctionnement de la Balance. Une Analyse Hiérarchique. Eur. J. Psychol. Educ. 1989, 4, 349–375. [Google Scholar] [CrossRef]
  22. Ravanis, K. The discovery of elementary magnetic properties in preschool age: Qualitative and quantitative research within a piagetian framework. Eur. Early Child. Educ. Res. J. 1994, 2, 79–91. [Google Scholar] [CrossRef]
  23. Weil-Barais, A. Heuristic value of the notion of zone of proximal development in the study of child and adolescent construction of concepts in physics. Eur. J. Psychol. Educ. 1994, 9, 367–383. [Google Scholar] [CrossRef]
  24. Kampeza, M. Preschool children’s ideas about the Earth as a cosmic body and the day/night cycle. J. Sci. Educ. 2006, 5, 119–122. [Google Scholar]
  25. Fragkiadaki, G.; Ravanis, K. Mapping the interactions between young children while approaching the natural phenomenon of clouds creation. Educ. J. Univ. Patras UNESCO Chair 2014, 1, 112–122. [Google Scholar] [CrossRef]
  26. Fleer, M. Science for Children; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
  27. Linebarger, D.L.; Norton-Meier, L. Scientific concepts, multiple modalities, and young children. In Using Multimodal Representations to Support Learning in the Science Classroom; Hand, B., McDermott, M., Prain, V., Eds.; Springer International Publishing: Cham, Switzerland, 2016; pp. 97–116. [Google Scholar]
  28. Ravanis, K. The physical sciences in early childhood education: Theoretical frameworks, strategies and activities. J. Phys. Conf. Ser. 2021, 1796, 012092. [Google Scholar] [CrossRef]
  29. Zacharia, Z.C.; Loizou, E.; Papaevripidou, M. Is physicality an important aspect of learning through science experimentation among kindergarten students? Early Child. Res. Q. 2012, 27, 447–457. [Google Scholar] [CrossRef]
  30. Chachlioutaki, M.E.; Pantidos, P. Semiotic multiplicities and contradictions in science learning. Rev. Sci. Math. ICT Educ. 2023, 17, 75–87. [Google Scholar] [CrossRef]
  31. Shapiro, L.; Stolz, S.A. Embodied cognition and its significance for education. Theory Res. Educ. 2019, 17, 19–39. [Google Scholar] [CrossRef]
  32. Clark, A. Embodied, situated and distributed cognition. In Companion to Cognitive Science, 1st ed.; Bechtel, W., Graham, G., Eds.; Blackwell Publishing Ltd.: Oxford, UK, 2017; pp. 506–517. [Google Scholar]
  33. Hamza, K.M.; Wickman, P.O. Describing and analyzing learning in action: An empirical study of the importance of misconceptions in learning science. Sci. Educ. 2008, 92, 141–164. [Google Scholar] [CrossRef]
  34. Zeidler, D.L.; Herman, B.C.; Sadler, T.D. New directions in socioscientific issues research. Discip. Interdiscip. Sci. Educ. Res. 2019, 1, 1–9. [Google Scholar] [CrossRef]
  35. Frank, B.W.; Kanim, S.E.; Gomez, L.S. Accounting for variability in student responses to motion questions. Phys. Rev. Spec. Top.-Phys. Educ. Res. 2008, 4, 020102. [Google Scholar] [CrossRef]
  36. Inhelder, B.; Piaget, J. The Growth of Logical Thinking from Childhood to Adolescence: An Essay on the Construction of Formal Operational Structures; Psychology Press: London, UK, 1958. [Google Scholar]
  37. Siegler, R.S. Three aspects of cognitive development. Cogn. Psychol. 1976, 8, 481–520. [Google Scholar] [CrossRef]
  38. Hardiman, P.T.; Pollatsek, A.; Well, A.D. Learning to understand the balance beam. Cogn. Instr. 1986, 3, 63–86. [Google Scholar] [CrossRef]
  39. Roth, W.M. The development of reasoning on the balance beam. J. Res. Sci. Teach. 1991, 28, 631–645. [Google Scholar] [CrossRef]
  40. Metz, K.E. Preschoolers’ developing knowledge of the pan balance: From new representation to transformed problem solving. Cogn. Instr. 1991, 11, 31–93. [Google Scholar] [CrossRef]
  41. Michael, D.; Goutas, T.; Tsigilis, N.; Michaelidou, V.; Gregoriadis, A.; Charalambous, V.; Vrasidas, C. Effects of the universal positive behavioral interventions and supports on collective teacher efficacy. Psychol. Sch. 2023, 60, 3188–3205. [Google Scholar] [CrossRef]
  42. Pramling, N.; Samuelsson, I.P. “It is Floating ‘Cause there is a Hole’”: A young child’s experience of natural science. Early Years Int. J. Res. Dev. 2001, 21, 139–149. [Google Scholar] [CrossRef]
  43. Gulsecen, S.; Kubat, A. Teaching ICT to teacher candidates using PBL: A qualitative and quantitative evaluation. Educ. Technol. Soc. 2006, 9, 96–106. [Google Scholar]
  44. Taber, K.S. Exploring conceptual integration in student thinking: Evidence from a case study. Int. J. Sci. Educ. 2008, 30, 1915–1943. [Google Scholar] [CrossRef]
  45. Howitt, C.; Lewis, S.; Upson, E. ‘It’s a Mystery’: A Case Study of Implementing Forensic Science in Preschool as Scientific Inquiry. Australas. J. Early Child. 2011, 36, 45–55. [Google Scholar] [CrossRef]
  46. Fragkiadaki, G.; Ravanis, K. Genetic research methodology meets Early Childhood Science Education Research: A Cultural-Historical study of child’s scientific thinking development. Cult. Hist. Psychol. 2016, 12, 310–330. [Google Scholar] [CrossRef]
  47. Givry, D.; Pantidos, P. Toward a multimodal approach of science teaching. In Proceedings of the Colloque SIEST Mediterranee Tunis 2012 Dispositifs, Démarches, Apprentissage dans l’Enseignement des Sciences et Technologies, Carthage, Tunisia, 26–28 April 2012; Volume 17, pp. 123–130. [Google Scholar]
  48. Hadzigeorgiou, Y. Imaginative Science Education; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar]
  49. Roth, W. From epistemic (ergotic) actions to scientific discourse: The bridging function of gestures. Pragmat. Cogn. 2003, 11, 141–170. [Google Scholar] [CrossRef]
  50. Ping, R.; Church, R.B.; Decatur, M.A.; Larson, S.W.; Zinchenko, E.; Goldin-Meadow, S. Unpacking the gestures of chemistry learners: What the hands tell us about correct and incorrect conceptions of stereochemistry. Discourse Process. 2021, 58, 213–232. [Google Scholar] [CrossRef]
  51. Trofatter, C.; Kontra, C.; Beilock, S.; Goldin-Meadow, S. Gesturing has a larger impact on problem-solving than action, even when action is accompanied by words. Lang. Cogn. Neurosci. 2015, 30, 251–260. [Google Scholar] [CrossRef] [PubMed]
  52. Gentsch, A.; Weber, A.; Synofzik, M.; Vosgerau, G.; Schütz-Bosbach, S. Towards a common framework of grounded action cognition: Relating motor control, perception and cognition. Cognition 2016, 146, 81–89. [Google Scholar] [CrossRef] [PubMed]
  53. Beilock, S.L.; Goldin-Meadow, S. Gesture changes thought by grounding it in action. Psychol. Sci. 2010, 21, 1605–1610. [Google Scholar] [CrossRef] [PubMed]
  54. Dawson, M. Embedded and situated cognition. In The Routledge Handbook of Embodied Cognition, 1st ed.; Shapiro, L., Ed.; Routledge: Oxford, UK, 2014; pp. 77–85. [Google Scholar]
  55. Alibali, M.W.; Nathan, M.J. Teachers’ gestures as a means of scaffolding students’ understanding: Evidence from an early algebra lesson. In Video Research in the Learning Sciences, 1st ed.; Goldman, R., Pea, R., Barron, B., Derry, S., Eds.; Routledge: Oxford, UK, 2007; pp. 349–365. [Google Scholar] [CrossRef]
  56. Gunderson, E.A.; Spaepen, E.; Gibson, D.; Goldin-Meadow, S.; Levine, S.C. Gesture as a window onto children’s number knowledge. Cognition 2015, 144, 14–28. [Google Scholar] [CrossRef]
  57. Novack, M.A.; Congdon, E.L.; Hemani-Lopez, N.; Goldin-Meadow, S. From action to abstraction: Using the hands to learn math. Psychol. Sci. 2014, 25, 903–910. [Google Scholar] [CrossRef]
  58. Jelinek, J.A. Children’s Astronomy. Shape of the earth, location of people on earth and the day/night cycle according to polish children between 5 and 8 years of age. Rev. Sci. Math. ICT Educ. 2020, 14, 69–87. [Google Scholar] [CrossRef]
  59. Singer, M.; Radinsky, J.; Goldman, S.R. The role of gesture in meaning construction. Discourse Process. 2008, 45, 365–386. [Google Scholar] [CrossRef]
  60. Pantidos, P.; Herakleioti, E.; Chachlioutaki, M.E. Reanalysing children’s responses on shadow formation: A comparative approach to bodily expressions and verbal discourse. Int. J. Sci. Educ. 2017, 39, 2508–2527. [Google Scholar] [CrossRef]
  61. Lemeignan, G.; Weil-Barais, A. Construire des Concepts en Physique; Hachette: New York, NY, USA, 1993. [Google Scholar]
  62. Weil-Barais, A. What is a precursor model? In Precursor Models for Teaching and Learning Science during Early Childhood; Boilevin, J.-M., Delserieys, A., Ravanis, K., Eds.; Springer: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
  63. Ravanis, K.; Boilevin, J.-M. What use is a precursor model in early science teaching and learning? Didactic perspectives. In Precursor Models for Teaching and Learning Science during Early Childhood; Boilevin, J.-M., Delserieys, A., Ravanis, K., Eds.; Springer: Berlin/Heidelberg, Germany, 2022; pp. 33–49. [Google Scholar]
  64. Delserieys, A.; Jegou, C.; Boilevin, J.-M.; Ravanis, K. Precursor model and preschool science learning about shadows formation. Res. Sci. Technol. Educ. 2018, 36, 147–164. [Google Scholar] [CrossRef]
  65. Kambouri-Danos, M.; Ravanis, K.; Jameau, A.; Boilevin, J.-M. Precursor models and early years science learning: A case study related to the mater state changes. Early Child. Educ. J. 2019, 47, 475–488. [Google Scholar] [CrossRef]
  66. Church, R.B. Using gesture and speech to capture transitions in learning. Cogn. Dev. 1999, 14, 313–342. [Google Scholar] [CrossRef]
  67. Siegler, R.S. Cognitive variability: A key to understanding cognitive development. Curr. Dir. Psychol. Sci. 1994, 3, 1–5. [Google Scholar] [CrossRef]
Education 14 00338 g001
Figure 1. Research design.
Figure 1. Research design.
Education 14 00338 g001
Education 14 00338 g002
Figure 2. The test consisted of three tasks.
Figure 2. The test consisted of three tasks.
Education 14 00338 g002
Education 14 00338 g003
Figure 3. The mathematical balance scale that was used.
Figure 3. The mathematical balance scale that was used.
Education 14 00338 g003
Table 1. Modalities and pieces of meaning.
Table 1. Modalities and pieces of meaning.
ReferentKey ConceptsSignifiers
Balancing equal weights (D1)Same weight + same position on the other side si, igi, dgi, egi, di
Balancing unequal weights (D2)Heavy object + inner position =
Light object + outer position 		si + igi, dgi,+si, di et.
Table 2. Complementarity 1.
Table 2. Complementarity 1.
DimensionsTest 1Test 2Test 3
Task 1D1s1 + ig1
D2
Task 2D1
D2
Task 3D1s1 + dg1 s1 + dg2
D2 s2 + dg3
s4 + dg1 = s2 + dg4
1 s1 + ig1: “same kilos” + “opens the arms wide, hands facing each other”, s1 + dg1: “same kilos” + “points to the right or left end of the balance”, s1 + dg2: “same kilos” + “points a little further in from the left or right end”, s2 + dg3: “large” + “points to the center of the balance”, s4 + dg1 = s2 + dg4: “small” + “points to the right or left end of the beam” = “large” + “points to the two bricks in the center of the one side of the balance”.
Table 3. Complementation structures.
Table 3. Complementation structures.
Complementation “Sentences”Modal Structures
si + igi (speech + iconic gesture)
si + dgi (speech + deictic gesture)		x + y
s2 + dg3(s3) [speech + deictic gesture (speech)]
s4 + dg1(s8) [speech + deictic gesture (speech)]		x + y(z)
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Chachlioutaki, M.-E.; Pantidos, P. Speech and Gesture Complementarity in a Preschooler’s Conceptualization of Mechanical Equilibrium. Educ. Sci. 2024, 14, 338. https://doi.org/10.3390/educsci14040338

AMA Style

Chachlioutaki M-E, Pantidos P. Speech and Gesture Complementarity in a Preschooler’s Conceptualization of Mechanical Equilibrium. Education Sciences. 2024; 14(4):338. https://doi.org/10.3390/educsci14040338

Chicago/Turabian Style

Chachlioutaki, Maria-Eleni, and Panagiotis Pantidos. 2024. "Speech and Gesture Complementarity in a Preschooler’s Conceptualization of Mechanical Equilibrium" Education Sciences 14, no. 4: 338. https://doi.org/10.3390/educsci14040338

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop