Perception of Symmetry and Spatial Reasoning in 11–12-Year-Old Pupils
Abstract
1. Introduction
1.1. Visual Thinking, Visualisation, and Spatial Reasoning
1.2. Perception of Symmetry
1.3. Placing Our Research in the Broader Context of STEAM Education Research
2. Materials and Methods
2.1. Research Questions
- Is there a strong relationship between spatial and planar symmetry perception in students?
- Is there any connection between symmetry perception and spatial ability globally?
- Is there a connection between mental rotation (and perhaps mental mirroring) and spatial orientation in the thinking of individual students?
2.2. Research Tools
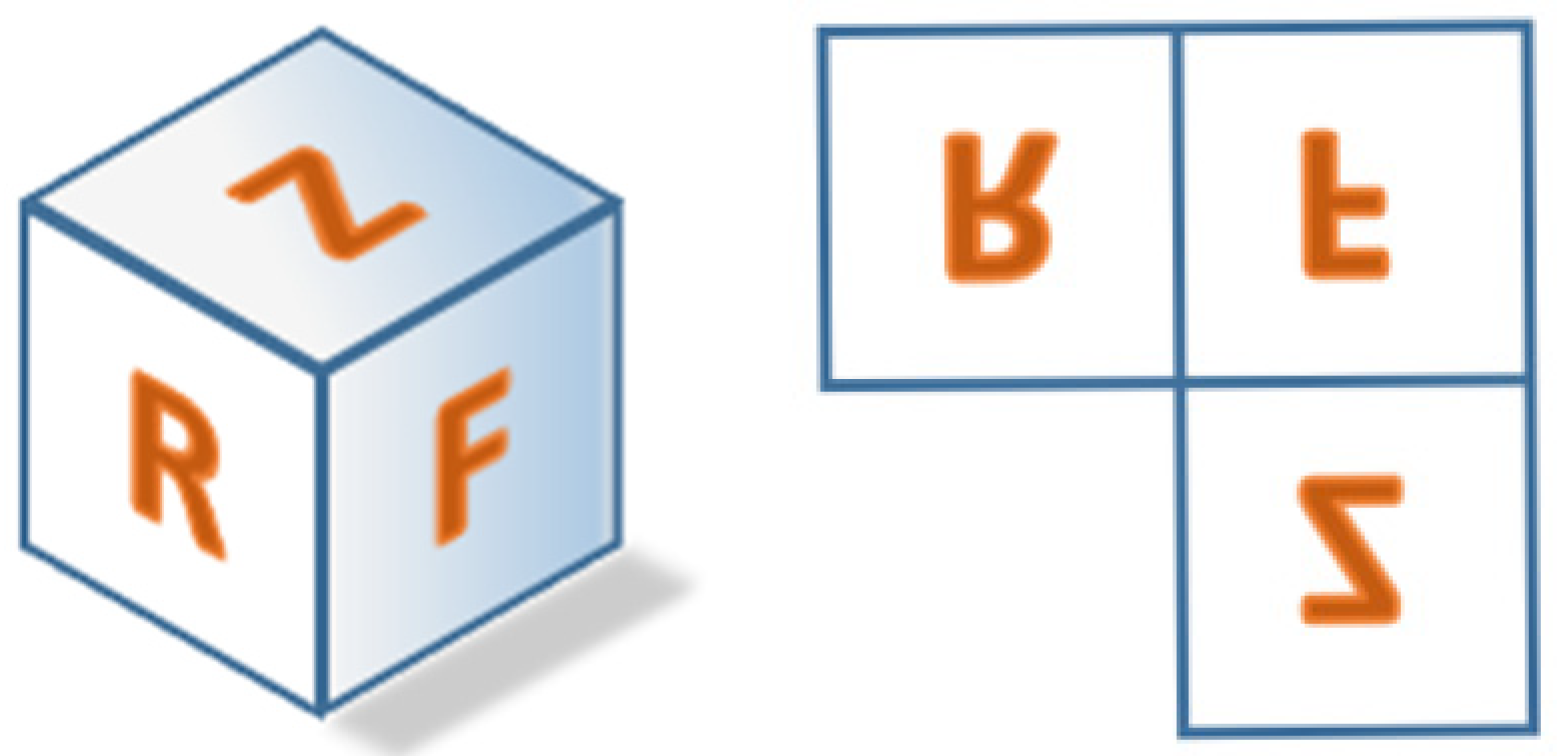
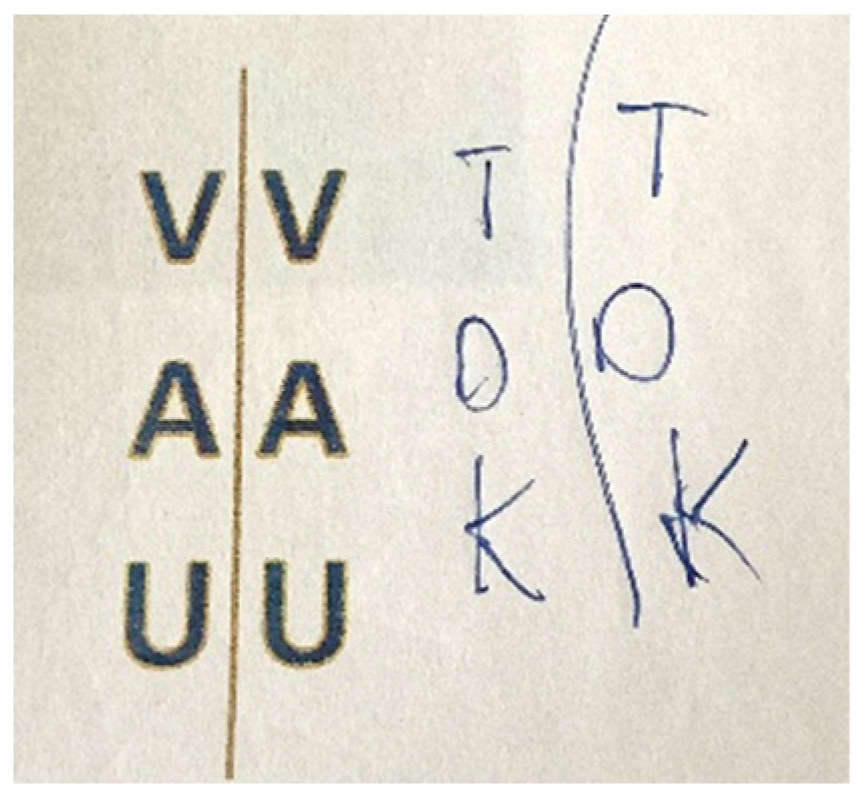
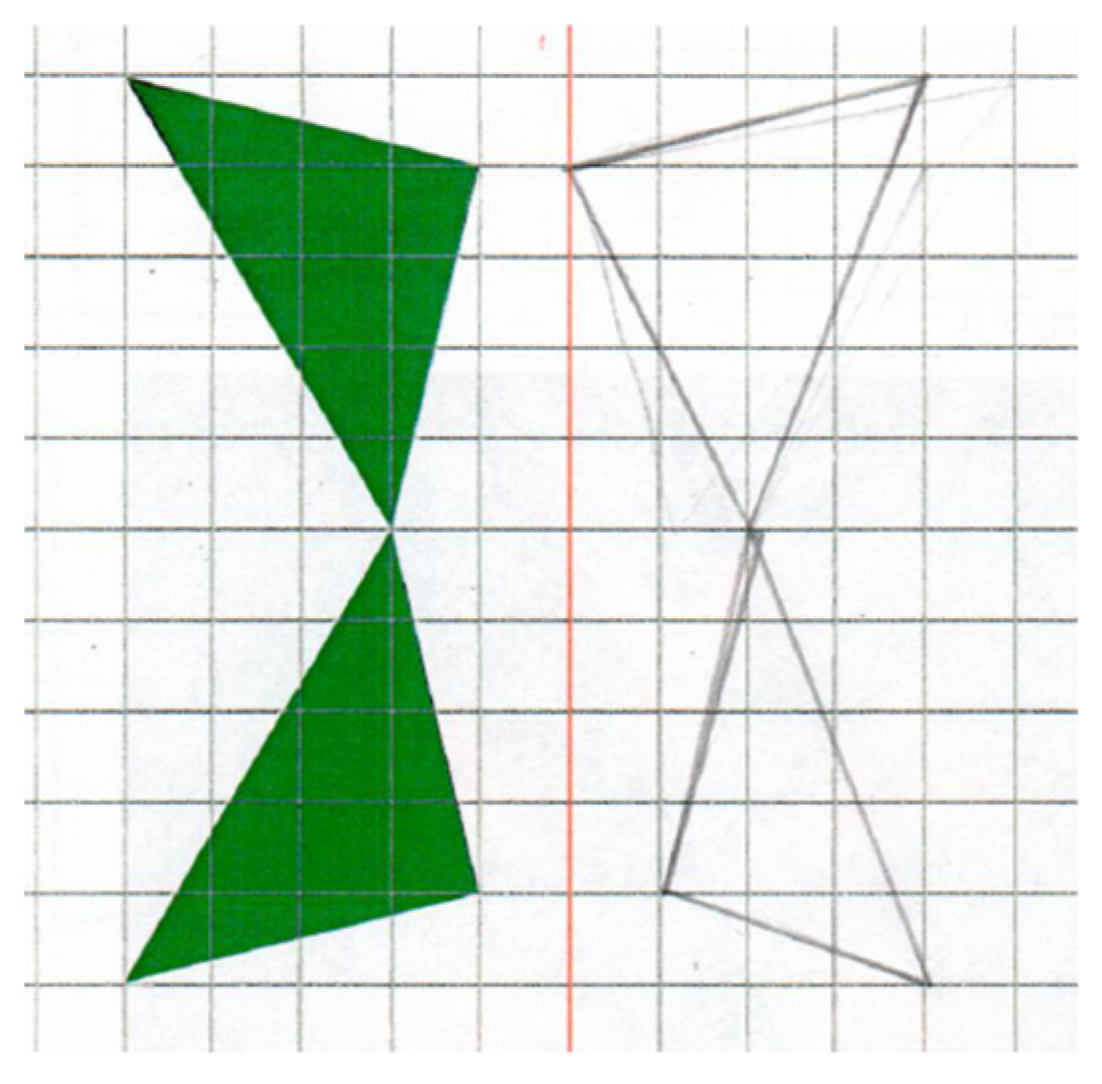
2.2.1. Axial Symmetry Test
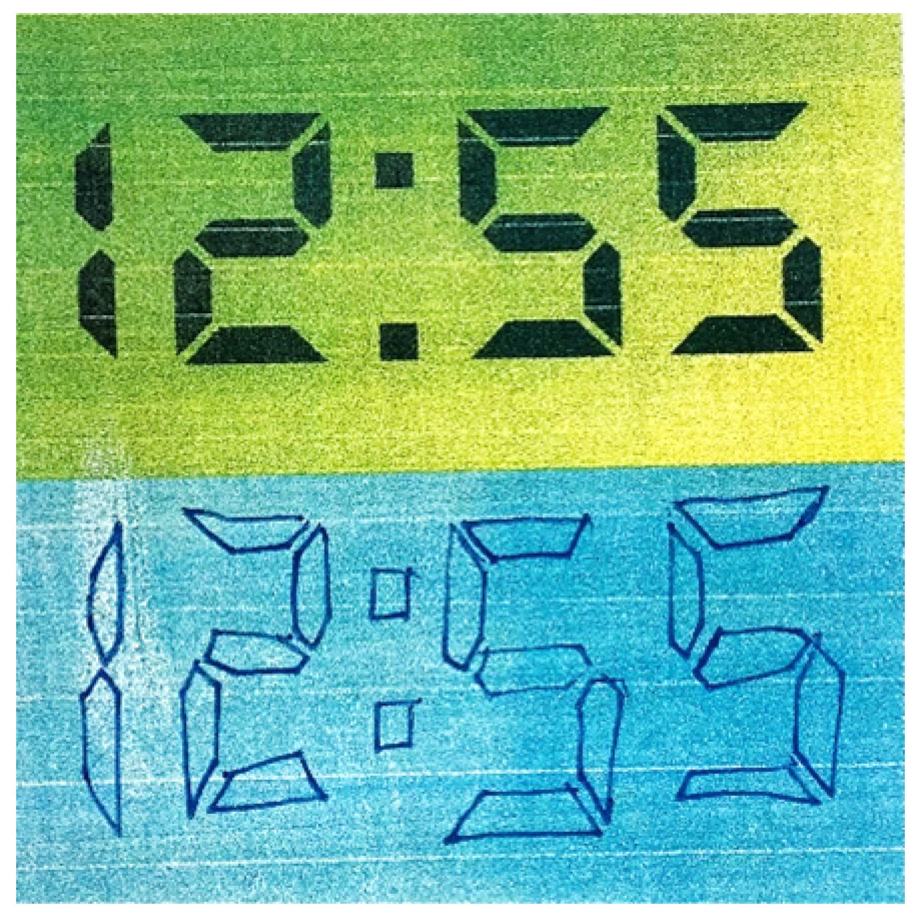
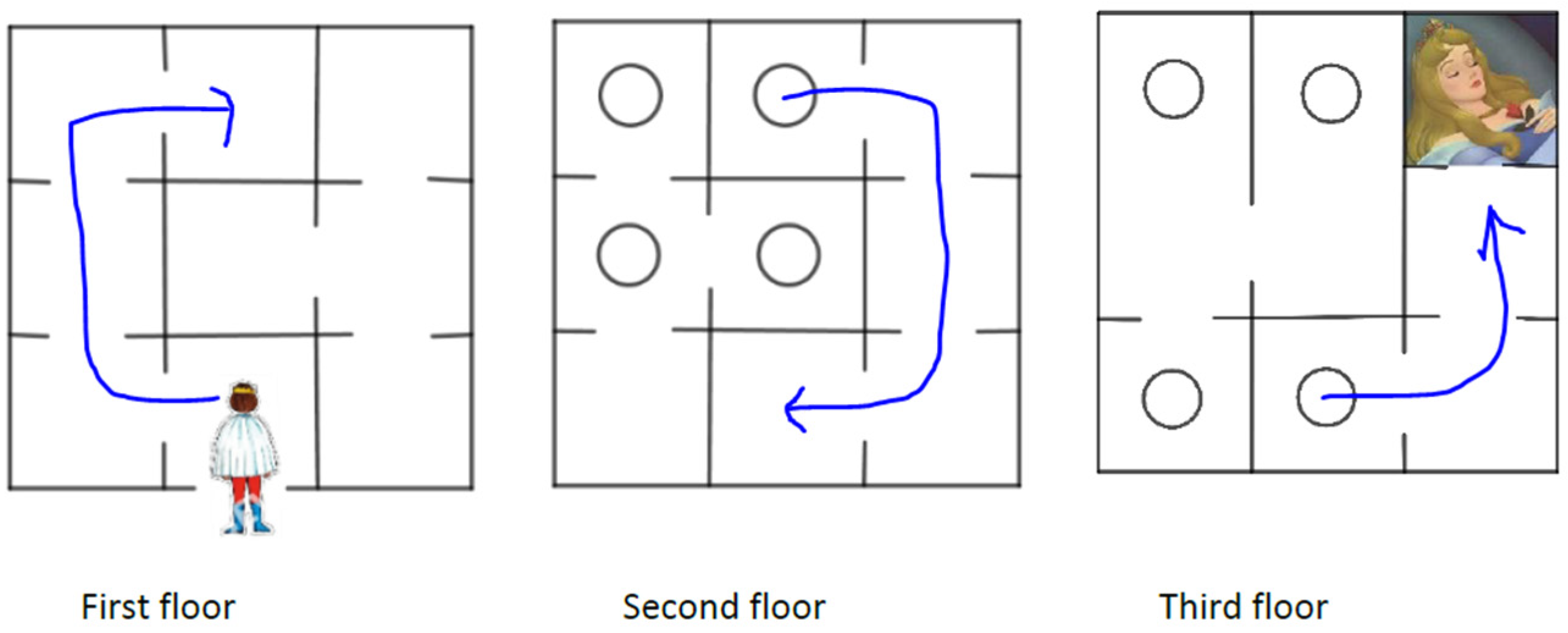
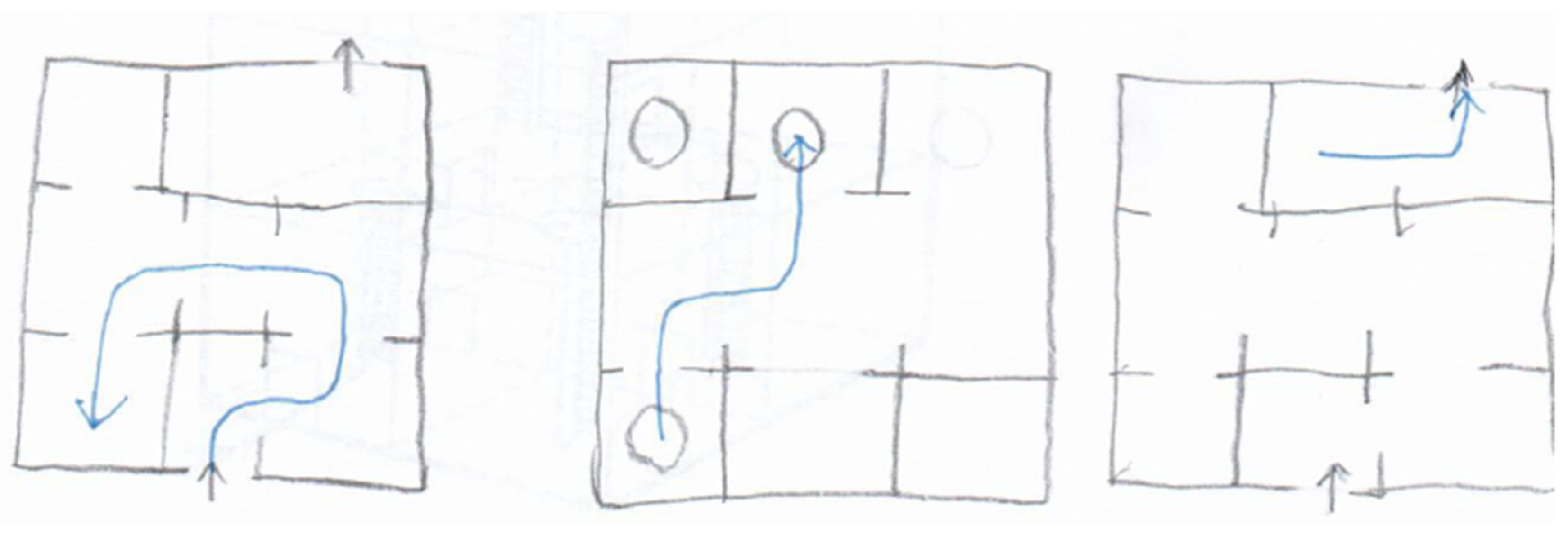
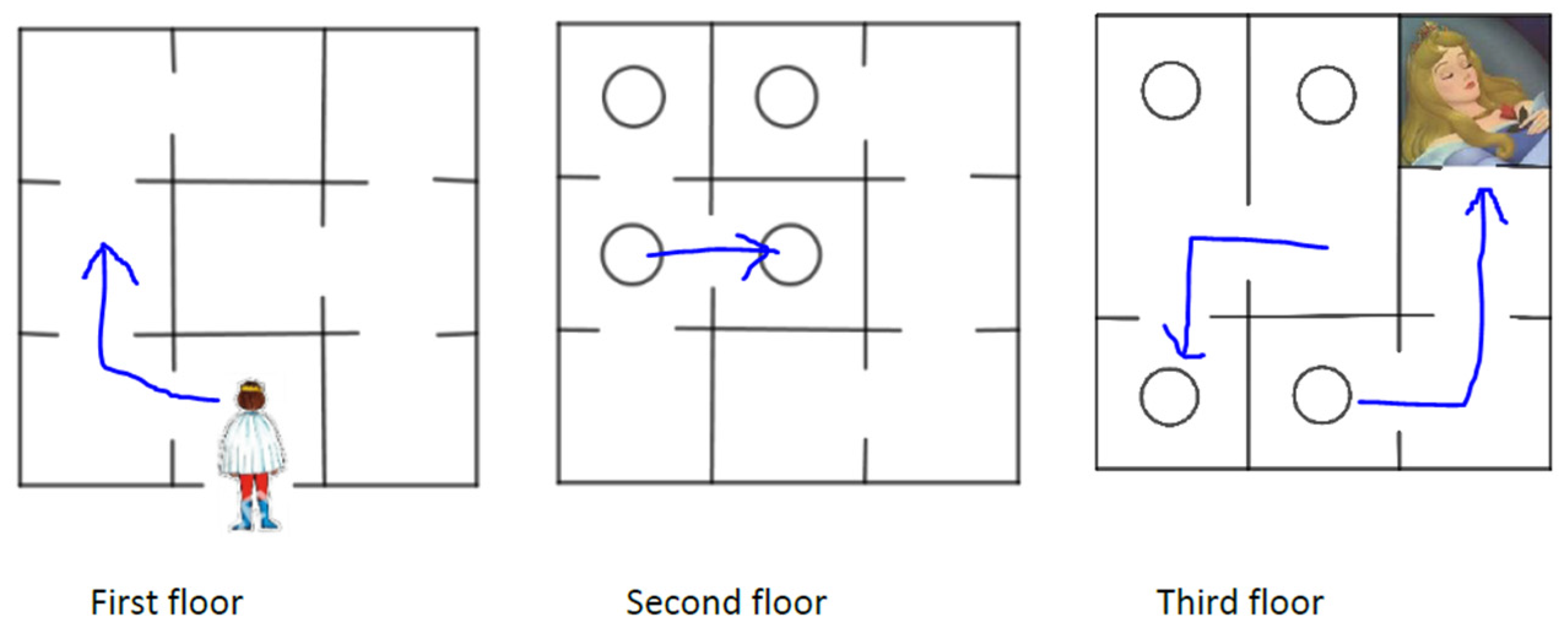
2.2.2. Spatial Labyrinths
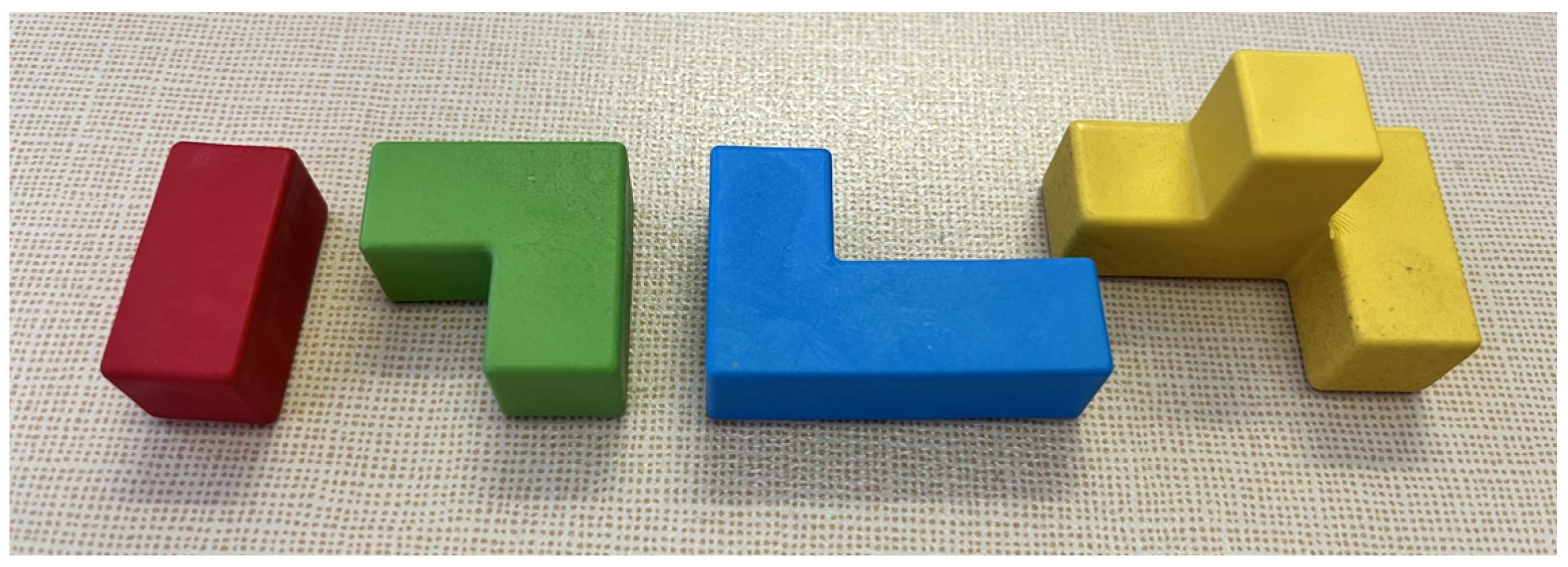
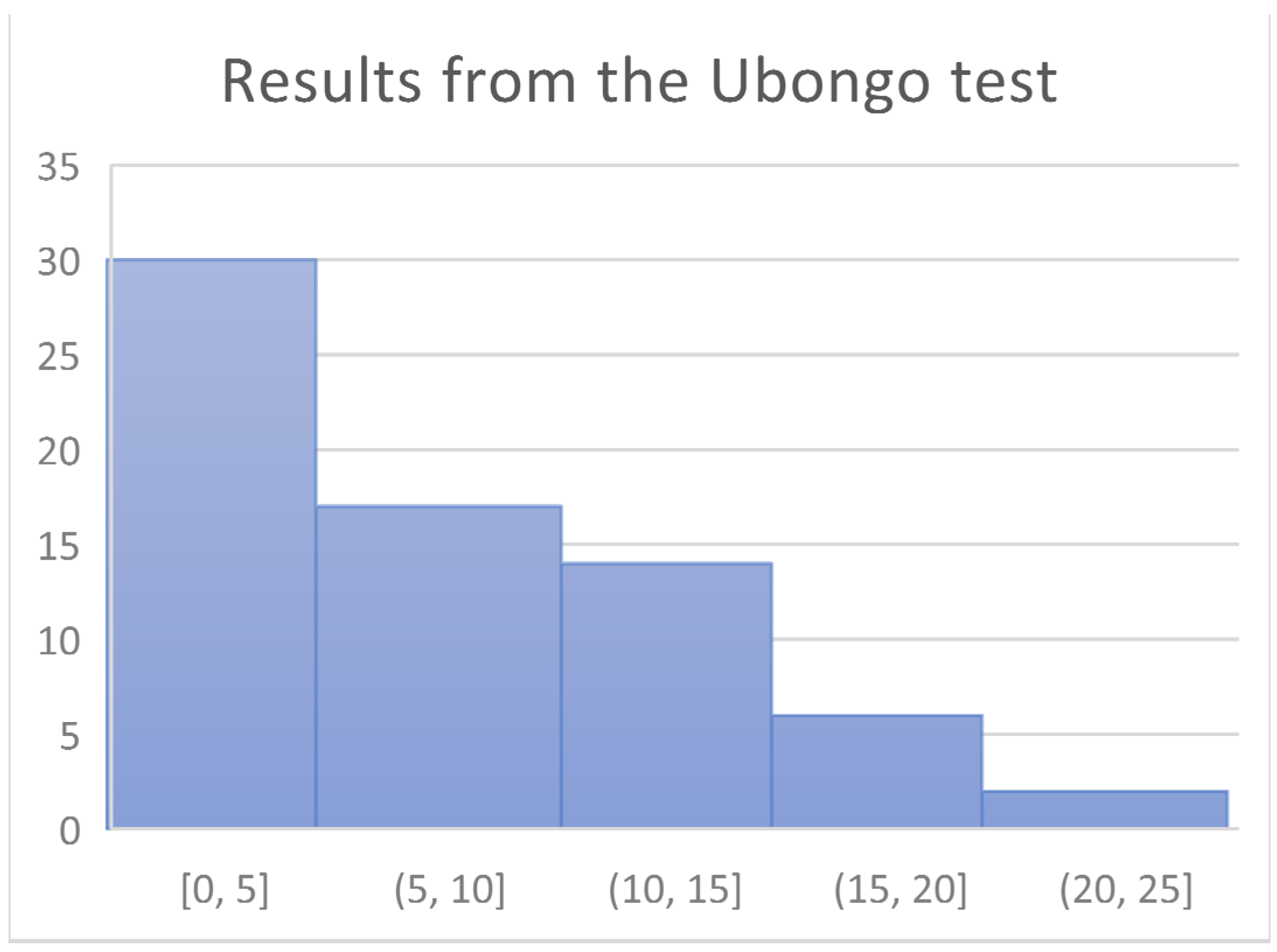
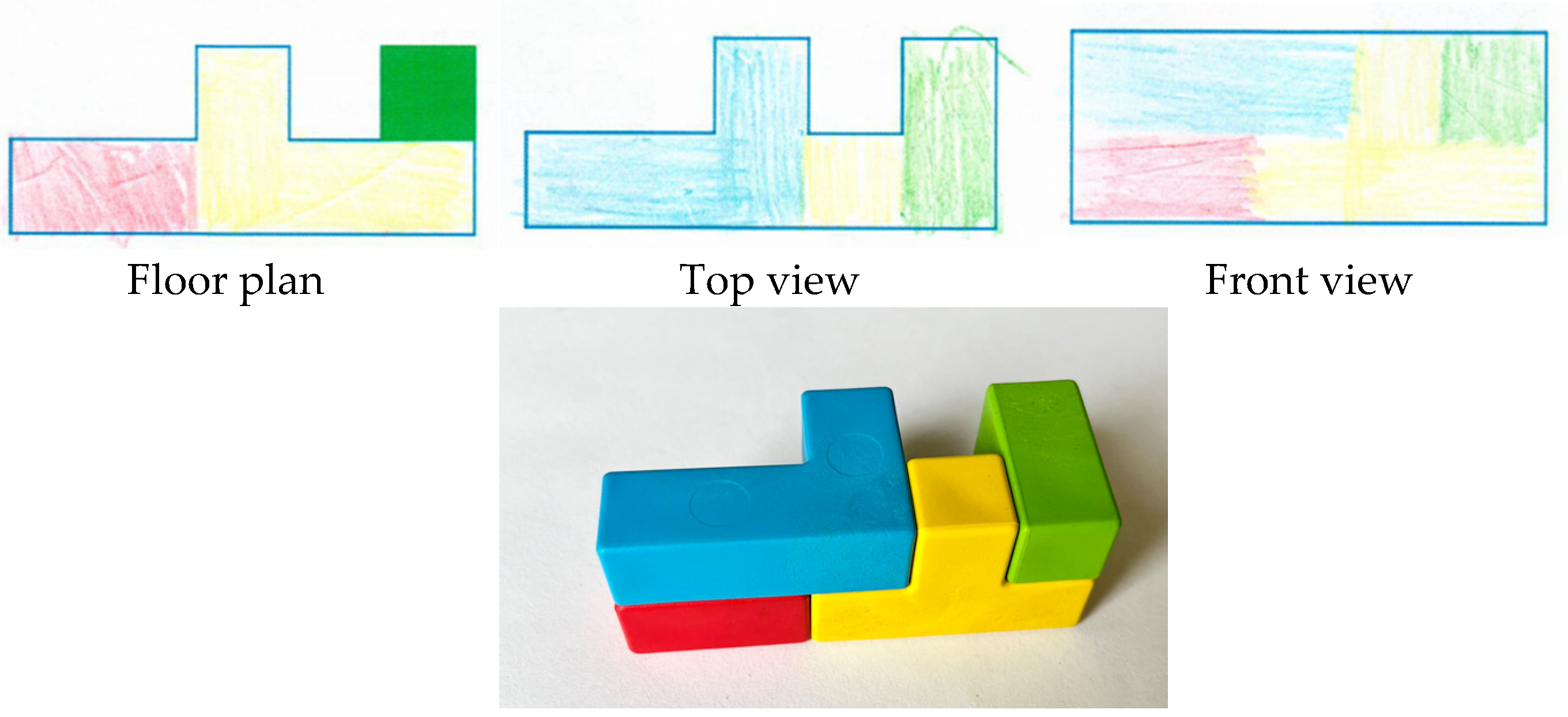
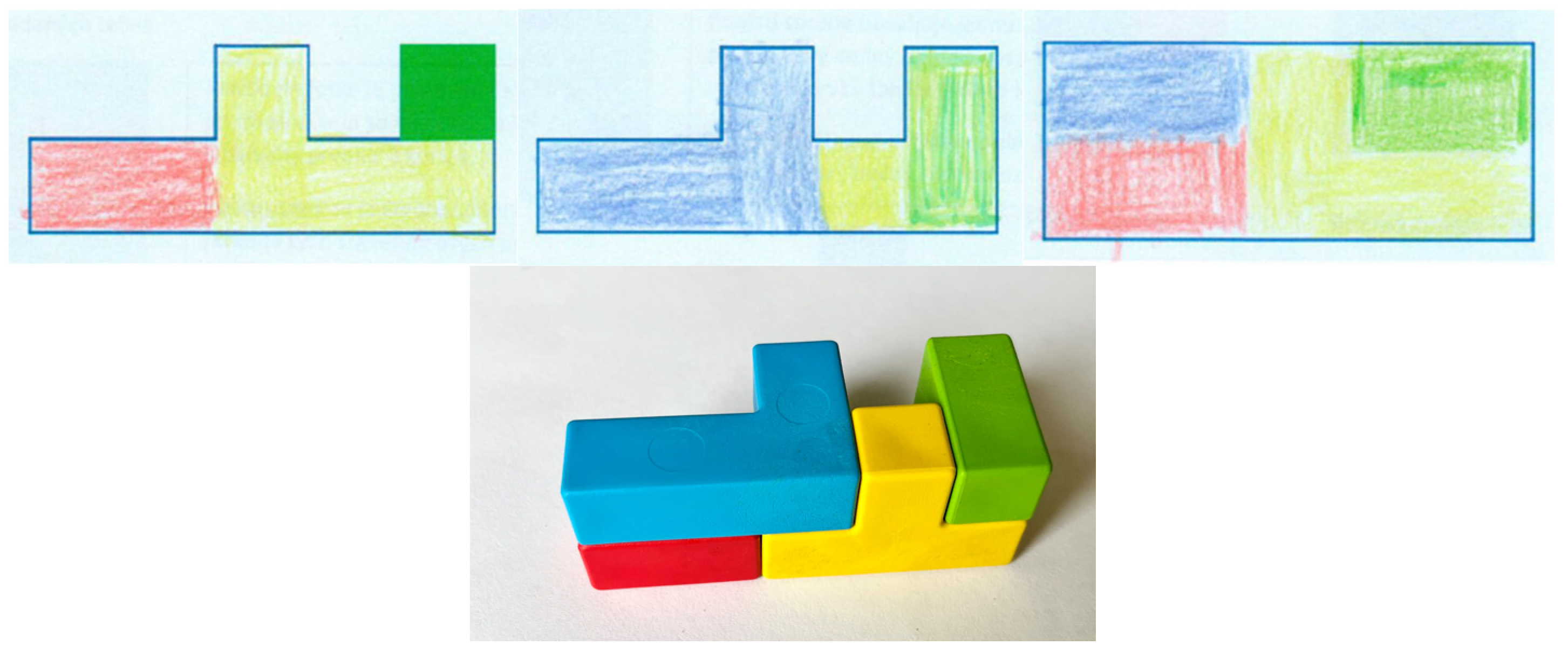
2.2.3. Ubongo Test
2.3. Participants
2.4. Case Studies
2.4.1. Exploratory Case Study
2.4.2. Atomic Analysis
- The revealed way of thinking will make it possible to advise the student how to overcome obstacles in learning.
- The researcher, who repeatedly tries to penetrate the pupil’s thinking, increases the sensitivity to understanding the pupil’s actions.
2.5. CHIC Analysis
3. Data Analysis and Results
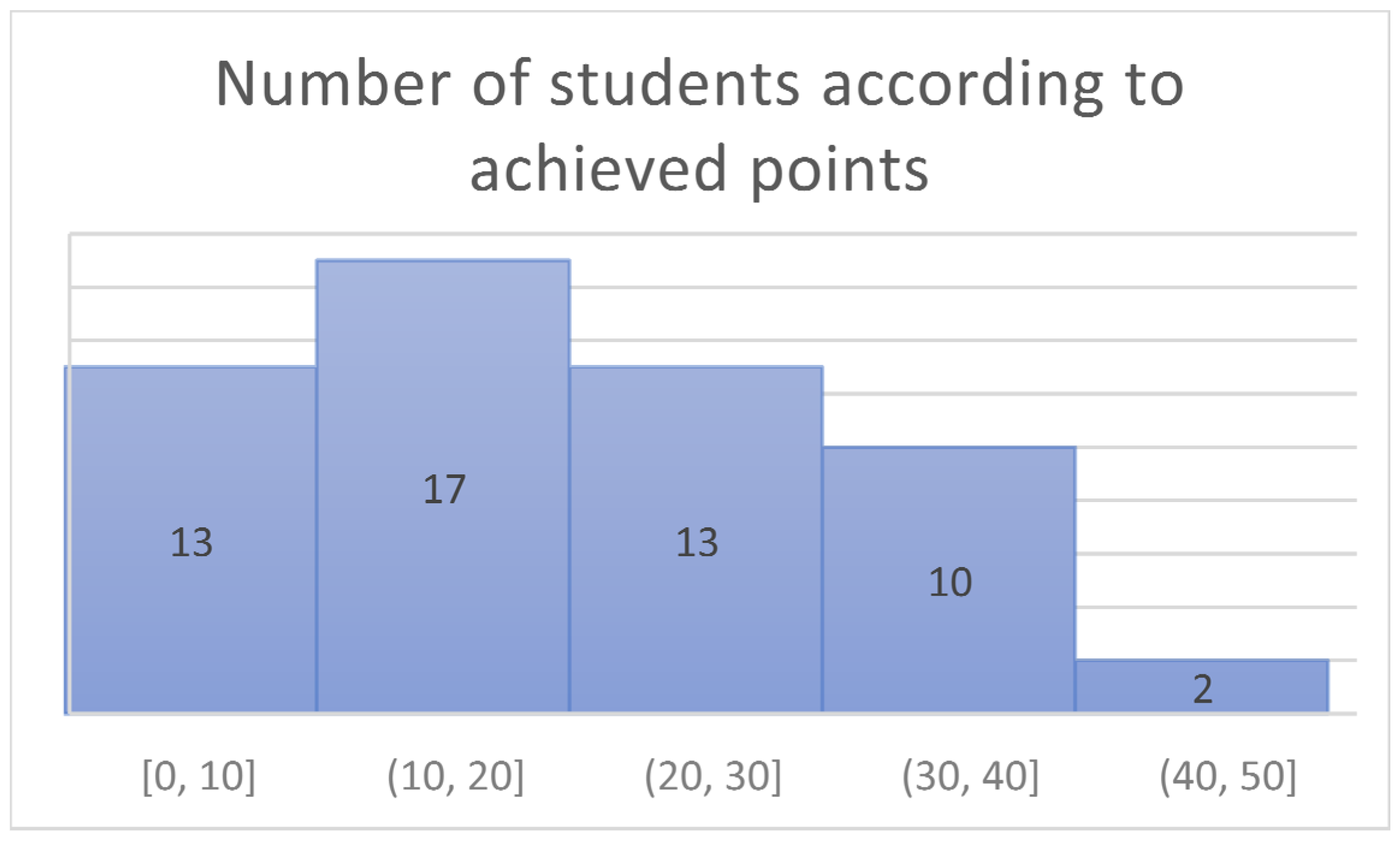
3.1. Quantitative Analysis of the Tests
3.2. The Case Studies
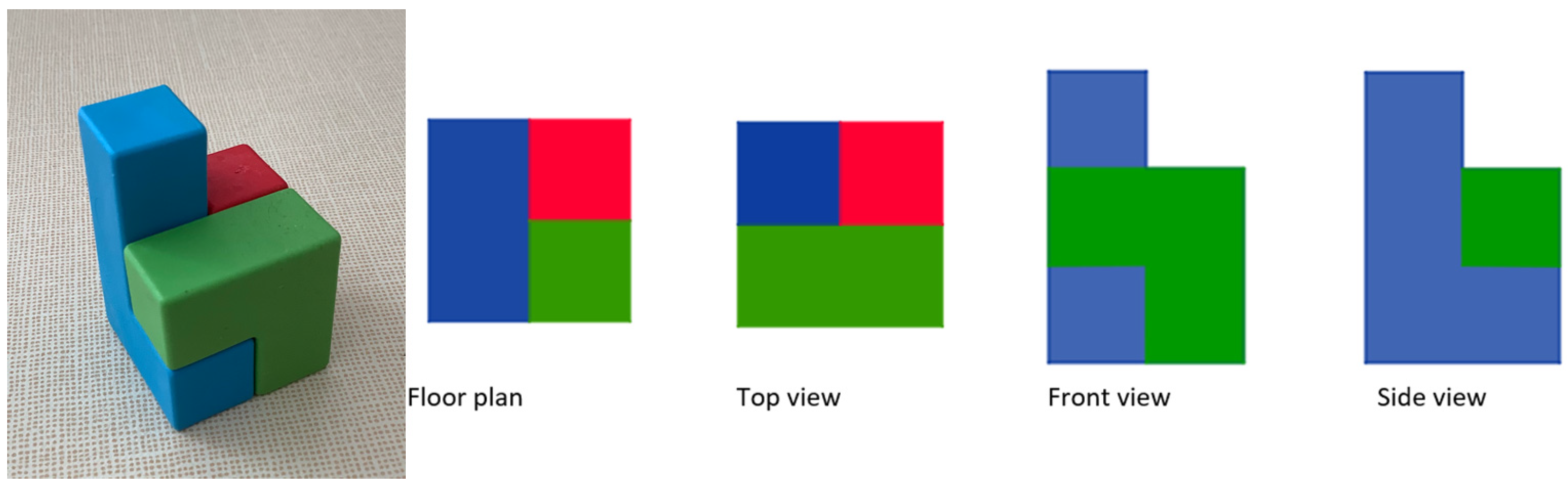
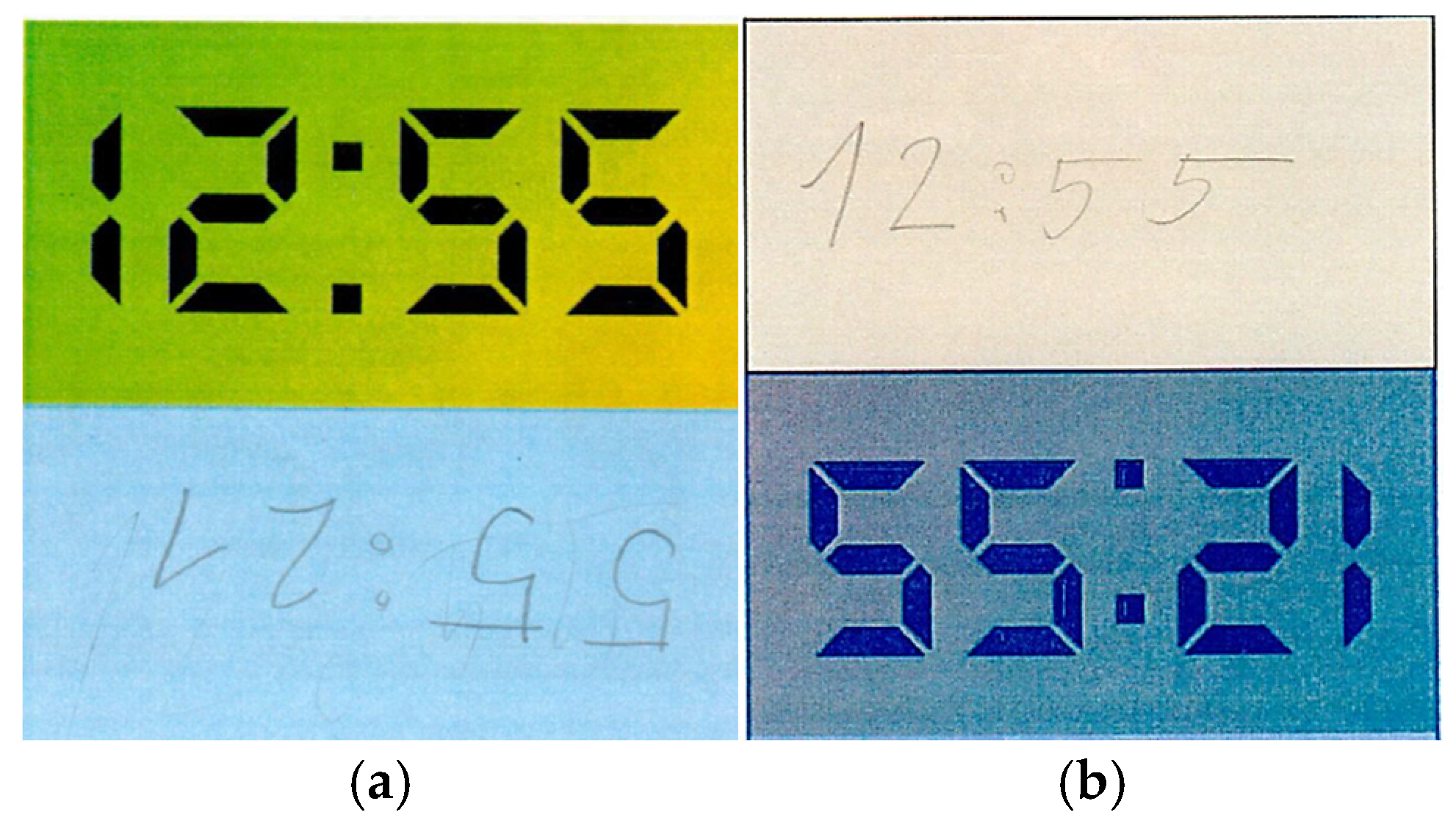
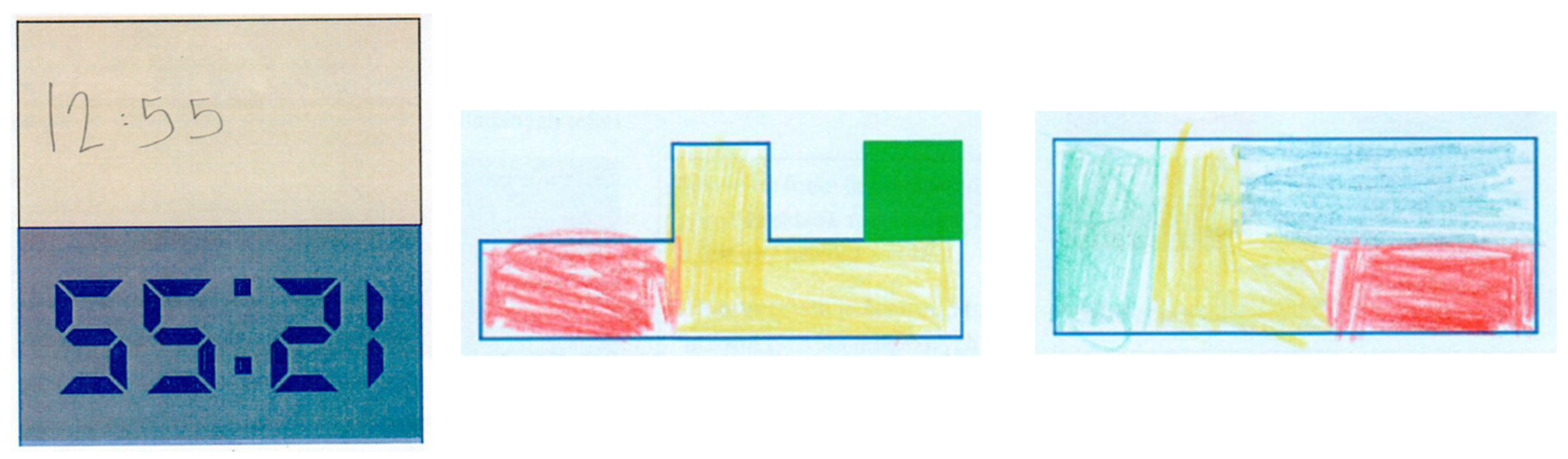
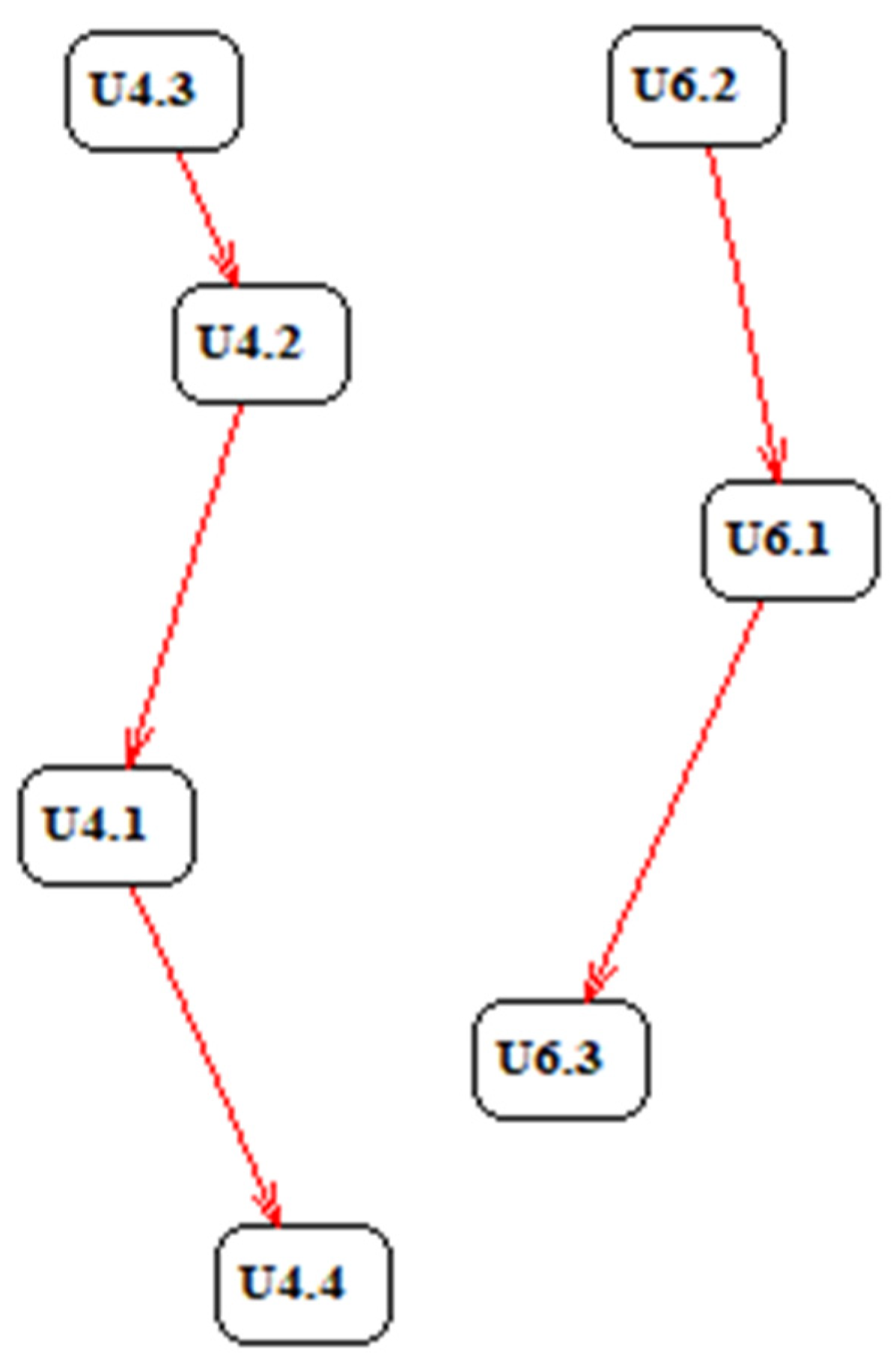
3.2.1. Atomic Analysis of a Good Solution
- The Ubongo test evaluation (Appendix C)
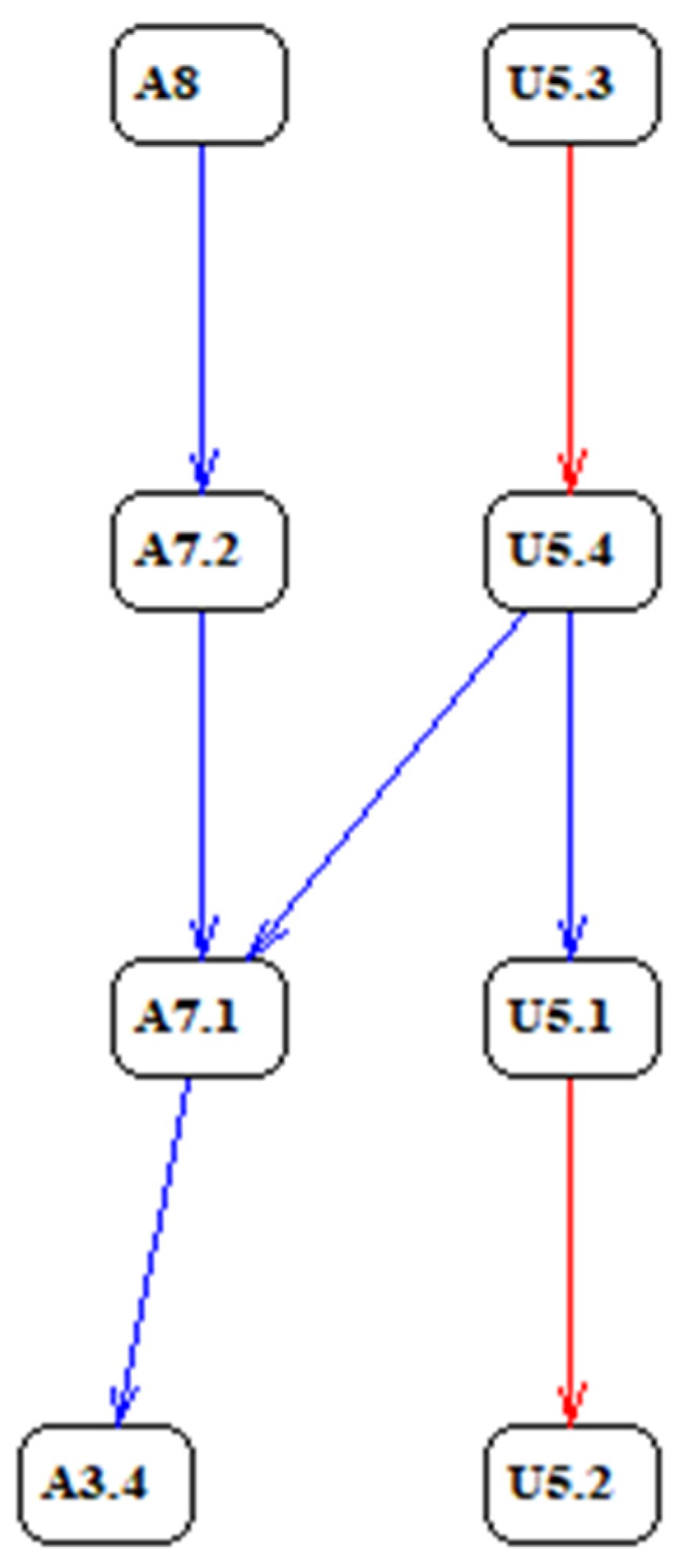
3.2.2. Atomic Analysis of a Mediocre Solution
- The Labyrinth test evaluation
- The Ubongo test evaluation.
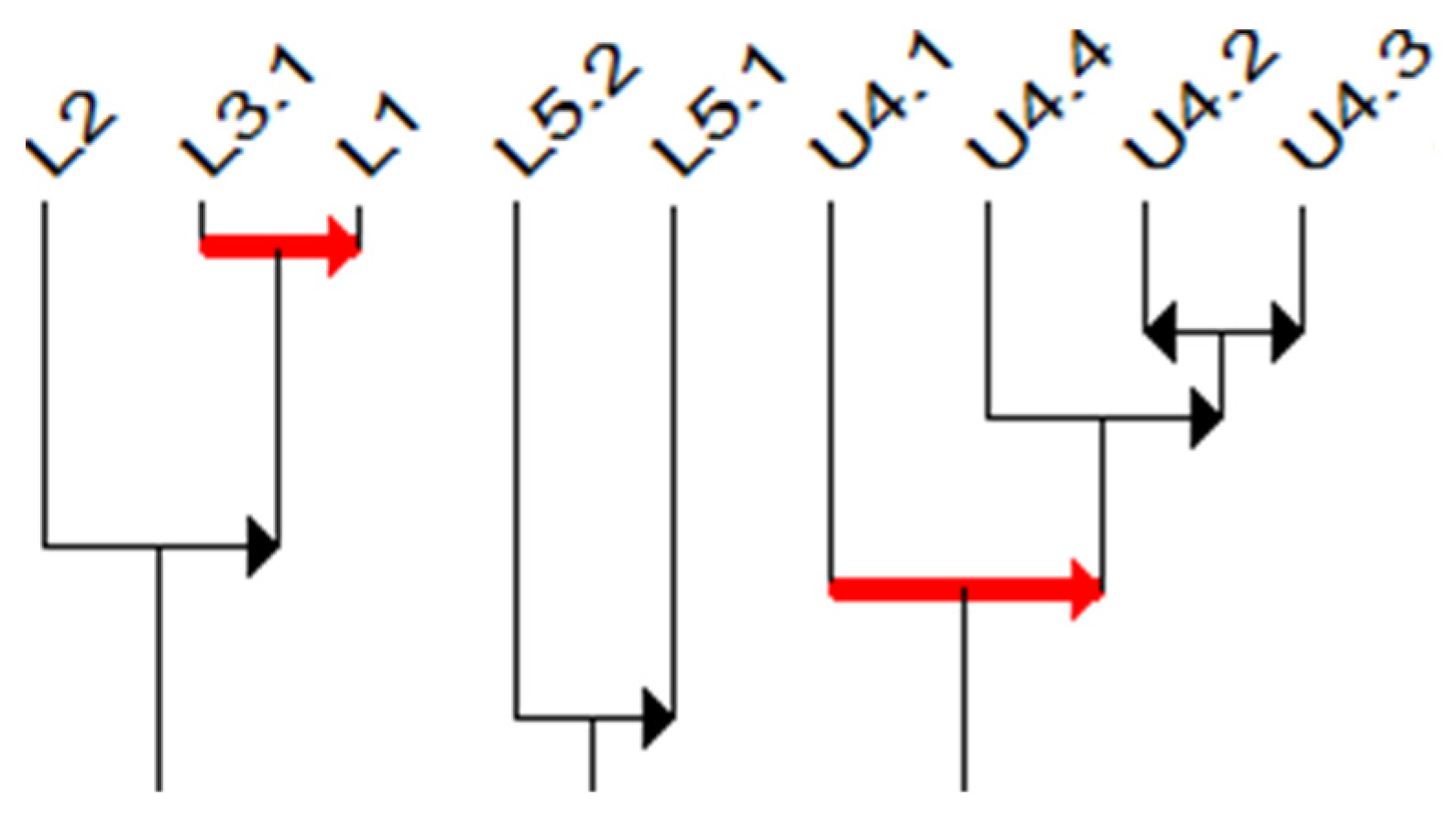
3.3. The CHIC Statistical Analysis
3.3.1. CHIC Results for Tests A, L, and U
3.3.2. CHIC Results for Tests A and U
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix C
References
- Vágová, R. Exploring the Interplay of Physical and Digital Manipulatives to Develop Students’ Visualisation in Solid Geometry. Dissertation Thesis, UKF, Nitra, Slovakia, 2021. [Google Scholar]
- Lean, G.A.; Clements, K. Spatial ability, visual imagery, and mathematical performance. Educ. Stud. Math. 1981, 12, 267–299. [Google Scholar] [CrossRef]
- Clements, K. Visual Imagery and School Mathematics. Part II. Learn. Math. 1982, 3, 33–38. [Google Scholar]
- Kosslyn, S.M. Image and Brain: The Resolution of the Imagery Debate; The USA, Massachusetts Institute of Technology: Cambridge, MA, USA, 1994; ISBN 0262611244. [Google Scholar]
- Kosslyn, S.M. Mental images and the brain. Cogn. Neuropsychol. 2005, 22, 333–347. [Google Scholar] [CrossRef] [PubMed]
- Duval, R. Representation, vision and visualization: Cognitive functions in mathematical thinking. In Proceedings of the 21st Annual Meeting North American Chapter of the International Group of PME, 1999; Hitt, F., Santos, M., Eds.; ERIC Clearinghouse for Science, Mathematics, and Environmental Education: Columbus, OH, USA, 1999; Volume 1, pp. 3–26. [Google Scholar]
- Battista, M.T. The development of geometric and spatial thinking. In Second Handbook of Research on Mathematics Teaching and Learning; Lester, F.K., Jr., Ed.; Information Age Publishing Inc.: Charlotte, NC, USA, 2007; pp. 843–908. ISBN 978-1-59311-586-9. [Google Scholar]
- Kuchemann, D. Children’s Difficulties with Single Reflections and Rotations, 1980. Available online: https://www.researchgate.net/publication/234728681_Children%27s_Difficulties_with_Single_Reflections_and_Rotations (accessed on 15 July 2024).
- Symmetry, a Foundational Concept in STEM. The Robertson Program for Inquiry-Based Teaching in Mathematics and Science; University of Toronto: Toronto, ON, Canada, 2022; Available online: https://wordpress.oise.utoronto.ca/robertson/2022/10/03/symmetry-a-foundational-concept-steam/ (accessed on 15 July 2024).
- Kmeťová, M.; Nagyová Lehocká, Z.; Szabó, T. Creativity and spatial skills in solid geometry and plane geometry in primary schools. In Proceedings of the 14th Annual International Conference of Education: Research and Innovation, Online, 8–9 November 2021; Available online: https://library.iated.org/publications/ICERI2021 (accessed on 15 July 2024).
- Tóth, A.; Žitný, R.; Szabó, T.; Csáky, A.; Kárpáti, A. Possibilities of Development of Spatial Imagination. In Proceedings of the 13th International Conference of Education, Research and Innovation: Transforming Education, Transforming Lives, Sevilla, Spain, 9–10 November 2020; pp. 565–573. [Google Scholar]
- Szabó, T.; Babály, B.; Pataiová, H.; Kárpáti, A. Development of Spatial Abilities of Preadolescents: What Works? Educ. Sci. 2023, 13, 312. [Google Scholar] [CrossRef]
- Kmeťová, M.; Nagyová Lehocká, Z. Using Tangram as a Manipulative Tool for Transition between 2D and 3D Perception in Geometry. Mathematics 2021, 9, 2185. [Google Scholar] [CrossRef]
- Markos, A.; Menexes, G.; Papadimitriou, I. The CHIC Analysis Software v1.0. In Classification as a Tool for Research. Studies in Classification, Data Analysis, and Knowledge Organization; Locarek-Junge, H., Weihs, C., Eds.; Springer: Berlin, Heidelberg, 2010. [Google Scholar] [CrossRef]
- Bassey, M. Case Study Research in Educational Settings; Open University Press: Buckinham, PA, USA, 1999. [Google Scholar]
- Hejný, M.; Niepel, L. Šetnásť Matematických Príbehov; Mladé letá: Bratislava, Slovakia, 1983. [Google Scholar]
- Mathematics, National Educational Program (in Slovak: Matematika, Štátny Vzdelávací Program ISCED 1). Available online: https://www.statpedu.sk/files/articles/dokumenty/statny-vzdelavaci-program/matematika_isced1.pdf (accessed on 18 February 2024).
- Babály, B.; Kárpáti, A. A téri képességek vizsgálata papír alapú és online tesztekkel. (Testing spatial abilities with paper-based and online tests, in Hungarian). Magyar Pedagógia 2015, 115, 67–92. [Google Scholar] [CrossRef]
- Kárpáti, A.; Babály, B.; Budai, L. Developmental Assessment of Spatial Abilities through Interactive, Online 2D and Virtual 3D Task. Int. J. Arts Educ. 2014, 12, 94–124. [Google Scholar]
- WAC Clearinghouse, Case Studies. Available online: https://wac.colostate.edu/repository/writing/guides/casestudies/ (accessed on 15 July 2024).
- Sturman, A. Case study methods. In Educational Research Methodology, and Measurement: An International Handbook, 2nd ed.; Keeves, J.P., Ed.; Pergamon: Oxford, UK, 1994. [Google Scholar]
- Yin, R.K. Case Study Research: Design and Methods, 2nd ed.; Sage: London, UK, 1994. [Google Scholar]
- Glaser, B.; Strauss, A. The Discovery of Grounded Theory: Strategies for Qualitative Research; Aldine: Chicago, IL, USA, 1967. [Google Scholar]
- Bryant, A.; Charmaz, K. The SAGE Handbook of Grounded Theory; SAGE Publications: London, UK, 2007. [Google Scholar]
- Hejný, M.; Michalcová, A. Skúmanie Matematického Riešiteľského Postupu; (Investigation of the mathematical solution process, in Slovak); MC: Bratislava, Slovakia, 2001. [Google Scholar]
- Stehlíková, N. The Research Methods Employed by Contributors to the Prague Didactics of Mathematics Seminars. In European Research in Mathematics Education I, Volume 2. First Conference of the European Society for Research in Mathematics Education. (CERME 1, August 1998); Schwank, I., Ed.; Forschungsinstitut für Mathematikdidaktik: Osnabrück, Germany, 1999; ISBN 3-925386-51-3. Available online: https://hal.science/hal-02270689/document (accessed on 15 July 2024).
- Couturier, R. CHIC: Cohesive Hierarchical Implicative Classification. In Statistical Implicative Analysis. Studies in Computational Intelligence; Gras, R., Suzuki, E., Guillet, F., Spagnolo, F., Eds.; Springer: Berlin/Heidelberg, Germany, 2008; Volume 127. [Google Scholar] [CrossRef]
- Rumanová, L.; Pavlovičová, G.; Švecová, V. Apply of statistical software CHIC in evaluation of didactical experiment. Forum Stat. Slovacum 2011, 7, 166–170, ISSN 1336-7420. [Google Scholar]
- Laššová, K.; Rumanová, L. Engaging STEM Learning Experience of Spatial Ability through Activities with Using Math Trail. Mathematics 2023, 11, 2541. [Google Scholar] [CrossRef]









Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kmetová, M.; Rumanová, L. Perception of Symmetry and Spatial Reasoning in 11–12-Year-Old Pupils. Educ. Sci. 2024, 14, 1402. https://doi.org/10.3390/educsci14121402
Kmetová M, Rumanová L. Perception of Symmetry and Spatial Reasoning in 11–12-Year-Old Pupils. Education Sciences. 2024; 14(12):1402. https://doi.org/10.3390/educsci14121402
Chicago/Turabian StyleKmetová, Mária, and Lucia Rumanová. 2024. "Perception of Symmetry and Spatial Reasoning in 11–12-Year-Old Pupils" Education Sciences 14, no. 12: 1402. https://doi.org/10.3390/educsci14121402
APA StyleKmetová, M., & Rumanová, L. (2024). Perception of Symmetry and Spatial Reasoning in 11–12-Year-Old Pupils. Education Sciences, 14(12), 1402. https://doi.org/10.3390/educsci14121402






