Teacher Learning towards Equitable Mathematics Classrooms: Reframing Problems of Practice

Abstract

This study responds to the debate on understanding and evaluating teacher learning in professional development programmes, with particular reference to the development of equitable mathematics classrooms. Conducted in the context of a year-long PD mathematics programme for primary teachers in Norway, designed to disrupt teachers’ assumptions about mathematics pedagogy and how it relates to students’ mathematical thinking, this study takes teachers’ entry goals as its point of departure. Sixteen teachers participated in interviews at the end of the course. Recognising the situated nature of the development of pedagogic judgement in our analysis of teachers’ reflections on their learning, we report on the shift in their “problems of practice” towards actionable concerns about student inclusion. We argue that this shift underpins a fundamental change in their assumptions about teaching and learning and a critical stance towards their own professional practice, suggesting an important indicator of what constitutes sustainable professional development for critical mathematics education.

1. Introduction

Success in school mathematics has a major impact on students’ futures in terms of both academic opportunities and participation in society. However, while equity in mathematics instruction is a widely shared aim, there is little consensus on how to achieve it. As mathematics teacher educators, our main interest in the issue is how to develop sustainable change in teachers’ practice, given the pressures of high-stakes accountability cultures that frequently undermine moves to equitable instruction [1]. In this article, we report on teachers’ learning in a year-long professional development programme that aims to challenge and redirect their existing pedagogic practice, with particular respect to connecting with children’s mathematical thinking. The programme is part of a national strategy in Norway, the Competency for Quality initiative (Kompetanse for Kvalitet, KfK), which addresses the fact that primary school teachers are generalists and that many lack specific training in mathematics. In operation from 2014 to 2025, the KfK strategy includes courses on a broad range of subjects and at multiple universities, with each institution having autonomy over the course design within some set parameters. In the particular version of the mathematics KfK course described here, the designers aim to disrupt teachers’ preconceptions about their students’ mathematical knowledge and thinking and develop a dialogic and inquiry-based approach to teaching for inclusion. We situate our study of these teachers’ learning trajectories as a contribution to our understanding of how we can move away from the snap-shot evaluation of professional development in terms of the implementation of “best practice” to a focus on teacher learning as the development of context-sensitive and critical pedagogical judgement.

2. Literature Review

Educators’ and researchers’ understanding of what constitutes equitable mathematics teaching has developed over time, and we adhere here to the multidimensional perspective proposed by Gutiérrez, combining issues of access, achievement, identity and power ([2] p. 21). Raising the question of what can be done for the realisation of equitable classrooms, she proposes that general initiatives targeting teaching resources, teacher knowledge, policy and so on are not sufficient, while attention to the particular contexts in which teachers work is key. This concern for the incorporation of the situated nature of teachers’ work into initiatives for equity raises serious questions for professional development programmes that focus on the top-down dissemination of principles for equitable teaching while ignoring the nature of teacher learning and the need to recontextualise the programme content in local school cultures [3]. Teachers are central in ensuring that values of equity permeate the reality of classrooms. For example, how they see students’ capability for learning influences the cognitive demand of tasks they choose and how they present them. Thus, seeing students as “weak” or “slow” creates apparent mismatches that lead teachers to doubt their capability for rich tasks [4], leading to simplification in order to reduce what they perceive as harmful challenges to learners who need “protective nurturance” [5]. Task design and selection may also privilege some students over others in terms of their background knowledge [6], specifically in terms of the use of context, which presents multiple challenges in representing diversity of gender, class, culture and so on [7], or unfounded assumptions that certain everyday contexts suit certain groups best [8]. Thus, Gutiérrez ([2] p. 24) provides a vivid account in which disadvantaged (poor, working class, minority ethnic) students’ academic success could be traced to a community of teachers who developed “a deep understanding of their students”, avoiding assumptions that “pre-scripted contexts for Latina/o students such as tortillas instead of bread” were key to addressing excluded student identities (p. 27). Similarly, Kinser-Traut and Turner [9] report how a teacher committed to building on students’ funds of knowledge struggled to incorporate this into her teaching, ending in appropriating the knowledge for herself as she attempted to share authority in the classroom, thus undermining the principle of building on students’ home knowledge. These issues underline the context sensitivity of connecting with students’ mathematical thinking and the need for awareness of the cultural and ideological dimensions of teacher noticing [10].

This emphasis on the importance of understanding teachers’ equity-based judgements in context is underlined in Goldsmith et al.’s [11] systematic review of 106 studies on the professional learning of practising mathematics teachers from 1985 to 2008. Reflecting the state of the field at the time, professional learning was defined in the review as a change in knowledge and/or in practices, as well as a change in beliefs and dispositions that might influence knowledge or practice (p. 7). While the research on teachers’ actual professional lives was already becoming more prominent, with more than twice as many studies considering changes in practice than changes in knowledge, changes in teachers’ pedagogical reasoning were largely overlooked (just 8 out of 106 studies consider teachers taking on an inquiry stance as learning). Although rare, some studies (e.g., [12,13]) reported on the contextual embedding (school discourse, district policies, etc.) that hindered or supported changes in pedagogical practice (p. 15). The authors of the review concluded that it is problematic that studies tended to seek an answer to the question of whether a programme is effective, rather than how it works, particularly how it works in different settings ([11] p. 21). The few studies that reported on how found that the process was far from direct in the sense of moving “from a single professional experience to a change in practice to improvement of student outcomes” but rather was “often incremental, nonlinear and iterative, proceeding through repeated cycles of inquiry outside the classroom and implementation in the classroom” (p. 20), with small advances in one domain (e.g., knowledge, beliefs or practice) triggering a new process leading to another small change.

Questioning the meaningfulness of evaluating teachers’ practices against theoretical “best practice” without examining the why behind the actions, Horn [14] proposed pedagogical judgement as a concept that acknowledges the complexity of change in teachers’ practice as far more than the acquisition of new skills or knowledge. Rather, teacher learning involves the development of a situated judgement, which includes the interpretation of local contexts and concerns; teacher learning is not just about conceptual change, but it “also involves developing ecological, holistic, integrated views of these aspects of teaching and continually exploring their interdependence in different situations” ([15] p. 46). This integration of multiple contextualised understandings that are in continual development is operationalised by Horn and Garner in terms of teachers’ ongoing refinement of “problems of practice”—the stories that teachers tell from their professional practice about the problems they encounter. The framing of such problems of practice (what is the reason for the problem?) is consequential for how teachers envisage possible solutions [16]. Babichenko et al. [17] argue that the resulting “actionability”—is there something that can be done?—is a factor in defining teachers’ agentic space. This research highlights the fact that framing in teacher talk is not fixed, as both the person raising the issue and others can contribute to its reframing and thus, implicitly, to the envisaged solutions. Thus, the framing and reframing of problems of practice is likely a productive site for teacher learning [18] when teachers engage with the issues and refine their pedagogical reasoning in a process that is closely related to shifts in teachers’ noticing as they make sense of classroom situations, including what their attention is drawn to and, depending on the definition used, how they interpret what they see and how they decide to act on their interpretations [19,20]. While this potential for reframing is not necessarily realised, even in settings that are intended to promote professional development, there is evidence of examples of how such concrete problems from one teacher’s practice can engage colleagues in processes of generative learning that transcend the specifics of the situation [21,22].

Thus, problems of practice define teachers’ own agendas for professional development, and Horn and Garner describe how teachers move from a view of their own learning as filling “knowledge/skills gaps” to a more complex understanding of teaching whereby “no amount of additional information would sufficiently account for all sources of variability” ([15] p. 147). In the absence of a single or final solution to a problem of practice, teachers’ pedagogical judgements are therefore based on iterative relationships between reasoning and actions, which are both connected to and contextualised by institutional obligations and ethical commitments, as depicted in Figure 1.

In this paper, we focus on shifts in teachers’ framing of problems of practice in connection to the KfK PD course, understanding this reframing in terms of their evolution of pedagogical judgement. Thus, we pose the following Research Questions:

• How did teachers’ problems of practice change during the course?
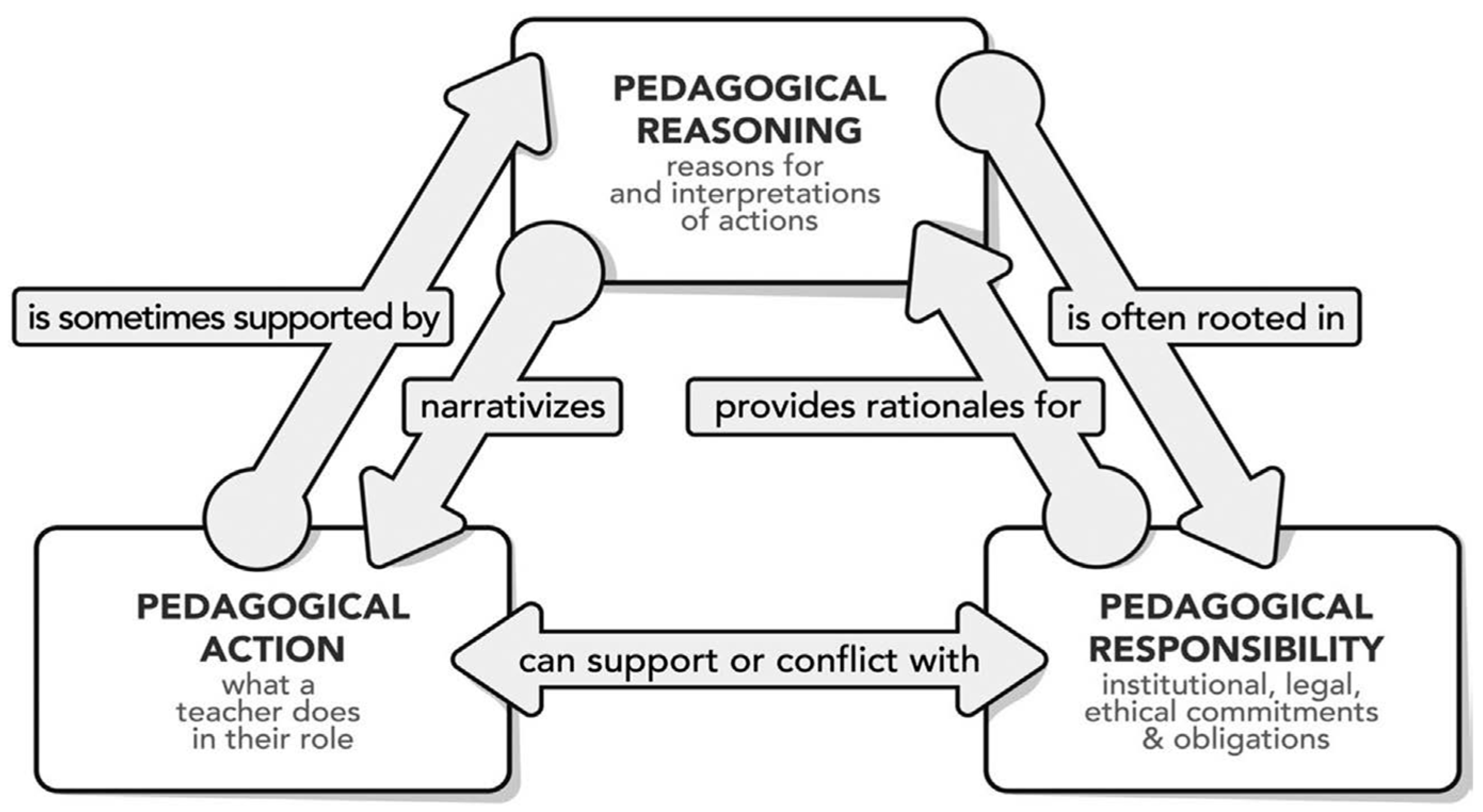
• How does their reframing of problems of practice relate to the development of pedagogical judgement?

3. Methodology

This study is part of the larger research project Inclusive Mathematics Teaching: Understanding and developing school and classroom strategies for raising attainment (IMaT), financed by the Research Council of Norway Grant 287132. Ethical approval for the overall project was obtained from the Norwegian national body for ethics in research (NSD, now Sikt—Norwegian Agency for Shared Services in Education and Research). We report on data collected from teachers enrolled in a mathematics professional development course for grades 1–7 (ages 6–13) in the academic year 2020–2021 at a large teacher education provider in Norway. All participating teachers worked at the time as teachers in schools; most held formal teaching qualifications, although some did not, having been hired as teachers under a special exemption granted to schools because of staffing shortages. Some of the qualified teachers had completed a half-year unit in mathematics education as part of their training, but others needed to top up their mathematics teaching education to meet new government requirements introduced retroactively for primary school teachers in Norway. Consequently, some teachers participated in the course for continuing professional development, while others enrolled to fulfil their licensure requirements.

The course was taught by two experienced mathematics teacher educators (MTEs), Silje and Daniel, working together in a two-teacher system, where Silje was the course designer. Teachers, working in groups through the year, attended six “gatherings” evenly distributed over two semesters, where each gathering was organised as two full consecutive days of teaching focusing on teachers’ lived experiences of being in the classroom and connecting this with research-based perspectives on mathematics teaching and learning. Furthermore, course assignments (“missions”) required teacher groups to create sites of professional inquiry in their school contexts (rather than implementing ideas supplied by the course tutors), reflecting critically in their groups on their observations, particularly student thinking, in light of issues raised in the course. They submitted jointly written reports, with mathematics teacher educators nurturing teachers’ criticality by giving feedback that raised new issues, pressed for justification or suggested alternative interpretations based on the empirical data included. Both the course design and the implementation were centred around the MTEs’ own “theory of change”, grounded in the stance that subject knowledge per se is not a main consideration for the facilitation of practical, inquiry-based and theoretical work that values and develops students’ mathematical knowledge and mathematical thinking. We summarise the theory of change here, focusing in particular on how the course designer aims to use confrontation to disrupt teachers’ preconceptions [23] and promote a radical change in their practice (for a detailed account, see Eriksen and Solomon [24]). Drawing on a Realistic Mathematics Education philosophy [25], Silje and Daniel aimed to enable participants to develop a reform-based approach by:

• Discussing authentic student work and noticing what students can do, considering what lies on their closest learning horizon, and how the teacher can challenge them to develop their thinking by:

  ○

  Discussing the learning landscape/student learning trajectories;

  ○

  Analysing and discussing the work of their own students (“missions”);

  ○

  Becoming familiar with, working with and accounting for informal and pre-formal methods that can eventually be used as teaching tools.

• Drawing on a range of theoretically driven approaches to support analysis and reflection to develop conversational features/rich discussion.
• Asking teachers to work in an investigative manner in and with mathematics throughout the course.
• Modelling the practice advocated by the course themselves.

As part of the larger project on inclusive mathematics teaching, data collection during the PD course was extensive. We draw here on two datasets drawn from the participating teachers. Dataset one, the entry data, was gathered from all course participants (31 out of 40) who responded to Silje and Daniel’s e-mail request prior to the course start asking them to explain in writing their motivation for enrolling, their expectations of how the course might support their mathematics teaching, and their vision of what they would ideally like their mathematics teaching to be. The second and third authors asked the teachers for permission to use these data at the end of the first gathering, which they had joined as researchers engaged in the project. Dataset two, the exit data, consists of audio-recorded interviews with all 16 of the 40 course participants who responded positively to our request for interviews towards the end of the course. Thirteen of them had also provided entry data. All participants worked as teachers in schools, leading to various time constraints; to accommodate these, we interviewed 3 teachers individually, while the remaining 13 were organised into four group interviews (see

Table 1. Overview of individual and group interviews.

Individual interviews	Jenny Ina Mathilde
Group interviews	Sofie, Rikke, Hedda, Vilde Rita, Ulf Gaute, Hedvig, Trude Aurora, Solvor, Nora, Gina

). Interview topics were provided to each teacher in advance and covered their background as mathematics teachers; comparisons between their earlier experience in teaching the four mathematical operations and the approach used in the course; and the teaching of mathematics through inquiry.

All interviews were conducted on Zoom in Norwegian and were transcribed and translated into English by the authors, who include both native Norwegian and native English speakers, with the aim of capturing sense in plausible English rather than producing a literal translation. Appropriate translations were extensively discussed between the authors. Dataset one was also written in Norwegian and was similarly translated and discussed.

We began the analysis of both datasets by identifying problems of practice, subscribing to the assumption that these are productive sites for teacher learning [21]. Specifically, we identified issues in professional practice that teachers brought up as problematic in some sense, be they actionable (there is a non-routine solution that teachers can develop within their own sphere of action) or nonactionable (solutions are obstructed by immutable student characteristics or structural constraints). Unlike Horn and Little [21], we included problems-of-practice issues that may not appear to be directly related to classroom activities (e.g., teachers’ efforts to communicate with colleagues). Our view is that, since teachers were asked about classrooms, by bringing up other school issues that may not physically be located in the classroom, they signal to us their perceived interrelatedness.

Having systematically looked for problems of practice in both datasets, we continued our analysis of dataset one by identifying teachers’ assumptions about their cause and what would constitute “help” (in terms of learning), that is, their framing of their particular problems of practice. In the analysis of dataset two, we drew more interpretively on Anderson and Justice’s definition of disruption as “an analytical construct that allows for the investigation of how individual learning and changes in local practice mutually influence the other within a purposefully designed learning context” ([23] p. 401). In doing so, we identified disruptions as described by the teachers when comparing differences between their previous experiences of, and beliefs about, teaching and the teaching approaches and activities advocated and used in the course. When teachers reframed their initial problems of practice, we endeavoured to pick out connections to the course design (trying new teaching strategies and tasks out in their own classrooms, group discussions and analysis of students’ work) and references to pedagogical judgement, as described in Horn and Garner’s [15] model (explanations/justifications of pedagogical actions in the classroom, resolution of ethical dilemmas).

Any qualitative study raises issues of trustworthiness, and this is heightened in the context of potential power imbalances, as in this study, where participants were aware that the researchers and their instructors were close colleagues. While this was not an issue for dataset one, which was produced for the instructors rather than the researchers, the interviews in dataset two could be seen to be overshadowed by this relationship. We are optimistic that this did not interfere with participants’ responses on the basis of (1) the overall ethos of the course in encouraging participants to express their opinions openly in discussion and (2) our actual experience of participants expressing views that they might have been hesitant to say to an instructor (“I do this because I have to”) or being critical of some aspects of the course. Another issue concerns our use of a mixture of individual and group interviews and whether these can be treated similarly in the analysis since group interviews include multiple voices. We found that problems of practice were at times co-constructed, with participants building on each other’s contributions (e.g., one might describe a situation and another would raise a question on how that situation might be handled). Since this is an important site of learning, we highlighted such co-constructions in our analysis. However, we argue that even individual interviews are co-constructions of more than one voice [26], and in our analysis, we attempted to capture teachers’ productive building of ideas in both individual and group interviews while focusing less on discussions deviating from our intended main topics. All three authors were involved in identifying themes from the data, with extensive discussion of extracts and their meaning and fit with the theoretical framework.

4. Findings

In this section, we first report on teachers’ accounts of disruption as a result of attending the course, noting how their initial assumptions that it would provide them with missing skills and knowledge were replaced by surprising revelations about mathematics teaching, particularly the role and value of informal understanding. We note, too, teachers’ enthusiastic embrace of the course principles as a feature of discussion with colleagues. We then move to an analysis of the ways in which they reframed their problems of practice, paying particular attention to the distinction between problems that were deemed actionable and those that were not.

4.1. Initial Problems of Practice and Underlying Assumptions

Responding to the written questions sent by the mathematics teacher educators ahead of the semester start, teachers expressed goals for learning that ranged from a simple wish to learn something “new” without a specific motivation (“get tips about different teaching methods in mathematics”, Thea) to more complex motivations related to particular problems they saw either in themselves or in their interactions with students. Many initial problems of practice simply focused on becoming more confident and competent teachers, something to be achieved by enlarging their skillset with new techniques and example tasks:

I imagine the course can give me a robust knowledge base, together with specific didactical approaches, methods and activities […] I want a good balance between exploration and repetition/retrieval for each student. (Emma)

Jenny was very confident as a mathematics teacher and wanted the course to provide “confirmation that what I do is right” but also to equip her with “new tools” and up-to-date knowledge:

I also want the course to give me a clearer understanding on how to teach maths following the new curriculum, instead of my trying to interpret it on my own, without knowing if I understand it right or not.

A recurring problem of practice was helping everyone, an issue that teachers frequently framed in terms of students’ emotional experiences of success, enjoyment and interest:

[I want my lessons to be] motivating, inspiring. That all students, irrespective of their starting point, [should have] the experience of being successful and the opportunity to develop their curiosity about this subject and across subjects. (Anna)

I hope to be introduced to teaching methods and tools that can help me vary my classes, make students interested and help them experience a sense of being successful in maths. (Serena)

Linnea wanted help with a similar problem of student engagement, framed this time in terms of difficulty in connecting theory and practice:

How to inspire a whole class in mathematics […]. A practical lesson, going from theory to practice without creating chaos. The students being engaged but still listening to the teacher.

A similar framing saw student engagement as an issue of the apparent distance between school mathematics and real life; teachers wanted to help students “to see connections between the maths and the real world” (Jenny), the teachers’ role being “to make maths relevant for the students, so that they feel they need it later in life”. Nina also wanted to foster a positive relationship with mathematics through practical relevance:

I want students to experience maths as something concrete and practical. In an ideal world my classes would include more discussions that included all students and more practical tasks.

Nina’s mention of “more discussions” that “include all students” introduces another aspect of teachers’ framing of student needs. Several teachers focused on the ideal of classrooms driven by sense-making, where all students take ownership of the mathematics and are challenged, for example:

I want to have time and knowledge to meet each child where they are in their mathematical understanding and guide him to reach his full potential. I want a classroom where everyone has enough mental and emotional surplus to wonder together about puzzling mathematical observations through a well-developed mathematical language. (Iselin)

Often, however, this problem of practice was framed in accordance with two assumptions: students have a fixed ability in mathematics, and different students need different methods. Having raised her ideal of the inclusive discussion-based classroom, Nina admits that “I found it difficult to [make this happen], being alone with 28 students in mathematics—naturally on very different levels”. Solving this problem is a main aim for her:

I hope to expand my understanding of how to help students who struggle with maths, to get more practical approaches and strategies.

Mathilde and Klara adopt the language of levels to frame the problem and, hence, what they want from the course:

Give me more methods and techniques to use in my teaching so that each student gets help on his own level. ‘I don’t understand why someone doesn’t understand. (Mathilde)

I want to adapt my teaching to the levels in the classroom and not let the lack of skills hold students back from [solving the tasks]. (Klara)

Trude is even more specific in describing the strugglers and what they need:

The course should give an overview of good sources for alternative mathematics teaching, so that one can reach all students, also those who struggle with thinking logically.

Perceptions of students such as Trude’s are common [4]. One of the main aims of the course is to disturb such assumptions about student ability and related preconceptions about teaching and the nature of mathematics. In the following sections, we show how the course appeared to achieve this: in their exit interviews, the teachers recounted numerous destabilising experiences and new insights that caused them to rethink their approach to mathematics teaching, and their ideas about what students bring to a classroom and the role of informal ideas in mathematics.

4.2. Challenging Assumptions and Initial Problem Frames

In the exit interviews, teachers gave frequent examples of how their professional reasoning had been disrupted (e.g., “it was a revelation”, “before I used to … but now…”) by certain experiences of the course (the missions in groups, the gatherings, the research-based readings). Three such shifts were most prominent in the data: how teachers saw mathematics teaching, the role of students’ informal methods and the value of productive friction among colleagues. We discuss these in the following sections.

4.2.1. Seeing Mathematics Teaching Differently

Many of the teachers talked about the ways in which the course disrupted their thinking about mathematics teaching, causing them to reframe the issues around what is involved in doing mathematics and the role of how children think in learning. For many, this led to a new criticality of their former teacher-centred approaches and their own schooling. Typical were Gaute’s comments about the impact of the course’s teaching style, in which teacher participants were encouraged to engage with mathematical problems and explore their own informal strategies: “already at the first gathering—it was a very aha experience in how to talk and think maths”. He describes how the experience of the course gatherings was very different from his own education in mathematics, where

we drilled, and we memorised numbers, … I don’t remember … having a lot of mathematical conversations, or different methods.

Jenny describes the inquiry approach of the course as “mind blowing”, because it made her see mathematics differently. She also recognises how traditional schooling means that

you have never thought about doing it this way […] And all of a sudden, they [the course tutors] show that things can be done in a different way. I remember the first gathering, it was … mind blowing. Because they showed maths from a completely new angle.

This was a major revelation for her:

I lacked a sort of an overarching understanding, right? I didn’t see it [mathematics] from above, and [didn’t] understand how things are tied together, right? I lacked a connection between all the issues, together.

Hedvig’s “aha” experience focuses on her reframing of the potential range of student thinking and what this means for changing traditional teaching:

…I have had a lot of aha experiences in a way … Talking about the world’s biggest numbers and stuff like that … there’s no limit to how children think, then. And that you kind of have to use what the kids think. Don’t just follow the textbook.

Rikke has also noticed the emphasis of the course on understanding how children think and hinted that, combined with the disruption that the course “missions” brought to her habitual practice, this has improved her relationship with mathematics and thus her confidence as a teacher:

Even though they are young children, I notice that I’m not always confident in those teaching situations, and I want … to stay in [my old ways]. [Then I’m] more confident about it. And [now we are] finding out a bit more about how children think, and ways of thinking and… yes. The whole process then, not just drawing two lines below the answer [to highlight the final solution to a problem]. And I think it’s been really nice that [the course is] very focused on that.

She is struck by the way that “right answers” are not the point of the course gatherings:

it’s never scary to answer [here]. Because if you answer incorrectly here, you kind of don’t get “caught” for it. [The MTEs] are more interested in thought processes—how you think—rather than [saying] “That’s wrong”. And I think that’s a pretty nice thing to bring into the teaching as well.

Hedda echoes these ideas, highlighting a change in how she sees mathematics teaching in terms of no longer feeling that she ought to know everything: there are different ways of knowing, which the course has made clear:

what I notice has happened is … that now we know that students think in different ways … And I think that—I’m not so afraid of not knowing everything now… That it’s no big deal [that I haven’t] heard of that particular way of thinking before. Or if I can’t keep up with it.

4.2.2. Recognising the Value of Informal Understanding

These new perceptions of mathematics lead to a reframing of informal understanding as legitimate and valuable mathematics. Gaute reflects that although he used informal strategies when doing mathematics himself, “I don’t think it was a conscious part of my maths teaching”. The course has caused him to think consciously about the connection between informal methods and algorithms and its relevance to teaching:

we’ve been given tasks … and then we’ve had to drill into how we’re going to do it. Especially those on the division algorithm, how to set it up. I’ve never thought about how it’s based on equal sharing […] even when you just draw up when you want to divide something by three […] then you hand out, a thousand first, and then a hundred afterwards. I’ve never seen that before, and I haven’t connected: that’s what the algorithm actually does. So it was very much an eye-opener for me. That whole way of thinking about maths, especially when dealing with children.

Jenny also talked about how encountering two different additive models gave her tools to engage in her students’ thinking more deeply:

… what was the best, was that I understood the two principles, right? Linear model and grouping. And I knew which students worked within which model […] all of a sudden I had all the dots above the i-es … I understood how things connect, and I can work within [the model] that I know suits the students.

This stood in stark contrast to how she saw it before, where her attempts to do things differently had been too random:

When I worked with the students, I used to just try to show them what I thought would work for them. [I did this] without knowing what those things [linear model or grouping] are … It was at a very intuitive level. I tried to guess how to do it.

Less mathematically trained and confident teachers such as Solvor talked about how they had realised that “helping” students by teaching them algorithms, as they had themselves been taught, was pointless:

I’ve been thinking like that in terms of addition, you’ve thought that that algorithm—you want to teach them that quickly—because it’s so convenient, right? You think of it as a help for them. But then you see now that it’s counterproductive if you rush towards that algorithm.

Gina now realises that “It’s not the goal to get there as quickly as possible”:

But I didn’t think that before. Before, I just thought, to help them [with addition, you need to say]: ‘… Look here: Just put them one above the other. Then you add them up. Right? So easy and great and straightforward’. But it’s not easy and great and straightforward if you don’t realise what you’re doing.

Instead, she realises that it is important to capture the informal stages that underlie the algorithms. Hedvig also notes that the power of algorithms has created a kind of trap, which the course has challenged:

You just don’t have to get in there [using algorithms] as soon as possible, and that’s… the trap that I think maybe I have fallen into, not knowing that it was a trap. I’ve sort of said that; “Learn this method, and you’ll be fine”. But that’s it—the understanding is not in using algorithms.

She argues that this different way is more inclusive:

Allowing kids to think in different ways to come up with an answer [...] so that more kids get it. That’s the point. And then they will [get it]—when they realise what they are doing—no matter which way they have got there…

4.2.3. Embracing Productive Friction

All teachers celebrated these new ways of seeing mathematics and their students and their new abilities to argue about pedagogical judgements. Solvor feels that the course has enabled her to articulate why “what’s underneath” is so important for inclusion:

… maths teaching has perhaps been adapted to those who get things easily, quickly, like arithmetic. Whereas now, we’re learning to have a maths lesson that targets everyone. I think. There’s a difference, and especially that there are different solution paths, because I had a discussion last year, with the neighbour saying, “No, but you just have to memorise multiplication tables. Some things you have to memorise.” And then I was like, “No, you don’t have to.” But I couldn’t argue why. But now, I can. Now I say, “No, you can use [relational thinking]”.

Sofie is outspoken about the importance of teaching for understanding at her school:

When they’re talking about standard algorithms—I’ve become quite an opponent of it—and I don’t think it’s right either, but I’m questioning the maths teacher, like that; why do you do that, and…

She rebuffs responses that defend the top-down teaching of algorithms:

They think it’s important, so […] I lost the discussion the other day, because they think that you have to [do it], because you have to find an effective strategy eventually, anyway. So if the student doesn’t understand something, in order for them to move on, […] you should [do it]. At least you’ve given them a tool to do the maths when they get to middle school. … But I stood my ground: “No, they have to know why they do what they do!”

Hedda also disagrees with other teachers’ practices in her school now, describing a colleague as “old school” when he focused on setting up a standard algorithm rather than listening to the students’ strategies. She is aware that this is exactly what she would have done in the past:

And it was really such a good example of how I probably would have done it if I suddenly had to be a maths teacher before taking this course here. I wouldn’t have been interested in hearing about different ways, because I thought I’d show them a standard algorithm…. And then I wasn’t … didn’t really know that it’s totally [reasonable] to spend a lot of time hearing from different students how they think … And that it’s really just as important [for the students] as continuing to calculate in your book one by one.

Vilde comments on the difficulty of talking to colleagues about what she sees as bad mathematics teaching, yet she is confident that, at some point, she will confront them and open the matter up for discussion: “I think that I can’t keep my mouth shut forever. I’m going to have to confront, or be curious and ask: ‘Why are you doing that?’”

4.3. Reframing Problems of Practice

As the previous section showed, the teachers developed strong positions on what kind of teaching they felt was right—fostering student understanding, promoting discussion and explanation, avoiding algorithmic teaching and recognising varied student contributions. Embracing these principles and their importance in equitable mathematics teaching led to new problems of practice: teachers were concerned with exploring the details of how they could put them into practice. These new problems of practice were not, however, always seen as actionable, and teachers struggled individually and jointly with these problems.

4.3.1. Refining Pedagogical Judgements—How Do We Make This Happen?

The teachers reflected on a number of problems in the classroom as they worked on how to incorporate the course principles into their daily practices, ranging from the need to elicit and understand students’ thinking, to refining questioning and discussion and promoting new classroom cultures, to grappling with problems of mixed class teaching and student progress.

A number of the teachers commented on the need to prepare carefully for lessons, because, as Sofie says, “now we want [to see] much more into the head of the student”, and this means being armed with strategies that are new to them, as Rikke points out:

What do you want to know and why? What kind of follow-up questions should be asked of students when they are stuck? […] It shouldn’t be the right answer, but how did you think? How did you arrive at the answer?… Can you prove it?… In other words, the good questions.

Mathilde frames her particular problem of practice in terms of needing to think about what the students know and how to incorporate that, given that mathematics is more than right or wrong answers:

… first of all, I have to—in preparation have a bit more focus on the students’ strengths and skills and knowledge—in order to be able to facilitate. … And then I have to prepare myself for how to start a conversation like that with them. And I have to be aware of what might come up. I have to be a little bit more ahead of the curve than before.

Gaute comments that he has become more skilled at asking good questions in classroom discussions in order to elicit student thinking, but he struggles to throw off his embedded ways of dealing with mathematics and the resulting desire to present the “easiest” method:

I’m definitely going to be better at asking for justification from students as to why that is. Maybe say: “Yes—can you show us on the blackboard? Has anyone else solved it any other way?” [But] I’d probably feel … a desire to give an easier method. If someone had drawn it, and sort of done it that way … there’s a voice that says “actually, you can skip this, and just… do like that. Then you’ve halved your workload”.

Gaute questions when it is appropriate to stop his students’ exploration and say, “This is a very, very good way to do it”, wondering how one can do this “without somehow overriding [the students’] understanding and exploration”. He struggles with striking a balance in terms of being “open to other ways of thinking, or the child’s, the student’s way of doing it, without in a way encouraging strategies that are really very convoluted”. For Gaute, the challenge is “daring” to let students sit longer, daring to give more open tasks and “giving students room to explore, to try and fail … Now you are free”. He is particularly exercised by issues of summative assessment:

It’s a bit more demanding to … get a summative assessment—at the end. … when do you know that you have actually learned what you are supposed to? And when have you got … when have the students got the strategies they need to do it effectively then? You don’t always have the time and opportunity to make a drawing to solve something you can actually do in a very simple and straightforward way. When do you say that understanding is kind of good enough, and you can give a bit more… tools based on recipes. Or algorithms?

Hedvig ruminates on similar issues but focuses on the pressure of time in forcing decisions:

How long do you stay, and dwell on the same topic, and task. When does it stop giving something more? And we very rarely have the time to stay very long with fractions. No, we have to move on because we’re going to have something else, …. When have the kids learned it? Or when have they learned enough…

Mathilde focuses on a related issue, which is getting students to change their expectations about how they should participate in class:

I think it’s difficult because the students are very used to the fact that there is either a right or wrong answer. … I noticed that the last time I was doing geometry that they’re sort of not used to that [new] way, so they get very silent and quiet. Because they probably expect me to be looking for the right or wrong answer. So it’s a challenge, and then it’s challenging to be able to drive the conversation forward, when they stop or don’t say anything. Getting them involved, it’s been challenging.

4.3.2. Refining Pedagogical Judgements: Can We Make This Happen?

Some teachers framed problems of practice in terms of student characteristics or contextual constraints which caused them to perceive problems as inactionable. Some talked about their struggle to sustain and act on the idea that all students can benefit from their new strategies. Hedvig finds it hard to adjust her framing of the issues, since she sees the “nurture group” students that she teaches as unable to maintain engagement in a mathematical problem:

[I have] children who have quite a short attention … span. Which may only be a short time with a mathematical problem. If it gets too difficult, we have to … [simplify the problem] or do something else. And I quickly resort to games.

She is keen to teach in mixed whole classes, where she can draw on the stronger students to help the others and reduce teacher authority at the same time:

I kind of want to try it out, because there are some strong kids in one class, and there are quite a few who have to hang on and need some help. So you can use the strong mathematical brains to explain to the others. Because sometimes it’s a bit like the teacher is standing and talking, and then they’re going to do some tasks. So I kind of want to try to distribute some responsibility, around to the kids themselves.

Trude does not see this as a solution to the problem of how to include weaker students, which she sees as basically inactionable. She struggles to act on the course message in favour of mixed teaching and has decided to put students into homogeneous groups. She believes that all students benefit from investigative activities but argues that the stronger students (she struggles to find the vocabulary to describe them) will be held back otherwise:

obviously, I could let the […] brightest, or call it what you will, help those who were less so. But, my experience is that the ones who had come a long way had more fun. Because they got farther. They sort of got it, and that’s a group of students who get short-changed in regular maths lessons. [Because] they are already there, and don’t get enough challenges, and think maths is getting boring, and they’re the ones who... there’s hope they may move forward [in the future] with maths.

While Trude’s hesitation about putting a principle into operation was clearly connected to one specific instance, Rita is more generally sceptical of teaching through inquiry. She tries to work out how the teacher and student roles have changed:

Whereas before I would have presented a few alternative methods, more … unambiguous strategies, as an adult now you have to hide it more. So that they get to think about it. Justifying is nothing new, nor is understanding. The parts about formulating how they would solve it, and the part about figuring out things themselves are new.

This analysis leads her to conclude that the approach disadvantages “the weakest students” because the teacher has to be “secretive”, and these students cannot then participate without help, which descends into mere funnelling. This problem of practice thus appears inactionable to her. Echoing many of the teachers’ earlier problem framing, she holds on to the assumption that struggling students need a different type of mathematics teaching, and she questions the principles:

Even if they say that maybe the weak benefit as well—you have some that just don’t figure out any […] way to solve it. You can keep hinting […] and keep going one step down […] When can you stop letting the child [laughs] figure it out? It’s silly, it will just confuse the student who won’t get anything out of it. Say—how do you use the numberline? Or find a correct answer. Because there will be a lot of guessing and trial and error and [they] still [won’t] figure it out.

Rita and Ulf, who were by chance interviewed together, both work with immigrants who recently arrived in Norway, teaching “welcome classes” at the primary school level: Norwegian education policy stipulates that all children entering the country should be prepared for integration into mainstream classes at their own age level within two years, regardless of their circumstances and prior language/educational achievements. Rita and Ulf’s job is to enable students to cope with Norwegian as a language for learning, as well as ensure that they are up to speed with the mathematics curriculum level appropriate for the school grade that they will eventually end up in. These twin aims create dilemmas for what to prioritise in their limited time with these students. Understanding Rita’s situation, Ulf attempts to reframe her problem by contextualising it in terms of Norwegian education policy (“It’s the eternal problem of adapted education, isn’t it? Cater for everyone”, Ulf) and, therefore, highlighting that it is difficult to work out in its generality. But she rejects the premise that all students can benefit from time for inquiry, acknowledging that her claim is controversial (“I am throwing a lit torch”):

Still, … I am throwing a lit torch here, but for exactly these students, trying to let them figure things out even though they can’t just … come up with mathematical ideas, “Oh, I can do it this way!” and give them so much time, too, and maybe still not come up with anything anyway. It’s a pity, I think. That they can’t figure it out. But in the end they have to just learn to add two-digit numbers, if they are sixth-graders. And so I’d end up having to just show them how to do it on the numberline.

Her position that inquiry is not suitable for everyone is challenged by Ulf. He sees the students as empowered by the approach rather than being left to struggle alone (“It’s not just about right and wrong [...] Students are taken more seriously nowadays, [...] Before it was the teacher who stood at the board”, Ulf) and envisages the teacher’s role extending beyond individual interactions:

I agree very strongly [about the problems] with Rita. But I also see that there are […] students who find things difficult and then find them easier once they see the strategies of their classmates. And they are no longer afraid to go and share their thoughts because [they see it’s not about] right or wrong.

The disagreement was not resolved during the interview, but Rita is open to discussion and admits that she falls short of arguments. She is able to list numerous arguments supporting the value of mathematical processes for children’s education, yet questions their value for her students’ learning:

It’s a good thing, and a useful thing. They learn to argue and to formulate [their thoughts]. And dare to stand up and say something. And try to convince others. And think outside the box. Creativity. There are many good things and I have no really strong argument for not using it. The only thing is that it takes time that needs to be taken from something else. […] And it’s a class for learning the language in at most two years. And even if I know it helps with their language development, I still have to take the time from something. And it doesn’t always work.

Although the disagreement between Rita and Ulf is not productive, in the sense that it does not impact Rita’s framing of the problem, it is one of several examples that speaks to the typical interactions in the course where different points of view are discussed. We return to the role of inactionable problems as sites of learning and productive friction in the discussion.

5. Discussion

This article contributes to ongoing efforts to understand teacher learning from a situational perspective [15,16]. Understanding learning as the development of pedagogical judgement [14,15], we have focused here on equity in the sense of catering to all students, the aspiration shared by participating teachers at the start of the course. However, our findings show that the course played a part in the significant reframing of teachers’ problems of practice, from seeking to acquire “best practice” for children “at their own level” to seeking to understand and build on children’s informal and productive mathematical ideas within the inevitable tensions of their own particular contexts.

Addressing Research Question 1—How did teachers’ problems of practice change during the course?—we identified a shift in their expectations that the course would simply “plug the gap” in their skills and knowledge (see Section 4.1) to recognising the importance of drilling down into their classrooms and considering multiple avenues for addressing the problem (see Section 4.3). These expectations were challenged by their experience of the course, as teachers describe in Section 4.2. Looking back on the year, they attributed their learning to disruption, rather than just new knowledge and skills. They described “aha” moments that were prompted by their participation as learners in the gatherings, their participation in making sense of student ideas during gatherings and in interaction with real students, and their participation in argumentation and productive friction with other teachers on the course. They remarked on the new experience of mathematical discussion, the irrelevance of “right” and “wrong” and the revelation of the value of informal thinking, and their new compulsion to reject standard algorithms as frequently excluding some children and thus to press “old school” colleagues for justification of their practice. We thus found that at the end of the course, teachers had embraced the principles of the course as global aims for their teaching. More importantly, these global aims raised many new questions, leading them to reframe their problems of practice. Our teachers voiced the struggles that were associated with this contextualisation; since the course had required them to experiment in their own classrooms, they were able to raise new, actionable questions on how to cope with specific problems and why: for example, Gaute questioned how he could judge when students had a “sufficient” understanding for him to be able to move on in a lesson, and Trude hesitated when forming groups, as she felt pulled between her ethical responsibilities of organisational inclusion (mixed groups) and realising individual potential.

The shift in teachers’ expectations of what might be useful ways of dealing with problems of practice is significant, as it recognises teacher learning as an ongoing recontextualisation in complex situations, rather than the addition of new knowledge ([15] p. 147). This interpretation opens up sustainable opportunities to learn in teachers’ own professional communities, moving past the typical sharing of teaching ideas, provided of course that their colleagues have the same stance. This prerequisite is difficult to meet; teachers may distrust the value of probing colleagues’ professional reasoning [3,15] or hesitate to voice alternatives to the main discourse of their community, especially when in a position with less power [27]. Our analysis of the changes reported by our teachers and the links that they make with particularities of the course led us to identify disruption as key. This is precisely Silje’s intention in her course design, which aims to confront teachers’ pre-existing assumptions [24] rather than relying mainly on the more common approach of fostering reflection and smoothly coordinating new ways of thinking and acting when intervening in professional practice [28].

Turning to Research Question 2—How does teachers’ framing of problems of practice relate to the development of pedagogical judgement?—we found that teachers drew enthusiastically on the general principles of the course, but, despite their criticism of colleagues, they did not see these principles as “rules” for best practice. Rather, they articulated the difficulties that they experienced in putting them into practice, emphasising in the interviews the new questions that arose for them. As we have seen in Section 4.3, some problems of practice were seen as clearly actionable, but addressing them was not straightforward: the teachers assumed that all claims about classrooms are subject to multiple interpretations, and that actions need to be justified within their particular context. However, some problems were seen as inactionable; Rita struggles with how to fit in practices that she agrees are beneficial but that take time away from the major goal of preparing students for mainstream school in a time frame that she has no control over. Despite her adherence to the pedagogical reasoning behind the core course principle of valuing inquiry learning for all students, Rita’s struggle with the mismatch problem identified by Horn [4], which makes her doubt the capacity of her students for inquiry mathematics, ultimately seems to lead to an assumption that her particular problem of practice is inactionable. However, her learning in the course appears to have led to a willingness and expectation to argue for her position, as we can see in her interaction with Ulf, as well as in the multiple voices she uses (“I don’t have any good arguments”, “I am throwing a lit torch…”). Rita positions herself as willing to engage in productive friction with colleagues, and this plays a part in the development of pedagogic judgement.

As we have argued in the literature review, teachers need to incorporate principles for equitable teaching into their own practice: they need to recontextualise the course content in their own settings [2,3]. In terms of Horn and Garner’s [15] model (Figure 1), not only do teachers need to develop new pedagogical actions, but these need to be connected to pedagogical reasoning and tuned to local pedagogical responsibilities. As Goldsmith et al. [11] argue, the important issue for studies on teacher learning is not to show that learning happened, but to shed light on how. Building on our findings with respect to Research Question 1, we can see evidence in the teachers’ narratives for four possible mechanisms that enriched their pedagogical reasoning and its bonds to pedagogic action and pedagogic responsibility:

• Disrupting previously held assumptions;
• Holding the expectation that principles for, and actions in, teaching are justified;
• Habitually unpacking classroom experimentation into actionable problems of practice that may well require the mitigation of conflicting priorities rather than the enactment of “best practices”;
• Embracing productive friction with colleagues.

These observations return us to our argument that teacher learning is not necessarily evidenced by a change in teacher actions. We see the main learning achieved by our teachers as being the pedagogical reasoning that they clearly appreciate: they see it as confirming what they do and providing a basis for going forward. Further than this, though, the problems of practice that are presented by their local contexts provide opportunities for the exercise of reasoning, which we can understand as sustaining learning rather than limiting it. Learning is not about enacting a practice perfectly but about experimenting: principles sometimes come into conflict, and principles do not always trump all other considerations. This is perhaps best summed up by Trude’s observation that orchestrating lessons where students are at the core is a principle that is easy to adhere to but difficult to realise. It requires focus and practice:

[This is] essential for me, because it is about such an extreme presence and awareness as you stand there and [words] fall out of your mouth. What [words] do you say and how do students […] then understand the mathematics? Being so aware, so present when twenty-five students [buzz] and I think about [teaching English next, and about them washing their hands for lunch] and you have such a short time to engage in these processes. So it’s this extreme form of being present, when you must ask the questions that make students think mathematically and so on. I need to practice, and I don’t know who to practice on. We barely have time to do that. So it’s something I have to focus on.

6. Implications for Future Research

In this article, we have explored the nature of teacher learning from the point of view of the development of pedagogic judgement as the product of relationships between the pedagogic actions and reasons promoted by professional development and local institutional ethics and responsibilities. We have suggested that the four mechanisms identified above are central to the development of pedagogical judgement in a complex system such as mathematics education. We see the disruptive nature of the course described in this article as a key element in teachers’ development and productive friction as a means of sustaining learning beyond the course.

Future research and PD development need to explore these mechanisms further. While this article focuses on the entry and exit data, further analysis will turn to data gathered during the course to develop insight into how this professional development promoted the mechanisms listed above. In particular, we believe that the structure of the assignments sending our teachers on “missions” in the classroom, requiring them to discuss and report in groups, even when they experimented in different school contexts, together with feedback on their reports from the MTEs, impacted teacher development at each stage. Such exploration can contribute to ongoing efforts to understand teachers’ learning in context and the design of experiences that support such learning, such as Japanese Lesson Study adaptations around the world [29], video-formative feedback cycles [15] or the Australian Learning from Lessons project [30].