Using the Van Hiele Theory to Explain Pre-Service Teachers’ Understanding of Similarity in Euclidean Geometry
Abstract
:1. Introduction
2. Literature Review
2.1. Geometry
2.2. Similarity and Proof
2.3. Errors and Misconceptions as Part of the Learning Process
2.4. The Van Hiele Model: Levels of Geometric Thought
3. Research Methodology
3.1. Research Approach and Sampling
3.2. Collection and Analysis of Data
4. Results and Discussion
4.1. Overall Performance across the Items
4.1.1. Least Challenging Items
Item 1.2
Item 3.2
Item 3.3
4.1.2. Most Challenging Items
Item 1.4
Items 2.4 and 2.5
4.1.3. General Insights about the Overall Performance
4.2. Misconceptions Associated with Particular VH Levels
4.2.1. Misconception That Similarity Applies to Triangles Only
4.2.2. Misconception That All Rectangles Are Similar to One Another
4.2.3. Misconception about Naming Figures in a Similarity Relationship
4.2.4. Misconception about Class Inclusion of Quadrilaterals
4.2.5. Misconceptions Related to Visual Appearance and Orientation of Diagrams in Proofs
5. Conclusions
6. Limitations
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Alex, J.K.; Mammen, K.J. An assessment of the readiness of grade 10 students for geometry in the context of curriculum and assessment policy statement (CAPS) expectations. Int. J. Educ. Sci. 2014, 7, 29–39. [Google Scholar]
- Bowie, L. What Is Mathematics Paper 3 for? Marang News; Marang Centre for Mathematics and Science Education: Johannesburg, South Africa, 2009. [Google Scholar]
- Van Putten, S.; Stols, G.; Howie, S.J. Making Euclidean geometry compulsory: Are we prepared? Perspect. Educ. 2010, 28, 22–31. [Google Scholar]
- Ndlovu, M.C. Re-envisioning the scholarship of engagement: Lessons from a university school partnership project for mathematics and science teaching. S. Afr. J. High. Educ. 2011, 25, 1397–1415. [Google Scholar]
- Atebe, H.U.; Schäfer, M. The face of geometry instruction and learning opportunities in selected Nigerian and South African high schools. In Proceedings of the 17th Annual Conference of the Southern African Association for Research in Mathematics, Science and Technology Education, Grahamstown, South Africa, 19–22 January 2009; Schäfer, M., McNamara, C., Eds.; SAARMSTE: Grahamstown, South Africa, 2010; pp. 7–21. [Google Scholar]
- Department of Basic Education (DBE). Curriculum and Assessment Policy Statement (CAPS) FET Band Mathematics Grades 10–12; Government Printers: Pretoria, South Africa, 2011.
- Haj-Yahya, A. Students’ conceptions of the definitions of congruent and similar triangles. Int. J. Math. Educ. Sci. Technol. 2022, 53, 2703–2727. [Google Scholar] [CrossRef]
- Amaral, R.B.; Hollebrands, K. An analysis of context-based similarity tasks in textbooks from Brazil and the United States. Int. J. Math. Educ. Sci. Technol. 2017, 48, 1166–1184. [Google Scholar] [CrossRef]
- Ubah, I.; Bansilal, S. The use of semiotic representations in reasoning about similar triangles in Euclidean geometry. Pythagoras 2019, 40, a480. [Google Scholar] [CrossRef]
- Bassarear, T. Mathematics for Elementary School Teachers, 5th ed.; Brooks /Cole: Pacific Grove, CA, USA, 2012. [Google Scholar]
- Knight, K.C. An Investigation into the Change in the Van Hiele Level of Understanding Geometry of Pre-Service Elementary and Secondary Mathematics Teachers. Master’s Thesis, University of Maine, Orono, ME, USA, 2006. Unpublished. [Google Scholar]
- De Villiers, M. Using dynamic geometry to expand mathematics teachers’ understanding of proof. Int. J. Math. Educ. Sci. Technol. 2004, 35, 703–724. [Google Scholar] [CrossRef]
- Ngirishi, H.; Bansilal, S. An exploration of high school learners’ understanding of geometric concepts. Probl. Educ. 21st Century 2019, 77, 82–96. [Google Scholar] [CrossRef]
- Lee, J.S.; Ginsburg, H.P. Early childhood teachers’ misconceptions about mathematics education for young children in the United States. Australas. J. Early Child. 2009, 34, 37–45. [Google Scholar] [CrossRef]
- Patkin, D.; Levenberg, I. Geometry from the world around us. Learn. Teach. Math. 2012, 13, 14–18. [Google Scholar]
- Cunningham, R.F.; Roberts, A. Reducing the Mismatch of Geometry Concept Definitions and Concept Images Held by Pre-Service Teachers. Issues in the Undergraduate Mathematics Preparation of School Teachers 2010, 1. Available online: http://https://eric.ed.gov/?id=EJ914254 (accessed on 24 July 2023).
- Mudaly, V. Pre-service teachers’ understanding of the concept of proof. Int. Sci. Res. J. 2016, 72, 137–158. [Google Scholar] [CrossRef]
- Mudaly, V.; De Villiers, M. Mathematical modelling and proof. In Proceedings of the 10th Annual National Congress of the Association for Mathematics Education of South Africa, University of the North West, Potchefstroom, South Africa, 30 June–4 July 2004. [Google Scholar]
- Msomi, A.M.; Bansilal, S. Analysis of students’ errors and misconceptions in solving linear ordinary differential equations using the method of Laplace transform. Int. Electron. J. Math. Educ. 2022, 17, em0670. [Google Scholar] [CrossRef] [PubMed]
- Mutambara, L.H.N.; Bansilal, S. An exploratory study on the understanding of the vector subspace concept. Afr. J. Res. Math. Sci. Technol. Educ. 2019, 1, 1–13. [Google Scholar] [CrossRef]
- Lannin, J.K.; Barker, D.D.; Townsend, B.E. How students view the general nature of their errors. Educ. Stud. Math. 2007, 66, 43–59. [Google Scholar] [CrossRef]
- Smith JP di Sessa, A.A.; Roschelle, J. Misconceptions reconceived: A constructivist analysis of knowledge in transition. J. Learn. Sci 1993, 3, 115–163. [Google Scholar] [CrossRef]
- Crowley, M.L. The van Hiele model of the development of geometric thought. In Learning and Teaching Geometry; Linquist, M.M., Shalte, A.P., Eds.; National Council of Teachers of Mathematics: Reston, VA, USA, 1987; Volume K-12, pp. 1–16. [Google Scholar]
- Jaime, A.; Gutierrez, A. Guidelines for teaching plane isometries in secondary school. Math. Teach. 1995, 88, 591–597. [Google Scholar] [CrossRef]
- Feza, N.; Webb, P. Assessment standards, Van Heile levels and grade seven learners understanding of Geometry. Pythagoras 2005, 62, 36–47. [Google Scholar]
- Clements, D.H. Teaching and Learning Geometry. In A Research Companion to Principles and Standards for School Mathematics; Kilpatrick, J., Martin, W.G., Schifter, D., Eds.; National Council of Teachers of Mathematics: Reston, VA, USA, 2003; pp. 15–78. [Google Scholar]
- Department of Basic Education (DBE). 2018 Examination Report; Department of Basic Education: Pretoria, South Africa, 2019.
- Bansilal, S. An exploration of students’ conversions from a symbolic to a verbal representation. J. Commun. 2015, 6, 38–47. [Google Scholar] [CrossRef]
- Zaslavsky, O.; Ron, G. Students’ understandings of the role of counterexamples. In Proceedings of the 22nd Conference of the International Group for the Psychology of Mathematics Education, Stellenbosch, South Africa, 12–17 July 1998; Olivier, A., Newstead, K., Olivier, A., Newstead, K., Eds.; PME: Stellenbosch, South Africa, 1998; Volume 4, pp. 225–232. [Google Scholar]
- Bansilal, S.; Brijlall, D.; Trigueros, M. An APOS study on pre-service teachers’ understanding of injections and surjections. J. Math. Behav. 2017, 48, 22–37. [Google Scholar] [CrossRef]
- Sinclair, N.; Bussi, M.G.B.; De Villiers, M.; Jones, K.; Kortenkamp, U.; Leung, A.; Owens, K. Recent research on geometry education: An ICME-13 survey team report. ZDM Int. J. Math. Educ. 2016, 48, 691–719. [Google Scholar] [CrossRef]
- Gal, H.; Linchevski, L. To see or not to see: Analyzing difficulties in geometry from the perspective of visual perception. Educ. Stud. Math. 2010, 74, 163–183. [Google Scholar] [CrossRef]
- Ndlovu, B. Exploring Pre-Service Teacher’s Knowledge of Proof in Geometry. A Dissertation Submitted in Partial Fulfilment of the Requirements for the Degree of Master of Education; University of KwaZulu-Natal: Durban, South Africa, 2012. [Google Scholar]
- Hasenbank, J.F. The Effects of a Framework for Procedural Understanding on College Algebra Students’ Procedural Skill and Understanding. Master’s Thesis, Montana State University, Bozeman, MT, USA, 2006. Unpublished. [Google Scholar]
- Jojo, Z. Students’ Understanding of the Chain Rule. Master’s Thesis, University of KwaZulu Natal, Durban, South Africa, 2010. Unpublished. [Google Scholar]
- Dhlamini, S.S. An Investigation into Grade 12 Teachers’ Understanding of Euclidean Geometry. Master’s Thesis, University of KwaZulu Natal, Durban, South Africa, 2012. Unpublished. [Google Scholar]
- Turnuklu, E.; Akkas, E.N.; Gundogdu-Alayli, F. Mathematics teachers’ perceptions of quadrilaterals and understanding the inclusion relations. In Proceedings of the Eighth Congress of European Research In Mathematics Education (CERME 8), Antalya, Turkey, 1 August 2012. [Google Scholar]
- Zeybek, Z. Understanding inclusion relations between quadrilaterals. Int. J. Res. Educ. Sci. (IJRES) 2018, 4, 595–612. [Google Scholar] [CrossRef]
- Ozdemir, E.E.; Dur, Z. Preservice Mathematics Teachers’ Personal Figural Concepts and Classifications About Quadrilaterals. Aust. J. Teach. Educ. 2014, 39, 107–133. [Google Scholar] [CrossRef]
- Zilkova, K. Misconceptions in pre-service primary education teachers about quadrilaterals. J. Educ. Psychol. Soc. Sci. 2015, 3, 30–37. [Google Scholar]
- Fujita, T. Learners’ level of understanding of the inclusion relations of quadrilaterals and prototype phenomenon. J. Math. Behav. 2012, 3, 60–72. [Google Scholar] [CrossRef]
- Stols, G.; Long, C.; Dunne, T. An application of the Rasch measurement theory to an assessment of geometric thinking levels. Afr. J. Res. Math. Sci. Technol. Educ. 2015, 19, 69–81. [Google Scholar] [CrossRef]


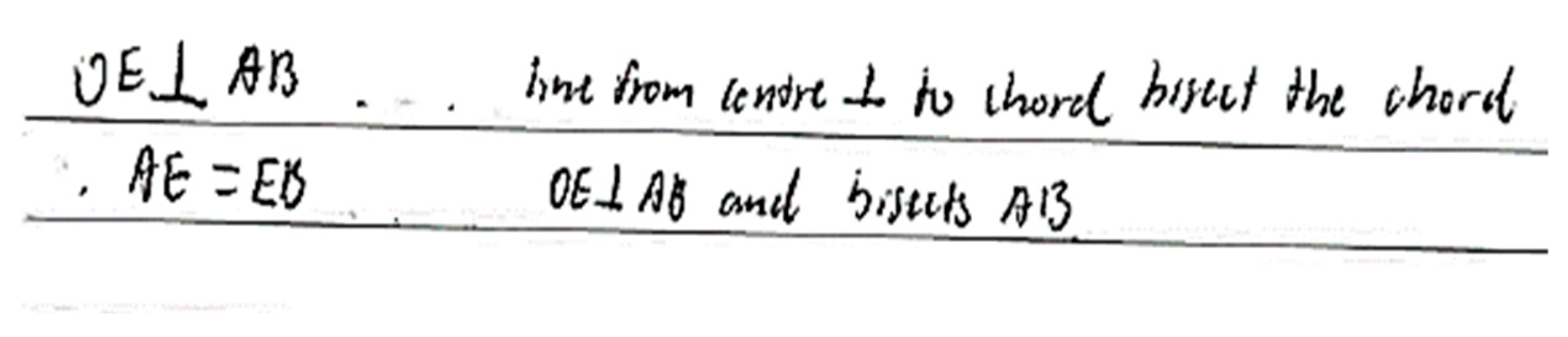







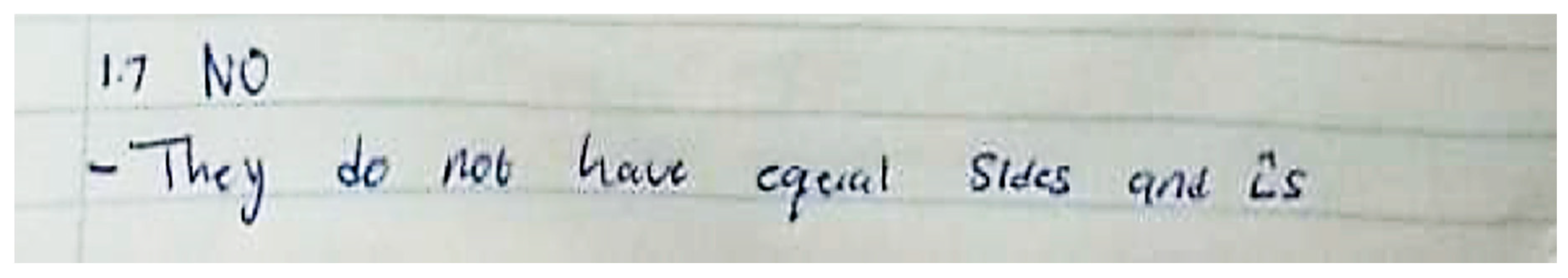
| Number | Item | Targeted VH Level | % Correct | |
|---|---|---|---|---|
| Question One | ||||
| 1.1 | Explain the meaning of similarity in mathematics as the best you can. | L2 Know similarity property | 85 | |
| 1.2 | State the conditions required to show that two polygons are similar. | L2 Know similarity property | 88 | |
| 1.3 | Given any two arbitrary squares of different sizes, are they similar or not? Explain | L3 can use informal deduction to see that properties of similarity hold | 82 | |
| 1.4 | Given any two arbitrary rectangles of different sizes, are they similar or not? Explain | L4 Can understand if the minimum conditions for similarity are not met—corresponding sides are not always in proportion | 0 | |
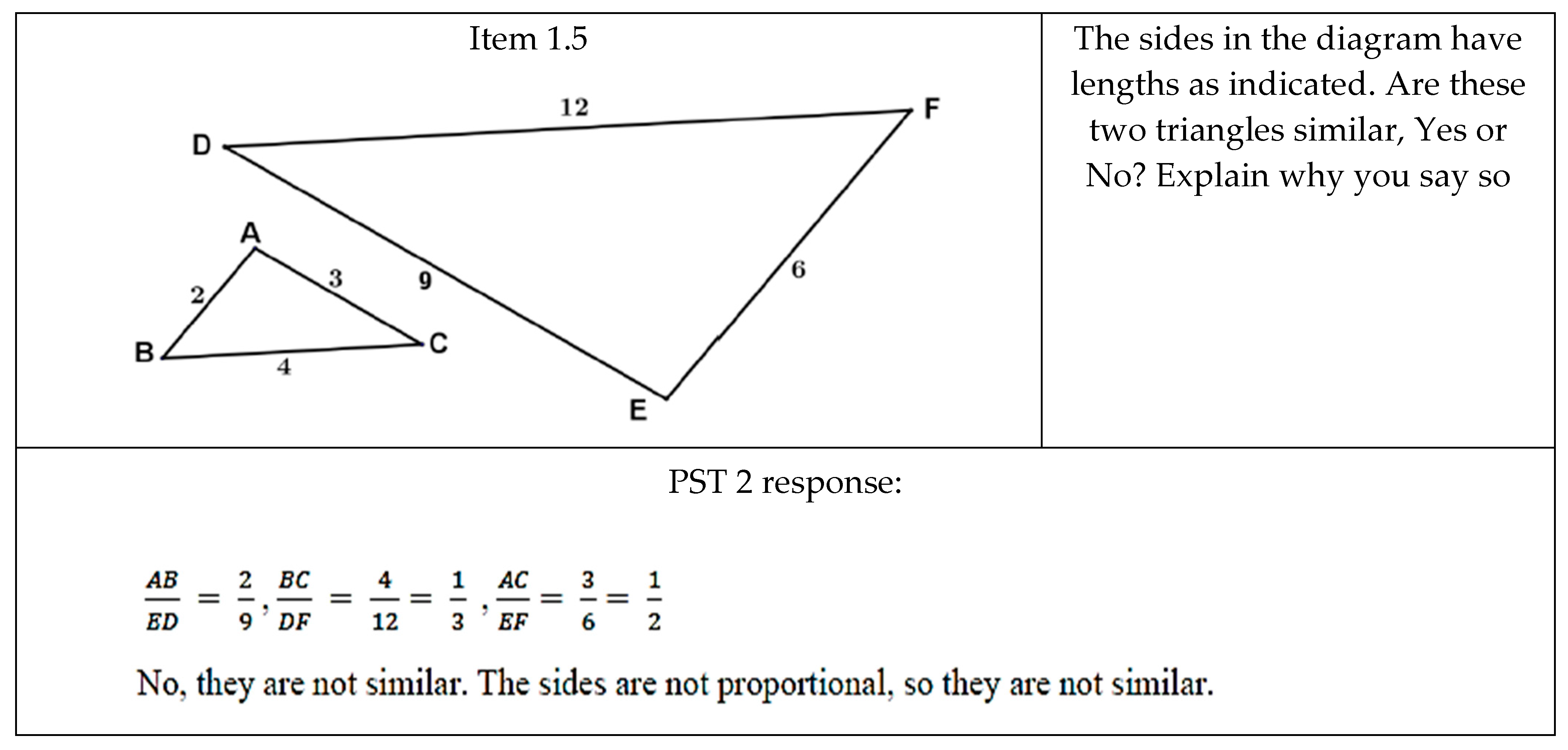
| 1.5 | The sides in the diagram have lengths as indicated. Are these two triangles similar? Explain why you say so. | 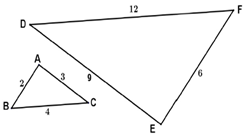 | L3 can use calculations to establish that corresponding sides are in proportion | 62 |
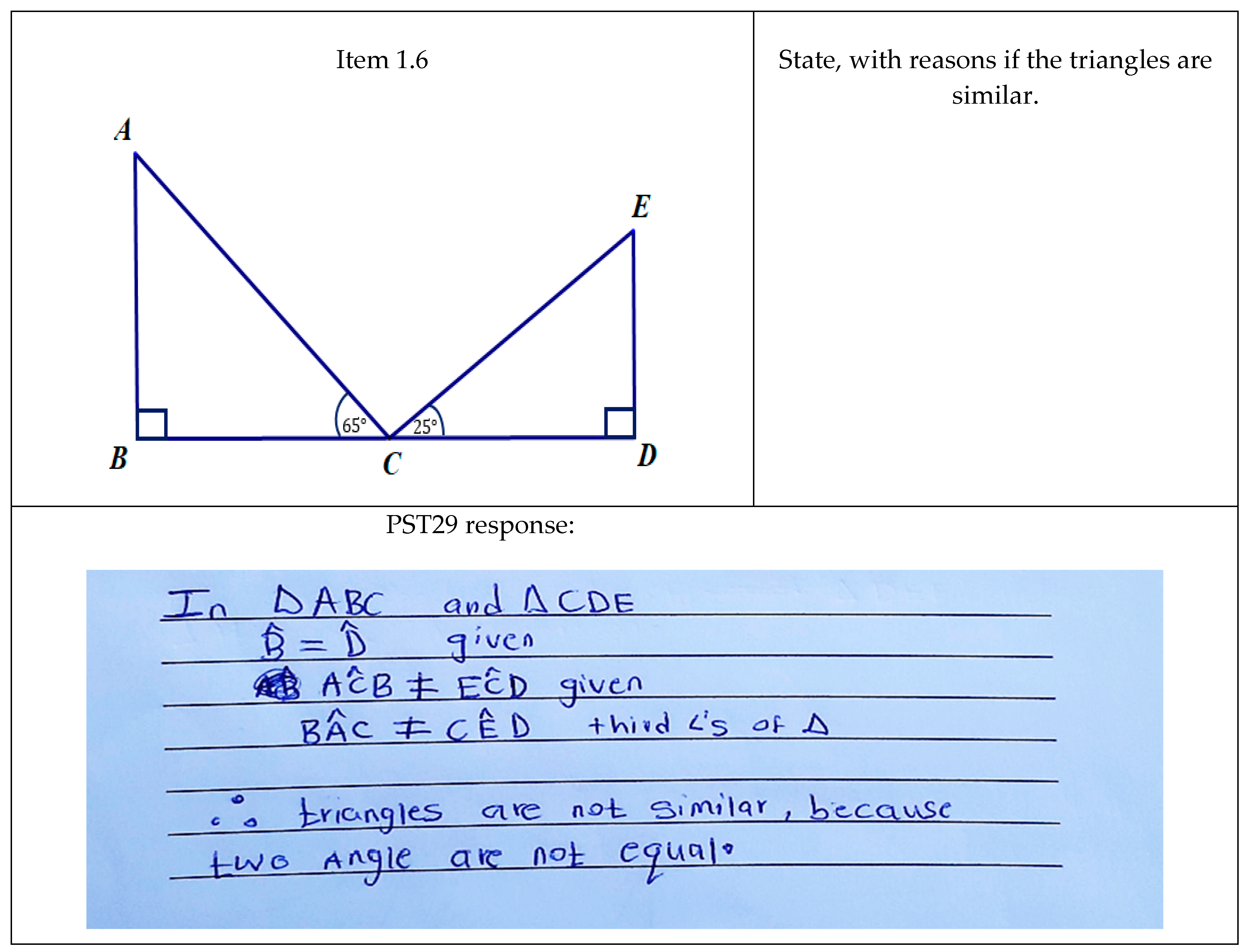
| 1.6 | State with reason whether or not the following triangles are similar. | 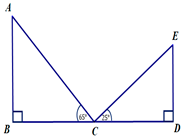 | L2 can see that triangles are equiangular | 76 |
| Question Two For each diagram below, write down a triangle similar to the given triangle. Naming must be in the correct order: | ||||
| 2.1 | PQ is a tangent to the circle at Q. ∆BQP///∆__ | 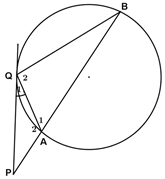 | L3 Can use informal deduction to identify the equal corresponding angles and, thus, order of naming | 62 |
| 2.2 | ∆PRS /// ∆______ | 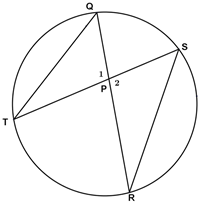 | L3 Can use informal deduction to identify the equal corresponding angles and, thus, order of naming | 71 |
| 2.3 | ∆ABE /// ∆______ | 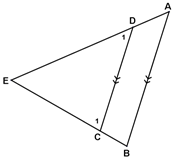 | L3 Can use informal deduction to identify the equal corresponding angles and, thus, order of naming | 74 |
| 2.4 (2.5) | ∆PQR /// ∆______ /// ∆______ |  | L3 (L3) Can use informal deduction to identify the equal corresponding angles and, thus, the correct order of naming, | 56 (53) |
| Question Three. Two circles touch each other at point A. The smaller circles pass through O, the centre of the larger circle. Point E is on the circumference of the smaller circle. A, D, B and C are points on the circumference of the larger circle. OE//CA. | ||||
| 3.1 | Prove, with reasons that AE = BE | 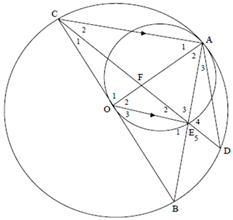 | L4 Can devise a formal proof using previous theorems | 59 |
| 3.2 | Show that | L3 Can use informal deduction to show that the corresponding angles according to the naming order are equal | 91 | |
| 3.3 | L3 can use informal deduction using the results in 3.2 and 3.3 to reach the required result | 97 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mbatha, M.; Bansilal, S. Using the Van Hiele Theory to Explain Pre-Service Teachers’ Understanding of Similarity in Euclidean Geometry. Educ. Sci. 2023, 13, 861. https://doi.org/10.3390/educsci13090861
Mbatha M, Bansilal S. Using the Van Hiele Theory to Explain Pre-Service Teachers’ Understanding of Similarity in Euclidean Geometry. Education Sciences. 2023; 13(9):861. https://doi.org/10.3390/educsci13090861
Chicago/Turabian StyleMbatha, Mduduzi, and Sarah Bansilal. 2023. "Using the Van Hiele Theory to Explain Pre-Service Teachers’ Understanding of Similarity in Euclidean Geometry" Education Sciences 13, no. 9: 861. https://doi.org/10.3390/educsci13090861
APA StyleMbatha, M., & Bansilal, S. (2023). Using the Van Hiele Theory to Explain Pre-Service Teachers’ Understanding of Similarity in Euclidean Geometry. Education Sciences, 13(9), 861. https://doi.org/10.3390/educsci13090861






