Investigating the Principle of Relativity and the Principle of Equivalence in Classical Mechanics: Design and Evaluation of a Teaching–Learning Sequence Based on Experiments and Simulations
Abstract
1. Introduction
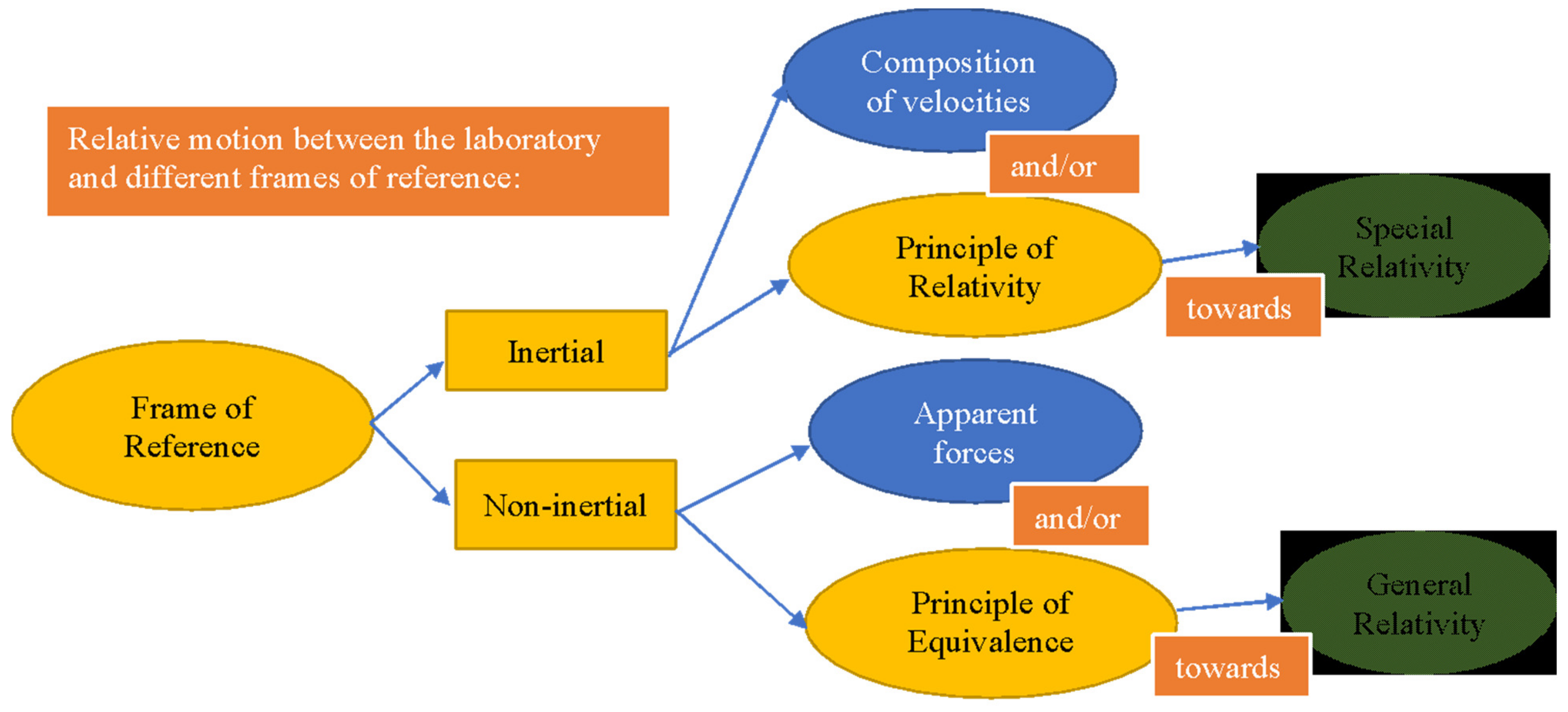
2. Theoretical and Methodological Framework
2.1. The Content Structure for Instruction
- (i)
- Analysis of science content (analysis from the perspective of theory),
- (ii)
- Analysis of students’ difficulties and assessment of the crucial features of students’ learning processes in this topic (analysis from the perspective of learners), contribute to the identification of the fundamental structure of the subject matter for instruction (i.e., the issue of elementarization) of the possibly fruitful strategies to improve learning outcomes and overcome student difficulties. From such outcomes, the third component of the MER stems:
- (iii)
- Construction and evaluation of learning environments and activities.
2.1.1. Analysis from the Perspective of Theory
2.1.2. Analysis from the Perspective of the Learner
2.1.2.1. Significance of the Subject Matter for Students
2.1.2.2. Research on Student Conceptions and Difficulties
2.1.2.3. Research on Effective Experimental Activities
2.2. Development of the Learning Environment
2.2.1. The Predict Observe Explain (POE) Strategy
2.2.2. Practical Choices for Creating a Productive Learning Environment
- (a)
- Propose activities based on a combination of real experiments and interactive simulations. Measurements were taken via the open-source Tracker Video Analysis tool [61] while interactive simulations were designed and run within the freeware 2D simulation environment Algodoo (v2.1.0) [73]. Barring all educational considerations on these, the choice of free/open-source software in teacher education is motivated primarily by the importance of adopting tools that teachers can continue to use in their future school teaching.
- (b)
- Let students perform experimental activities even in the absence of specific equipment. The challenge facing remote education is the potential absence of practical interaction with measurement tools and experimental apparatuses. So, we asked students to use the resources they had at home, taking advantage of the spread of smartphones among them. When it was not possible for the students to perform the experiments themselves at home, the experiments were replaced by demonstration videos with experiments also performed with objects of common use by the teacher. Sometimes the real experiments were replaced or supported by Algodoo simulations, designed and realized by students, converted in video, and analyzed, thanks to Video Analysis, as if they were real experiments.
- (c)
- Let students perform the data analysis, modelling activities and explanation phase in groups, guided by the instructors who stimulated discussion about experimental results. Collaborative learning opportunities are at the heart of the socio-constructivist paradigm, and play an important part in our learning environment, on which they were made possible by exploiting breakout rooms in Zoom [74]. Within cooperative environments, students were guided in the construction of qualitative, conceptual mental models of phenomena connected with relative motion, which were refined reinforced with the progress of the experimental sequence.
- (d)
- In some cases, exploit the possibilities of Algodoo, to “break the rules” of POE and encourage students to more open and autonomous investigations starting from motivating questions. This strategy was used in the case of the thought experiment of Einstein’s elevator, with the stimulating question concerning the behavior of swinging pendulums and other objects after the start of free fall. Students in this case investigated the problem with fewer constraints by autonomously designing Algodoo simulations. In addition to the educational relevance of this strategy, observing the work of students and discussing the activity in groups provided us with crucial information on the role that the modeling activity has in scaffolding students’ knowledge.
3. Description of the Sequence
- -
- Inertial reference frame (PoR),
- -
- Non-inertial reference frame in accelerated motion with uniform acceleration: in the horizontal plane and on inclined planes, and
- -
- Non-inertial reference frame: Einstein’s elevator, free fall (PoE).
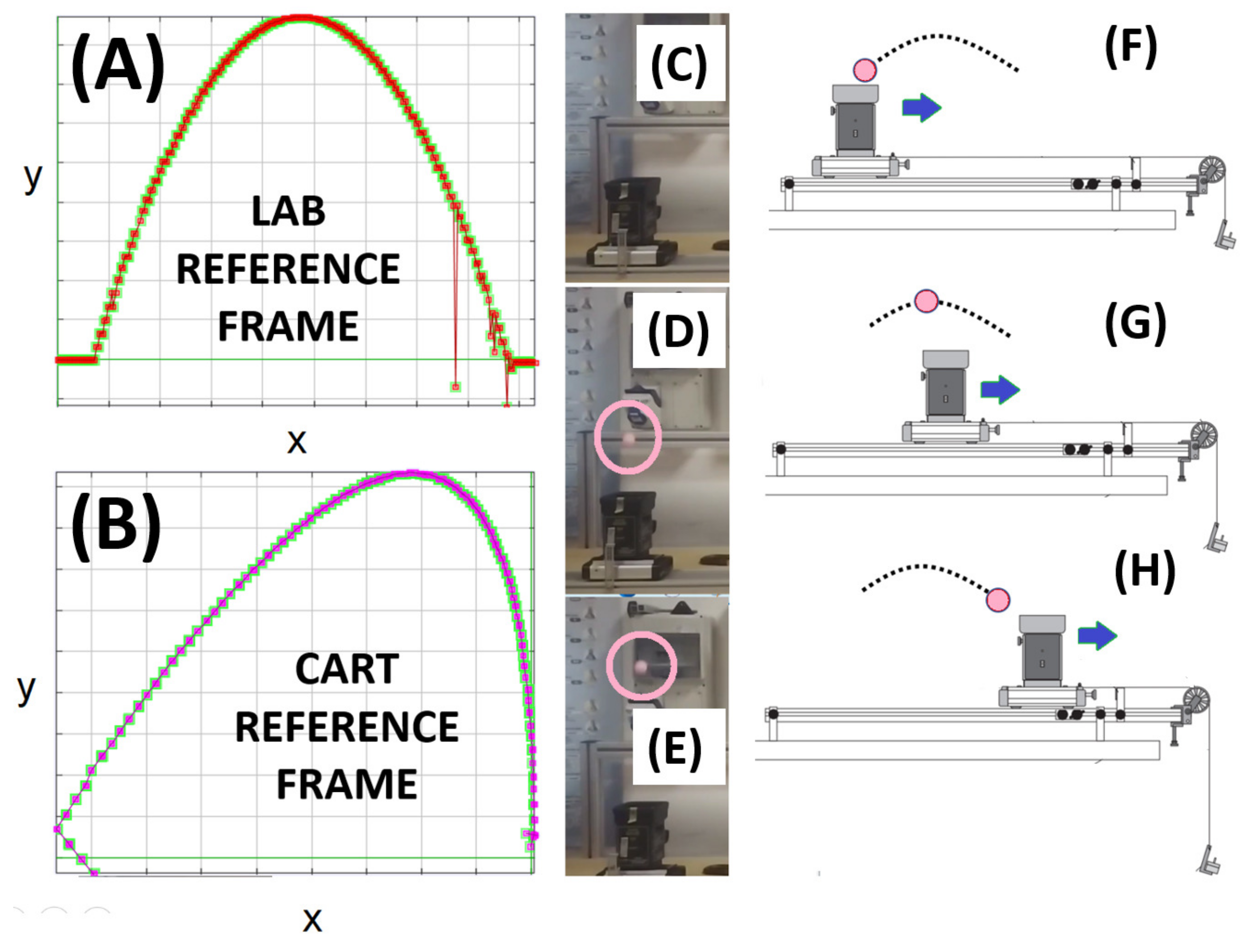
3.1. Inertial Frame of Reference
3.2. Non-Inertial Reference Frame in 1D
3.3. D Non-Inertial Reference Frame: Liquids’ Surface Shape in Accelerated Reference Frame
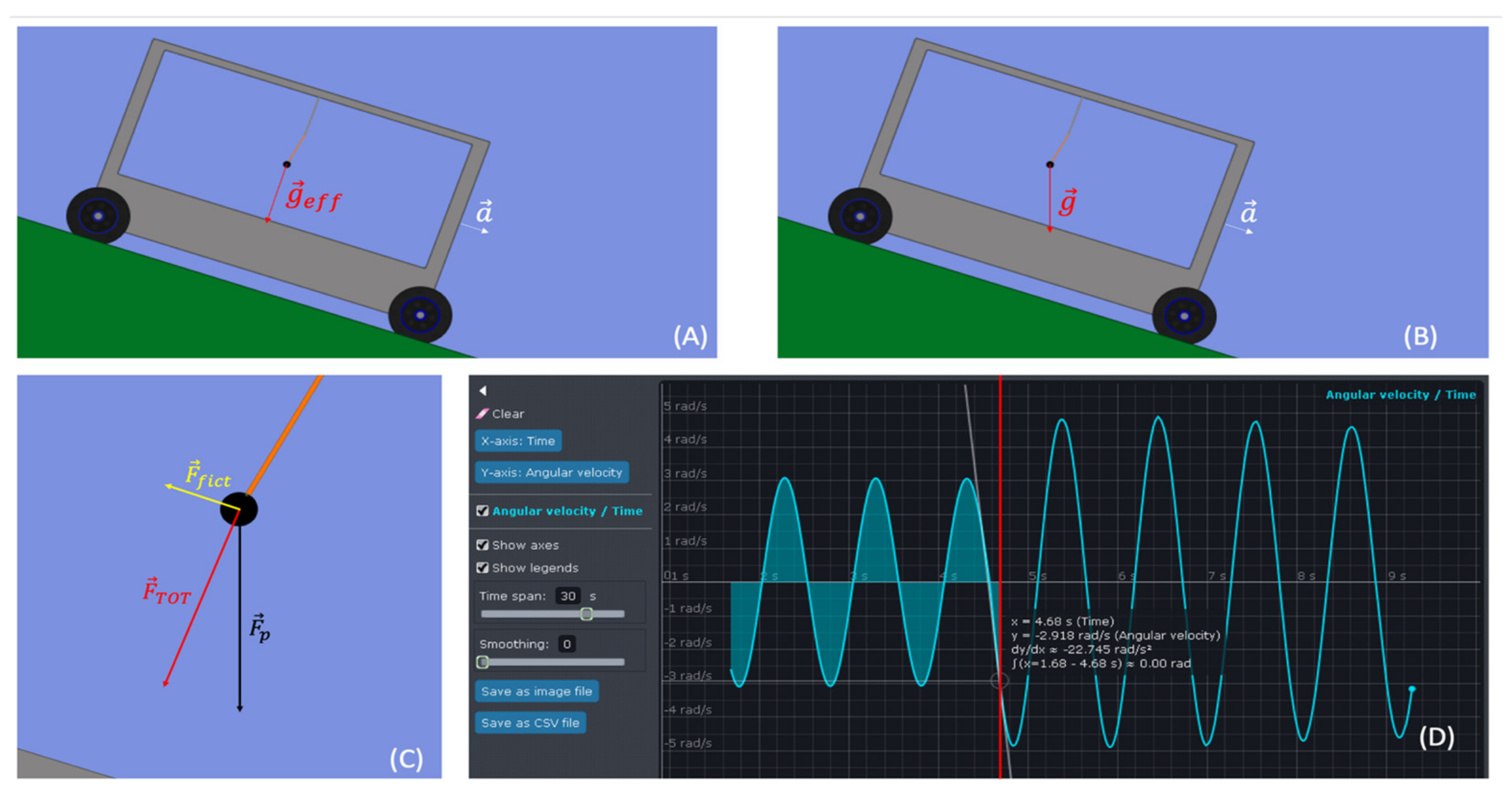
3.4. Non-Inertial Reference Frame in 1D: Pendulum Oscillations
3.5. Free-Falling Reference Frame: The Equivalence Principle and Einstein’s Elevator
4. Results
4.1. Student Understanding of Key Concepts
4.1.1. Quantitative Results
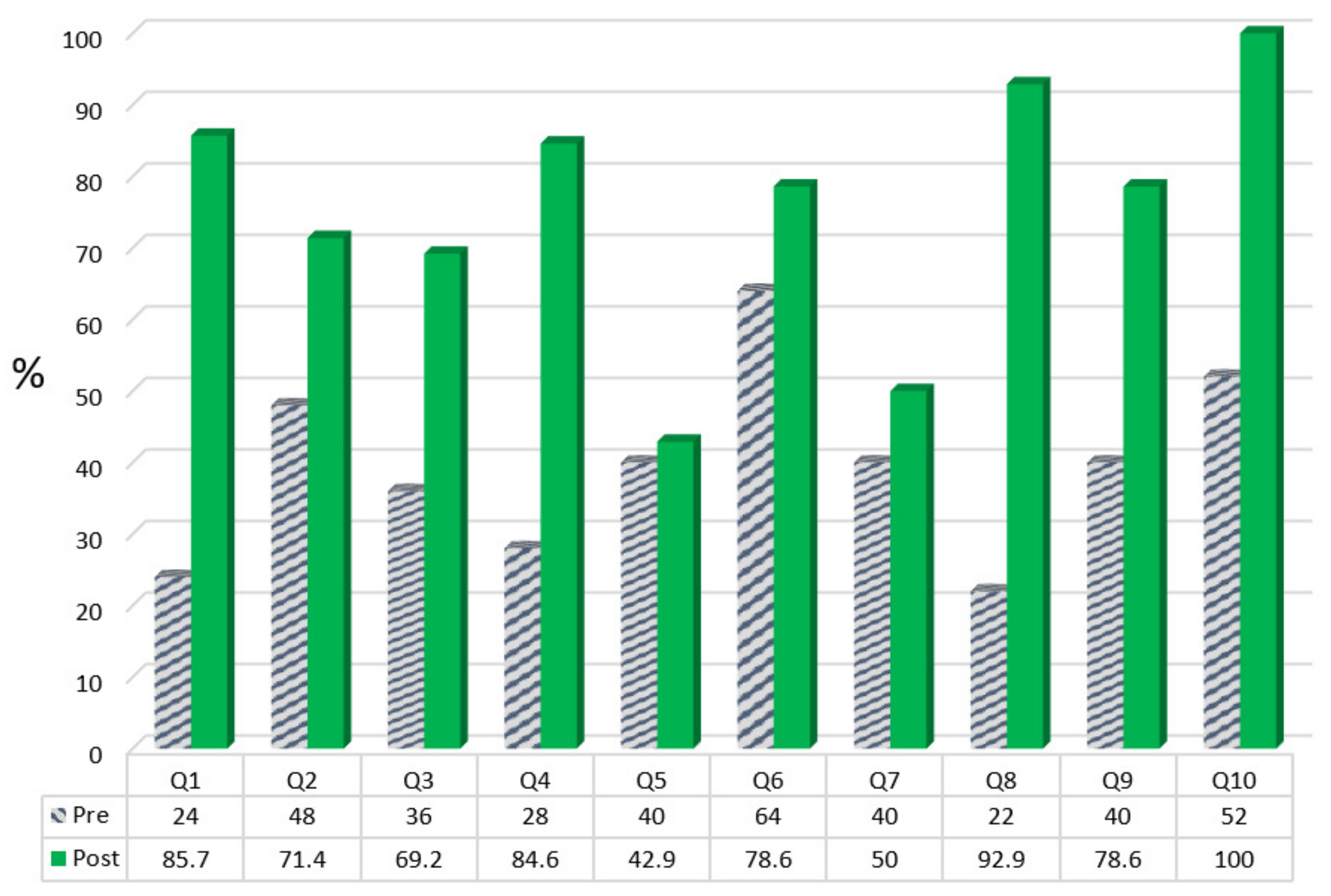
- (i)
- Students showed big difficulties distinguishing between the trajectories of a projectile with respect to two inertial FoRs. Only six students (24%) identified the correct trajectory in the moving FoR while one-half of students recognized the path in the FoR of the Lab.
- (ii)
- Only a small fraction of students identified the real path of the drone of Q3 as seen by an observer sitting still on the bank of the river) at a constant speed with respect to a moving FoR (9 students, 36%) while just one-half recognize that there are no fictitious forces acting on the drone in this FoR.
- (iii)
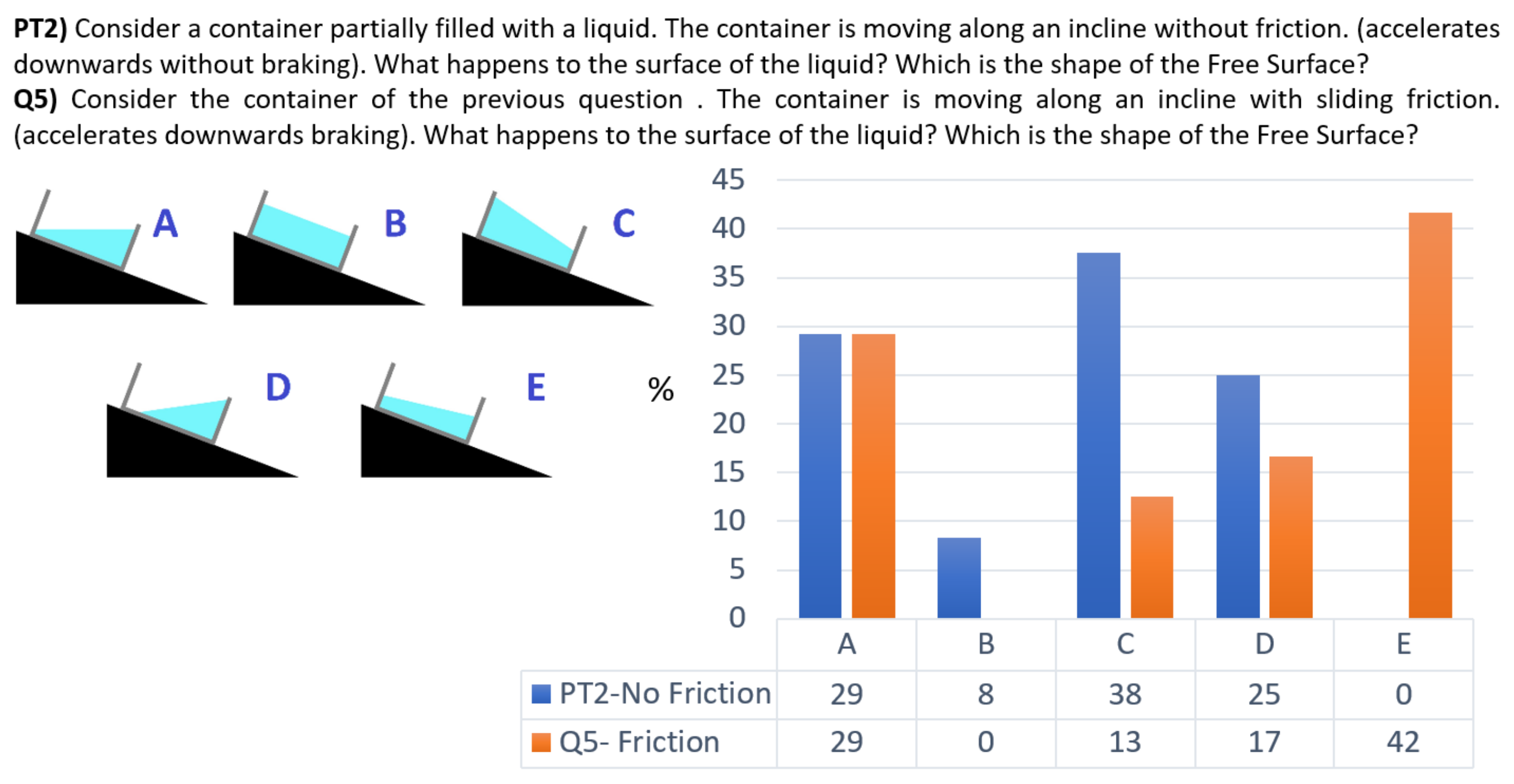
- Most students (22, i.e., 92%) did not predict properly what happens to the shape of the Free Surface of a liquid which fills a container in accelerated motion along a slope.
- (iv)
- One-third of students did not identify what will be the center of oscillations of a pendulum hanging from the ceiling of a car descending along an incline and just 10 (42%) understood that the period of oscillations increases.
- (v)
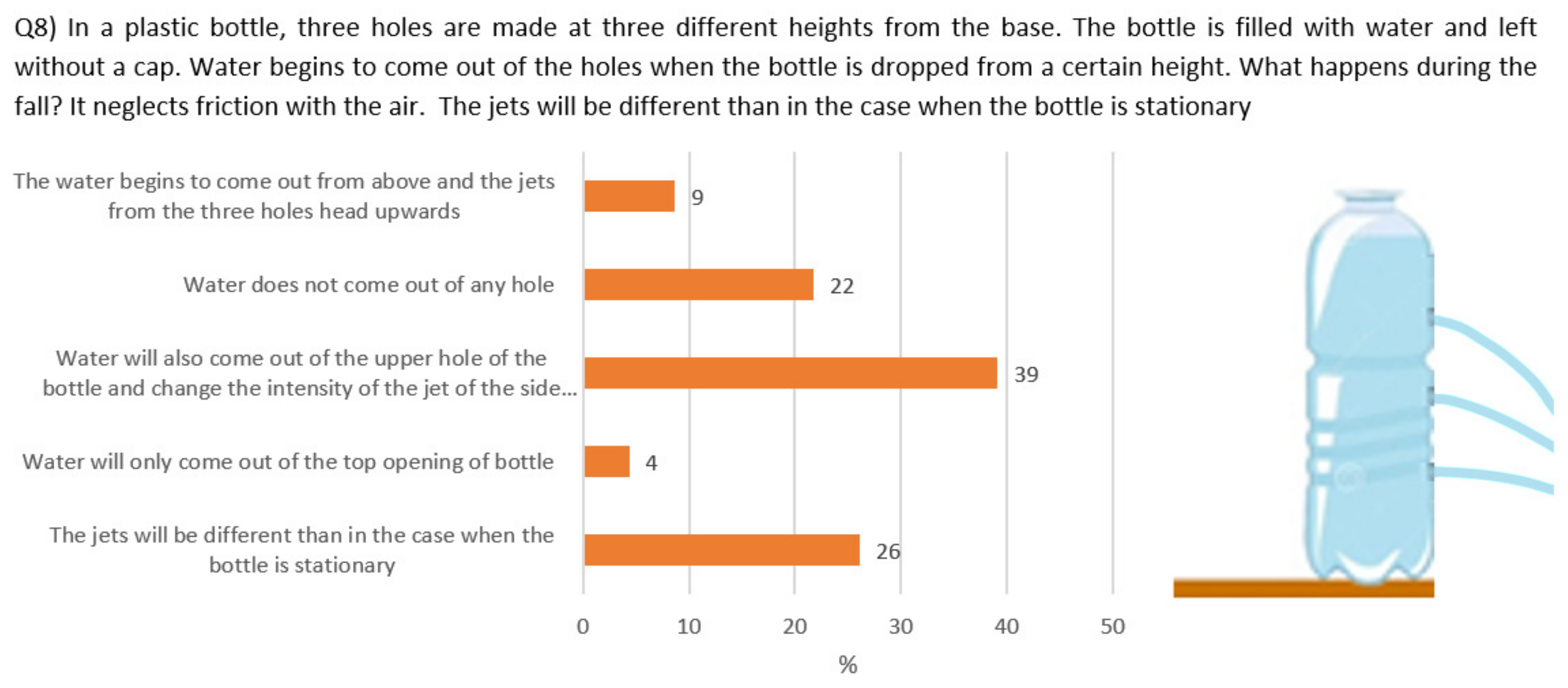
- Only 5 (21%) students were convinced that during the free fall, the water in a bottle is in state of weightlessness and does not push through a hole in the side. Most students (13, i.e., 54%) predicted that an object moving horizontally in an elevator is deflected upward when the elevator starts to fall freely.
- (vi)
- 70% of students knew that a body in the ISS (International Space Station) in orbit around the Earth does not fall. Among them, a significant fraction (7, i.e., 28% of the total) believed that this happens because in the space station the gravitational field is negligible because of the great distance from the center of the Earth. Among the students who gave a correct answer one-half resorted to the presence of a fictitious force and one-half to the weightless due to the free-falling motion of the satellite.
- (i)
- Answers to the post-test (Q1–Q4) confirmed an improvement of students’ understanding of how trajectories in an inertial frame can be converted to trajectories in another by a simple transformation (correct answers, average of Q1–Q4, from 35% to 80%).
- (ii)
- Additionally, after the sequence most students did not predict properly what happens to the shape of the Free Surface of a liquid which fills a container in accelerated motion along an incline. However, if we consider the difficulty increased by the presence of friction, we can conclude that assuming as correct both B and E just 40% before while after 64%.
- (iii)
- Nearly 80% of the students, after the sequence, recognized what will be the center of oscillations of a pendulum hanging from the ceiling of a car descending along an inclined plane while, also after the sequence just 50% understood that the period increases.
- (iv)
- After the sequence a large percentage of students (90%) were able to recognize that during the free fall, the water in a bottle is in state of weightlessness and does not push through a hole in the side. Most students (80%) predicted correctly that an object moving horizontally in an elevator is not deflected by the gravitational force when the elevator starts to free fall.
- (v)
- All the students recognize that in the ISS in orbit around the Earth a body does not fall. Among them, nobody, after the sequence, believed that this happens because in the space station the gravitational field is negligible given the great distance from the center of the Earth. Most students (72%) resorted to the free-falling motion of the satellite.
4.1.2. Qualitative Results: Interviews
4.2. Evaluation of the Learning Environment: Student Ratings
- -
- Effectiveness in terms of understanding a phenomenon thanks to the proposed activities (possible responses are presented in a range from 1 “not effective” to 5 “very effective”).
- -
- Enjoyment during each workshop activity (possible responses are presented in a sequence 1 boring, …, 5 very nice).
- -
- Engagement and personal interest during the activities (possible responses are presented in a sequence 1 unpleasant, …, 5 very engaging).
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. The Pre-Post Test
| Inertial Reference of Frame | |
|---|---|
| Alice is standing in a train moving at velocity v from left to right relative to Bob, who is standing on a platform. As Alice passes Bob, she drops a bowling ball out of the train’s window: (Q1) Ignoring air resistance, which path of the ball would Bob observe, standing on the platform? (A) Path (A) (B) Path (B) (C) Path (C) | 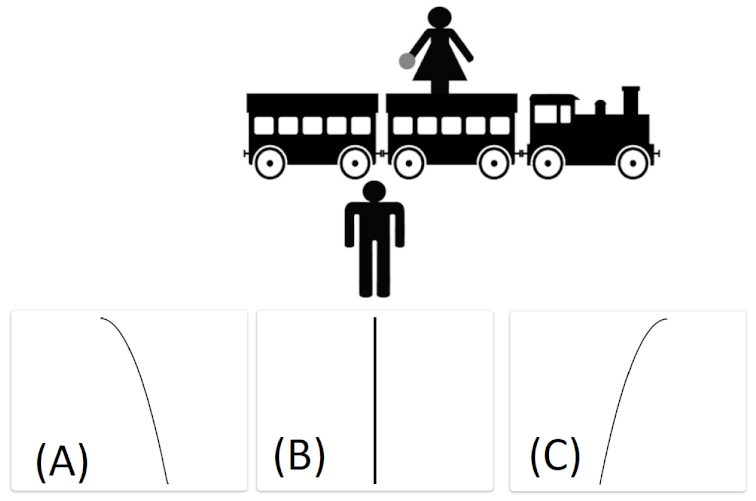 |
| (Q2) Ignoring air resistance, which path of the ball would Alice observe, standing in the train? (A) Path (A) (B) Path (B) (C) Path (C) | |
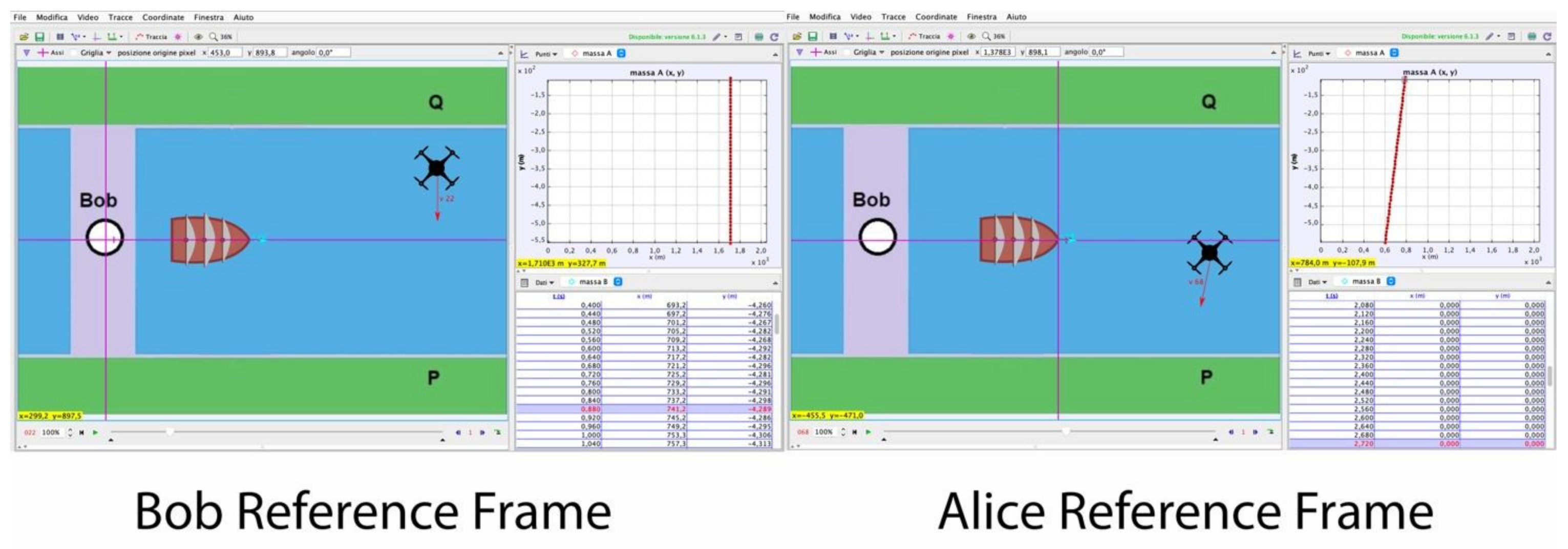
| (Q3) Bob is sitting on a bridge on the river. Alice is standing on a boat moving along the river at velocity v from left to right relative to Bob. As Alice passes the bridge, a drone takes off from point Q to reach point P flying at a constant speed. Which path of the drone would Alice observe, standing on the boat? (A) Path (A) (B) Path (B) (C) Path (C) (D) Path (D) (E) Path (E) | 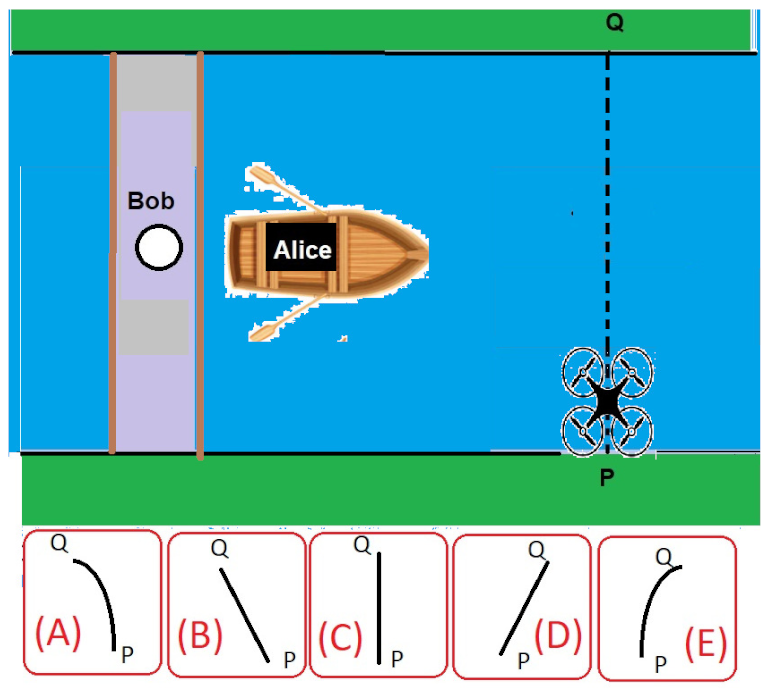 |
| (Q4) Thinking about the previous question: in Alice’s reference frame what will be the “fictitious” force acting on the drone (A) Constant towards the right (B) Not constant towards the left (C) There is no apparent force (D) Constant towards the left (E) Not constant towards the right | |
| Non-Inertial Reference of Frame | |
| (PT1) Open: Alice is standing in a train from left to right relative to Bob, who is standing on a platform. As Alice passes Bob, a ball is thrown vertically upwards from the train while the train is accelerating: Ignoring air resistance, what path of the ball would Bob observe, standing on the platform? What path of the ball would Alice observe, standing in the train? |  |
| (PT2) Consider a container partially filled with a liquid. The container is moving along an incline without friction. (accelerates downwards without braking). What happens to the surface of the liquid? Which is the shape of the Free Surface? A B C D E |  |
| (Q5) Consider the container of the previous question. The container is moving along an incline with sliding friction. (accelerates downwards braking). What happens to the surface of the liquid? Which is the shape of the Free Surface? A B C D E | |
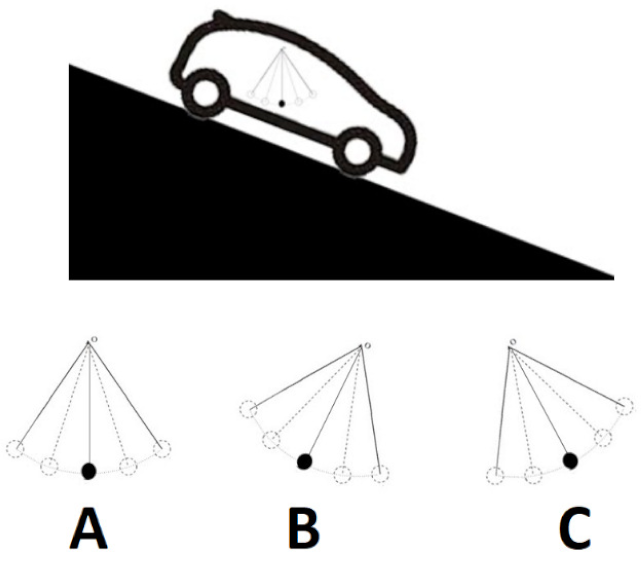
| (Q6) A pendulum hanging from the ceiling of a car swings with small amplitudes, when the car starts to descend from an inclined plane without friction (accelerates downwards without braking). What will the pendulum center of oscillations be? A B C (Q7) Consider the pendulum of the previous question How will the period of the pendulum be while the car descends from the inclined plane compared to the period when the car is at rest?
|  |
| Free-Falling Reference Frame | |
(Q8) In a plastic bottle, three holes are made at three different heights from the base. The bottle is filled with water and left without a cap. Water begins to come out of the holes when the bottle is dropped from a certain height. What happens during the fall? It neglects friction with the air.
|  |
| (Q9) Consider an elevator with a table anchored to it, as represented in the figure. A small cube is placed on the table, with an initial speed that allows it to reach the edge of the table, despite the friction with it. The elevator has a hole which would allow the cube to exit the elevator if it moved horizontally with respect to the surface of the table. After the cube is thrown, when it is in the center of the table, the cable that supports the elevator is cut. What happens? A B C | 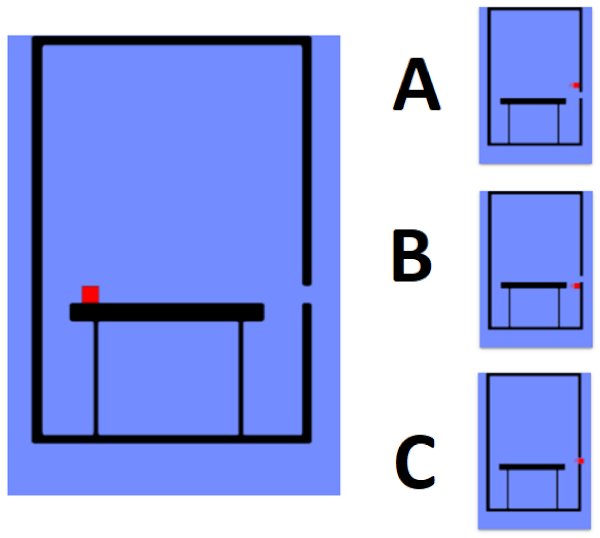 |
| (PT3) A rigid pendulum hanging from the ceiling of an elevator is swinging, when suddenly the cable supporting the elevator is removed. At the time of cable breakage the pendulum was at the point of equilibrium. Describe the motion of the pendulum observed by the reference frame S’ integral with the elevator, neglecting air friction. What happens if at the time of cable breakage the pendulum was at the point of maximum amplitude? | |
(Q10) Astronaut Samantha is on the ISS (International Space Station) in orbit around the Earth. Samantha has a small ball in her fist. Suddenly she opens her hand leaving the grip.
| |
References
- Kersting, M.; Henriksen, E.K.; Bøe, M.V.; Angell, C. General relativity in upper secondary school: Design and evaluation of an online learning environment using the model of educational reconstruction. Phys. Rev. Phys. Educ. Res. 2018, 14, 010130. [Google Scholar] [CrossRef]
- Levrini, O. Reconstructing the basic concepts of general relativity from an educational and cultural point of view. In Science Education and Culture; Springer: Dordrecht, The Netherlands, 2001; pp. 311–326. [Google Scholar]
- Bandyopadhyay, A.; Kumar, A. Probing students’ understanding of some conceptual themes in general relativity. Phys. Rev. Spec. Top. Phys. Educ. Res. 2010, 6, 020104. [Google Scholar] [CrossRef]
- De Ambrosis, A.; Malgieri, M.; Mascheretti, P.; Onorato, P. Investigating the role of sliding friction in rolling motion: A teaching sequence based on experiments and simulations. Eur. J. Phys. 2015, 36, 035020. [Google Scholar] [CrossRef]
- Onorato, P.; Malgieri, M.; De Ambrosis, A. Three years evaluation of a teaching learning sequence on rolling motion based on a blended learning environment. J. Phys. Conf. Ser. 2019, 1286, 012053. [Google Scholar] [CrossRef]
- Duit, R.; Gropengießer, H.; Kattmann, U.; Komorek, M.; Parchmann, I. The model of educational reconstruction–A framework for improving teaching and learning science. In Science Education Research and Practice in Europe; Brill: Leiden, The Netherlands, 2012; pp. 13–37. [Google Scholar]
- Klafki, W. Didactic analysis as the core of preparation of instruction (Didaktische Analyse als Kern der Unterrichtsvorbereitung). J. Curric. Stud. 1995, 27, 13–30. [Google Scholar] [CrossRef]
- Duit, R.; Komorek, M.; Wilbers, J. Studies on educational reconstruction of chaos theory. Res. Sci. Educ. 1997, 27, 339–357. [Google Scholar] [CrossRef]
- Niebert, K.; Gropengiesser, H. The model of educational reconstruction: A framework for the design of theory-based content specific interventions. The example of climate change. In Educational Design Research–Part B: Illustrative Cases; SLO: Enschede, The Netherlands, 2013; pp. 511–531. [Google Scholar]
- Kersting, M. General Relativity in Secondary School: Research-Based Development of Learning Resources and Analyses of Students’ Conceptual Understanding Using the Model of Educational Reconstruction. Ph.D. Thesis, University of Oslo, Oslo, Norway, 2019. [Google Scholar]
- Kamphorst, F.; Vollebregt, M.J.; Savelsbergh, E.R.; van Joolingen, W.R. An Educational Reconstruction of Special Relativity Theory for Secondary Education. Sci. Educ. 2023, 32, 57–100. [Google Scholar] [CrossRef]
- Metaxas, I.; Michailidi, E.; Stavrou, D.; Pavlidis, I.V. Educational reconstruction of size-depended-properties in nanotechnology for teaching in tertiary education. Chem. Teach. Int. 2021, 3, 413–422. [Google Scholar] [CrossRef]
- Italian Ministry of Education. Indicazioni Nazionali per i Licei Scientifici; Italian Ministry of Education: Rome, Italy, 2012.
- Scherr, R.E.; Shaffer, P.S.; Vokos, S. Student understanding of time in special relativity: Simultaneity and reference frames. Am. J. Phys. 2001, 69 (Suppl. S1), S24–S35. [Google Scholar] [CrossRef]
- Mortimer, E.F. Conceptual change or conceptual profile change? Sci. Educ. 1995, 4, 267–285. [Google Scholar] [CrossRef]
- Ayala Filho, Á.L. A construção de um perfil para o conceito de referencial em Física e os obstáculos epistemológicos à aprendizagem da teoria da relatividade restrita. Investig. Ensino Ciências 2010, 15, 155–179. [Google Scholar]
- Alstein, P.; Krijtenburg-Lewerissa, K.; van Joolingen, W.R. Teaching and learning special relativity theory in secondary and lower undergraduate education: A literature review. Phys. Rev. Phys. Educ. Res. 2021, 17, 023101. [Google Scholar] [CrossRef]
- Arlego, M.; Otero, M.R. Teaching basic special relativity in high school: The role of classical kinematics. In Contemporary Science Education and Challenges in the Present Society: Perspectives in Physics Teaching and Learning; FEUSP: São Paulo, Brazil, 2017. [Google Scholar]
- Scherr, R.E. An Investigation of Student Understanding of Basic Concepts in Special Relativity. Ph.D. Thesis, University of Washington, Seattle, WA, USA, 2001. [Google Scholar]
- Desloge, E.A. Classical Mechanics; John Wiley Sons: Hoboken, NJ, USA, 1982; Volume 1. [Google Scholar]
- Sommerfeld, A. Mechanics: Lectures on Theoretical Physics; Elsevier: Amsterdam, The Netherlands, 2016; Volume 1. [Google Scholar]
- Halliday, D.; Resnick, R.; Walker, J. Fundamentals of Physics; John Wiley Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Galilei, G. Dialogo Sopra i due Massimi Sistemi del Mondo; Rizzoli: Milano, Italy, 2013. [Google Scholar]
- Browne, K.M. Galilei proposed the principle of relativity, but not the “Galilean transformation”. Am. J. Phys. 2020, 88, 207–213. [Google Scholar] [CrossRef]
- Norton, J.D. Einstein’s Special Theory of Relativity and the Problems in the Electrodynamics of Moving Bodies that Led him to it. In The Cambrridge Companion to Einstein; Cambridge University Press: Cambridge, UK, 2014; pp. 72–102. [Google Scholar]
- Pietrocola, M.; Zylbersztajn, A. The use of the Principle of Relativity in the interpretation of phenomena by undergraduate physics students. Int. J. Sci. Educ. 1999, 21, 261–276. [Google Scholar] [CrossRef]
- Mencuccini, C.; Silvestrini, V. Fisica: Meccanica e Termodinamica: Con Esempi ed Esercizi; Casa Editrice Ambrosiana: Milano, Italy, 2016. [Google Scholar]
- Amaldi, U. La Fisica di Amaldi; Zanichelli: Bologna, Italy, 2011. [Google Scholar]
- Múnera, H.A. The principle of equivalence: Demonstrations of local effective vertical and horizontal. Phys. Teach. 2010, 48, 131–133. [Google Scholar] [CrossRef]
- Fägerlind, C.O.; Pendrill, A.M. Liquid in accelerated motion. Phys. Educ. 2015, 50, 648. [Google Scholar] [CrossRef]
- Norton, J.D. What was Einstein’s principle of equivalence? Stud. Hist. Philos. Sci. Part A 1985, 16, 203–246. [Google Scholar] [CrossRef]
- Norton, J.D. General covariance and the foundations of general relativity: Eight decades of dispute. Rep. Prog. Phys. 1993, 56, 791. [Google Scholar] [CrossRef]
- Norton, J.D. Einstein’s Conflicting Heuristics: The Discovery of General Relativity. In Thinking about Space and Time; Birkhäuser: Cham, Switzerland, 2020; pp. 17–48. [Google Scholar]
- Di Casola, E.; Liberati, S.; Sonego, S. Nonequivalence of equivalence principles. Am. J. Phys. 2015, 83, 39–46. [Google Scholar] [CrossRef]
- Thorne, K.S.; Lee, D.L.; Lightman, A.P. Foundations for a theory of gravitation theories. Phys. Rev. D 1973, 7, 3563. [Google Scholar] [CrossRef]
- Krane, K. Modern Physics, 3rd ed.; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- Levrini, O.; Altamore, A.; Balzano, E.; Bertozzi, E.; Gagliardi, M.; Giordano, E.; Guidoni, P.; Rinaudo, G.; Tarsitani, C. Looking at the physics curriculum in terms of framing ideas. In Proceedings of the GIREP 2008 Conference, Nicosia, Cyprus, 18–22 August 2008; pp. 18–22. [Google Scholar]
- Giliberti, M.; Organtini, G. Reconsidering Physics Education for better understanding of modern physics. J. Phys.: Conf. Ser. 2021, 1929, 012046. [Google Scholar] [CrossRef]
- Villani, A.; Arruda, S.M. Special theory of relativity, conceptual change and history of science. Sci. Educ. 1998, 7, 85–100. [Google Scholar] [CrossRef]
- Doménech, A.; Casasús, E.; Doménech, M.T.; Buñol, I.B. The classical concept of mass: Theoretical difficulties and students’ definitions. Int. J. Sci. Educ. 1993, 15, 163–173. [Google Scholar] [CrossRef]
- Michelini, M.; Pugliese, E.; Santi, L. Mass from classical physics to special relativity: Learning results. In Proceedings of the World Conference on Physics Education 2012, Istanbul, Turkey, 1–6 July 2012; pp. 141–154. [Google Scholar]
- Saltiel, E.M.; Malgrange, J.L. Spontaneous ways of reasoning in elementary kinematics. Eur. J. Phys. 1980, 1, 73–80. [Google Scholar] [CrossRef]
- Maury, L.; Saltiel, E.; Viennot, L. Étude de la notion de mouvement chez l’enfant à partir des changements de référentiels. Rev. Française Pédagogie 1977, 40, 15–29. [Google Scholar] [CrossRef]
- McCloskey, M. Naïve Theories of Motion. In Mental Models; Gentner, D., Stevens, A.L., Eds.; Lawrence Erlbaum Associates: Hillsdale, NJ, USA, 1983; pp. 299–324. [Google Scholar]
- Nersessian, N.J.; Resnick, L.B. Comparing historical and intuitive explanations of motion: Does “naive physics” have a structure. In Proceedings of the Eleventh Annual Conference of the Cognitive Science Society, Ann Arbor, MI, USA, 16–19 August 1989; Erlbaum: Mahwah, NJ, USA; pp. 412–420. [Google Scholar]
- McCloskey, M.; Washburn, A.; Felch, L. Intuitive physics: The straight-down belief and its origin. J. Exp. Psychol. Learn. Mem. Cogn. 1989, 9, 636. [Google Scholar] [CrossRef]
- Aslanides, J.S.; Savage, C.M. Relativity concept inventory: Development, analysis, and results. Phys. Rev. Spec. Top. Phys. Educ. Res. 2013, 9, 010118. [Google Scholar] [CrossRef]
- Panse, S.; Ramadas, J.; Kumar, A. Alternative conceptions in Galilean relativity: Frames of reference. Int. J. Sci. Educ. 1994, 16, 63–82. [Google Scholar] [CrossRef]
- Ramadas, J.; Barve, S.; Kumar, A. Alternative conceptions in Galilean relativity: Inertial and non-inertial observers. Int. J. Sci. Educ. 1996, 18, 615–629. [Google Scholar] [CrossRef]
- de Hosson, C.; Kermen, I.; Parizot, E. Exploring students’ understanding of reference frames and time in Galilean and special relativity. Eur. J. Phys. 2010, 31, 1527. [Google Scholar] [CrossRef]
- Dimitriadi, K.; Halkia, K. Secondary students’ understanding of basic ideas of special relativity. Int. J. Sci. Educ. 2012, 34, 2565–2582. [Google Scholar] [CrossRef]
- Viennot, L. Spontaneous reasoning in elementary dynamics. Eur. J. Sci. Educ. 1979, 1, 205–221. [Google Scholar] [CrossRef]
- Bandyopadhyay, A. Students’ ideas of the meaning of the relativity principle. Eur. J. Phys. 2009, 30, 1239. [Google Scholar] [CrossRef]
- Bandyopadhyay, A.; Kumar, A. Probing students’ ideas of the principle of equivalence. Eur. J. Phys. 2010, 32, 139. [Google Scholar] [CrossRef]
- Mayer, V.V.; Varaksina, E.I. A simple demonstration of Einstein’s lift: A body thrown upwards moves rectilinearly and uniformly relative to a free-falling model of the lift. Eur. J. Phys. 2015, 36, 055020. [Google Scholar] [CrossRef]
- Kapotis, E.; Kalkanis, G. Einstein’s elevator in class: A self-construction by students for the study of the equivalence principle. Phys. Teach. 2016, 54, 404–407. [Google Scholar] [CrossRef]
- Bozzo, G. Free-Fall Demonstrations in the High School Laboratory. Phys. Teach. 2020, 58, 23–27. [Google Scholar] [CrossRef]
- Monteiro, M.; Cabeza, C.; Marti, A.C. Acceleration measurements using smartphone sensors: Dealing with the equivalence principle. Rev. Bras. Ensino Fis. 2015, 37, 1303. [Google Scholar] [CrossRef]
- Kuhn, J. Analyzing free fall with a smartphone acceleration sensor. Phys. Teach. 2012, 50, 182–183. [Google Scholar] [CrossRef]
- Kuhn, J.; Vogt, P. Smartphones as experimental tools: Different methods to determine the gravitational acceleration in classroom physics by using everyday devices. Eur. J. Phys. Educ. 2013, 4, 16–27. [Google Scholar]
- OSP, Tracker, Video Analysis and Modeling Tool. Available online: http://physlets.org/tracker/ (accessed on 10 July 2023).
- White, R.; Gunstone, R. Probing Understanding; Routledge: Abingdon, UK, 2014. [Google Scholar]
- Liew, C.W.; Treagust, D.F. A predict-observe-explain teaching sequence for learning about students’ understanding of heat and expansion of liquids. Aust. Sci. Teach. J. 1995, 41, 68–71. [Google Scholar]
- Treagust, D.F.; Mthembu, Z.; Chandrasegaran, A.L. Evaluation of the Predict-Observe-Explain instructional strategy to enhance students’ understanding of redox reactions. In Learning with Understanding in the Chemistry Classroom; Springer: Dordrecht, The Netherlands, 2014; pp. 265–286. [Google Scholar]
- Liew, C.W.; Treagust, D.F. The effectiveness of predict-observe-explain tasks in diagnosing students’ understanding of science and in identifying their levels of achievement. In Proceedings of the Paper Presented at the Annual Meeting of the American Educational Research Association, San Diego, CA, USA, 13–17 April 1998. [Google Scholar]
- Onorato, P.; Rosi, T.; Tufino, E.; Caprara, C.; Malgieri, M. Using smartphone cameras and ambient light sensors in distance learning: The attenuation law as experimental determination of gamma correction. Phys. Educ. 2021, 56, 045007. [Google Scholar] [CrossRef]
- Al Mamun, M.A.; Lawrie, G.; Wright, T. Exploration of learner-content interactions and learning approaches: The role of guided inquiry in the self-directed online environments. Comput. Educ. 2022, 178, 104398. [Google Scholar] [CrossRef]
- Jiang, F.; McComas, W.F. The effects of inquiry teaching on student science achievement and attitudes: Evidence from propensity score analysis of PISA data. Int. J. Sci. Educ. 2015, 37, 554–576. [Google Scholar] [CrossRef]
- Zacharia, Z.C. The impact of interactive computer simulations on the nature and quality of postgraduate science teachers’ explanations in physics. Int. J. Sci. Educ. 2005, 27, 1741–1767. [Google Scholar] [CrossRef]
- Alfiyanti, I.F.; Jatmiko, B. The Effectiveness of Predict Observe Explain (POE) Model with PhET to Improve Critical Thinking Skills of Senior High School Students. Stud. Learn. Teach. 2020, 1, 76–85. [Google Scholar] [CrossRef]
- Kearney, M.; Treagust, D.F.; Yeo, S.; Zadnik, M.G. Student and teacher perceptions of the use of multimedia supported predict–observe–explain tasks to probe understanding. Res. Sci. Educ. 2001, 31, 589–615. [Google Scholar] [CrossRef]
- Al Mamun, M.A.; Lawrie, G.; Wright, T. Instructional design of scaffolded online learning modules for self-directed and inquiry-based learning environments. Comput. Educ. 2020, 144, 103695. [Google Scholar] [CrossRef]
- Algodoo. Available online: http://www.algodoo.com/ (accessed on 10 July 2023).
- Zoom. Available online: https://zoom.us/ (accessed on 10 July 2023).
- Haber-Schaim, U.; Dodge, J.H.; Gardner, R.; Shore, E.A.; Walter, F. PSSC Physics, 7th ed.; Kendall/Hunt: Dubuque, Iowa, 1991. [Google Scholar]
- Marzari, A.; Rosi, T.; Onorato, P. Three experiments on the Einstein’s lift. Eur. J. Phys. 2021, 42, 045404. [Google Scholar] [CrossRef]



| Part | Curriculum Goals | Key Concept | Description of the Activity | Items |
|---|---|---|---|---|
| (A) | Concept of Frame of Reference (FoR) Principle of Relativity (PoR) and Galilean transformations | Inertial reference frame | Video analysis of a motion seen by two different reference frames in uniform rectilinear motion with respect to each other. Algodoo simulation (exporting the video and then proceeding with Tracker analysis). | Q1, Q2 Q3, Q4 |
| (B) | Concept of FoR and classification as inertial and non-inertial | Non-inertial reference frame (with constant acceleration in the horizontal plane) | Video analysis of a motion seen by two different reference frames in straight motion uniformly accelerated relative to each other. | PT1 (open) |
| (C) | Concept of FoR and their classification as inertial and non-inertial | Non-inertial reference frame (inclined plane) | Video analysis of the shape of the surface of a liquid in a vessel descending along an inclined plane. Simulation of the surface of a fluid in an accelerated vessel. | PT2, Q5 |
| (D) | The principle of equivalence (PoE, i.e., the equivalence of gravitational and inertial mass | Algodoo simulation of the oscillations of a pendulum in a car descending along an inclined plane. | Q6,Q7 | |
| (E) | The PoE, i.e., the equivalence of gravitational and inertial mass | Free fall reference frame | Video of a qualitative experiment with a perforated bottle in free fall. | Q8 |
| Simulation of horizontal motion in an elevator in free fall. | Q9 | |||
| Discussion about PoE and fictitious forces for different FR in free fall. | Q10 | |||
| Video analysis and simulations of the motion of a pendulum in the elevator in an elevator in free fall. | PT3 (open) |
| Activity | Effectiveness | Enjoyment | Engagement |
|---|---|---|---|
| Experiments performed at home by students, on classical relative motion | 4.1 | 3.5 | 3.8 |
| Experiments performed at home by the teacher | 4 | 3.7 | 3.5 |
| Using Algodoo to create virtual simulations | 4.3 | 3.9 | 3.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marzari, A.; Di Mauro, M.; Rosi, T.; Onorato, P.; Malgieri, M. Investigating the Principle of Relativity and the Principle of Equivalence in Classical Mechanics: Design and Evaluation of a Teaching–Learning Sequence Based on Experiments and Simulations. Educ. Sci. 2023, 13, 712. https://doi.org/10.3390/educsci13070712
Marzari A, Di Mauro M, Rosi T, Onorato P, Malgieri M. Investigating the Principle of Relativity and the Principle of Equivalence in Classical Mechanics: Design and Evaluation of a Teaching–Learning Sequence Based on Experiments and Simulations. Education Sciences. 2023; 13(7):712. https://doi.org/10.3390/educsci13070712
Chicago/Turabian StyleMarzari, Alessio, Marco Di Mauro, Tommaso Rosi, Pasquale Onorato, and Massimiliano Malgieri. 2023. "Investigating the Principle of Relativity and the Principle of Equivalence in Classical Mechanics: Design and Evaluation of a Teaching–Learning Sequence Based on Experiments and Simulations" Education Sciences 13, no. 7: 712. https://doi.org/10.3390/educsci13070712
APA StyleMarzari, A., Di Mauro, M., Rosi, T., Onorato, P., & Malgieri, M. (2023). Investigating the Principle of Relativity and the Principle of Equivalence in Classical Mechanics: Design and Evaluation of a Teaching–Learning Sequence Based on Experiments and Simulations. Education Sciences, 13(7), 712. https://doi.org/10.3390/educsci13070712








