Self-Assessment in the Development of Mathematical Problem-Solving Skills
Abstract
1. Introduction
- RQ1: Is there a relation between external (tutors’) assessment and students’ self-assessment?
- RQ2: In which problem-solving phase did students show more difficulties in self-assessing their work?
2. Theoretical Framework
2.1. Self-Assessment
2.2. Doing and Assessing Problem Solving with Technologies
3. Materials and Methods
3.1. Self-Assessment within the Digital Math Training Project
- A weekly synchronous online tutoring in web-conference, conducted by a tutor and focused on how to use the ACE to solve the problems;
- A discussion forum monitored by tutors in which students can interrelate with the other participants and discuss their solving strategies;
- A questionnaire which guides students to the self-assessment of the submitted problems according to the parameters chosen for grading the problems [12].
- Comprehension: Analyze the problematic situation, represent, and interpret the data and then turn them into mathematical language;
- Identification of a solving strategy: Employ solving strategies by modeling the problem and by using the most suitable strategy;
- Development of the solving process: Solve the problematic situation consistently, completely, and correctly by applying mathematical rules and by performing the necessary calculations;
- Argumentation: Explain and comment on the chosen strategy, the key steps of the building process and the consistency of the results;
- Use of an ACE: Use the ACE commands appropriately and effectively in order to solve the problem.
- To what level do you think you understood—and showed that you understood—the problematic situation?
- To what level do you think you identified and described the solution strategy?
- To what level do you think you developed the chosen solving process?
- To what level did you discuss your steps clearly and in detail?
- To what level do you think you effectively used Maple?
- Did you find some difficulties in solving this problem?
- To establish and share the evaluation criteria, an assessment rubric has been created and shared through the DLE;
- To show how to apply the established criteria and clarify what a good performance is, the section “Get ready for the training!” has been designed; moreover, proposed solutions to the problems are published after the submission deadline. To increment the range of solving approaches, besides the tutors’ resolution, also some of the most original participants’ submissions are selected;
- To provide feedback to students, the tutors’ assessment is provided though the rubric, which has explicit descriptors; moreover, detailed and personalized comments and tips are released by tutors together with the evaluation;
- To encourage self-assessment, participants receive an explanation about the importance of filling the self-assessment questionnaire. Moreover, they are rewarded with 3 DMC for each questionnaire filled.
3.2. Participants
3.3. Research Method
“In solving the problems, how much did the following aspects hinder you? Comprehension of the problematic situation; Identification of a solving strategy; Completion of the solving process; Argumentation; Generalization by using interactive components; Use of Maple.”
4. Results
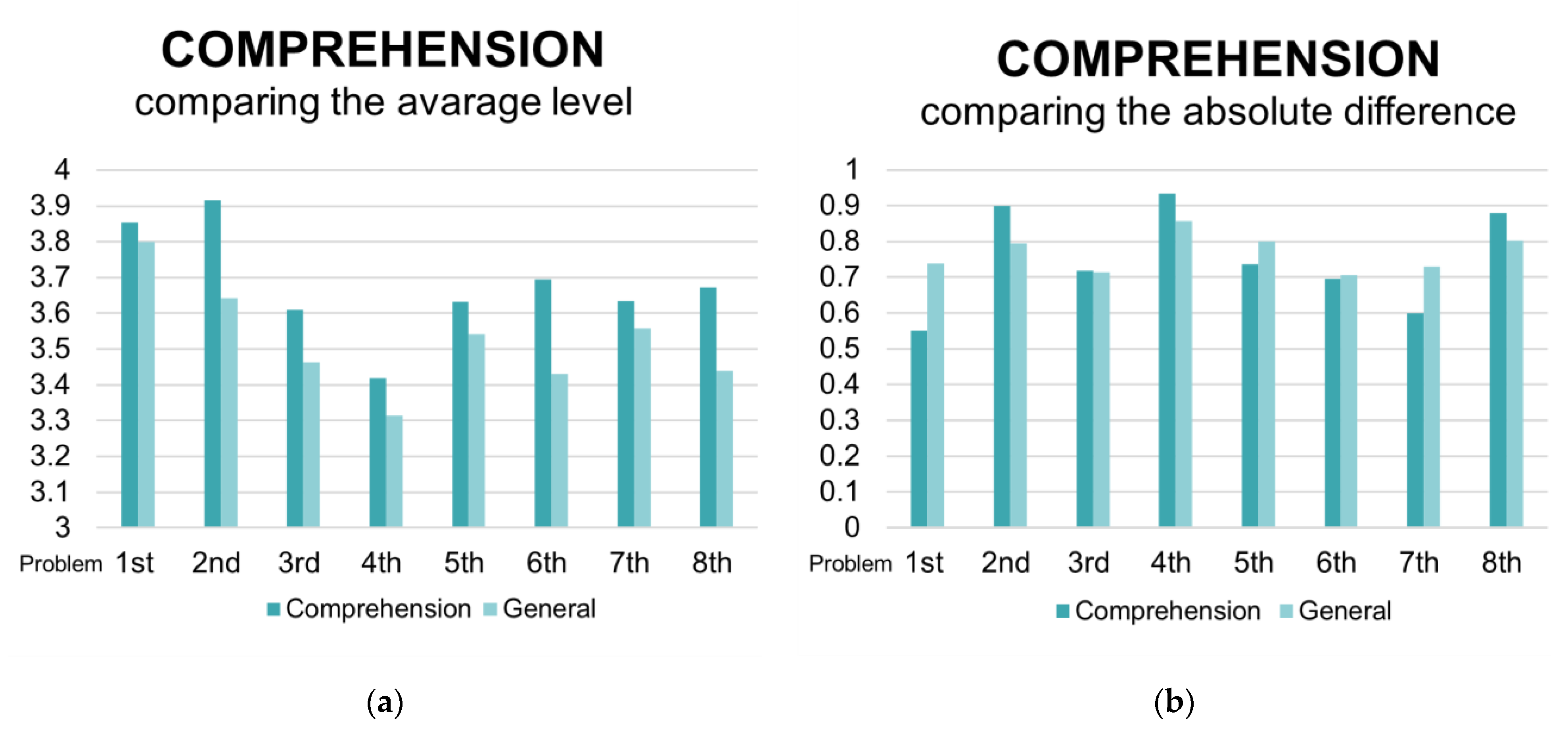
4.1. Comprehension of the Problematic Situation
“I found it difficult to select only one strategy because the text could be interpreted differently”;“The text wasn’t so clear”;“I found it more difficult to understand the text than to use Maple. That is why I tried to underline the points in the text from which the different interpretations originated and then I employed my strategy. Furthermore, I found the forum useful because other participants had my doubts and reading the answers to their posts helped me.”
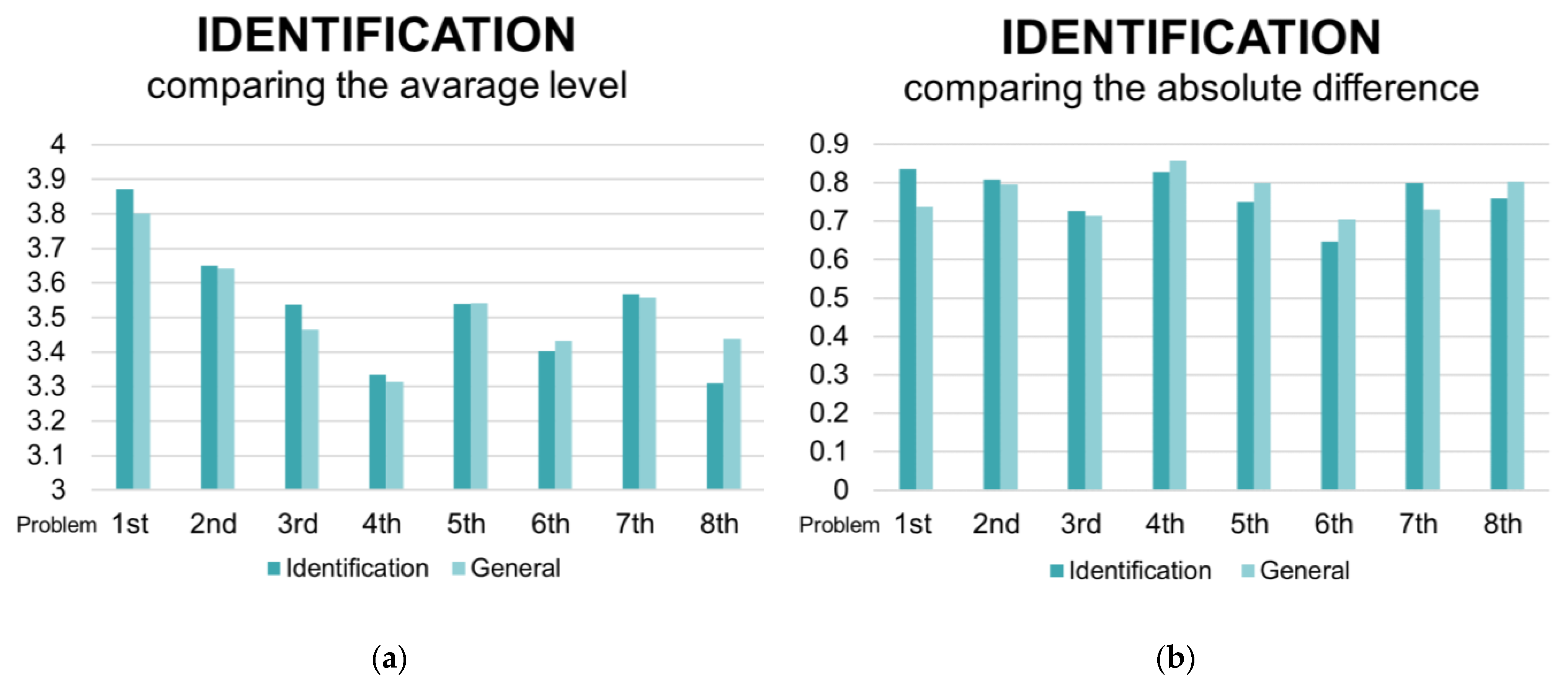
4.2. Identification of a Solving Strategy
4.3. Development of the Solving Process
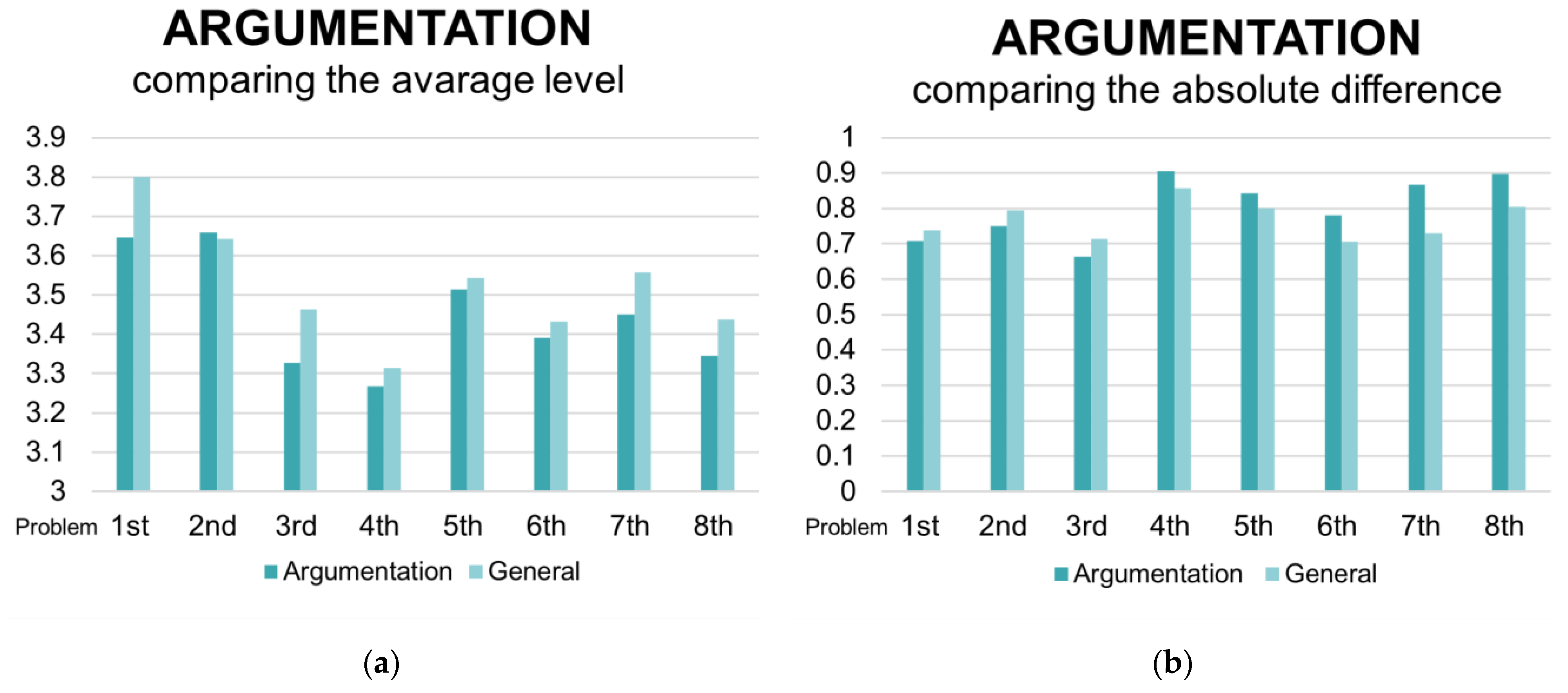
4.4. Argumentation
4.5. Use of the ACE
“I found it difficult to solve the third task. I had problems in programming the interactive components”;“I found it difficult to develop the interactive components by plotting the moving average”;“I found it difficult to understand some tasks and so I did the best I could, even though I’m not so sure I satisfied the requests”.
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
- Problem 1—Gasoline
- How many km can he travel with a liter of gasoline?
- 2.
- How much will the full tank of gas cost this once?
- 3.
- How many liters does Marco’s tank contain, if the gasoline consumption remained the same throughout the journey?
- 4.
- Assuming that the refueling stops were 305th km and at 940th km, plot the value pointed by the tank indicator of Marco’s car in terms of the crossed km.
- Problem 8—Antibiotic therapy
- If the therapy starts the first day after the medical prescription and is done properly, how many days would it take to remove both bacteria from the organism? Discuss it by using graphical representations.
- Supposing that Marco begins to feel better when only one of the two bacteria has disappeared, and he decides to stop the treatment. What happens to type A bacteria in the 3 following weeks? Does it grow more or less than it would grow if the therapy hadn’t started? Discuss it by using graphical representations.
- Create a system of interactive components which estimates how many days it would take to remove one of the 2 types of bacteria by inserting the initial concentration, the natural increment of the 2 types of bacteria, the competitive decrease (assuming they are the same), the antibiotic sensitivity (in term of the absolute decrease of concentrations). Be careful: It isn’t required to display how many days it would take to remove both bacteria.
- The other problems
Appendix B
| Indicators | Level (Score) | Description |
|---|---|---|
| Comprehension Analyze the problematic situation, represent and interpretate the data and then turn them in mathematical language | L1 (0–3) | You don’t understand the tasks, or you do incorrectly or partially, so that you fail to recognize key points and information, or you recognize some of them, but you interpret them incorrectly. You incorrectly link information, and you use mathematical codes insufficiently and/or with big mistakes. |
| L2 (4–8) | You analyze and you understand the tasks only partially, so that you select just some key points and essential information or, if you identify all of them, you make mistakes by interpreting some of them, by linking topics and/or by using mathematical codes. | |
| L3 (9–13) | You properly analyze the problematic situation by identifying and correctly interpreting the key points, the information and the links between them by recognizing and skipping distractors. You properly use the mathematical codes by employing plots and symbols, but there are some inaccuracies and/or mistakes. | |
| L4 (14–18) | You analyze and interpret the key points, the essential information and the links between them completely and in a relevant way. You are able to skip distractors and use mathematical codes by employing plots and symbols with mastery and accuracy. Even if there are some inaccuracies, these don’t influence the complex comprehension of the problematic situation. | |
| Identification of solving strategy Employ solving strategies by modeling the problem and by using the most suitable strategy | L1 (0–4) | You don’t identify operating strategies, or you identify them improperly. You aren’t able to identify relevant standard model. There isn’t any creative effort to find the solving process. You don’t establish the appropriate formal instruments. |
| L2 (5–10) | You identify operating strategies that are not very effective and sometimes you employ them not very consistently. You use known models with some difficulties. You show little creativity in setting the operating steps. You establish the appropriate formal instruments with difficulties and by doing some mistakes. | |
| L3 (11-16) | You identify operating strategies, even if they aren’t the most appropriate and efficient. You show your knowledge about standard processes and models which you learned in class, but sometimes you don’t employ them correctly. You use some original strategies. You employ the appropriate formal instruments, even though with some uncertainties. | |
| L4 (17–21) | You employ logical links clearly and with mastery. You efficiently identify the correct operating strategies. You employ known models in the best way, and you also propose some new ones. You show creativity and authenticity in employing operating steps. You carefully and accurately identify the appropriate formal instruments. | |
| Development of the solving process Solve the problematic situation consistently, completely and correctly by applying mathematical rules and by performing the necessary calculations | L1 (0–4) | You don’t implement the chosen strategies, or you implement them incorrectly. You don’t develop the solving process, or you employ it incompletely and/or incorrectly. You aren’t able to use procedures and/or theorems or you employ them incorrectly and/or with several mistakes in calculating. The solution isn’t consistent with the problem’s context. |
| L2 (5–10) | You employ the chosen strategies partially and not always properly. You develop the solving process incompletely. You aren’t always able to use procedures and/or theorems or you employ them partially correctly and/or with several mistakes in calculating. The solution is partially consistent with the problem’s context. | |
| L3 (11–16) | You employ the chosen strategy even though with some inaccuracy. You develop the solving process almost completely. You are able to use procedures and/or theorems or rules and you employ them correctly and properly. You make a few mistakes in calculating. The solution is generally consistent with the problem’s context. | |
| L4 (17–21) | You correctly employ the chosen strategy by using models and/or charts and/or symbols. You develop the solving process analytically, completely, clearly and correctly. You employ procedures and/or theorems or rules correctly and properly, with ability and originality. The solution is consistent with the problem’s context. | |
| Argumentation Explain and comment on the chosen strategy, the key steps of the building process and the consistency of the results | L1 (0–3) | You don’t argue or you argue the solving strategy/process and the test phase wrongly by using mathematical language that is improper or very inaccurate. |
| L2 (4–7) | You argue the solving strategy/process or the test phase in a fragmentary way and/or not always consistently. You use broadly suitable, but not always rigorous mathematical language. | |
| L3 (8–11) | You argue the solving process and the test phase correctly but incompletely. You explain the answer, but not the solving strategies employed (or vice versa). You use a pertinent mathematical language, although with some uncertainty. | |
| L4 (12–15) | You argue both the employed strategies and the obtained results consistently, accurately, exhaustively and in depth. You show an excellent command of the scientific language. | |
| Use of an ACE (Maple) Use the ACE commands appropriately and effectively which is the software Maple, in order to solve the problem | L1 (0–5) | You use Maple as a plain white sheet on which you transpose calculus and arguments which are somewhere else employed. You don’t use Maple’s capabilities in order to plot, to perform mathematical operations and to solve the problem. |
| L2 (6–12) | You partially use Maple’s commands in order to perform some non-basic calculus by making decisions about commands and instruments which aren’t always the most pertinent. You only use basic functions and show that you aren’t able to employ advanced features. | |
| L3 (13–19) | You appropriately use basic Maple’s commands and show that you are able to employ advanced features, even with some indecision, several attempts or by making some mistakes, or in a non-effective way in order to represent the data, the solutions and to solve the problem. | |
| L4 (20–25) | You display mastery in the use of Maple, you make correct and efficient decisions about commands and instruments which you employ. You are able to employ them gracefully and with originality by using Maple’s capabilities in order to solve the problem. |
References
- Klenowski, V. Student Self-evaluation Processes in Student-centred Teaching and Learning Contexts of Australia and England. Assess. Educ. Princ. Policy Pract. 1995, 2, 145–163. [Google Scholar] [CrossRef]
- Black, P.; Wiliam, D. Assessment and Classroom Learning. Assess. Educ. Princ. Policy Pract. 1998, 5, 7–74. [Google Scholar] [CrossRef]
- Ross, J.A. The Reliability, Validity, and Utility of Self-Assessment. Pract. Assess. Res. Eval. 2006, 11, 10. [Google Scholar] [CrossRef]
- Brookhart, S.M.; Andolina, M.; Zuza, M.; Furman, R. Minute Math: An Action Research Study of Student Self-Assessment. Educ. Stud. Math. 2004, 57, 213–227. [Google Scholar] [CrossRef]
- Nicol, D.J.; Macfarlane-Dick, D. Formative Assessment and Self-regulated Learning: A Model and Seven Principles of Good Feedback Practice. Stud. High. Educ. 2006, 31, 199–218. [Google Scholar] [CrossRef]
- Andrade, H.; Valtcheva, A. Promoting Learning and Achievement Through Self-Assessment. Theory Pract. 2009, 48, 12–19. [Google Scholar] [CrossRef]
- Tachie, S.A. Meta-Cognitive Skills and Strategies Application: How This Helps Learners in Mathematics Problem-Solving. EURASIA J. Math. Sci. Technol. Educ. 2019, 15, em1702. [Google Scholar] [CrossRef]
- Yoong, D.W.K. Helping Your Students to Become Metacognitive in Mathematics: A Decade Later. Math. Newsl. 2002, 12, 1–7. [Google Scholar]
- Schoenfeld, A. Learning to think mathematically: Problem solving, metacognition, and sense making in mathematics. In Handbook of Research on Mathematics Teaching and Learning; Macmillian: New York, NY, USA, 1992; pp. 334–370. [Google Scholar]
- Kilpatrick, J. A retrospective account of the past 25 years of research on teaching mathematical problem solving. In Teaching and Learning Mathematical Problem Solving: Multiple Research Perspectives; Silver, E.A., Ed.; Lawrence Erlbaum: Hillsdale, NJ, USA, 1985; pp. 1–15. [Google Scholar]
- Liljedahl, P.; Santos-Trigo, M.; Malaspina, U.; Bruder, R. Problem Solving in Mathematics Education; ICME-13 Topical Surveys; Springer: New York, NY, USA, 2016; ISBN 978-3-319-40729-6. [Google Scholar]
- Barana, A.; Marchisio, M. From digital mate training experience to alternating school work activities. Mondo Digit. 2016, 15, 63–82. [Google Scholar]
- Barana, A.; Brancaccio, A.; Conte, A.; Fissore, C.; Floris, F.; Marchisio, M.; Pardini, C. The Role of an Advanced Computing Environment in Teaching and Learning Mathematics through Problem Posing and Solving. In Proceedings of the 15th International Scientific Conference eLearning and Software for Education, Bucharest, Romania, 11–12 April 2019; Volume 2, pp. 11–18. [Google Scholar]
- Ninness, H.A.C.; Ninness, S.K.; Sherman, S.; Schotta, C. Augmenting Computer-Interactive Self-Assessment with and Without Feedback. Psychol. Rec. 1998, 48, 601–616. [Google Scholar] [CrossRef][Green Version]
- McDonald, B.; Boud, D. The Impact of Self-Assessment on Achievement: The Effects of Self-Assessment Training on Performance in External Examinations. Assess. Educ. Princ. Policy Pract. 2003, 10, 209–220. [Google Scholar] [CrossRef]
- Black, P.; Wiliam, D. ‘In Praise of Educational Research’: Formative Assessment. Br. Educ. Res. J. 2003, 29, 623–637. [Google Scholar] [CrossRef]
- Black, P.; Wiliam, D. Developing the Theory of Formative Assessment. Educ. Assess. Eval. Account. 2009, 21, 5–31. [Google Scholar] [CrossRef]
- Sánchez-Ruiz, L.-M.; Moll-López, S.; Moraño-Fernández, J.-A.; Roselló, M.-D. Dynamical Continuous Discrete Assessment of Competencies Achievement: An Approach to Continuous Assessment. Mathematics 2021, 9, 2082. [Google Scholar] [CrossRef]
- Castoldi, M. Le rubriche valutative. L’Educatore 2006, 5, 6–10. [Google Scholar]
- Yan, Z. Self-Assessment in the Process of Self-Regulated Learning and Its Relationship with Academic Achievement. Assess. Eval. High. Educ. 2020, 45, 224–238. [Google Scholar] [CrossRef]
- Zimmerman, B.J. Attaining Self-Regulation: A Social Cognitive Perspective. In Handbook of Self-Regulation; Boekaerts, M., Pintrich, P.R., Zeidner, M., Eds.; Elsevier: San Diego, CA, USA, 2000; pp. 13–39. [Google Scholar]
- Ross, J.A.; Hogaboam-Gray, A.; Rolheiser, C. Student Self-Evaluation in Grade 5-6 Mathematics Effects on Problem- Solving Achievement. Educ. Assess. 2002, 8, 43–58. [Google Scholar] [CrossRef]
- English, L.D.; Sriraman, B. Problem Solving for the 21st Century. In Theories of Mathematics Education; Springer: Berlin/Heidelberg, Germany, 2010; pp. 263–290. [Google Scholar]
- Schoenfeld, A. Mathematical Problem Solving; Academic Press: Cambridge, MA, USA, 1985. [Google Scholar]
- Fan, L.; Zhu, Y. From Convergence to Divergence: The Development of Mathematical Problem Solving in Research, Curriculum, and Classroom Practice in Singapore. ZDM 2007, 39, 491–501. [Google Scholar] [CrossRef]
- Chen, P.P. Relationship between Students’ Self-Assessment of Their Capabilities and Their Teachers’ Judgments of Students’ Capabilities in Mathematics Problem-Solving. Psychol. Rep. 2006, 98, 765–778. [Google Scholar] [CrossRef]
- Krathwohl, D.R. A Revision of Bloom’s Taxonomy: An Overview. Theory Pract. 2002, 41, 212–218. [Google Scholar] [CrossRef]
- Semana, S.; Santos, L. Self-Regulation Capacity of Middle School Students in Mathematics. ZDM 2018, 50, 743–755. [Google Scholar] [CrossRef]
- National Council of Teachers of Mathematics. Executive Summary Principles and Standards for School Mathematics; National Council of Teachers of Mathema: Reston, VA, USA, 2000. [Google Scholar]
- Council of the European Union. European Parliament and Council Council Recommendation of 22 May 2018 on Key Competences for Lifelong Learning. Off. J. Eur. Union 2018, C 189/1, 1–13.
- Pólya, G. How to Solve It; Princeton University: Princeton, NJ, USA, 1945. [Google Scholar]
- D’Amore, B.; Pinilla, M.I.F. Che problema i problemi! L’insegnamento Della Mat. E Delle Sci. Integr. 2006, 6, 645–664. [Google Scholar]
- Samo, D.D.; Darhim, D.; Kartasasmita, B. Culture-Based Contextual Learning to Increase Problem-Solving Ability of First Year University Student. J. Math. Educ. 2017, 9, 81–94. [Google Scholar] [CrossRef]
- Bonotto, C.; Baroni, M. Using maths in a daily context: Experiences in Italian compulsory education. In Planting Mathematics, Publication of the Comenius Network Developing Quality in Mathematics Education II—DQME II; Henn, H.W., Meier, S., Eds.; TU Dortmund: Dortmund, Germany, 2006; pp. 19–47. [Google Scholar]
- Acevedo Nistal, A.; Dooren, W.; Clarebout, G.; Elen, J.; Verschaffel, L. Conceptualising, Investigating and Stimulating Representational Flexibility in Mathematical Problem Solving and Learning: A Critical Review. ZDM 2009, 41, 627–636. [Google Scholar] [CrossRef]
- Silver, E.A. Fostering Creativity through Instruction Rich in Mathematical Problem Solving and Problem Posing. ZDM 1997, 29, 75–80. [Google Scholar] [CrossRef]
- Olivares, D.; Lupiáñez, J.L.; Segovia, I. Roles and Characteristics of Problem Solving in the Mathematics Curriculum: A Review. Int. J. Math. Educ. Sci. Technol. 2021, 52, 1079–1096. [Google Scholar] [CrossRef]
- Toh, T.L.; Chan, C.M.E.; Tay, E.G.; Leong, Y.H.; Quek, K.S.; Toh, P.C.; Ho, W.K.; Dindyal, J.; Ho, F.H.; Dong, F. Problem Solving in the Singapore School Mathematics Curriculum. In Mathematics Education in Singapore; Toh, T.L., Kaur, B., Tay, E.G., Eds.; Mathematics Education—An Asian Perspective; Springer: Singapore, 2019; pp. 141–164. ISBN 9789811335723. [Google Scholar]
- Yap, R.A.S.; Leong, Y.H. Using Video Clubs to Learn for Mathematical Problem-Solving Instruction in the Philippines: The Case of Teaching Extensions. In Cases of Mathematics Professional Development in East Asian Countries; Ng, S.F., Ed.; Springer: Singapore, 2015; pp. 83–106. ISBN 978-981-287-404-7. [Google Scholar]
- Leong, Y.H.; Tay, E.G.; Toh, T.L.; Quek, K.S.; Dindyal, J. Reviving Pólya’s “Look Back” in a Singapore School. J. Math. Behav. 2011, 30, 181–193. [Google Scholar] [CrossRef]
- Carreira, S.; Jones, K.; Amado, N.; Jacinto, H.; Nobre, S. Youngsters Solving Mathematical Problems with Technology; Mathematics Education in the Digital Era; Springer: Cham, Switzerland, 2016; Volume 5, ISBN 978-3-319-24908-7. [Google Scholar]
- Lesh, R.; Lehrer, R. Models and Modeling Perspectives on the Development of Students and Teachers. Math. Think. Learn. 2003, 5, 109–129. [Google Scholar] [CrossRef]
- Santos-Trigo, M.; Moreno-Armella, L.; Camacho-Machín, M. Problem Solving and the Use of Digital Technologies within the Mathematical Working Space Framework. ZDM 2016, 48, 827–842. [Google Scholar] [CrossRef]
- Artigue, M. Instrumentation Issues and the Integration of Computer Technologies into Secondary Mathematics Teaching. In Proceedings of the Annual Meeting of the GDM, Potsdam, Germany; 2000; pp. 7–17. Available online: http://webdoc.sub.gwdg.de/ebook/e/gdm/2000/artigue_2000.pdf (accessed on 19 January 2022).
- Kuzniak, A.; Parzysz, B.; Vivier, L. Trajectory of a Problem: A Study in Teacher Training. Math. Enthus. 2013, 10, 407–440. [Google Scholar] [CrossRef]
- Moreno-Armella, L.; Santos-Trigo, M. The use of digital technologies in mathematical practices: Reconciling traditional and emerging approaches. In Handbook of International Research in Mathematics Education; English, L.D., Kirshner, D., Eds.; Taylor & Francis: New York, NY, USA, 2016; pp. 595–616. [Google Scholar]
- Avitzur, R. Graphing Calculator (Version 4.0); Pacific Tech.: Berkeley, CA, USA, 2011. [Google Scholar]
- Dimiceli, V.A.; Lang, A.S.I.D.; Locke, L.A. Teaching Calculus with Wolfram|Alpha. Int. J. Math. Educ. Sci. Technol. 2010, 41, 1061–1071. [Google Scholar] [CrossRef]
- Hohenwarter, M.; Hohenwarter, J.; Kreis, Y.; Lavicza, Z. Teaching and Learning Calculus with Free Dynamic Mathematics Software GeoGebra. In Proceedings of the 11th International Congress on Mathematics Education, Monterrey, Mexico, 6–13 July 2008; pp. 1–10. [Google Scholar]
- Artigue, M. Learning Mathematics in a CAS Environment: The Genesis of a Reflection about Instrumentation and the Dialectics between Technical and Conceptual Work. Int. J. Comput. Math. Learn. 2002, 7, 245–274. [Google Scholar] [CrossRef]
- Barana, A.; Marchisio, M. Analyzing Interactions in Automatic Formative Assessment Activities for Mathematics in Digital Learning Environments. In Proceedings of the 13th International Conference on Computer Supported Education, SCITEPRESS, Online, 23–25 April 2021; Volume 1, pp. 497–504. [Google Scholar]
- Barana, A.; Marchisio, M.; Sacchet, M. Interactive Feedback for Learning Mathematics in a Digital Learning Environment. Educ. Sci. 2021, 11, 279. [Google Scholar] [CrossRef]
- Beevers, C.E.; Paterson, J.S. Automatic Assessment of Problem Solving Skills in Mathematics. Act. Learn. High. Educ. 2003, 4, 127–144. [Google Scholar] [CrossRef]
- Goos, M.; Galbraith, P.; Renshaw, P.; Geiger, V. Perspectives on Technology Mediated Learning in Secondary School Mathematics Classrooms. J. Math. Behav. 2003, 22, 73–89. [Google Scholar] [CrossRef]
- Barana, A.; Fissore, C.; Marchisio, M.; Roman, F. Enhancement of Mathematical Problem Solving by Discussing and Collaborating Asynchronously. In Proceedings of the Edulearn20 Conference, Online, 6–7 July 2020; pp. 2827–2836. [Google Scholar]
- Tan, D.Y.; Cheah, C.W. Developing a Gamified AI-Enabled Online Learning Application to Improve Students’ Perception of University Physics. Comput. Educ. Artif. Intell. 2021, 2, 100032. [Google Scholar] [CrossRef]
- Kang, K.; Kushnarev, S.; Wei Pin, W.; Ortiz, O.; Chen Shihang, J. Impact of Virtual Reality on the Visualization of Partial Derivatives in a Multivariable Calculus Class. IEEE Access 2020, 8, 58940–58947. [Google Scholar] [CrossRef]
- Buentello-Montoya, D.A.; Lomelí-Plascencia, M.G.; Medina-Herrera, L.M. The Role of Reality Enhancing Technologies in Teaching and Learning of Mathematics. Comput. Electr. Eng. 2021, 94, 107287. [Google Scholar] [CrossRef]
- Kushnarev, S.; Kang, K.; Goyal, S. Assessing the Efficacy of Personalized Online Homework in a First-Year Engineering Multivariate Calculus Course. In Proceedings of the 2020 IEEE International Conference on Teaching, Assessment, and Learning for Engineering (TALE), Takamatsu, Japan, 8–11 December 2020; pp. 770–773. [Google Scholar]
- Cheong, K.H.; Koh, J.M. Integrated Virtual Laboratory in Engineering Mathematics Education: Fourier Theory. IEEE Access 2018, 6, 58231–58243. [Google Scholar] [CrossRef]
- Cheong, K.H.; Koh, J.M.; Yeo, D.J.; Tan, Z.X.; Boo, B.O.E.; Lee, G.Y. Paradoxical Simulations to Enhance Education in Mathematics. IEEE Access 2019, 7, 17941–17950. [Google Scholar] [CrossRef]
- Comoglio, M. Le Nuove Prospettive Della Valutazione Scolasica. L’Educatore 2008, 11, 29–34. [Google Scholar]
- Leong, Y.H.; Janjaruporn, R. Teaching of Problem Solving in School Mathematics Classrooms. In The Proceedings of the 12th International Congress on Mathematical Education; Cho, S.J., Ed.; Springer: Cham, Switzerland, 2015; pp. 645–648. ISBN 978-3-319-10685-4. [Google Scholar]
- Comoglio, M. La Valutazione Autentica. Orientamenti Pedagog. 2002, 49, 93–112. [Google Scholar]
- Maple. Available online: https://www.maplesoft.com/products/Maple/ (accessed on 7 August 2019).
- Pajares, F.; Miller, M.D. Mathematics Self-Efficacy and Mathematics Performances: The Need for Specificity of Assessment. J. Couns. Psychol. 1995, 42, 190–198. [Google Scholar] [CrossRef]
- Ewers, C.A.; Wood, N.L. Sex and Ability Differences in Children’s Math Self-Efficacy and Prediction Accuracy. Learn. Individ. Differ. 1993, 5, 259–267. [Google Scholar] [CrossRef]
- Sriraman, B.; Umland, K. Argumentation in Mathematics Education. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: Dordrecht, Germany, 2014; pp. 46–48. ISBN 978-94-007-4977-1. [Google Scholar]
- Cusi, A.; Olsher, S. Design of Classroom Discussions and the Role of the Expert in Fostering an Effective and Aware Use of Examples as a Means of Argumentation. Int. J. Sci. Math. Educ. 2021. [Google Scholar] [CrossRef]
- Boero, P. Argumentation and Mathematical Proof: A Complex, Productive, Unavoidable Relationship in Mathematics and Mathematics Education. Int. Newsl. Teach. Learn. Math. Proof 1999, 7, 8. [Google Scholar]


| Number of Students | Comprehension | Identification | Development | Argumentation | Use of Maple | |
|---|---|---|---|---|---|---|
| 1st problem | 116 | +0.48 | +0.78 | +0.65 | +0.43 | +0.83 |
| 2nd problem | 120 | +0.83 | +0.64 | +0.45 | +0.45 | +0.57 |
| 3rd problem | 110 | +0.66 | +0.67 | +0.54 | +0.23 | +0.60 |
| 4th problem | 105 | +0.67 | +0.56 | +0.51 | +0.60 | +0.53 |
| 5th problem | 76 | +0.53 | +0.59 | +0.49 | +0.50 | +0.68 |
| 6th problem | 82 | +0.65 | +0.38 | +0.37 | +0.39 | +0.57 |
| 7th problem | 60 | +0.57 | +0.73 | +0.58 | +0.70 | +0.60 |
| 8th problem | 58 | +0.64 | +0.38 | +0.41 | +0.52 | +0.62 |
| Pearson Coefficient | p-Value | |
|---|---|---|
| 1st problem | 0.407 | <0.001 |
| 2nd problem | 0.259 | 0.004 |
| 3rd problem | 0.731 | <0.001 |
| 4th problem | 0.535 | <0.001 |
| 5th problem | 0.556 | <0.001 |
| 6th problem | 0.615 | <0.001 |
| 7th problem | 0.735 | <0.001 |
| 8th problem | 0.515 | <0.001 |
| Pearson Coefficient | p-Value | |
|---|---|---|
| 1st problem | 0.356 | <0.001 |
| 2nd problem | 0.074 | 0.422 |
| 3rd problem | 0.652 | <0.001 |
| 4th problem | 0.379 | <0.001 |
| 5th problem | 0.404 | <0.001 |
| 6th problem | 0.483 | <0.001 |
| 7th problem | 0.662 | <0.001 |
| 8th problem | 0.228 | 0.086 |
| Students’ Answers | Mean | Standard Deviation |
|---|---|---|
| 1 | 0.4143 | 0.15278 |
| 2 | 0.7352 | 0.49100 |
| 3 | 0.8014 | 0.46268 |
| 4 | 0.8586 | 0.61427 |
| 5 | 0.8542 | 0.51875 |
| Pearson Coefficient | p-Value | |
|---|---|---|
| 1st problem | 0.243 | 0.009 |
| 2nd problem | 0.200 | 0.029 |
| 3rd problem | 0.639 | <0.001 |
| 4th problem | 0.411 | <0.001 |
| 5th problem | 0.509 | <0.001 |
| 6th problem | 0.468 | <0.001 |
| 7th problem | 0.653 | <0.001 |
| 8th problem | 0.421 | 0.001 |
| Students’ Answers | Mean | Standard Deviation |
|---|---|---|
| 1 | 0.3452 | 0.13521 |
| 2 | 0.6847 | 0.44823 |
| 3 | 0.8374 | 0.42753 |
| 4 | 0.9793 | 0.31618 |
| 5 | - | - |
| Pearson Coefficient | p-Value | |
|---|---|---|
| 1st problem | 0.300 | 0.001 |
| 2nd problem | 0.277 | 0.002 |
| 3rd problem | 0.632 | <0.001 |
| 4th problem | 0.460 | <0.001 |
| 5th problem | 0.518 | <0.001 |
| 6th problem | 0.553 | <0.001 |
| 7th problem | 0.740 | <0.001 |
| 8th problem | 0.465 | <0.001 |
| Pearson Coefficient | p-Value | |
|---|---|---|
| 1st problem | 0.269 | 0.004 |
| 2nd problem | 0.182 | 0.046 |
| 3rd problem | 0.525 | <0.001 |
| 4th problem | 0.488 | <0.001 |
| 5th problem | 0.307 | 0.007 |
| 6th problem | 0.256 | 0.020 |
| 7th problem | 0.576 | <0.001 |
| 8th problem | 0.346 | 0.008 |
| Students’ Answers | Mean | Standard Deviation |
|---|---|---|
| 1 | 0.4571 | 0.37319 |
| 2 | 0.8515 | 0.49796 |
| 3 | 0.7836 | 0.47839 |
| 4 | 0.9877 | 0.50577 |
| 5 | 0.7143 | 0.62270 |
| Pearson Coefficient | p-Value | |
|---|---|---|
| 1st problem | 0.390 | <0.001 |
| 2nd problem | 0.322 | <0.001 |
| 3rd problem | 0.609 | <0.001 |
| 4th problem | 0.469 | <0.001 |
| 5th problem | 0.477 | <0.001 |
| 6th problem | 0.488 | <0.001 |
| 7th problem | 0.740 | <0.001 |
| 8th problem | 0.465 | <0.001 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barana, A.; Boetti, G.; Marchisio, M. Self-Assessment in the Development of Mathematical Problem-Solving Skills. Educ. Sci. 2022, 12, 81. https://doi.org/10.3390/educsci12020081
Barana A, Boetti G, Marchisio M. Self-Assessment in the Development of Mathematical Problem-Solving Skills. Education Sciences. 2022; 12(2):81. https://doi.org/10.3390/educsci12020081
Chicago/Turabian StyleBarana, Alice, Giulia Boetti, and Marina Marchisio. 2022. "Self-Assessment in the Development of Mathematical Problem-Solving Skills" Education Sciences 12, no. 2: 81. https://doi.org/10.3390/educsci12020081
APA StyleBarana, A., Boetti, G., & Marchisio, M. (2022). Self-Assessment in the Development of Mathematical Problem-Solving Skills. Education Sciences, 12(2), 81. https://doi.org/10.3390/educsci12020081








