Teaching Mathematics to Non-Mathematics Majors through Problem Solving and New Technologies

Abstract

The role of mathematics in several scientific disciplines is undisputed; work and everyday life take great advantage of its application. Nevertheless, students often tend to not particularly like it and to consider it of little interest. It is also believed that only people with a certain attitude are capable of mastering the subject. In consideration of this, we aimed to help science students develop mathematical competences by designing a course specifically oriented to applications and problem solving. We administered our course to students attending the first year of a program in biotechnology, asking them to work with technologies instilling curiosity and interest, thus achieving a better proficiency as a consequence. Two questionnaires, along with access and proficiency data, allowed us to collect information about students’ attitudes, beliefs, and activity, which we analyzed by means of descriptive statistics. The promotion of the interaction among learners made them active users of the contents, thus allowing for the adaptation of their learning paths according to their personal necessities, as well as the development of teamwork skills and flexibility. Finally, students recognized the usefulness of the problem-solving approach and the role played by software.

1. Introduction

The COVID-19 pandemic highlighted the use of technologies, such as learning management systems (LMS), advanced computing environments (ACE), and automated assessment systems (AAS). They became pivotal in distance education and integrated into a digital learning environment, thanks to methodologies such as formative assessment [1,2]. Their use has been underway since well before the beginning of the health emergency, given, on one hand, that the need for remote teaching and learning has its roots in the organization of university life itself, for example, to deal with overlapping timetables or courses provided in places that might be difficult to reach. On the other hand, these tools are used also during in-person activities, usually in a lab or at a location that slightly differs from the traditional classroom in which lectures take place. These instruments are even more important when used in a collateral subject, as is the case of STEM specialties for students whose curriculum is not strictly based on the main STEM fields; this applies particularly to mathematics but also to related subjects, such as computer science [3]. Indeed, a difficulty arises when students consider these topics secondary compared to the main areas relative to the degree course they chose. In this case, they will study such topics only to pass the required exams and to move forward with their studies, regardless of gaining a real mastery of the topic, which would be the true goal of the module within the degree program. The poor motivation that often accompanies this state of mind can also lead students to postpone these exams. If a STEM exam is planned for the first year of a certain degree course, as is often the case, it can be put off by students and become one of the last courses to be taken before graduating, even if this means a two-year delay or more. As a borderline case, students may end up repeatedly failing one or more exams, to an extent that they decide to withdraw from the whole degree course, completely rethinking—or even giving up—their university career. Stakeholders may be led to the conclusion that STEM subjects can be handled superficially in these degree courses because having difficulties in understanding them is believed to be inevitable for some learners. This does not help the students to become aware of their importance.

In the present article, we formulate the following research question: how can we help the students of a scientific program that is not mainly focused on mathematics develop mathematical competences? For this purpose, we consider a course that was provided during the first semester of the academic year 2019–2020, starting in September 2019 and ending in January 2020. It is named “Mathematics and Biostatistics with applications relative to Computer Science”, and it is provided to students from the first year of a degree course in biotechnologies belonging to the Department of Molecular Biotechnology and Health Sciences. In order to overcome the above-described difficulties and in light of some theoretical concepts in mathematics education, such as affect, attitude, and beliefs, an equilibrium between theory, practice, and applications is set, on the one side using the problem-solving approach, consisting in starting from real contexts and then modeling them into mathematical problems. At the same time, it is considered how today, numerical and symbolic models are devised with the support of computers for computations, thus requiring a correct use of digital tools, therefore also awarding computer science a role in the course. This allows students to develop mathematical and related competences, knowledge, and abilities useful in the applications that they have to study. Since they were evaluated face to face, with the first exam calls of the course taking place in January and February 2020, before the outbreak of the pandemic in Italy that forced closures from the end of February onwards, it was possible to assess them as planned at the beginning of the course. Both this summative evaluation and the study itself, along with its implications, such as the enhancement of the capabilities acquired, showed a significant improvement, thus confirming that the described approach is effective. This paper, which is an extension of the work originally presented in the 2020 IEEE 44th Annual Computers, Software, and Applications Conference (COMPSAC) [4], is structured as follows. Section 2 depicts some theoretical frameworks concerning the main topics relative to our study. In Section 3, we present the research question and describe the methodology applied, while in Section 4, we describe the course we provided. Section 5 is devoted to the presentation of the results, with their discussion constituting Section 6. Finally, in Section 7 some final remarks are presented.

2. Materials and Methods

2.1. Blended Learning

Recent advances in learning techniques include the concept of blended learning, which has raised interest among both researchers and teachers. More than a definition exists for it; one among the most widespread ones defines this practice as follows. Blended learning is an approach to education in which a student learns, at least partially, through the delivery of content and instruction via digital and online media, where students can control some elements of their track, relative to time, place, path, or pace. On the other side, the student learns at least partially in a supervised brick-and-mortar location away from home, provided that the modalities along each student’s learning path within a course or subject are connected in an integrated learning experience [5,6]. Depending on the specific subject, the steady state between online and personal interaction can be different, thus prompting different interpretations of the blended learning concept concerning how teachers optimally relate to students within their specialty and vice versa [7]. Some synonyms of blended learning that have been proposed are hybrid learning, personalized learning, technology-enabled learning, technology-enhanced learning, and differential instruction. Each of these synonyms underlines a different aspect of blended learning [8]. The focus of blended learning is often directed to technological aspects, such as learning design and course delivery (some authors even proposed the form blended teaching), although the involvement of mindset and pedagogy, central factors for learning itself, also have to be taken into account [9,10,11]. All of this agrees with UNESCO’s Sustainable Goals [12], thanks to the improved availability of internet connections and mobile devices, which has characterized these past few years. Indeed, accessible and equitable quality education, along with lifelong learning opportunities for everyone across all learning modalities, both formal and informal, is thus possible in large areas of the world. While this is crucial in developing countries, it is also very important in developed contexts. Young people are encouraged to see the internet not only as a means of fun, but firstly as a valuable resource for developing knowledge, competences, and skills, as well as a tool to build their future as citizens and as workers [13].

2.2. STEM Education

The various subjects constituting the STEM acronym (science, technology, engineering, mathematics), are not distinct blocks, but they are rather intertwined in almost every application in which they are used. For example, it is impossible to think about the practical implications of several sciences without the applied role of engineering; on the other hand, engineers require scientists for the very making of their specialties, a basic example being the mathematics required at the beginning of every undergraduate course in Engineering. If we consider a commercial electronic device, for example, a smartphone, a tablet, or a computer, its manufacturing is possible only using both theoretical and practical aspects of the STEM paradigm. Therefore, interdisciplinary integration of these subjects is essential to reduce the gap between education and job-market requirements. Concerning the latter, it has to be noted how the beginning of a career in a STEM job generally results in higher earnings compared to the majority of other jobs. Nevertheless, this is not always sufficient to foster specific interest in the specialties, even when good salaries are accompanied by desirable possibilities to advance more rapidly than in other areas [14]. In order to make STEM subjects more attractive—because there is often a need for workers in the area—it is important to educate pupils in STEM from the very first stages of their education. Indeed, children are more eager to gain interest in new things compared to adolescents, who may already have a clear idea about what they are planning to do in the future. Concerning secondary education, a considerable number of students follow learning paths in high school that are not scientific by preferring, for example, a humanistic, linguistic, or artistic path, and then enroll in a scientific course at university. Because the preparation is inevitably different, in that the nonscientific high schools are at least less detailed, it is important to help those students fill possible gaps as soon as possible as they begin their university path, thus enabling them to reach their goals, even when starting from a lower level. In recent years, our university has performed various research projects on new technologies in STEM teaching and learning [1], with the development of a model of automatic formative assessment, extensively tested in the context of secondary education.

2.3. Applications and Problem Solving

By expanding on what we said in Section 1 regarding some misconceptions about the consideration of mathematics, we can add that one possible reason for students’ low interest is the fact that mathematics is considered of little use in real life. Indeed, students may believe that this subject is mainly of theoretical interest, with only a part of it taking shape as practical applications. This also fuels the idea that mathematics is “difficult”, a consideration that is also often heard from the general audience outside school and academic contexts. Some of the specialties belonging to other areas, which could be those constituting the main topics of the course the students are attending, may, on the contrary, be more difficult than mathematics, but their perception is biased by the apparent ease of putting them into practice. To deal with this hindrance, interest can be fueled by bringing students to the true meaning of what they are studying. This can lead to higher attentiveness because it can refer to dynamics that they are interested in understanding and for which mathematical tools can be used to give a proper description. If students are given concrete points from which to start related to real life and closer to the main specialties of their course of study, they can be more easily introduced to more abstract concepts, such as prominently mathematical concepts [15,16]. By giving proper attention to the relations between mathematics and the main subjects of the non-mathematical program involved, it is possible to present the aspects of the subject that are most attractive for the students, thus making them fond of the specific applications. For example, a general approach could involve electronics, which is of common use among a large section of society, including smartphones, tablets, or infotainment systems. Given that their functioning essentially based on mathematics, students would be able to appreciate how the subject influences these tools, which are ubiquitous in their lives. For scientific programs, it is possible to refer to the numerous relations between mathematics and other sciences. As examples, biologists could discover what biomathematics is and its importance in the study of the evolution of populations; geologists could analyze how to find, from a mathematical point of view, the hypocenter and the epicenter of an earthquake, along with the computation of its magnitude; and so on.

2.4. Affect, Attitude, and Beliefs in Mathematics

The premises on which the previous subsection is based can be formalized in the context of mathematical education by the concepts of affect, attitude, and beliefs. Although their general meaning is clear, the way they relate to learning has been the subject of an extensive debate. In [17], a survey relative to the construct of attitude, as developed in more than 60 years of studies, is presented. By following the framework of [18], the constructs of beliefs and emotions are recalled as crucial in order to conceptualize the research on affect in mathematics education. It is stated how, in order to obtain the best impact on all the stakeholders, affective issues have to be considered with more importance relative to how much they were taken into account in previous research. In general, studies agree on the fact that the relationship between a certain belief and the consequent emotions cannot be reduced as a simple cause-and-effect scheme, but it has to be characterized with more individuality [19], with the aim of understanding the reasons beyond the intentional actions of an individual in the mathematical context [20]. This has consequences for research regarding possible reasons for low motivation. If on the one hand, it is possible to successfully look for causes, as we have done, determining a set in which every cause lies is not a straightforward process. [21] presents consistent research on motivation by analyzing various convergent perspectives. A cause of low motivation presented therein is learned helplessness, the perception of the unattainability of success due to lacking in previous successes and the belief that only lack of ability causes failures, which can be the case of university students already facing difficulties in mathematics during high school. Another cause is related to gender differences. Although such issues have been progressively decreasing thanks to social progress, some girls still sometimes believe that mathematics is better handled by boys in terms of predisposition, thus giving up the effort they would be capable of making. This is part of a broader problem that goes well beyond gender. Often, various factors, both social and environmental, bring groups of students to view mathematics from a biased perspective, influencing them in such a way that they see their relation with motivation as essentially negative. This issue extends, with some differences, to other STEM specialties, such as physics [22].

3. Methodology

The methodology for the present study was guided by the research question from Section 1, focusing on how a scientific program not mainly focused on mathematics can support the development of mathematical competences. In the following subsections, we will go into detail about the approaches, strategies, and tools adopted for the blended learning course in mathematics. We will introduce learning management systems (LMS), advanced computing environments (ACE), and automatic assessment systems (AAS). These allowed us also to retrieve the data we needed to carry out our analyses. First of all, we developed two questionnaires, administering the first one at the beginning of the course and the second one at the end. They had several questions in common: a self-evaluation about the problem-solving approach, the use of an AAS, and students’ perception of their competences were proposed both before and after the course in order to oversee students’ learning path. The questions for which we considered the evolution of their answers were:

(i)

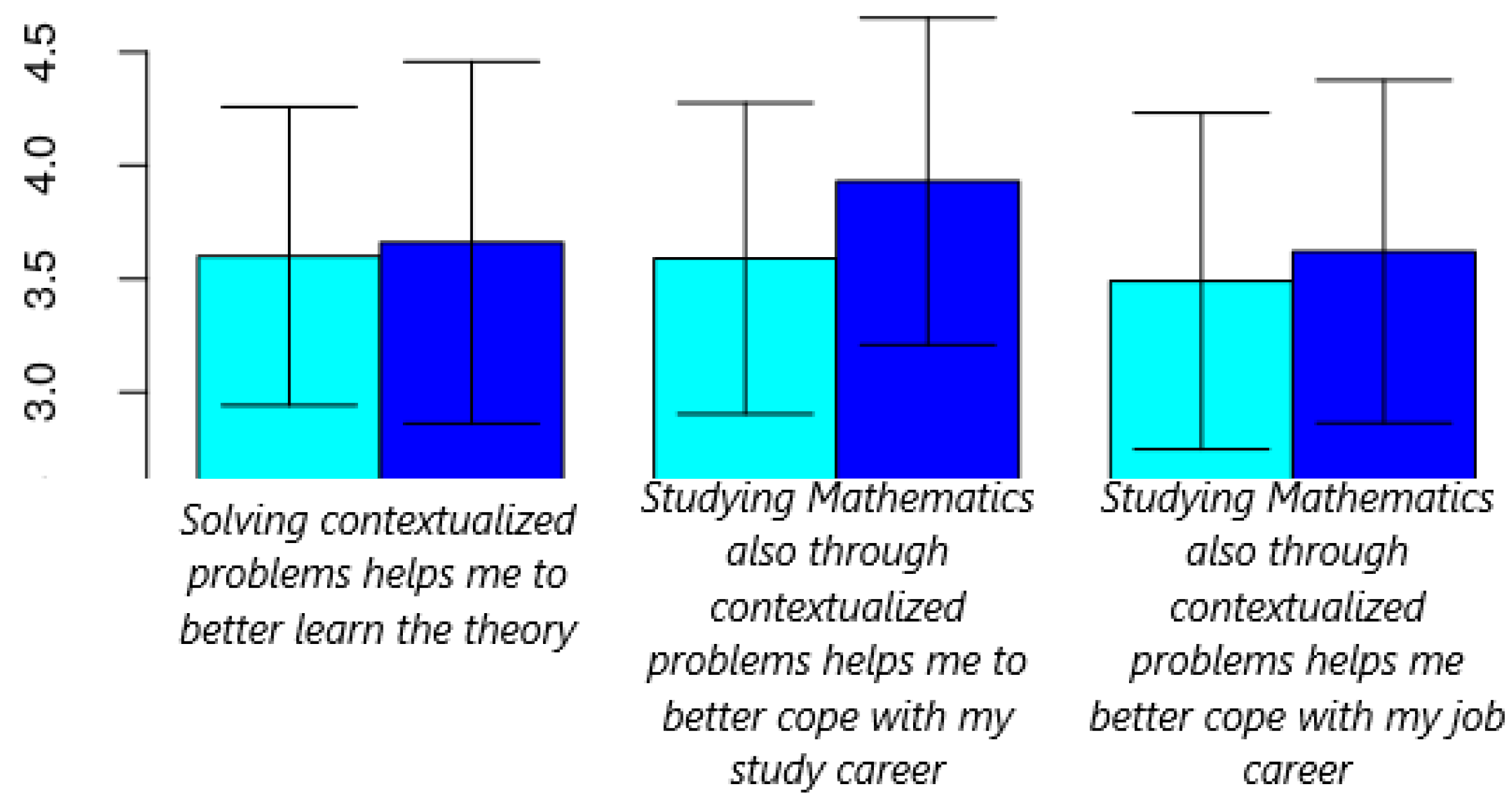
Concerning the problem-solving approach, how much do you identify yourself with the following statements:

• Solving contextualized problems helps me to better learn the theory;
• Studying mathematics through contextualized problems also helps me to better cope with my academic career;
• Studying mathematics through contextualized problems also helps me better cope with my job career.

(ii)

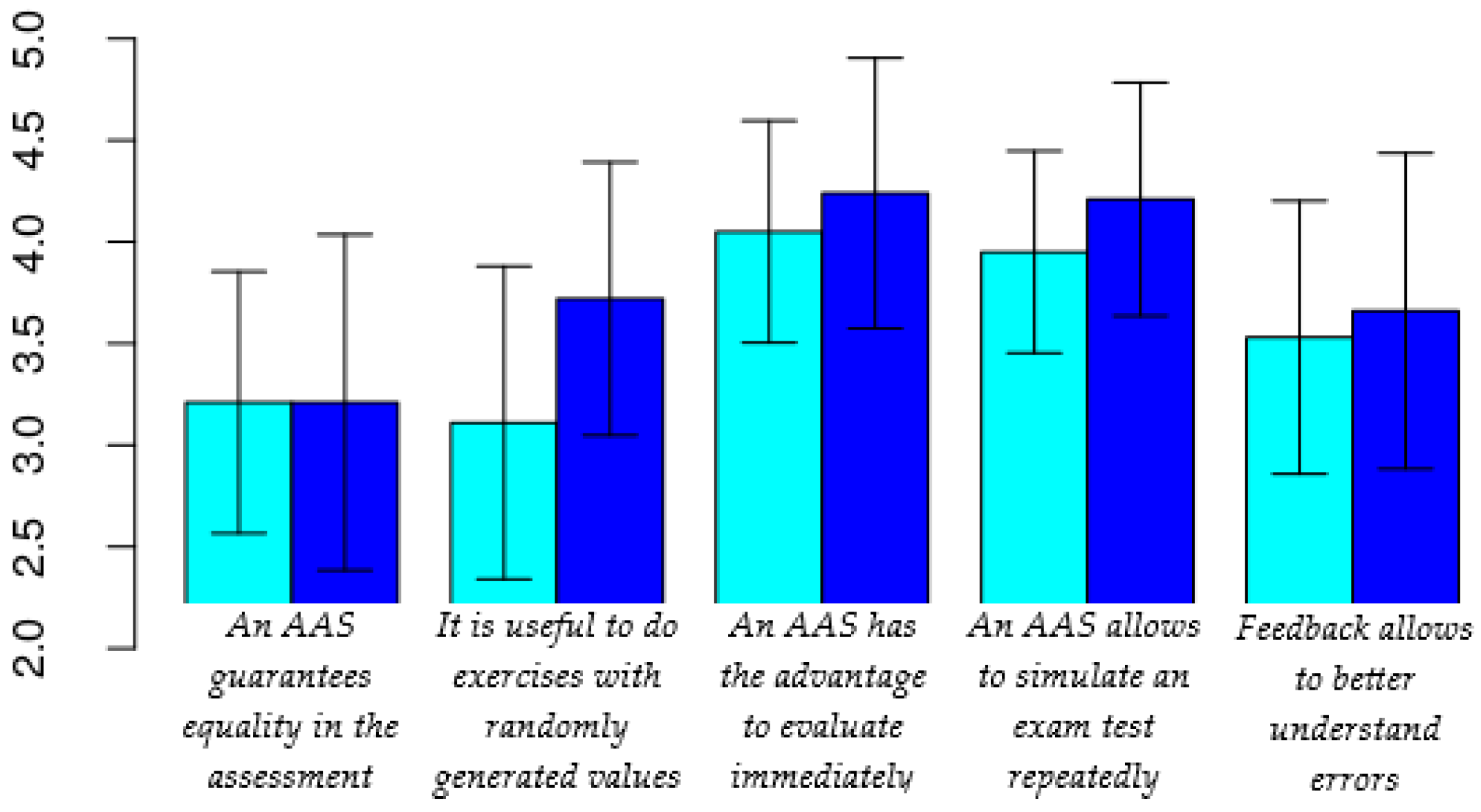
How much do you agree with the following statements concerning the use of an automated assessment system, AAS?

• An AAS guarantees equality in the assessment;
• It is useful to do exercises with randomly generated values;
• An AAS has the advantage to evaluate immediately;
• An AAS allows for repeated simulation of an exam;
• Feedback allows for better understanding of errors.

(iii)

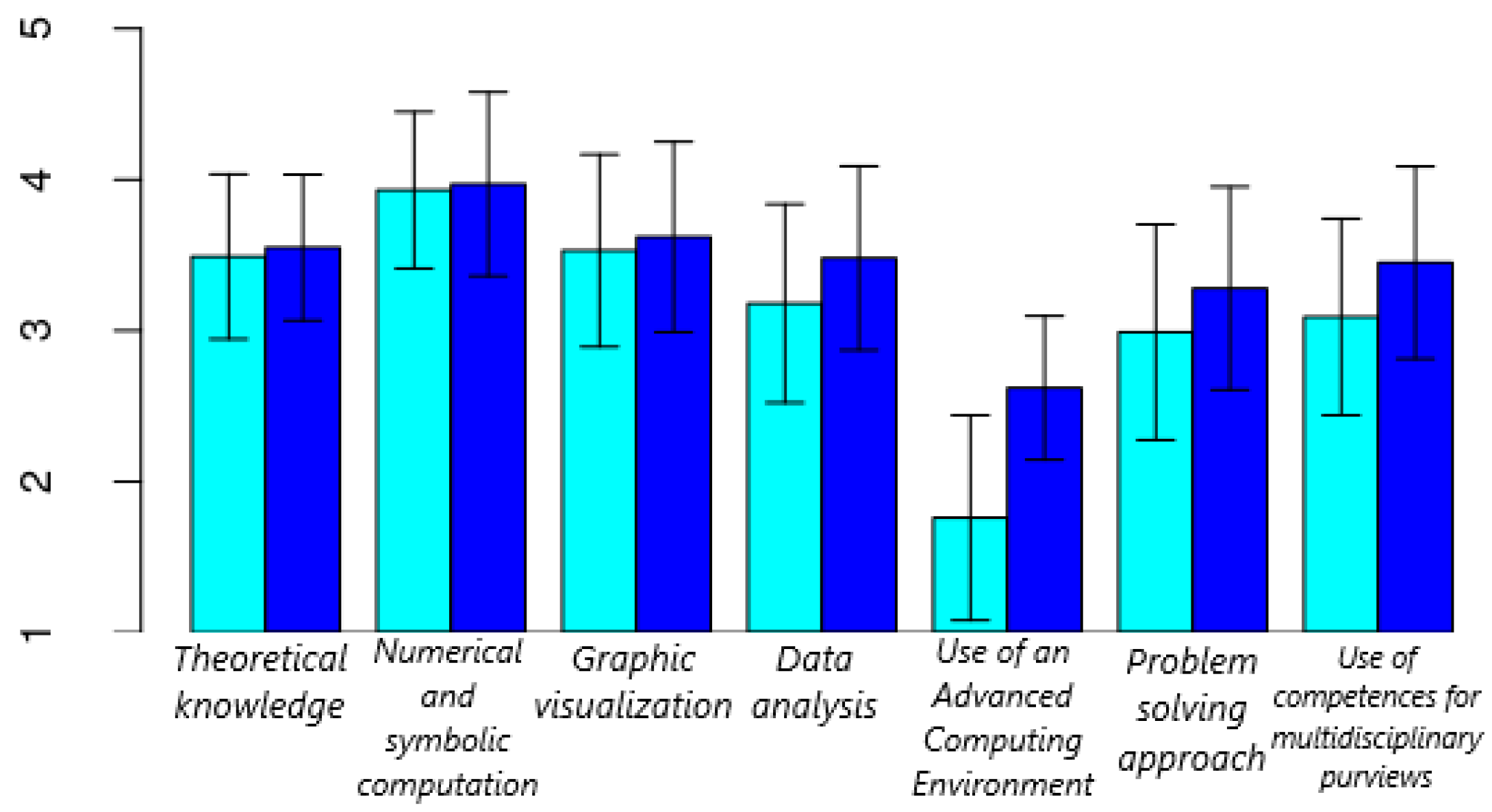
How would you assess, in the following areas, your competences in mathematics and statistics after your studies in secondary school?

• Theoretical knowledge;
• Numerical and symbolic computation;
• Graphic visualization;
• Data analysis;
• Use of an advanced computing environment;
• Problem-solving approach;
• Use of competences for multidisciplinary purviews.

Furthermore, the second questionnaire also asked questions relative to the subject and the topics of the course: mathematics, statistics, computer science, problem-solving approach. Moreover, the analyses relative to how students responded to the proposed activities were developed by using data from both the LMS and the AAS, with ACE resources playing an important role as key items based on which to analyze users and access. Finally, the AAS was pivotal for grading and analyzing performance.

The research was designed with a mixed-method approach [23] in order to better understand the situation thanks to the incorporation of qualitative aspects in a mainly quantitative setting; more specifically, a sequential explanatory strategy was followed in which some qualitative data support quantitative findings. Students of scientific programs in which mathematics does not play a central role, all attending the same university, constituted the target population; the samples were cohorts of students enrolled in a study program in biotechnologies, where they attended the first year of the program. A total of 29 students answered to all the questions of the two questionnaires, but since in the first questionnaire, some questions were posed only to students giving specific answers to previous questions, other questions were answered by more students—sometimes dozens more. The access data were based on the whole class, and ultimately, 20 students were considered for proficiency purposes. Measurement of questionnaire responses was performed by means of Likert scales, while access and proficiency were measured with dedicated automatic features of the LMS and the AAS. As researchers who were also teachers of the course, we performed the quantitative analyses by using descriptive statistics, while the qualitative part was explanatory of the quantitative portion.

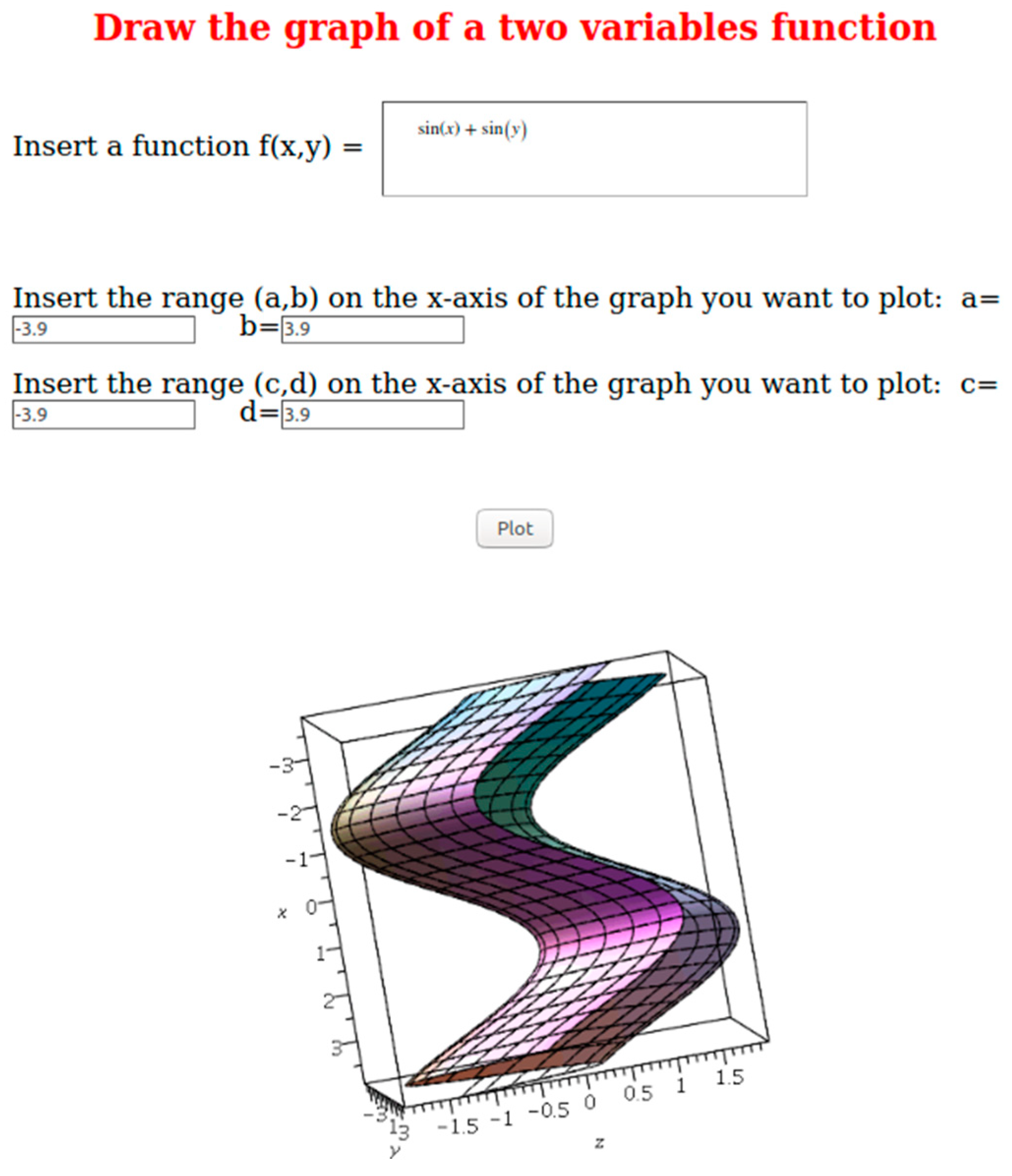
3.1. Learning Management System

A learning management system is an online platform for creating and distributing course contents. Features of an LMS include students’ subscription and activity tracking, allowing, on the one hand, for students to have extensive access to the resources and, on the other hand, for teachers to monitor their activity on the platform. For our course, we used the LMS Moodle, one of the most common choices, both academically and for business applications. A course can be designed using several learning objects, which allow (but not mandatorily) dynamicity and interactivity, providing a wide range of possibilities for creators to operate within. An excerpt from a section of a Moodle course containing several types of learning items is shown in Figure 1.

3.2. Advanced Computing Environment

An advanced computing environment is a user-friendly software allowing for simple and functional representation of mathematical objects. For instance, an ACE allows for the redaction of documents constituted of both text and code, where the former can be formatted as in a word processor and the latter can be executed by a computational engine. It is also possible to insert graphics and interactive elements, for example, by drawing animated graphs, which are useful for developing computational thinking and to support STEM education. This is obtained through some interactive components that permit the intuitive exploration of changes in quantities or figures while certain mathematical entities (algebraic, geometric, etc.) on which they depend vary. Students’ reasoning can thus be improved by helping them keep track of their learning process. For the development of our course, we made use of the ACE Maple, which allows for the creation of interactive files. These interactive files have the advantage of being embeddable within a Moodle course as a regular learning object. This allows students to work with one of the best mathematical engines available without the need to possess the software (which is commercial) on their PCs and to save the money required to buy it and the time necessary for its installation and configuration. Figure 2 shows an example of an interactive file.

3.3. Automated Assessment System

An automated assessment system is a system that allows for the evaluation of a student’s preparation by automatic means. Using an AAS allows various courses of action, such as regular testing, thanks to feedback, which can be personalized to monitor learning over time. Thanks to automatic correction procedures, it is possible to activate self-evaluation, which can be useful in courses where physical interaction with the teacher is minimal. In addition, an AAS is able to better deal with students’ needs, even when minimal physical interaction with the teacher is not the case. For example, an automatic system is always able to answer immediately, while a teacher, in many cases, is not. At least at first, there could also be some limitations, for example, sometimes multiple-choice questions, although traditionally used in automatic-evaluation contexts, are not always appropriate to solve doubts and test students’ skills in using knowledge and competences when considering a problem-solving setting [24]. Speaking of mathematics, for example, it could be useful to ask students to explicitly write various mathematical entities (formulas, equations, sets, etc.) that constitute some intermediate or final step of the solution to a certain problem rather than ask them to choose from a limited number of choices. An AAS is able to evaluate the correctness of a mathematical expression using an ACE, which can recognize all (or at least most) of the correct forms of a certain expression. In fact, there are entities that can be represented in more than a single correct form; the possibilities may also be infinite, such as the implicit equation of a line on a plane, for example:

x + y = 0, 2x + 2y = 0, 3x + 3y = 0, …, kx + ky = 0

(1)

the set (1) containing, by varying k among real non-zero numbers, the infinite forms of the unique line passing through the points with Cartesian coordinates (0, 0) and (1, −1). The AAS Möbius Assessment allows one to take advantage of both an AAS and an ACE. Indeed, it works with the Maple engine, supporting all its commands, which can also be used to insert graphics, computations, and random parameters. In particular, random variables allow for the creation of questions, which are generated with different values every time they are executed. By fixing the question structure, with only numerical values being modified, students are allowed to learn from their errors. Indeed, by answering a new version of the algorithmic question after giving the wrong answer, the student must repeat the reasoning in order to avoid the errors that led to the incorrect answer. This causes the error to become part of the learning process [25]. Möbius Assessment tests can be also inserted within a Moodle course as regular learning items, thus allowing students to retrieve them directly from the LMS. An important feature of Möbius Assessment is so-called adaptive questions, which are multi-part questions in which the path proposed to students is dependent on their answers. More precisely, in the beginning, a question is proposed for which the students can freely reason, usually with more than a single way to answer correctly. Then, if they answer incorrectly, a multi-step path starts, guiding them through the solution by requiring an answer to some intermediate steps. In the end, a similar problem can be proposed, likely with different numerical values and data, to allow students to repeat and learn the procedure that is necessary to obtain the correct answer. This methodology permits the introduction of immediate feedback, a very useful tool for formative assessment. Thus, the relation between the answers given by students and the paths that are consequently proposed to them can be interpreted as immediate feedback from the assessment system relative to the problem-solving method followed by the students themselves. There is a relevant number of studies [26] agreeing on these factors. Evaluation of the effectiveness of feedback has to be based upon its quick availability (the answer should be clearly shown to be correct or not), moderate length (students are generally not very eager to read long feedback), and specificity. Regarding the last factor, since general considerations are usually less appreciated than specific ones, it becomes particularly important for the guidance to the right answer to occur through an active process. In this way, students are guided by a procedure in which they are directly involved and not just limited to the mere proposition of contents independent of their actions. This allows the creation of personalized paths (adaptive teaching and learning), which have been shown to improve student outcomes. Additionally, students’ self-awareness is improved, making them more aware of the results achieved thanks to their studies. This allows for more efficient learning and personal involvement in the process of improvement. Figure 3 shows an example of an adaptive question.

4. Mathematics for Biotechnologists

The methodology we presented brought us to design our course as follows: addressed to students of the first year of a study program in biotechnologies called Matematica e Biostatistica con Applicazioni Informatiche (Mathematics and Biostatistics with Applications relative to Computer Science), lasting three years and resulting in a bachelor’s degree at its end, referred to in the Italian university framework as Laurea Triennale. The course was aimed both at providing competences in mathematics and at improving several soft and transversal skills, such as competences in the digital world and a problem-solving setting. It lasted a total of 76 h, of which about 68% (52 h) were devoted to classroom lessons divided between classical topics of mathematics (mainly, but not limited to, linear algebra and calculus, for 36 h) and biostatistics (16 h). The remaining 24 h were provided half as lab sessions in a room equipped with PCs, allowing students to work with the ACE, and half as a general review, with exercises similar to those they would be asked to solve on the incumbent exam also presented to make students confident of the modalities. Outside the brick-and-mortar locations, students were able to access an online course specifically devoted to this academic course available 24/7. In that course, apart from general information, students were encouraged to take part in various activities, often involving the ACE or the AAS. For the mathematics part, a section for every topic (vector calculus, matrix algebra, univariate calculus, integrals, etc.) was created, generally containing the following educational resources and activities: files, Maple worksheets with interactive components, and Möbius Assessment tests. For the biostatistics part, we created a section containing files, tests, and a book, since we also took advantage of the computer-room hours to complete the theoretical explanations. For both parts, the tests were constructed in such a way that almost every question was algorithmic; thus, students were able to take advantage of various formative assessment capabilities since questions could be attempted multiple times and students did not need to search for exercises and similar resources or activities elsewhere outside our course. The adaptivity inherently present in some of the questions presented even more advantages, fully benefiting from the features of the feedback system, such as immediateness and interactivity. At the end of the course, students completed the two questionnaires. In the initial questionnaire, students were asked, among other questions, about their knowledge of ACEs and AASs. On the other hand, in the final questionnaire, they had to review the experience with the same methodology, but some more general questions about the subject and the topics of the course were also asked, such as questions about mathematics and statistics themselves, but also concerning computer-science competences and the problem-solving approach. It is noteworthy that the AAS was used not only for formative assessment purposes, as just described, but also for the final exam and even for an additional exam that some students were asked to complete. Indeed, some students had to take an OFA (Obbligo Formativo Aggiuntivo, additional formative obligation), which consisted of a test in mathematics focused on topics that are considered prerequisites. This test, also part of another LMS course, also took advantage of the features granted by the AAS. Students were required to answer 12 questions during the final exam, eight of which referred to classical topics of mathematics, while the other four referred to biostatistics. These proportions were in line with the amount of time devoted to the two areas. The questions possessed random parameters, thus being algorithmic, but they were not designed as adaptive since this kind of adaptivity is more effective and suitable for formative assessment than for summative assessment. About 85% of correct answers were sufficient to achieve full marks, as it is often deemed proper to give the maximum even if a limited percentage of questions is incorrect or unanswered. This test was not the only evaluation, though; students also had to submit an examination paper with the ACE. The idea behind this requirement was to highlight the signs of progress of the students in mathematics and biostatistics, on the one hand, and in mastering the ACE, on the other hand. Indeed, the work consisted of considering and putting into practice a problem that had been examined during the course and in discussing it orally after the test, with the possibility of achieving a bonus or withstanding a malus, modifying the test score earned. The approach undertaken for teaching was integrated, following the blended learning paradigm. During classroom hours, an alternation of lectures, group, and individual activities related to solving exercises and problems, use of the platform for accessing interactive materials, and short automatically assessed tests, both with the use of the teacher’s computer and the students’ smartphones, was carried out. During computer-room hours, students worked with the ACE installed on the PCs they used, but they were able to access the platform and, more generally, the Web, along with the use of pen and paper to reason on exercises and problems, either alone or in small groups. At home, they were able to use the platform as a virtual environment in which to access materials, view short videos, take self-evaluation tests, receive interactive feedback while doing an exercise or a problem, upload the requested homework, and receive individual comments. Students were also given the possibility to use a forum to communicate, receive information about noteworthy activities available, do comparisons, and work with their peers. The platform collected data [27] allowing for monitoring by teachers, for instance, concerning the activities students undertook and their errors, giving room for interventions when particular difficulties arose around specific concepts.

5. Results

The analysis of the questionnaires allowed us to track changes in students’ opinions during the course since, as mentioned in the previous sections, the first questionnaire was administered at the beginning of the course, while the second one was made available at its end. Figure 4 shows students’ improvement in recognizing the importance of the problem-solving approach for several reasons. In this and the following figures, a score 1 is the lowest, while a score of 5 is the highest. Furthermore, the cyan bars correspond to scores relative to the first questionnaire, while the blue bars represent the scores relative to the second one; the values reached by the bars are the averages, and the lines over them highlight, for every bar, both the average minus the standard deviation and the average plus the standard deviation.

Students gave particular importance to needs related to their academic career. Indeed, they thought about how the problem-solving approach would help them learn the theory and their job career, but the best score in the second questionnaire was obtained on the question concerning students’ future studies, maybe because they are, at the moment, their main interest. The most important feature is that they are all positive beliefs. Figure 5 depicts the improvements in recognizing how some noteworthy features regarding an AAS are important and useful.

The immediate assessment, differently from a textbook-like framework, was the preferred feature, but all the presented features were appreciated. The possibility of repeating simulations, thanks to the use of parameters that are generated randomly, was awarded a very high score, while they also recognized how errors can be better understood with such a system. The poorest result concerns the guarantee of equality, an aspect that we will develop in detail in the next section. Figure 6 depicts the recognition by students of how their competences improved.

The items for which the best results were obtained in the first questionnaire were again at the top in the second one, but they underwent only slight improvements; generally, the lower the initial score, the greater the increase. This is particularly true for the use of an ACE, which started with a very low score but gained almost one point at the end.

The analysis of the activities carried out by the students gives other interesting insights in terms of boosting interest and augmenting competences. Nine tests with the AAS and relative to single topics were provided about the classical themes of mathematics, while four were about biostatistics, for a total of 13. Two general tests, one limited to biostatistics and the other simulating the structure of the final exam, were also made available to students. If we consider their use until the date of the first exam call, because the students involved were likely those who took better advantage of the features our course provided them, we obtained the results of Figure 7. Note that another reason to limit the consideration to the first call is to ensure the availability of a sample that is consistent with our framework of mathematics in certain scientific programs, thus preferring not to take into account students who are more prone to change path after having enrolled in biotechnologies. For various reasons, such as career transferability, they usually do not take the mathematics exam as soon as possible.

The tests and the simulation were generally taken by more people than those taking the exam; only one student took the exam without taking the general test simulating it. As shown in Figure 8, there were some specific tests done by even more students, likely because they preferred to train rather than to prepare themselves to take the exam at the first call.

Additionally, the ACE worksheets were opened by several students, more than those who took the exam as soon as it was possible, as shown in Figure 9; accesses to computer-science applications are reported as two averages, one related to theory and the other concerning exercises and problems.

Summing up what we depicted in Figure 7, Figure 8 and Figure 9, the fact that students took a consistent number of specific AAS tests and accessed an important number of ACE resources suggests that creating and making available such materials could foster interest, thus helping students to develop mathematical competences. For both the AAS and the ACE, we found various occurrences of multiple accesses, when students opened a certain resource more than once. Figure 10 shows how notable resources were accessed, on average, far more than once.

It could be interesting to also mention how much the students interacted on the LMS (including its ACE-type and AAS-type resources). During the period starting from the third week of October 2019 (when the course was brought online) and ending the second week of February 2020, we registered a weekly maximum of more than 1000 posts (quiz submissions, forum discussion answers, other active responses) in the last week of November, near the end of the classroom hours. We also detected a maximum of more than 2500 views (instances of a user accessing a resource or activity for reading or downloading purposes) in the week immediately preceding the first exam call in January. In general, numbers were higher when the lessons were coming to their end and near the exam dates, but they were high in general throughout the period, strengthening the suggestion of using resources and activities to generate engagement and favor the development of mathematical competences.

We will now present the qualitative part of our research by giving an example of an examination paper, which we introduced in Section 4. Figure 11 shows a representative excerpt of the submitted student responses from one of these examination papers. We see how the ACE capabilities allow for generalization of the evolution of a population modeled with Verhulst’s equation. Indeed, while it can be considered initially (not shown here) with fixed values of the parameters involved in the form of a traditional exercise, it admits an easy generalization to arbitrary values for the parameters themselves. A form allows for the insertion of the data characterizing the evolution process, which are the initial number of individuals, the carrying capacity, and the growth rate of the population (with the last one being inserted using a slider). The result is a plot of the number of individuals versus time, showing how the population tends to stabilize toward the carrying capacity, according to the theory. The usefulness of the interactive exploration depicted in § 3.2 is boosted by the fact that students are not only users anymore, as for the worksheets made available during the course; they become creators, a role which again supports persistence and development of competences.

If we then analyze grades, we obtain surprising results. Indeed, if we consider the grades relative to students taking the exam on the first available date (again, because they were likely the students that took better advantage of the features of the course), without the effects of the ACE examination papers (by means of evaluation, they did not substantially modify the marks), we had no failures and several full scores. What is shown in Figure 12 configures better outcomes than expected. In the set of 20 students, no failure was registered. At the same time, among the five students getting a 30/30 grade, four passed the exam Cum Laude, reserved to those who obtained more than 30 points on the test (as we said in Section 4, 85% of correct answers was sufficient to achieve a full mark).

6. Discussion

We will now discuss some of the most noteworthy results emerging from Section 5. Concerning Figure 4, we briefly discussed our interpretation of the scores in loco. Regarding Figure 5, we saw how the guarantee of equality obtained an intermediate result around 3 (the midpoint of the interval 1–5), which has to be noted as being low compared to the other items. This is likely caused by the fact that students are not completely at ease with some features of the AAS. For example, some of them doubt the role that miscalculations could have in the assessment. By reading several of their comments concerning observations on the course in general, they feared suffering excessive penalties due to errors in computations rather than conceptual ones or, more generally, regarding the solving process. It is nonetheless possible to deal with this issue by constructing questions with the requirement of step-by-step answers accompanied by partial grading that allows an evaluation that reflects the actual preparation of the students. Furthermore, the capabilities of the AAS, including various symbolic syntaxes and numerical answers within a prescribed tolerance, can help in giving a fair assessment. By considering how mathematics is usually (albeit not always) assessed in secondary schools, it can be inferred that students usually wrote solutions within a framework wherein their teacher evaluated them by reading everything they jotted down. Evaluation only on specific answers is rarer, something students tend not to be used to. Summing up, the above constitutes some sort of a wrong belief but more on a technical aspect of the tool.

In Figure 6, we saw the score relative to the use of the ACE steadily increase, but that augmentation did not prevent the item from achieving the lowest score again after the course. In particular, it remained the only question to be evaluated below 3. Since before the course, students made clear that the majority of them did not properly consider (or even at all) using an ACE during secondary school, our course had been the first (and only, up to the considered semester) occasion to become familiar with it. This triggered the great boost but, at the same time, caused the extremely low mark from which ACE competences started. What emerges from both marks is the advisability to begin a computer-supported education well before entering university in order to let students feel at ease with these tools, which, as other scores show, can be advantageous. Indeed, the fact that there is no guarantee of employing them in secondary school constitutes a limitation for reaching our goals, which led to the recommendation to anticipate their introduction and make sure that they will actually be used. The result could have also conceivably been influenced by a certain dose of learned helplessness; students with initial difficulties with the ACE were plausibly prone to believe that they would have failed in overcoming them anyway, thus working below their capabilities, although the examination papers showed they were, in fact, able to perform various tasks.

The results of Figure 8 can be interpreted as an occurrence of personalized learning, which is also important because of the individual relation between beliefs and emotions. While training, rather than directly preparing for the exam, could have been agreed upon both at the beginning or during the course, since the amount of individual study necessary may be known only after the course has started, it still fits with the blended learning paradigm. In terms of instructional design, it could be recommended to devote some work to overcoming this limitation, for example, by making clearer estimates in advance regarding how much study is required for the various parts of the course, always respecting the personalization features.

The multiple accesses of Figure 10 are also in agreement with a feature of the blended learning paradigm—that is, the ability of the student to control their own pace, again recalling the individuality of some features. With the AAS, it is natural to relate behavior with the algorithmic features of several questions, allowing students to attempt them repeatedly, thanks to the randomly generated parameters and learning through errors. However, with the ACE, it also makes much more sense to repeat access to the resources; indeed, it is reasonable to think about students only superficially viewing the resources at a first instance and then exploring them in more detail with subsequent access, for example, by making use of interactivity.

In the examination paper of Figure 11, we see that students could count on some help from teachers and tutors, but they worked, for the most consistent part, autonomously, giving proof of competence acquisition in both mathematics and use of the ACE, as well as in the transversal areas of problem solving and digital skills. This is not in contradiction with what we said at the end of the discussion relative to Figure 6. In fact, acquiring competences does not imply acquiring the kind of confidence that allows the student to feel how they have effectively gained those competences—at least not completely. With a certain frequency, it does trigger an awareness, though only a partial awareness.

Finally, the outcome of the exam (Figure 12) allowed us to confirm that higher grades were generally awarded to students who made better use of the platform, of the resources, and of the activities provided, thus further supporting their usefulness.

It should be noted that it is difficult to prove that the results are statistically significant with the traditional methods of inference since the search for a numerical significance would have required provisions that are hardly compatible with the nature of the course, such as subdividing the students in two groups. Indeed, it also would not have been completely ethical to assign students, regardless of their will (otherwise, the introduction of a bias would have occurred), to a group providing more traditional teaching, or to a group featuring our format. Nevertheless, this does not constitute a limitation, by keeping intact the educational meaning of the results, since it is not so obvious that students who enroll in a course, even if equipped with online features, will persist by improving their opinion of its facets, as, for example, ref. [28] and some references therein discuss.

7. Conclusions

The use of a problem-solving approach within a digital learning environment, along with methodologies, such as formative assessment, and technologies like a learning management system, an advanced computing environment, and an automated assessment system allowed students of a non-mathematical science to better learn mathematics. This happened not only in terms of summative evaluation, such as by considering the grades obtained on the exam, which were nonetheless generally higher than expected, if compared to the previous years with a traditional course and assessment, but also in terms of an improvement concerning the study of mathematics, along with affect, attitude, and beliefs. Indeed, the help that the problem-solving approach and the use of an ACE and AAS gave to students has been recognized as essential, making them aware of how pivotal they had been in smoothing out their learning path. The latter feature is even more important, in a way, because the valorization of mathematical capabilities to a greater extent allows for an advantageous approach to both potential future courses in mathematics and the scientific job market. All of this also proves the effectiveness of the blended learning approach. If teachers and tutors used said approach throughout the course, the improvement would be primarily (but not exclusively) on the students’ side, initially with reference to self-evaluation and competences and then in terms of grades. Ideas for future research include the extension of this study for fields even more markedly distanced from mathematics than a scientific program not strictly based on the primary STEM threads. Given that several of the competences provided by mathematics are transversal—that is, they can be of various uses inside and outside university—it would be an interesting challenge to extend our work to other fields, for example, linguistics or humanities. This is supported by experiences implementing the use of the AAS in these fields. The main features of AAS, such as random parameters and adaptive questions, can be made applicable for working in the contexts of sentences and text, as these fields of knowledge require. If students are allowed to use the same technological means, with correspondence to an analogous methodology (e.g., problem solving), to handle both the main subjects of their fields of study and the mathematical courses, it would be easier for them to study mathematics and take the respective exams.