On Augmented Reality for the Learning of 3D-Geometric Contents: A Preliminary Exploratory Study with 6-Grade Primary Students
Abstract
1. Introduction
2. AR for Geometric and Spatial Learning
Geometry comprises those branches of mathematics that exploit visual intuition (the most dominant of our senses) to remember theorems, understand proofs, inspire conjectures, perceive realities, and give global insight.(Zeeman, quoted in [24], p. 12)
3. Aim
4. Materials and Methods
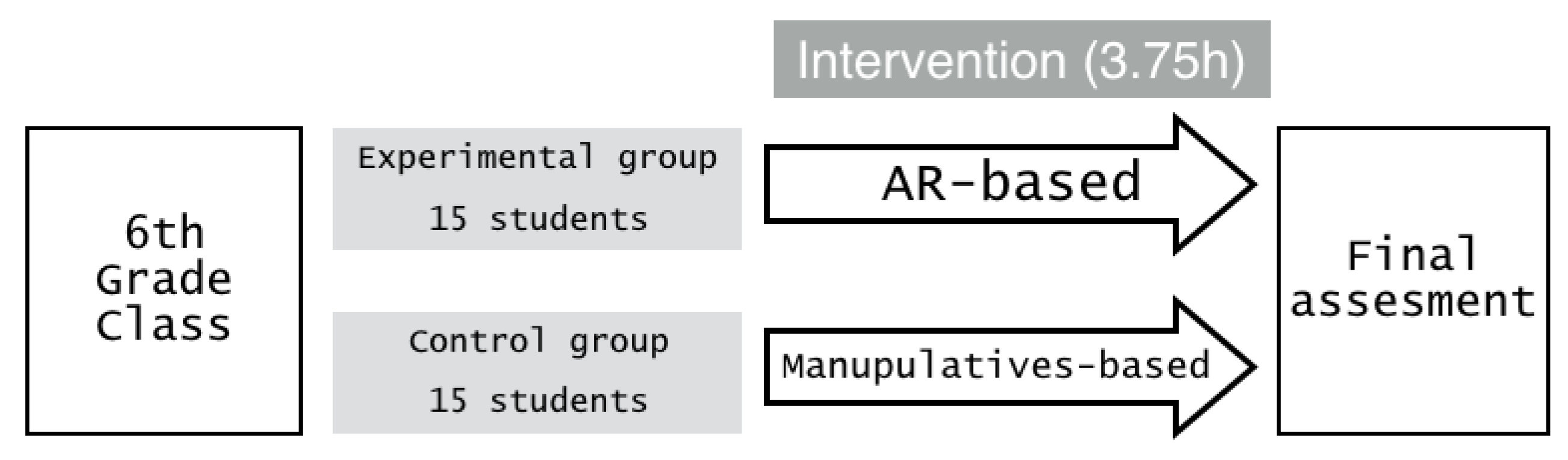
4.1. The Intervention Design
4.2. Participants
4.3. Procedure and Materials
4.4. Instrument
5. Results and Discussion
5.1. Mean Scores on the Initial Test
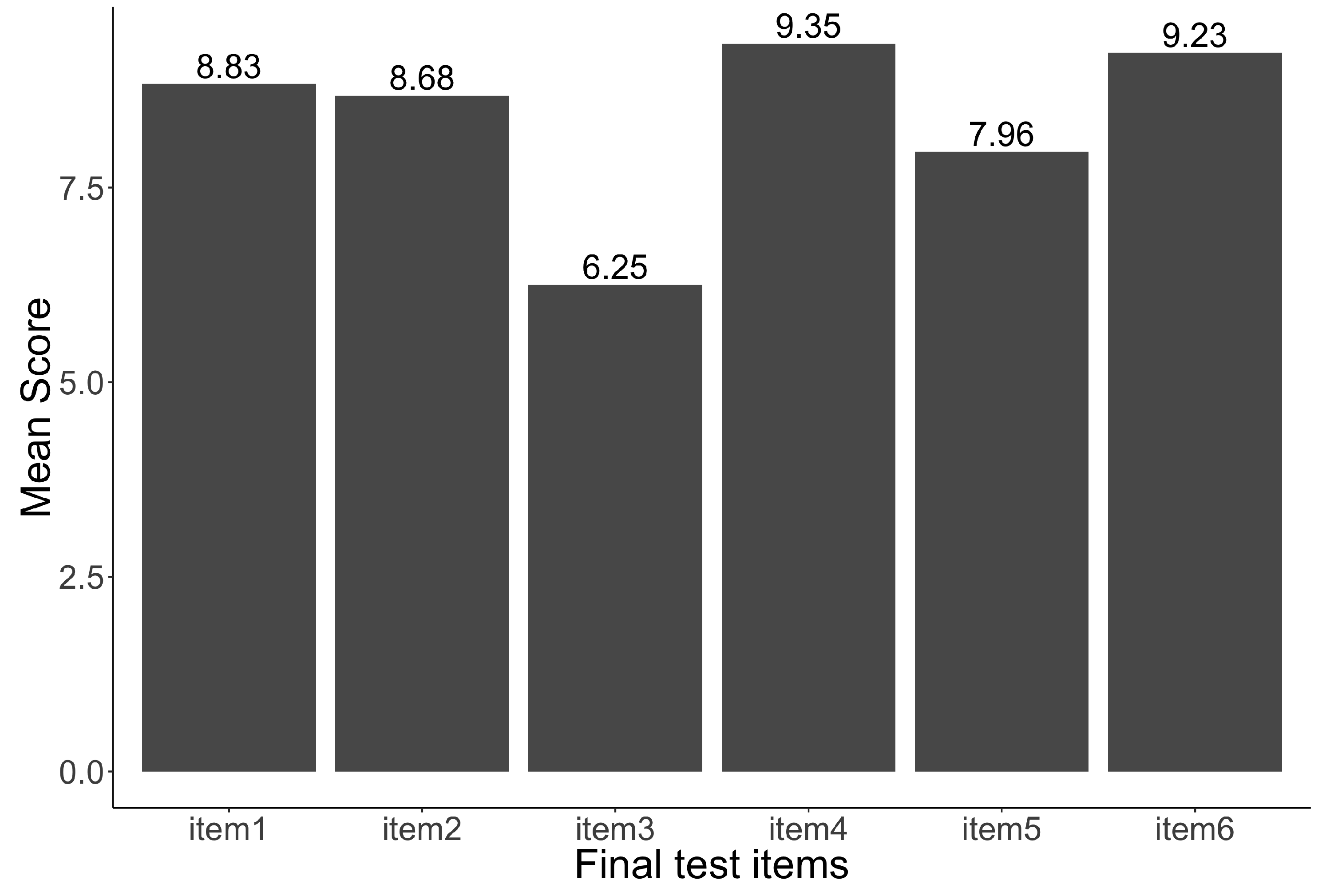
5.2. Mean Scores on the Final Assessment Test
5.3. Comparison between Control and Experimental Groups
6. Final Remarks
Author Contributions
Funding
Conflicts of Interest
References
- Fernández, B. Adopción de la realidad aumentada por estudiantes del grado de educación primaria. In Tecnología, innovación e investigación en los procesos de enseñanza-aprendizaje; Roig-Vila, R., Ed.; Octaedro: Barcelona, Spain, 2016; pp. 432–439. [Google Scholar]
- Sarracino, F. ¿Mejora la realidad aumentada el aprendizaje de los alumnos? Una propuesta de experiencia de museo aumentado. Profesorado. Revista de Currículum y formación del profesurrículum y formación del profesorado 2014, 3, 1–18. [Google Scholar]
- Duh, H.B.; Klopfer, E. Augmented reality learning: New learning paradigm in co-space. Comput. Educ. 2013, 68, 534–535. [Google Scholar] [CrossRef]
- Bacca, J.; Baldiris, S.; Fabregat, R.; Graf, S.; Kinshuk, G. Augmented Reality Trends in Education: A Systematic Review of Research and Applications. Educ. Technol. Soc. 2014, 17, 133–149. [Google Scholar]
- Azuma, R.; Billinghurst, M.; Klinker, G. Special section on mobile augmented reality. Comput. Graph. 2011, 35. [Google Scholar] [CrossRef]
- Cuendet, S.; Bonnard, Q.; Do-Lenh, S.; Dillenbourg, P. Designing augmented reality for the classroom. Comput. Educ. 2013, 68, 557–569. [Google Scholar] [CrossRef]
- Iftene, A.; Trandabat, D. Enhancing the Attractiveness of Learning through Augmented Reality. Procedia Comput. Sci. 2018, 126, 166–175. [Google Scholar] [CrossRef]
- Freeman, A.; Adams Becker, S.; Cummins, M.; Davis, A.; Hall Giesinger, C. NMC/CoSN Horizon Report: 2017 K–12 Edition; The New Media Consortium: Austin, TX, USA, 2017. [Google Scholar]
- Adams Becker, S.; Brown, M.; Dahlstrom, E.; Davis, A.; DePaul, K.; Diaz, V.; Pomerantz, J. NMC Horizon Report: 2018 Higher Education Edition; EDUCAUSE: Louisville, CO, USA, 2018. [Google Scholar]
- Cózar, R.; Sáez, J.M. Realidad aumentada, proyectos en el aula de primaria: Experiencias y casos en Ciencias Sociales. EDMETIC 2016, 6, 165–180. [Google Scholar] [CrossRef]
- Cabero, J.; Barroso, J. The educational possibilities of Augmented Reality. J. New Approaches Educ. Res. 2016, 5, 44–50. [Google Scholar]
- Leiva, J.J.; Moreno, N.M. Tecnologías de geolocalización y realidad aumentada en contextos educativos: Experiencias y herramientas didácticas. Rev. DIM 2015, 31, 1–18. [Google Scholar]
- Cubillo, J.; Martín, S.; Castro, M.; Colmenar, A. Recursos Digitales Autónomos Mediante Realidad Aumentada. Rev. Iberoam. Educac. Distancia 2014, 17, 241–274. [Google Scholar]
- Kesim, M.; Ozarslan, Y. Augmented Reality in Education: Current Technologies and the Potential for Education. Procedia Soc. Behav. Sci. 2012, 47, 297–302. [Google Scholar] [CrossRef]
- Wu, H.K.; Wen-Yu, S.; Chang, H.Y.; Liang, J.C. Current status, opportunities and challenges of augmented reality in education. Comput. Educ. 2013, 62, 41–49. [Google Scholar] [CrossRef]
- Orcos, L.; Jordán, C.; Magreñán, A. 3D visualization through the hologram for the learning of area and volume concepts. Mathematics 2019, 7, 247. [Google Scholar] [CrossRef]
- Jamali, S.S.; Shiratuddin, M.F.; Wong, K.W.; Oskam, C.L. Utilising Mobile-Augmented Reality for Learning Human Anatomy. Procedia Soc. Behav. Sci. 2015, 197, 659–668. [Google Scholar] [CrossRef]
- Luna, Ú.; Ibáñez-Etxeberria, A.; Rivero, P. El patrimonio aumentado. 8 apps de Realidad Aumentada para la enseñanza-aprendizaje del patrimonio. Rev. Interuniv. Formación del Profesor. 2019, 94, 43–62. [Google Scholar]
- Piqueras, E.M.; Cózar, R.; González-Calero, J.A. Incidencia de la realidad aumentada en la enseñanza de la historia. Una experiencia en tercer curso de educación primaria. Enseñan. Teach. Rev. Interuniv. Didáct. 2018, 36, 23–39. [Google Scholar]
- Prendes, C. Realidad aumentada y educación: Análisis de experiencias prácticas. Píxel-Bit. Rev. de Medios y Educ. 2015, 46, 187–203. [Google Scholar]
- Fonseca, D.; Redondo, E.; Valls, F. Motivación y mejora académica utilizando realidad aumentada para el estudio de modelos tridimensionales arquitectónicos. Educ. Knowl. Soc. 2016, 17, 45. [Google Scholar] [CrossRef]
- Escrivà, M.T.; Jaime, A.; Gutiérrez, Á. Educación Matemática en la Infancia. Edma 0-6 Educ. Mat. Infanc. 2018, 7, 42–62. [Google Scholar]
- Bujak, K.R.; Radu, I.; Catrambone, R.; MacIntyre, B.; Zheng, R.; Golubski, G. A psychological perspective on augmented reality in the mathematics classroom. Comput. Educ. 2013, 68, 536–544. [Google Scholar] [CrossRef]
- Royal Society. Teaching and Learning Geometry; Royal Society: London, UK, 2001; pp. 11–19. [Google Scholar]
- Bishop, A.J. Spatial abilities and mathematics achievement—A review. Educ. Stud. Math. 1980, 11, 257–269. [Google Scholar] [CrossRef]
- Jones, K.; Tzekaki, M. Research on the teaching and learning of geometry. In The Second Handbook of Research on the Psychology of Mathematics Education: The Journey Continues; Gutiérrez, Á., Leder, G.C., Boero, P., Eds.; Sense Publishers: Rotterdam, The Netherlands, 2016; pp. 109–149. [Google Scholar]
- Whiteley, W.; Sinclair, N.; Davis, B. What is spatial reasoning? In Spatial Reasoning in the Early Years; Davis, B., The Spatial Reasoning Study Group, Eds.; Routledge: New York, NY, USA, 2015; pp. 3–14. [Google Scholar]
- Battista, M.T. The development of geometric and spatial thinking. In Second Handbook of Research on Mathematics Teaching and Learning; Lester, F.K., Ed.; National Council of Teachers of Mathematics: Charlotte, NC, USA, 2007; pp. 843–908. [Google Scholar]
- Dowker, A.; Cheriton, O.; Horton, R.; Mark, W. Relationships between attitudes and performance in young children’s mathematics. Educ. Stud. Math. 2019, 100, 211. [Google Scholar] [CrossRef]
- National Council of Teachers of Mathematics. Principles and Standards for School Mathematics; NCTM: Reston, VA, USA, 2000. [Google Scholar]
- Duval, R. Geometry from a cognitive point of view. In Perspectives on the Teaching of the Geometry for the 21st Century; Mammana, C., Villani, V., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1998; pp. 37–51. [Google Scholar]
- Clements, D.H.; Battista, M.T.; Sarama, J.; Swaminathan, S. Development of Students’ Spatial Thinking in a Unit on Geometric Motions and Area. Elem. Sch. J. 1997, 98, 171–186. [Google Scholar] [CrossRef]
- Bartolini, M.G.; Martignone, F. Manipulatives in mathematics education. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: Dordrecht, The Netherlands, 2014; pp. 365–372. [Google Scholar]
- Guillén, G.; Gutiérrez, Á.; Jaime, A.; Cáceres, M. La enseñanza de la geometría de sólidos en la E.G.B.; Technical Report; Universitat de València: Valencia, Spain, 1992. [Google Scholar]
- Gutiérrez, Á. Visualization in 3-dimensional geometry: In search of a framework. In Proceedings of the 20th PME Conference, Valencia, Spain, 8–12 July 1996; Volume 1, pp. 3–19. [Google Scholar]
- Kaufmann, H.; Schmalstieg, D. Mathematics and geometry education with collaborative augmented reality. Comput. Graph. 2003, 27, 339–345. [Google Scholar] [CrossRef]
- Kaufmann, H. Geometry Education with Augmented Reality; Technical Report; Technischen Universität Wien: Wien, Austria, 2004. [Google Scholar]
- Marrades, R.; Gutiérrez, Á. Proofs produced by secondary school students learning geometry in a dynamic computer environment. Educ. Stud. Math. 2000, 44, 87–125. [Google Scholar] [CrossRef]
- Saha, R.A.; Ayub, A.F.M.; Tarmizi, R.A. The effects of GeoGebra on mathematics achievement: Enlightening Coordinate Geometry learning. Procedia Soc. Behav. Sci. 2010, 8, 686–693. [Google Scholar] [CrossRef]

| 1 | What is a polyhedron? |
| 2 | What types of polyhedrons you know? |
| 3 | Can you identify the notable elements of a polyhedron? |
| 4 | True of false:
|
| 5 | Draw the developments of a cone and of a prism. |
| Session | Content |
|---|---|
| 1 | 3D-geometric shapes, definition of polyhedron |
| 2 | Identification of 3D-geometric shapes through using videos |
| 3 | Review of 2D shapes and identification. Identification of a polyhedron using his development |
| 4 | Identification 3D-geometric shapes in our everyday life |
| 5 | Characteristics and classification of 3D-geometric shapes |
| Final Assesment Items | |
|---|---|
| 1 | Explain what 3D-geometric shapes are and in which two groups we can classify them |
| 2 | Put the parts of these 3D-geometric shapes (Shapes: hexagonal prism, cone and sphere. Parts: base, face, edge, vertex, body and radius) |
| 3 | Complete the table with the characteristics of the 3D-geometric shapes (name, number of faces, number of edges and number of vertices) |
| 4 | Circle with red the 3D-geometric shapes that are polyhedrons and circle with blue those that are round shapes |
| 5 | Write the name of the following developed 3D-geometric shapes |
| 6 | Match the 3D-geometric shape with arrows to its development and try to draw the missing one |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Flores-Bascuñana, M.; Diago, P.D.; Villena-Taranilla, R.; Yáñez, D.F. On Augmented Reality for the Learning of 3D-Geometric Contents: A Preliminary Exploratory Study with 6-Grade Primary Students. Educ. Sci. 2020, 10, 4. https://doi.org/10.3390/educsci10010004
Flores-Bascuñana M, Diago PD, Villena-Taranilla R, Yáñez DF. On Augmented Reality for the Learning of 3D-Geometric Contents: A Preliminary Exploratory Study with 6-Grade Primary Students. Education Sciences. 2020; 10(1):4. https://doi.org/10.3390/educsci10010004
Chicago/Turabian StyleFlores-Bascuñana, Míriam, Pascual D. Diago, Rafael Villena-Taranilla, and Dionisio F. Yáñez. 2020. "On Augmented Reality for the Learning of 3D-Geometric Contents: A Preliminary Exploratory Study with 6-Grade Primary Students" Education Sciences 10, no. 1: 4. https://doi.org/10.3390/educsci10010004
APA StyleFlores-Bascuñana, M., Diago, P. D., Villena-Taranilla, R., & Yáñez, D. F. (2020). On Augmented Reality for the Learning of 3D-Geometric Contents: A Preliminary Exploratory Study with 6-Grade Primary Students. Education Sciences, 10(1), 4. https://doi.org/10.3390/educsci10010004







