Time Jitter, Turbulence and Chromatic Dispersion in Underwater Optical Wireless Links
Abstract
1. Introduction
2. The Underwater Channel Model
3. The Chromatic Dispersion Effect
4. The Time Jitter Effect
5. The Joint Influence of GVD, Time Jitter and Turbulence
6. The Probability of Fade
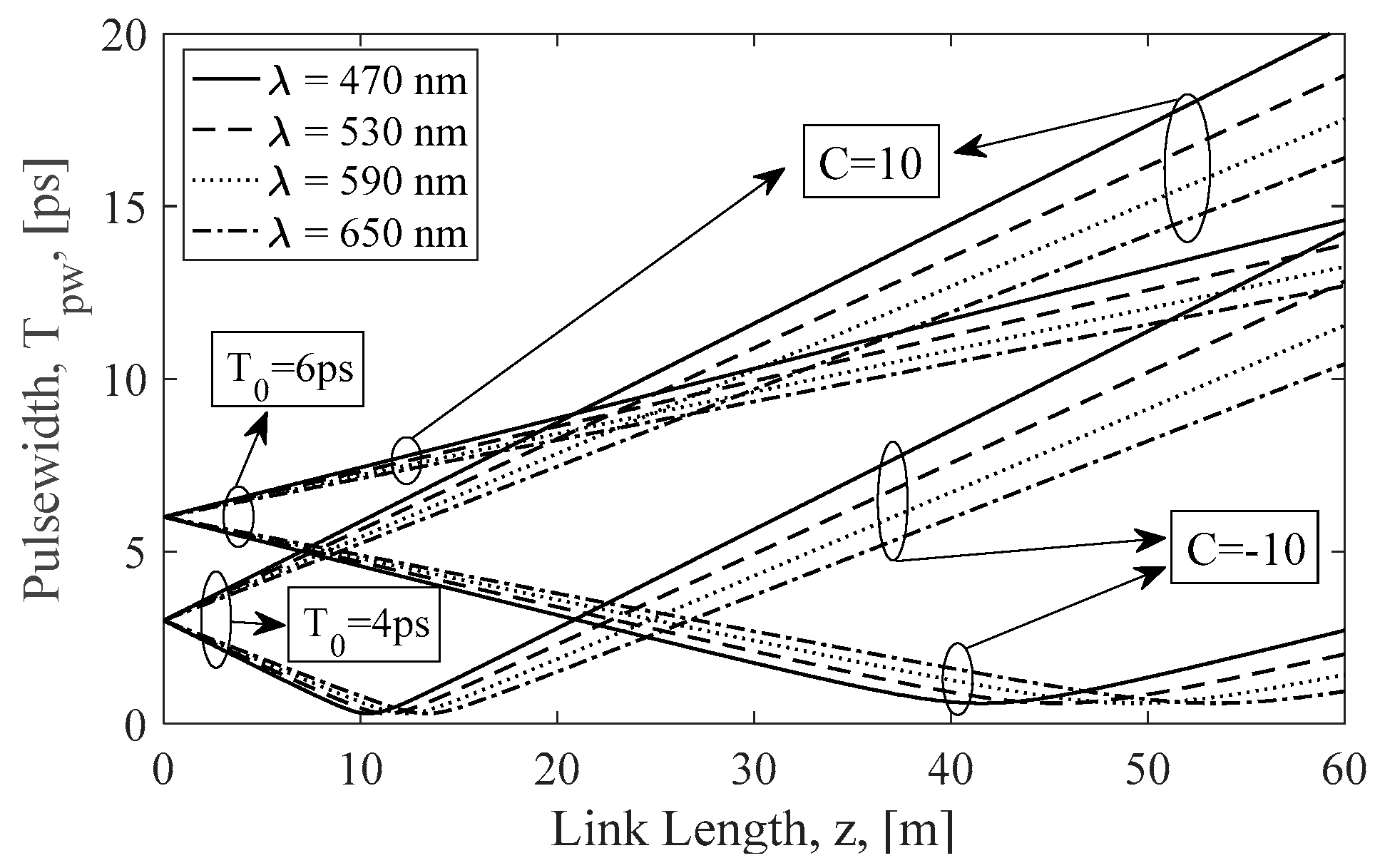
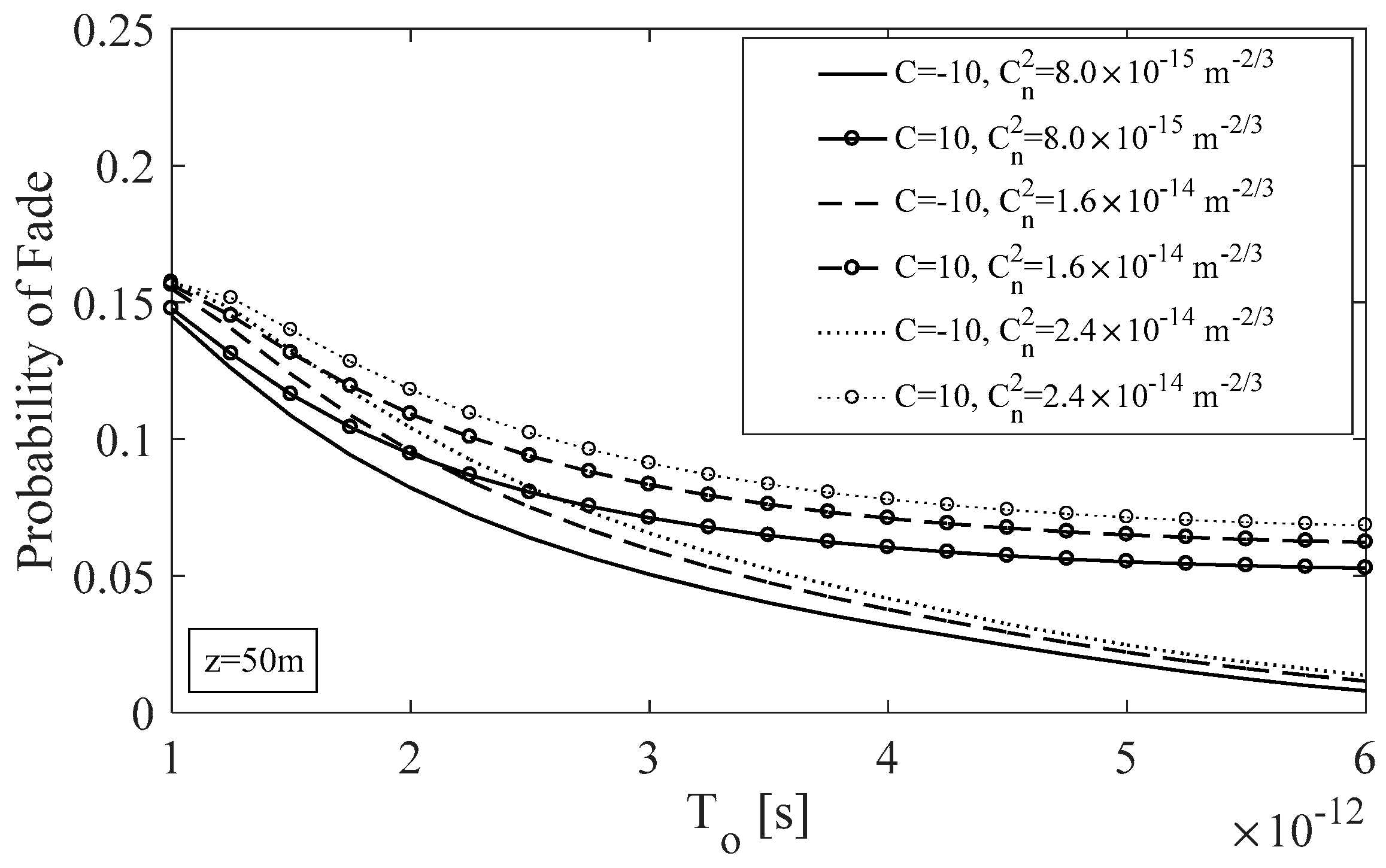
7. Numerical Results
8. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Arnon, S. Underwater Optical Wireless Communication Network. Opt. Eng. 2010, 49, 015001. [Google Scholar] [CrossRef]
- Tsonev, D.; Chun, H.; Rajbhandari, S.; McKendry, J.J.D.; Videv, S.; Gu, E.; Haji, M.; Watson, S.; Kelly, A.E.; Faulkner, G.; et al. A 3-Gb/s Single-LED OFDM-Based Wireless VLC Link Using a Gallium Nitride μLED. IEEE Photonics Technol. Lett. 2014, 26, 637–640. [Google Scholar] [CrossRef]
- Wu, T.C.; Chi, Y.C.; Wang, H.Y.; Tsai, C.T.; Lin, G.R. Blue laser diode enables underwater communication at 12.4 gbps. Sci. Rep. 2017, 7, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Kaushal, H.; Kaddoum, G. Underwater Optical Wireless Communication. IEEE Access 2016, 4, 1518–1547. [Google Scholar] [CrossRef]
- Chi, Y.-C.; Hsieh, D.-H.; Tsai, C.-T.; Chen, H.-Y.; Kuo, H.-C.; Lin, G.-R. 450-nm GaN laser diode enables high-speed visible light communication with 9-Gbps QAM-OFDM. Opt. Express 2015, 23, 13051. [Google Scholar] [CrossRef]
- Gkoura, L.K.; Roumelas, G.D.; Nistazakis, H.E.; Sandalidis, H.G.; Vavoulas, A.; Tsigopoulos, A.D.; Tombras, G.S. Underwater Optical Wireless Communication Systems: A Concise Review. In Turbulence Modelling Approaches—Current State, Development Prospects, Applications; Volkov, K., Ed.; InTech: Vienna, Austria, 2017. [Google Scholar] [CrossRef]
- Huang, Y.-F.; Tsai, C.-T.; Chi, Y.-C.; Huang, D.-W.; Lin, G.-R. Filtered Multicarrier OFDM Encoding on Blue Laser Diode for 14.8-Gbps Seawater Transmission. J. Light. Technol. 2018, 36, 1739–1745. [Google Scholar] [CrossRef]
- Saeed, N.; Celik, A.; Al-Naffouri, T.Y.; Alouini, M.-S. Underwater optical wireless communications, networking, and localization: A survey. Ad Hoc Netw. 2019, 94, 101935. [Google Scholar] [CrossRef]
- Coello, Y.; Xu, B.; Miller, T.L.; Lozovoy, V.V.; Dantus, M. Group-velocity dispersion measurements of water, seawater, and ocular components using multiphoton intrapulse interference phase scan. Appl. Opt. 2007, 46, 8394–8401. [Google Scholar] [CrossRef]
- Cheng, C.-H.; Shen, C.-C.; Kao, H.-Y.; Hsieh, D.-H.; Wang, H.-Y.; Yeh, Y.-W.; Lu, Y.-T.; Huang Chen, S.-W.; Tsai, C.-T.; Chi, Y.-C.; et al. 850/940-nm VCSEL for optical communication and 3D sensing. Opto-Electronic Adv. 2018, 1, 18000501–18000511. [Google Scholar] [CrossRef]
- Mullen, L.; Alley, D.; Cochenour, B. Investigation of the effect of scattering agent and scattering albedo on modulated light propagation in water. Appl. Opt. 2011, 50, 1396–1404. [Google Scholar] [CrossRef]
- Cochenour, B.; Mullen, L.; Laux, A. Spatial and temporal dispersion in high bandwidth underwater laser communication links. In MILCOM 2008—2008 IEEE Military Communications Conference; IEEE: Piscataway, NJ, USA, 2008; pp. 1–7. ISBN 978-1-42-442676-8. [Google Scholar]
- Liu, L.; Zhou, S.; Cui, J. Prospects and Problems of Wireless Communication for Underwater Sensor Networks. Wirel. Commun. Mob. Comput. 2008, 8, 977–994. [Google Scholar] [CrossRef]
- Baykal, Y. Signal-to-noise ratio reduction due to oceanic turbulence in oceanic wireless optical communication links. Opt. Commun. 2018, 427, 44–47. [Google Scholar] [CrossRef]
- Baykal, Y. Scintillations of optical plane and spherical waves in underwater turbulence. J. Opt. Soc. Am. A 2014, 31, 1552–1556. [Google Scholar] [CrossRef]
- Korotkova, O.; Farwell, N.; Shchepakina, E. Light scintillation in oceanic turbulence. Waves Random Complex Media 2012, 22, 260–266. [Google Scholar] [CrossRef]
- Andrews, L.C.; Phillips, R.L.; Hopen, C.Y. Laser Beam Scintillation with Applications; SPIE: Bellingham, WA, USA, 2001; ISBN 978-0-81-944103-4. [Google Scholar]
- Jamali, M.V.; Khorramshahi, P.; Tashakori, A.; Chizari, A.; Shahsavari, S.; Abdollahramezani, S.; Fazelian, M.; Bahrani, S.; Salehi, J.A. Statistical distribution of intensity fluctuations for underwater wireless optical channels in the presence of air bubbles. IWCIT 2016 Iran Workshop Commun. Inf. Theory 2016. [Google Scholar] [CrossRef]
- Arpali, S.; Baykal, Y.; Arpali, C. BER evaluations for multimode beams in underwater turbulence. J. Mod. Opt. 2016, 340, 1–4. [Google Scholar] [CrossRef]
- Liu, J.; Dong, Y. On capacity of underwater optical wireless links under weak oceanic turbulence. Ocean. 2016 Shanghai 2016. [Google Scholar] [CrossRef]
- Agrawal, G.P. Fiber-Optic Communication Systems; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2010; Volume 6. [Google Scholar]
- Underhill, M.J. Time jitter and phase noise—Now and in the future? In 2012 IEEE International Frequency Control Symposium Proceedings; IEEE: Piscataway, NJ, USA, 2012; pp. 1–8. [Google Scholar]
- Grigoryan, V.S.; Menyuk, C.R.; Mu, R.M. Calculation of timing and amplitude jitter in dispersion-managed optical fiber communications using linearization. J. Light. Technol. 1999, 17, 1347–1356. [Google Scholar] [CrossRef]
- Gnauck, A.H.; Mecozzi, A.; Clausen, C.B.; Sang-Gyu, P.; Shtaif, M. Cancellation of timing and amplitude jitter in symmetric links using highly dispersed pulses. IEEE Photonics Technol. Lett. 2002, 13, 445–447. [Google Scholar] [CrossRef]
- Santhanam, J.; Agrawal, G.P. Raman-induced timing jitter in dispersion-managed optical communication systems. IEEE J. Sel. Top. Quantum Electron. 2002, 8, 632–639. [Google Scholar] [CrossRef]
- Ghassemlooy, Z.; Arnon, S.; Uysal, M.; Xu, Z.; Cheng, J. Emerging Optical Wireless Communications-Advances and Challenges. IEEE J. Sel. Areas Commun. 2015, 33, 1738–1749. [Google Scholar] [CrossRef]
- Roumelas, G.D.; Nistazakis, H.E.; Stassinakis, A.N.; Volos, C.K.; Tsigopoulos, A.D. Underwater Optical Wireless Communications with Chromatic Dispersion and Time Jitter. Computation 2019, 7, 35. [Google Scholar] [CrossRef]
- Agrawal, G.G.P. Nonlinear Fiber Optics, 3rd ed.; Academic Press: San Diego, CA, USA, 2001. [Google Scholar]
- Crisp, J. Introduction to Fiber Optics, 2nd ed.; Newnes: Oxford, UK, 2001; Volume 1, ISBN 0750650303. [Google Scholar]
- Lu, H.; Zhao, W.; Xie, X. Analysis of temporal broadening of optical pulses by atmospheric dispersion in laser communication system. Opt. Commun. 2012, 285, 3169–3173. [Google Scholar] [CrossRef]
- Nistazakis, H.E.; Frantzeskakis, D.J.; Atai, J.; Malomed, B.A.; Efremidis, N.; Hizanidis, K. Multichannel pulse dynamics in a stabilized Ginzburg-Landau system. Phys. Rev. E Stat. Phys. Plasma Fluids Relat. Interdiscip. Top. 2002, 65, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Hizanidis, K.; Malomed, B.A.; Nistazakis, H.E.; Frantzeskakis, D.J. Stabilizing soliton transmission by third-order dispersion in dispersion-compensated fibre links. Pure Appl. Opt. 1998, 7, 57–62. [Google Scholar] [CrossRef]
- Stassinakis, A.N.; Nistazakis, H.E.; Peppas, K.P.; Tombras, G.S. Improving the availability of terrestrial FSO links over log-normal atmospheric turbulence channels using dispersive chirped Gaussian pulses. Opt. Laser Technol. 2013, 54, 329–334. [Google Scholar] [CrossRef]
- Djordjevic, G.T.; Petkovic, M.I.; Spasic, M.; Antic, D.S. Outage capacity of FSO link with pointing errors and link blockage. Opt. Express 2016, 24, 219–230. [Google Scholar] [CrossRef]
- Gappmair, W.; Hranilovic, S.; Leitgeb, E. Performance of PPM on terrestrial FSO links with turbulence and pointing errors. IEEE Commun. Lett. 2010, 14, 468–470. [Google Scholar] [CrossRef]
- Aarthi, G.; Ramachandra Reddy, G. Average spectral efficiency analysis of FSO links over turbulence channel with adaptive transmissions and aperture averaging. Opt. Commun. 2018, 410, 896–902. [Google Scholar] [CrossRef]
- Varotsos, G.K.; Nistazakis, H.E.; Petkovic, M.I.; Djordjevic, G.T.; Tombras, G.S. SIMO optical wireless links with nonzero boresight pointing errors over M modeled turbulence channels. Opt. Commun. 2017, 403, 391–400. [Google Scholar] [CrossRef]
- Varotsos, G.K.; Nistazakis, H.E.; Gappmair, W.; Sandalidis, H.G.; Tombras, G.S. DF relayed subcarrier FSO links over Malaga turbulence channels with phase noise and non-zero boresight pointing errors. Appl. Sci. 2018, 8, 664. [Google Scholar] [CrossRef]
- Jagadeesh, V.K.; Palliyembil, V.; Ansari, I.S.; Muthuchidambaranathan, P.; Qaraqe, K.A. Performance Analysis of Relaying FSO System over M -Distributed Turbulent Channel with Variable Gain AF Protocol. In Law and Regulation of Common Carriers in the Communications Industry, 2nd ed.; Springer: Singapore, 2019; ISBN 978-0-42-996785-6. [Google Scholar]
- Prabu, K.; Kumar, D.S.; Srinivas, T. Performance analysis of FSO links under Strong atmospheric turbulence conditions using various modulation schemes. Optik (Stuttg) 2014, 125, 5573–5581. [Google Scholar] [CrossRef]
- Sandalidis, H.G.; Tsiftsis, T.A. Outage probability and ergodic capacity of free-space optical links over strong turbulence. Electron. Lett. 2008, 44, 46–47. [Google Scholar] [CrossRef]
- Gappmair, W. Novel results on pulse-position modulation performance for terrestrial free-space optical links impaired by turbulent atmosphere and pointing errors. IET Commun. 2012, 6, 1300–1305. [Google Scholar] [CrossRef]
- Li, Y.; Liang, H.; Gao, C.; Miao, M.; Li, X. Temporal dispersion compensation for turbid underwater optical wireless communication links. Opt. Commun. 2019, 435, 355–361. [Google Scholar] [CrossRef]
- Vali, Z.; Gholami, A.; Ghassemlooy, Z.; Michelson, D.G. System parameters effect on the turbulent underwater optical wireless communications link. Optik (Stuttg) 2019, 198, 163153. [Google Scholar] [CrossRef]
- Majumdar, A.K. Advanced Free Space Optics (Fso)—A System Approach; Springer: New York, NY, USA, 2015; Volume 140. [Google Scholar]
- Kaushik, R.; Khandelwal, V.; Jain, R.C. A New Closed Form Approximation for BER for Optical Wireless Systems in Weak Atmospheric Turbulence. J. Opt. Commun. 2018, 39, 247–253. [Google Scholar] [CrossRef]
- Katsis, A.; Nistazakis, H.E.; Tombras, G.S. Bayesian and frequentist estimation of the performance of free space optical channels under weak turbulence conditions. J. Franklin Inst. 2009, 346, 315–327. [Google Scholar] [CrossRef]
- McNeil, G.T. Metrical Fundamentals of Underwater Lens System. Opt. Eng. 1977, 16, 162128. [Google Scholar] [CrossRef]
- Driben, R.; Malomed, B.A.; Chu, P.L. Transmission of pulses in a dispersion-managed fiber link with extra nonlinear segments. Opt. Commun. 2005, 245, 227–236. [Google Scholar] [CrossRef]
- Varotsos, G.K.; Nistazakis, H.E.; Volos, C.K.; Tombras, G.S. FSO links with diversity pointing errors and temporal broadening of the pulses over weak to strong atmospheric turbulence channels. Optik (Stuttg) 2016, 127, 3402–3409. [Google Scholar] [CrossRef]
- Varotsos, G.K.; Stassinakis, A.N.; Nistazakis, H.E.; Tsigopoulos, A.D.; Peppas, K.P.; Aidinis, C.J.; Tombras, G.S. Probability of fade estimation for FSO links with time dispersion and turbulence modeled with the gamma–gamma or the I-K distribution. Optik (Stuttg) 2014, 125, 7191–7197. [Google Scholar] [CrossRef]
- Papoulis, A. Probability, Random Variables, and Stochastic Processes, 3rd ed.; McGraw-Hill, Inc.: New York, NY, USA, 1991; ISBN 0070484775. [Google Scholar]
- Weber, H.; Arfken, G.B. Essentials of Math Methods for Physicists; Elsevier: Amsterdam, The Netherlands, 1966; ISBN 978-1-48-320059-0. [Google Scholar]
- Farid, A.A.; Hranilovic, S. Outage capacity optimization for free-space optical links with pointing errors. J. Light. Technol. 2007, 25, 1702–1710. [Google Scholar] [CrossRef]
- Ninos, M.P.; Nistazakis, H.E.; Tombras, G.S. On the BER performance of FSO links with multiple receivers and spatial jitter over gamma-gamma or exponential turbulence channels. Optik (Stuttg) 2017, 138, 269–279. [Google Scholar] [CrossRef]
- Barrios, R.; Dios, F. Probability of fade and BER performance of FSO links over the exponentiated Weibull fading channel under aperture averaging. Unmanned/Unattended Sens. Sens. Netw. IX 2012, 8540, 85400D. [Google Scholar] [CrossRef]
- Vetelino, F.S.; Young, C.; Andrews, L. Fade statistics and aperture averaging for Gaussian beam waves in moderate-to-strong turbulence. Appl. Opt. 2007, 46, 3780–3789. [Google Scholar] [CrossRef]
- Wang, C.; Yu, H.-Y.; Zhu, Y.-J. A Long Distance Underwater Visible Light Communication System With Single Photon Avalanche Diode. IEEE Photonics J. 2016, 8, 1–11. [Google Scholar] [CrossRef]
- Partan, J.; Kurose, J.; Levine, B.N. A survey of practical issues in underwater networks. ACM SIGMOBILE Mob. Comput. Commun. Rev. 2007, 11, 23. [Google Scholar] [CrossRef]
- Farr, N.; Chave, A.D.; Freitag, L.; Preisig, J.; White, S.N.; Yoerger, D.; Sonnichsen, F. Optical Modem Technology for Seafloor Observatories. In OCEANS 2006; IEEE: Piscataway, NJ, USA, 2006; pp. 1–6. ISBN 1424401143. [Google Scholar]


| Wavelength (λ) in [nm] | Refractive Index (n) | GVD Parameter (β2) in [ps2/km] |
|---|---|---|
| 470 | 1.3461 | 86.74 |
| 500 | 1.3445 | 83.05 |
| 530 | 1.3432 | 79.53 |
| 560 | 1.3420 | 76.22 |
| 590 | 1.3411 | 73.10 |
| 620 | 1.3402 | 70.19 |
| 650 | 1.3395 | 67.46 |
| 680 | 1.3388 | 64.91 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roumelas, G.D.; Nistazakis, H.E.; Stassinakis, A.N.; Varotsos, G.K.; Tsigopoulos, A.D.; Tombras, G.S. Time Jitter, Turbulence and Chromatic Dispersion in Underwater Optical Wireless Links. Technologies 2020, 8, 3. https://doi.org/10.3390/technologies8010003
Roumelas GD, Nistazakis HE, Stassinakis AN, Varotsos GK, Tsigopoulos AD, Tombras GS. Time Jitter, Turbulence and Chromatic Dispersion in Underwater Optical Wireless Links. Technologies. 2020; 8(1):3. https://doi.org/10.3390/technologies8010003
Chicago/Turabian StyleRoumelas, George D., Hector E. Nistazakis, Argyris N. Stassinakis, George K. Varotsos, Andreas D. Tsigopoulos, and George S. Tombras. 2020. "Time Jitter, Turbulence and Chromatic Dispersion in Underwater Optical Wireless Links" Technologies 8, no. 1: 3. https://doi.org/10.3390/technologies8010003
APA StyleRoumelas, G. D., Nistazakis, H. E., Stassinakis, A. N., Varotsos, G. K., Tsigopoulos, A. D., & Tombras, G. S. (2020). Time Jitter, Turbulence and Chromatic Dispersion in Underwater Optical Wireless Links. Technologies, 8(1), 3. https://doi.org/10.3390/technologies8010003






