Electron Energy-Loss Spectroscopy Method for Thin-Film Thickness Calculations with a Low Incident Energy Electron Beam
Abstract
1. Introduction
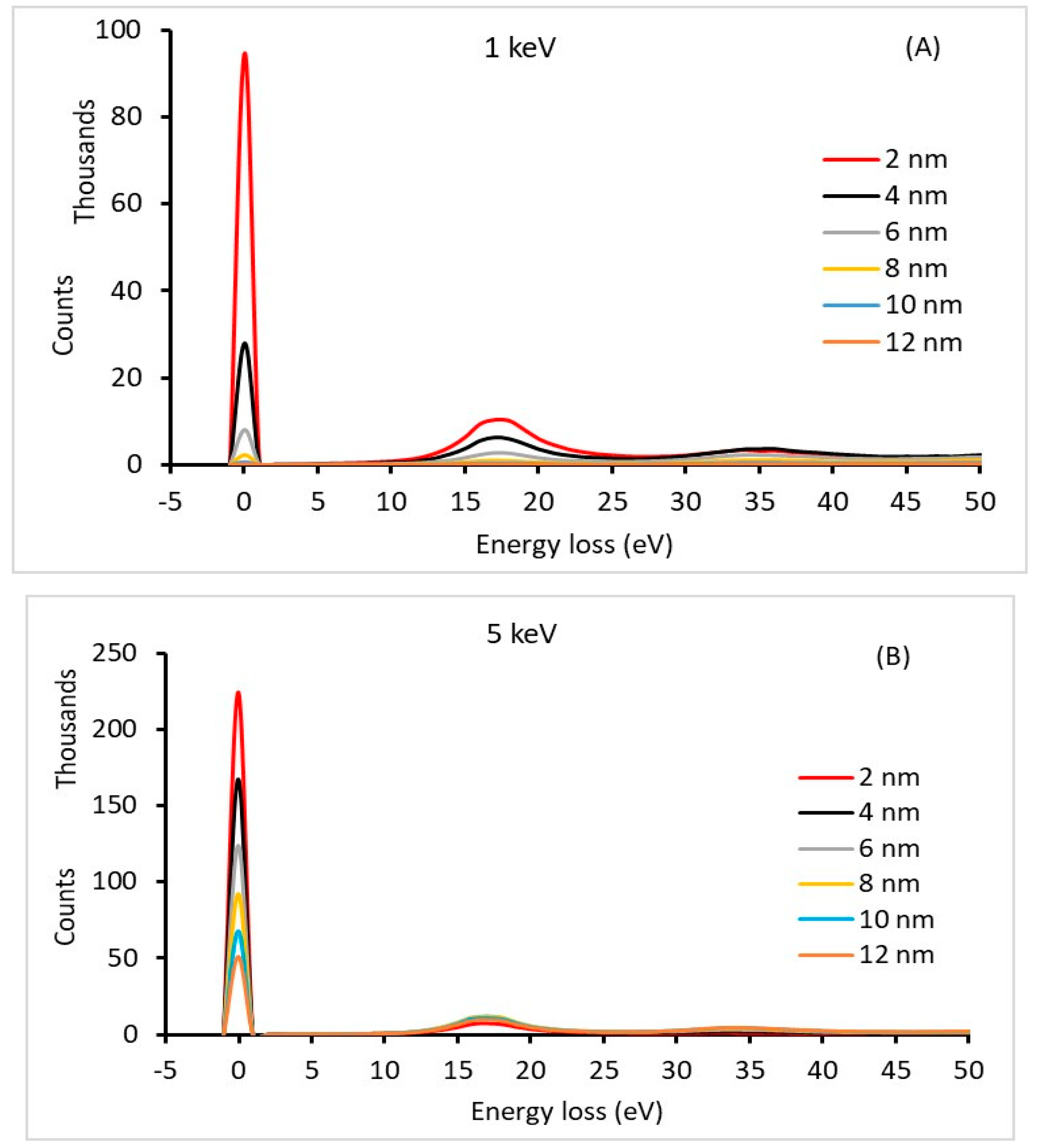
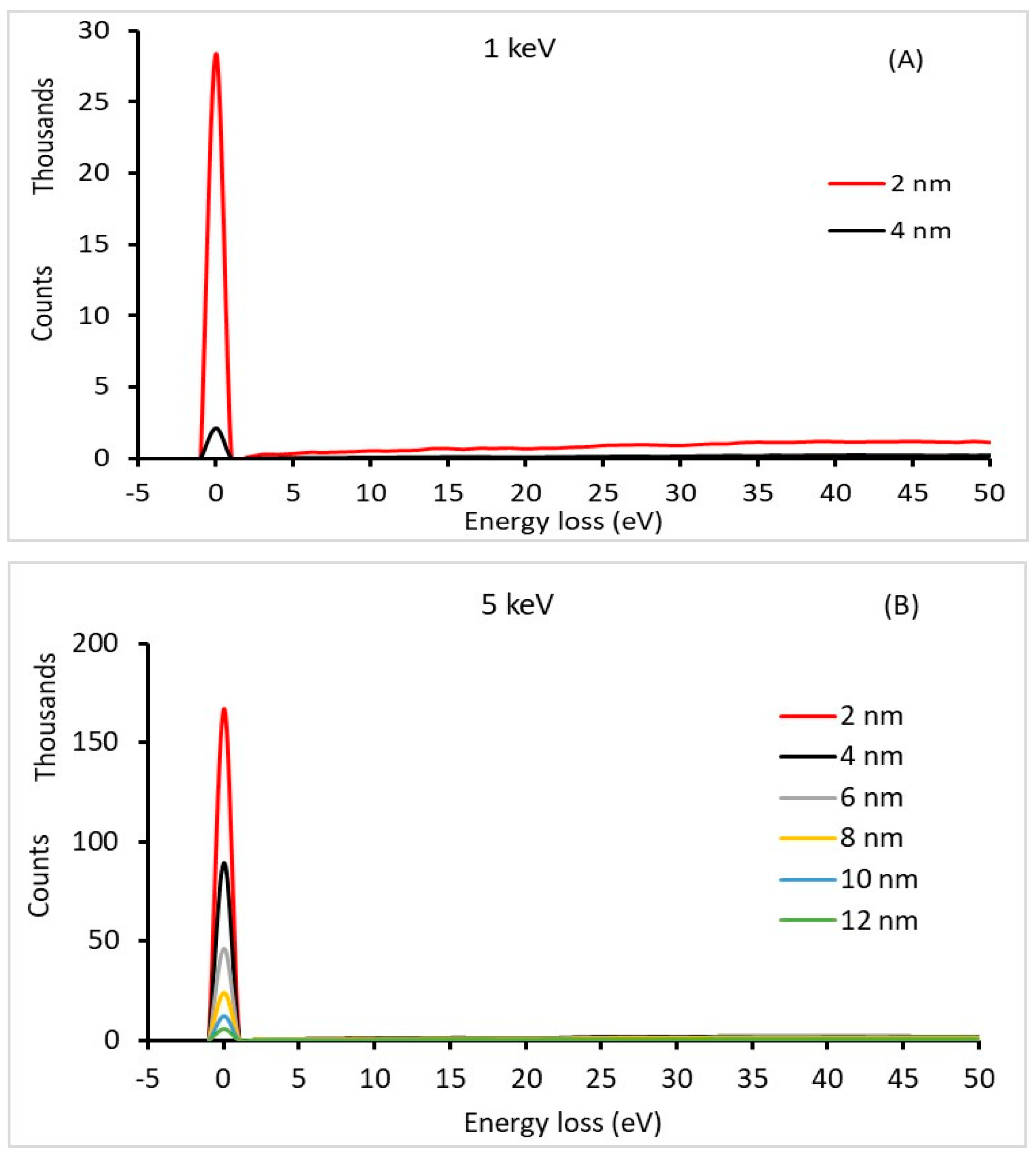
2. Monte Carlo Simulations
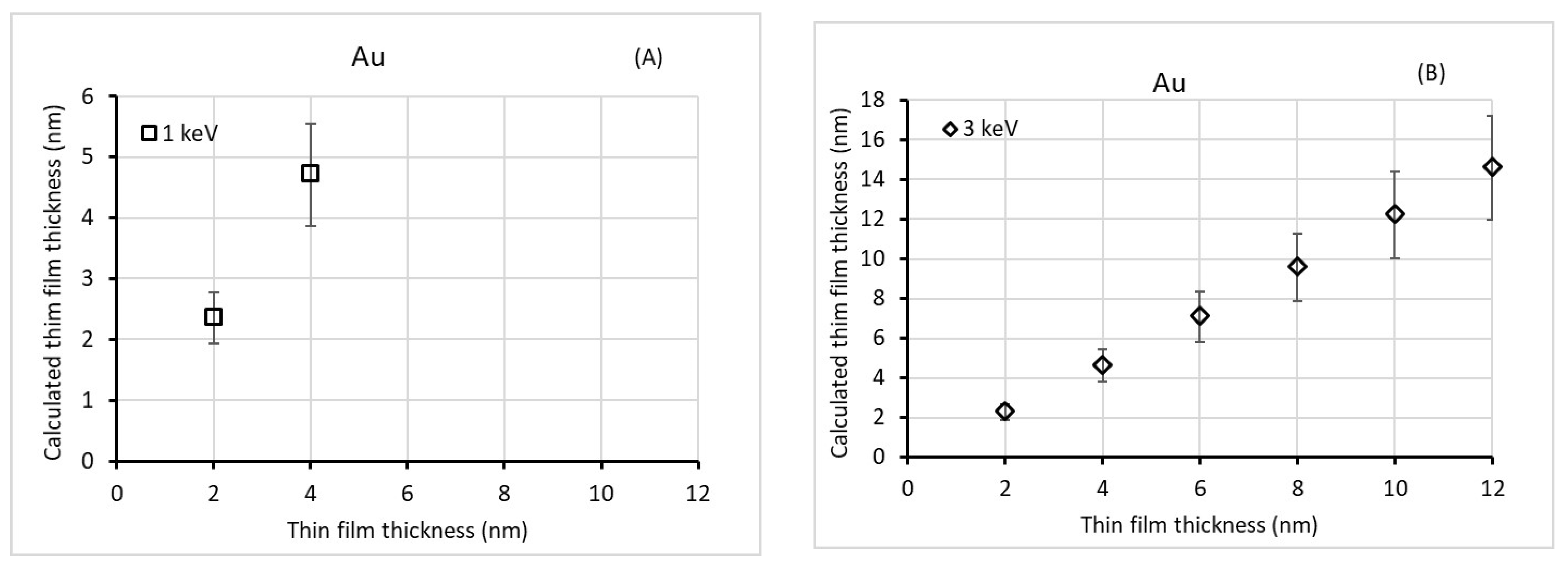
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Biebl, F.; Glawar, R.; Jalali, A.; Ansari, F.; Haslhofer, B.; de Boer, P.; Sihn, W. A conceptual model to enable prescriptive maintenance for etching equipment in semiconductor manufacturing. Procedia CIRP 2020, 88, 64–69. [Google Scholar] [CrossRef]
- Weber, A. Smart manufacturing in the semiconductor industry: An evolving nexus of business drivers, technologies, and standards. In Smart Manufacturing; Elsevier: New York, NY, USA, 2020; pp. 59–105. [Google Scholar] [CrossRef]
- Huang, H.-T.; Terry, F.L., Jr. Spectroscopic ellipsometry and reflectometry from gratings (Scatterometry) for critical dimension measurement and in situ, real-time process monitoring. Thin Solid Films 2004, 455–456, 828–836. [Google Scholar] [CrossRef]
- Llovet, X.; Moy, A.; Pinard, P.T.; Fournelle, J.H. Electron probe microanalysis: A review of recent developments and applications in materials science and engineering. Prog. Mater. Sci. 2021, 116, 100673. [Google Scholar] [CrossRef]
- Terborg, R.; Kim, K.J.; Hodoroaba, V.-D. Determination of Thin Film Thickness and Composition using Energy Dispersive EPMA. Microsc. Microanal. 2022, 28, 672–673. [Google Scholar] [CrossRef]
- Terborg, R.; Kim, K.J.; Hodoroaba, V. Elemental composition and thickness determination of thin films by electron probe microanalysis. Surf. Interface Anal. 2023, 55, 496–500. [Google Scholar] [CrossRef]
- Sokolov, S.A.; Milovanov, R.A.; Sidorov, L.N. Determination of the Thickness of Thin Films Based on Scanning Electron Microscopy and Energy Dispersive X-Ray Analysis. J. Surf. Investig. 2019, 13, 836–847. [Google Scholar] [CrossRef]
- Egerton, R.F. Electron Energy-Loss Spectroscopy in the Electron Microscope; Springer US: Boston, MA, USA, 2011. [Google Scholar] [CrossRef]
- Egerton, R.F.; Cheng, S.C. Measurement of local thickness by electron energy-loss spectroscopy. Ultramicroscopy 1987, 21, 231–244. [Google Scholar] [CrossRef]
- Niedrig, H. Film-thickness Determination in Electron Microscopy: The Electron Backscattering Method. Opt. Acta 1977, 24, 679–691. [Google Scholar] [CrossRef]
- Egerton, R.F.; Malac, M. EELS in the TEM. J. Electron. Spectros. Relat. Phenom. 2005, 143, 43–50. [Google Scholar] [CrossRef]
- Stephens, A.P. Quantitative microanalysis by electron energy-loss spectroscopy: Two corrections. Ultramicroscopy 1980, 5, 343–349. [Google Scholar] [CrossRef]
- Assa’d, A.M.D. Monte Carlo computation of the influence of carbon contamination layer on the energy distribution of backscattered electrons emerging from Al and Au. Jordan J. Phys. 2019, 12, 37–44. [Google Scholar]
- Egerton, R.F. Electron energy-loss spectroscopy in the TEM. Rep. Prog. Phys. 2009, 72, 016502. [Google Scholar] [CrossRef]
- Malis, T.; Cheng, S.C.; Egerton, R.F. EELS log-ratio technique for specimen-thickness measurement in the TEM. J. Electron. Microsc. Tech. 1988, 8, 193–200. [Google Scholar] [CrossRef] [PubMed]
- Botton, G.A.; L’espérance, G.; Gallerneault, C.E.; Ball, M.D. Volume fraction measurement of dispersoids in a thin foil by parallel energy-loss spectroscopy: Development and assessment of the technique. J. Microsc. 1995, 180, 217–229. [Google Scholar] [CrossRef]
- Haasch, R.T. X-Ray Photoelectron Spectroscopy (XPS) and Auger Electron Spectroscopy (AES). In Practical Materials Characterization; Springer: New York, NY, USA, 2014; pp. 93–132. [Google Scholar] [CrossRef]
- Leapman, R.D.; Fiori, C.E.; Swyt, C.R. Mass thickness determination by electron energy loss for quantitative X-ray microanalysis in biology. J. Microsc. 1984, 133, 239–253. [Google Scholar] [CrossRef] [PubMed]
- Joy, D.C.; Egerton, R.F.; Maher, D.M. Progress in the quantitation of EELS. In Scanning Electron Microscopy; SEM: Washington, DC, USA, 1979; Volume 2, pp. 817–826. [Google Scholar]
- Iakoubovskii, K.; Mitsuishi, K.; Nakayama, Y.; Furuya, K. Thickness measurements with electron energy loss spectroscopy. Microsc. Res. Tech. 2008, 71, 626–631. [Google Scholar] [CrossRef] [PubMed]
- Jaber, A.M.D.; Kawariq, H.H.; Walker, C.G.H.; Mousa, M.S. Monte Carlo Simulation of Free-standing Thin Films under Low Energy Electron Bombardment: Electron Inelastic Mean Free Path (IMFP) Determination Using Elastic Peak of the Transmitted Electrons. Jordan J. Phys. 2023, 16, 381–393. [Google Scholar] [CrossRef]
- Tanuma, S.; Powell, C.J.; Penn, D.R. Calculations of electron inelastic mean free paths. IX. Data for 41 elemental solids over the 50 eV to 30 keV range. Surf. Interface Anal. 2011, 43, 689–713. [Google Scholar] [CrossRef]
- Kieft, E.; Bosch, E. Refinement of Monte Carlo simulations of electron–specimen interaction in low-voltage SEM. J. Phys. D Appl. Phys. 2008, 41, 215310. [Google Scholar] [CrossRef]
- Ashley, J.C. Interaction of low-energy electrons with condensed matter: Stopping powers and inelastic mean free paths from optical data. J. Electron. Spectros. Relat. Phenom. 1988, 46, 199–214. [Google Scholar] [CrossRef]
- Kenway, P.B.; Duke, P.J. X-Ray Optics and Microanalysis 1992, Proceedings of the 13th INT Conference, Manchester, UK, 31 August-4 September 1992; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar] [CrossRef]
- Demers, H.; Poirier-Demers, N.; de Jonge, N.; Drouin, D. Three-Dimensional Electron Microscopy Simulation with the CASINO Monte Carlo Software. Microsc. Microanal. 2011, 17, 612–613. [Google Scholar] [CrossRef]
- Agostinelli, S.; Allison, J.; Amako, K.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.; et al. Geant4—A simulation toolkit. Nucl. Instrum. Methods Phys. Res. A 2003, 506, 250–303. [Google Scholar] [CrossRef]
- Rising, M.E.; Armstrong, J.C.; Bolding, S.R.; Brown, F.B.; Bull, J.S.; Burke, T.P.; Clark, A.R.; Dixon, D.A.; Forster, R.A., III; Giron, J.F.; et al. MCNP® Code V.6.3.0 Release Notes; Los Alamos National Laboratory (LANL): Los Alamos, NM, USA, 2023. [Google Scholar] [CrossRef]
- Sobel’man, I.I.; Vainshtein, L.A.; Yukov, E.A. Theory of Atomic Collisions; Springer: New York, NY, USA, 1933. [Google Scholar]
- Massey, H.S.W. Theory of Atomic Collisions. In Atoms II/Atome II. Encyclopedia of Physics/Handbuch der Physik; Springer: Berlin/Heidelberg, Germany, 1956; pp. 232–306. [Google Scholar] [CrossRef]
- Werner, W.S.M. Electron transport in solids for quantitative surface analysis. Surf. Interface Anal. 2001, 31, 141–176. [Google Scholar] [CrossRef]
- Reimer, L. Scanning Electron Microscopy: Physics of Image Formation and Microanalysis, Second Edition. Meas. Sci. Technol. 2000, 11, 1826. [Google Scholar] [CrossRef]
- Goldstein, J.I.; Newbury, D.E.; Echlin, P.; Joy, D.C.; Fiori, C.; Lifshin, E. Scanning Electron Microscopy and X-Ray Microanalysis; Springer US: Boston, MA, USA, 1981. [Google Scholar] [CrossRef]
- Walker, C.G.H.; El-Gomati, M.M.; Assa’d, A.M.D.; Zadražil, M. The secondary electron emission yield for 24 solid elements excited by primary electrons in the range 250–5000 ev: A theory/experiment comparison. Scanning 2008, 30, 365–380. [Google Scholar] [CrossRef]
- Assa’d, A.M.D. Monte Carlo calculation of the backscattering coefficient of thin films of low on high atomic number materials and the reverse as a function of the incident electron energy and film thickness. Appl. Phys. A 2018, 124, 699. [Google Scholar] [CrossRef]


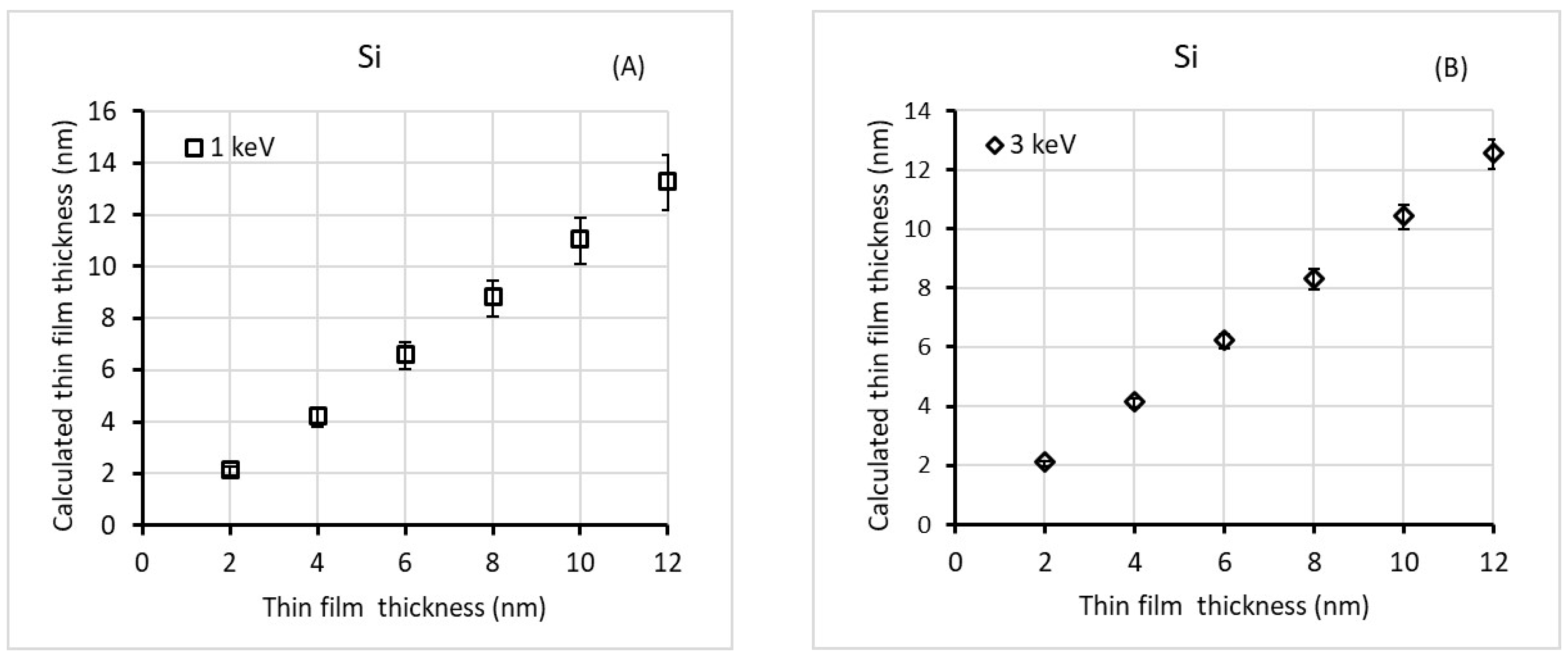


| t (nm) | 1 keV | 3 keV | 5 keV | 7.5 keV | 10 keV | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | |
| 2.00 | 2.10 | 0.050 | 2.04 | 0.020 | 2.03 | 0.015 | 2.04 | 0.020 | 2.08 | 0.040 |
| 4.00 | 4.24 | 0.060 | 4.08 | 0.020 | 4.07 | 0.018 | 4.06 | 0.015 | 4.10 | 0.025 |
| 6.00 | 6.38 | 0.063 | 6.13 | 0.022 | 6.08 | 0.013 | 6.08 | 0.013 | 6.16 | 0.027 |
| 8.00 | 8.44 | 0.055 | 8.19 | 0.024 | 8.11 | 0.014 | 8.08 | 0.010 | 8.19 | 0.024 |
| 10.00 | 10.77 | 0.077 | 10.29 | 0.029 | 10.19 | 0.019 | 10.08 | 0.008 | 10.21 | 0.021 |
| 12.00 | - | - | 12.39 | 0.033 | 12.24 | 0.020 | 12.15 | 0.013 | 12.34 | 0.028 |
| t (nm) | 1 keV | 3 keV | 5 keV | 7.5 keV | 10 keV | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | |
| 2.00 | 2.11 | 0.055 | 2.06 | 0.030 | 2.05 | 0.025 | 2.04 | 0.020 | 2.06 | 0.030 |
| 4.00 | 4.31 | 0.078 | 4.10 | 0.025 | 4.06 | 0.015 | 4.09 | 0.023 | 4.06 | 0.015 |
| 6.00 | 6.51 | 0.085 | 6.20 | 0.033 | 6.13 | 0.022 | 6.10 | 0.017 | 6.11 | 0.018 |
| 8.00 | 8.68 | 0.085 | 8.29 | 0.036 | 8.17 | 0.021 | 8.15 | 0.019 | 8.17 | 0.021 |
| 10.00 | 10.99 | 0.099 | 10.40 | 0.040 | 10.29 | 0.029 | 10.19 | 0.019 | 10.17 | 0.017 |
| 12.00 | 13.25 | 0.104 | 12.54 | 0.045 | 12.28 | 0.023 | 12.22 | 0.018 | 12.23 | 0.019 |
| t (nm) | 1 keV | 3 keV | 5 keV | 7.5 keV | 10 keV | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | |
| 2.00 | 2.28 | 0.140 | 2.15 | 0.075 | 2.07 | 0.035 | 2.08 | 0.040 | 2.07 | 0.035 |
| 4.00 | 4.63 | 0.158 | 4.30 | 0.075 | 4.10 | 0.025 | 4.16 | 0.040 | 4.13 | 0.033 |
| 6.00 | 6.92 | 0.153 | 6.57 | 0.095 | 6.25 | 0.042 | 6.24 | 0.040 | 6.24 | 0.040 |
| 8.00 | - | - | 8.87 | 0.109 | 8.39 | 0.049 | 8.40 | 0.050 | 8.34 | 0.043 |
| 10.00 | - | - | 11.2 | 0.120 | 10.62 | 0.062 | 10.50 | 0.050 | 10.50 | 0.050 |
| 12.00 | - | - | 13.5 | 0.125 | 12.90 | 0.075 | 12.70 | 0.070 | 12.60 | 0.050 |
| t (nm) | 1 keV | 3 keV | 5 keV | 7.5 keV | 10 keV | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | |
| 2.00 | 2.32 | 0.160 | 2.20 | 0.100 | 2.15 | 0.075 | 2.08 | 0.040 | 2.10 | 0.050 |
| 4.00 | 4.66 | 0.165 | 4.47 | 0.118 | 4.27 | 0.068 | 4.19 | 0.048 | 4.21 | 0.053 |
| 6.00 | 6.87 | 0.145 | 6.80 | 0.133 | 6.49 | 0.082 | 6.35 | 0.060 | 6.34 | 0.057 |
| 8.00 | - | - | 9.20 | 0.150 | 8.75 | 0.094 | 8.52 | 0.065 | 8.46 | 0.058 |
| 10.00 | - | - | 11.59 | 0.159 | 11.05 | 0.105 | 10.71 | 0.071 | 10.59 | 0.059 |
| 12.00 | - | - | 14.2 | 0.183 | 13.4 | 0.116 | 12.93 | 0.078 | 12.82 | 0.068 |
| t (nm) | 1 keV | 3 keV | 5 keV | 7.5 keV | 10 keV | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | |
| 2.00 | 2.30 | 0.150 | 2.17 | 0.085 | 2.14 | 0.070 | 2.12 | 0.060 | 2.11 | 0.055 |
| 4.00 | 4.48 | 0.120 | 4.41 | 0.104 | 4.30 | 0.075 | 4.23 | 0.058 | 4.21 | 0.053 |
| 6.00 | - | - | 6.71 | 0.118 | 6.49 | 0.082 | 6.40 | 0.067 | 6.30 | 0.050 |
| 8.00 | - | - | 9.04 | 0.130 | 8.74 | 0.093 | 8.57 | 0.071 | 8.39 | 0.049 |
| 10.00 | - | - | 11.35 | 0.135 | 11.04 | 0.104 | 10.84 | 0.084 | 10.54 | 0.054 |
| 12.00 | - | - | 13.79 | 0.149 | 13.38 | 0.115 | 13.05 | 0.088 | 12.74 | 0.062 |
| t (nm) | 1 keV | 3 keV | 5 keV | 7.5 keV | 10 keV | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | tc | ±∆ tc | |
| 2.00 | 2.36 | 0.180 | 2.26 | 0.130 | 2.18 | 0.090 | 2.17 | 0.085 | 2.15 | 0.075 |
| 4.00 | 4.71 | 0.178 | 4.62 | 0.155 | 4.41 | 0.103 | 4.38 | 0.095 | 4.33 | 0.083 |
| 6.00 | - | - | 7.10 | 0.183 | 6.75 | 0.125 | 6.68 | 0.113 | 6.54 | 0.090 |
| 8.00 | - | - | 9.57 | 0.196 | 9.09 | 0.136 | 8.99 | 0.124 | 8.81 | 0.101 |
| 10.00 | - | - | 12.22 | 0.222 | 11.55 | 0.155 | 11.39 | 0.139 | 11.16 | 0.116 |
| 12.00 | - | - | 14.60 | 0.217 | 14.01 | 0.168 | 13.81 | 0.151 | 13.44 | 0.120 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jaber, A.M.D.; Alsoud, A.; Al-Bashaish, S.R.; Al Dmour, H.; Mousa, M.S.; Trčka, T.; Holcman, V.; Sobola, D. Electron Energy-Loss Spectroscopy Method for Thin-Film Thickness Calculations with a Low Incident Energy Electron Beam. Technologies 2024, 12, 87. https://doi.org/10.3390/technologies12060087
Jaber AMD, Alsoud A, Al-Bashaish SR, Al Dmour H, Mousa MS, Trčka T, Holcman V, Sobola D. Electron Energy-Loss Spectroscopy Method for Thin-Film Thickness Calculations with a Low Incident Energy Electron Beam. Technologies. 2024; 12(6):87. https://doi.org/10.3390/technologies12060087
Chicago/Turabian StyleJaber, Ahmad M. D. (Assa’d), Ammar Alsoud, Saleh R. Al-Bashaish, Hmoud Al Dmour, Marwan S. Mousa, Tomáš Trčka, Vladimír Holcman, and Dinara Sobola. 2024. "Electron Energy-Loss Spectroscopy Method for Thin-Film Thickness Calculations with a Low Incident Energy Electron Beam" Technologies 12, no. 6: 87. https://doi.org/10.3390/technologies12060087
APA StyleJaber, A. M. D., Alsoud, A., Al-Bashaish, S. R., Al Dmour, H., Mousa, M. S., Trčka, T., Holcman, V., & Sobola, D. (2024). Electron Energy-Loss Spectroscopy Method for Thin-Film Thickness Calculations with a Low Incident Energy Electron Beam. Technologies, 12(6), 87. https://doi.org/10.3390/technologies12060087








