Atomic Quantum Technologies for Quantum Matter and Fundamental Physics Applications
Abstract
1. Introduction
2. Theoretical and Experimental Concepts and Tools
2.1. Quantum Simulators Platforms
2.1.1. Platforms Overview
Hardware Developments towards Quantum Communication
Ultracold Atoms
2.1.2. Atoms with Tunable Short-Range Interaction Strength
2.1.3. Atoms in Optical Lattices
2.1.4. Trapped Ions
2.1.5. Rydberg Atoms
2.1.6. Atoms with Dipolar Interactions
2.1.7. Atoms in Optical Cavities
2.1.8. Miniaturization and Atom Chips Technology
2.2. Theoretical and Simulational Paradigms for Atomic Systems
2.2.1. Driven-Dissipative Quantum Systems
Open Systems: Reservoir Engineering
Describing Dissipation in Quantum Systems: Gorini-Kossakowski-Sudarshan- Lindblad Master Equation
- Born Approximation: this approximation assumes that system and environment are weakly coupled, implying that the dynamics induced by this coupling are small compared to the system or environment ones given by .
- Markov Approximation: Firstly, it implies the S-E coupling to be frequency-independent over short timescales, that we can define with a rate . Secondly, it requires that the information transmitted from the system into the environment, decays exponentially fast in a way that this information cannot return to the system S itself. This, together with the Born approximation, implies that the environment can be considered static throughout the evolution.This approximation is valid in a wide range of scenarios; formalising the condition that the bath correlations decay in timescales . These , on the other hand, are given by the inverse of the spectral width of the bath [301]. E.g., if we assume a bath in thermal equilibrium, the decay of correlations will be much faster than the rate of change of the system [302,303].
Stochastic Unraveling Description of Open Quantum System
Beyond the Born-Markov Approximation
2.2.2. Quantum System Description via Tensor Networks
Matrix Product States (MPS) and Operators (MPO)
Algorithms with MPSs and MPOs: Equilibrium, Dynamics and Open Systems
Beyond One-Dimension
Tensor Networks beyond the Simulation of Quantum Matter
2.3. Quantum Computing
- Architecture scalability. The qubit is a well-defined physical system that can be isolated from the external environment. Moreover, increasing the number of qubits does not modify the device’s functioning principles.
- Initialization ability. It is possible to initialise qubits in a custom desired state.
- Coherence. Qubits have long coherence times.
- Universality. It is possible to perform quantum gate operations that form a universal set of gates. In this way, every quantum operation can be realized as a composition of gates from the universal set.
- Addressability. The device is capable of addressing and selecting the qubit that is intended to be measured, isolating it from the remaining ones and performing the measurement without information leaking or cross-talks.
2.3.1. Fault-Tolerant, Error-Corrected Quantum Computation
2.3.2. Quantum Optimization
Quantum Variational Algorithms
2.3.3. Quantum Control
2.4. Quantum Metrology and Sensing
2.4.1. Atomic Clocks and Atom Interferometry
Atomic Clocks
Atom Interferometry
2.4.2. Entanglement as a Resource for Quantum Metrology and Sensing
3. Condensed Matter and Many-Body Physics
3.1. Condensed Matter and Many-Body Physics: Evergreen Paradigms
3.1.1. BEC-BCS Crossover
3.1.2. Commensurate—Incommensurate Transitions
Aubry-like Transitions
Meissner to Vortex Transition
3.2. Condensed Matter: Sprouting Paradigms
3.2.1. Eigenstate Thermalisation Hypothesis and Anderson/Many-Body Localisation
3.2.2. Periodically-Driven Systems: Floquet Engineering
3.2.3. Measurement, Control and Feedback in Open Quantum Systems
3.2.4. Out-of-Equilibrium Systems, Dynamical Phase Transitions and Dissipation
4. Fundamental Physics
4.1. Electric Dipole Moment
4.2. Fundamental Equations and Symmetries
4.3. Ultracompact Stars and Quark Matter
5. Cosmology and Astrophysics
5.1. Gravity and General Relativity Tests
5.1.1. Measurement of Big G
5.1.2. Variation of Fundamental Constants
5.1.3. Equivalence Principle Tests
5.1.4. Detection of Gravitational Waves
5.1.5. Quest for Ultra-Light Dark Matter
5.1.6. A Special Perspective
5.2. Quantum Simulators for Gravity and Cosmology Problems
5.2.1. What Is Meant by Analogue Gravity
5.2.2. Analogue Horizons and Hawking Radiation
5.2.3. Information-Loss Paradox
5.2.4. Viscosity to Entropy Density Ratio
6. Foundations of Quantum Mechanics
7. Quantum Simulators for Biology and Chemistry
7.1. Quantum-like Paradigm and the Brain
7.2. Transport and Quantum Effects in Biology
8. Responsible Research and Innovation, Research-Based Education and Outreach in Quantum Technologies
9. Discussion
10. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Adams, A.; Carr, L.D.; Schäfer, T.; Steinberg, P.; Thomas, J.E. Strongly correlated quantum fluids: Ultracold quantum gases, quantum chromodynamic plasmas and holographic duality. New J. Phys. 2012, 14, 115009. [Google Scholar] [CrossRef]
- Pezzè, L.; Smerzi, A.; Oberthaler, M.K.; Schmied, R.; Treutlein, P. Quantum metrology with nonclassical states of atomic ensembles. Rev. Mod. Phys. 2018, 90, 035005. [Google Scholar] [CrossRef]
- Bauer, B.; Bravyi, S.; Motta, M.; Chan, G.K.L. Quantum Algorithms for Quantum Chemistry and Quantum Materials Science. Chem. Rev. 2020, 120, 12685–12717. [Google Scholar] [CrossRef] [PubMed]
- Chaikin, P.M.; Lubensky, T.C. Principles of Condensed Matter Physics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar] [CrossRef]
- Baym, G. BCS from Nuclei and Neutron Stars to Quark Matter and Cold Atoms. Int. J. Mod. Phys. B 2010, 24, 3968–3982. [Google Scholar] [CrossRef]
- Ball, P. Beyond the bond. Nature 2011, 469, 26–28. [Google Scholar] [CrossRef] [PubMed]
- Al-Khalili, J.; McFadden, J. Life on the Edge: The Coming of Age of Quantum Biology; Bantam Press: London, UK, 2014. [Google Scholar]
- Cao, J.; Cogdell, R.J.; Coker, D.F.; Duan, H.G.; Hauer, J.; Kleinekathöfer, U.; Jansen, T.L.C.; Mančal, T.; Miller, R.J.D.; Ogilvie, J.P.; et al. Quantum biology revisited. Sci. Adv. 2020, 6, eaaz4888. [Google Scholar] [CrossRef] [PubMed]
- Feynman, R.P. Simulating physics with computers. Int. J. Theor. Phys. 1982, 21, 467–488. [Google Scholar] [CrossRef]
- Barzanjeh, S.; Xuereb, A.; Gröblacher, S.; Paternostro, M.; Regal, C.A.; Weig, E.M. Optomechanics for quantum technologies. Nat. Phys. 2022, 18, 15–24. [Google Scholar] [CrossRef]
- Atatüre, M.; Englund, D.; Vamivakas, N.; Lee, S.Y.; Wrachtrup, J. Material platforms for spin-based photonic quantum technologies. Nat. Rev. Mater. 2018, 3, 38–51. [Google Scholar] [CrossRef]
- Pelucchi, E.; Fagas, G.; Aharonovich, I.; Englund, D.; Figueroa, E.; Gong, Q.; Hannes, H.; Liu, J.; Lu, C.Y.; Matsuda, N.; et al. The potential and global outlook of integrated photonics for quantum technologies. Nat. Rev. Phys. 2022, 4, 194–208. [Google Scholar] [CrossRef]
- Debnath, S.; Linke, N.M.; Figgatt, C.; Landsman, K.A.; Wright, K.; Monroe, C. Demonstration of a small programmable quantum computer with atomic qubits. Nature 2016, 536, 63–66. [Google Scholar] [CrossRef] [PubMed]
- Postler, L.; Heuβen, S.; Pogorelov, I.; Rispler, M.; Feldker, T.; Meth, M.; Marciniak, C.D.; Stricker, R.; Ringbauer, M.; Blatt, R.; et al. Demonstration of fault-tolerant universal quantum gate operations. Nature 2022, 605, 675–680. [Google Scholar] [CrossRef] [PubMed]
- Wurtz, J.; Bylinskii, A.; Braverman, B.; Amato-Grill, J.; Cantu, S.H.; Huber, F.; Lukin, A.; Liu, F.; Weinberg, P.; Long, J.; et al. Aquila: QuEra’s 256-qubit neutral-atom quantum computer. arXiv 2023, arXiv:2306.11727. [Google Scholar]
- DLR Institute of Quantum Technologies. DLR QCI Awards Contract Worth 29 Million Euros for the Development of a Quantum Computer Based on Neutral Atoms. Available online: https://qci.dlr.de/en/dlr-qci-awards-contract-worth-29-million-euros-for-the-development-of-a-quantum-computer-based-on-neutral-atoms/ (accessed on 1 February 2023).
- Pasqal. Towards Regenerative Quantum Computing with Proven Positive Sustainability Impact; Pasqal: Massy, France, 2023. [Google Scholar]
- Atom Computing. High Scalable Quantum Computing with Atomic Arrays; Atom Computing: Berkeley, CA, USA, 2023. [Google Scholar]
- Zapata Computing. The Near Term Promise of Quantum Generative AI; Zapata Computing: Boston, MA, USA, 2023. [Google Scholar]
- Bloch, I.; Dalibard, J.; Nascimbène, S. Quantum simulations with ultracold quantum gases. Nat. Phys. 2012, 8, 267. [Google Scholar] [CrossRef]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Theory of Superconductivity. Phys. Rev. 1957, 108, 1175–1204. [Google Scholar] [CrossRef]
- Greiner, M.; Regal, C.; Jin, D.S. Emergence of a Molecular Bose-Einstein Condensate from a Fermi Gas. Nature 2003, 426, 537–540. [Google Scholar] [CrossRef]
- Zwierlein, M.W.; Stan, C.A.; Schunck, C.H.; Raupach, S.M.F.; Gupta, S.; Hadzibabic, Z.; Ketterle, W. Observation of Bose-Einstein Condensation of Molecules. Phys. Rev. Lett. 2003, 91, 250401. [Google Scholar] [CrossRef]
- Chiu, C.S.; Ji, G.; Mazurenko, A.; Greif, D.; Greiner, M. Quantum State Engineering of a Hubbard System with Ultracold Fermions. Phys. Rev. Lett. 2018, 120, 243201. [Google Scholar] [CrossRef] [PubMed]
- Greiner, M.; Mandel, O.; Esslinger, T.; Hänsch, T.W.; Bloch, I. Quantum phase transition from a superfluid to a Mott insulator in a gas of ultracold atoms. Nature 2002, 415, 39–44. [Google Scholar] [CrossRef] [PubMed]
- Endres, M.; Fukuhara, T.; Pekker, D.; Cheneau, M.; Schauss, P.; Gross, C.; Demler, E.; Kuhr, S.; Bloch, I. The ‘Higgs’ amplitude mode at the two-dimensional superfluid-Mott insulator transition. Nature 2012, 487, 454. [Google Scholar] [CrossRef] [PubMed]
- Atala, M.; Aidelsburger, M.; Lohse, M.; Barreiro, J.T.; Paredes, B.; Bloch, I. Observation of chiral currents with ultracold atoms in bosonic ladders. Nat. Phys. 2014, 10, 588–593. [Google Scholar] [CrossRef]
- Bylinskii, A.; Gangloff, D.; Counts, I.; Vuletić, V. Observation of Aubry-type transition in finite atom chains via friction. Nat. Mater. 2016, 15, 717–721. [Google Scholar] [CrossRef] [PubMed]
- Köhl, M.; Moritz, H.; Stöferle, T.; Günter, K.; Esslinger, T. Fermionic Atoms in a Three Dimensional Optical Lattice: Observing Fermi Surfaces, Dynamics, and Interactions. Phys. Rev. Lett. 2005, 94, 080403. [Google Scholar] [CrossRef]
- Esslinger, T. Fermi-Hubbard Physics with Atoms in an Optical Lattice. Annu. Rev. Condens. Matter Phys. 2010, 1, 129–152. [Google Scholar] [CrossRef]
- Tarruell, L.; Sanchez-Palencia, L. Quantum simulation of the Hubbard model with ultracold fermions in optical lattices. Comptes Rendus Phys. 2018, 19, 365–393. [Google Scholar] [CrossRef]
- Tarruell, L.; Greif, D.; Uehlinger, T.; Jotzu, G.; Esslinger, T. Creating, moving and merging Dirac points with a Fermi gas in a tunable honeycomb lattice. Nature 2012, 483, 302–305. [Google Scholar] [CrossRef] [PubMed]
- Schreiber, M.; Hodgman, S.S.; Bordia, P.; Lüschen, H.P.; Fischer, M.H.; Vosk, R.; Altman, E.; Schneider, U.; Bloch, I. Observation of many-body localization of interacting fermions in a quasirandom optical lattice. Science 2015, 349, 842–845. [Google Scholar] [CrossRef] [PubMed]
- Alet, F.; Laflorencie, N. Many-body localization: An introduction and selected topics. Comptes Rendus Phys. 2018, 19, 498–525. [Google Scholar] [CrossRef]
- Vaidya, V.D.; Guo, Y.; Kroeze, R.M.; Ballantine, K.E.; Kollár, A.J.; Keeling, J.; Lev, B.L. Tunable-Range, Photon-Mediated Atomic Interactions in Multimode Cavity QED. Phys. Rev. X 2018, 8, 011002. [Google Scholar] [CrossRef]
- Daley, A.J. Quantum trajectories and open many-body quantum systems. Adv. Phys. 2014, 63, 77–149. [Google Scholar] [CrossRef]
- Barceló, C.; Liberati, S.; Visser, M. Analogue Gravity. Living Rev. Relativ. 2005, 8, 12. [Google Scholar] [CrossRef] [PubMed]
- Volovik, G. Superfluid analogies of cosmological phenomena. Phys. Rep. 2001, 351, 195. [Google Scholar] [CrossRef]
- Steinhauer, J. Observation of quantum Hawking radiation and its entanglement in an analogue black hole. Nat. Phys. 2016, 12, 959–965. [Google Scholar] [CrossRef]
- Hu, J.; Feng, L.; Zhang, Z.; Chin, C. Quantum simulation of Unruh radiation. Nat. Phys. 2019, 15, 785–789. [Google Scholar] [CrossRef]
- Mannarelli, M.; Grasso, D.; Trabucco, S.; Chiofalo, M.L. Hawking temperature and phonon emission in acoustic holes. Phys. Rev. D 2021, 103, 076001. [Google Scholar] [CrossRef]
- Fixler, J.B.; Foster, G.; McGuirk, J.; Kasevich, M. Atom interferometer measurement of the Newtonian constant of gravity. Science 2007, 315, 74–77. [Google Scholar] [CrossRef] [PubMed]
- Rosi, G.; Sorrentino, F.; Cacciapuoti, L.; Prevedelli, M.; Tino, G.M. Precision measurement of the Newtonian gravitational constant using cold atoms. Nature 2014, 510, 518–521. [Google Scholar] [CrossRef] [PubMed]
- Tino, G.M. Testing gravity with cold atom interferometry: Results and prospects. Quantum Sci. Technol. 2021, 6, 024014. [Google Scholar] [CrossRef]
- Cairncross, W.B.; Gresh, D.N.; Grau, M.; Cossel, K.C.; Roussy, T.S.; Ni, Y.; Zhou, Y.; Ye, J.; Cornell, E.A. Precision measurement of the electron’s electric dipole moment using trapped molecular ions. Phys. Rev. Lett. 2017, 119, 153001. [Google Scholar] [CrossRef] [PubMed]
- ACME Collaboration; Baron, J.; Campbell, W.C.; DeMille, D.; Doyle, J.M.; Gabrielse, G.; Gurevich, Y.V.; Hess, P.W.; Hutzler, N.R.; Kirilov, E.; et al. Order of magnitude smaller limit on the electric dipole moment of the electron. Science 2013, 343, 269–272. [Google Scholar]
- DeMille, D.; Doyle, J.M.; Sushkov, A.O. Probing the frontiers of particle physics with tabletop-scale experiments. Science 2017, 357, 990–994. [Google Scholar] [CrossRef]
- Tino, G.M.; Bassi, A.; Bianco, G.; Bongs, K.; Bouyer, P.; Cacciapuoti, L.; Capozziello, S.; Chen, X.; Chiofalo, M.L.; Derevianko, A.; et al. SAGE: A proposal for a space atomic gravity explorer. Eur. Phys. J. D 2019, 73, 228. [Google Scholar] [CrossRef]
- Ivanov, V.V.; Alberti, A.; Schioppo, M.; Ferrari, G.; Artoni, M.; Chiofalo, M.L.; Tino, G.M. Coherent delocalization of atomic wave packets in driven lattice potentials. Phys. Rev. Lett. 2008, 100, 043602. [Google Scholar] [CrossRef] [PubMed]
- El-Neaj, Y.A.; Alpigiani, C.; Amairi-Pyka, S.; Araújo, H.; Balaž, A.; Bassi, A.; Bathe-Peters, L.; Battelier, B.; Belić, A.; Bentine, E.; et al. AEDGE: Atomic Experiment for Dark Matter and Gravity Exploration in Space. EPJ Quantum Technol. 2020, 7, 6. [Google Scholar] [CrossRef]
- Bothwell, T.; Kennedy, C.J.; Aeppli, A.; Kedar, D.; Robinson, J.M.; Oelker, E.; Staron, A.; Ye, J. Resolving the gravitational redshift across a millimetre-scale atomic sample. Nature 2022, 602, 420–424. [Google Scholar] [CrossRef] [PubMed]
- Ahlers, H.; Badurina, L.; Bassi, A.; Battelier, B.; Beaufils, Q.; Bongs, K.; Bouyer, P.; Braxmaier, C.; Buchmueller, O.; Carlesso, M.; et al. STE-QUEST: Space Time Explorer and QUantum Equivalence principle Space Test. arXiv 2022, arXiv:2211.15412. [Google Scholar]
- Alonso, I.; Alpigiani, C.; Altschul, B.; Araújo, H.; Arduini, G.; Arlt, J.; Badurina, L.; Balaž, A.; Bandarupally, S.; Barish, B.C.; et al. Cold atoms in space: Community workshop summary and proposed road-map. EPJ Quantum Technol. 2022, 9, 30. [Google Scholar] [CrossRef]
- Zohar, E.; Cirac, J.I.; Reznik, B. Cold-Atom Quantum Simulator for SU(2) Yang-Mills Lattice Gauge Theory. Phys. Rev. Lett. 2013, 110, 125304. [Google Scholar] [CrossRef] [PubMed]
- Zohar, E.; Cirac, J.I.; Reznik, B. Quantum simulations of gauge theories with ultracold atoms: Local gauge invariance from angular-momentum conservation. Phys. Rev. A 2013, 88, 023617. [Google Scholar] [CrossRef]
- Bernien, H.; Schwartz, S.; Keesling, A.; Levine, H.; Omran, A.; Pichler, H.; Choi, S.; Zibrov, A.S.; Endres, M.; Greiner, M.; et al. Probing many-body dynamics on a 51-atom quantum simulator. Nature 2017, 551, 579. [Google Scholar] [CrossRef] [PubMed]
- Martinez, E.A.; Muschik, C.A.; Schindler, P.; Nigg, D.; Erhard, A.; Heyl, M.; Hauke, P.; Dalmonte, M.; Monz, T.; Zoller, P.; et al. Real-time dynamics of lattice gauge theories with a few-qubit quantum computer. Nature 2016, 534, 516–519. [Google Scholar] [CrossRef] [PubMed]
- Al-Khalili, J. Overview of the quantum biology session at the 19th IUPAB congress and 11th EBSA congress. Biophys. Rev. 2017, 9, 293–294. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Huelga, S.; Plenio, M. Vibrations, quanta and biology. Contemp. Phys. 2013, 54, 181–207. [Google Scholar] [CrossRef]
- Adams, B.; Petruccione, F. Quantum effects in the brain: A review. AVS Quantum Sci. 2020, 2, 022901. [Google Scholar] [CrossRef]
- Goorney, S.; Foti, C.; Santi, L.; Sherson, J.; Yago Malo, J.; Chiofalo, M.L. Culturo-Scientific Storytelling. Educ. Sci. 2022, 12, 474. [Google Scholar] [CrossRef]
- Chiofalo, M.; Michelini, M. Responsible Research and Innovation in Quantum Technologies. Front. Quantum Sci. Technol. 2023. Available online: https://www.frontiersin.org/research-topics/48870/responsible-research-and-innovation-in-quantum-science-and-technologies/articles (accessed on 1 February 2023).
- Müller, R.; Greinert, F. Competence Framework for Quantum Technologies: Methodology and Version History; European Commission: Brussels, Belgium, 2021. [Google Scholar]
- Raymer, M.G.; Monroe, C. The US National Quantum Initiative. Quantum Sci. Technol. 2019, 4, 020504. [Google Scholar]
- The Quantum Flagship Initiative. Available online: https://qt.eu/ (accessed on 1 February 2023).
- Hasan, S.R.; Chowdhury, M.Z.; Saiam, M.; Jang, Y.M. Quantum Communication Systems: Vision, Protocols, Applications, and Challenges. IEEE Access 2023, 11, 15855–15877. [Google Scholar] [CrossRef]
- Zhang, P.; Chen, N.; Shen, S.; Yu, S.; Wu, S.; Kumar, N. Future Quantum Communications and Networking: A Review and Vision. IEEE Wirel. Commun. 2024, 31, 141–148. [Google Scholar] [CrossRef]
- Gisin, N.; Thew, R. Quantum communication. Nat. Photonics 2007, 1, 165–171. [Google Scholar] [CrossRef]
- Orieux, A.; Diamanti, E. Recent advances on integrated quantum communications. J. Opt. 2016, 18, 083002. [Google Scholar] [CrossRef]
- Devoret, M.H.; Wallraff, A.; Martinis, J.M. Superconducting qubits: A short review. arXiv 2004, arXiv:cond-mat/0411174. [Google Scholar]
- Koch, J.; Yu, T.M.; Gambetta, J.M.; Houck, A.A.; Schuster, D.I.; Majer, J.; Blais, A.; Devoret, M.H.; Girvin, S.M.; Schoelkopf, R.J. Charge-insensitive qubit design derived from the Cooper pair box. Phys. Rev. A 2007, 76, 042319. [Google Scholar] [CrossRef]
- Kjaergaard, M.; Schwartz, M.E.; Braumüller, J.; Krantz, P.; Wang, J.I.J.; Gustavsson, S.; Oliver, W.D. Superconducting qubits: Current state of play. Annu. Rev. Condens. Matter Phys. 2020, 11, 369–395. [Google Scholar]
- Linke, N.M.; Maslov, D.; Roetteler, M.; Debnath, S.; Figgatt, C.; Landsman, K.A.; Wright, K.; Monroe, C. Experimental comparison of two quantum computing architectures. Proc. Natl. Acad. Sci. USA 2017, 114, 3305–3310. [Google Scholar] [CrossRef]
- Dutt, M.G.; Childress, L.; Jiang, L.; Togan, E.; Maze, J.; Jelezko, F.; Zibrov, A.; Hemmer, P.; Lukin, M. Quantum register based on individual electronic and nuclear spin qubits in diamond. Science 2007, 316, 1312–1316. [Google Scholar] [CrossRef] [PubMed]
- Fedyanin, D.Y. Optoelectronics of Color Centers in Diamond and Silicon Carbide: From Single-Photon Luminescence to Electrically Controlled Spin Qubits. Adv. Quantum Technol. 2021, 4, 2100048. [Google Scholar] [CrossRef]
- Nakazato, T.; Reyes, R.; Imaike, N.; Matsuda, K.; Tsurumoto, K.; Sekiguchi, Y.; Kosaka, H. Quantum error correction of spin quantum memories in diamond under a zero magnetic field. Commun. Phys. 2022, 5, 102. [Google Scholar] [CrossRef]
- Saraiva, A.; Lim, W.H.; Yang, C.H.; Escott, C.C.; Laucht, A.; Dzurak, A.S. Materials for silicon quantum dots and their impact on electron spin qubits. Adv. Funct. Mater. 2022, 32, 2105488. [Google Scholar] [CrossRef]
- Pla, J.J.; Tan, K.Y.; Dehollain, J.P.; Lim, W.H.; Morton, J.J.; Zwanenburg, F.A.; Jamieson, D.N.; Dzurak, A.S.; Morello, A. High-fidelity readout and control of a nuclear spin qubit in silicon. Nature 2013, 496, 334–338. [Google Scholar] [PubMed]
- Carusotto, I.; Ciuti, C. Quantum fluids of light. Rev. Mod. Phys. 2013, 85, 299–366. [Google Scholar] [CrossRef]
- Gerace, D.; Carusotto, I. Analog Hawking radiation from an acoustic black hole in a flowing polariton superfluid. Phys. Rev. B 2012, 86, 144505. [Google Scholar] [CrossRef]
- Kavokin, A.; Liew, T.C.; Schneider, C.; Lagoudakis, P.G.; Klembt, S.; Hoefling, S. Polariton condensates for classical and quantum computing. Nat. Rev. Phys. 2022, 4, 435–451. [Google Scholar] [CrossRef]
- Yang, H.; Kim, N.Y. Microcavity exciton-polariton quantum spin fluids. Adv. Quantum Technol. 2022, 5, 2100137. [Google Scholar] [CrossRef]
- Basov, D.N.; Asenjo-Garcia, A.; Schuck, P.J.; Zhu, X.; Rubio, A. Polariton panorama. Nanophotonics 2020, 10, 549–577. [Google Scholar] [CrossRef]
- Sammak, A.; Sabbagh, D.; Hendrickx, N.W.; Lodari, M.; Paquelet Wuetz, B.; Tosato, A.; Yeoh, L.; Bollani, M.; Virgilio, M.; Schubert, M.A.; et al. Shallow and undoped germanium quantum wells: A playground for spin and hybrid quantum technology. Adv. Funct. Mater. 2019, 29, 1807613. [Google Scholar] [CrossRef]
- Liao, P.F.; Kelley, P. Quantum Well Lasers; Elsevier: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Das, R.; Bandyopadhyay, R.; Pramanik, P. Carbon quantum dots from natural resource: A review. Mater. Today Chem. 2018, 8, 96–109. [Google Scholar] [CrossRef]
- Vajner, D.A.; Rickert, L.; Gao, T.; Kaymazlar, K.; Heindel, T. Quantum communication using semiconductor quantum dots. Adv. Quantum Technol. 2022, 5, 2100116. [Google Scholar] [CrossRef]
- Sun, H.; Wu, L.; Wei, W.; Qu, X. Recent advances in graphene quantum dots for sensing. Mater. Today 2013, 16, 433–442. [Google Scholar] [CrossRef]
- Burkard, G.; Engel, H.A.; Loss, D. Spintronics and quantum dots for quantum computing and quantum communication. Fortschritte Phys. Prog. Phys. 2000, 48, 965–986. [Google Scholar] [CrossRef]
- Barthelemy, P.; Vandersypen, L.M.K. Quantum Dot Systems: A versatile platform for quantum simulations. Ann. Phys. 2013, 525, 808–826. [Google Scholar] [CrossRef]
- García de Arquer, F.P.; Talapin, D.V.; Klimov, V.I.; Arakawa, Y.; Bayer, M.; Sargent, E.H. Semiconductor quantum dots: Technological progress and future challenges. Science 2021, 373, eaaz8541. [Google Scholar] [CrossRef]
- Carretta, S.; Zueco, D.; Chiesa, A.; Gómez-León, A.; Luis, F. A perspective on scaling up quantum computation with molecular spins. Appl. Phys. Lett. 2021, 118, 240501. [Google Scholar] [CrossRef]
- Chicco, S.; Allodi, G.; Chiesa, A.; Garlatti, E.; Buch, C.D.; Santini, P.; De Renzi, R.; Piligkos, S.; Carretta, S. Proof-of-Concept Quantum Simulator Based on Molecular Spin Qudits. J. Am. Chem. Soc. 2024, 146, 1053–1061. [Google Scholar] [CrossRef]
- Lockyer, S.J.; Chiesa, A.; Brookfield, A.; Timco, G.A.; Whitehead, G.F.S.; McInnes, E.J.L.; Carretta, S.; Winpenny, R.E.P. Five-Spin Supramolecule for Simulating Quantum Decoherence of Bell States. J. Am. Chem. Soc. 2022, 144, 16086–16092. [Google Scholar] [CrossRef]
- Wei, S.H.; Jing, B.; Zhang, X.Y.; Liao, J.Y.; Yuan, C.Z.; Fan, B.Y.; Lyu, C.; Zhou, D.L.; Wang, Y.; Deng, G.W.; et al. Towards Real-World Quantum Networks: A Review. Laser Photonics Rev. 2022, 16, 2100219. [Google Scholar] [CrossRef]
- Paraïso, T.K.; Woodward, R.I.; Marangon, D.G.; Lovic, V.; Yuan, Z.; Shields, A.J. Advanced Laser Technology for Quantum Communications (Tutorial Review). Adv. Quantum Technol. 2021, 4, 2100062. [Google Scholar] [CrossRef]
- Bennett, C.H.; Brassard, G.; Crépeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1993, 70, 1895–1899. [Google Scholar] [CrossRef]
- Anderson, M.H.; Ensher, J.R.; Matthews, M.R.; Wieman, C.E.; Cornell, E.A. Observation of Bose-Einstein Condensation in a Dilute Atomic Vapor. Science 1995, 269, 198–201. [Google Scholar] [CrossRef]
- Davis, K.B.; Mewes, M.O.; Andrews, M.R.; van Druten, N.J.; Durfee, D.S.; Kurn, D.M.; Ketterle, W. Bose-Einstein Condensation in a Gas of Sodium Atoms. Phys. Rev. Lett. 1995, 75, 3969–3973. [Google Scholar] [CrossRef]
- Zwierlein, M.W.; Stan, C.A.; Schunck, C.H.; Raupach, S.M.F.; Kerman, A.J.; Ketterle, W. Condensation of Pairs of Fermionic Atoms near a Feshbach Resonance. Phys. Rev. Lett. 2004, 92, 120403. [Google Scholar] [CrossRef]
- Dalfovo, F.; Giorgini, S.; Pitaevskii, L.P.; Stringari, S. Theory of Bose-Einstein condensation in trapped gases. Rev. Mod. Phys. 1999, 71, 463–512. [Google Scholar] [CrossRef]
- Giorgini, S.; Pitaevskii, L.P.; Stringari, S. Theory of ultracold atomic Fermi gases. Rev. Mod. Phys. 2008, 80, 1215–1274. [Google Scholar] [CrossRef]
- Inguscio, M.; Stringari, S.; Weiman, C. (Eds.) Bose Einstein Condensation in Atomic Gases; IOS Press: Amsterdam, The Netherlands, 1999. [Google Scholar]
- Metcalf, H.; Stratenvan, P. Laser Cooling and Trapping; Graduate Texts in Contemporary Physics; Springer: New York, NY, USA, 1999. [Google Scholar]
- Holland, M.J.; Jin, D.S.; Chiofalo, M.L.; Cooper, J. Emergence of Interaction Effects in Bose-Einstein Condensation. Phys. Rev. Lett. 1997, 78, 3801–3805. [Google Scholar] [CrossRef]
- Gaunt, A.L.; Schmidutz, T.F.; Gotlibovych, I.; Smith, R.P.; Hadzibabic, Z. Bose-Einstein Condensation of Atoms in a Uniform Potential. Phys. Rev. Lett. 2013, 110, 200406. [Google Scholar] [CrossRef] [PubMed]
- Hilker, T.A.; Dogra, L.H.; Eigen, C.; Glidden, J.A.P.; Smith, R.P.; Hadzibabic, Z. First and Second Sound in a Compressible 3D Bose Fluid. Phys. Rev. Lett. 2022, 128, 223601. [Google Scholar] [CrossRef] [PubMed]
- Bakr, W.; Gillen, J.; Peng, A.; Fölling, S.; Greiner, M. A quantum gas microscope for detecting single atoms in a Hubbard-regime optical lattice. Nature 2009, 462, 74. [Google Scholar] [CrossRef] [PubMed]
- Bloch, I.; Dalibard, J.; Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 2008, 80, 885–964. [Google Scholar] [CrossRef]
- Fano, U. Effects of Configuration Interaction on Intensities and Phase Shifts. Phys. Rev. 1961, 124, 1866–1878. [Google Scholar] [CrossRef]
- Feshbach, H. A unified theory of nuclear reactions. II. Ann. Phys. 1962, 19, 287–313. [Google Scholar] [CrossRef]
- Moerdijk, A.J.; Verhaar, B.J.; Axelsson, A. Resonances in Ultracold Collisions of 6Li, 7Li, and 23Na. Phys. Rev. A 1995, 51, 4852–4861. [Google Scholar] [CrossRef] [PubMed]
- Gurarie, V.; Radzihovsky, L. Resonantly Paired Fermionic Superfluids. Ann. Phys. 2007, 322, 2–119. [Google Scholar] [CrossRef]
- Chin, C.; Grimm, R.; Julienne, P.; Tiesinga, E. Feshbach Resonances in Ultracold Gases. Rev. Mod. Phys. 2010, 82, 1225–1286. [Google Scholar] [CrossRef]
- Periwal, A.; Cooper, E.S.; Kunkel, P.; Wienand, J.F.; Davis, E.J.; Schleier-Smith, M. Programmable interactions and emergent geometry in an array of atom clouds. Nature 2021, 600, 630–635. [Google Scholar] [CrossRef] [PubMed]
- Bloch, I. Ultracold quantum gases in optical lattices. Nat. Phys. 2005, 1, 23–30. [Google Scholar] [CrossRef]
- Lewenstein, M.; Sanpera, A.; Ahufinger, V. Ultracold Atoms in Optical Lattices: Simulating Quantum Many-Body Systems; OUP Oxford: Oxford, UK, 2012. [Google Scholar]
- Lye, J.E.; Fallani, L.; Modugno, M.; Wiersma, D.S.; Fort, C.; Inguscio, M. Bose-Einstein Condensate in a Random Potential. Phys. Rev. Lett. 2005, 95, 070401. [Google Scholar] [CrossRef]
- Dalibard, J.; Gerbier, F.; Juzeliūnas, G.; Öhberg, P. Colloquium: Artificial gauge potentials for neutral atoms. Rev. Mod. Phys. 2011, 83, 1523–1543. [Google Scholar] [CrossRef]
- Goldman, N.; Juzeliūnas, G.; Öhberg, P.; Spielman, I.B. Light-induced gauge fields for ultracold atoms. Rep. Prog. Phys. 2014, 77, 126401. [Google Scholar] [CrossRef] [PubMed]
- Burger, S.; Cataliotti, F.S.; Fort, C.; Minardi, F.; Inguscio, M.; Chiofalo, M.L.; Tosi, M.P. Superfluid and Dissipative Dynamics of a Bose-Einstein Condensate in a Periodic Optical Potential. Phys. Rev. Lett. 2001, 86, 4447–4450. [Google Scholar] [CrossRef] [PubMed]
- Witthaut, D.; Trimborn, F.; Wimberger, S. Dissipation Induced Coherence of a Two-Mode Bose-Einstein Condensate. Phys. Rev. Lett. 2008, 101, 200402. [Google Scholar] [CrossRef] [PubMed]
- Cataliotti, F.S.; Burger, S.; Fort, C.; Maddaloni, P.; Minardi, F.; Trombettoni, A.; Smerzi, A.; Inguscio, M. Josephson junction arrays with Bose-Einstein condensates. Science 2001, 293, 843–846. [Google Scholar] [CrossRef] [PubMed]
- Chiofalo, M.L.; Tosi, M.P. Josephson-type oscillations of a driven Bose-Einstein condensate in an optical lattice. Europhys. Lett. 2001, 56, 326. [Google Scholar] [CrossRef]
- Micheli, A.; Daley, A.J.; Jaksch, D.; Zoller, P. Single Atom Transistor in a 1D Optical Lattice. Phys. Rev. Lett. 2004, 93, 140408. [Google Scholar] [CrossRef] [PubMed]
- Levy, S.; Lahoud, E.; Shomroni, I.; Steinhauer, J. The A.C. and D.C. Josephson effects in a Bose-Einstein condensate. Nature 2007, 449, 579–583. [Google Scholar] [CrossRef] [PubMed]
- Bloch, I.; Zoller, P. Chapter 5—Ultracold Atoms and Molecules in Optical Lattices. In Ultracold Bosonic and Fermionic Gases; Levin, K., Fetter, A.L., Stamper-Kurn, D.M., Eds.; Contemporary Concepts of Condensed Matter Science; Elsevier: Amsterdam, The Netherlands, 2012; Volume 5, pp. 121–156. [Google Scholar]
- Valtolina, G.; Matsuda, K.; Tobias, W.G.; Li, J.R.; De Marco, L.; Ye, J. Dipolar evaporation of reactive molecules to below the Fermi temperature. Nature 2020, 588, 239–243. [Google Scholar] [CrossRef] [PubMed]
- Briegel, H.J.; Calarco, T.; Jaksch, D.; Cirac, J.I.; Zoller, P. Quantum computing with neutral atoms. J. Mod. Opt. 2000, 47, 415–451. [Google Scholar] [CrossRef]
- Dumke, R.; Lu, Z.; Close, J.; Robins, N.; Weis, A.; Mukherjee, M.; Birkl, G.; Hufnagel, C.; Amico, L.; Boshier, M.G.; et al. Roadmap on quantum optical systems. J. Opt. 2016, 18, 093001. [Google Scholar] [CrossRef]
- Dutta, O.; Gajda, M.; Hauke, P.; Lewenstein, M.; Lühmann, D.S.; Malomed, B.A.; Sowiński, T.; Zakrzewski, J. Non-standard Hubbard models in optical lattices: A review. Rep. Prog. Phys. 2015, 78, 066001. [Google Scholar] [CrossRef]
- Schäfer, F.; Fukuhara, T.; Sugawa, S.; Takasu, Y.; Takahashi, Y. Tools for quantum simulation with ultracold atoms in optical lattices. Nat. Rev. Phys. 2020, 2, 411–425. [Google Scholar] [CrossRef]
- Gross, C.; Bakr, W.S. Quantum gas microscopy for single atom and spin detection. Nat. Phys. 2021, 17, 1316–1323. [Google Scholar] [CrossRef]
- Hemmerich, A.; Hänsch, T.W. Two-dimesional atomic crystal bound by light. Phys. Rev. Lett. 1993, 70, 410–413. [Google Scholar] [CrossRef] [PubMed]
- Grynberg, G.; Lounis, B.; Verkerk, P.; Courtois, J.Y.; Salomon, C. Quantized motion of cold cesium atoms in two- and three-dimensional optical potentials. Phys. Rev. Lett. 1993, 70, 2249–2252. [Google Scholar] [CrossRef] [PubMed]
- Modugno, G.; Ferlaino, F.; Heidemann, R.; Roati, G.; Inguscio, M. Production of a Fermi gas of atoms in an optical lattice. Phys. Rev. A 2003, 68, 011601. [Google Scholar] [CrossRef]
- Ritsch, H.; Domokos, P.; Brennecke, F.; Esslinger, T. Cold atoms in cavity-generated dynamical optical potentials. Rev. Mod. Phys. 2013, 85, 553–601. [Google Scholar] [CrossRef]
- Zhu, C.; Chen, L.; Hu, H.; Liu, X.J.; Pu, H. Spin-exchange-induced exotic superfluids in a Bose-Fermi spinor mixture. Phys. Rev. A 2019, 100, 031602. [Google Scholar] [CrossRef]
- Wu, Z.; Bruun, G.M. Topological superfluid in a Fermi-Bose mixture with a high critical temperature. Phys. Rev. Lett. 2016, 117, 245302. [Google Scholar] [CrossRef] [PubMed]
- Ruostekoski, J. Cooperative quantum-optical planar arrays of atoms. Phys. Rev. A 2023, 108, 030101. [Google Scholar] [CrossRef]
- Grimm, R.; Weidemüller, M.; Ovchinnikov, Y.B. Optical Dipole Traps for Neutral Atoms. In Advances in Atomic, Molecular, and Optical Physics; Academic Press: Cambridge, MA, USA, 2000; Volume 42, pp. 95–170. [Google Scholar] [CrossRef]
- Cirac, J.I.; Zoller, P. Quantum Computations with Cold Trapped Ions. Phys. Rev. Lett. 1995, 74, 4091–4094. [Google Scholar] [CrossRef]
- Wineland, D.J.; Monroe, C.; Itano, W.M.; Leibfried, D.; King, B.E.; Meekhof, D.M. A Experimental Issues in Coherent Quantum-State Manipulation of Trapped Atomic Ions. J. Res. Natl. Inst. Stand. Technol. 1998, 103, 259. [Google Scholar] [CrossRef] [PubMed]
- Bruzewicz, C.D.; Chiaverini, J.; McConnell, R.; Sage, J.M. Trapped-ion quantum computing: Progress and challenges. Appl. Phys. Rev. 2019, 6, 021314. [Google Scholar] [CrossRef]
- Brown, K.R.; Chiaverini, J.; Sage, J.M.; Häffner, H. Materials challenges for trapped-ion quantum computers. Nat. Rev. Mater. 2021, 6, 892–905. [Google Scholar] [CrossRef]
- Benhelm, J.; Kirchmair, G.; Roos, C.F.; Blatt, R. Towards fault-tolerant quantum computing with trapped ions. Nat. Phys. 2008, 4, 463–466. [Google Scholar] [CrossRef]
- Myerson, A.; Szwer, D.; Webster, S.; Allcock, D.; Curtis, M.; Imreh, G.; Sherman, J.; Stacey, D.; Steane, A.; Lucas, D. High-fidelity readout of trapped-ion qubits. Phys. Rev. Lett. 2008, 100, 200502. [Google Scholar] [CrossRef] [PubMed]
- Häffner, H.; Roos, C.F.; Blatt, R. Quantum computing with trapped ions. Phys. Rep. 2008, 469, 155–203. [Google Scholar] [CrossRef]
- Monroe, C.; Kim, J. Scaling the ion trap quantum processor. Science 2013, 339, 1164–1169. [Google Scholar] [CrossRef] [PubMed]
- Webb, A.E.; Webster, S.C.; Collingbourne, S.; Bretaud, D.; Lawrence, A.M.; Weidt, S.; Mintert, F.; Hensinger, W.K. Resilient entangling gates for trapped ions. Phys. Rev. Lett. 2018, 121, 180501. [Google Scholar] [CrossRef] [PubMed]
- Ospelkaus, C.; Warring, U.; Colombe, Y.; Brown, K.; Amini, J.; Leibfried, D.; Wineland, D.J. Microwave quantum logic gates for trapped ions. Nature 2011, 476, 181–184. [Google Scholar] [CrossRef] [PubMed]
- Jonathan, D.; Plenio, M.; Knight, P. Fast quantum gates for cold trapped ions. Phys. Rev. A 2000, 62, 042307. [Google Scholar] [CrossRef]
- Katz, O.; Cetina, M.; Monroe, C. N-Body Interactions between Trapped Ion Qubits via Spin-Dependent Squeezing. Phys. Rev. Lett. 2022, 129, 063603. [Google Scholar] [CrossRef]
- Wang, Y.; Um, M.; Zhang, J.; An, S.; Lyu, M.; Zhang, J.N.; Duan, L.M.; Yum, D.; Kim, K. Single-qubit quantum memory exceeding ten-minute coherence time. Nat. Photonics 2017, 11, 646–650. [Google Scholar] [CrossRef]
- Zhang, C.; Pokorny, F.; Li, W.; Higgins, G.; Pöschl, A.; Lesanovsky, I.; Hennrich, M. Submicrosecond entangling gate between trapped ions via Rydberg interaction. Nature 2020, 580, 345–349. [Google Scholar] [CrossRef] [PubMed]
- Monz, T.; Nigg, D.; Martinez, E.A.; Brandl, M.F.; Schindler, P.; Rines, R.; Wang, S.X.; Chuang, I.L.; Blatt, R. Realization of a scalable Shor algorithm. Science 2016, 351, 1068–1070. [Google Scholar] [CrossRef]
- Graß, T.; Lewenstein, M. Trapped-ion quantum simulation of tunable-range Heisenberg chains. EPJ Quantum Technol. 2014, 1, 8. [Google Scholar] [CrossRef]
- Shimshoni, E.; Morigi, G.; Fishman, S. Quantum Zigzag Transition in Ion Chains. Phys. Rev. Lett. 2011, 106, 010401. [Google Scholar] [CrossRef] [PubMed]
- Porras, D.; Cirac, J.I. Effective Quantum Spin Systems with Trapped Ions. Phys. Rev. Lett. 2004, 92, 207901. [Google Scholar] [CrossRef] [PubMed]
- Blatt, R.; Roos, C.F. Quantum simulations with trapped ions. Nat. Phys. 2012, 8, 277–284. [Google Scholar] [CrossRef]
- Monroe, C.; Campbell, W.C.; Duan, L.M.; Gong, Z.X.; Gorshkov, A.V.; Hess, P.W.; Islam, R.; Kim, K.; Linke, N.M.; Pagano, G.; et al. Programmable quantum simulations of spin systems with trapped ions. Rev. Mod. Phys. 2021, 93, 025001. [Google Scholar] [CrossRef]
- Hempel, C.; Maier, C.; Romero, J.; McClean, J.; Monz, T.; Shen, H.; Jurcevic, P.; Lanyon, B.P.; Love, P.; Babbush, R.; et al. Quantum Chemistry Calculations on a Trapped-Ion Quantum Simulator. Phys. Rev. X 2018, 8, 031022. [Google Scholar] [CrossRef]
- Reiter, F.; Sørensen, A.S.; Zoller, P.; Muschik, C. Dissipative quantum error correction and application to quantum sensing with trapped ions. Nat. Commun. 2017, 8, 1822. [Google Scholar] [CrossRef] [PubMed]
- Elben, A.; Flammia, S.T.; Huang, H.Y.; Kueng, R.; Preskill, J.; Vermersch, B.; Zoller, P. The randomized measurement toolbox. Nat. Rev. Phys. 2023, 5, 9–24. [Google Scholar] [CrossRef]
- Adams, C.S.; Pritchard, J.D.; Shaffer, J.P. Rydberg atom quantum technologies. J. Phys. B At. Mol. Opt. Phys. 2019, 53, 012002. [Google Scholar] [CrossRef]
- Gallagher, T.F. Rydberg Atoms; Cambridge Monographs on Atomic, Molecular and Chemical Physics; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar] [CrossRef]
- Sibalic, N.; Adams, C.S. Rydberg Physics; IOP Publishing: Bristol, UK, 2018; pp. 2399–2891. [Google Scholar] [CrossRef]
- Ashkin, A. Acceleration and Trapping of Particles by Radiation Pressure. Phys. Rev. Lett. 1970, 24, 156–159. [Google Scholar] [CrossRef]
- Browaeys, A.; Lahaye, T. Many-body physics with individually controlled Rydberg atoms. Nat. Phys. 2020, 16, 132–142. [Google Scholar] [CrossRef]
- Urban, E.; Johnson, T.A.; Henage, T.; Isenhower, L.; Yavuz, D.D.; Walker, T.G.; Saffman, M. Observation of Rydberg blockade between two atoms. Nat. Phys. 2009, 5, 110–114. [Google Scholar] [CrossRef]
- Ryabtsev, I.I.; Tretyakov, D.B.; Beterov, I.I. Applicability of Rydberg atoms to quantum computers. J. Phys. At. Mol. Opt. Phys. 2005, 38, S421. [Google Scholar] [CrossRef]
- Weimer, H.; Müller, M.; Lesanovsky, I.; Zoller, P.; Büchler, H.P. A Rydberg quantum simulator. Nat. Phys. 2010, 6, 382–388. [Google Scholar] [CrossRef]
- Degen, C.L.; Reinhard, F.; Cappellaro, P. Quantum sensing. Rev. Mod. Phys. 2017, 89, 035002. [Google Scholar] [CrossRef]
- Saffman, M.; Walker, T.G.; Mølmer, K. Quantum information with Rydberg atoms. Rev. Mod. Phys. 2010, 82, 2313–2363. [Google Scholar] [CrossRef]
- Hermann-Avigliano, C.; Teixeira, R.C.; Nguyen, T.; Cantat-Moltrecht, T.; Nogues, G.; Dotsenko, I.; Gleyzes, S.; Raimond, J.; Haroche, S.; Brune, M. Long coherence times for Rydberg qubits on a superconducting atom chip. Phys. Rev. A 2014, 90, 040502. [Google Scholar] [CrossRef]
- Saffman, M. Quantum computing with atomic qubits and Rydberg interactions: Progress and challenges. J. Phys. At. Mol. Opt. Phys. 2016, 49, 202001. [Google Scholar] [CrossRef]
- Ebadi, S.; Wang, T.T.; Levine, H.; Keesling, A.; Semeghini, G.; Omran, A.; Bluvstein, D.; Samajdar, R.; Pichler, H.; Ho, W.W.; et al. Quantum phases of matter on a 256-atom programmable quantum simulator. Nature 2021, 595, 227–232. [Google Scholar] [CrossRef] [PubMed]
- Barredo, D.; Lienhard, V.; Scholl, P.; de Léséleuc, S.; Boulier, T.; Browaeys, A.; Lahaye, T. Three-dimensional trapping of individual Rydberg atoms in ponderomotive bottle beam traps. Phys. Rev. Lett. 2020, 124, 023201. [Google Scholar] [CrossRef] [PubMed]
- Wilson, J.; Saskin, S.; Meng, Y.; Ma, S.; Dilip, R.; Burgers, A.; Thompson, J. Trapping alkaline earth Rydberg atoms optical tweezer arrays. Phys. Rev. Lett. 2022, 128, 033201. [Google Scholar] [CrossRef] [PubMed]
- Evered, S.J.; Bluvstein, D.; Kalinowski, M.; Ebadi, S.; Manovitz, T.; Zhou, H.; Li, S.H.; Geim, A.A.; Wang, T.T.; Maskara, N.; et al. High-fidelity parallel entangling gates on a neutral-atom quantum computer. Nature 2023, 622, 268–272. [Google Scholar] [CrossRef]
- Bluvstein, D.; Evered, S.J.; Geim, A.A.; Li, S.H.; Zhou, H.; Manovitz, T.; Ebadi, S.; Cain, M.; Kalinowski, M.; Hangleiter, D.; et al. Logical quantum processor based on reconfigurable atom arrays. Nature 2024, 626, 58–65. [Google Scholar] [CrossRef] [PubMed]
- Santos, L.; Shlyapnikov, G.V.; Zoller, P.; Lewenstein, M. Bose-Einstein Condensation in Trapped Dipolar Gases. Phys. Rev. Lett. 2000, 85, 1791–1794. [Google Scholar] [CrossRef] [PubMed]
- Schulz, H.J. Wigner crystal in one dimension. Phys. Rev. Lett. 1993, 71, 1864–1867. [Google Scholar] [CrossRef] [PubMed]
- Capponi, S.; Poilblanc, D.; Giamarchi, T. Effects of long-range electronic interactions on a one-dimensional electron system. Phys. Rev. B 2000, 61, 13410–13417. [Google Scholar] [CrossRef]
- Richter, R.; Barashenkov, I.V. Two-Dimensional Solitons on the Surface of Magnetic Fluids. Phys. Rev. Lett. 2005, 94, 184503. [Google Scholar] [CrossRef] [PubMed]
- Ronen, S.; Bortolotti, D.C.E.; Bohn, J.L. Radial and Angular Rotons in Trapped Dipolar Gases. Phys. Rev. Lett. 2007, 98, 030406. [Google Scholar] [CrossRef]
- Góral, K.; Santos, L.; Lewenstein, M. Quantum Phases of Dipolar Bosons in Optical Lattices. Phys. Rev. Lett. 2002, 88, 170406. [Google Scholar] [CrossRef]
- Dalla Torre, E.G.; Berg, E.; Altman, E. Hidden Order in 1D Bose Insulators. Phys. Rev. Lett. 2006, 97, 260401. [Google Scholar] [CrossRef] [PubMed]
- Kao, W.; Lin, K.Y.; Gopalakrishnan, S.; Lev, B. Topological pumping of a 1D dipolar gas into strongly correlated prethermal states. Science 2021, 371, 296. [Google Scholar] [CrossRef] [PubMed]
- Wei, X.; Gao, C.; Asgari, R.; Wang, P.; Xianlong, G. Fulde-Ferrell-Larkin-Ovchinnikov pairing states of a polarized dipolar Fermi gas trapped in a one-dimensional optical lattice. Phys. Rev. A 2018, 98, 023631. [Google Scholar] [CrossRef]
- Chomaz, L.; Ferrier-Barbut, I.; Ferlaino, F.; Laburthe-Tolra, B.; Lev, B.L.; Pfau, T. Dipolar physics: A review of experiments with magnetic quantum gases. Rep. Prog. Phys. 2022, 86, 026401. [Google Scholar] [CrossRef]
- Carr, L.D.; DeMille, D.; Krems, R.V.; Ye, J. Cold and ultracold molecules: Science, technology and applications. New J. Phys. 2009, 11, 055049. [Google Scholar] [CrossRef]
- Bohn, J.L.; Rey, A.M.; Ye, J. Cold molecules: Progress in quantum engineering of chemistry and quantum matter. Science 2017, 357, 1002–1010. [Google Scholar] [CrossRef]
- Moses, S.A.; Covey, J.P.; Miecnikowski, M.T.; Jin, D.S.; Ye, J. New frontiers for quantum gases of polar molecules. Nat. Phys. 2017, 13, 13. [Google Scholar] [CrossRef]
- Löw, R.; Weimer, H.; Nipper, J.; Balewski, J.B.; Butscher, B.; Büchler, H.P.; Pfau, T. An experimental and theoretical guide to strongly interacting Rydberg gases. J. Phys. At. Mol. Opt. Phys. 2012, 45, 113001. [Google Scholar] [CrossRef]
- Labuhn, H.; Barredo, D.; Ravets, S.; Léséleuc, S.D.; Macrì, T.; Lahaye, T.; Browaeys, A. Tunable two-dimensional arrays of single Rydberg atoms for realizing quantum Ising models. Nature 2016, 534, 667. [Google Scholar] [CrossRef] [PubMed]
- Lahaye, T.; Menotti, C.; Santos, L.; Lewenstein, M.; Pfau, T. The physics of dipolar bosonic quantum gases. Rep. Prog. Phys. 2009, 72, 126401. [Google Scholar] [CrossRef]
- Menotti, C.; Stringari, S. Collective oscillations of a one-dimensional trapped Bose-Einstein gas. Phys. Rev. A 2002, 66, 043610. [Google Scholar] [CrossRef]
- Petrov, D.; Gangardt, D.; Shlyapnikov, G. Low-dimensional trapped gases. J. Phys. IV 2004, 116, 3. [Google Scholar] [CrossRef]
- Lieb, E.H.; Liniger, W. Exact analysis of an interacting Bose gas. I. The general solution and the ground state. Phys. Rev. 1963, 130, 1605. [Google Scholar] [CrossRef]
- Girardeau, M. Relationship between Systems of Impenetrable Bosons and Fermions in One Dimension. J. Math. Phys. 1960, 1, 516. [Google Scholar] [CrossRef]
- Citro, R.; Orignac, E.; De Palo, S.; Chiofalo, M.L. Evidence of Luttinger-liquid behavior in one-dimensional dipolar quantum gases. Phys. Rev. A 2007, 75, 051602. [Google Scholar] [CrossRef]
- Citro, R.; Palo, S.D.; Orignac, E.; Pedri, P.; Chiofalo, M.L. Luttinger hydrodynamics of confined one-dimensional Bose gases with dipolar interactions. New J. Phys. 2008, 10, 045011. [Google Scholar] [CrossRef]
- Orignac, E.; Citro, R.; De Palo, S.; Chiofalo, M.L. Light scattering in inhomogeneous Tomonaga-Luttinger liquids. Phys. Rev. A 2012, 85, 013634. [Google Scholar] [CrossRef]
- De Palo, S.; Orignac, E.; Citro, R.; Chiofalo, M.L. Low-energy excitation spectrum of one-dimensional dipolar quantum gases. Phys. Rev. B 2008, 77, 212101. [Google Scholar] [CrossRef]
- Schmitz, R.; Krönke, S.; Cao, L.; Schmelcher, P. Quantum breathing dynamics of ultracold bosons in one-dimensional harmonic traps: Unraveling the pathway from few- to many-body systems. Phys. Rev. A 2013, 88, 043601. [Google Scholar] [CrossRef]
- Parisi, L.; Giorgini, S. Quantum Monte Carlo study of the Bose-polaron problem in a one-dimensional gas with contact interactions. Phys. Rev. A 2017, 95, 023619. [Google Scholar] [CrossRef]
- Gudyma, A.I.; Astrakharchik, G.E.; Zvonarev, M.B. Reentrant behavior of the breathing-mode-oscillation frequency in a one-dimensional Bose gas. Phys. Rev. A 2015, 92, 021601. [Google Scholar] [CrossRef]
- De Palo, S.; Orignac, E.; Chiofalo, M.L.; Citro, R. Polarization angle dependence of the breathing mode in confined one-dimensional dipolar bosons. Phys. Rev. B 2021, 103, 115109. [Google Scholar] [CrossRef]
- Koch, T.; Lahaye, T.; Metz, J.; Fröhlich, B.; Griesmaier, A.; Pfau, T. Stabilization of a purely dipolar quantum gas against collapse. Nat. Phys. 2008, 4, 218–222. [Google Scholar] [CrossRef]
- Santos, L.; Shlyapnikov, G.V.; Lewenstein, M. Roton-Maxon Spectrum and Stability of Trapped Dipolar Bose-Einstein Condensates. Phys. Rev. Lett. 2003, 90, 250403. [Google Scholar] [CrossRef]
- Tanzi, L.; Lucioni, E.; Famà, F.; Catani, J.; Fioretti, A.; Gabbanini, C.; Bisset, R.N.; Santos, L.; Modugno, G. Observation of a Dipolar Quantum Gas with Metastable Supersolid Properties. Phys. Rev. Lett. 2019, 122, 130405. [Google Scholar] [CrossRef] [PubMed]
- Norcia, M.A.; Politi, C.; Klaus, L.; Poli, E.; Sohmen, M.; Mark, M.J.; Bisset, R.N.; Santos, L.; Ferlaino, F. Two-dimensional supersolidity in a dipolar quantum gas. Nature 2021, 596, 357–361. [Google Scholar] [CrossRef] [PubMed]
- Tang, Y.; Kao, W.; Li, K.Y.; Seo, S.; Mallayya, K.; Rigol, M.; Gopalakrishnan, S.; Lev, B.L. Thermalization near Integrability in a Dipolar Quantum Newton’s Cradle. Phys. Rev. X 2018, 8, 021030. [Google Scholar] [CrossRef]
- Baier, S.; Petter, D.; Becher, J.H.; Patscheider, A.; Natale, G.; Chomaz, L.; Mark, M.J.; Ferlaino, F. Realization of a Strongly Interacting Fermi Gas of Dipolar Atoms. Phys. Rev. Lett. 2018, 121, 093602. [Google Scholar] [CrossRef] [PubMed]
- Ferrier-Barbut, I.; Kadau, H.; Schmitt, M.; Wenzel, M.; Pfau, T. Observation of Quantum Droplets in a Strongly Dipolar Bose Gas. Phys. Rev. Lett. 2016, 116, 215301. [Google Scholar] [CrossRef] [PubMed]
- Santos, L. Theory of dipolar gases (I). In Many-Body Physics with Ultracold Gases: Lecture Notes of the Les Houches Summer School: Volume 94, July 2010; Oxford University Press: Oxford, UK, 2012; Volume 94, p. 231. [Google Scholar]
- Tanji-Suzuki, H.; Leroux, I.D.; Schleier-Smith, M.H.; Cetina, M.; Grier, A.T.; Simon, J.; Vuletić, V. Interaction between Atomic Ensembles and Optical Resonators. Adv. At. Mol. Opt. Phys. 2011, 60, 201–237. [Google Scholar] [CrossRef]
- Mivehvar, F.; Piazza, F.; Donner, T.; Ritsch, H. Cavity QED with quantum gases: New paradigms in many-body physics. Adv. Phys. 2021, 70, 1–153. [Google Scholar] [CrossRef]
- Kaluzny, Y.; Goy, P.; Gross, M.; Raimond, J.M.; Haroche, S. Observation of self-induced Rabi oscillations in two-level atoms excited inside a resonant cavity: The ringing regime of superradiance. Phys. Rev. Lett. 1983, 51, 1175. [Google Scholar] [CrossRef]
- Vuletić, V.; Chu, S. Laser Cooling of Atoms, Ions, or Molecules by Coherent Scattering. Phys. Rev. Lett. 2000, 84, 6090. [Google Scholar] [CrossRef]
- Wolke, M.; Klinner, J.; Keßler, H.; Hemmerich, A. Cavity cooling below the recoil limit. Science 2012, 337, 3787. [Google Scholar] [CrossRef] [PubMed]
- Ma, J.; Wang, X.; Sun, C.P.; Nori, F. Quantum spin squeezing. Phys. Rep. 2011, 509, 89–165. [Google Scholar] [CrossRef]
- Peden, B.M.; Meiser, D.; Chiofalo, M.L.; Holland, M.J. Nondestructive cavity QED probe of Bloch oscillations in a gas of ultracold atoms. Phys. Rev. A 2009, 80, 063614. [Google Scholar] [CrossRef]
- Meiser, D.; Ye, J.; Holland, M.J. Spin squeezing in optical lattice clocks via lattice-based QND measurements. New J. Phys. 2008, 10, 045014. [Google Scholar] [CrossRef]
- Maschler, C.; Ritsch, H. Cold atom dynamics in a quantum optical lattice potential. Phys. Rev. Lett. 2005, 95, 260401. [Google Scholar] [CrossRef]
- Jäger, S.B.; Cooper, J.; Holland, M.J.; Morigi, G. Dynamical phase transitions to optomechanical superradiance. Phys. Rev. Lett. 2019, 123, 053601. [Google Scholar] [CrossRef] [PubMed]
- Black, A.T.; Chan, H.W.; Vuletić, V. Observation of Collective Friction Forces due to Spatial Self-Organization of Atoms: From Rayleigh to Bragg Scattering. Phys. Rev. Lett. 2003, 91, 203001. [Google Scholar] [CrossRef] [PubMed]
- Baumann, K.; Guerlin, C.; Brennecke, F.; Esslinger, T. Dicke quantum phase transition with a superfluid gas in an optical cavity. Nature 2010, 464, 1301–1306. [Google Scholar] [CrossRef] [PubMed]
- Nagy, D.; Szirmai, G.; Domokos, P. Self-organization of a Bose-Einstein condensate in an optical cavity. Eur. Phys. J. D 2007, 48, 127–136. [Google Scholar] [CrossRef]
- Colella, E.; Citro, R.; Barsanti, M.; Rossini, D.; Chiofalo, M.L. Quantum phases of spinful Fermi gases in optical cavities. Phys. Rev. B 2018, 97, 134502. [Google Scholar] [CrossRef]
- Kollár, A.J.; Papageorge, A.T.; Baumann, K.; Armen, M.A.; Lev, B.L. An adjustable-length cavity and Bose–Einstein condensate apparatus for multimode cavity QED. New J. Phys. 2015, 17, 043012. [Google Scholar] [CrossRef]
- Niedenzu, W.; Schulze, R.; Vukics, A.; Ritsch, H. Microscopic dynamics of ultracold particles in a ring-cavity optical lattice. Phys. Rev. A 2010, 82, 043605. [Google Scholar] [CrossRef]
- Gopalakrishnan, S.; Lev, B.L.; Goldbart, P.M. Emergent crystallinity and frustration with Bose–Einstein condensates in multimode cavities. Nat. Phys. 2009, 5, 845–850. [Google Scholar] [CrossRef]
- Ritsch, H. Crystals of atoms and light. Nat. Phys. 2009, 5, 781–782. [Google Scholar] [CrossRef]
- Colella, E.; Ostermann, S.; Niedenzu, W.; Mivehvar, F.; Ritsch, H. Antiferromagnetic self-ordering of a Fermi gas in a ring cavity. New J. Phys. 2019, 21, 043019. [Google Scholar] [CrossRef]
- Gopalakrishnan, S.; Lev, B.L.; Goldbart, P.M. Frustration and Glassiness in Spin Models with Cavity-Mediated Interactions. Phys. Rev. Lett. 2011, 107, 277201. [Google Scholar] [CrossRef] [PubMed]
- Strack, P.; Sachdev, S. Dicke Quantum Spin Glass of Atoms and Photons. Phys. Rev. Lett. 2011, 107, 277202. [Google Scholar] [CrossRef]
- Lucchesi, L.; Chiofalo, M.L. Many-Body Entanglement in Short-Range Interacting Fermi Gases for Metrology. Phys. Rev. Lett. 2019, 123, 060406. [Google Scholar] [CrossRef] [PubMed]
- Müller, M.; Strack, P.; Sachdev, S. Quantum charge glasses of itinerant fermions with cavity-mediated long-range interactions. Phys. Rev. A 2012, 86, 023604. [Google Scholar] [CrossRef]
- Bentsen, G.S.; Hashizume, T.; Davis, E.J.; Buyskikh, A.S.; Schleier-Smith, M.H.; Daley, A.J. Tunable geometries from a sparse quantum spin network. In Optical, Opto-Atomic, and Entanglement-Enhanced Precision Metrology II; Shahriar, S.M., Scheuer, J., Eds.; International Society for Optics and Photonics, SPIE: Bellingham, WA, USA, 2020; Volume 11296, p. 112963W. [Google Scholar] [CrossRef]
- Kruse, D.; Ruder, M.; Benhelm, J.; von Cube, C.; Zimmermann, C.; Courteille, P.; Elsässer, T.; Nagorny, B.; Hemmerich, A. Cold atoms in a high-Q ring cavity. Phys. Rev. A 2003, 67, 051802. [Google Scholar] [CrossRef]
- Nagorny, B.; Elsässer, T.; Richter, H.; Hemmerich, A.; Kruse, D.; Zimmermann, C.; Courteille, P. Optical lattice in a high-finesse ring resonator. Phys. Rev. A 2003, 67, 031401. [Google Scholar] [CrossRef]
- Elsässer, T.; Nagorny, B.; Hemmerich, A. Collective sideband cooling in an optical ring cavity. Phys. Rev. A 2003, 67, 051401. [Google Scholar] [CrossRef]
- Slama, S.; Bux, S.; Krenz, G.; Zimmermann, C.; Courteille, P. Superradiant Rayleigh scattering and collective atomic recoil lasing in a ring cavity. Phys. Rev. Lett. 2007, 98, 053603. [Google Scholar] [CrossRef] [PubMed]
- Mivehvar, F.; Ostermann, S.; Piazza, F.; Ritsch, H. Driven-Dissipative Supersolid in a Ring Cavity. Phys. Rev. Lett. 2018, 120, 123601. [Google Scholar] [CrossRef] [PubMed]
- Schuster, S.; Wolf, P.; Ostermann, S.; Slama, S.; Zimmermann, C. Supersolid Properties of a Bose-Einstein Condensate in a Ring Resonator. Phys. Rev. Lett. 2020, 124, 143602. [Google Scholar] [CrossRef] [PubMed]
- Bentsen, G.; Hashizume, T.; Buyskikh, A.S.; Davis, E.J.; Daley, A.J.; Gubser, S.S.; Schleier-Smith, M. Treelike Interactions and Fast Scrambling with Cold Atoms. Phys. Rev. Lett. 2019, 123, 130601. [Google Scholar] [CrossRef] [PubMed]
- Sheremet, A.S.; Petrov, M.I.; Iorsh, I.V.; Poshakinskiy, A.V.; Poddubny, A.N. Waveguide quantum electrodynamics: Collective radiance and photon-photon correlations. Rev. Mod. Phys. 2023, 95, 015002. [Google Scholar] [CrossRef]
- Goban, A.; Hung, C.L.; Hood, J.D.; Yu, S.P.; Muniz, J.A.; Painter, O.; Kimble, H.J. Superradiance for Atoms Trapped along a Photonic Crystal Waveguide. Phys. Rev. Lett. 2015, 115, 063601. [Google Scholar] [CrossRef]
- Chang, D.E.; Douglas, J.S.; González-Tudela, A.; Hung, C.L.; Kimble, H.J. Colloquium: Quantum matter built from nanoscopic lattices of atoms and photons. Rev. Mod. Phys. 2018, 90, 031002. [Google Scholar] [CrossRef]
- Da Ros, E.; Cooper, N.; Nute, J.; Hackermueller, L. Cold atoms in micromachined waveguides: A new platform for atom-photon interactions. Phys. Rev. Res. 2020, 2, 033098. [Google Scholar] [CrossRef]
- Lodahl, P.; Mahmoodian, S.; Stobbe, S.; Rauschenbeutel, A.; Schneeweiss, P.; Volz, J.; Pichler, H.; Zoller, P. Chiral quantum optics. Nature 2017, 541, 473–480. [Google Scholar] [CrossRef] [PubMed]
- González-Tudela, A.; Hung, C.L.; Chang, D.E.; Cirac, J.I.; Kimble, H.J. Subwavelength vacuum lattices and atom–atom interactions in two-dimensional photonic crystals. Nat. Photonics 2015, 9, 320–325. [Google Scholar] [CrossRef]
- Gouraud, B.; Maxein, D.; Nicolas, A.; Morin, O.; Laurat, J. Demonstration of a Memory for Tightly Guided Light in an Optical Nanofiber. Phys. Rev. Lett. 2015, 114, 180503. [Google Scholar] [CrossRef] [PubMed]
- González-Tudela, A.; Cirac, J.I. Quantum Emitters in Two-Dimensional Structured Reservoirs in the Nonperturbative Regime. Phys. Rev. Lett. 2017, 119, 143602. [Google Scholar] [CrossRef] [PubMed]
- Yver-Leduc, F.; Cheinet, P.; Fils, J.; Clairon, A.; Dimarcq, N.; Holleville, D.; Bouyer, P.; Landragin, A. Reaching the quantum noise limit in a high-sensitivity cold-atom inertial sensor. J. Opt. Quantum Semiclassical Opt. 2003, 5, S136. [Google Scholar] [CrossRef]
- Budker, D.; Romalis, M. Optical magnetometry. Nat. Phys. 2007, 3, 227–234. [Google Scholar] [CrossRef]
- Wildermuth, S.; Hofferberth, S.; Lesanovsky, I.; Groth, S.; Krüger, P.; Schmiedmayer, J.; Bar-Joseph, I. Sensing electric and magnetic fields with Bose-Einstein condensates. Appl. Phys. Lett. 2006, 88, 264103. [Google Scholar] [CrossRef]
- Napolitano, M.; Koschorreck, M.; Dubost, B.; Behbood, N.; Sewell, R.J.; Mitchell, M.W. Interaction-based quantum metrology showing scaling beyond the Heisenberg limit. Nature 2011, 471, 486–489. [Google Scholar] [CrossRef] [PubMed]
- Kozhekin, A.E.; Mølmer, K.; Polzik, E. Quantum memory for light. Phys. Rev. A 2000, 62, 033809. [Google Scholar] [CrossRef]
- Duan, L.M.; Lukin, M.D.; Cirac, J.I.; Zoller, P. Long-distance quantum communication with atomic ensembles and linear optics. Nature 2001, 414, 413–418. [Google Scholar] [CrossRef] [PubMed]
- Bize, S.; Diddams, S.A.; Tanaka, U.; Tanner, C.E.; Oskay, W.H.; Drullinger, R.E.; Parker, T.E.; Heavner, T.P.; Jefferts, S.R.; Hollberg, L.; et al. Testing the Stability of Fundamental Constants with the 199Hg+ Single-Ion Optical Clock. Phys. Rev. Lett. 2003, 90, 150802. [Google Scholar] [CrossRef] [PubMed]
- Dimopoulos, S.; Graham, P.; Hogan, J.; Kasevich, M. Testing general relativity with atom interferometry. Phys. Rev. Lett. 2007, 98, 111102. [Google Scholar] [CrossRef] [PubMed]
- McGilligan, J.P.; Griffin, P.F.; Elvin, R.; Ingleby, S.J.; Riis, E.; Arnold, A.S. Grating chips for quantum technologies. Sci. Rep. 2017, 7, 384. [Google Scholar] [CrossRef] [PubMed]
- Singh, V.; Tiwari, V.; Chaudhary, A.; Shukla, R.; Mukherjee, C.; Mishra, S. Development and characterization of atom chip for magnetic trapping of atoms. J. Appl. Phys. 2023, 133. [Google Scholar] [CrossRef]
- Cooper, N.; Coles, L.; Everton, S.; Maskery, I.; Campion, R.; Madkhaly, S.; Morley, C.; O’Shea, J.; Evans, W.; Saint, R.; et al. Additively manufactured ultra-high vacuum chamber for portable quantum technologies. Addit. Manuf. 2021, 40, 101898. [Google Scholar] [CrossRef]
- Fortágh, J.; Zimmermann, C. Magnetic microtraps for ultracold atoms. Rev. Mod. Phys. 2007, 79, 235. [Google Scholar] [CrossRef]
- Rushton, J.; Aldous, M.; Himsworth, M. Contributed review: The feasibility of a fully miniaturized magneto-optical trap for portable ultracold quantum technology. Rev. Sci. Instrum. 2014, 85, 121501. [Google Scholar] [CrossRef] [PubMed]
- Ketterle, W.; Druten, N.V. Evaporative Cooling of Trapped Atoms. In Evaporative Cooling of Trapped Atoms; Advances in Atomic, Molecular, and Optical Physics; Bederson, B., Walther, H., Eds.; Academic Press: Cambridge, MA, USA, 1996; Volume 37, pp. 181–236. [Google Scholar]
- Rabl, P.; Daley, A.J.; Fedichev, P.O.; Cirac, J.I.; Zoller, P. Defect-Suppressed Atomic Crystals in an Optical Lattice. Phys. Rev. Lett. 2003, 91, 110403. [Google Scholar] [CrossRef] [PubMed]
- Kantian, A.; Daley, A.J.; Zoller, P. η Condensate of Fermionic Atom Pairs via Adiabatic State Preparation. Phys. Rev. Lett. 2010, 104, 240406. [Google Scholar] [CrossRef] [PubMed]
- Müller, M.; Diehl, S.; Pupillo, G.; Zoller, P. Engineered Open Systems and Quantum Simulations with Atoms and Ions. In Advances in Atomic, Molecular, and Optical Physics; Berman, P., Arimondo, E., Lin, C., Eds.; Academic Press: Cambridge, MA, USA, 2012; Volume 61, pp. 1–80. [Google Scholar]
- Brossel, J.; Kastler, A.; Winter, J. Gréation optique d’une inégalité de population entre les sous-niveaux Zeeman de l’état fondamental des atomes. J. Phys. Radium 1952, 13, 668. [Google Scholar] [CrossRef]
- Cohen-Tannoudji, C.; Kastler, A. I Optical Pumping; Wolf, E., Ed.; Progress in Optics; Elsevier: Amsterdam, The Netherlands, 1966; Volume 5, pp. 1–81. [Google Scholar]
- Haller, E.; Hudson, J.; Kelly, A.; Cotta, D.A.; Peaudecerf, B.; Bruce, G.D.; Kuhr, S. Single-atom imaging of fermions in a quantum-gas microscope. Nat. Phys. 2015, 11, 738–742. [Google Scholar] [CrossRef]
- Cheuk, L.W.; Nichols, M.A.; Okan, M.; Gersdorf, T.; Ramasesh, V.V.; Bakr, W.S.; Lompe, T.; Zwierlein, M.W. Quantum-Gas Microscope for Fermionic Atoms. Phys. Rev. Lett. 2015, 114, 193001. [Google Scholar] [CrossRef] [PubMed]
- Parsons, M.F.; Huber, F.; Mazurenko, A.; Chiu, C.S.; Setiawan, W.; Wooley-Brown, K.; Blatt, S.; Greiner, M. Site-Resolved Imaging of Fermionic 6Li in an Optical Lattice. Phys. Rev. Lett. 2015, 114, 213002. [Google Scholar] [CrossRef] [PubMed]
- McKay, D.C.; DeMarco, B. Cooling in strongly correlated optical lattices: Prospects and challenges. Rep. Prog. Phys. 2011, 74, 054401. [Google Scholar] [CrossRef]
- Daley, A.J.; Fedichev, P.O.; Zoller, P. Single-atom cooling by superfluid immersion: A nondestructive method for qubits. Phys. Rev. A 2004, 69, 022306. [Google Scholar] [CrossRef]
- Aspect, A.; Arimondo, E.; Kaiser, R.; Vansteenkiste, N.; Cohen-Tannoudji, C. Laser Cooling below the One-Photon Recoil Energy by Velocity-Selective Coherent Population Trapping. Phys. Rev. Lett. 1988, 61, 826–829. [Google Scholar] [CrossRef] [PubMed]
- Kasevich, M.; Chu, S. Laser cooling below a photon recoil with three-level atoms. Phys. Rev. Lett. 1992, 69, 1741–1744. [Google Scholar] [CrossRef] [PubMed]
- Griessner, A.; Daley, A.J.; Clark, S.R.; Jaksch, D.; Zoller, P. Dark-State Cooling of Atoms by Superfluid Immersion. Phys. Rev. Lett. 2006, 97, 220403. [Google Scholar] [CrossRef] [PubMed]
- Diehl, S.; Micheli, A.; Kantian, A.; Kraus, B.; Büchler, H.P.; Zoller, P. Quantum states and phases in driven open quantum systems with cold atoms. Nat. Phys. 2008, 4, 878–883. [Google Scholar] [CrossRef]
- Kraus, B.; Büchler, H.P.; Diehl, S.; Kantian, A.; Micheli, A.; Zoller, P. Preparation of entangled states by quantum Markov processes. Phys. Rev. A 2008, 78, 042307. [Google Scholar] [CrossRef]
- Diehl, S.; Rico, E.; Baranov, M.A.; Zoller, P. Topology by dissipation in atomic quantum wires. Nat. Phys. 2011, 7, 971–977. [Google Scholar] [CrossRef]
- Yago Malo, J.; van Nieuwenburg, E.P.L.; Fischer, M.H.; Daley, A.J. Particle statistics and lossy dynamics of ultracold atoms in optical lattices. Phys. Rev. A 2018, 97, 053614. [Google Scholar] [CrossRef]
- Daley, A.J.; Taylor, J.M.; Diehl, S.; Baranov, M.; Zoller, P. Atomic Three-Body Loss as a Dynamical Three-Body Interaction. Phys. Rev. Lett. 2009, 102, 040402. [Google Scholar] [CrossRef] [PubMed]
- Kantian, A.; Dalmonte, M.; Diehl, S.; Hofstetter, W.; Zoller, P.; Daley, A.J. Atomic Color Superfluid via Three-Body Loss. Phys. Rev. Lett. 2009, 103, 240401. [Google Scholar] [CrossRef] [PubMed]
- Sieberer, L.M.; Huber, S.D.; Altman, E.; Diehl, S. Dynamical Critical Phenomena in Driven-Dissipative Systems. Phys. Rev. Lett. 2013, 110, 195301. [Google Scholar] [CrossRef] [PubMed]
- Sieberer, L.M.; Huber, S.D.; Altman, E.; Diehl, S. Nonequilibrium functional renormalization for driven-dissipative Bose-Einstein condensation. Phys. Rev. B 2014, 89, 134310. [Google Scholar] [CrossRef]
- Li, Y.; Chen, X.; Fisher, M.P.A. Quantum Zeno effect and the many-body entanglement transition. Phys. Rev. B 2018, 98, 205136. [Google Scholar] [CrossRef]
- Skinner, B.; Ruhman, J.; Nahum, A. Measurement-Induced Phase Transitions in the Dynamics of Entanglement. Phys. Rev. X 2019, 9, 031009. [Google Scholar] [CrossRef]
- Li, Y.; Chen, X.; Fisher, M.P.A. Measurement-driven entanglement transition in hybrid quantum circuits. Phys. Rev. B 2019, 100, 134306. [Google Scholar] [CrossRef]
- Potter, A.C.; Vasseur, R. Entanglement Dynamics in Hybrid Quantum Circuits. In Entanglement in Spin Chains; Springer International Publishing: Cham, Switzerland, 2022; pp. 211–249. [Google Scholar]
- Bhattacharyya, A.; Joshi, L.K.; Sundar, B. Quantum information scrambling: From holography to quantum simulators. Eur. Phys. J. C 2022, 82, 458. [Google Scholar] [CrossRef]
- Nassar, A.B.; Miret-Artés, S. Bohmian Mechanics, Open Quantum Systems and Continuous Measurements; Springer International Publishing: Berlin/Heidelberg, Germany, 2017. [Google Scholar] [CrossRef]
- Zurek, W.H. Quantum Darwinism. Nat. Phys. 2009, 5, 181–188. [Google Scholar] [CrossRef]
- Brandão, F.G.S.L.; Piani, M.; Horodecki, P. Generic emergence of classical features in quantum Darwinism. Nat. Commun. 2015, 6, 7908. [Google Scholar] [CrossRef] [PubMed]
- Hirsbrunner, M.R.; Philip, T.M.; Basa, B.; Kim, Y.; Park, M.J.; Gilbert, M.J. A review of modeling interacting transient phenomena with non-equilibrium Green functions. Rep. Prog. Phys. 2019, 82, 046001. [Google Scholar] [CrossRef]
- Cohen-Tannoudji, C.; Guéry-Odelin, D. Advances in Atomic Physics: An Overview; World Scientific Publishing: Singapore, 2011. [Google Scholar]
- Breuer, H.P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Alicki, R.; Lendi, K. Quantum Dynamical Semigroups and Applications; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 2007; Volume 717. [Google Scholar]
- Milburn, G.; Wiseman, H. Quantum Measurement and Control; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Gardiner, C.W. Quantum Noise; Springer series in synergetics; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Carmichael, H. An Open Systems Approach to Quantum Optics; Lecture Notes in Physics Monographs; Springer: Berlin/Heidelberg, Germany, 1993; Volume 18. [Google Scholar] [CrossRef]
- Gardiner, C.; Zoller, P. Quantum Noise: A Handbook of Markovian and Non-Markovian Quantum Stochastic Methods with Applications to Quantum Optics; Springer Series in Synergetics; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Barchielli, A.; Belavkin, V.P. Measurements continuous in time and a posteriori states in quantum mechanics. J. Phys. A Math. Gen. 1991, 24, 1495. [Google Scholar] [CrossRef]
- Plenio, M.B.; Knight, P.L. The quantum-jump approach to dissipative dynamics in quantum optics. Rev. Mod. Phys. 1998, 70, 101–144. [Google Scholar] [CrossRef]
- Clerk, A.A.; Devoret, M.H.; Girvin, S.M.; Marquardt, F.; Schoelkopf, R.J. Introduction to quantum noise, measurement, and amplification. Rev. Mod. Phys. 2010, 82, 1155–1208. [Google Scholar] [CrossRef]
- Dalibard, J.; Castin, Y.; Mølmer, K. Wave-function approach to dissipative processes in quantum optics. Phys. Rev. Lett. 1992, 68, 580–583. [Google Scholar] [CrossRef] [PubMed]
- Mølmer, K.; Castin, Y.; Dalibard, J. Monte Carlo wave-function method in quantum optics. J. Opt. Soc. Am. B 1993, 10, 524–538. [Google Scholar] [CrossRef]
- Dum, R.; Zoller, P.; Ritsch, H. Monte Carlo simulation of the atomic master equation for spontaneous emission. Phys. Rev. A 1992, 45, 4879–4887. [Google Scholar] [CrossRef] [PubMed]
- Dum, R.; Parkins, A.S.; Zoller, P.; Gardiner, C.W. Monte Carlo simulation of master equations in quantum optics for vacuum, thermal, and squeezed reservoirs. Phys. Rev. A 1992, 46, 4382–4396. [Google Scholar] [CrossRef] [PubMed]
- Bergquist, J.C.; Hulet, R.G.; Itano, W.M.; Wineland, D.J. Observation of Quantum Jumps in a Single Atom. Phys. Rev. Lett. 1986, 57, 1699–1702. [Google Scholar] [CrossRef] [PubMed]
- Nagourney, W.; Sandberg, J.; Dehmelt, H. Shelved optical electron amplifier: Observation of quantum jumps. Phys. Rev. Lett. 1986, 56, 2797–2799. [Google Scholar] [CrossRef] [PubMed]
- Sauter, T.; Neuhauser, W.; Blatt, R.; Toschek, P.E. Observation of Quantum Jumps. Phys. Rev. Lett. 1986, 57, 1696–1698. [Google Scholar] [CrossRef] [PubMed]
- Yu, Y.; Zhu, S.L.; Sun, G.; Wen, X.; Dong, N.; Chen, J.; Wu, P.; Han, S. Quantum Jumps between Macroscopic Quantum States of a Superconducting Qubit Coupled to a Microscopic Two-Level System. Phys. Rev. Lett. 2008, 101, 157001. [Google Scholar] [CrossRef] [PubMed]
- Vamivakas, A.N.; Lu, C.Y.; Matthiesen, C.; Zhao, Y.; Fält, S.; Badolato, A.; Atatüre, M. Observation of spin-dependent quantum jumps via quantum dot resonance fluorescence. Nature 2010, 467, 297–300. [Google Scholar] [CrossRef] [PubMed]
- Minev, Z.K.; Mundhada, S.O.; Shankar, S.; Reinhold, P.; Gutiérrez-Jáuregui, R.; Schoelkopf, R.J.; Mirrahimi, M.; Carmichael, H.J.; Devoret, M.H. To catch and reverse a quantum jump mid-flight. Nature 2019, 570, 200–204. [Google Scholar] [CrossRef]
- Wiseman, H.M. Quantum Trajectories and Feedback. PhD Thesis, University of Queensland, Brisbane, Australia, 1994. [Google Scholar]
- Belavkin, V.P. Quantum stochastic semigroups and their generators. In Irreversibility and Causality Semigroups and Rigged Hilbert Spaces; Bohm, A., Doebner, H.D., Kielanowski, P., Eds.; Springer: Berlin/Heidelberg, Germany, 1998; pp. 82–109. [Google Scholar]
- Barchielli, A. Direct and heterodyne detection and other applications of quantum stochastic calculus to quantum optics. Quantum Opt. J. Eur. Opt. Soc. Part B 1990, 2, 423. [Google Scholar] [CrossRef]
- Wiseman, H.M.; Milburn, G.J. Quantum theory of optical feedback via homodyne detection. Phys. Rev. Lett. 1993, 70, 548–551. [Google Scholar] [CrossRef]
- Wiseman, H.M. Quantum theory of continuous feedback. Phys. Rev. A 1994, 49, 2133–2150. [Google Scholar] [CrossRef] [PubMed]
- Murch, K.W.; Weber, S.J.; Macklin, C.; Siddiqi, I. Observing single quantum trajectories of a superconducting quantum bit. Nature 2013, 502, 211–214. [Google Scholar] [CrossRef] [PubMed]
- Hatridge, M.; Shankar, S.; Mirrahimi, M.; Schackert, F.; Geerlings, K.; Brecht, T.; Sliwa, K.M.; Abdo, B.; Frunzio, L.; Girvin, S.M.; et al. Quantum Back-Action of an Individual Variable-Strength Measurement. Science 2013, 339, 178–181. [Google Scholar] [CrossRef] [PubMed]
- Wiseman, H. Using feedback to eliminate back-action in quantum measurements. Phys. Rev. A 1995, 51, 2459. [Google Scholar] [CrossRef] [PubMed]
- Korotkov, A.N. Simple quantum feedback of a solid-state qubit. Phys. Rev. B 2005, 71, 201305. [Google Scholar] [CrossRef]
- Korotkov, A.N.; Jordan, A.N. Undoing a Weak Quantum Measurement of a Solid-State Qubit. Phys. Rev. Lett. 2006, 97, 166805. [Google Scholar] [CrossRef] [PubMed]
- De Lange, G.; Ristè, D.; Tiggelman, M.J.; Eichler, C.; Tornberg, L.; Johansson, G.; Wallraff, A.; Schouten, R.N.; DiCarlo, L. Reversing Quantum Trajectories with Analog Feedback. Phys. Rev. Lett. 2014, 112, 080501. [Google Scholar] [CrossRef]
- Brun, T.A. A simple model of quantum trajectories. Am. J. Phys. 2002, 70, 719–737. [Google Scholar] [CrossRef]
- Scarani, V.; Ziman, M.; Štelmachovič, P.; Gisin, N.; Bužek, V. Thermalizing Quantum Machines: Dissipation and Entanglement. Phys. Rev. Lett. 2002, 88, 097905. [Google Scholar] [CrossRef] [PubMed]
- Ciccarello, F.; Lorenzo, S.; Giovannetti, V.; Palma, G.M. Quantum collision models: Open system dynamics from repeated interactions. Phys. Rep. 2022, 954, 1–70. [Google Scholar] [CrossRef]
- Campbell, S.; Vacchini, B. Collision models in open system dynamics: A versatile tool for deeper insights? Europhys. Lett. 2021, 133, 60001. [Google Scholar] [CrossRef]
- Rau, J. Relaxation Phenomena in Spin and Harmonic Oscillator Systems. Phys. Rev. 1963, 129, 1880–1888. [Google Scholar] [CrossRef]
- Facchi, P.; Marzolino, U.; Parisi, G.; Pascazio, S.; Scardicchio, A. Phase Transitions of Bipartite Entanglement. Phys. Rev. Lett. 2008, 101, 050502. [Google Scholar] [CrossRef] [PubMed]
- Tian, F.; Zou, J.; Li, L.; Li, H.; Shao, B. Effect of Inter-System Coupling on Heat Transport in a Microscopic Collision Model. Entropy 2021, 23, 471. [Google Scholar] [CrossRef] [PubMed]
- Chisholm, D.A.; García-Pérez, G.; Rossi, M.A.C.; Palma, G.M.; Maniscalco, S. Stochastic collision model approach to transport phenomena in quantum networks. New J. Phys. 2021, 23, 033031. [Google Scholar] [CrossRef]
- Cakmak, B.; Campbell, S.; Vacchini, B.; Müstecaplıoğlu, O.E.; Paternostro, M. Robust multipartite entanglement generation via a collision model. Phys. Rev. A 2019, 99, 012319. [Google Scholar] [CrossRef]
- Rybár, T.; Filippov, S.N.; Ziman, M.; Bužek, V. Simulation of indivisible qubit channels in collision models. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 154006. [Google Scholar] [CrossRef]
- Ciccarello, F.; Palma, G.M.; Giovannetti, V. Collision-model-based approach to non-Markovian quantum dynamics. Phys. Rev. A 2013, 87, 040103. [Google Scholar] [CrossRef]
- Rivas, Á.; Huelga, S.F.; Plenio, M.B. Quantum non-Markovianity: Characterization, quantification and detection. Rep. Prog. Phys. 2014, 77, 094001. [Google Scholar] [CrossRef] [PubMed]
- Breuer, H.P.; Laine, E.M.; Piilo, J.; Vacchini, B. Colloquium: Non-Markovian dynamics in open quantum systems. Rev. Mod. Phys. 2016, 88, 021002. [Google Scholar] [CrossRef]
- De Vega, I.; Alonso, D. Dynamics of non-Markovian open quantum systems. Rev. Mod. Phys. 2017, 89, 015001. [Google Scholar] [CrossRef]
- Li, L.; Hall, M.J.; Wiseman, H.M. Concepts of quantum non-Markovianity: A hierarchy. Phys. Rep. 2018, 759, 1–51. [Google Scholar] [CrossRef]
- Breuer, H.P. Foundations and measures of quantum non-Markovianity. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 154001. [Google Scholar] [CrossRef]
- Guo, Y.; Kroeze, R.M.; Marsh, B.P.; Gopalakrishnan, S.; Keeling, J.; Lev, B.L. An optical lattice with sound. Nature 2021, 599, 211–215. [Google Scholar] [CrossRef] [PubMed]
- Maldacena, J.; Shenker, S.H.; Stanford, D. A bound on chaos. J. High Energy Phys. 2016, 2016, 106. [Google Scholar] [CrossRef]
- Preskill, J. Quantum Shannon Theory. arXiv 2016, arXiv:1604.07450. [Google Scholar]
- Jaksch, D.; Zoller, P. The cold atom Hubbard toolbox. Ann. Phys. 2005, 315, 52–79. [Google Scholar] [CrossRef]
- Navarrete-Benlloch, C.; de Vega, I.; Porras, D.; Cirac, J.I. Simulating quantum-optical phenomena with cold atoms in optical lattices. New J. Phys. 2011, 13, 023024. [Google Scholar] [CrossRef]
- Schön, G.; Zaikin, A. Quantum coherent effects, phase transitions, and the dissipative dynamics of ultra small tunnel junctions. Phys. Rep. 1990, 198, 237–412. [Google Scholar] [CrossRef]
- Cohen-Tannoudji, J. Dupont-Roc, G.G. Atom-Photon Interactions: Basic Processes and Applications; Wiley: Hoboken, NJ, USA, 1992. [Google Scholar]
- Whitney, R.S. Staying positive: Going beyond Lindblad with perturbative master equations. J. Phys. A Math. Theor. 2008, 41, 175304. [Google Scholar] [CrossRef]
- Li, A.C.Y.; Petruccione, F.; Koch, J. Resummation for Nonequilibrium Perturbation Theory and Application to Open Quantum Lattices. Phys. Rev. X 2016, 6, 021037. [Google Scholar] [CrossRef]
- Piilo, J.; Härkönen, K.; Maniscalco, S.; Suominen, K.A. Open system dynamics with non-Markovian quantum jumps. Phys. Rev. A 2009, 79, 062112. [Google Scholar] [CrossRef]
- Diósi, L.; Gisin, N.; Strunz, W.T. Non-Markovian quantum state diffusion. Phys. Rev. A 1998, 58, 1699–1712. [Google Scholar] [CrossRef]
- Hartmann, R.; Strunz, W.T. Exact Open Quantum System Dynamics Using the Hierarchy of Pure States (HOPS). J. Chem. Theory Comput. 2017, 13, 5834–5845. [Google Scholar] [CrossRef] [PubMed]
- Ishizaki, A.; Fleming, G.R. Unified treatment of quantum coherent and incoherent hopping dynamics in electronic energy transfer: Reduced hierarchy equation approach. J. Chem. Phys. 2009, 130, 234111. [Google Scholar] [CrossRef] [PubMed]
- Engel, G.S.; Calhoun, T.R.; Read, E.L.; Ahn, T.K.; Mančal, T.; Cheng, Y.C.; Blankenship, R.E.; Fleming, G.R. Evidence for wavelike energy transfer through quantum coherence in photosynthetic systems. Nature 2007, 446, 782–786. [Google Scholar] [CrossRef] [PubMed]
- Lu, X.M.; Wang, X.; Sun, C.P. Quantum Fisher information flow and non-Markovian processes of open systems. Phys. Rev. A 2010, 82, 042103. [Google Scholar] [CrossRef]
- Breuer, H.P.; Laine, E.M.; Piilo, J. Measure for the Degree of Non-Markovian Behavior of Quantum Processes in Open Systems. Phys. Rev. Lett. 2009, 103, 210401. [Google Scholar] [CrossRef] [PubMed]
- White, S.R. Density matrix formulation for quantum renormalization groups. Phys. Rev. Lett. 1992, 69, 2863–2866. [Google Scholar] [CrossRef] [PubMed]
- White, S.R. Density-matrix algorithms for quantum renormalization groups. Phys. Rev. B 1993, 48, 10345–10356. [Google Scholar] [CrossRef]
- Östlund, S.; Rommer, S. Thermodynamic Limit of Density Matrix Renormalization. Phys. Rev. Lett. 1995, 75, 3537–3540. [Google Scholar] [CrossRef] [PubMed]
- Rommer, S.; Östlund, S. Class of ansatz wave functions for one-dimensional spin systems and their relation to the density matrix renormalization group. Phys. Rev. B 1997, 55, 2164–2181. [Google Scholar] [CrossRef]
- Vidal, G. Efficient Classical Simulation of Slightly Entangled Quantum Computations. Phys. Rev. Lett. 2003, 91, 147902. [Google Scholar] [CrossRef] [PubMed]
- Daley, A.J.; Kollath, C.; Schollwöck, U.; Vidal, G. Time-dependent density-matrix renormalization-group using adaptive effective Hilbert spaces. J. Stat. Mech. Theory Exp. 2004, 2004, P04005. [Google Scholar] [CrossRef]
- White, S.R.; Feiguin, A.E. Real-Time Evolution Using the Density Matrix Renormalization Group. Phys. Rev. Lett. 2004, 93, 076401. [Google Scholar] [CrossRef] [PubMed]
- Eisert, J.; Cramer, M.; Plenio, M.B. Colloquium: Area laws for the entanglement entropy. Rev. Mod. Phys. 2010, 82, 277–306. [Google Scholar] [CrossRef]
- Page, D.N. Average entropy of a subsystem. Phys. Rev. Lett. 1993, 71, 1291–1294. [Google Scholar] [CrossRef] [PubMed]
- Verstraete, F.; García-Ripoll, J.J.; Cirac, J.I. Matrix Product Density Operators: Simulation of Finite-Temperature and Dissipative Systems. Phys. Rev. Lett. 2004, 93, 207204. [Google Scholar] [CrossRef]
- Pirvu, B.; Murg, V.; Cirac, J.I.; Verstraete, F. Matrix product operator representations. New J. Phys. 2010, 12, 025012. [Google Scholar] [CrossRef]
- Wall, M.L.; Carr, L.D. Out-of-equilibrium dynamics with matrix product states. New J. Phys. 2012, 14, 125015. [Google Scholar] [CrossRef]
- Verstraete, F.; Murg, V.; Cirac, J. Matrix product states, projected entangled pair states, and variational renormalization group methods for quantum spin systems. Adv. Phys. 2008, 57, 143–224. [Google Scholar] [CrossRef]
- Schollwöck, U. The density-matrix renormalization group in the age of matrix product states. Ann. Phys. 2011, 326, 96–192. [Google Scholar] [CrossRef]
- Cirac, J.I.; Pérez-García, D.; Schuch, N.; Verstraete, F. Matrix product states and projected entangled pair states: Concepts, symmetries, theorems. Rev. Mod. Phys. 2021, 93, 045003. [Google Scholar] [CrossRef]
- Orús, R. Tensor networks for complex quantum systems. Nat. Rev. Phys. 2019, 1, 538–550. [Google Scholar] [CrossRef]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information: 10th Anniversary Edition; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Malo, J.Y. Dissipative Engineering of Cold Atoms in Optical Lattices. University of Strathclyde. 2019. Available online: http://localhost/files/g732d907d (accessed on 26 August 2021).
- Zauner, V.; Draxler, D.; Vanderstraeten, L.; Degroote, M.; Haegeman, J.; Rams, M.M.; Stojevic, V.; Schuch, N.; Verstraete, F. Transfer matrices and excitations with matrix product states. New J. Phys. 2015, 17, 053002. [Google Scholar] [CrossRef]
- Van Damme, M.; Vanhove, R.; Haegeman, J.; Verstraete, F.; Vanderstraeten, L. Efficient matrix product state methods for extracting spectral information on rings and cylinders. Phys. Rev. B 2021, 104, 115142. [Google Scholar] [CrossRef]
- Yu, X.; Pekker, D.; Clark, B.K. Finding Matrix Product State Representations of Highly Excited Eigenstates of Many-Body Localized Hamiltonians. Phys. Rev. Lett. 2017, 118, 017201. [Google Scholar] [CrossRef] [PubMed]
- Dutta, S.; Buyskikh, A.; Daley, A.J.; Mueller, E.J. Density Matrix Renormalization Group for Continuous Quantum Systems. Phys. Rev. Lett. 2022, 128, 230401. [Google Scholar] [CrossRef] [PubMed]
- Pirvu, B.; Vidal, G.; Verstraete, F.; Tagliacozzo, L. Matrix product states for critical spin chains: Finite-size versus finite-entanglement scaling. Phys. Rev. B 2012, 86, 075117. [Google Scholar] [CrossRef]
- Milsted, A.; Haegeman, J.; Osborne, T.J. Matrix product states and variational methods applied to critical quantum field theory. Phys. Rev. D 2013, 88, 085030. [Google Scholar] [CrossRef]
- Haegeman, J.; Cirac, J.I.; Osborne, T.J.; Pižorn, I.; Verschelde, H.; Verstraete, F. Time-Dependent Variational Principle for Quantum Lattices. Phys. Rev. Lett. 2011, 107, 070601. [Google Scholar] [CrossRef] [PubMed]
- Haegeman, J.; Lubich, C.; Oseledets, I.; Vandereycken, B.; Verstraete, F. Unifying time evolution and optimization with matrix product states. Phys. Rev. B 2016, 94, 165116. [Google Scholar] [CrossRef]
- Cui, J.; Cirac, J.I.; Bañuls, M.C. Variational Matrix Product Operators for the Steady State of Dissipative Quantum Systems. Phys. Rev. Lett. 2015, 114, 220601. [Google Scholar] [CrossRef] [PubMed]
- Strathearn, A.; Kirton, P.; Kilda, D.; Keeling, J.; Lovett, B.W. Efficient non-Markovian quantum dynamics using time-evolving matrix product operators. Nat. Commun. 2018, 9, 3322. [Google Scholar] [CrossRef]
- Flannigan, S.; Damanet, F.; Daley, A.J. Many-Body Quantum State Diffusion for Non-Markovian Dynamics in Strongly Interacting Systems. Phys. Rev. Lett. 2022, 128, 063601. [Google Scholar] [CrossRef] [PubMed]
- Weimer, H.; Kshetrimayum, A.; Orús, R. Simulation methods for open quantum many-body systems. Rev. Mod. Phys. 2021, 93, 015008. [Google Scholar] [CrossRef]
- Vidal, G. Entanglement Renormalization. Phys. Rev. Lett. 2007, 99, 220405. [Google Scholar] [CrossRef] [PubMed]
- Kadow, W.; Pollmann, F.; Knap, M. Isometric tensor network representations of two-dimensional thermal states. Phys. Rev. B 2023, 107, 205106. [Google Scholar] [CrossRef]
- Felser, T.; Notarnicola, S.; Montangero, S. Efficient Tensor Network Ansatz for High-Dimensional Quantum Many-Body Problems. Phys. Rev. Lett. 2021, 126, 170603. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.P.; Fu, Y.F.; Xie, Z.Y.; Xiang, T. Efficient calculation of three-dimensional tensor networks. Phys. Rev. B 2023, 107, 165127. [Google Scholar] [CrossRef]
- Bañuls, M.C. Tensor Network Algorithms: A Route Map. Annu. Rev. Condens. Matter Phys. 2023, 14, 173–191. [Google Scholar] [CrossRef]
- Zohar, E. Quantum simulation of lattice gauge theories in more than one space dimension—Requirements, challenges and methods. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2022, 380, 20210069. [Google Scholar] [CrossRef] [PubMed]
- Chanda, T.; Zakrzewski, J.; Lewenstein, M.; Tagliacozzo, L. Confinement and Lack of Thermalization after Quenches in the Bosonic Schwinger Model. Phys. Rev. Lett. 2020, 124, 180602. [Google Scholar] [CrossRef] [PubMed]
- Bañuls, M.C.; Cichy, K. Review on novel methods for lattice gauge theories. Rep. Prog. Phys. 2020, 83, 024401. [Google Scholar] [CrossRef] [PubMed]
- Shachar, T.; Zohar, E. Approximating relativistic quantum field theories with continuous tensor networks. Phys. Rev. D 2022, 105, 045016. [Google Scholar] [CrossRef]
- Jahn, A.; Eisert, J. Holographic tensor network models and quantum error correction: A topical review. Quantum Sci. Technol. 2021, 6, 033002. [Google Scholar] [CrossRef]
- Gasull, A.; Tilloy, A.; Cirac, J.I.; Sierra, G. Symmetries and field tensor network states. Phys. Rev. B 2023, 107, 155102. [Google Scholar] [CrossRef]
- Huang, R.Z.; Zhang, L.; Läuchli, A.M.; Haegeman, J.; Verstraete, F.; Vanderstraeten, L. Emergent conformal boundaries from finite-entanglement scaling in matrix product states. arXiv 2023, arXiv:2306.08163. [Google Scholar] [CrossRef] [PubMed]
- Bañuls, M.C.; Garrahan, J.P. Using Matrix Product States to Study the Dynamical Large Deviations of Kinetically Constrained Models. Phys. Rev. Lett. 2019, 123, 200601. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Iblisdir, S.; Cirac, J.I.; Bañuls, M.C. Probing Thermalization through Spectral Analysis with Matrix Product Operators. Phys. Rev. Lett. 2020, 124, 100602. [Google Scholar] [CrossRef] [PubMed]
- Cheng, S.; Cao, C.; Zhang, C.; Liu, Y.; Hou, S.Y.; Xu, P.; Zeng, B. Simulating noisy quantum circuits with matrix product density operators. Phys. Rev. Res. 2021, 3, 023005. [Google Scholar] [CrossRef]
- Lanyon, B.P.; Maier, C.; Holzäpfel, M.; Baumgratz, T.; Hempel, C.; Jurcevic, P.; Dhand, I.; Buyskikh, A.S.; Daley, A.J.; Cramer, M.; et al. Efficient tomography of a quantum many-body system. Nat. Phys. 2017, 13, 1158–1162. [Google Scholar] [CrossRef]
- Guo, Y.; Yang, S. Quantum Error Mitigation via Matrix Product Operators. PRX Quantum 2022, 3, 040313. [Google Scholar] [CrossRef]
- Wall, M.L.; Titum, P.; Quiroz, G.; Foss-Feig, M.; Hazzard, K.R.A. Tensor-network discriminator architecture for classification of quantum data on quantum computers. Phys. Rev. A 2022, 105, 062439. [Google Scholar] [CrossRef]
- Schiffer, B.F.; Tura, J.; Cirac, J.I. Adiabatic Spectroscopy and a Variational Quantum Adiabatic Algorithm. PRX Quantum 2022, 3, 020347. [Google Scholar] [CrossRef]
- Napp, J.C.; La Placa, R.L.; Dalzell, A.M.; Brandão, F.G.S.L.; Harrow, A.W. Efficient Classical Simulation of Random Shallow 2D Quantum Circuits. Phys. Rev. X 2022, 12, 021021. [Google Scholar] [CrossRef]
- Carleo, G.; Troyer, M. Solving the quantum many-body problem with artificial neural networks. Science 2017, 355, 602–606. [Google Scholar] [CrossRef] [PubMed]
- Melko, R.G.; Carleo, G.; Carrasquilla, J.; Cirac, J.I. Restricted Boltzmann machines in quantum physics. Nat. Phys. 2019, 15, 887–892. [Google Scholar] [CrossRef]
- Huang, H.Y.; Kueng, R.; Torlai, G.; Albert, V.V.; Preskill, J. Provably efficient machine learning for quantum many-body problems. Science 2022, 377, eabk3333. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Cheng, S.; Xie, H.; Wang, L.; Xiang, T. Equivalence of restricted Boltzmann machines and tensor network states. Phys. Rev. B 2018, 97, 085104. [Google Scholar] [CrossRef]
- Huggins, W.; Patil, P.; Mitchell, B.; Whaley, K.B.; Stoudenmire, E.M. Towards quantum machine learning with tensor networks. Quantum Sci. Technol. 2019, 4, 024001. [Google Scholar] [CrossRef]
- Sharir, O.; Shashua, A.; Carleo, G. Neural tensor contractions and the expressive power of deep neural quantum states. Phys. Rev. B 2022, 106, 205136. [Google Scholar] [CrossRef]
- Ganahl, M.; Beall, J.; Hauru, M.; Lewis, A.G.; Wojno, T.; Yoo, J.H.; Zou, Y.; Vidal, G. Density Matrix Renormalization Group with Tensor Processing Units. PRX Quantum 2023, 4, 010317. [Google Scholar] [CrossRef]
- Xu, L.; Cheng, L.; Wong, N.; Wu, Y.C. Tensor train factorization under noisy and incomplete data with automatic rank estimation. Pattern Recognit. 2023, 141, 109650. [Google Scholar] [CrossRef]
- Hur, Y.; Hoskins, J.G.; Lindsey, M.; Stoudenmire, E.M.; Khoo, Y. Generative modeling via tensor train sketching. arXiv 2022, arXiv:2202.11788. [Google Scholar] [CrossRef]
- Ghosh, S.K.; Ghosh, D. Machine learning matrix product state ansatz for strongly correlated systems. J. Chem. Phys. 2023, 158, 064108. [Google Scholar] [CrossRef]
- Derbyshire, E.; Malo, J.Y.; Daley, A.J.; Kashefi, E.; Wallden, P. Randomized benchmarking in the analogue setting. Quantum Sci. Technol. 2020, 5, 034001. [Google Scholar] [CrossRef]
- Argüello-Luengo, J.; González-Tudela, A.; Shi, T.; Zoller, P.; Cirac, J.I. Analogue quantum chemistry simulation. Nature 2019, 574, 215–218. [Google Scholar] [CrossRef] [PubMed]
- Lubasch, M.; Joo, J.; Moinier, P.; Kiffner, M.; Jaksch, D. Variational quantum algorithms for nonlinear problems. Phys. Rev. A 2020, 101, 010301. [Google Scholar] [CrossRef]
- Cao, Y.; Romero, J.; Olson, J.P.; Degroote, M.; Johnson, P.D.; Kieferová, M.; Kivlichan, I.D.; Menke, T.; Peropadre, B.; Sawaya, N.P.D.; et al. Quantum Chemistry in the Age of Quantum Computing. Chem. Rev. 2019, 119, 10856–10915. [Google Scholar] [CrossRef] [PubMed]
- Baiardi, A.; Reiher, M. The density matrix renormalization group in chemistry and molecular physics: Recent developments and new challenges. J. Chem. Phys. 2020, 152, 040903. [Google Scholar] [CrossRef] [PubMed]
- Pollet, L. Recent developments in quantum Monte Carlo simulations with applications for cold gases. Rep. Prog. Phys. 2012, 75, 094501. [Google Scholar] [CrossRef] [PubMed]
- Sieberer, L.M.; Buchhold, M.; Diehl, S. Keldysh field theory for driven open quantum systems. Rep. Prog. Phys. 2016, 79, 096001. [Google Scholar] [CrossRef] [PubMed]
- Sánchez-Soto, L.L.; Monzón, J.J.; Barriuso, A.G.; Cariñena, J.F. The transfer matrix: A geometrical perspective. Phys. Rep. 2012, 513, 191–227. [Google Scholar] [CrossRef]
- Haegeman, J.; Verstraete, F. Diagonalizing Transfer Matrices and Matrix Product Operators: A Medley of Exact and Computational Methods. Annu. Rev. Condens. Matter Phys. 2017, 8, 355–406. [Google Scholar] [CrossRef]
- Sinatra, A.; Lobo, C.; Castin, Y. The truncated Wigner method for Bose-condensed gases: Limits of validity and applications. J. Phys. B At. Mol. Opt. Phys. 2002, 35, 3599. [Google Scholar] [CrossRef]
- Polkovnikov, A. Phase space representation of quantum dynamics. Ann. Phys. 2010, 325, 1790–1852. [Google Scholar] [CrossRef]
- Motta, M.; Rice, J.E. Emerging quantum computing algorithms for quantum chemistry. WIREs Comput. Mol. Sci. 2022, 12, e1580. [Google Scholar] [CrossRef]
- Blunt, N.S.; Camps, J.; Crawford, O.; Izsák, R.; Leontica, S.; Mirani, A.; Moylett, A.E.; Scivier, S.A.; Sünderhauf, C.; Schopf, P.; et al. Perspective on the Current State-of-the-Art of Quantum Computing for Drug Discovery Applications. J. Chem. Theory Comput. 2022, 18, 7001–7023. [Google Scholar] [CrossRef] [PubMed]
- Orús, R.; Mugel, S.; Lizaso, E. Quantum computing for finance: Overview and prospects. Rev. Phys. 2019, 4, 100028. [Google Scholar] [CrossRef]
- Biamonte, J.; Wittek, P.; Pancotti, N.; Rebentrost, P.; Wiebe, N.; Lloyd, S. Quantum machine learning. Nature 2017, 549, 195–202. [Google Scholar] [CrossRef] [PubMed]
- Shor, P.W. Polynomial-Time Algorithms for Prime Factorization and Discrete Logarithms on a Quantum Computer. SIAM J. Comput. 1997, 26, 1484–1509. [Google Scholar] [CrossRef]
- Bharti, K.; Cervera-Lierta, A.; Kyaw, T.H.; Haug, T.; Alperin-Lea, S.; Anand, A.; Degroote, M.; Heimonen, H.; Kottmann, J.S.; Menke, T.; et al. Noisy intermediate-scale quantum algorithms. Rev. Mod. Phys. 2022, 94, 015004. [Google Scholar] [CrossRef]
- DiVincenzo, D.P. The Physical Implementation of Quantum Computation. Fortschritte Der Phys. 2000, 48, 771–783. [Google Scholar] [CrossRef]
- Preskill, J. Quantum Computing in the NISQ era and beyond. Quantum 2018, 2, 79. [Google Scholar] [CrossRef]
- Gill, S.S.; Kumar, A.; Singh, H.; Singh, M.; Kaur, K.; Usman, M.; Buyya, R. Quantum computing: A taxonomy, systematic review and future directions. Softw. Pract. Exp. 2022, 52, 66–114. [Google Scholar] [CrossRef]
- Escofet, P.; Rached, S.B.; Rodrigo, S.; Almudever, C.G.; Alarcón, E.; Abadal, S. Interconnect Fabrics for Multi-Core Quantum Processors: A Context Analysis. In Proceedings of the 16th International Workshop on Network on Chip Architectures, NoCArc’23, Toronto, ON, Canada, 28 October 2023; pp. 34–39. [Google Scholar] [CrossRef]
- Singh, A.; Dev, K.; Siljak, H.; Joshi, H.D.; Magarini, M. Quantum Internet—Applications, Functionalities, Enabling Technologies, Challenges, and Research Directions. IEEE Commun. Surv. Tutorials 2021, 23, 2218–2247. [Google Scholar] [CrossRef]
- Shor, P.W. Scheme for reducing decoherence in quantum computer memory. Phys. Rev. A 1995, 52, R2493–R2496. [Google Scholar] [CrossRef] [PubMed]
- Terhal, B.M. Quantum error correction for quantum memories. Rev. Mod. Phys. 2015, 87, 307–346. [Google Scholar] [CrossRef]
- Aharonov, D.; Ben-Or, M. Fault-Tolerant Quantum Computation with Constant Error Rate. SIAM J. Comput. 2008, 38, 1207–1282. [Google Scholar] [CrossRef]
- Knill, E.; Laflamme, R.; Zurek, W.H. Resilient Quantum Computation. Science 1998, 279, 342–345. [Google Scholar] [CrossRef]
- Kitaev, A. Fault-tolerant quantum computation by anyons. Ann. Phys. 2003, 303, 2–30. [Google Scholar] [CrossRef]
- Noiri, A.; Takeda, K.; Nakajima, T.; Kobayashi, T.; Sammak, A.; Scappucci, G.; Tarucha, S. Fast universal quantum gate above the fault-tolerance threshold in silicon. Nature 2022, 601, 338–342. [Google Scholar] [CrossRef] [PubMed]
- Abobeih, M.H.; Wang, Y.; Randall, J.; Loenen, S.J.H.; Bradley, C.E.; Markham, M.; Twitchen, D.J.; Terhal, B.M.; Taminiau, T.H. Fault-tolerant operation of a logical qubit in a diamond quantum processor. Nature 2022, 606, 884–889. [Google Scholar] [CrossRef] [PubMed]
- Sun, K.; Hao, Z.Y.; Wang, Y.; Li, J.K.; Xu, X.Y.; Xu, J.S.; Han, Y.J.; Li, C.F.; Guo, G.C. Optical demonstration of quantum fault-tolerant threshold. Light Sci. Appl. 2022, 11, 203. [Google Scholar] [CrossRef] [PubMed]
- Campagne-Ibarcq, P.; Eickbusch, A.; Touzard, S.; Zalys-Geller, E.; Frattini, N.E.; Sivak, V.V.; Reinhold, P.; Puri, S.; Shankar, S.; Schoelkopf, R.J.; et al. Quantum error correction of a qubit encoded in grid states of an oscillator. Nature 2020, 584, 368–372. [Google Scholar] [CrossRef] [PubMed]
- Noh, K.; Chamberland, C. Fault-tolerant bosonic quantum error correction with the surface—Gottesman-Kitaev-Preskill code. Phys. Rev. A 2020, 101, 012316. [Google Scholar] [CrossRef]
- Bombin, H.; Andrist, R.S.; Ohzeki, M.; Katzgraber, H.G.; Martin-Delgado, M.A. Strong Resilience of Topological Codes to Depolarization. Phys. Rev. X 2012, 2, 021004. [Google Scholar] [CrossRef]
- Crosson, E.J.; Lidar, D.A. Prospects for quantum enhancement with diabatic quantum annealing. Nat. Rev. Phys. 2021, 3, 466–489. [Google Scholar] [CrossRef]
- Egan, L.; Debroy, D.M.; Noel, C.; Risinger, A.; Zhu, D.; Biswas, D.; Newman, M.; Li, M.; Brown, K.R.; Cetina, M.; et al. Fault-tolerant control of an error-corrected qubit. Nature 2021, 598, 281–286. [Google Scholar] [CrossRef] [PubMed]
- Lockwood, O. An Empirical Review of Optimization Techniques for Quantum Variational Circuits. arXiv 2022, arXiv:2202.01389. [Google Scholar]
- Peruzzo, A.; McClean, J.; Shadbolt, P.; Yung, M.H.; Zhou, X.Q.; Love, P.J.; Aspuru-Guzik, A.; O’brien, J.L. A variational eigenvalue solver on a photonic quantum processor. Nat. Commun. 2014, 5, 4213. [Google Scholar] [CrossRef] [PubMed]
- Fitzpatrick, A.; Nykänen, A.; Talarico, N.W.; Lunghi, A.; Maniscalco, S.; García-Pérez, G.; Knecht, S. A self-consistent field approach for the variational quantum eigensolver: Orbital optimization goes adaptive. arXiv 2022, arXiv:2212.11405. [Google Scholar] [CrossRef]
- Nykänen, A.; Rossi, M.A.C.; Borrelli, E.M.; Maniscalco, S.; García-Pérez, G. Mitigating the measurement overhead of ADAPT-VQE with optimised informationally complete generalised measurements. arXiv 2022, arXiv:2212.09719. [Google Scholar]
- McClean, J.R.; Romero, J.; Babbush, R.; Aspuru-Guzik, A. The theory of variational hybrid quantum-classical algorithms. New J. Phys. 2016, 18, 023023. [Google Scholar] [CrossRef]
- Cerezo, M.; Arrasmith, A.; Babbush, R.; Benjamin, S.C.; Endo, S.; Fujii, K.; McClean, J.R.; Mitarai, K.; Yuan, X.; Cincio, L.; et al. Variational quantum algorithms. Nat. Rev. Phys. 2021, 3, 625–644. [Google Scholar] [CrossRef]
- Kadowaki, T.; Nishimori, H. Quantum annealing in the transverse Ising model. Phys. Rev. E 1998, 58, 5355. [Google Scholar] [CrossRef]
- Yarkoni, S.; Raponi, E.; Bäck, T.; Schmitt, S. Quantum annealing for industry applications: Introduction and review. Rep. Prog. Phys. 2022, 85, 104001. [Google Scholar] [CrossRef] [PubMed]
- Edward Farhi, J.G. A Quantum Approximate Optimization Algorithm. arXiv 2014, arXiv:1411.4028. [Google Scholar]
- Khan, S.U.; Awan, A.J.; Vall-Llosera, G. K-means clustering on noisy intermediate scale quantum computers. arXiv 2019, arXiv:1909.12183. [Google Scholar]
- Ding, C.; Xu, X.Y.; Zhang, S.; Huang, H.L.; Bao, W.S. Evaluating the resilience of variational quantum algorithms to leakage noise. Phys. Rev. A 2022, 106, 042421. [Google Scholar] [CrossRef]
- Fontana, E.; Fitzpatrick, N.; Ramo, D.M.; Duncan, R.; Rungger, I. Evaluating the noise resilience of variational quantum algorithms. Phys. Rev. A 2021, 104, 022403. [Google Scholar] [CrossRef]
- Gentini, L.; Cuccoli, A.; Pirandola, S.; Verrucchi, P.; Banchi, L. Noise-resilient variational hybrid quantum-classical optimization. Phys. Rev. A 2020, 102, 052414. [Google Scholar] [CrossRef]
- Holmes, Z.; Sharma, K.; Cerezo, M.; Coles, P.J. Connecting Ansatz Expressibility to Gradient Magnitudes and Barren Plateaus. PRX Quantum 2022, 3, 010313. [Google Scholar] [CrossRef]
- McClean, J.R.; Boixo, S.; Smelyanskiy, V.N.; Babbush, R.; Neven, H. Barren plateaus in quantum neural network training landscapes. Nat. Commun. 2018, 9, 4812. [Google Scholar] [CrossRef] [PubMed]
- Cerezo, M.; Sone, A.; Volkoff, T.; Cincio, L.; Coles, P.J. Cost function dependent barren plateaus in shallow parametrized quantum circuits. Nat. Commun. 2021, 12, 1791. [Google Scholar] [CrossRef] [PubMed]
- Sack, S.H.; Medina, R.A.; Michailidis, A.A.; Kueng, R.; Serbyn, M. Avoiding Barren Plateaus Using Classical Shadows. PRX Quantum 2022, 3, 020365. [Google Scholar] [CrossRef]
- Lyu, C.; Montenegro, V.; Bayat, A. Accelerated variational algorithms for digital quantum simulation of many-body ground states. Quantum 2020, 4, 324. [Google Scholar] [CrossRef]
- Skolik, A.; McClean, J.R.; Mohseni, M.; van der Smagt, P.; Leib, M. Layerwise learning for quantum neural networks. Quantum Mach. Intell. 2021, 3, 5. [Google Scholar] [CrossRef]
- Harrow, A.; Napp, J. Low-depth gradient measurements can improve convergence in variational hybrid quantum-classical algorithms. arXiv 2019, arXiv:1901.05374v1. [Google Scholar] [CrossRef]
- Warren, W.S.; Rabitz, H.; Dahleh, M. Coherent control of quantum dynamics: The dream is alive. Science 1993, 259, 1581–1589. [Google Scholar] [CrossRef] [PubMed]
- Dong, D.; Petersen, I.R. Quantum control theory and applications: A survey. IET Control. Theory Appl. 2010, 4, 2651–2671. [Google Scholar] [CrossRef]
- Rabitz, H.; de Vivie-Riedle, R.; Motzkus, M.; Kompa, K.L. Whither the future of controlling quantum phenomena? Science 2000, 288, 824–828. [Google Scholar] [CrossRef] [PubMed]
- Chu, S. Cold atoms and quantum control. Nature 2002, 416, 206–210. [Google Scholar] [CrossRef] [PubMed]
- Lloyd, S. Coherent quantum feedback. Phys. Rev. A 2000, 62, 022108. [Google Scholar] [CrossRef]
- Ball, H.; Biercuk, M.J.; Carvalho, A.R.; Chen, J.; Hush, M.; De Castro, L.A.; Li, L.; Liebermann, P.J.; Slatyer, H.J.; Edmunds, C.; et al. Software tools for quantum control: Improving quantum computer performance through noise and error suppression. Quantum Sci. Technol. 2021, 6, 044011. [Google Scholar] [CrossRef]
- Wu, R.; Pechen, A.; Brif, C.; Rabitz, H. Controllability of open quantum systems with Kraus-map dynamics. J. Phys. Math. Theor. 2007, 40, 5681–5693. [Google Scholar] [CrossRef]
- Koch, C.P.; Boscain, U.; Calarco, T.; Dirr, G.; Filipp, S.; Glaser, S.J.; Kosloff, R.; Montangero, S.; Schulte-Herbrüggen, T.; Sugny, D.; et al. Quantum optimal control in quantum technologies. Strategic report on current status, visions and goals for research in Europe. EPJ Quantum Technol. 2022, 9, 19. [Google Scholar] [CrossRef]
- Dong, D.; Petersen, I.R. Controllability of quantum systems with switching control. Int. J. Control. 2010, 83, 518–525. [Google Scholar] [CrossRef]
- Jacobs, K.; Shabani, A. Quantum feedback control: How to use verification theorems and viscosity solutions to find optimal protocols. Contemp. Phys. 2008, 49, 435–448. [Google Scholar] [CrossRef][Green Version]
- Ludlow, A.D.; Boyd, M.M.; Ye, J.; Peik, E.; Schmidt, P.O. Optical atomic clocks. Rev. Mod. Phys. 2015, 87, 637–701. [Google Scholar] [CrossRef]
- Ye, J.; Kimble, H.J.; Katori, H. Quantum state engineering and precision metrology using state-insensitive light traps. Science 2008, 320, 1734. [Google Scholar] [CrossRef] [PubMed]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Advances in quantum metrology. Nat. Photonics 2011, 5, 222. [Google Scholar] [CrossRef]
- Mølmer, K.; Sørensen, A. Multiparticle entanglement of hot trapped ions. Phys. Rev. Lett. 1999, 82, 1835. [Google Scholar] [CrossRef]
- Kasevich, M.A. Coherence with atoms. Science 2002, 298, 1363. [Google Scholar] [CrossRef] [PubMed]
- Tino, G.M.; Kasevich, M.A. Atom Interferometry. In Proceedings of the International School of Physics “Enrico Fermi”, Course CLXXXVIII, Varenna, Italy, 27 June–9 July 1966; Tino, G.M., Kasevich, M.A., Eds.; Società Italiana di Fisica; IOS Press: Bologna, Italy, 2013. [Google Scholar]
- Bongs, K.; Holynski, M.; Vovrosh, J.; Bouyer, P.; Condon, G.; Rasel, E.; Schubert, C.; Schleich, W.P.; Roura, A. Taking atom interferometric quantum sensors from the laboratory to real-world applications. Nat. Rev. Phys. 2019, 1, 731–739. [Google Scholar] [CrossRef]
- Allan, D.W. Statistics of atomic frequency standards. Proc. IEEE 1966, 54, 221–230. [Google Scholar] [CrossRef]
- Silverman, M.P. Neutron Interferometry: Lessons in Experimental Quantum Mechanics, Wave–Particle Duality, and Entanglement. J. Appl. Crystallogr. 2015, 48, 1607–1608. [Google Scholar] [CrossRef]
- Gerlich, S.; Eibenberger, S.; Tomandl, M.; Nimmrichter, S.; Hornberger, K.; Fagan, P.J.; Tüxen, J.; Mayor, M.; Arndt, M. Quantum interference of large organic molecules. Nat. Commun. 2011, 2, 263. [Google Scholar] [CrossRef]
- Kasevich, M.; Chu, S. Atomic interferometry using stimulated Raman transitions. Phys. Rev. Lett. 1991, 67, 181–184. [Google Scholar] [CrossRef] [PubMed]
- Cronin, A.D.; Schmiedmayer, J.; Pritchard, D.E. Optics and interferometry with atoms and molecules. Rev. Mod. Phys. 2009, 81, 1051. [Google Scholar] [CrossRef]
- Müller, H.; Peters, A.; Chu, S. A precision measurement of the gravitational redshift by the interference of matter waves. Nature 2010, 463, 926. [Google Scholar] [CrossRef]
- Graham, P.W.; Hogan, J.M.; Kasevich, M.A.; Rajendran, S. New method for gravitational wave detection with atomic sensors. Phys. Rev. Lett. 2013, 110, 171102. [Google Scholar] [CrossRef] [PubMed]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Quantum metrology. Phys. Rev. Lett. 2006, 96, 010401. [Google Scholar] [CrossRef] [PubMed]
- Pezzè, L.; Smerzi, A. Quantum theory of phase estimation. In Atom Interferometry; Tino, G.M., Kasevich, M.A., Eds.; IOS Press: Bologna, Italy, 2013; p. 691. [Google Scholar]
- Gross, C.; Zibold, T.; Nicklas, E.; Estève, J.; Oberthaler, M.K. Nonlinear atom interferometer surpasses classical precision limit. Nature 2010, 464, 1165. [Google Scholar] [CrossRef] [PubMed]
- Kitagawa, M.; Ueda, M. Squeezed spin states. Phys. Rev. A 1993, 47, 5138. [Google Scholar] [CrossRef] [PubMed]
- Walls, D.F. Squeezed states of light. Nature 1983, 306, 141. [Google Scholar] [CrossRef]
- Leroux, I.D.; Schleier-Smith, M.H.; Vuletić, V. Implementation of Cavity Squeezing of a Collective Atomic Spin. Phys. Rev. Lett. 2010, 104, 073602. [Google Scholar] [CrossRef] [PubMed]
- Bohnet, J.G.; Cox, K.C.; Norcia, M.A.; Weiner, J.M.; Chen, Z.; Thompson, J.K. Reduced spin measurement back-action for a phase sensitivity ten times beyond the standard quantum limit. Nat. Photonics 2014, 8, 731. [Google Scholar] [CrossRef]
- Norcia, M.A.; Lewis-Swan, R.J.; Cline, J.R.K.; Zhu, B.; Rey, A.M.; Thompson, J.K. Cavity-mediated collective spin-exchange interactions in a strontium superradiant laser. Science 2018, 361, 259. [Google Scholar] [CrossRef]
- Spagnolli, G.; Semeghini, G.; Masi, L.; Ferioli, G.; Trenkwalder, A.; Coop, S.; Landini, M.; Pezzè, L.; Modugno, G.; Inguscio, M.; et al. Crossing Over from Attractive to Repulsive Interactions in a Tunneling Bosonic Josephson Junction. Phys. Rev. Lett. 2017, 118, 230403. [Google Scholar] [CrossRef]
- Baroni, C.; Gori, G.; Chiofalo, M.L.; Trombettoni, A. Effect of Inter-Well Interactions on Non-Linear Beam Splitters for Matter-Wave Interferometers. Condensed Matter 2020, 5, 31. [Google Scholar] [CrossRef]
- Salvi, L.; Poli, N.; Vuletić, V.; Tino, G.M. Squeezing on Momentum States for Atom Interferometry. Phys. Rev. Lett. 2018, 120, 033601. [Google Scholar] [CrossRef]
- Luo, C.; Zhang, H.; Koh, V.P.W.; Wilson, J.D.; Chu, A.; Holland, M.J.; Rey, A.M.; Thompson, J.K. Cavity-Mediated Collective Momentum-Exchange Interactions. arXiv 2023, arXiv:2304.01411. [Google Scholar]
- Shankar, A.; Salvi, L.; Chiofalo, M.L.; Poli, N.; Holland, M.J. Squeezed state metrology with Bragg interferometers operating in a cavity. Quantum Sci. Technol. 2019, 4, 045010. [Google Scholar] [CrossRef]
- Wilson, J.D.; Jäger, S.B.; Reilly, J.T.; Shankar, A.; Chiofalo, M.L.; Holland, M.J. Beyond one-axis twisting: Simultaneous spin-momentum squeezing. Phys. Rev. A 2022, 106, 043711. [Google Scholar] [CrossRef]
- Tóth, G. Multipartite entanglement and high-precision metrology. Phys. Rev. A 2012, 85, 022322. [Google Scholar] [CrossRef]
- Hyllus, P.; Laskowski, W.; Krischek, R.; Schwemmer, C.; Wieczorek, W.; Weinfurter, H.; Pezzé, L.; Smerzi, A. Fisher information and multiparticle entanglement. Phys. Rev. A 2012, 85, 022321. [Google Scholar] [CrossRef]
- Ren, Z.; Li, W.; Smerzi, A.; Gessner, M. Metrological Detection of Multipartite Entanglement from Young Diagrams. Phys. Rev. Lett. 2021, 126, 080502. [Google Scholar] [CrossRef] [PubMed]
- Lepori, L.; Trombettoni, A.; Giuliano, D.; Kombe, J.; Yago Malo, J.; Daley, A.; Smerzi, A.; Chiofalo, M.L. Can multipartite entanglement be characterized by two-point connected correlation functions? J. Phys. A Math. Theor. 2023, 56, 305302. [Google Scholar] [CrossRef]
- Zanardi, P. Quantum entanglement in fermionic lattices. Phys. Rev. A 2002, 65, 042101. [Google Scholar] [CrossRef]
- Islam, R.; Ma, R.; Preiss, P.M.; Eric Tai, M.; Lukin, A.; Rispoli, M.; Greiner, M. Measuring entanglement entropy in a quantum many-body system. Nature 2015, 528, 77–83. [Google Scholar] [CrossRef] [PubMed]
- Lo Franco, R.; Compagno, G. Quantum entanglement of identical particles by standard information-theoretic notions. Sci. Rep. 2016, 6, 20603. [Google Scholar] [CrossRef] [PubMed]
- Hauke, P.; Heyl, M.; Tagliacozzo, L.; Zoller, P. Measuring multipartite entanglement through dynamic susceptibilities. Nat. Photonics 2016, 12, 778. [Google Scholar] [CrossRef]
- Lourenço, A.C.; Debarba, T.; Duzzioni, E.I. Entanglement of indistinguishable particles: A comparative study. Phys. Rev. A 2019, 99, 012341. [Google Scholar] [CrossRef]
- Lukin, A.; Rispoli, M.; Schittko, R.; Tai, M.E.; Kaufman, A.M.; Choi, S.; Khemani, V.; Léonard, J.; Greiner, M. Probing entanglement in a many-body localized system. Science 2019, 364, 256–260. [Google Scholar] [CrossRef] [PubMed]
- Naldesi, P.; Elben, A.; Minguzzi, A.; Clément, D.; Zoller, P.; Vermersch, B. Fermionic correlation functions from randomized measurements in programmable atomic quantum devices. arXiv 2022, arXiv:2205.00981. [Google Scholar] [CrossRef] [PubMed]
- Mitchell, M.W.; Palacios Alvarez, S. Colloquium: Quantum limits to the energy resolution of magnetic field sensors. Rev. Mod. Phys. 2020, 92, 021001. [Google Scholar] [CrossRef]
- Strobel, H.; Muessel, W.; Linnemann, D.; Zibold, T.; Hume, D.B.; Pezzè, L.; Smerzi, A.; Oberthaler, M.K. Fisher information and entanglement of non-Gaussian spin states. Science 2014, 345, 424–427. [Google Scholar] [CrossRef] [PubMed]
- Pezzè, L.; Li, Y.; Li, W.; Smerzi, A. Witnessing entanglement without entanglement witness operators. Proc. Natl. Acad. Sci. USA 2016, 113, 11459–11464. [Google Scholar] [CrossRef]
- Aspect, A.; Grangier, P.; Roger, G. Experimental Realization of Einstein-Podolsky-Rosen-Bohm Gedankenexperiment: A New Violation of Bell’s Inequalities. Phys. Rev. Lett. 1982, 49, 91–94. [Google Scholar] [CrossRef]
- Aspect, A.; Dalibard, J.; Roger, G. Experimental Test of Bell’s Inequalities Using Time-Varying Analyzers. Phys. Rev. Lett. 1982, 49, 1804–1807. [Google Scholar] [CrossRef]
- Greenberger, D.M.; Horne, M.A.; Shimony, A.; Zeilinger, A. Bell’s theorem without inequalities. Am. J. Phys. 1990, 58, 1131–1143. [Google Scholar] [CrossRef]
- Weihs, G.; Jennewein, T.; Simon, C.; Weinfurter, H.; Zeilinger, A. Violation of Bell’s Inequality under Strict Einstein Locality Conditions. Phys. Rev. Lett. 1998, 81, 5039–5043. [Google Scholar] [CrossRef]
- Luo, X.Y.; Zou, Y.Q.; Wu, L.N.; Liu, Q.; Han, M.F.; Tey, M.K.; You, L. Deterministic entanglement generation from driving through quantum phase transitions. Science 2017, 355, 620–623. [Google Scholar] [CrossRef] [PubMed]
- Lücke, B.; Scherer, M.; Kruse, J.; Pezzé, L.; Deuretzbacher, F.; Hyllus, P.; Topic, O.; Peise, J.; Ertmer, W.; Arlt, J.; et al. Twin Matter Waves for Interferometry Beyond the Classical Limit. Science 2011, 334, 773–776. [Google Scholar] [CrossRef] [PubMed]
- Eckert, K.; Schliemann, J.; Bruß, D.; Lewenstein, M. Quantum correlations in systems of indistinguishable particles. Ann. Phys. 2002, 299, 88. [Google Scholar] [CrossRef]
- Gabbrielli, M.; Lepori, L.; Pezzè, L. Multipartite entanglement tomography of a quantum simulator. New J. Phys. 2019, 21, 033039. [Google Scholar] [CrossRef]
- Buyskikh, A.S.; Fagotti, M.; Schachenmayer, J.; Essler, F.; Daley, A.J. Entanglement growth and correlation spreading with variable-range interactions in spin and fermionic tunneling models. Phys. Rev. A 2016, 93, 053620. [Google Scholar] [CrossRef]
- Foss-Feig, M.; Gong, Z.X.; Gorshkov, A.; Clark, C. Entanglement and spin-squeezing without infinite-range interactions. arXiv 2016, arXiv:1612.07805. [Google Scholar]
- Szigeti, S.S.; Hosten, O.; Haine, S.A. Improving cold-atom sensors with quantum entanglement: Prospects and challenges. Appl. Phys. Lett. 2021, 118, 140501. [Google Scholar] [CrossRef]
- Sachdev, S. Quantum Phase Transitions; Cambridge Press: Cambridge, UK, 2000. [Google Scholar]
- Mukherjee, B.; Shaffer, A.; Patel, P.B.; Yan, Z.; Wilson, C.C.; Crépel, V.; Fletcher, R.J.; Zwierlein, M. Crystallization of bosonic quantum Hall states in a rotating quantum gas. Nature 2022, 601, 58–62. [Google Scholar] [CrossRef] [PubMed]
- Léonard, J.; Kim, S.; Kwan, J.; Segura, P.; Grusdt, F.; Repellin, C.; Goldman, N.; Greiner, M. Realization of a fractional quantum Hall state with ultracold atoms. Nature 2023, 619, 495–499. [Google Scholar] [CrossRef] [PubMed]
- Patel, P.B.; Yan, Z.; Mukherjee, B.; Fletcher, R.J.; Struck, J.; Zwierlein, M.W. Universal sound diffusion in a strongly interacting Fermi gas. Science 2020, 370, 1222–1226. [Google Scholar] [CrossRef] [PubMed]
- Hartke, T.; Oreg, B.; Jia, N.; Zwierlein, M. Doublon-Hole Correlations and Fluctuation Thermometry in a Fermi-Hubbard Gas. Phys. Rev. Lett. 2020, 125, 113601. [Google Scholar] [CrossRef] [PubMed]
- Baroni, C.; Huang, B.; Fritsche, I.; Dobler, E.; Anich, G.; Kirilov, E.; Grimm, R.; Bastarrachea-Magnani, M.A.; Massignan, P.; Bruun, G.M. Mediated interactions between Fermi polarons and the role of impurity quantum statistics. Nat. Phys. 2023, 20, 68–73. [Google Scholar] [CrossRef]
- Strinati, G.C.; Pieri, P.; Röpke, G.; Schuck, P.; Urban, M. The BCS-BEC Crossover: From Ultra-Cold Fermi Gases to Nuclear Systems. Phys. Rep. 2018, 738, 1–76. [Google Scholar] [CrossRef]
- Holland, M.; Kokkelmans, S.J.J.M.F.; Chiofalo, M.L.; Walser, R. Resonance Superfluidity in a Quantum Degenerate Fermi Gas. Phys. Rev. Lett. 2001, 87, 120406. [Google Scholar] [CrossRef] [PubMed]
- Timmermans, E.; Tommasini, P.; Hussein, M.; Kerman, A. Feshbach Resonances in Atomic Bose-Einstein Condensates. Phys. Rep. 1999, 315, 199–230. [Google Scholar] [CrossRef]
- Pitaevskii, L.; Stringari, S. Bose-Einstein Condensation; International Series of Monographs on Physics; Clarendon Press: Oxford, UK, 2003. [Google Scholar]
- Zwerger, W. The BCS-BEC Crossover and the Unitary Fermi Gas; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Randeria, M.; Taylor, E. Crossover from Bardeen-Cooper-Schrieffer to Bose-Einstein Condensation and the Unitary Fermi Gas. Annu. Rev. Condens. Matter Phys. 2014, 5, 209–232. [Google Scholar] [CrossRef]
- Chen, Q.; Stajic, J.; Tan, S.; Levin, K. BCS–BEC crossover: From high temperature superconductors to ultracold superfluids. Phys. Rep. 2005, 412, 1–88. [Google Scholar] [CrossRef]
- Nozieres, P.; Schmitt-Rink, S. Bose condensation in an attractive fermion gas: From weak to strong coupling superconductivity. J. Low Temp. Phys. 1985, 59, 195–211. [Google Scholar] [CrossRef]
- Iadonisi, G.; Schrieffer, J.R.; Chiofalo, M.L. (Eds.) Models and Phenomenology for Conventional and High-Temperature Superconductivity; Proceedings of the International School “Enrico Fermi”, Course CXXXVI; IOS Press: Bologna, Italy, 1998. [Google Scholar]
- Eagles, D.M. Possible Pairing without Superconductivity at Low Carrier Concentrations in Bulk and Thin-Film Superconducting Semiconductors. Phys. Rev. 1969, 186, 456–463. [Google Scholar] [CrossRef]
- Leggett, A.J. Diatomic Molecules and Cooper Pairs. In Proceedings of the Modern Trends in the Theory of Condensed Matter, Karpacz, Poland, 19 February–3 March 1979; Pkekalski, A., Przystawa, J.A., Eds.; Springer: Berlin/Heidelberg, Germany, 1980; pp. 13–27. [Google Scholar]
- Uemura, Y.J.; Le, L.P.; Luke, G.M.; Sternlieb, B.J.; Wu, W.D.; Brewer, J.H.; Riseman, T.M.; Seaman, C.L.; Maple, M.B.; Ishikawa, M.; et al. Basic Similarities among Cuprate, Bismuthate, Organic, Chevrel-Phase, and Heavy-Fermion Superconductors Shown by Penetration-Depth Measurements. Phys. Rev. Lett. 1991, 66, 2665–2668. [Google Scholar] [CrossRef] [PubMed]
- Pistolesi, F.; Strinati, G.C. Evolution from BCS superconductivity to Bose condensation: Role of the Parameter kFξ. Phys. Rev. B 1994, 49, 6356–6359. [Google Scholar] [CrossRef] [PubMed]
- Gezerlis, A.; Pethick, C.J.; Schwenk, A. Pairing and superfluidity of nucleaons in neutron stars. arXiv 2014, arXiv:1406.6109. [Google Scholar]
- Schwenk, A.; Pethick, C.J. Resonant Fermi Gases with a Large Effective Range. Phys. Rev. Lett. 2005, 95, 160401. [Google Scholar] [CrossRef] [PubMed]
- Regal, C.A.; Jin, D.S. Measurement of Positive and Negative Scattering Lengths in a Fermi Gas of Atoms. Phys. Rev. Lett. 2003, 90, 230404. [Google Scholar] [CrossRef] [PubMed]
- Chiofalo, M.L.; Kokkelmans, S.J.J.M.F.; Milstein, J.N.; Holland, M.J. Signatures of Resonance Superfluidity in a Quantum Fermi Gas. Phys. Rev. Lett. 2002, 88, 090402. [Google Scholar] [CrossRef] [PubMed]
- Kokkelmans, S.J.J.M.F.; Milstein, J.N.; Chiofalo, M.L.; Walser, R.; Holland, M.J. Resonance Superfluidity: Renormalization of Resonance Scattering Theory. Phys. Rev. A 2002, 65, 053617. [Google Scholar] [CrossRef]
- Regal, C.A.; Ticknor, C.; Bohn, J.L.; Jin, D.S. Creation of ultracold molecules from a Fermi gas of atoms. Nature 2003, 424, 47–50. [Google Scholar] [CrossRef]
- Regal, C.A.; Greiner, M.; Jin, D.S. Observation of Resonance Condensation of Fermionic Atom Pairs. Phys. Rev. Lett. 2004, 92, 040403. [Google Scholar] [CrossRef]
- Zwierlein, M.W.; Abo-Shaeer, J.R.; Schirotzek, A.; Schunck, C.H.; Ketterle, W. Vortices and superfluidity in a strongly interacting Fermi gas. Nature 2005, 435, 1047–1051. [Google Scholar] [CrossRef] [PubMed]
- Adhikari, S.K.; Salasnich, L. Vortex lattice in the crossover of a Bose gas from weak coupling to unitarity. Sci. Rep. 2018, 8, 8825. [Google Scholar] [CrossRef] [PubMed]
- Hoffmann, D.K.; Singh, V.P.; Paintner, T.; Jäger, M.; Limmer, W.; Mathey, L.; Hecker Denschlag, J. Second sound in the crossover from the Bose-Einstein condensate to the Bardeen-Cooper-Schrieffer superfluid. Nat. Commun. 2021, 12, 7074. [Google Scholar] [CrossRef] [PubMed]
- He, M.; Lv, C.; Lin, H.Q.; Zhou, Q. Universal relations for ultracold reactive molecules. Sci. Adv. 2020, 6, eabd4699. [Google Scholar] [CrossRef]
- Gao, X.Y.; Blume, D.; Yan, Y. Temperature-Dependent Contact of Weakly Interacting Single-Component Fermi Gases and Loss Rate of Degenerate Polar Molecules. Phys. Rev. Lett. 2023, 131, 043401. [Google Scholar] [CrossRef] [PubMed]
- Tobias, W.G.; Matsuda, K.; Li, J.R.; Miller, C.; Carroll, A.N.; Bilitewski, T.; Rey, A.M.; Ye, J. Reactions between layer-resolved molecules mediated by dipolar spin exchange. Science 2022, 375, 1299–1303. [Google Scholar] [CrossRef] [PubMed]
- Li, J.R.; Matsuda, K.; Miller, C.; Carroll, A.N.; Tobias, W.G.; Higgins, J.S.; Ye, J. Tunable itinerant spin dynamics with polar molecules. Nature 2023, 614, 70–74. [Google Scholar] [CrossRef] [PubMed]
- Hartke, T.; Oreg, B.; Turnbaugh, C.; Jia, N.; Zwierlein, M. Direct observation of nonlocal fermion pairing in an attractive Fermi-Hubbard gas. Science 2023, 381, 82–86. [Google Scholar] [CrossRef] [PubMed]
- Xu, M.; Kendrick, L.H.; Kale, A.; Gang, Y.; Ji, G.; Scalettar, R.T.; Lebrat, M.; Greiner, M. Frustration- and doping-induced magnetism in a Fermi–Hubbard simulator. Nature 2023, 620, 971–976. [Google Scholar] [CrossRef] [PubMed]
- Bourdel, T.; Khaykovich, L.; Cubizolles, J.; Zhang, J.; Chevy, F.; Teichmann, M.; Tarruell, L.; Kokkelmans, S.J.J.M.; Salomon, C. Experimental Study of the BEC-BCS Crossover Region in Lithium 6. Phys. Rev. Lett. 2004, 93, 050401. [Google Scholar] [CrossRef] [PubMed]
- Jochim, S.; Bartenstein, M.; Altmeyer, A.; Hendl, G.; Riedl, S.; Chin, C.; Denschlag, J.H.; Grimm, R. Bose-Einstein condensation of molecules. Science 2003, 302, 2101. [Google Scholar] [CrossRef] [PubMed]
- Bonetti, P.M.; Chiofalo, M.L. Local-field Theory of the BCS-BEC Crossover. arXiv 2019, arXiv:1908.10648. [Google Scholar]
- Chen, Q.; Kosztin, I.; Jankó, B.; Levin, K. Phase separation and vortex states in rotating trapped Bose-Einstein condensates. Phys. Rev. Lett. 1998, 81, 4708. [Google Scholar] [CrossRef]
- Pieri, P.; Pisani, L.; Strinati, G.C. BCS-BEC Crossover at Finite Temperature in the Broken-Symmetry Phase. Phys. Rev. B 2004, 70, 094508. [Google Scholar] [CrossRef]
- Perali, A.; Pieri, P.; Pisani, L.; Strinati, G.C. Pseudogap, superfluidity, and BCS-BEC crossover in a trapped Fermi gas. Phys. Rev. Lett. 2004, 92, 220404. [Google Scholar] [CrossRef] [PubMed]
- Haussmann, R.; Rantner, W.; Cerrito, S.; Zwerger, W. Thermodynamics of the BCS-BEC crossover. Phys. Rev. A 2007, 75, 023610. [Google Scholar] [CrossRef]
- Chiofalo, M.L.; Giorgini, S.; Holland, M. Released Momentum Distribution of a Fermi Gas in the BCS-BEC Crossover. Phys. Rev. Lett. 2006, 97, 070404. [Google Scholar] [CrossRef] [PubMed]
- Astrakharchik, G.E.; Boronat, J.; Casulleras, J.; Giorgini, S. Equation of State of a Fermi Gas in the BEC-BCS Crossover: A Quantum Monte Carlo Study. Phys. Rev. Lett. 2004, 93, 200404. [Google Scholar] [CrossRef]
- Bulgac, A.; Drut, J.E.; Magierski, P. Spin 1/2 Fermions in the Unitary Regime: A Superfluid of a New Type. Phys. Rev. Lett. 2006, 96, 090404. [Google Scholar] [CrossRef] [PubMed]
- Burovski, E.; Prokofév, N.; Svistunov, B.; Troyer, M. Critical Temperature and Thermodynamics of Attractive Fermions at Unitarity. Phys. Rev. Lett. 2006, 96, 160402. [Google Scholar] [CrossRef] [PubMed]
- Akkineni, V.K.; Ceperley, D.M.; Trivedi, N. Pairing Symmetry in Dilute Fermi Gases with Attractive Interactions. Phys. Rev. B 2007, 76, 165116. [Google Scholar] [CrossRef]
- Mazurenko, A.; Chiu, C.S.; Ji, G.; Parsons, M.F.; Kanász-Nagy, M.; Schmidt, R.; Grusdt, F.; Demler, E.; Greif, D.; Greiner, M. A cold-atom Fermi–Hubbard antiferromagnet. Nature 2017, 545, 462–466. [Google Scholar] [CrossRef] [PubMed]
- Lercher, A.; Takekoshi, T.; Debatin, M.; Schuster, B.; Rameshan, R.; Ferlaino, F.; Grimm, R.; Nägerl, H.C. Production of a dual-species Bose-Einstein condensate of Rb and Cs atoms. Eur. Phys. J. D 2011, 65, 3–9. [Google Scholar] [CrossRef]
- Diener, R.B.; Ho, T.L. The Condition for Universality at Resonance and Direct Measurement of Pair Wavefunctions Using rf Spectroscopy. arXi 2004, arXiv:cond-mat/0405174v2. [Google Scholar]
- Forbes, M.M.; Gandolfi, S.; Gezerlis, A. Resonantly Interacting Fermions in a Box. Phys. Rev. Lett. 2011, 106, 235303. [Google Scholar] [CrossRef] [PubMed]
- De Palo, S.; Chiofalo, M.; Holland, M.; Kokkelmans, S. Resonance effects on the crossover of bosonic to fermionic superfluidity. Phys. Lett. A 2004, 327, 490–499. [Google Scholar] [CrossRef][Green Version]
- Friedberg, R.; Lee, T.D. Gap Energy and Long-Range Order in the Boson-Fermion Model of Superconductivity. Phys. Rev. B 1989, 40, 6745–6762. [Google Scholar] [CrossRef]
- Ranninger, J.; Robin, J. The Boson-Fermion Model of High-Tc Superconductivity. Doping Dependence. Phys. C Supercond. 1995, 253, 279–291. [Google Scholar] [CrossRef]
- Ohashi, Y.; Griffin, A. Superfluidity and Collective Modes in a Uniform Gas of Fermi Atoms with a Feshbach Resonance. Phys. Rev. A 2003, 67, 063612. [Google Scholar] [CrossRef]
- Ohashi, Y.; Griffin, A. BCS-BEC Crossover in a Gas of Fermi Atoms with a Feshbach Resonance. Phys. Rev. Lett. 2002, 89, 130402. [Google Scholar] [CrossRef] [PubMed]
- Manini, N.; Salasnich, L. Bulk and collective properties of a dilute Fermi gas in the BCS-BEC crossover. Phys. Rev. A 2005, 71, 033625. [Google Scholar] [CrossRef]
- Stajic, J.; Milstein, J.; Chen, Q.; Chiofalo, M.; Holland, M.; Levin, K. Nature of superfluidity in ultracold Fermi gases near Feshbach resonances. Phys. Rev. A 2004, 69, 063610. [Google Scholar] [CrossRef]
- Liu, X.J.; Hu, H. Self-consistent theory of atomic Fermi gases with a Feshbach resonance at the superfluid transition. Phys. Rev. A 2005, 72, 063613. [Google Scholar] [CrossRef]
- Floerchinger, S.; Scherer, M.; Diehl, S.; Wetterich, C. Particle-hole fluctuations in BCS-BEC crossover. Phys. Rev. B 2008, 78, 174528. [Google Scholar] [CrossRef]
- Diehl, S.; Gies, H.; Pawlowski, J.M.; Wetterich, C. Renormalization flow of Wilsonian effective actions and Translationally invariant nonperturbative Functionals. Phys. Rev. A 2007, 76, 053627. [Google Scholar] [CrossRef]
- Diehl, S.; Gies, H.; Pawlowski, J.M.; Wetterich, C. Universality in Bose-Einstein condensates: Particle correlations beyond Landau and Bogoliubov. Phys. Rev. A 2007, 76, 021602(R). [Google Scholar] [CrossRef]
- Singwi, K.S.; Tosi, M.P.; Land, R.H.; Sjölander, A. Electron Correlations at Metallic Densities. Phys. Rev. 1968, 176, 589–599. [Google Scholar] [CrossRef]
- Hasegawa, T.; Shmizu, M. Electron Correlations at Metallic Densities, II. Quantum Mechanical Expression of Dielectric Function with Wigner Distribution Function. J. Phys. Soc. Jpn. 1975, 38, 965–973. [Google Scholar] [CrossRef]
- Giuliani, G.; Vignale, G. Quantum Theory of the Electron Liquid; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Musolino, S.; Chiofalo, M.L. Correlation Length and Universality in the BCS-BEC Crossover for Energy-Dependent Resonance Superfluidity. Eur. Phys. J. Spec. Top. 2017, 226, 2793–2803. [Google Scholar] [CrossRef]
- Giambastiani, D.; Barsanti, M.; Chiofalo, M.L. Interaction-range effects and universality in the BCS-BEC crossover of spin-orbit–coupled Fermi gases. Europhys. Lett. 2018, 123, 66001. [Google Scholar] [CrossRef]
- Grüner, G. The dynamics of charge-density waves. Rev. Mod. Phys. 1988, 60, 1129–1181. [Google Scholar] [CrossRef]
- Mo, Y.; Turner, K.; Szlufarska, I. Friction Laws at the Nanoscale. Nature 2009, 457, 1116–1119. [Google Scholar] [CrossRef] [PubMed]
- Braun, O.M.; Kivshar, Y.S. The Frenkel-Kontorova Model: Concepts, Methods, and Applications; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Braun, O.; Naumovets, A. Nanotribology: Microscopic mechanisms of friction. Surf. Sci. Rep. 2006, 60, 79–158. [Google Scholar] [CrossRef]
- Bormuth, V.; Varga, V.; Howard, J.; Schäffer, E. Protein friction limits diffusive and directed movements of kinesin motors on microtubules. Science 2009, 325, 870–873. [Google Scholar] [CrossRef] [PubMed]
- Chiang, C.K.; Fincher, C.R.; Park, Y.W.; Heeger, A.J.; Shirakawa, H.; Louis, E.J.; Gau, S.C.; MacDiarmid, A.G. Electrical Conductivity in Doped Polyacetylene. Phys. Rev. Lett. 1977, 39, 1098–1101. [Google Scholar] [CrossRef]
- Bak, P. Commensurate phases, incommensurate phases and the devil’s staircase. Rep. Prog. Phys. 1982, 45, 587. [Google Scholar] [CrossRef]
- Kürten, K.; Krattenthaler, C. Multistability and Multi 2π-kinks in the Frenkel-Kontorova model: An Application to Arrays of Josephson Junctions. In Condensed Matter Theories, Part E; World Scientific: Singapore, 2012; pp. 290–300. [Google Scholar]
- Haller, E.; Hart, R.; Mark, M.J.; Danzl, J.; Reichsöllner, L.; Gustavsson, M.; Dalmonte, M.; Pupillo, G.; Nägerl, H.C. Pinning quantum phase transition for a Luttinger liquid of strongly interacting bosons. Nature 2010, 466, 597. [Google Scholar] [CrossRef]
- Orignac, E.; Citro, R.; Dio, M.D.; Palo, S.D.; Chiofalo, M.L. Incommensurate phases of a bosonic two-leg ladder under a flux. New J. Phys. 2016, 18, 055017. [Google Scholar] [CrossRef]
- Consoli, L.; Knops, H.J.F.; Fasolino, A. Onset of Sliding Friction in Incommensurate Systems. Phys. Rev. Lett. 2000, 85, 302–305. [Google Scholar] [CrossRef] [PubMed]
- Aubry, S. The twist map, the extended Frenkel-Kontorova model and the devil’s staircase. Phys. D 1983, 7, 240–258. [Google Scholar] [CrossRef][Green Version]
- Frenkel, Y.; Kontorova, T.K. On the theory of plastic deformation and twinning. II. Zh. Eksp. Teor. Fiz. 1938, 8, 1340. [Google Scholar]
- Frank, F.C.; Van der Merwe, J.H. One-dimensional dislocations. I. Static theory. Proc. R. Soc. 1949, 198, 205. [Google Scholar] [CrossRef]
- Sharma, S.R.; Bergersen, B.; Joos, B. Aubry transition in a finite modulated chain. Phys. Rev. B 1984, 29, 6335–6340. [Google Scholar] [CrossRef]
- Borgonovi, F.; Guarneri, I.; Shepelyansky, D. Destruction of classical cantori in the quantum Frenkel-Kontorova model. Z. Phys.B. 1990, 79, 133. [Google Scholar] [CrossRef][Green Version]
- Hu, B.; Li, B. Quantum Frenkel-Kontorova model. Phys. A 2000, 288, 81–97. [Google Scholar] [CrossRef]
- Zhirov, O.V.; Casati, G.; Shepelyansky, D.L. Quantum phase transition in the Frenkel-Kontorova chain: From pinned instanton glass to sliding phonon gas. Phys. Rev. E 2003, 67, 056209. [Google Scholar] [CrossRef] [PubMed]
- Ma, Y.; Wang, J.; Xu, X.; Wei, Q.; Kais, S. Quantum Phase Transition in One-Dimensional Commensurate Frenkel–Kontorova Model. J. Phys. Soc. Jpn. 2014, 83, 124603. [Google Scholar] [CrossRef][Green Version]
- Krajewski, F.R.; Muser, M.H. Quantum dynamics in the highly discrete, commensurate Frenkel-Kontorova model: A path-integral molecular dynamics study. J. Chem. Phys. 2005, 122, 124711. [Google Scholar] [CrossRef]
- Hu, B.; Wang, J.X. Density-matrix renormalization group study of the incommensurate quantum Frenkel-Kontorova model. Phys. Rev. B 2006, 73, 184305. [Google Scholar] [CrossRef]
- Pokrovsky, V.; Virosztek, A. Solitary wave solutions of nonlocal Sine-Gordon equations. J. Phys. C 1983, 16, 4513. [Google Scholar] [CrossRef]
- Braun, O.M.; Kivshar, Y.S.; Zelenskaya, I.I. Kinks in the Frenkel-Kontorova model with long-range interparticle interactions. Phys. Rev. B 1990, 41, 7118–7138. [Google Scholar] [CrossRef] [PubMed]
- Silvi, P.; De Chiara, G.; Calarco, T.; Morigi, G.; Montangero, S. Full characterization of the quantum linear-zigzag transition in atomic chains. Ann. Phys. 2013, 525, 827–832. [Google Scholar] [CrossRef]
- Cormick, C.; Morigi, G. Structural Transitions of Ion Strings in Quantum Potentials. Phys. Rev. Lett. 2012, 109, 053003. [Google Scholar] [CrossRef] [PubMed]
- Gangloff, D.A.; Bylinskii, A.; Vuletić, V. Kinks and nanofriction: Structural phases in few-atom chains. Phys. Rev. Res. 2020, 2, 013380. [Google Scholar] [CrossRef]
- Fogarty, T.; Cormick, C.; Landa, H.; Stojanović, V.M.; Demler, E.; Morigi, G. Nanofriction in Cavity Quantum Electrodynamics. Phys. Rev. Lett. 2015, 115, 233602. [Google Scholar] [CrossRef] [PubMed]
- Bak, P.; Bruinsma, R. One-Dimensional Ising Model and the Complete Devil’s Staircase. Phys. Rev. Lett. 1982, 49, 249–251. [Google Scholar] [CrossRef]
- Gangloff, D.; Bylinskii, A.; Counts, I.; Jhe, W.; Vuletić, V. Velocity tuning of friction with two trapped atoms. Nat. Phys. 2015, 11, 915–919. [Google Scholar] [CrossRef]
- Bylinskii, A.; Gangloff, D.; Vuletić, V. Tuning friction atom-by-atom in an ion-crystal simulator. Science 2015, 348, 1115–1118. [Google Scholar] [CrossRef] [PubMed]
- Benassi, A.; Vanossi, A.; Tosatti, E. Nanofriction in cold ion traps. Nat. Commun. 2011, 2, 236. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Mandelli, D.; Vanossi, A.; Tosatti, E. Stick-slip nanofriction in trapped cold ion chains. Phys. Rev. B 2013, 87, 195418. [Google Scholar] [CrossRef]
- Pruttivarasin, T.; Ramm, M.; Talukdar, I.; Kreuter, A.; Häffner, H. Trapped ions in optical lattices for probing oscillator chain models. New J. Phys. 2011, 13, 075012. [Google Scholar] [CrossRef][Green Version]
- Kiethe, J.; Nigmatullin, R.; Kalincev, D.; Schmirander, T.; Mehlstäubler, T.E. Probing nanofriction and Aubry-type signatures in a finite self-organized system. Nat. Commun. 2017, 8, 15364. [Google Scholar] [CrossRef]
- Bonetti, P.M.; Rucci, A.; Chiofalo, M.L.; Vuletić, V. Quantum effects in the Aubry transition. Phys. Rev. Res. 2021, 3, 013031. [Google Scholar] [CrossRef]
- Tinkham, M. Introduction to Superconductivity; McGraw-Hill: New York, NY, USA, 1975. [Google Scholar]
- Japaridze, G.I.; Nersesyan, A.A. One-dimensional electron system with attractive interaction in a magnetic field. J. Low Temp. Phys. 1979, 37, 95–110. [Google Scholar] [CrossRef]
- Pokrovsky, V.L.; Talapov, A.L. Ground State, Spectrum, and Phase Diagram of Two-Dimensional Incommensurate Crystals. Phys. Rev. Lett. 1979, 42, 65–67. [Google Scholar] [CrossRef]
- Osterloh, K.; Baig, M.; Santos, L.; Zoller, P.; Lewenstein, M. Cold Atoms in Non-Abelian Gauge Potentials: From the Hofstadter “Moth” to Lattice Gauge Theory. Phys. Rev. Lett. 2005, 95, 010403. [Google Scholar] [CrossRef] [PubMed]
- Ruseckas, J.; Juzeliūnas, G.; Öhberg, P.; Fleischhauer, M. Non-Abelian gauge potentials for ultracold atoms with degenerate dark states. Phys. Rev. Lett. 2005, 95, 010404. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.J.; Jiménez-García, K.; Spielman, I.B. Spin-orbit-coupled Bose-Einstein condensates. Nature 2011, 471, 83–86. [Google Scholar] [CrossRef] [PubMed]
- Mermin, N.D.; Wagner, H. Absence of Ferromagnetism or Antiferromagnetism in One- or Two-Dimensional Isotropic Heisenberg Models. Phys. Rev. Lett. 1966, 17, 1133–1136. [Google Scholar] [CrossRef]
- Hohenberg, P.C. Existence of Long-Range Order in One and Two Dimensions. Phys. Rev. 1967, 158, 383–386. [Google Scholar] [CrossRef]
- Kardar, M. Josephson-junction ladders and quantum fluctuations. Phys. Rev. B 1986, 33, 3125–3128. [Google Scholar] [CrossRef] [PubMed]
- Orignac, E.; Giamarchi, T. Meissner effect in a bosonic ladder. Phys. Rev. B 2001, 64, 144515. [Google Scholar] [CrossRef]
- Cha, M.C.; Shin, J.G. Two peaks in the momentum distribution of bosons in a weakly frustrated two-leg optical ladder. Phys. Rev. 2011, 83, 055602. [Google Scholar] [CrossRef]
- Di Dio, M.; De Palo, S.; Orignac, E.; Citro, R.; Chiofalo, M.L. Persisting Meissner state and incommensurate phases of hard-core boson ladders in a flux. Phys. Rev. B 2015, 92, 060506. [Google Scholar] [CrossRef]
- Granato, E. Phase transitions in Josephson-junction ladders in a magnetic field. Phys. Rev. B 1990, 42, 4797–4799. [Google Scholar] [CrossRef]
- Nishiyama, Y. Finite-size-scaling analyses of the chiral ordert in the Josephson-junction ladder with half a flux quantum per plaquette. Eur. Phys. J. -Condens. Matter Complex Syst. 2000, 17, 295–299. [Google Scholar] [CrossRef]
- Dhar, A.; Maji, M.; Mishra, T.; Pai, R.V.; Mukerjee, S.; Paramekanti, A. Bose-Hubbard model in a strong effective magnetic field: Emergence of a chiral Mott insulator ground state. Phys. Rev. A 2012, 85, 041602. [Google Scholar] [CrossRef]
- Dhar, A.; Mishra, T.; Maji, M.; Pai, R.V.; Mukerjee, S.; Paramekanti, A. Chiral Mott insulator with staggered loop currents in the fully frustrated Bose-Hubbard model. Phys. Rev. B 2013, 87, 174501. [Google Scholar] [CrossRef]
- Petrescu, A.; Le Hur, K. Bosonic Mott Insulator with Meissner Currents. Phys. Rev. Lett. 2013, 111, 150601. [Google Scholar] [CrossRef] [PubMed]
- Tokuno, A.; Georges, A. Ground states of a Bose–Hubbard ladder in an artificial magnetic field: Field-theoretical approach. New J. Phys. 2014, 16, 073005. [Google Scholar] [CrossRef]
- Petrescu, A.; Le Hur, K. Chiral Mott insulators, Meissner effect, and Laughlin states in quantum ladders. Phys. Rev. B 2015, 91, 054520. [Google Scholar] [CrossRef]
- Zhao, J.; Hu, S.; Chang, J.; Zhang, P.; Wang, X. Ferromagnetism in a two-component Bose-Hubbard model with synthetic spin-orbit coupling. Phys. Rev. A 2014, 89, 043611. [Google Scholar] [CrossRef]
- Zhao, J.; Hu, S.; Chang, J.; Zheng, F.; Zhang, P.; Wang, X. Evolution of magnetic structure driven by synthetic spin-orbit coupling in a two-component Bose-Hubbard model. Phys. Rev. B 2014, 90, 085117. [Google Scholar] [CrossRef]
- Xu, Z.; Cole, W.S.; Zhang, S. Mott-superfluid transition for spin-orbit-coupled bosons in one-dimensional optical lattices. Phys. Rev. A 2014, 89, 051604. [Google Scholar] [CrossRef]
- Peotta, S.; Mazza, L.; Vicari, E.; Polini, M.; Fazio, R.; Rossini, D. The XYZ chain with Dzyaloshinsky–Moriya interactions: From spin–orbit-coupled lattice bosons to interacting Kitaev chains. J. Stat. Mech. Theory Exp. 2014, 2014, P09005. [Google Scholar] [CrossRef][Green Version]
- Piraud, M.; Cai, Z.; McCulloch, I.P.; Schollwöck, U. Quantum magnetism of bosons with synthetic gauge fields in one-dimensional optical lattices: A density-matrix renormalization-group study. Phys. Rev. A 2014, 89, 063618. [Google Scholar] [CrossRef]
- Barbiero, L.; Abad, M.; Recati, A. Magnetic phase transition in coherently coupled Bose gases in optical lattices. Phys. Rev. A 2016, 93, 033645. [Google Scholar] [CrossRef]
- Deutsch, J.M. Quantum statistical mechanics in a closed system. Phys. Rev. A 1991, 43, 2046–2049. [Google Scholar] [CrossRef] [PubMed]
- Srednicki, M. Chaos and quantum thermalization. Phys. Rev. E 1994, 50, 888–901. [Google Scholar] [CrossRef] [PubMed]
- Rigol, M.; Dunjko, V.; Olshanii, M. Thermalization and its mechanism for generic isolated quantum systems. Nature 2008, 452, 854. [Google Scholar] [CrossRef] [PubMed]
- Deutsch, J.M. Eigenstate thermalization hypothesis. Rep. Prog. Phys. 2018, 81, 082001. [Google Scholar] [CrossRef] [PubMed]
- Lux, J.; Müller, J.; Mitra, A.; Rosch, A. Hydrodynamic long-time tails after a quantum quench. Phys. Rev. A 2014, 89, 053608. [Google Scholar] [CrossRef]
- Kaufman, A.M.; Tai, M.E.; Lukin, A.; Rispoli, M.; Schittko, R.; Preiss, P.M.; Greiner, M. Quantum thermalization through entanglement in an isolated many-body system. Science 2016, 353, 794–800. [Google Scholar] [CrossRef] [PubMed]
- Kranzl, F.; Lasek, A.; Joshi, M.K.; Kalev, A.; Blatt, R.; Roos, C.F.; Yunger Halpern, N. Experimental Observation of Thermalization with Noncommuting Charges. PRX Quantum 2023, 4, 020318. [Google Scholar] [CrossRef]
- Anderson, P.W. Absence of Diffusion in Certain Random Lattices. Phys. Rev. 1958, 109, 1492–1505. [Google Scholar] [CrossRef]
- Wiersma, D.S.; Bartolini, P.; Lagendijk, A.; Righini, R. Localization of light in a disordered medium. Nature 1997, 390, 671. [Google Scholar] [CrossRef]
- Schwartz, T.; Bartal, G.; Fishman, S.; Segev, M. Transport and Anderson localization in disordered two-dimensional photonic lattices. Nature 2007, 446, 52. [Google Scholar] [CrossRef] [PubMed]
- Lahini, Y.; Avidan, A.; Pozzi, F.; Sorel, M.; Morandotti, R.; Christodoulides, D.N.; Silberberg, Y. Anderson Localization and Nonlinearity in One-Dimensional Disordered Photonic Lattices. Phys. Rev. Lett. 2008, 100, 013906. [Google Scholar] [CrossRef]
- Billy, J.; Josse, V.; Zuo, Z.; Bernard, A.; Hambrecht, B.; Lugan, P.; Clément, D.; Sanchez-Palencia, L.; Bouyer, P.; Aspect, A. Direct observation of Anderson localization of matter waves in a controlled disorder. Nature 2008, 453, 891. [Google Scholar] [CrossRef]
- Kondov, S.S.; McGehee, W.R.; Zirbel, J.J.; DeMarco, B. Three-Dimensional Anderson Localization of Ultracold Matter. Science 2011, 334, 66–68. [Google Scholar] [CrossRef] [PubMed]
- Jendrzejewski, F.; Bernard, A.; Müller, K.; Cheinet, P.; Josse, V.; Piraud, M.; Pezzé, L.; Sanchez-Palencia, L.; Aspect, A.; Bouyer, P. Three-dimensional localization of ultracold atoms in an optical disordered potential. Nat. Phys. 2012, 8, 398–403. [Google Scholar] [CrossRef]
- Žnidarič, M.; Prosen, T.; Prelovšek, P. Many-body localization in the Heisenberg XXZ magnet in a random field. Phys. Rev. B 2008, 77, 064426. [Google Scholar] [CrossRef]
- Bardarson, J.H.; Pollmann, F.; Moore, J.E. Unbounded Growth of Entanglement in Models of Many-Body Localization. Phys. Rev. Lett. 2012, 109, 017202. [Google Scholar] [CrossRef] [PubMed]
- Nandkishore, R.; Huse, D.A. Many-Body Localization and Thermalization in Quantum Statistical Mechanics. Annu. Rev. Condens. Matter Phys. 2015, 6, 15–38. [Google Scholar] [CrossRef]
- Basko, D.; Aleiner, I.; Altshuler, B. Metal–insulator transition in a weakly interacting many-electron system with localized single-particle states. Ann. Phys. 2006, 321, 1126–1205. [Google Scholar] [CrossRef]
- Pal, A.; Huse, D.A. Many-body localization phase transition. Phys. Rev. B 2010, 82, 174411. [Google Scholar] [CrossRef]
- Iyer, S.; Oganesyan, V.; Refael, G.; Huse, D.A. Many-body localization in a quasiperiodic system. Phys. Rev. B 2013, 87, 134202. [Google Scholar] [CrossRef]
- Aubry, S.; André, G. Analyticity breaking and Anderson localization in incommensurate lattices. Ann. Israel Phys. Soc 1980, 3, 18. [Google Scholar]
- Smith, J.; Lee, A.; Richerme, P.; Neyenhuis, B.; Hess, P.W.; Hauke, P.; Heyl, M.; Huse, D.A.; Monroe, C. Many-body localization in a quantum simulator with programmable random disorder. Nat. Phys. 2016, 12, 907–911. [Google Scholar] [CrossRef]
- Bordia, P.; Lüschen, H.P.; Hodgman, S.S.; Schreiber, M.; Bloch, I.; Schneider, U. Coupling Identical one-dimensional Many-Body Localized Systems. Phys. Rev. Lett. 2016, 116, 140401. [Google Scholar] [CrossRef] [PubMed]
- Choi, J.Y.; Hild, S.; Zeiher, J.; Schauß, P.; Rubio-Abadal, A.; Yefsah, T.; Khemani, V.; Huse, D.A.; Bloch, I.; Gross, C. Exploring the many-body localization transition in two dimensions. Science 2016, 352, 1547–1552. [Google Scholar] [CrossRef]
- Van Horssen, M.; Levi, E.; Garrahan, J.P. Dynamics of many-body localization in a translation-invariant quantum glass model. Phys. Rev. B 2015, 92, 100305. [Google Scholar] [CrossRef]
- Turner, C.J.; Michailidis, A.A.; Abanin, D.A.; Serbyn, M.; Papić, Z. Weak ergodicity breaking from quantum many-body scars. Nat. Phys. 2018, 14, 745–749. [Google Scholar] [CrossRef]
- Brenes, M.; Dalmonte, M.; Heyl, M.; Scardicchio, A. Many-Body Localization Dynamics from Gauge Invariance. Phys. Rev. Lett. 2018, 120, 030601. [Google Scholar] [CrossRef] [PubMed]
- Altman, E.; Vosk, R. Universal Dynamics and Renormalization in Many-Body-Localized Systems. Annu. Rev. Condens. Matter Phys. 2015, 6, 383–409. [Google Scholar] [CrossRef]
- Lüschen, H.P.; Bordia, P.; Hodgman, S.S.; Schreiber, M.; Sarkar, S.; Daley, A.J.; Fischer, M.H.; Altman, E.; Bloch, I.; Schneider, U. Signatures of Many-Body Localization in a Controlled Open Quantum System. Phys. Rev. X 2017, 7, 011034. [Google Scholar] [CrossRef]
- Medvedyeva, M.V.; Prosen, T.; Žnidarič, M. Influence of dephasing on many-body localization. Phys. Rev. B 2016, 93, 094205. [Google Scholar] [CrossRef]
- Levi, E.; Heyl, M.; Lesanovsky, I.; Garrahan, J.P. Robustness of Many-Body Localization in the Presence of Dissipation. Phys. Rev. Lett. 2016, 116, 237203. [Google Scholar] [CrossRef] [PubMed]
- Fischer, M.H.; Maksymenko, M.; Altman, E. Dynamics of a Many-Body-Localized System Coupled to a Bath. Phys. Rev. Lett. 2016, 116, 160401. [Google Scholar] [CrossRef] [PubMed]
- Van Nieuwenburg, E.; Malo, J.Y.; Daley, A.; Fischer, M. Dynamics of many-body localization in the presence of particle loss. Quantum Sci. Technol. 2017, 3, 01LT02. [Google Scholar] [CrossRef]
- Serbyn, M.; Papić, Z.; Abanin, D.A. Criterion for Many-Body Localization-Delocalization Phase Transition. Phys. Rev. X 2015, 5, 041047. [Google Scholar] [CrossRef]
- Bañuls, M.C.; Yao, N.Y.; Choi, S.; Lukin, M.D.; Cirac, J.I. Dynamics of quantum information in many-body localized systems. Phys. Rev. B 2017, 96, 174201. [Google Scholar] [CrossRef]
- De Roeck, W.; Huveneers, F.M.C. Stability and instability towards delocalization in many-body localization systems. Phys. Rev. B 2017, 95, 155129. [Google Scholar] [CrossRef]
- Pancotti, N.; Knap, M.; Huse, D.A.; Cirac, J.I.; Bañuls, M.C. Almost conserved operators in nearly many-body localized systems. Phys. Rev. B 2018, 97, 094206. [Google Scholar] [CrossRef]
- Li, X.; Ganeshan, S.; Pixley, J.H.; Das Sarma, S. Many-Body Localization and Quantum Nonergodicity in a Model with a Single-Particle Mobility Edge. Phys. Rev. Lett. 2015, 115, 186601. [Google Scholar] [CrossRef]
- Agarwal, K.; Altman, E.; Demler, E.; Gopalakrishnan, S.; Huse, D.A.; Knap, M. Rare-region effects and dynamics near the many-body localization transition. Ann. Phys. 2017, 529, 1600326. [Google Scholar] [CrossRef]
- Rubio-Abadal, A.; Choi, J.y.; Zeiher, J.; Hollerith, S.; Rui, J.; Bloch, I.; Gross, C. Many-Body Delocalization in the Presence of a Quantum Bath. Phys. Rev. X 2019, 9, 041014. [Google Scholar] [CrossRef]
- Léonard, J.; Kim, S.; Rispoli, M.; Lukin, A.; Schittko, R.; Kwan, J.; Demler, E.; Sels, D.; Greiner, M. Probing the onset of quantum avalanches in a many-body localized system. Nat. Phys. 2023, 19, 481–485. [Google Scholar] [CrossRef]
- Rispoli, M.; Lukin, A.; Schittko, R.; Kim, S.; Tai, M.E.; Léonard, J.; Greiner, M. Quantum critical behaviour at the many-body localization transition. Nature 2019, 573, 385–389. [Google Scholar] [CrossRef]
- Huse, D.A.; Nandkishore, R.; Oganesyan, V.; Pal, A.; Sondhi, S.L. Localization-protected quantum order. Phys. Rev. B 2013, 88, 014206. [Google Scholar] [CrossRef]
- Bahri, Y.; Vosk, R.; Altman, E.; Vishwanath, A. Localization and topology protected quantum coherence at the edge of hot matter. Nat. Commun. 2015, 6, 7341. [Google Scholar] [CrossRef] [PubMed]
- Guo, L.; Liang, P. Condensed matter physics in time crystals. New J. Phys. 2020, 22, 075003. [Google Scholar] [CrossRef]
- Serbyn, M.; Abanin, D.A.; Papić, Z. Quantum many-body scars and weak breaking of ergodicity. Nat. Phys. 2021, 17, 675–685. [Google Scholar] [CrossRef]
- Bluvstein, D.; Omran, A.; Levine, H.; Keesling, A.; Semeghini, G.; Ebadi, S.; Wang, T.T.; Michailidis, A.A.; Maskara, N.; Ho, W.W.; et al. Controlling quantum many-body dynamics in driven Rydberg atom arrays. Science 2021, 371, 1355–1359. [Google Scholar] [CrossRef] [PubMed]
- Chandran, A.; Iadecola, T.; Khemani, V.; Moessner, R. Quantum Many-Body Scars: A Quasiparticle Perspective. Annu. Rev. Condens. Matter Phys. 2023, 14, 443–469. [Google Scholar] [CrossRef]
- Lewenstein, M.; Sanpera, A.; Ahufinger, V.; Damski, B.; De Sen, A.; Sen, U. Ultracold atomic gases in optical lattices: Mimicking condensed matter physics and beyond. Adv. Phys. 2007, 56, 243–379. [Google Scholar] [CrossRef]
- Weitenberg, C.; Simonet, J. Tailoring quantum gases by Floquet engineering. Nat. Phys. 2021, 17, 1342–1348. [Google Scholar] [CrossRef]
- Rahav, S.; Gilary, I.; Fishman, S. Effective Hamiltonians for periodically driven systems. Phys. Rev. A 2003, 68, 013820. [Google Scholar] [CrossRef]
- Goldman, N.; Dalibard, J. Periodically Driven Quantum Systems: Effective Hamiltonians and Engineered Gauge Fields. Phys. Rev. X 2014, 4, 031027. [Google Scholar] [CrossRef]
- Aoki, H.; Tsuji, N.; Eckstein, M.; Kollar, M.; Oka, T.; Werner, P. Nonequilibrium dynamical mean-field theory and its applications. Rev. Mod. Phys. 2014, 86, 779–837. [Google Scholar] [CrossRef]
- Sørensen, A.S.; Demler, E.; Lukin, M.D. Fractional Quantum Hall States of Atoms in Optical Lattices. Phys. Rev. Lett. 2005, 94, 086803. [Google Scholar] [CrossRef] [PubMed]
- Eckardt, A.; Hauke, P.; Soltan-Panahi, P.; Becker, C.; Sengstock, K.; Lewenstein, M. Frustrated quantum antiferromagnetism with ultracold bosons in a triangular lattice. Europhys. Lett. 2010, 89, 10010. [Google Scholar] [CrossRef]
- Creffield, C.E.; Sols, F. Directed transport in driven optical lattices by gauge generation. Phys. Rev. A 2011, 84, 023630. [Google Scholar] [CrossRef]
- Lim, L.K.; Smith, C.M.; Hemmerich, A. Staggered-Vortex Superfluid of Ultracold Bosons in an Optical Lattice. Phys. Rev. Lett. 2008, 100, 130402. [Google Scholar] [CrossRef] [PubMed]
- Jiang, L.; Kitagawa, T.; Alicea, J.; Akhmerov, A.R.; Pekker, D.; Refael, G.; Cirac, J.I.; Demler, E.; Lukin, M.D.; Zoller, P. Majorana Fermions in Equilibrium and in Driven Cold-Atom Quantum Wires. Phys. Rev. Lett. 2011, 106, 220402. [Google Scholar] [CrossRef] [PubMed]
- Hauke, P.; Tieleman, O.; Celi, A.; Ölschläger, C.; Simonet, J.; Struck, J.; Weinberg, M.; Windpassinger, P.; Sengstock, K.; Lewenstein, M.; et al. Non-Abelian Gauge Fields and Topological Insulators in Shaken Optical Lattices. Phys. Rev. Lett. 2012, 109, 145301. [Google Scholar] [CrossRef] [PubMed]
- Yagüe Bosch, L.S.; Ehret, T.; Petiziol, F.; Arimondo, E.; Wimberger, S. Shortcut-to-Adiabatic Controlled-Phase Gate in Rydberg Atoms. Ann. Phys. 2023, 535, 2300275. [Google Scholar] [CrossRef]
- Landi, G.T.; Poletti, D.; Schaller, G. Nonequilibrium boundary-driven quantum systems: Models, methods, and properties. Rev. Mod. Phys. 2022, 94, 045006. [Google Scholar] [CrossRef]
- Sato, S.A.; Giovannini, U.D.; Aeschlimann, S.; Gierz, I.; Hübener, H.; Rubio, A. Floquet states in dissipative open quantum systems. J. Phys. At. Mol. Opt. Phys. 2020, 53, 225601. [Google Scholar] [CrossRef]
- Mori, T. Floquet States in Open Quantum Systems. Annu. Rev. Condens. Matter Phys. 2023, 14, 35–56. [Google Scholar] [CrossRef]
- Berdanier, W.; Kolodrubetz, M.; Vasseur, R.; Moore, J.E. Floquet Dynamics of Boundary-Driven Systems at Criticality. Phys. Rev. Lett. 2017, 118, 260602. [Google Scholar] [CrossRef] [PubMed]
- Li, N.; Ren, J.; Wang, L.; Zhang, G.; Hänggi, P.; Li, B. Colloquium: Phononics: Manipulating heat flow with electronic analogs and beyond. Rev. Mod. Phys. 2012, 84, 1045–1066. [Google Scholar] [CrossRef]
- Olaya-Castro, A.; Lee, C.F.; Olsen, F.F.; Johnson, N.F. Efficiency of energy transfer in a light-harvesting system under quantum coherence. Phys. Rev. B 2008, 78, 085115. [Google Scholar] [CrossRef]
- Plenio, M.B.; Huelga, S.F. Dephasing-assisted transport: Quantum networks and biomolecules. New J. Phys. 2008, 10, 113019. [Google Scholar] [CrossRef]
- Itano, W.M. Perspectives on the quantum Zeno paradox. arXiv 2006, arXiv:quant-ph/quant-ph/0612187. [Google Scholar]
- Bushev, P.; Rotter, D.; Wilson, A.; Dubin, F.; Becher, C.; Eschner, J.; Blatt, R.; Steixner, V.; Rabl, P.; Zoller, P. Feedback Cooling of a Single Trapped Ion. Phys. Rev. Lett. 2006, 96, 043003. [Google Scholar] [CrossRef] [PubMed]
- Maunz, P.; Puppe, T.; Schuster, I.; Syassen, N.; Pinkse, P.W.H.; Rempe, G. Cavity cooling of a single atom. Nature 2004, 428, 50–52. [Google Scholar] [CrossRef] [PubMed]
- Mazzucchi, G.; Caballero-Benitez, S.F.; Ivanov, D.A.; Mekhov, I.B. Quantum optical feedback control for creating strong correlations in many-body systems. Optica 2016, 3, 1213–1219. [Google Scholar] [CrossRef]
- Ivanov, D.A.; Ivanova, T.Y.; Caballero-Benitez, S.F.; Mekhov, I.B. Feedback-Induced Quantum Phase Transitions Using Weak Measurements. Phys. Rev. Lett. 2020, 124, 010603. [Google Scholar] [CrossRef] [PubMed]
- Young, J.T.; Gorshkov, A.V.; Spielman, I.B. Feedback-stabilized dynamical steady states in the Bose-Hubbard model. Phys. Rev. Res. 2021, 3, 043075. [Google Scholar] [CrossRef]
- Ostermann, S.; Piazza, F.; Ritsch, H. Spontaneous Crystallization of Light and Ultracold Atoms. Phys. Rev. X 2016, 6, 021026. [Google Scholar] [CrossRef]
- Yamaguchi, E.P.; Hurst, H.M.; Spielman, I.B. Feedback-cooled Bose-Einstein condensation: Near and far from equilibrium. Phys. Rev. A 2023, 107, 063306. [Google Scholar] [CrossRef]
- Koch, C.P. Controlling open quantum systems: Tools, achievements, and limitations. J. Phys. Condens. Matter 2016, 28, 213001. [Google Scholar] [CrossRef] [PubMed]
- Caballero-Benitez, S.F.; Mazzucchi, G.; Mekhov, I.B. Quantum simulators based on the global collective light-matter interaction. Phys. Rev. A 2016, 93, 063632. [Google Scholar] [CrossRef]
- Zhang, J.; Xi Liu, Y.; Wu, R.B.; Jacobs, K.; Nori, F. Quantum feedback: Theory, experiments, and applications. Phys. Rep. 2017, 679, 1–60. [Google Scholar] [CrossRef]
- Polkovnikov, A.; Sengupta, K.; Silva, A.; Vengalattore, M. Colloquium: Nonequilibrium dynamics of closed interacting quantum systems. Rev. Mod. Phys. 2011, 83, 863–883. [Google Scholar] [CrossRef]
- Heyl, M. Dynamical quantum phase transitions: A review. Rep. Prog. Phys. 2018, 81, 054001. [Google Scholar] [CrossRef] [PubMed]
- Hohenberg, P.C.; Halperin, B.I. Theory of dynamic critical phenomena. Rev. Mod. Phys. 1977, 49, 435–479. [Google Scholar] [CrossRef]
- Albert, V.V.; Jiang, L. Symmetries and conserved quantities in Lindblad master equations. Phys. Rev. A 2014, 89, 022118. [Google Scholar] [CrossRef]
- Minganti, F.; Biella, A.; Bartolo, N.; Ciuti, C. Spectral theory of Liouvillians for dissipative phase transitions. Phys. Rev. A 2018, 98, 042118. [Google Scholar] [CrossRef]
- Jurcevic, P.; Shen, H.; Hauke, P.; Maier, C.; Brydges, T.; Hempel, C.; Lanyon, B.P.; Heyl, M.; Blatt, R.; Roos, C.F. Direct Observation of Dynamical Quantum Phase Transitions in an Interacting Many-Body System. Phys. Rev. Lett. 2017, 119, 080501. [Google Scholar] [CrossRef] [PubMed]
- Fläschner, N.; Vogel, D.; Tarnowski, M.; Rem, B.S.; Lühmann, D.S.; Heyl, M.; Budich, J.C.; Mathey, L.; Sengstock, K.; Weitenberg, C. Observation of dynamical vortices after quenches in a system with topology. Nat. Phys. 2018, 14, 265–268. [Google Scholar] [CrossRef]
- Alberton, O.; Buchhold, M.; Diehl, S. Entanglement Transition in a Monitored Free-Fermion Chain: From Extended Criticality to Area Law. Phys. Rev. Lett. 2021, 126, 170602. [Google Scholar] [CrossRef] [PubMed]
- Gullans, M.J.; Huse, D.A. Scalable Probes of Measurement-Induced Criticality. Phys. Rev. Lett. 2020, 125, 070606. [Google Scholar] [CrossRef] [PubMed]
- Gullans, M.J.; Huse, D.A. Dynamical Purification Phase Transition Induced by Quantum Measurements. Phys. Rev. X 2020, 10, 041020. [Google Scholar] [CrossRef]
- Choi, S.; Bao, Y.; Qi, X.L.; Altman, E. Quantum Error Correction in Scrambling Dynamics and Measurement-Induced Phase Transition. Phys. Rev. Lett. 2020, 125, 030505. [Google Scholar] [CrossRef] [PubMed]
- Kuriyattil, S.; Hashizume, T.; Bentsen, G.; Daley, A.J. Onset of Scrambling as a Dynamical Transition in Tunable-Range Quantum Circuits. PRX Quantum 2023, 4, 030325. [Google Scholar] [CrossRef]
- Dine, M.; Kusenko, A. Origin of the matter-antimatter asymmetry. Rev. Mod. Phys. 2003, 76, 1–30. [Google Scholar] [CrossRef]
- Barr, S. A review of CP violation in Atoms. Int. J. Mod. Phys. 1993, 8, 209–236. [Google Scholar] [CrossRef]
- Roussy, T.S.; Caldwell, L.; Wright, T.; Cairncross, W.B.; Shagam, Y.; Ng, K.B.; Schlossberger, N.; Park, S.Y.; Wang, A.; Ye, J.; et al. An improved bound on the electron’s electric dipole moment. Science 2023, 381, 46–50. [Google Scholar] [CrossRef] [PubMed]
- Wallace, P.R. The Band Theory of Graphite. Phys. Rev. 1947, 71, 622–634. [Google Scholar] [CrossRef]
- Castro Neto, A.H.; Guinea, F.; Peres, N.M.R.; Novoselov, K.S.; Geim, A.K. The electronic properties of graphene. Rev. Mod. Phys. 2009, 81, 109–162. [Google Scholar] [CrossRef]
- Katsnelson, M.; Novoselov, K. Graphene: New bridge between condensed matter physics and quantum electrodynamics. Solid State Commun. 2007, 143, 3–13. [Google Scholar] [CrossRef]
- Affleck, I.; Marston, J.B. Large-n limit of the Heisenberg-Hubbard model: Implications for high-Tc superconductors. Phys. Rev. B 1988, 37, 3774–3777. [Google Scholar] [CrossRef]
- Mazzucchi, G.; Lepori, L.; Trombettoni, A. Semimetal–superfluid quantum phase transitions in 2D and 3D lattices with Dirac points. J. Phys. At. Mol. Opt. Phys. 2013, 46, 134014. [Google Scholar] [CrossRef]
- Lepori, L.; Mussardo, G.; Trombettoni, A. (3+1) massive Dirac fermions with ultracold atoms in frustrated cubic optical lattices. Europhys. Lett. 2010, 92, 50003. [Google Scholar] [CrossRef]
- Creutz, M. Quarks, Gluons and Lattices; Cambridge Monographs on Mathemathics; Cambridge University Press: Cambridge, UK, 2023. [Google Scholar]
- Bermudez, A.; Mazza, L.; Rizzi, M.; Goldman, N.; Lewenstein, M.; Martin-Delgado, M.A. Wilson Fermions and Axion Electrodynamics in Optical Lattices. Phys. Rev. Lett. 2010, 105, 190404. [Google Scholar] [CrossRef] [PubMed]
- Peccei, R.D.; Quinn, H.R. CP Conservation in the Presence of Pseudoparticles. Phys. Rev. Lett. 1977, 38, 1440–1443. [Google Scholar] [CrossRef]
- Qi, X.L.; Zhang, S.C. Topological insulators and superconductors. Rev. Mod. Phys. 2011, 83, 1057–1110. [Google Scholar] [CrossRef]
- Casanova, J.; Sabín, C.; León, J.; Egusquiza, I.L.; Gerritsma, R.; Roos, C.F.; García-Ripoll, J.J.; Solano, E. Quantum Simulation of the Majorana Equation and Unphysical Operations. Phys. Rev. X 2011, 1, 021018. [Google Scholar] [CrossRef]
- Lepori, L.; Celi, A.; Trombettoni, A.; Mannarelli, M. Synthesis of Majorana mass terms in low-energy quantum systems. New J. Phys. 2018, 20, 063032. [Google Scholar] [CrossRef]
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. I. Phys. Rev. 1961, 122, 345–358. [Google Scholar] [CrossRef]
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. II. Phys. Rev. 1961, 124, 246–254. [Google Scholar] [CrossRef]
- Cirac, J.I.; Maraner, P.; Pachos, J.K. Cold Atom Simulation of Interacting Relativistic Quantum Field Theories. Phys. Rev. Lett. 2010, 105, 190403. [Google Scholar] [CrossRef] [PubMed]
- Inguscio, M.; Fallani, L. Atomic Physics: Precise Measurements and Ultracold Matter; Oxford University Press: Oxford, UK, 2013. [Google Scholar] [CrossRef]
- Schweikhard, V.; Coddington, I.; Engels, P.; Mogendorff, V.P.; Cornell, E.A. Rapidly Rotating Bose-Einstein Condensates in and near the Lowest Landau Level. Phys. Rev. Lett. 2004, 92, 040404. [Google Scholar] [CrossRef]
- Williams, R.A.; Al-Assam, S.; Foot, C.J. Observation of Vortex Nucleation in a Rotating Two-Dimensional Lattice of Bose-Einstein Condensates. Phys. Rev. Lett. 2010, 104, 050404. [Google Scholar] [CrossRef] [PubMed]
- Banerjee, D.; Dalmonte, M.; Müller, M.; Rico, E.; Stebler, P.; Wiese, U.J.; Zoller, P. Atomic Quantum Simulation of Dynamical Gauge Fields Coupled to Fermionic Matter: From String Breaking to Evolution after a Quench. Phys. Rev. Lett. 2012, 109, 175302. [Google Scholar] [CrossRef] [PubMed]
- Banerjee, D.; Bögli, M.; Dalmonte, M.; Rico, E.; Stebler, P.; Wiese, U.J.; Zoller, P. Atomic Quantum Simulation of U(N) and SU(N) Non-Abelian Lattice Gauge Theories. Phys. Rev. Lett. 2013, 110, 125303. [Google Scholar] [CrossRef] [PubMed]
- Chandrasekharan, S.; Wiese, U.J. Quantum link models: A discrete approach to gauge theories. Nucl. Phys. B 1997, 492, 455–471. [Google Scholar] [CrossRef]
- Brower, R.; Chandrasekharan, S.; Wiese, U.J. QCD as a quantum link model. Phys. Rev. D 1999, 60, 094502. [Google Scholar] [CrossRef]
- Bañuls, M.C.; Blatt, R.; Catani, J.; Celi, A.; Cirac, J.I.; Dalmonte, M.; Fallani, L.; Jansen, K.; Lewenstein, M.; Montangero, S.; et al. Simulating lattice gauge theories within quantum technologies. Eur. Phys. J. D 2020, 74, 165. [Google Scholar] [CrossRef]
- Aidelsburger, M.; Barbiero, L.; Bermudez, A.; Chanda, T.; Dauphin, A.; González-Cuadra, D.; Grzybowski, P.R.; Hands, S.; Jendrzejewski, F.; Jünemann, J.; et al. Cold atoms meet lattice gauge theory. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2022, 380, 20210064. [Google Scholar] [CrossRef] [PubMed]
- Rico, E.; Pichler, T.; Dalmonte, M.; Zoller, P.; Montangero, S. Tensor Networks for Lattice Gauge Theories and Atomic Quantum Simulation. Phys. Rev. Lett. 2014, 112, 201601. [Google Scholar] [CrossRef]
- Pichler, T.; Dalmonte, M.; Rico, E.; Zoller, P.; Montangero, S. Real-Time Dynamics in U(1) Lattice Gauge Theories with Tensor Networks. Phys. Rev. X 2016, 6, 011023. [Google Scholar] [CrossRef]
- Zohar, E.; Cirac, J.I. Combining tensor networks with Monte Carlo methods for lattice gauge theories. Phys. Rev. D 2018, 97, 034510. [Google Scholar] [CrossRef]
- Büchler, H.P.; Hermele, M.; Huber, S.D.; Fisher, M.P.A.; Zoller, P. Atomic Quantum Simulator for Lattice Gauge Theories and Ring Exchange Models. Phys. Rev. Lett. 2005, 95, 040402. [Google Scholar] [CrossRef] [PubMed]
- Zohar, E.; Reznik, B. Confinement and Lattice Quantum-Electrodynamic Electric Flux Tubes Simulated with Ultracold Atoms. Phys. Rev. Lett. 2011, 107, 275301. [Google Scholar] [CrossRef] [PubMed]
- Szirmai, G.; Szirmai, E.; Zamora, A.; Lewenstein, M. Gauge fields emerging from time-reversal symmetry breaking for spin-5/2 fermions in a honeycomb lattice. Phys. Rev. A 2011, 84, 011611. [Google Scholar] [CrossRef]
- Wen, X. Quantum Field Theory of Many-body Systems: From the Origin of Sound to an Origin of Light and Electrons; Oxford Graduate Texts; Oxford University Press: Oxford, UK, 2004. [Google Scholar]
- Zohar, E.; Cirac, J.I.; Reznik, B. Simulating Compact Quantum Electrodynamics with Ultracold Atoms: Probing Confinement and Nonperturbative Effects. Phys. Rev. Lett. 2012, 109, 125302. [Google Scholar] [CrossRef] [PubMed]
- Tagliacozzo, L.; Celi, A.; Orland, P.; Mitchell, M.W.; Lewenstein, M. Simulation of non-Abelian gauge theories with optical lattices. Nat. Commun. 2013, 4, 2615. [Google Scholar] [CrossRef] [PubMed]
- Georgi, H. Lie Algebras In Particle Physics: From Isospin To Unified Theories; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Yip, S.K. Theory of a fermionic superfluid with SU(2) × SU(6) symmetry. Phys. Rev. A 2011, 83, 063607. [Google Scholar] [CrossRef]
- Lepori, L.; Trombettoni, A.; Vinci, W. Simulation of two-flavor symmetry-locking phases in ultracold fermionic mixtures. Europhys. Lett. 2015, 109, 50002. [Google Scholar] [CrossRef]
- Boada, O.; Celi, A.; Latorre, J.I.; Lewenstein, M. Quantum Simulation of an Extra Dimension. Phys. Rev. Lett. 2012, 108, 133001. [Google Scholar] [CrossRef]
- Celi, A.; Massignan, P.; Ruseckas, J.; Goldman, N.; Spielman, I.B.; Juzeliūnas, G.; Lewenstein, M. Synthetic Gauge Fields in Synthetic Dimensions. Phys. Rev. Lett. 2014, 112, 043001. [Google Scholar] [CrossRef] [PubMed]
- Mancini, M.; Pagano, G.; Cappellini, G.; Livi, L.; Rider, M.; Catani, J.; Sias, C.; Zoller, P.; Inguscio, M.; Dalmonte, M.; et al. Observation of chiral edge states with neutral fermions in synthetic Hall ribbons. Science 2015, 349, 1510–1513. [Google Scholar] [CrossRef] [PubMed]
- Weinberg, S. The Quantum Theory of Fields: Volume 2, Modern Applications; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Zee, A. Quantum Field Theory in a Nutshell, 2nd ed.; In a Nutshell; Princeton University Press: Princeton, NJ, USA, 2010. [Google Scholar]
- Huber, S.D.; Altman, E.; Büchler, H.P.; Blatter, G. Dynamical properties of ultracold bosons in an optical lattice. Phys. Rev. B 2007, 75, 085106. [Google Scholar] [CrossRef]
- Pollet, L.; Prokofév, N. Higgs Mode in a Two-Dimensional Superfluid. Phys. Rev. Lett. 2012, 109, 010401. [Google Scholar] [CrossRef] [PubMed]
- Pekker, D.; Varma, C. Amplitude/Higgs Modes in Condensed Matter Physics. Annu. Rev. Condens. Matter Phys. 2015, 6, 269–297. [Google Scholar] [CrossRef]
- Hill, C.T.; Simmons, E.H. Strong Dynamics and Electroweak Symmetry Breaking. Phys. Rep. 2003, 381, 235–402, Erratum in Phys. Rep. 2004, 390, 553–554. [Google Scholar] [CrossRef]
- Alford, M.; Rajagopal, K.; Wilczek, F. Color-flavor locking and chiral symmetry breaking in high density QCD. Nucl. Phys. B 1999, 537, 443–458. [Google Scholar] [CrossRef]
- Alford, M.G.; Schmitt, A.; Rajagopal, K.; Schäfer, T. Color superconductivity in dense quark matter. Rev. Mod. Phys. 2008, 80, 1455–1515. [Google Scholar] [CrossRef]
- Anglani, R.; Casalbuoni, R.; Ciminale, M.; Ippolito, N.; Gatto, R.; Mannarelli, M.; Ruggieri, M. Crystalline color superconductors. Rev. Mod. Phys. 2014, 86, 509–561. [Google Scholar] [CrossRef]
- Mannarelli, M. The amazing properties of crystalline color superconductors. J. Physics: Conf. Ser. 2014, 527, 012020. [Google Scholar] [CrossRef]
- Peskin, M.; Schroeder, D. An Introduction To Quantum Field Theory; Frontiers in Physics; Avalon Publishing: New York, NY, USA, 1995. [Google Scholar]
- ’t Hooft, G. Symmetry Breaking through Bell-Jackiw Anomalies. Phys. Rev. Lett. 1976, 37, 8–11. [Google Scholar] [CrossRef]
- Nielsen, H.; Ninomiya, M. The Adler-Bell-Jackiw anomaly and Weyl fermions in a crystal. Phys. Lett. B 1983, 130, 389–396. [Google Scholar] [CrossRef]
- Wan, X.; Turner, A.M.; Vishwanath, A.; Savrasov, S.Y. Topological semimetal and Fermi-arc surface states in the electronic structure of pyrochlore iridates. Phys. Rev. B 2011, 83, 205101. [Google Scholar] [CrossRef]
- Fang, C.; Gilbert, M.J.; Dai, X.; Bernevig, B.A. Multi-Weyl Topological Semimetals Stabilized by Point Group Symmetry. Phys. Rev. Lett. 2012, 108, 266802. [Google Scholar] [CrossRef] [PubMed]
- Bradlyn, B.; Cano, J.; Wang, Z.; Vergniory, M.G.; Felser, C.; Cava, R.J.; Bernevig, B.A. Beyond Dirac and Weyl fermions: Unconventional quasiparticles in conventional crystals. Science 2016, 353, aaf5037. [Google Scholar] [CrossRef]
- Lepori, L.; Burrello, M.; Guadagnini, E. Axial anomaly in multi-Weyl and triple-point semimetals. J. High Energy Phys. 2018, 2018, 110. [Google Scholar] [CrossRef]
- Semenoff, G.W. Condensed-Matter Simulation of a Three-Dimensional Anomaly. Phys. Rev. Lett. 1984, 53, 2449–2452. [Google Scholar] [CrossRef]
- Mannarelli, M. Meson Condensation. Particles 2019, 2, 411–443. [Google Scholar] [CrossRef]
- Borsányi, S.; Endrodi, G.; Fodor, Z.; Katz, S.D.; Szabó, K.K. Precision SU(3) lattice thermodynamics for a large temperature range. J. High Energy Phys. 2012, 2012, 56. [Google Scholar] [CrossRef]
- Zhitnitsky, A.R. Conformal window in QCD for large numbers of colors and flavors. Nucl. Phys. A 2014, 921, 1–18. [Google Scholar] [CrossRef][Green Version]
- Deuzeman, A.; Lombardo, M.P.; Pallante, E. Evidence for a conformal phase in SU(N) gauge theories. Phys. Rev. D 2010, 82, 074503. [Google Scholar] [CrossRef]
- Surace, F.M.; Mazza, P.P.; Giudici, G.; Lerose, A.; Gambassi, A.; Dalmonte, M. Lattice Gauge Theories and String Dynamics in Rydberg Atom Quantum Simulators. Phys. Rev. X 2020, 10, 021041. [Google Scholar] [CrossRef]
- Celi, A.; Vermersch, B.; Viyuela, O.; Pichler, H.; Lukin, M.D.; Zoller, P. Emerging Two-Dimensional Gauge Theories in Rydberg Configurable Arrays. Phys. Rev. X 2020, 10, 021057. [Google Scholar] [CrossRef]
- Dumitrescu, P.T.; Bohnet, J.G.; Gaebler, J.P.; Hankin, A.; Hayes, D.; Kumar, A.; Neyenhuis, B.; Vasseur, R.; Potter, A.C. Dynamical topological phase realized in a trapped-ion quantum simulator. Nature 2022, 607, 463–467. [Google Scholar] [CrossRef] [PubMed]
- Ringbauer, M.; Meth, M.; Postler, L.; Stricker, R.; Blatt, R.; Schindler, P.; Monz, T. A universal qudit quantum processor with trapped ions. Nat. Phys. 2022, 18, 1053–1057. [Google Scholar] [CrossRef]
- Chertkov, E.; Bohnet, J.; Francois, D.; Gaebler, J.; Gresh, D.; Hankin, A.; Lee, K.; Hayes, D.; Neyenhuis, B.; Stutz, R.; et al. Holographic dynamics simulations with a trapped-ion quantum computer. Nat. Phys. 2022, 18, 1074–1079. [Google Scholar] [CrossRef]
- Richerme, P.; Gong, Z.X.; Lee, A.; Senko, C.; Smith, J.; Foss-Feig, M.; Michalakis, S.; Gorshkov, A.V.; Monroe, C. Non-local propagation of correlations in quantum systems with long-range interactions. Nature 2014, 511, 198–201. [Google Scholar] [CrossRef] [PubMed]
- Jurcevic, P.; Lanyon, B.P.; Hauke, P.; Hempel, C.; Zoller, P.; Blatt, R.; Roos, C.F. Quasiparticle engineering and entanglement propagation in a quantum many-body system. Nature 2014, 511, 202–205. [Google Scholar] [CrossRef] [PubMed]
- Vodola, D.; Lepori, L.; Ercolessi, E.; Gorshkov, A.V.; Pupillo, G. Kitaev Chains with Long-Range Pairing. Phys. Rev. Lett. 2014, 113, 156402. [Google Scholar] [CrossRef] [PubMed]
- Lepori, L.; Giuliano, D.; Paganelli, S. Edge insulating topological phases in a two-dimensional superconductor with long-range pairing. Phys. Rev. B 2018, 97, 041109. [Google Scholar] [CrossRef]
- Lucas, A. Ising formulations of many NP problems. Front. Phys. 2014, 2, 74887. [Google Scholar] [CrossRef]
- Defenu, N.; Donner, T.; Macrì, T.; Pagano, G.; Ruffo, S.; Trombettoni, A. Long-range interacting quantum systems. arXiv 2021, arXiv:cond-mat.quant-gas/2109.01063. [Google Scholar] [CrossRef]
- Diessel, O.K.; Diehl, S.; Defenu, N.; Rosch, A.; Chiocchetta, A. Generalized Higgs mechanism in long-range interacting quantum systems. arXiv 2022, arXiv:2208.10487. [Google Scholar] [CrossRef]
- Song, M.; Zhao, J.; Zhou, C.; Meng, Z.Y. Dynamical properties of quantum many-body systems with long range interactions. arXiv 2023, arXiv:2301.00829. [Google Scholar] [CrossRef]
- Qiu, Z. Supersymmetry, two-dimensional critical phenomena and the tricritical Ising model. Nucl. Phys. B 1986, 270, 205–234. [Google Scholar] [CrossRef]
- Mussardo, G. Statistical Field Theory: An Introduction to Exactly Solved Models in Statistical Physics; Oxford Graduate Texts; Oxford University Press: Oxford, UK, 2010. [Google Scholar]
- Yu, Y.; Yang, K. Supersymmetry and the Goldstino-Like Mode in Bose-Fermi Mixtures. Phys. Rev. Lett. 2008, 100, 090404. [Google Scholar] [CrossRef] [PubMed]
- Tomka, M.; Pletyukhov, M.; Gritsev, V. Supersymmetry in quantum optics and in spin-orbit coupled systems. Sci. Rep. 2015, 5, 13097. [Google Scholar] [CrossRef] [PubMed]
- Yu, Y.; Yang, K. Simulating the Wess-Zumino Supersymmetry Model in Optical Lattices. Phys. Rev. Lett. 2010, 105, 150605. [Google Scholar] [CrossRef] [PubMed]
- Cooper, L.; Feldman, D. BCS: 50 Years; World Scientific: Singapore, 2011. [Google Scholar]
- Rapp, A.; Zaránd, G.; Honerkamp, C.; Hofstetter, W. Color Superfluidity and “Baryon” Formation in Ultracold Fermions. Phys. Rev. Lett. 2007, 98, 160405. [Google Scholar] [CrossRef] [PubMed]
- Baym, G. The Microscopic Description of Superfluidity. In Mathematical Methods in Solid State and Superfluid Theory; Clark, R.C., Derrick, G.H., Eds.; Springer: Berlin/Heidelberg, Germany, 1968; Chapter 3; pp. 121–156. [Google Scholar]
- Guenther, J.N. Overview of the QCD phase diagram. Eur. Phys. J. A 2021, 57, 136. [Google Scholar] [CrossRef] [PubMed]
- Fulde, P.; Ferrell, R.A. Superconductivity in a Strong Spin-Exchange Field. Phys. Rev. 1964, 135, A550–A563. [Google Scholar] [CrossRef]
- Larkin, A.I.; Ovchinnikov, Y.N. Non-uniform state of superconductors. Zh. Eksperim. Teor. Fiz. 1964, 47, 847. [Google Scholar]
- Alford, M.; Kapustin, A.; Wilczek, F. Imaginary chemical potential and finite fermion density on the lattice. Phys. Rev. D 1999, 59, 054502. [Google Scholar] [CrossRef]
- Lombardo, M.P. Finite density [might well be easier] at finite temperature. Nucl. Phys. B Proc. Suppl. 2000, 83-84, 375–377. [Google Scholar]
- Nishida, Y. Phase structures of strong coupling lattice QCD with finite baryon and isospin density. Phys. Rev. D 2004, 69, 094501. [Google Scholar] [CrossRef]
- Cea, P.; Cosmai, L.; D’Elia, M.; Papa, A.; Sanfilippo, F. Critical line of two-flavor QCD at finite isospin or baryon densities from imaginary chemical potentials. Phys. Rev. D 2012, 85, 094512. [Google Scholar] [CrossRef]
- Brandt, B.B.; Cuteri, F.; Endrődi, G.; Schmalzbauer, S. Dirac spectrum and the BEC-BCS crossover in QCD at nonzero isospin asymmetry. arXiv 1912. https://doi.org/10.48550/arXiv.1912.07451. [Google Scholar]
- Carignano, S.; Lepori, L.; Mammarella, A.; Mannarelli, M.; Pagliaroli, G. Scrutinizing the pion condensed phase. Eur. Phys. J. 2017, 53, 35. [Google Scholar] [CrossRef]
- Parish, M.M.; Marchetti, F.M.; Lamacraft, A.; Simons, B.D. Finite-temperature phase diagram of a polarized Fermi condensate. Nat. Phys. 2007, 3, 124–128. [Google Scholar] [CrossRef]
- Ravensbergen, C.; Soave, E.; Corre, V.; Kreyer, M.; Huang, B.; Kirilov, E.; Grimm, R. Resonantly Interacting Fermi-Fermi Mixture of 161Dy and 40K. Phys. Rev. Lett. 2020, 124, 203402. [Google Scholar] [CrossRef] [PubMed]
- Hawking, S.; Ellis, G. The Large Scale Structure of Space-Time, 1st ed.; Cambridge University Press: Cambridge, UK, 1973. [Google Scholar]
- Tino, G.; Cacciapuoti, L.; Capozziello, S.; Lambiase, G.; Sorrentino, F. Precision gravity tests and the Einstein Equivalence Principle. Prog. Part. Nucl. Phys. 2020, 112, 103772. [Google Scholar] [CrossRef]
- Damour, T.; Polyakov, A. The string dilation and a least coupling principle. Nucl. Phys. B 1994, 423, 532–558. [Google Scholar] [CrossRef]
- Tino, G.M.; Vetrano, F. Is it possible to detect gravitational waves with atom interferometers? Class. Quantum Gravity 2007, 24, 2167. [Google Scholar] [CrossRef]
- Dimopoulos, S.; Graham, P.W.; Hogan, J.M.; Kasevich, M.A.; Rajendran, S. Atomic gravitational wave interferometric sensor. Phys. Rev. D 2008, 78, 122002. [Google Scholar] [CrossRef]
- Kolkowitz, S.; Pikovski, I.; Langellier, N.; Lukin, M.D.; Walsworth, R.L.; Ye, J. Gravitational wave detection with optical lattice atomic clocks. Phys. Rev. D 2016, 94, 124043. [Google Scholar] [CrossRef]
- Counts, I.; Hur, J.; Craik, D.P.A.; Jeon, H.; Leung, C.; Berengut, J.C.; Geddes, A.; Kawasaki, A.; Jhe, W.; Vuletić, V. Evidence for Nonlinear Isotope Shift in Yb+ Search for New Boson. Phys. Rev. Lett. 2020, 125, 123002. [Google Scholar] [CrossRef] [PubMed]
- Solaro, C.; Meyer, S.; Fisher, K.; Berengut, J.C.; Fuchs, E.; Drewsen, M. Improved Isotope-Shift-Based Bounds on Bosons beyond the Standard Model through Measurements of the 2D3/2-2D5/2 Interval in Ca+. Phys. Rev. Lett. 2020, 125, 123003. [Google Scholar] [CrossRef] [PubMed]
- Hinkley, N.; Sherman, J.A.; Phillips, N.B.; Schioppo, M.; Lemke, N.D.; Beloy, K.; Pizzocaro, M.; Oates, C.W.; Ludlow, A.D. An atomic clock with 10−18 instability. Science 2013, 341, 1215–1218. [Google Scholar] [CrossRef]
- Nicholson, T.L.; Martin, M.J.; Williams, J.R.; Bloom, B.J.; Bishof, M.; Swallows, M.D.; Campbell, S.L.; Ye, J. Comparison of Two Independent Sr Optical Clocks with 1×10−17 Stability at 103s. Phys. Rev. Lett. 2012, 109, 230801. [Google Scholar] [CrossRef]
- Campbell, S.L.; Hutson, R.B.; Marti, G.E.; Goban, A.; Oppong, N.D.; McNally, R.L.; Sonderhouse, L.; Robinson, J.M.; Zhang, W.; Bloom, B.J.; et al. A Fermi-degenerate three-dimensional optical lattice clock. Science 2017, 358, 90–94. [Google Scholar] [CrossRef] [PubMed]
- Kim, K.; Aeppli, A.; Bothwell, T.; Ye, J. Evaluation of Lattice Light Shift at Low 10−19 Uncertainty for a Shallow Lattice Sr Optical Clock. Phys. Rev. Lett. 2023, 130, 113203. [Google Scholar] [CrossRef] [PubMed]
- Zheng, X.; Dolde, J.; Lochab, V.; Merriman, B.N.; Li, H.; Kolkowitz, S. Differential clock comparisons with a multiplexed optical lattice clock. Nature 2022, 602, 425–430. [Google Scholar] [CrossRef] [PubMed]
- Derevianko, A.; Pospelov, M. Hunting for topological dark matter with atomic clocks. Nat. Phys. 2014, 10, 933–936. [Google Scholar] [CrossRef]
- Van Tilburg, K.; Leefer, N.; Bougas, L.; Budker, D. Search for Ultralight Scalar Dark Matter with Atomic Spectroscopy. Phys. Rev. Lett. 2015, 115, 011802. [Google Scholar] [CrossRef] [PubMed]
- Arvanitaki, A.; Huang, J.; Van Tilburg, K. Searching for dilaton dark matter with atomic clocks. Phys. Rev. D 2015, 91, 015015. [Google Scholar] [CrossRef]
- Stadnik, Y.V.; Flambaum, V.V. Enhanced effects of variation of the fundamental constants in laser interferometers and application to dark-matter detection. Phys. Rev. A 2016, 93, 063630. [Google Scholar] [CrossRef]
- Kovachy, T.; Asenbaum, P.; Overstreet, C.; Donnelly, C.A.; Dickerson, S.M.; Sugarbaker, A.; Hogan, J.M.; Kasevich, M.A. Quantum superposition at the half-metre scale. Nature 2015, 528, 530–533. [Google Scholar] [CrossRef] [PubMed]
- Poli, N.; Wang, F.Y.; Tarallo, M.G.; Alberti, A.; Prevedelli, M.; Tino, G.M. Precision Measurement of Gravity with Cold Atoms in an Optical Lattice and Comparison with a Classical Gravimeter. Phys. Rev. Lett. 2011, 106, 038501. [Google Scholar] [CrossRef] [PubMed]
- Peters, A.; Chung, K.Y.; Chu, S. Measurement of gravitational acceleration by dropping atoms. Nature 1999, 400, 849–852. [Google Scholar] [CrossRef]
- McGuirk, J.M.; Foster, G.T.; Fixler, J.B.; Snadden, M.J.; Kasevich, M.A. Sensitive absolute-gravity gradiometry using atom interferometry. Phys. Rev. A 2002, 65, 033608. [Google Scholar] [CrossRef]
- D’Amico, G.; Borselli, F.; Cacciapuoti, L.; Prevedelli, M.; Rosi, G.; Sorrentino, F.; Tino, G.M. Bragg interferometer for gravity gradient measurements. Phys. Rev. A 2016, 93, 063628. [Google Scholar] [CrossRef]
- Tarallo, M.G.; Alberti, A.; Poli, N.; Chiofalo, M.L.; Wang, F.Y.; Tino, G.M. Delocalization-enhanced Bloch oscillations and driven resonant tunneling in optical lattices for precision force measurements. Phys. Rev. A 2012, 86, 033615. [Google Scholar] [CrossRef]
- Alberti, A.; Ferrari, G.; Ivanov, V.V.; Chiofalo, M.L.; Tino, G.M. Atomic wave packets in amplitude-modulated vertical optical lattices. New J. Phys. 2010, 12, 065037. [Google Scholar] [CrossRef]
- McAlpine, K.E.; Gochnauer, D.; Gupta, S. Excited-band Bloch oscillations for precision atom interferometry. Phys. Rev. A 2020, 101, 023614. [Google Scholar] [CrossRef]
- Hu, L.; Wang, E.; Salvi, L.; Tinsley, J.N.; Tino, G.M.; Poli, N. Sr atom interferometry with the optical clock transition as a gravimeter and a gravity gradiometer. Class. Quantum Gravity 2019, 37, 014001. [Google Scholar] [CrossRef]
- Asenbaum, P.; Overstreet, C.; Kovachy, T.; Brown, D.D.; Hogan, J.M.; Kasevich, M.A. Phase Shift in an Atom Interferometer due to Spacetime Curvature across its Wave Function. Phys. Rev. Lett. 2017, 118, 183602. [Google Scholar] [CrossRef] [PubMed]
- Conlon, L.O.; Michel, T.; Guccione, G.; McKenzie, K.; Assad, S.M.; Lam, P.K. Enhancing the precision limits of interferometric satellite geodesy missions. NPJ Microgravity 2022, 8, 21. [Google Scholar] [CrossRef] [PubMed]
- Durfee, D.S.; Shaham, Y.K.; Kasevich, M.A. Gravity gradiometry using a Bose-Einstein condensate. Phys. Rev. Lett. 2006, 97, 240801. [Google Scholar] [CrossRef] [PubMed]
- Gustavson, T.L.; Landragin, A.; Kasevich, M.A. Rotation sensing with a dual atom-interferometer Sagnac gyroscope. Class. Quantum Gravity 2000, 17, 2385. [Google Scholar] [CrossRef]
- Ferrari, G.; Poli, N.; Sorrentino, F.; Tino, G.M. Long-lived Bloch oscillations with bosonic Sr atoms and application to gravity measurement at the micrometer scale. Phys. Rev. Lett. 2006, 97, 060402. [Google Scholar] [CrossRef] [PubMed]
- Amelino-Camelia, G.; Lämmerzahl, C.; Mercati, F.; Tino, G.M. Constraining the energy-momentum dispersion relation with Planck-scale sensitivity using cold atoms. Phys. Rev. Lett. 2009, 103, 171302. [Google Scholar] [CrossRef] [PubMed]
- De Angelis, M.; Bertoldi, A.; Cacciapuoti, L.; Giorgini, A.; Lamporesi, G.; Prevedelli, M.; Saccorotti, G.; Sorrentino, F.; Tino, G.M. Precision gravimetry with atomic sensors. Meas. Sci. Technol. 2009, 20, 022001. [Google Scholar] [CrossRef]
- Damour, T.; Donoghue, J.F. Equivalence principle violations and couplings of a light dilaton. Phys. Rev. D 2010, 82, 084033. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D. History of dark matter. Rev. Mod. Phys. 2018, 90, 045002. [Google Scholar] [CrossRef]
- Hees, A.; Minazzoli, O.; Savalle, E.; Stadnik, Y.V.; Wolf, P. Violation of the equivalence principle from light scalar dark matter. Phys. Rev. D 2018, 98, 064051. [Google Scholar] [CrossRef]
- Rogers, K.K.; Peiris, H.V. Strong Bound on Canonical Ultralight Axion Dark Matter from the Lyman-Alpha Forest. Phys. Rev. Lett. 2021, 126, 071302. [Google Scholar] [CrossRef] [PubMed]
- Ellis, J.; Hagelin, J.S.; Nanopoulos, D.; Srednicki, M. Search for violations of quantum mechanics. Nucl. Phys. B 1984, 241, 381–405. [Google Scholar] [CrossRef]
- Diósi, L. Models for universal reduction of macroscopic quantum fluctuations. Phys. Rev. A 1989, 40, 1165–1174. [Google Scholar] [CrossRef] [PubMed]
- Penrose, R. On Gravity’s Role in Quantum State Reduction. Gen. Relativ. Gravit. 1996, 28, 581–600. [Google Scholar] [CrossRef]
- Gasbarri, G.; Belenchia, A.; Carlesso, M.; Donadi, S.; Bassi, A.; Kaltenbaek, R.; Paternostro, M.; Ulbricht, H. Testing the Foundation of Quantum Physics in Space via Interferometric and Non-Interferometric Experiments with Mesoscopic Nanoparticles. Commun. Phys. 2021, 4, 155. [Google Scholar] [CrossRef]
- Reynaud, S.; Salomon, C.; Wolf, P. Testing General Relativity with Atomic Clocks. Space Sci. Rev. 2009, 148, 233–247. [Google Scholar] [CrossRef][Green Version]
- Schiller, S.; Tino, G.M.; Gill, P.; Salomon, C.; Sterr, U.; Peik, E.; Nevsky, A.; Görlitz, A.; Svehla, D.; Ferrari, G.; et al. Einstein Gravity Explorer—A medium-class fundamental physics mission. Exp. Astron. 2009, 23, 573–610. [Google Scholar] [CrossRef]
- Lilley, M.; Savalle, E.; Angonin, M.C.; Delva, P.; Guerlin, C.; Le Poncin-Lafitte, C.; Meynadier, F.; Wolf, P. ACES/PHARAO: High-performance space-to-ground and ground-to-ground clock comparison for fundamental physics. GPS Solut. 2021, 25, 34. [Google Scholar] [CrossRef]
- Cacciapuoti, L.; Armano, M.; Much, R.; Sy, O.; Helm, A.; Hess, M.P.; Kehrer, J.; Koller, S.; Niedermaier, T.; Esnault, F.X.; et al. Testing gravity with cold-atom clocks in space. Eur. Phys. J. D 2020, 74, 164. [Google Scholar] [CrossRef]
- Schlamminger, S.; Choi, K.Y.; Wagner, T.A.; Gundlach, J.H.; Adelberger, E.G. Test of the Equivalence Principle Using a Rotating Torsion Balance. Phys. Rev. Lett. 2008, 100, 041101. [Google Scholar] [CrossRef] [PubMed]
- Nobili, A.; Bramanti, D.; Comandi, G.; Toncelli, R.; Polacco, E.; Chiofalo, M. “Galileo Galilei-GG”: Design, requirements, error budget and significance of the ground prototype. Phys. Lett. A 2003, 318, 172–183. [Google Scholar] [CrossRef]
- Blaser, J.; Cornelisse, J.; Cruise, T.; Damour, F.; Hechler, M.; Hechler, Y.; Jafry, B.; Kent, N.; Lockerbie, H.; Pik, A.; et al. STEP: “Satellite Test of the Equivalence Principle”; Report on the Phase A Study, Technical Report, ESA SCI (96)5; NASA: Washington, DC, USA, 1996. [Google Scholar]
- Touboul, P.; Métris, G.; Rodrigues, M.; André, Y.; Baghi, Q.; Bergé, J.; Boulanger, D.; Bremer, S.; Carle, P.; Chhun, R.; et al. MICROSCOPE Mission: First Results of a Space Test of the Equivalence Principle. Phys. Rev. Lett. 2017, 119, 231101. [Google Scholar] [CrossRef] [PubMed]
- Touboul, P.; Métris, G.; Rodrigues, M.; André, Y.; Baghi, Q.; Bergé, J.; Boulanger, D.; Bremer, S.; Chhun, R.; Christophe, B.; et al. Space test of the equivalence principle: First results of the MICROSCOPE mission. Class. Quantum Gravity 2019, 36, 225006. [Google Scholar] [CrossRef]
- MICROSCOPE Collaboration; Touboul, P. MICROSCOPE Mission: Final Results of the Test of the Equivalence Principle. Phys. Rev. Lett. 2022, 129, 121102. [Google Scholar]
- Asenbaum, P.; Overstreet, C.; Kim, M.; Curti, J.; Kasevich, M.A. Atom-Interferometric Test of the Equivalence Principle at the 10−12 Level. Phys. Rev. Lett. 2020, 125, 191101. [Google Scholar] [CrossRef] [PubMed]
- Battelier, B.; Bergé, J.; Bertoldi, A.; Blanchet, L.; Bongs, K.; Bouyer, P.; Braxmaier, C.; Calonico, D.; Fayet, P.; Gaaloul, N.; et al. Exploring the foundations of the physical universe with space tests of the equivalence principle. Exp. Astron. 2021, 51, 1695–1736. [Google Scholar] [CrossRef]
- Müntinga, H.; Ahlers, H.; Krutzik, M.; Wenzlawski, A.; Arnold, S.; Becker, D.; Bongs, K.; Dittus, H.; Duncker, H.; Gaaloul, N.; et al. Interferometry with Bose-Einstein condensates in microgravity. Phys. Rev. Lett. 2013, 110, 093602. [Google Scholar] [CrossRef] [PubMed]
- Deppner, C.; Herr, W.; Cornelius, M.; Stromberger, P.; Sternke, T.; Grzeschik, C.; Grote, A.; Rudolph, J.; Herrmann, S.; Krutzik, M.; et al. Collective-mode enhanced matter-wave optics. Phys. Rev. Lett. 2021, 127, 100401. [Google Scholar] [CrossRef] [PubMed]
- Nyman, R.A.; Varoquaux, G.; Lienhart, F.; Chambon, D.; Boussen, S.; Cl’ement, J.-F.; Muller, T.; Santarelli, G.; Santos, F.P.D.; Clairon, A.; et al. I.C.E.: A transportable atomic inertial sensor for test in microgravity. Appl. Phys. B 2006, 84, 673–681. [Google Scholar] [CrossRef][Green Version]
- Lachmann, M.D.; Ahlers, H.; Becker, D.; Dinkelaker, A.N.; Grosse, J.; Hellmig, O.; Müntinga, H.; Schkolnik, V.; Seidel, S.T.; Wendrich, T.; et al. Ultracold atom interferometry in space. Nature Comm. 2021, 12, 1317. [Google Scholar] [CrossRef] [PubMed]
- Aveline, D.C.; Williams, J.R.; Elliott, E.R.; Dutenhoffer, C.; Kellogg, J.R.; Kohel, J.M.; Lay, N.E.; Oudrhiri, K.; Shotwell, R.F.; Yu, N.; et al. Observation of Bose-Einstein condensates in an earth-orbiting research lab. Nature 2020, 582, 193–197. [Google Scholar] [CrossRef] [PubMed]
- Loriani, S.; Schubert, C.; Schlippert, D.; Ertmer, W.; Santos, F.P.D.; Rasel, E.M.; Gaaloul, N.; Wolf, P. Resolution of the colocation problem in satellite quantum tests of the universality of free fall. Phys. Rev. D 2020, 102, 124043. [Google Scholar] [CrossRef]
- Bongs, K.; Bouyer, P.; Buchmueller, O.; Canuel, B.; Chiofalo, M.; Ellis, J.; Gaaloul, N.; Hogan, J.; Kovachy, T.; Rasel, E.; et al. In Proceedings of the Terrestrial Very-Long-Baseline Atom Interferometry Workshop, Geneva, Switzerland, 13–14 March 2023.
- Canuel, B.; Bertoldi, A.; Amand, L.; Pozzo di Borgo, E.; Chantrait, T.; Danquigny, C.; Dovale Álvarez, M.; Fang, B.; Freise, A.; Geiger, J.; et al. Exploring gravity with the MIGA large scale atom interferometer. Sci. Rep. 2018, 8, 14064. [Google Scholar] [CrossRef] [PubMed]
- Canuel, B.; Abend, S.; Amaro-Seoane, P.; Badaracco, F.; Beaufils, Q.; Bertoldi, A.; Bongs, K.; Bouyer, P.; Braxmaier, C.; Chaibi, W.; et al. ELGAR—A European Laboratory for Gravitation and Atom-interferometric Research. Class. Quantum Gravity 2020, 37, 225017. [Google Scholar] [CrossRef]
- Zhan, M.S.; Wang, J.; Ni, W.T.; Gao, D.F.; Wang, G.; He, L.X.; Li, R.B.; Zhou, L.; Chen, X.; Zhong, J.Q.; et al. ZAIGA: Zhaoshan long-baseline atom interferometer gravitation antenna. Int. J. Mod. Phys. D 2020, 29, 1940005. [Google Scholar] [CrossRef]
- Ufrecht, C.; Di Pumpo, F.; Friedrich, A.; Roura, A.; Schubert, C.; Schlippert, D.; Rasel, E.M.; Schleich, W.P.; Giese, E. Atom-interferometric test of the universality of gravitational redshift and free fall. Phys. Rev. Res. 2020, 2, 043240. [Google Scholar] [CrossRef]
- Badurina, L.; Bentine, E.; Blas, D.; Bongs, K.; Bortoletto, D.; Bowcock, T.; Bridges, K.; Bowden, W.; Buchmueller, O.; Burrage, C.; et al. AION: An atom interferometer observatory and network. J. Cosmol. Astropart. Phys. 2020, 2020, 011. [Google Scholar] [CrossRef]
- Abe, M.; Adamson, P.; Borcean, M.; Bortoletto, D.; Bridges, K.; Carman, S.P.; Chattopadhyay, S.; Coleman, J.; Curfman, N.M.; DeRose, K.; et al. Matter-wave Atomic Gradiometer Interferometric Sensor (MAGIS-100). Quantum Sci. Technol. 2021, 6, 044003. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. GW150914: The Advanced LIGO Detectors in the Era of First Discoveries. Phys. Rev. Lett. 2016, 116, 131103. [Google Scholar] [CrossRef] [PubMed]
- Amaro-Seoane, P.; Andrews, J.; Arca Sedda, M.; Askar, A.; Baghi, Q.; Balasov, R.; Bartos, I.; Bavera, S.S.; Bellovary, J.; Berry, C.P.L.; et al. Astrophysics with the Laser Interferometer Space Antenna. Living Rev. Relativ. 2023, 26, 2. [Google Scholar] [CrossRef]
- Belenchia, A.; Carlesso, M.; Bayraktar, Ö.; Dequal, D.; Derkach, I.; Gasbarri, G.; Herr, W.; Li, Y.L.; Rademacher, M.; Sidhu, J.; et al. Quantum Physics in Space. Phys. Rep. 2022, 951, 1–70. [Google Scholar] [CrossRef]
- Foster, J.W.; Rodd, N.L.; Safdi, B.R. Revealing the dark matter halo with axion direct detection. Phys. Rev. D 2018, 97, 123006. [Google Scholar] [CrossRef]
- Derevianko, A. Detecting dark-matter waves with a network of precision-measurement tools. Phys. Rev. A 2018, 97, 042506. [Google Scholar] [CrossRef]
- Lee, J.W. Brief History of Ultra-light Scalar Dark Matter Models. EPJ Web Conf. 2018, 168, 06005. [Google Scholar] [CrossRef]
- Hees, A.; Guéna, J.; Abgrall, M.; Bize, S.; Wolf, P. Searching for an Oscillating Massive Scalar Field as a Dark Matter Candidate Using Atomic Hyperfine Frequency Comparisons. Phys. Rev. Lett. 2016, 117, 061301. [Google Scholar] [CrossRef]
- Wagner, T.A.; Schlamminger, S.; Gundlach, J.H.; Adelberger, E.G. Torsion-balance tests of the weak equivalence principle. Class. Quantum Gravity 2012, 29, 184002. [Google Scholar] [CrossRef]
- Graham, P.W.; Kaplan, D.E.; Mardon, J.; Rajendran, S.; Terrano, W.A.; Trahms, L.; Wilkason, T. Spin precession experiments for light axionic dark matter. Phys. Rev. D 2018, 97, 055006. [Google Scholar] [CrossRef]
- Graham, P.W.; Kaplan, D.E.; Mardon, J.; Rajendran, S.; Terrano, W.A. Dark matter direct detection with accelerometers. Phys. Rev. D 2016, 93, 075029. [Google Scholar] [CrossRef]
- Bailes, M.; Berger, B.K.; Brady, P.R.; Branchesi, M.; Danzmann, K.; Evans, M.; Holley-Bockelmann, K.; Iyer, B.R.; Kajita, T.; Katsanevas, S.; et al. Gravitational-wave physics and astronomy in the 2020s and 2030s. Nat. Rev. Phys. 2021, 3, 344–366. [Google Scholar] [CrossRef]
- ECFA Detectors R&D Roadmap Process Group. The 2021 ECFA Detector Research and Developement Roadmap; CERN: Geneva, Switzerland, 2021. [Google Scholar] [CrossRef]
- Abend, S.; Allard, B.; Alonso, I.; Antoniadis, J.; Araujo, H.; Arduini, G.; Arnold, A.; Aßmann, T.; Augst, N.; Badurina, L.; et al. Terrestrial Very-Long-Baseline Atom Interferometry: Workshop Summary. arXiv 2023, arXiv:hep-ex/2310.08183. [Google Scholar]
- Schive, H.Y.; Chiueh, T.; Broadhurst, T. Cosmic structure as the quantum interference of a coherent dark wave. Nat. Phys. 2014, 10, 496–499. [Google Scholar] [CrossRef]
- Angulo, R.E.; Hahn, O. Large-scale dark matter simulations. Living Rev. Comput. Astrophys. 2022, 8, 1. [Google Scholar] [CrossRef]
- Faccio, D.; Belgiorno, F.; Cacciatori, S.; Gorini, V.; Liberati, S.; Moschella, U. Lecture Notes in Physics Analogue Gravity Phonomenology; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar] [CrossRef]
- Wald, R.M. General Relativity, 1984 ed.; University of Chicago Press: Chicago, IL, USA, 1984; p. 473. [Google Scholar]
- Schützhold, R.; Unruh, W.G. Gravity wave analogs of black holes. Phys. Rev. D 2002, 66, 044019. [Google Scholar] [CrossRef]
- Weinfurtner, S.; Tedford, E.W.; Penrice, M.C.J.; Unruh, W.G.; Lawrence, G.A. Measurement of stimulated Hawking emission in an analogue system. Phys. Rev. Lett. 2011, 106, 021302. [Google Scholar] [CrossRef] [PubMed]
- Euvé, L.P.; Michel, F.; Parentani, R.; Philbin, T.G.; Rousseaux, G. Observation of noise correlated by the Hawking effect in a water tank. Phys. Rev. Lett. 2016, 117, 121301. [Google Scholar] [CrossRef]
- Torres, T.; Patrick, S.; Coutant, A.; Richartz, M.; Tedford, E.W.; Weinfurtner, S. Observation of superradiance in a vortex flow. Nat. Phys. 2017, 13, 833–836. [Google Scholar] [CrossRef]
- Plebanski, J. Electromagnetic Waves in Gravitational Fields. Phys. Rev. 1959, 118, 1396–1408. [Google Scholar] [CrossRef]
- De Felice, F. On the gravitational field acting as an optical medium. Gen. Relativ. Gravit. 1971, 2, 347–367. [Google Scholar] [CrossRef]
- Schuster, S.; Visser, M. Effective metrics and a fully covariant description of constitutive tensors in electrodynamics. Phys. Rev. D 2017, 96, 124019. [Google Scholar] [CrossRef]
- Schuster, S.; Visser, M. Boyer-Lindquist space-times and beyond: Meta-material analogues. arXiv 2018, arXiv:1802.09807. [Google Scholar]
- Garay, L.J.; Anglin, J.R.; Cirac, J.I.; Zoller, P. Black holes in Bose-Einstein condensates. Phys. Rev. Lett. 2000, 85, 4643–4647. [Google Scholar] [CrossRef]
- Visser, M.; Barceló, C.; Liberati, S. Analog models of and for gravity. Gen. Relativ. Gravit. 2002, 34, 1719–1734. [Google Scholar] [CrossRef]
- Jacobson, T. Black hole evaporation and ultrashort distances. Phys. Rev. D 1991, 44, 1731–1739. [Google Scholar] [CrossRef]
- Unruh, W.G. Sonic analog of black holes and the effects of high frequencies on black hole evaporation. Phys. Rev. D 1995, 51, 2827–2838. [Google Scholar] [CrossRef]
- Finazzi, S.; Parentani, R. On the robustness of acoustic black hole spectra. J. Phys. Conf. Ser. 2011, 314, 012030. [Google Scholar] [CrossRef]
- Jacobson, T. Thermodynamics of space-time: The Einstein equation of state. Phys. Rev. Lett. 1995, 75, 1260–1263. [Google Scholar] [CrossRef] [PubMed]
- Liberati, S.; Visser, M.; Weinfurtner, S. Analogue quantum gravity phenomenology from a two-component Bose-Einstein condensate. Class. Quantum Gravity 2006, 23, 3129. [Google Scholar] [CrossRef]
- Howl, R.; Penrose, R.; Fuentes, I. Exploring the unification of quantum theory and general relativity with a Bose-Einstein condensate. New J. Phys. 2019, 21, 043047. [Google Scholar] [CrossRef]
- Jain, P.; Weinfurtner, S.; Visser, M.; Gardiner, C.W. Analog model of a Friedmann-Robertson-Walker universe in Bose-Einstein condensates: Application of the classical field method. Phys. Rev. A 2007, 76, 033616. [Google Scholar] [CrossRef]
- Sindoni, L.; Girelli, F.; Liberati, S. Emergent Gravitational Dynamics in Bose-Einstein Condensates. AIP Conf. Proc. 2009, 1196, 258–265. [Google Scholar] [CrossRef]
- Unruh, W. Experimental Black Hole Evaporation. Phys. Rev. Lett. 1981, 46, 1351. [Google Scholar] [CrossRef]
- Balbinot, R.; Fabbri, A.; Fagnocchi, S.; Parentani, R. Non-local density correlations as a signal of Hawking radiation in BEC acoustic black holes. Phys. Rev. 2008, 78, 024035. [Google Scholar] [CrossRef]
- Finazzi, S.; Parentani, R. Black-hole lasers in Bose-Einstein condensates. New J. Phys. 2010, 12, 095015. [Google Scholar] [CrossRef]
- Carusotto, I.; Fagnocchi, S.; Recati, A.; Balbinot, R.; Fabbri, A. Numerical observation of Hawking radiation from acoustic black holes in atomic Bose–Einstein condensates. New J. Phys. 2008, 10, 103001. [Google Scholar] [CrossRef]
- Parola, A.; Tettamanti, M.; Cacciatori, S.L. Analogue Hawking radiation in an exactly solvable model of BEC. EPL Europhys. Lett. 2017, 119, 50002. [Google Scholar] [CrossRef][Green Version]
- Kolobov, V.I.; Golubkov, K.; Muñoz de Nova, J.R.; Steinhauer, J. Spontaneous Hawking Radiation and Beyond: Observing the Time Evolution of an Analogue Black Hole. arXiv 2019, arXiv:1910.10677. [Google Scholar]
- Kovtun, P.K.; Son, D.T.; Starinets, A.O. Viscosity in strongly interacting quantum field theories from black hole physics. Phys. Rev. Lett. 2005, 94, 111601. [Google Scholar] [CrossRef] [PubMed]
- Brout, R.; Massar, S.; Parentani, R.; Spindel, P. A primer for black hole quantum physics. Phys. Rep. 1995, 260, 329. [Google Scholar] [CrossRef]
- Corley, S. Computing the spectrum of black hole radiation in the presence of high-frequency dispersion: An analytical approach. Phys. Rev. D 1998, 57, 6280. [Google Scholar] [CrossRef]
- Saida, H.; Sakagami, M.A. Black hole radiation with high-frequency dispersion. Phys. Rev. D 2000, 61, 084023. [Google Scholar] [CrossRef]
- Himemoto, Y.; Tanaka, T. Generalization of the model of Hawking radiation with modified high-frequency dispersion relation. Phys. Rev. D 2000, 61, 064004. [Google Scholar] [CrossRef]
- Unruh, W.G.; Schutzhold, R. On the universality of the Hawking effect. Phys. Rev. D 2005, 71, 024028. [Google Scholar] [CrossRef]
- Balbinot, R.; Fabbri, A.; Fagnocchi, S.; Parentani, R. Hawking radiation from acoustic black holes, short distance and back-reaction effects. Riv. Del Nuovo C. 2005, 28, 1. [Google Scholar]
- Lindquist, R.W. Relativistic transport theory. Ann. Phys. 1966, 37, 487. [Google Scholar] [CrossRef]
- Stewart, J. Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 1969; Volume 10. [Google Scholar]
- Bardeen, J.M.; Carter, B.; Hawking, S.W. The four laws of black hole mechanics. Commun. Math. Phys. 1973, 31, 161. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Black holes and entropy. Phys. Rev. D 1973, 7, 2333. [Google Scholar] [CrossRef]
- Jacobson, T. Black Hole Entropy and Induced Gravity. arXiv 1994, arXiv:gr-qc/9404039. [Google Scholar]
- Liberati, S.; Tricella, G.; Trombettoni, A. The Information Loss Problem: An Analogue Gravity Perspective. Entropy 2019, 21, 940. [Google Scholar] [CrossRef]
- Chirco, G.; Liberati, S. Non-equilibrium thermodynamics of spacetime: The role of gravitational dissipation. Phys. Rev. D 2010, 81, 024016. [Google Scholar] [CrossRef]
- Chirco, G.; Eling, C.; Liberati, S. The universal viscosity to entropy density ratio from entanglement. Phys. Rev. D 2010, 82, 024010. [Google Scholar] [CrossRef]
- Penrose, R. Gravitational collapse and space-time singularities. Phys. Rev. Lett. 1965, 14, 57–59. [Google Scholar] [CrossRef]
- Shuryak, E. Strongly coupled quark-gluon plasma in heavy ion collisions. Rev. Mod. Phys. 2017, 89, 035001. [Google Scholar] [CrossRef]
- Meyer, H.B. Calculation of the Bulk Viscosity in SU(3) Gluodynamics. Phys. Rev. Lett. 2008, 100, 162001. [Google Scholar] [CrossRef] [PubMed]
- Romatschke, P. Shear Viscosity at Infinite Coupling: A Field Theory Calculation. Phys. Rev. Lett. 2021, 127, 111603. [Google Scholar] [CrossRef]
- Rais, J.; Gallmeister, K.; Greiner, C. Shear viscosity to entropy density ratio of Hagedorn states. Phys. Rev. D 2020, 102, 036009. [Google Scholar] [CrossRef]
- Enss, T.; Haussmann, R.; Zwerger, W. Viscosity and scale invariance in the unitary Fermi gas. Ann. Phys. 2011, 326, 770–796. [Google Scholar] [CrossRef]
- Chiofalo, M.L.; Grasso, D.; Mannarelli, M.; Trabucco, S. Dissipative processes at the acoustic horizon. arXiv 2022, arXiv:2202.13790. [Google Scholar] [CrossRef]
- Chiofalo, M.L.; Tosi, M.P. Time-dependent density-functional theory for superfluids. Europhys. Lett. 2001, 53, 162. [Google Scholar] [CrossRef]
- Chiofalo, M.; Minguzzi, A.; Tosi, M. Time-dependent linear response of an inhomogeneous Bose superfluid: Microscopic theory and connection to current-density functional theory. Phys. Condens. Matter 1998, 254, 188–201. [Google Scholar] [CrossRef][Green Version]
- Iadonisi, G.; Cantele, G.; Chiofalo, M.L. Introduction to Solid State Physics and Crystalline Nanostructures; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Arndt, M.; Hornberger, K. Testing the Limits of Quantum Mechanical Superpositions. Nat. Phys. 2014, 10, 271–277. [Google Scholar] [CrossRef]
- Fein, Y.Y.; Geyer, P.; Zwick, P.; Kiałka, F.; Pedalino, S.; Mayor, M.; Gerlich, S.; Arndt, M. Quantum Superposition of Molecules beyond 25 kDa. Nat. Phys. 2019, 15, 1242–1245. [Google Scholar] [CrossRef]
- Isham, C.J. Canonical Quantum Gravity and the Problem of Time. In Integrable Systems, Quantum Groups, and Quantum Field Theories; Springer: Berlin/Heidelberg, Germany, 1993; pp. 157–287. [Google Scholar]
- Page, D.N.; Wootters, W.K. Evolution without evolution: Dynamics described by stationary observables. Phys. Rev. D 1983, 27, 2885–2892. [Google Scholar] [CrossRef]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Quantum time. Phys. Rev. D 2015, 92, 045033. [Google Scholar] [CrossRef]
- Castro-Ruiz, E.; Giacomini, F.; Belenchia, A.; Brukner, Č. Quantum clocks and the temporal localisability of events in the presence of gravitating quantum systems. Nat. Commun. 2020, 11, 2672. [Google Scholar] [CrossRef]
- Castro-Ruiz, E.; Giacomini, F.; Brukner, Č. Entanglement of quantum clocks through gravity. Proc. Natl. Acad. Sci. USA 2017, 114, E2303–E2309. [Google Scholar] [CrossRef] [PubMed]
- Foti, C.; Coppo, A.; Barni, G.; Cuccoli, A.; Verrucchi, P. Time and classical equations of motion from quantum entanglement via the Page and Wootters mechanism with generalized coherent states. Nat. Commun. 2021, 12, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Adler, S. Quantum Theory as an Emergent Phenomenon; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Leggett, A.J. The Quantum Measurement Problem. Science 2005, 307, 871–872. [Google Scholar] [CrossRef] [PubMed]
- Weinberg, S. The Trouble with Quantum Mechanics; The New York Review of Books: New York, NY, USA, 2017. [Google Scholar]
- Schlosshauer, M. Quantum decoherence. Phys. Rep. 2019, 831, 1–57. [Google Scholar] [CrossRef]
- Bohm, D. A suggested interpretation of the quantum theory in terms of “hidden” variables. i. Phys. Rev. 1952, 85, 166. [Google Scholar] [CrossRef]
- Walleczek, J.; Grössing, G.; Pylkkänen, P. Emergent Quantum Mechanics: David Bohm Centennial Perspectives; MDPI: Basel, Switzerland, 2019. [Google Scholar]
- Griffiths, R.B. Consistent histories and the interpretation of quantum mechanics. J. Stat. Phys. 1984, 36, 219–272. [Google Scholar] [CrossRef]
- Everett III, H. “Relative state” formulation of quantum mechanics. Rev. Mod. Phys. 1957, 29, 454. [Google Scholar] [CrossRef]
- Diosi, L. A Universal Master Equation for the Gravitational Violation of Quantum Mechanics. Phys. Lett. A 1987, 120, 377–381. [Google Scholar] [CrossRef]
- Penrose, R. On the Gravitization of Quantum Mechanics 1: Quantum State Reduction. Found. Phys. 2014, 44, 557–575. [Google Scholar] [CrossRef]
- Bassi, A.; Lochan, K.; Satin, S.; Singh, T.P.; Ulbricht, H. Models of Wave-Function Collapse, Underlying Theories, and Experimental Tests. Rev. Mod. Phys. 2013, 85, 471–527. [Google Scholar] [CrossRef]
- Adler, S.L.; Bassi, A. Is Quantum Theory Exact? Science 2009, 325, 275–276. [Google Scholar] [CrossRef] [PubMed]
- Riedinger, R.; Wallucks, A.; Marinković, I.; Löffler, W.; Aspelmeyer, M.; Hammerer, K. Remote quantum entanglement between two micromechanical oscillators. Nature 2018, 556, 473. [Google Scholar] [CrossRef]
- Ockeloen-Korppi, C.F.; Damskägg, E.; Pirkkalainen, J.M.; Clerk, A.A.; Massel, F.; Sillanpää, M.A. Stabilized entanglement of massive mechanical oscillators. Nature 2018, 556, 478. [Google Scholar] [CrossRef] [PubMed]
- Marinković, I.; Wallucks, A.; Milburn, G.J.; Aspelmeyer, M.; Hammerer, K. Optomechanical Bell test. Phys. Rev. Lett. 2018, 121, 220404. [Google Scholar] [CrossRef]
- Friedman, J.R.; Patel, V.; Chen, W.; Tolpygo, S.K.; Lukens, J.E. Quantum superposition of distinct macroscopic states. Nature 2000, 406, 43–46. [Google Scholar] [CrossRef] [PubMed]
- Andrews, M.R.; Townsend, C.G.; Miesner, H.J.; Durfee, D.S.; Kurn, D.M.; Ketterle, W. Observation of interference between two Bose condensates. Science 1997, 275, 637–641. [Google Scholar] [CrossRef]
- Berrada, T.; van Frank, S.; Bücker, R.; Schumm, T.; Schmiedmayer, J. Integrated Mach–Zehnder interferometer for Bose–Einstein condensates. Nat. Commun. 2013, 4, 2077. [Google Scholar] [CrossRef] [PubMed]
- Xu, M.; Jäger, S.B.; Schütz, S.; Cooper, J.; Morigi, G.; Holland, M. Supercooling of Atoms in an Optical Resonator. Phys. Rev. Lett. 2016, 116, 153002. [Google Scholar] [CrossRef] [PubMed]
- Brand, C.; Kiałka, F.; Troyer, M.; Mayor, M. Bragg diffraction of large organic molecules. Phys. Rev. Lett. 2020, 125, 033604. [Google Scholar] [CrossRef] [PubMed]
- Nimmrichter, S.; Hornberger, K. Macroscopicity of mechanical quantum superposition states. Phys. Rev. Lett. 2013, 110, 160403. [Google Scholar] [CrossRef] [PubMed]
- Pearle, P. Combining Stochastic Dynamical State-Vector Reduction with Spontaneous Localization. Phys. Rev. A 1989, 39, 2277–2289. [Google Scholar] [CrossRef] [PubMed]
- Ghirardi, G.C.; Pearle, P.; Rimini, A. Markov Processes in Hilbert Space and Continuous Spontaneous Localization of Systems of Identical Particles. Phys. Rev. A 1990, 42, 78. [Google Scholar] [CrossRef] [PubMed]
- Ghirardi, G.C.; Rimini, A.; Weber, T. A Unified Dynamics for Micro and MACRO Systems. Phys. Rev. D 1986, 34, 470. [Google Scholar] [CrossRef] [PubMed]
- Adler, S.L. Lower and Upper Bounds on CSL Parameters from Latent Image Formation and IGM Heating. J. Phys. A 2007, 40, 2935. [Google Scholar] [CrossRef]
- Belli, S.; Bonsignori, R.; D’Auria, G.; Fant, L.; Martini, M.; Peirone, S.; Donadi, S.; Bassi, A. Entangling Macroscopic Diamonds at Room Temperature: Bounds on the Continuous-Spontaneous-Localization Parameters. Phys. Rev. A 2016, 94, 012108. [Google Scholar] [CrossRef]
- Toroš, M.; Gasbarri, G.; Bassi, A. Colored and Dissipative Continuous Spontaneous Localization Model and Bounds from Matter-Wave Interferometry. Phys. Lett. A 2017, 381, 3921–3927. [Google Scholar] [CrossRef]
- Carlesso, M.; Bassi, A.; Falferi, P.; Vinante, A. Experimental Bounds on Collapse Models from Gravitational Wave Detectors. Phys. Rev. D 2016, 94, 124036. [Google Scholar] [CrossRef]
- Vinante, A.; Mezzena, R.; Falferi, P.; Carlesso, M.; Bassi, A. Improved Noninterferometric Test of Collapse Models Using Ultracold Cantilevers. Phys. Rev. Lett. 2017, 119, 110401. [Google Scholar] [CrossRef] [PubMed]
- Adler, S.L.; Bassi, A.; Carlesso, M.; Vinante, A. Testing Continuous Spontaneous Localization with Fermi Liquids. Phys. Rev. D 2019, 99, 103001. [Google Scholar] [CrossRef]
- Vinante, A.; Carlesso, M.; Bassi, A.; Chiasera, A.; Varas, S.; Falferi, P.; Margesin, B.; Mezzena, R.; Ulbricht, H. Narrowing the Parameter Space of Collapse Models with Ultracold Layered Force Sensors. Phys. Rev. Lett. 2020, 125, 100404. [Google Scholar] [CrossRef] [PubMed]
- Donadi, S.; Piscicchia, K.; Grande, R.D.; Curceanu, C.; Laubenstein, M.; Bassi, A. Novel CSL Bounds from the Noise-Induced Radiation Emission from Atoms. Eur. Phys. J. C 2021, 81, 1–10. [Google Scholar] [CrossRef]
- Bilardello, M.; Donadi, S.; Vinante, A.; Bassi, A. Bounds on Collapse Models from Cold-Atom Experiments. Phys. A 2016, 462, 764–782. [Google Scholar] [CrossRef]
- Helou, B.; Slagmolen, B.J.J.; McClelland, D.E.; Chen, Y. LISA Pathfinder Appreciably Constrains Collapse Models. Phys. Rev. D 2017, 95, 084054. [Google Scholar] [CrossRef]
- Carlesso, M.; Donadi, S.; Ferialdi, L.; Paternostro, M.; Ulbricht, H.; Bassi, A. Present Status and Future Challenges of Non-Interferometric Tests of Collapse Models. Nat. Phys. 2022, 18, 243–250. [Google Scholar] [CrossRef]
- Vinante, A.; Ulbricht, H. Gravity-Related Collapse of the Wave Function and Spontaneous Heating: Revisiting the Experimental Bounds. AVS Quantum Sci. 2021, 3, 045602. [Google Scholar] [CrossRef]
- Howl, R.; Milburn, G.J. Penrose–Fuentes gravitationally-induced wave-function collapse. New J. Phys. 2019, 21, 043047. [Google Scholar] [CrossRef]
- Belenchia, A.; Carlesso, M.; Donadi, S.; Gasbarri, G.; Ulbricht, C.; Bassi, A.; Paternostro, M. Test Quantum Mechanics in Space—Invest US $1 Billion. Nature 2021, 596, 32–34. [Google Scholar] [CrossRef]
- Kaltenbaek, R.; Aspelmeyer, M.; Barker, P.F.; Bassi, A.; Bateman, J.; Bongs, K.; Bose, S.; Braxmaier, C.; Brukner, C.; Christophe, B.; et al. Macroscopic Quantum Resonators (MAQRO): 2015 Update. EPJ Quantum Technol. 2016, 3, 1–47. [Google Scholar] [CrossRef]
- O’Brien, T.E.; Streif, M.; Rubin, N.C.; Santagati, R.; Su, Y.; Huggins, W.J.; Goings, J.J.; Moll, N.; Kyoseva, E.; Degroote, M.; et al. Efficient quantum computation of molecular forces and other energy gradients. Phys. Rev. Res. 2022, 4, 043210. [Google Scholar] [CrossRef]
- Malone, F.D.; Parrish, R.M.; Welden, A.R.; Fox, T.; Degroote, M.; Kyoseva, E.; Moll, N.; Santagati, R.; Streif, M. Towards the simulation of large scale protein–ligand interactions on -era quantum computers. Chem. Sci. 2022, 13, 3094–3108. [Google Scholar] [CrossRef]
- Khrennikov, A. Ubiquitous Quantum Structure: From Psychology to Finances; Springer: Berlin, Switzerland, 2010. [Google Scholar] [CrossRef]
- Khrennikov, A. Order stability via Fröhlich condensation in bio, eco, and social systems: The quantum-like approach. Biosystems 2022, 212, 104593. [Google Scholar] [CrossRef]
- Jedlicka, P. Revisiting the Quantum Brain Hypothesis: Toward Quantum (Neuro)biology? Front. Mol. Neurosci. 2017, 10, 366. [Google Scholar] [CrossRef] [PubMed]
- Satinover, J. The Quantum Brain: The Search for Freedom and the Next Generation of Man; John Wiley & Sons: Hoboken, NJ, USA, 2001. [Google Scholar]
- Tononi, G.; Koch, C. Consciousness: Here, there and everywhere? Philos. Trans. R. Soc. Biol. Sci. 2015, 370, 20140167. [Google Scholar] [CrossRef] [PubMed]
- Barbosa, L.S.; Marshall, W.; Albantakis, L.; Tononi, G. Mechanism Integrated Information. Entropy 2021, 23, 362. [Google Scholar] [CrossRef] [PubMed]
- Sabbadini, S.A.; Vitiello, G. Entanglement and Phase-Mediated Correlations in Quantum Field Theory. Application to Brain-Mind States. Appl. Sci. 2019, 9, 3203. [Google Scholar] [CrossRef]
- Basieva, I.; Khrennikov, A.; Ozawa, M. Quantum-like modeling in biology with open quantum systems and instruments. Biosystems 2021, 201, 104328. [Google Scholar] [CrossRef] [PubMed]
- Li, J.A.; Dong, D.; Wei, Z.; Liu, Y.; Pan, Y.; Nori, F.; Zhang, X. Quantum reinforcement learning during human decision-making. Nat. Hum. Behav. 2020, 4, 294–307. [Google Scholar] [CrossRef] [PubMed]
- Knill, D.C.; Pouget, A. The Bayesian brain: The role of uncertainty in neural coding and computation. Trends Neurosci. 2004, 27, 712–719. [Google Scholar] [CrossRef]
- Friston, K. The free-energy principle: A unified brain theory? Nat. Rev. Neurosci. 2010, 11, 127–138. [Google Scholar] [CrossRef] [PubMed]
- Nasr, K.; Viswanathan, P.; Nieder, A. Number detectors spontaneously emerge in a deep neural network designed for visual object recognition. Sci. Adv. 2019, 5, eaav7903. [Google Scholar] [CrossRef] [PubMed]
- Kim, G.; Jang, J.; Baek, S.; Song, M.; Paik, S. Visual number sense in untrained deep neural networks. Sci. Adv. 2021, 7, 6127. [Google Scholar] [CrossRef] [PubMed]
- Dehaene, S. The neural basis of the Weber—Fechner law: A logarithmic mental number line. Trends Cogn. Sci. 2003, 7, 145. [Google Scholar] [CrossRef] [PubMed]
- Gallistel, C.R.; Gelman, R. Non-verbal numerical cognition: From reals to integers. Trends Cogn. Sci. 2000, 4, 59–65. [Google Scholar] [CrossRef] [PubMed]
- Burr, D.; Ross, J. A visual sense of number. Current Biol. 2008, 18, 425. [Google Scholar] [CrossRef] [PubMed]
- Yago Malo, J.; Cicchini, G.M.; Morrone, M.C.; Chiofalo, M.L. Quantum spin models for numerosity perception. PLoS ONE 2023, 18, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Santos, L.F.; Borgonovi, F.; Celardo, G.L. Cooperative Shielding in Many-Body Systems with Long-Range Interaction. Phys. Rev. Lett. 2016, 116, 250402. [Google Scholar] [CrossRef] [PubMed]
- Nandkishore, R.M.; Sondhi, S.L. Many-Body Localization with Long-Range Interactions. Phys. Rev. X 2017, 7, 041021. [Google Scholar] [CrossRef]
- Liu, F.; Lundgren, R.; Titum, P.; Pagano, G.; Zhang, J.; Monroe, C.; Gorshkov, A.V. Confined Quasiparticle Dynamics in Long-Range Interacting Quantum Spin Chains. Phys. Rev. Lett. 2019, 122, 150601. [Google Scholar] [CrossRef] [PubMed]
- Thomson, S.J.; Schiró, M. Quasi-many-body localization of interacting fermions with long-range couplings. Phys. Rev. Res. 2020, 2, 043368. [Google Scholar] [CrossRef]
- Bhakuni, D.S.; Santos, L.F.; Lev, Y.B. Suppression of heating by long-range interactions in periodically driven spin chains. Phys. Rev. B 2021, 104, L140301. [Google Scholar] [CrossRef]
- Celardo, G.L.; Giusteri, G.G.; Borgonovi, F. Cooperative robustness to static disorder: Superradiance and localization in a nanoscale ring to model light-harvesting systems found in nature. Phys. Rev. B 2014, 90, 075113. [Google Scholar] [CrossRef]
- Celardo, G.L.; Poli, P.; Lussardi, L.; Borgonovi, F. Cooperative robustness to dephasing: Single-exciton superradiance in a nanoscale ring to model natural light-harvesting systems. Phys. Rev. B 2014, 90, 085142. [Google Scholar] [CrossRef]
- Cornelius, J.; Xu, Z.; Saxena, A.; Chenu, A.; del Campo, A. Spectral Filtering Induced by Non-Hermitian Evolution with Balanced Gain and Loss: Enhancing Quantum Chaos. Phys. Rev. Lett. 2022, 128, 190402. [Google Scholar] [CrossRef] [PubMed]
- Vattay, G.; Kauffman, S.; Niiranen, S. Quantum Biology on the Edge of Quantum Chaos. PLoS ONE 2014, 9, e89017. [Google Scholar] [CrossRef] [PubMed]
- Altland, A.; Sonner, J. Late time physics of holographic quantum chaos. SciPost Phys. 2021, 11, 034. [Google Scholar] [CrossRef]
- Saxena, K.; Singh, P.; Sahoo, P.; Sahu, S.; Ghosh, S.; Ray, K.; Fujita, D.; Bandyopadhyay, A. Fractal, Scale Free Electromagnetic Resonance of a Single Brain Extracted Microtubule Nanowire, a Single Tubulin Protein and a Single Neuron. Fractal Fract. 2020, 4, 11. [Google Scholar] [CrossRef]
- Wilczek, F. Quantum Time Crystals. Phys. Rev. Lett. 2012, 109, 160401. [Google Scholar] [CrossRef]
- Witthaut, D.; Wimberger, S.; Burioni, R.; Timme, M. Classical synchronization indicates persistent entanglement in isolated quantum systems. Nat. Commun. 2017, 8, 14829. [Google Scholar] [CrossRef] [PubMed]
- Singh, P.; Saxena, K.; Singhania, A.; Sahoo, P.; Ghosh, S.; Chhajed, R.; Ray, K.; Fujita, D.; Bandyopadhyay, A. A Self-Operating Time Crystal Model of the Human Brain: Can We Replace Entire Brain Hardware with a 3D Fractal Architecture of Clocks Alone? Information 2020, 11, 238. [Google Scholar] [CrossRef]
- Panitchayangkoon, G.; Hayes, D.; Fransted, K.A.; Caram, J.R.; Harel, E.; Wen, J.; Blankenship, R.E.; Engel, G.S. Long-lived quantum coherence in photosynthetic complexes at physiological temperature. Proc. Natl. Acad. Sci. USA 2010, 107, 12766–12770. [Google Scholar] [CrossRef] [PubMed]
- Ball, P. Physics of life: The dawn of quantum biology. Nature 2011, 474, 272–274. [Google Scholar] [CrossRef] [PubMed]
- Fleming, G.R.; Scholes, G.D.; Cheng, Y.C. Quantum effects in biology. Procedia Chem. 2011, 3, 38–57. [Google Scholar] [CrossRef]
- Collini, E.; Wong, C.Y.; Wilk, K.E.; Curmi, P.M.G.; Brumer, P.; Scholes, G.D. Coherently wired light-harvesting in photosynthetic marine algae at ambient temperature. Nature 2010, 463, 644–647. [Google Scholar] [CrossRef] [PubMed]
- Fröhlich, H. Bose condensation of strongly excited longitudinal electric modes. Phys. Lett. A 1968, 26, 402–403. [Google Scholar] [CrossRef]
- Vasconcellos, Á.R.; Vannucchi, F.S.; Mascarenhas, S.; Luzzi, R. Fröhlich Condensate: Emergence of Synergetic Dissipative Structures in Information Processing Biological and Condensed Matter Systems. Information 2012, 3, 601–620. [Google Scholar] [CrossRef]
- Zhang, Z.; Agarwal, G.S.; Scully, M.O. Quantum Fluctuations in the Fröhlich Condensate of Molecular Vibrations Driven Far From Equilibrium. Phys. Rev. Lett. 2019, 122, 158101. [Google Scholar] [CrossRef] [PubMed]
- Biamonte, J.; Faccin, M.; De Domenico, M. Complex networks from classical to quantum. Commun. Phys. 2019, 2, 53. [Google Scholar] [CrossRef]
- Watts, D.J.; Strogatz, S.H. Collective dynamics of ‘small-world’networks. Nature 1998, 393, 440–442. [Google Scholar] [CrossRef]
- Barabási, A.L.; Albert, R. Emergence of Scaling in Random Networks. Science 1999, 286, 509–512. [Google Scholar] [CrossRef] [PubMed]
- Boccaletti, S.; Latora, V.; Moreno, Y.; Chavez, M.; Hwang, D.U. Complex networks: Structure and dynamics. Phys. Rep. 2006, 424, 175–308. [Google Scholar] [CrossRef]
- Cirac, J.I.; Zoller, P.; Kimble, H.J.; Mabuchi, H. Quantum State Transfer and Entanglement Distribution among Distant Nodes in a Quantum Network. Phys. Rev. Lett. 1997, 78, 3221–3224. [Google Scholar] [CrossRef]
- Ritter, S.; Nölleke, C.; Hahn, C.; Reiserer, A.; Neuzner, A.; Uphoff, M.; Mücke, M.; Figueroa, E.; Bochmann, J.; Rempe, G. An elementary quantum network of single atoms in optical cavities. Nature 2012, 484, 195–200. [Google Scholar] [CrossRef] [PubMed]
- De Domenico, M.; Biamonte, J. Spectral Entropies as Information-Theoretic Tools for Complex Network Comparison. Phys. Rev. X 2016, 6, 041062. [Google Scholar] [CrossRef]
- Valdez, M.A.; Jaschke, D.; Vargas, D.L.; Carr, L.D. Quantifying Complexity in Quantum Phase Transitions via Mutual Information Complex Networks. Phys. Rev. Lett. 2017, 119, 225301. [Google Scholar] [CrossRef] [PubMed]
- García-Pérez, G.; Rossi, M.A.C.; Sokolov, B.; Borrelli, E.M.; Maniscalco, S. Pairwise tomography networks for many-body quantum systems. Phys. Rev. Res. 2020, 2, 023393. [Google Scholar] [CrossRef]
- Kadian, K.; Garhwal, S.; Kumar, A. Quantum walk and its application domains: A systematic review. Comput. Sci. Rev. 2021, 41, 100419. [Google Scholar] [CrossRef]
- Whitfield, J.D.; Rodríguez-Rosario, C.A.; Aspuru-Guzik, A. Quantum stochastic walks: A generalization of classical random walks and quantum walks. Phys. Rev. A 2010, 81, 022323. [Google Scholar] [CrossRef]
- Rossi, M.A.C.; Benedetti, C.; Borrelli, M.; Maniscalco, S.; Paris, M.G.A. Continuous-time quantum walks on spatially correlated noisy lattices. Phys. Rev. A 2017, 96, 040301. [Google Scholar] [CrossRef]
- Kurt, A.; Rossi, M.A.C.; Piilo, J. Quantum transport efficiency in noisy random-removal and small-world networks. J. Phys. A Math. Theor. 2023, 56, 145301. [Google Scholar] [CrossRef]
- Burget, M.; Bardone, E.; Pedaste, M. Definitions and conceptual dimensions of responsible research and innovation: A literature review. Sci. Eng. Ethics 2017, 23, 1–19. [Google Scholar] [CrossRef] [PubMed]
- About RRI. Available online: https://www.rri-leaders.eu/about-rri/ (accessed on 30 June 2023).
- Shelley-Egan, C.; Gjefsen, M.D.; Nydal, R. Consolidating RRI and Open Science: Understanding the potential for transformative change. Life Sci. Soc. Policy 2020, 16, 7. [Google Scholar] [CrossRef] [PubMed]
- Groves, C. Review of RRI Tools Project. J. Responsible Innov. 2017, 4, 371–374. Available online: https://rri-tools.eu/ (accessed on 1 March 2023). [CrossRef]
- Kuzma, J.; Cummings, C.L. Cultural beliefs and stakeholder affiliation influence attitudes towards responsible research and innovation among United States stakeholders involved in biotechnology and gene editing. Front. Political Sci. 2021, 3, 677003. [Google Scholar] [CrossRef]
- Mackay, S.M.; Tan, E.W.; Warren, D.S. Developing a new generation of scientist communicators through effective public outreach. Commun. Chem. 2020, 3, 76. [Google Scholar] [CrossRef] [PubMed]
- Science outreach in the post-truth age. Nat. Nanotechnol. 2017, 12, 929. [CrossRef] [PubMed][Green Version]
- McCartney, M.; Childers, C.; Baiduc, R.R.; Barnicle, K. Annotated Primary Literature: A Professional Development Opportunity in Science Communication for Graduate Students and Postdocs. J. Microbiol. Biol. Educ. 2018, 19, 1–11. [Google Scholar] [CrossRef]
- Foti, C.; Anttila, D.; Maniscalco, S.; Chiofalo, M.L. Quantum Physics Literacy Aimed at K12 and the General Public. Universe 2021, 7, 86. [Google Scholar] [CrossRef]
- Schwab, J. The Teaching of Science as Enquiry; Simon and Schuster: New York, NY, USA, 1962. [Google Scholar]
- Bloomfield, L.A. How Things Work: The Physics of Everyday Life, 6th ed.; Wiley: Hoboken, NJ, USA, 2015. [Google Scholar]
- Bondani, M.; Chiofalo, M.L.; Ercolessi, E.; Macchiavello, C.; Malgieri, M.; Michelini, M.; Mishina, O.; Onorato, P.; Pallotta, F.; Satanassi, S.; et al. Introducing Quantum Technologies at Secondary School Level: Challenges and Potential Impact of an Online Extracurricular Course. Physics 2022, 4, 1150–1167. [Google Scholar] [CrossRef]
- Montagnani, S.; Stefanel, A.; Chiofalo, M.L.M.; Santi, L.; Michelini, M. An experiential program on the foundations of quantum mechanics for final-year high-school students. Phys. Educ. 2023, 58, 035003. [Google Scholar] [CrossRef]
- Chiofalo, M.L.; Michelini, M. An Interview with Marisa Michelini: IUPAP-ICPE Medal, Professor of Physics-Education Research at Udine University, GIREP President. Eurasia J. Math. Sci. Technol. Educ. 2023, 19, em2243. [Google Scholar] [CrossRef] [PubMed]
- Quantum Technology Education (QTEdu), Action of Quantum Flagship Initiative. Available online: https://qtedu.eu/ (accessed on 1 March 2023).
- DigiQ: Digitally Enhanced European Quantum Technology Master. 2022. Available online: https://www.digiq.eu/ (accessed on 1 February 2023).
- Quantum Technology Education for Everyone, QTEdu Pilot Project. Available online: https://qtedu.eu/project/quantum-technologies-education-everyone (accessed on 1 March 2023).
- Tseitlin, M.; Galili, I. Physics Teaching in the Search for Its Self. Sci. Educ. 2005, 14, 235–261. [Google Scholar] [CrossRef]
- Chiofalo, M.L.; Giudici, C.; Gardner, H. An Interview with Howard Gardner: John H. and Elisabeth A. Hobbs Research Professor of Cognition and Education at the Harvard Graduate School of Education. Eurasia J. Math. Sci. Technol. Educ. 2022, 18, em2112. [Google Scholar] [CrossRef] [PubMed]
- Seskir, Z.C.; Migdał, P.; Weidner, C.; Anupam, A.; Case, N.; Davis, N.; Decaroli, C.; Ercan, İ.; Foti, C.; Gora, P. Quantum games and interactive tools for quantum technologies outreach and education. Opt. Eng. 2022, 61, 081809. [Google Scholar] [CrossRef]
- Chiofalo, M.L.; Foti, C.; Michelini, M.; Santi, L.; Stefanel, A. Games for Teaching/Learning Quantum Mechanics: A Pilot Study with High-School Students. Educ. Sci. 2022, 12, 446. [Google Scholar] [CrossRef]
- Gentini, L.; Yago Malo, J.; Chiofalo, M. The Quantum Bit Woman: Promoting the Cultural Heritage with Quantum Games. In Cultural Physics Awareness and Education: The Challenge of Digitalization 2023; Springer Book Series Challenges in Physics Education; Bonivento, W., Michelini, M., Streit-Bianchi, M., Tuveri, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2023. [Google Scholar]



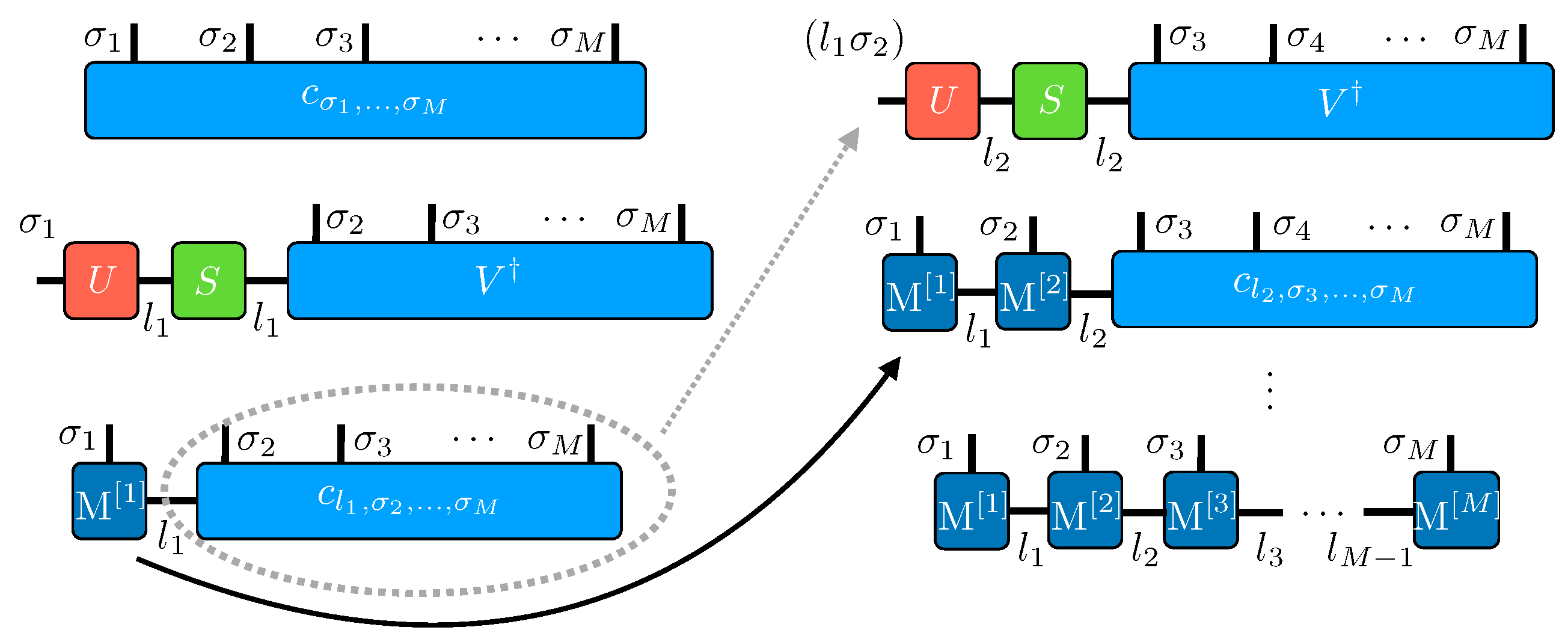

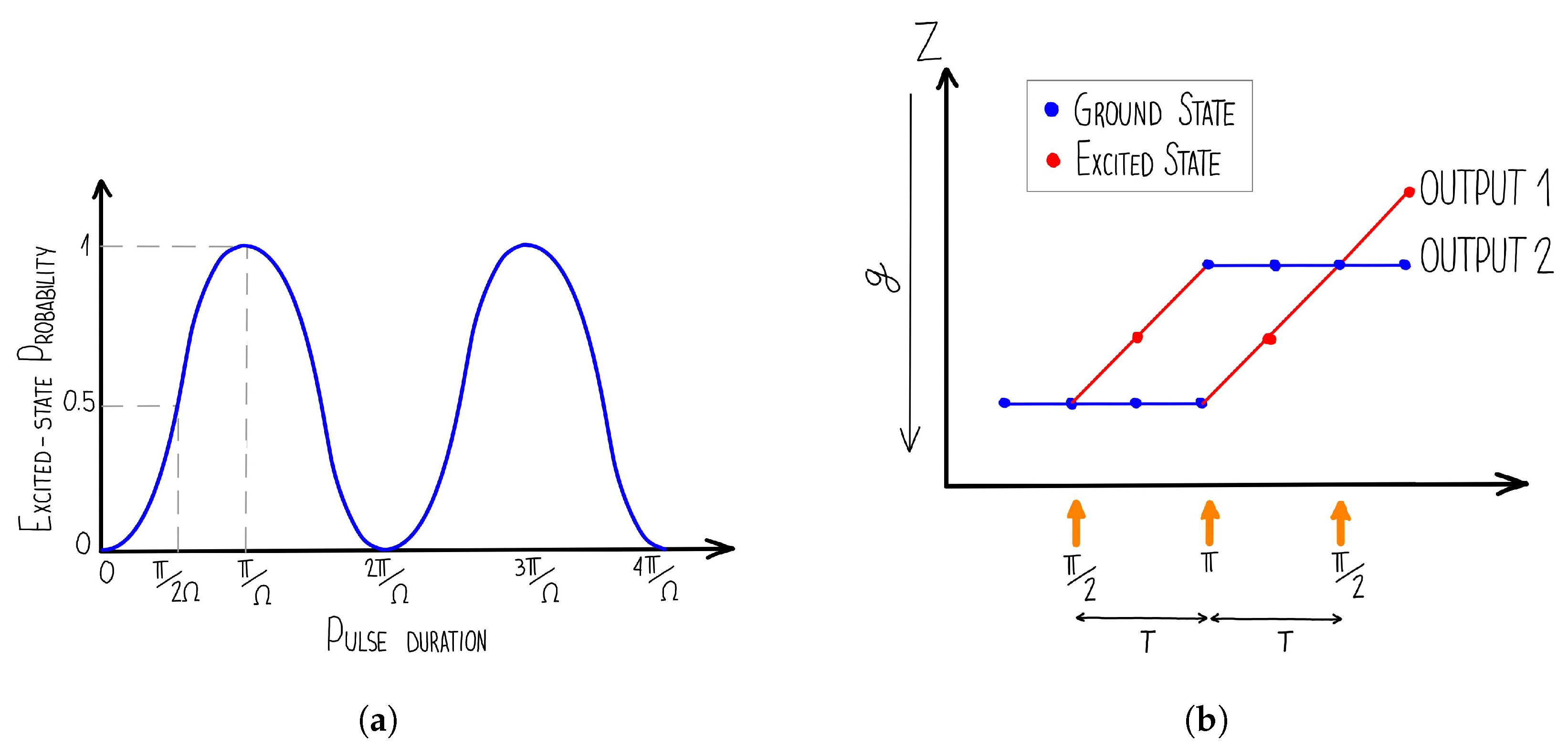

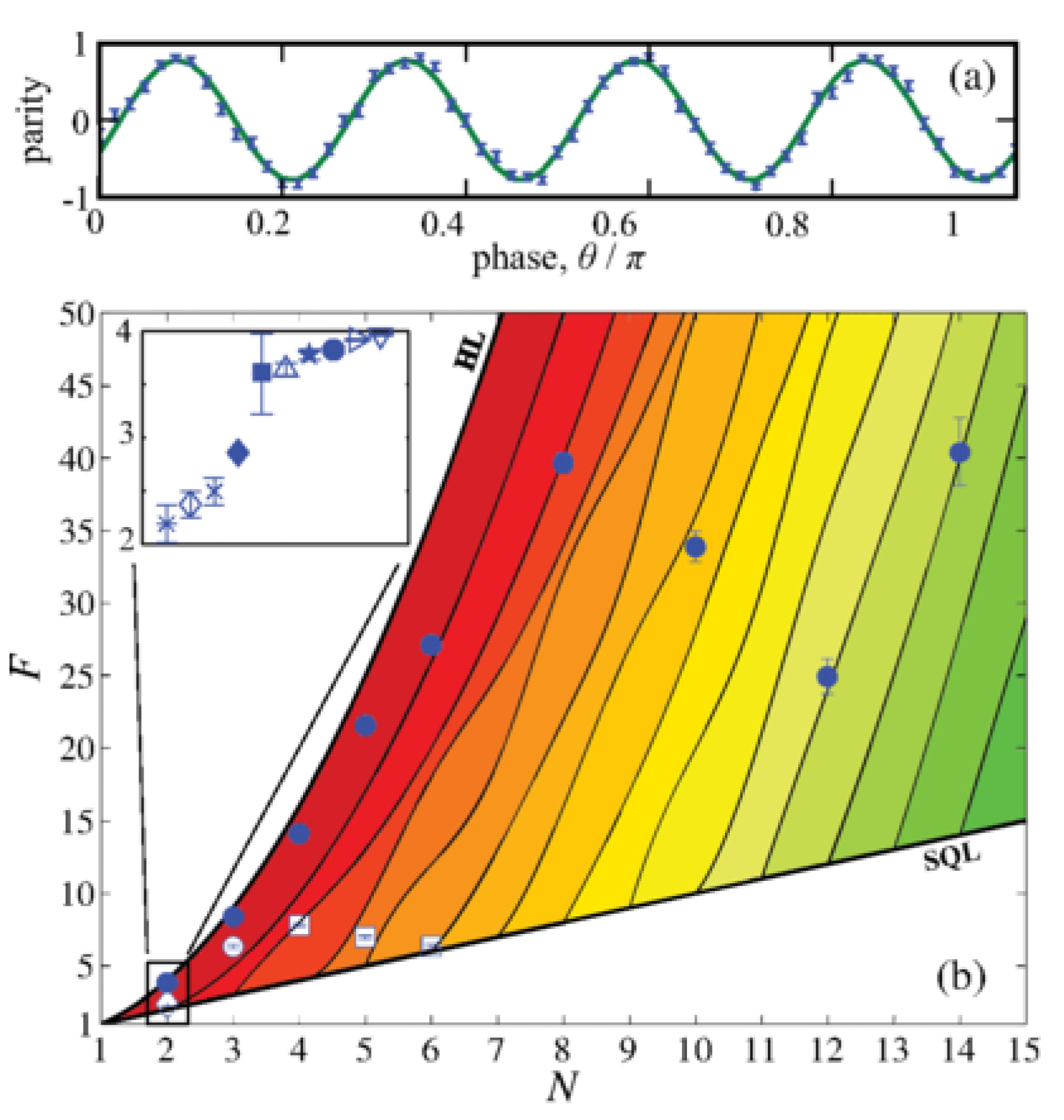
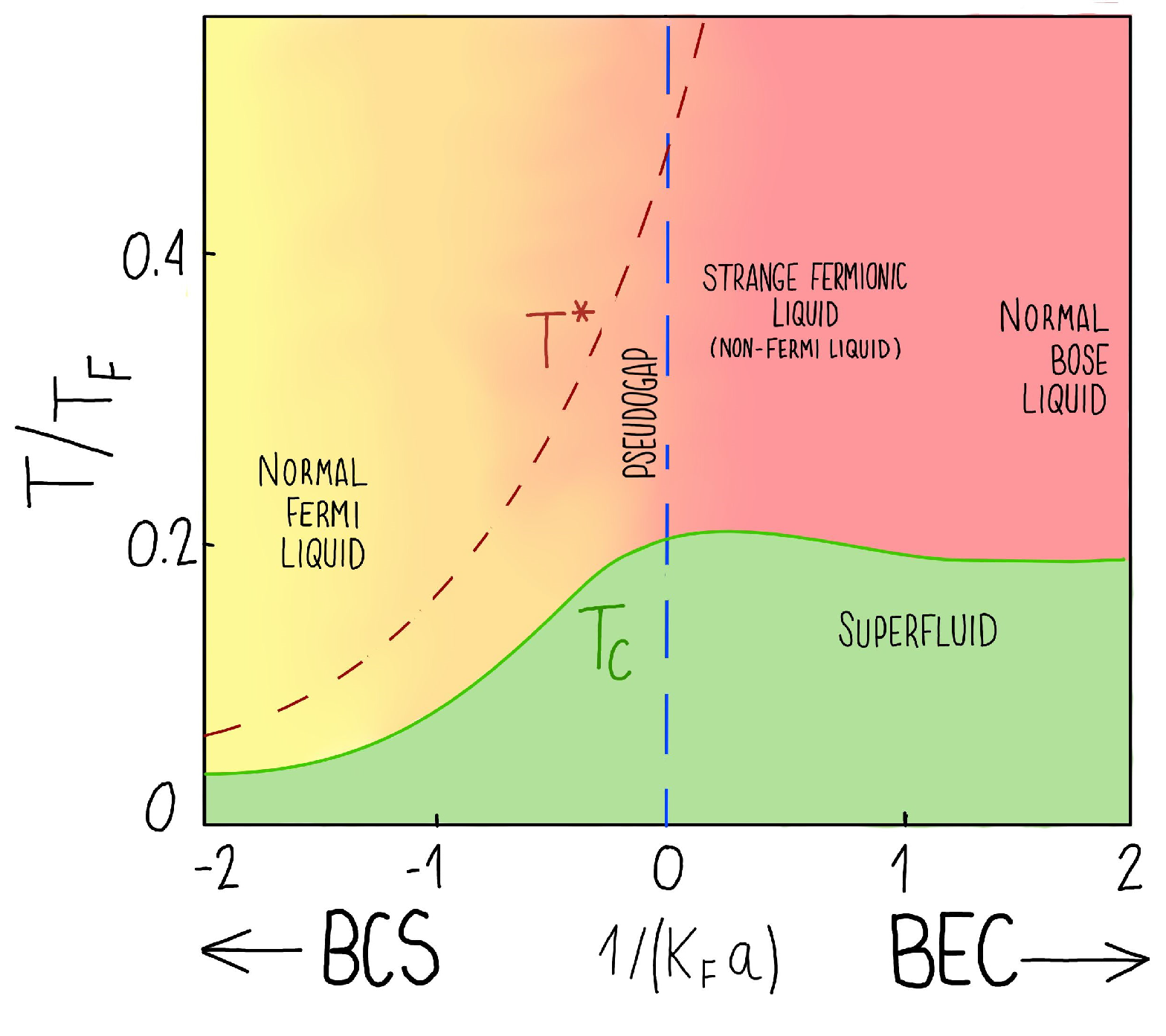

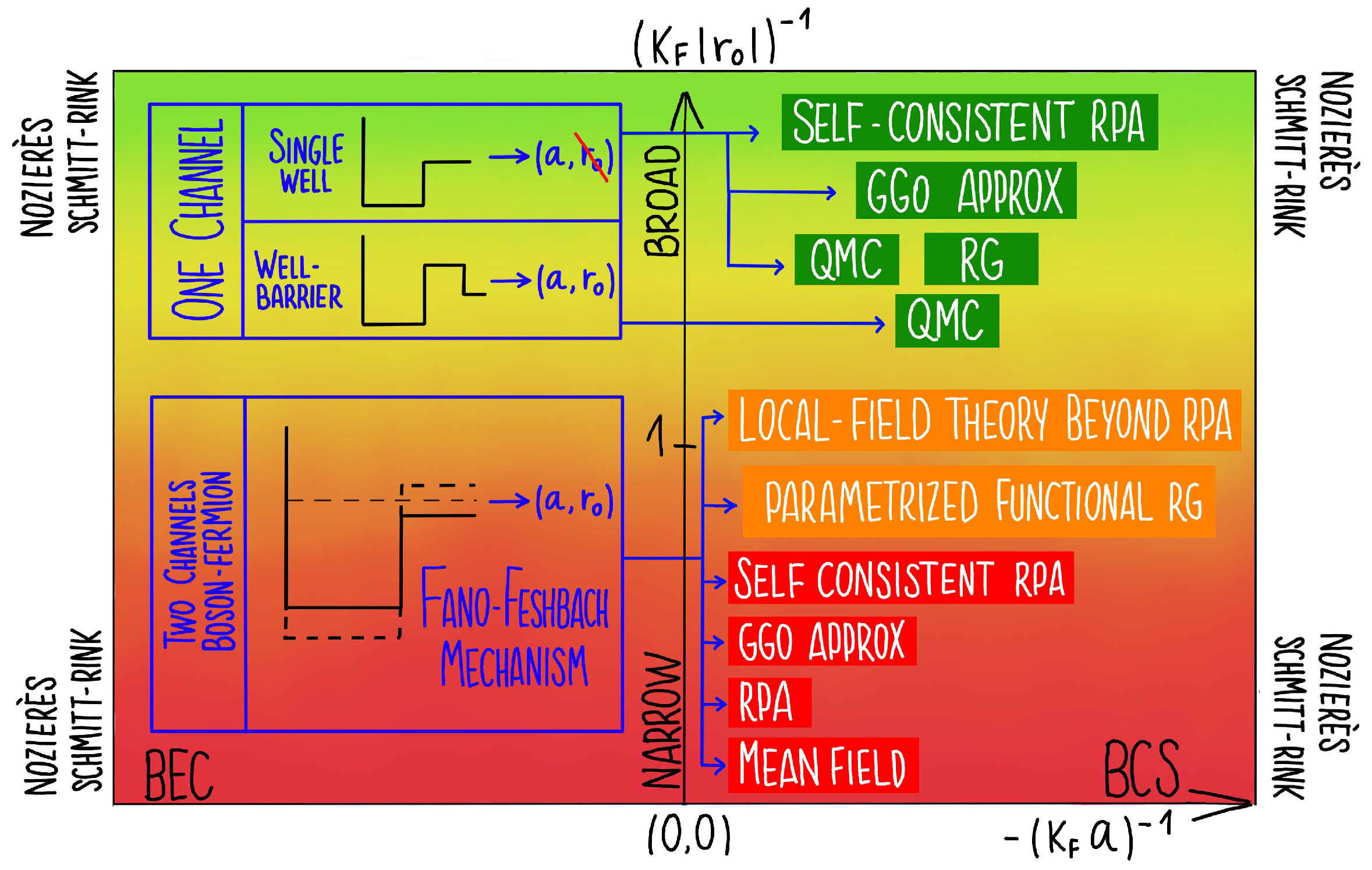
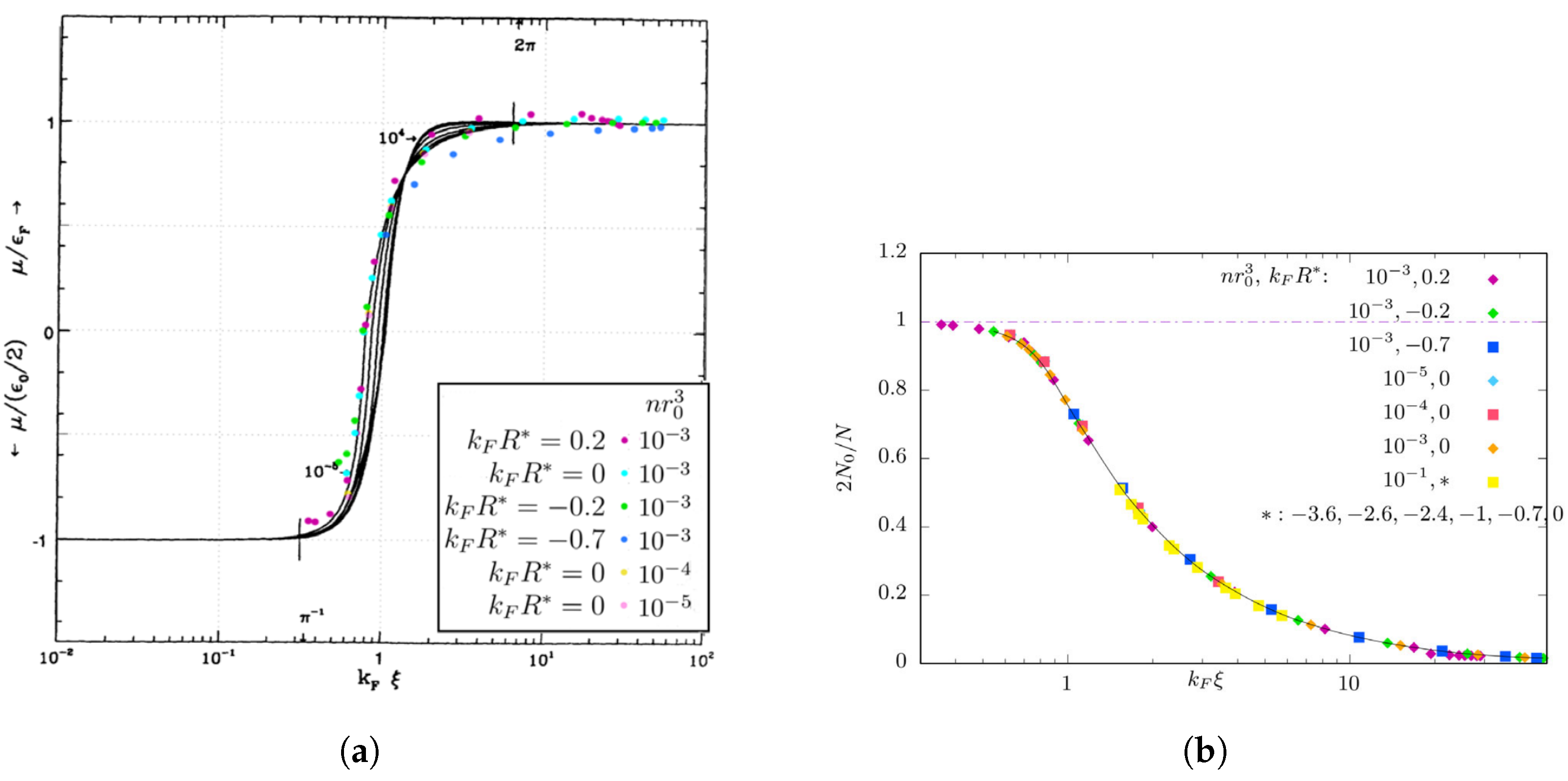
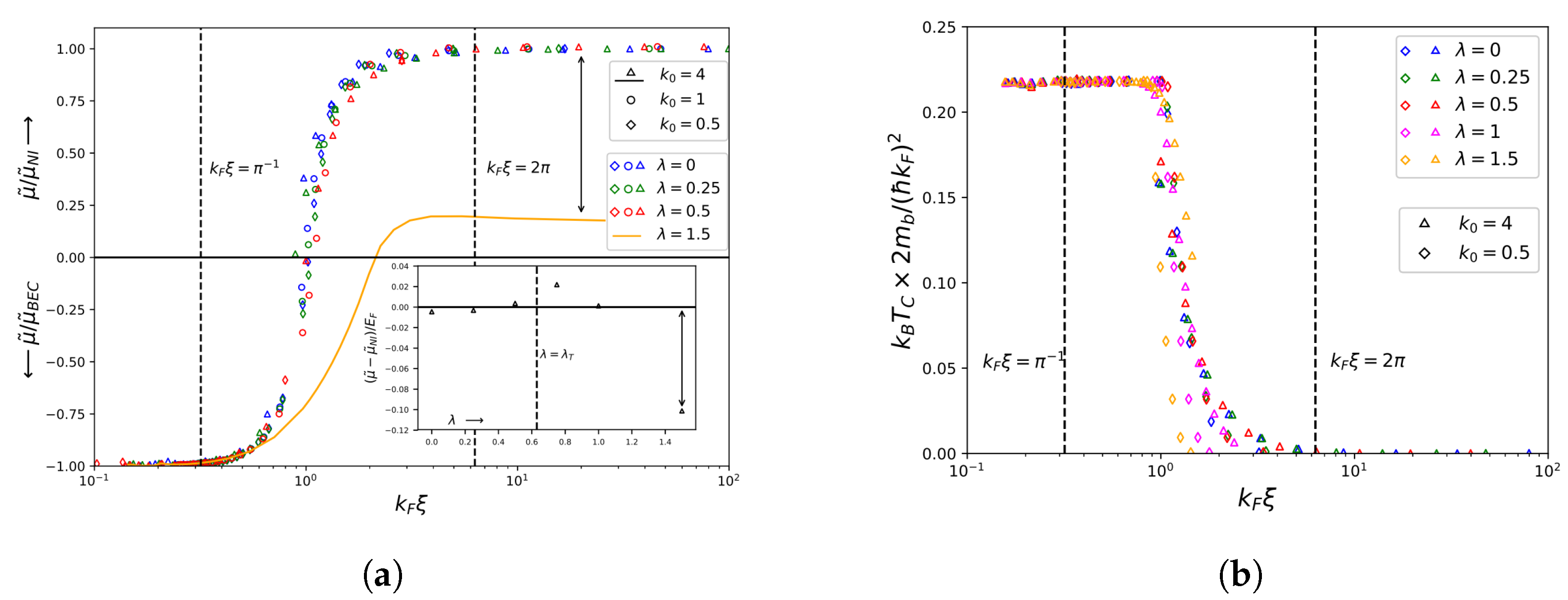
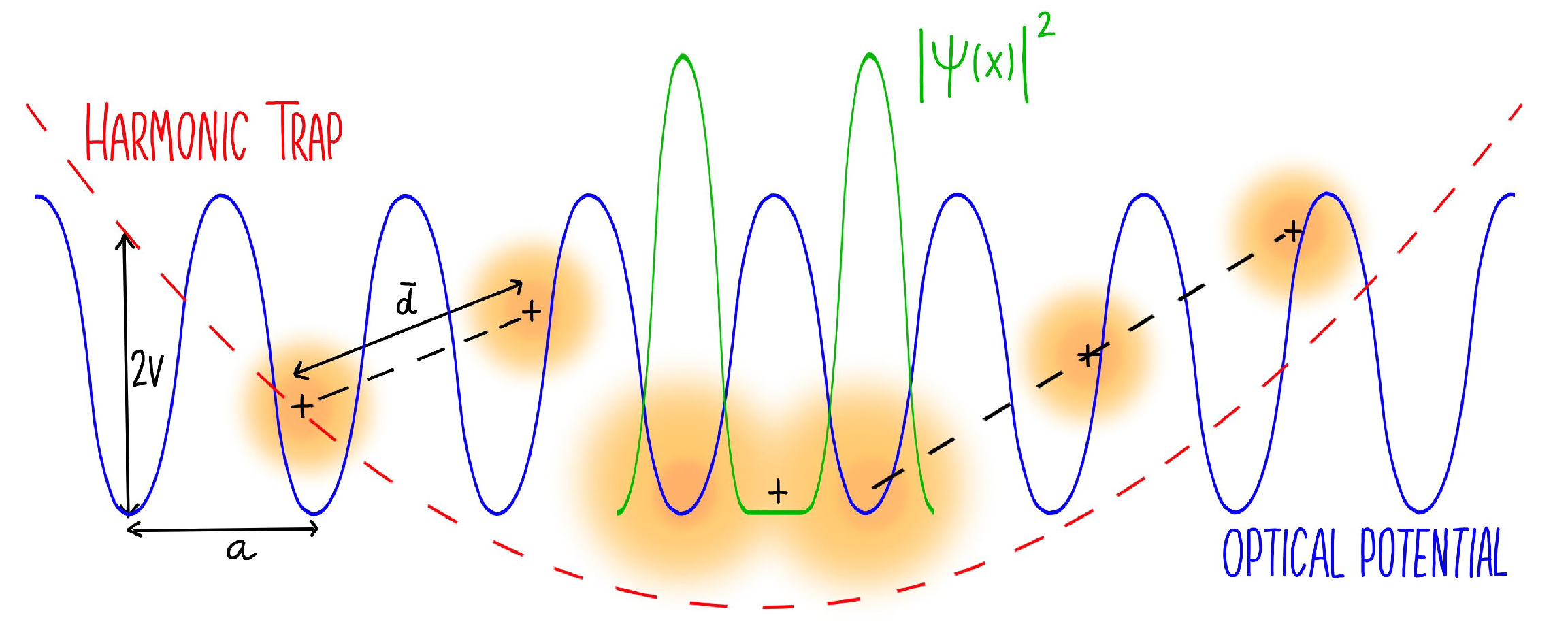


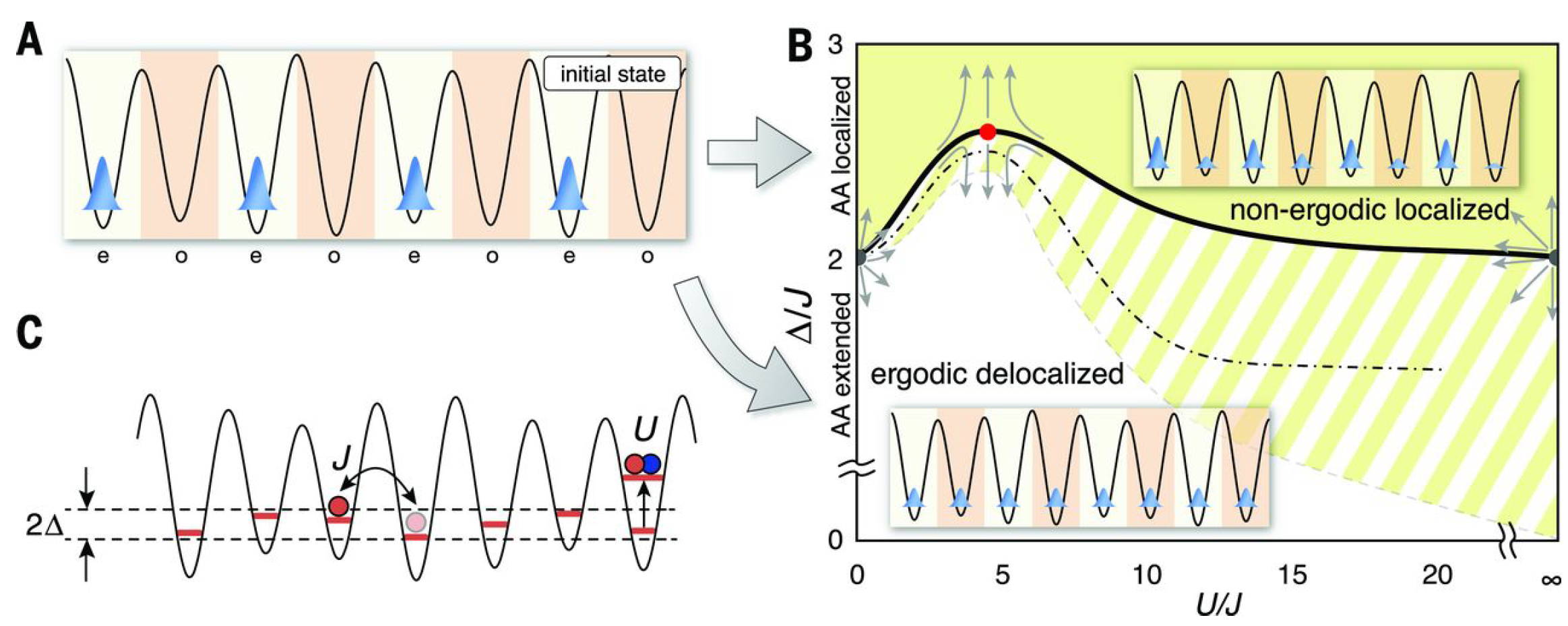



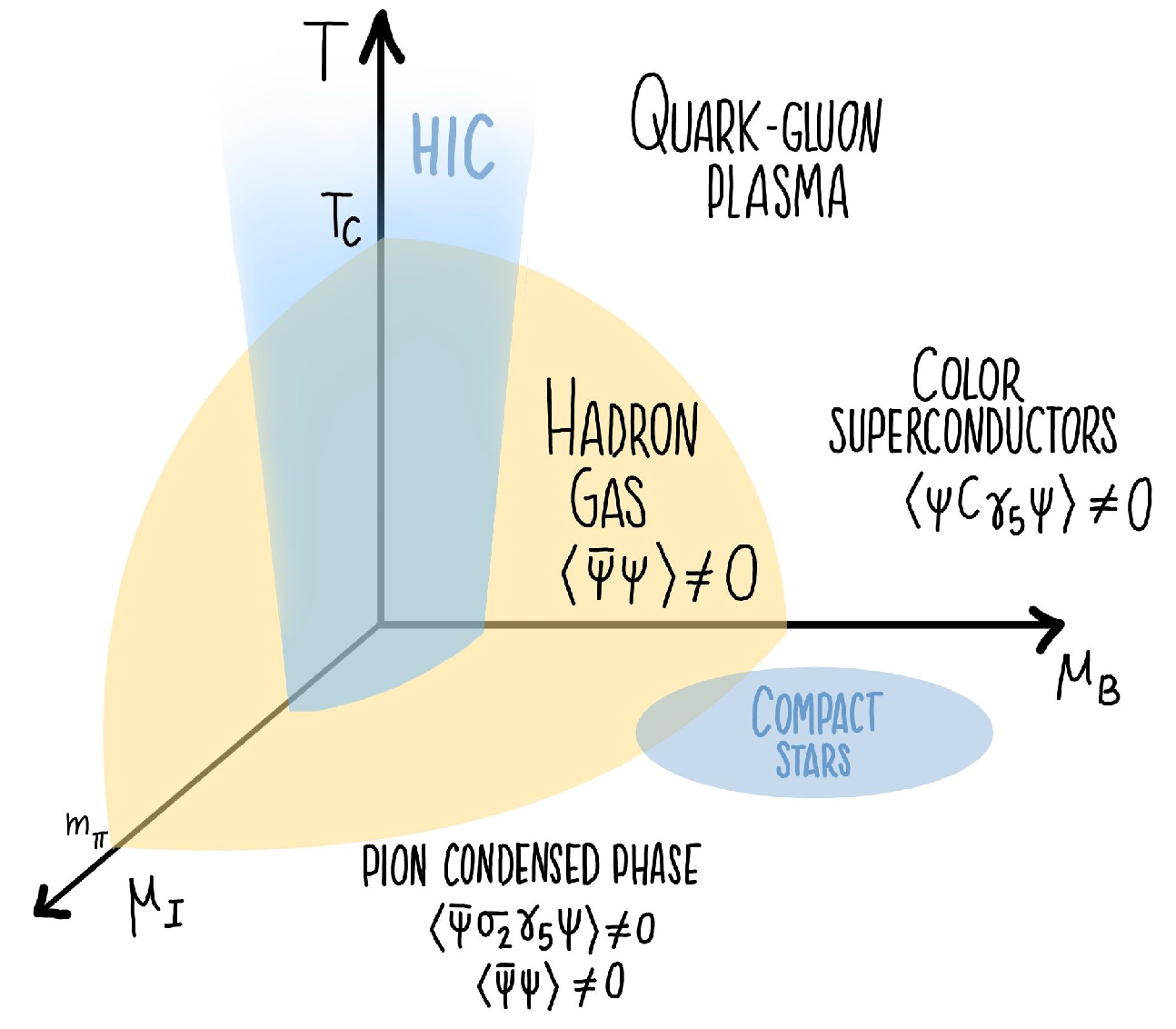
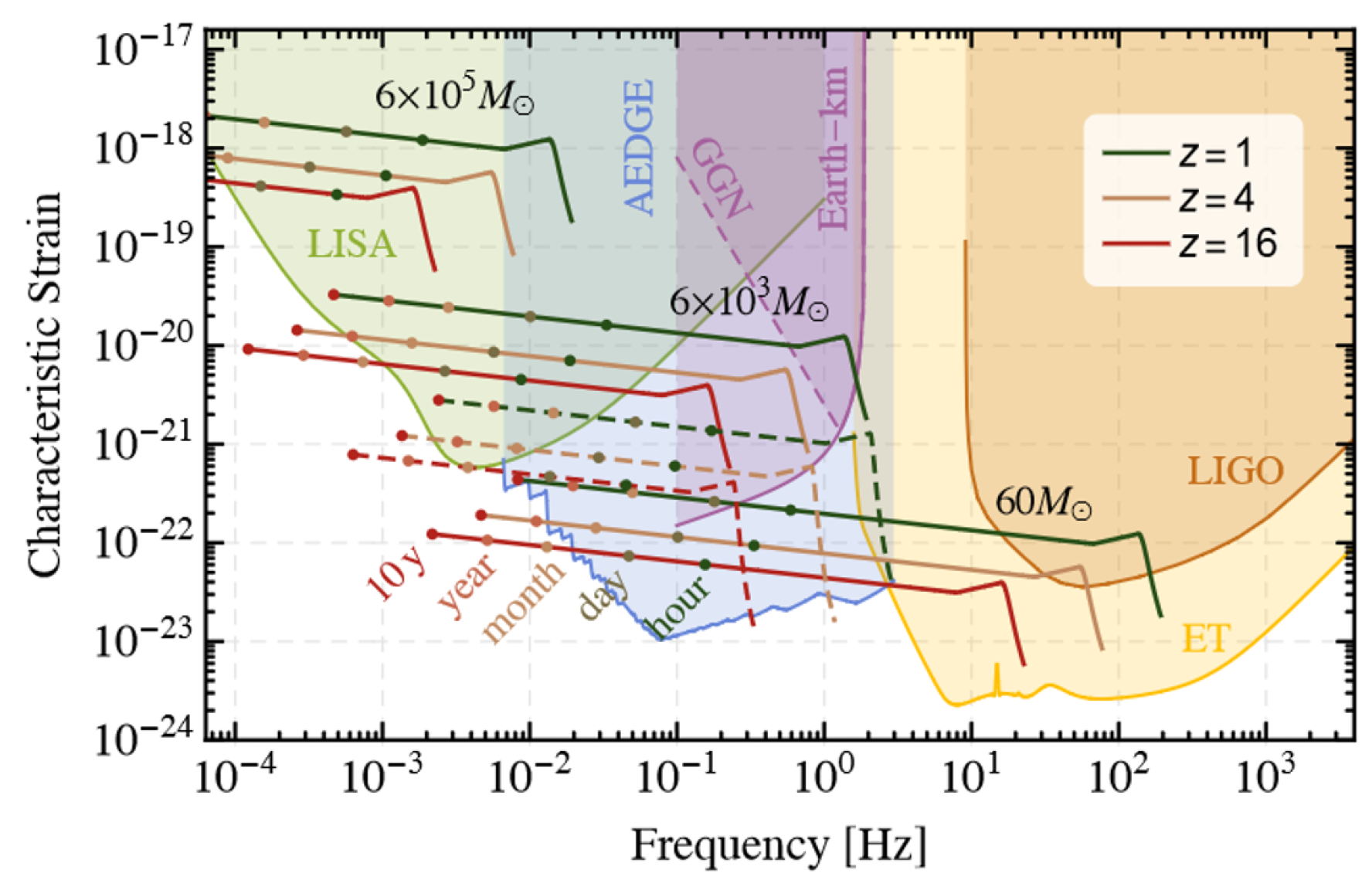
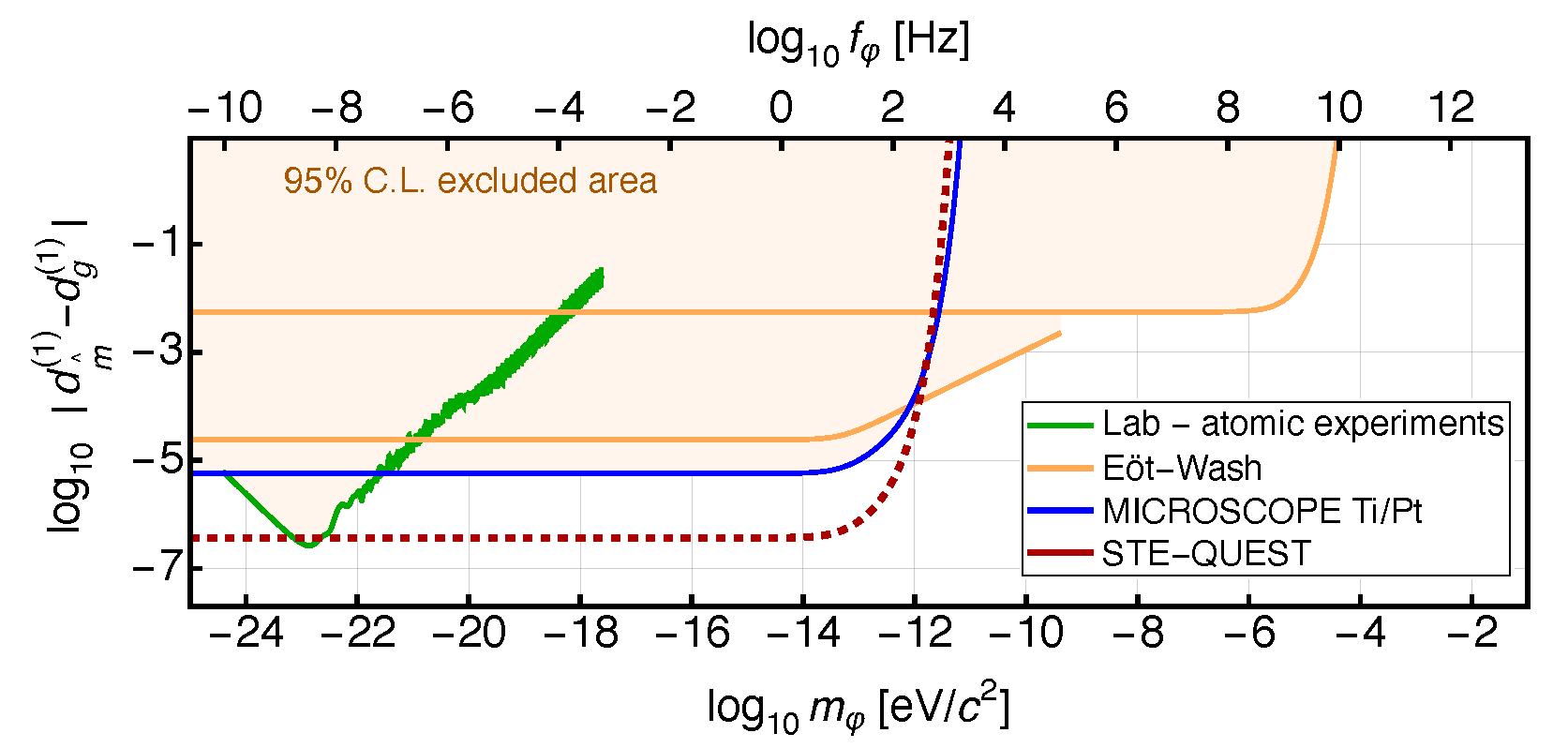
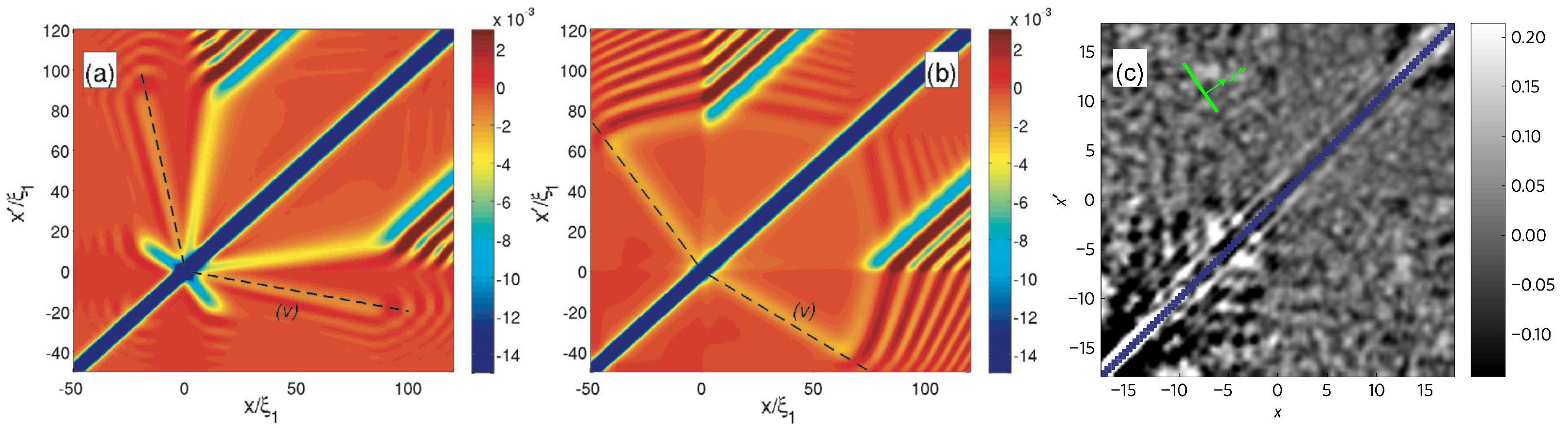
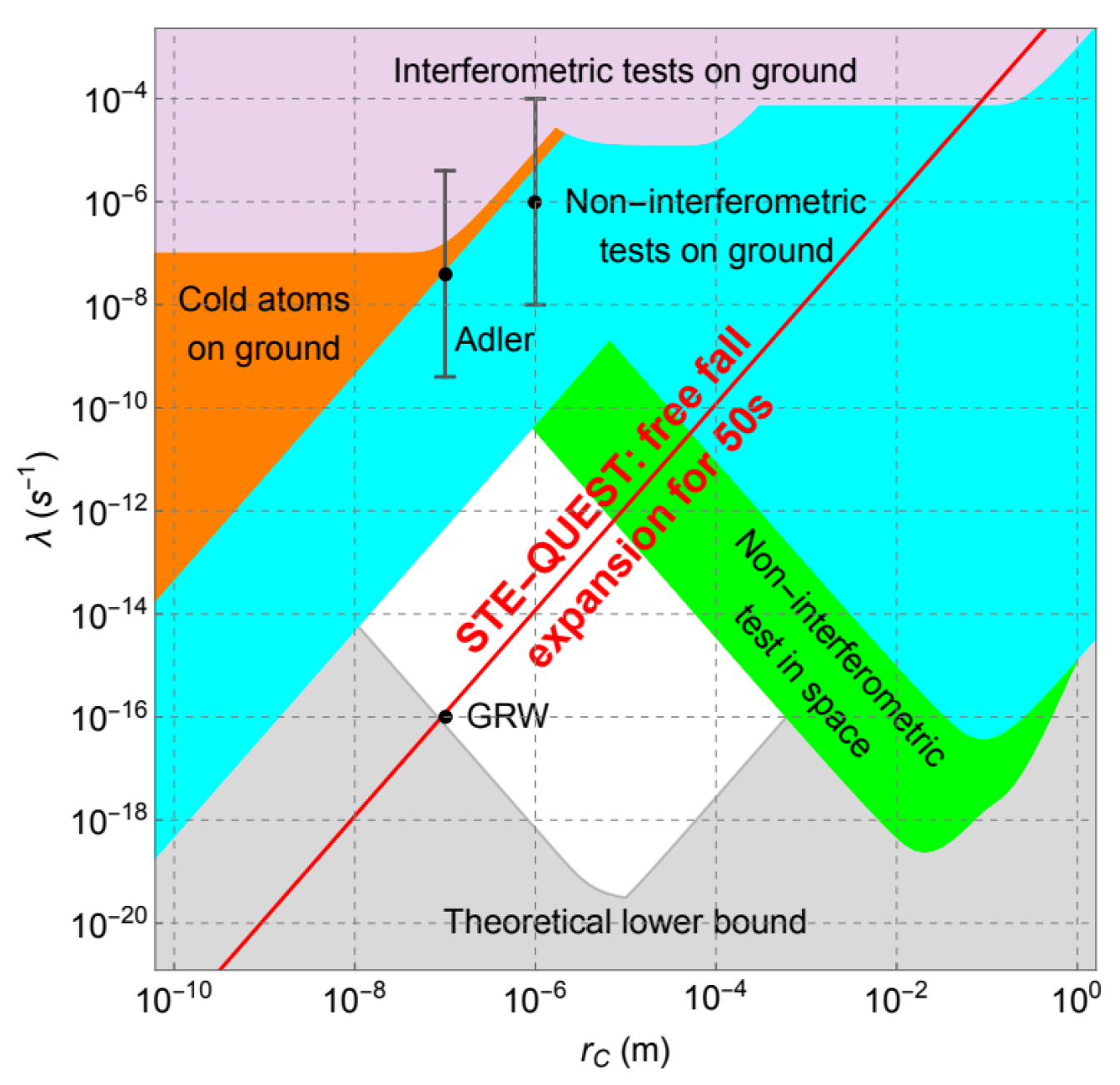
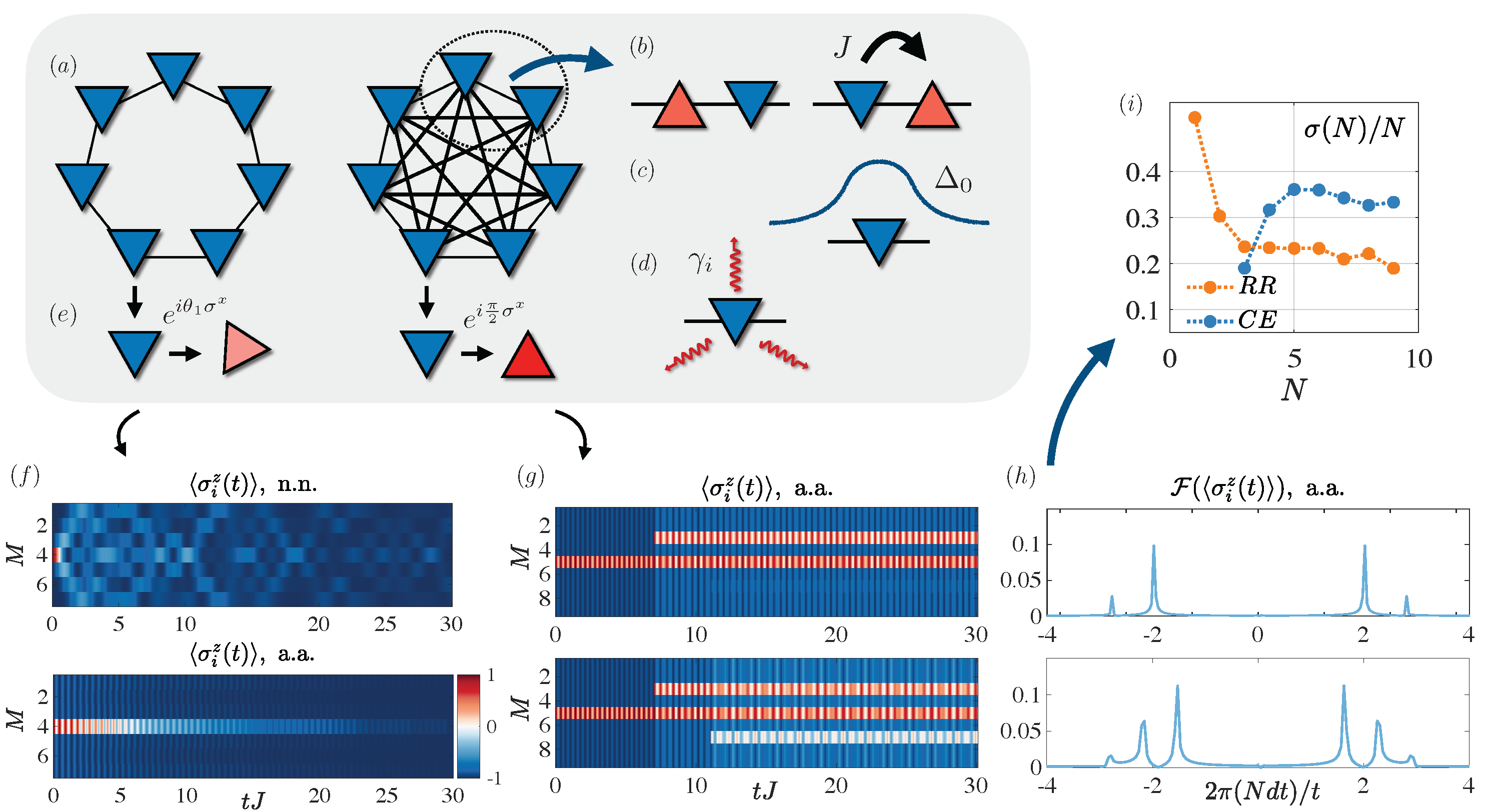
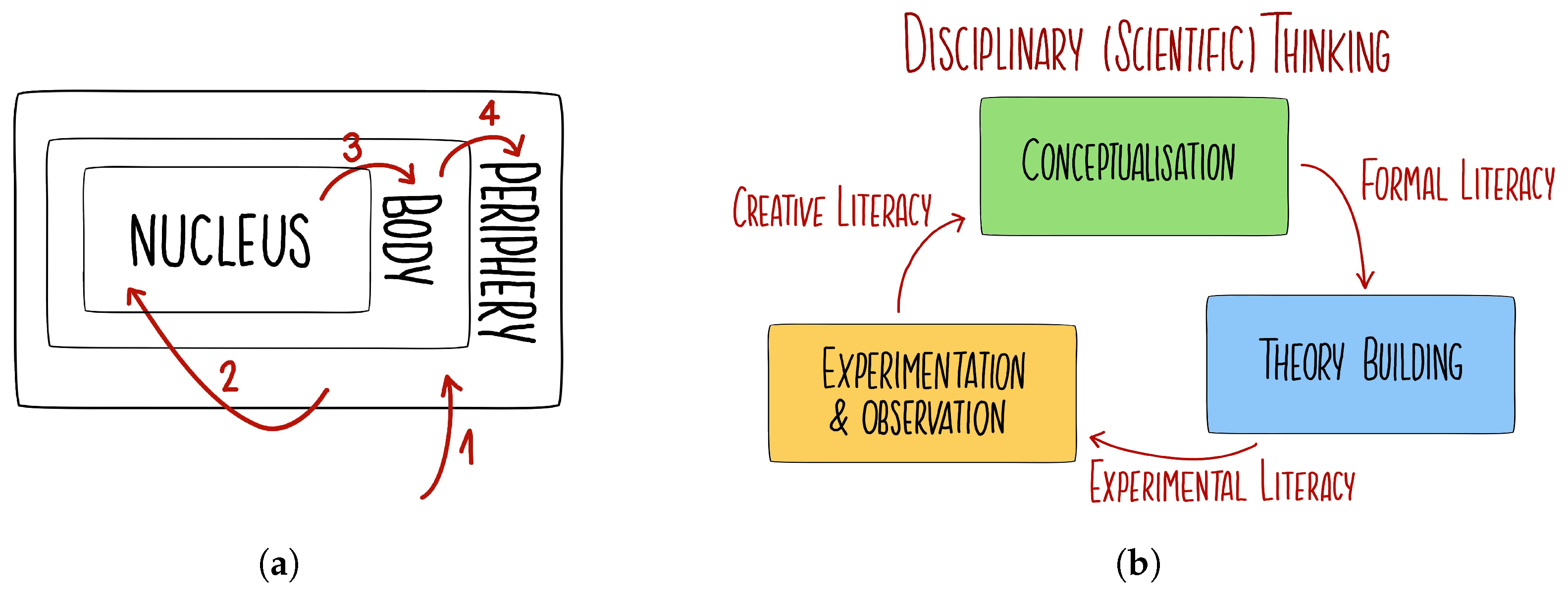
| Class | Elements | Year | Comments | |
|---|---|---|---|---|
| Be–Ti | 2008 | Torsion balance | ||
| Classical | Pt–Ti | 2017 | MICROSCOPE first results | |
| Pt–Ti | 2022 | MICROSCOPE full data | ||
| Hybrid | 133Cs–CC | 2001 | Atom Interferometry | |
| 87Rb–CC | 2010 | and macroscopic corner cube (CC) | ||
| 39K–87Rb | 2020 | different elements | ||
| 87Sr–88Sr | 2014 | same element, fermion vs. boson | ||
| Quantum | 85Rb–87Rb | 2015 | same element, different isotopes | |
| 85Rb–87Rb | 2020 | 10 m drop tower | ||
| 41K–87Rb | 2037 | STE-QUEST | ||
| Antimatter | –H | 2023+ | under construction at CERN |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yago Malo, J.; Lepori, L.; Gentini, L.; Chiofalo, M.L. Atomic Quantum Technologies for Quantum Matter and Fundamental Physics Applications. Technologies 2024, 12, 64. https://doi.org/10.3390/technologies12050064
Yago Malo J, Lepori L, Gentini L, Chiofalo ML. Atomic Quantum Technologies for Quantum Matter and Fundamental Physics Applications. Technologies. 2024; 12(5):64. https://doi.org/10.3390/technologies12050064
Chicago/Turabian StyleYago Malo, Jorge, Luca Lepori, Laura Gentini, and Maria Luisa (Marilù) Chiofalo. 2024. "Atomic Quantum Technologies for Quantum Matter and Fundamental Physics Applications" Technologies 12, no. 5: 64. https://doi.org/10.3390/technologies12050064
APA StyleYago Malo, J., Lepori, L., Gentini, L., & Chiofalo, M. L. (2024). Atomic Quantum Technologies for Quantum Matter and Fundamental Physics Applications. Technologies, 12(5), 64. https://doi.org/10.3390/technologies12050064








