Parametric Research and Aerodynamic Characteristic of a Two-Stage Transonic Compressor for a Turbine Based Combined Cycle Engine
Abstract
:1. Introduction
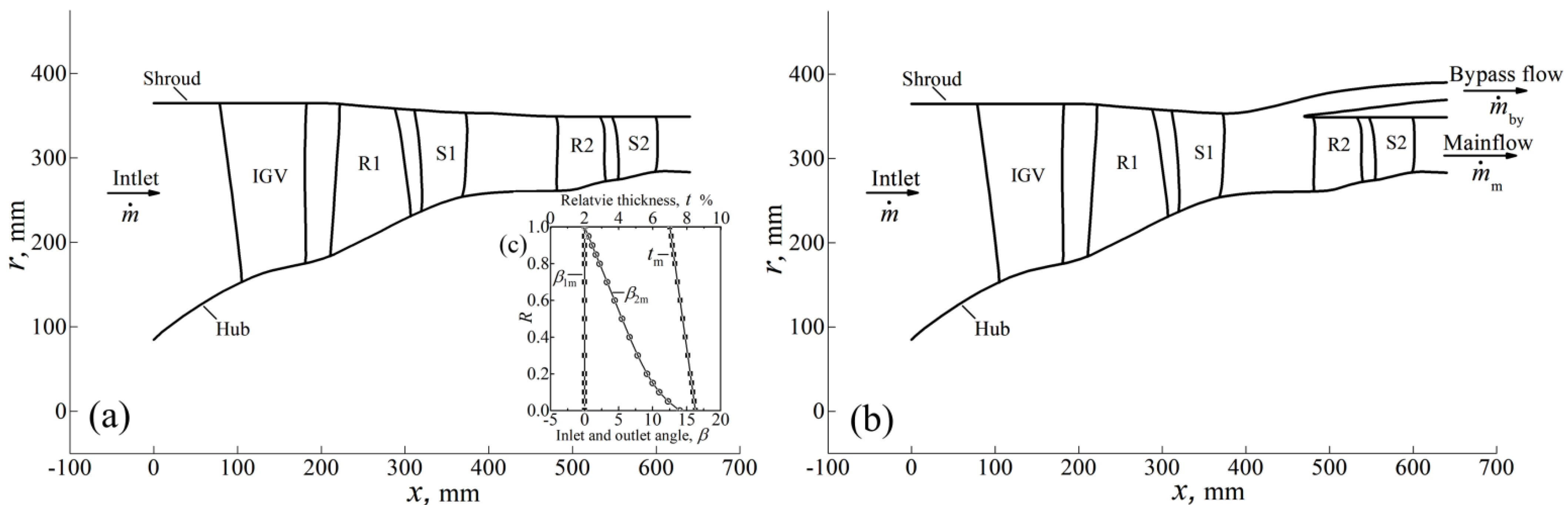
2. General Consideration of the Transonic Compressor: A Large Bypass Flow-Path in Stages to Improve Compressor Mass-Flow Rate at Partial Speed
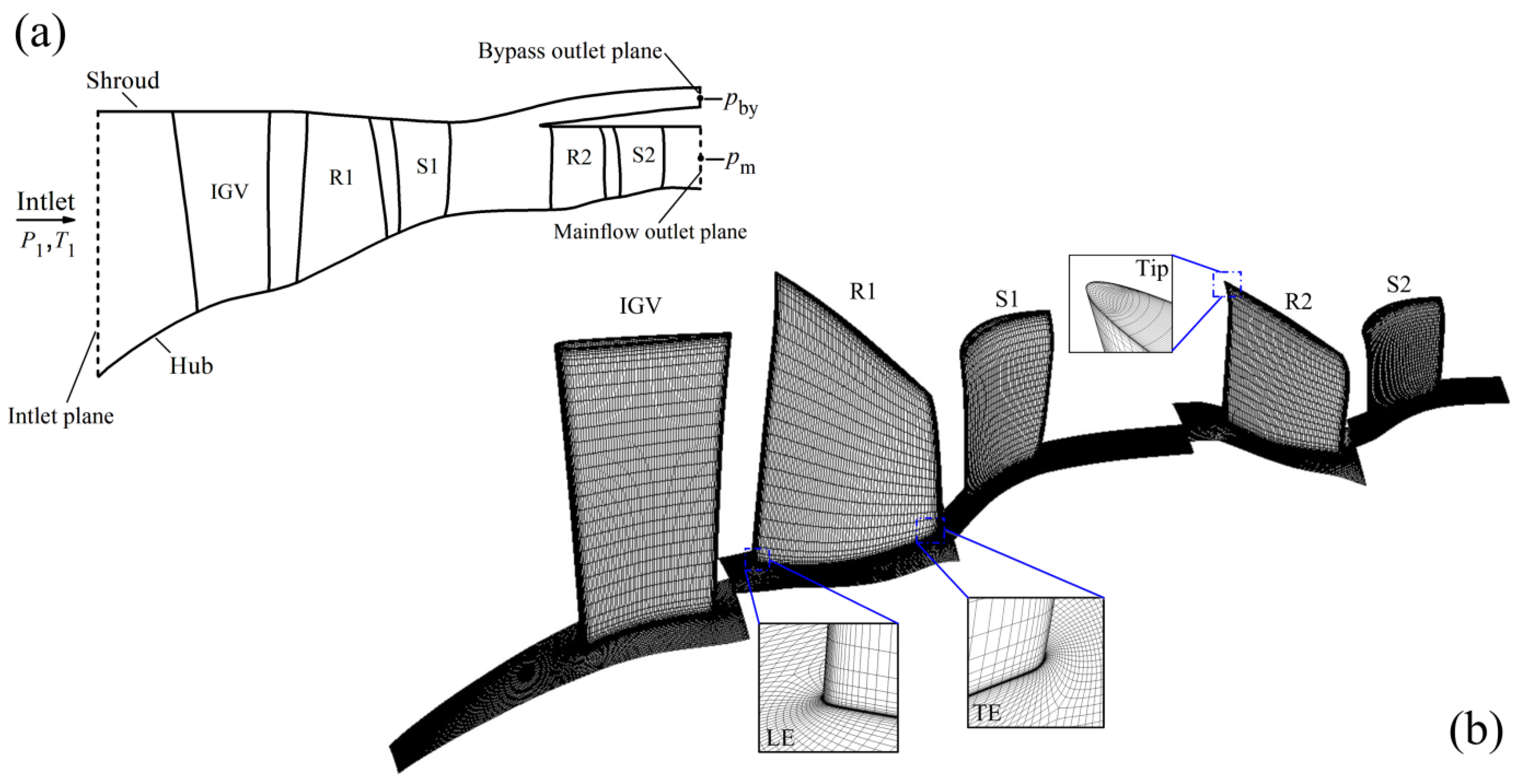
Introduction of the Baseline Two-Stage Transonic Compressor
3. Effect of Adding the Bypass Flow Channel
3.1. Numerical Method
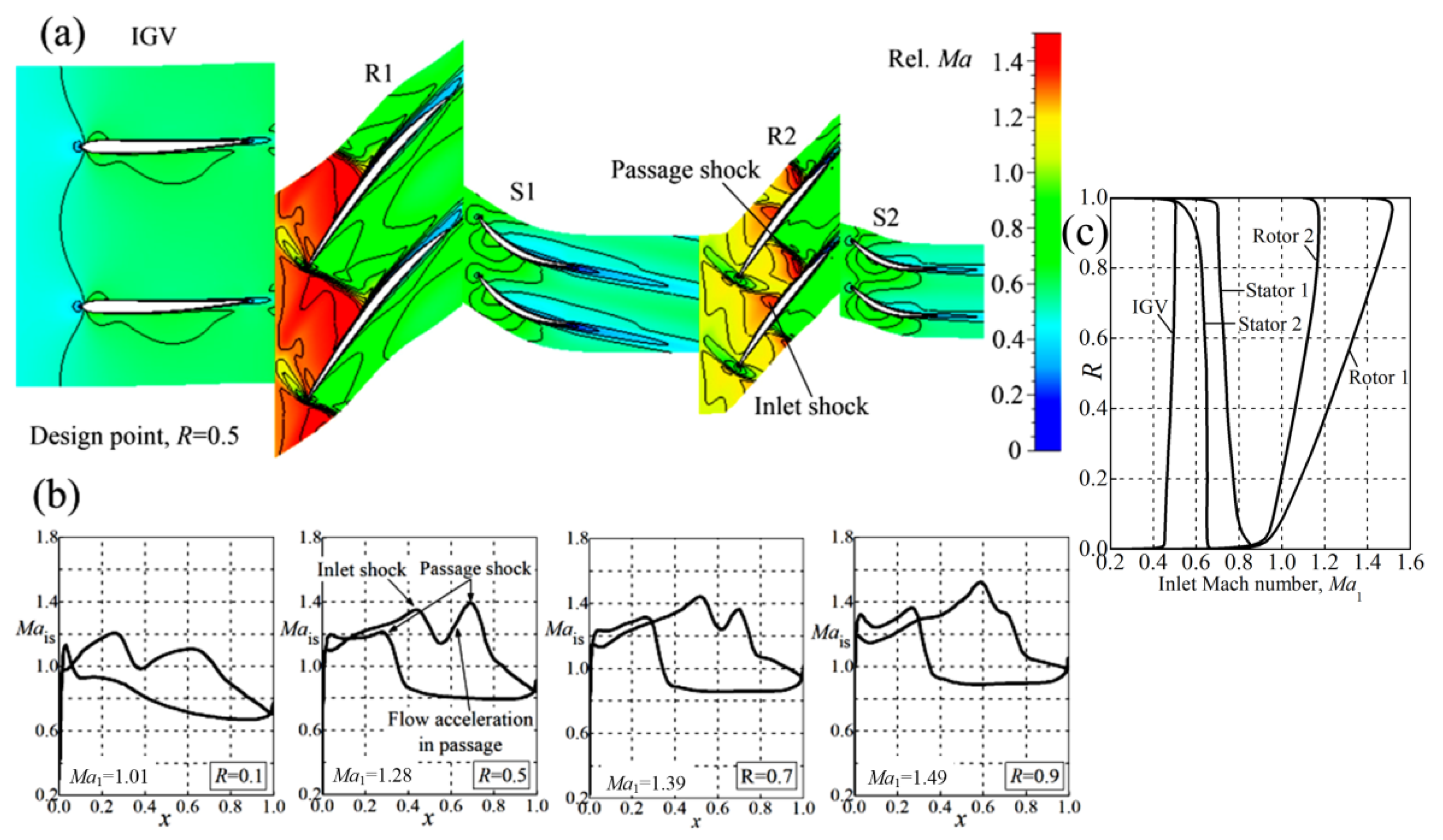
3.2. Baseline Compressor Performance
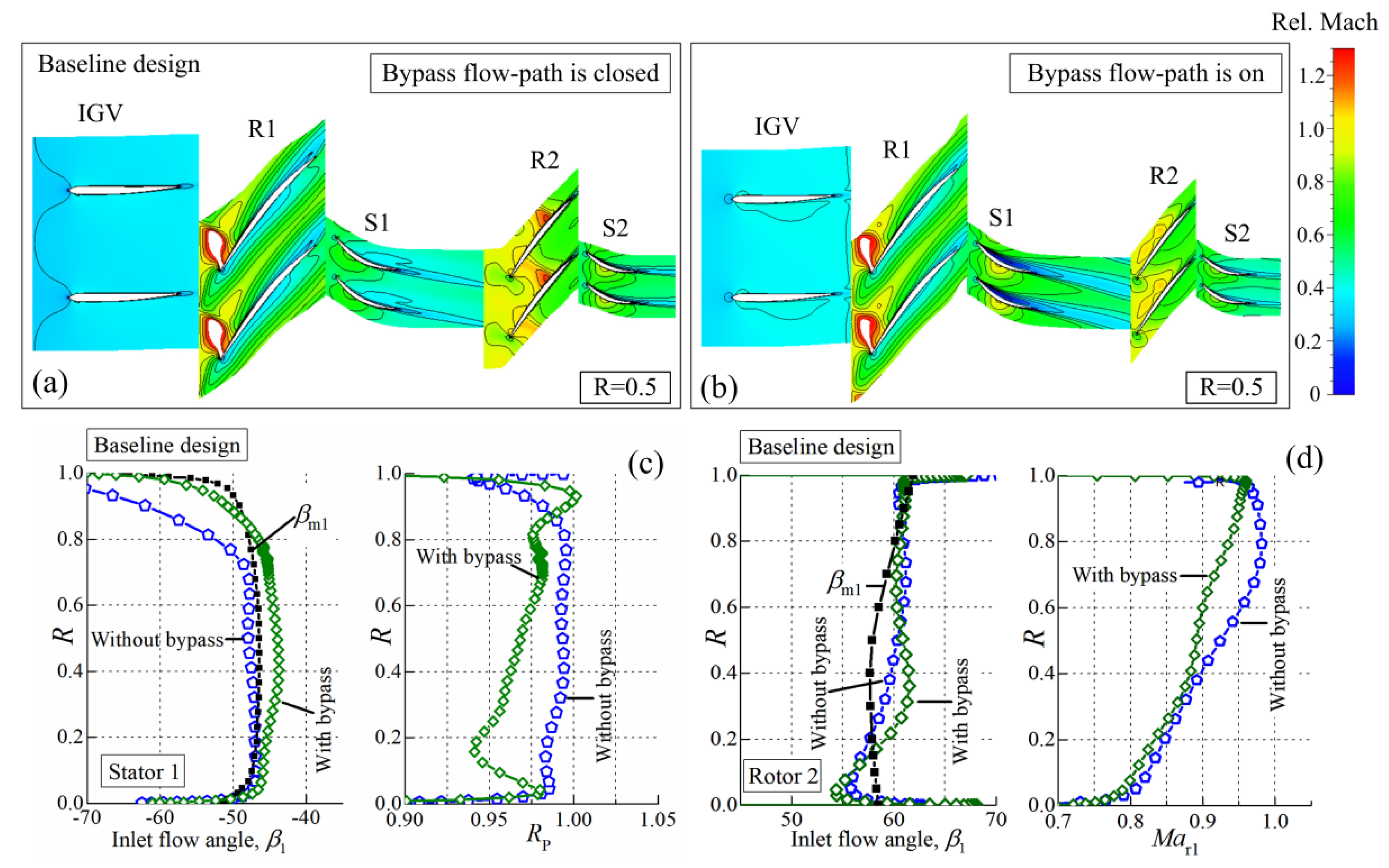
3.3. Effect of the Bypass Flow-Path
4. Parametric Research of Rotor 2
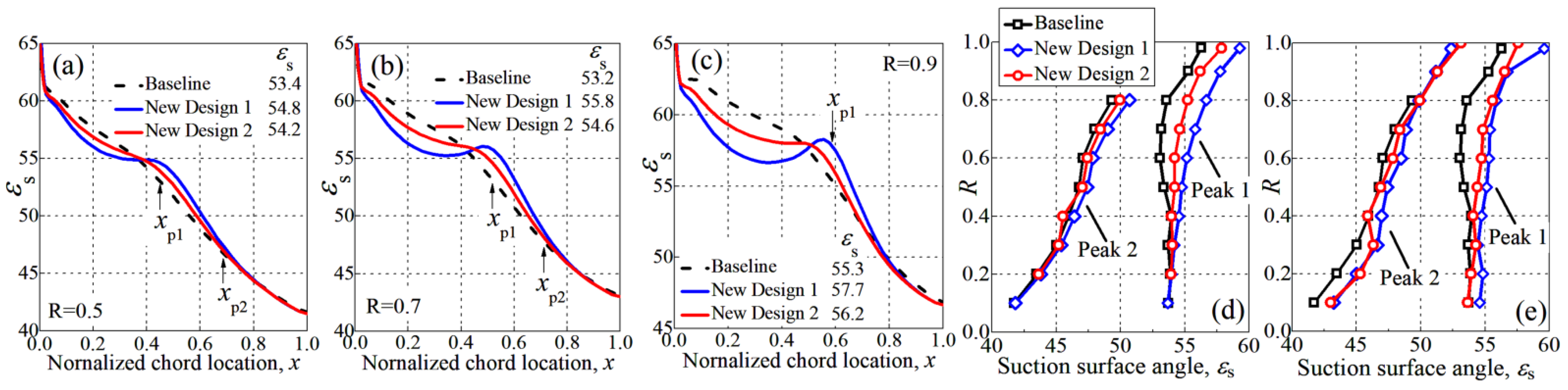
4.1. Modification of the Rotor 2 Normalized Camber Angle Distribution
- (1)
- Keeping the blade suction surface angle at inlet shock impinging point higher than that of baseline design, aiming at decreasing the inlet shock strength by reducing the flow expansion along the blade suction surface. This is because the pre-shock Mach number at suction surface impinging point increases with the enhancement of the inflow Mach number and flow turning angle :
- (2)
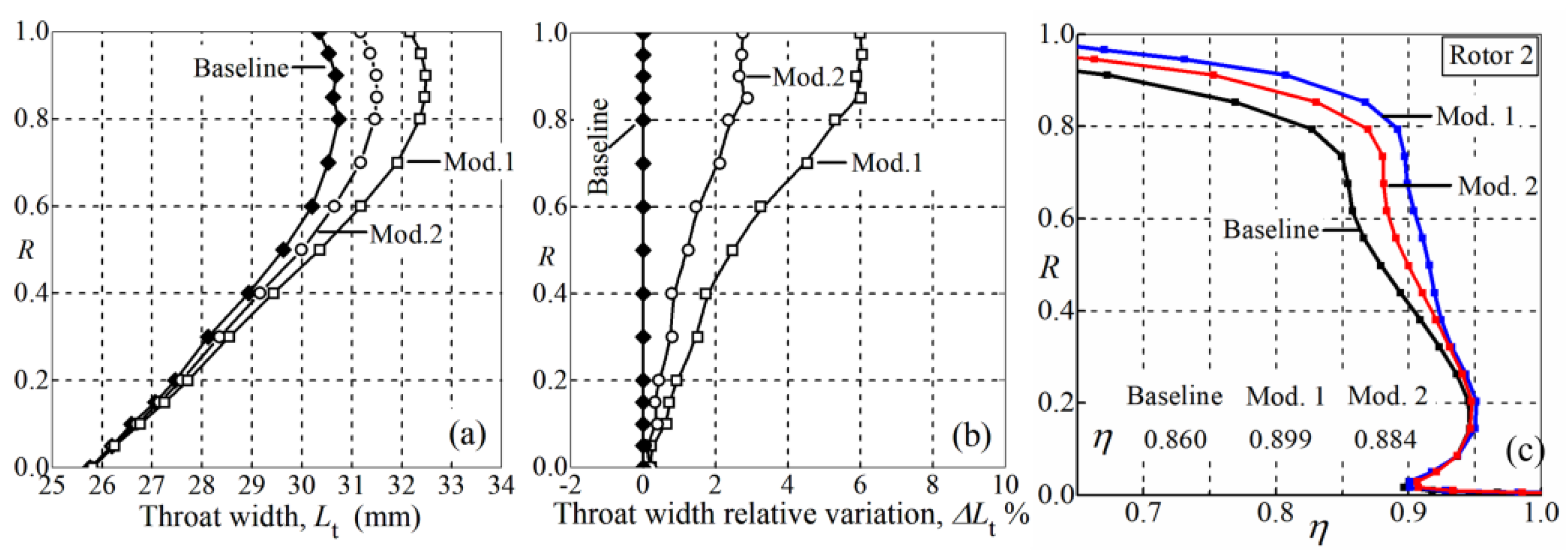
- The larger blade cascade throat width is able to reduce the degree of flow blockage around the throat location for decreasing the strength of passage shock.
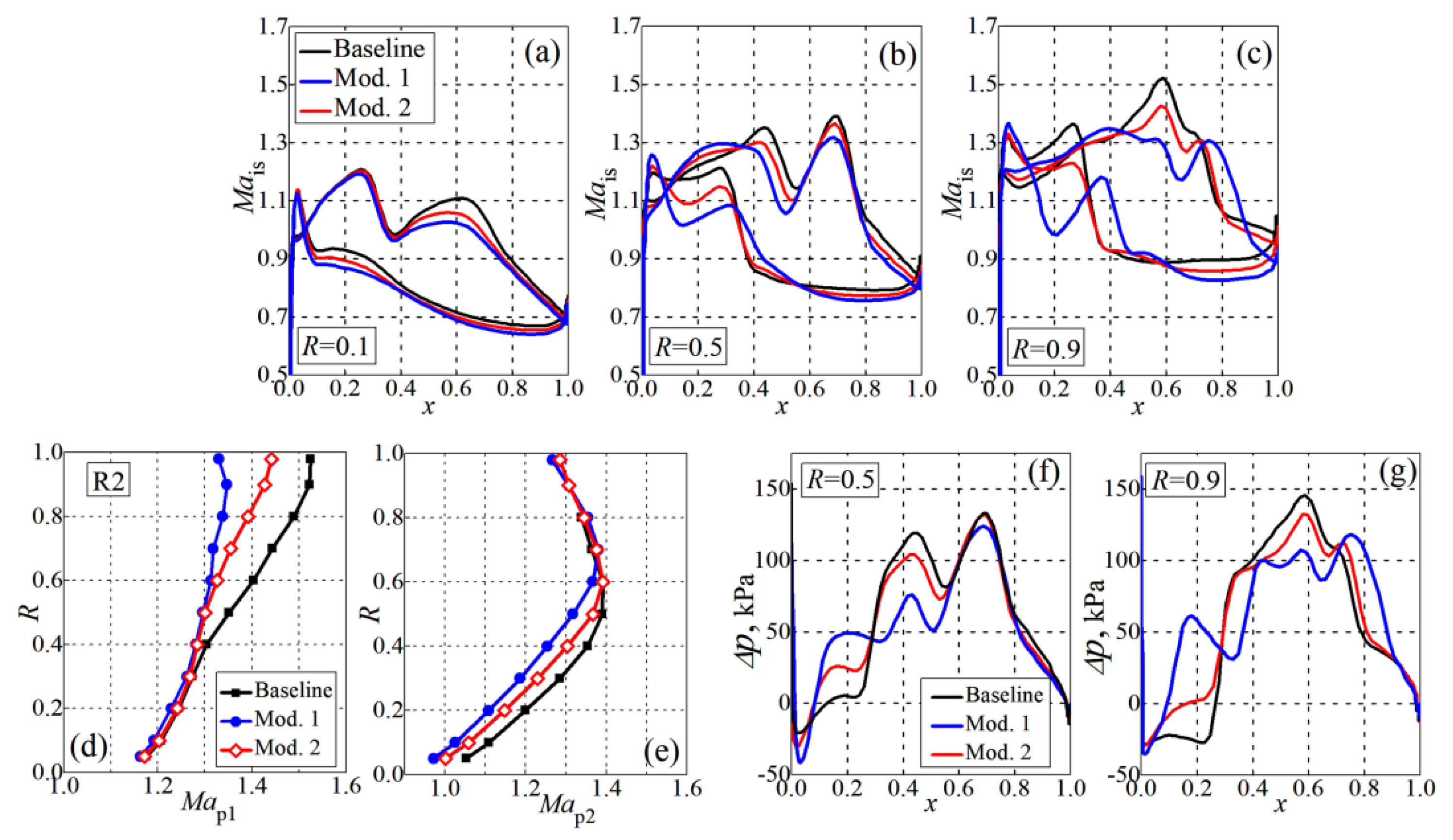
4.2. Aerodynamic Influence of the Rotor 2 Modifications
5. Parametric Research of the Stator S1
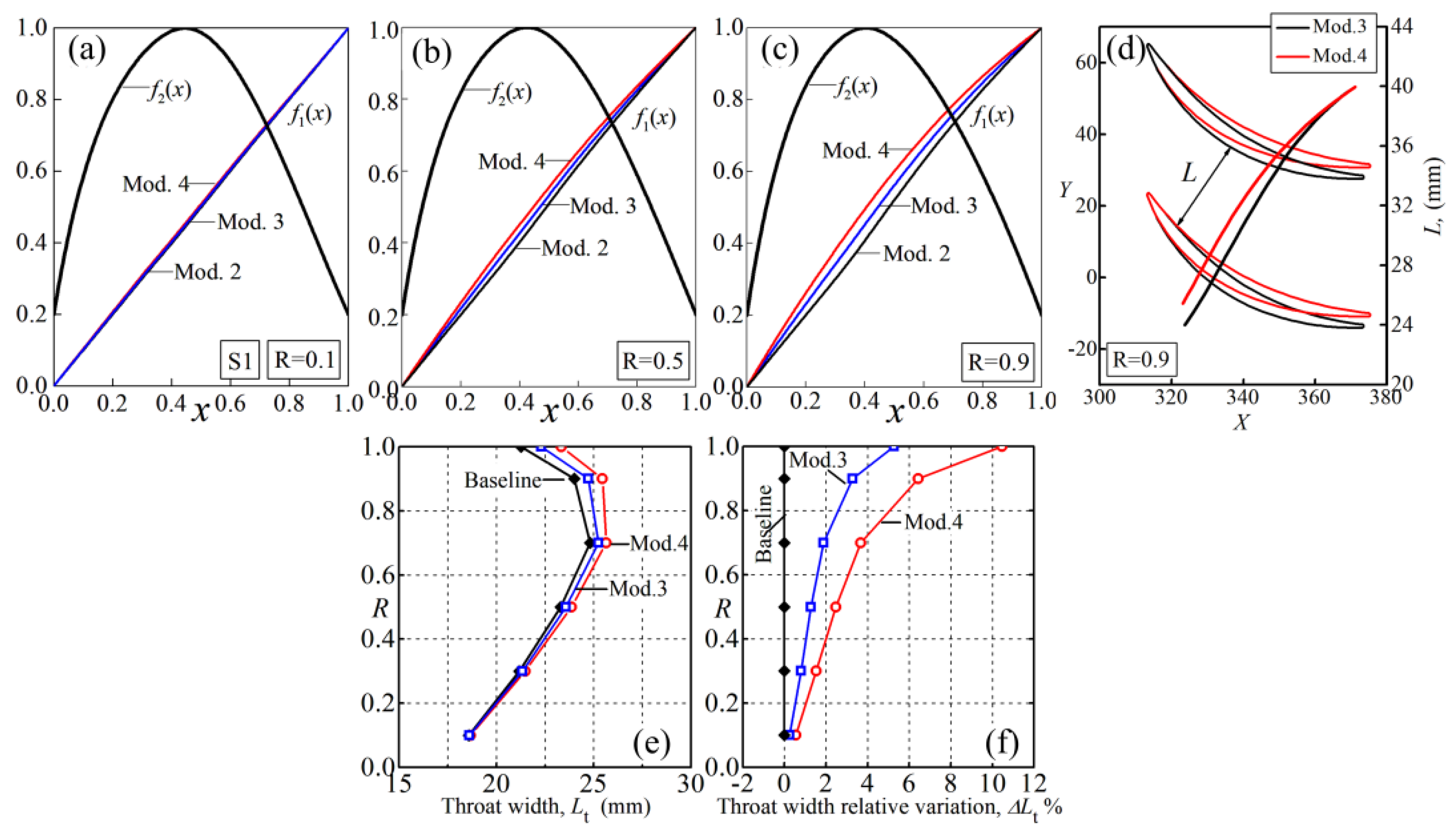
5.1. Modification of Stator S1 Normalized Camber Angle Distribution
5.2. Aerodynamic Influence of the Stator 1 Modifications
6. Conclusions
- (1)
- The target of Rotor 2 modification is to improve efficiency at choke condition by decreasing the shock system strength. The modification of Rotor 2 is achieved by altering the normalized camber angle distribution for reducing the turning angle of the suction surface at the inlet shock impinging point while slightly increasing the blade throat width. Simulations indicate the strength of shock structure is obviously reduced and the blade loading distribution becomes more uniform, leading to the efficiency improvement of modified Rotor 2 at design point. Compared with Mod.1, Mod.2 has positive efficiency gain at more operating conditions, which atrributes to the weaker inlet shock-wave strength of Mod.2 at single shock mode due to the higher suction surface angle in the front portion.
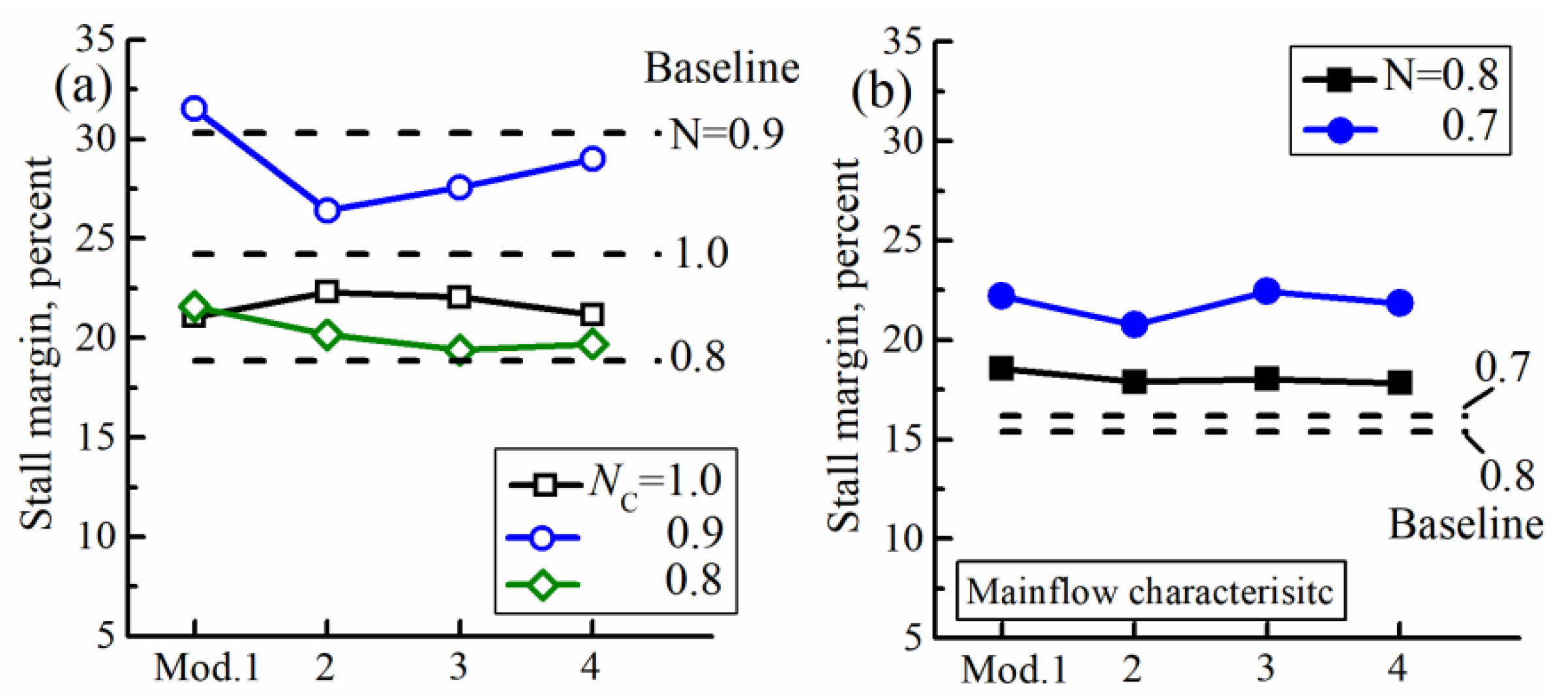
- (2)
- The target of stator S1 modification is to enhance the compressor mass-flow rate at partial rotating speeds by improving the flow capacity. With Mod.2 as the basis, the front loading level of normalized camber angle distribution of S1 is increased to enhance the blade throat width. Simulation of Mod.3 and 4 with enhanced S1 blade throat width achieves higher mass-flow rate at choke conditions of partial speeds. In addition, Mod.3 and 4 show efficiency improvement over Mod.2 at most of the representative operation conditions at all rotating speeds And the efficiency improvement is positively correlated to the enhancement of S1 flow capacity due to the further decreased flow blockage and lower loss coefficient.
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Specific heat ratio, k =1.4 | |
| Mass flow rate () | |
| Static pressure (Pa) | |
| Radius (mm) | |
| Normalized axial chord location of first peak isentropic Mach number | |
| Normalized axial chord location of second peak isentropic Mach number | |
| C | Airfoil chord |
| CP | Choke point |
| DP | Design point |
| L | Blade passage width |
| Blade passage width at throat location | |
| LE | Leading edge |
| Mach number | |
| Blade surface isentropic Mach number | |
| Relative inlet Mach number | |
| Relative rotating speed, the ratio of actual rotating speed to design rotating speed | |
| NS | Near stall point |
| Total pressure (Pa) | |
| PE | Peak efficiency point |
| Blade relative height, | |
| Total pressure recovery coefficient, | |
| Spacing (mm) | |
| Total temperature (K) | |
| Flow angle measured from axial direction (degree) | |
| Blade metal angle measured from axial direction (degree) | |
| Blade surface angle, the angle between surface tangential line and axial direction (degree) | |
| Isentropic efficiency, | |
| Flow coefficient, | |
| Load coefficient, | |
| Total pressure ratio, | |
| Solidity, | |
| Blade aerodynamic loading distribution, the pressure difference between pressure surface and suction surface (kPa) | |
| Variation of efficiency relative to baseline design, | |
| Relative variation of blade throat width, | |
| Subscripts | |
| 1 | Inlet |
| 2 | Outlet |
| ax | Axial direction |
| by | Bypass flow |
| D | Value at design point |
| m | Main-flow |
| is | Isentropic |
| t | Blade tip |
References
- Viola, N.; Roncioni, P.; Gori, O.; Fusaro, R. Aerodynamic Characterization of Hypersonic Transportation Systems and Its Impact on Mission Analysis. Energies 2021, 14, 3580. [Google Scholar] [CrossRef]
- Viola, N.; Ferretto, D.; Fusaro, R.; Scigliano, R. Performance Assessment of an Integrated Environmental Control System of Civil Hypersonic Vehicles. Aerospace 2022, 9, 201. [Google Scholar] [CrossRef]
- Zhen, W.; Wang, Y.; Wu, Q.; Shao, P. Coordinated attitude control of hypersonic flight vehicles based on the coupling analysis. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2017, 232, 1002–1011. [Google Scholar] [CrossRef]
- Sen, D.; Pesyridis, A.; Lenton, A. A Scramjet Compression System for Hypersonic Air Transportation Vehicle Combined Cycle Engines. Energies 2018, 11, 1568. [Google Scholar] [CrossRef] [Green Version]
- Feil, M.; Staudacher, S. Uncertainty quantification of a generic scramjet engine using a probabilistic collocation and a hybrid approach. CEAS Aeronaut. J. 2018, 9, 649–659. [Google Scholar] [CrossRef]
- Bruno, C.; Ingenito, A. Some Key Issues in Hypersonic Propulsion. Energies 2021, 14, 3690. [Google Scholar] [CrossRef]
- Walker, S.; Tang, M.; Mamplata, C. TBCC Propulsion for a Mach 6 Hypersonic Airplane. In Proceedings of the 16th AIAA/DLR/DGLR International Space Planes and Hypersonic Systems and Technologies Conference, Bremen, Germany, 19–22 October 2009. [Google Scholar]
- Clough, J. Modeling and Optimization of Turbine-Based Combined-Cycle Engine Performance; University of Maryland: College Park, MD, USA, 2004. [Google Scholar]
- Colville, J.R.; Lewis, M.J. An Aerodynamic Redesign of the SR-71 Inlet with Applications to Turbine Based Combined Cycle Engines. In Proceedings of the 40th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Fort Lauderdale, FL, USA, 11–14 July 2004. [Google Scholar]
- Sippel, M. Research on TBCC Propulsion for a Mach 4.5 Supersonic Cruise Airliner. In Proceedings of the 14th AIAA/AHI Space Planes and Hypersonic Systems and Technologies Conference, Canberra, Australia, 6–9 November 2006. [Google Scholar]
- Gamble, E.; Haid, D.; D’Alessandro, S.; DeFrancesco, R. Dual-Mode Scramjet Performance Model for TBCC Simulation. In Proceedings of the 45th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Denver, CO, USA, 2–5 August 2009. [Google Scholar]
- Gamble, E.; Haid, D.; D’Alessandro, S. Hydraulic and Kinematic System Model for TBCC Dynamic Simulation. In Proceedings of the 46th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Nashville, TN, USA, 25–28 July 2010. [Google Scholar]
- Gamble, E.; Haid, D.; D’Alessandro, S. Thermal Management and Fuel System Model for TBCC Dynamic Simulation. In Proceedings of the 46th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Nashville, TN, USA, 25–28 July 2012. [Google Scholar]
- Slater, J.; Saunders, J. CFD Simulation of Hypersonic TBCC Inlet Mode Transition. In Proceedings of the 16th AIAA/DLR/DGLR International Space Planes and Hypersonic Systems and Technologies Conference, Bremen, Germany, 19–22 October 2009. [Google Scholar]
- Zhang, M.; Wang, Z.; Liu, Z.; Zhang, X. Analysis of Mode Transition Performance for a Tandem TBCC Engine. In Proceedings of the 52nd AIAA/SAE/ASEE Joint Propulsion Conference, Salt Lake City, UT, USA, 25–27 July 2016. [Google Scholar]
- Ou-Yang, H.; Zhu, Z.l.; Chen, M. Conceptual Design of Geometry-Variable Hypersonic Intake for TBCC. In Proceedings of the 44th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Hartford, CT, USA, 21–23 July 2008. [Google Scholar]
- Huang, H.; Huang, G.; Zuo, F.; Chen, X. Research on a Novel Internal waverider TBCC Inlet for Ramjet Mode. In Proceedings of the 52nd AIAA/SAE/ASEE Joint Propulsion Conference, Salt Lake City, UT, USA, 25–27 July 2016. [Google Scholar]
- Vanna, F.D.; Picano, F.; Benini, E.; Quinn, M.K. Large-Eddy Simulations of the Unsteady Behavior of a Hypersonic Intake at Mach 5. AIAA J. 2021, 59, 3859–3872. [Google Scholar] [CrossRef]
- Vanna, F.D.; Bof, D.; Benini, E. Multi-Objective RANS Aerodynamic Optimization of a Hypersonic Intake Ramp at Mach 5. Energies 2022, 15, 2811. [Google Scholar] [CrossRef]
- Foster, L.; Saunders, J.; Sanders, B.; Weir, L. Highlights from a Mach 4 Experimental Demonstration of Inlet Mode Transition for Turbine-Based Combined Cycle Hypersonic Propulsion. In Proceedings of the 48th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Atlanta, GA, USA, 30 July–1 August 2012. [Google Scholar]
- Shi, H. A Parametric Blade Design Method for High-Speed Axial Compressor. Aerospace 2021, 8, 271. [Google Scholar] [CrossRef]
- Mao, X.; Liu, B. Numerical investigation of tip clearance size effect on the performance and tip leakage flow in a dual-stage counter-rotating axial compressor. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2017, 231, 474–484. [Google Scholar] [CrossRef]
- Miller, G.R.; Lewis, G.W.; Hartmann, M.J. Shock Losses in Transonic Compressor Blade Rows. ASME J. Eng. Gas Turbines Power 1961, 83, 235–241. [Google Scholar] [CrossRef]
- Venturelli, G.; Benini, E. Kriging-assisted design optimization of S-shape supersonic compressor cascades. Aerosp. Sci. Technol. 2016, 58, 275–297. [Google Scholar] [CrossRef]
- Wadia, A.R.; Copenhaver, W.W. An Investigation of the Effect of Cascade Area Ratios on Transonic Compressor Performance. ASME J. Turbomach. 1996, 118, 760–770. [Google Scholar] [CrossRef]
- Burguburu, S.p.; Toussaint, C.; Bonhomme, C.; Leroy, G. Numerical Optimization of Turbomachinery Bladings. ASME J. Turbomach. 2004, 126, 91–100. [Google Scholar] [CrossRef]
- Wang, D.X.; He, L.; Li, Y.S.; Wells, R.G. Adjoint Aerodynamic Design Optimization for Blades in Multistage Turbomachines—Part II: Validation and Application. ASME J. Turbomach. 2010, 132, 021012. [Google Scholar] [CrossRef]
- Wang, D.X.; He, L. Adjoint Aerodynamic Design Optimization for Blades in Multistage Turbomachines—Part I: Methodology and Verification. ASME J. Turbomach. 2010, 132, 021011. [Google Scholar] [CrossRef]
- Aungier, R.H. Axial-Flow Compressors; ASME: New York, NY, USA, 2003; p. 361. [Google Scholar]





| (kg/s) | Stall Margin | |||
|---|---|---|---|---|
| 1.0 | 60.0 | 3.30 | >84.5% | >20.0% |
| 0.9 | ≮50.0 | ≮2.50 | >82.0% | >15.0% |
| 0.8 | ≮47.0 | ≮1.85 | >81.0% | >15.0% |
| 0.7 | ≮41.5 | ≮1.45 | -- | >15.0% |
| 0.80 | 0.74 | 0.70 | |
|---|---|---|---|
| ≮14.0% | ≮22.0% | ≮24.5% |
| H | AR | |||
|---|---|---|---|---|
| Rotor 1 | 463.5 (m/s) | 153.6 (mm) | 0.505 | 1.19 |
| Rotor 2 | 450.0 (m/s) | 84.3 (mm) | 0.748 | 0.92 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, H. Parametric Research and Aerodynamic Characteristic of a Two-Stage Transonic Compressor for a Turbine Based Combined Cycle Engine. Aerospace 2022, 9, 346. https://doi.org/10.3390/aerospace9070346
Shi H. Parametric Research and Aerodynamic Characteristic of a Two-Stage Transonic Compressor for a Turbine Based Combined Cycle Engine. Aerospace. 2022; 9(7):346. https://doi.org/10.3390/aerospace9070346
Chicago/Turabian StyleShi, Hengtao. 2022. "Parametric Research and Aerodynamic Characteristic of a Two-Stage Transonic Compressor for a Turbine Based Combined Cycle Engine" Aerospace 9, no. 7: 346. https://doi.org/10.3390/aerospace9070346
APA StyleShi, H. (2022). Parametric Research and Aerodynamic Characteristic of a Two-Stage Transonic Compressor for a Turbine Based Combined Cycle Engine. Aerospace, 9(7), 346. https://doi.org/10.3390/aerospace9070346






