Layout Design and Verification of a Space Payload Distributed Capture and Lock System
Abstract
:1. Introduction
2. Materials and Methods
2.1. Positioning and Layout Design of the Capture and Lock System
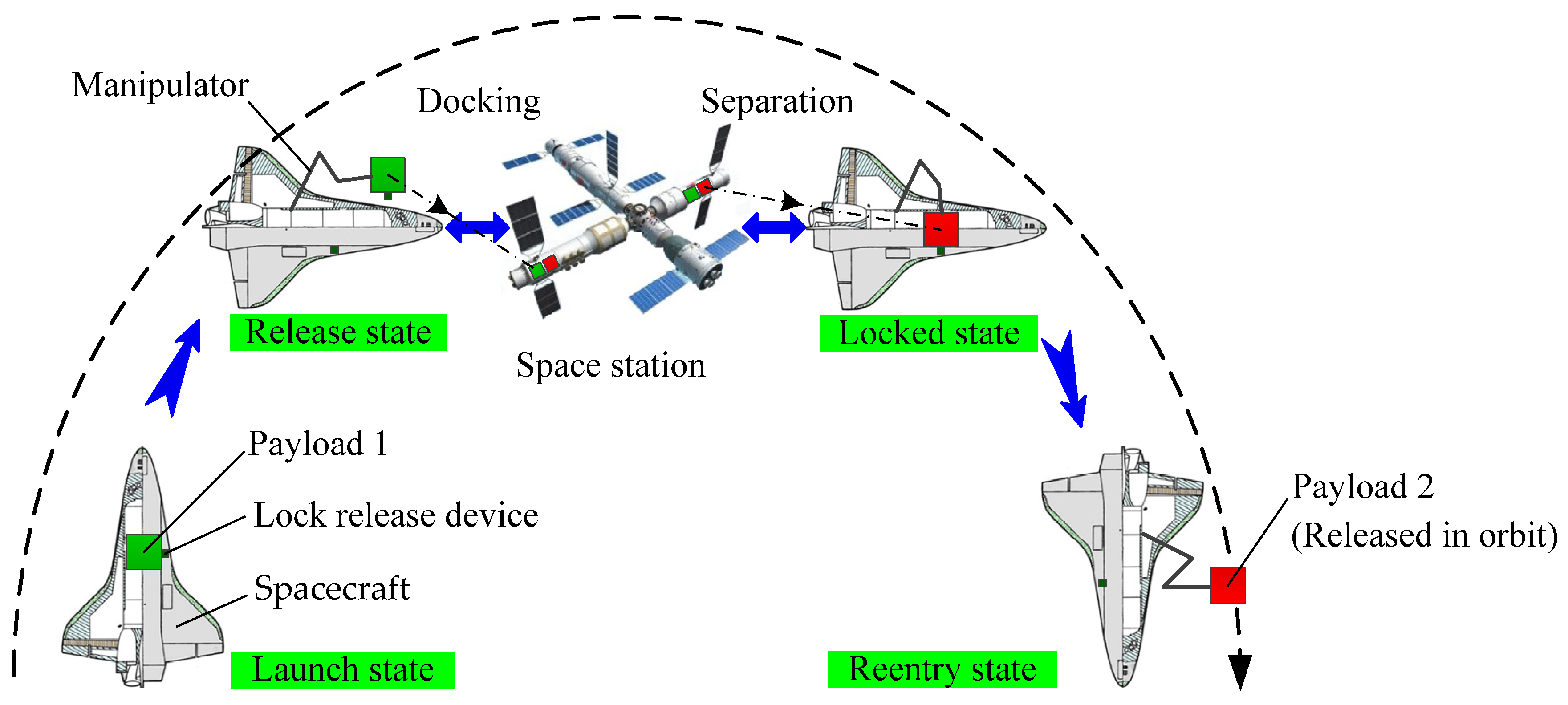
2.1.1. Analysis of the Working Process of the Capture and Lock System
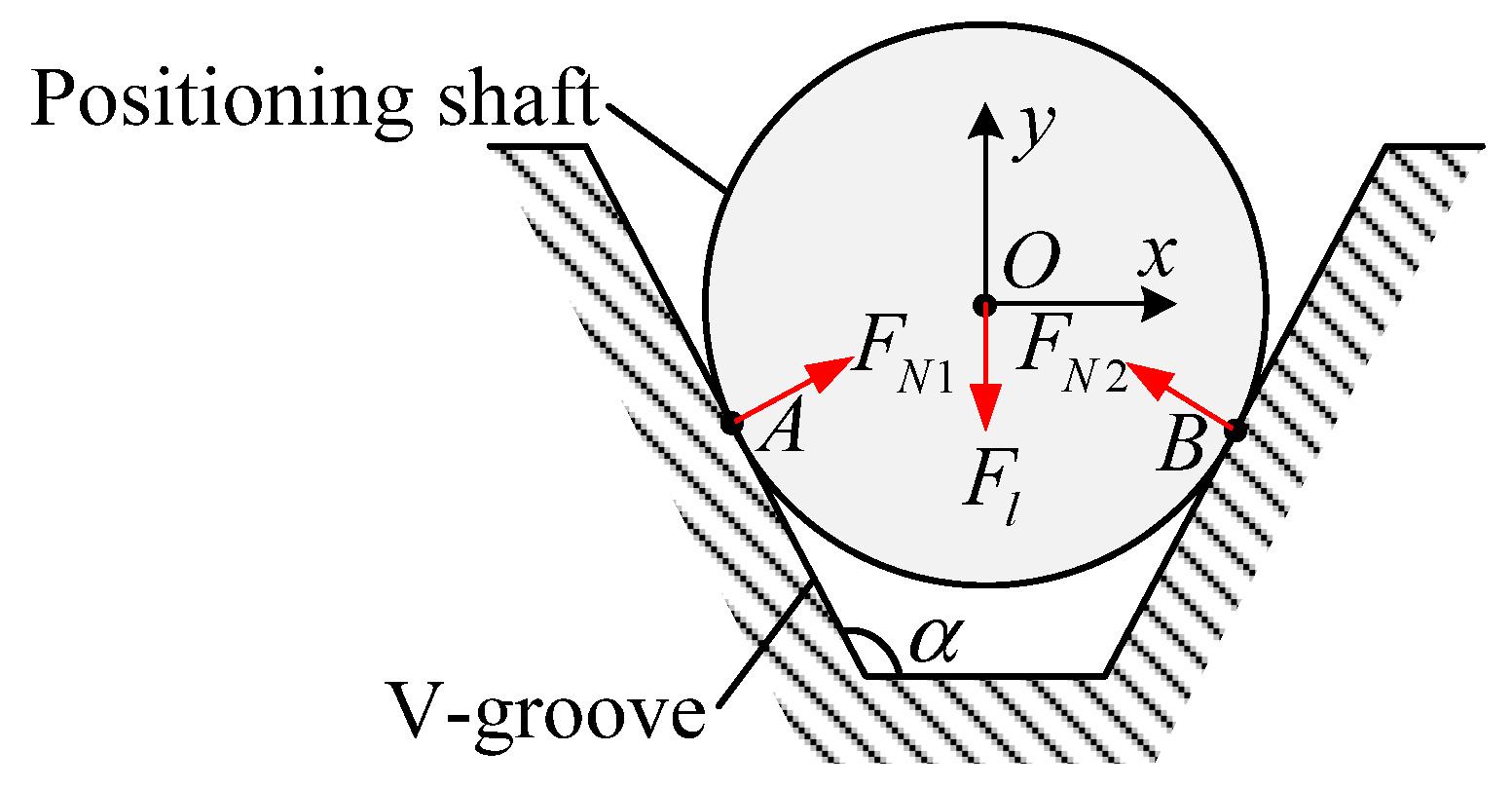
2.1.2. Single Point Positioning Analysis and Multi-Point Over-Positioning Evaluation Model
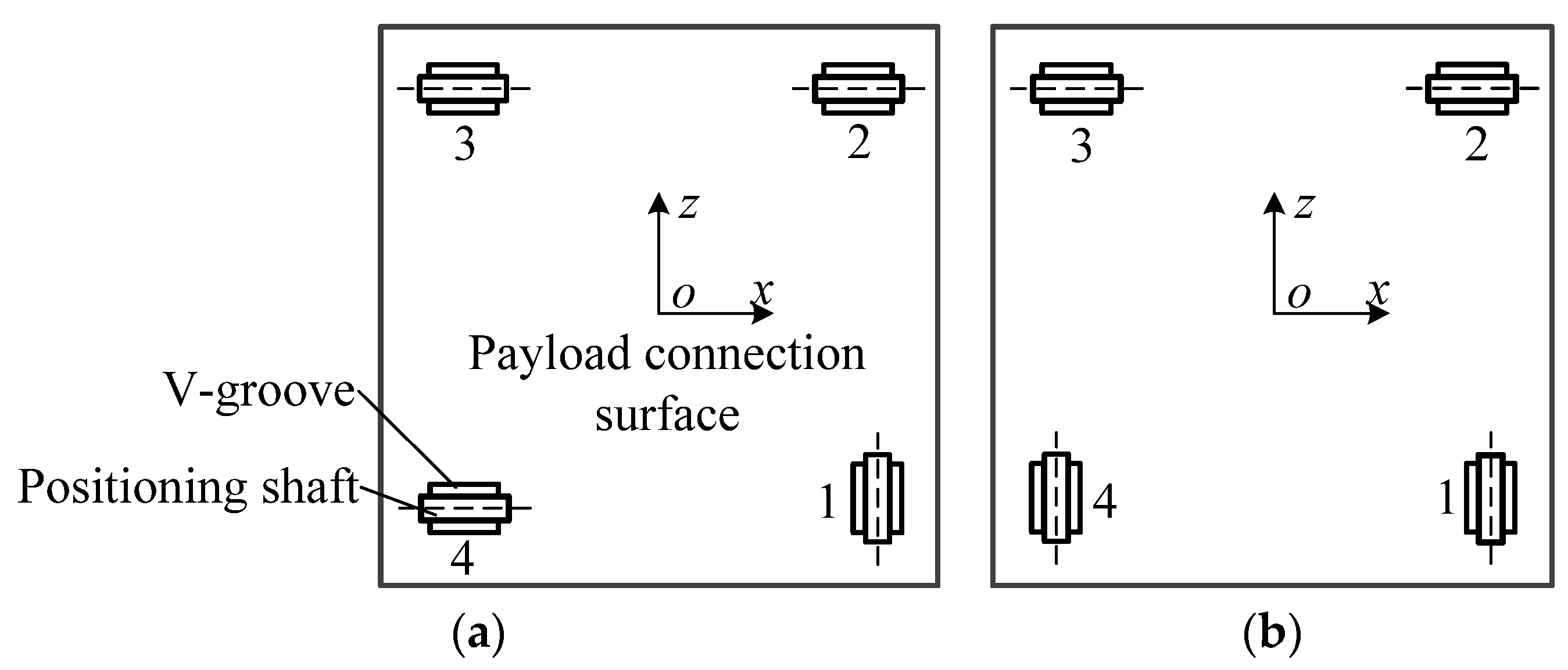
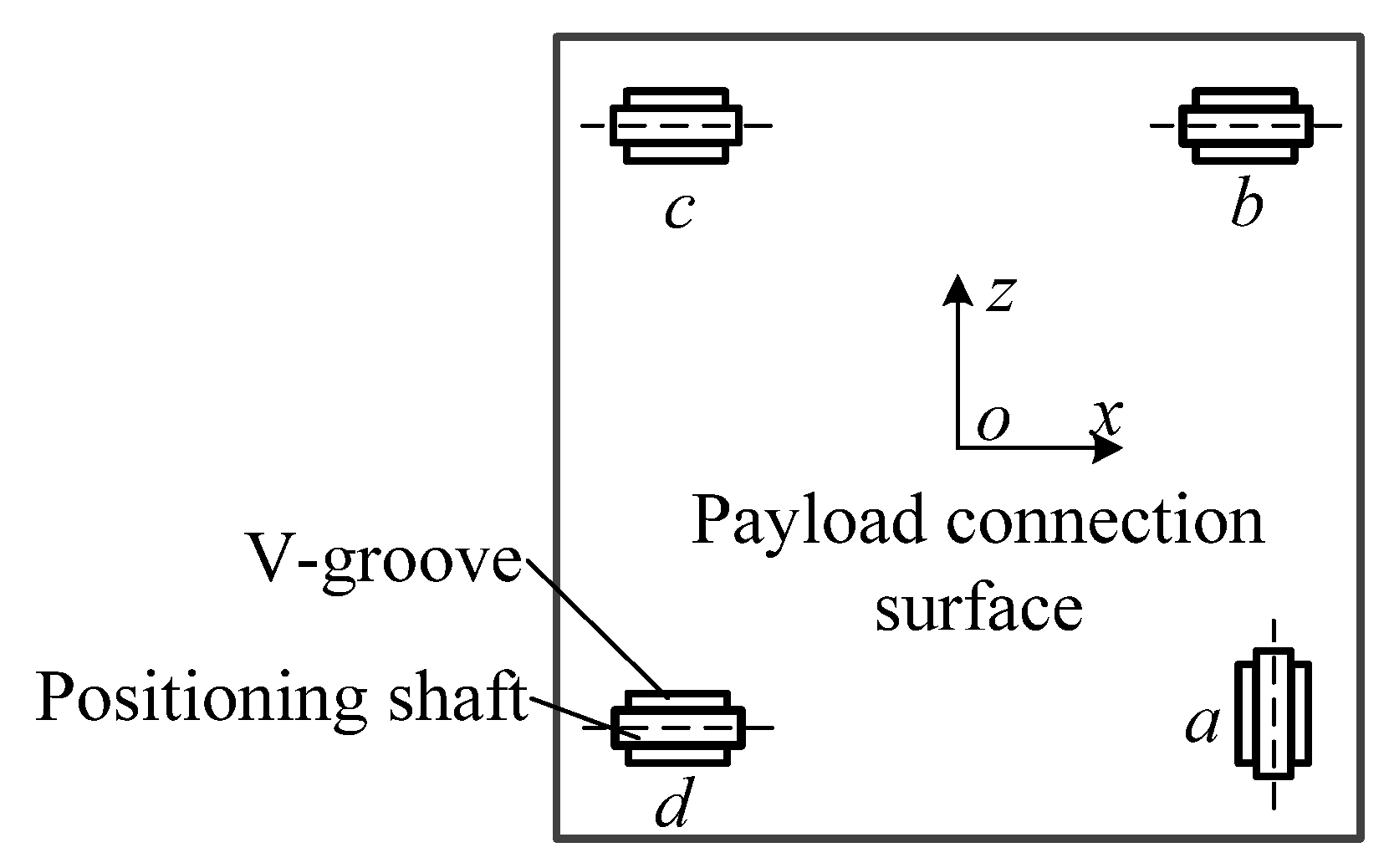
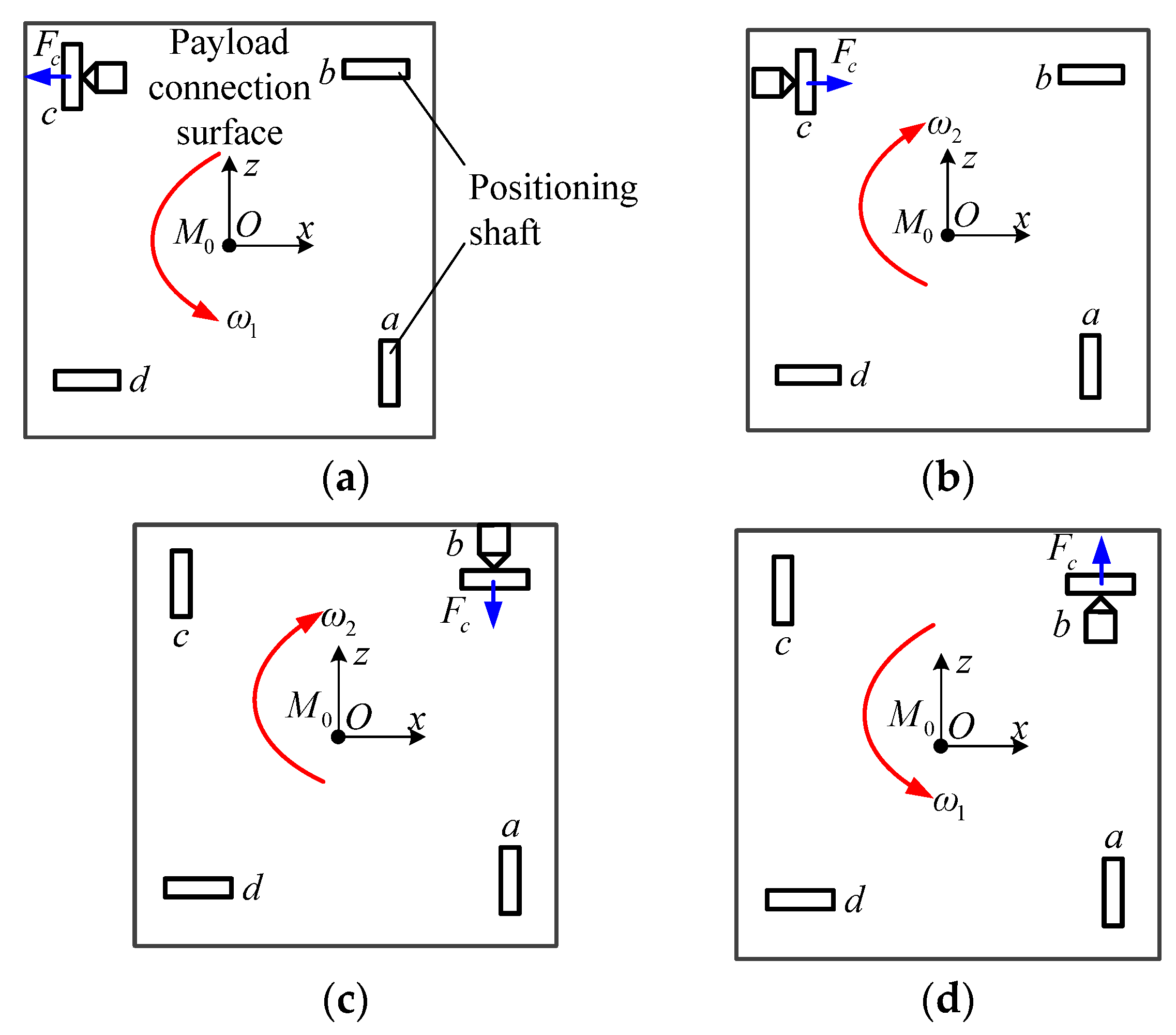
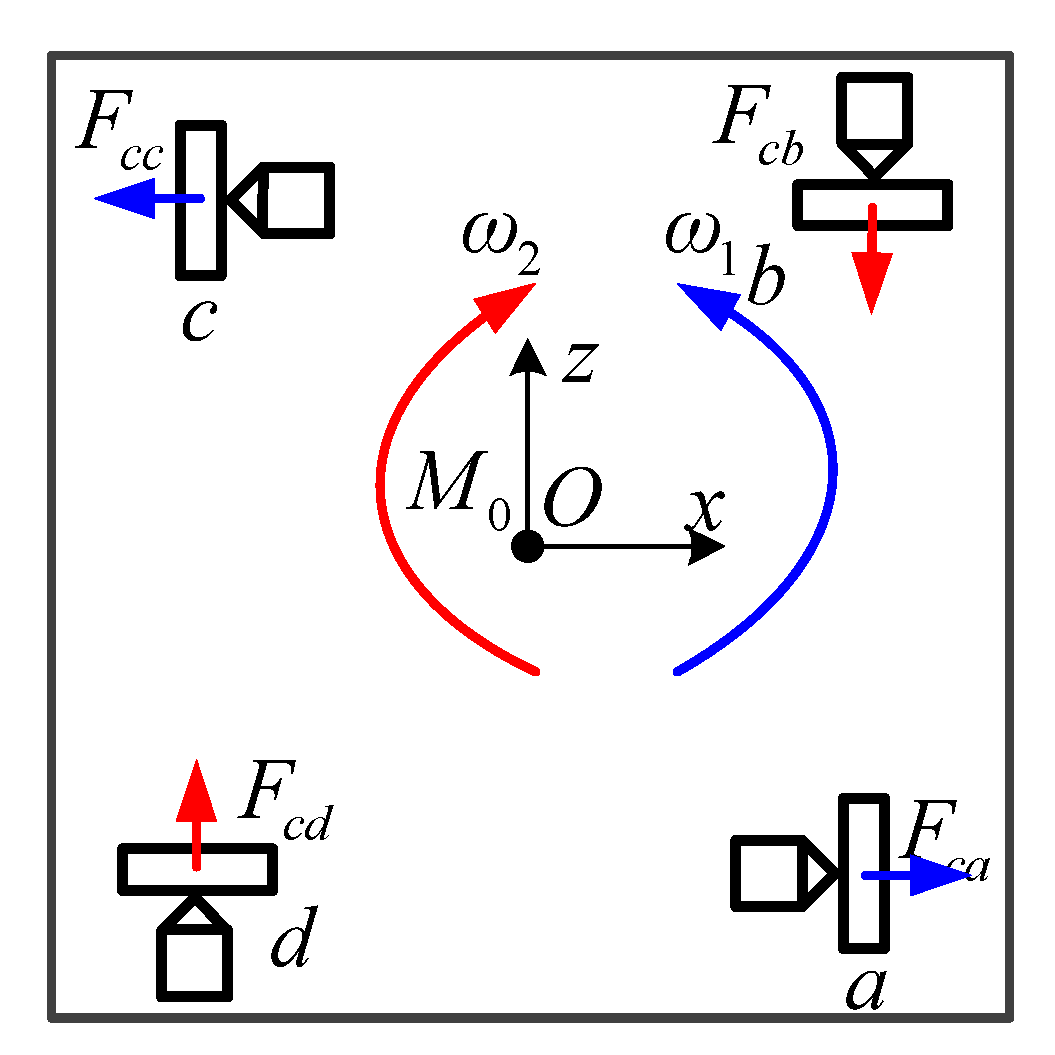
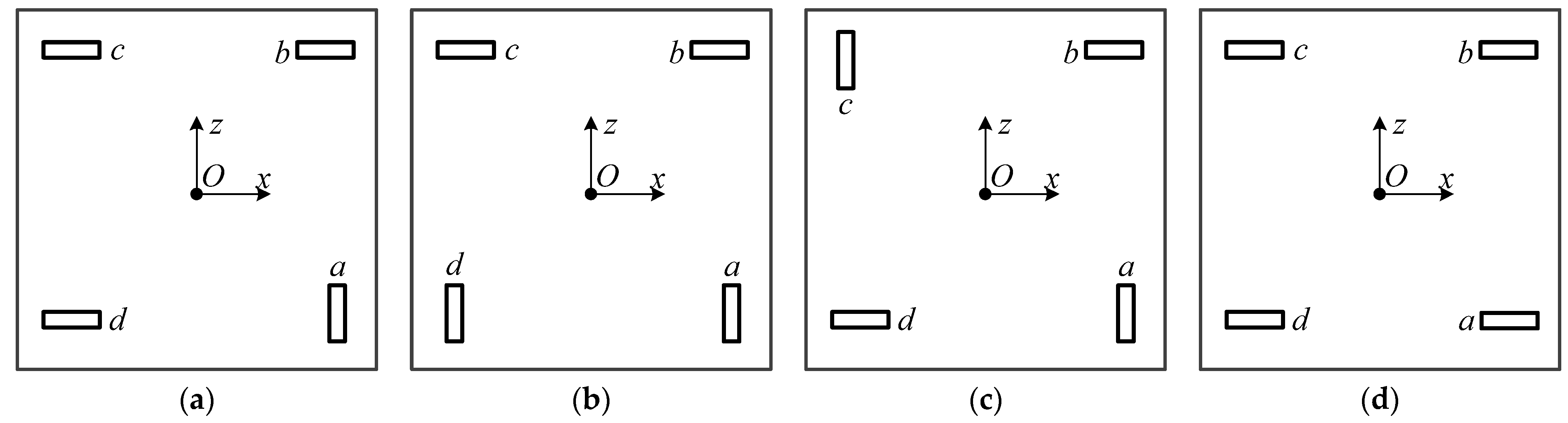
2.1.3. Analysis of Positioning Mode and Layout Design for the Capture and Lock System
2.2. Structural Design and Trajectory Planning for the Capture and Lock System
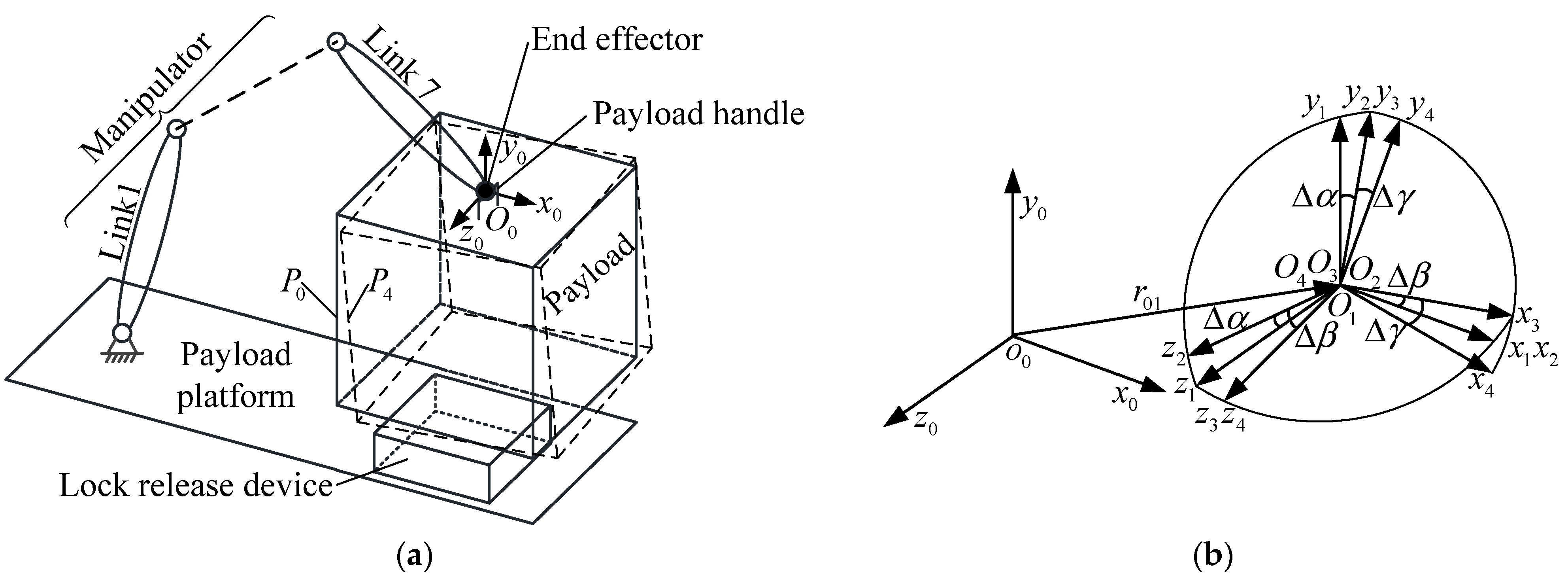
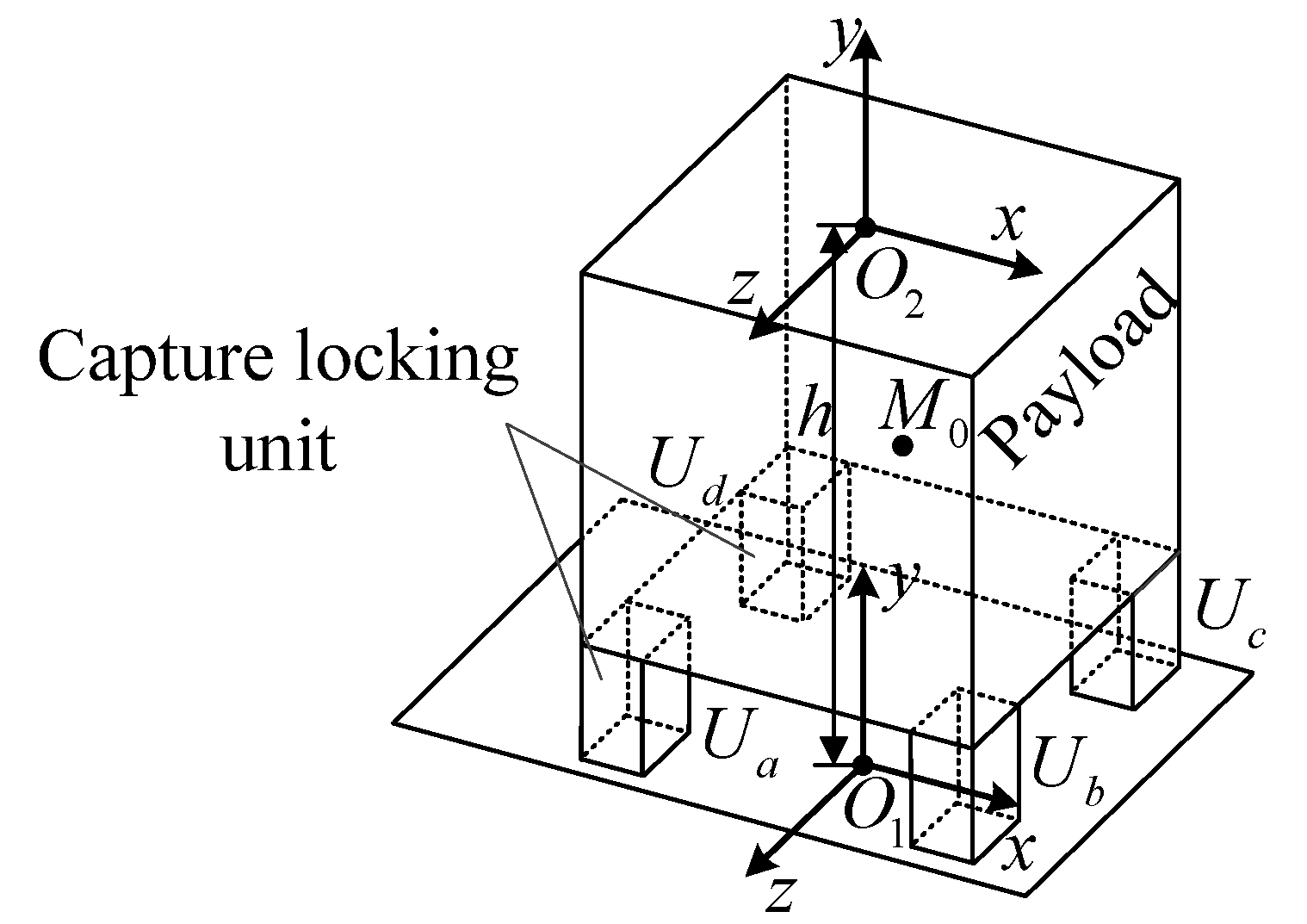
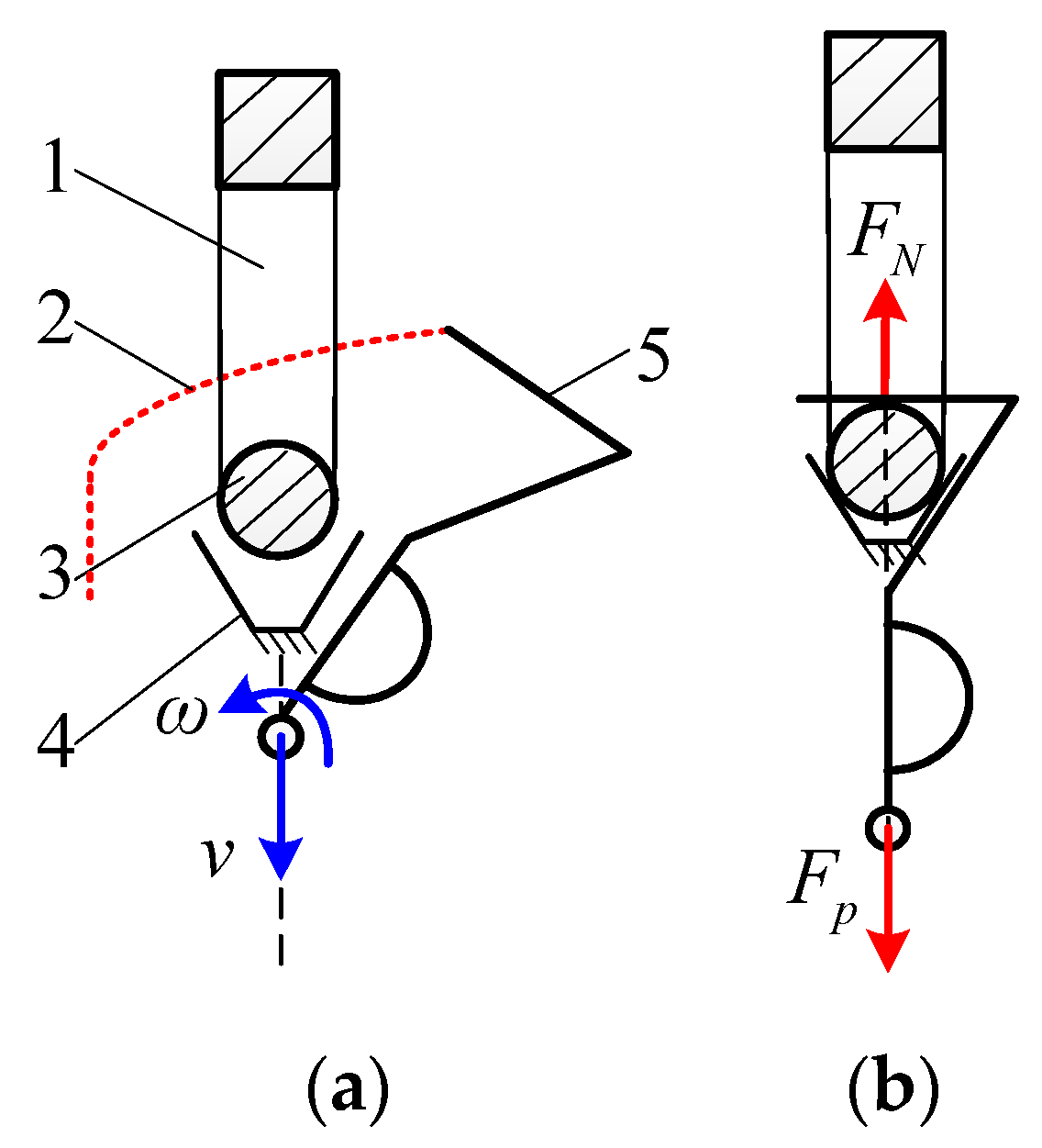
2.2.1. Analysis of Passive End Error Domain and Configuration Design of Capture Frame
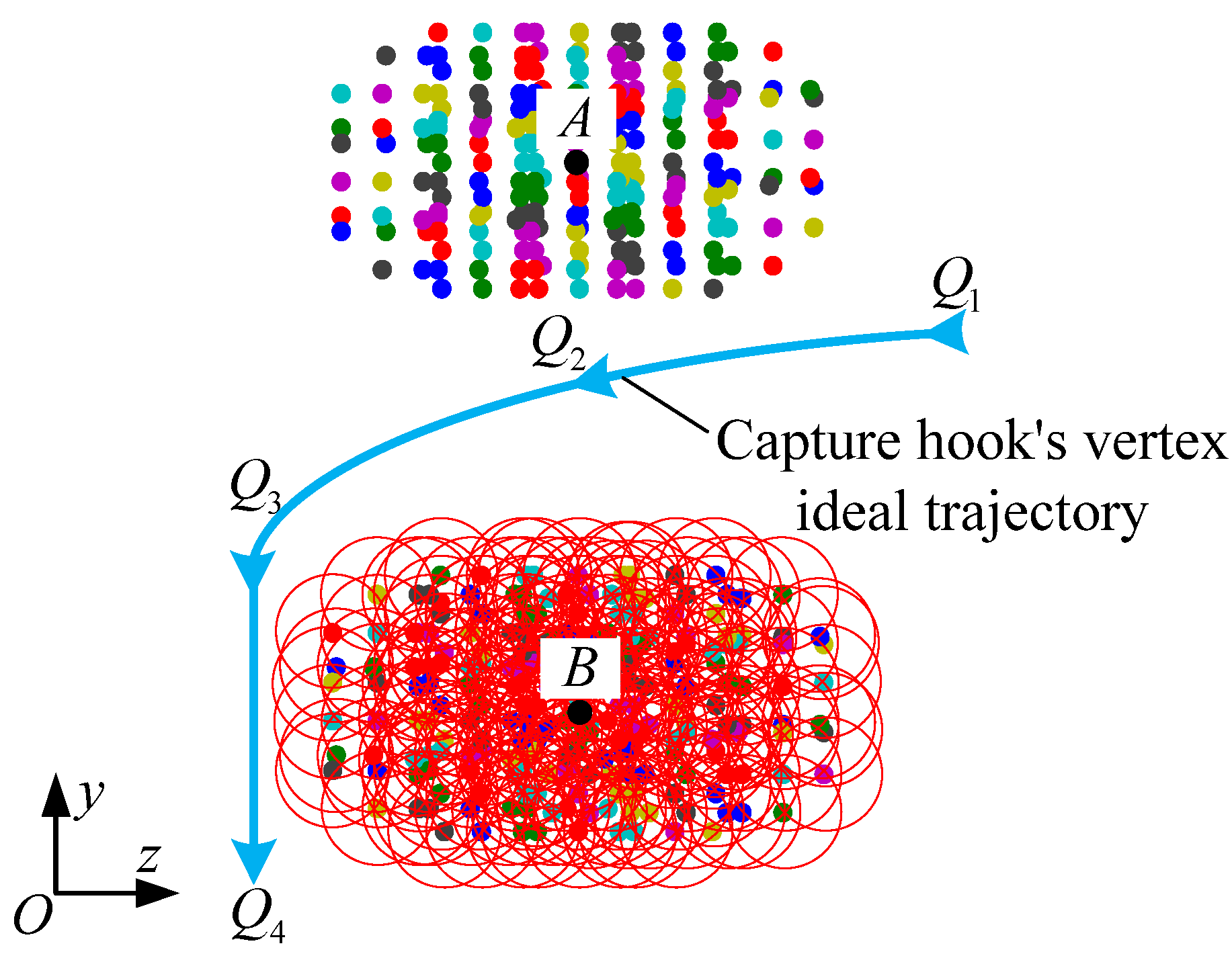
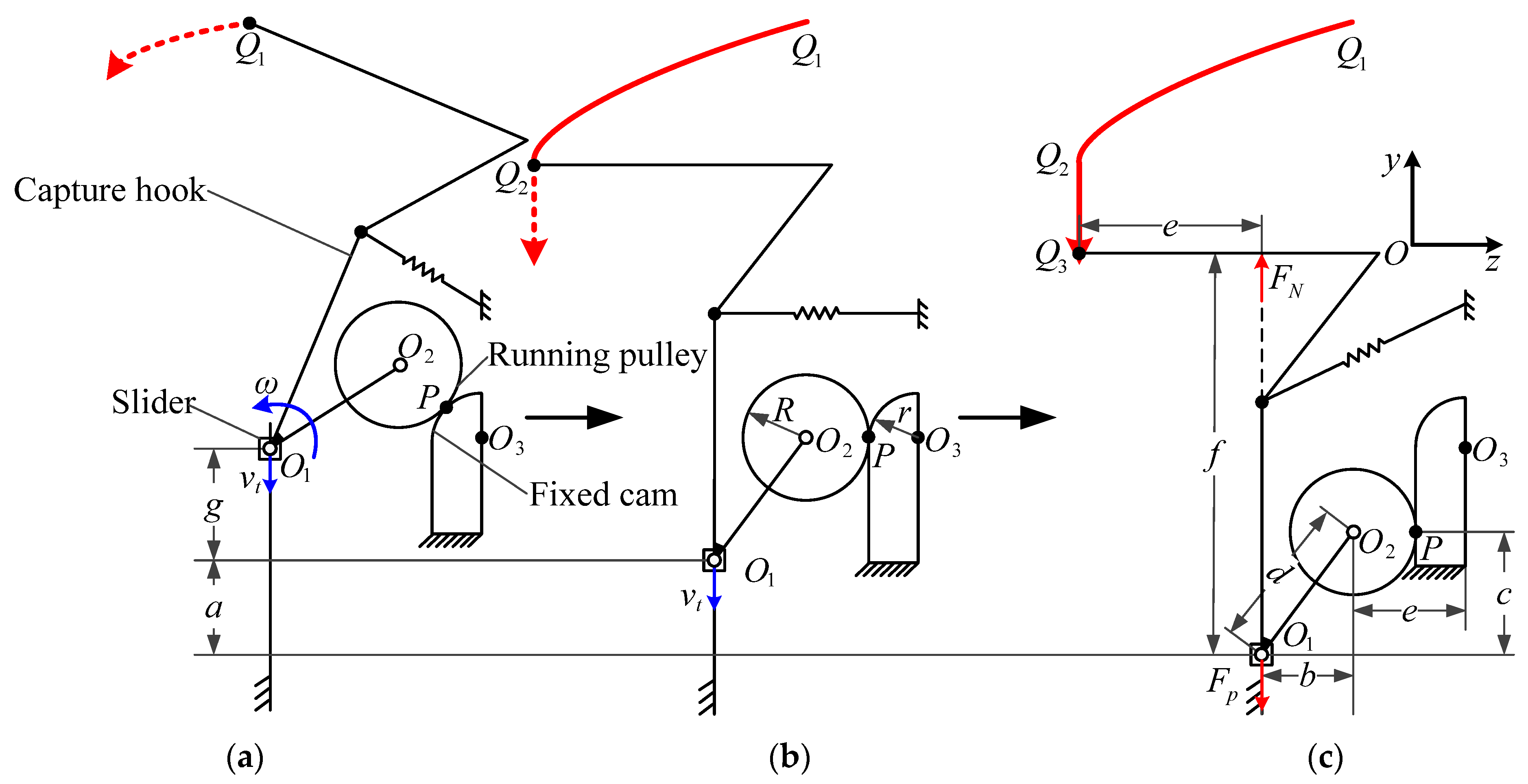
2.2.2. Planning the Capture Trajectory of the Active End and the Configuration Design of the Capture Hook
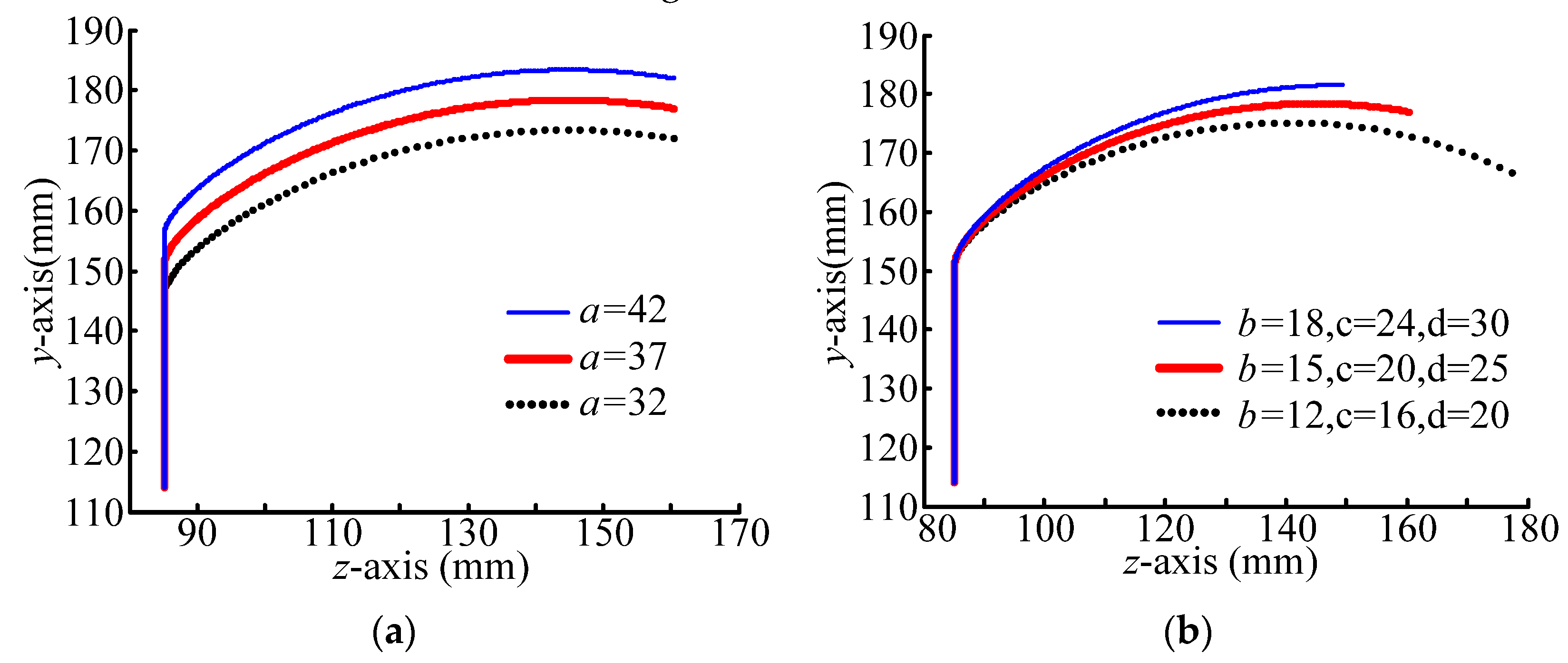
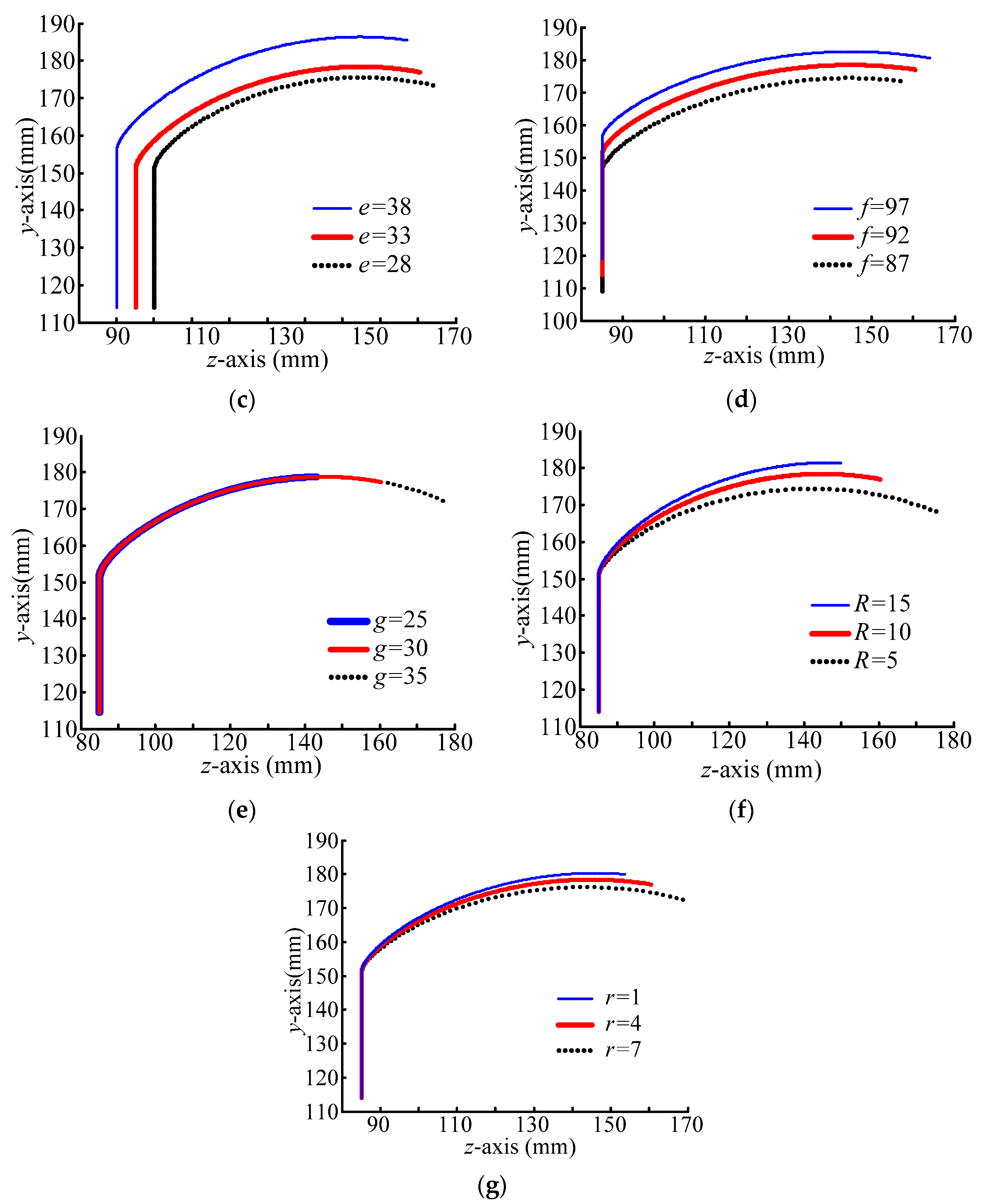
2.2.3. Configuration Parameter Optimization for the Capture and Lock System
2.3. Capture Hook Layout Design
2.3.1. Analysis of Capture Hook Layout
2.3.2. Simulation Verification of Tolerance Capability for the Layout of Capture and Lock System
- According to the boundary conditions of the structure size of the space payload and the working requirements of the capture and lock system, determine the simplest layout for the capture and lock system that will fully reflect the working conditions of the system;
- Determine the initial tolerance T0 and increase the tolerance by , then the system tolerance will be ;
- Convert the tolerance index Tm into a limit boundary pose that can be tested;
- Carry out the capture judgments for the limit boundary pose, and if all poses are successfully captured, set n = n+1, and continuously increase the tolerance for capture. If not all captures are successful, the system tolerance will be .
3. Results
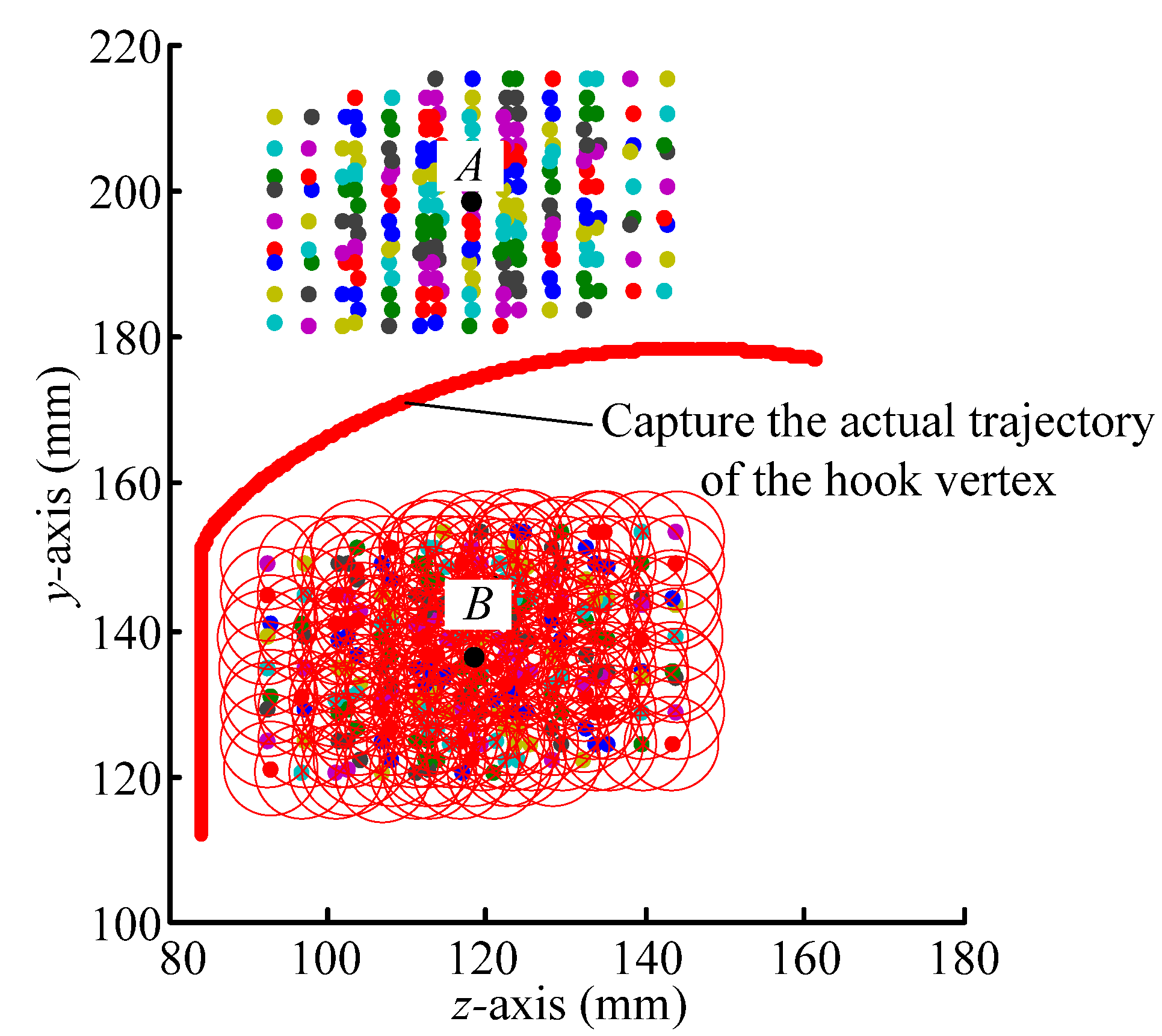
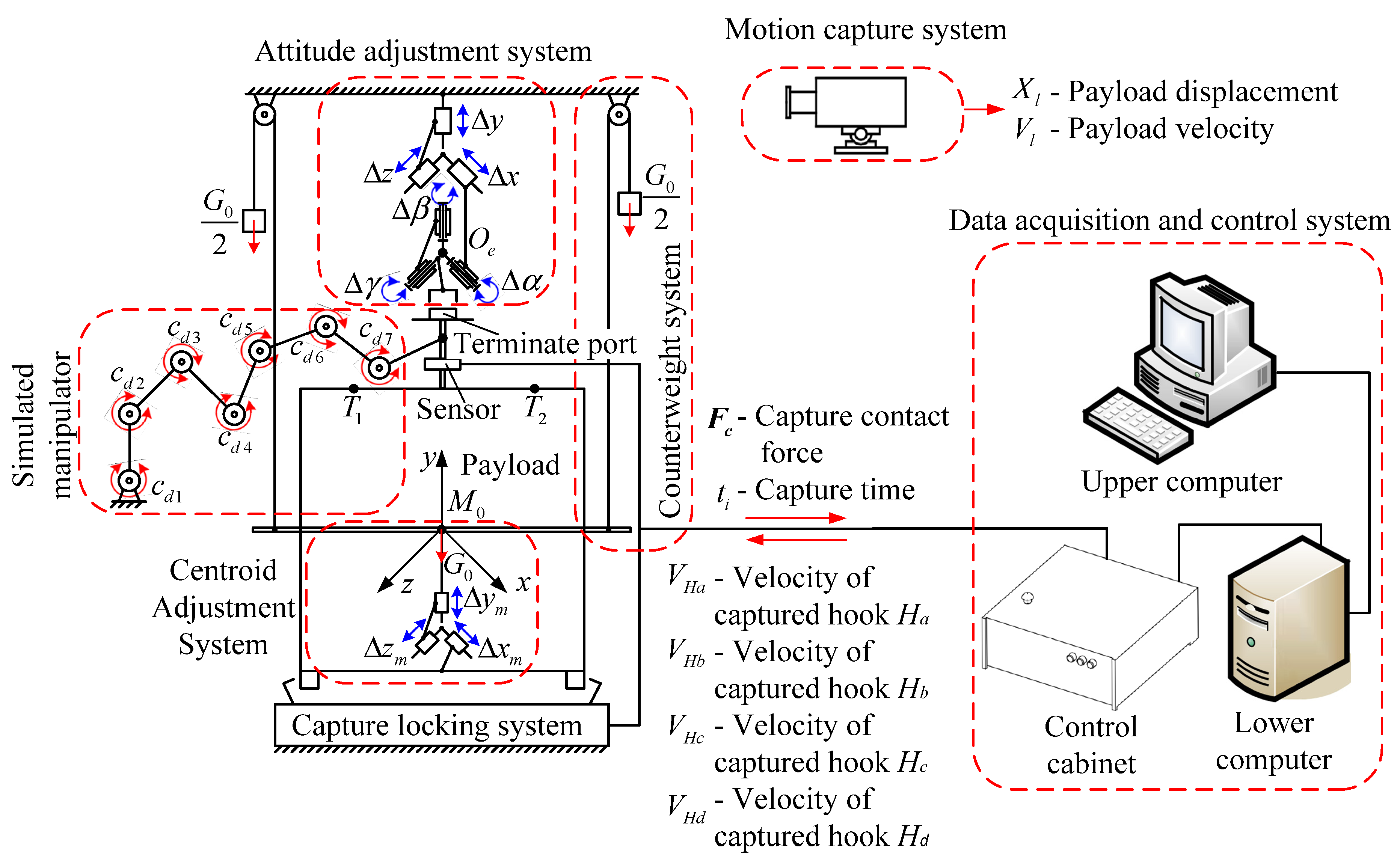
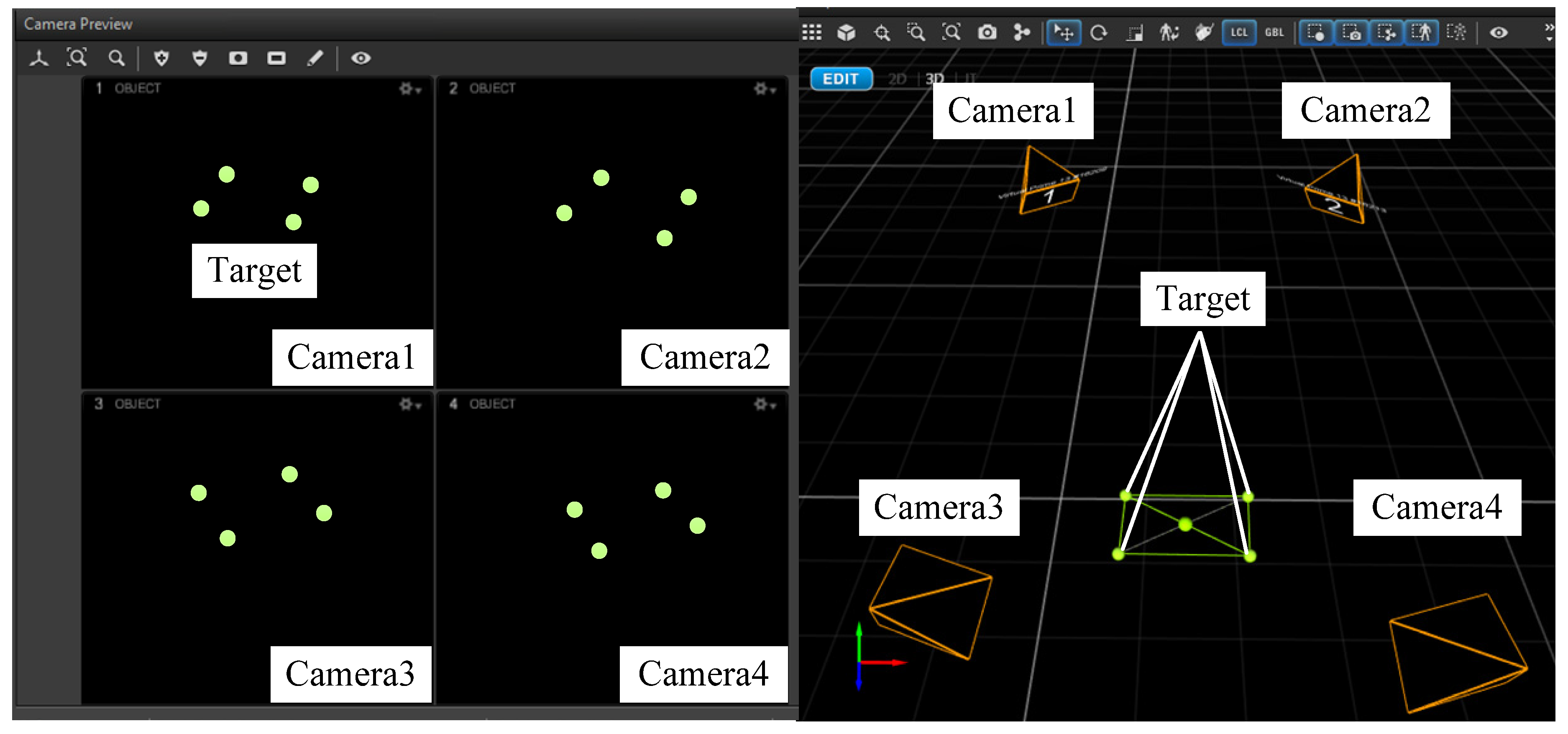
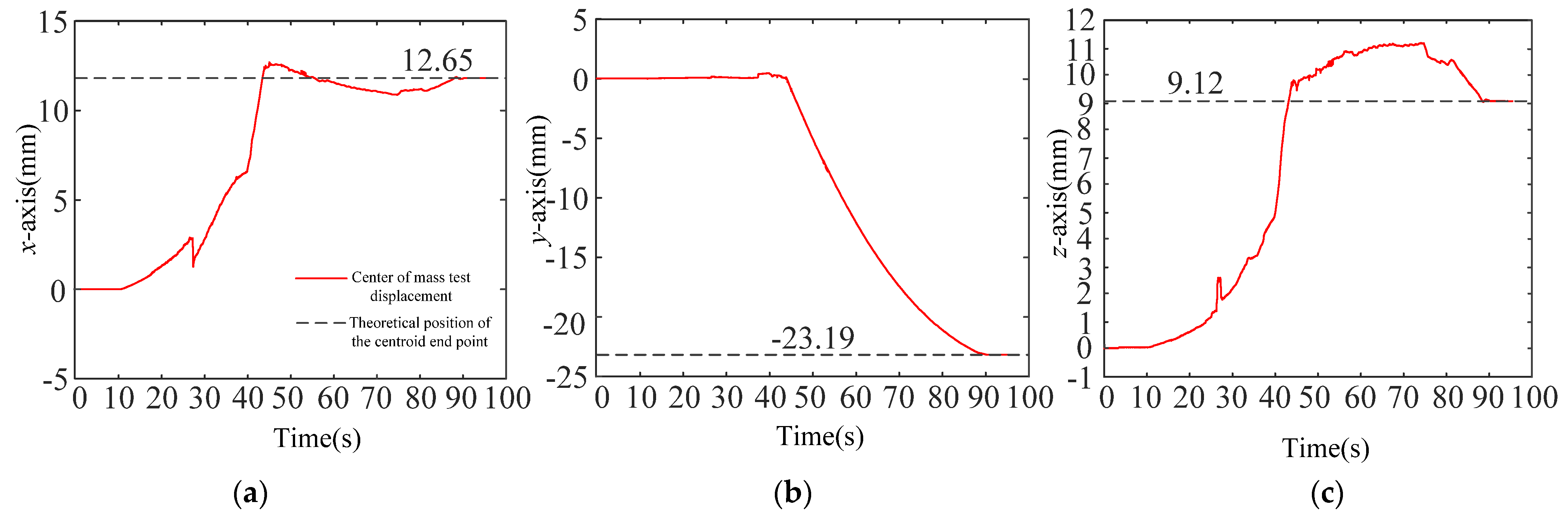
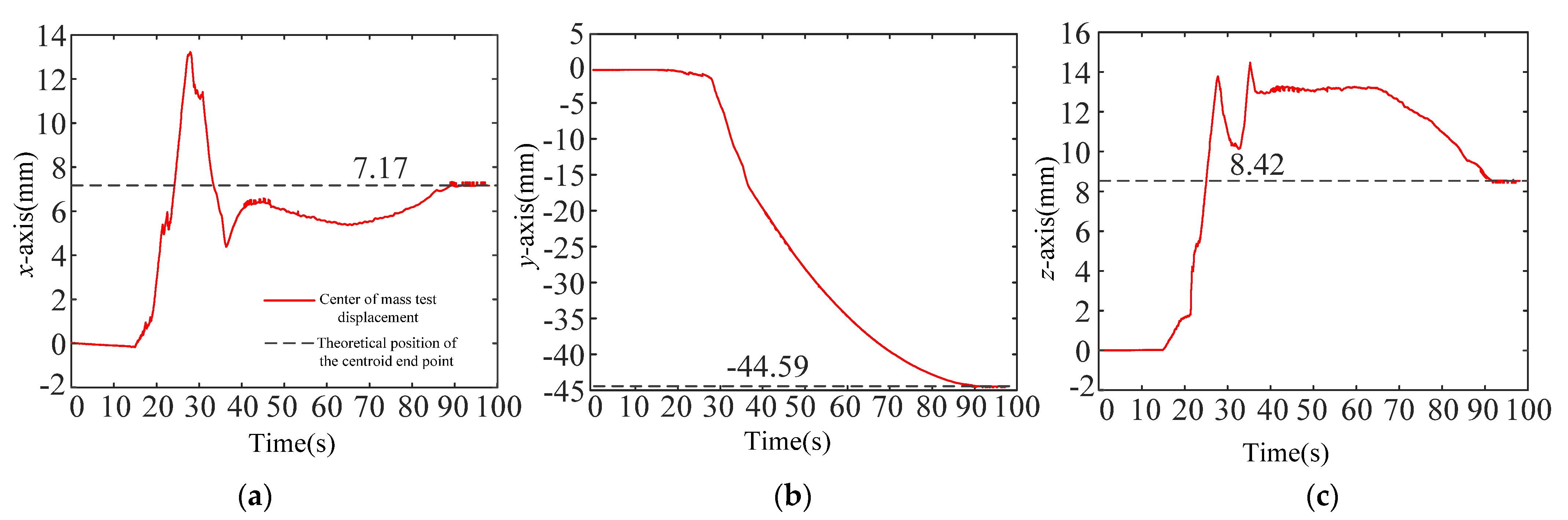
3.1. Development of a Space Payload Ground Capture Test System
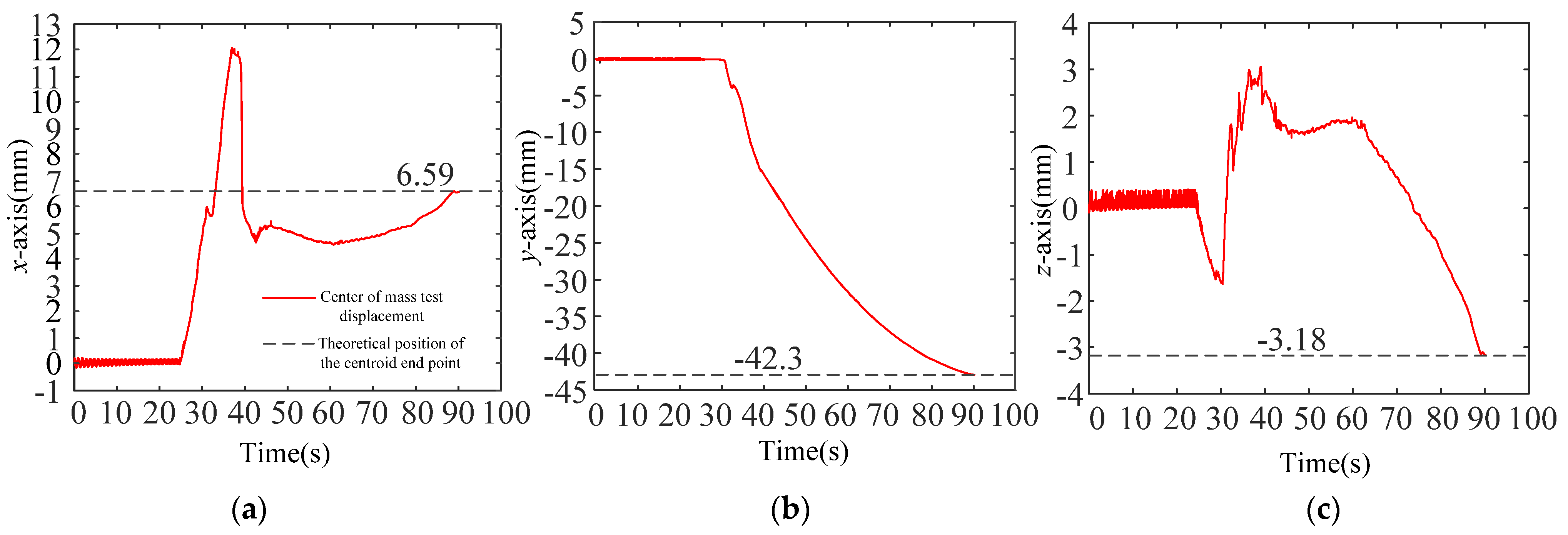
3.2. Experimental Verification of Capturing Tolerance Capability
4. Discussion
- A multi-point over-positioning evaluation model was established, the positioning design of the capture and lock mechanism was carried out, and a positioning mechanism with an orthogonal layout was obtained, which created a foundation for designing the configuration of the capture and lock mechanism;
- Through the analysis of the payload attitude and the error domain for the passive end, the planning and design of the capture trajectory of the active end was completed, and the configuration of the passive end was obtained by comprehensively considering the optimal dynamic performance of the system. A mathematical model for the capture and lock mechanism was established, and the ideal trajectory parameters for the active end were obtained. Through the analysis of the capture trajectory curve, optimization of the configuration parameters for the capture lock system was completed;
- To improve payload attitude adjustment, the system positioning method was comprehensively considered, the layout design of the active end was carried out, and the system layout with the largest tolerance capacity and optimal attitude adjustment was obtained;
- A simulation was used to verify the layout tolerance capability of the capture lock system, a space payload ground capture test system was developed, and a system layout tolerance capability test was carried out to verify the large-tolerance capability of the developed capture system.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhu, A.; Ai, H.; Chen, L. A Fuzzy Logic Reinforcement Learning Control with Spring-Damper Device for Space Robot Capturing Satellite. Appl. Sci. 2022, 12, 2662. [Google Scholar] [CrossRef]
- Carignan, C.; Scott, N.; Roderick, S. Hardware-in-the-loop simulation of satellite capture on a ground-based robotic testbed. In Proceedings of the International Symposium on Artificial Intelligence, Robotics and Automation in Space (i-SAIRAS), Montreal, QC, Canada, 17–19 July 2014; p. 6. [Google Scholar]
- Rekleitis, I.; Martin, E.; Rouleau, G.; L’Archevêque, R.; Parsa, K.; Dupuis, E. Autonomous capture of a tumbling satellite. J. Field Robot. 2007, 24, 275–296. [Google Scholar] [CrossRef]
- Kaiser, C.; Rank, P.; Krenn, R. Simulation of the docking phase for the SMART-OLEV satellite servicing mission. In Proceedings of the i-SAIRAS: International Symposium on Artificial Intelligence, Robotics and Automation in Space, Cologne, Germany, 25 February 2008; pp. 26–29. [Google Scholar]
- Huang, J.; Li, Z.; Huang, L.; Meng, B.; Han, X.; Pang, Y. Docking mechanism design and dynamic analysis for the GEO tumbling satellite. Assem. Autom. 2019, 39, 432–444. [Google Scholar] [CrossRef]
- Jaworski, J.; Dudek, L.; Wolski, M.; Mateja, A.; Wittels, P.; Labecki, M. Grippers for Launch Adapter Rings of Non-cooperative Satellites Capture for Active Debris Removal, Space Tug and On-Orbit Satellite Servicing Applications Holl. ASTRA ESTEC 2017. Available online: https://www.semanticscholar.org/paper/GRIPPERS-FOR-LAUNCH-ADAPTER-RINGS-OF-SATELLITES-FOR-Jaworski-Dudek/edd6822509bbc83b1be9640dfa840e1d39983576 (accessed on 12 May 2022).
- Rouleaut, G.; Martin, E.; Sharf, I. Trajectory Generation for Satellite Capture Using a Redundant Manipulator. In Romansy 16; Springer: Vienna, Austria, 2006; pp. 413–420. [Google Scholar]
- Wang, G.; Xie, Z.; Lu, Y.; Wang, J.; Wu, M.; Yang, F.; Jiang, S. Analysis method of the capture tolerance capability for an orthogonally distributed satellite capture device. IEEE Access 2019, 7, 55022–55034. [Google Scholar] [CrossRef]
- Wang, G.; Yang, F.; Jiang, S.; Yue, H.; Lu, Y.; Wu, M. Determination method of capture for an orthogonal distributed satellite capture device. IEEE Access 2018, 6, 61800–61811. [Google Scholar] [CrossRef]
- Wang, G.; Xie, Z.; Mu, X.; Li, S.; Yang, F.; Yue, H.; Jiang, S. Docking Strategy for a Space Station Container Docking Device Based on Adaptive Sensing. IEEE Access 2019, 7, 100867–100880. [Google Scholar] [CrossRef]
- Ma, Z.; Ma, O.; Shashikanth, B.N. Optimal approach to and alignment with a rotating rigid body for capture. J. Astronaut. Sci. 2007, 55, 407–419. [Google Scholar] [CrossRef]
- Wang, H.; Xie, Y. On the uniform positive definiteness of the estimated inertia for robot manipulators. IFAC Proc. Vol. 2011, 44, 4089–4094. [Google Scholar] [CrossRef] [Green Version]
- Wang, F.; Sun, F.; Liu, H. Space robot modeling and control considering the effect of orbital mechanics. In Proceedings of the 2006 1st International Symposium on Systems and Control in Aerospace and Astronautics, Harbin, China, 19–21 January 2006; Volume 6, p. 198. [Google Scholar]
- Rybus, T.; Seweryn, K.; Sasiadek, J.Z. Trajectory optimization of space manipulator with non-zero angular momentum during orbital capture maneuver. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, San Diego, CA, USA, 4–8 January 2016; p. 0885. [Google Scholar]
- Xu, W.; Liu, Y.; Xu, Y. The coordinated motion planning of a dual-arm space robot for target capturing. Robotica 2012, 30, 755–771. [Google Scholar] [CrossRef]
- Tortopidis, I.; Papadopoulos, E. On point-to-point motion planning for underactuated space manipulator systems. Robot. Auton. Syst. 2007, 55, 122–131. [Google Scholar] [CrossRef]
- Aghili, F. Coordination control of a free-flying manipulator and its base attitude to capture and detumble a noncooperative satellite. In Proceedings of the 2009 IEEE/RSJ International Conference on Intelligent Robots and Systems, St. Louis, MO, USA, 10–15 October 2009; pp. 2365–2372. [Google Scholar]
- Piersigilli, P.; Sharf, I.; Misra, A.K. Reactionless capture of a satellite by a two degree-of-freedom manipulator. Acta Astronautica 2010, 66, 183–192. [Google Scholar] [CrossRef]
- Wang, G.; Yang, F.; Yue, H.; Jiang, S. Optimal layout method of distributed locking device of satellite based on plant root growth theory. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 2802–2818. [Google Scholar] [CrossRef]
- Wang, G.; Yang, F.; Yue, H.; Jiang, S.; Wang, K. A new approach for design of a satellite modular reusable locking-release device. In Proceedings of the 2017 IEEE International Conference on Information and Automation (ICI(A), Macau, China, 18–20 July 2017; pp. 559–564. [Google Scholar]
- Lim, J.; Chung, J. Dynamic analysis of a tethered satellite system for space debris capture. Nonlinear Dyn. 2018, 94, 2391–2408. [Google Scholar] [CrossRef]
- Yoshida, K.; Dimitrov, D.; Nakanishi, H. On the capture of tumbling satellite by a space robot. In Proceedings of the 2006 IEEE/RSJ International Conference on Intelligent Robots and Systems, Beijing, China, 9–13 October 2006; pp. 4127–4132. [Google Scholar]
- Yoshida, K.; Nakanishi, H.; Ueno, H.; Inaba, N.; Nishimaki, T.; Oda, M. Dynamics, control and impedance matching for robotic capture of a non-cooperative satellite. Adv. Robot. 2004, 18, 175–198. [Google Scholar] [CrossRef]










| Serial Number | Positioning Form | Positioning Piece (a) | Positioning Piece (b) | Positioning Diagram | Restricted Degrees of Freedom | |
|---|---|---|---|---|---|---|
| 1 | Point positioning | Support nails | Flat piece | 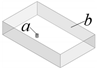 | One movement degree of freedom | |
| 2 | Line positioning | Linear positioning | Long support plate | Flat piece | 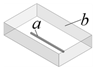 | One movement degree of freedom and one rotational degree of freedom |
| Curve positioning | Taper pin | Cylinder | 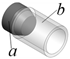 | Two movement degrees of freedom | ||
| V-groove | Circular shaft | 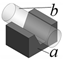 | Two movement degrees of freedom and two rotational degrees of freedom | |||
| 3 | Face positioning | Plane positioning | Support plate | Flat piece |  | One movement degree of freedom and two rotational degrees of freedom |
| Rectangular pin | Rectangular tube |  | Two movement degrees of freedom and three rotational degrees of freedom | |||
| V-groove | V-block | 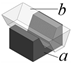 | ||||
| Surface positioning | Round pin | Cylinder | 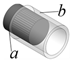 | Two movement degrees of freedom and two rotational degrees of freedom | ||
| Circular cone | Tapered hole |  | Three movement degrees of freedom and two rotational degrees of freedom | |||
| Positioning Method | C1 | C2 | C2l | C2k | C3 | C3l | C3k | C4 | C4l | C4k | C | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Circular shaft-V-groove | Three-one orthogonal | 4 | 4 | 0 | 0 | 4 | 1 | 1 | 4 | 1 | 1 | 10 |
| Two-two orthogonal | 4 | 4 | 0 | 0 | 4 | 2 | 2 | 4 | 0 | 0 | 10 | |
| V-block-V-groove | Three-one orthogonal | 5 | 5 | 0 | 0 | 5 | 0 | 0 | 5 | 1 | 1 | 14 |
| Two-two orthogonal | 5 | 5 | 0 | 0 | 5 | 1 | 1 | 5 | 0 | 0 | 14 | |
| Circular cone-tapered hole | 5 | 5 | 1 | 1 | 5 | 0 | 0 | 5 | 0 | 0 | 14 | |
| Parameter | a/mm | b/mm | c/mm | d/mm | e/mm | f/mm | g/mm | R/mm | r/mm |
|---|---|---|---|---|---|---|---|---|---|
| Value | 37 | 15 | 20 | 25 | 33 | 92 | 30 | 10 | 4 |
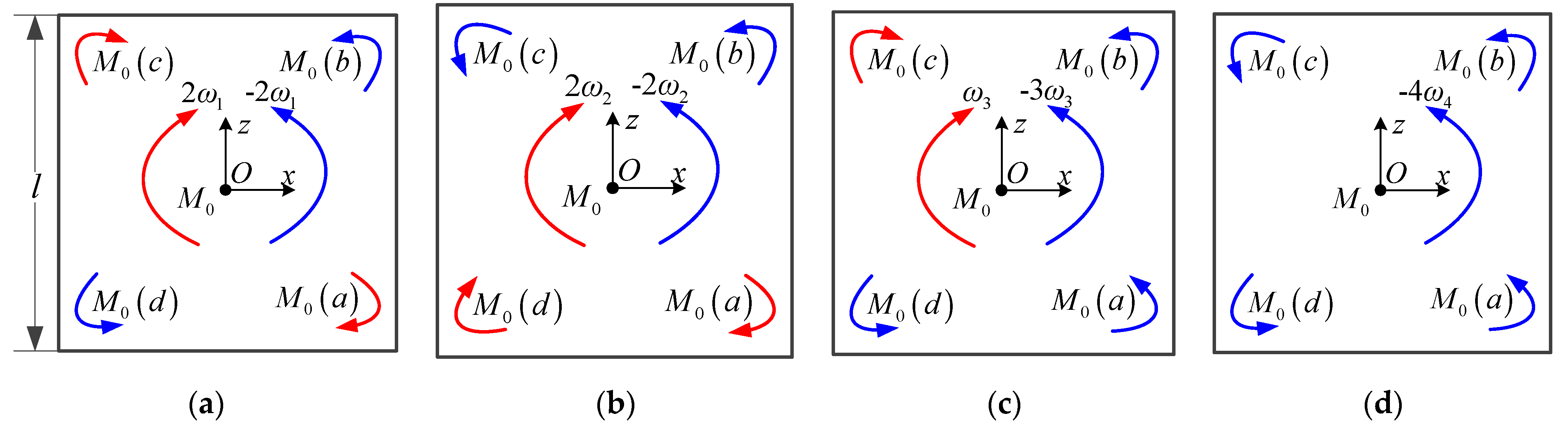
| Three-One Orthogonal Layout | Two-Two Diagonal Orthogonal Layout | Two-Two Unilateral Orthogonal Layout | Parallel Layout | |
|---|---|---|---|---|
| Two-two moments unilateral balance | ||||
| Two-two moments diagonal balance | ||||
| Three-one moments reversal | ||||
| Four moments equal |
| Boundary Pose | x/mm | y/mm | z/mm | |||
|---|---|---|---|---|---|---|
| P1 | 10 | −10 | 10 | 1 | −1 | −1 |
| P2 | −10 | 10 | 10 | 1 | 1 | −1 |
| P3 | 10 | 10 | −10 | −1 | −1 | 1 |
| P4 | 10 | 10 | 10 | −1 | 1 | −1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Yao, Y.; Wang, J.; Huo, W.; Xu, G.; Hu, X. Layout Design and Verification of a Space Payload Distributed Capture and Lock System. Aerospace 2022, 9, 345. https://doi.org/10.3390/aerospace9070345
Wang G, Yao Y, Wang J, Huo W, Xu G, Hu X. Layout Design and Verification of a Space Payload Distributed Capture and Lock System. Aerospace. 2022; 9(7):345. https://doi.org/10.3390/aerospace9070345
Chicago/Turabian StyleWang, Gang, Yimeng Yao, Jingtian Wang, Weiye Huo, Guosheng Xu, and Xi Hu. 2022. "Layout Design and Verification of a Space Payload Distributed Capture and Lock System" Aerospace 9, no. 7: 345. https://doi.org/10.3390/aerospace9070345
APA StyleWang, G., Yao, Y., Wang, J., Huo, W., Xu, G., & Hu, X. (2022). Layout Design and Verification of a Space Payload Distributed Capture and Lock System. Aerospace, 9(7), 345. https://doi.org/10.3390/aerospace9070345






