Influence of Fluid Viscosity and Compressibility on Nonlinearities in Generalized Aerodynamic Forces for T-Tail Flutter
Abstract
:1. Introduction
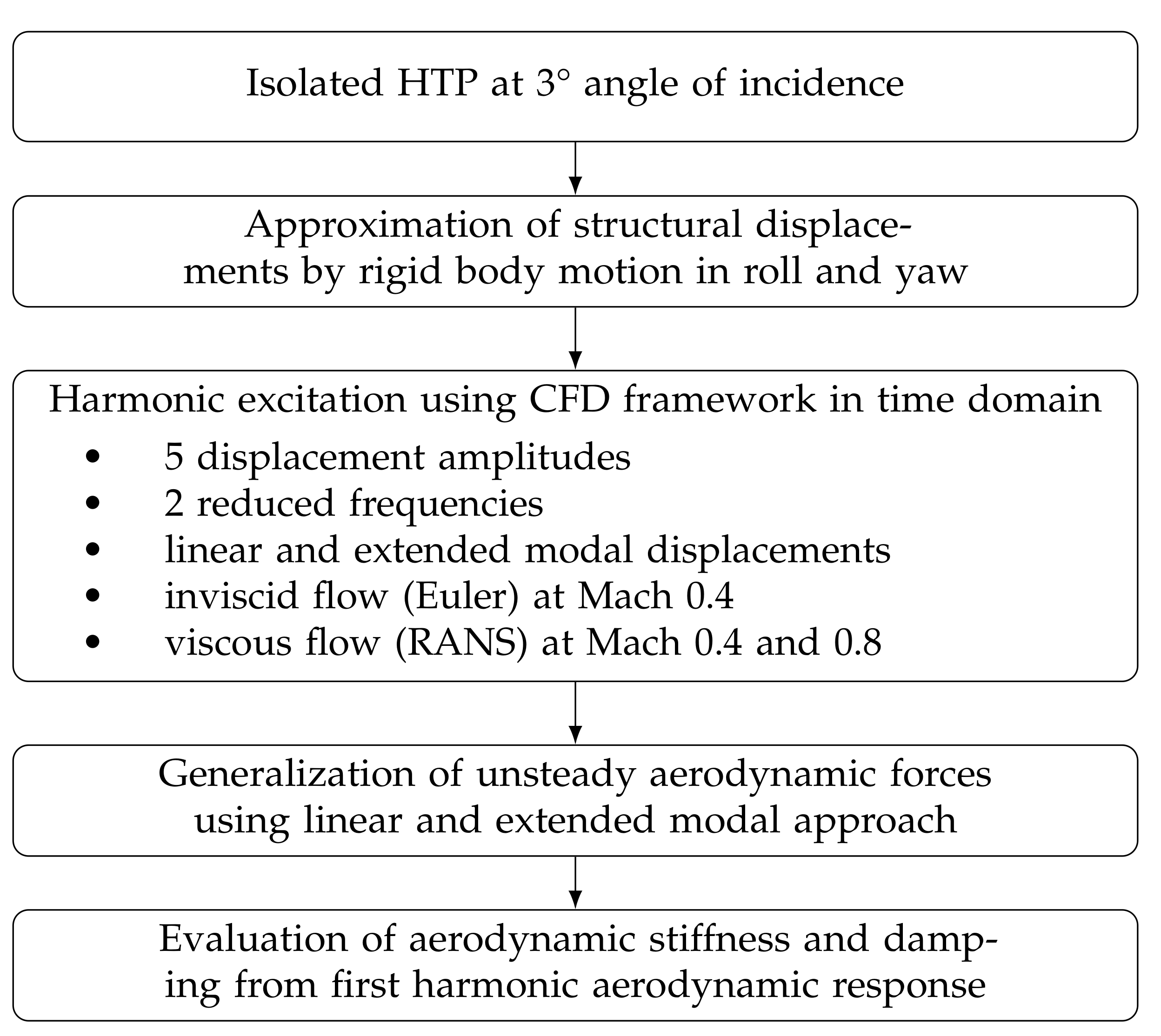
2. Approach and Simulation Models
2.1. Extended Modal Approach
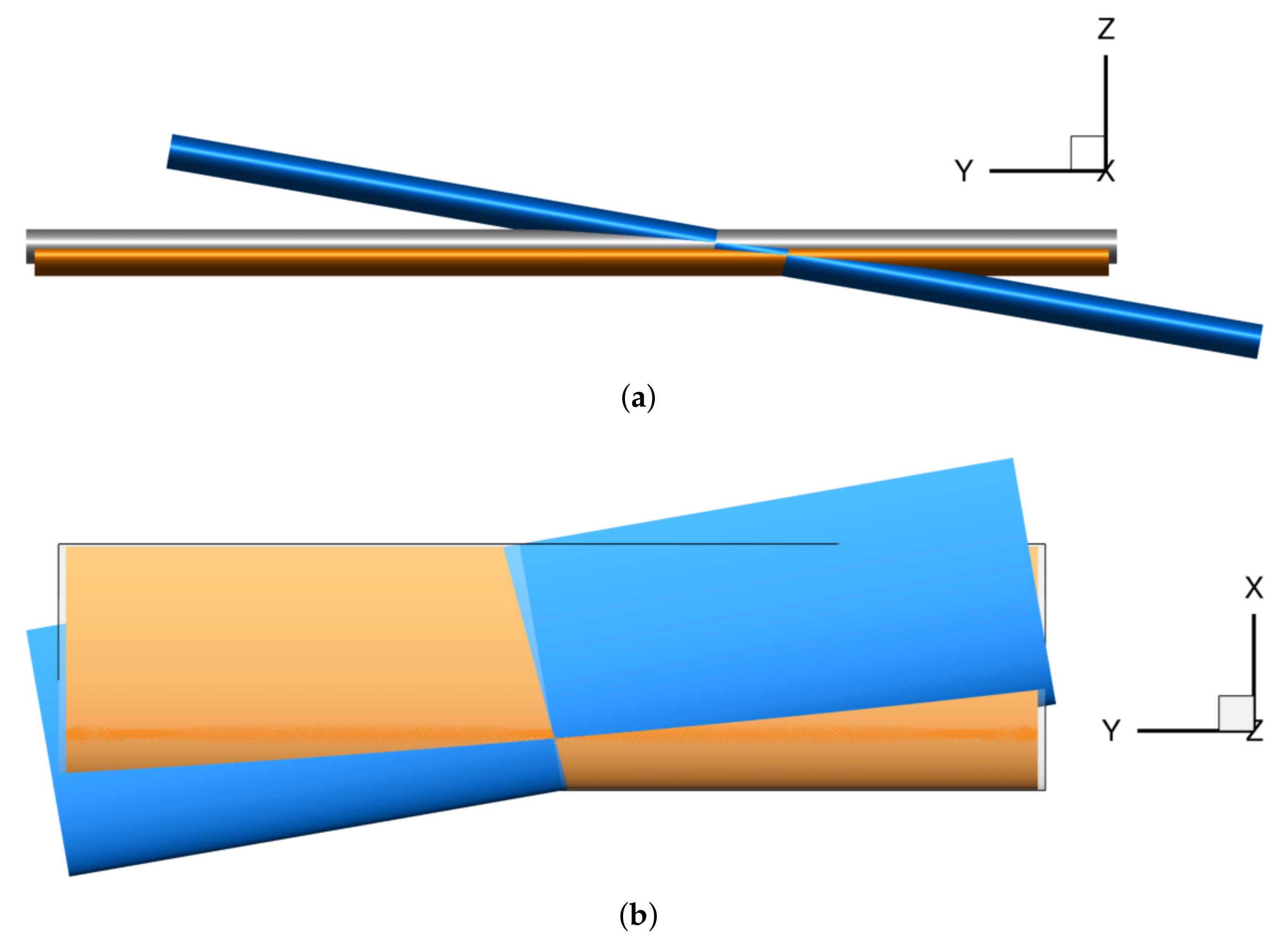
2.2. Obtaining Quadratic Mode Shape Components
2.3. Time Domain Harmonic Forced Motion
2.4. Aerodynamic Stiffness and Damping from Time Domain Results
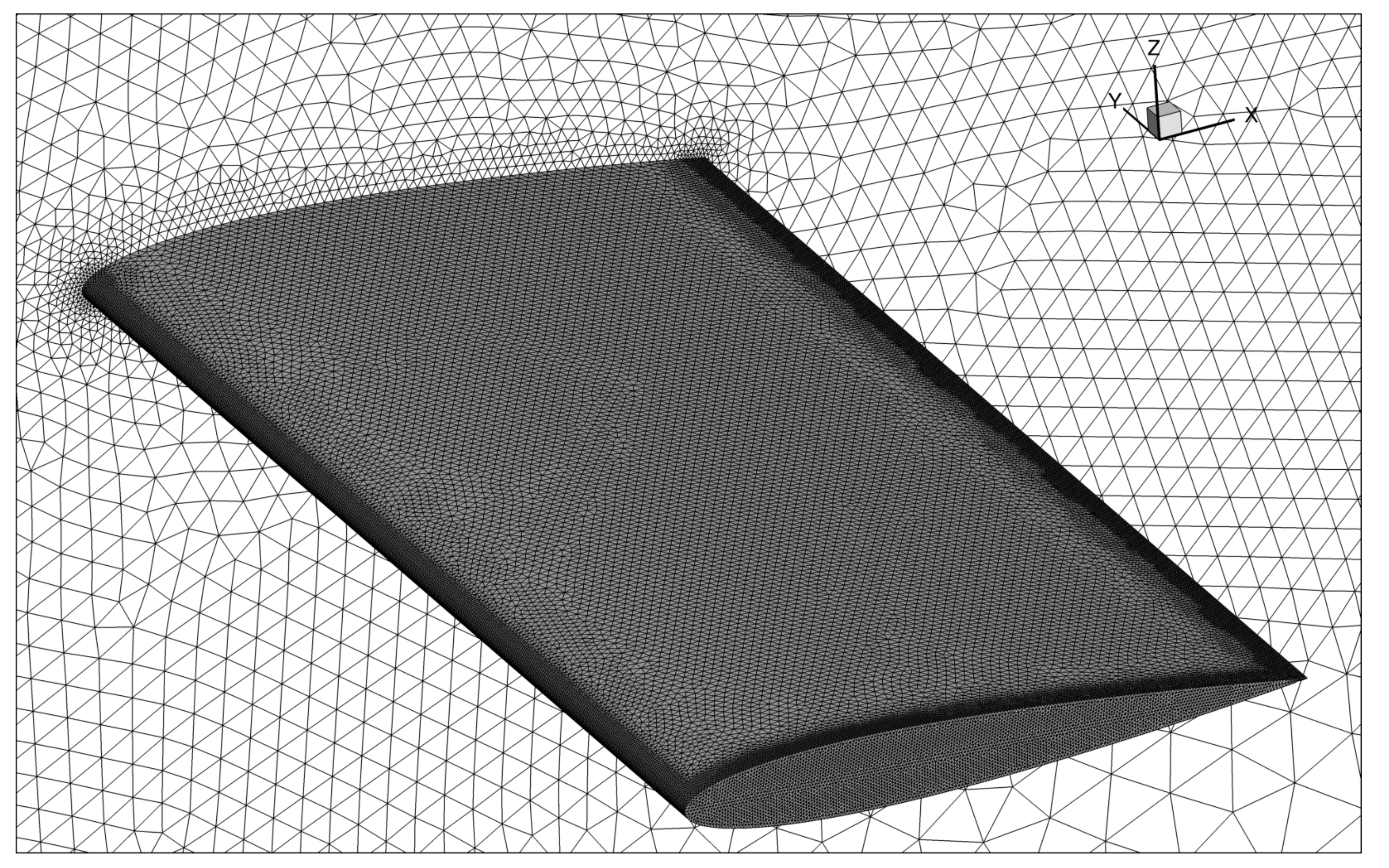
2.5. CFD Models
2.5.1. Inviscid Flow
2.5.2. Viscous Flow
2.6. Temporal Discretization
3. Results
3.1. Inviscid Flow
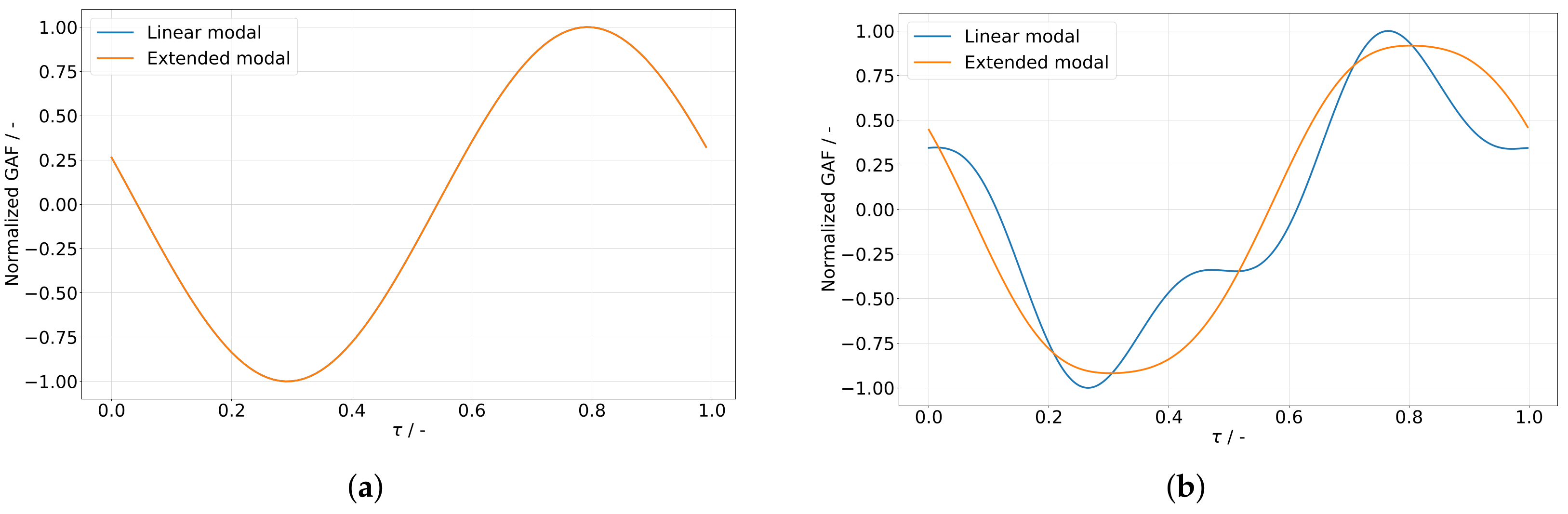
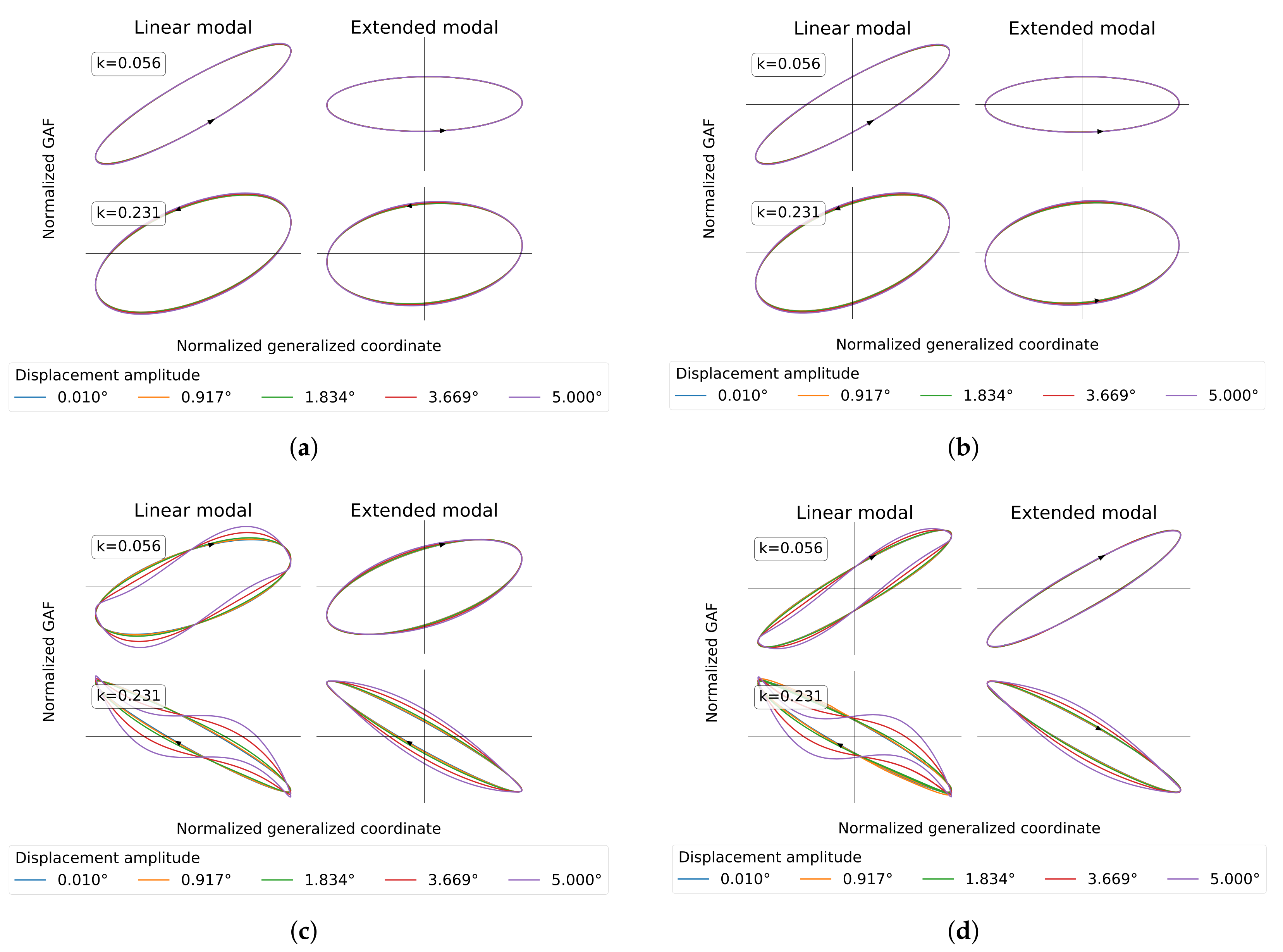
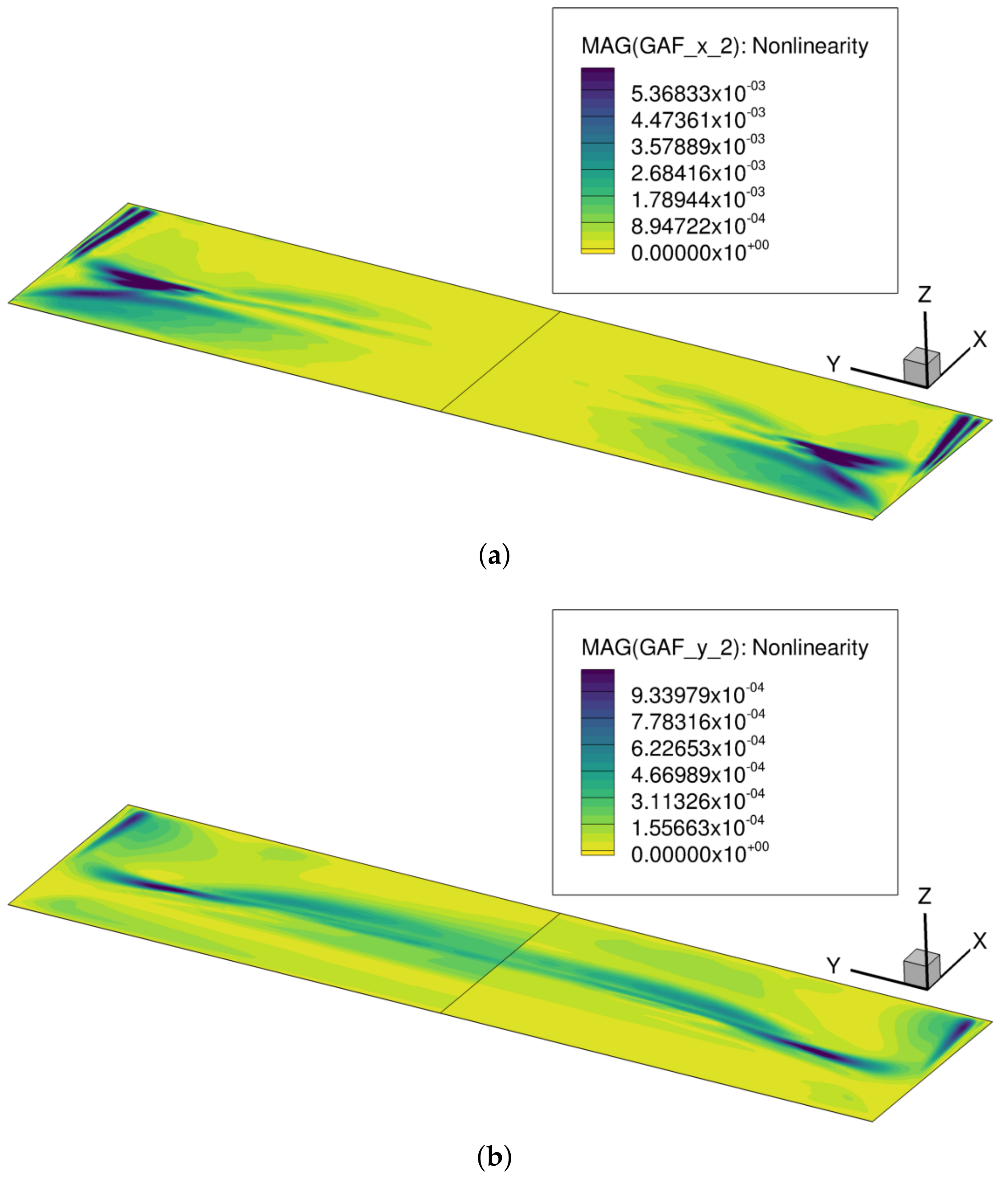
3.1.1. GAF Hystereses
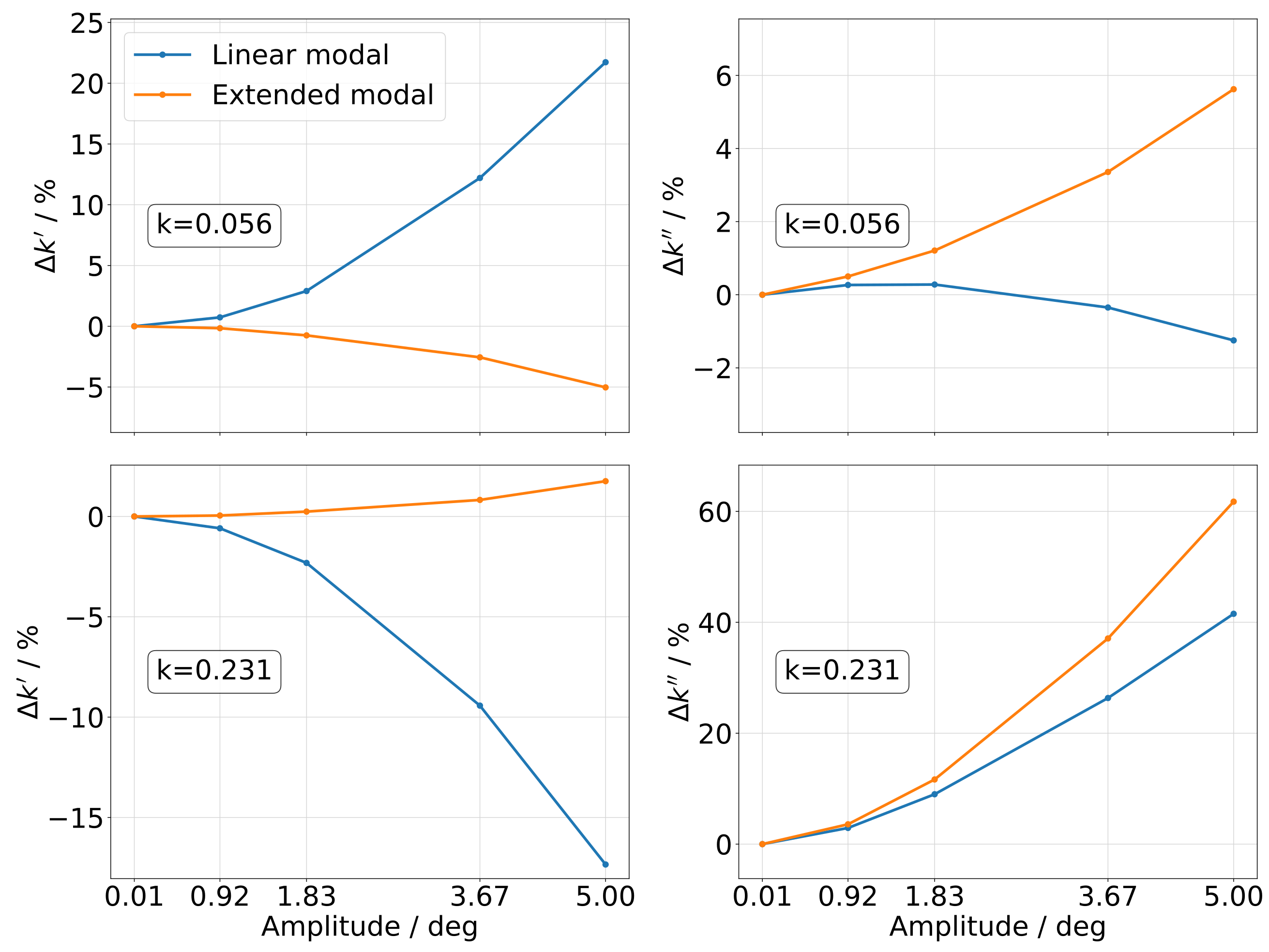
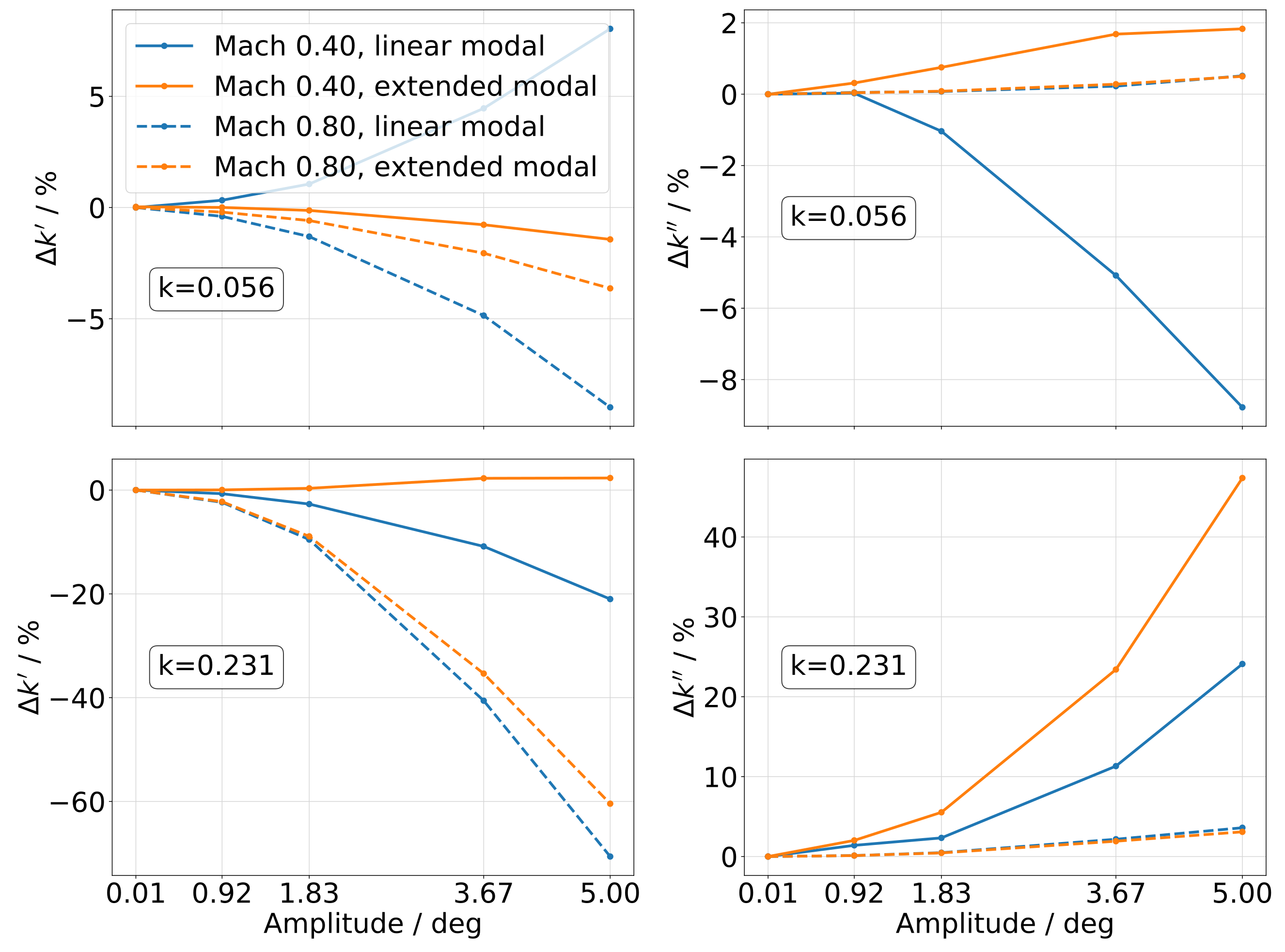
3.1.2. Aerodynamic Stiffness and Damping
- Aerodynamic stiffness:
- –
- Nonlinear for low and high reduced frequencies with linear displacements;
- –
- Only marginally nonlinear for low and high reduced frequencies with quadratic displacements.
- Aerodynamic damping:
- –
- Marginally nonlinear for low reduced frequencies and both displacement descriptions;
- –
- Nonlinear for high reduced frequencies and both displacement descriptions;
- –
- Increased nonlinearity with quadratic displacements.
3.2. Impact of Fluid Viscosity
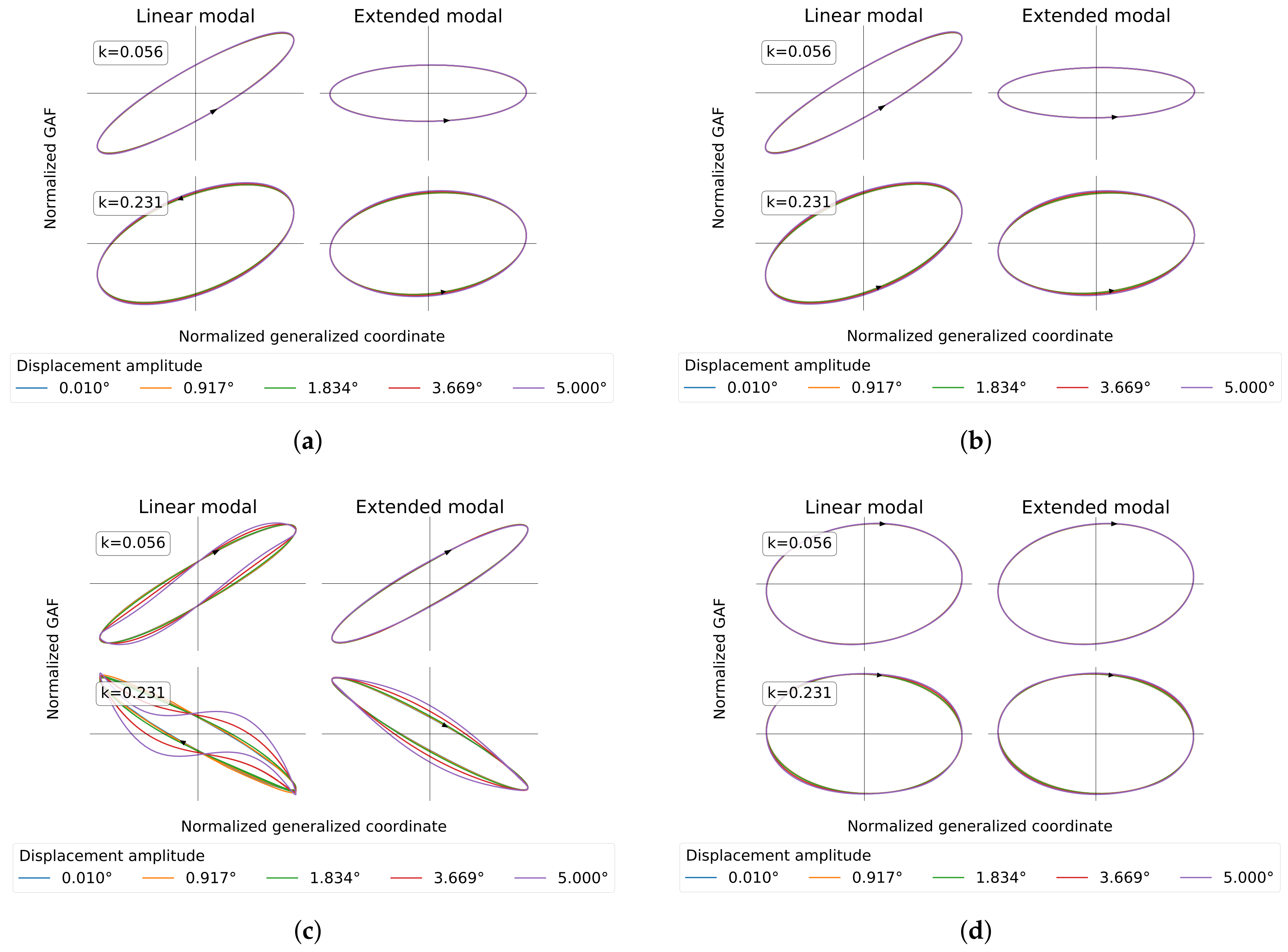
3.2.1. GAF Hystereses
3.2.2. Aerodynamic Stiffness and Damping
- Aerodynamic stiffness:
- –
- Significant and amplitude-independent offset with respect to inviscid flow results;
- –
- No remarkable impact on nonlinear character.
- Aerodynamic damping:
- –
- Marginally larger damping at small displacement amplitudes and low reduced frequencies;
- –
- Marginally lower damping at small displacement amplitudes and high reduced frequencies;
- –
- Increased nonlinearity for linear modal displacement at low reduced frequencies;
- –
- Decreased nonlinearity for extended modal displacement at low reduced frequencies;
- –
- Decreased nonlinearity for linear and extended modal displacement at high reduced frequencies.
3.3. Impact of Fluid Compressibility
3.3.1. GAF Hystereses
3.3.2. Aerodynamic Stiffness and Damping
- Aerodynamic stiffness:
- –
- Increased nonlinearity for low and high reduced frequencies;
- –
- Reduced impact of geometric nonlinearity, especially at high reduced frequencies.
- Aerodynamic damping:
- –
- Nonlinearity is almost entirely canceled;
- –
- No impact of geometric nonlinearity.
4. Discussion
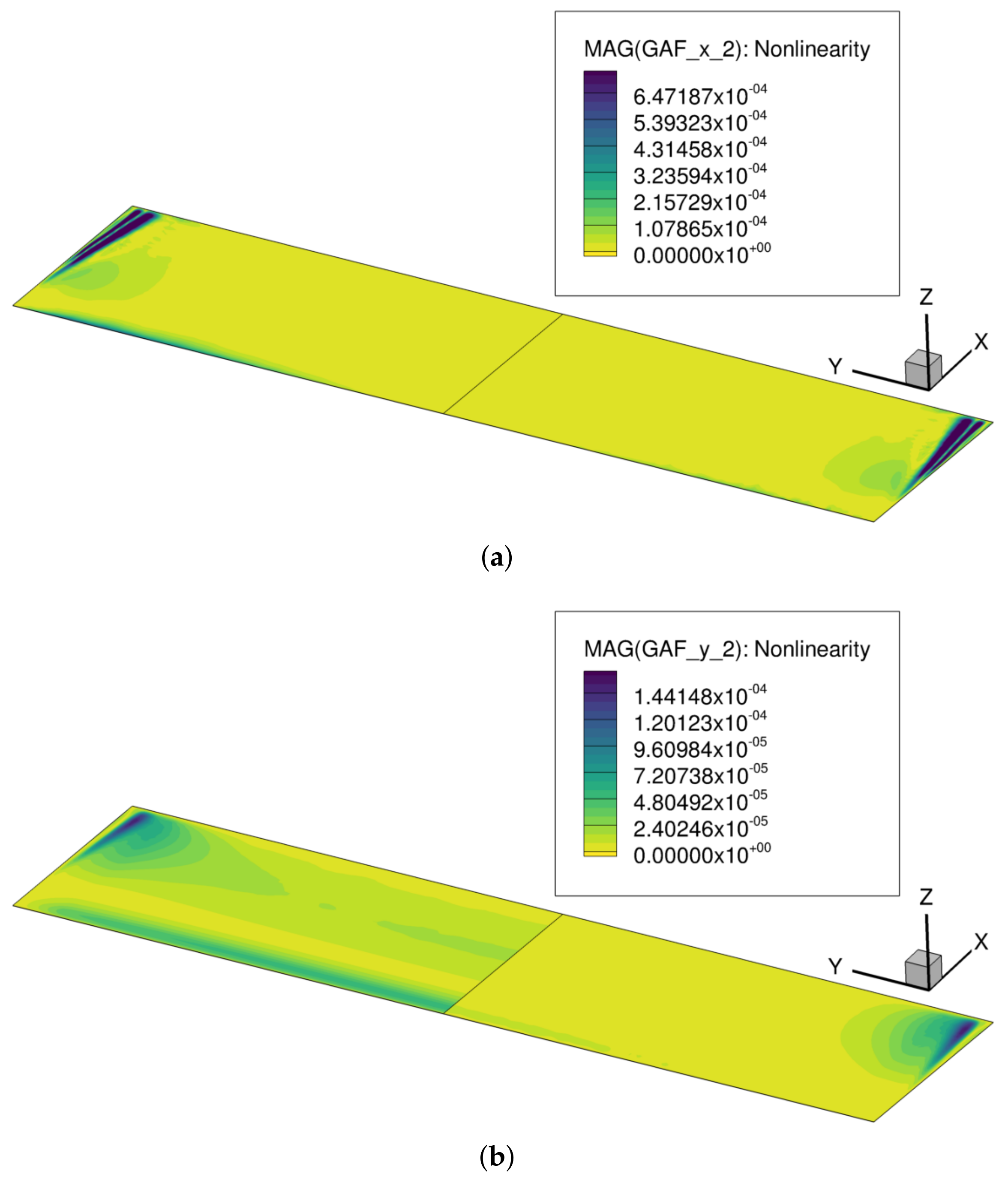
4.1. Physical Sources for Aerodynamic Nonlinearities
4.2. Impact of Fluid Compressibility
4.3. Summary of Aerodynamic Coupling Term Nonlinearity and Implications for T-Tail Flutter
5. Conclusions and Outlook
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jennings, W.P.; Berry, M.A. Effect of Stabilizer Dihedral and Static Lift on T-Tail Flutter. J. Aircr. 1977, 14, 364–367. [Google Scholar] [CrossRef]
- Suciu, E. MSC/Nastran Flutter Analyses of T-Tails Including Horizontal Stabilizer Static Lift Effects and T-Tail Transonic Dip. In Proceedings of the 1996 MSC/Nastran World Users’ Conference, Newport Beach, CA, USA, 3–7 June 1996. [Google Scholar]
- Suciu, E.; Stathopoulos, N.; Dickinson, M.; Glaser, J. A DLM-Based MSC Nastran Aerodynamic Flutter Simulator for Aircraft Lifting Surfaces. In Proceedings of the MSC Software 2013 Users’ Conference, Irvine, CA, USA, 7 May 2013. [Google Scholar]
- Queijo, M.J. Theory For Computing Span Loads and Stability Derivatives Due to Sideslip, Yawing, and Rolling for Wings in Subsonic Compressible Flow; Technical Report; NASA Langley Research Center: Washington, DC, USA, 1968. [Google Scholar]
- Theodorsen, T. General Theory of Aerodynamic Instability and the Mechanism of Flutter; Technical Report; National Advisory Committee for Aeronautics, Langley Aeronautical Lab: Langley Field, VA, USA, 1935. [Google Scholar]
- Van Zyl, L. A DLM for T-Tails. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics, Stockholm, Sweden, 24–26 June 2007. [Google Scholar]
- Van Zyl, L. Advanced Linear Methods for T-Tail Aeroelasticity. Ph.D. Thesis, North-West University, Potchefstroom Campus, Potchefstroom, South Africa, 2011. [Google Scholar]
- Murua, J.; van Zyl, L.; Palacios, R. T-Tail flutter: Potential-flow modelling, experimental validation and flight tests. Prog. Aerosp. Sci. 2014, 71, 54–84. [Google Scholar] [CrossRef]
- Arizono, H.; Kheirandisch, H.R.; Nakamichi, J. Flutter simulations of a T-tail configuration using non-linear aerodynamics. Int. J. Numer. Methods Eng. 2007, 72, 1513–1523. [Google Scholar] [CrossRef]
- Attorni, A.; Cavagna, L.; Quaranta, G. Aircraft T-tail flutter predictions using computational fluid dynamics. J. Fluids Struct. 2011, 27, 161–174. [Google Scholar] [CrossRef]
- Isogai, K. Subsonic and Transonic Flow Simulation for an Oscillating T-Tail—Effects of In-plane Motion of Horizontal Tail Plane—. Trans. Jpn. Soc. Aeronaut. Space Sci. 2016, 59, 25–32. [Google Scholar] [CrossRef] [Green Version]
- Isogai, K. Numerical Simulation of Transonic Flutter of a T-Tail. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics, Como, Italy, 25–28 June 2017. [Google Scholar]
- Ruhlin, C.; Sandford, M. Experimental Parametric Studies of Transonic T-Tail Flutter; Technical Report; National Aeronautics and Space Administration: Washington, DC, USA, 1975. [Google Scholar]
- Santos, E.; Berrera, J.; Martinez, A.; Martinez, P.; Rafael de la Cruz, V.; Arevalo, F.; Karpel, M.; Climent, H. Development of a CFD-based industrial procedure for supporting the aircraft certification with application to A400M T-Tail flutter. In Proceedings of the Second International Symposium on Flutter and Its Application, Paris, France, 12–14 May 2020. [Google Scholar]
- Baldock, J. The Determination of the Flutter Speed of a T-Tail Unit by Calculations, Model Tests and Flight Flutter Tests; Technical Report; Advisory Group for Aerospace Research and Development: Paris, France, 1958. [Google Scholar]
- Van Zyl, L.; Mathews, E.H. Aeroelastic Analysis of T-Tails Using an Enhanced Doublet Lattice Method. J. Aircr. 2011, 48, 823–831. [Google Scholar] [CrossRef] [Green Version]
- Van Zyl, L.H.; Sutherland, A.N.; Rossouw, P.S. Parabolic mode shapes: What they are, where to get them and what to do with them. In Proceedings of the International Forum on Aeroelasticity and Structural Dynamics, Seattle, WA, USA, 21–25 June 2009. [Google Scholar]
- Segalman, D.J.; Dohrmann, C.R. A method for calculating the dynamics of rotating flexible structures, part 1: Derivation. J. Vib. Acoust. 1996, 118, 313–317. [Google Scholar] [CrossRef]
- Ritter, M.R. An Extended Modal Approach for Nonlinear Aeroelastic Simulations of Highly Flexible Aircraft Structures. Ph.D. Thesis, Technische Universität Berlin, Berlin, Germany, 2019. [Google Scholar]
- Jung, K.J.; Lee, J.; Kwon, J.H.; Kang, I. The quadratic mode method for fluid-structure coupling of high aspect ratio wing. In Proceedings of the 12th AIAA/ISSMO Multidisciplinary Analysis and Optimization Conference, Victoria, BC, Canada, 10–12 September 2008. [Google Scholar]
- Farao, J.; Malan, A.G.; Gambioli, F. Towards a Non-Linear Full Aircraft Model For Passenger Aircraft Loads Calculations. In Proceedings of the International Forum Aeroelasticity and Structural Dynamics, Como, Italy, 25–28 June 2017. [Google Scholar]
- Schäfer, D. Nonlinearities in generalized aerodynamic forces in the context of T-tail flutter. In Proceedings of the Aerospace Europe Conference, Warsaw, Poland, 23–26 November 2021. [Google Scholar]
- Schäfer, D. T-tail flutter simulations with regard to quadratic mode shape components. CEAS Aeronaut. J. 2021, 12, 621–632. [Google Scholar] [CrossRef]
- Allmaras, S.R.; Johnson, F.T.; Spalart, P.R. Modifications and clarifications for the implementation of the Spalart-Allmaras turbulence model. In Proceedings of the Seventh International Conference on Computational Fluid Dynamics (ICCFD7), Big Island, HI, USA, 9–13 July 2012. [Google Scholar]
- Van Zyl, L.; Mathews, E.H. Quadratic Mode Shape Components From Linear Finite Element Analysis. J. Vib. Acoust. 2012, 134, 014501. [Google Scholar] [CrossRef]
- Bronstein, I.N.; Semendjajew, K.A.; Musiol, G.; Mühlig, H. Taschenbuch der Mathematik, 10th ed.; Verlag Harri Deutsch: Frankfurt am Main, Germany, 2016. [Google Scholar]
- Wahle, M. Maschinendynamik; Lecture Notes; University of Applied Sciences Aachen: Aachen, Germany, 2008. [Google Scholar]
- Genta, G. Vibration Dynamics and Control; Springer: New York, NY, USA, 2009. [Google Scholar]
- Harris, C.M.; Piersol, A.G. Harris’ Shock and Vibration Handbook; McGraw-Hill: New York, NY, USA, 2002. [Google Scholar]
- Anderson, J.D. Fundamentals of Aerodynamics; McGraw-Hill, Inc.: New York, NY, USA, 1991. [Google Scholar]
- Tips & Tricks: Estimating the First Cell Height for Correct y+. Available online: https://www.computationalfluiddynamics.com.au/tips-tricks-cfd-estimate-first-cell-height/ (accessed on 17 June 2020).
- Gulfstream Aerospace. Available online: https://www.gulfstream.com (accessed on 18 March 2022).
- Van Rooij, A.C.L.M. Aeroelastic Limit-Cycle Oscillations Resulting from Aerodynamic Non-Linearities. Ph.D. Thesis, Technische Universiteit Delft, Delft, The Netherlands, 2017. [Google Scholar]
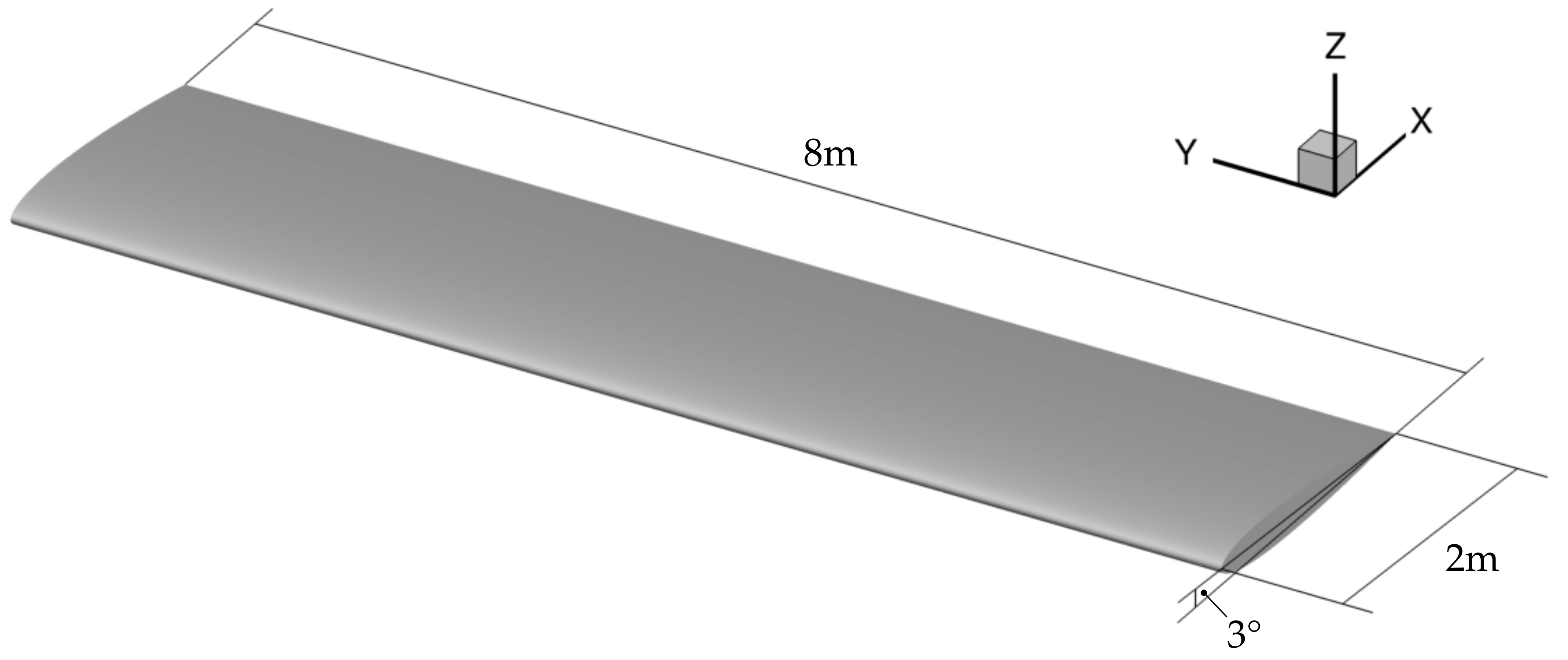


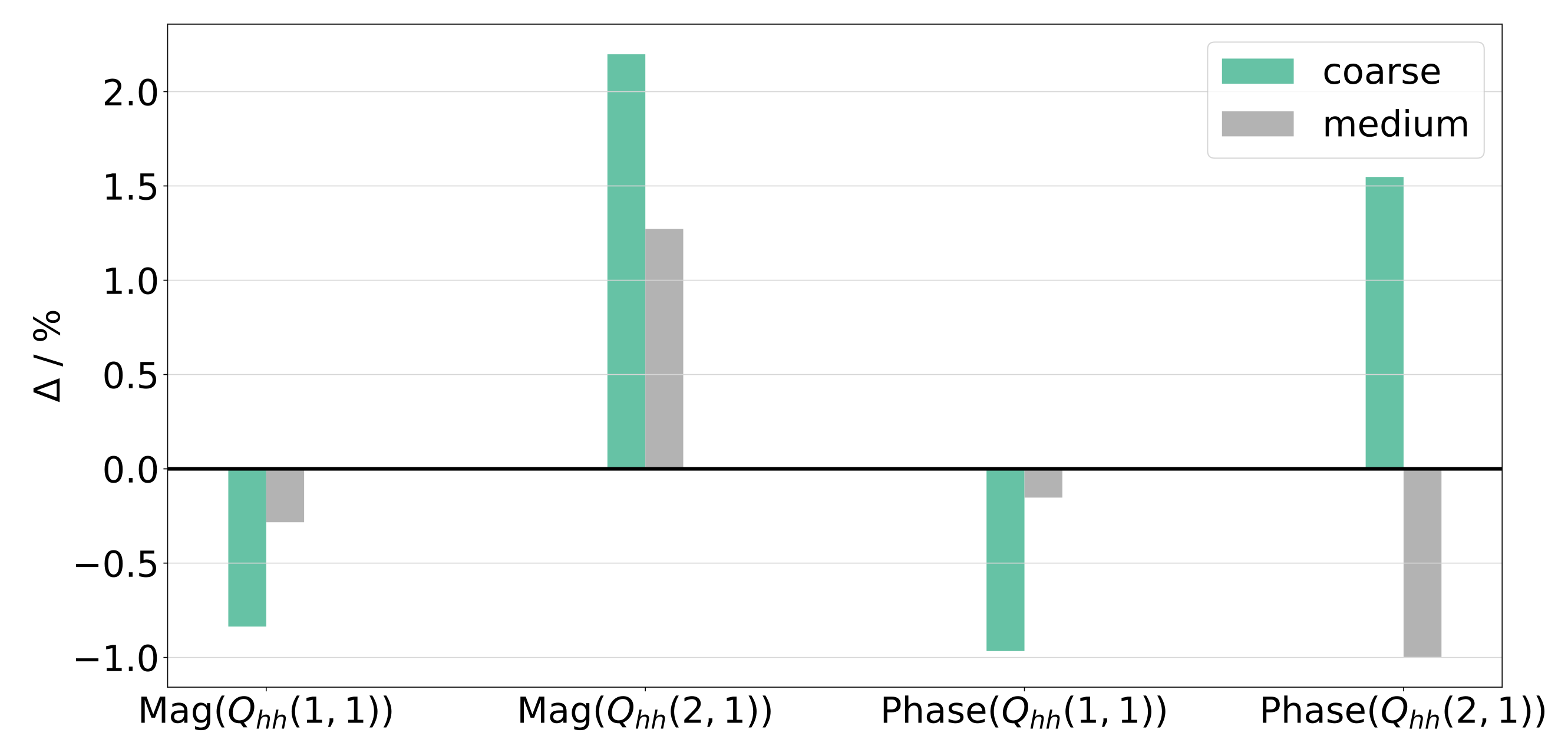
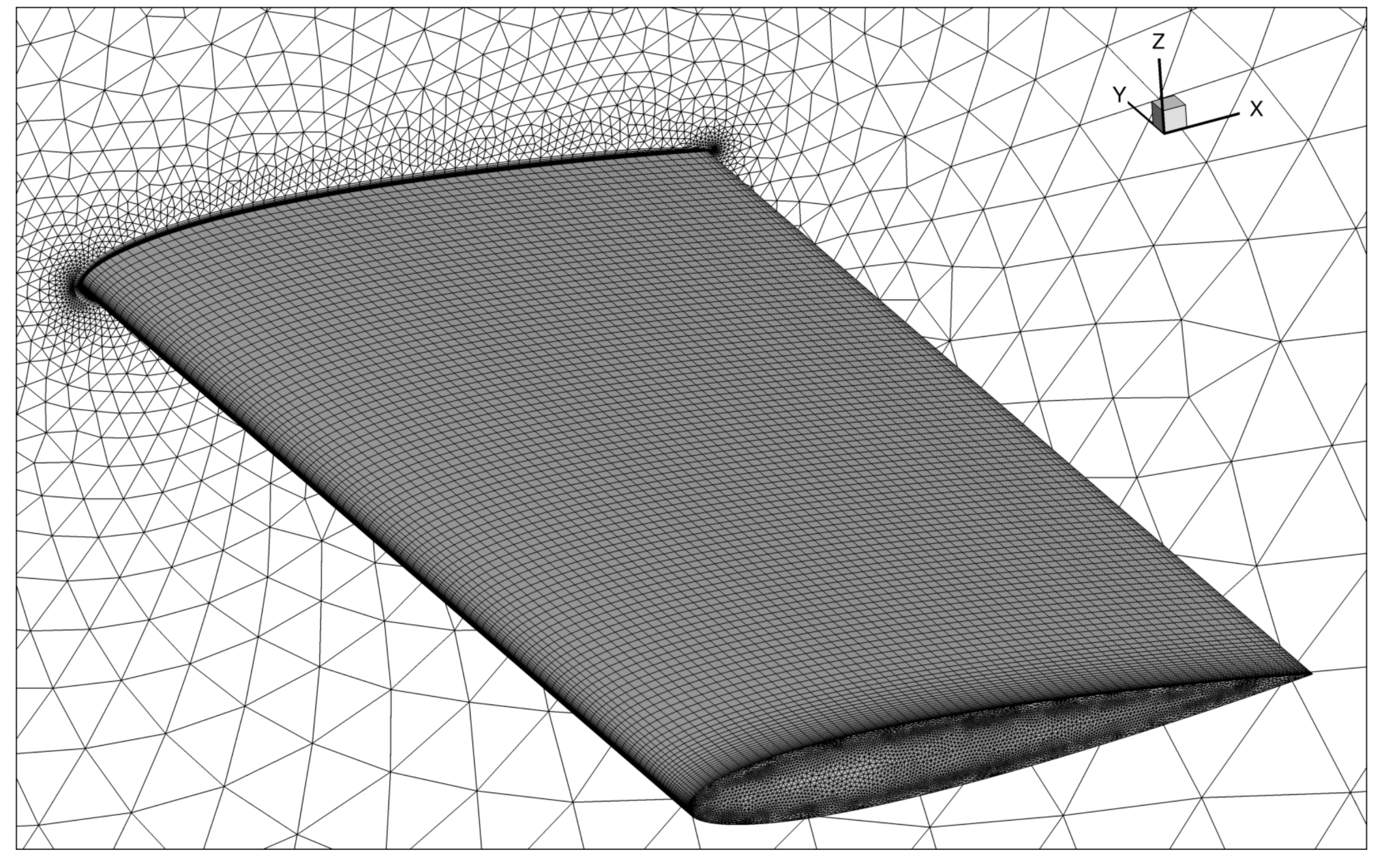






| Parameter | Symbol | Value |
|---|---|---|
| Mach numbers/- | 0.4|0.8 | |
| Reduced frequencies/- | k | 0.056, 0.231 |
| Frequencies/ | f | 1.213, 5.004 |2.426, 10.008 |
| Rotational amplitudes/ | 0.01, 0.917, 1.834, 3.669, 5.000 | |
| Temperature/ | T | 288.15 |
| Density/ | 1.225 | |
| Dynamic viscosity/ | 17.89 × 10−6 | |
| Ratio of specific heats/- | 1.4 | |
| Ideal gas constant/ | R | 287 |
| Reduced frequency reference length/ | 1.0 | |
| Reynolds number reference length/ | L | 2.0 |
| Reynolds numbers/- | 15.216 × 106|30.432 × 106 | |
| Relative Cauchy error for ⋯ | ||
| ⋯lift coefficient/- | 1 × 10−6 | |
| ⋯drag coefficient/- | 1 × 10−6 | |
| ⋯lateral force/- | 1 × 10−4 | |
| ⋯longitudinal moment coefficient/- | 1 × 10−3 | |
| ⋯lateral moment coefficient/- | 5 × 10−6 |
| Parameter | Coarse | Medium | Fine |
|---|---|---|---|
| Number of grid points | 0.432 million | 0.865 million | 1.412 million |
| Number of surface triangles | 85.732 k | 178.066 k | 412.094 k |
| Number of volume tetrahedrons | 2.407 million | 4.816 million | 7.689 million |
| Parameter | Value |
|---|---|
| First layer thickness | |
| Number of layers | 38 |
| Stretching ratio | 1.25 |
| Total prism layer height |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schäfer, D. Influence of Fluid Viscosity and Compressibility on Nonlinearities in Generalized Aerodynamic Forces for T-Tail Flutter. Aerospace 2022, 9, 256. https://doi.org/10.3390/aerospace9050256
Schäfer D. Influence of Fluid Viscosity and Compressibility on Nonlinearities in Generalized Aerodynamic Forces for T-Tail Flutter. Aerospace. 2022; 9(5):256. https://doi.org/10.3390/aerospace9050256
Chicago/Turabian StyleSchäfer, Dominik. 2022. "Influence of Fluid Viscosity and Compressibility on Nonlinearities in Generalized Aerodynamic Forces for T-Tail Flutter" Aerospace 9, no. 5: 256. https://doi.org/10.3390/aerospace9050256
APA StyleSchäfer, D. (2022). Influence of Fluid Viscosity and Compressibility on Nonlinearities in Generalized Aerodynamic Forces for T-Tail Flutter. Aerospace, 9(5), 256. https://doi.org/10.3390/aerospace9050256






