Hybrid Guidance Optimization for Multipulse Glideslope Approach with Bearing-Only Navigation
Abstract
:1. Introduction
2. Navigation Accuracy Criteria Based on the FIM
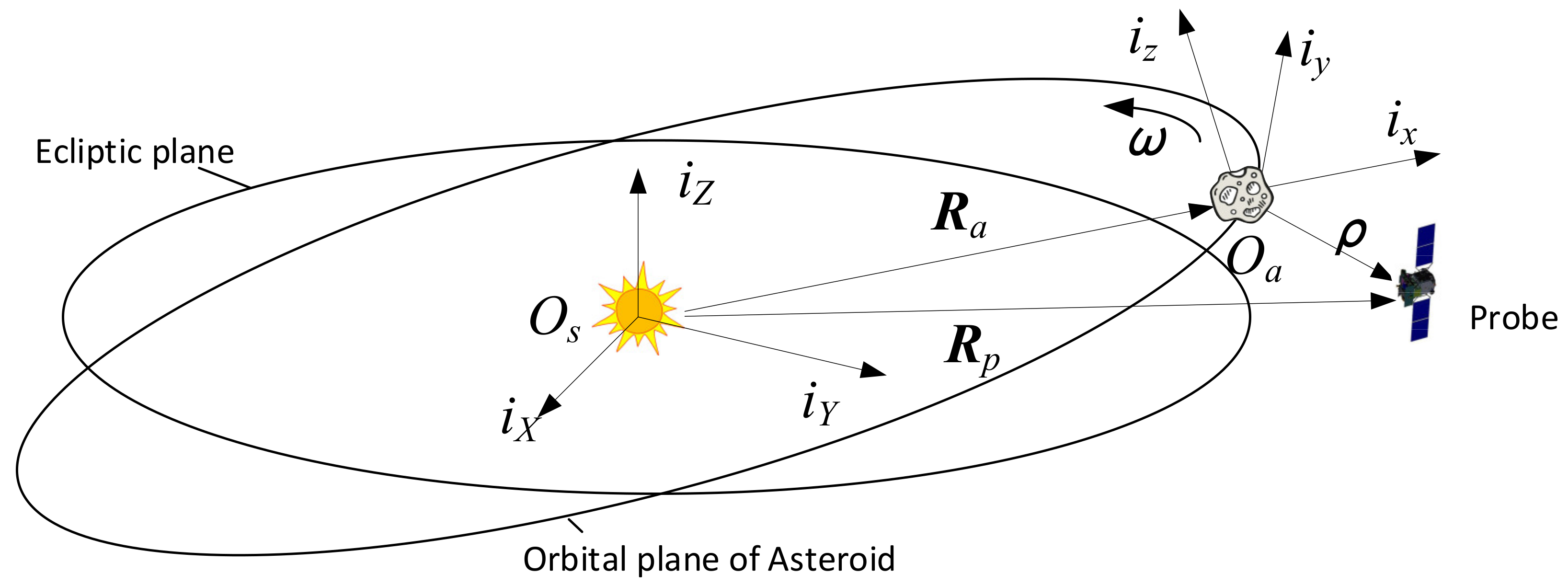
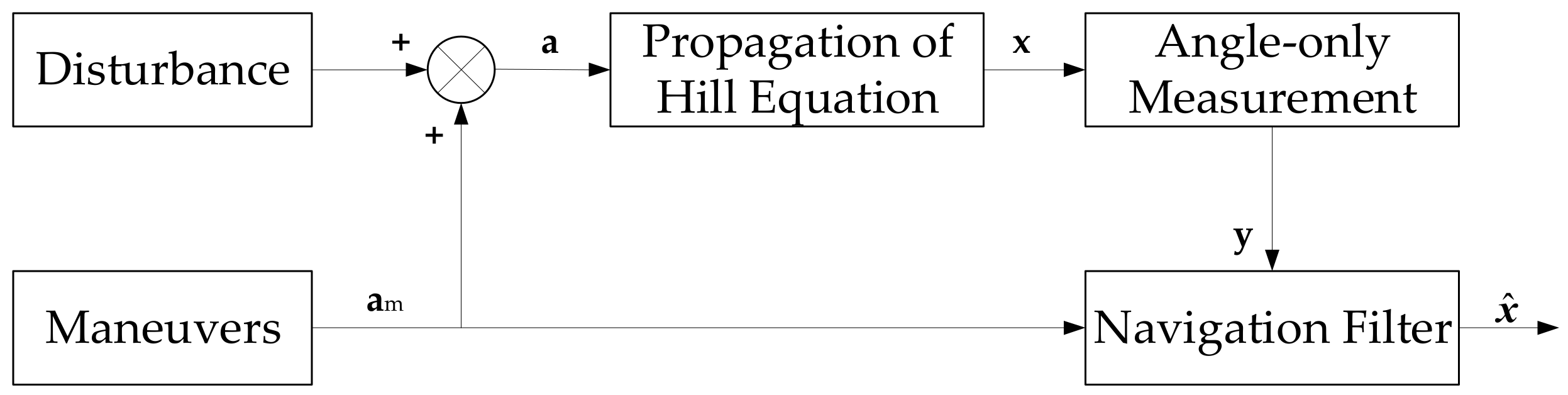
2.1. AON System with an Orbit Maneuver
2.2. Pseudorange Measurement and Its Error Noise
2.3. Navigation Accuracy Estimation Based on the FIM and the CRLB
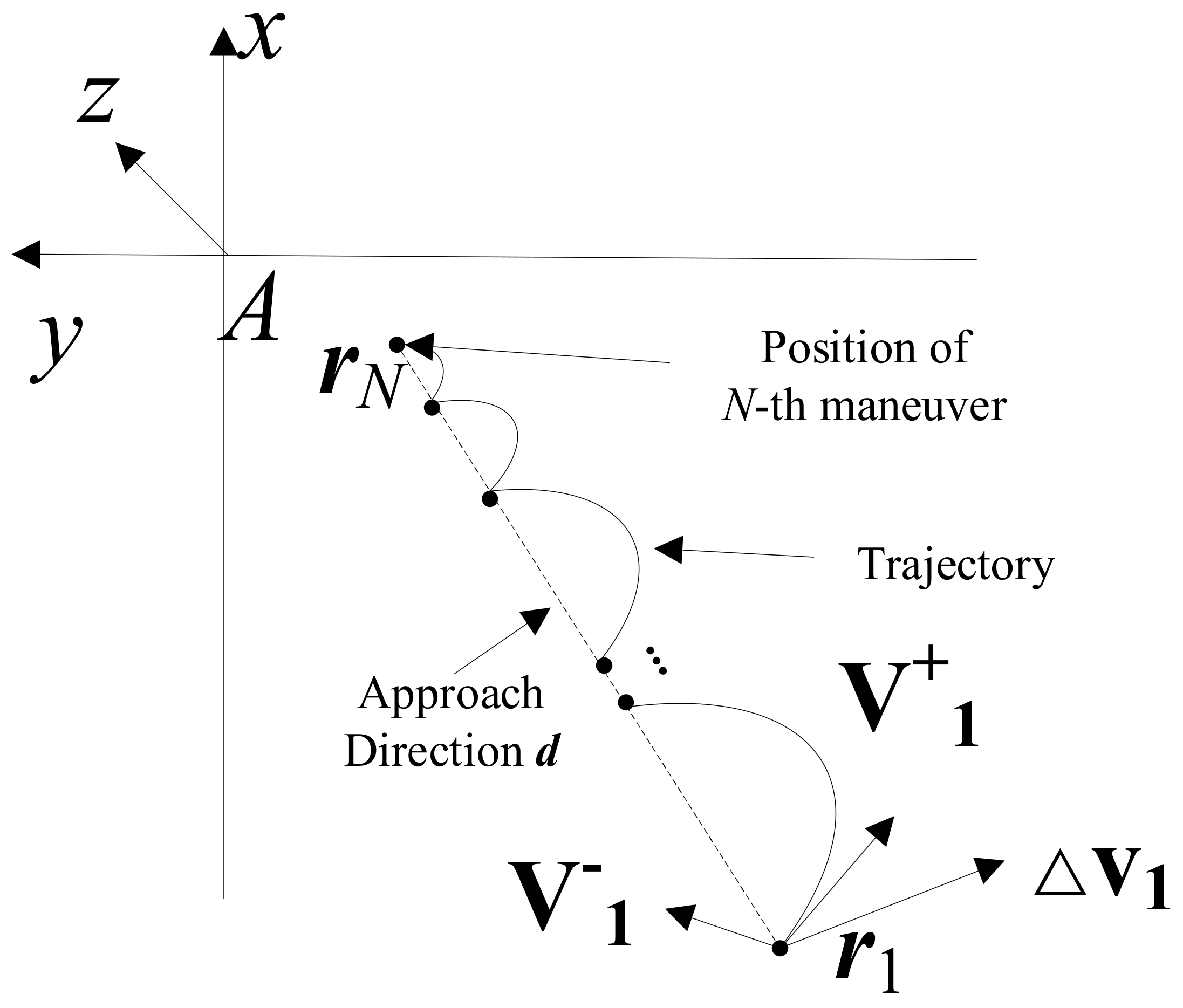
3. Multipulse Glideslope Guidance
4. Navigation Accuracy-Enhanced Multipulse Glideslope Guidance Method
4.1. Problem Description
4.2. Fuel Consumption Objective
4.3. Navigation Error Objective
4.4. Optimization Objectives
4.5. Constraints
4.6. Optimization Problem
5. Numerical Simulation
5.1. Simulation Conditions
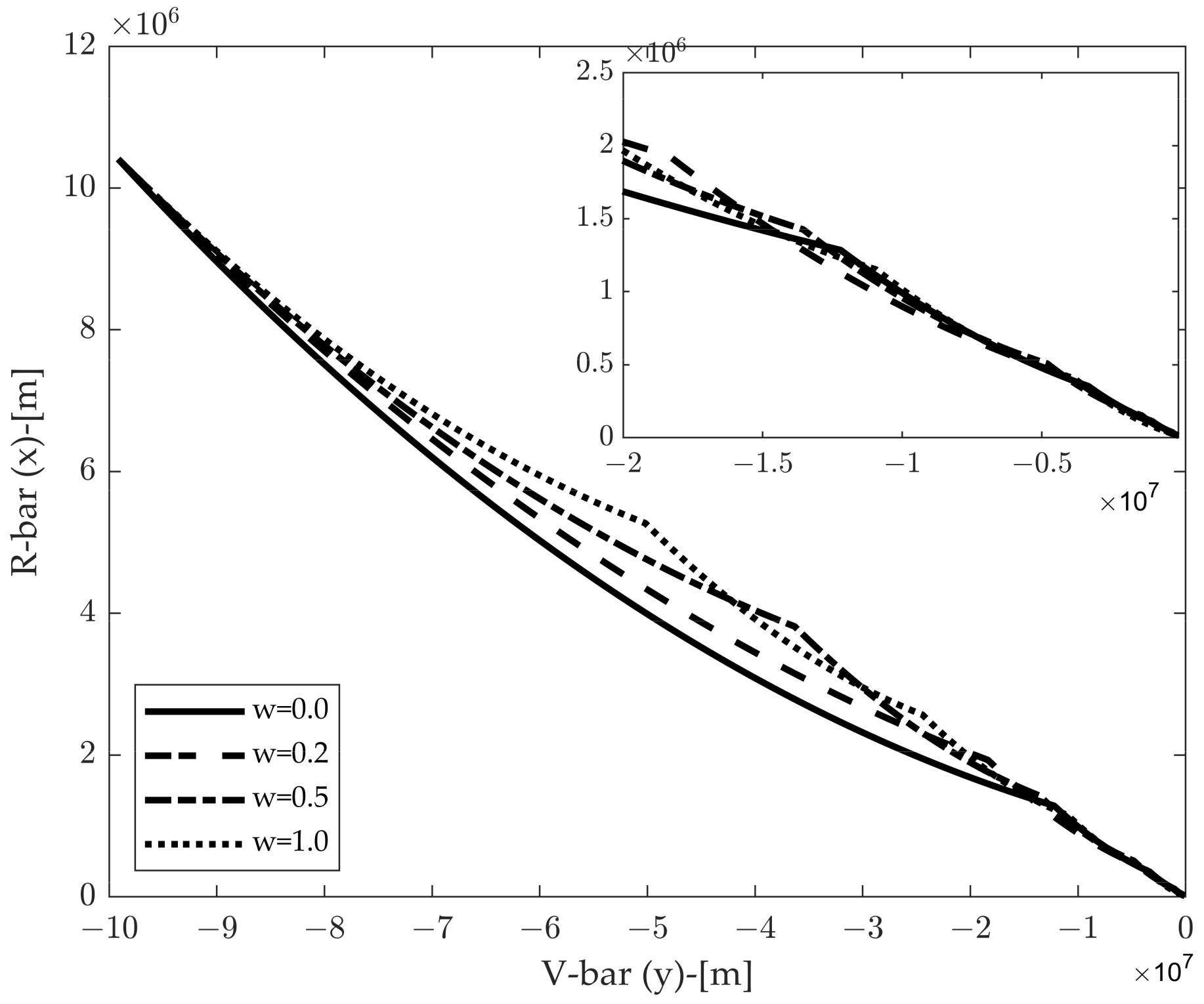
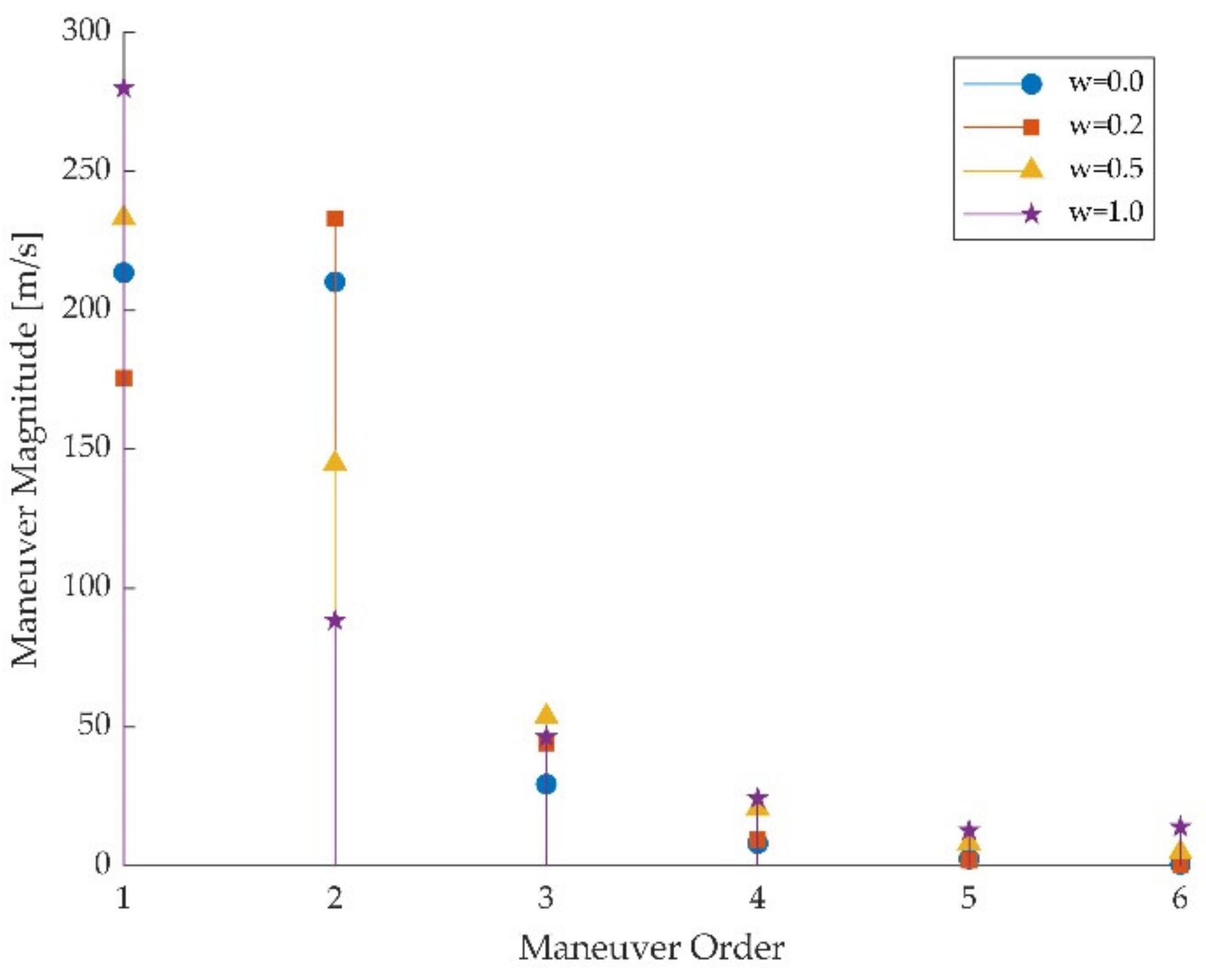
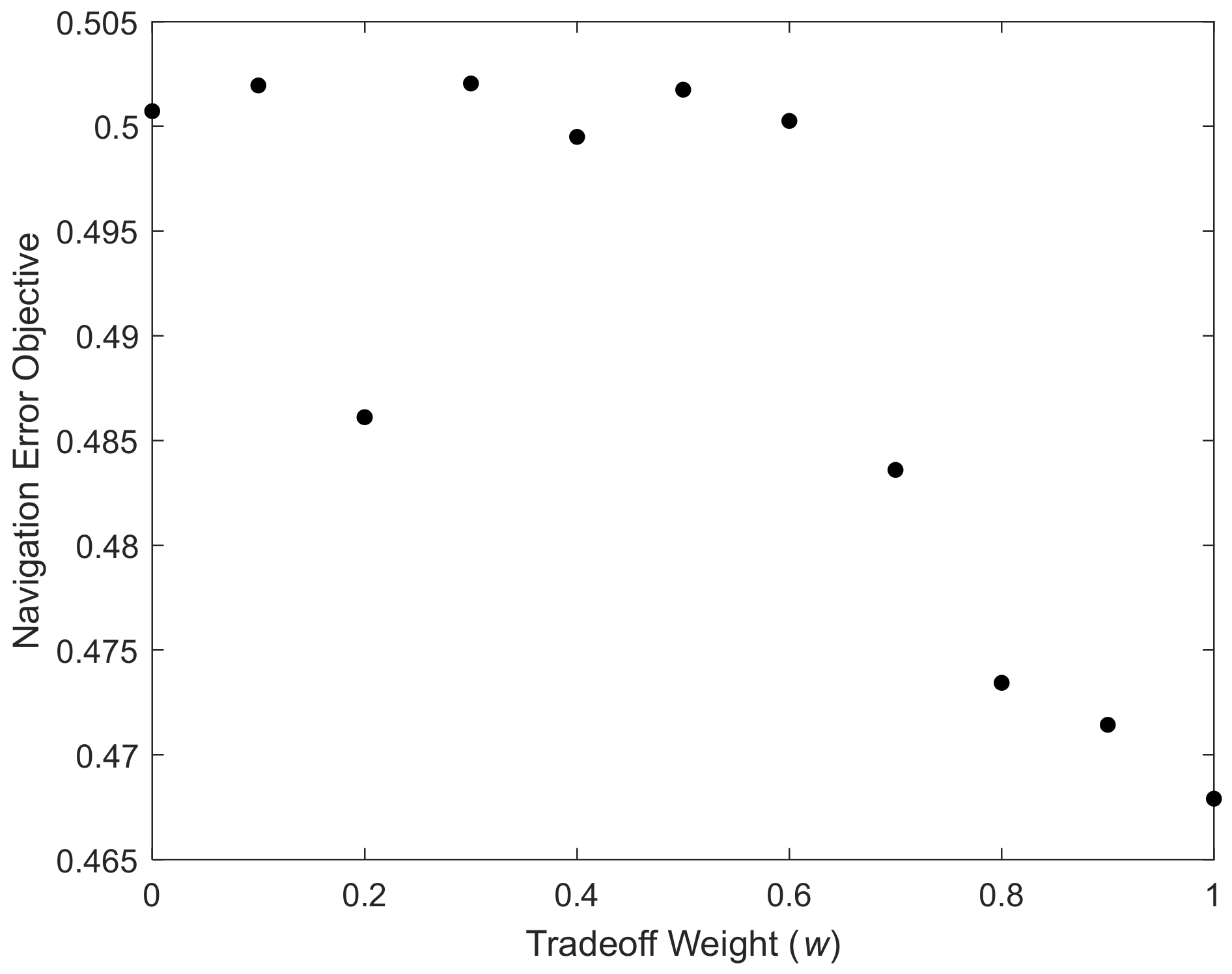
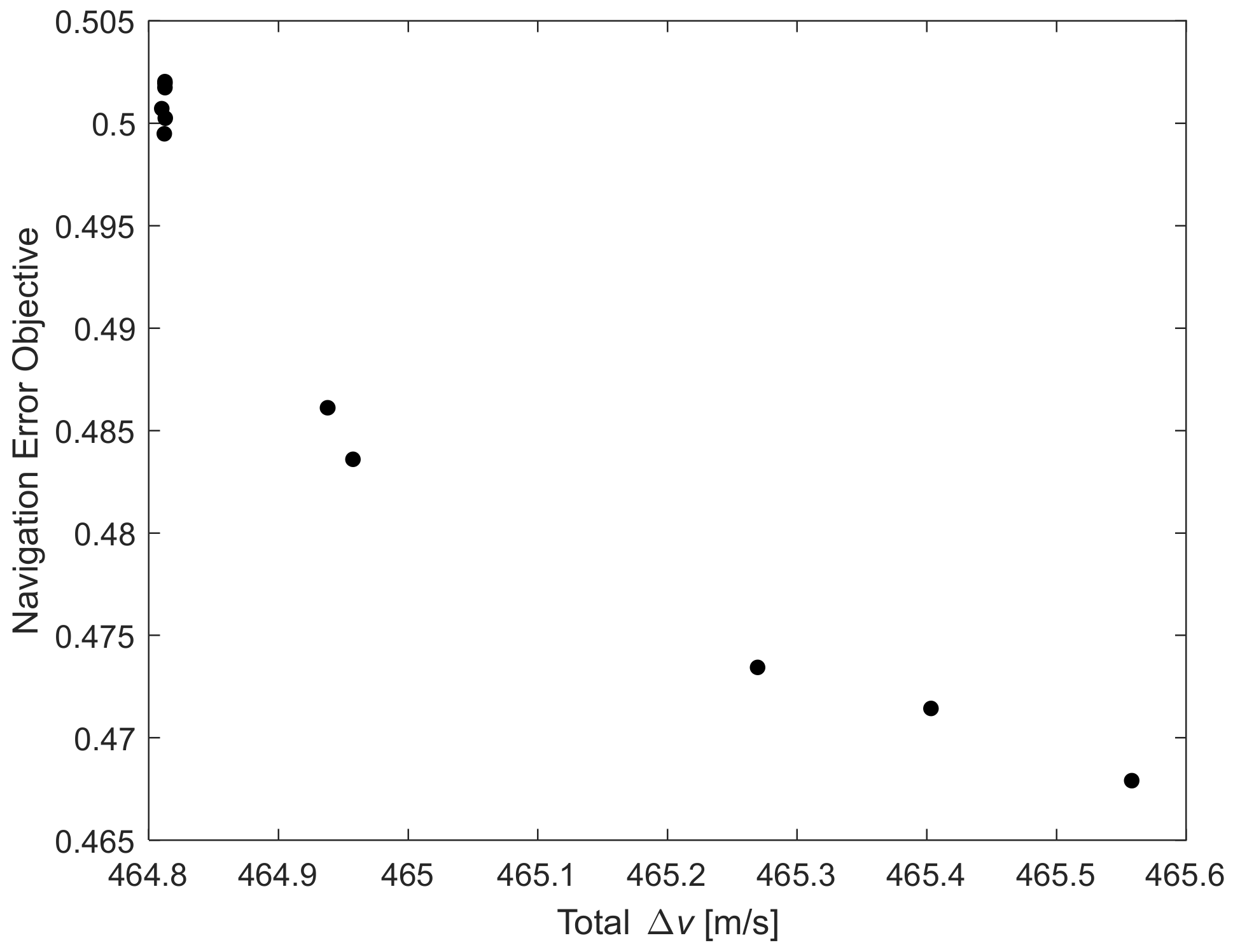
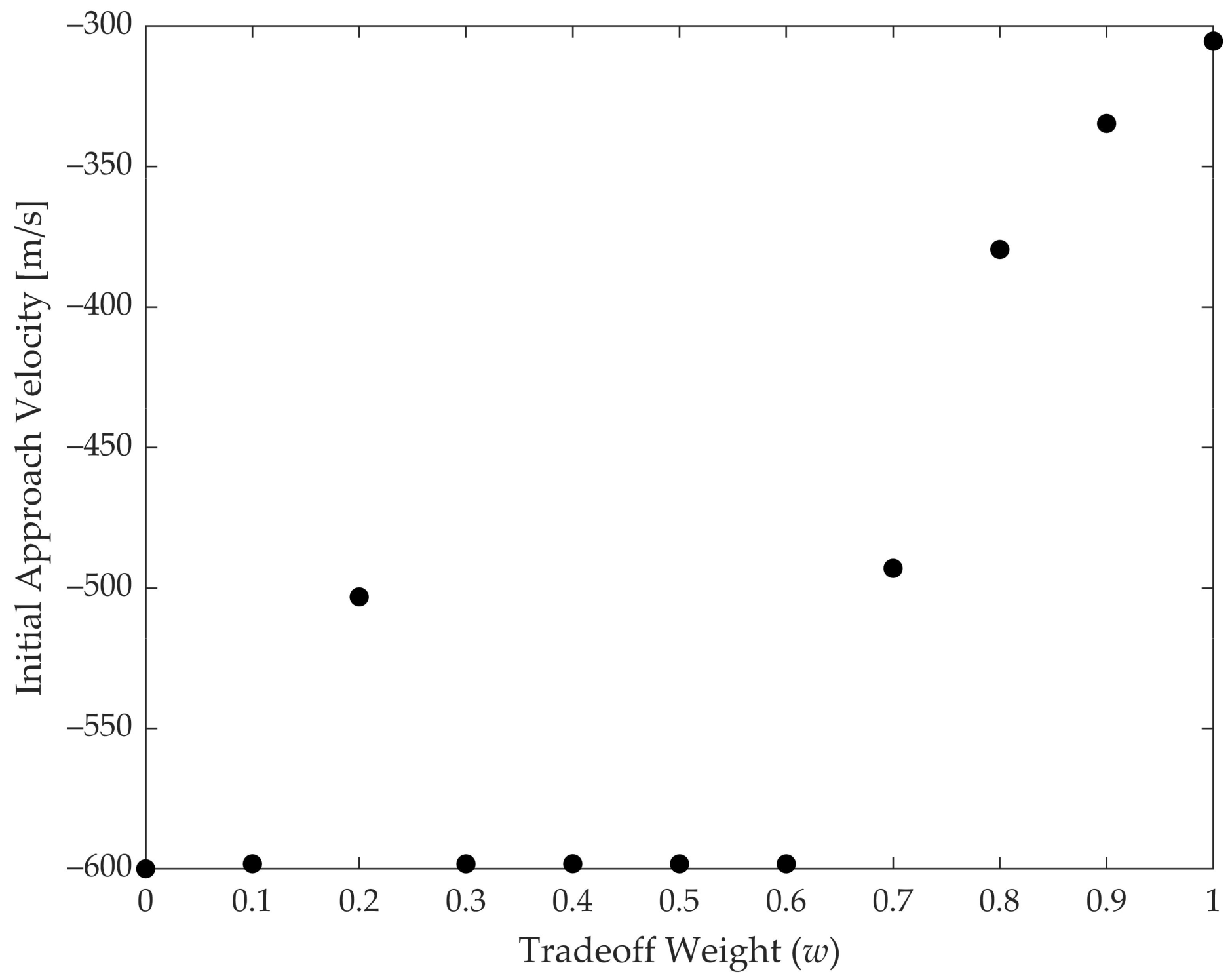
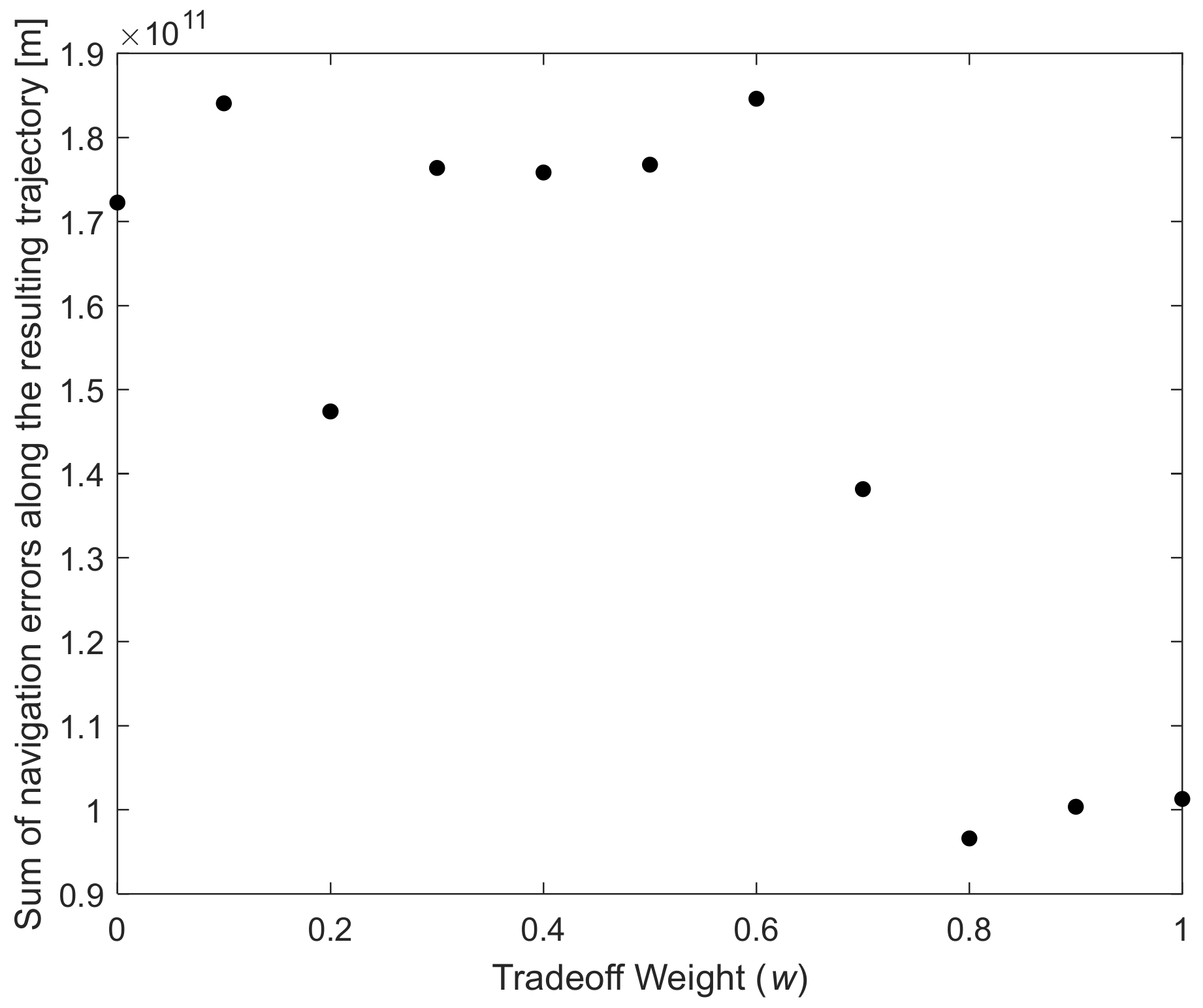
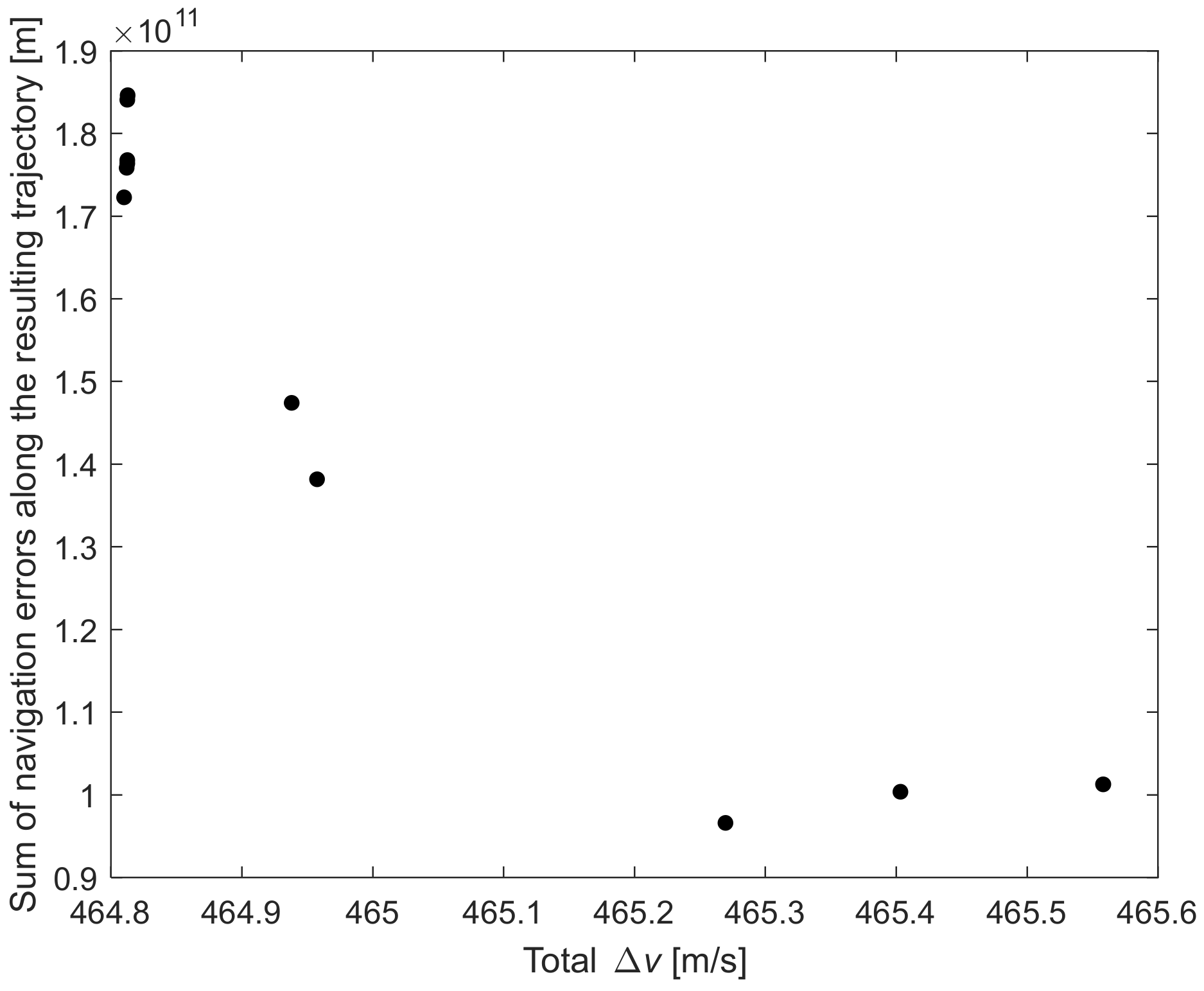
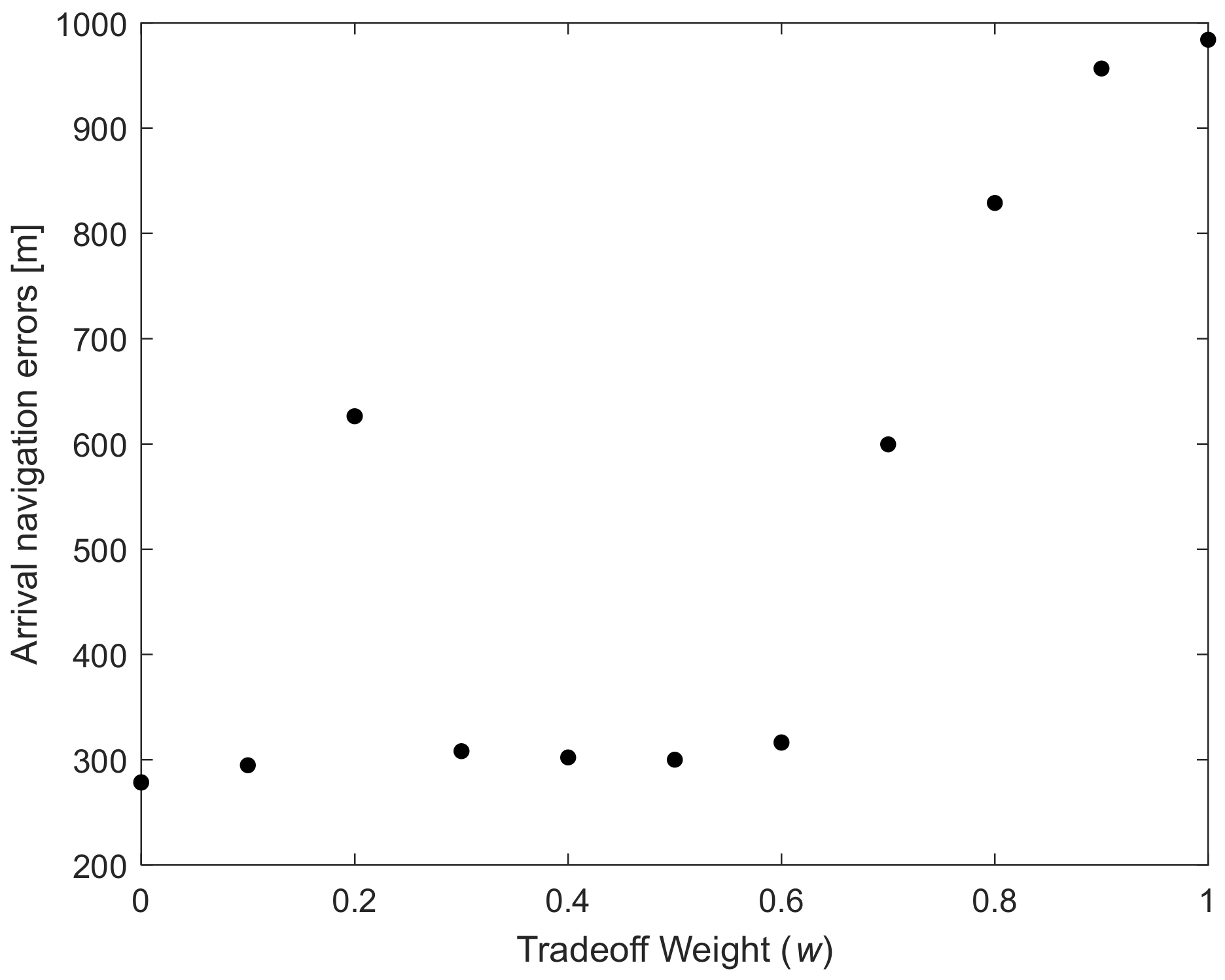
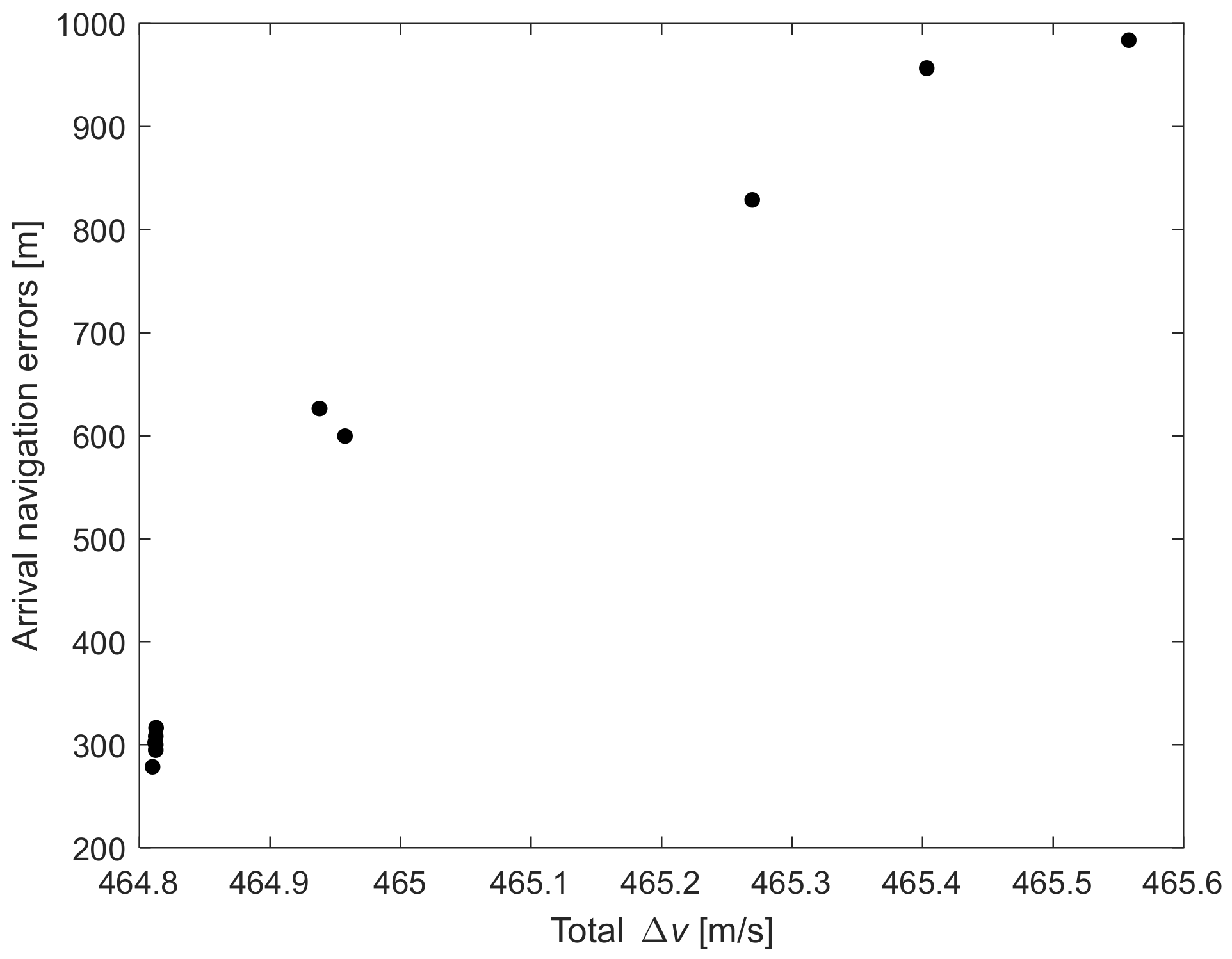
5.2. Navigation Accuracy-Enhanced Multipulse Glideslope Guidance Optimum Results
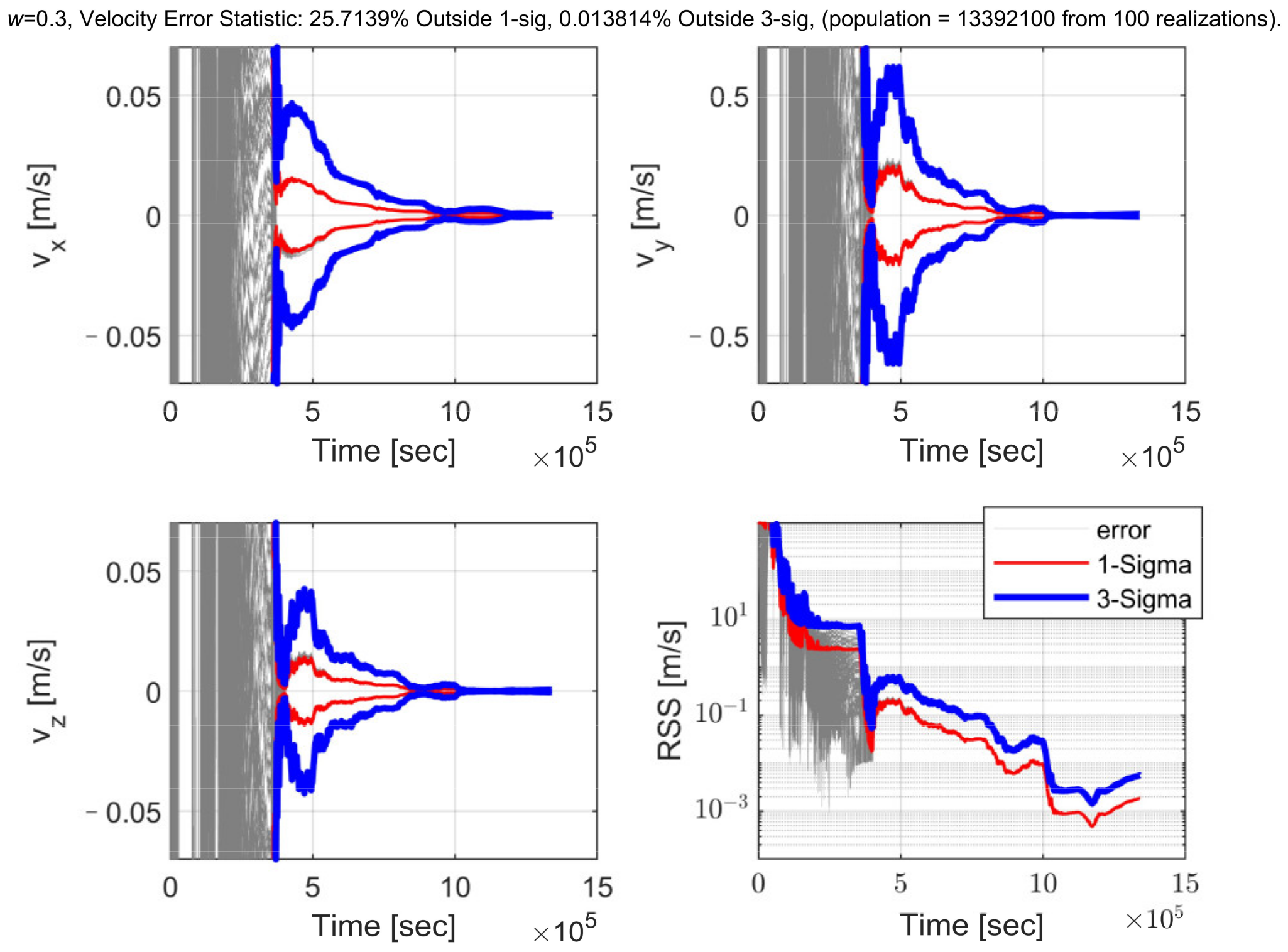
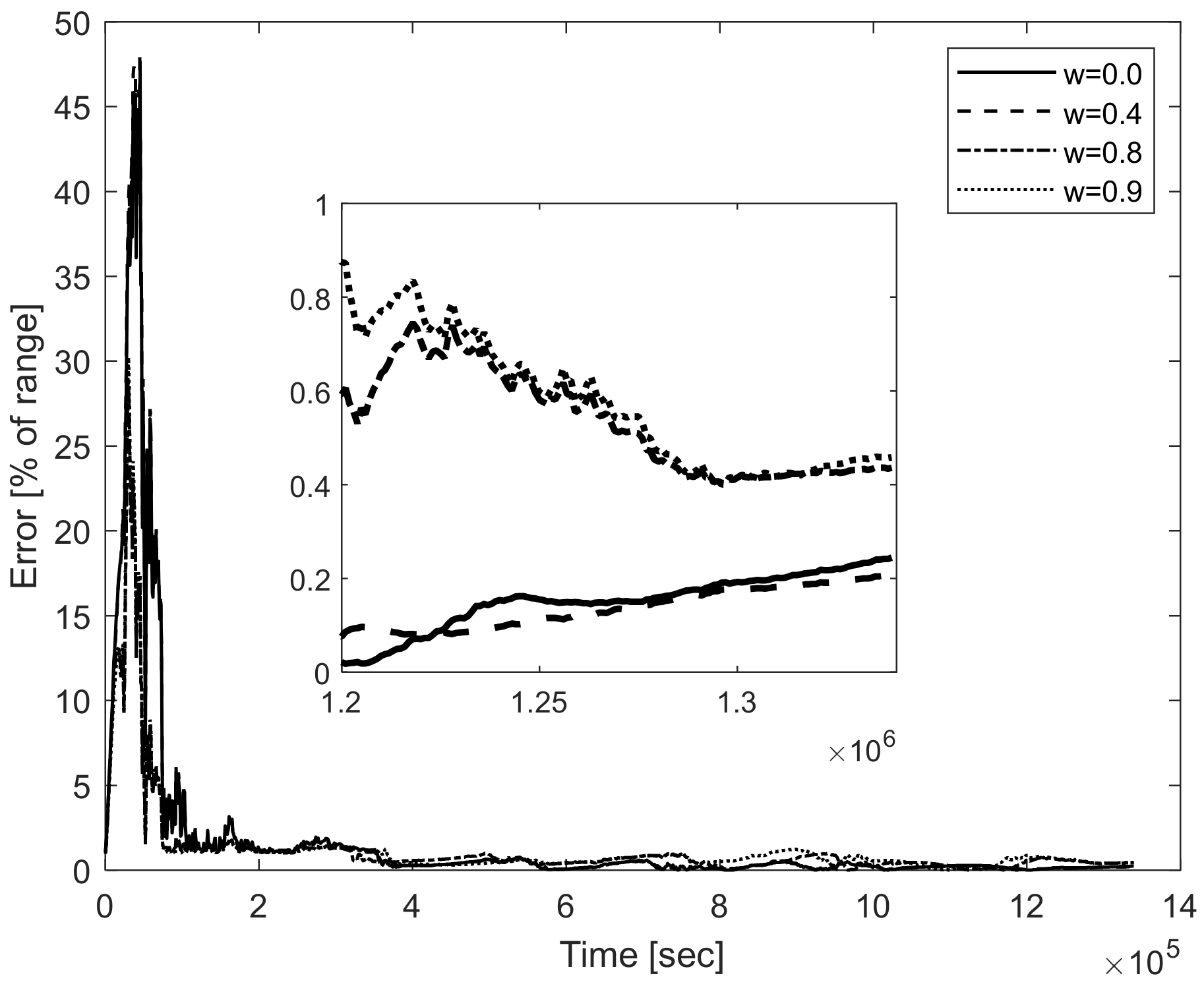
5.3. AON Using Resulting Trajectories
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Asteroid Hill Rotation Reference Frame
- The axis is inward along the orbit radius;
- The axis follows the angular momentum direction of the asteroid’s orbit;
- The axis completes the right-handed coordinate system and is perpendicular to the axis in the orbital plane.
Appendix B. Tschauner–Hempel Equation of a Relative Motion
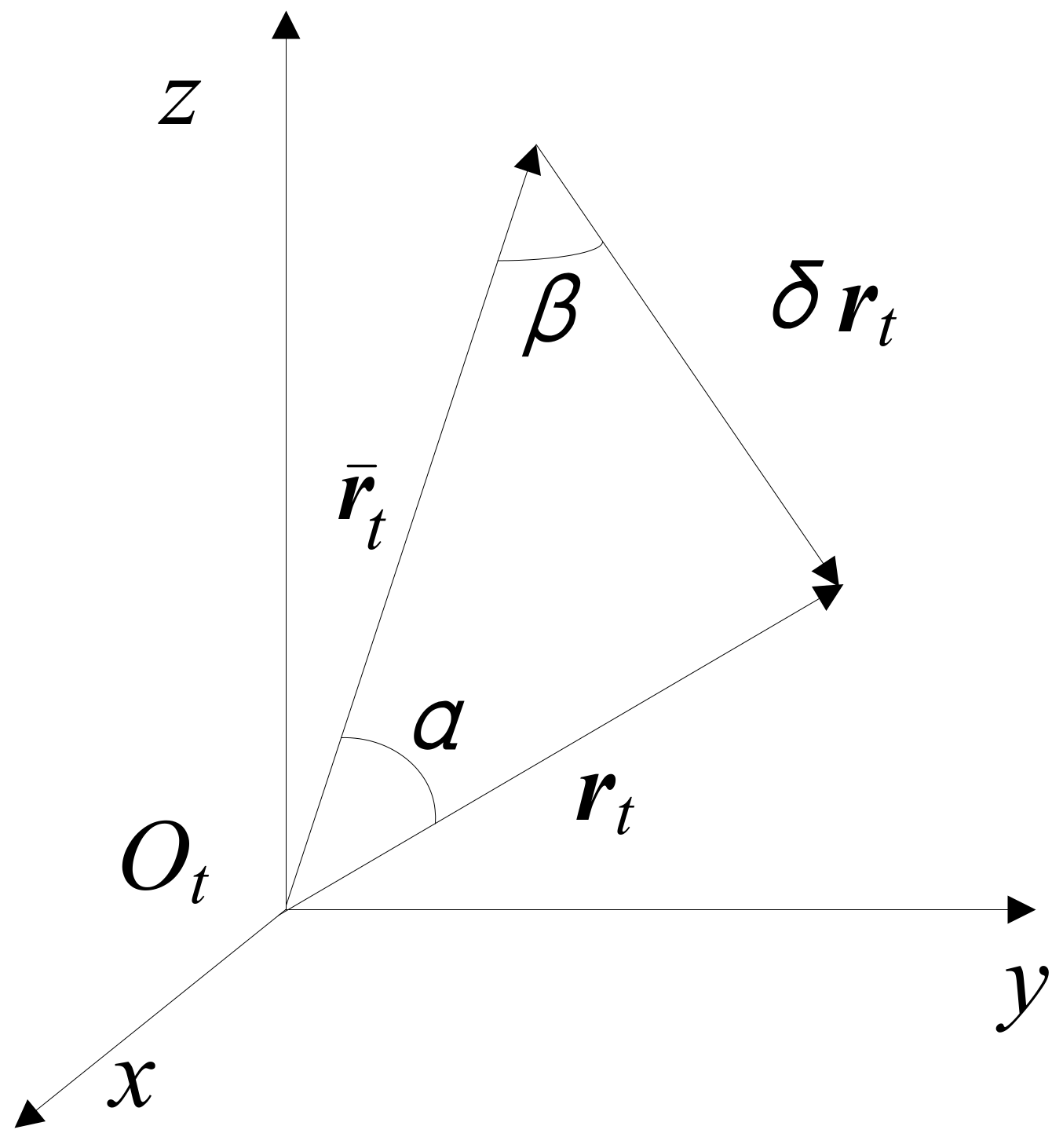
Appendix C. Angle Measurement and Pseudodistance Measurement

References
- Rivkin, A.S.; Chabot, N.L.; Stickle, A.M.; Thomas, C.A.; Richardson, D.C.; Barnouin, O.; Fahnestock, E.G.; Ernst, C.M.; Cheng, A.F.; Chesley, S.; et al. The Double Asteroid Redirection Test (DART): Planetary Defense Investigations and Requirements. Planet. Sci. J. 2021, 2, 173. [Google Scholar] [CrossRef]
- Vaughan, R.; Riedel, J.; Davis, R.; Owen, J.W.; Synnott, S. Optical navigation for the Galileo Gaspra encounter. In Proceedings of the Astrodynamics Conference, Hilton Head Island, SC, USA, 10–12 August 1992; p. 4522. [Google Scholar]
- Miller, J.K.; Williams, B.G.; Bollman, W.E.; Davis, R.P.; Yeomans, D.K. Navigation analysis for Eros rendezvous and orbital phases. J. Astronaut. Sci. 1995, 43, 453–476. [Google Scholar]
- Broschart, S.; Bhaskaran, S.; Bellerose, J.; Dietrich, A.; Han, D.; Haw, R.; Mastrodemos, N.; Owen, W.M.; Rush, B.; Surovik, D. Shadow navigation support at jpl for the rosetta landing on comet 67p/churyumov-gerasimenko. In Proceedings of the 26th International Symposium on Space Flight Dynamics ISSFD, number ISSFD-2017-096, Matsuyama, Japan, 3–9 June 2017. [Google Scholar]
- Kominato, T.; Matsuoka, M.; Uo, M.; Hashimoto, T.; Kawaguchi, J.I. Optical hybrid navigation and station keeping around Itokawa. In Proceedings of the AIAA/AAS Astrodynamics Specialist Conference and Exhibit, Keystone, Colorado, 21–24 August 2006; p. 6535. [Google Scholar]
- Tsuda, Y.; Takeuchi, H.; Ogawa, N.; Ono, G.; Kikuchi, S.; Oki, Y.; Ishiguro, M.; Kuroda, D.; Urakawa, S.; Okumura, S.-I. Rendezvous to asteroid with highly uncertain ephemeris: Hayabusa2’s Ryugu-approach operation result. Astrodynamics 2020, 4, 137–147. [Google Scholar] [CrossRef]
- Lamey, Q.; Vance, L.D.; Thangavelautham, J. The Impact of the Yarkosvky Effect on Satellite Navigation around Small Bodies. ASCEND 2021 2021, 2021, 4173. [Google Scholar]
- Yu, Z.; Shang, H.; Wei, B. Accessibility assessment and trajectory design for multiple Near-Earth-asteroids exploration using stand-alone CubeSats. Aerosp. Sci. Technol. 2021, 118, 106944. [Google Scholar] [CrossRef]
- Gil-Fernandez, J.; Prieto-Llanos, T.; Cadenas-Gorgojo, R.; Graziano, M.; Drai, R. Autonomous GNC Algorithms for Rendezvous Missions to Near-Earth-Objects. In Proceedings of the AIAA/AAS Astrodynamics Specialist Conference & Exhibit, Honolulu, HI, USA, 18–21 August 2008. [Google Scholar]
- Bhaskaran, S. Autonomous navigation for deep space missions. SpaceOps 2012 2012, 2012, 1267135. [Google Scholar]
- Vetrisano, M.; Yarnoz, D.G.; Branco, J. Effective approach navigation prior to small body deflection. In Proceedings of the Space Generation Congress, Beijing, China, 23–27 September 2013. [Google Scholar]
- Richards, A.; Feron, E.; How, J.P.; Schouwenaars, T. Spacecraft Trajectory Planning with Avoidance Constraints Using Mixed-Integer Linear Programming. J. Guid. Control. Dyn. 2002, 25, 755–764. [Google Scholar] [CrossRef] [Green Version]
- Boyd, S.; Vandenberghe, L. Convex Optimization; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Weiss, A.; Baldwin, M.; Erwin, R.S.; Kolmanovsky, I. Model Predictive Control for Spacecraft Rendezvous and Docking: Strategies for Handling Constraints and Case Studies. IEEE Trans. Control. Syst. Technol. 2015, 23, 1638–1647. [Google Scholar] [CrossRef]
- Hartley, E. A tutorial on model predictive control for spacecraft rendezvous. In Proceedings of the Control Conference, Osaka, Japan, 15–18 December 2015. [Google Scholar]
- Hablani, H.B.; Tapper, M.L.; Dana-Bashian, D.J. Guidance and Relative Navigation for Autonomous Rendezvous in a Circular Orbit. J. Guid. Control. Dyn. 2002, 25, 553–562. [Google Scholar] [CrossRef]
- Benedikter, B.; Zavoli, A. Convex Optimization of Linear Impulsive Rendezvous. arXiv 2019, arXiv:1912.08038. [Google Scholar]
- Grzymisch, J.; Fichter, W. Optimal Rendezvous Guidance with Enhanced Bearings-Only Observability. J. Guid. Control. Dyn. 2015, 38, 1131–1140. [Google Scholar] [CrossRef]
- Woffinden, D.C.; Geller, D.K. Observability Criteria for Angles-Only Navigation. IEEE Trans. Aerosp. Electron. Syst. 2009, 45, 1194–1208. [Google Scholar] [CrossRef]
- Woffinden, D.C.; Geller, D.K. Optimal Orbital Rendezvous Maneuvering for Angles-Only Navigation. J. Guid. Control Dyn. 2009, 32, 1382–1387. [Google Scholar] [CrossRef]
- Grzymisch, J.; Fichter, W. Observability Criteria and Unobservable Maneuvers for In-Orbit Bearings-Only Navigation. J. Guid. Control Dyn. 2014, 37, 1250–1259. [Google Scholar] [CrossRef]
- Grzymisch, J.; Fichter, W. Analytic Optimal Observability Maneuvers for In-Orbit Bearings-Only Rendezvous. J. Guid. Control Dyn. 2014, 37, 1658–1664. [Google Scholar] [CrossRef]
- Mok, S.-H.; Pi, J.; Bang, H. One-step rendezvous guidance for improving observability in bearings-only navigation. Adv. Space Res. 2020, 66, 2689–2702. [Google Scholar] [CrossRef]
- Hou, B.; Wang, D.; Wang, J.; Ge, D.; Zhou, H.; Zhou, X. Optimal Maneuvering for Autonomous Relative Navigation Using Monocular Camera Sequential Images. J. Guid. Control Dyn. 2021, 44, 1947–1960. [Google Scholar] [CrossRef]
- D’Amico, S.; Ardaens, J.S.; Gaias, G.; Benninghoff, H.; Schlepp, B.; Jorgensen, J.L. Noncooperative Rendezvous Using Angles-Only Optical Navigation: System Design and Flight Results. J. Guid. Control Dyn. 2013, 36, 1576–1595. [Google Scholar] [CrossRef]
- Pesce, V.; Opromolla, R.; Sarno, S.; Lavagna, M.; Grassi, M. Autonomous relative navigation around uncooperative spacecraft based on a single camera. Aerosp. Sci. Technol. 2019, 84, 1070–1080. [Google Scholar] [CrossRef]
- Clohessy, W.H.; Wiltshire, R.S. Terminal Guidance System for Satellite Rendezvous. J. Aerosp. Sci. 1960, 27, 653–658. [Google Scholar] [CrossRef]
- Okasha, M.; Newman, B. Guidance, Navigation and Control for Satellite Proximity Operations using Tschauner-Hempel Equations. J. Astronaut. Sci. 2013, 60, 109–136. [Google Scholar] [CrossRef]
- Yamanaka, K.; Ankersen, F. New State Transition Matrix for Relative Motion on an Arbitrary Elliptical Orbit. J. Guid. Control Dyn. 2002, 25, 60–66. [Google Scholar] [CrossRef]
- Arthur, G. Applied Optimal Estimation; The MIT Press: Cambreidge, MA, USA, 1974. [Google Scholar]




| Epoch 2459600.5 (2022-Jan-21.0) TDB Reference: Heliocentric J2000 Ecliptic | ||
|---|---|---|
| Element | Value | Units |
| Semimajor axis | 1.001137344063433 | AU |
| Eccentricity | 0.1029843787386461 | |
| Inclination | 7.788928644671124 | deg |
| Longitude of the ascending node | 66.0142959682462 | deg |
| Perihelion argument angle | 305.6646720090911 | deg |
| Mean anomaly | 107.172338605596 | deg |
| Parameter | Value | Unit |
|---|---|---|
| Environment | ||
| Equivalent disturbance 1σ | 1 × 10–8 | m/s2 |
| Equivalent disturbance 1σ | 1 | mrad |
| Sample time | 10 | s |
| Rendezvous Mission | ||
| Initial condition | km km km km/s km/s km/s | |
| Target condition | km km km km/s km/s km/s | |
| Number of pulses N | 6 | |
| 2023-May-7 | ||
| Total time T | 15.5 | day |
| Optimization Parameter | ||
| Minimum maneuver interval time | 5 | hour |
| Navigation Initiation | ||
| Initial navigation error 1σ | km km km km/s km/s km/s |
| Tradeoff Weight w | Maneuver Time Instant (/T) | |||
|---|---|---|---|---|
| 2nd Maneuver | 3rd Maneuver | 4th Maneuver | 5th Maneuver | |
| 0 | 0.260 | 0.424 | 0.575 | 0.739 |
| 0.1 | 0.263 | 0.425 | 0.574 | 0.736 |
| 0.2 | 0.268 | 0.427 | 0.572 | 0.731 |
| 0.3 | 0.263 | 0.425 | 0.574 | 0.736 |
| 0.4 | 0.262 | 0.425 | 0.574 | 0.737 |
| 0.5 | 0.264 | 0.426 | 0.573 | 0.735 |
| 0.6 | 0.264 | 0.425 | 0.574 | 0.735 |
| 0.7 | 0.257 | 0.422 | 0.577 | 0.742 |
| 0.8 | 0.230 | 0.411 | 0.588 | 0.769 |
| 0.9 | 0.263 | 0.425 | 0.574 | 0.736 |
| 1 | 0.257 | 0.422 | 0.577 | 0.742 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, H.; Li, D.; Wang, J. Hybrid Guidance Optimization for Multipulse Glideslope Approach with Bearing-Only Navigation. Aerospace 2022, 9, 242. https://doi.org/10.3390/aerospace9050242
Yuan H, Li D, Wang J. Hybrid Guidance Optimization for Multipulse Glideslope Approach with Bearing-Only Navigation. Aerospace. 2022; 9(5):242. https://doi.org/10.3390/aerospace9050242
Chicago/Turabian StyleYuan, Hao, Dongxu Li, and Jie Wang. 2022. "Hybrid Guidance Optimization for Multipulse Glideslope Approach with Bearing-Only Navigation" Aerospace 9, no. 5: 242. https://doi.org/10.3390/aerospace9050242
APA StyleYuan, H., Li, D., & Wang, J. (2022). Hybrid Guidance Optimization for Multipulse Glideslope Approach with Bearing-Only Navigation. Aerospace, 9(5), 242. https://doi.org/10.3390/aerospace9050242






