An Investigation into the Flow of Rotating Orifices with Euler Angle and the Calculation Model of Discharge Coefficient Considering the Effect of Comprehensive Incidence Angle
Abstract
:1. Introduction
- The length to diameter ratio of the orifice (l/d);
- Radiusing or chamfering of the orifice;
- Euler angles of the orifice (radial inclination angles and circumferential inclination angles ).
- The Reynolds number of the flow inside the orifice (Re);
- The pressure ratio across the orifice ();
- The internal and external cross-flow of the orifice;
- Pre-swirl of the inlet;
- Rotation.
2. Theory of Rotating Orifices
3. Experimental Apparatus
4. Numerical Calculation Method
4.1. Geometric Model
4.2. CFD Model and Boundary Conditions
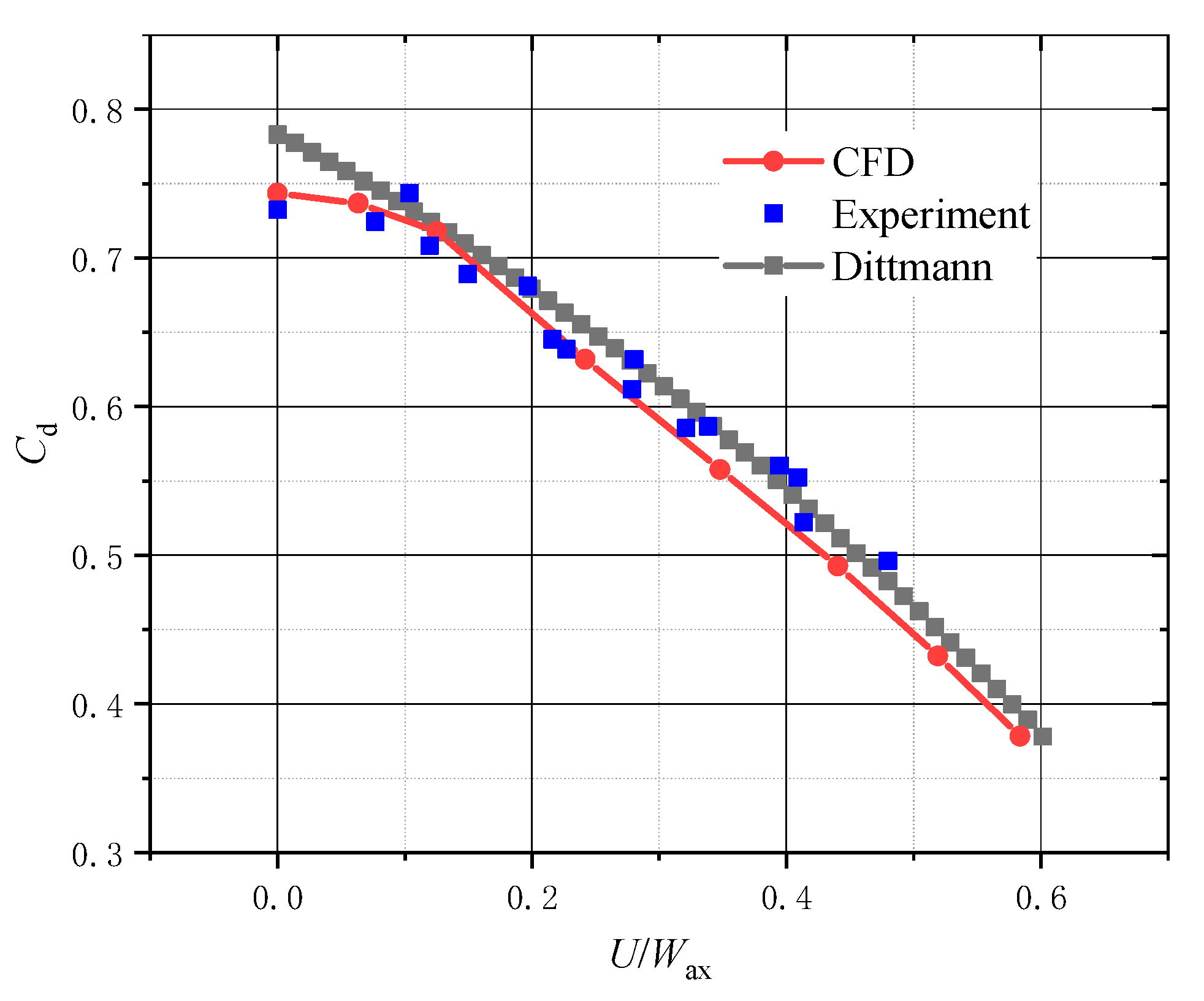
4.3. Verification of CFD Model
4.3.1. Verification of Grid Independence
4.3.2. Verification of Computational Model Reliability
5. Results and Discussion
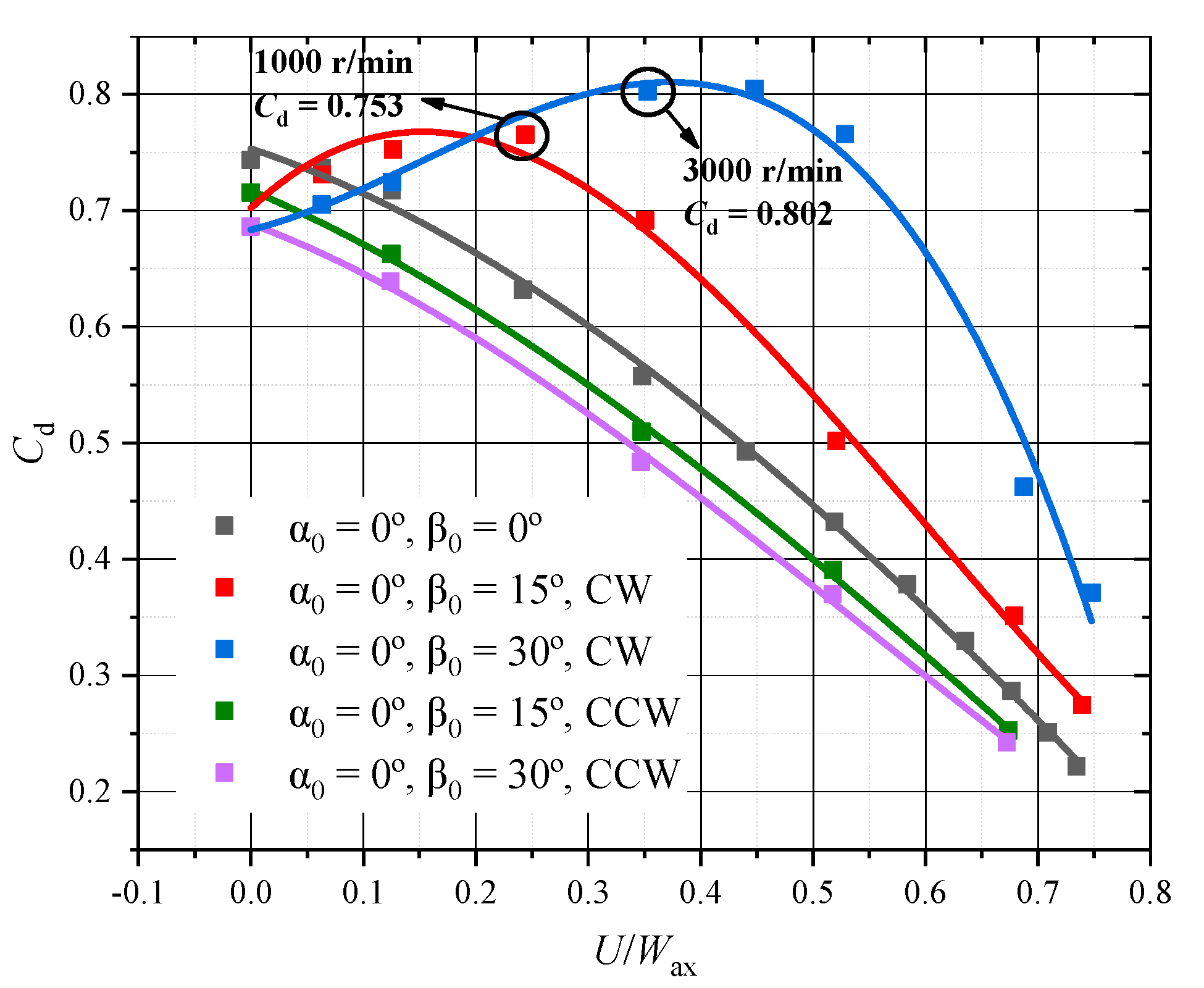
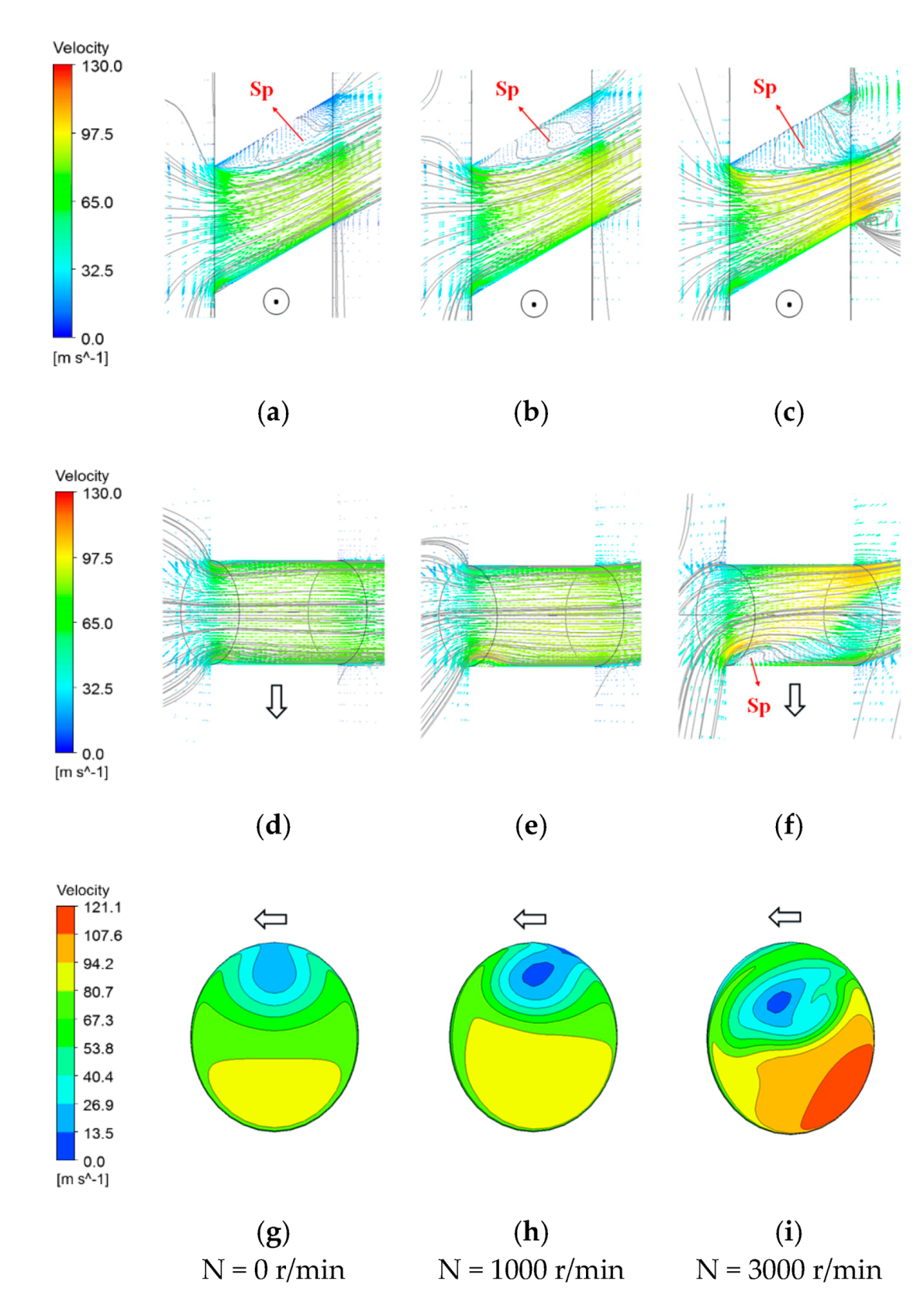
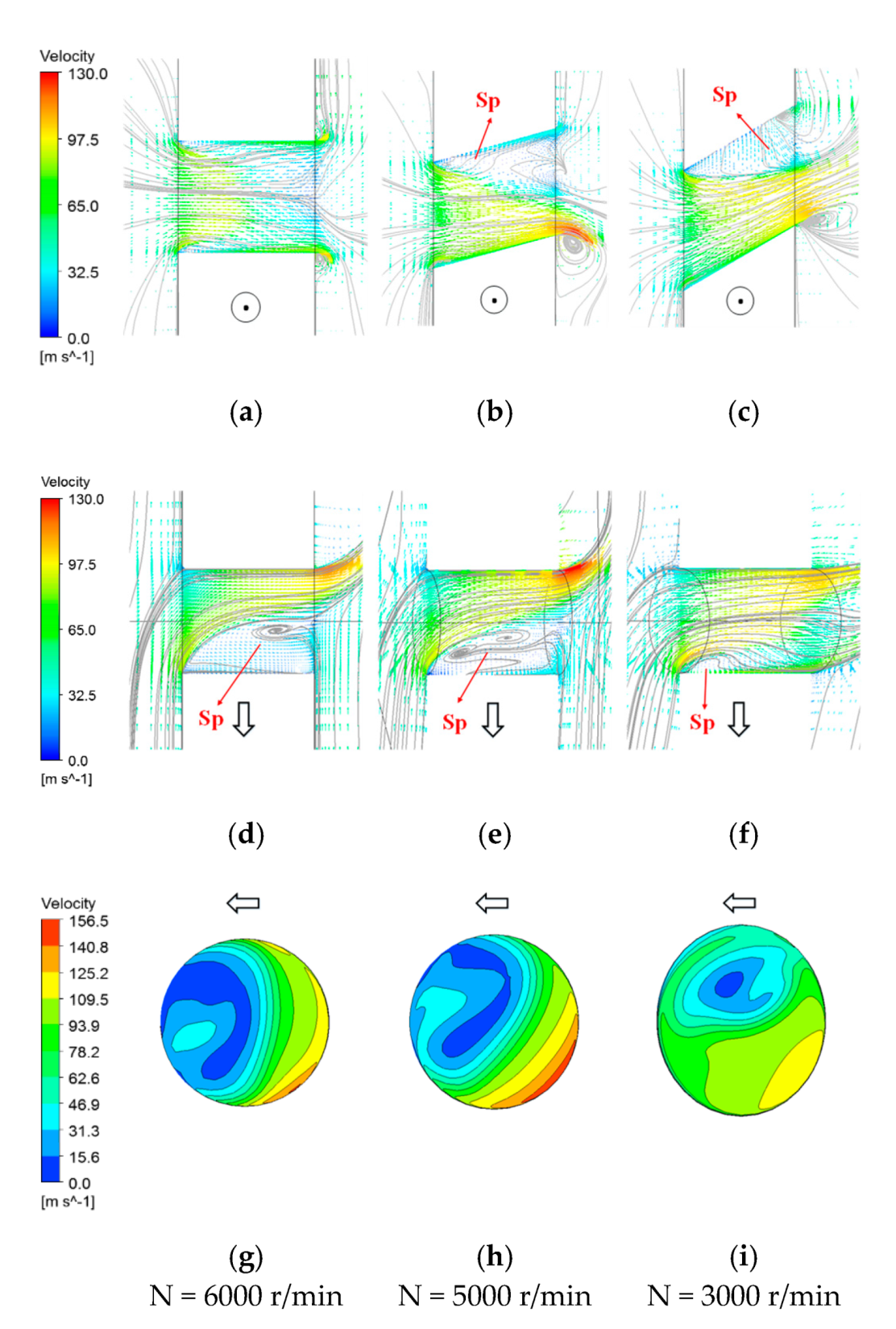
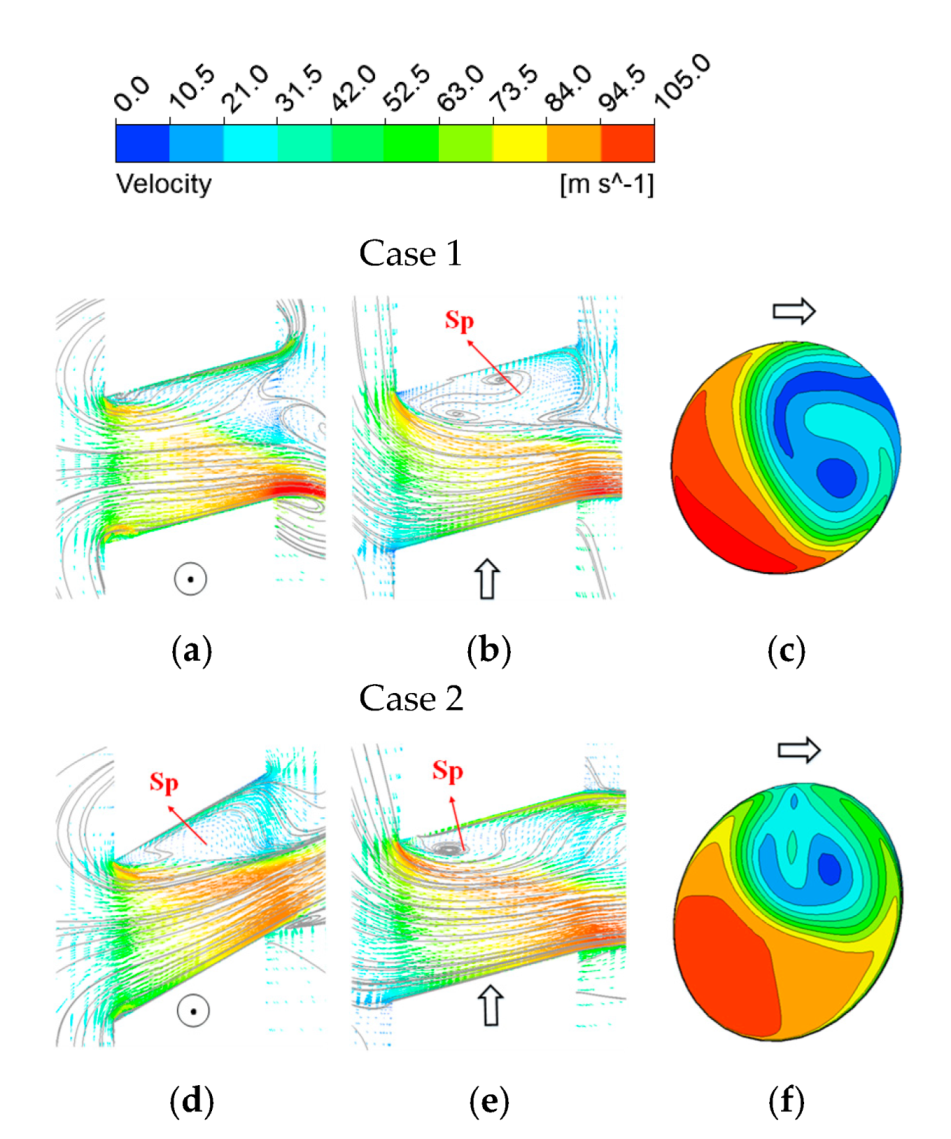
5.1. Effect of Circumferential Inclination Angle on the Discharge Coefficient
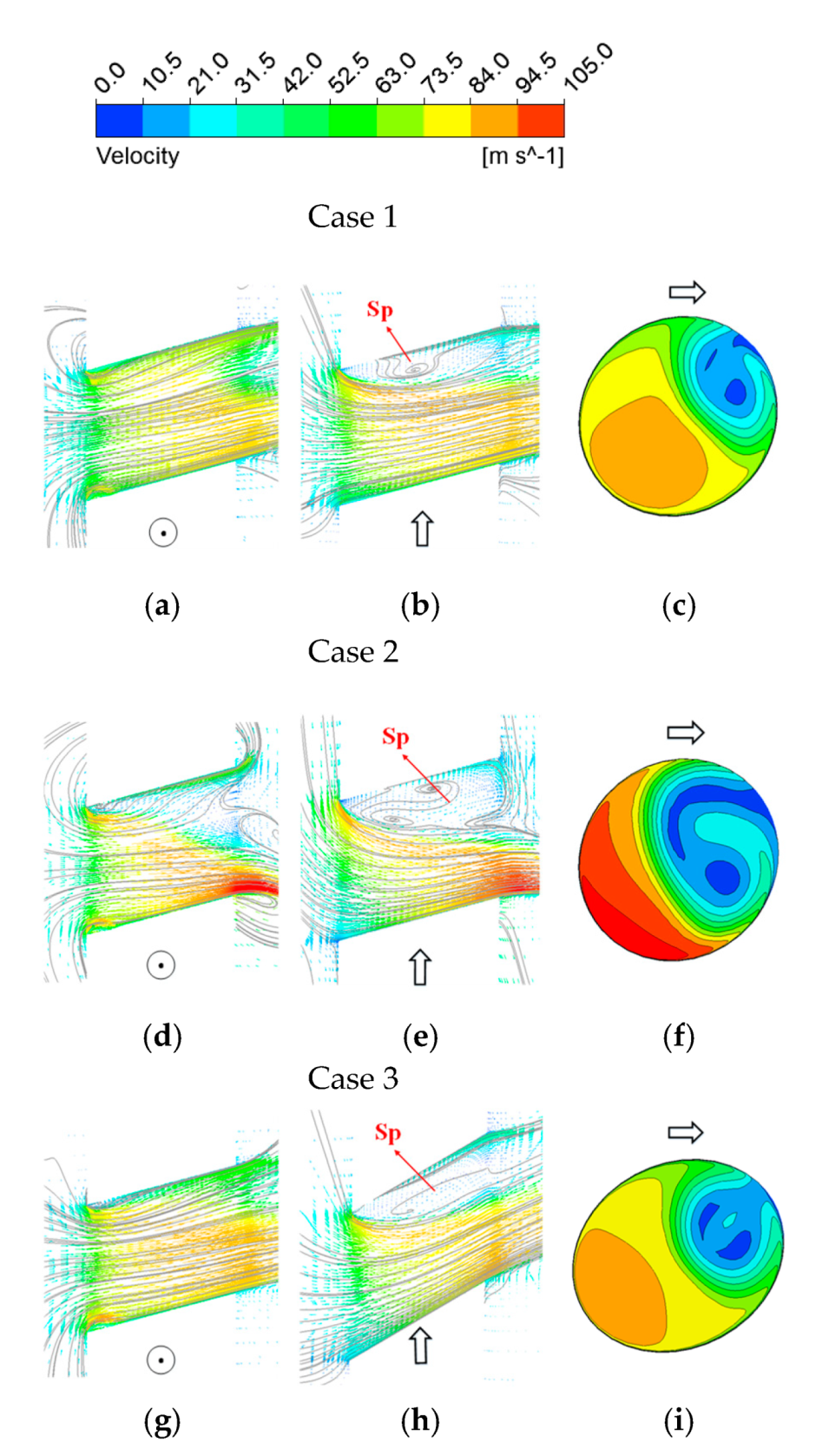
5.2. Effect of Radial Inclination Angle on the Discharge Coefficient
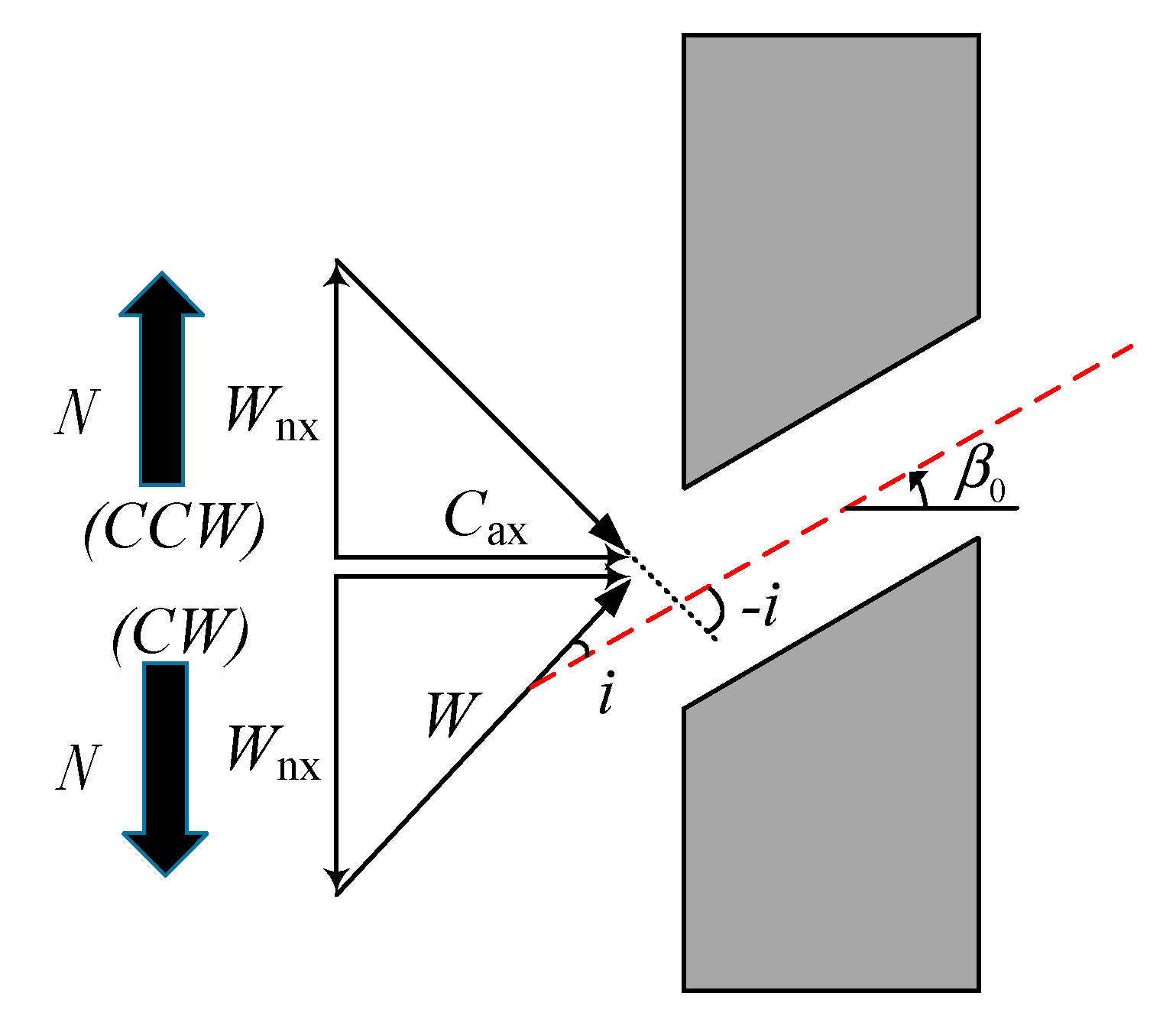
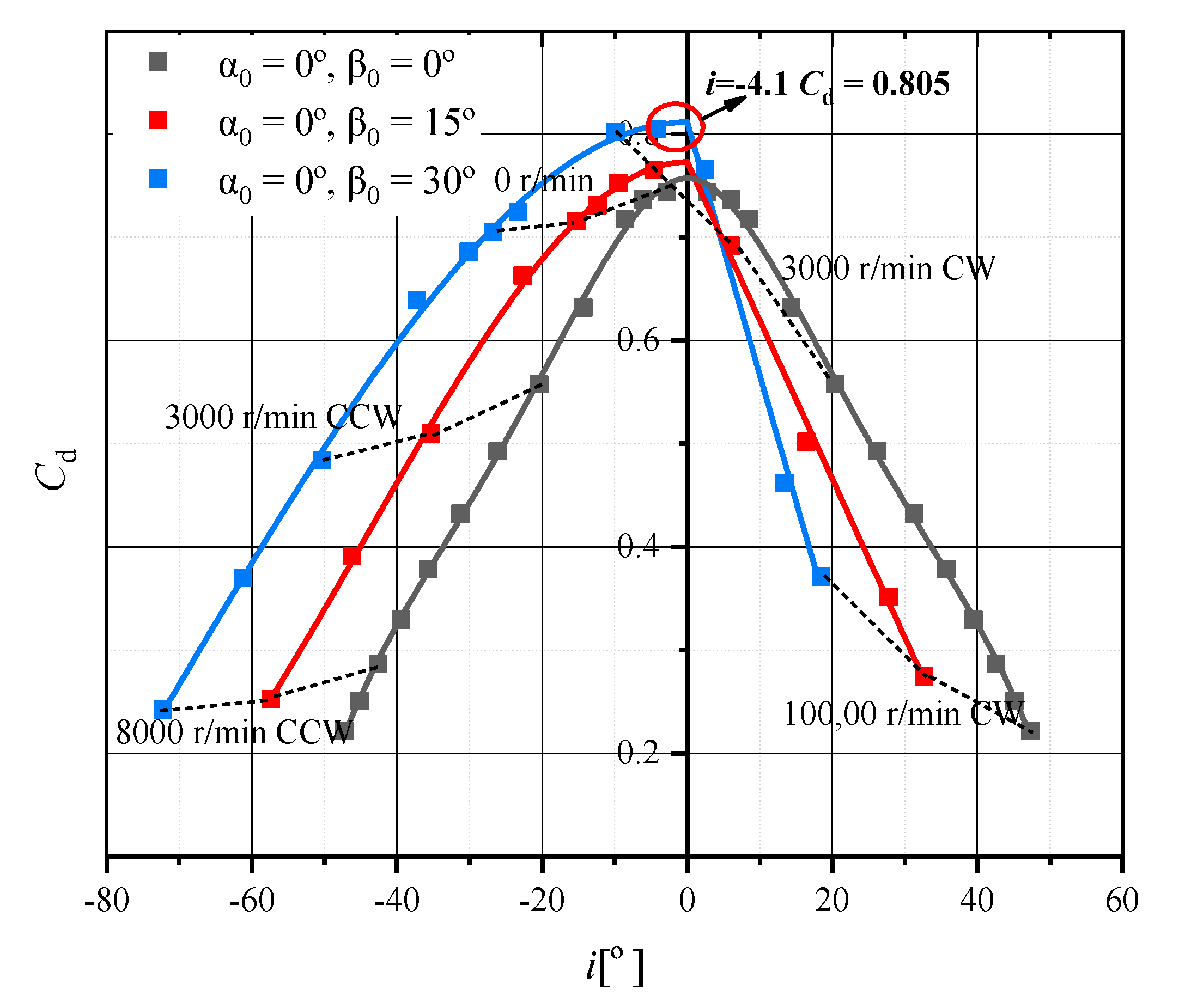
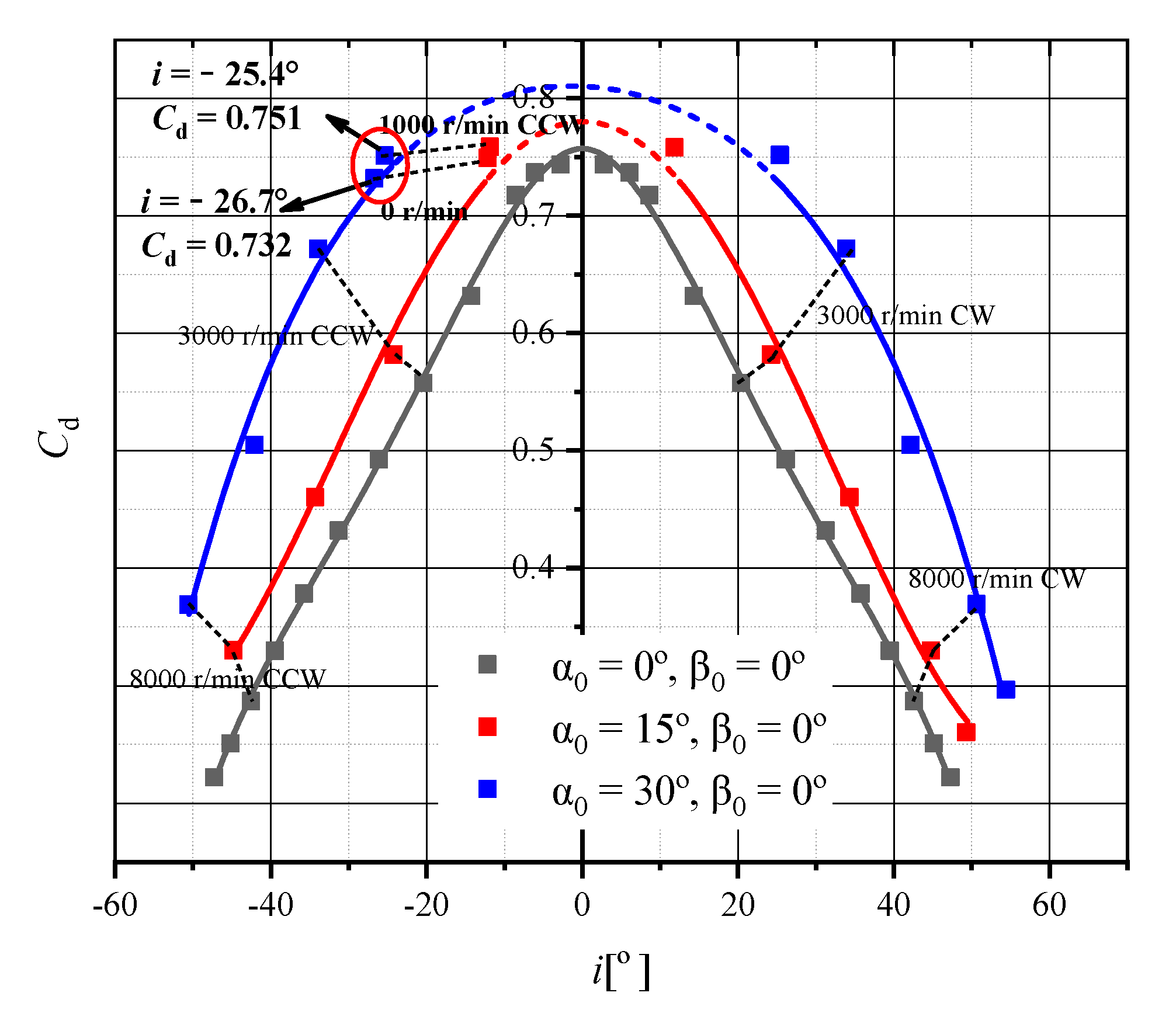
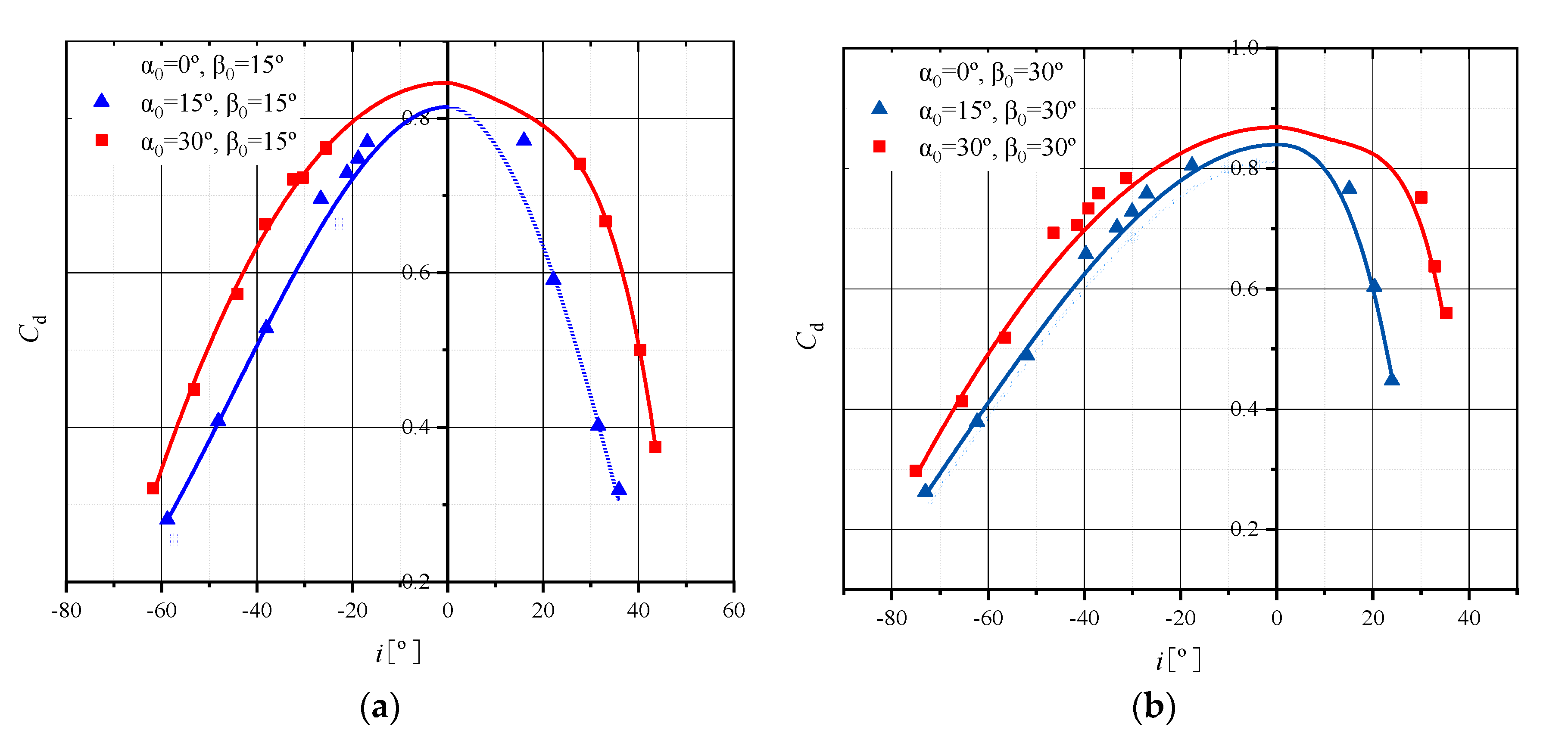
5.3. Effect of the Compound Angle on the Discharge Coefficient
5.3.1. Variation in Discharge Coefficients for the Same and Different
5.3.2. Variation in Discharge Coefficients for the Same and Different
5.4. A General Calculation Model of the Rotating Orifice Considering the Effect of the Comprehensive Incidence Angle
6. Conclusions
- (1)
- The Euler angles have a significant effect on the discharge coefficient of the rotating orifices. The flow separation caused by the Euler angle may change the actual cross-section flow area and result in a decrease in the discharge coefficient.
- (2)
- For the circumferential inclination angle, the magnitude and direction of the rotational speed are the main factors affecting the flow separation in the circumferential cross-section. For the radial inclination angle, the pump effect arising from rotation can reduce the flow separation region at the meridian section and improve the discharge coefficient to some extent.
- (3)
- A large radial inclination angle results in a greater Coriolis force, which may weaken the flow separation effect caused by rotation and the circumferential inclination angle, thus improving the discharge coefficient.
- (4)
- The comprehensive incidence angle is determined by the Euler angle (radial inclination angle and circumferential inclination angle) and rotation. It is clear that the discharge coefficient of the orifice increases with the decline in the comprehensive incidence angle. The discharge coefficient of orifices would reach the peak when the comprehensive incidence angle is 0.
- (5)
- The general calculation model of rotating orifices considering the effect of the comprehensive incidence angle is developed. The overall relative error between the calculation results and the experimental data in the published literature is within 6%, which meets the requirements of engineering design in the secondary air system.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Cross-sectional area [] | |
| Specific heat at constant pressure [] | |
| Axial velocity in the abs. frame of reference [] | |
| Discharge coefficient | |
| Diameter of orifice [m] | |
| Comprehensive incidence angle [] | |
| Circumferential incidence angle [] | |
| Length of orifice [m] | |
| Mass flow rate [] | |
| Disk rotating speed [] | |
| Pressure [] | |
| Radius of the cavity [m] | |
| Pitch radius [m] | |
| Inlet radius of the cavity [m] | |
| Inlet radius of the orifice [m] | |
| Ideal gas constant [] | |
| Re | Reynolds number |
| Sp | Separation region |
| Temperature [] | |
| Orifices rotating velocity []] | |
| Circumferential velocity []] | |
| Radial velocity [] | |
| Chamfering depth | |
| Relative velocity [] | |
| Axial velocity in the rel. frame of reference [] | |
| Greek symbols | |
| Radial inclination angle [] | |
| Circumferential inclination angle [] | |
| Specific heat ratio | |
| Angle of chamfering [] | |
| Pre-swirl angle [] | |
| Subscripts | |
| actual | |
| circumferential | |
| ideal | |
| inlet, outlet | |
| non-axial | |
| the rel. frame of reference | |
| static | |
| total | |
| upstream, downstream of orifices | |
Appendix A
| i | Positive | Negative | Positive | Negative | Positive | Negative |
|---|---|---|---|---|---|---|
| a | 0.757 | 0.757 | 0.773 | 0.773 | 0.811 | 0.811 |
| b | 0 | 0 | −0.0154 | 0 | −0.0245 | 0 |
| c | −8.62 × 10−4 | −8.62 × 10−4 | 0 | −2.78 × 10−4 | 0 | −1.63 × 10−4 |
| d | 2.4 × 10−5 | −2.4 × 10−5 | 0 | −2.09 × 10−6 | 0 | −7.48 × 10−7 |
| e | −2.29 × 10−7 | −2.29 × 10−7 | 0 | 0 | 0 | 0 |
| i | Positive/Negative | Positive | Negative | Positive | Negative | |
| a | 0.78 | 0.815 | 0.815 | 0.84 | 0.84 | |
| b | 2.35 × 10−5 | 0 | 0 | 0 | 0 | |
| c | −3.36 × 10−4 | −5.06 × 10−4 | −2.75 × 10−4 | −2.24 × 10−4 | −1.66 × 10−4 | |
| d | −8.16 × 10−8 | 3.15 × 1068 | −2.04 × 10−6 | −1.88 × 10−5 | −7.73 × 10−7 | |
| e | 5.35 × 10−8 | - | - | - | - | |
| i | Positive/Negative | Positive | Negative | Positive | Negative | |
| a | 0.81 | 0.846 | 0.846 | 0.869 | 0.869 | |
| b | −2.93 × 10−4 | −8.42 × 10−4 | 0 | 0 | 0 | |
| c | −1.05 × 10−4 | −2.1 × 10−4 | −1.21 × 10−4 | −3.8 × 10−4 | −1.11 × 10−4 | |
| d | 1.87 × 10−7 | 1.08 × 10−5 | 2.98 × 10−7 | 2.72 × 10−5 | −1.13 × 10−7 | |
| e | −2.63 × 10−8 | −2.57 × 10−7 | 0 | 6.85 × 10−7 | 0 | |
References
- Sehra, A.K.; Whitlow, W. Propulsion and power for 21st century aviation. Prog. Aerosp. Sci. 2004, 40, 199–235. [Google Scholar] [CrossRef]
- Setlak, L.; Kowalik, R.; Lusiak, T. Practical Use of Composite Materials Used in Military Aircraft. Materials 2021, 14, 4812. [Google Scholar] [CrossRef] [PubMed]
- Lewis, P.; Wilson, M.; Lock, G.D.; Owen, J.M. Effect of radial location of nozzles on performance of pre-swirl systems: A computational and theoretical study. J. Power Energy 2008, 223, 179–190. [Google Scholar] [CrossRef]
- Hay, N.; Lampard, D. Discharge coefficient of turbine cooling holes: A review. ASME J. Turbomach. 1998, 120, 314–319. [Google Scholar] [CrossRef]
- Lichtarowicz, A.; Duggins, R.K.; Markland, E. Discharge Coefficients for Incompressible Non-Cavitating Flow through Long Orifices. J. Mech. Eng. Sci. 1965, 7, 210–219. [Google Scholar] [CrossRef]
- Benedict, R.P.; Wyler, J.D.; Brandt, G.B. The Effect of Edge Sharpness on the Discharge Coefficient of an Orifice. J. Eng. Gas Turbines Power 1975, 97, 576–581. [Google Scholar] [CrossRef]
- Rohde, J.E.; Richards, H.T.; Metger, G.W. Discharge Coefficients for Thick Plate Orifices with Approach Flow Perpendicular and Inclined to the Orifice Axis. Washington: National Aeronautics and Space Administration. 1969. Available online: https://ntrs.nasa.gov/citations/19690028630 (accessed on 1 March 2022).
- Gritsch, M.; Schulz, A.; Wittig, S. Effect of Crossflows on the Discharge Coefficient of Film Cooling Holes With Varying Angles of Inclination and Orientation. ASME J. Turbomach. 2001, 123, 781–787. [Google Scholar] [CrossRef]
- Feseker, D.; Kinell, M.; Neef, M. Experimental Study on Pressure Losses in Circular Orifices With Inlet Cross Flow. ASME J. Turbomach. 2018, 140, 071006. [Google Scholar] [CrossRef]
- Meyfarth, P.F.; Shine, A.J. Experimental Study of Flow through Moving Orifices. J. Fluids Eng. 1965, 87, 1082–1083. [Google Scholar] [CrossRef]
- Wittig, S.; Kim, S.; Jakoby, R. Experimental and Numerical Study of Orifice Discharge Coefficients in High-Speed Rotating Disks. ASME J. Turbomach. 1996, 118, 400–407. [Google Scholar] [CrossRef]
- Maeng, D.J.; Lee, J.S.; Jakoby, R. Characteristics of Discharge Coefficient in a Rotating Disk System. J. Eng. Gas Turbines Power 1999, 121, 663–669. [Google Scholar] [CrossRef]
- Zimmermann, H.; Kutz, J.; Fischer, R. Air System Correlations: Part 2—Rotating Holes and Two Phase Flow. In Proceedings of the ASME 1998 International Gas Turbine and Aeroengine Congress and Exhibition, Stockholm, Sweden, 2–5 June 1998. [Google Scholar]
- Dittmann, M.; Dullenkopf, K.; Wittig, S. Discharge Coefficients of Rotating Short Orifices with Radiused and Chamfered Inlets. In Proceedings of the ASME Turbo Expo 2003: Power for Land, Sea, and Air, Atlanta, GA, USA, 16–19 June 2003; pp. 1001–1009. [Google Scholar]
- Du, Q.; Xie, L.; Liu, G. Influence of pumping effect on axial rotating holes. Energy Rep. 2021, 7, 2343–2353. [Google Scholar] [CrossRef]
- Sousek, J.; Pfitzner, M.; Niehuis, R. Experimental Study of Discharge Coefficients for Radial Orifices in High-Speed Rotating Shafts. In Proceedings of the ASME Turbo Expo 2010: Power for Land, Sea, and Air, Glasgow, UK, 14–18 June 2010; pp. 1051–1060. [Google Scholar]
- Sousek, J.; Riedmuller, D.; Pfitzner, M. Experimental and Numerical Investigation of the Flow Field at Radial Holes in High-Speed Rotating Shafts. In Proceedings of the ASME Turbo Expo 2012: Turbine Technical Conference and Exposition, Copenhagen, Denmark, 11–15 June 2012; pp. 1899–1912. [Google Scholar]
- Hay, N.; Henshall, S.E.; Manning, A. Discharge Coefficients of Holes Angled to the Flow Direction. ASME J. Turbomach. 1994, 116, 92–96. [Google Scholar] [CrossRef]
- Idris, A.; Pullen, K.; Barnes, D. An Investigation into the Flow Within Inclined Rotating Orifices and the Influence of Incidence Angle on the Discharge Coefficient. Proc. Inst. Mech. Eng. Part A J. Power Energy 2004, 218, 55–68. [Google Scholar] [CrossRef]
- Idris, A.; Pullen, K.R.; Read, R. The Influence of Incidence Angle on the Discharge Coefficient for Rotating Radial Orifices. In Proceedings of the ASME Turbo Expo 2004: Power for Land, Sea, and Air, Vienna, Austria, 14–17 June 2004; pp. 307–320. [Google Scholar]
- Lee, J.; Lee, H.; Kim, D.; Cho, J. The effect of rotating receiver hole shape on a gas turbine pre-swirl system. J. Mech. Sci. Technol. 2020, 34, 2179–2187. [Google Scholar] [CrossRef]
- Ahn, J.; Schobeiri, M.T.; Han, J.C.; Moon, H.K. Effect of rotation on leading edge region film cooling of a gas turbine blade with three rows of film cooling holes. Int. J. Heat Mass Transf. 2007, 50, 15–25. [Google Scholar] [CrossRef]
- Chang, J.L.; XU, J.J.; Duan, X.L. Investigations of effect for different influencing factors on film cooling effectiveness—Steady coolant ejected. Energy Rep. 2021, 7, 1453–1465. [Google Scholar] [CrossRef]
- Li, W.; Lu, X.; Li, X. High resolution measurements of film cooling performance of simple and compound angle cylindrical holes with varying hole length-to-diameter ratio—Part I: Adiabatic film effectiveness. Int. J. Therm. Sci. 2018, 124, 146–161. [Google Scholar] [CrossRef]
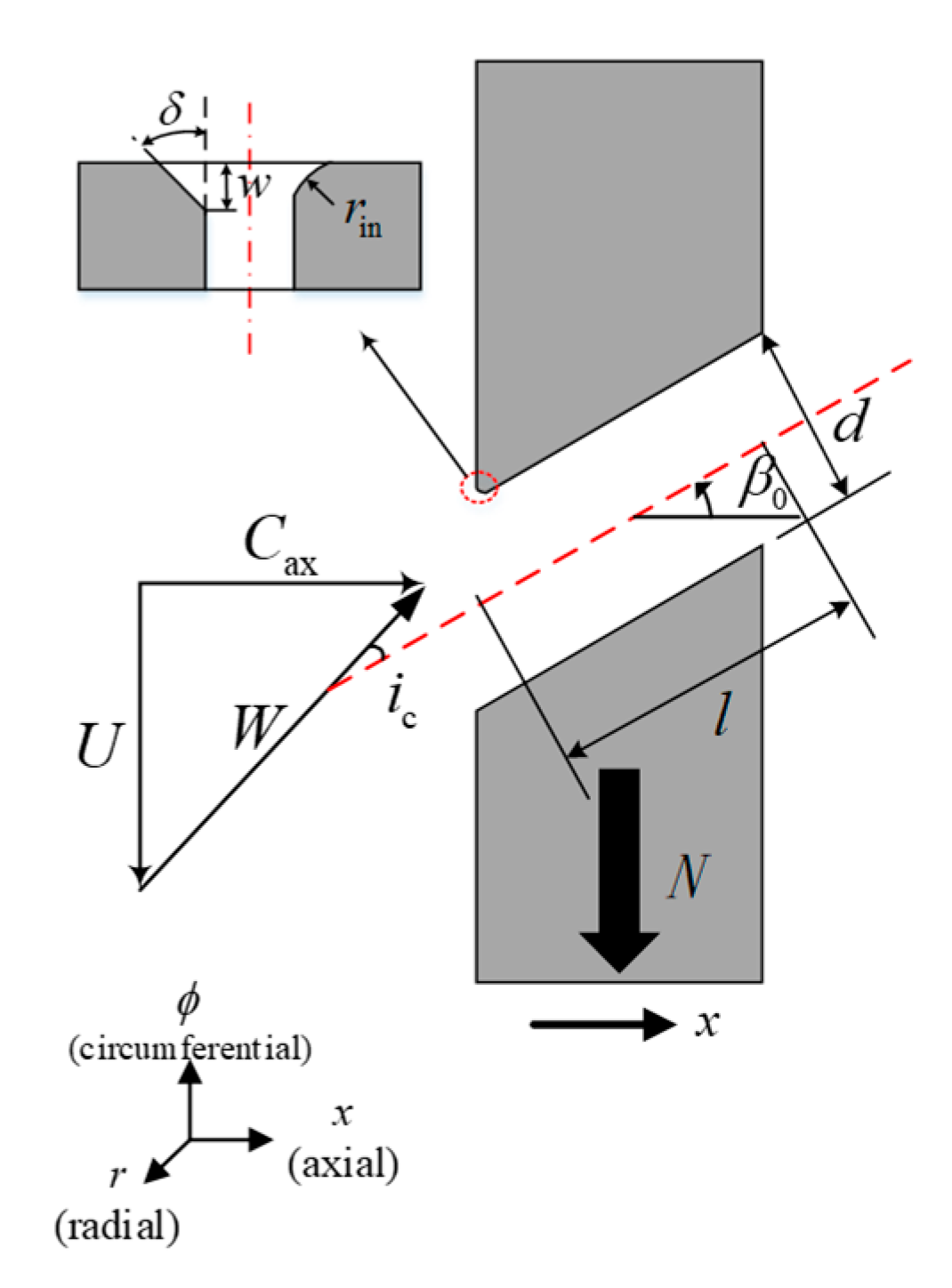
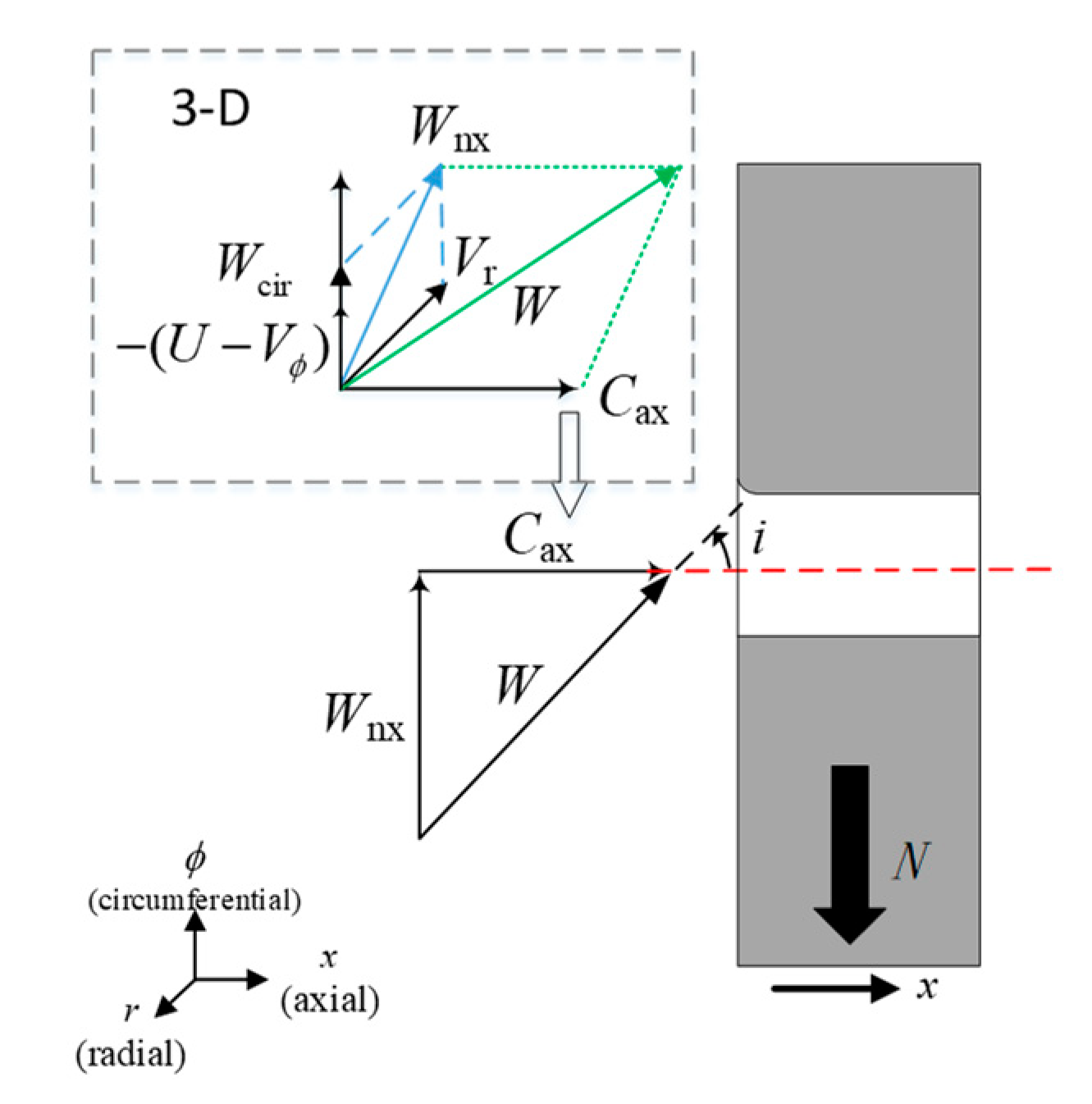

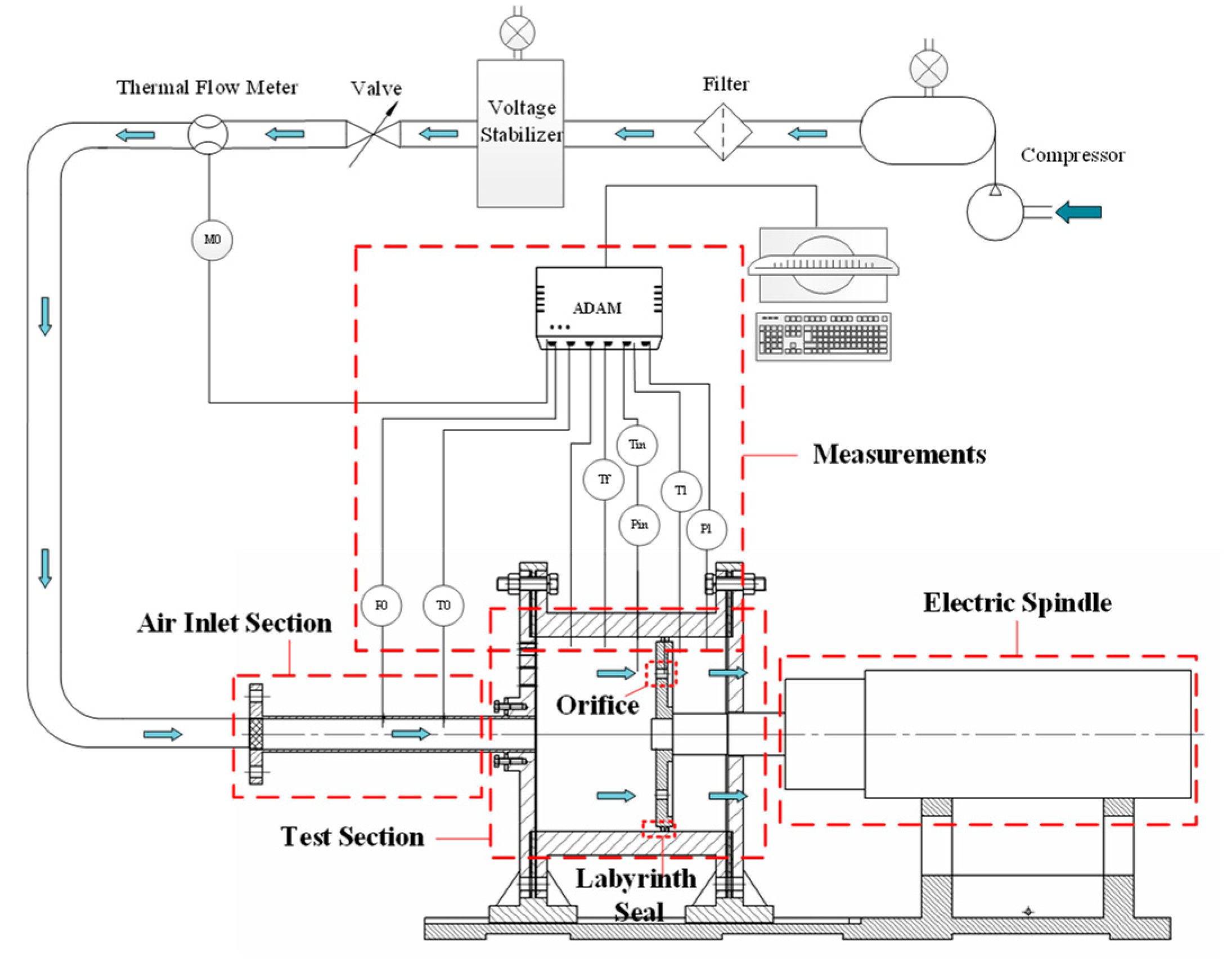

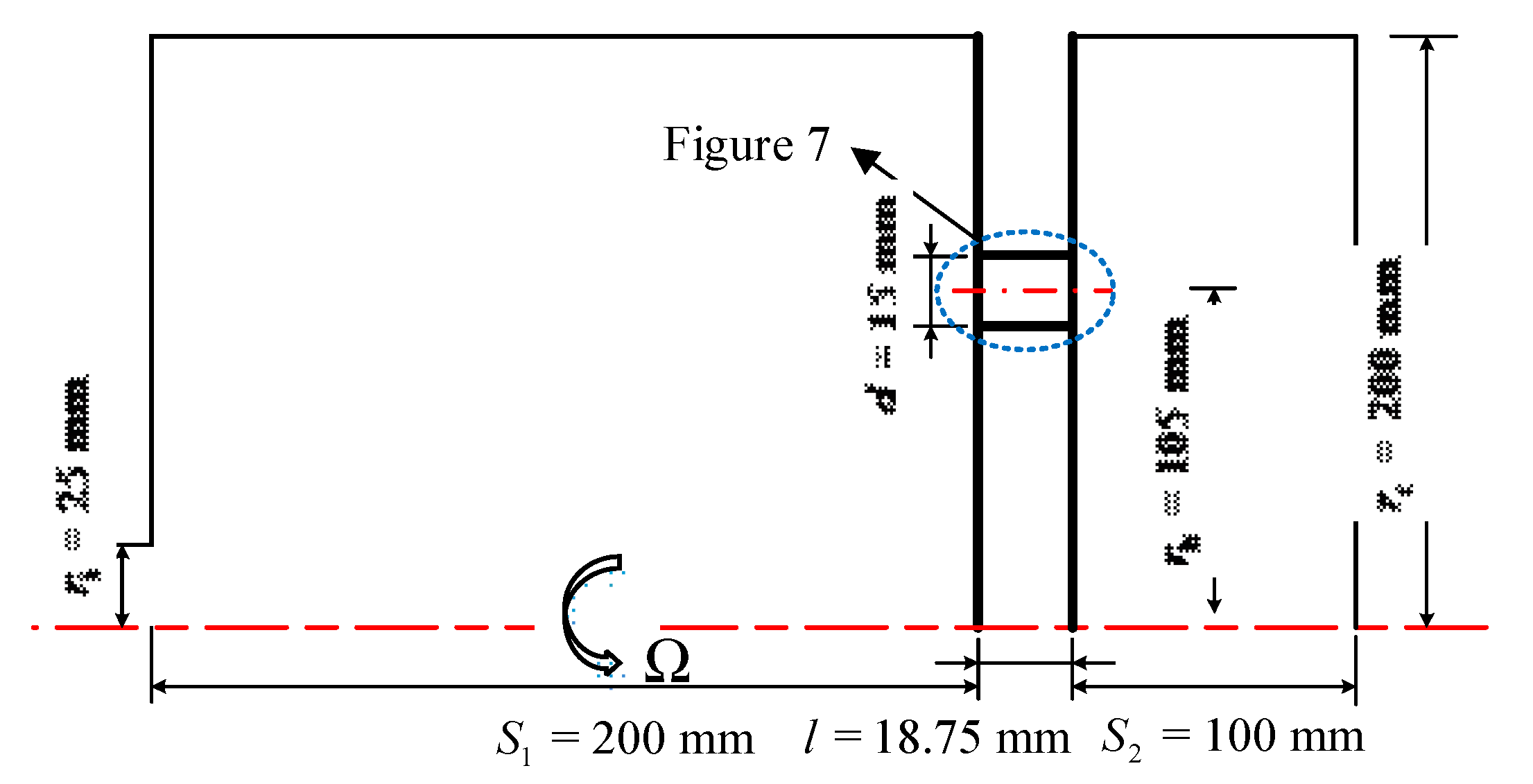

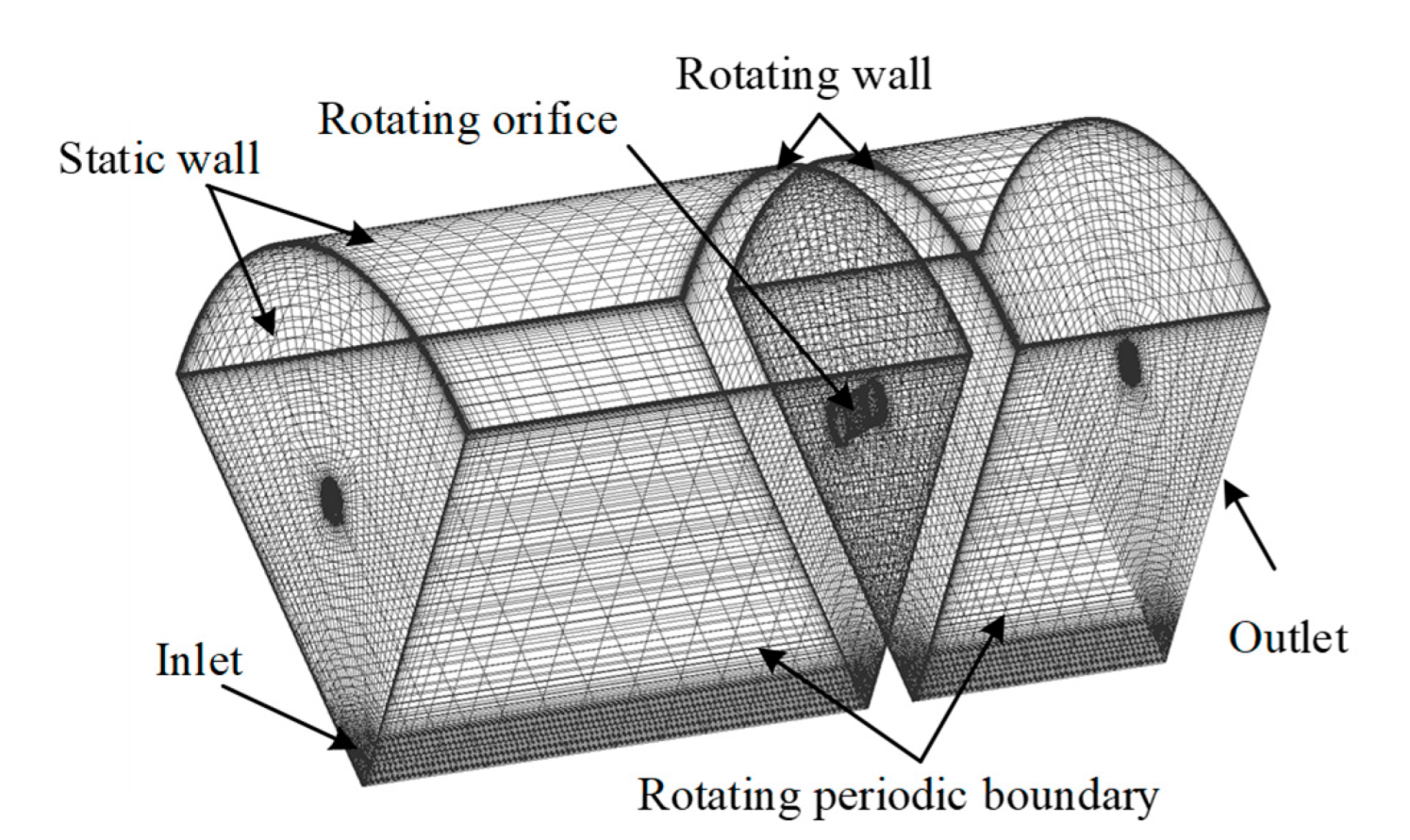
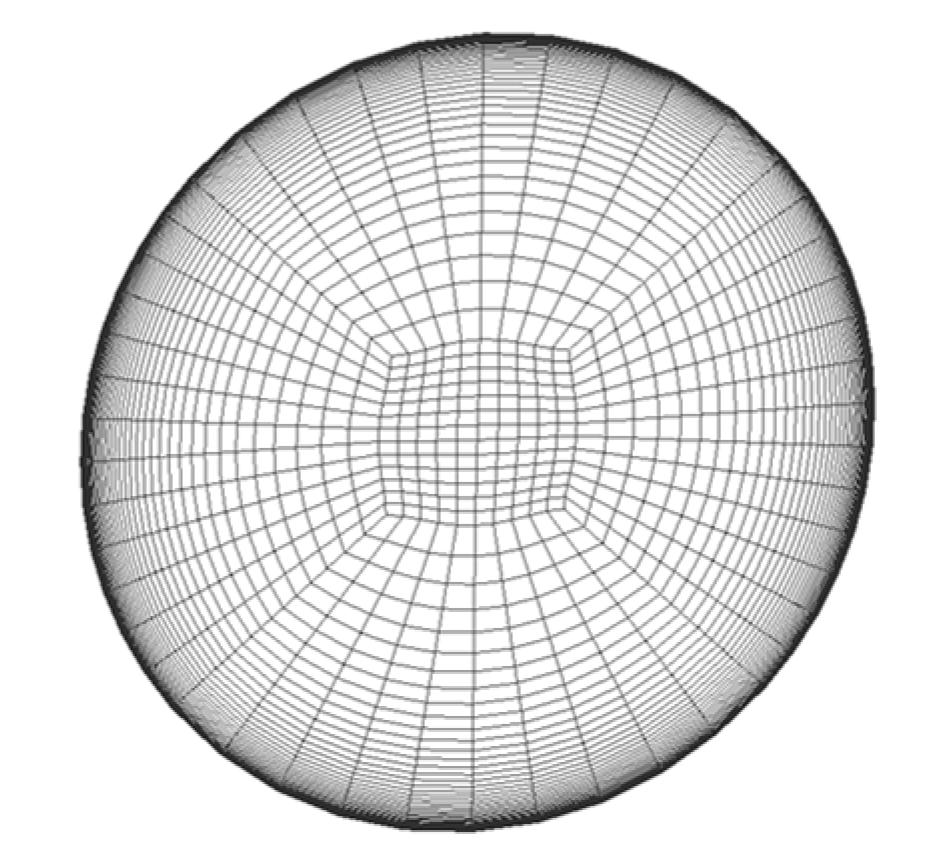
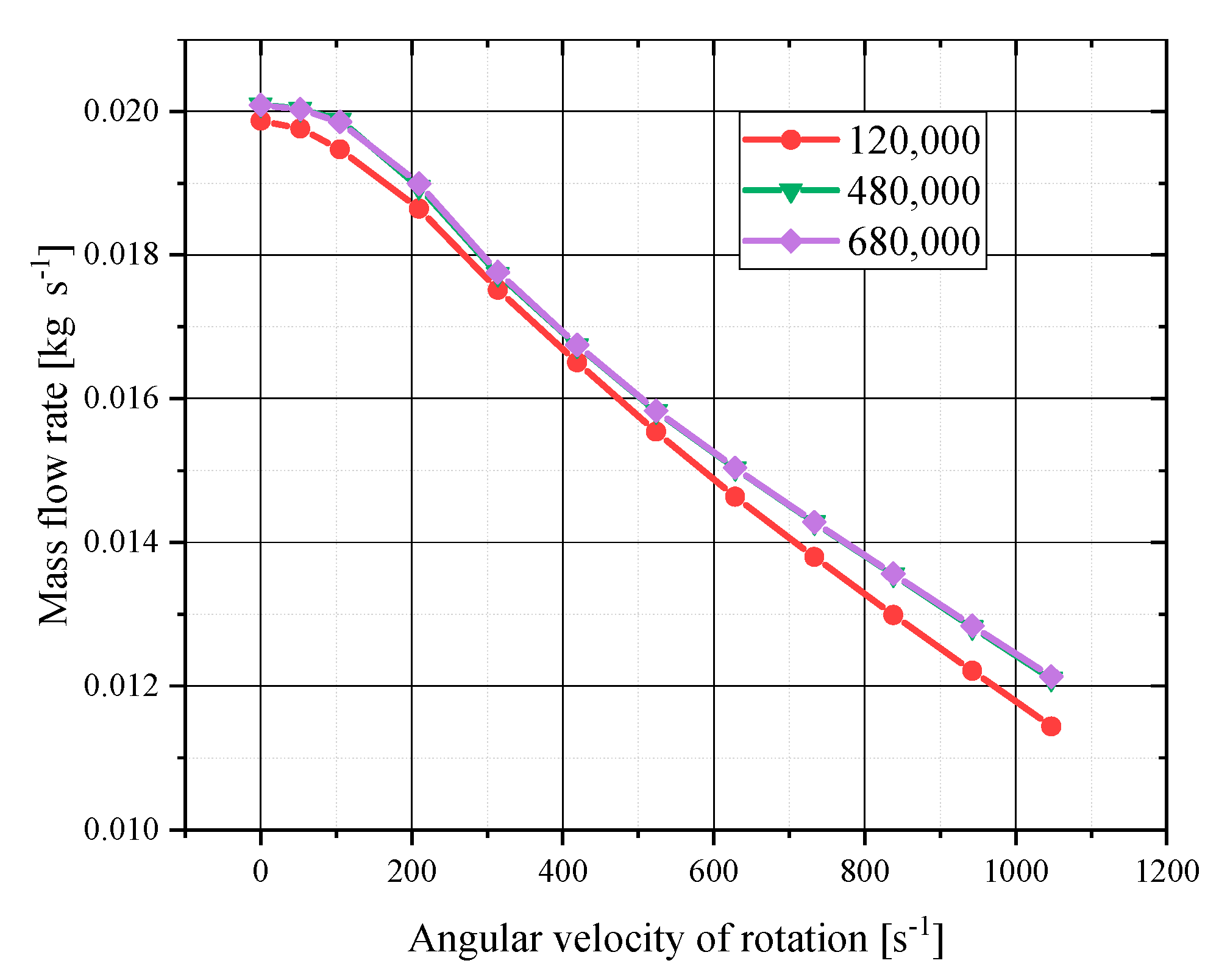
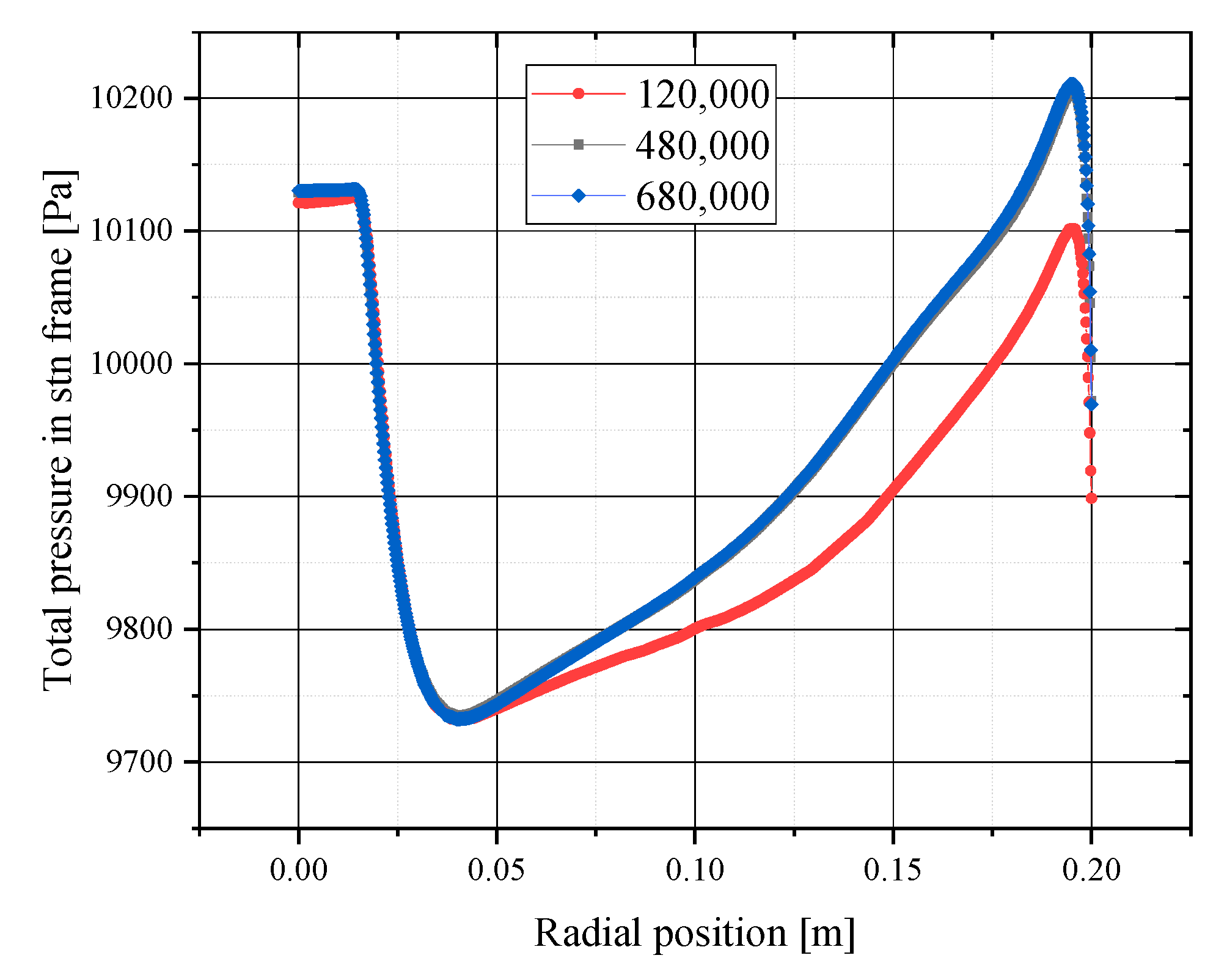


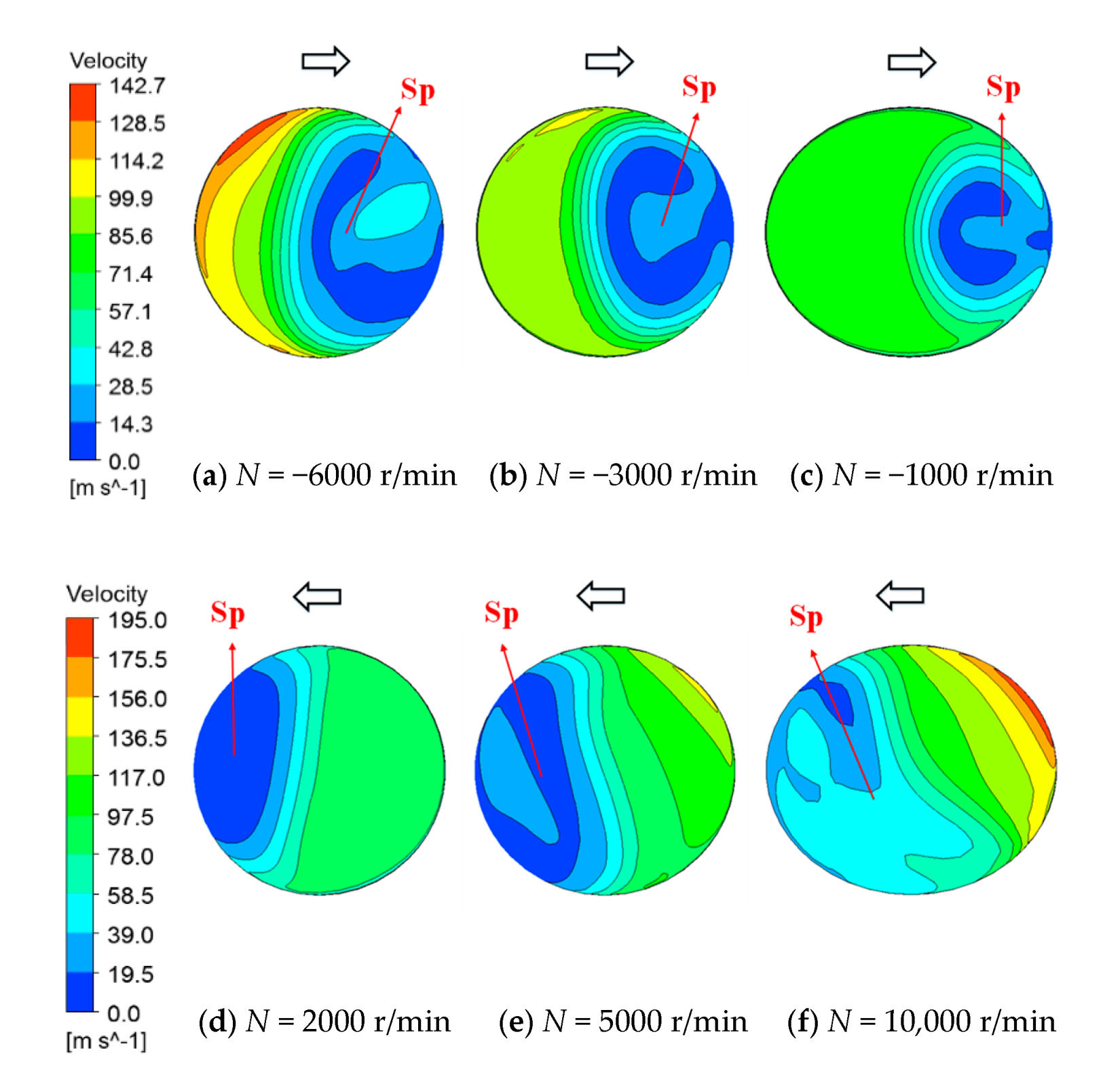
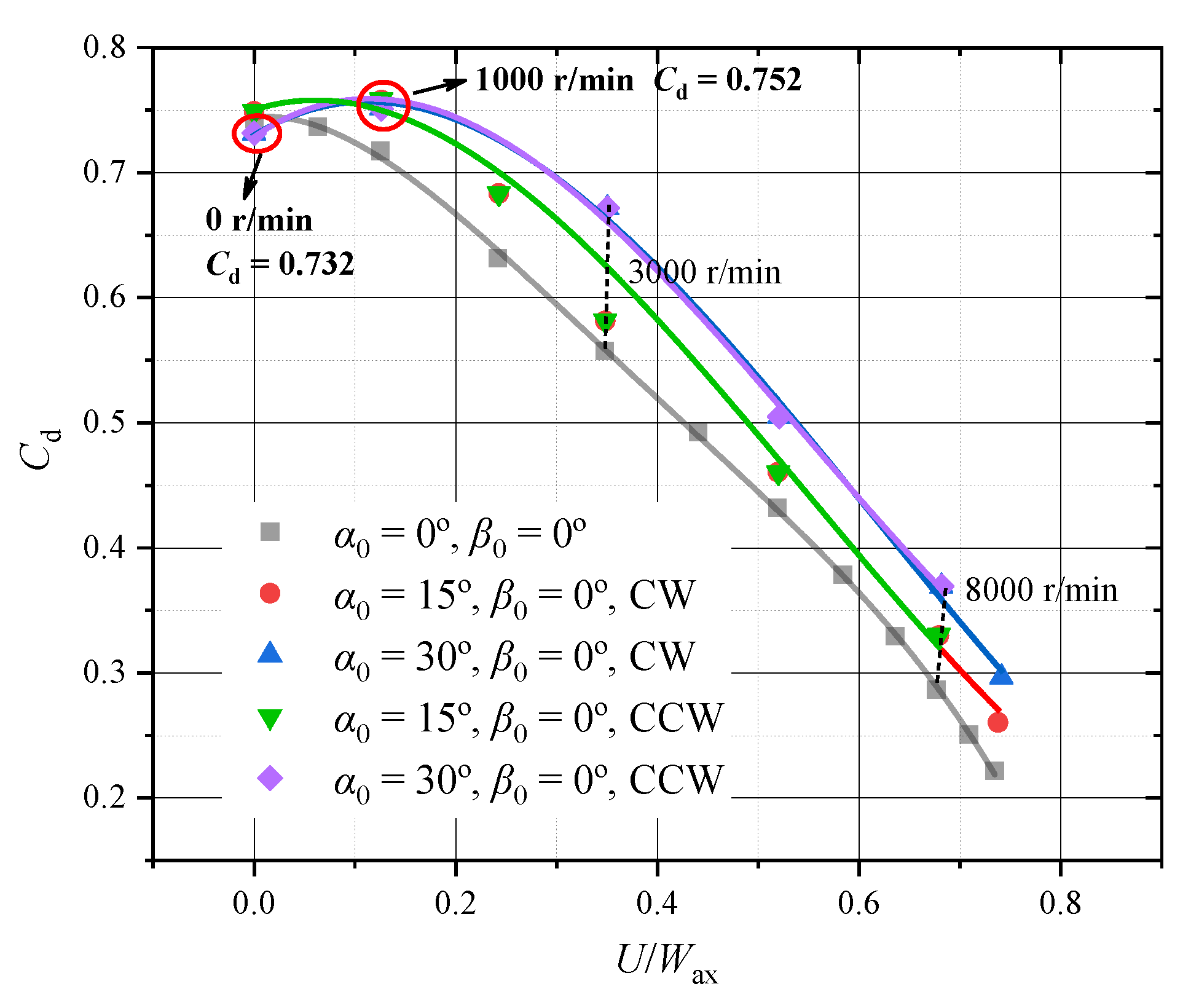
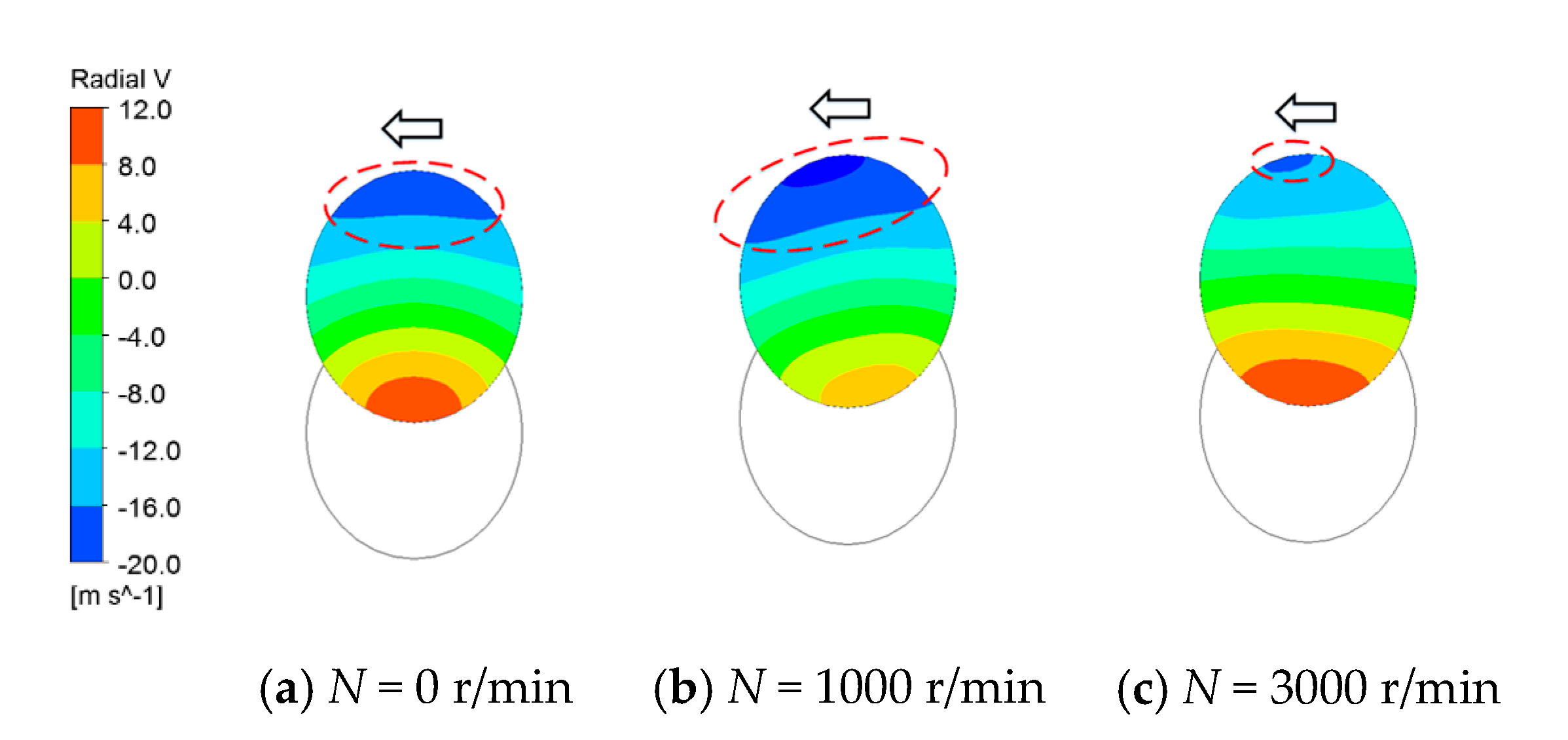
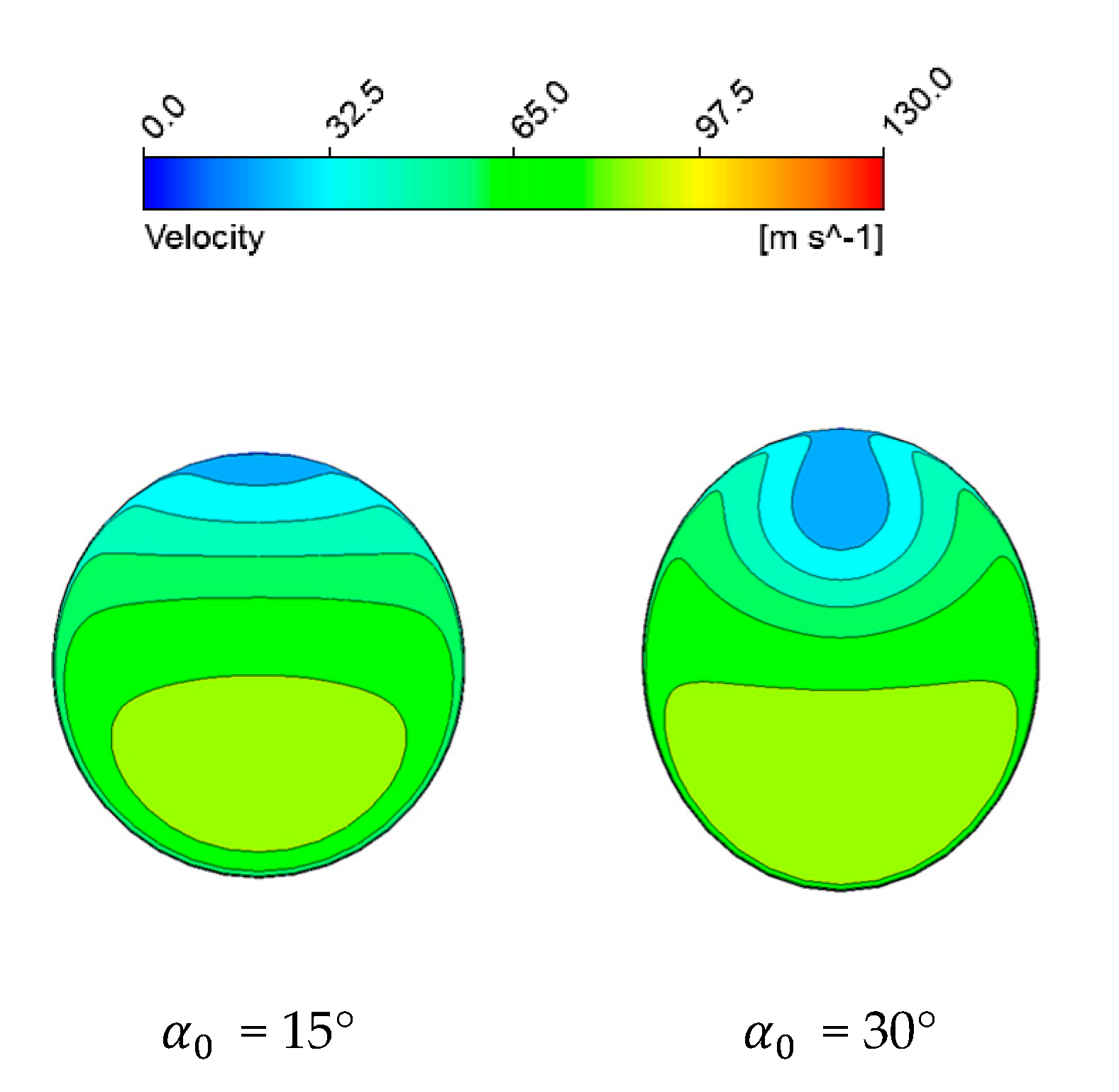




| Orifice | l/d | π | Inlet Crossflow (Ma) | Outlet Crossflow (Ma) | Rotation | Pre-Swirl | ||
|---|---|---|---|---|---|---|---|---|
| Rohde [7] | 0 | 45 | 0.51–4 | 1.1–1.55 | 0.1–0.7 | 0 | Non | - |
| Hay [4] | 0–180 | 30 | 6 | 1–2 | 0 | 0.31 | Non | - |
| Gritsch [8] | 0,45,90 | 0–60 | 3–6 | 1–2.25 | 0–0.6 | 0–1.2 | Non | - |
| Dittmann [14] | 0 | 0 | 0.4,1.25 | 1.05–1.6 | 0 | 0 | 0–9500 | - |
| Du Qiang [15] | 0 | 0 | 0.5 | 1.0–1.5 | 0 | 0 | 2000–8000 | - |
| Sousek [16] | 0 | 0 | 1.2 | 1.05–1.5 | 0–0.2 | 0 | 0–5000 | 25° |
| Idris [19] | 0 | 0–30 | 1.4–1.62 | 1.06 | 0 | 0 | 0–21,000 | - |
| Jungsoo Lee [21] | 0 | 45 | - | 1.2–1.35 | - | - | 0–3600 | Swirl ratio 0.8–1.4 |
| Jaeyong Ahn [22] | 20 | - | d = 1.19 | <1.41 | - | - | 2400–3000 | - |
| Present | 0,15,30 | 0,15,30 | d = 1.25 | 1.06 | 0 | 0 | 0–10,000 | - |
| Orifice | l/d | ||
|---|---|---|---|
| 1 | 0 | 0 | 1.25 |
| 2 | 0 | 15 | 1.25 |
| 3 | 0 | 30 | 1.25 |
| 4 | 15 | 0 | 1.25 |
| 5 | 15 | 15 | 1.25 |
| 6 | 15 | 30 | 1.25 |
| 7 | 30 | 0 | 1.25 |
| 8 | 30 | 15 | 1.25 |
| 9 | 30 | 30 | 1.25 |
| (Pa) | 106,391 |
| (K) | 288.15 |
| (Pa) | 101,325 |
| (N/min) | 0, 1000, 2000, 3000, 5000, 8000, 10,000, −1000, −3000, −5000, −8000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Liu, P.; Qiu, T.; Ding, S. An Investigation into the Flow of Rotating Orifices with Euler Angle and the Calculation Model of Discharge Coefficient Considering the Effect of Comprehensive Incidence Angle. Aerospace 2022, 9, 179. https://doi.org/10.3390/aerospace9040179
Wang J, Liu P, Qiu T, Ding S. An Investigation into the Flow of Rotating Orifices with Euler Angle and the Calculation Model of Discharge Coefficient Considering the Effect of Comprehensive Incidence Angle. Aerospace. 2022; 9(4):179. https://doi.org/10.3390/aerospace9040179
Chicago/Turabian StyleWang, Jie, Peng Liu, Tian Qiu, and Shuiting Ding. 2022. "An Investigation into the Flow of Rotating Orifices with Euler Angle and the Calculation Model of Discharge Coefficient Considering the Effect of Comprehensive Incidence Angle" Aerospace 9, no. 4: 179. https://doi.org/10.3390/aerospace9040179
APA StyleWang, J., Liu, P., Qiu, T., & Ding, S. (2022). An Investigation into the Flow of Rotating Orifices with Euler Angle and the Calculation Model of Discharge Coefficient Considering the Effect of Comprehensive Incidence Angle. Aerospace, 9(4), 179. https://doi.org/10.3390/aerospace9040179






