Aeroservoelastic Stability Evaluation for Slender Vehicles Based on the Ground Frequency Response Test
Abstract
:1. Introduction
2. ASE System Modeling
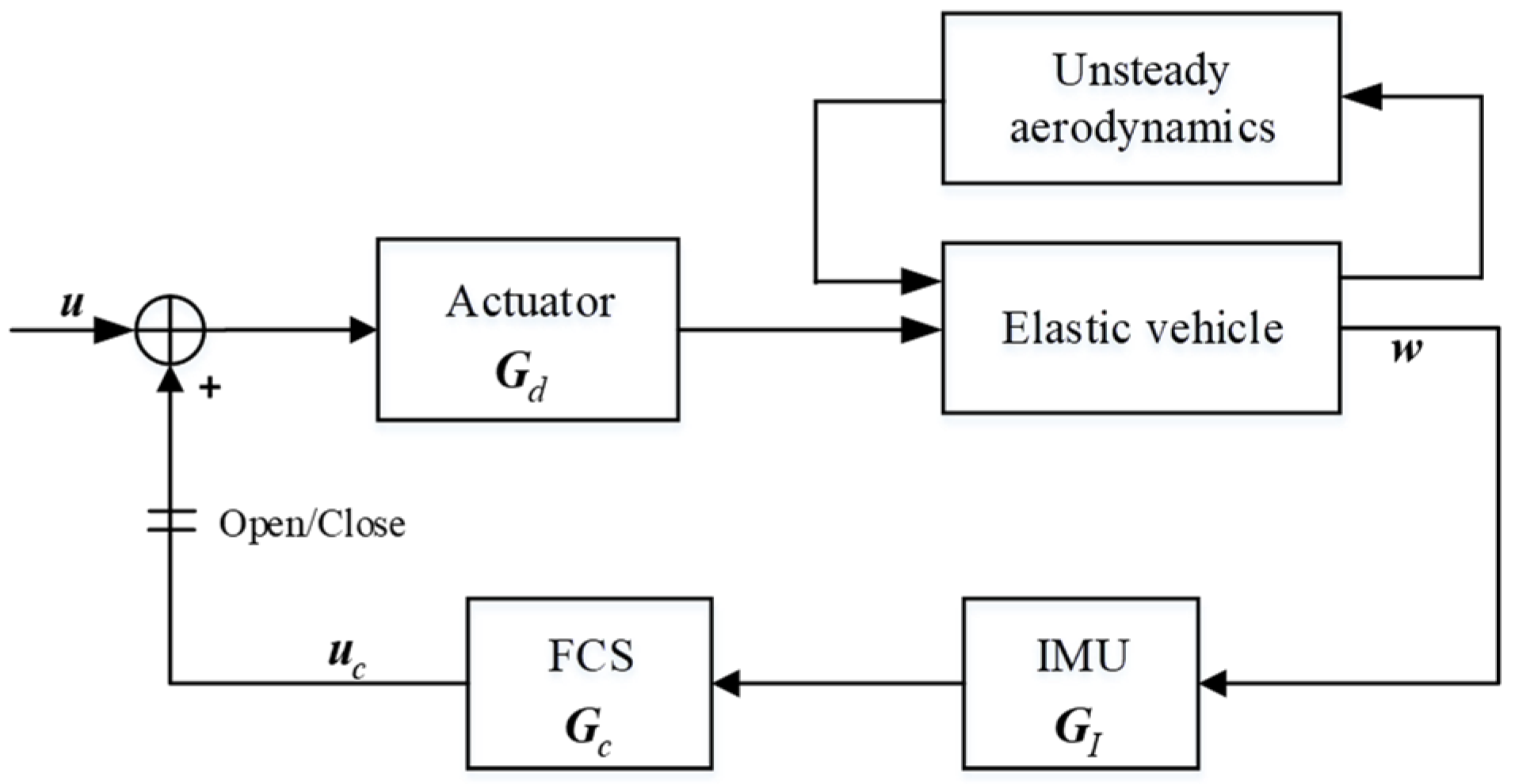
2.1. Theoretical ASE System
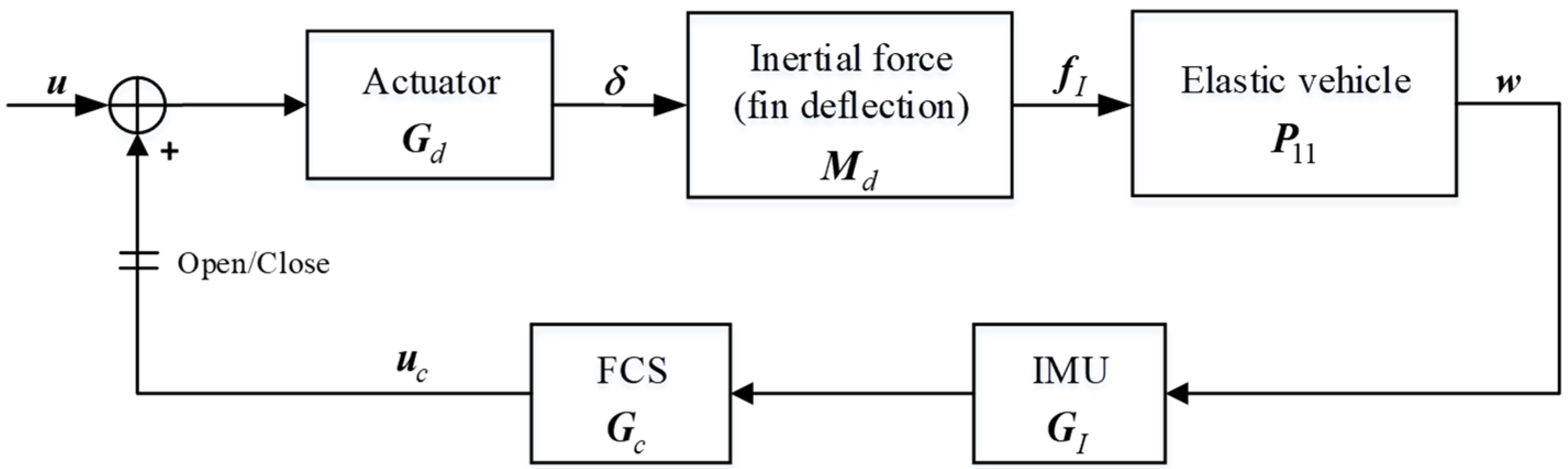
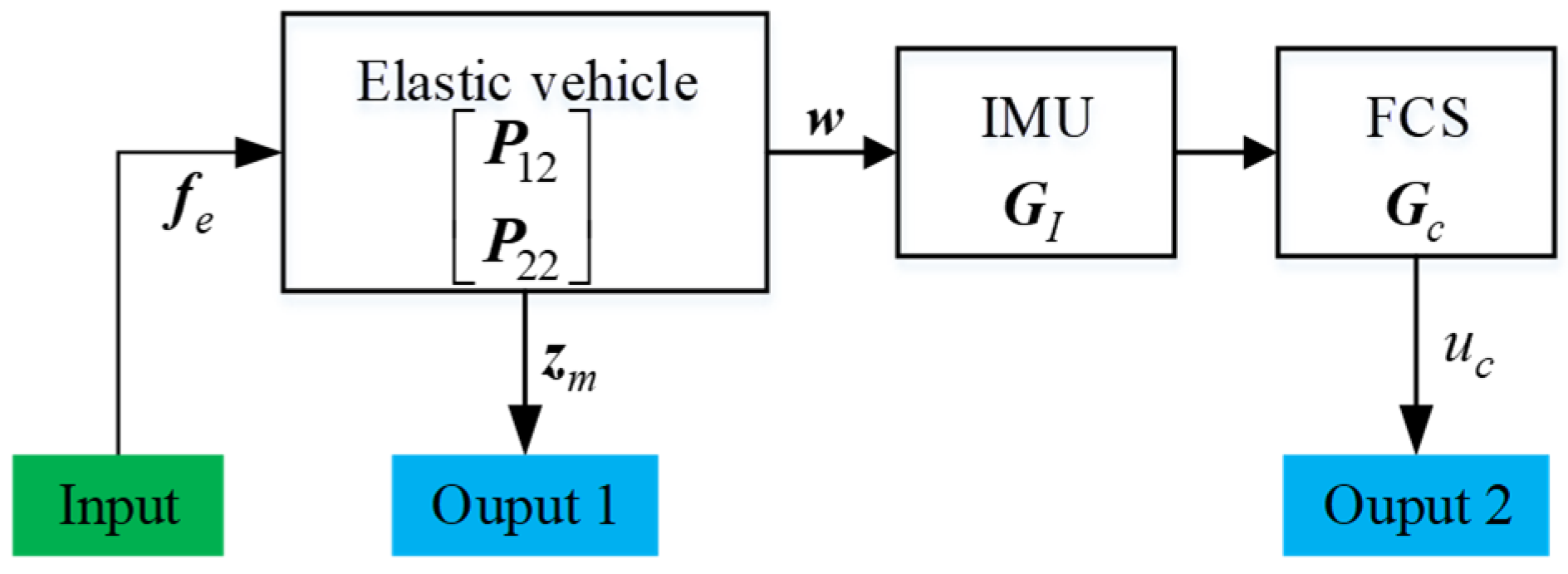
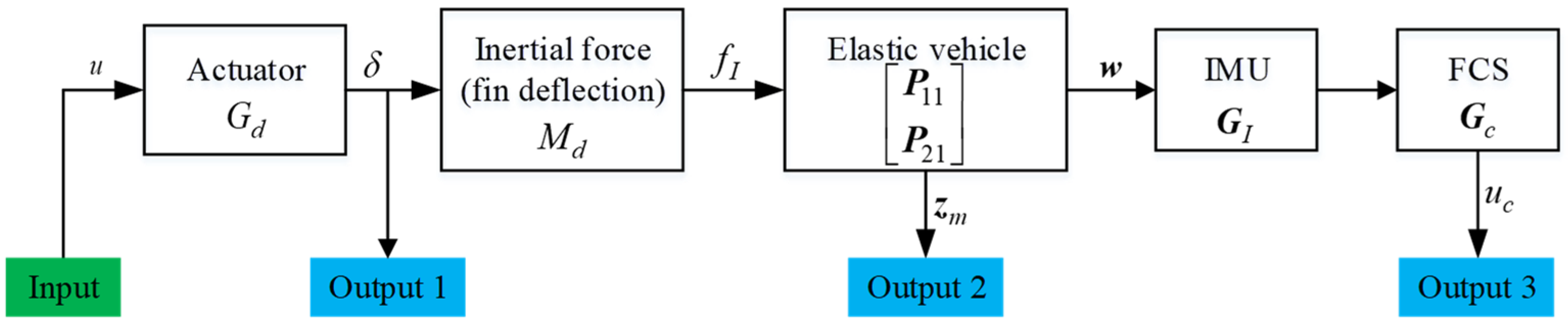
2.2. SCT Systems
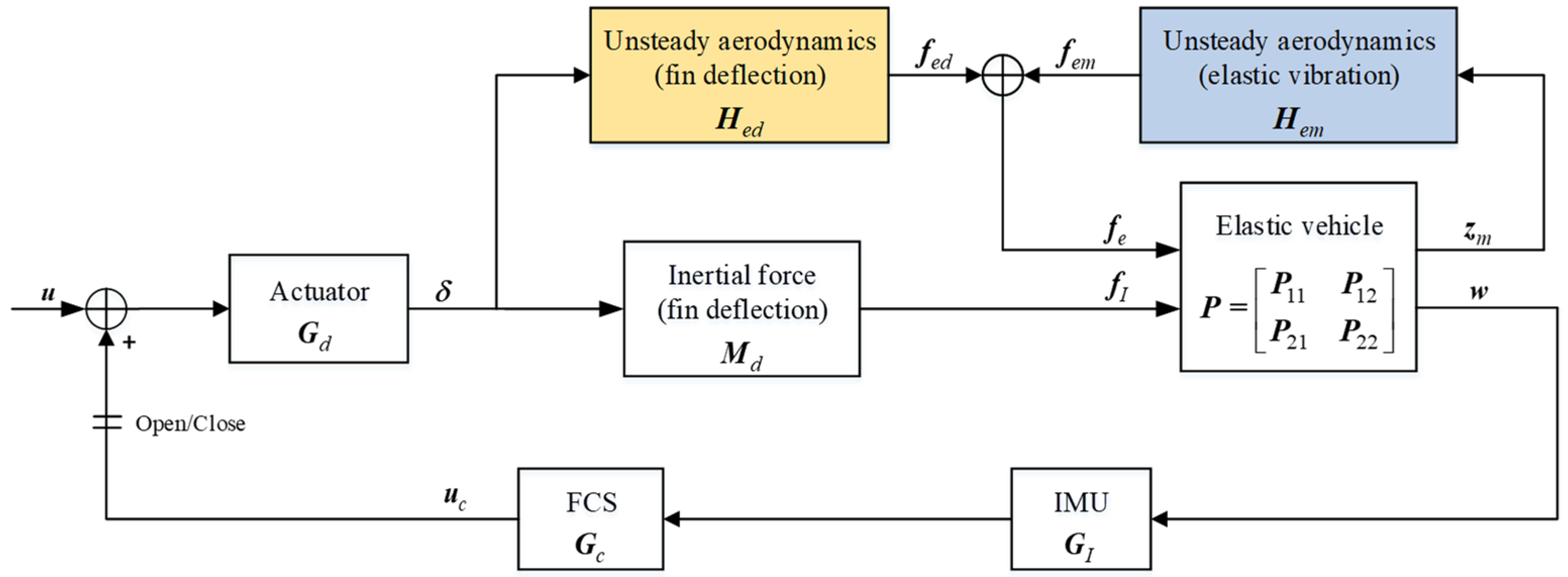
2.3. Improved ASE System
3. ASE Stability Evaluation for Slender Vehicles
3.1. Preliminaries
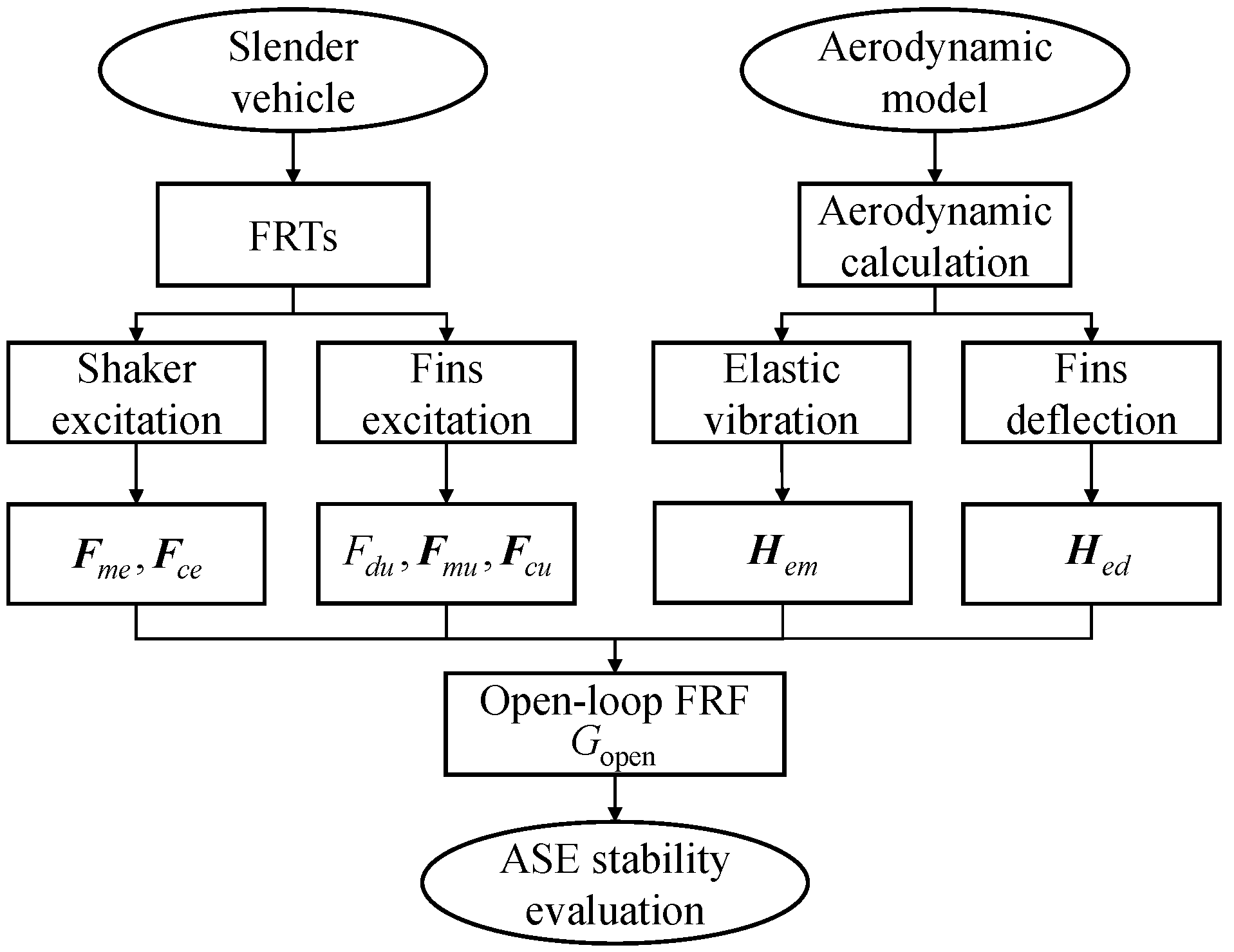
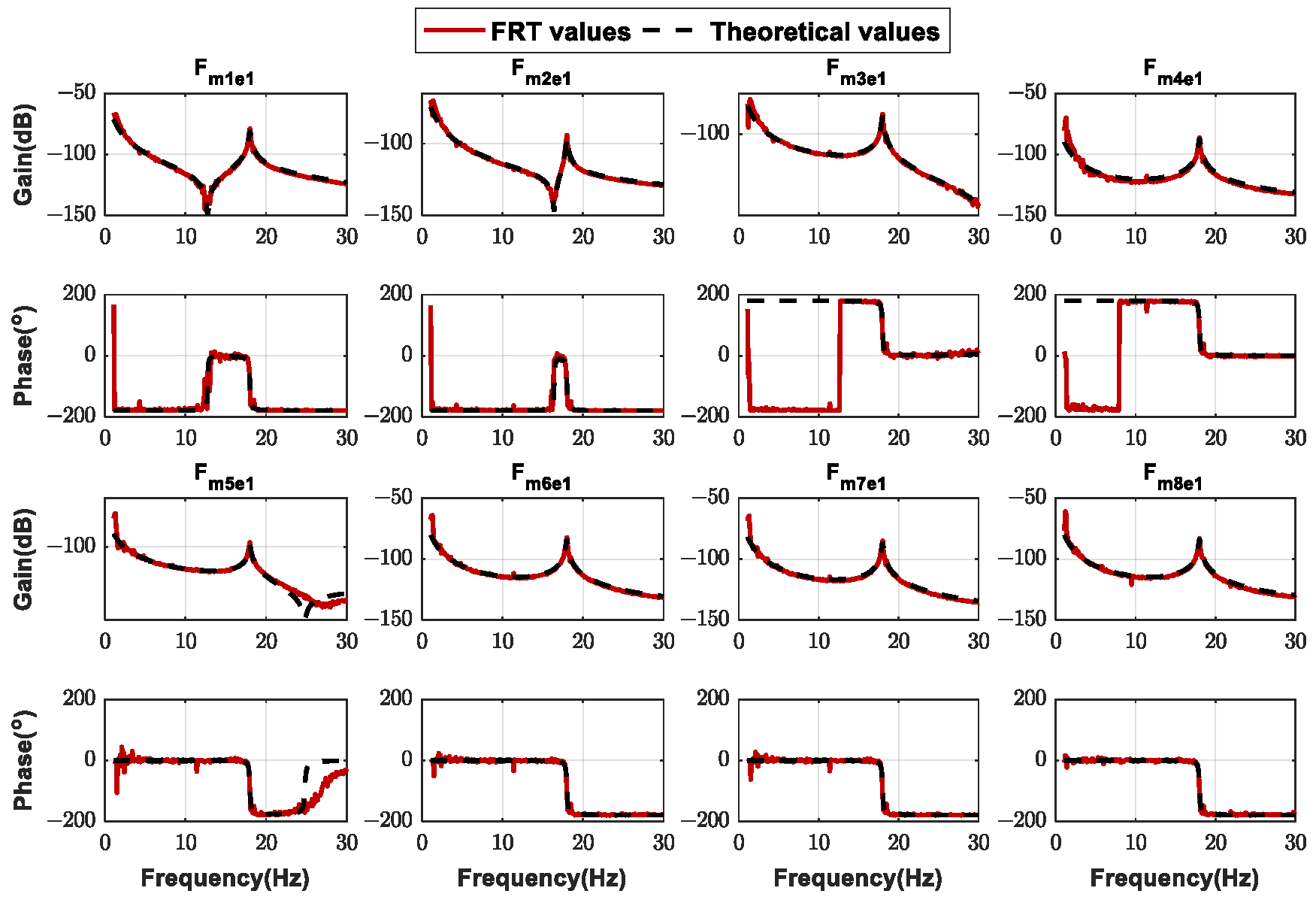
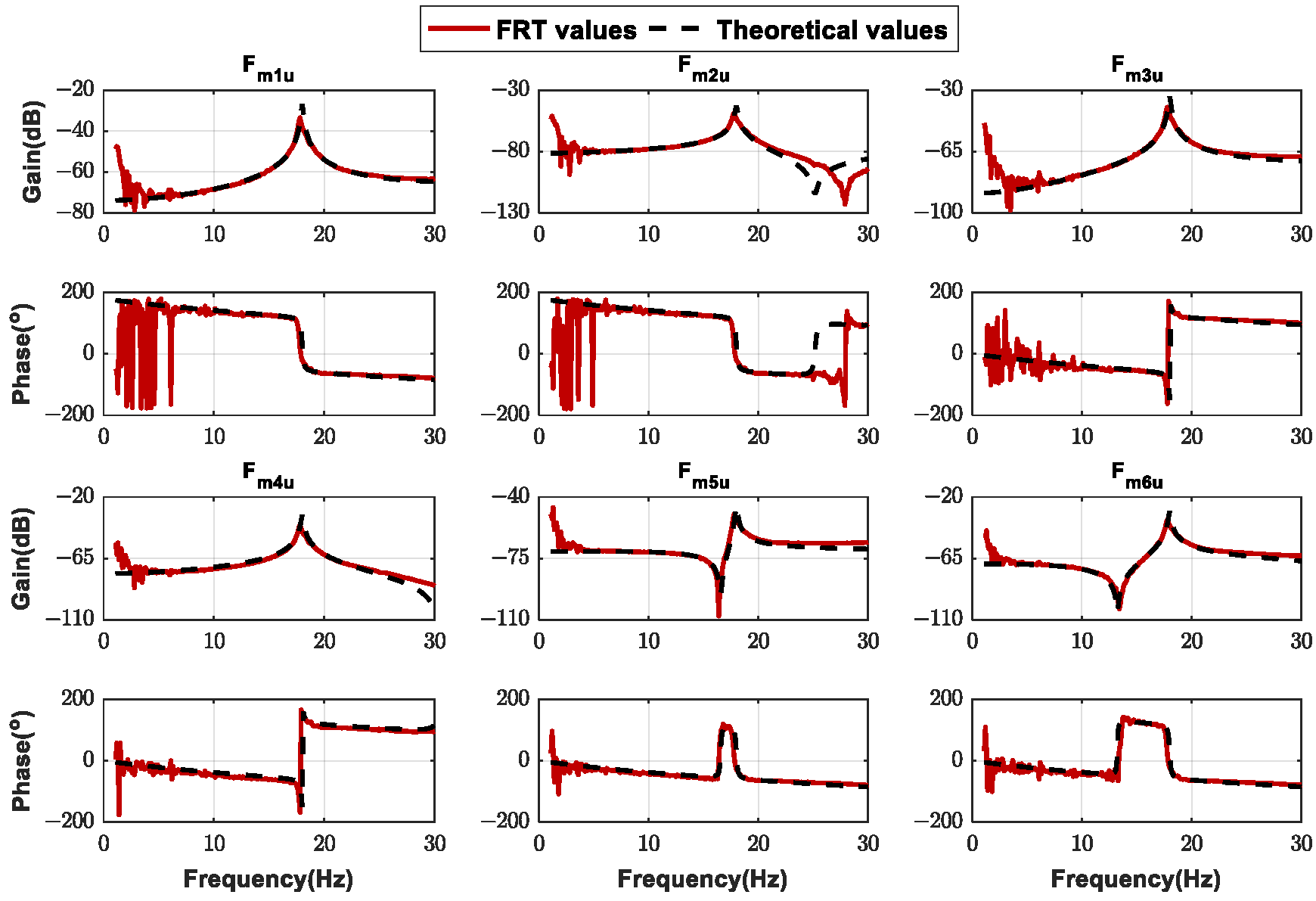
3.2. FRFs Acquired from the FRT
3.2.1. Shaker Excitation
3.2.2. Fin Excitation
3.3. FRFs of Unsteady Aerodynamics
3.3.1. Unsteady Aerodynamics of Elastic Vibration
3.3.2. Unsteady Aerodynamics of Fin Deflections
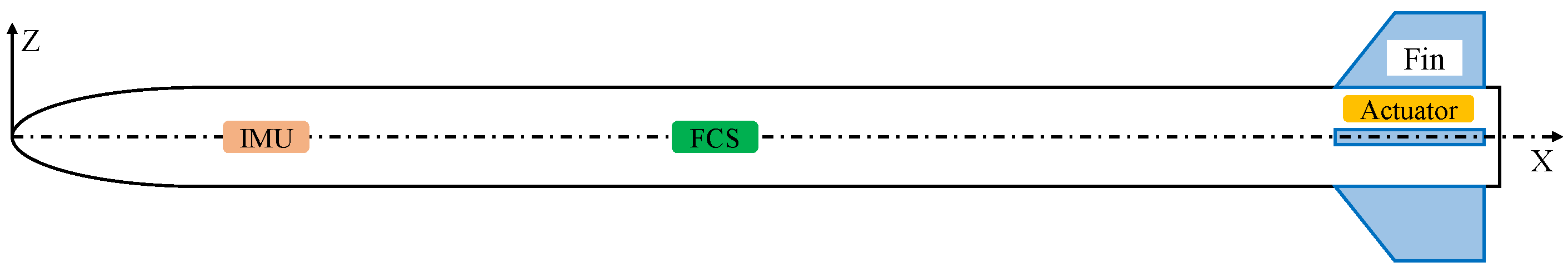
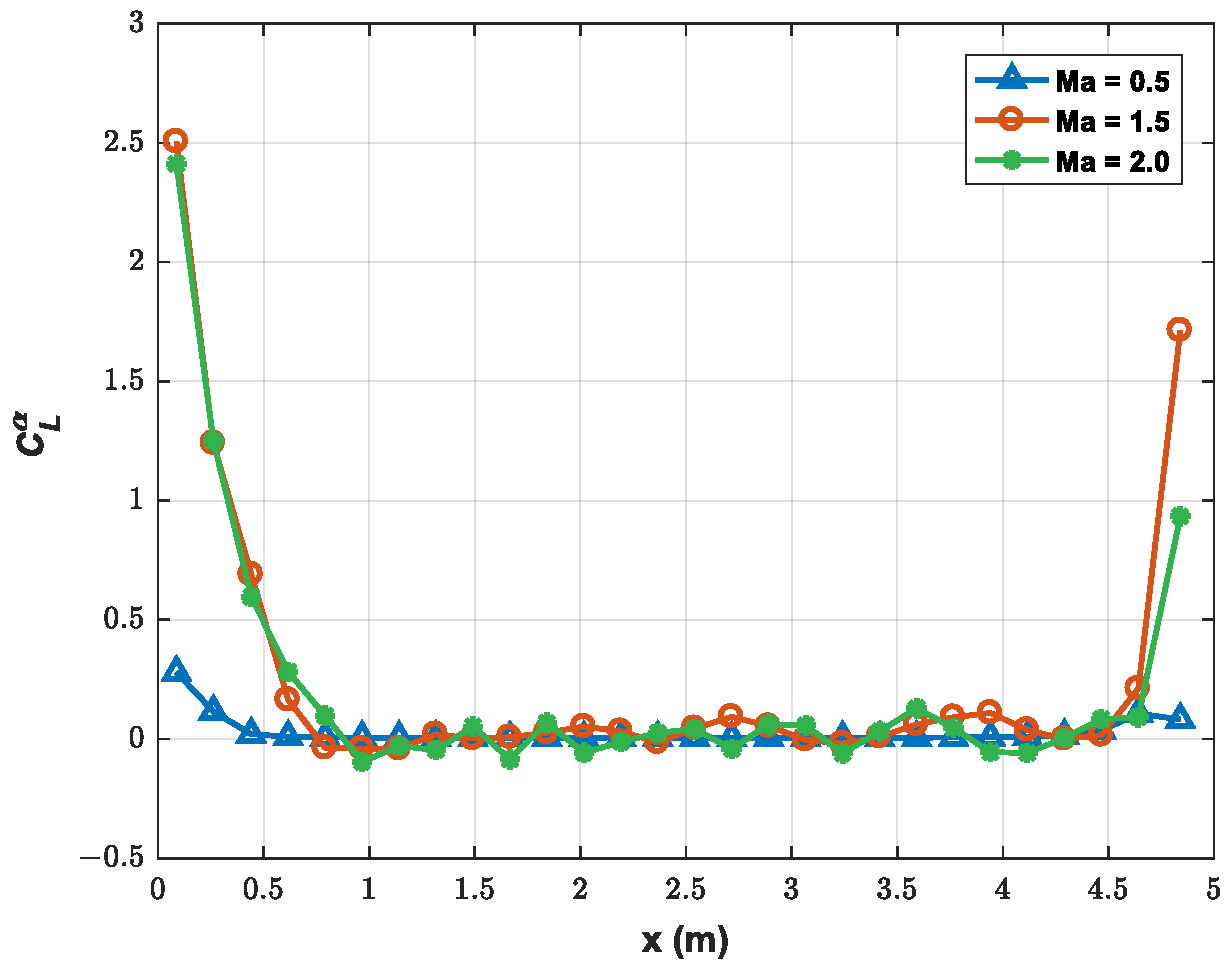
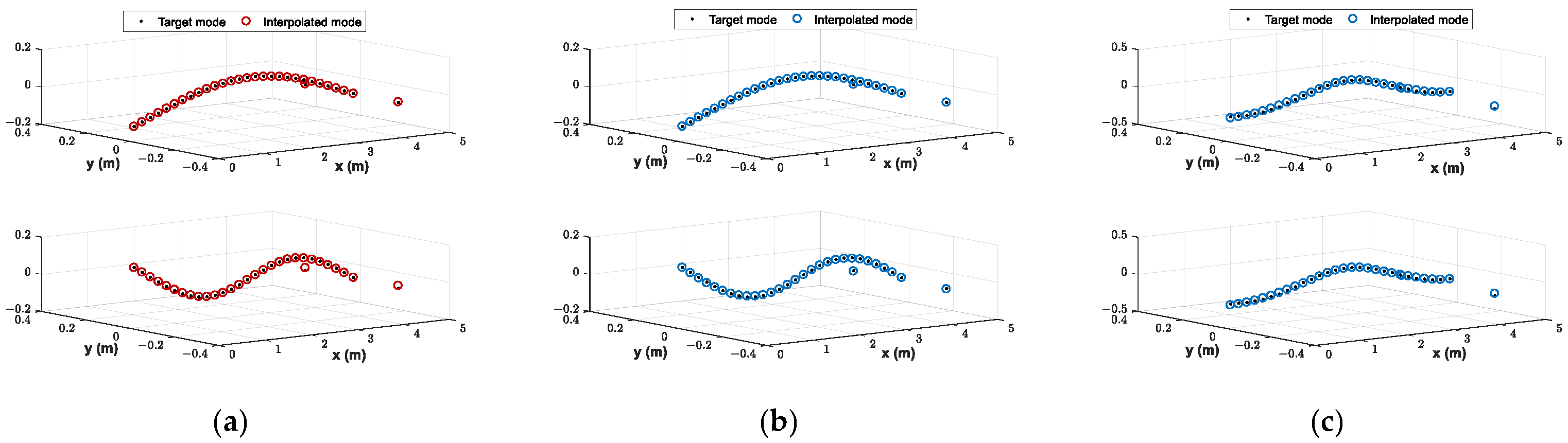
3.3.3. Placement of Excitation and Measurement Points
3.4. Stability Evaluation
4. Application of the Proposed Method
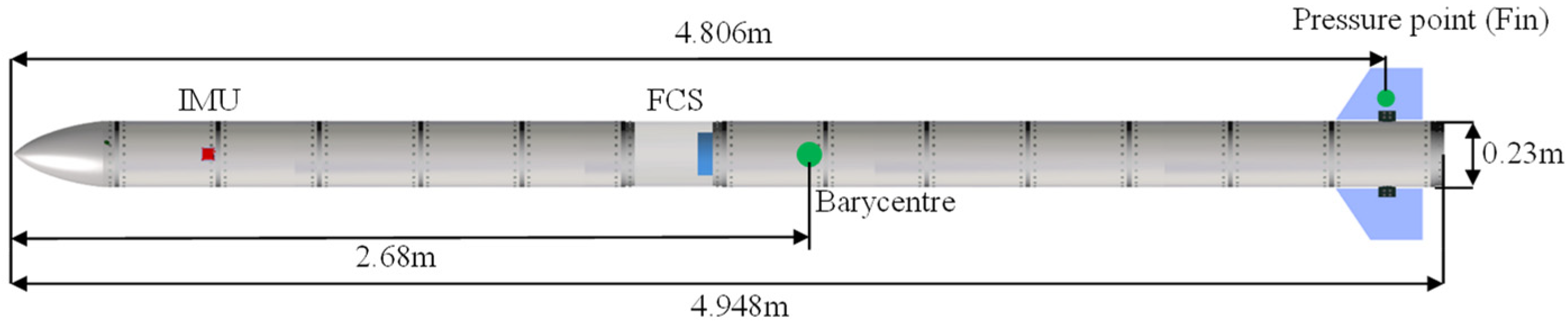
4.1. Description of the Slender Vehicle
4.1.1. Structural Parameters
4.1.2. Servo Control Systems
4.1.3. Aerodynamic Derivative
4.2. Model of Theoretical ASE
4.3. Locations of Excitation and Measurement Points
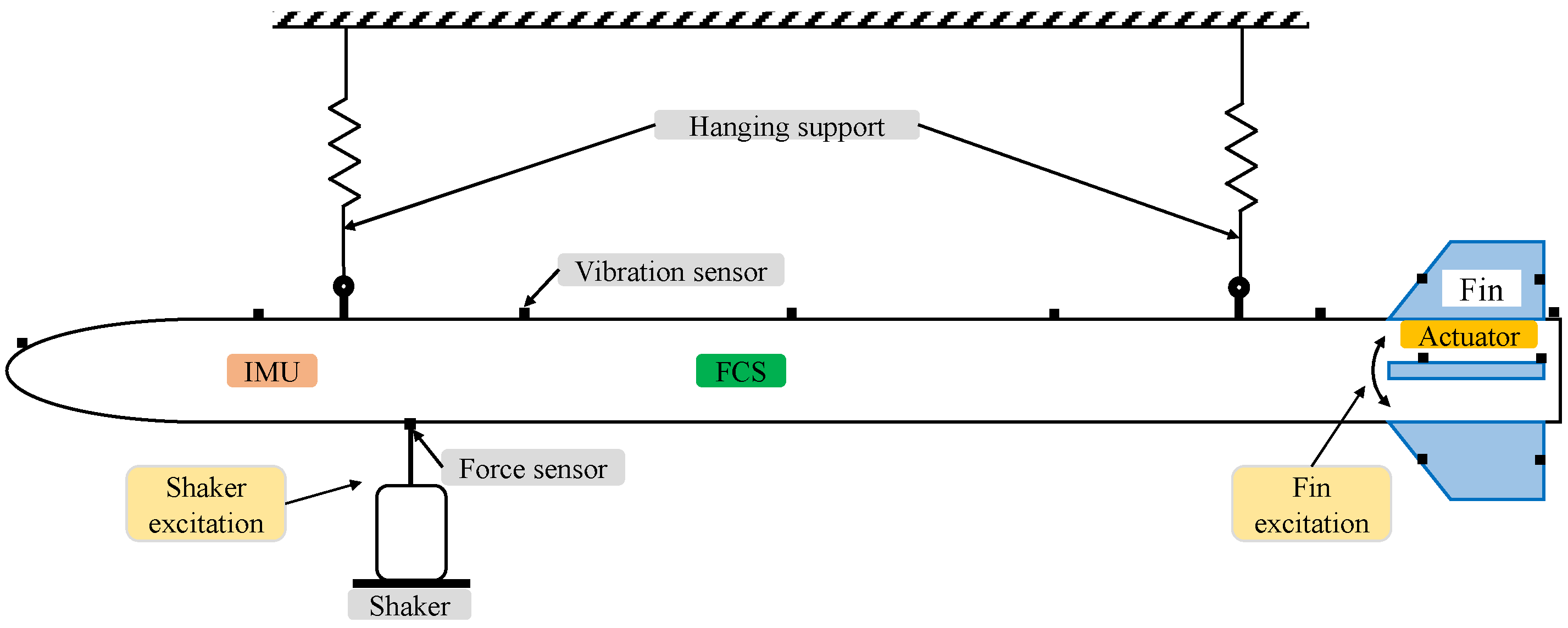
4.4. FRT Procedure
4.4.1. FRT Setup and Implementation
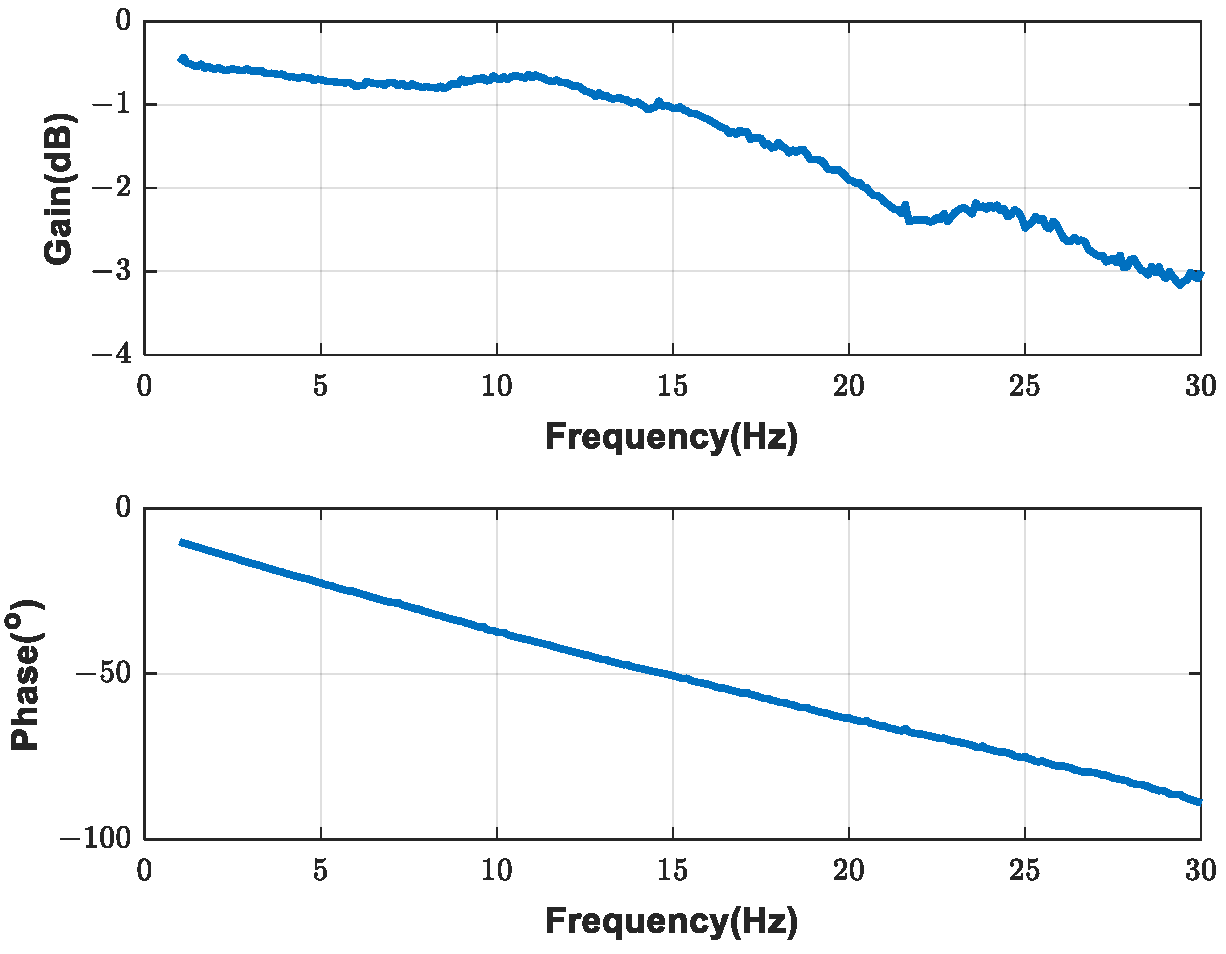
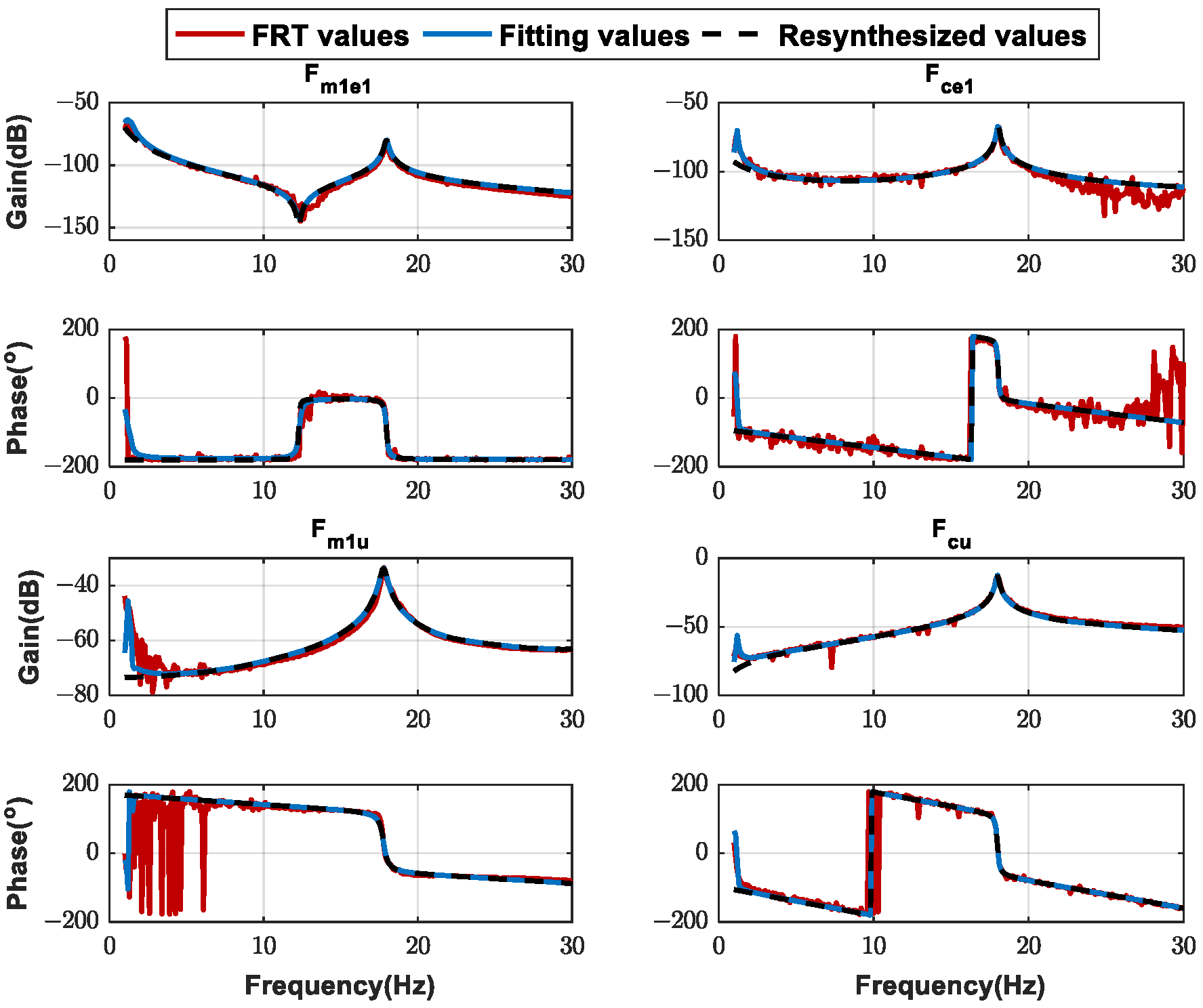
4.4.2. FRF Identification
5. Results and Discussion
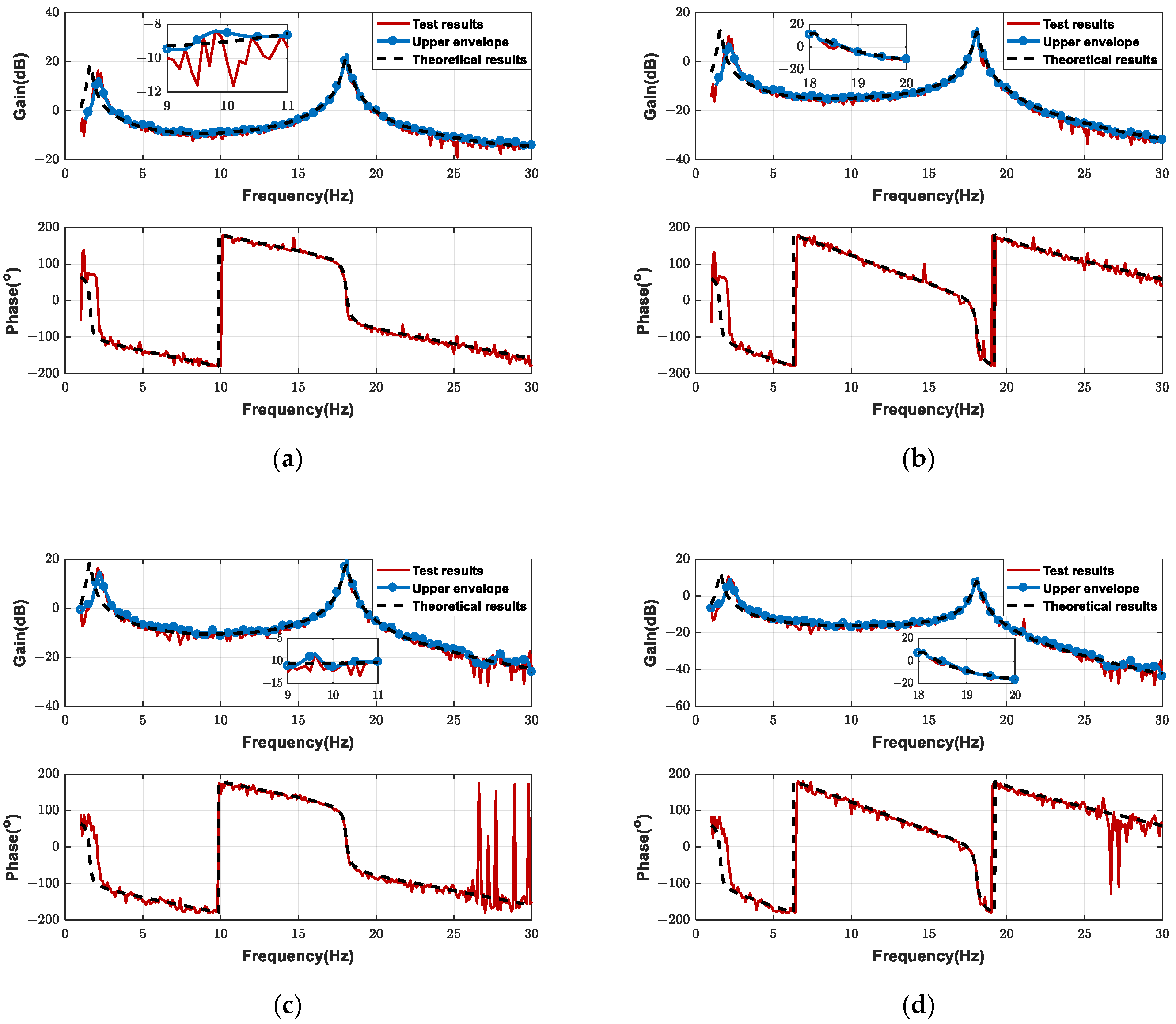
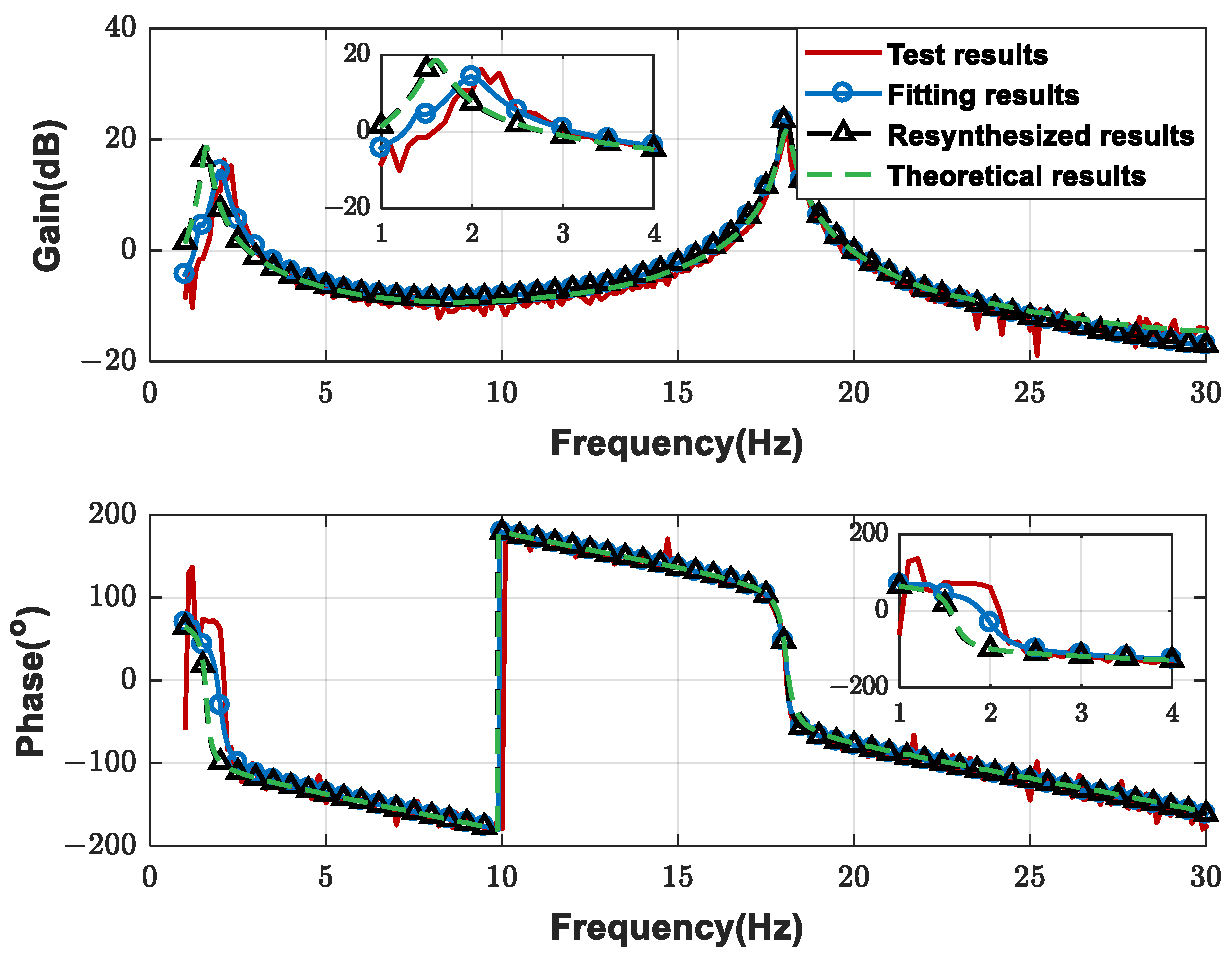
5.1. Stability Results of Single Group Test Data
5.2. Statistical Results of Stability Evaluation
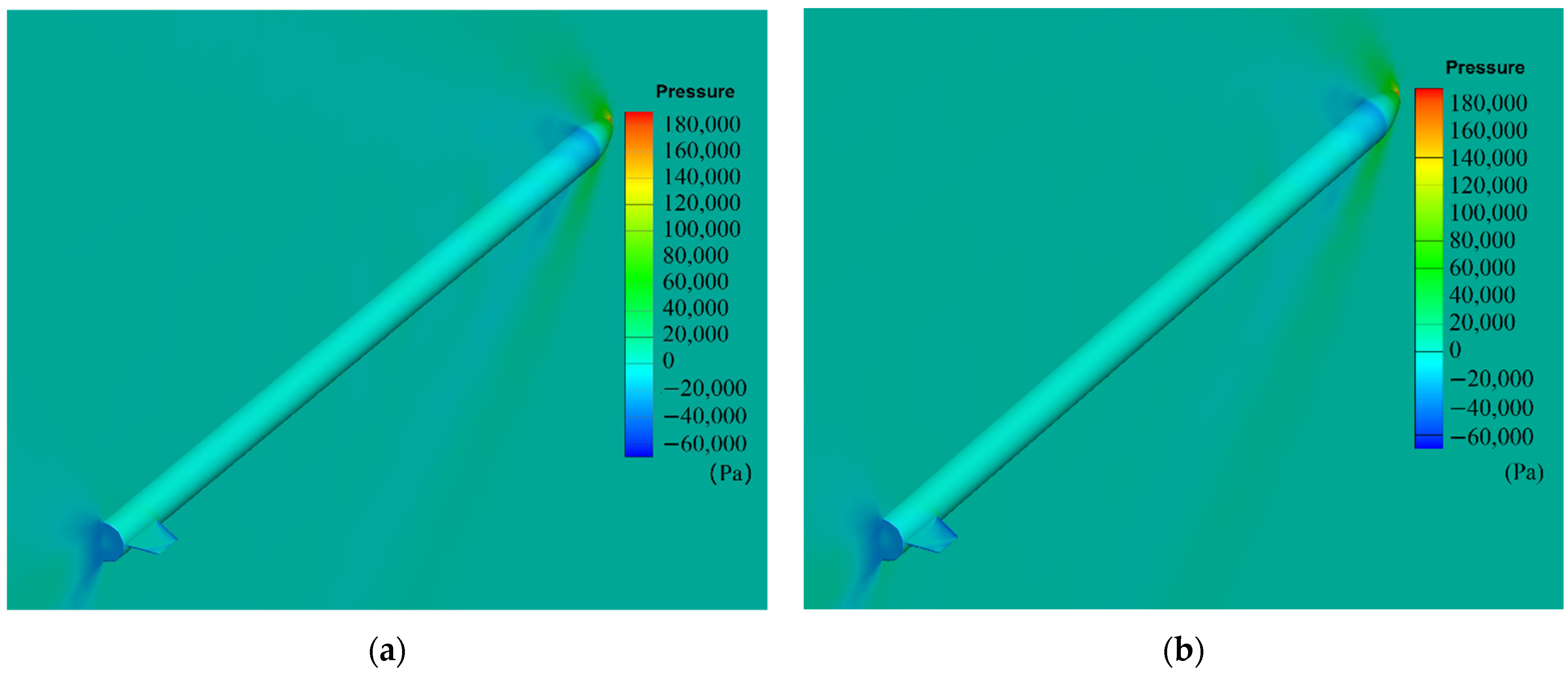
5.3. Removal of the Influence of Hanging Supports
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Felt, L.R.; Huttsell, L.J.; Noll, T.E.; Cooley, D.E. Aeroservoelastic encounters. J. Aircr. 1979, 16, 477–483. [Google Scholar] [CrossRef]
- Pak, C. Aeroservoelastic Stability Analysis of the X-43A Stack; Technical report, NASA-TM-2008-214635; NASA Dryden Flight Research Center: Edwards, CA, USA, 2008. [Google Scholar]
- Karpel, M. Procedures and models for aeroservoelastic analysis and design. Z. Angew. Math. Mech. 2001, 81, 579–592. [Google Scholar] [CrossRef]
- Botez, R.; Cotoi, I.; Doin, A.; Biskri, D. Method validation for aeroservoelastic analysis. In Proceedings of the 43rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Denver, CO, USA, 22–25 April 2002; p. 1481. [Google Scholar]
- Moulin, B. Modeling of aeroservoelastic systems with structural and aerodynamic variations. AIAA J. 2005, 43, 2503–2513. [Google Scholar] [CrossRef]
- Beal, T. Dynamic stability of a flexible missile under constant and pulsating thrusts. AIAA J. 1965, 3, 486–494. [Google Scholar] [CrossRef]
- Park, Y. Dynamic stability of a free Timoshenko beam under a controlled follower force. J. Sound Vib. 1987, 113, 407–415. [Google Scholar] [CrossRef]
- Gupta, K.K. Finite Element Multidisciplinary Analysis; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2003. [Google Scholar]
- Zhang, C.; Ye, Z.; Zhang, W. Aeroservoelastic Analysis for Supersonic and Hypersonic Missiles. In Proceedings of the 45th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2007; p. 1073. [Google Scholar]
- Verhaegen, A.; Żbikowski, R. Aeroservoelastic modelling and control of a slender anti-air missile for active damping of longitudinal bending vibrations. Aerosp. Sci. Technol. 2017, 66, 20–27. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Xie, C. Aeroservoelastic stability analysis for flexible aircraft based on a nonlinear coupled dynamic model. Chin. J. Aeronaut. 2018, 31, 2185–2198. [Google Scholar] [CrossRef]
- Dessena, G.; Ignatyev, D.I.; Whidborne, J.F.; Pontillo, A.; Zanotti Fragonara, L. Ground vibration testing of a flexible wing: A benchmark and case study. Aerospace 2022, 9, 438. [Google Scholar] [CrossRef]
- Zhang, R.; Wu, Z.; Yang, C. Ground Structural Coupling Testing and Data Processing Methods for Frequency Response Function Estimation. In Proceedings of the 54th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Boston, MA, USA, 8–11 April 2013; p. 1776. [Google Scholar]
- Allen, M.; Pollock, S. AFTI/F-16 aeroservoelastic analyses and ground test with a digital flight control system. In Proceedings of the 24th Structures, Structural Dynamics and Materials Conference, Lake Tahoe, NV, USA, 2–4 May 1983; p. 994. [Google Scholar]
- Zislin, A.; Laurie, E.; Wilkinson, K.; Goldstein, R. X-29 Aeroservoelastic Analysis and Ground Test Validation Procedures. In Proceedings of the Aircraft Design Systems and Operations Meeting, Colorado Springs, CO, USA, 14–16 October 1985; p. 3091. [Google Scholar]
- Vaccaro, V.; Caldwell, B.; Becker, J. Ground Structural Coupling Testing Model Updating in the Aeroservoelastic Qualification of a Combat Aircraft; Technical report; Alenia Aeronautica Turin (Italy) Aircraft Engineering: Rome, Italy, 2000. [Google Scholar]
- Zeng, J.; Kingsbury, D.; Ritz, E.; Chen, P.C.; Lee, D.H.; Mignolet, M. GVT-based ground flutter test without wind tunnel. In Proceedings of the 52nd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference 19th AIAA/ASME/AHS Adaptive Structures Conference, Denver, CO, USA, 4–7 April 2011; p. 1942. [Google Scholar]
- Kearns, J.P. Flutter Simulation; Technical report; Johns Hopkins University Applied Physics Laboratory: Laurel, MD, USA, 1962. [Google Scholar]
- Wu, Z.G.; Ma, C.J.; Yang, C. New approach to the ground flutter simulation test. J. Aircr. 2016, 53, 1578–1580. [Google Scholar] [CrossRef]
- Wu, Z.G.; Zhang, R.J.; Ma, C.J.; Yang, C. Aeroelastic semiphysical simulation and wind-tunnel testing validation of a fin–actuator system. J. Aircr. 2017, 54, 235–245. [Google Scholar] [CrossRef]
- Wang, B.W.; Fan, X.L. Ground flutter simulation test based on reduced order modeling of aerodynamics by CFD/CSD coupling method. Int. J. Appl. Mech. 2019, 11, 1950008. [Google Scholar] [CrossRef]
- Yun, J.M.; Han, J.H. Development of ground vibration test based flutter emulation technique. Aeronaut. J. 2020, 124, 1436–1461. [Google Scholar] [CrossRef]
- Wu, Z.; Chu, L.; Yuan, R.; Yang, C.; Tang, C. Studies on aeroservoelasticity semi-physical simulation test for missiles. Sci. China Technol. Sci. 2012, 55, 2482–2488. [Google Scholar] [CrossRef]
- Rodden, W.P.; Giesing, J.P.; Kalman, T.P. Refinement of the nonplanar aspects of the subsonic doublet-lattice lifting surface method. J. Aircr. 1972, 9, 69–73. [Google Scholar] [CrossRef]
- Brock, B.; Griffin, J. The supersonic doublet-lattice method-A comparison of two approaches. In Proceedings of the 16th Structural Dynamics, and Materials Conference, Denver, CO, USA, 27–29 May 1975; p. 760. [Google Scholar]
- Zhang, W.W.; Ye, Z.Y.; Zhang, C.A. Supersonic flutter analysis based on a local piston theory. AIAA J. 2009, 47, 2321–2328. [Google Scholar] [CrossRef]
- Schmidt, D.K.; Raney, D.L. Modeling and simulation of flexible flight vehicles. J. Guid. Control. Dyn. 2001, 24, 539–546. [Google Scholar] [CrossRef]
- ZONA Technology. ZAERO Theoretical Manual Version 8.2; ZONA Technology: Scottsdale, AZ, USA, 2011. [Google Scholar]
- Harder, R.L.; Desmarais, R.N. Interpolation using surface splines. J. Aircr. 1972, 9, 189–191. [Google Scholar] [CrossRef]
- Duchon, J. Splines minimizing rotation-invariant semi-norms in Sobolev spaces. In Constructive Theory of Functions of Several Variables; Schempp, W., Zeller, K., Eds.; Springer: Berlin/Heidelberg, Germany, 1977; pp. 85–100. [Google Scholar]
- Xu, Y.T.; Wu, Z.G.; Yang, C. Simulation of the Unsteady Aerodynamic Forces for Ground Flutter Simulation Test. Acta Aeronaut. Astronaut. Sin. 2012, 33, 1947–1957. [Google Scholar]
- Wright, J.R.; Cooper, J.E. Introduction to Aircraft Aeroelasticity and Loads; John Wiley and Sons: Hoboken, NJ, USA, 2008; Volume 20, pp. 105–115. [Google Scholar]
- LMS. The LMS Test.Lab Modal Analysis Manual; LMS Test.Lab rev 9A; LMS International: Laredo, TX, USA, 2008. [Google Scholar]
- Schoukens, J.; Pintelon, R. Measurement of frequency response functions in noisy environments. IEEE Trans. Instrum. Meas. 1990, 39, 905–909. [Google Scholar] [CrossRef]










| Excitation Points (m) | Measurement Points (m) | |
|---|---|---|
| Body | 0.358, 1.758, 3.508, 4.806 | 0.05, 1.058, 2.108, 3.158, 4.208, 4.876 |
| Fin | (4.806, 0.0, 0.0) | (4.656, −0.21, 0.0), (4.876, −0.21, 0.0) |
| State | Mean Values of Test | Theoretical Results | ||
|---|---|---|---|---|
| Gain Margin (dB) | Phase Crossover Frequency (Hz) | Gain Margin (dB) | Phase Crossover Frequency (Hz) | |
| U1C1 | 8.72 | 9.89 | 9.04 | 9.88 |
| U1C2 | 5.55 | 19.12 | 5.93 | 19.21 |
| U2C1 | 10.37 | 9.91 | 10.49 | 9.88 |
| U2C2 | 10.44 | 19.22 | 10.61 | 19.23 |
| Ma | Mean Values of Test | Theoretical Results | ||
|---|---|---|---|---|
| Gain Margin (dB) | Phase Crossover Frequency (Hz) | Gain Margin (dB) | Phase Crossover Frequency (Hz) | |
| 0.5 | 31.13 | 10.32 | 31.50 | 10.33 |
| 1.5 | 8.72 | 9.89 | 9.04 | 9.88 |
| 2.0 | 5.88 | 9.83 | 6.38 | 9.84 |
| Gain Margin (dB) | Phase Crossover Frequency (Hz) | Phase Margin (°) | Gain Crossover Frequency (Hz) | |
|---|---|---|---|---|
| Test results | 8.50 | 10.01 | 71.19 | 2.99 |
| Fitting results | 8.21 | 9.92 | 65.42 | 3.13 |
| Resynthesized results | 8.30 | 9.86 | 64.84 | 2.78 |
| Theoretical results | 9.04 | 9.88 | 64.69 | 2.80 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, C.; Wu, Z.; Yang, C. Aeroservoelastic Stability Evaluation for Slender Vehicles Based on the Ground Frequency Response Test. Aerospace 2022, 9, 850. https://doi.org/10.3390/aerospace9120850
Yu C, Wu Z, Yang C. Aeroservoelastic Stability Evaluation for Slender Vehicles Based on the Ground Frequency Response Test. Aerospace. 2022; 9(12):850. https://doi.org/10.3390/aerospace9120850
Chicago/Turabian StyleYu, Changkun, Zhigang Wu, and Chao Yang. 2022. "Aeroservoelastic Stability Evaluation for Slender Vehicles Based on the Ground Frequency Response Test" Aerospace 9, no. 12: 850. https://doi.org/10.3390/aerospace9120850
APA StyleYu, C., Wu, Z., & Yang, C. (2022). Aeroservoelastic Stability Evaluation for Slender Vehicles Based on the Ground Frequency Response Test. Aerospace, 9(12), 850. https://doi.org/10.3390/aerospace9120850






