Description of a Eulerian–Lagrangian Approach for the Modeling of Cooling Water Droplets
Abstract
:1. Introduction
2. Governing Equations
Cooling Process
3. Numerical Algorithm
3.1. Coupling of Continuous and Dispersed Phases
| Algorithm 1: Iterative procedure for the two-way coupling of the dispersed and continuous phases. |
 |
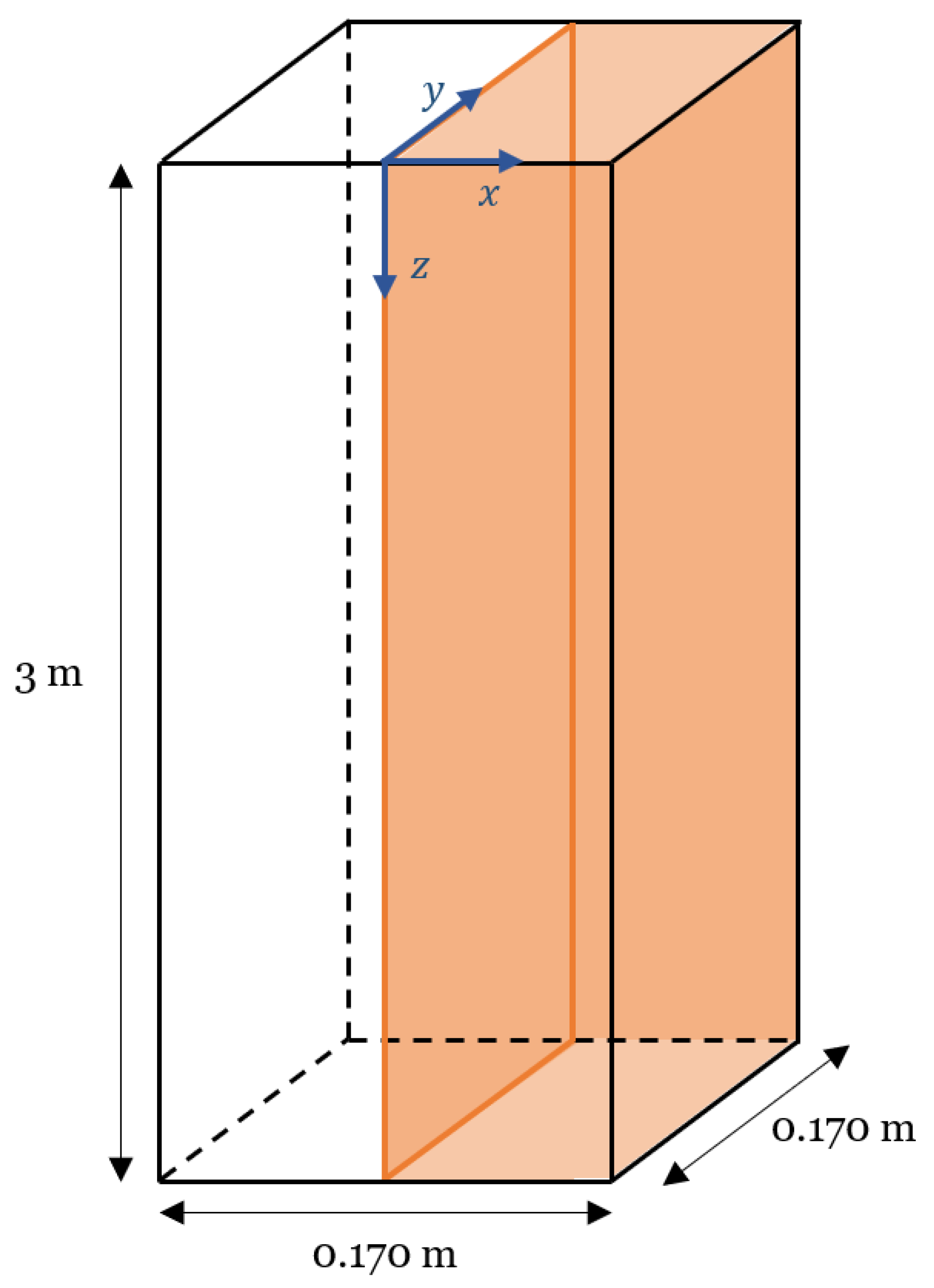
3.2. Initial and Boundary Conditions
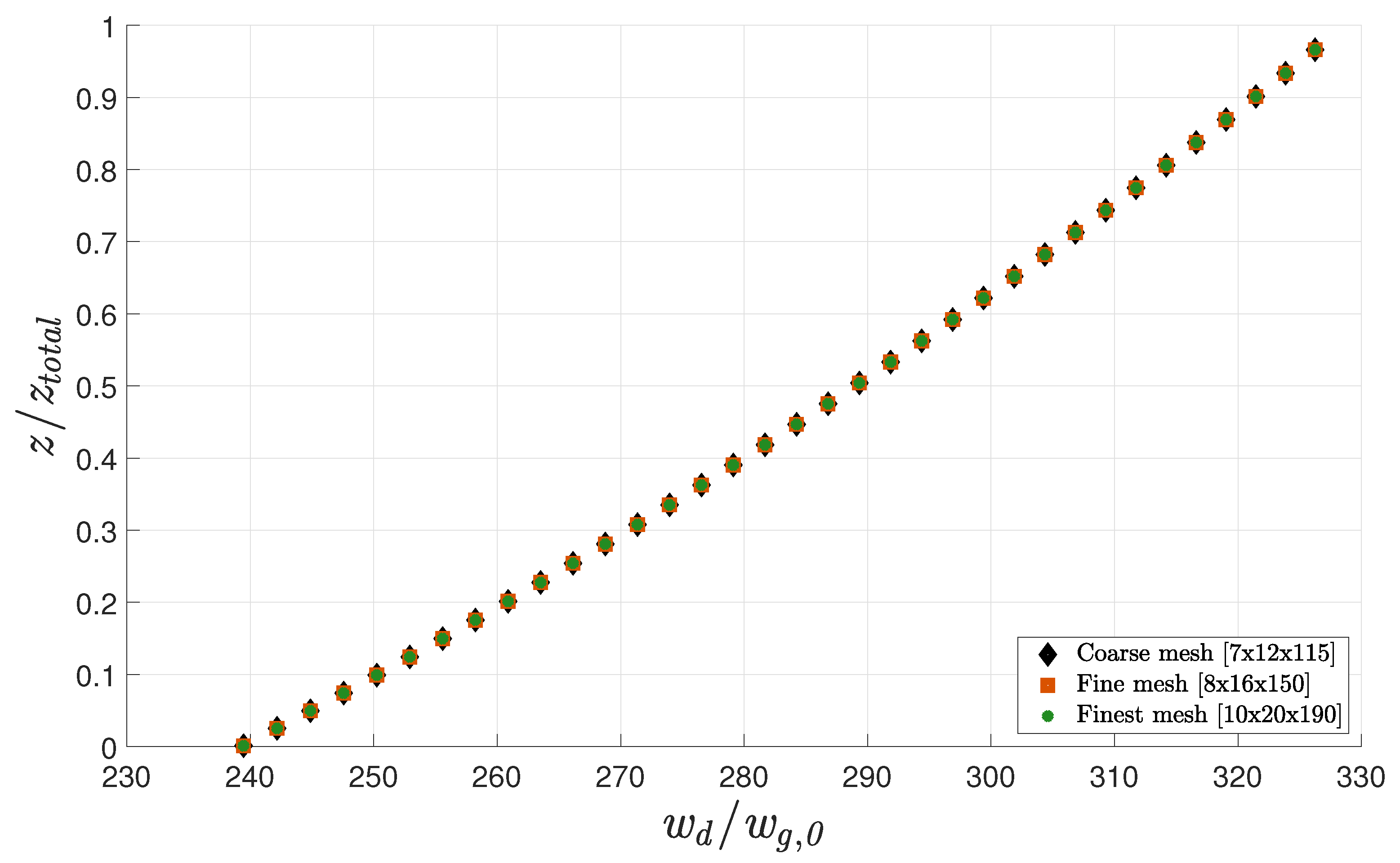
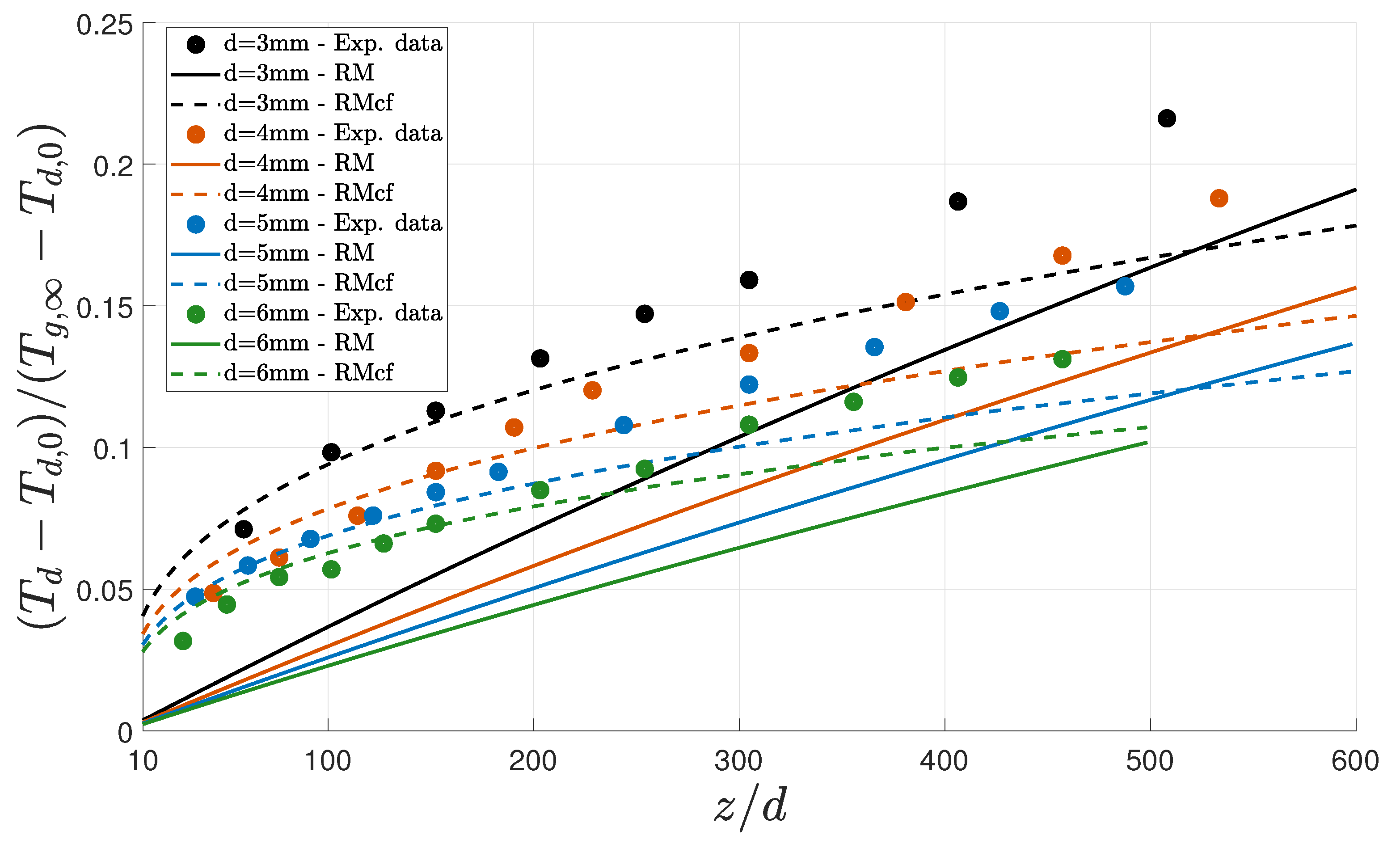
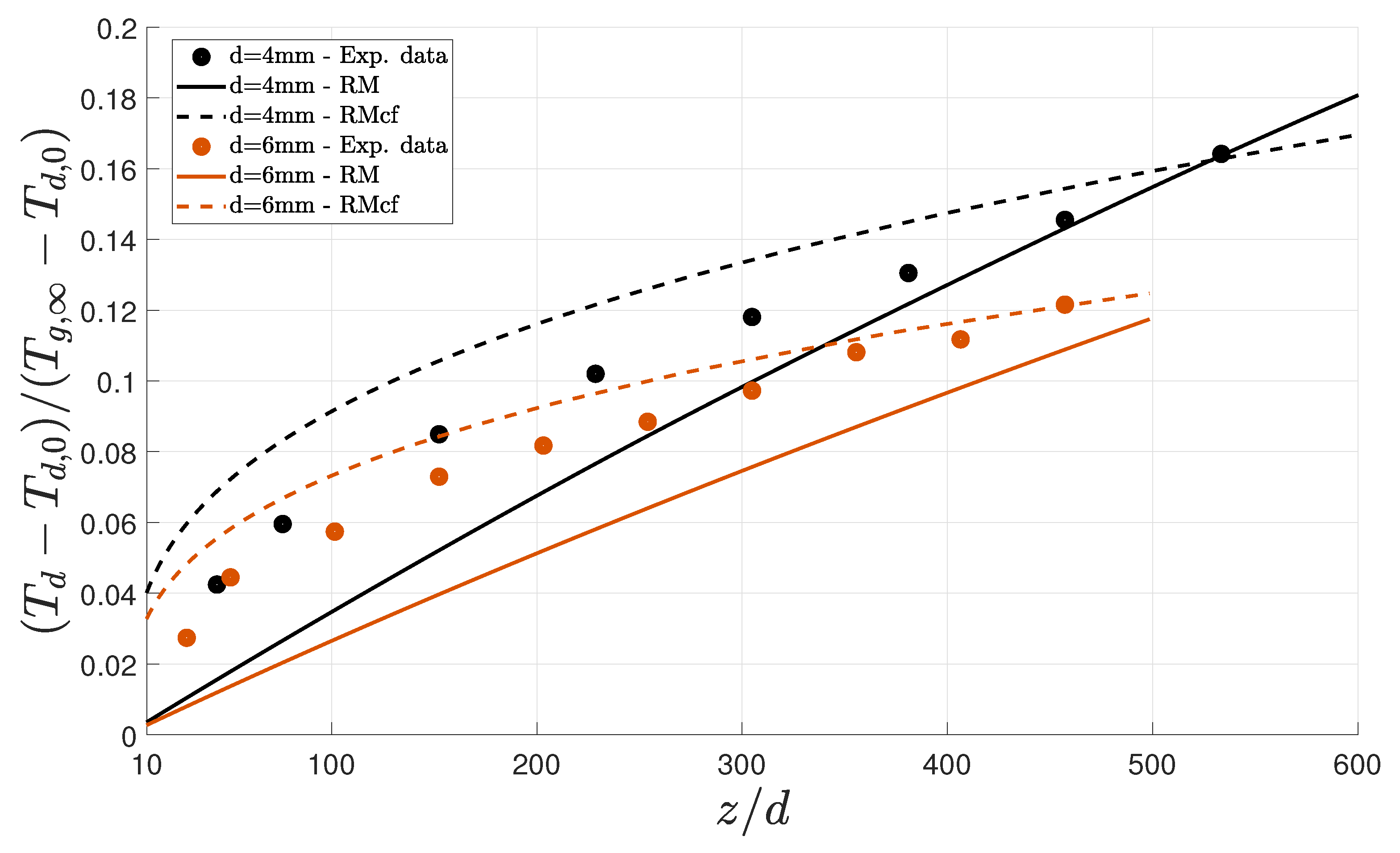
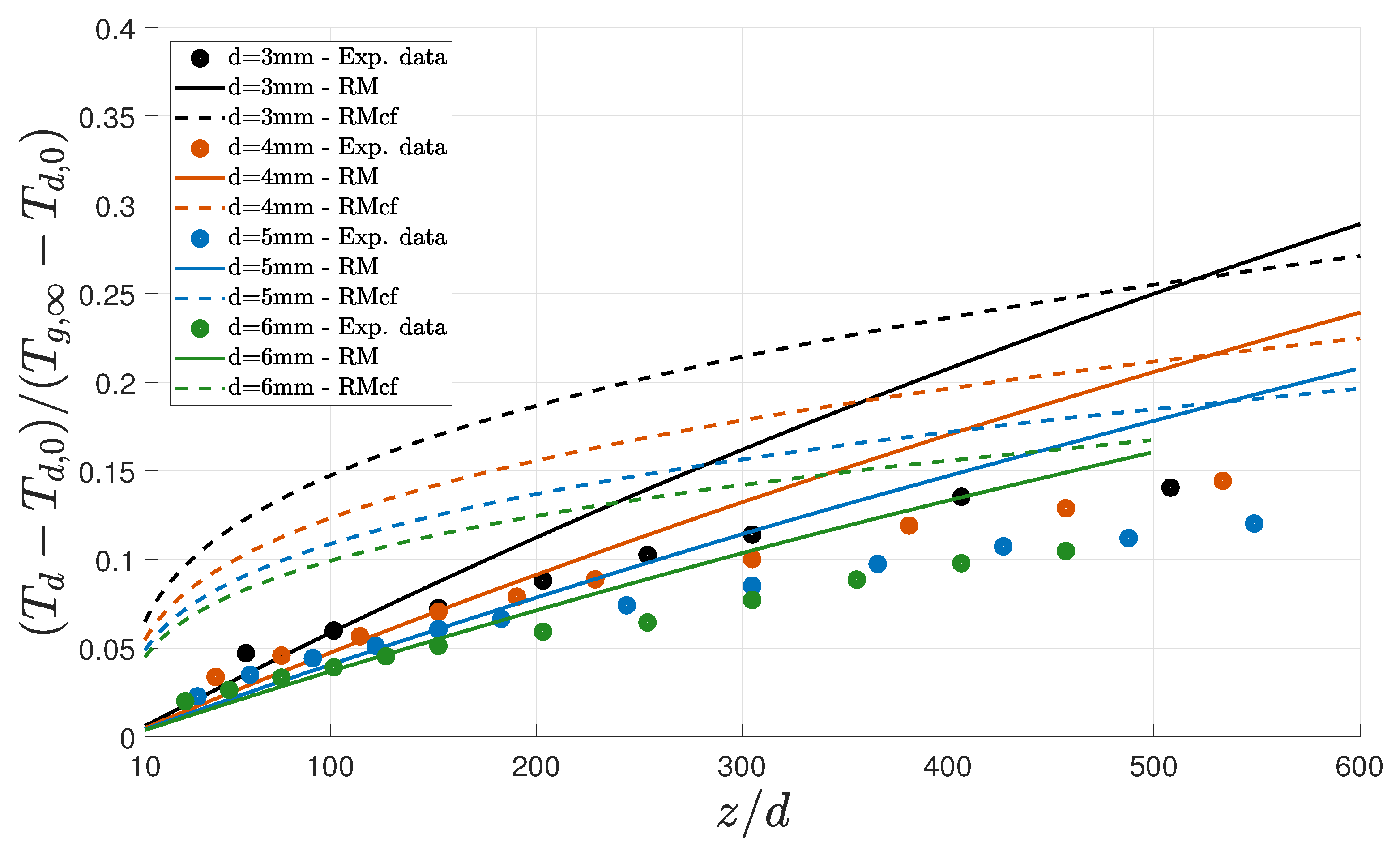
4. Numerical Tests and Validation
5. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DPM | Discrete Phase Model |
| FVM | Finite Volume Method |
| LHL | Locally Homogeneous Flow |
| NACA | National Advisory Committee for Aeronautics |
| NIST | National Institute of Standards |
| PDE | Partial Differential Equation |
| Probability Density Function | |
| QUICK | Quadratic Upstream Interpolation for Convective Kinematics |
| RANS | Reynolds-Averaged Navier–Stokes |
| RM | Ranz–Marshall Classical Correlation |
| RMcf | Ranz–Marshall Corrected Correlation |
| SIMPLE | Semi-Implicit Method for Pressure-Linked Equations |
| SF | Separated Flow |
| SSF | Stochastic Separated Flow |
| TDMA | Tridiagonal Matrix Algorithm |
| VoF | Volume of Fluid |
Appendix A. Thermodynamic Relations
Appendix B. Thermophysical Properties of Dry Air and Water
| Physical Property | Expression |
|---|---|
| Physical Property | Expression |
|---|---|
References
- Cao, Y.; Wu, Z.; Su, Y.; Xu, Z. Aircraft flight characteristics in icing conditions. Prog. Aerosp. Sci. 2015, 74, 62–80. [Google Scholar] [CrossRef]
- Cao, Y.; Tan, W.; Wu, Z. Aircraft icing: An ongoing threat to aviation safety. Aerosp. Sci. Technol. 2018, 75, 353–385. [Google Scholar] [CrossRef]
- Stebbins, S.J.; Loth, E.; Broeren, A.P.; Potapczuk, M. Review of computational methods for aerodynamic analysis of iced lifting surfaces. Prog. Aerosp. Sci. 2019, 111, 100583. [Google Scholar] [CrossRef]
- Khalil, E.E.; Sobhi, M. CFD Simulation of Thermal and Energy Performance for a Display Cabinet Refrigerator Containing a Phase Change Material (PCM). In Proceedings of the AIAA Propulsion and Energy 2020 Forum, Virtual, 24–28 August 2020. [Google Scholar] [CrossRef]
- Fakorede, O.; Feger, Z.; Ibrahim, H.; Ilinca, A.; Perron, J.; Masson, C. Ice protection systems for wind turbines in cold climate: Characteristics, comparisons and analysis. Renew. Sustain. Energy Rev. 2016, 65, 662–675. [Google Scholar] [CrossRef]
- Yancheshme, A.A.; Allahdini, A.; Maghsoudi, K.; Jafari, R.; Momen, G. Potential anti-icing applications of encapsulated phase change material-embedded coatings: A review. J. Energy Storage 2020, 31, 101638. [Google Scholar] [CrossRef]
- Shirolkar, J.S.; Coimbra, C.F.M.; McQuay, M.Q. Fundamental aspects of modeling turbulent particle dispersion in dilute flows. Prog. Energy Combust. Sci. 1996, 22, 363–399. [Google Scholar] [CrossRef]
- Gouesbet, G.; Berlemont, A. Eulerian and Lagrangian approaches for predicting the behaviour of discrete particles in turbulent flows. Prog. Energy Combust. Sci. 1999, 25, 133–159. [Google Scholar] [CrossRef]
- Patankar, N.A.; Joseph, D.D. Modeling and numerical simulation of particulate flows by the Eulerian–Lagrangian approach. Int. J. Multiph. Flow 2001, 27, 1659–1684. [Google Scholar] [CrossRef] [Green Version]
- Subramaniam, S. Lagrangian–Eulerian methods for multiphase flows. Prog. Energy Combust. Sci. 2013, 39, 215–245. [Google Scholar] [CrossRef] [Green Version]
- Uranai, S.; Fukudome, K.; Mamori, H.; Fukushima, N.; Yamamoto, M. Numerical simulation of the anti-icing performance of electric heaters for icing on the NACA 0012 airfoil. Aerospace 2020, 7, 123. [Google Scholar] [CrossRef]
- Messinger, B.L. Equilibrium temperature of an unheated icing surface as a function of air speed. J. Aeronaut. Sci. 1953, 20, 29–42. [Google Scholar] [CrossRef]
- Peng, K.; Xing, T.; Yi, L.; Yun, Y.H. Effects of turbulent dispersion on water droplet impingement based on statistics method. Int. J. Aeronaut. Space Sci. 2018, 19, 330–339. [Google Scholar] [CrossRef]
- Fatahian, H.; Salarian, H.; Eshagh Nimvari, M.; Khaleghinia, J. Effect of Gurney flap on flow separation and aerodynamic performance of an airfoil under rain and icing conditions. Acta Mech. Sin. 2020, 36, 659–677. [Google Scholar] [CrossRef]
- Lian, W.; Zhao, L.; Xuan, Y.; Shen, J. A modified spongy icing model considering the effect of droplets retention on the ice accretion process. Appl. Therm. Eng. 2018, 134, 54–61. [Google Scholar] [CrossRef]
- Mason, J.; Strapp, W.; Chow, P. The ice particle threat to engines in flight. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006. [Google Scholar]
- Bucknell, A.; McGilvray, M.; Gillespie, D.R.H.; Jones, G.; Collier, B. A thermodynamic model for ice crystal accretion in aircraft engines: EMM-C. Int. J. Heat Mass Transf. 2021, 174, 121270. [Google Scholar] [CrossRef]
- Mason, J.G.; Chow, P.; Fuleki, D.M. Understanding Ice Crystal Accretion and Shedding Phenomenon in Jet Engines Using a Rig Test. J. Eng. Gas Turbines Power 2010, 133, 041201. [Google Scholar] [CrossRef]
- Al-Khalil, K.M.; Keith, T.G.; De Witt, K.J. Icing calculations on a typical commercial jet engine inlet nacelle. J. Aircr. 1997, 34, 87–93. [Google Scholar] [CrossRef]
- Zheng, M.; Guo, Z.; Dong, W.; Guo, X. Experimental investigation on ice accretion on a rotating aero-engine spinner with hydrophobic coating. Int. J. Heat Mass Transf. 2019, 136, 404–414. [Google Scholar] [CrossRef]
- Norde, E.; Senoner, J.M.; van der Weide, E.T.A.; Trontin, P.; Hoeijmakers, H.W.M.; Villedieu, P. Eulerian and Lagrangian ice-crystal trajectory simulations in a generic turbofan compressor. J. Propuls. Power 2019, 35, 26–40. [Google Scholar] [CrossRef]
- Feng, Z.G.; Michaelides, E.E. The immersed boundary-lattice Boltzmann method for solving fluid–particles interaction problems. J. Comput. Phys. 2004, 195, 602–628. [Google Scholar] [CrossRef]
- Ireland, P.J.; Desjardins, O. Improving particle drag predictions in Euler–Lagrange simulations with two-way coupling. J. Comput. Phys. 2017, 338, 405–430. [Google Scholar] [CrossRef] [Green Version]
- Ching, E.J.; Brill, S.R.; Barnhardt, M.; Ihme, M. A two-way coupled Euler-Lagrange method for simulating multiphase flows with discontinuous Galerkin schemes on arbitrary curved elements. J. Comput. Phys. 2020, 405, 109096. [Google Scholar] [CrossRef]
- Oefelin, J.; Yang, V. High pressure spray field dynamics in turbulent mixing layers. In Proceedings of the 31st Joint Propulsion Conference and Exhibit, AIAA. San Diego, CA, USA, 10–12 July 1995. [Google Scholar] [CrossRef]
- Gosman, A.D.; loannides, E. Aspects of computer simulation of liquid-fueled combustors. J. Energy 1983, 7, 482–490. [Google Scholar] [CrossRef]
- Kazemi, S.; Adib, M.; Amani, E. Numerical study of advanced dispersion models in particle-laden swirling flows. Int. J. Multiph. Flow 2018, 101, 167–185. [Google Scholar] [CrossRef]
- Faeth, G.M. Evaporation and combustion of sprays. Prog. Energy Combust. Sci. 1983, 9, 1–76. [Google Scholar] [CrossRef]
- Faeth, G.M. Mixing, transport and combustion in sprays. Prog. Energy Combust. Sci. 1987, 13, 293–345. [Google Scholar] [CrossRef] [Green Version]
- Sommerfeld, M. Analysis of isothermal and evaporating turbulent sprays by phase-Doppler anemometry and numerical calculations. Int. J. Heat Fluid Flow 1998, 19, 173–186. [Google Scholar] [CrossRef]
- Barata, J. Modelling of biofuel droplets dispersion and evaporation. Renew. Energy 2008, 33, 769–779. [Google Scholar] [CrossRef]
- Rodrigues, C.; Barata, J.; Silva, A. Modeling of evaporating sprays impinging onto solid surfaces. J. Thermophys. Heat Trans. 2017, 31, 109–119. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Methods Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Crowe, C.T.; Schwarzkopf, J.D.; Sommerfeld, M.; Tsuji, Y. Multiphase Flows with Droplets and Particles, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar] [CrossRef]
- Hindmarsh, J.P.; Russell, A.B.; Chen, X.D. Experimental and numerical analysis of the temperature transition of a suspended freezing water droplet. Int. J. Heat Mass Transf. 2003, 46, 1199–1213. [Google Scholar] [CrossRef]
- Strub, M.; Jabbour, O.; Strub, F.; Bédécarrats, J.P. Experimental study and modelling of the crystallization of a water droplet. Int. J. Refrig. 2003, 26, 59–68. [Google Scholar] [CrossRef]
- Tabakova, S.; Feuillebois, F.; Radev, S. Freezing of a suspended supercooled droplet with a heat transfer mixed condition on its outer surface. AIP Conf. Proc. 2009, 1186, 240–247. [Google Scholar] [CrossRef]
- Tanner, F.X. Droplet freezing and solidification. In Handbook of Atomization and Sprays: Theory and Applications; Ashgriz, N., Ed.; Springer: Boston, MA, USA, 2011. [Google Scholar] [CrossRef]
- Akhtar, S.; Xu, M.; Sasmito, A.P. Development and validation of a semi-analytical framework for droplet freezing with heterogeneous nucleation and non-linear interface kinetics. Int. J. Heat Mass Transf. 2020, 166, 120734. [Google Scholar] [CrossRef]
- Meng, Z.; Zhang, P. Dynamic propagation of ice-water phase front in a supercooled water droplet. Int. J. Heat Mass Transf. 2020, 152, 119468. [Google Scholar] [CrossRef]
- Myers, T.G.; Hennessy, M.G.; Calvo-Schwarzwälder, M. The Stefan problem with variable thermophysical properties and phase change temperature. Int. J. Heat Mass Transf. 2020, 149, 118975. [Google Scholar] [CrossRef] [Green Version]
- Ranz, W.E.; Marshall, W.R. Evaporation from drops: Part 1. Chem. Eng. Prog. 1952, 48, 141–146. [Google Scholar]
- Ranz, W.E.; Marshall, W.R. Evaporation from drops: Part 2. Chem. Eng. Prog. 1952, 48, 173–180. [Google Scholar]
- Yao, S.C.; Schrock, V.E. Heat and Mass Transfer From Freely Falling Drops. J. Heat Transf. 1976, 98, 120–126. [Google Scholar] [CrossRef]
- Leonard, B.P. A stable and accurate convective modelling procedure based on quadratic upstream interpolation. Comput. Methods Appl. Mech. Eng. 1979, 19, 59–98. [Google Scholar] [CrossRef]
- Patankar, S.V.; Spalding, D.B. A calculation procedure for heat, mass and momentum transfer in three-dimensional parabolic flows. Int. J. Heat Mass Transf. 1972, 15, 1787–1806. [Google Scholar] [CrossRef]
- Lefebvre, A.H.; McDonell, V.G. Atomization and Sprays, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar] [CrossRef]
- Eckert, E.R.G.; Drake, R.M. Heat and Mass Transfer, 1st ed.; McGraw-Hill Inc.: New York, NY, USA, 1959. [Google Scholar]
- McQuillan, F.J.; Culham, J.R.; Yovanovich, M.M. Properties of Dry Air at One Atmosphere; Microelectronics Heat Transfer Lab—University of Waterloo: Waterloo, ON, Canada, 1984. [Google Scholar]
- Lemmon, E.W.; McLinden, M.O.; Friend, D.G. Thermophysical Properties of Fluid Systems in NIST Chemistry WebBook, NIST Standard Reference Database Number 69; Checked on 8th March 2021; National Institute of Standards and Technology: Gaithersburg, MD, USA, 1997; p. 20899. [Google Scholar] [CrossRef]

| 1 | - | 0 | - | |
| T | 0 | |||
| 0 | 0 | 0 | ||
| 0 | 0 | |||
| k | ||||
| Droplet Diameter () | Relative Humidity Ratio (-) | Initial Droplet Temperature () | Environment Temperature () |
|---|---|---|---|
| 3 | |||
| 3 | |||
| 3 | |||
| 4 | |||
| 4 | |||
| 4 | |||
| 5 | |||
| 5 | |||
| 5 | |||
| 6 | |||
| 6 | |||
| 6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meireles, R.; Magalhães, L.; Silva, A.; Barata, J. Description of a Eulerian–Lagrangian Approach for the Modeling of Cooling Water Droplets. Aerospace 2021, 8, 270. https://doi.org/10.3390/aerospace8090270
Meireles R, Magalhães L, Silva A, Barata J. Description of a Eulerian–Lagrangian Approach for the Modeling of Cooling Water Droplets. Aerospace. 2021; 8(9):270. https://doi.org/10.3390/aerospace8090270
Chicago/Turabian StyleMeireles, Rúben, Leandro Magalhães, André Silva, and Jorge Barata. 2021. "Description of a Eulerian–Lagrangian Approach for the Modeling of Cooling Water Droplets" Aerospace 8, no. 9: 270. https://doi.org/10.3390/aerospace8090270
APA StyleMeireles, R., Magalhães, L., Silva, A., & Barata, J. (2021). Description of a Eulerian–Lagrangian Approach for the Modeling of Cooling Water Droplets. Aerospace, 8(9), 270. https://doi.org/10.3390/aerospace8090270









