Vibration Response Aspects of a Main Landing Gear Composite Door Designed for High-Speed Rotorcraft
Abstract
1. Introduction
1.1. Industrial Advance Scenario
1.2. Scope of the Research

2. Material and Methods
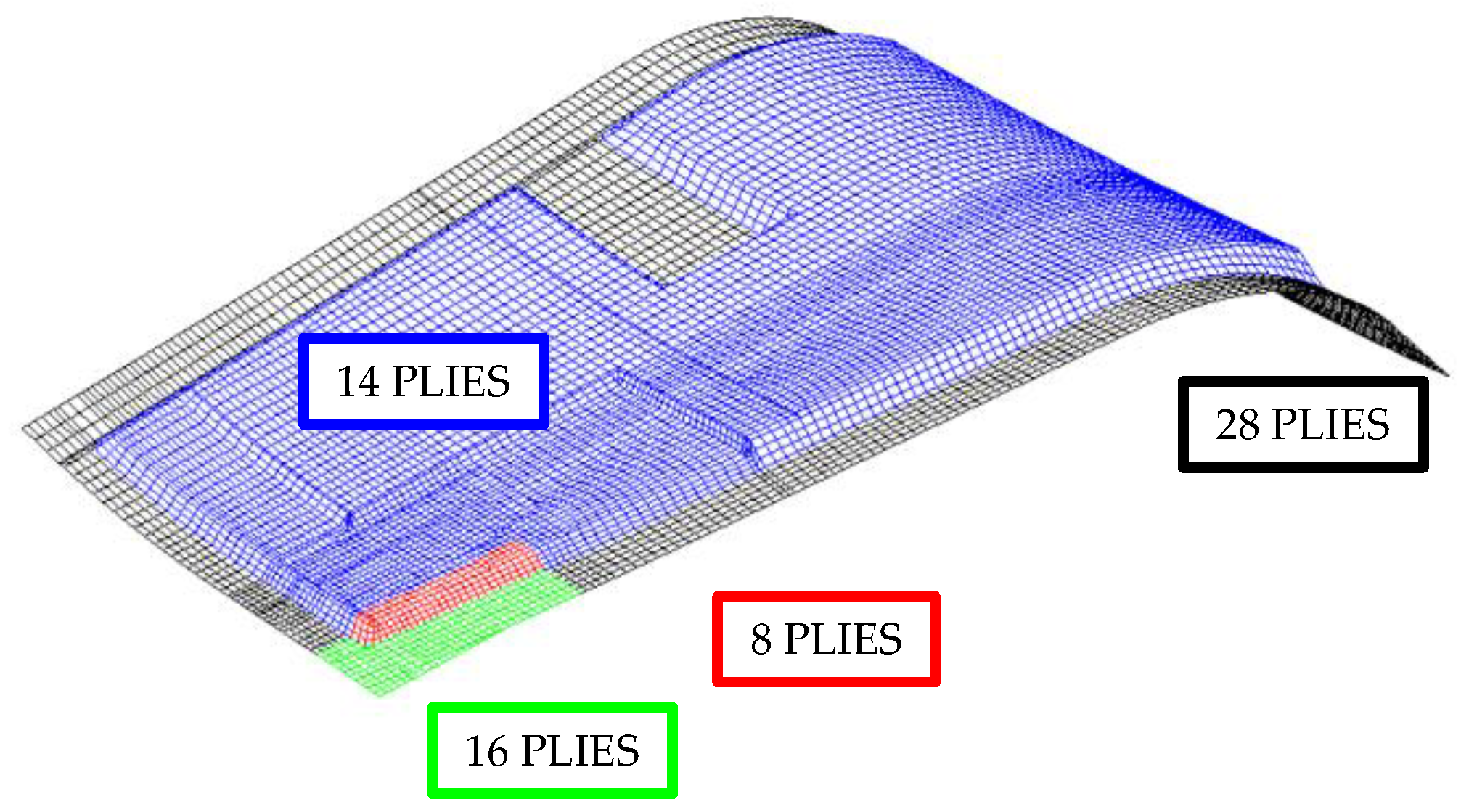
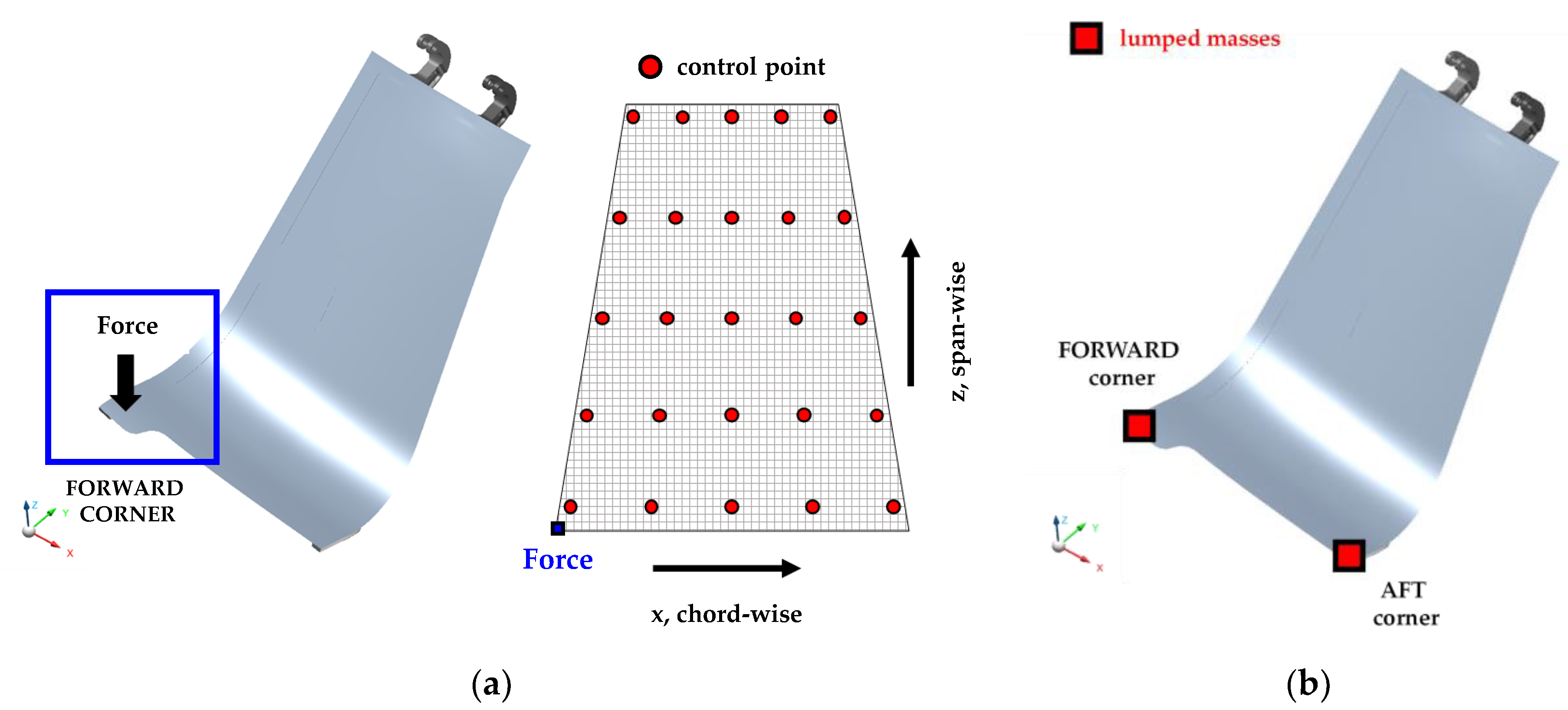
2.1. Architecture Overview
2.2. FE Model Description
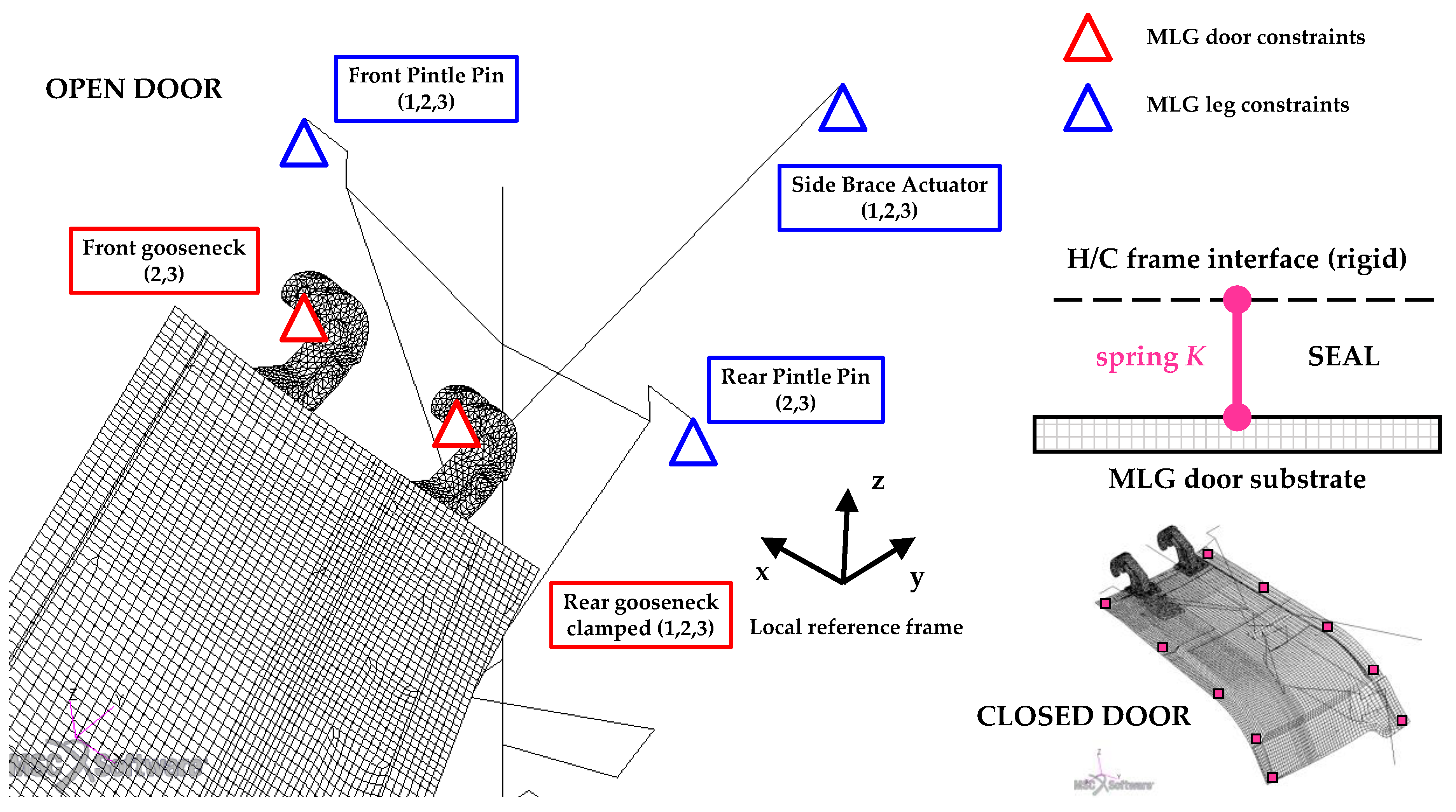
2.3. Boundary Conditions
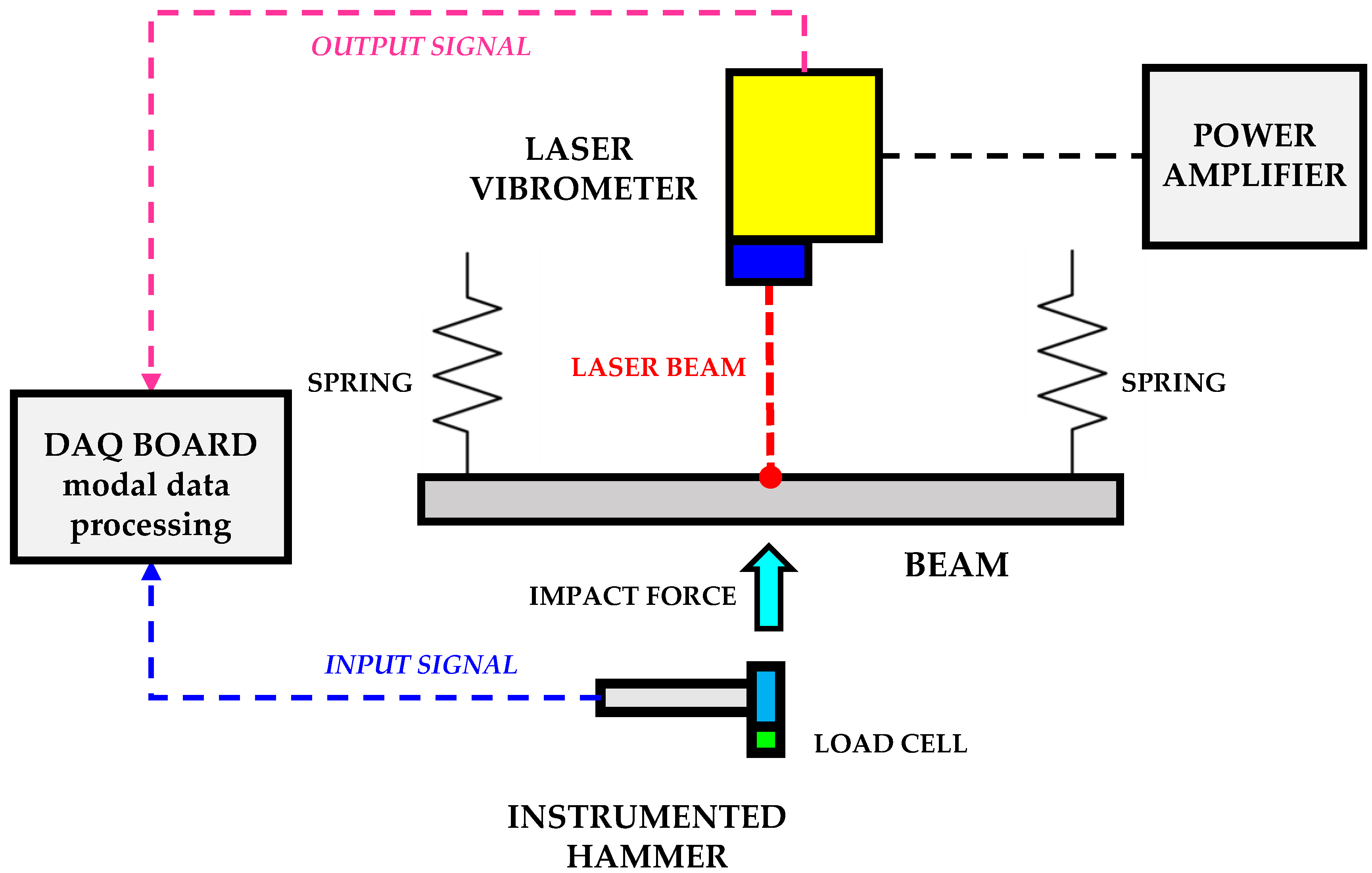
2.4. Damping Characterization of Metallic Fittings and Composite Parts
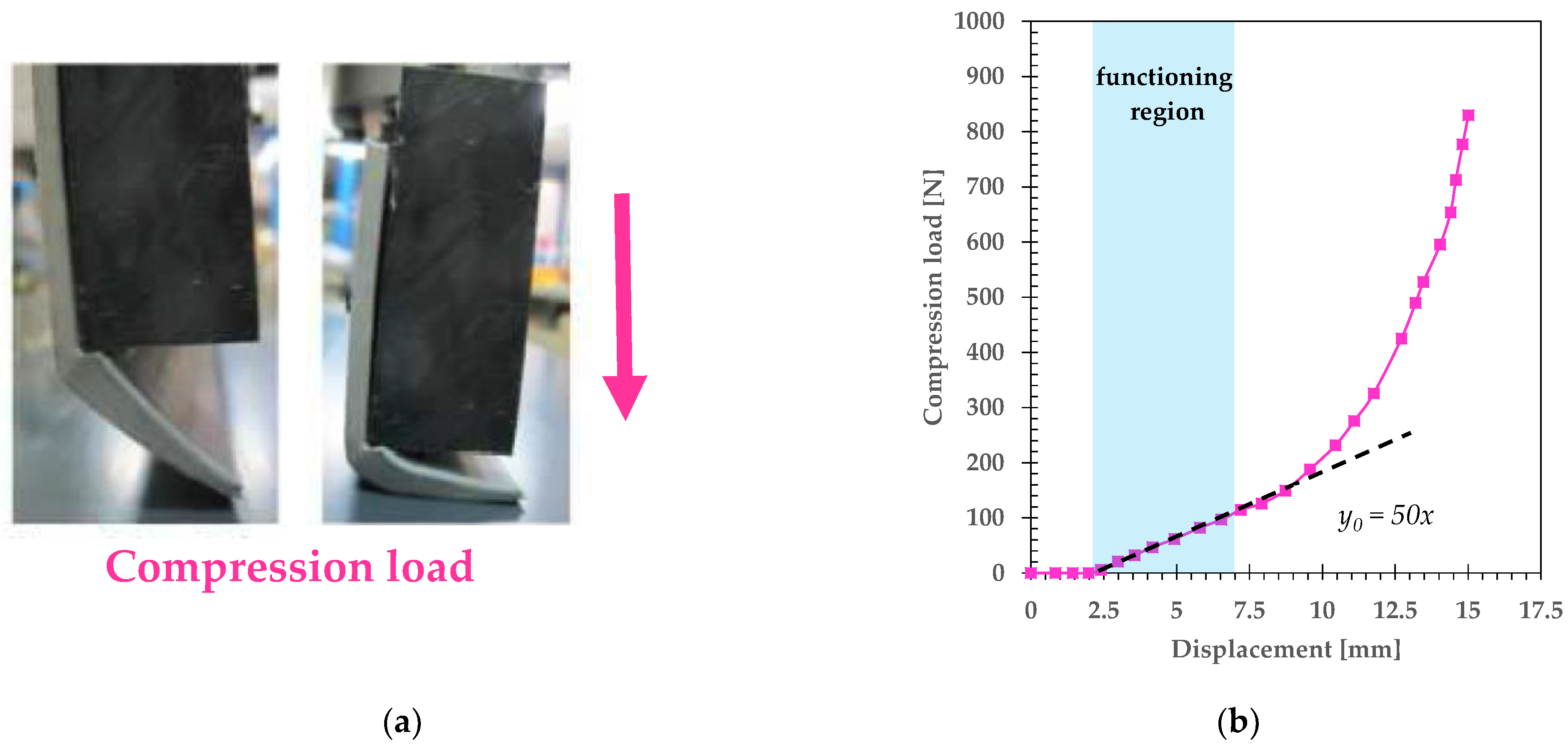
2.5. Gasket Stiffness Characterization
3. Results
3.1. Dynamic Constraints
3.2. MLG Door Baseline Configuration: Normal Modes Analysis
3.3. Spectral Analysis
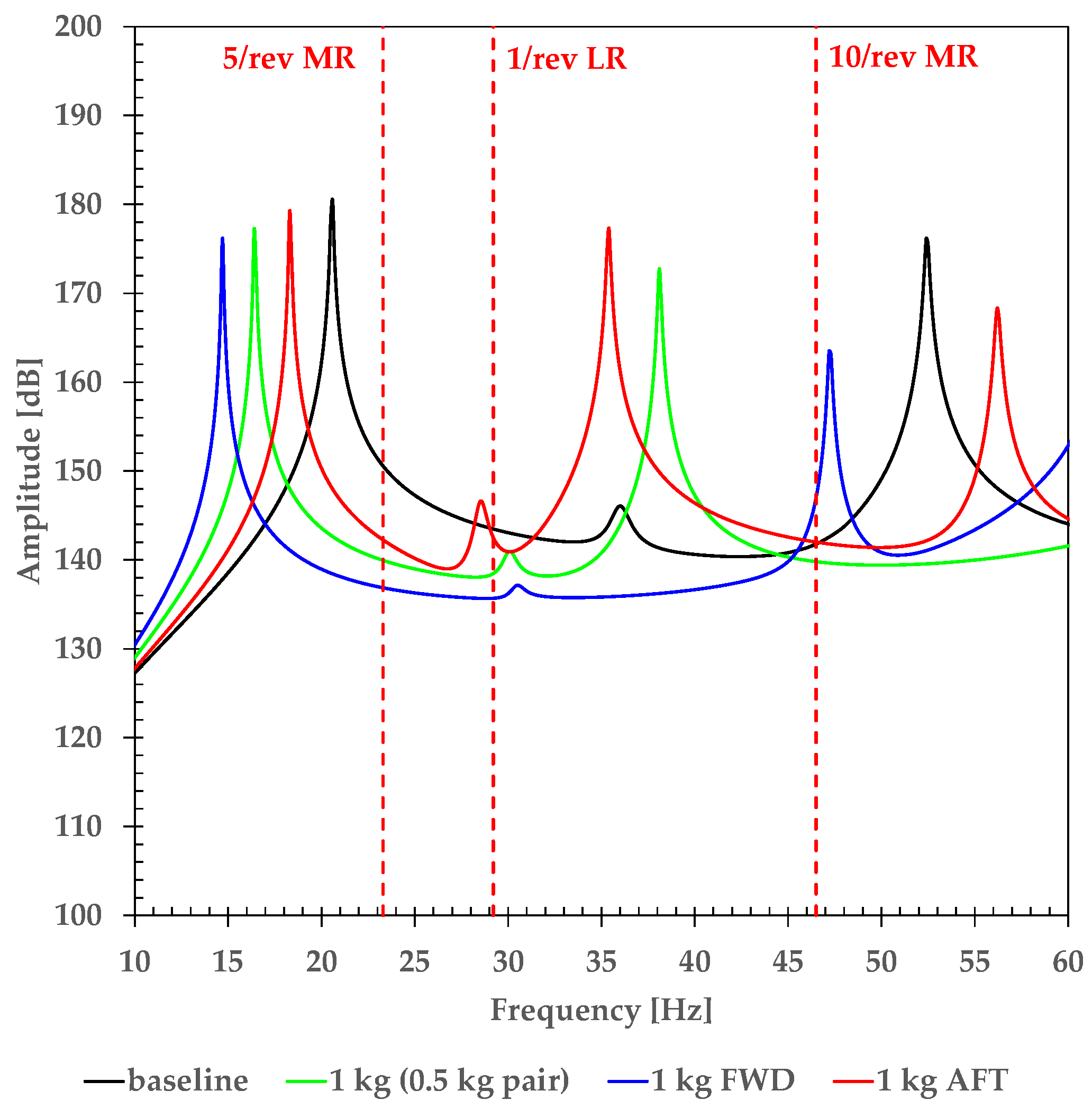
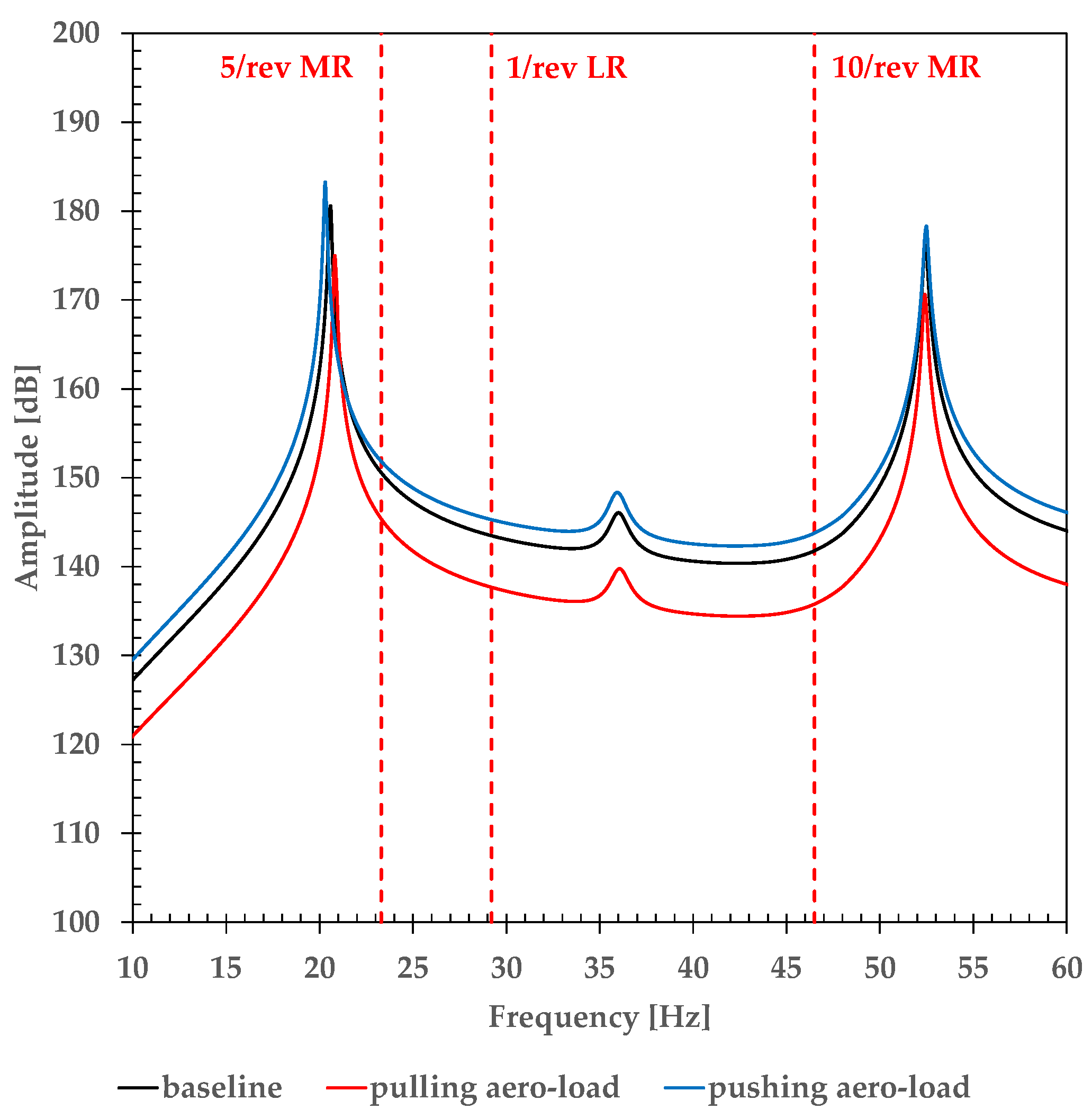
3.3.1. Nominal Case: Ballast Mass and Effect of Aerodynamic Action
3.3.2. Failure Case
- Hazard description: door uncontrolled dynamic motion;
- Potential impact: door moving undamped in airflow, which may cause structural damages to the H/C frame;
- Recovery action: Immediate speed reduction.
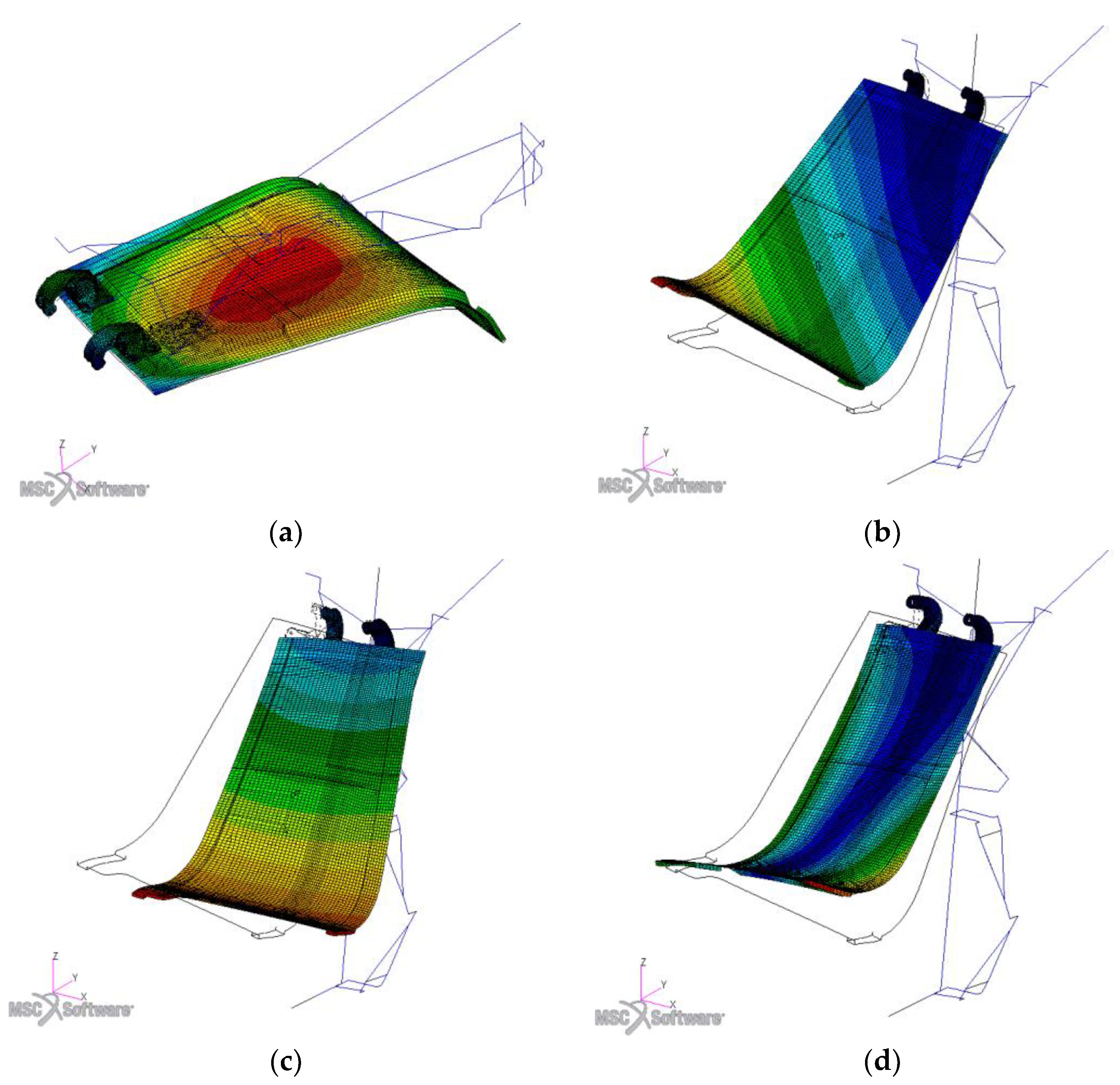
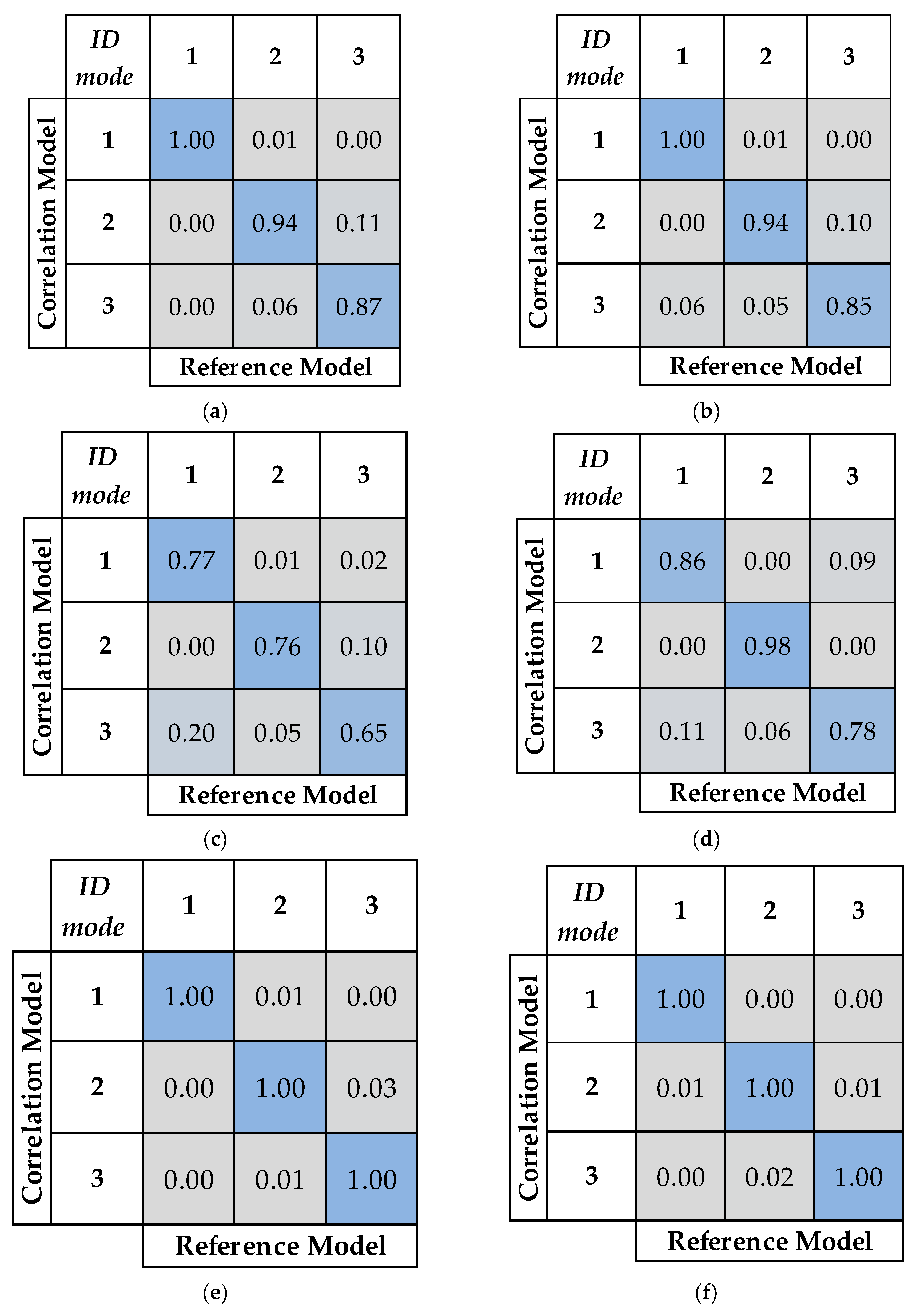
3.4. Mode Shapes Cross-Correlation
4. Conclusions and Next Developments
Author Contributions
Funding

Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zweben, C. Advanced composites for aerospace applications: A review of current status and future prospects. Composites 1981, 12, 235–240. [Google Scholar] [CrossRef]
- Harris, C.E.; Starnes, J.H.; Shuart, M.J. Design and Manufacturing of Aerospace Composite Structures, State-of-the-Art Assessment. J. Aircr. 2002, 39, 545–560. [Google Scholar] [CrossRef]
- Stone, R.H.; Harvill, W.E. Service experience of composite parts on the L-1011 and C-130. In Proceedings of the 9th National SAMPE Technical Conference, Atlanta, GA, USA, 4–6 October 1977. [Google Scholar]
- Hoffman, D.J.; Stoecklin, R.L. The 737 Graphite Composite Flight Spoiler Flight Service Evaluation; NASA Langley Technical Report Server; NASA Langley Research Center: Hampton, VA, USA, 1980. [Google Scholar]
- Cowles, A.; Forsch, H. Design, fabrication and test of an F-14 composite overwing fairing. In Proceedings of the Annual Conference SPI Reinforced Plastics/Composites Institute, Society of the Plastics Industry, SPI 30th Annual Conference, Washington, DC, USA, 4–7 February 1975. [Google Scholar]
- Dastin, S.J. Design and concepts of composite structures. In Proceedings of the Third International Conference on Composite Materials, Paris, France, 26–29 August 1980; pp. 46–68. [Google Scholar]
- Hadcock, R.N.; Dastin, S.J.; Erbacher, H.A. Design and fabrication of a mixed composite wing box. In Proceedings of the 5th SAMPE National Technical Conference, Kiamesha Lake, NY, USA, 9–11 October 1973. [Google Scholar]
- Baker, A.; Scott, M.L. (Eds.) Composite Materials for Aircraft Structures, 3th ed.; AIAA Education Series; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2016. [Google Scholar]
- Vosteen, L.F.; Hadcock, R.N. Composite Chronicles: A Study in the Lessons Learned in the Development, Production, and Service of Composite Structures; NASA Contractor Report 4620; NASA Langley Research Center: Hampton, VA, USA, 1994; p. 23. [Google Scholar]
- Shuart, M.J.; Johnston, N.J.; Dexter, H.B.; Marchello, J.M.; Grenoble, R.W. Automated Fabrication Technologies for High Performance Polymer Composites Composite Fabrication; NASA Langley Technical Report Server; NASA Langley Research Center: Hampton, VA, USA, 1998. [Google Scholar]
- Simsiriwong, J.; Warsi Sullivan, R. Experimental Vibration Analysis of a Composite UAV Wing. Mech. Adv. Mater. Struct. 2012, 19, 196–206. [Google Scholar] [CrossRef]
- Sullivan, R.; Rais-Rohani, M.; Lacy, T.; Alday, N. Structural testing of an ultralight UAV composite wing. In Proceedings of the 47th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Newport, RI, USA, 1–4 May 2006. [Google Scholar]
- Sullivan, R.W.; Hwang, Y.; Rais-Rohani, M.; Lacy, T. Structure analysis and testing of an ultralight UAV carbon composite wing. J. Aircr. 2009, 46, 814–820. [Google Scholar] [CrossRef]
- Simsiriwong, J.; Sullivan, R. Vibration testing of a carbon composite fuselage. In Proceedings of the 23rd American Society for Composites Conference, Memphis, TN, USA, 9–11 September 2008. [Google Scholar]
- Viscardi, M.; Arena, M.; Ciminello, M.; Guida, M.; Meola, C.; Cerreta, P. Experimental Technologies Comparison for Strain Measurement of a Composite Main Landing Gear Bay Specimen. In Nondestructive Characterization and Monitoring of Advanced Materials, Aerospace, Civil Infrastructure, and Transportation XII, Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, Denver, CO, USA, 4–8 March 2018; International Society for Optics and Photonics: Bellingham, WA, USA, 2018; p. 105990N. [Google Scholar]
- Official AFLoNext. Available online: http://www.aflonext.eu (accessed on 8 December 2020).
- Schwochow, J.; Sinske, J.; Buchbach, R. Inflight-Measurements of Aircraft Undercarriage Vibration during Deployment. In ettc2018-Proceedings, Proceedings of the ettc2018—European Test and Telemetry Conference 2018, Nürnberg, Germany, 26–28 June 2018; AMA Service GmbH: Wunstorf, Germany, 2018; p. 54. [Google Scholar]
- Official Clean Sky. Available online: http://cleansky.eu (accessed on 8 December 2020).
- Lienard, C.; Salah el Din, I.; Renaud, T.; Fukari, R. RACER high-speed demonstrator: Rotor and rotor-head wake interactions with tail unit. In Proceedings of the AHS International 74th Annual Forum & Technology Display, Phoenix, AZ, USA, 14–17 May 2018; Vertical Flight Society (VFS): Fairfax, VA, USA, 2018. [Google Scholar]
- Stokkermans, T.; Veldhuis, L.; Soemarwoto, B.; Fukari, R.; Eglin, P. Breakdown of aerodynamic interactions for the lateral rotors on a compound helicopter. Aerosp. Sci. Technol. 2020, 101, 105845. [Google Scholar] [CrossRef]
- Thiemeier, J.; Öhrle, C.; Frey, F.; Keßler, M.; Krämer, E. Aerodynamics and flight mechanics analysis of airbus helicopters’ compound helicopter RACER in hover under crosswind conditions. CEAS Aeronaut. J. 2019, 11, 49–66. [Google Scholar] [CrossRef]
- Belardo, M.; Paletta, N.; Di Palma, L.; Pecora, M. Structural and aeroelastic design of a joined-wing UAV. J. Aerosp. Eng. 2014, 27, 93–111. [Google Scholar] [CrossRef]
- Paletta, N.; Belardo, M.; Di Palma, L.; Pecora, M. Evaluation of wing loads during the flight drop test of the Italian Unmanned Space Vehicle. In Proceedings of the 5th International Operational Modal Analysis Conference, IOMAC 2013, Guimarães, Portugal, 13–15 May 2013; pp. 1–12. [Google Scholar]
- Viúdez-Moreiras, D.; Martín, M.; Abarca, R.; Andrés, E.; Ponsín, J.; Monge, F. Surrogate modeling for the main landing gear doors of an airbus passenger aircraft. Aerosp. Sci. Technol. 2017, 68, 135–148. [Google Scholar] [CrossRef]
- Barnes, J.; McMichael, J.; Walker, J.; Olliffee, R.; Baker, B. Nose Landing Gear Door Re-design. In Proceedings of the 48th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, AIAA Meeting Paper, Honolulu, HI, USA, 23–26 April 2007. [Google Scholar]
- Chiariello, A.; Orlando, S.; Vitale, P.; Linari, M.; Longobardi, R.; Di Palma, L. Development of a Morphing Landing Gear Composite Door for High Speed Compound Rotorcraft. Aerospace 2020, 7, 88. [Google Scholar] [CrossRef]
- Berger, E.J. Friction modelling for dynamic system simulation. Appl. Mech. Rev. 2002, 55, 430–453. [Google Scholar] [CrossRef]
- Ferri, A.A. Friction damping and isolation systems. J. Mech. Des. 1995, 117, 196–206. [Google Scholar] [CrossRef]
- Gaul, L.; Nitsche, R. The role of friction in mechanical joints. Appl. Mech. Rev. 2001, 52, 93–106. [Google Scholar] [CrossRef]
- Tleilia, A.; Nasri, R.; Chakhari, J. Damping in Bolted Structures. In Condition Monitoring of Machinery in Non-Stationary Operations; Springer: Berlin/Heidelberg, Germany, 2012; pp. 617–626. [Google Scholar]
- Gaul, L.; Becker, J. Damping prediction of structures with bolted joints. Shock Vib. 2010, 17, 359–371. [Google Scholar] [CrossRef]
- Goyder, H.; Lancereau, D.; Ind, P.; Brown, D. Friction and damping associated with bolted joints: Results and signal processing. In Proceedings of the 27th International Conference on Noise and Vibration Engineering (ISMA2016) and 5th International Conference on Uncertainty in Structural Dynamics (USD2016), Leuven, Belgium, 19–21 September 2016. [Google Scholar]
- Helicopter History Site (HELIS). Available online: https://www.helis.com/database/model/Airbus-Racer (accessed on 8 December 2020).
- MSC Nastran®, Quick Reference Guide 2019; MSC Software: Newport Beach, CA, USA, 2019.
- Huth, H. Zum Einfluβ der Nietnachgiebigkeit Mehrreihiger Nietverbindungen auf die Lastübertragungs und Lebensddauervorhersage; Bericht Nr. FB-172; LBF-Bericht: Darmstadt, Germany, 1984. [Google Scholar]
- Huth, H. Influence on Fastener Flexibility on the Prediction of Load Transfer and Fatigue Life for Multiple-Row Joints; ASTM STP 927; ASTM International: West Conshohocken, PA, USA, 1986. [Google Scholar]
- Caputo, F.; De Luca, A.; Greco, A.; Marro, A.; Apicella, A.; Sepe, R.; Armentani, E. Established Numerical Techniques for the Structural Analysis of a Regional Aircraft Landing Gear. Adv. Mater. Sci. Eng. 2018, 2018, 8536581. [Google Scholar] [CrossRef]
- ESDU online engineering manual: ESDU Vibration and Acoustic Fatigue Series, Featured Product from IHS ESDU. Available online: https://www.esdu.com/cgi-bin/ps.pl?sess=unlicensed_1210222080433dxf&t=ser&p=ser_vacf (accessed on 20 December 2020).
- North Atlantic Treaty Organization; Advisory Group for Aerospace Research and Development; Structures and Materials Panel. Damping Effects in Aerospace Structures: Papers. In Proceedings of the 48th Meeting of the AGARD Structures and Materials Panel, AGARD, Williamsburg, VA, USA,, 2–3 April 1979. [Google Scholar]
- Casiano, M.J. Extracting Damping Ratio from Dynamic Data and Numerical Solutions; Technical Memorandum; NASA/TM—2016–218227; NASA Marshall Space Flight Center: Huntsville, AL, USA, 2016. [Google Scholar]
- ASTM F3270/F3270M-17, Standard Practice for Compression versus Load Properties of Gasket Materials; ASTM International: West Conshohocken, PA, USA, 2017. Available online: www.astm.org (accessed on 8 December 2020).
- Sun, J.Q.; Jolly, M.R.; Norris, M.A. Passive, Adaptive and Active Tuned Vibration Absorbers-A Survey. Trans. Am. Soc. Mech. Eng. (ASME) 1995, 117, 234–242. [Google Scholar]
- Wright, R.I.; Kidner, M.R.F. Vibration Absorbers: A Review of Applications in Interior Noise Control of Propeller Aircraft. J. Vib. Control 2004, 10, 1221–1237. [Google Scholar] [CrossRef]
- Marano, G.C.; Greco, R.; Chiaia, B. A comparison between different optimization criteria for tuned mass dampers design. J. Sound Vib. 2010, 329, 4880–4890. [Google Scholar] [CrossRef]
- EASA. Certification Specifications and Acceptable Means of Compliance for Large Rotorcrafts; CS-29; Amendment 8; EASA: Cologne, Germany, 2020. [Google Scholar]
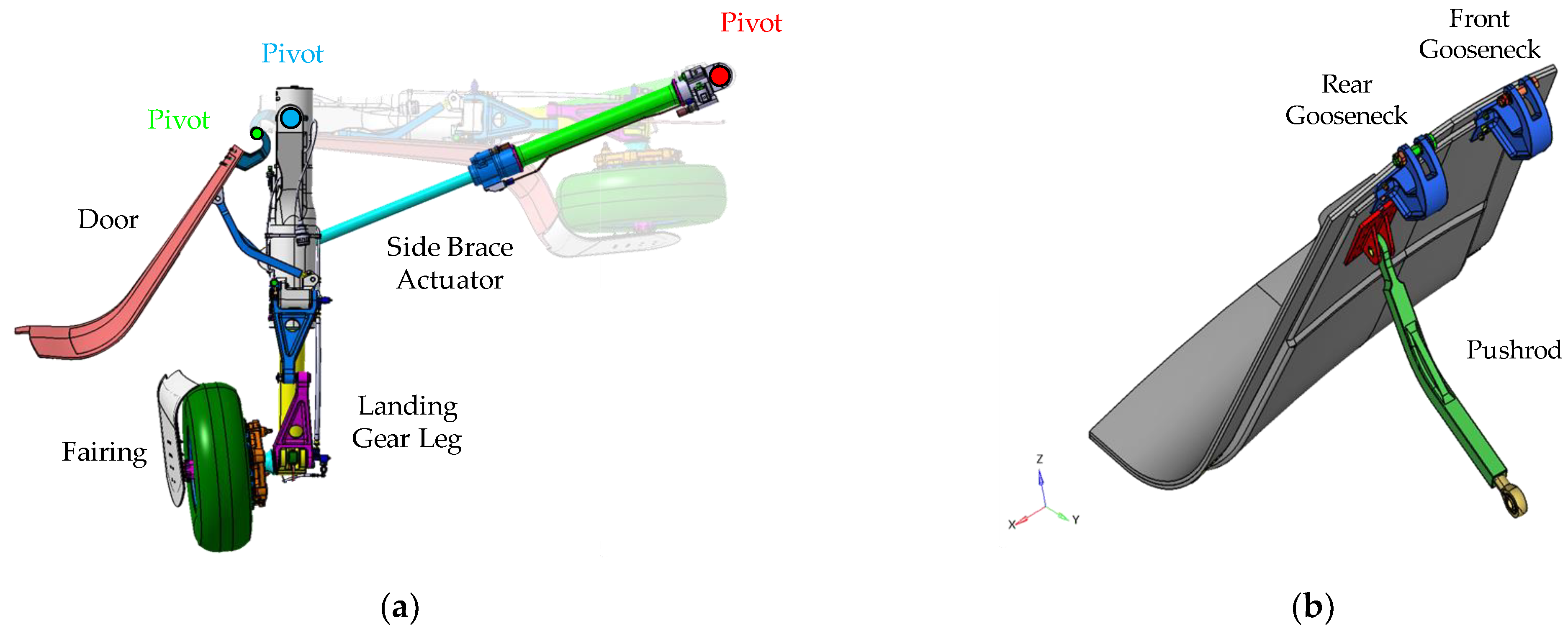
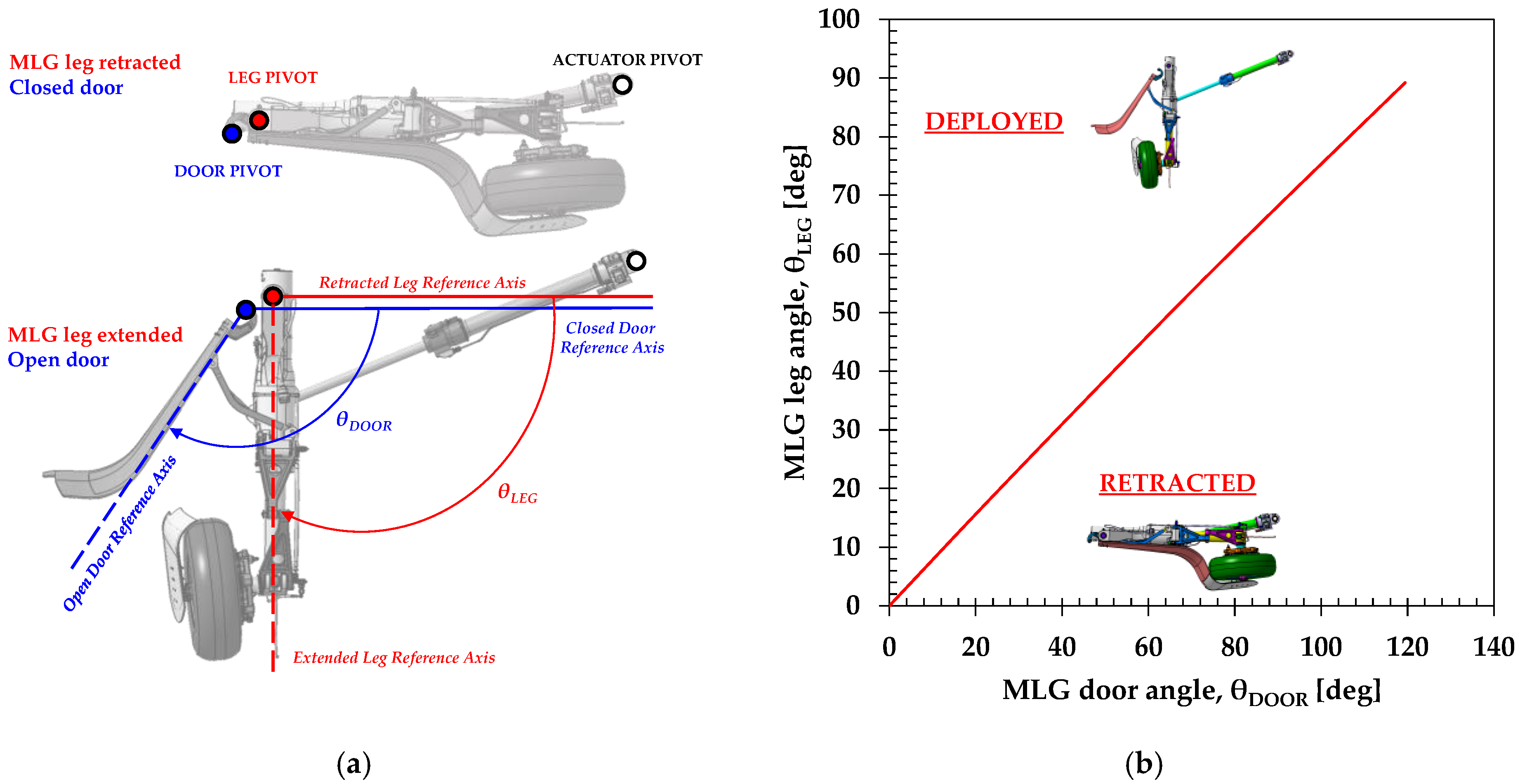
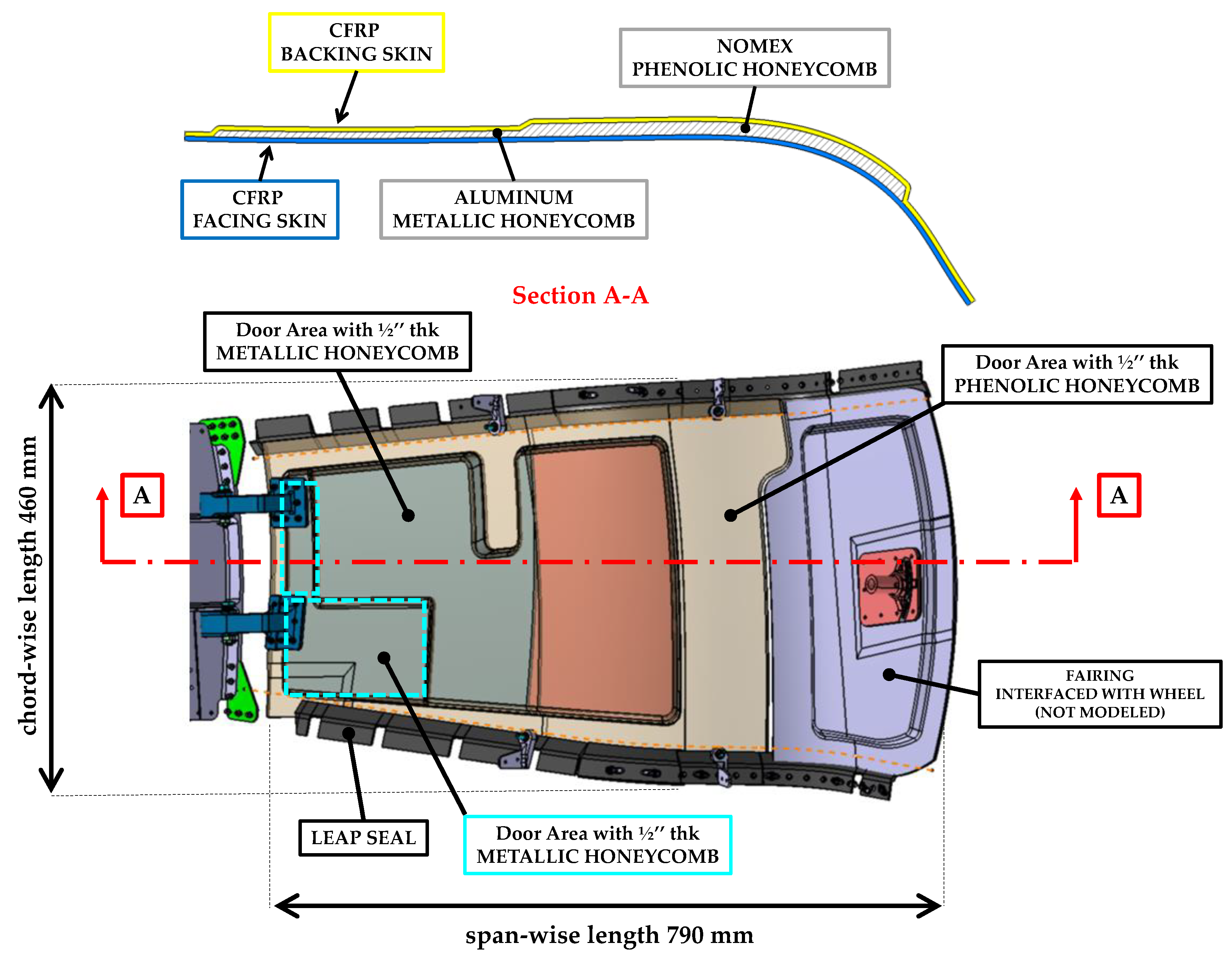
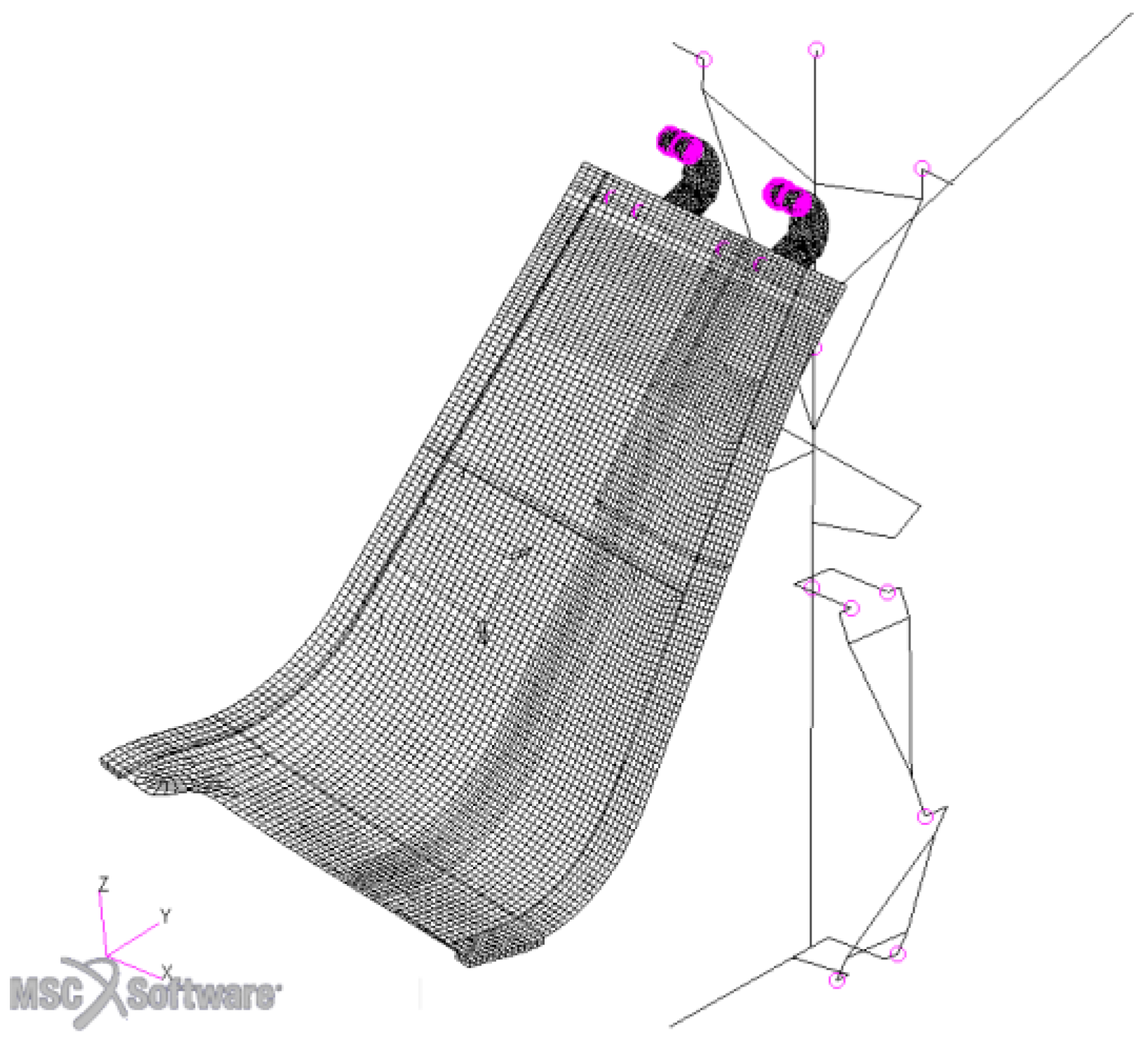

| Entity Type | Number of Entities |
|---|---|
| Grid nodes | 49,810 |
| 1D cbar | 49 |
| 1D cbeam | 20 |
| 1D cbush | 24 |
| 1D crod | 2 |
| 2D cquad4 | 12,415 |
| 2D ctria3 | 107 |
| 3D chex4 | 14,939 |
| 3D cpent4 | 233 |
| 3D ctet4 | 14,235 |
| rbe2 | 62 |
| rjoint | 24 |
| Number of Plies | Orientation | Single Ply Thickness (mm) | Laminate Thickness (mm) |
|---|---|---|---|
| 28 | (45°/0°/45°/0°/45°/0°/45°/45°/0°/45°/0°/45°/0°/45°)S | 0.31877 | 8.925 |
| 16 | (45°/0°45°/0°/0°/45°/0°/45°)S | 0.31877 | 5.1 |
| 14 | (45°/0°/45°/0°/45°/0°/45°)S | 0.31877 | 4.463 |
| 8 | (45°/0°/45°/0°)S | 0.31877 | 2.55 |
| Region | Structural Damping |
|---|---|
| Metallic fittings | 0.017 |
| Laminate panel | 0.025 |
| Honeycomb | 0.014–0.055 |
| Description | Frequency (Hz) |
|---|---|
| 5/rev MR | 23.3 |
| 1/rev LR | 29.2 |
| 10/rev MR | 46.5 |
| MLG Door Configuration | Mode I | Mode II | Mode III | |
|---|---|---|---|---|
| f [Hz] | f [Hz] | f [Hz] | ||
| baseline | 20.5 | 36.0 | 52.4 | |
| extra mass configuration | 1 kg on fwd corner | 14.7 | 30.5 | 47.3 |
| 1 kg on aft corner | 18.2 | 28.7 | 35.4 | |
| 0.5 kg pair | 16.4 | 30.1 | 38.1 | |
| 0.4 kg pair | 17.0 | 31.2 | 40.0 | |
| 0.3 kg pair | 17.7 | 32.3 | 42.1 | |
| 0.2 kg pair | 18.5 | 33.5 | 44.8 | |
| 0.1 kg pair | 19.4 | 34.7 | 48.2 | |
| aero load action | blowing pressure | 21.0 | 36.2 | 52.9 |
| pulling pressure | 20.2 | 35.8 | 51.8 | |
| helicopter rotors harmonics | 23.3 | 29.2 | 46.5 | |
| MLG Door Configuration | Mode I | Mode II | Mode III | |
|---|---|---|---|---|
| f (Hz) | f (Hz) | f (Hz) | ||
| baseline (nominal) | 20.5 | 36.0 | 52.4 | |
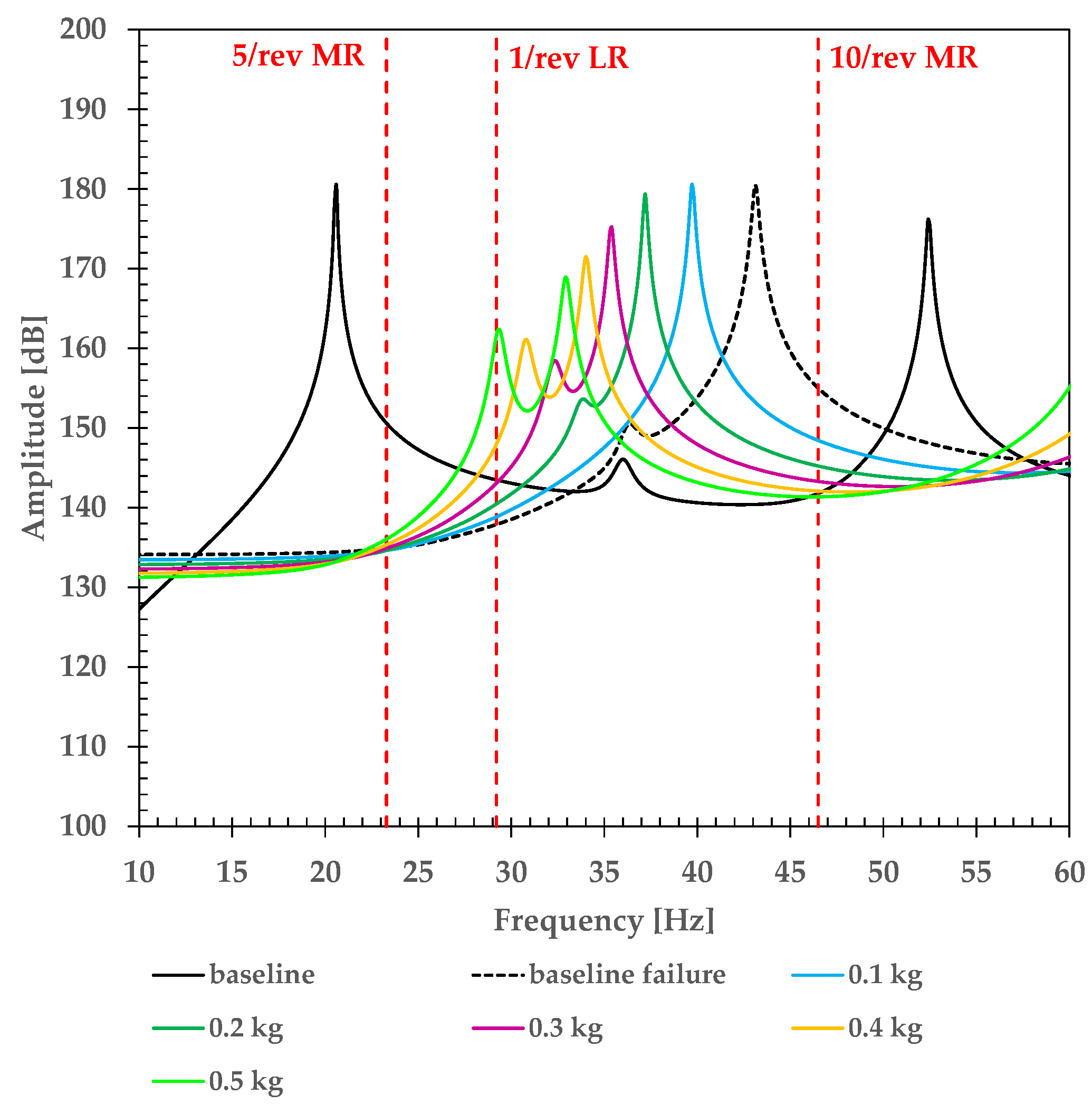
| baseline (failure) | 0.0 | 36.3 | 43.1 | |
| extra mass configuration | 1 kg on fwd corner | 0.0 | 29.2 | 34.0 |
| 1 kg on aft corner | 0.0 | 28.5 | 34.4 | |
| 0.5 kg pair | 0.0 | 29.3 | 32.9 | |
| 0.4 kg pair | 0.0 | 30.8 | 34.0 | |
| 0.3 kg pair | 0.0 | 32.3 | 35.4 | |
| 0.2 kg pair | 0.0 | 33.8 | 37.2 | |
| 0.1 kg pair | 0.0 | 35.1 | 39.7 | |
| helicopter rotors harmonics | 23.3 | 29.2 | 46.5 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arena, M.; Chiariello, A.; Castaldo, M.; Di Palma, L. Vibration Response Aspects of a Main Landing Gear Composite Door Designed for High-Speed Rotorcraft. Aerospace 2021, 8, 52. https://doi.org/10.3390/aerospace8020052
Arena M, Chiariello A, Castaldo M, Di Palma L. Vibration Response Aspects of a Main Landing Gear Composite Door Designed for High-Speed Rotorcraft. Aerospace. 2021; 8(2):52. https://doi.org/10.3390/aerospace8020052
Chicago/Turabian StyleArena, Maurizio, Antonio Chiariello, Martina Castaldo, and Luigi Di Palma. 2021. "Vibration Response Aspects of a Main Landing Gear Composite Door Designed for High-Speed Rotorcraft" Aerospace 8, no. 2: 52. https://doi.org/10.3390/aerospace8020052
APA StyleArena, M., Chiariello, A., Castaldo, M., & Di Palma, L. (2021). Vibration Response Aspects of a Main Landing Gear Composite Door Designed for High-Speed Rotorcraft. Aerospace, 8(2), 52. https://doi.org/10.3390/aerospace8020052








