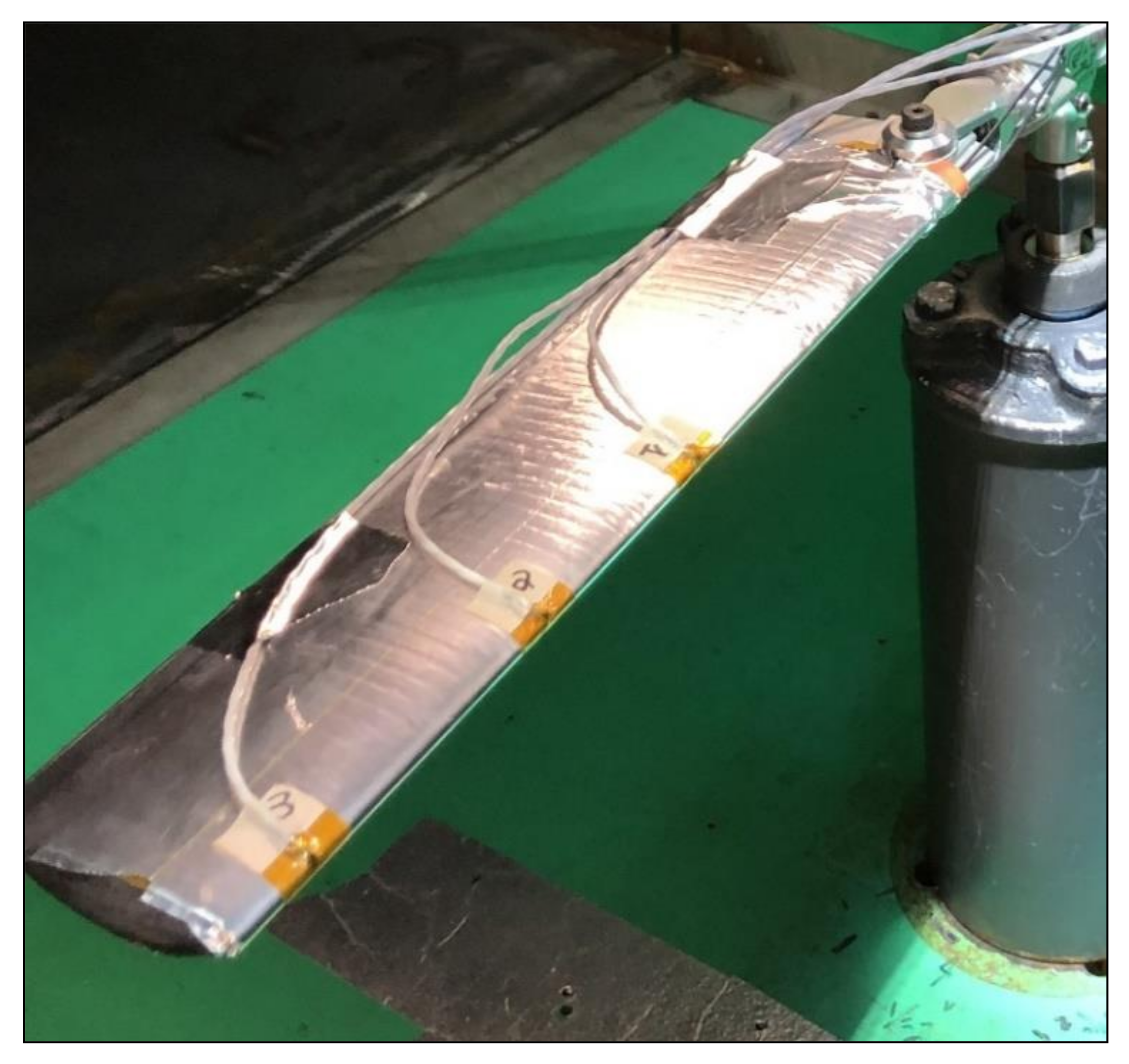
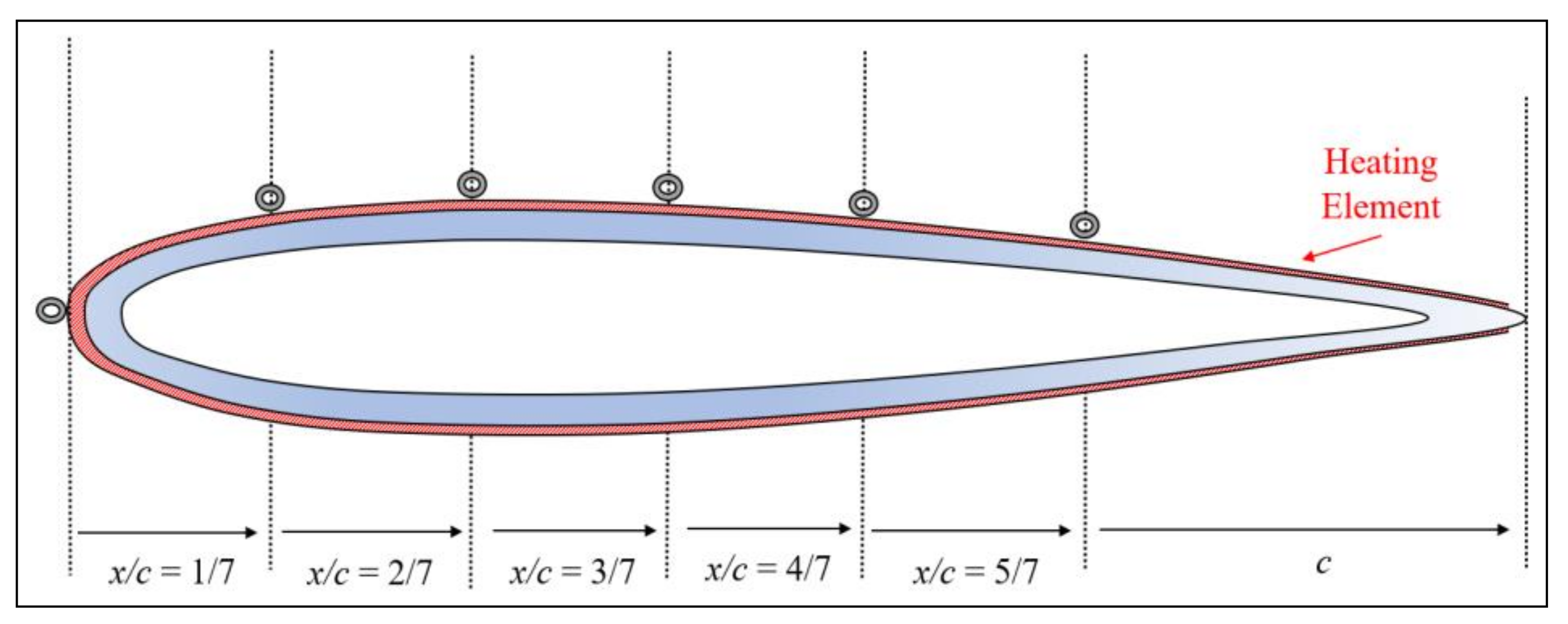
1. Introduction
Aircraft are vulnerable to in-flight and pre-flight icing that limit their operations. Indeed, icing causes flight delays during ground de-icing interventions, flight cancellations because of forecast or actual in-cloud icing [
1]. During flight, liquid water impinging on an aircraft will freeze to its surfaces even when the outside air temperature (OAT) is above 0 °C [
2]. According to [
2,
3], pilot-reduced visibility is a direct result of in-flight ice accumulation on windscreen and instrument ports. In addition, added weight on the airframe reduces the load capacity and increases fuel consumption. In the case of a helicopter, the most adverse effect of blade contamination comes from the aerodynamics of the iced main rotor blade, which will result in reduced lift and an increase in drag [
3]. A subsequent loss of thrust and possible flow separation generated by the main and tail rotors may lead to the total loss of control. Severe vibrations may also be induced by the shedding of ice that can force emergency landings. Although the primary concern is with the main rotor, the protection of the tail rotor requires similar considerations.
Calculating the convective heat transfer on airfoils is an important first step in the operation of icing codes. According to a study on the assessment of thermodynamic models for ice accretion [
4], heat transfer by convection is one of the most important terms in the heat balance for a successful icing/de-icing simulation of aircraft. In the literature, the convective heat transfer from standard geometrical configurations such as flat plates, cylinders, and spheres has been studied and reported extensively. Correlations were developed for the Nusselt (
Nu) or (
Fr) Frossling numbers based on a product of the Reynolds (
Re) and Prandtl (
Pr) numbers, in the form of Equation (1) [
5,
6], where
Fr =
Nu ×
Re−0.5. These correlations are associated with parameters (
A) and (
m) that differ if the flow is laminar or turbulent, or if the thermal boundary conditions (TBC) are either a constant surface temperature (
TS) or a constant surface heat flux (
QS) [
6]. Of the most recent studies, a correlation is proposed even for the
Nu in the transition region of the flat plate [
7].
The case of airfoil heat transfer is more complex, given the thicknesses involved and the effects of angles of attack (
α). Yet, the literature indicates that for fixed-wing airfoils, the convective heat transfer has been thoroughly studied. The work of Poinsatte et al. [
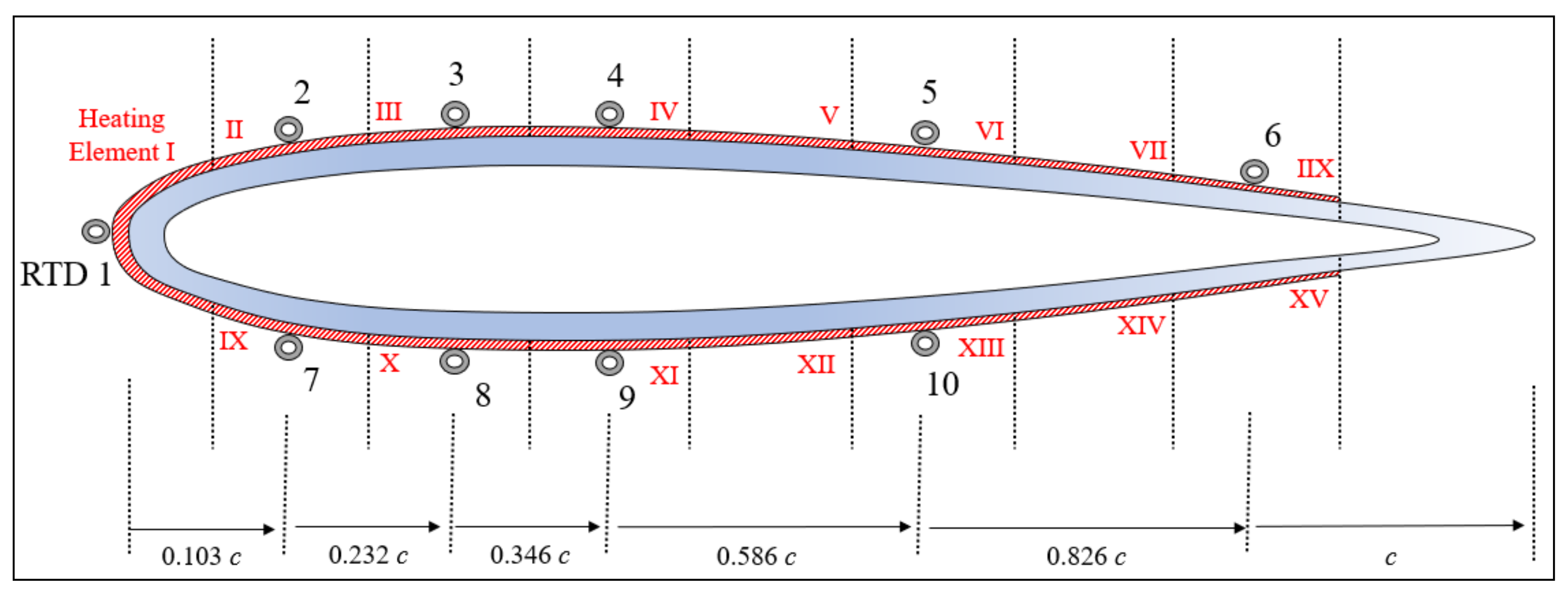
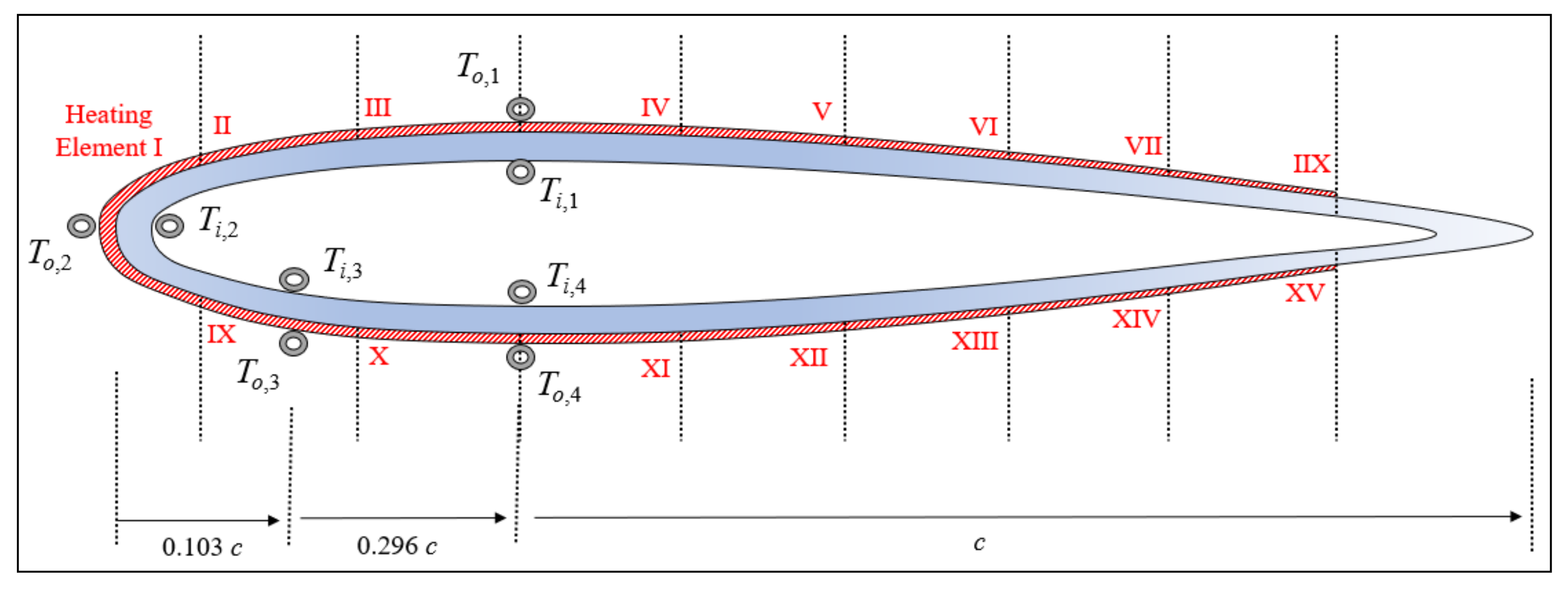
8] provided a comprehensive analysis of airfoil heat transfer when they quantified the heat transfer on the leading edge (LE) of a smooth and roughened NACA 0012. Their data were gathered from in-flight measurements of the NASA Lewis Twin Otter (NASA Lewis Research Center Cleveland, OH, USA) icing research aircraft and experiments in the Icing Research Tunnel (IRT). The experimented airfoil’s LE up was maintained on a constant surface temperature using embedded heating gauges located between −3.6% and 9.5% of the dimensionless wrap distance
S/
c. A total of 46 tests were carried out for −6° ≤
α ≤ 8° and 1.2 × 10
6 ≤
Re ≤ 2.4 × 10
6. The measured heat transfer on the airfoil was compared to the results of the flat plate under laminar and turbulent flow conditions and the cylinder correlations. For the smooth surface tests, their work affirmed the use of the
Fr and showed that the behavior of heat transfer in the laminar flow portion was similar to the behavior seen on the cylinder and the flat plate for the same flow conditions. They used Equation (1) to successfully correlate the
Frx at each
S/
c with
Re and found that on the stagnation point and near the LE, the correlations show values of
m = 0. The
Fr curves collapse into one in the laminar flow region making the
Fr independent of
Re.
A set of experiments between 2006 and 2008 by Wang et al. [
9,
10,
11,
12] measured the average Nusselt number (
NuAvg) on the surface of a hollowed NACA 63-421. The experimented airfoil was equipped with heating strips installed on the inner edges of the airfoil. The heaters were set to provide a constant heat flow of 500 W that was transferred to the airflow by conduction through the airfoil material. A total of 25 thermocouples were distributed across the chord on the exterior and interior surfaces of the airfoil to measure the temperature differences. They also correlated their data, in terms of the
NuAvg, based on Equation (1). Their main contribution was in the expansion of the
A term in Equation (1) to account for the
α and liquid water content (
LWC). Correlations were formed for dry air at
α = 0° [
9] and 0° ≤
α ≤ 25° [
10], in addition to 0 ≤
LWC ≤ 4.98 g/m
3 at
α = 0° [
11] and 0° ≤
α ≤ 25° [
12].
The static pressure and heat transfer rates were measured by Li et al. on the surface of a thick BO28 airfoil at locations covering 90% of both the top and bottom surfaces for −8.5° ≤
α ≤ 19.5° and
Re = 2.5 × 10
5, 5.82 × 10
5 and 1.085 × 10
6 [
13]. The airfoil had 23 embedded heating tiles that were heated to a specified constant temperature. They paid close attention to the effect of flow transition on the increased heat transfer between the laminar and turbulent flow regions by calculating the
Fr on various chord locations. Their tests again showed independence of the
Fr on the
Re in the laminar flow region on the airfoil, confirming the results of [
8]. When the
Re was fixed and the
α was increased, flow transition on the suction side of the airfoil moved closer to the LE, and an abrupt increase of heat transfer was detected. On the pressure side, the transition point was pushed towards the trailing edge and a similar abrupt increase of heat transfer was also observed. When the
α was fixed and the
Re was increased, the increase of heat transfer due to flow transition was pushed closer to LE on both surfaces of the airfoil. For all tests, high
Fr values were observed on the stagnation point however, the highest
Fr values were actually observed on locations where the flow transitioned from laminar to turbulent. The abrupt increase of
Fr at the transition point was followed by a less severe yet rapid decrease of
Fr values downstream towards the trailing edge.
Many factors add to the complexity of rotor heat transfer estimation compared to fixed-wing platforms. For instance, the rotation of the blades causes the blade local velocity (and associated
Re) to increase from root to tip. Moreover, tip vortices are formed when the pitch angle is varied, creating a range of
αEff across the blade radius. To further complicate the problem, the interaction of a freestream velocity (
V∞) with the rotating blades (as in the case of forward flight) produces advancing and retreating regions in the plane of rotation. The blade local velocity, therefore, experiences fluctuations that in turn generate high levels of turbulence intensity (
TI). Turbulent heat transfer augmentation is a direct result of the
TI that, according to [
14], affects the stagnation, laminar, and turbulent regions of flows on airfoils.
To the best of the authors’ knowledge, there are no previous experimental attempts to correlate heat transfer data measured on the surface of a small helicopter tail rotor. Although extensive experimental efforts have been made on rotor icing in the past [
15,
16,
17,
18,
19], the literature still lacks a correlation that considers both radial and chordwise variations of the
Frx. Moreover, a recent study by Aubert [
20] also found that the entire rotor simulation process from ice accretion to impact to shedding needs refinement. He reviewed previous icing rotor studies and concluded that the ice structure formed on rotor blades is not fully understood and that the type of accreted ice from the blade root to tip and the mechanical properties can vary. He also concluded that most numerical solvers, including their heat transfer calculation schemes, need enhancement for better icing and de-icing predictions.
In an earlier publication, Samad et al. [
21] proposed the use of low- and medium-fidelity numerical tools for heat transfer calculation of a small helicopter tail rotor. The tools are known as the BEMT–RHT and UVLM–RHT. They implement the Blade element momentum theory (BEMT) and the unsteady vortex lattice method (UVLM), on one hand, and a CFD-determined heat transfer correlation for an airfoil under fully turbulent flow conditions, on the other. The correlation is an expanded version of Equation (1) and describes the airfoil
FrAvg in the form of Equation (2). The coupling leads to an increase of fidelity, maintains the relatively computationally inexpensive solution, and provides an added layer of heat transfer prediction.
The objective of this paper is to correlate the measured Frx on the rotor surface with Re and αEff in a similar way as Equation (2). If the objective is met, it is believed the heat transfer prediction methodology of the BEMT–RHT and UVLM–RHT could be validated.
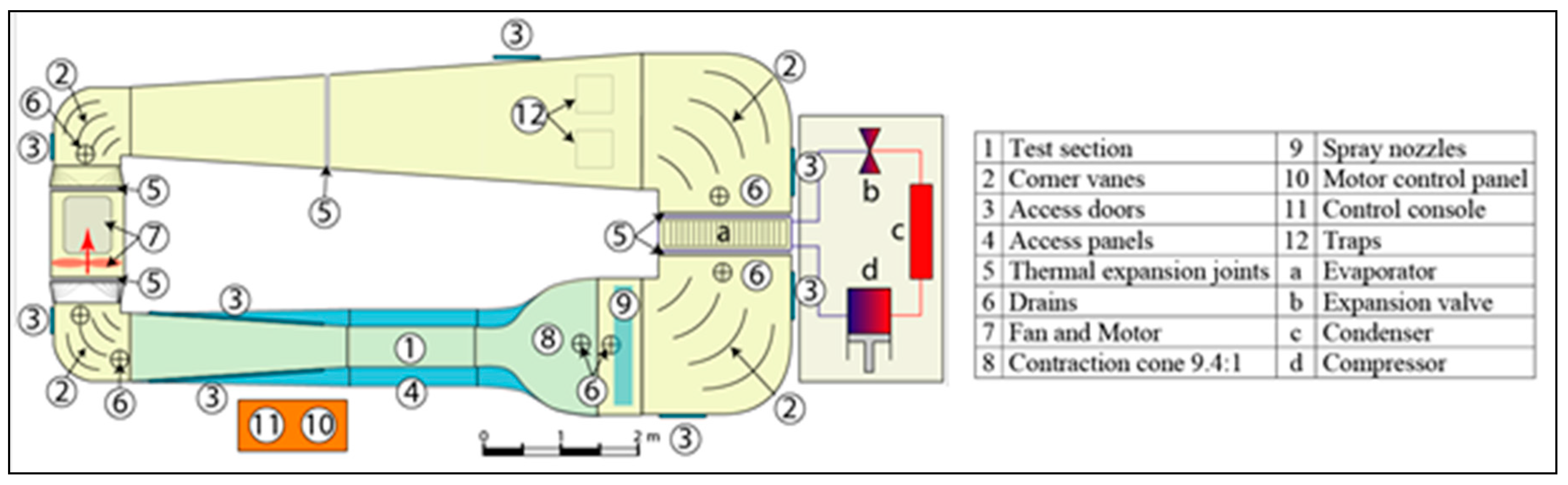
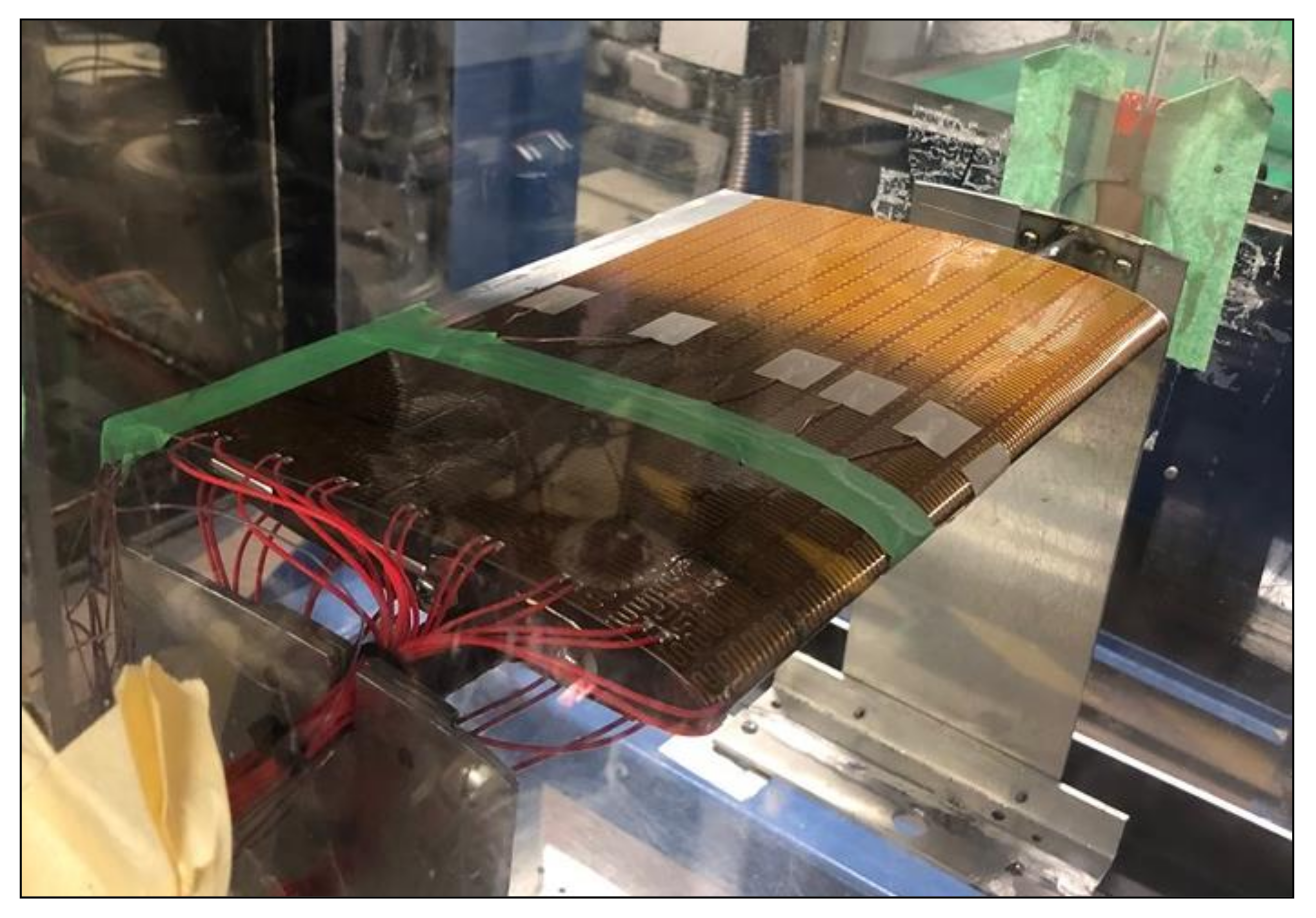
In the following sections, a description of the icing wind tunnel (IWT) is presented first. This is followed by a description of the fixed-wing and rotor experimental setups, along with the details of design and construction. Next, the methodology followed to calculate the convective heat transfer is outlined, together with the procedure to estimate heat losses, calculate the TI, and the experimental error. The test plan is laid out, and the procedure is followed for data acquisition and averaging. In the Results section, the calculated TI and the estimated heat losses due to radiation and conduction are discussed first. Heat transfer measurements are reported in terms of the Frx at the specified measurement locations and the FrAvg. The fixed-wing measurements are used for verification, where the stagnation point data are compared to previous experimental data from the literature and to CFD predictions. Finally, the rotor Frx data at each S/c and θ are then correlated with Re and αEff, in a similar form as Equation (2).
4. Discussion
Previous studies that correlated
Frx with
Re (using Equation (1)) were all made using non-rotating geometries, and the
Re was mostly constant during the test. Contrary to a fixed-wing, the
Re for rotating blades varies during the test. As an example,
Figure 18 shows the variation of the calculated
Re (Equation (5)) at the three
r/
R of rotor test #2 versus time. Because of the freestream velocity and blade rotation, the
Re depends on
Ω,
V∝ and
r/
R. This triple dependency creates relatively large variations of the
Re, as shown in
Figure 18, where the
Re increases when the blade is on the advancing side and decreases on the retreating side of the blade. This goes in parallel with variations of the
αEff, as shown in
Figure 17 for the same reasons.
In this study, the proposed correlations were built based on the averaged values of
Fr,
Re, and
αEff. (
ReAvg) is defined as the average value of
Re of each test and radial position and is calculated the mean of the varying
Re, as shown in
Figure 18. Similarly, the average values of
αEff from
Figure 17 are also used. The range of
ReAvg is between 9.5 × 10
4 ≤
ReAvg ≤ 3.71 × 10
5 corresponding to the lowest
ReAvg at
r/
R = 0.6 for
Ω = 500 RPM and the highest at
r/
R = 0.95 for
Ω = 1500 RPM.
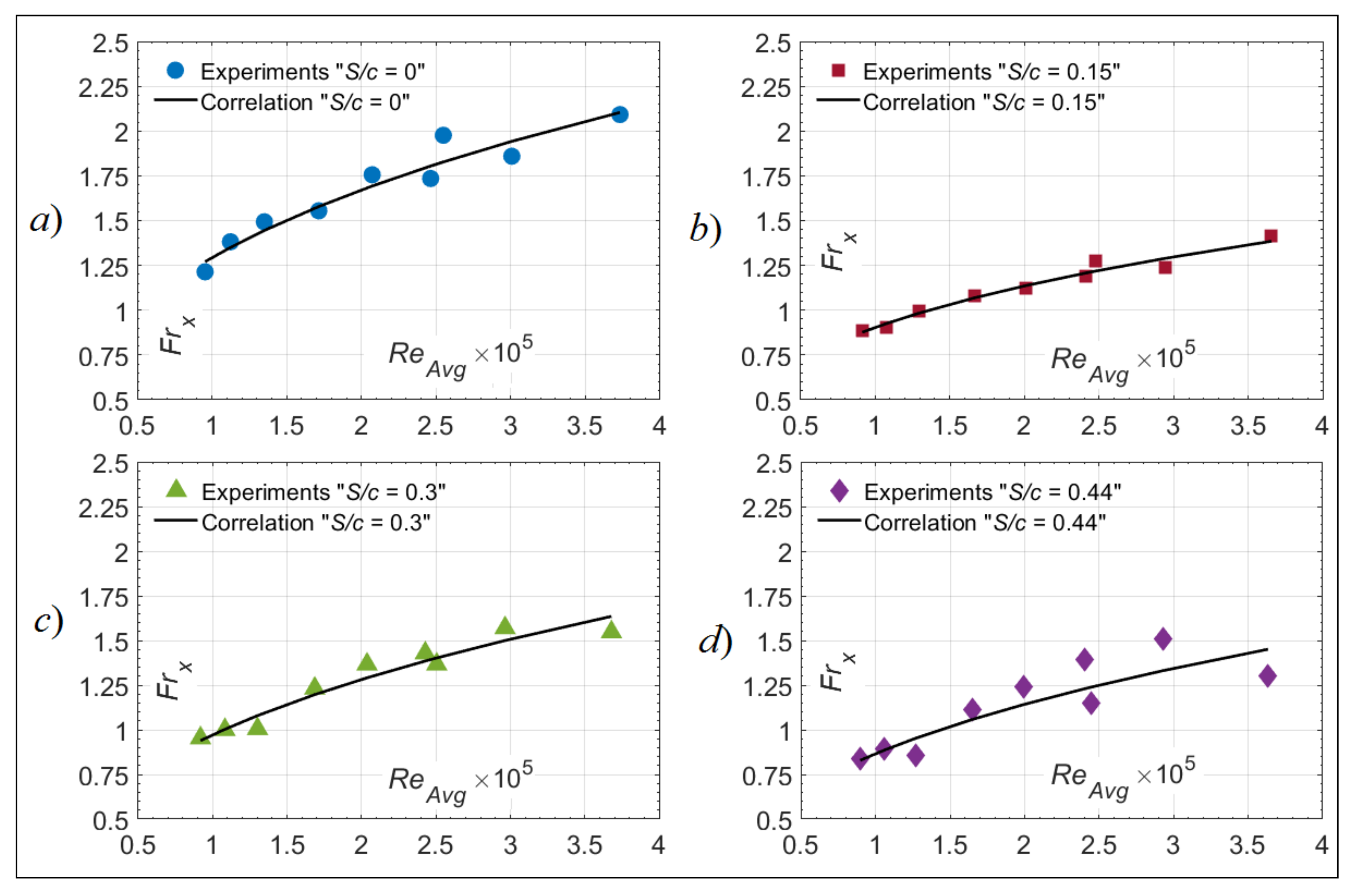
The first correlation attempt was for the tests at
θ = 0° given that the
αEff = 0° throughout the blade. This way, the correlation is expected to satisfy the form of Equation (1). In this study, the
Frx was correlated with
ReAvg at each
S/c. Curve fitting was carried out using MATLAB, and the correlation constants
A and
m are presented in
Table 10, together with the average error found between the experimental data and the correlation prediction. The
Pr used was the one at
T∞ = 248.15 K and is equal to
Pr = 0.70.
If the parameters are analyzed, it is found that for
θ = 0°, the
Frx correlates with
ReAvg by 0.369 ≤
m ≤ 0.399 at all
S/
c. This contradicts the results of [
8], who obtained
m = 0 at the stagnation point and laminar region downstream for a fixed-wing airfoil with
α = 0°. Furthermore, the range of
m for
θ = 6° is 0.220 ≤
m ≤ 0.373, which is also not expected near the leading edge of the airfoil. These values for
m could only be explained by the relatively high
TI found in the rotor tests. Turbulent heat transfer augmentation is a direct result of the
TI that, according to [
14], affects the stagnation, laminar, and turbulent regions of flows on airfoils.
Figure 19 shows a graphical representation of the correlations in
Table 10, compared to the measured values of
Frx for each
S/
c at
θ = 0°. The correlations fit very well the data, with an average error between 2.14% and 7.69%. This was also the case in [
8], where the fitting of the fixed-wing data at
α = 0° and different
S/
c was successful, and with a similar average error similar to the one found for the rotor at
θ = 0°. Recalling that the correlations are fit based on Equation (1) and that the
αEff = 0° for the non-lifting rotor, then
Figure 19 proves that the possibility of correlating rotor data only with
ReAvg is successful.
Because the aim of this paper is to propose a correlation for the
Frx in terms of the
Re and
αEff, the data for the rotor tests at
θ = 6° are now fitted in the form of Equation (23), which is a generalized form of Equation (2) for the BEMT–RHT and UVLM–RHT. The
A and
m parameters used were the ones from the tests at
θ = 0°, and the values of
αEff (converted to radians) were the ones determined through the UVLM calculations from
Section 3.4. For the tests at
θ = 0°, the
αEff is 0° throughout the blade and Equation (23) reduces to the same correlations previously presented in
Table 10. This way, Equation (23) could be used to calculate the
Frx at any blade section, even when the
αEff varies with
θ.
The curve fitting of Equation (23) was applied for each
S/
c of the tests at
θ = 6°, and the results for
C1 and
C2 are presented in
Table 11 along with the average error between the experimental data and correlation predictions.
Figure 20 also shows a graphical representation of the comparison between the predictions of Equation (23) and the experimental data at
θ = 6°. At
S/
c = 0,
S/
c = 0.30, and
S/
c = 0.58, the correlation fits the data very well and within 3% to 7% error. For
S/
c = 0.15, the error is higher, i.e., just under 15%. By examining
Figure 20, the elevated error originates mostly from a single test at
r/
R = 0.6. Given the experimental error involved, however, it is believed an acceptable estimate of the
Frx can be obtained with the novel correlation of Equation (23).
5. Conclusions
This paper presented heat transfer measurements made on the surfaces of a fixed-wing and a small two-blade rotor. The goal of this study was to propose correlations for the experimentally measured rotor Frx values based on Re and αEff. The fixed-wing tests were used to verify the heat transfer measurements in the IWT and had a total experimental between 16% and 21%. Results indicate that the fixed-wing Frx at the stagnation point was in the range of experimental data from the literature, and within 8% of fully turbulent CFD simulations. The FrAvg also agrees with CFD predictions, with an average discrepancy of 1.4%.
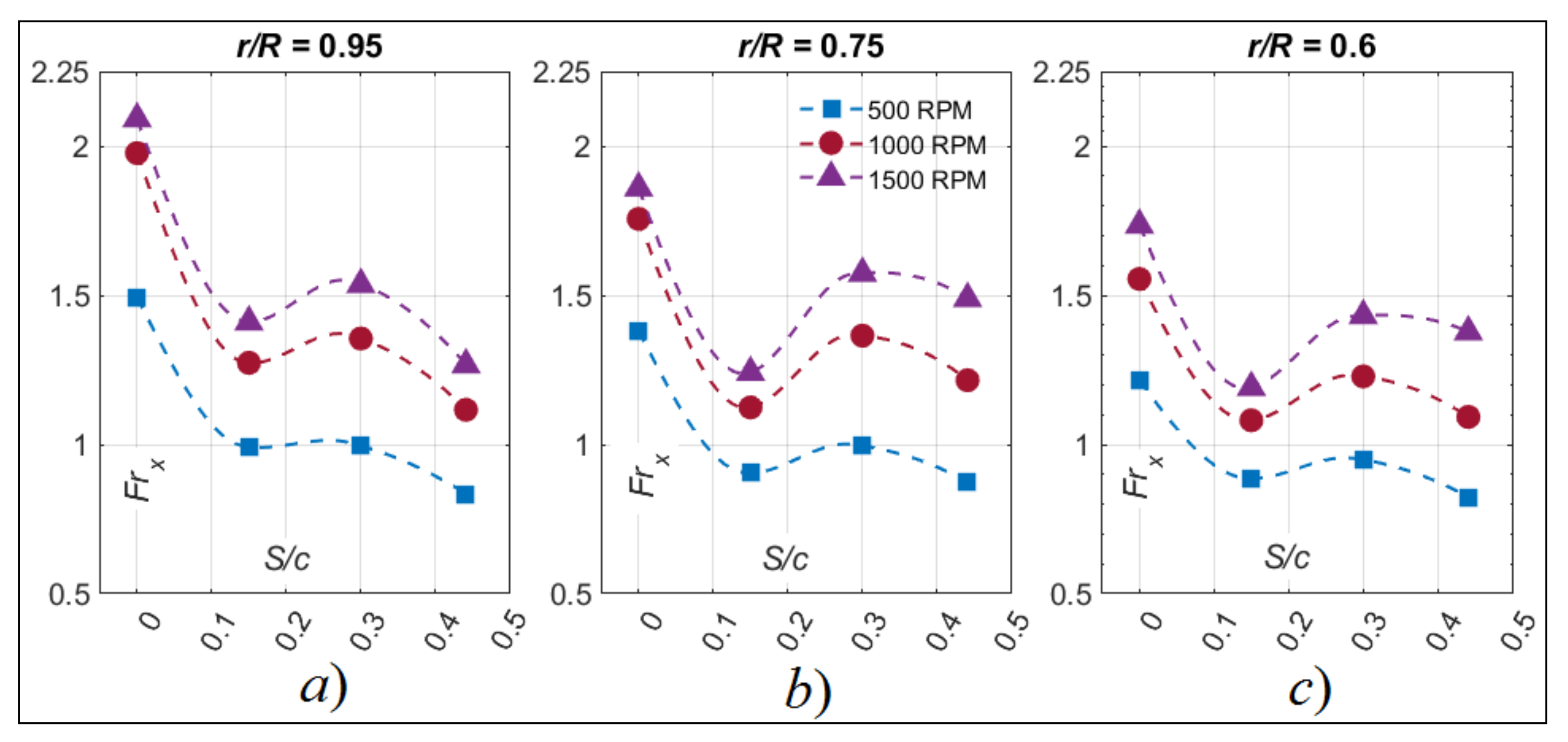
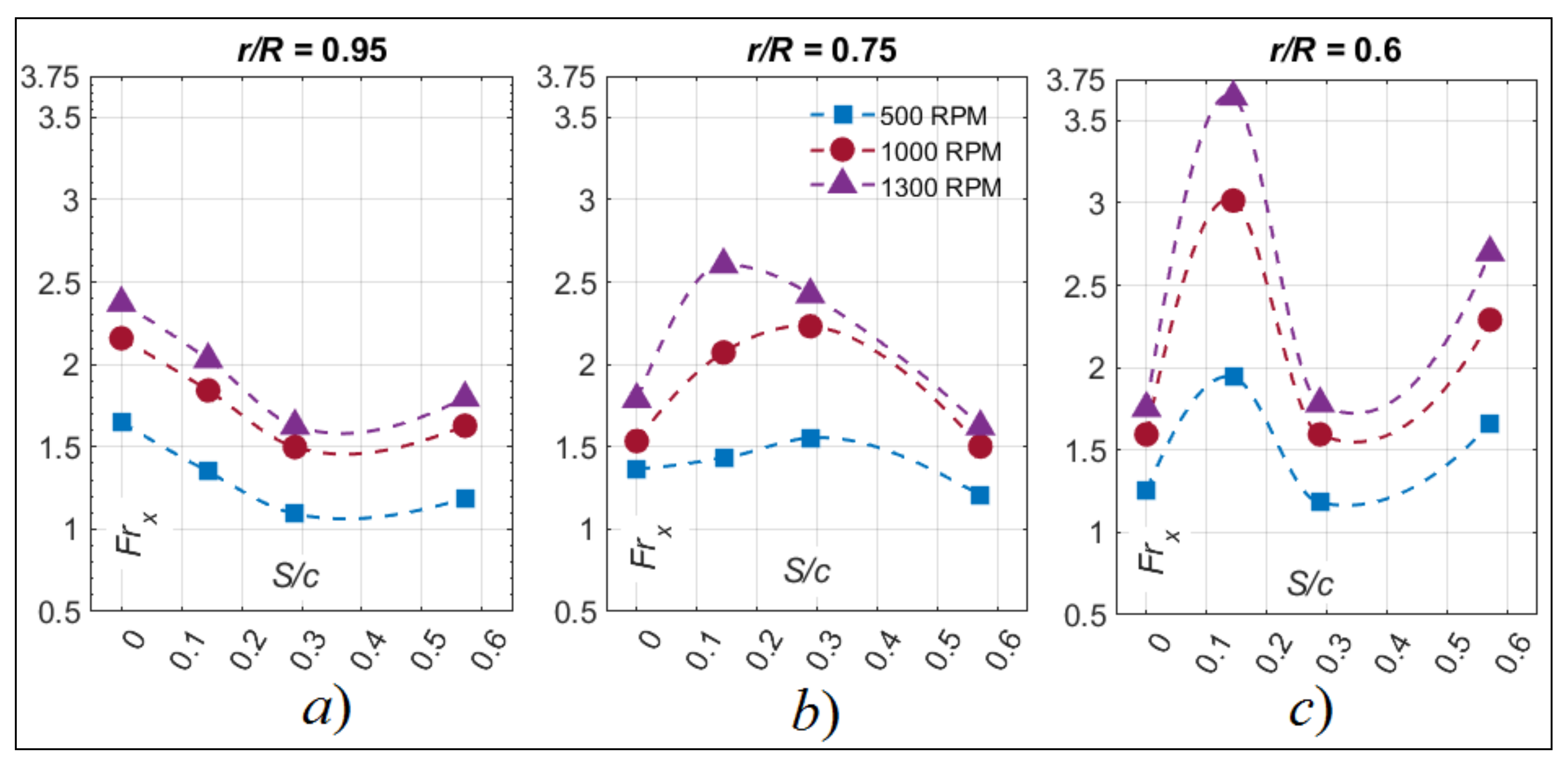
For the rotor tests, the total experimental error was estimated at 25%. The Ω directly increased the Frx values for all rotor tests due to an increase of Re. The rotor tests at θ = 0° showed that Frx behavior was rather insensitive to changes in r/R whereas the behavior changed significantly between r/R for the tests at θ = 6°. As the UVLM–RHT simulations proved, this was due to the varying αEff across the blade for the tests with the positive pitch angle, whereas for the non-lifting rotor, the αEff is 0° from root to tip.
The Frx data from the tests at θ = 0° were successfully correlated with Re at each S/c and with an error between 2.14% to 7.69%. When a term for the αEff was incorporated, a novel correlation for the Frx (at each S/c) in the form of Equation (23) that satisfies the data at θ = 0° and θ = 6° could then be formed and with a maximum error 14%. The results of this paper, therefore, show that the Frx across the rotor blade could be successfully correlated with Re and αEff; thus, justifying the methodology implemented in the previously developed BEMT–RHT and UVLM–RHT.
On a side note, the Frx correlations with RemAvg showed values of m that are consistently larger than 0.329. Given the locations of measurements and the range of Re involved, it was expected that some of the proposed correlations show values of m = 0, which is expected for laminar flow on flat plates or fixed-wing airfoils. The relatively high TI values are suspected to be the reason for this, which could eventually cause heat transfer augmentation and therefore explain the Frx variation with Re. In this study, no corrections for TI were implemented, and further investigation is recommended.
Future work in this research project includes further testing of the present rotor setup but under icing conditions. Several ice-accreting/de-icing tests will be run and compared to the presently ice-free heat transfer measurements. The gathered data will be studied and included in the BEMT–RHT and UVLM–RHT to account for heat transfer calculation under icing conditions.