Ion Source—Mathematical Simulation Results versus Experimental Data
Abstract
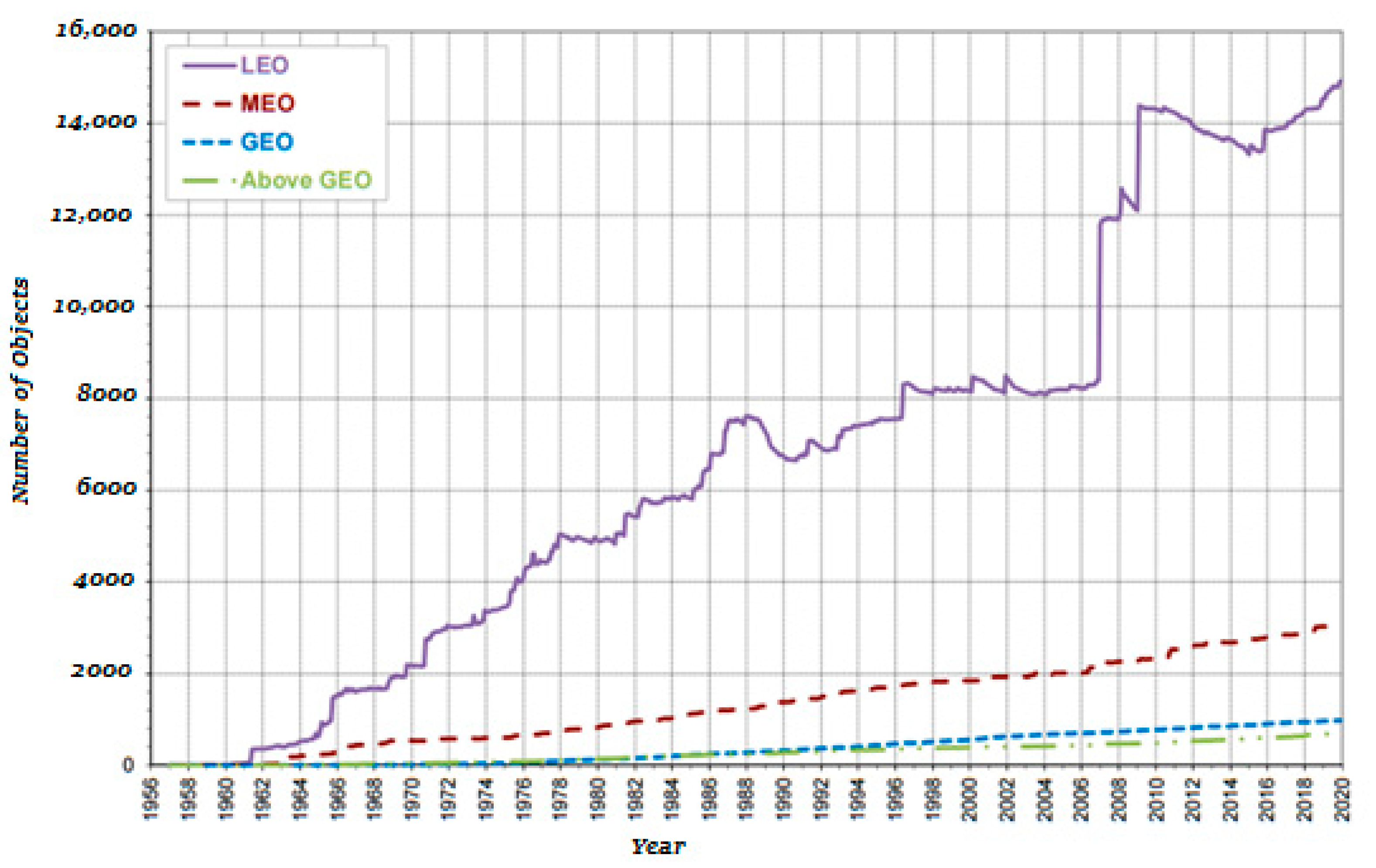
1. Introduction
2. The Status of the Problem
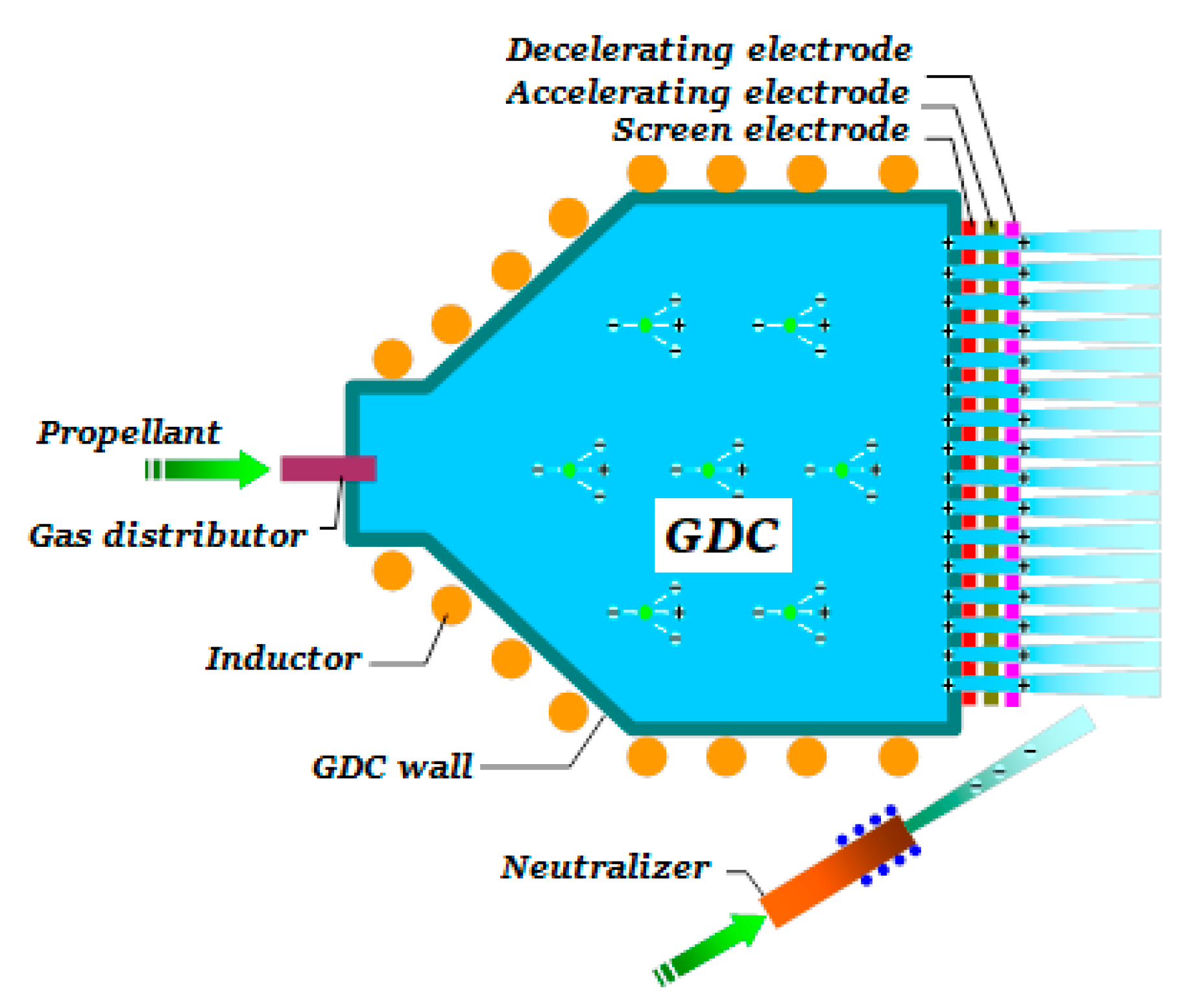
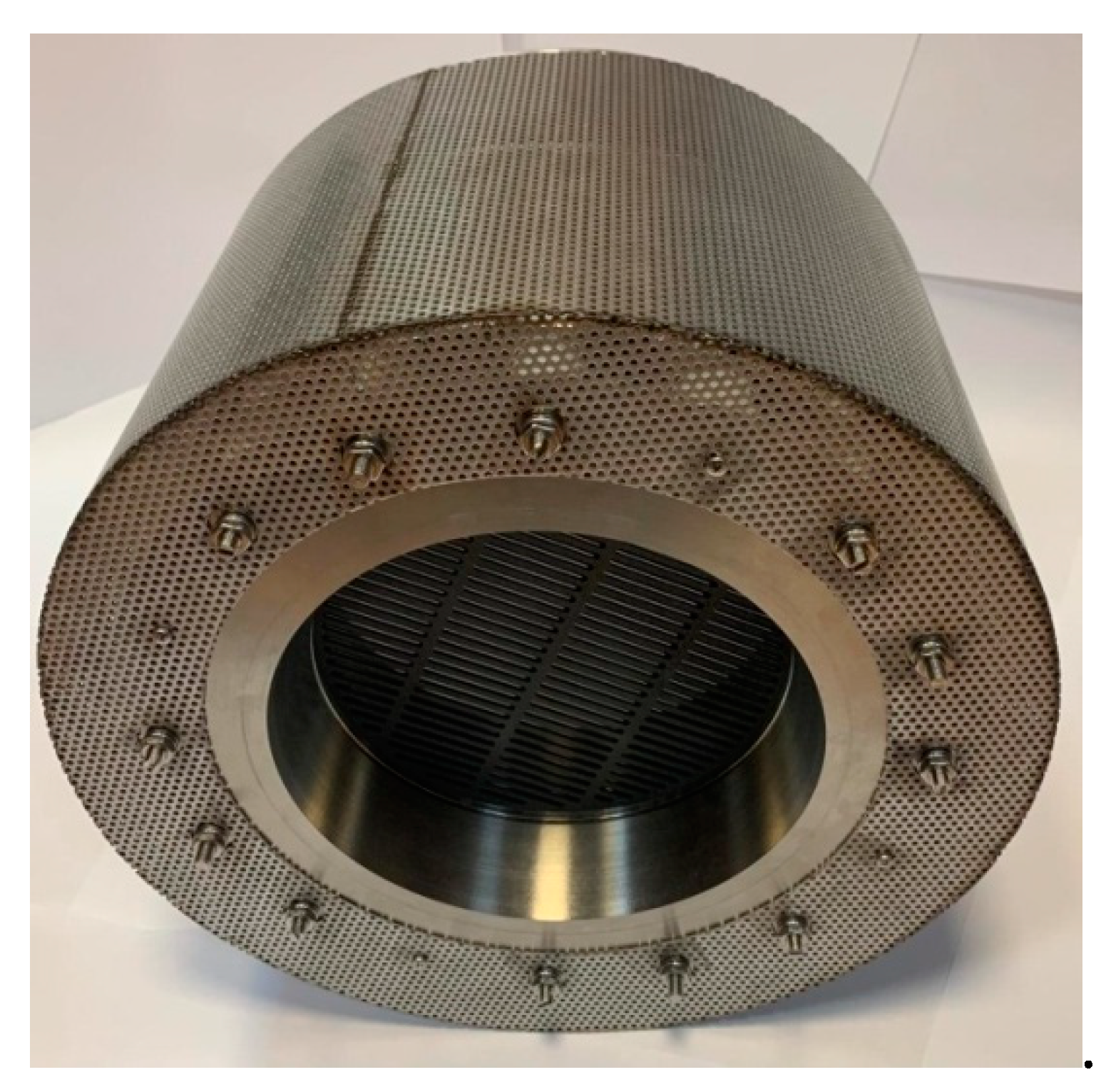
2.1. The Ion Source (IS)
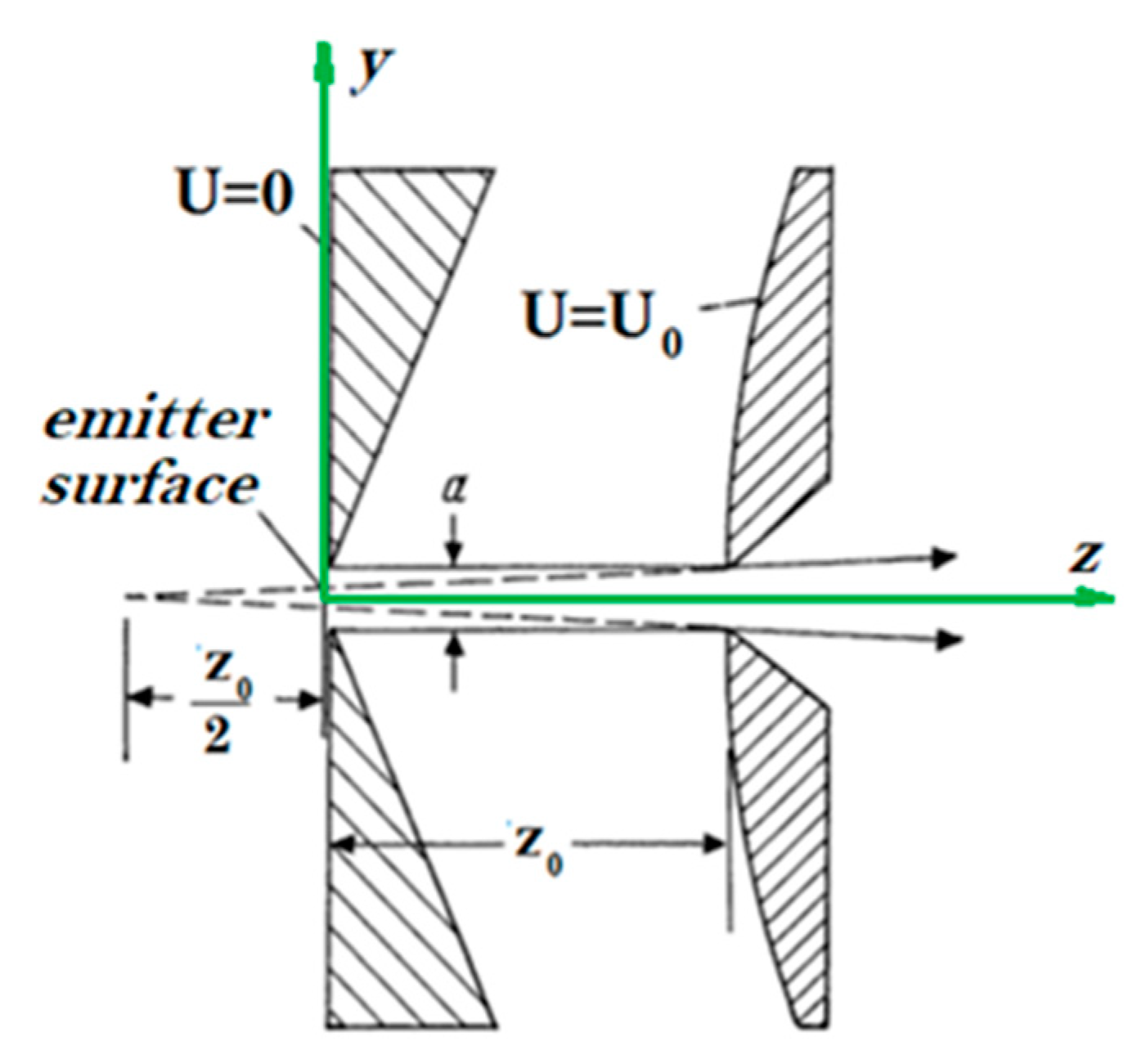
2.2. The Laminar Ion Beam Model
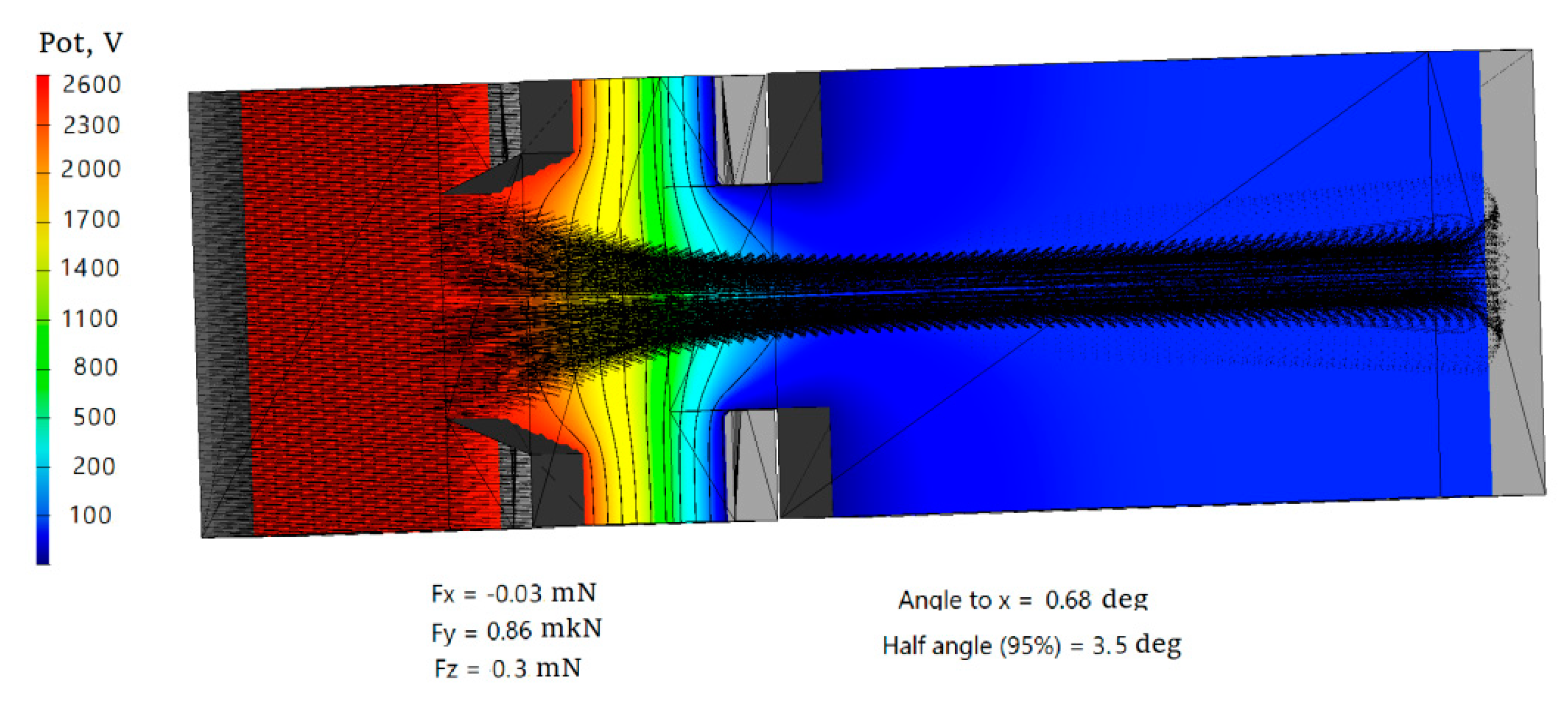
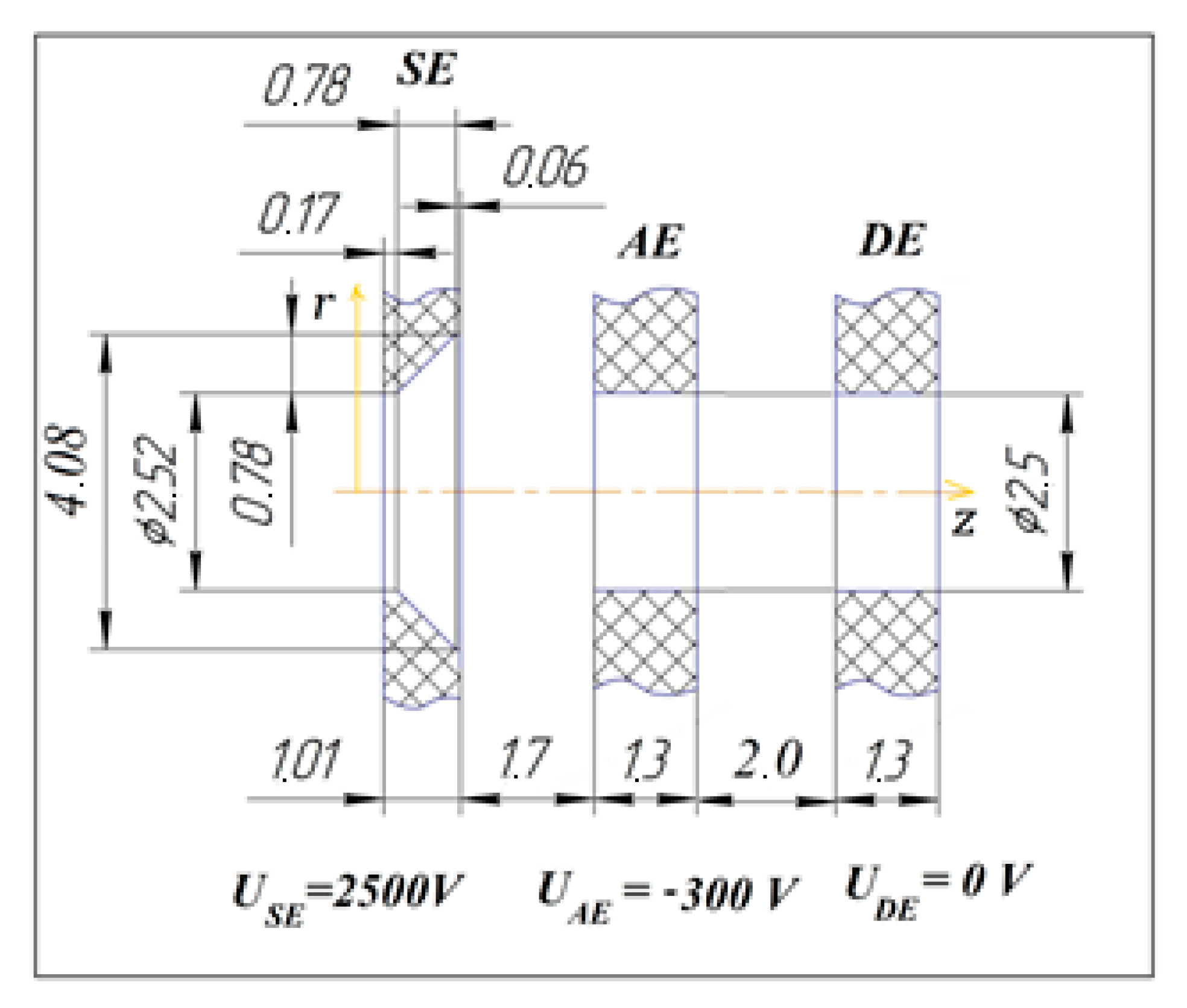
2.3. Simulation of ES with Slit-Type and Round-Type Apertures
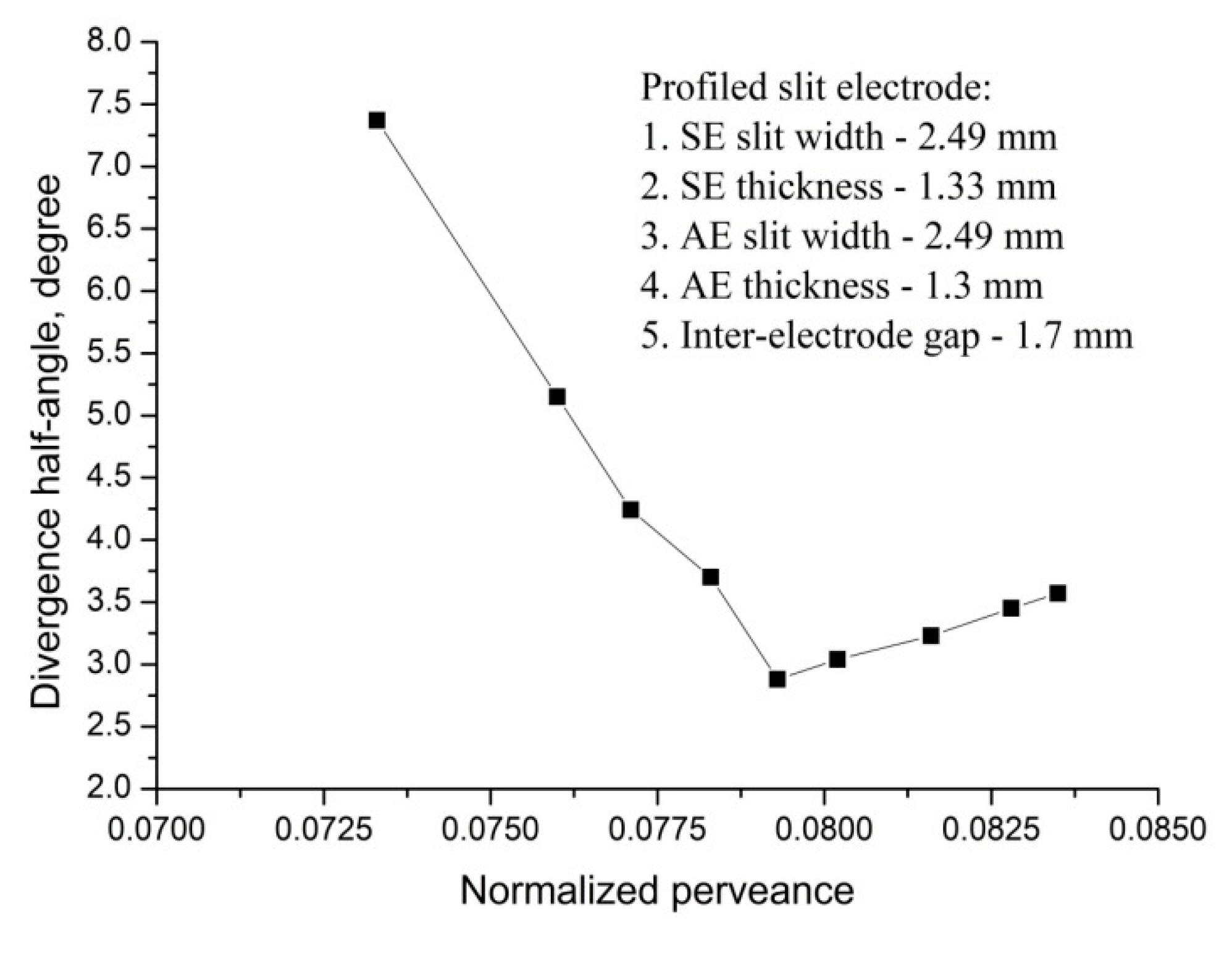
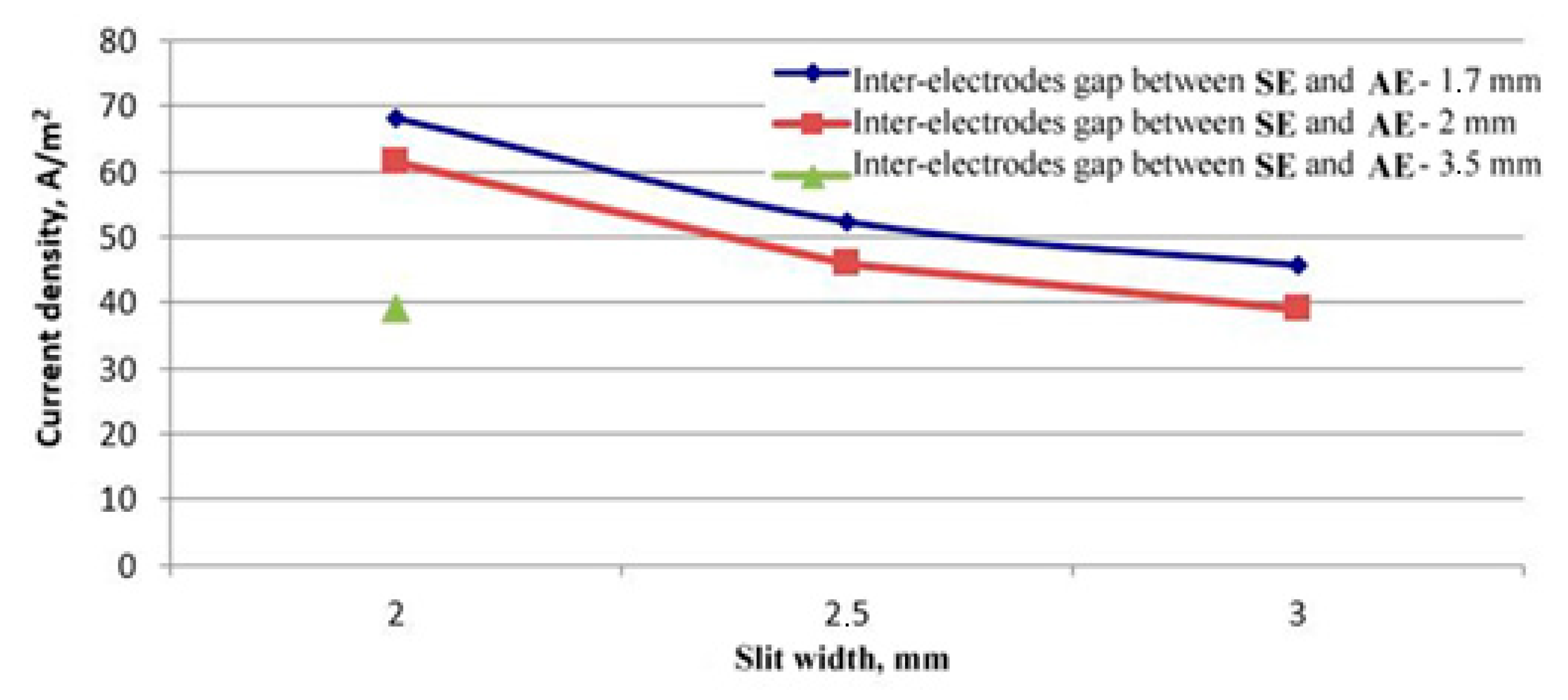
- Slit-Type ES Simulation
- b
- Experimental Results for Slit-Type ES
- c
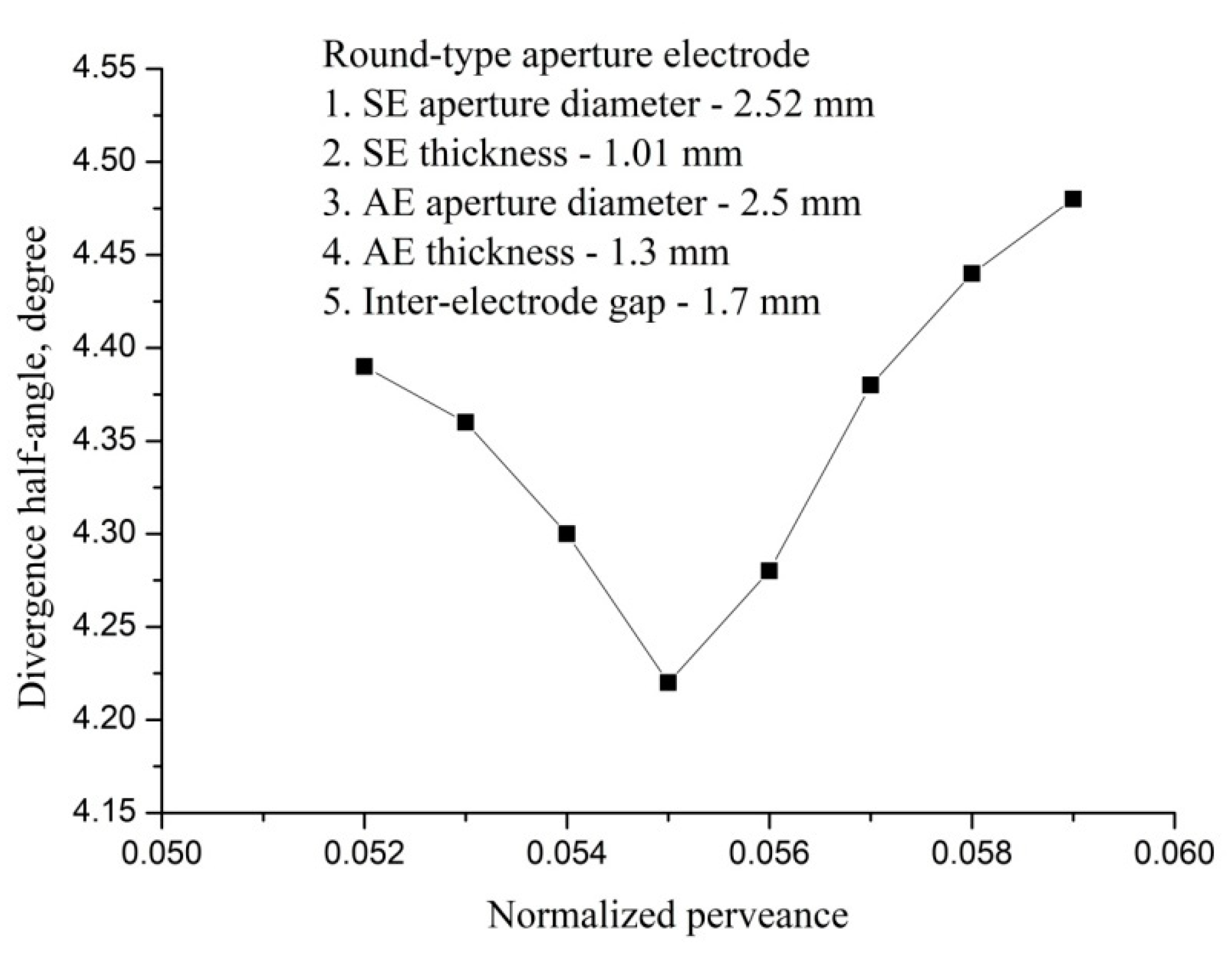
- Simulation of ES with Round Apertures
- d
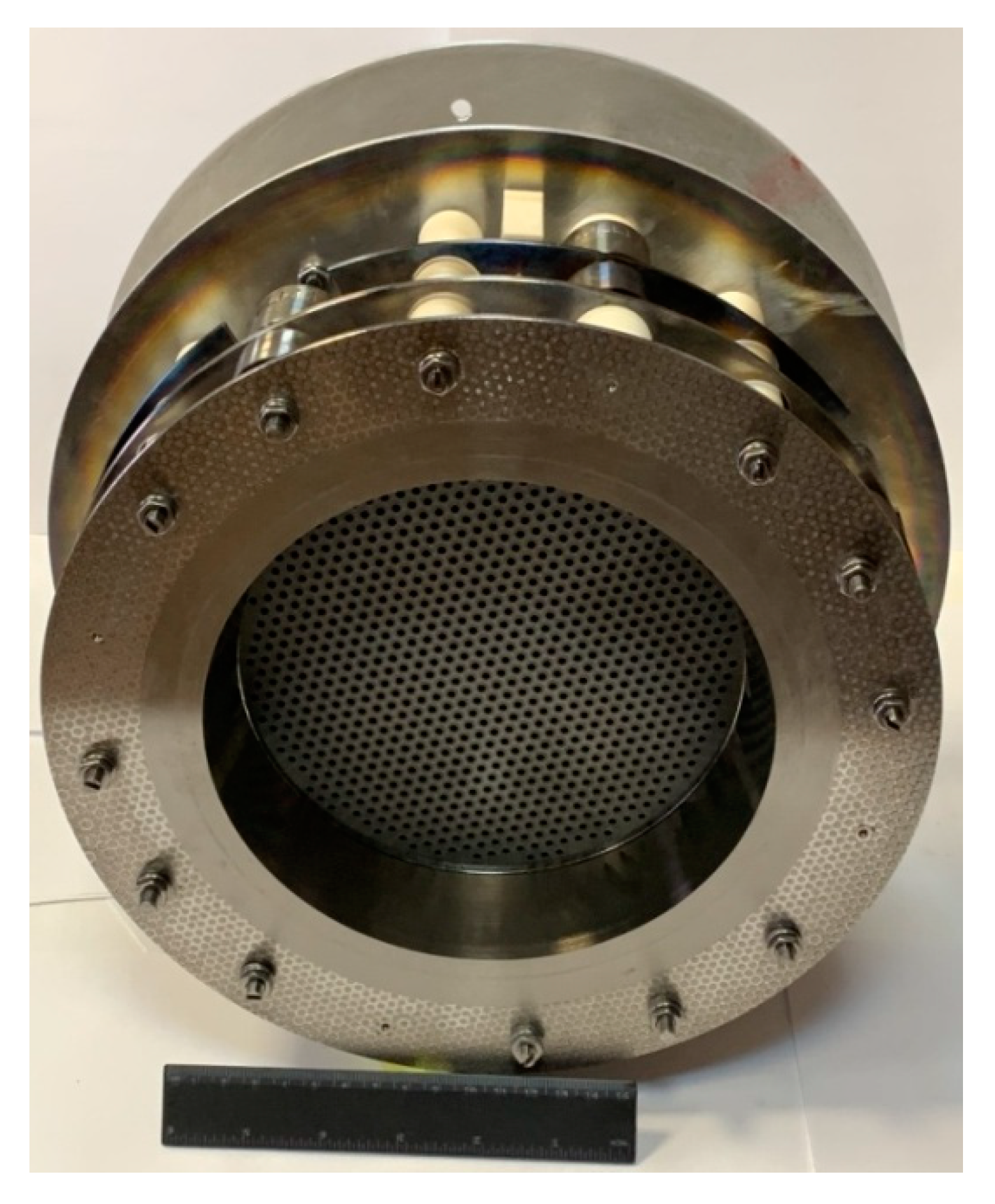
- Experimental Results for ES with Round-Type Perforation
3. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AE | accelerating electrode |
| DE | decelerating electrode |
| ES | extraction system |
| GDC | gas discharge chamber |
| GEO | geostationary orbit |
| HF | high-frequency |
| IES | ion-extraction system |
| IS | ion source |
| LEO | low Earth orbits |
| MEO | medium Earth orbits |
| RF | radio-frequency |
| RFG | radio-frequency generator |
| RIT | radio-frequency ion thruster |
| SC | spacecraft |
| SD | space debris |
| SE | screen electrode |
| SO | space object |
| SSC | service spacecraft |
Nomenclature
| Indexes | |
| AE | index of accelerating electrode parameter |
| ES | index of extraction system parameter |
| SE | index of screen electrode parameter |
| Parameters | |
| a | width of the slit-type aperture |
| b | length of the slit-type aperture |
| e | electron charge |
| IAE | current in AE circuit |
| ISE | current in SE circuit |
| Ip | beam current |
| Isp | specific impulse |
| j | ion current density |
| L | the effective length of the accelerating gap (the distance between SE and AE) |
| me | mass of electrons |
| M | propellant gas atom (ion) mass |
| P | perveance |
| Pi | injector specific thrust |
| PRF | radio-frequency generator power |
| Te | electron temperature (eV) |
| U | potential difference between SE and AE |
| UAE | potential of the acceleration electrode |
| UES | potential of the extraction system |
| USE | potential of the screen electrode |
| Vp | floating potential |
| W | ion energy |
| x | Child’s constant |
| y | axis perpendicular to the ion flow direction |
| z | coordinate measured from the plasma boundary along the ion flow |
| z0 | inter-electrode distance |
| ε0 | electrical permittivity of free space |
| normalized perveance |
References
- Debus, T.J.; Dougherty, S.P. Overview and Performance of the Front-end Robotics Enabling Near-Term Demonstration (FREND) Robotic Arm. In Proceedings of the AIAA Infotech@Aerospace Conference, AIAA 2009–1870, Seattle, WA, USA, 6–9 April 2009. [Google Scholar]
- Kaiser, C.; Sjoberg, F.; Delcura, J.M.; Eilertsen, B. SMART-OLEV—An orbital life extension vehicle for servicing commercial spacecraft in GEO. Acta Astronaut. 2008, 63, 400–410. [Google Scholar] [CrossRef]
- Melamed, N.; Griffice, C.P.; Chobotov, V. Survey of GEO Debris Removal Concepts. In Proceedings of the 59th International Astronautical Congress, IAC–08–D4.1.8, Glasgow, UK, 29 September–3 October 2008. [Google Scholar]
- Bosse, A.B.; Brands, W.J.; Brown, M.A.; Creamer, G. SUMO: Spacecraft for the Universal Modification of Orbits. In Proceedings of the SPIE—The International Society for Optical Engineering, Orlando, FL, USA, 16 June 2004. [Google Scholar] [CrossRef]
- Norman, T.L.; Gaskin, D. Conceptual Design of an Orbital Debris Defense System, NASA-CR-197211. In Proceedings of the 10th Annual Summer Conference, NASA/USRA Advanced Space Design Program, Pasadena, CA, USA, 13 June 1994; p. 64. [Google Scholar]
- DLR. Institute of Robotics and Mechatronics. Close Space Robotics Missions. ROTEX (1988–1993). Available online: http://www.dlr.de/rm/en/desktopdefault.aspx/tabid-3827/5969_read-8744/ (accessed on 10 December 2020).
- DLR. Institute of Robotics and Mechatronics. Close Space Robotics Missions. Available online: http://www.dlr.de/rm/en/desktopdefault.aspx/tabid-3827/5969_read-8750/ (accessed on 10 December 2020).
- Murdoch, N.; Izzo, D.; Bombardelli, C.; Carnelli, I.; Hilgers, A.; Rodgers, D. Electrostatic Tractor for Near Earth Object Deflection. In Proceedings of the IAC-08-A.3.1.5, 59th International Astronautical Congress, Glasgow, UK, 29 September–3 October 2008; p. 11. [Google Scholar]
- Nishida, S.; Kawamoto, S.; Okawa, Y.; Terui, F.; Kitamura, S. Space debris removal system using a small satellite. Acta Astronaut. 2009, 65, 95–102. [Google Scholar] [CrossRef]
- Kawamoto, S.; Nishida, S.; Kibe, S. Research on a Space Debris Removal System, NAL Research Progress 2002–2003; p. 84–87, ISSN 1340-5977. Available online: https://repository.exst.jaxa.jp/dspace/bitstream/a-is/26112/1/nalrp2003000.pdf (accessed on 10 December 2019).
- Kaplan, M.H. Space Debris Realities and Removal. SOSTC Improving Space Operations Workshop Spacecraft Collision Avoidance and Co–Location; The Johns Hopkins University, Applied Physics Laboratory: Baltimore, MD, USA, 2010; p. 12. [Google Scholar]
- Starke, J.; Bischof, B.; Foth, W.-O.; Gunther, J.J. Roger a Potential Orbital Space Debris Removal System. In Proceedings of the 38th COSPAR Scientific Assembly, Bremen, Germany, 15–18 July 2010; p. 2. [Google Scholar]
- Bondarenko, S.G.; Lyagushin, S.F.; Shifri, G.A. Prospects of Using Lasers and Military Space Technology for Space Debris Removal, ESA-SP 393. In Proceeding of the 2nd European Conference on Space Debris, ESOC, Darmstadt, Germany, 17–19 March 1997; pp. 703–706. [Google Scholar]
- Raikunov, G.G. (Ed.) Space debris prevention. In Space Debris; FIZMATLIT: Moscow, Russia, 2014; Volume 2, p. 188. ISBN 978-5-9221-1504-9. (In Russian) [Google Scholar]
- Brown, I.; Lane, J.; Youngquist, R. A Lunar-Based Spacecraft Propulsion Concept—The Ion Beam Sail. Acta Astronaut. 2007, 60, 834–845. [Google Scholar] [CrossRef][Green Version]
- Kitamura, S. Large Space Debris Reorbiter Using Ion Beam Irradiation. Paper IAC–10–A6.4.8. In Proceedings of the 61st International Astronautical Congress, Prague, Czech Republic, 27 September–1 October 2010. [Google Scholar]
- Bombardelli, C.; Peláez, J. Ion Beam Shepherd for Contactless Space Debris Removal. J. Guid. Control Dynamics. 2011, 34, 916–920. [Google Scholar] [CrossRef]
- Bombardelli, C.; Pelaez, J. Universidad Politecnica de Madrid. Sistema de Modificación de la Posicióny Actitud de Cuerpos en Órbita por Medio de Satélites Guía. ES Patent 2365394B2, 3 October 2011. [Google Scholar]
- NASAARES. Available online: https://orbitaldebris.jsc.nasa.gov (accessed on 10 December 2020).
- United Nations. General Assembly. Official records. 62 session. Supplement No. 20 (A/62/20). Report of the Committee on the Peaceful Uses of Outer Space. Available online: https://www.unoosa.org/pdf/gadocs/A_62_20E.pdf (accessed on 10 December 2019).
- NASA. Orbital Debris. Quarterly News. 2020.—V. 24, Is. 2. Available online: https://orbitaldebris.jsc.nasa.gov/quarterly-news/pdfs/odqnv24i2.pdf (accessed on 10 December 2020).
- Space Debris by the Numbers. ESA, January 2019. Retrieved 5 March 2019. Available online: https://www.esa.int/Safety_Security/Space_Debris/Space_debris_by_the_numbers (accessed on 10 December 2019).
- GEO Protected Region: ISON Capabilities to Provide Informational ISON Capabilities to Provide Informational Support for Tasks of Spacecraft Support for Tasks of Spacecraft Flight Safety and Space Debris Removal. 47session of the COPUOS STSC Session of the COPUOS STSC. 8–19 February 2010. Vienna. Available online: http://lfvn.astronomer.ru/report/0000059/Agapov_47sessionSTSC_2010.pdf (accessed on 10 December 2020).
- DeSelding, P.B. Space Station Required No Evasive Maneuvers in 2013 Despite Growing Debris Threat. SpaceNews. Available online: https://spacenews.com/39121space-station-required-no-evasive-maneuvers-in-2013-despite-growing-debris/ (accessed on 10 December 2020).
- Nadiradze, A.B.; Obukhov, V.A.; Popov, G.A.; Svotina, V.V. Modeling of Force Impact on Large-Sized Object of Space Debris by Ion Injection, IEPC-2015-66/ISTS-2015-b-66. In Proceedings of the Joint Conference of 30 ISTS, 34 IEPC, 6 NSAT, Kobe, Japan, 4–10 July 2015; p. 8. [Google Scholar]
- Balashov, V.V.; Cherkasova, M.V.; Kudryavtsev, A.V.; Masherov, P.E.; Mogulkin, A.I.; Obukhov, V.A.; Svotina, V.V.; Riaby, V.A.; Usovik, I.V. Radio Frequency Source of Weakly Expanding Wage-Shaped Xenon Ion Beam for Contactless Removal of Large-Sized Space Debris Objects. In Proceedings of the 7 European Conference on Space Debris, Darmstadt, Germany, 18–21 April 2017. [Google Scholar]
- Obukhov, V.A.; Pokryshkin, A.I.; Popov, G.A.; Svotina, V.V. Problems of the Method of Space Debris Objects Withdrawal by an Ion Beam. In Proceedings of the 7th Russian-German Conference on Electric Propulsion and Their Applications Electric Propulsion—New Challenges, Marburg, Germany, 21–26 October 2018. [Google Scholar]
- Certificate of state registration of the computer program No. 2018662928. In «Program for Simulating the Control of a Service Spacecraft with Non-Contact Means of Impact on Objects of Space Debris in the Process of Removal from the Protected Area of the Geostationary orbit. Disposeeso»/A.I. Pokryshkin (Russian Federation); Moscow Aviation Institute (National Research University): Moscow, Russia, 2018. (In Russian)
- Obukhov, V.A.; Kirillov, V.A.; Petukhov, V.G.; Popov, G.A.; Svotina, V.V.; Testoedov, N.A.; Usovik, I.V. Problematic issues of spacecraft development for contactless removal of space debris by ion beam. Act. Astronaut. 2021, 181, 569–578. [Google Scholar] [CrossRef]
- Langmuir, I. The Effect of Space Charge and Residual Gases on Thermionic Currents in High Vacuum. Phys. Rev. J. 1913, 2, 450–486. [Google Scholar] [CrossRef]
- Pierce, J.R. Theory and Design of Electron Beams; Van Nostrand: New York, NY, USA, 1954; p. 222. ISBN 978-1124091303. [Google Scholar]
- Forrester, A.T. Large Ion Beams, Fundamentals of Generation and Propagation, 1st ed.; John Wiley and Sons Inc.: Hoboken, NJ, USA, 1987; 325p, ISBN -0-471-62557-4. [Google Scholar]
- Bundesmann, C.; Tartz, M.; Scholze, F.; Neumann, H.; Leiter, H.J.; Scortecci, F.; Feili, D. In-Situ Temperature, Grid Curvature, Erosion, Beam and Plasma Characterization of a Gridded Ion Thruster RIT-22, IEPC-2009-160. In Proceedings of the 31st International Electric Propulsion Conference, Ann Arbor, MI, USA, 20–24 September 2009; p. 10. [Google Scholar]
- Snyder, J.; Goebel, D.M.; Hofer, R.R.; Polk, J.E.; Wallace, N.C.; Simpson, H. Performance Evaluation of T6 Ion Engine. J. Propuls. Power 2012, 28, 371–379. [Google Scholar] [CrossRef]
- Cooper, W.S.; Berkner, K.H.; Pyle, R.V. Multiple-Aperture Extractor Design for Producing Intense Ion and Neutral Beams. Nucl. Fusion 1972, 12, 263–265. [Google Scholar] [CrossRef]
- Obukhov, V.A.; Sosnovsky, V.E. Calculation of the transition layer in the emission hole of a gas-discharge ion source. In Proceedings of the 5th All-union Conference on Plasma Accelerators and Ion Injectors, Moscow, Russia, 19–22 October 1982; pp. 105–106. (In Russian). [Google Scholar]
- Becker, R. Modeling of Extraction of Positive Ions from Plasma IGUN (1997–2011). Available online: www.egun_igun.com (accessed on 20 December 2019).
- Certificate of State Registration of the Computer Program No. 2014610277. In «Modeling of Charged Particle Flows in Ion-Optical Systems of Ion Thrusters (IOS-3D)»/A.A. Shagayda (Russian Federation); Keldysh Research Center: Moscow, Russia, 20 April 2014. (In Russian)





| Parameter | Value (Test 1) | Value (Test 2) |
|---|---|---|
| AE slit width, mm | 2.49 | 2.49 |
| SE slit width, mm | 2.49 | 2.49 |
| Inter-electrode gap, mm | 1.7 | 1.7 |
| SE thickness, mm | 1.33 | 1.33 |
| AE thickness, mm | 1.3 | 1.3 |
| SE potential, V | 2500 | 3000 |
| AE potential, V | –200 | –300 |
| Measured Parameters | Calculated, Based on the Experiment | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Mass flow rate, mg/s | PRF, W | USE, V | ISE, A | UAE, V | IAE, A | Isp, sec | Ip, A | Pi, mN | W, keV |
| 0.771 | 220 | 2500 | 0.190 | 200 | 0.001 | 2083 | 0.18 | 16.0 | 2.50 |
| 0.947 | 235 | 3000 | 0.280 | 300 | 0.010 | 2737 | 0.27 | 25.97 | 3.00 |
| Parameter | Experimental Result | Simulation Result |
|---|---|---|
| SE potential, V | 2500 | 2500 |
| AE potential, V | –200 | –200 |
| Ion current, A | 0.19 | 0.15 |
| Perveance, A/kV3/2 | 0.043 | 0.034 |
| Inter-electrode electric field intensity, kV/mm | 1.59 | 1.59 |
| SE potential, V | 3000 | 3000 |
| AE potential, V | –300 | –300 |
| Ion current, A | 0.28 | 0.21 |
| Perveance, A/kV3/2 | 0.047 | 0.035 |
| Inter-electrode electric field intensity, kV/mm | 1.94 | 1.94 |
| Measured Parameters | Calculated, Based on the Experiment | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Mass flow rate, mg/s | PRF, W | USE, V | ISE, A | UAE, V | IAE, A | Isp, s | Ip, A | Pi, mN | W, keV |
| 0.561 | 230 | 2500 | 0.204 | 300 | 0.0050 | 3073 | 0.204 | 17.4 | 2.50 |
| 0.732 | 270 | 3000 | 0.285 | 300 | 0.0075 | 3604 | 0.285 | 26.4 | 3.00 |
| Parameter | Experimental Result | Simulation Result |
|---|---|---|
| SE potential, V | 2500 | 2500 |
| AE potential, V | –300 | –300 |
| Ion current, A | 0.204 | 0.210 |
| Perveance, A/kV3/2 | 0.044 | 0.045 |
| Inter-electrode electric field intensity, kV/mm | 1.65 | 1.65 |
| SE potential, V | 3000 | 3000 |
| AE potential, V | –300 | –300 |
| Ion current, A | 0.285 | 0.245 |
| Perveance, A/kV3/2 | 0.048 | 0.041 |
| Inter-electrode electric field intensity, kV/mm | 1.94 | 1.94 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Svotina, V.V.; Cherkasova, M.V.; Mogulkin, A.I.; Melnikov, A.V.; Peysakhovich, O.D. Ion Source—Mathematical Simulation Results versus Experimental Data. Aerospace 2021, 8, 276. https://doi.org/10.3390/aerospace8100276
Svotina VV, Cherkasova MV, Mogulkin AI, Melnikov AV, Peysakhovich OD. Ion Source—Mathematical Simulation Results versus Experimental Data. Aerospace. 2021; 8(10):276. https://doi.org/10.3390/aerospace8100276
Chicago/Turabian StyleSvotina, Victoria V., Maria V. Cherkasova, Andrey I. Mogulkin, Andrey V. Melnikov, and Oleg D. Peysakhovich. 2021. "Ion Source—Mathematical Simulation Results versus Experimental Data" Aerospace 8, no. 10: 276. https://doi.org/10.3390/aerospace8100276
APA StyleSvotina, V. V., Cherkasova, M. V., Mogulkin, A. I., Melnikov, A. V., & Peysakhovich, O. D. (2021). Ion Source—Mathematical Simulation Results versus Experimental Data. Aerospace, 8(10), 276. https://doi.org/10.3390/aerospace8100276







