Optimal Attitude Maneuvering Analyses for Imaging at Squint Staring and Sliding Spotlight Modes of SAR Satellite
Abstract
:1. Introduction
2. Analysis of Attitude Maneuvering and Maneuver Time of Squint SAR Satellite Spotlight Mode
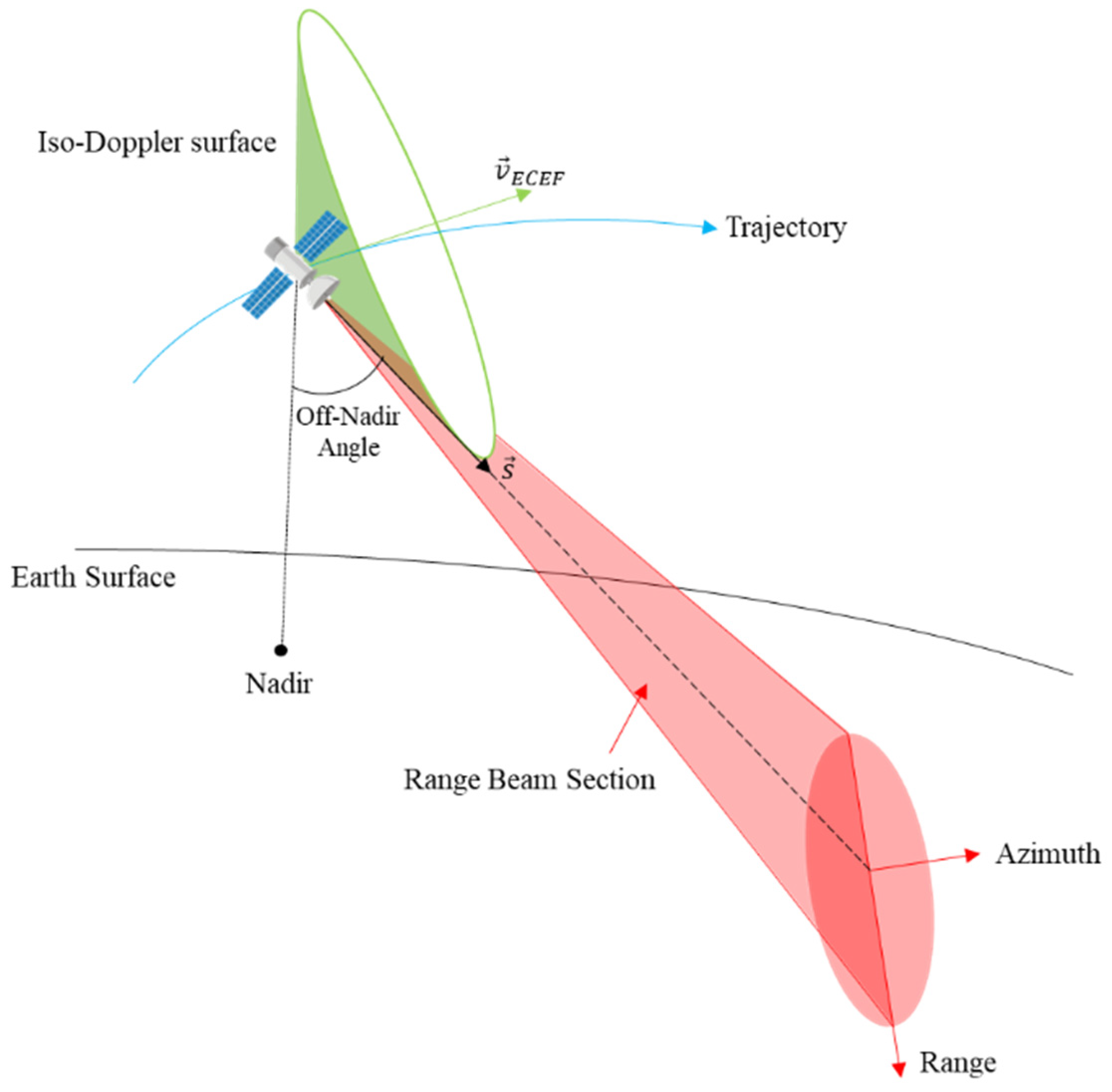
2.1. Attitude Maneuvering Problem in Squint Spotlight Mode
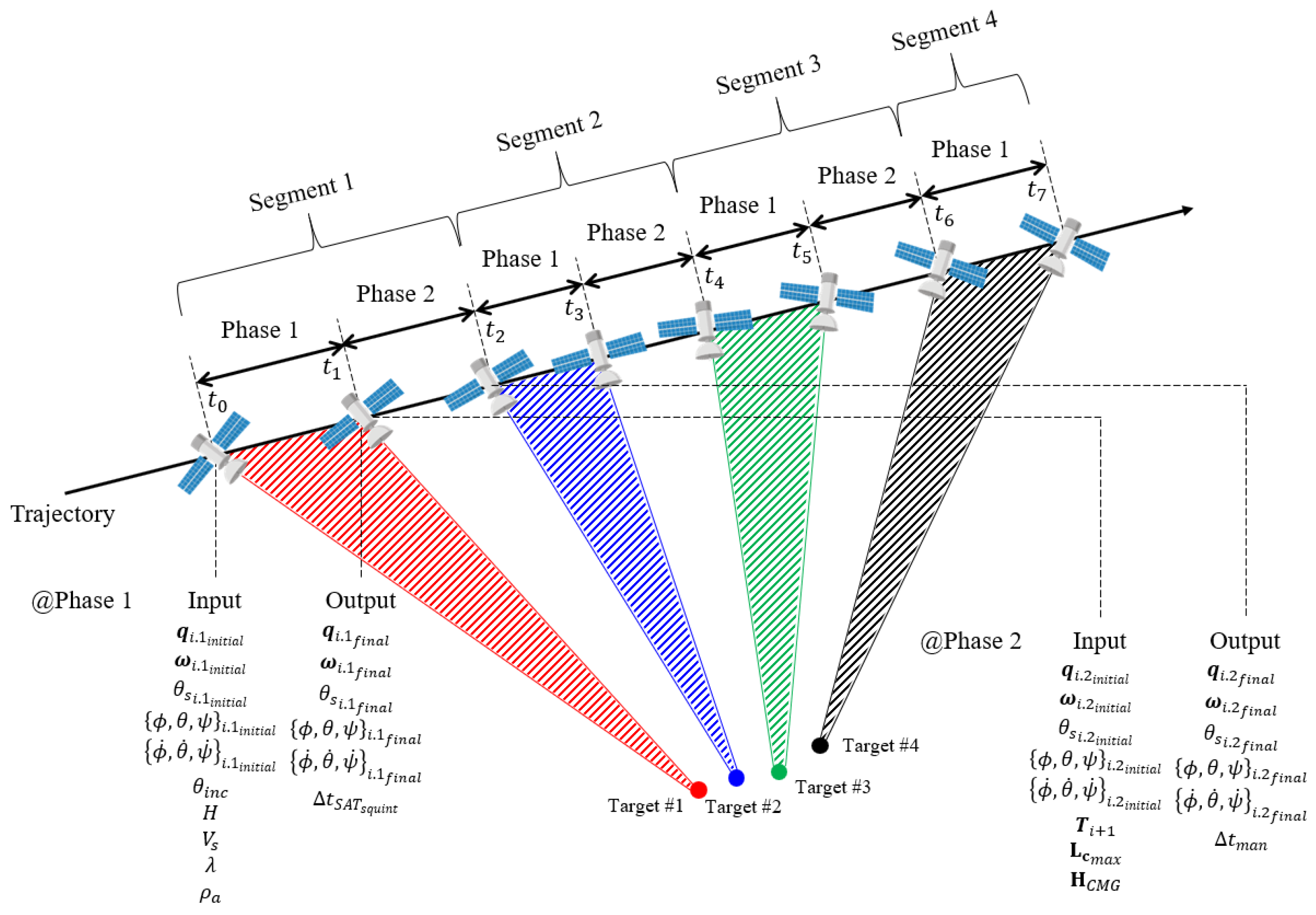
2.2. Timeline Elements of Mission Operation in Spotlight Mode
2.3. Synthetic Aperture Time and Attitude Maneuvering during Imaging (Phase 1)
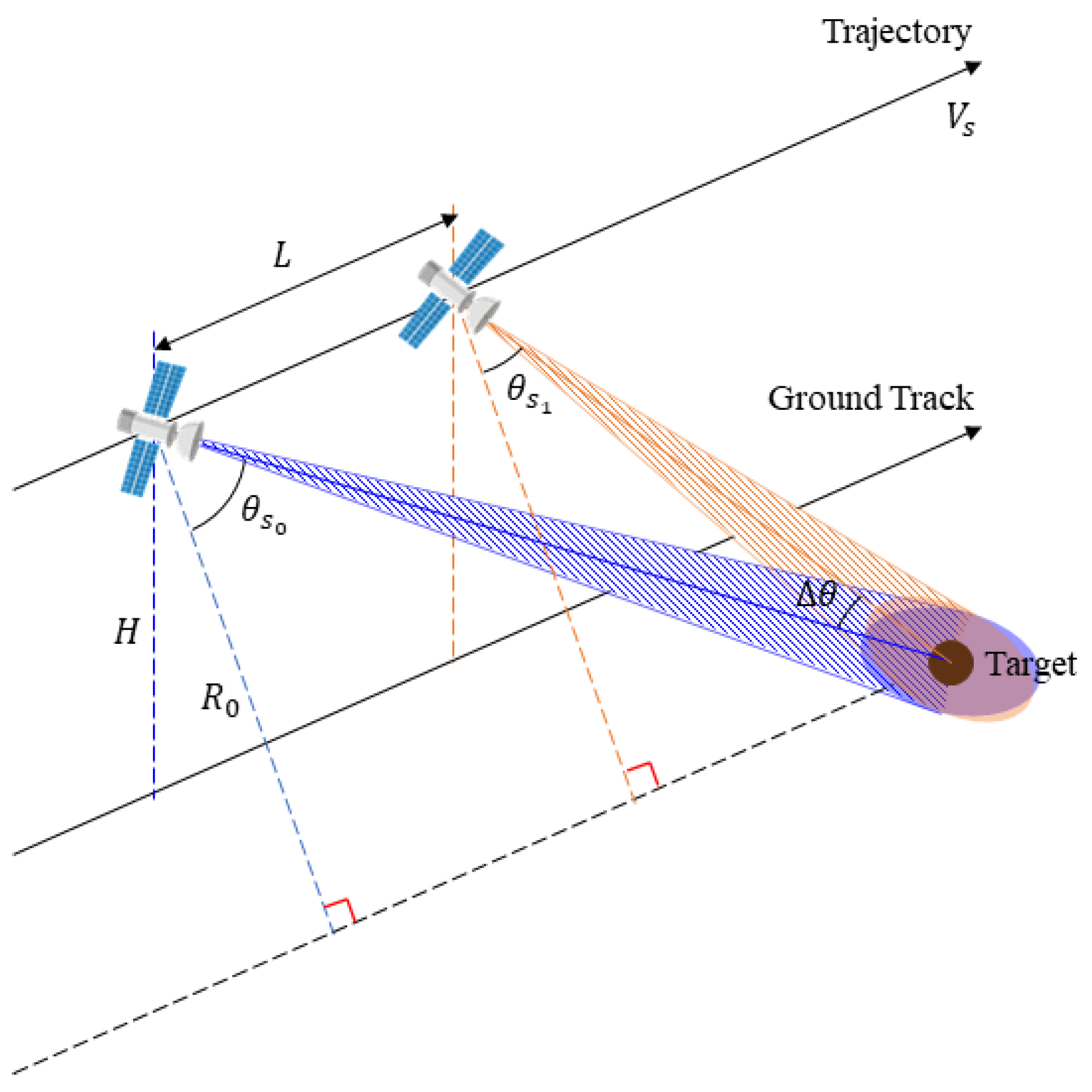
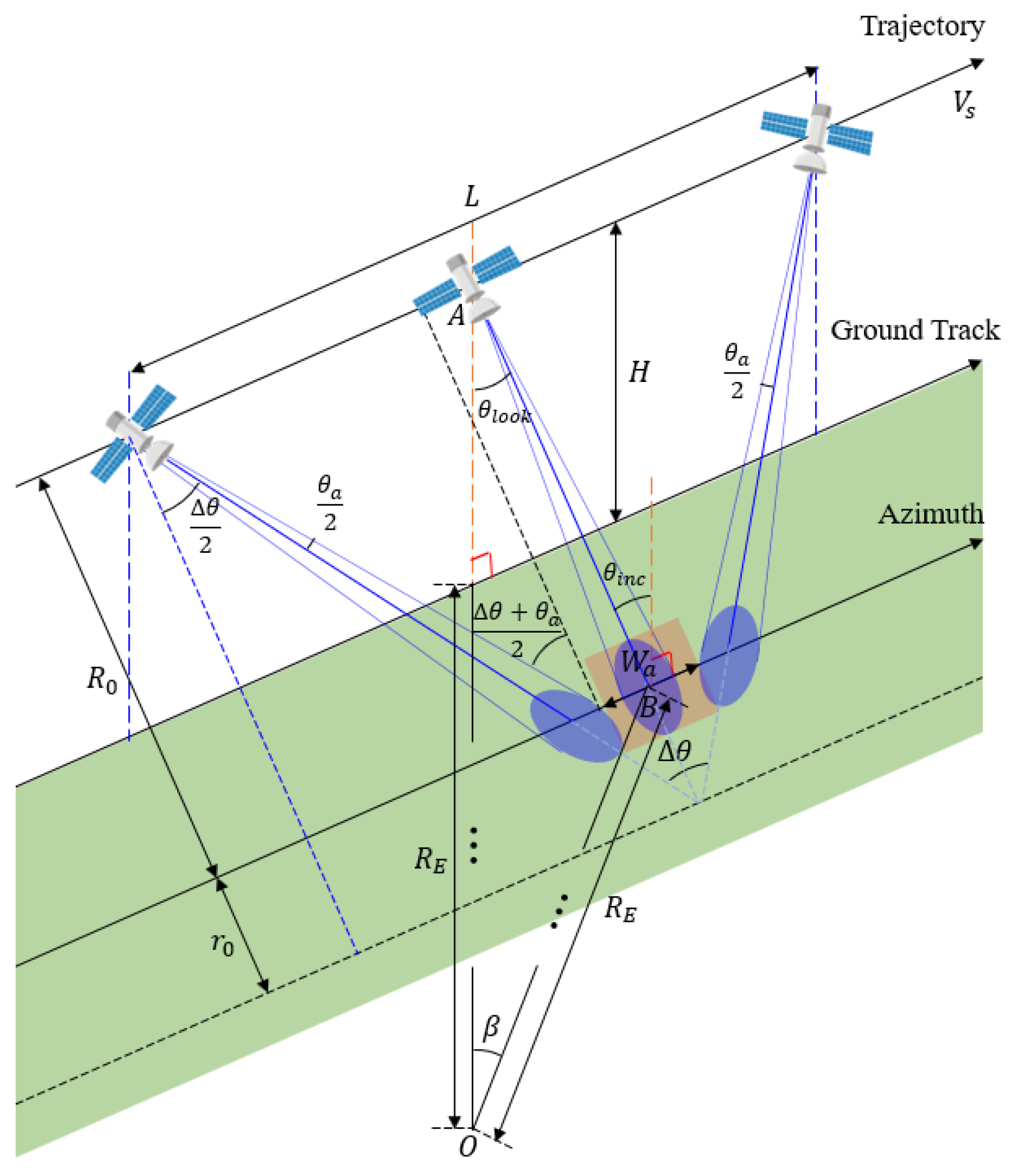
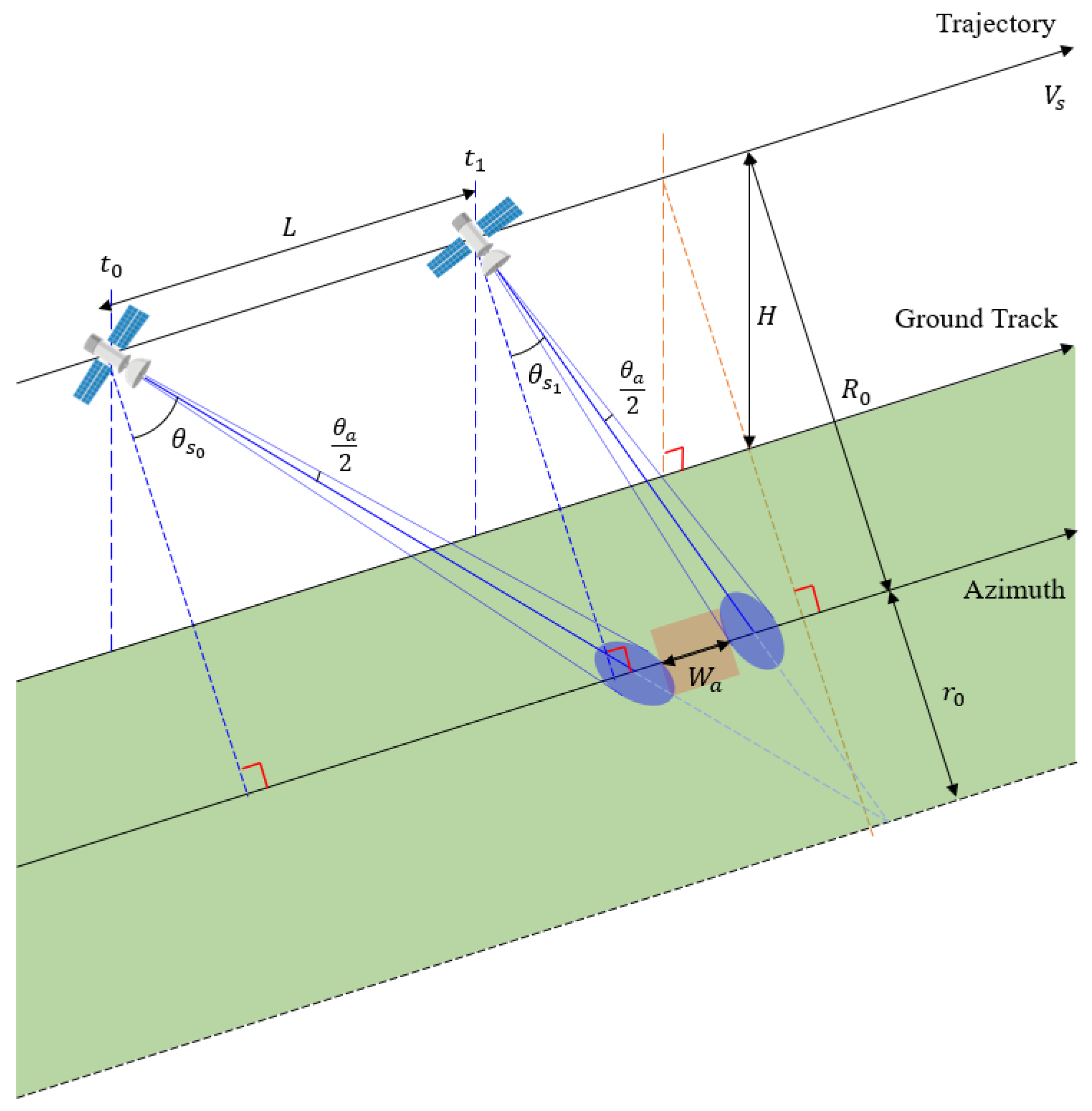
2.3.1. Synthetic Aperture Time in Broadside and Squint Staring Spotlight Modes
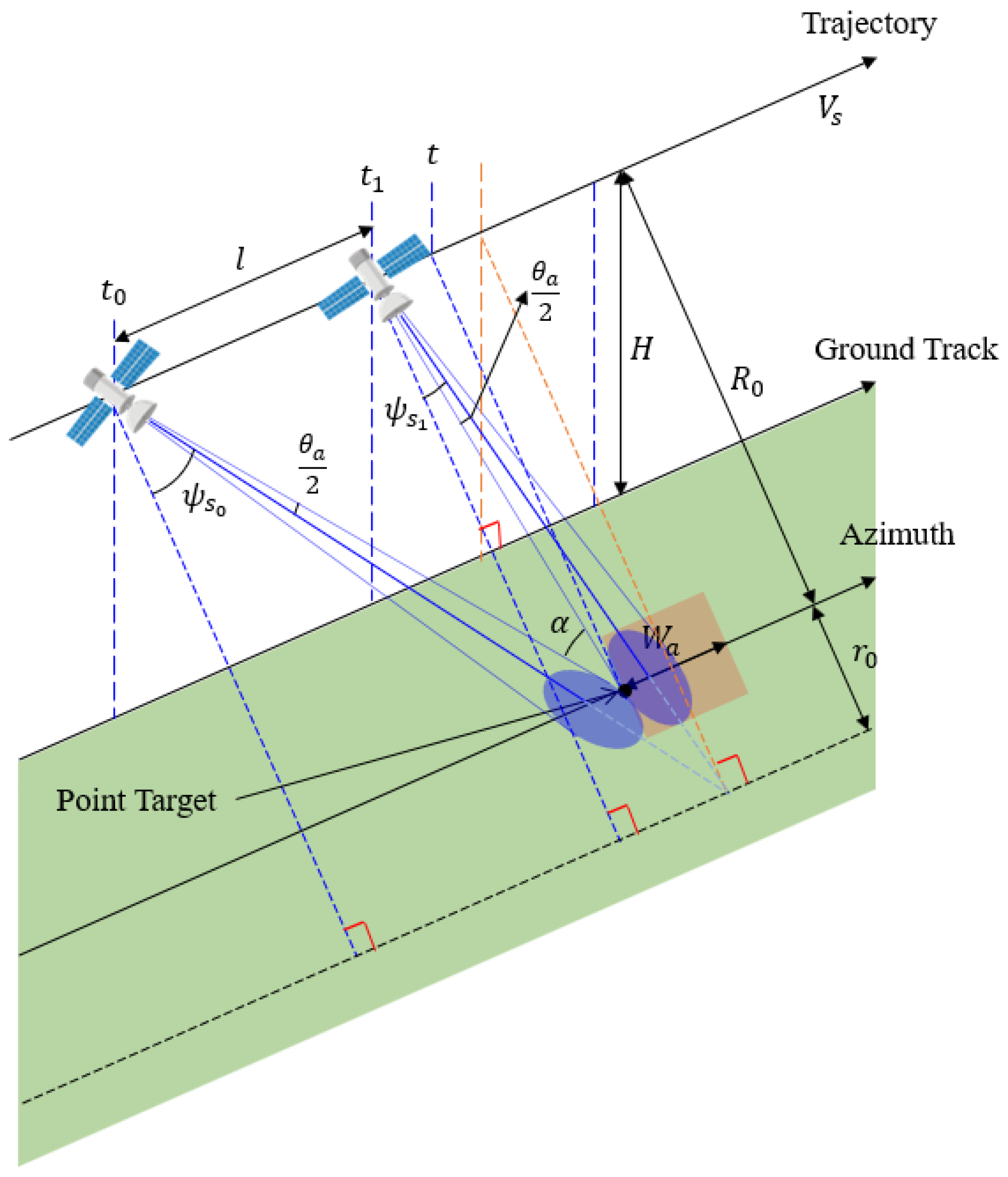
2.3.2. Synthetic Aperture Time in Broadside and Squint Sliding Spotlight Mode
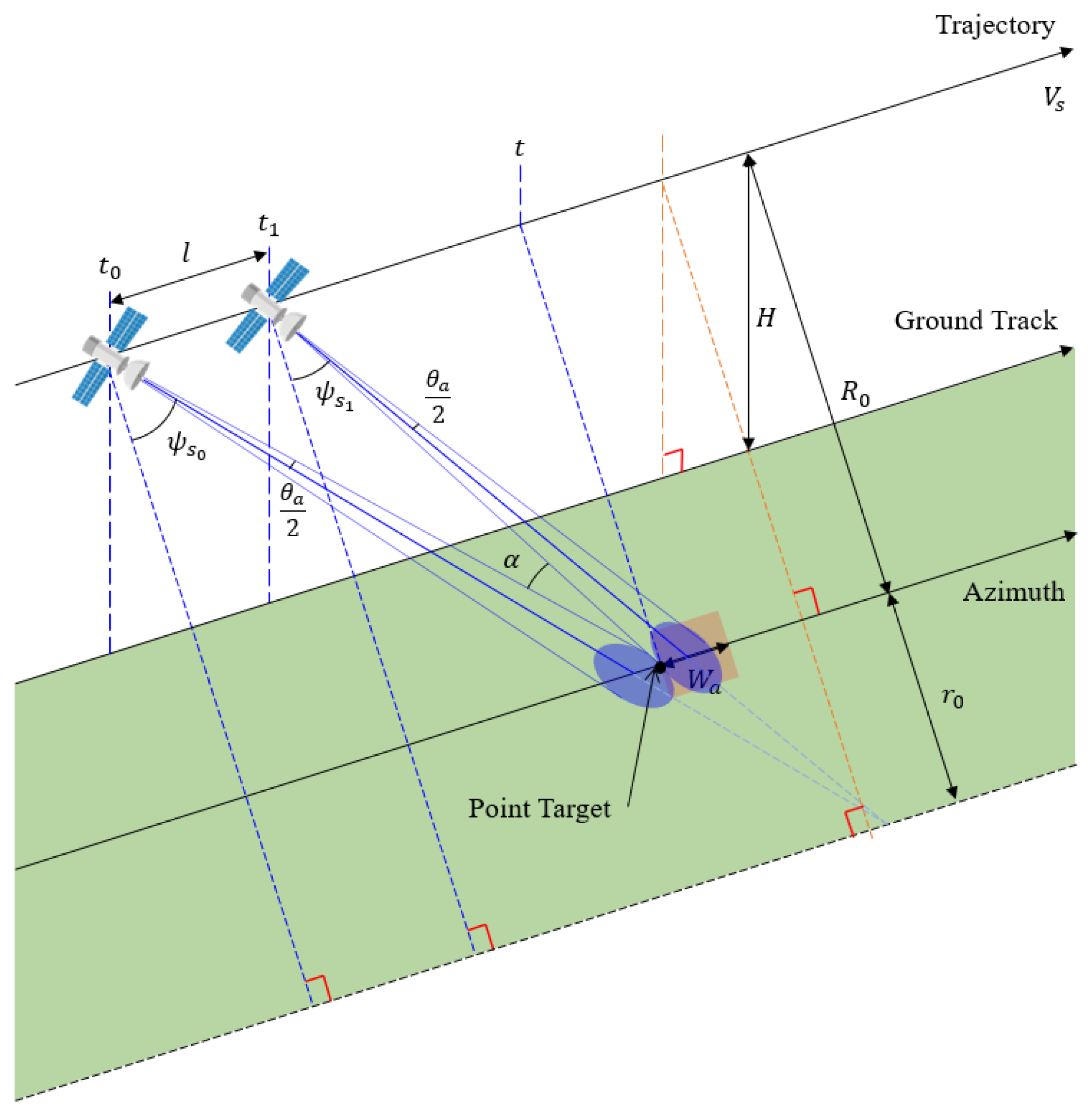
2.3.3. Attitude Maneuvering for Imaging in Squint Staring and Squint Sliding Spotlight Modes
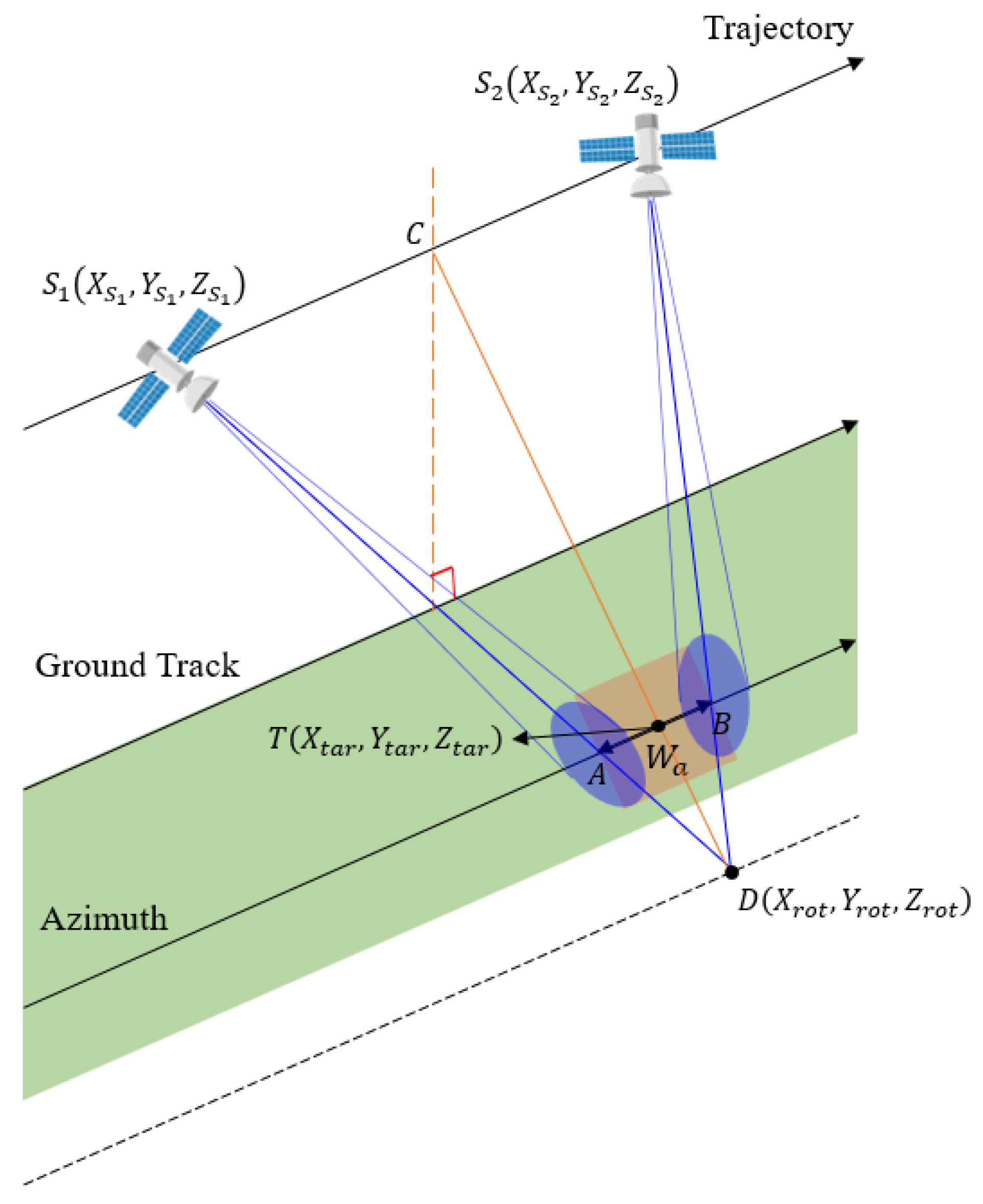
2.4. Retargeting Maneuver and Optimal Retargeting Maneuver Time in Spotlight Mode (Phase 2)
2.4.1. Analysis of Optimal Retargeting Maneuver
2.4.2. Analysis of Optimal Maneuvering Time in the Retargeting Maneuver
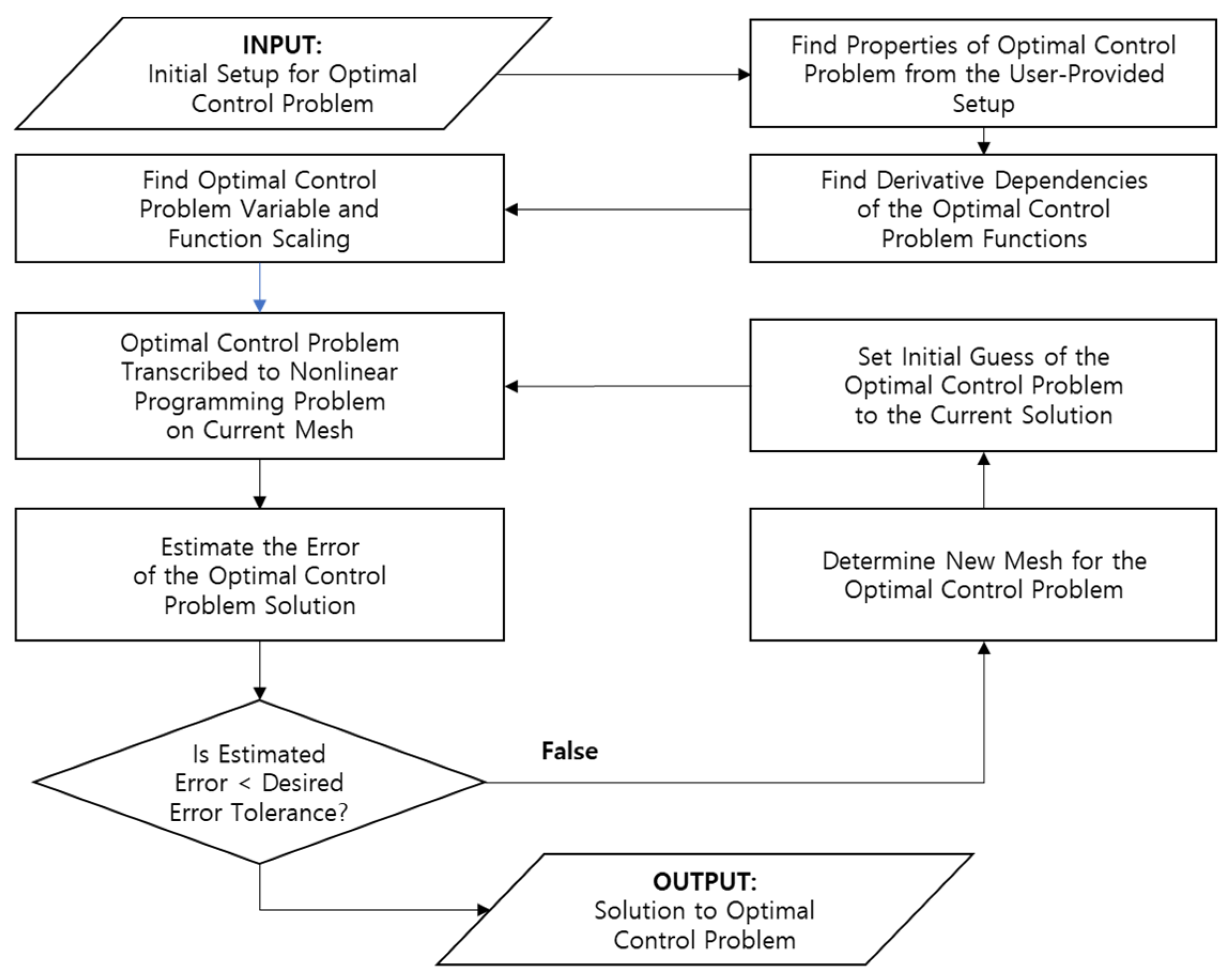
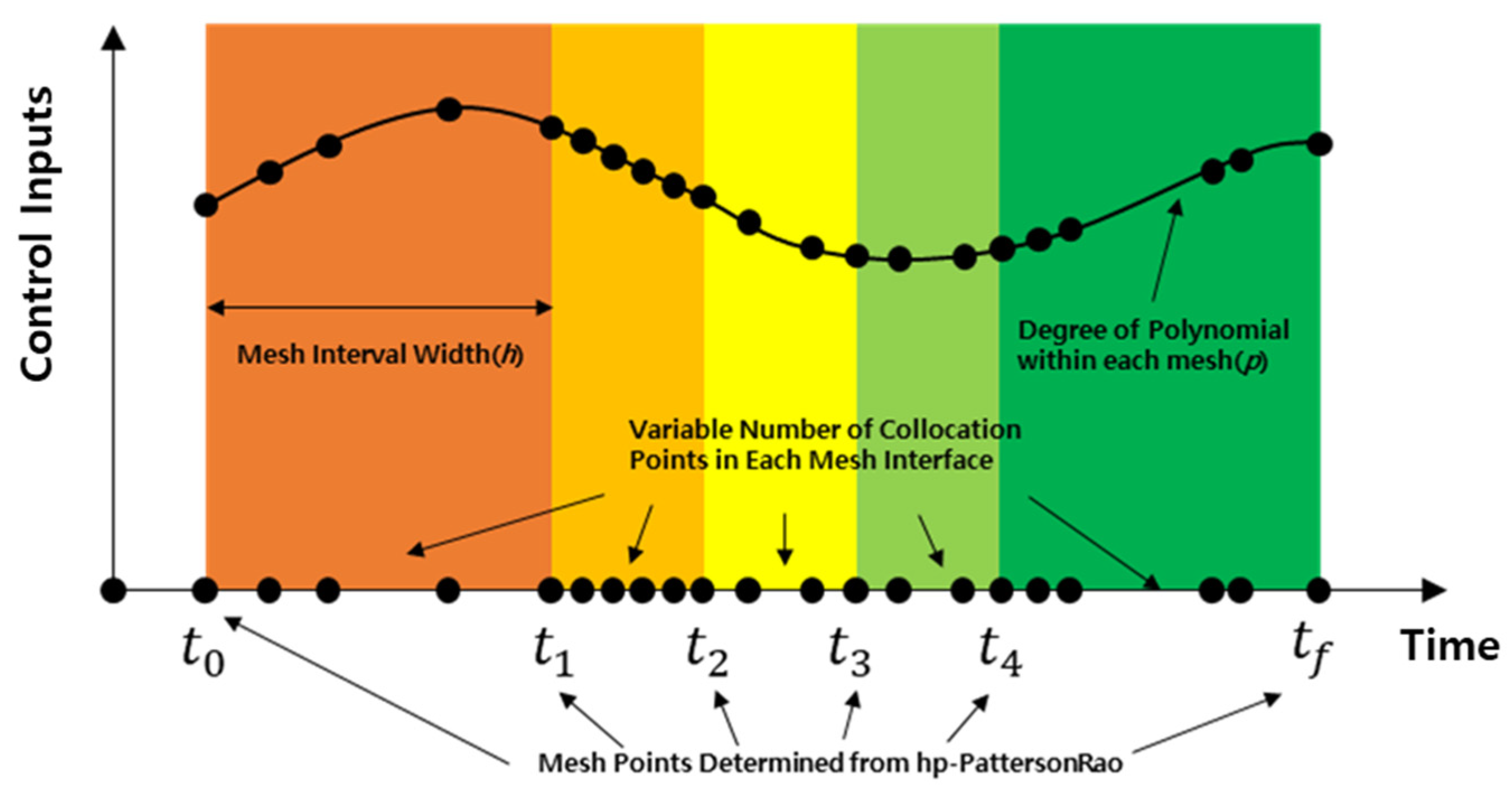
2.4.3. Optimization Algorithm of the Retargeting Maneuver
- -
- The cost function (i.e., performance index).
- -
- The continuous function (i.e., differential equations based on dynamics).
- -
- The time at the start and terminus of a phase.
- -
- The state at the start of a phase, during a phase, and at the terminus of a phase.
- -
- The control during a phase.
- -
- The path constraints.
- -
- The event constraints.
- -
- The static parameters.
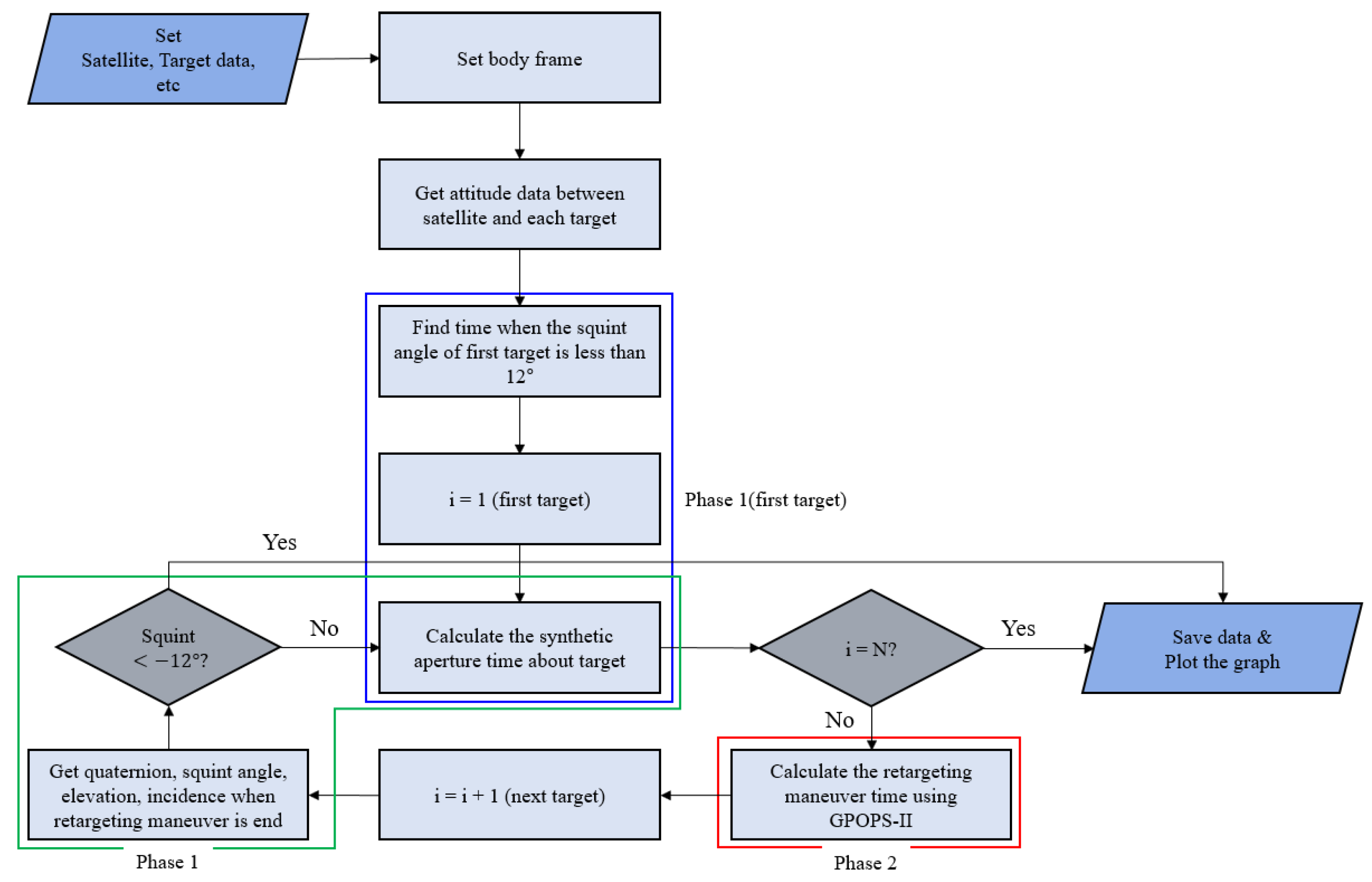
2.5. Development of Algorithm for Imaging Continuous Targets
3. Simulation and Results of Target Imaging
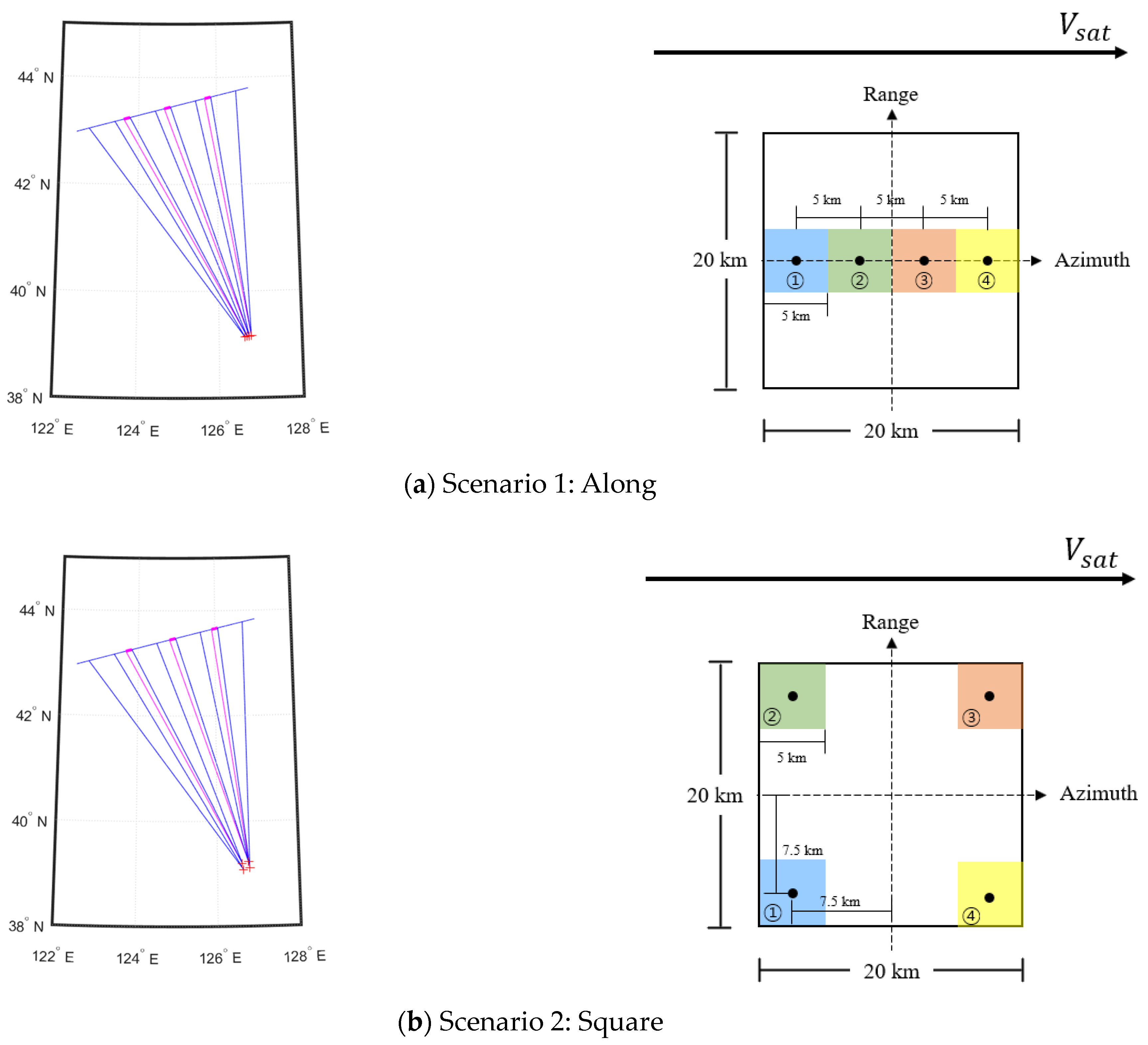
3.1. Targets Arrangement Scenario and Simulation Parameters
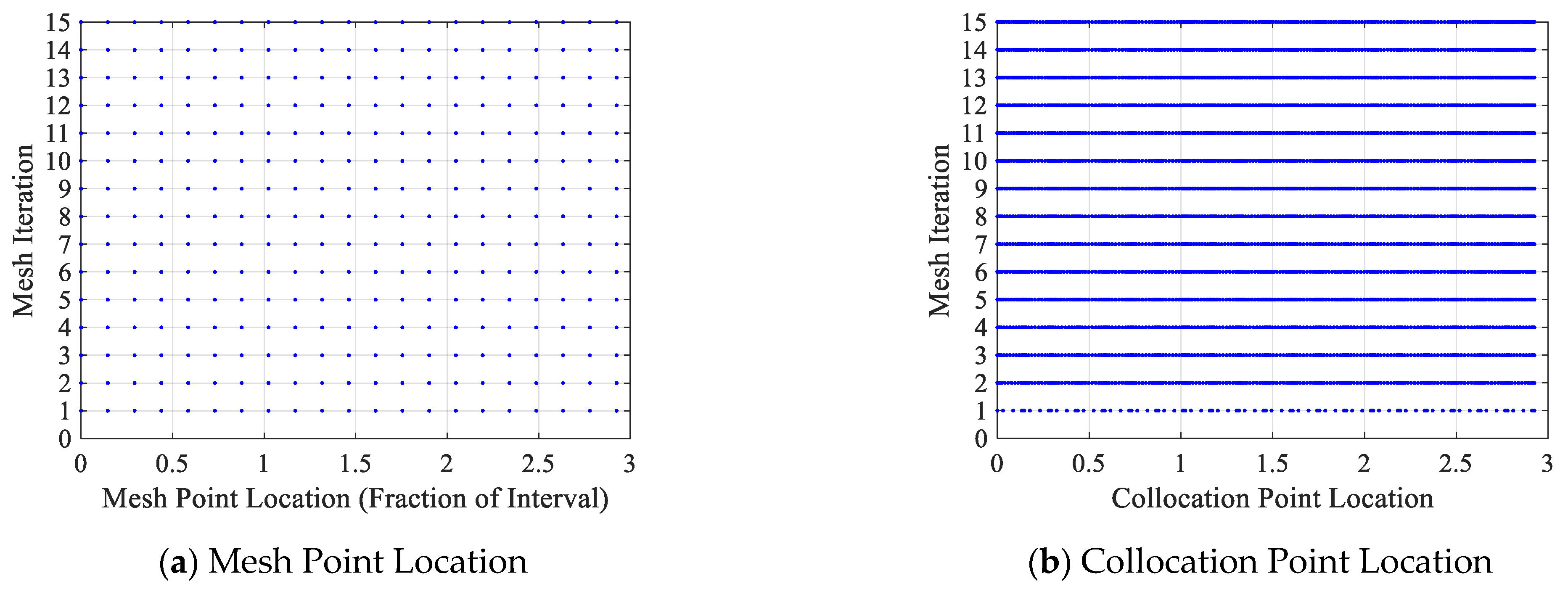
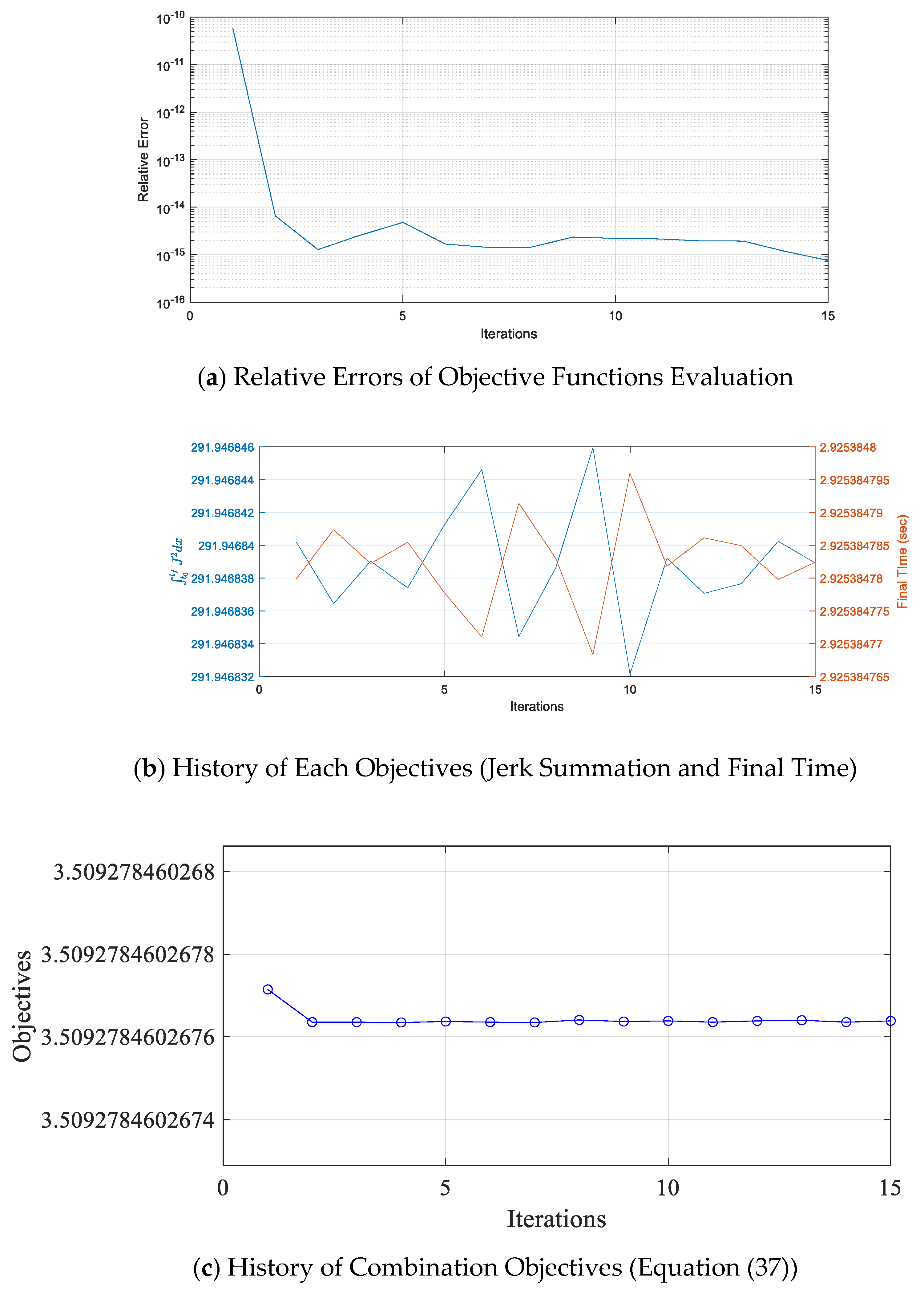
3.2. Verification of Optimization Algorithm
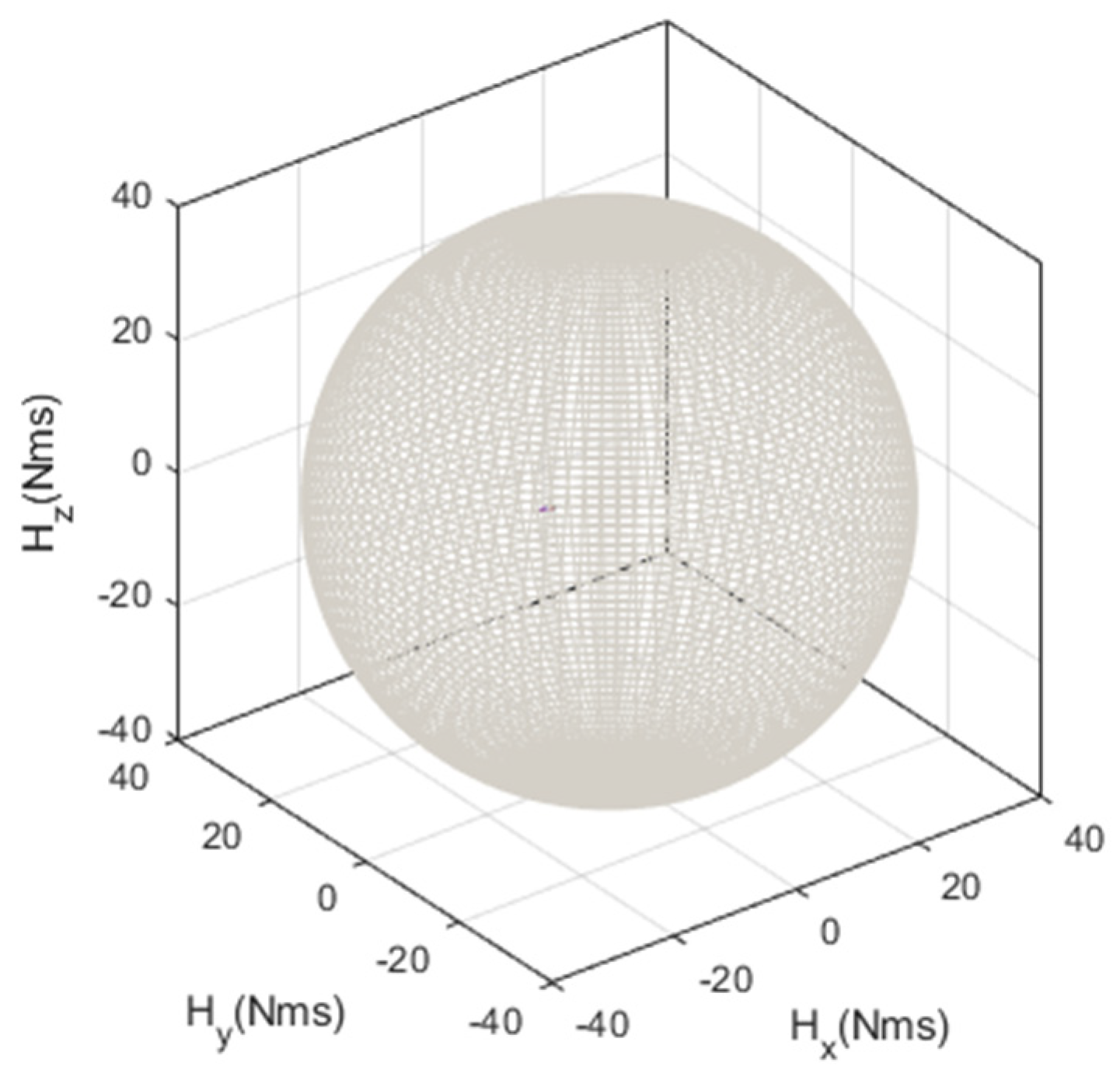
3.3. Results of Simulation Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Abbreviations | |
| AoI | Area of interest |
| CMG | Control moment gyro |
| DCM | Direction Cosine Matrix |
| ECEF | Earth center Earth fixed |
| GPOPS-II | General purpose optimal control software-II |
| LVLH | Local vertical local horizontal |
| MoI | Moment of inertia |
| PoI | Product of inertia |
| RMT | Retargeting maneuver time |
| SAA | Synthetic aperture angle |
| SAL | Synthetic aperture length |
| SAR | Synthetic aperture radar |
| SAT | Synthetic aperture time |
| TPBVP | Two points boundary value problem |
| Notation | |
| Quaternion of satellite | |
| Angular velocity of satellite [rad/sec] | |
| , | Initial & final quaternions of -th target during imaging maneuver, respectively |
| , | Initial & final angular velocity of -th target during imaging maneuver, respectively [rad/sec] |
| , | Initial & final squint angle of -th target during imaging maneuver, respectively |
| Initial roll/pitch/yaw angle of -th target during imaging maneuver [deg] | |
| Final roll/pitch/yaw angle of -th target during imaging maneuver [deg] | |
| Initial roll/pitch/yaw rate of -th target during imaging maneuver [rad/sec] | |
| Final roll/pitch/yaw rate of -th target during imaging maneuver [rad/sec] | |
| , | Initial & final quaternion of -th target during retargeting maneuver, respectively |
| , | Initial & final angular velocity of -th target during retargeting maneuver, respectively [rad/sec] |
| , | Initial & final squint angle of -th target during retargeting maneuver, respectively [deg] |
| Initial roll/pitch/yaw angle of -th target during retargeting maneuver [deg] | |
| Final roll/pitch/yaw angle of -th target during retargeting maneuver [deg] | |
| Initial roll/pitch/yaw rate of -th target during retargeting maneuver [rad/sec] | |
| Final roll/pitch/yaw rate of -th target during retargeting maneuver[rad/sec] | |
| Position of -th target at sliding spotlight mode [Latitude, Longitude] | |
| Position of satellite at point A in ECEF frame [km] | |
| Moment of inertia [kg m2] | |
| Torque of CMG [ Nm] | |
| Max torque of CMG [Nm] | |
| Max angular momentum of CMG [Nms] | |
| Jerk of CMG [rad/sec3] | |
| Synthetic aperture time at broadside staring spotlight mode [sec] | |
| Synthetic aperture time at squint staring spotlight mode [sec] | |
| Synthetic aperture time at broadside sliding spotlight mode [sec] | |
| Synthetic aperture time at squint sliding spotlight mode [sec] | |
| Retargeting maneuver time [sec] | |
| Stabilization time [sec] | |
| Slant range at aperture center [km] | |
| Radius of Earth [km] | |
| Slant range from the rotation center to target area center at aperture center [km] | |
| Synthetic aperture angle [deg] | |
| Incidence angle at aperture center [deg] | |
| Look angle at aperture center [deg] | |
| , | Starting & ending squint angles of image-taking at staring spotlight mode [deg] |
| Antenna beamwidth [deg] | |
| , | Starting & ending squint angles of image-taking at sliding spotlight mode [deg] |
| Earth central angle [deg] | |
| Latitude of satellite [deg] | |
| Longitude of satellite [deg] | |
| Synthetic aperture length [km] | |
| Swath width of target area[km] | |
| Altitude of satellite [km] | |
| Velocity of satellite [km/sec] | |
| Azimuth resolution [m] | |
| Doppler bandwidth [Hz] | |
| Wavelength of synthetic aperture radar [m] | |
| Direction vector of the target from satellite | |
| Velocity vector of satellite in ECEF frame | |
| Objective function | |
| Initial time at retargeting maneuver [Modified Julian Date, GSFC] | |
| Final time at retargeting maneuver [Modified Julian Date, GSFC] |
References
- Naftaly, U.; Levy-Nathansohn, R. Overview of the TECSAR Satellite Hardware and Mosaic Mode. IEEE Geosci. Remote Sens. Lett. 2008, 5, 423–426. [Google Scholar] [CrossRef]
- L’Abbate, M.; Germani, C.; Torre, A.; Campolo, G.; Cascone, D.; Bombaci, O.; Soccorsi, M.; Iorio, M.; Varchetta, S.; Federici, S. Compact SAR and micro satellite solutions for Earth observation. In Proceedings of the 31st Space Symposium, Colorado Springs, CO, USA, 13–14 April 2015; pp. 1–17. [Google Scholar]
- Sanfourche, J.-P. ‘SAR-lupe’, an important German initiative. Air Space Eur. 2000, 2, 26–27. [Google Scholar] [CrossRef]
- Yokota, Y.; Okada, Y.; Iribe, K.; Tsuji, M.; Ando, A.; Kunii, Y. Newly developed X-band SAR system onboard Japanese small sat-ellite “ASNARO-2”. In Proceedings of the Conference Proceedings of 2013 Asia-Pacific Conference on Syn-thetic Aperture Radar (APSAR), Tsukuba, Japan, 23–27 September 2013; pp. 81–83. [Google Scholar]
- Farquharson, G.; Woods, W.; Stringham, C.; Sankarambadi, N.; Riggi, L. The Capella synthetic aperture radar constellation, EUSAR 2018. In Proceedings of the 12th European Conference on Synthetic Aperture Radar, Verband Deutscher Elektrotrchniker (VDE), Aachen, Germany, 4–7 June 2018; pp. 1–5. [Google Scholar]
- Faizullina, D.; Uetsuhara, M.; Takahira, R.; Murayama, J.; Katayama, M.; Onishi, S.; Yasaka, T. Attitude Determination and Control System for the first SAR Satellite in a Constellation of iQPS. In Proceedings of the 71st International Astronautical Congress (IAC), Dubai, United Arab Emmirates, 12–14 October 2020. [Google Scholar]
- Davidson, G.W.; Cumming, I.G. Signal Properties of Spaceborne Squint-Mode SAR. IEEE Transact. Geosci. Remote Sens. 1997, 35, 611–617. [Google Scholar] [CrossRef] [Green Version]
- Chang, C.Y.; Jin, M.; Curlander, J.C. Squint mode SAR processing algorithms. In Proceedings of the IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Vancouver, BC, Canada, 10–14 July 1989; pp. 1702–1706. [Google Scholar]
- Wang, Y.; Zhimin, Z.; Yunkai, D. Squint spotlight SAR raw signal sim-ulation in the frequency domain using optical principles. IEEE Transact. Geosci. Remote Sens. 2008, 46, 2208–2215. [Google Scholar] [CrossRef]
- Vandewal, M.; Rainer, S.; Helmut, S. Efficient and precise pro-cessing for squinted spotlight SAR through a modified Stolt mapping. EURASIP J. Adv. Sig. Proc. 2006, 2007, 1–7. [Google Scholar]
- Mok, S.-H.; Bang, H.; Kim, H.-S. Analytical Solution for Attitude Command Generation of Agile Spacecraft. J. Korean Soc. Aeronaut. Space Sci. 2018, 46, 639–651. [Google Scholar] [CrossRef]
- Wie, B.; Weiss, H.; Arapostathis, A. Quarternion feedback regulator for spacecraft eigenaxis rotations. J. Guid. Control. Dyn. 1989, 12, 375–380. [Google Scholar] [CrossRef]
- Patterson, M.A.; Anil, V.; Rao, A. GPOPS-II: A MATLAB software for solv-ing multiple-phase optimal control problems using hp-adaptive Gaussian quadrature collocation methods and sparse nonlinear programming. ACM Transact. Math. Softw. 2014, 41, 1–37. [Google Scholar] [CrossRef] [Green Version]
- National Center for Geospatial Intelligence Standards. Spotlight SAR Sensor Model. Supporting Precise Geopositioning; NGA: Springfield, MA, USA, 2010. [Google Scholar]
- Mittermayer, J.; Richard, L.; Elke, B. Sliding spotlight SAR pro-cessing for TerraSAR-X using a new formulation of the extended chirp scaling algo-rithm, IGARSS 2003. In Proceedings of the 2003 IEEE International Geoscience and Remote Sensing Sympo-sium. Proceedings (IEEE Cat. No. 03CH37477), Toulouse, France, 21–25 July 2003; pp. 1462–1464. [Google Scholar]
- Boerner, E.; Lord, R.; Mittermayer, J.; Balmer, R. Evaluation of TerraSAR-X spotlight processing accuracy based on a new spotlight raw data simulator, IGARSS 2003. In Proceedings of the 2003 IEEE International Geoscience and Remote Sensing Symposium, Proceedings (IEEE Cat. No. 03CH37477), Toulouse, France, 21–25 July 2003; pp. 1323–1325. [Google Scholar]
- Omelyan, I.P. On the numerical integration of motion for rigid polyatomics: The modified quaternion approach. Comput. Phys. 1998, 12, 97. [Google Scholar] [CrossRef] [Green Version]
- Diebel, J. Representing attitude: Euler angles, unit quaternions, and rotation vectors. Matrix 2006, 58, 1–35. [Google Scholar]
- Zhao, S.; Deng, Y.; Wang, R. Attitude-Steering Strategy for Squint Spaceborne Synthetic Aperture Radar. IEEE Geosci. Remote Sens. Lett. 2016, 13, 1163–1167. [Google Scholar] [CrossRef]
- Pirnay, H.; Rodrigo, L.-N.; Lorenz, T.B. Optimal sensitivity based on IPOPT. Math. Program. Comput. 2012, 4, 307–331. [Google Scholar] [CrossRef]
- Patterson, M.A.; William, W.H.; Anil, V.R. A ph mesh refinement method for optimal control. Optim. Contr. Appl. Methods 2015, 36, 398–421. [Google Scholar] [CrossRef]
- Kim, H.; Chang, Y.-K. Optimal mission scheduling for hybrid synthetic aperture radar satellite constellation based on weighting factors. Aerosp. Sci. Technol. 2020, 107, 106287. [Google Scholar] [CrossRef]



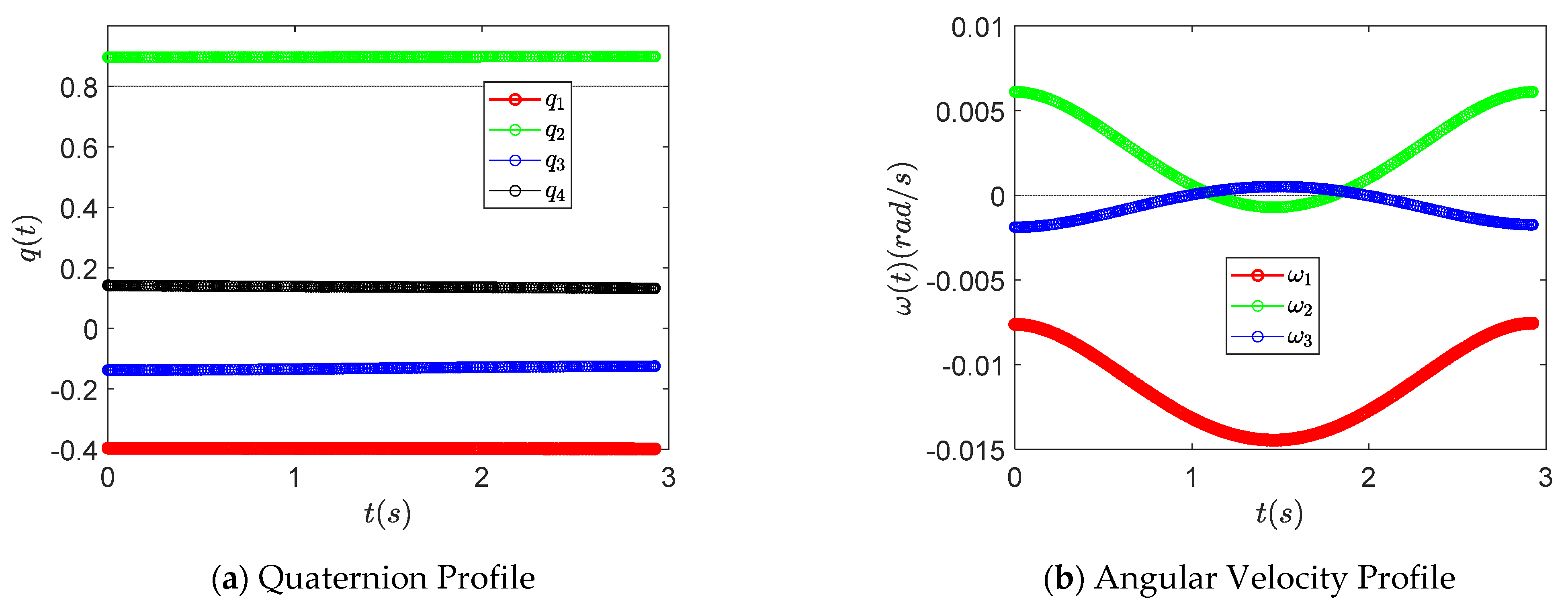
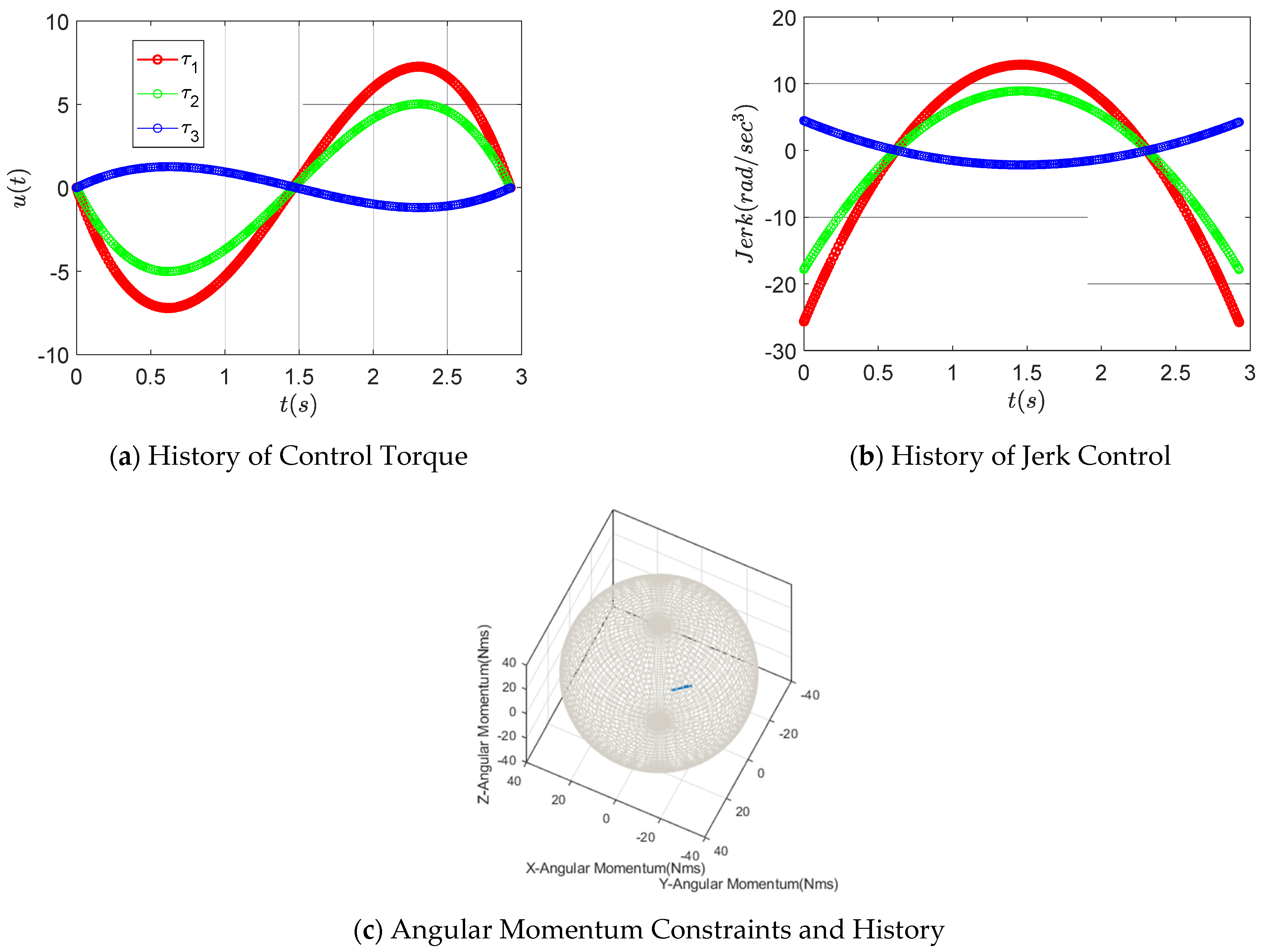
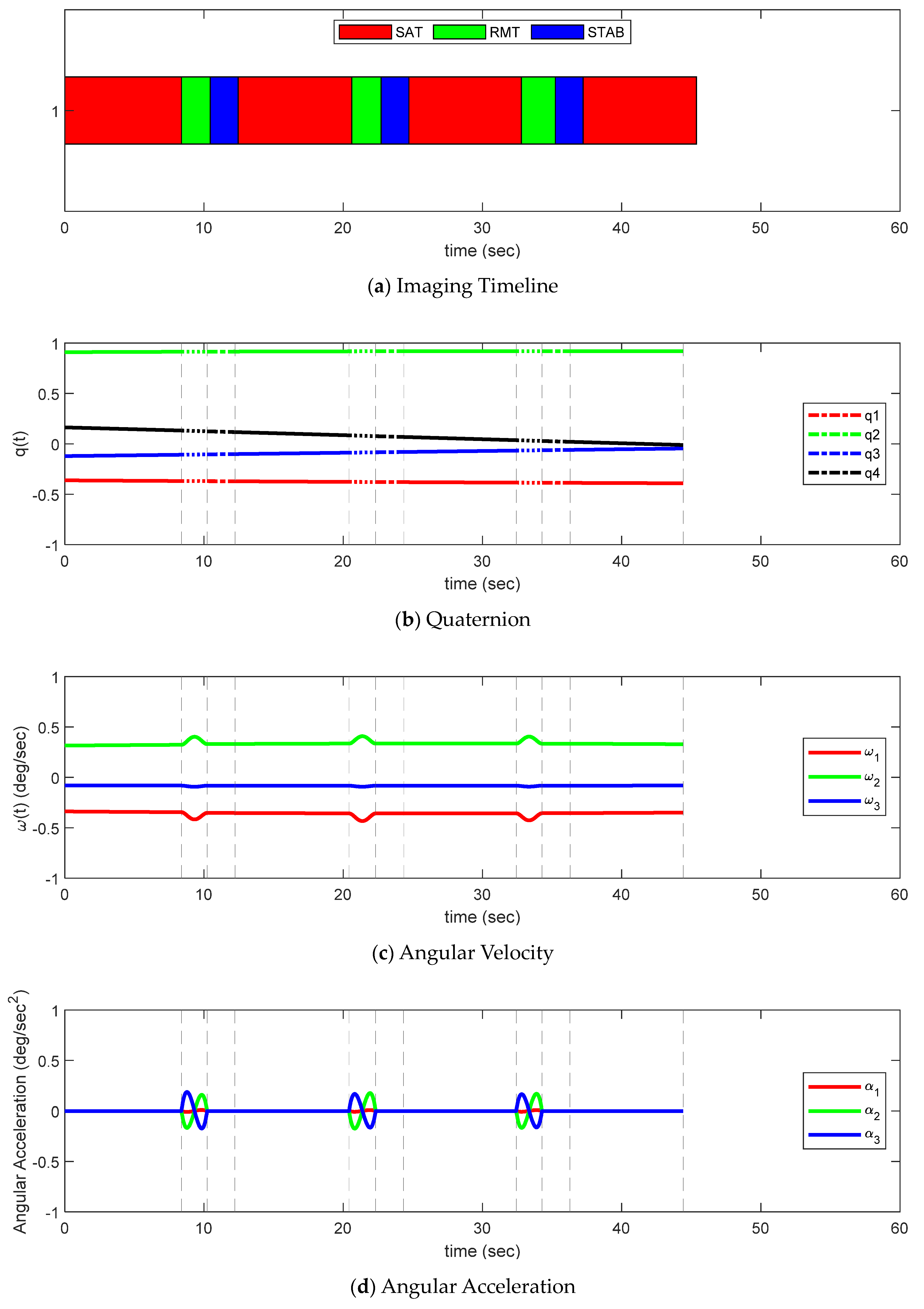

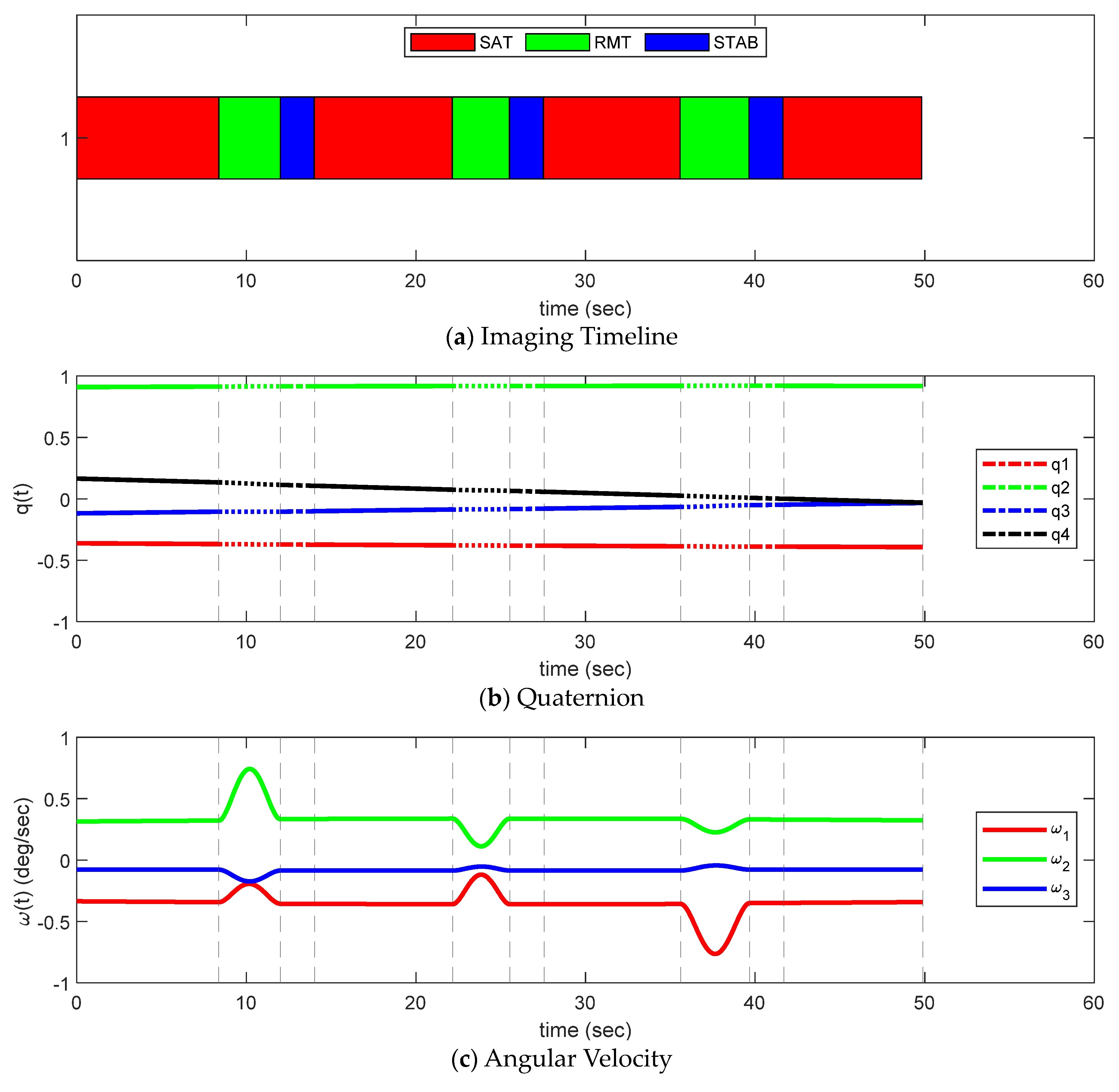
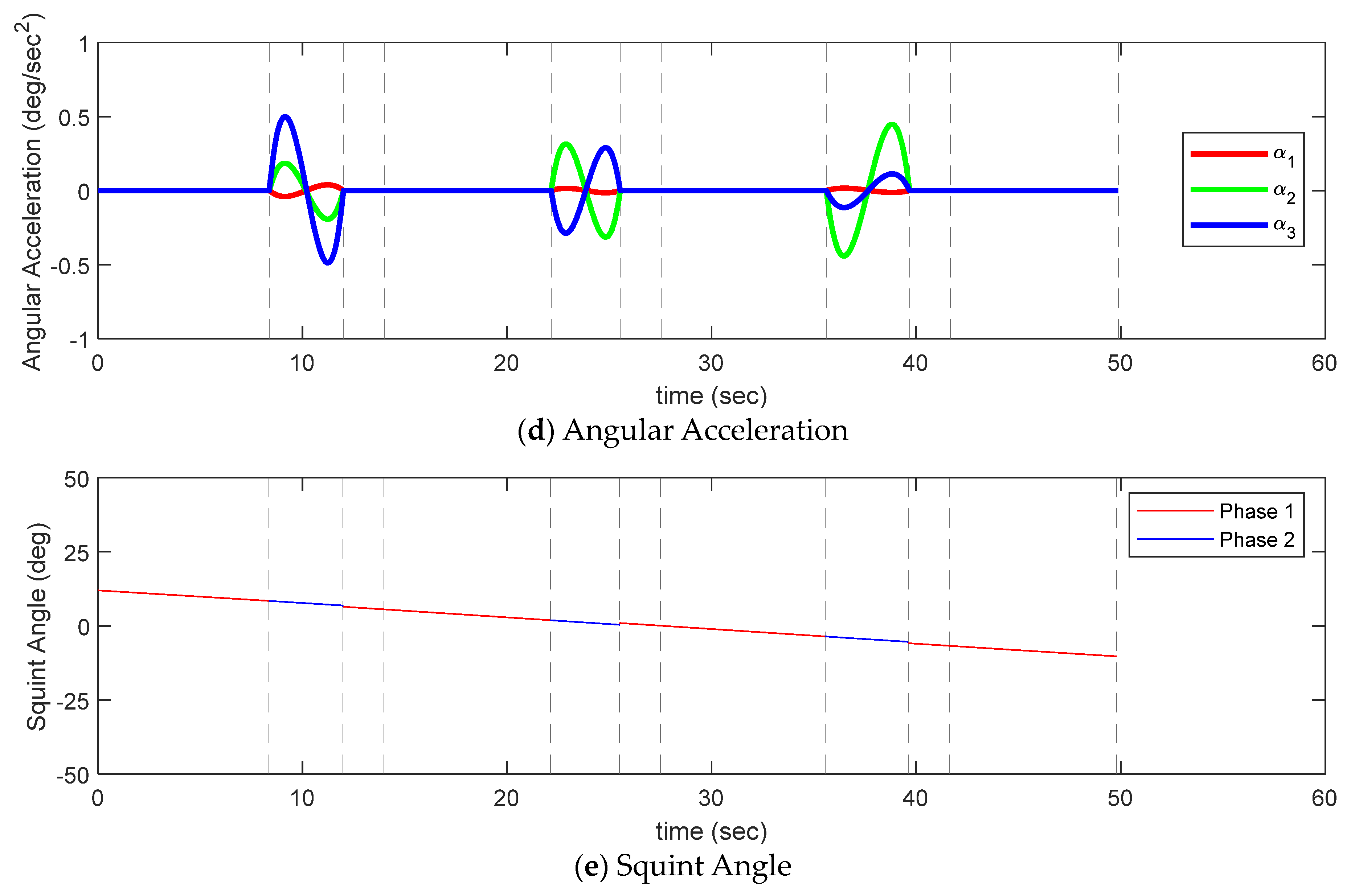
| Initial Conditions | Final Conditions | |
|---|---|---|
| Attitude | Attitude in which the image-taking is ended | Attitude for next image-taking at |
| Angular Velocity | Angular velocity in which the image-taking is ended | Angular velocity for next image-taking at |
| Parameters | Numerical Values |
|---|---|
| Satellite MOI | |
| CMG Max Torque | |
| CMG Angular Momentum | |
| Satellite Altitude | 570 km |
| Orbit Inclination | 45 deg. |
| Resolution | 0.5 m × 0.5 m |
| Swath Width | 5 km × 5 km |
| Squint Angle | ±12 deg. |
| Wavelength | 0.031 m |
| Stabilization Time | 2 s |
| Area of Interest | 20 km × 20 km |
| Number of Targets | 4 |
| Beamwidth | 0.36 deg. |
| Scenarios | Phase 1 (Synthetic Aperture Time; sec) | Phase 2 (Retargeting Maneuver Time; sec) | Stabilization Time; sec | Total Time for Taking 4 Sequential Images; sec |
|---|---|---|---|---|
| Scenario 1 (Target 1~4) | 32.77 (8.38/8.18/8.08/8.13) | 5.60 (1.84/1.91/1.85) | 6 (2/2/2) | 44.37 |
| Scenario 2 (Target 1~4) | 32.81 (8.38/8.15/8.08/8.20) | 11.06 (3.62/3.37/4.07) | 6 (2/2/2) | 49.87 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, H.; Park, J.; Chang, Y.-K.; Lee, S.-H. Optimal Attitude Maneuvering Analyses for Imaging at Squint Staring and Sliding Spotlight Modes of SAR Satellite. Aerospace 2021, 8, 277. https://doi.org/10.3390/aerospace8100277
Kim H, Park J, Chang Y-K, Lee S-H. Optimal Attitude Maneuvering Analyses for Imaging at Squint Staring and Sliding Spotlight Modes of SAR Satellite. Aerospace. 2021; 8(10):277. https://doi.org/10.3390/aerospace8100277
Chicago/Turabian StyleKim, Hongrae, Joonil Park, Young-Keun Chang, and Soo-Ho Lee. 2021. "Optimal Attitude Maneuvering Analyses for Imaging at Squint Staring and Sliding Spotlight Modes of SAR Satellite" Aerospace 8, no. 10: 277. https://doi.org/10.3390/aerospace8100277
APA StyleKim, H., Park, J., Chang, Y.-K., & Lee, S.-H. (2021). Optimal Attitude Maneuvering Analyses for Imaging at Squint Staring and Sliding Spotlight Modes of SAR Satellite. Aerospace, 8(10), 277. https://doi.org/10.3390/aerospace8100277






