Design and Optimization of Ram Air–Based Thermal Management Systems for Hybrid-Electric Aircraft
Abstract
1. Introduction
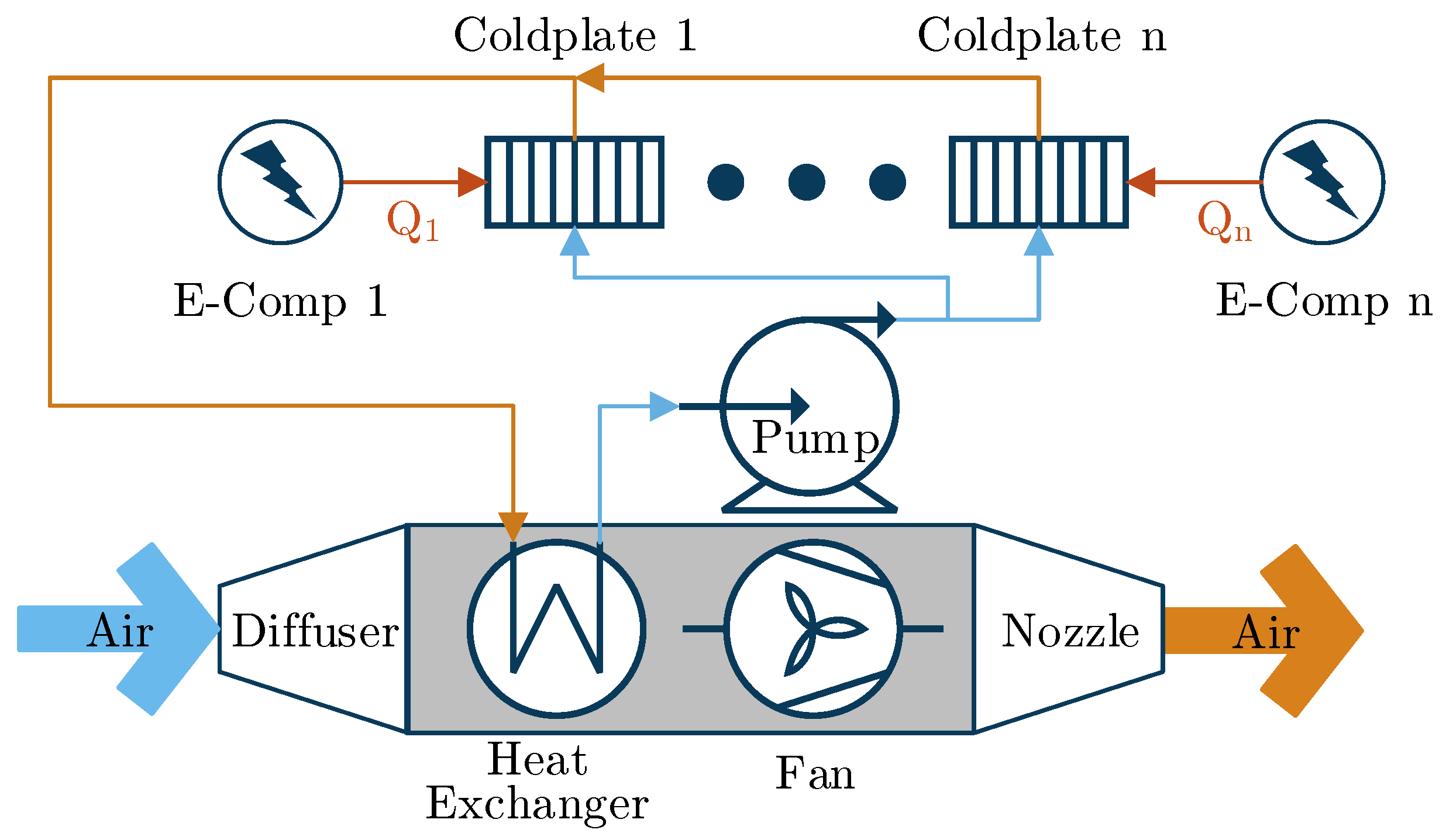
2. Models and Methods
- Coldplates to receive heat from the electric components and transfer it to the coolant.
- A compact HEX to reject the collected heat to ambiance.
- A diffuser to reduce cooling air speed and thereby the cold-side pressure loss of the compact HEX.
- Optionally, a puller fan to increase cooling air flow.
- A nozzle to recover some of the momentum of the cooling air and thereby reduce drag.
- Pipes to transfer the coolant.
- A pump to recover the pressure loss of the coolant.
2.1. Coldplates
2.2. Compact Heat Exchanger
- Rectangular microchannels.
- Offset-strip fins.
- Louvered fins.
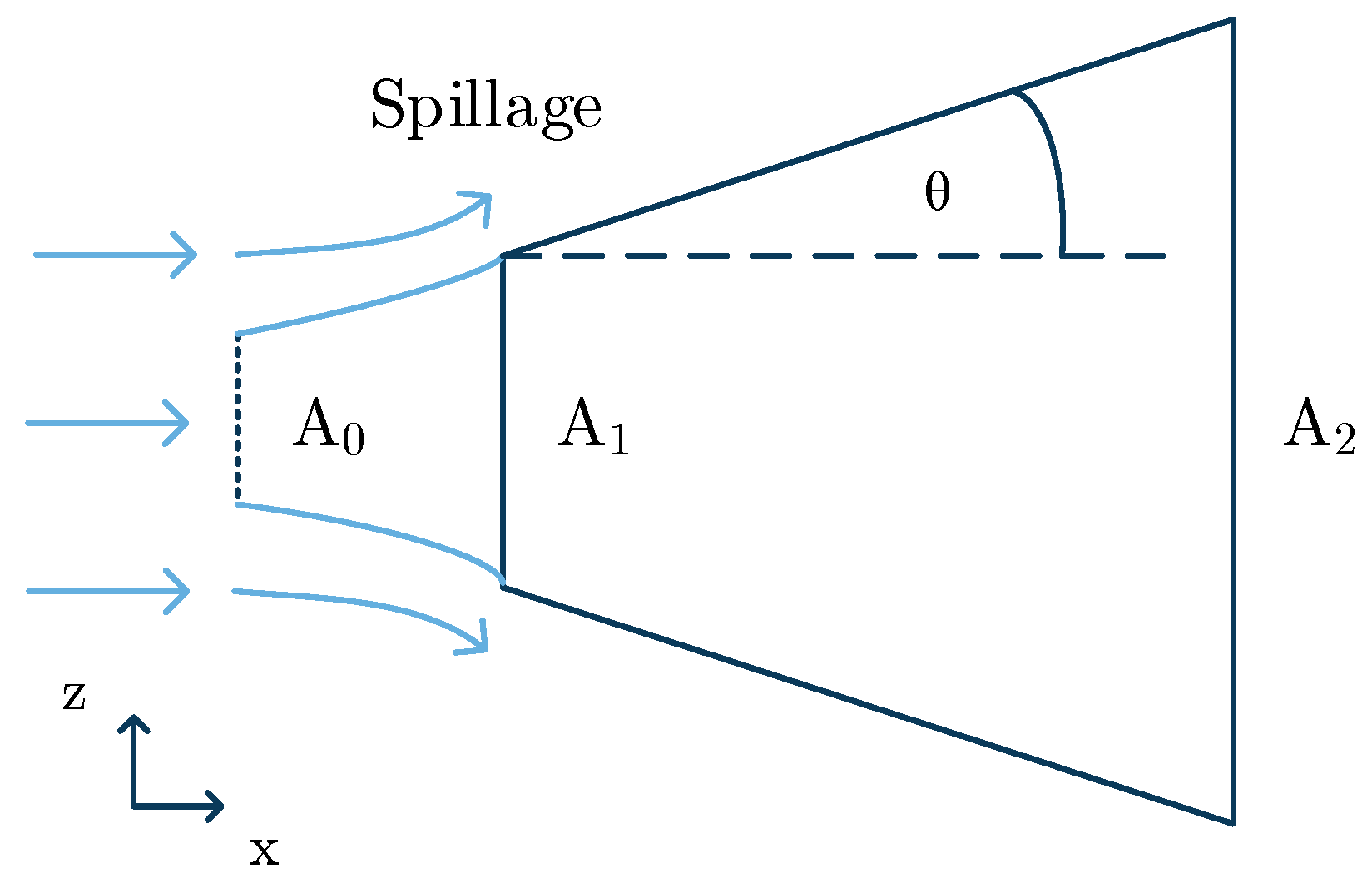
2.3. Diffuser, Nozzle, and Pipes
2.4. Pump and Fan
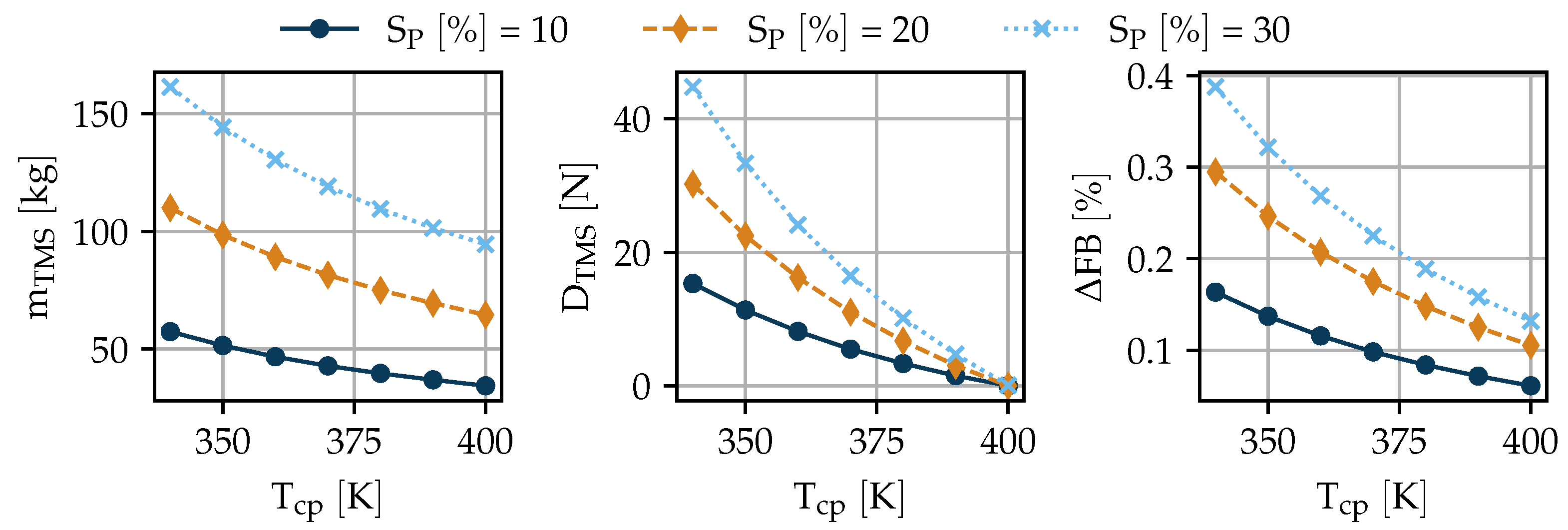
2.5. Aircraft Fuel Burn Sensitivities
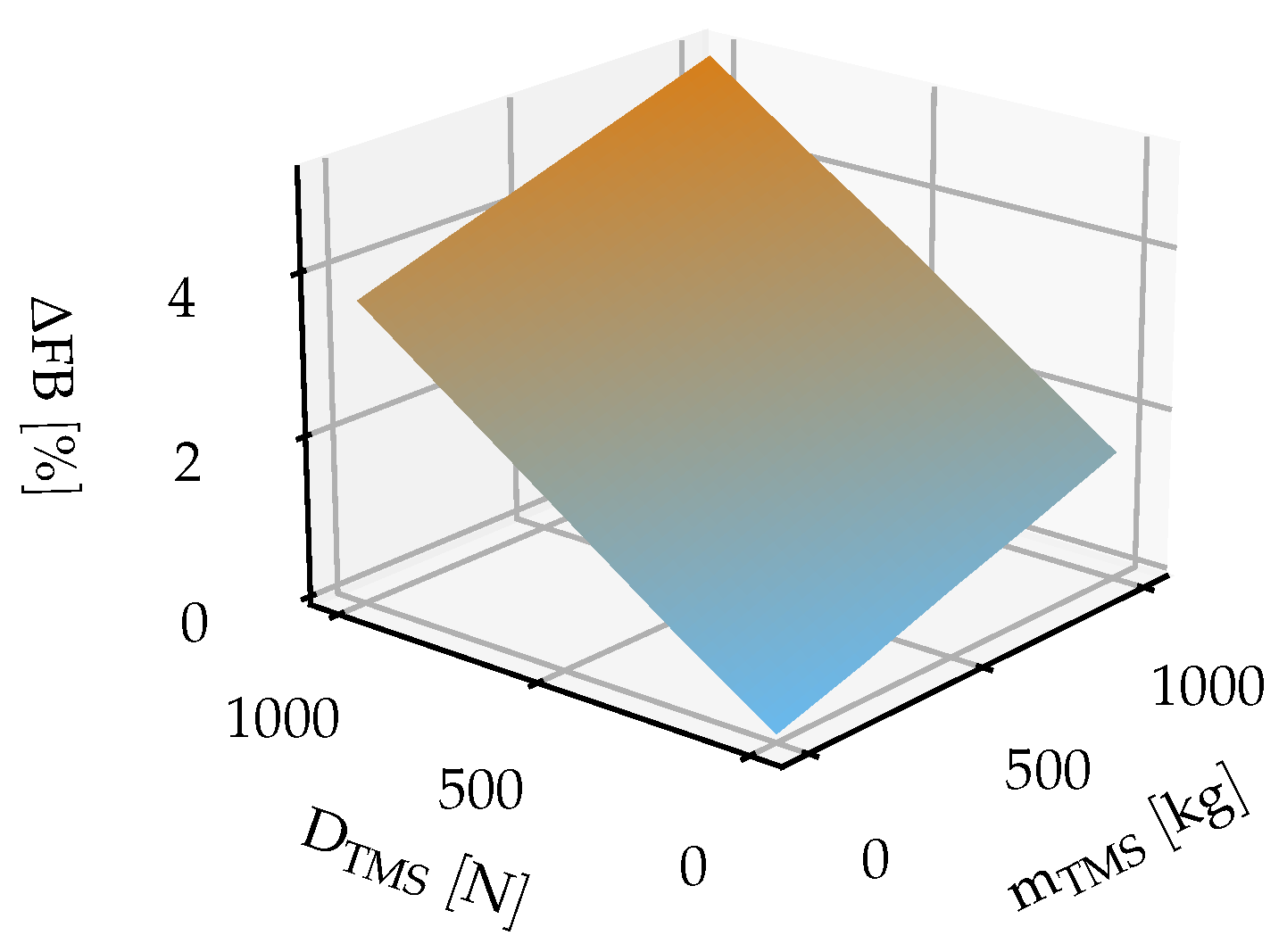
3. System Sensitivity Analysis
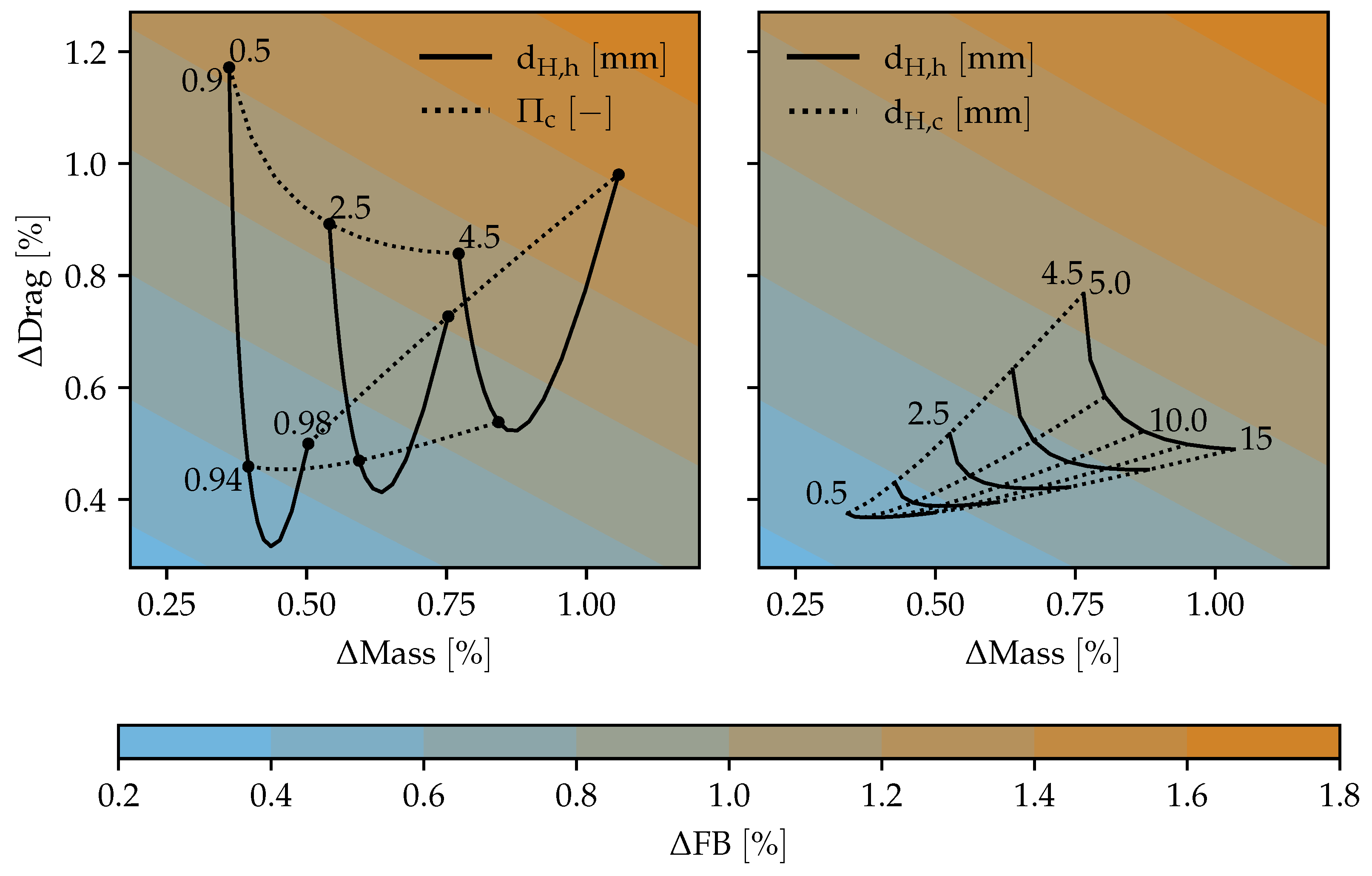
3.1. One-Dimensional Sensitivities
3.2. Multi-Dimensional Sensitivities
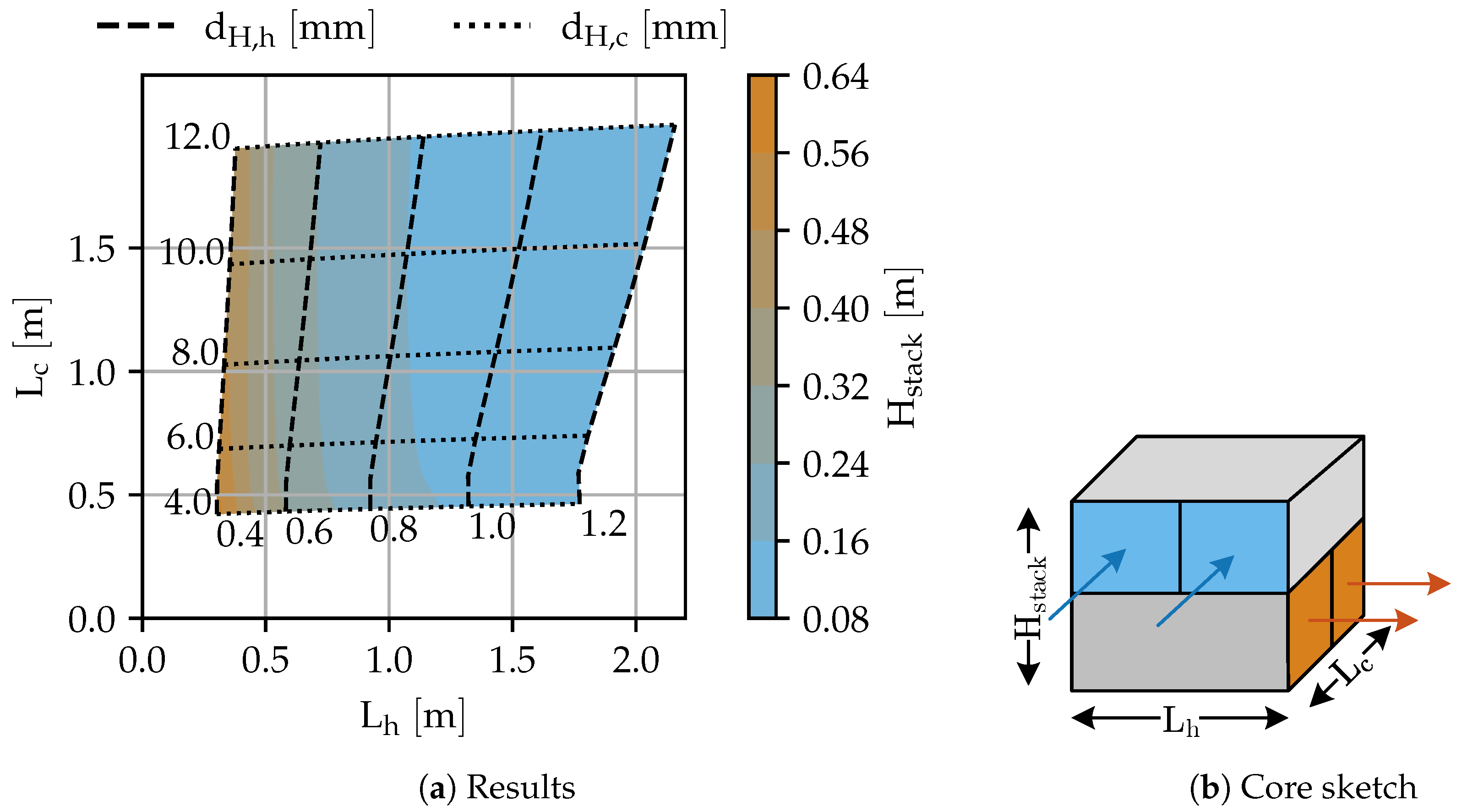
3.3. Heat Exchanger Size
4. Design and Off-Design Optimization for the Application Case
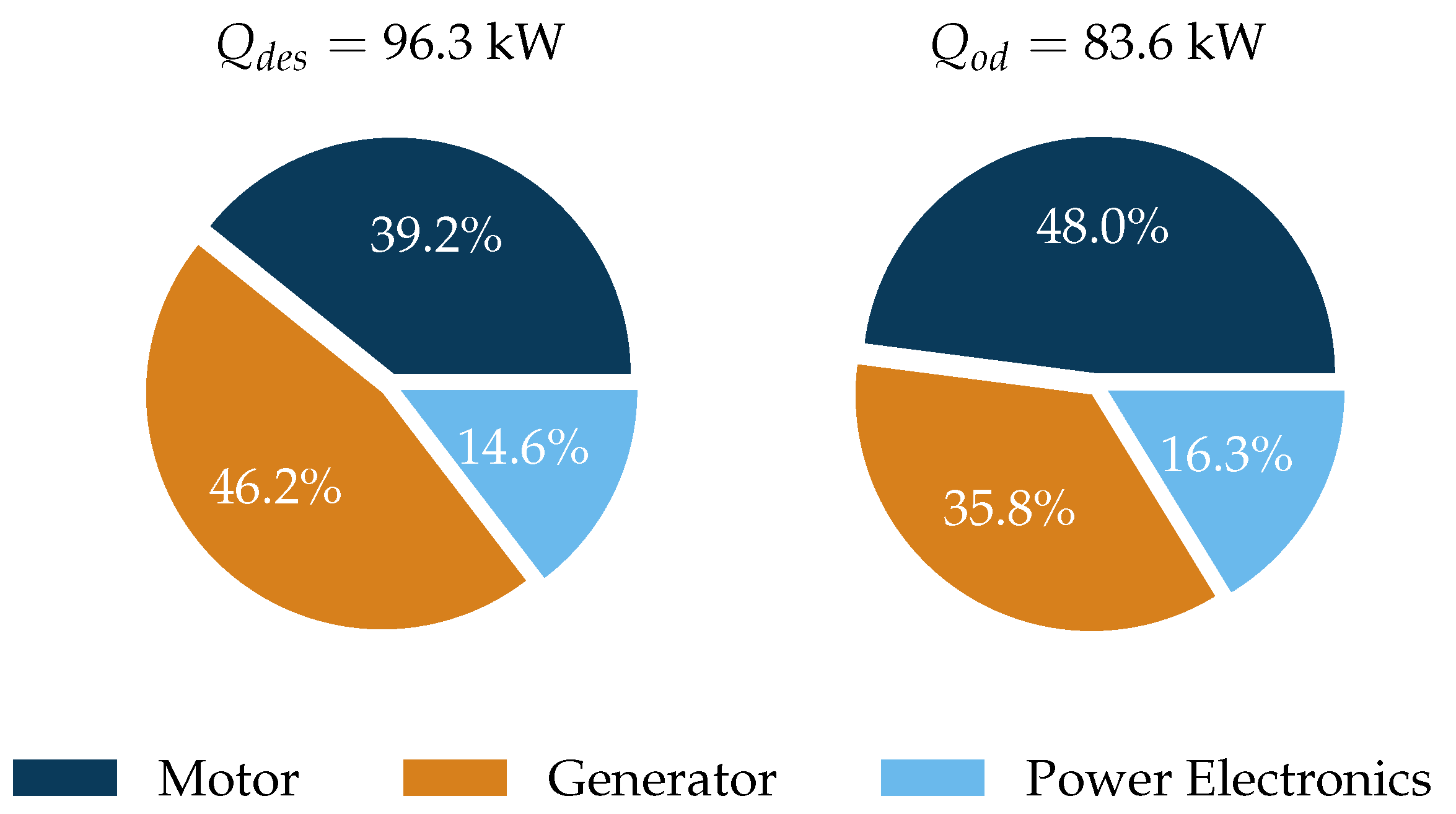
4.1. Design Point Optimization
4.2. Off-Design Point Optimization
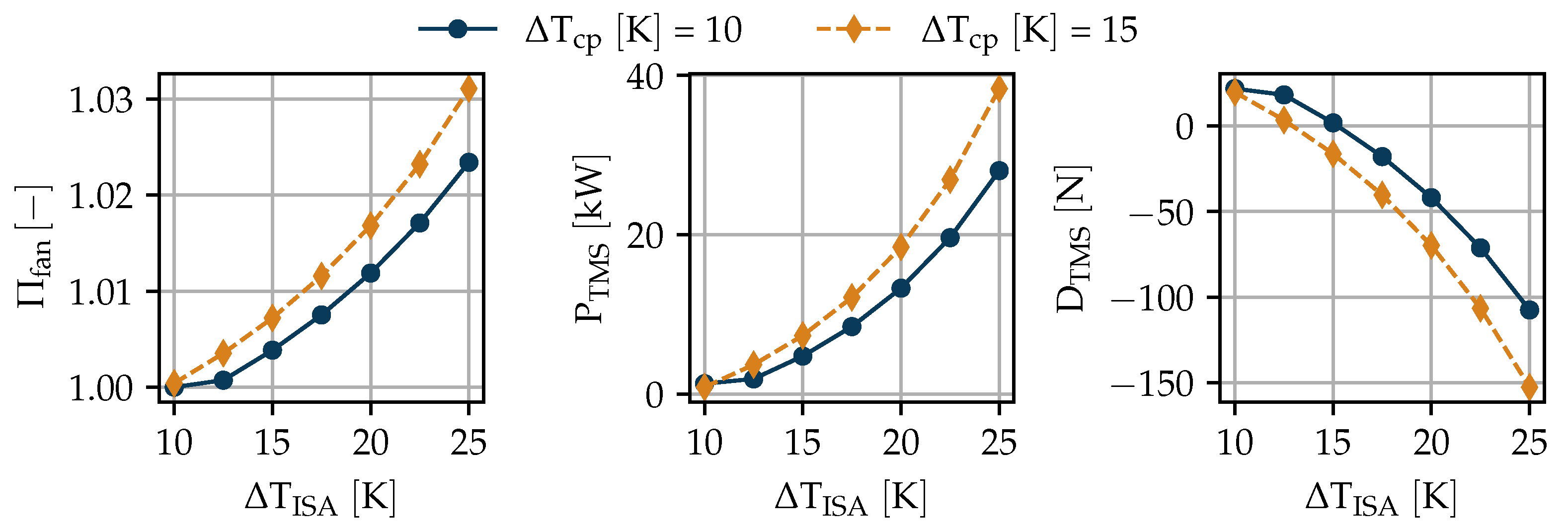
4.3. Multi-Point Optimization
5. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| HDTO | Hot-day takeoff | |
| HEA | Hybrid-electric aircraft | |
| HEX | Heat exchanger | |
| ISA | International standard atmosphere | |
| MP | Main propeller | |
| NASA | National Aeronautics and Space Administration | |
| OEM | Operating empty mass | |
| PCHE | Printed circuit heat exchanger | |
| PFHE | Plate fin heat exchanger | |
| TMS | Thermal management system | |
| TOC | Top of climb | |
| VTOL | Vertical takeoff and landing | |
| WTP | Wingtip propeller | |
| Roman Symbols | ||
| A | Area | m2 |
| Diffuser area ratio | - | |
| Corrected diffuser area ratio | - | |
| b | Heat exchanger plate space | m |
| B | Diffuser inlet blockage | - |
| Specific heat capacity at constant pressure | ||
| Ideal diffuser pressure recovery factor | - | |
| Specific heat capacity at constant volume | ||
| C | Absolute heat capacity | W/K |
| Heat capacity ratio () | - | |
| Side-specific heat capacity ratio () | - | |
| Core mass velocity | kg/(m2s) | |
| Hydraulic diameter | m | |
| D | Drag | N |
| f | Fanning friction factor | - |
| Fuel burn | ||
| g | Diffuser pressure recovery geometry factor | - |
| j | Colburn factor | - |
| Bend loss coefficient | - | |
| Inlet loss coefficient | - | |
| Outlet loss coefficient | - | |
| Nozzle pressure loss coefficient | - | |
| Spillage coefficient | - | |
| L | Length | m |
| m | Mass | kg |
| Ma | Mach number | - |
| Number of passes | - | |
| Number of transfer units on one side | - | |
| Number of transfer units | - | |
| p | Pressure | Pa |
| P | Power | W |
| Prandtl number | - | |
| q | Area-specific heat flow rate | W/m2 |
| Q | Heat flow rate | W |
| Thermal insulance | m2K/W | |
| Thermal resistance | ||
| Reynolds number | - | |
| Power split | % | |
| t | Channel width | m |
| T | Temperature | K |
| U | Overall heat transfer coefficient | W/(m2K) |
| v | Velocity | m/s |
| V | Volume | m3 |
| w | Mass flow rate | |
| Greek Symbols | ||
| α | Heat transfer coefficient | W/(m2K) |
| δ | Fin thickness | m |
| Δ | Difference | - |
| ϵ | Heat exchanger effectiveness | - |
| ηo | Overall fin efficiency | - |
| Φ | Aspect ratio | - |
| Π | Pressure ratio | - |
| ρ | Density | |
| Area density | kg/m2 | |
| σ | Heat exchanger ratio of free flow to frontal area | - |
| θ | Diffuser opening angle | deg |
| Subscripts | ||
| c | Cold | |
| cond | Conductive | |
| conv | Convective | |
| corr | Corrected | |
| cp | Coldplate | |
| cs | Cross section | |
| des | Design | |
| f | Finned | |
| h | Hot | |
| i | Inlet | |
| m | Mean | |
| o | Outlet | |
| od | Off-design | |
| s | Static | |
| spill | Spillage | |
| tot | Total | |
Appendix A. Coldplate Model
Appendix A.1. Model Description
| Parameter | Symbol | Unit |
|---|---|---|
| Inputs | ||
| Inlet pressure | ||
| Inlet temperature | K | |
| Effectiveness | - | |
| Heat load | ||
| Coldplate surface temperature | ||
| Thermal insulance | m2K/W | |
| Area density | kg/m2 | |
| Pressure drop | ||
| Outputs | ||
| Design mass flow | ||
| Outlet pressure | ||
| Outlet temperature | ||
| Area-specific heat load | W/m2 | |
| Coldplate area | m2 | |
| Dry mass | ||
| Number of transfer units | - | |
| U-A product | ||
| Parameter | Symbol | Unit |
|---|---|---|
| Inputs | ||
| Inlet pressure | ||
| Inlet temperature | ||
| Outlet temperature | ||
| Heat load | ||
| Outputs | ||
| Off-design mass flow | ||
| Outlet pressure | ||
| Coldplate temperature | ||
| Area-specific thermal resistance | m2K/W | |
| Effectiveness | - | |
Appendix A.2. Coldplate Validation Design Inputs
| Parameter | Unit | Value |
|---|---|---|
| 294 | ||
| - | ||
| 100 | ||
| 330 | ||
| m2K/W | 2.88 × 10−5 | |
| 50 × 103 |
Appendix B. Compact Heat Exchanger Core Model
- Rectangular microchannels. j and f are calculated according to the methods described for rectangular channels in [24]. Of the parameters in Table 1, and are used as known inputs, and the other parameters are calculated. The aspect ratio of the channels is also an input and defined as:with channel width (t). Starting from (A26) and the definition of :with channel cross section area and perimeter P, rearrangement leads to:
- Offset-strip fins. The model for this core is entirely based on [33]. j and f correlations were directly adapted and used within the given limits. For offset-strip fins, the fin length () is required as an additional input parameter. The missing geometries were derived from Figure 1 in [33]. If offset-strip fins could be realized without additional material on the top or bottom b, , and could be calculated from (A28), (A29), and (A30), respectively. With enhanced manufacturing techniques, it may become possible. Hence, for this model, the additional material thickness on the top and bottom is neglected.
- Louvered fins. The correlation for j was directly implemented from [34] and for f from [35]. b is used as a direct input for this model. and were calculated with (8.76–8.84) from [17]. Additional input parameters to be considered here are louver angle, louver pitch, and louver cut length, which should be selected carefully within the valid ranges given in [34,35].
References
- Advisory Council for Aviation Research and Innovation in Europe. Strategic Research and Innovation Agenda: Volume 1: 2017 Update; Advisory Council for Aviation Research and Innovation in Europe: Brussels, Belgium, 2017. [Google Scholar]
- Singh, R.; Freeman, J.; Osterkamp, P.; Green, M.; Gibson, A.; Schiltgen, B. Challenges and opportunities for electric aircraft thermal management. Aircr. Eng. Aerosp. Technol. 2014, 86, 519–524. [Google Scholar]
- Piancastelli, L.; Frizziero, L.; Donnici, G. The Meredith Ramjet: An Efficient Way To Recover The Heat Wasted in Piston Engine Cooling. J. Eng. Appl. Sci. 2015, 10, 5327–5333. [Google Scholar]
- Pérez-Grande, I.; Leo, T.J. Optimization of a commercial aircraft environmental control system. Appl. Therm. Eng. 2002, 22, 1885–1904. [Google Scholar] [CrossRef]
- Jafari, S.; Nikolaidis, T. Thermal Management Systems for Civil Aircraft Engines: Review, Challenges and Exploring the Future. Appl. Sci. 2018, 8, 2044. [Google Scholar] [CrossRef]
- Brelje, B.J.; Martins, J.R. Electric, hybrid, and turboelectric fixed-wing aircraft: A review of concepts, models, and design approaches. Prog. Aerosp. Sci. 2018, 104, 1–19. [Google Scholar] [CrossRef]
- National Acadamy of Sciences. Commercial Aircraft Propulsion and Energy Systems Research; National Academies Press: Washington, DC, USA, 2016. [Google Scholar]
- Jansen, R.; Bowman, C.; Jankovsky, A. Sizing Power Components of an Electrically Driven Tail Cone Thruster and a Range Extender. In Proceedings of the 16th AIAA Aviation Technology, Integration, and Operations Conference, Washington, DC, USA, 13–17 June 2016. [Google Scholar]
- Lents, C.E.; Hardin, L.W.; Rheaume, J.; Kohlman, L. Parallel Hybrid Gas-Electric Geared Turbofan Engine Conceptual Design and Benefits Analysis. In Proceedings of the 52nd AIAA/SAE/ASEE Joint Propulsion Conference, Salt Lake City, UT, USA, 25–27 July 2016. [Google Scholar]
- Rheaume, J.; Lents, C.E. Design and Simulation of a Commercial Hybrid Electric Aircraft Thermal Management System. In Proceedings of the 2018 AIAA/IEEE Electric Aircraft Technologies Symposium, Cincinnati, OH, USA, 9–11 July 2018. [Google Scholar]
- Rheaume, J.M.; MacDonald, M.; Lents, C.E. Commercial Hybrid Electric Aircraft Thermal Management System Design, Simulation, and Operation Improvements. In Proceedings of the 2019 AIAA/IEEE Electric Aircraft Technologies, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar]
- Chapman, J.W.; Schnulo, S.L. Development of a Thermal Management System for Electrified Aircraft. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; p. 2273. [Google Scholar]
- Kellermann, H.; Habermann, A.L.; Hornung, M. Assessment of Aircraft Surface Heat Exchanger Potential. Aerospace 2020, 7, 1. [Google Scholar] [CrossRef]
- Kellermann, H.; Habermann, A.L.; Vratny, P.C.; Hornung, M. Assessment of fuel as alternative heat sink for future aircraft. Appl. Therm. Eng. 2020, 170, 114985. [Google Scholar] [CrossRef]
- Wei, T. All-in-one design integrates microfluidic cooling into electronic chips. Nature 2020, 585, 188–189. [Google Scholar] [CrossRef] [PubMed]
- Xie, G.; Li, S.; Sunden, B.; Zhang, W.; Li, H. A Numerical Study of the Thermal Performance of Microchannel Heat Sinks with Multiple Length Bifurcation in Laminar Liquid Flow. Numer. Heat Transf. A Appl. 2014, 65, 107–126. [Google Scholar] [CrossRef]
- Shah, R.K.; Sekulić, D.P. Fundamentals of Heat Exchanger Design; Wiley-Interscience: Hoboken, NJ, USA, 2003. [Google Scholar]
- Kays, W.M.; London, A.L. Compact Heat Exchangers, 3rd ed.; Krieger: Malabar, FL, USA, 1998. [Google Scholar]
- Sundén, B.; Fu, J. Heat Transfer in Aerospace Applications; Academic Press: London, UK, 2017. [Google Scholar]
- Pittaluga, F. A set of correlations proposed for diffuser performance prediction. Mech. Res. Commun. 1981, 8, 161–168. [Google Scholar] [CrossRef]
- Malan, P.; Brown, E.F. Inlet drag prediction for aircraft conceptual design. J. Aircr. 1994, 31, 616–622. [Google Scholar] [CrossRef]
- Bräunling, W.J.G. Flugzeugtriebwerke: Grundlagen, Aero-Thermodynamik, Ideale und Reale Kreisprozesse, Thermische Turbomaschinen, Komponenten, Emissionen und Systeme, 4th ed.; VDI-Buch; Springer: Berlin, Germany, 2015. [Google Scholar]
- Walsh, P.P.; Fletcher, P. Gas Turbine Performance, 2nd ed.; Blackwell Science: Oxford, UK, 2008. [Google Scholar]
- Incropera, F.P.; DeWitt, D.P.; Bergman, T.L.; Lavine, A.S. Principles of Heat and Mass Transfer, 7th ed.; International Student Version, Ed.; Wiley: Singapore, 2013. [Google Scholar]
- Haaland, S.E. Simple and Explicit Formulas for the Friction Factor in Turbulent Pipe Flow. J. Fluids Eng. 1983, 105, 89. [Google Scholar] [CrossRef]
- Pohl, M.; Köhler, J.; Jeschke, P.; Kellermann, H.; Lüdemann, M.; Hornung, M.; Weintraub, D. Integrated Preliminary Design of Turboelectric Aircraft Propulsion Systems. 2021. Manuscript in preparation. [Google Scholar]
- Matsuda, M.; Mashiko, K.; Saito, Y.; Nguyen, T.; Nguyen, T. Mico-Channel Cold Plate Units for Cooling Super Computer; Fujikura: Tokyo, Japan, 2015. [Google Scholar]
- Advanced Thermal Solutions. The Thermal Resistance of Microchannel Cold Plates. Qpedia Therm. Emagazine 2012, 2012, 6–9. [Google Scholar]
- Bell, I.H.; Wronski, J.; Quoilin, S.; Lemort, V. Pure and Pseudo-pure Fluid Thermophysical Property Evaluation and the Open-Source Thermophysical Property Library CoolProp. Ind. Eng. Chem. Res. 2014, 53, 2498–2508. [Google Scholar] [CrossRef] [PubMed]
- VDI-Wärmeatlas. Mit 320 Tabellen, 11th ed.; VDI-Buch; Springer: Berlin, Germany, 2013. [Google Scholar]
- Denkenberger, D.C.; Brandemuehl, M.J.; Pearce, J.M.; Zhai, J. Expanded microchannel heat exchanger: Design, fabrication, and preliminary experimental test. Proc. Inst. Mech. Eng. A J. Power Energy 2012, 226, 532–544. [Google Scholar] [CrossRef]
- Nakayama, Y.; Boucher, R.F. Introduction to Fluid Mechanics; Butterworth Heinemann: Oxford, UK; Boston, MA, USA, 1999. [Google Scholar]
- Wieting, A.R. Empirical Correlations for Heat Transfer and Flow Friction Characteristics of Rectangular Offset-Fin Plate-Fin Heat Exchangers. J. Heat Transf. 1975, 97, 488–490. [Google Scholar] [CrossRef]
- Chang, Y.J.; Wang, C.C. A generalized heat transfer correlation for Iouver fin geometry. Int. J. Heat Mass Transf. 1997, 40, 533–544. [Google Scholar] [CrossRef]
- Chang, Y.J.; Hsu, K.C.; Lin, Y.T.; Wang, C.C. A generalized friction correlation for louver fin geometry. Int. J. Heat Mass Transf. 2000, 43, 2237–2243. [Google Scholar] [CrossRef]



| Name | Symbol | Unit |
|---|---|---|
| Colburn factor | j | - |
| Fanning friction factor | f | - |
| Hydraulic diameter | ||
| Plate space | b | |
| Area density | m2/m3 | |
| Fin thickness | ||
| Fin thermal conductivity | ||
| Ratio finned to total heat transfer area | - |
| Parameter | Symbol | Unit | Default Value |
|---|---|---|---|
| Coldplate surface temperature | 370 | ||
| Heat capacity ratio HEX cold to hot side | - | ||
| Coldplate coolant inlet temperature | 275 | ||
| Pressure ratio HEX cold side | - | ||
| Hydraulic diameter HEX cold side | mm | ||
| Coldplate effectiveness | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kellermann, H.; Lüdemann, M.; Pohl, M.; Hornung, M. Design and Optimization of Ram Air–Based Thermal Management Systems for Hybrid-Electric Aircraft. Aerospace 2021, 8, 3. https://doi.org/10.3390/aerospace8010003
Kellermann H, Lüdemann M, Pohl M, Hornung M. Design and Optimization of Ram Air–Based Thermal Management Systems for Hybrid-Electric Aircraft. Aerospace. 2021; 8(1):3. https://doi.org/10.3390/aerospace8010003
Chicago/Turabian StyleKellermann, Hagen, Michael Lüdemann, Markus Pohl, and Mirko Hornung. 2021. "Design and Optimization of Ram Air–Based Thermal Management Systems for Hybrid-Electric Aircraft" Aerospace 8, no. 1: 3. https://doi.org/10.3390/aerospace8010003
APA StyleKellermann, H., Lüdemann, M., Pohl, M., & Hornung, M. (2021). Design and Optimization of Ram Air–Based Thermal Management Systems for Hybrid-Electric Aircraft. Aerospace, 8(1), 3. https://doi.org/10.3390/aerospace8010003





