CFD-Based Aeroelastic Sensitivity Study of a Low-Speed Flutter Demonstrator
Abstract
1. Introduction
2. Theory and Numerical Methods
2.1. Equations of Aeroelasticity
2.2. Modelling of Unsteady Aerodynamics by Means of SD CFD Methodology
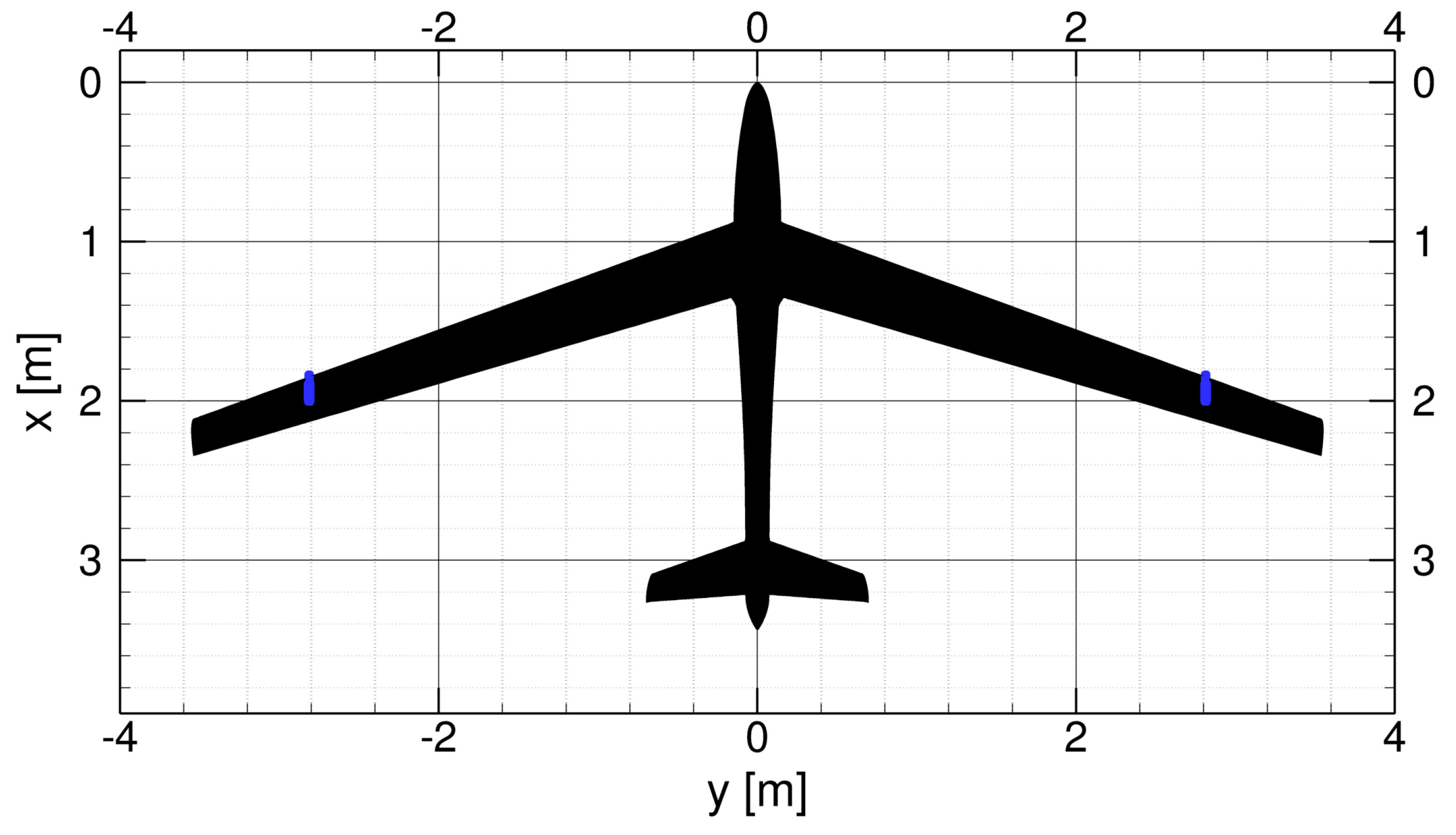
3. The Flutter Suppression Demonstrator FLEXOP
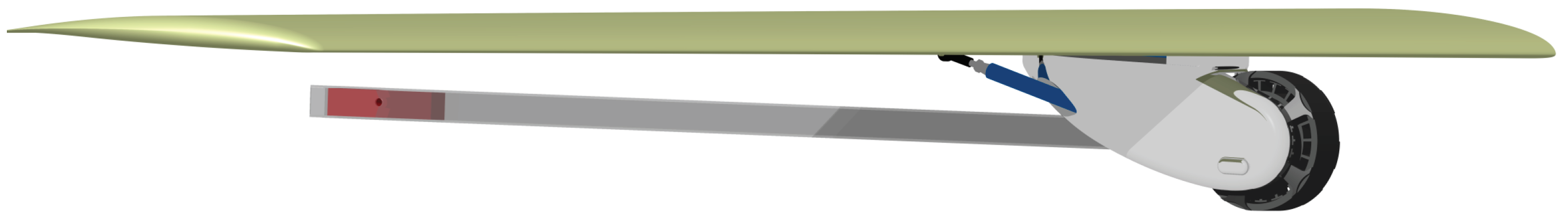
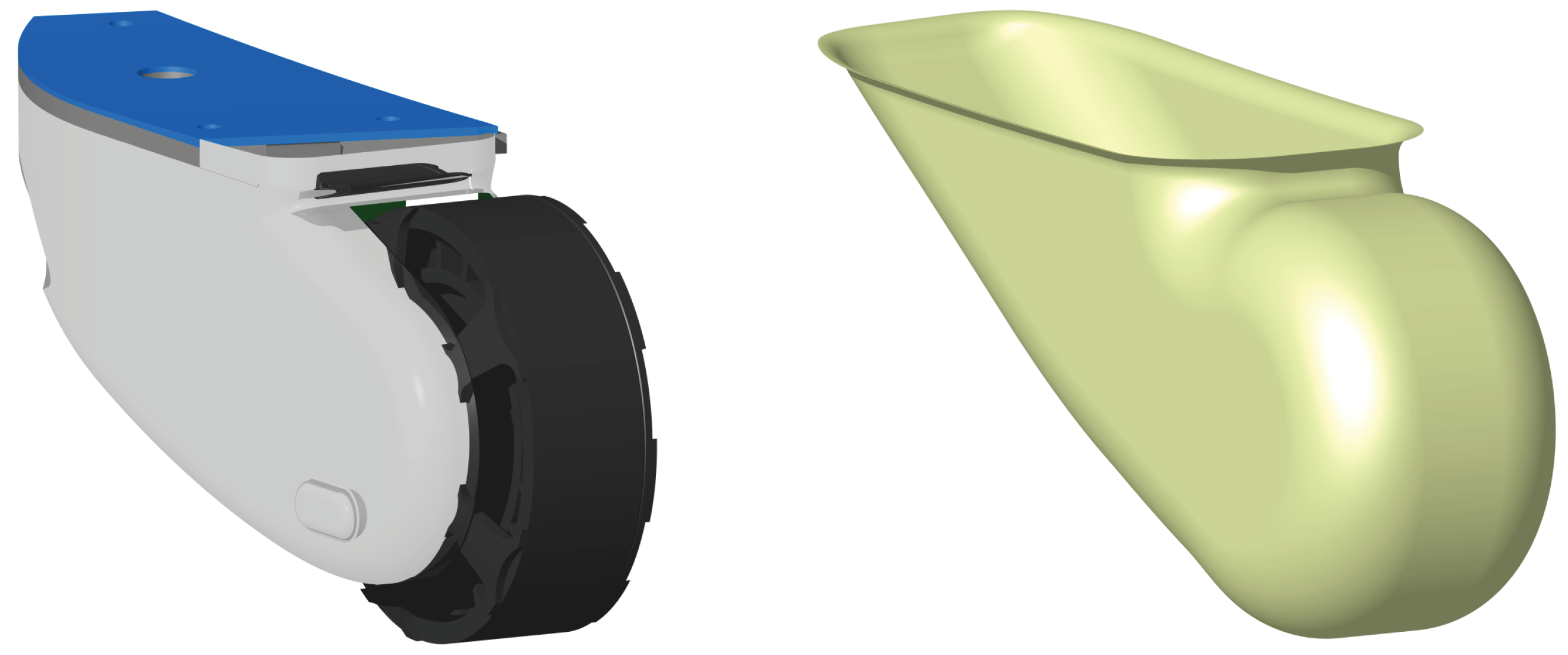
3.1. UAV Configuration
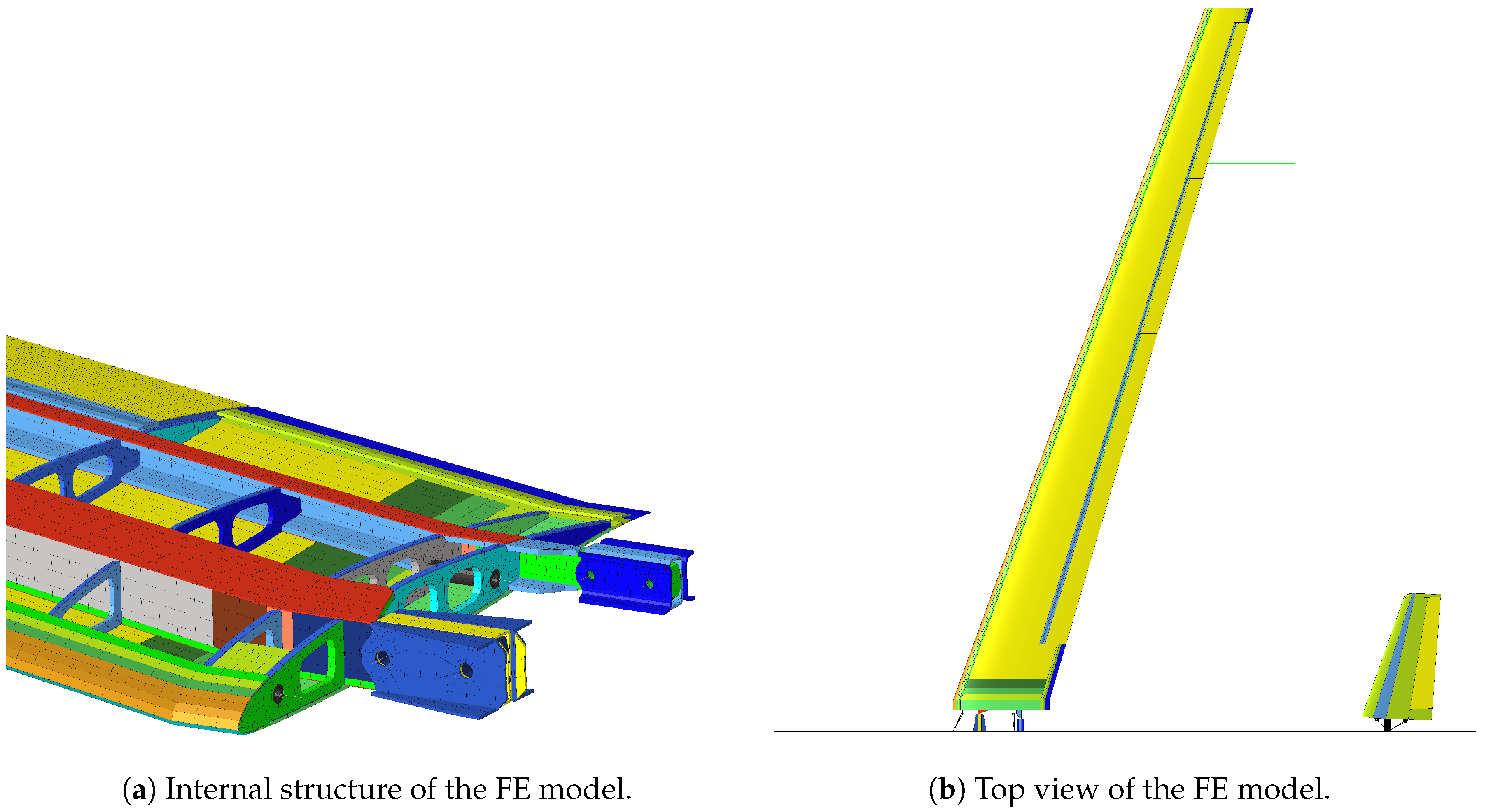
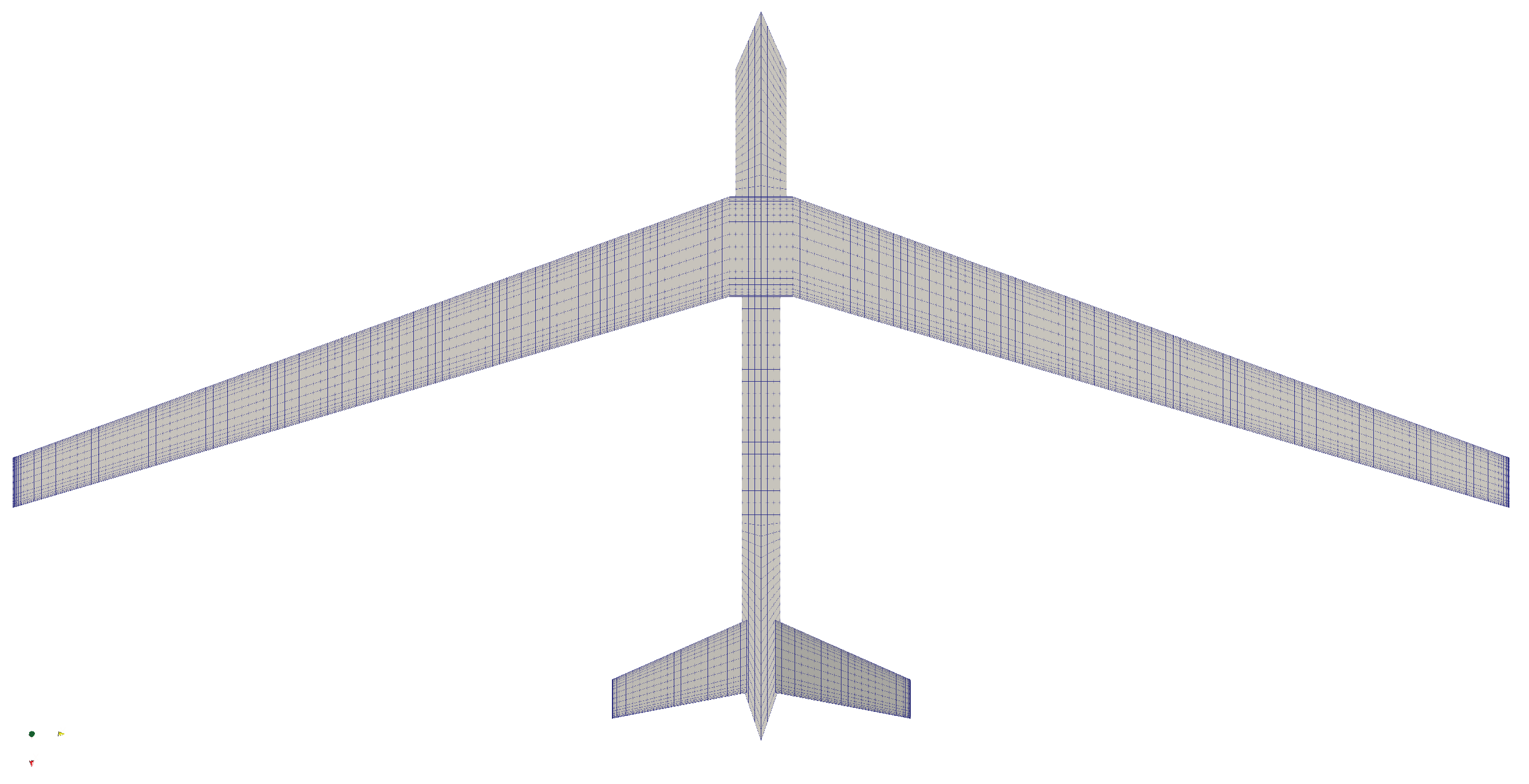
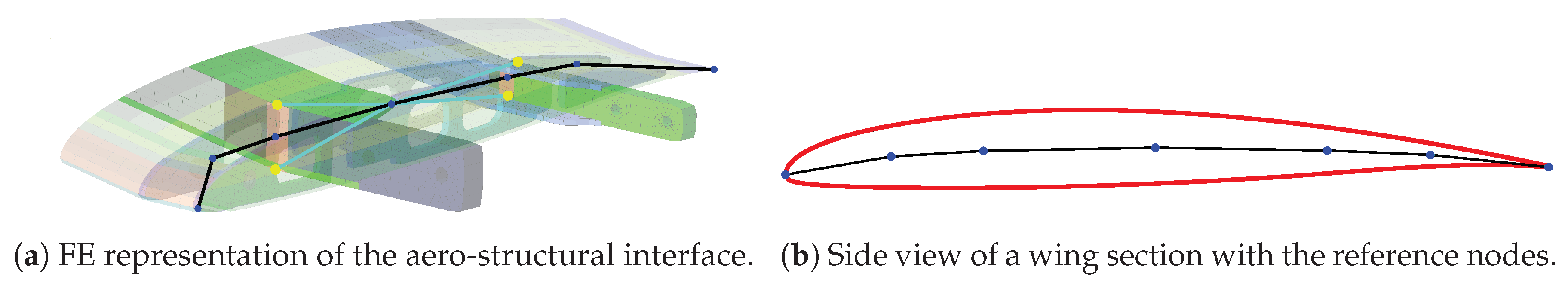
3.2. Finite Element Model
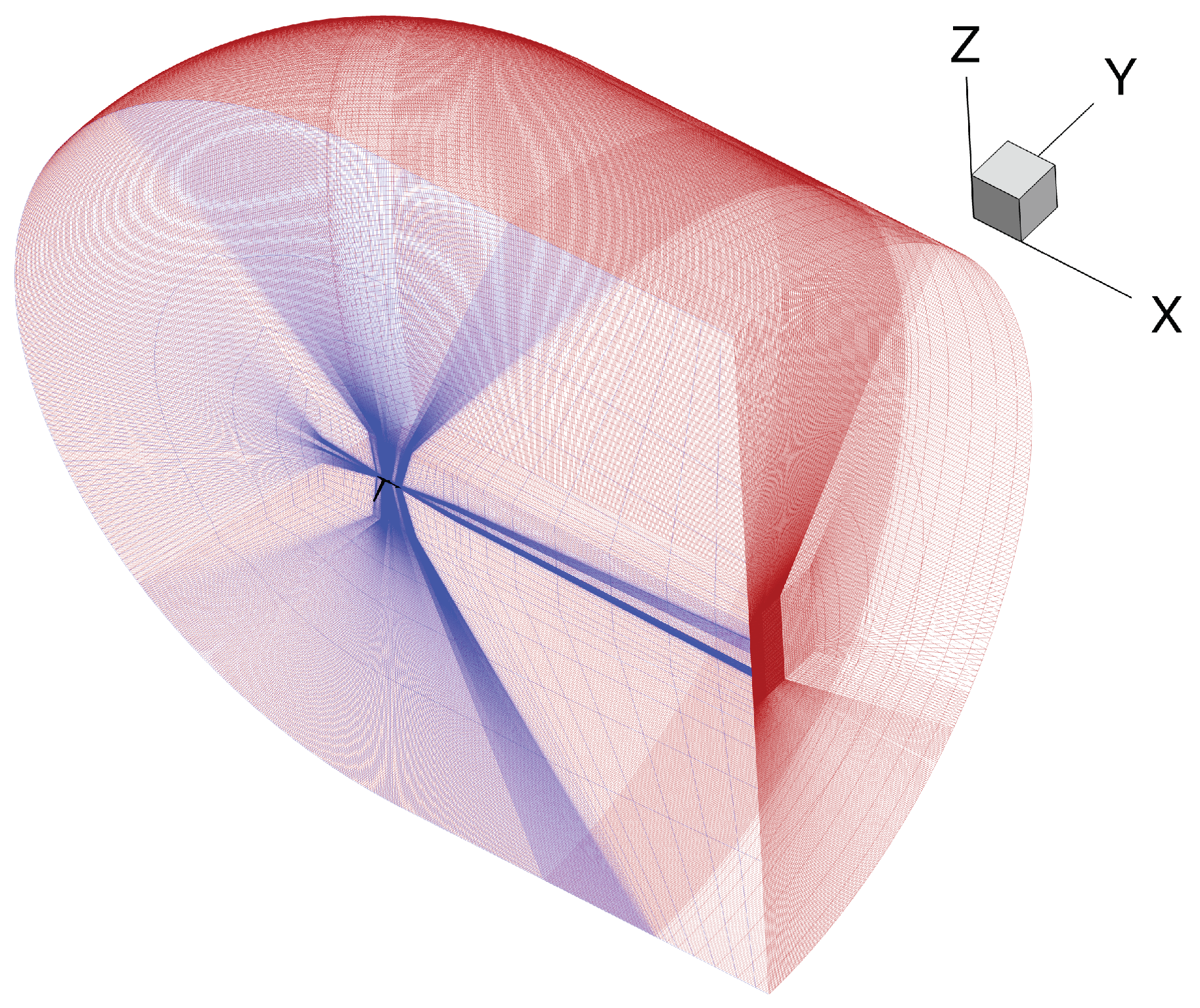
3.3. CFD Setup
4. Results
4.1. Results of the Steady State CFD Simulation
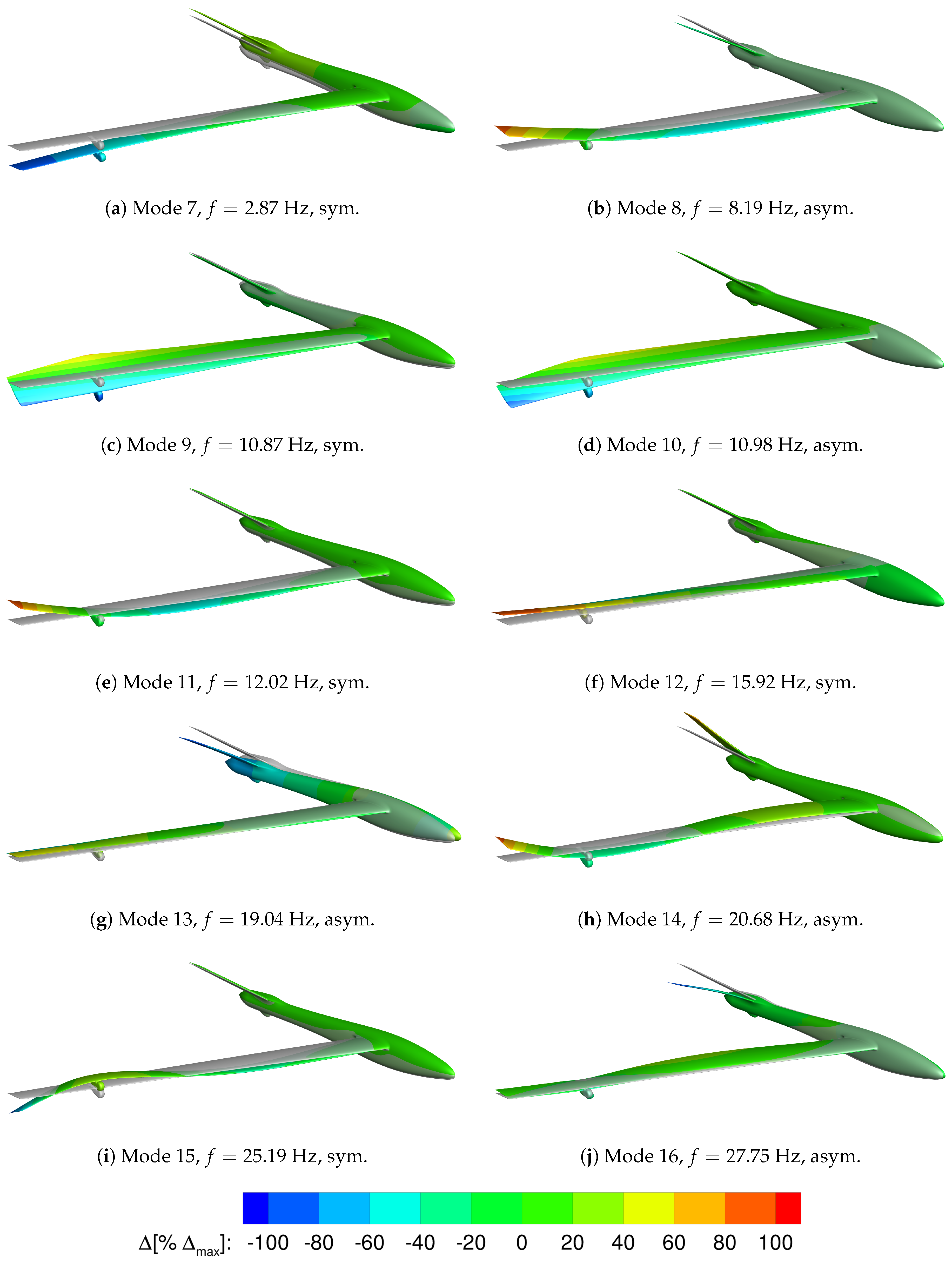
4.2. Aeroelastic Behavior of the Baseline Configuration
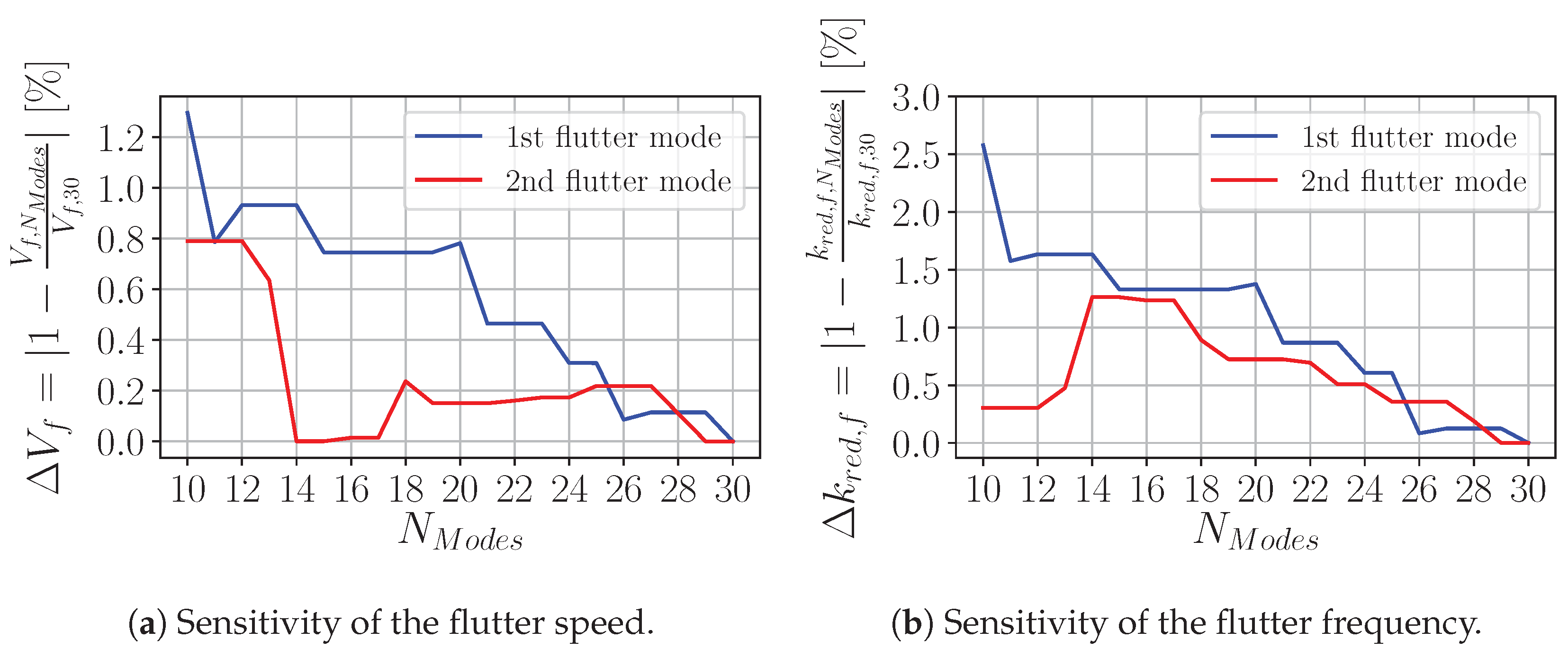
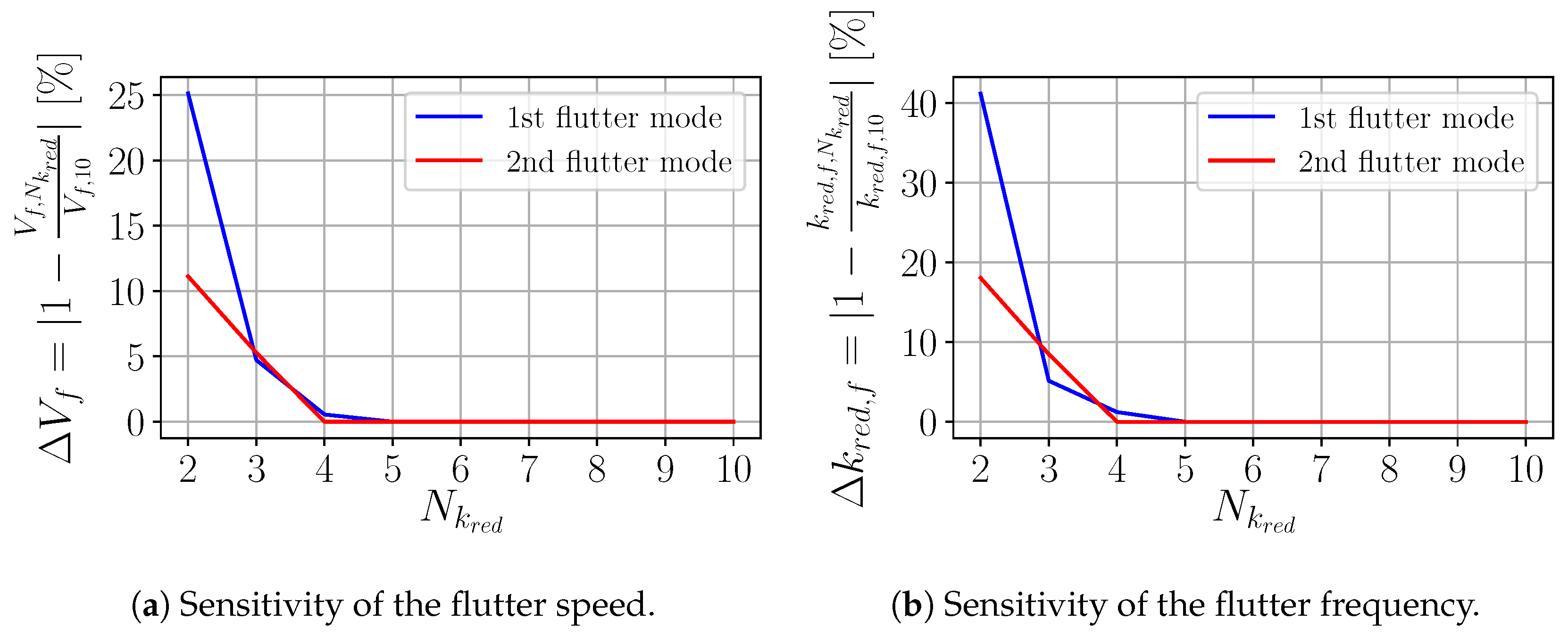
4.3. Sensitivity of the Flutter Limits Concerning the Size of the GAF Dataset
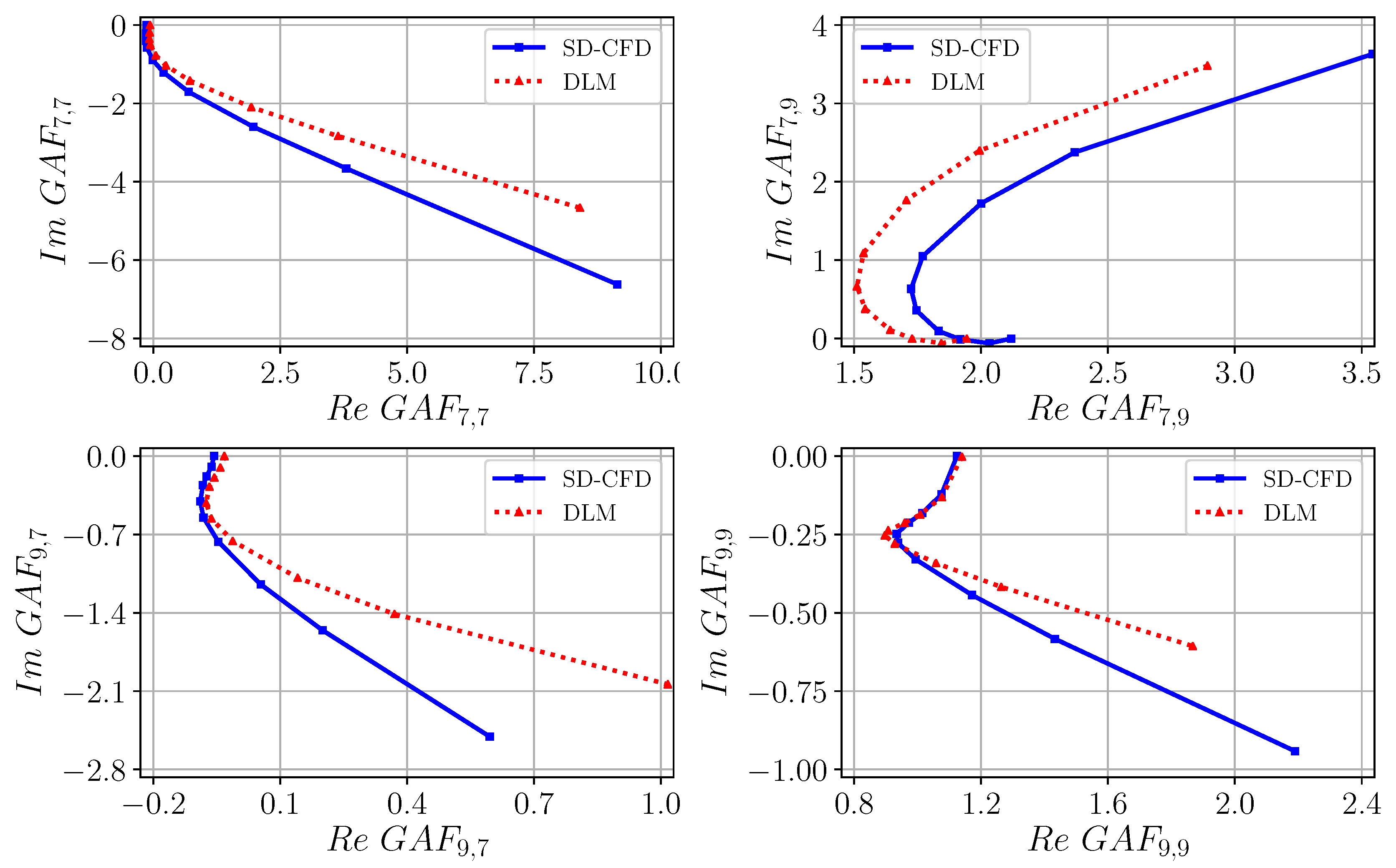
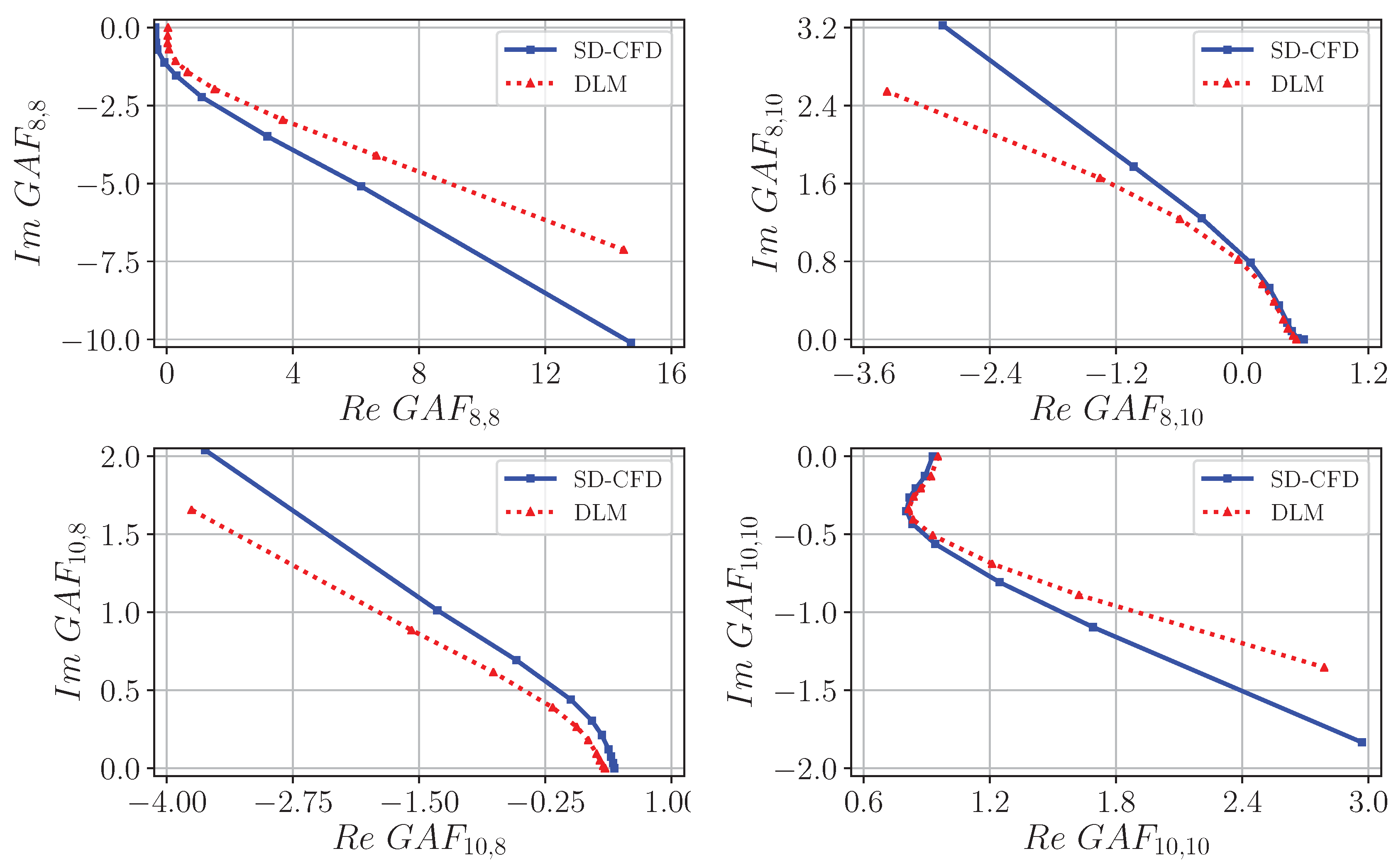
4.4. Comparison of SD-CFD-Based and DLM-Based Results
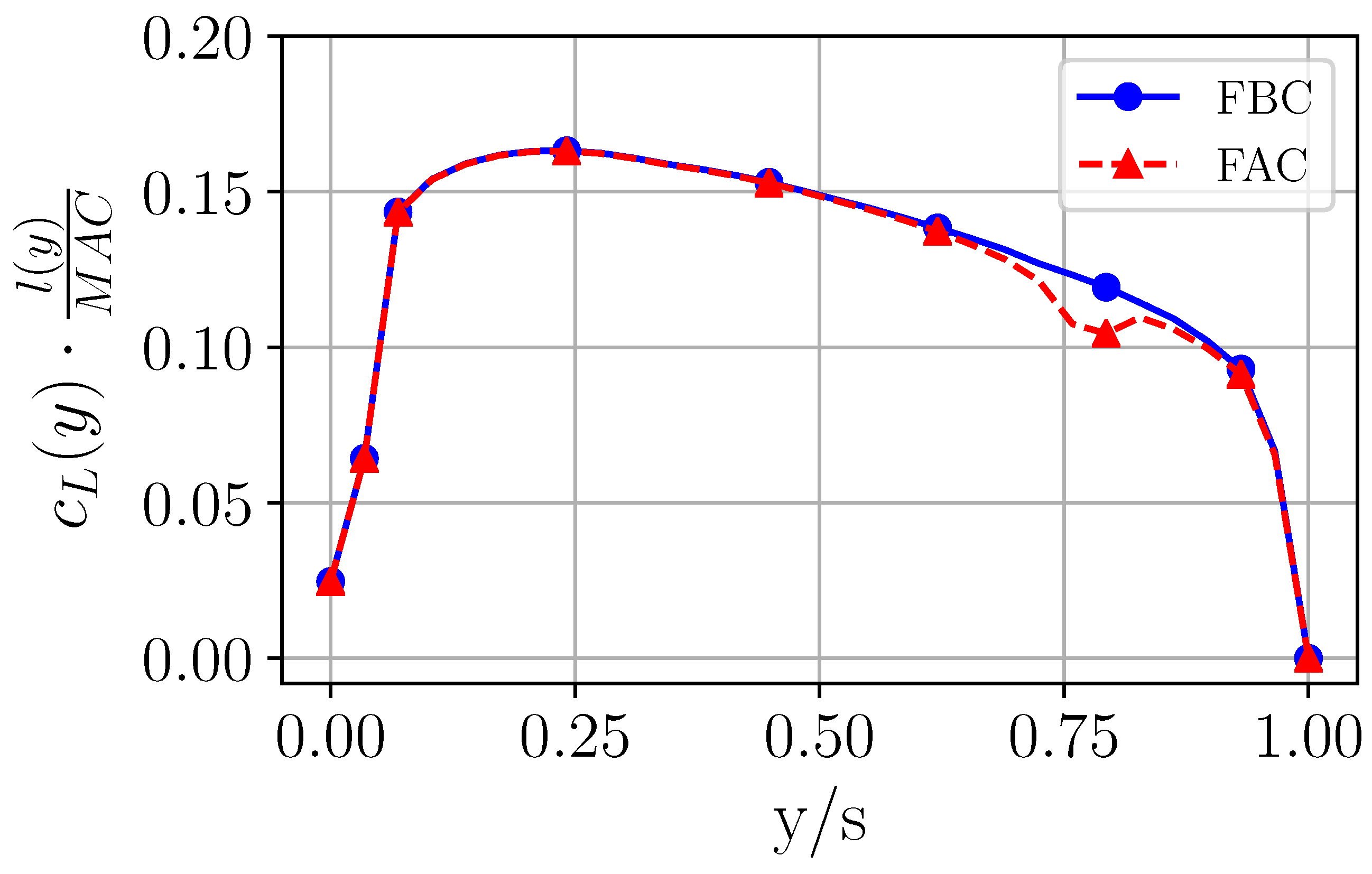
4.5. Aeroelastic Influence of the Actuator
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Scheelhaase, J.; Grimme, W.; O’Sullivan, M.; Naegler, T.; Klötzke, M.; Kugler, U.; Scheier, B.; Standfuß, T. Klimaschutz im Verkehrssektor—Aktuelle Beispiele aus der Verkehrsforschung. Wirtschaftsdienst 2018, 98, 655–663. [Google Scholar] [CrossRef]
- Price, M.; Raghunathan, S.; Curran, R. An Integrated Systems Engineering Approach to Aircraft Design. Prog. Aerosp. Sci. 2006, 42, 331–376. [Google Scholar] [CrossRef]
- Hall, K.C.; Crawley, E.F. Calculation of Unsteady Flows in Turbomachinery Using the Linearized Euler Equations. AIAA J. 1989, 27, 777–787. [Google Scholar] [CrossRef]
- Hall, K.C.; Clark, W.S. Linearized Euler Predictions of Unsteady Aerodynamic Loads in Cascades. AIAA J. 1993, 31, 540–550. [Google Scholar] [CrossRef]
- Kreiselmaier, E.; Laschka, B. Small Disturbance Euler Equations: Efficient and Accurate Tool for Unsteady Load Prediction. J. Aircr. 2000, 37, 770–778. [Google Scholar] [CrossRef]
- Pechloff, A.; Laschka, B. Small Disturbance Navier-Stokes Method: Efficient Tool for Predicting Unsteady Air Loads. J. Aircr. 2006, 43, 17–29. [Google Scholar] [CrossRef]
- Iatrou, M. Ein Navier-Stokes-Verfahren Kleiner Störungen Für Instationäre Vorgänge—Anwendung auf Transportflugzeuge. Ph.D. Thesis, Technische Universität München, München, Germany, 2010. [Google Scholar]
- Dufour, G.; Sicot, F.; Puigt, G.; Liauzun, C.; Dugeai, A. Contrasting the Harmonic Balance and Linearized Methods for Oscillating-Flap Simulations. AIAA J. 2010, 48, 788–797. [Google Scholar] [CrossRef]
- Thormann, R.; Widhalm, M. Linear-Frequency-Domain Predictions of Dynamic-Response Data for Viscous Transonic Flows. AIAA J. 2013, 51, 2540–2557. [Google Scholar] [CrossRef]
- Weishäupl, C.; Laschka, B. Small Disturbance Euler Simulations for Delta Wing Unsteady Flows due to Harmonic Oscillations. J. Aircr. 2004, 41, 782–789. [Google Scholar] [CrossRef]
- Da Ronch, A.; McCracken, A.; Badcock, K.J.; Widhalm, M.; Campobasso, M.S. Linear Frequency Domain and Harmonic Balance Predictions of Dynamic Derivatives. J. Aircr. 2013, 50, 694–707. [Google Scholar] [CrossRef]
- Revalor, Y.; Daumas, L.; Forestier, N. Industrial Use of CFD for Loads and Aero-Servo-Elastic Stability Computations at Dassault Aviation. In Proceedings of the 14th International Forum on Aeroelasticity and Structural Dynamics, Paris, France, 26–30 June 2011. [Google Scholar]
- Winter, M.; Heckmeier, F.M.; Breitsamter, C. CFD-based Aeroelastic Reduced-Order Modeling Robust to Structural Parameter Variations. Aerosp. Sci. Technol. 2017, 67, 13–30. [Google Scholar] [CrossRef]
- Albano, E.; Rodden, W.P. A Doublet-Lattice Method for Calculating Lift Distributions on Oscillating Surfaces in Subsonic Flows. AIAA J. 1969, 7, 279–285. [Google Scholar] [CrossRef]
- Giesing, J.P.; Kalman, T.P.; Rodden, W.P. Subsonic Unsteady Aerodynamics for General Configurations. In Proceedings of the 10th Aerospace Sciences Meeting, San Diego, CA, USA, 17–19 January 1972. [Google Scholar]
- Rodden, W.P.; Giesing, J.P.; Kalman, T.P. Refinement of the Nonplanar Aspects of the Subsonic Doublet-Lattice Lifting Surface Method. J. Aircr. 1972, 9, 69–73. [Google Scholar] [CrossRef]
- Wright, J.R.; Cooper, J.E. Introduction to Aircraft Aeroelasticity and Loads; Aerospace series; Wiley: Chichester, UK, 2008. [Google Scholar]
- Fleischer, D. Verfahren Reduzierter Ordnung zur Ermittlung Instationärer Luftkräfte. Ph.D. Thesis, Technische Universität München, München, Germany, 2014. [Google Scholar]
- Rozov, V.; Hermanutz, A.; Breitsamter, C.; Hornung, M. Aeroelastic Analysis of a Flutter Demonstrator with a Very Flexible High-Aspect-Ratio Swept Wing. In Proceedings of the 17th International Forum on Aeroelasticity and Structural Dynamics, Como, Italy, 25–28 June 2017. [Google Scholar]
- Wuestenhagen, M.; Kier, T.; Meddaikar, Y.M.; Pusch, M.; Ossmann, D.; Hermanutz, A. Aeroservoelastic Modeling and Analysis of a Highly Flexible Flutter Demonstrator. In 2018 Atmospheric Flight Mechanics Conference; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2018. [Google Scholar] [CrossRef]
- MSC. Software. Release Guide; MSC.Software Corporation: Newport Beach, CA, USA, 2018. [Google Scholar]
- Gürdal, Z.; Haftka, R.T.; Hajela, P. Design and optimization of laminated composite materials; A Wiley-Interscience Publication, Wiley: New York, NY, USA, 1999. [Google Scholar]
- MSC. Software. Aeroelastic Analysis User’s Guide; MSC.Software Corporation: Newport Beach, CA, USA, 2018. [Google Scholar]
- Duchon, J. Splines Minimizing Rotation-Invariant Semi-Norms in Sobolev Spaces. Construct. Theory Funct. Sev. Var 1977, 85–100. [Google Scholar]
- Lanczos, C. An Iteration Method for the Solution of the Eigenvalue Problem of Linear Differential and Integral Operators. J. Res. Natl. Bur. Stand. 1950, 45, 255–282. [Google Scholar] [CrossRef]
- ANSYS. ANSYS ICEM CFD User Manual; ANSYS, Inc.: Canonsburg, PA, USA, 2012. [Google Scholar]
- Rozov, V.; Winter, M.; Breitsamter, C. Antisymmetric Boundary Condition for Small Disturbance CFD. J. Fluids Struct. 2019, 85, 229–248. [Google Scholar] [CrossRef]
- Gordon, W.J.; Hall, C.A. Construction of Curvilinear Co-Ordinate Systems and Applications to Mesh Generation. Int. J. Nume. Methods Eng. 1973, 7, 461–477. [Google Scholar] [CrossRef]
- Hassig, H.J. An Approximate True Damping Solution of the Flutter Equation by Determinant Iteration. J. Aircr. 1971, 8, 885–889. [Google Scholar] [CrossRef]


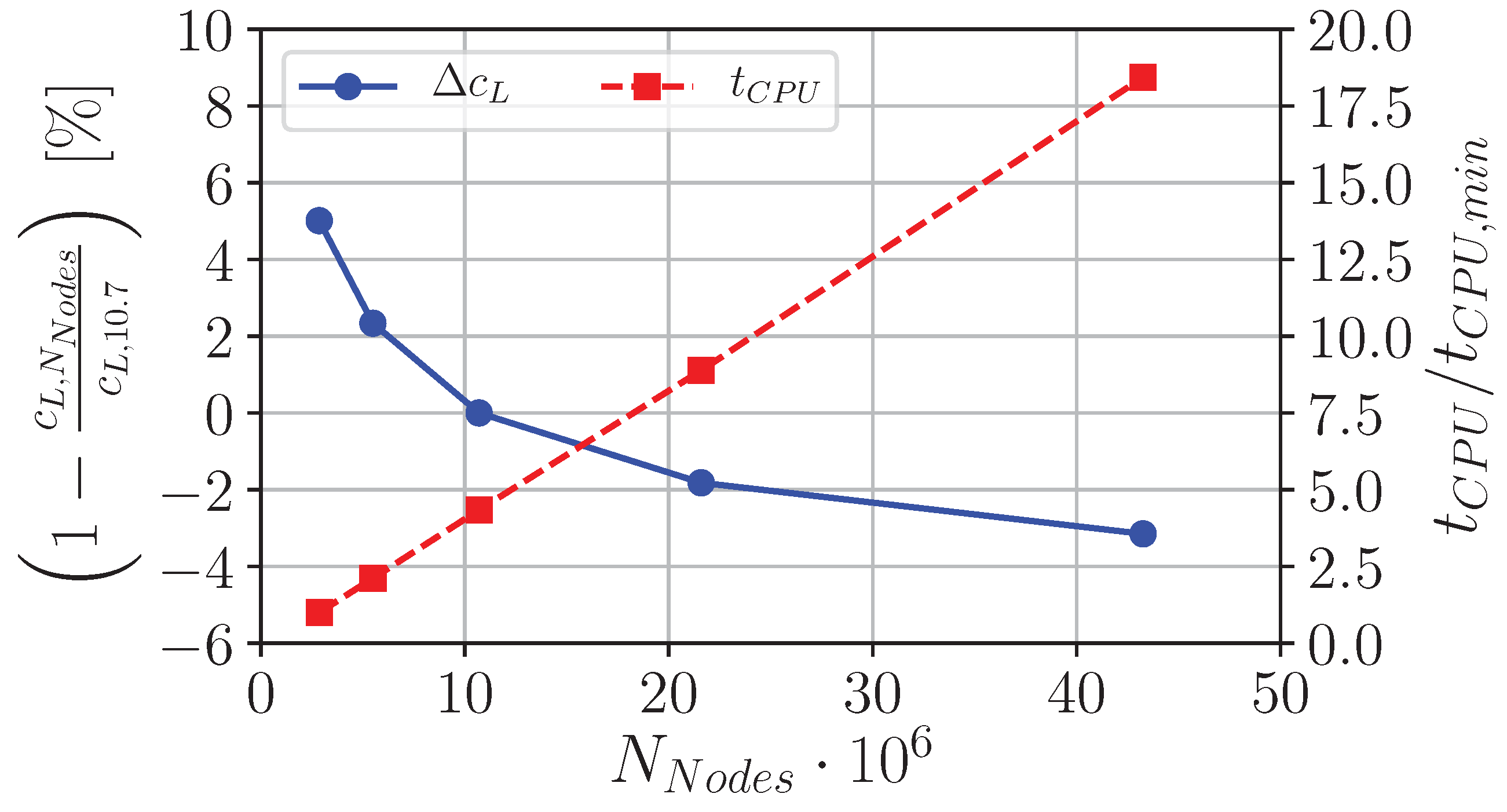
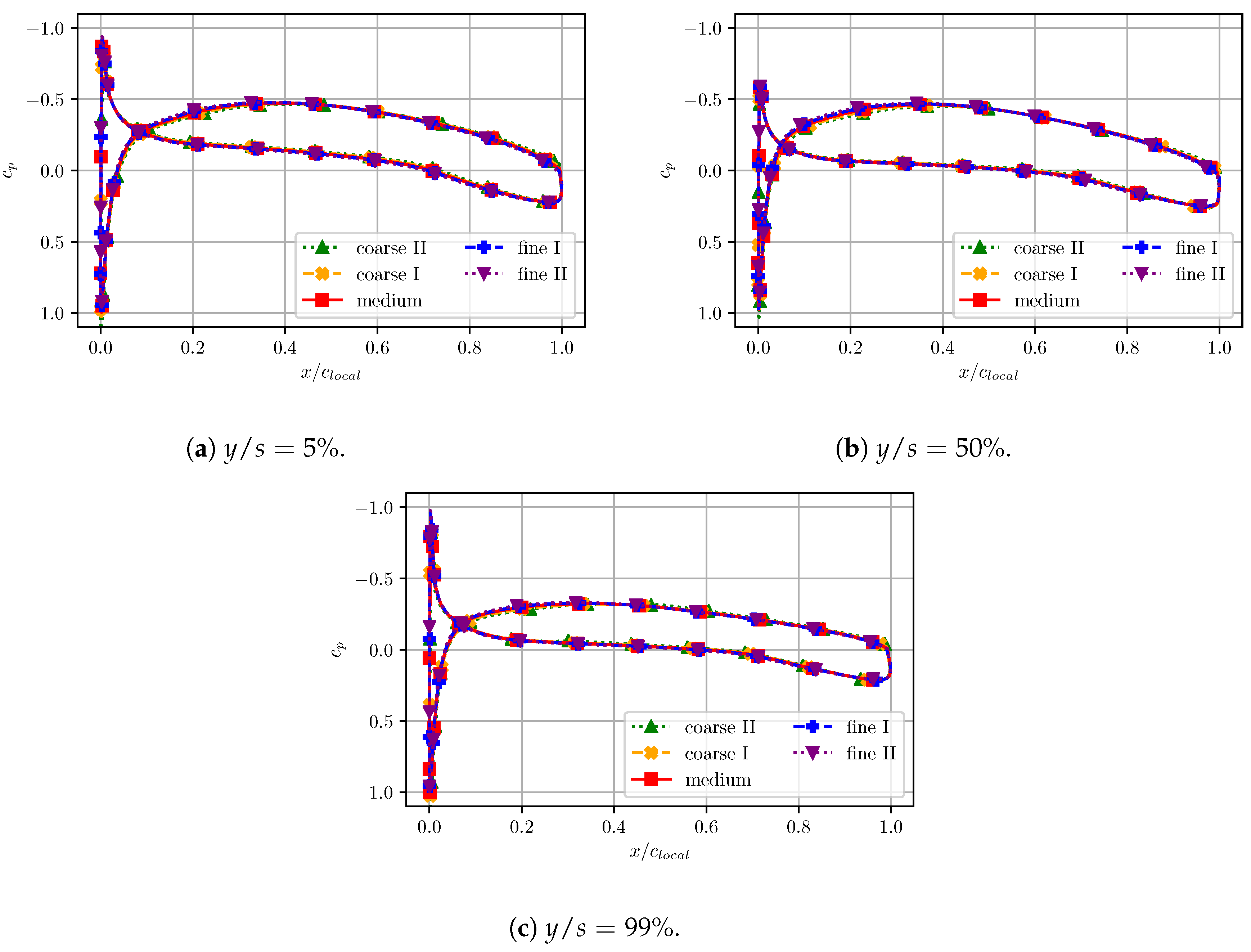
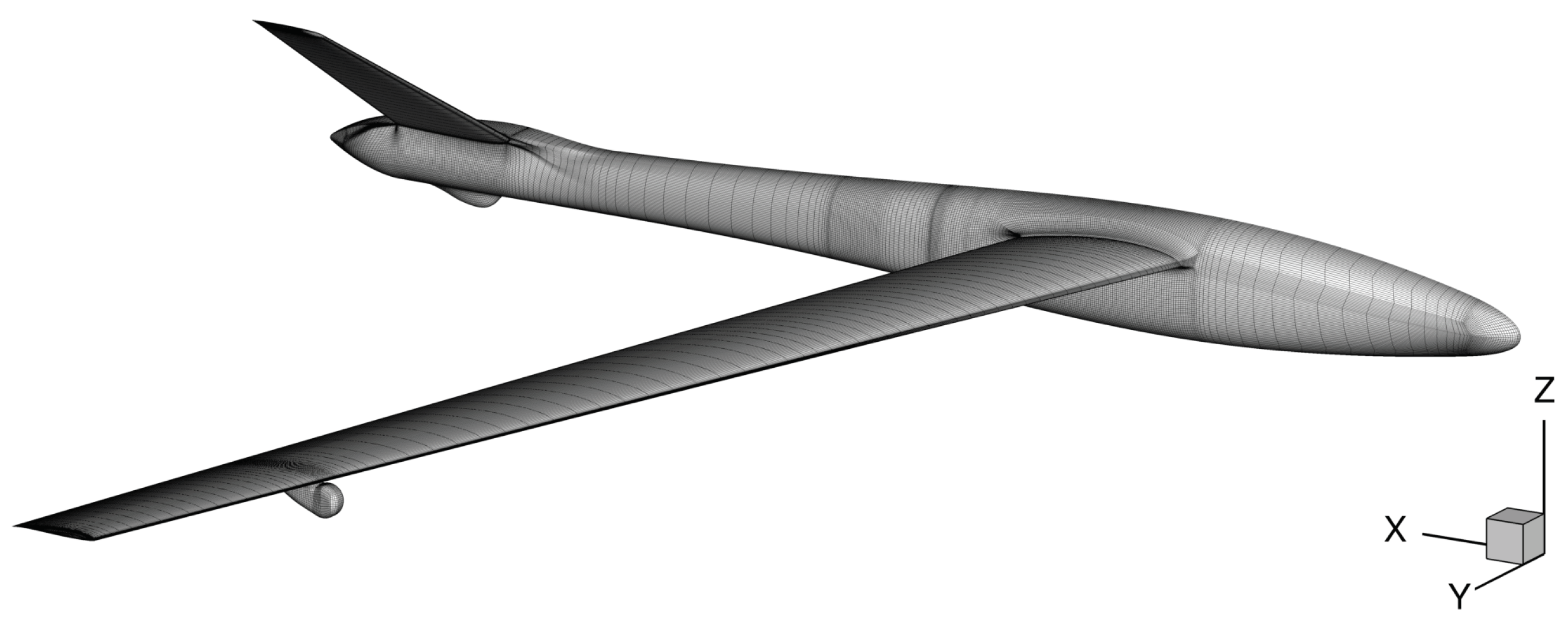

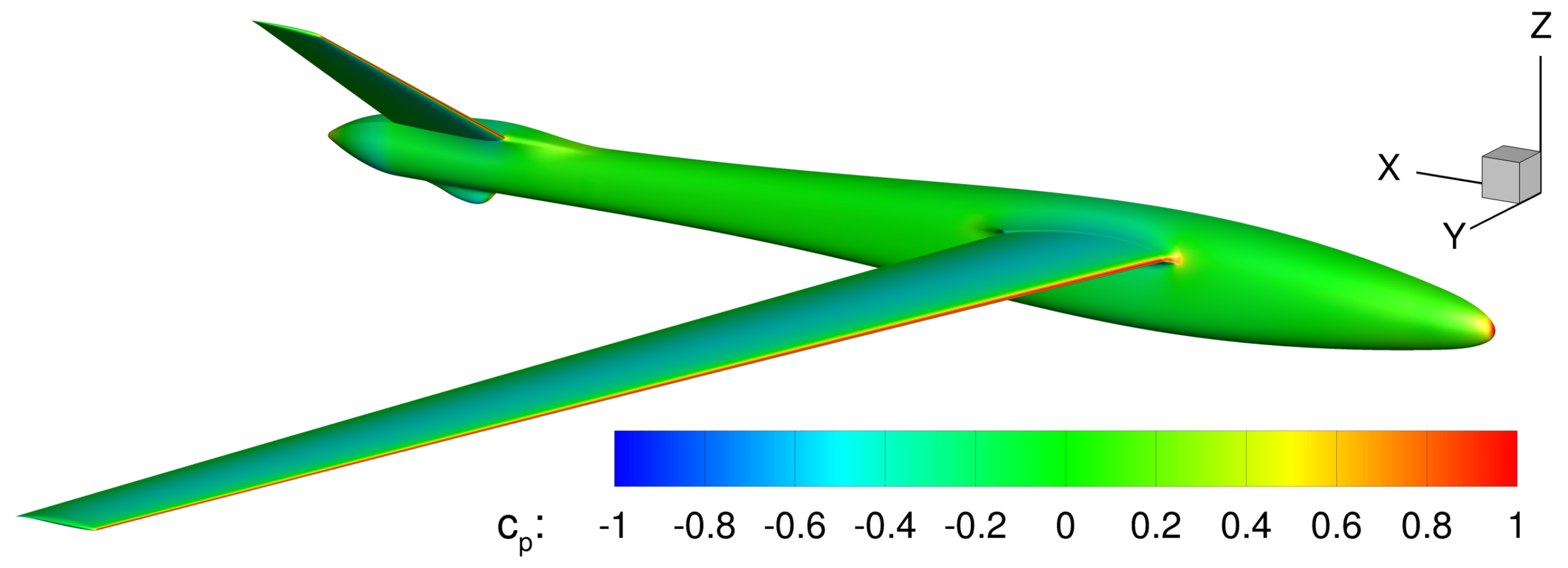

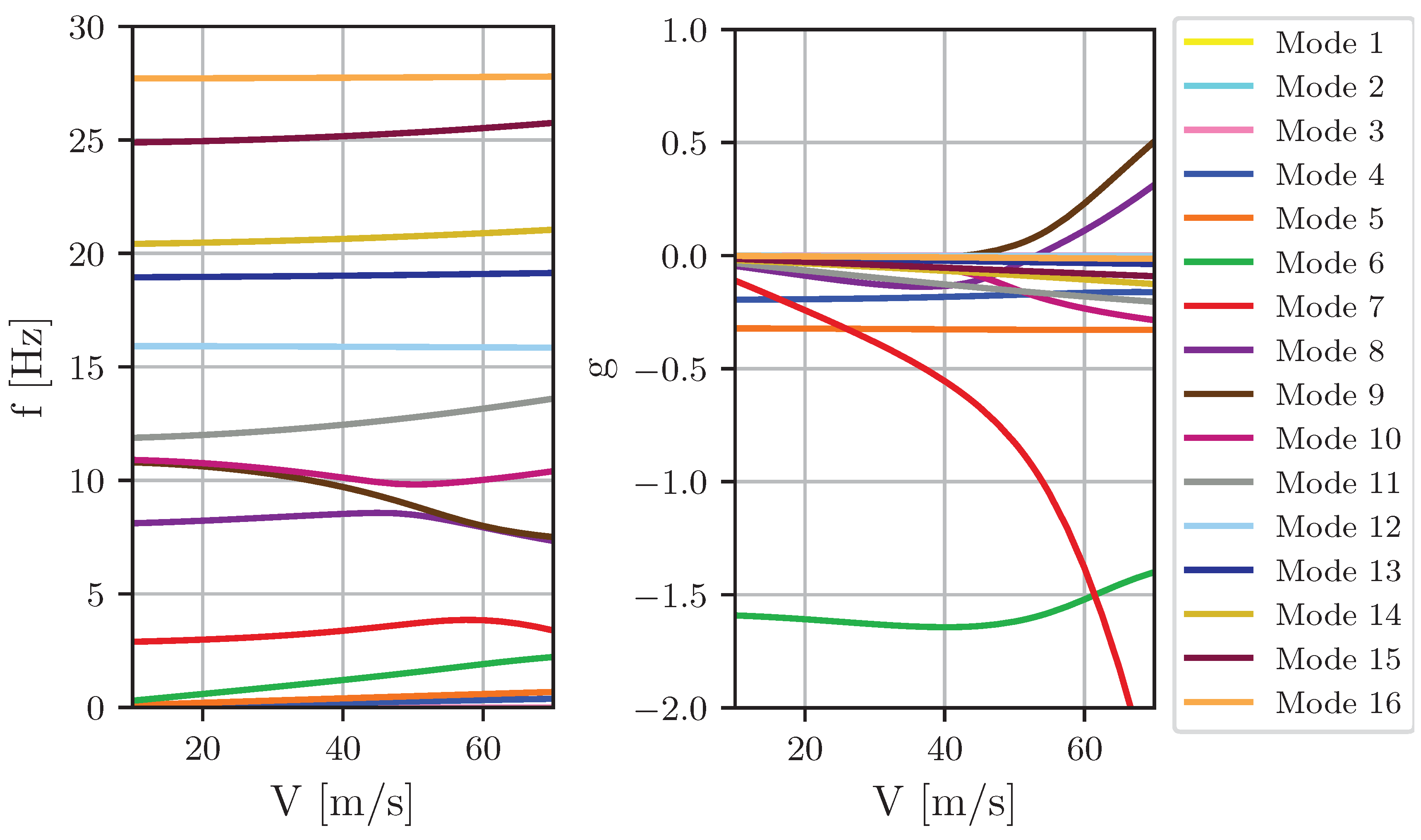
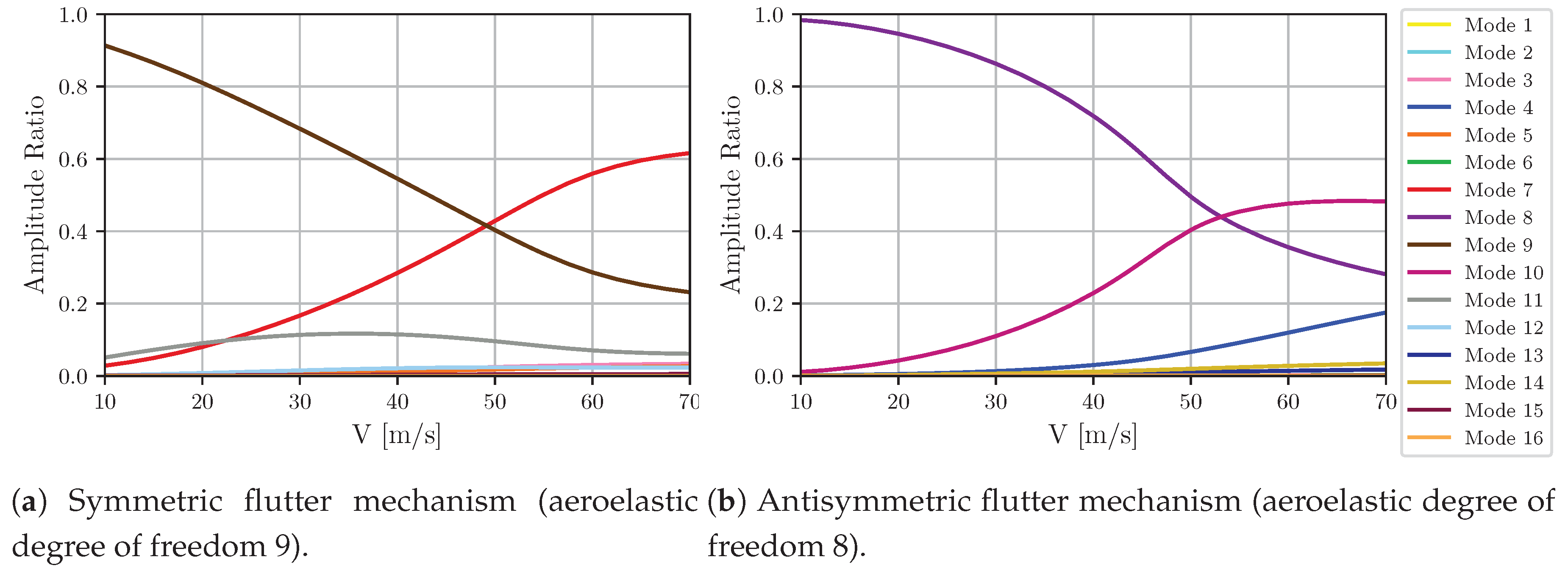
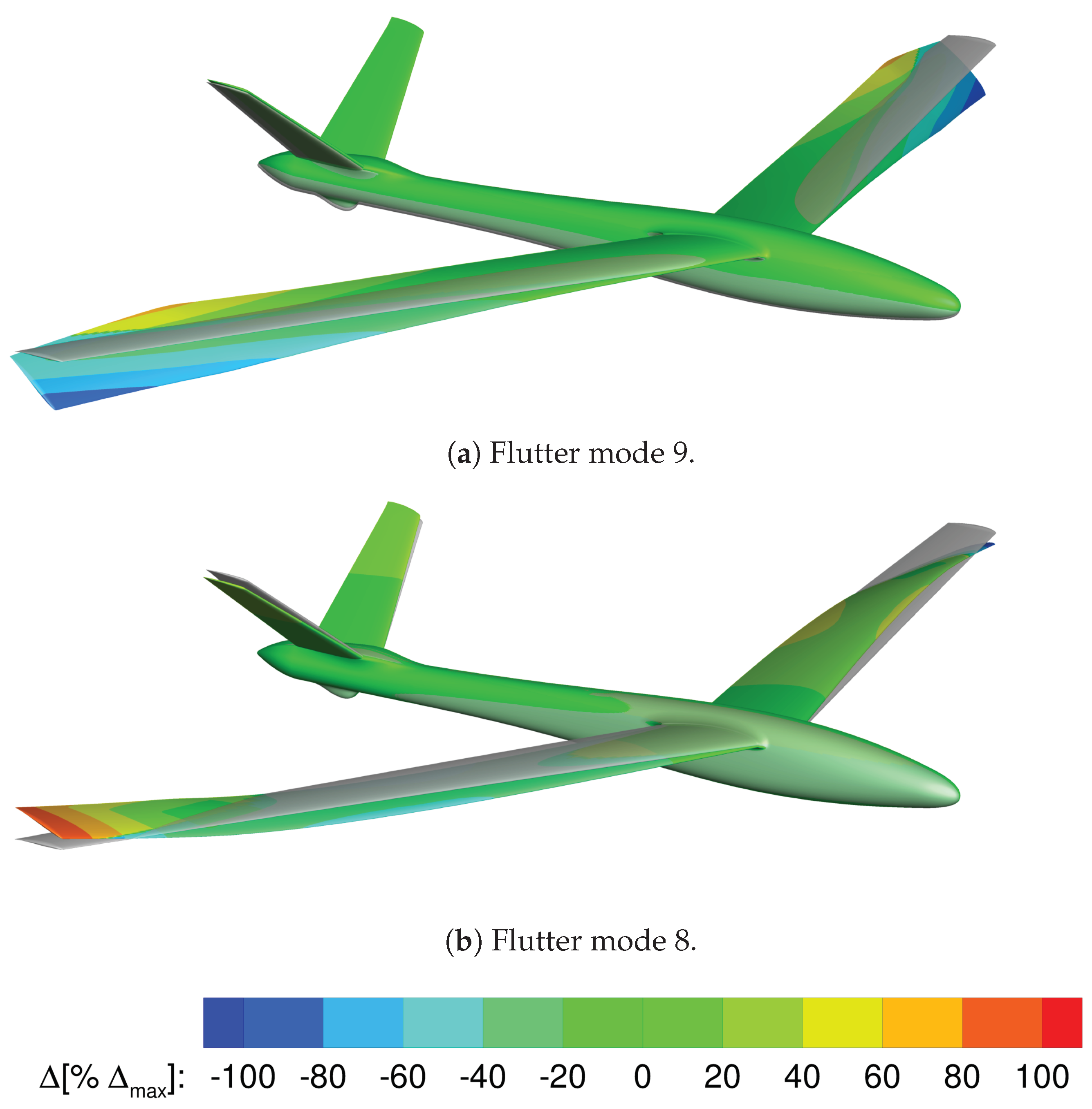

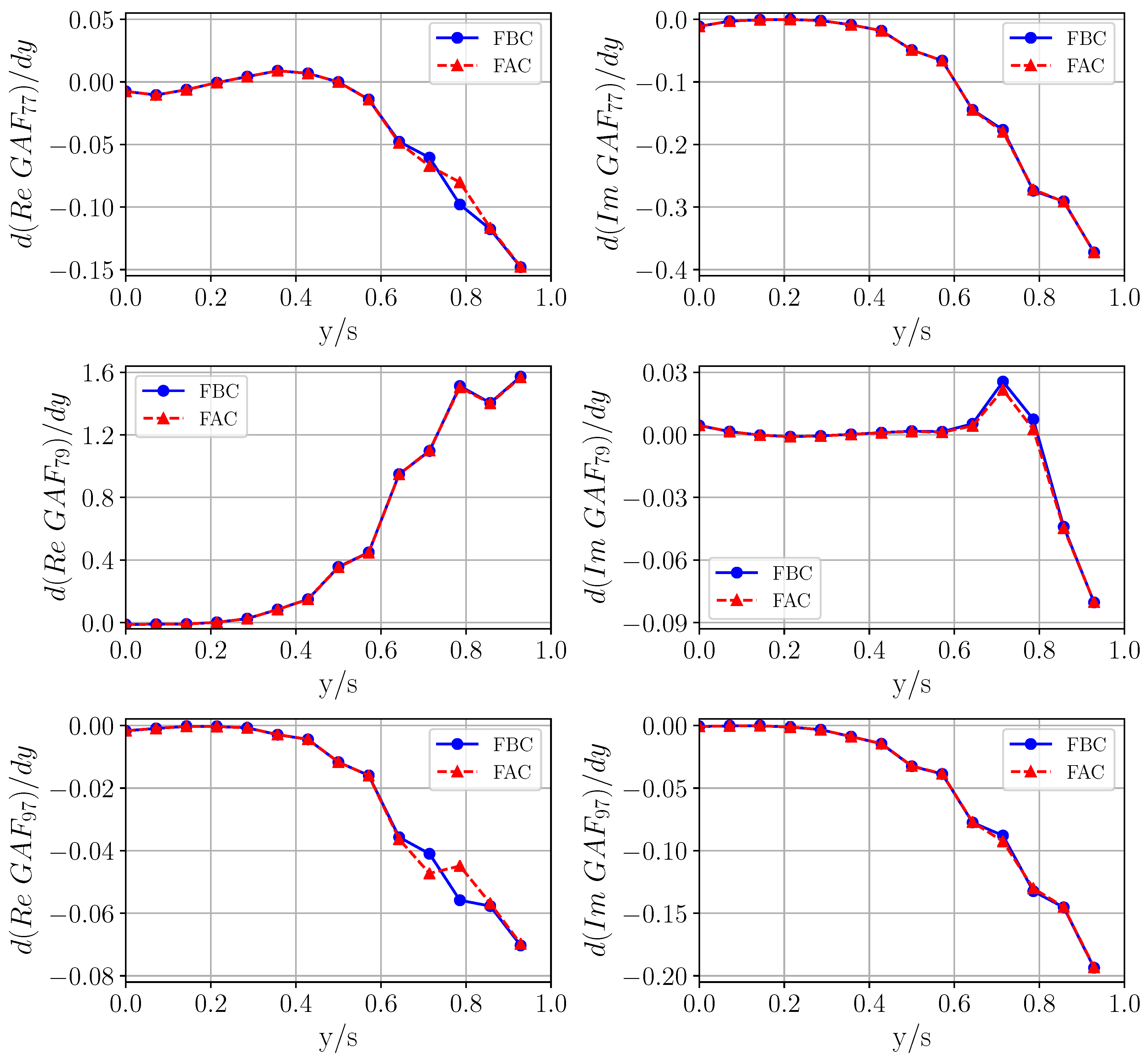


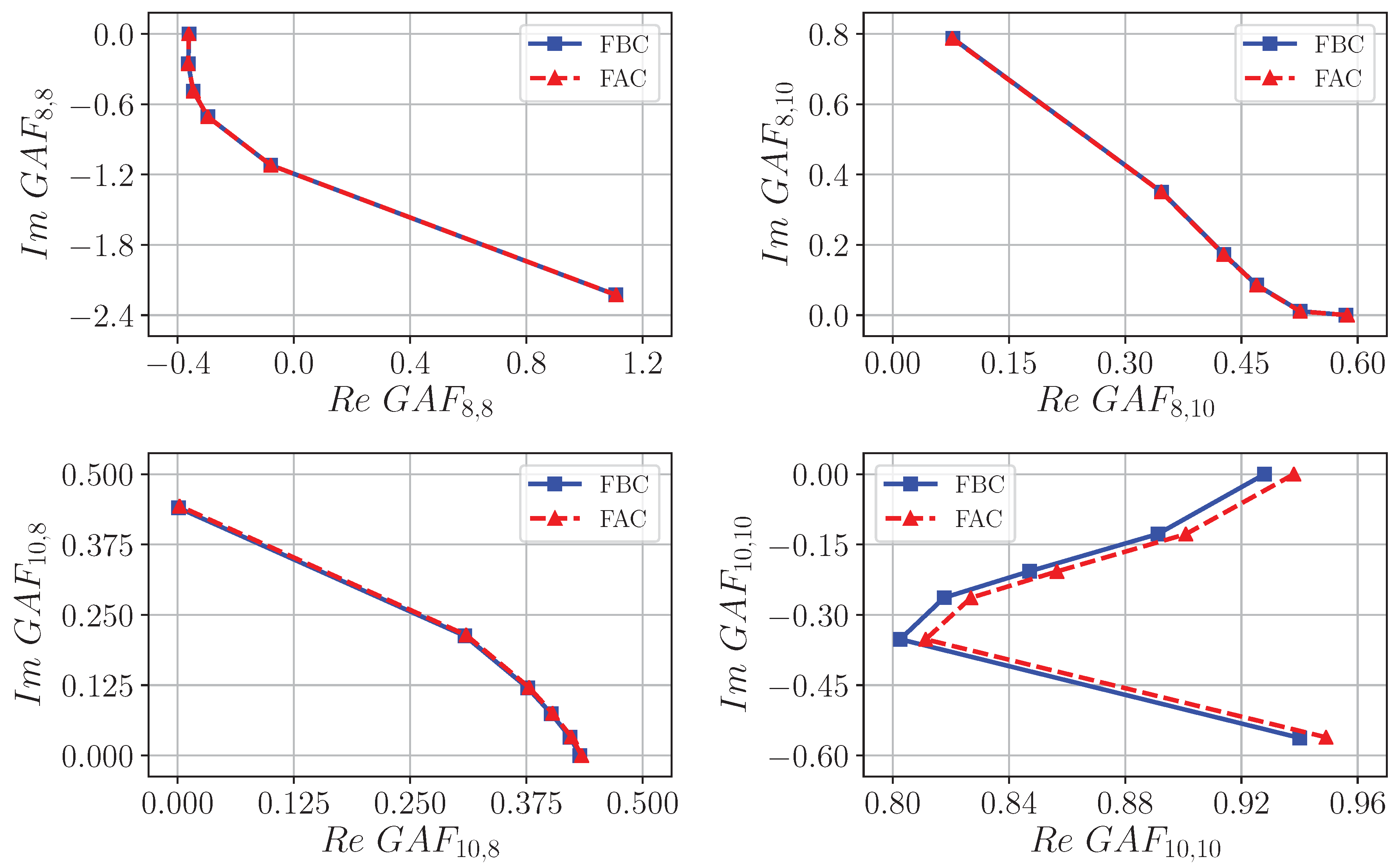

| Symmetric Boundary Condition | Antisymmetric Boundary Condition |
|---|---|
| Mode 9 | Mode 8 | |
|---|---|---|
| Mode 9 | Mode 8 | ||||
|---|---|---|---|---|---|
| SD-CFD | DLM | SD-CFD | DLM | ||
| − | − | ||||
| − | − | ||||
| − | − | ||||
| Mode 9 | Mode 8 | ||||
|---|---|---|---|---|---|
| FBC | FAC | FBC | FAC | ||
| − | − | ||||
| − | − | ||||
| − | − | ||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rozov, V.; Volmering, A.; Hermanutz, A.; Hornung, M.; Breitsamter, C. CFD-Based Aeroelastic Sensitivity Study of a Low-Speed Flutter Demonstrator. Aerospace 2019, 6, 30. https://doi.org/10.3390/aerospace6030030
Rozov V, Volmering A, Hermanutz A, Hornung M, Breitsamter C. CFD-Based Aeroelastic Sensitivity Study of a Low-Speed Flutter Demonstrator. Aerospace. 2019; 6(3):30. https://doi.org/10.3390/aerospace6030030
Chicago/Turabian StyleRozov, Vladyslav, Andreas Volmering, Andreas Hermanutz, Mirko Hornung, and Christian Breitsamter. 2019. "CFD-Based Aeroelastic Sensitivity Study of a Low-Speed Flutter Demonstrator" Aerospace 6, no. 3: 30. https://doi.org/10.3390/aerospace6030030
APA StyleRozov, V., Volmering, A., Hermanutz, A., Hornung, M., & Breitsamter, C. (2019). CFD-Based Aeroelastic Sensitivity Study of a Low-Speed Flutter Demonstrator. Aerospace, 6(3), 30. https://doi.org/10.3390/aerospace6030030





