Figure 1.
Seagull Arising Star V2 Unmanned Aerial Vehicle (UAV) platform [
11].
Figure 1.
Seagull Arising Star V2 Unmanned Aerial Vehicle (UAV) platform [
11].
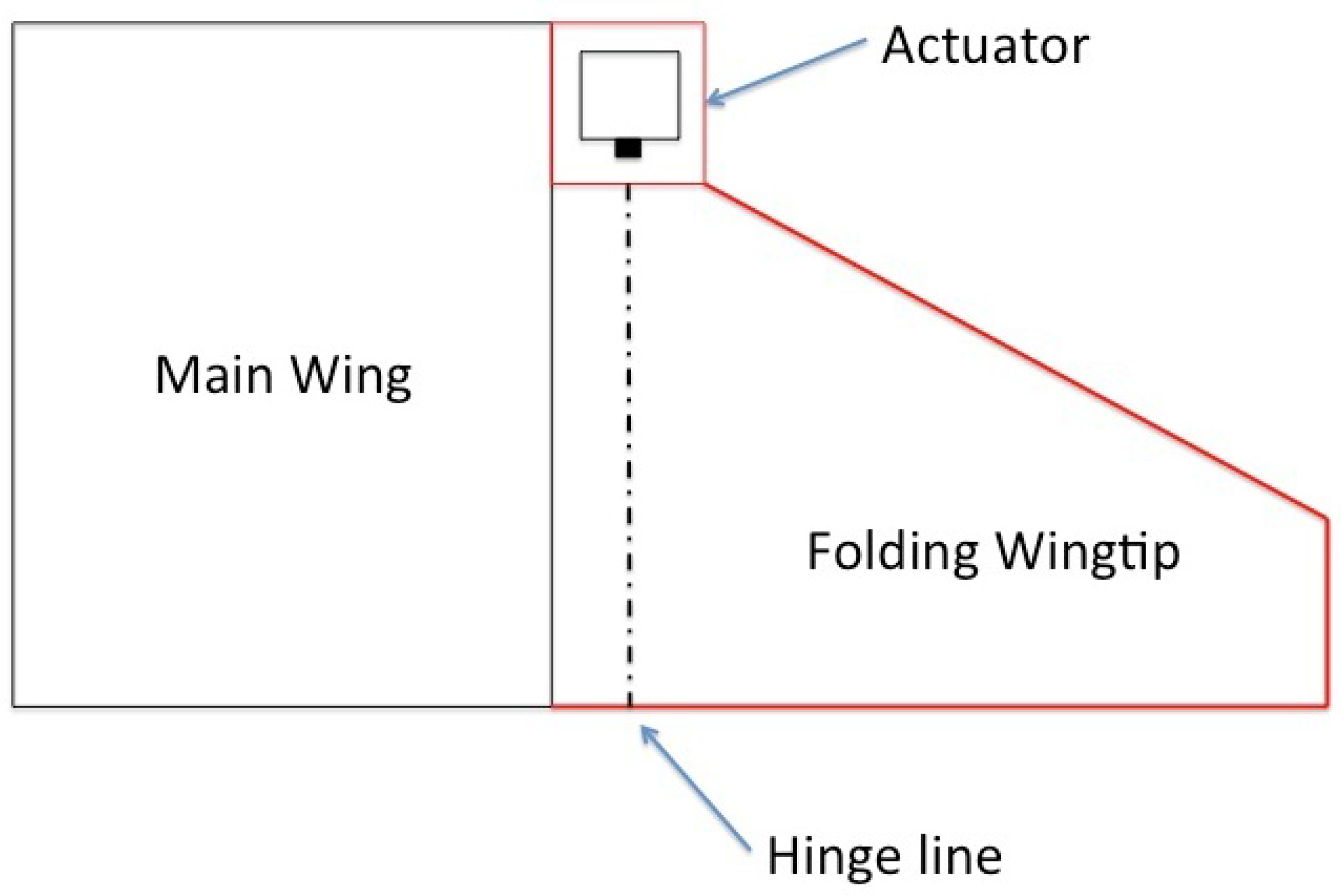
Figure 2.
Concept 1 utilising hinge line actuators (top view).
Figure 2.
Concept 1 utilising hinge line actuators (top view).
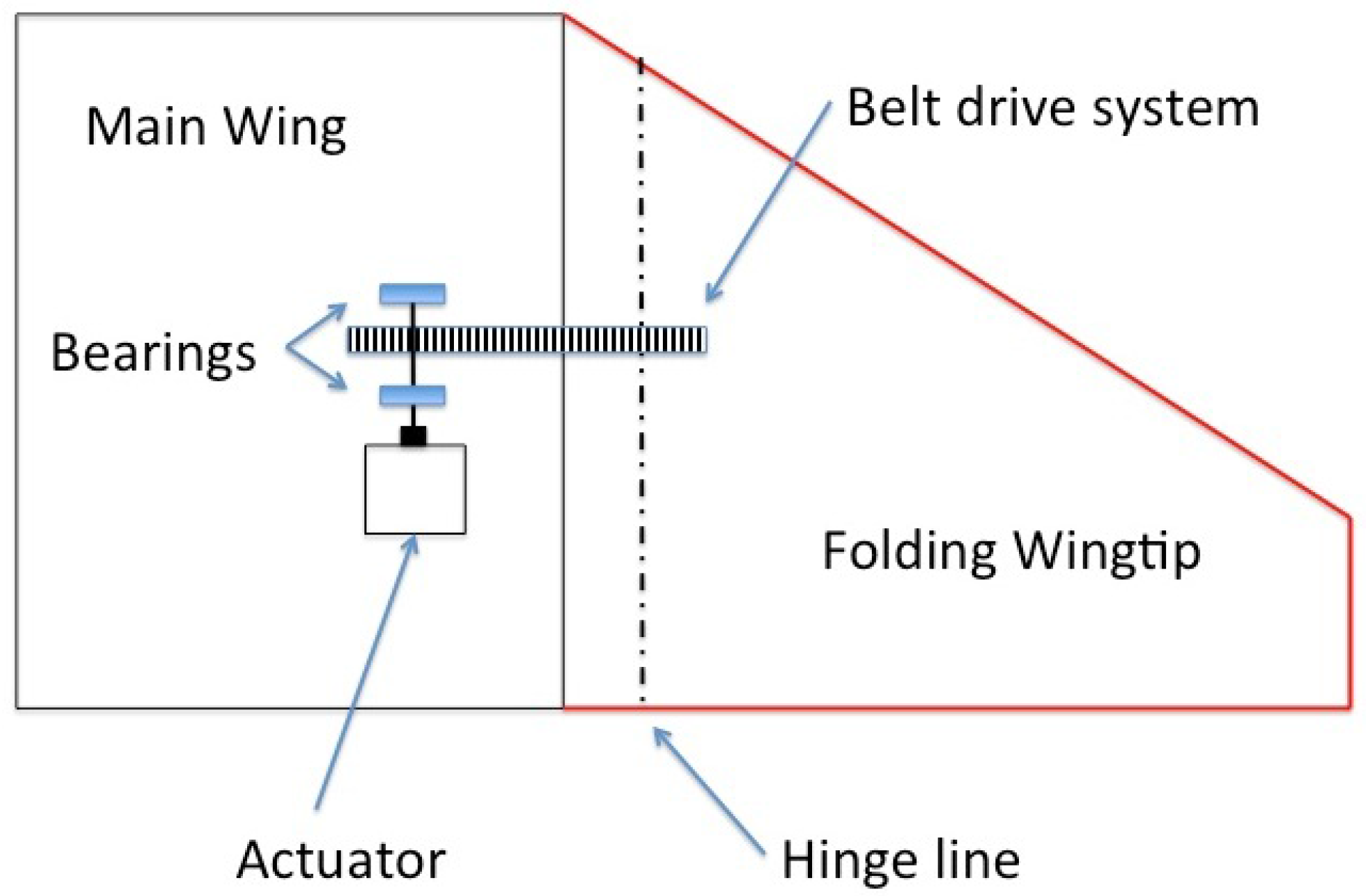
Figure 3.
Concept 2 using a system of pulley gears (top view).
Figure 3.
Concept 2 using a system of pulley gears (top view).
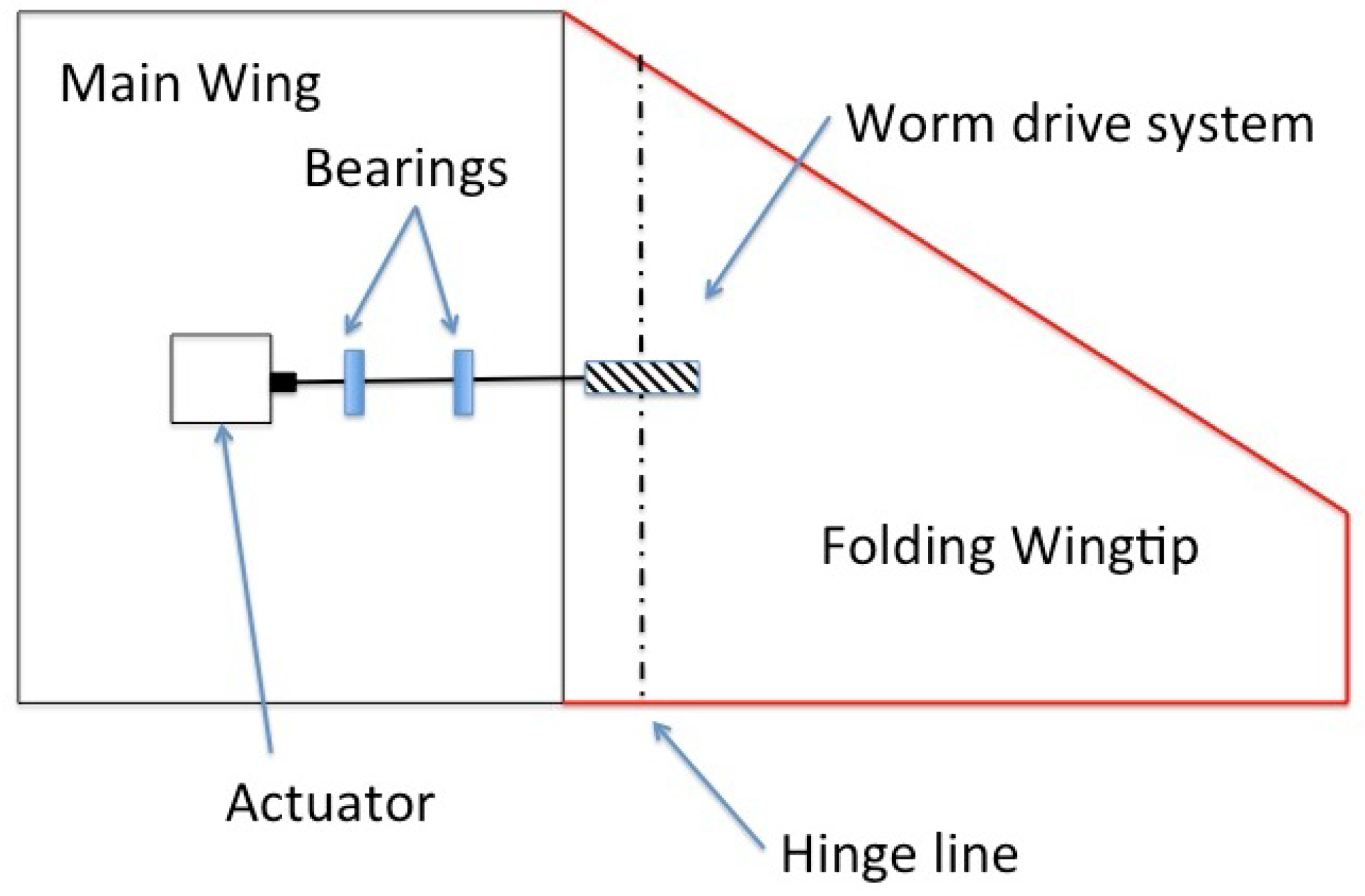
Figure 4.
Concept 3 using a worm gear system (top view).
Figure 4.
Concept 3 using a worm gear system (top view).
Figure 5.
Flexible latex skin investigation.
Figure 5.
Flexible latex skin investigation.
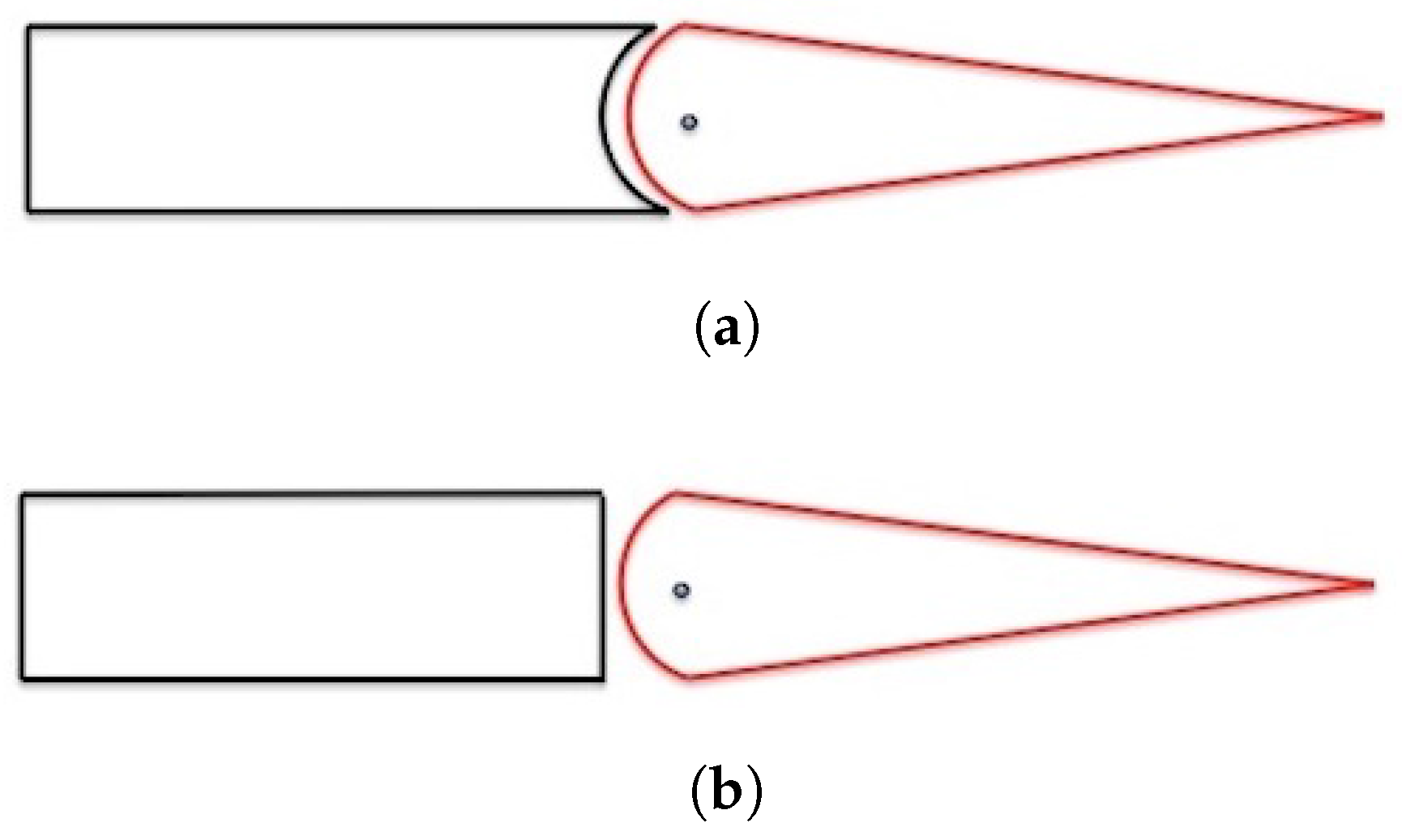
Figure 6.
Gap reduction hinge designs. (a) Aileron-style hinge with encapsulating inboard section preventing full range of motion (front view); (b) selected hinge style to enable full range of motion (front view).
Figure 6.
Gap reduction hinge designs. (a) Aileron-style hinge with encapsulating inboard section preventing full range of motion (front view); (b) selected hinge style to enable full range of motion (front view).
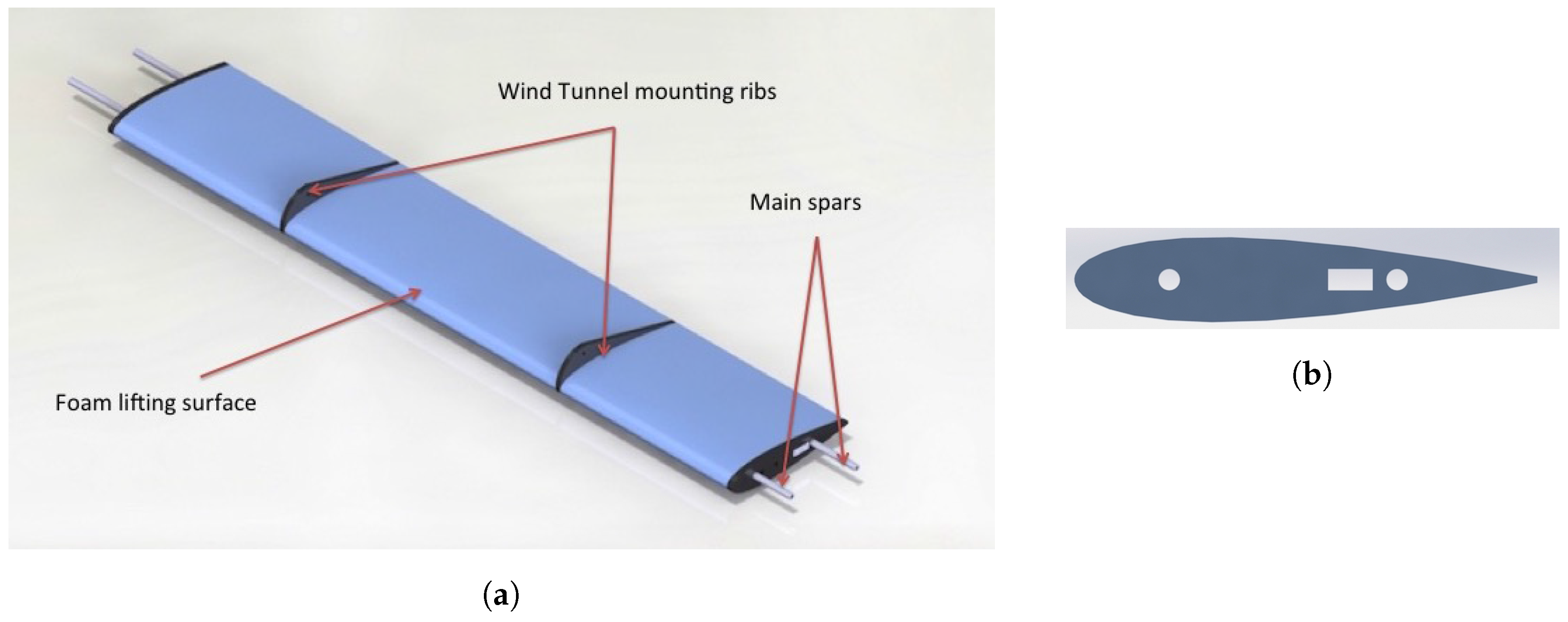
Figure 7.
Main wing structural layout. (a) Wing structure including main spars and ribs; (b) main wing cross-section showing internal cut outs for main spars and electrical cable runs.
Figure 7.
Main wing structural layout. (a) Wing structure including main spars and ribs; (b) main wing cross-section showing internal cut outs for main spars and electrical cable runs.
Figure 8.
Full prototype design.
Figure 8.
Full prototype design.
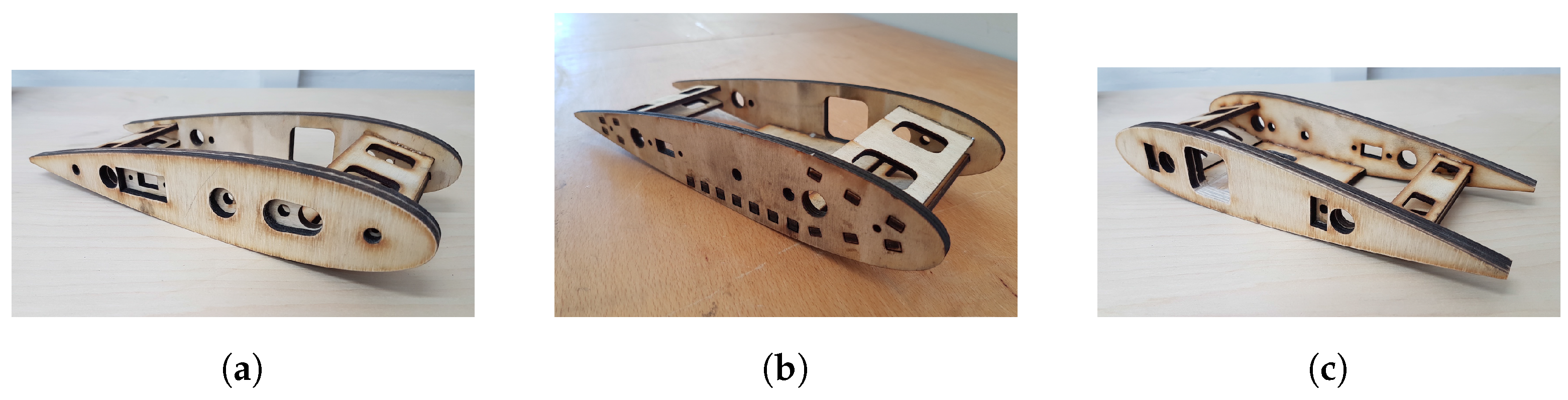
Figure 9.
FOLDERONS structure manufactured from 6-mm birch plywood. (a) Inboard side of the FOLDERONS structure; (b) internal joint structure; (c) outboard side of the FOLDERONS structure.
Figure 9.
FOLDERONS structure manufactured from 6-mm birch plywood. (a) Inboard side of the FOLDERONS structure; (b) internal joint structure; (c) outboard side of the FOLDERONS structure.
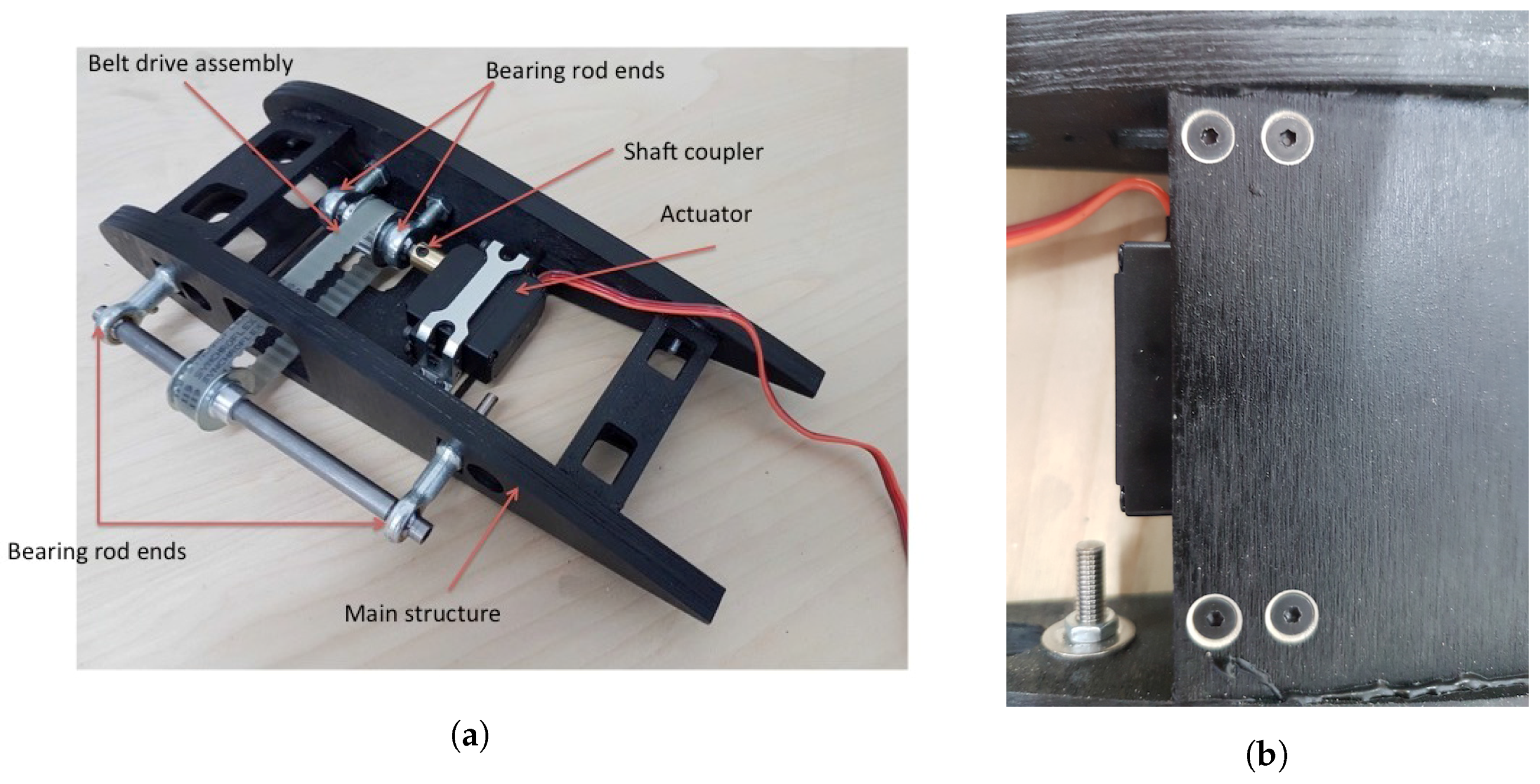
Figure 10.
Final installation of the servo motor actuator. (a) Final actuator installation; (b) countersunk actuator mounting bolts.
Figure 10.
Final installation of the servo motor actuator. (a) Final actuator installation; (b) countersunk actuator mounting bolts.
Figure 11.
Assembled modular tip unit.
Figure 11.
Assembled modular tip unit.
Figure 12.
Assembled wing.
Figure 12.
Assembled wing.
Figure 13.
Arduino controller used during testing. (a) A schematic to show the electrical connections of 2 servo motors and an Arduino; (b) the Arduino layout during tunnel testing.
Figure 13.
Arduino controller used during testing. (a) A schematic to show the electrical connections of 2 servo motors and an Arduino; (b) the Arduino layout during tunnel testing.
Figure 14.
UAV Installation in the RJ Mitchell Tunnel.
Figure 14.
UAV Installation in the RJ Mitchell Tunnel.
Figure 15.
An example of static deflection cases. (a) Symmetric deflection; (b) asymmetric deflection.
Figure 15.
An example of static deflection cases. (a) Symmetric deflection; (b) asymmetric deflection.
Figure 16.
Zero degrees AOA static test results.
Figure 16.
Zero degrees AOA static test results.
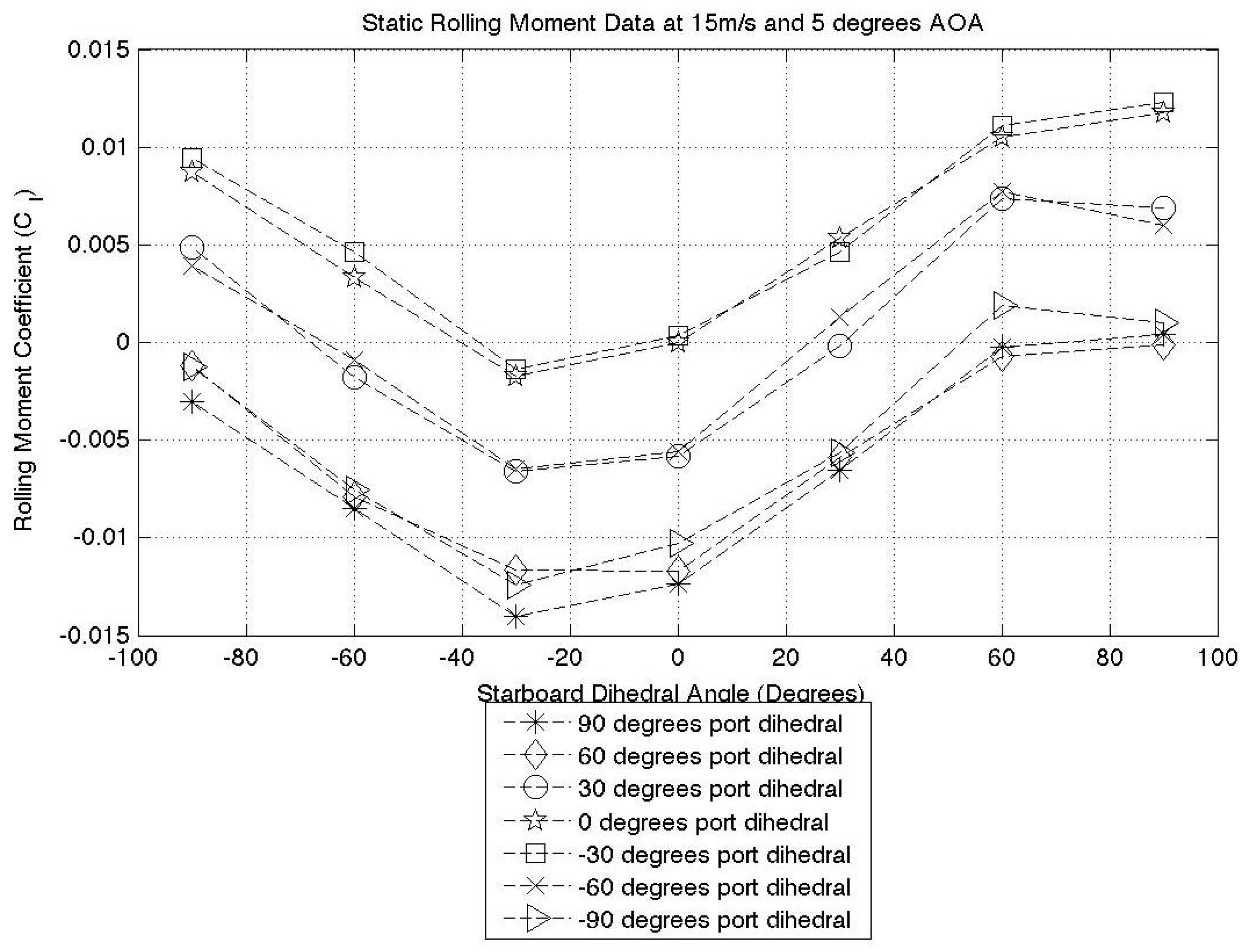
Figure 17.
Five degrees AOA static test results.
Figure 17.
Five degrees AOA static test results.
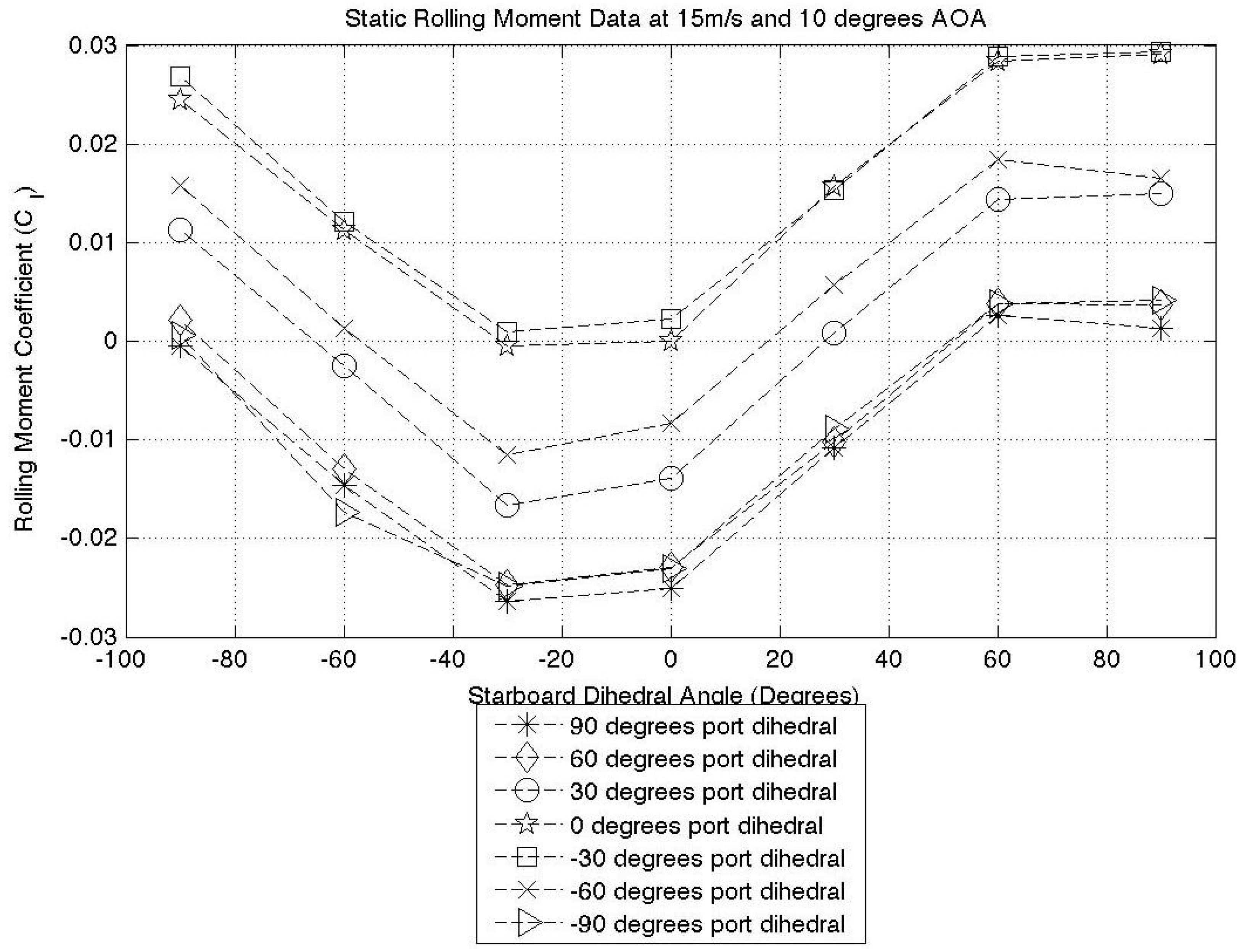
Figure 18.
Ten degrees AOA static test results.
Figure 18.
Ten degrees AOA static test results.
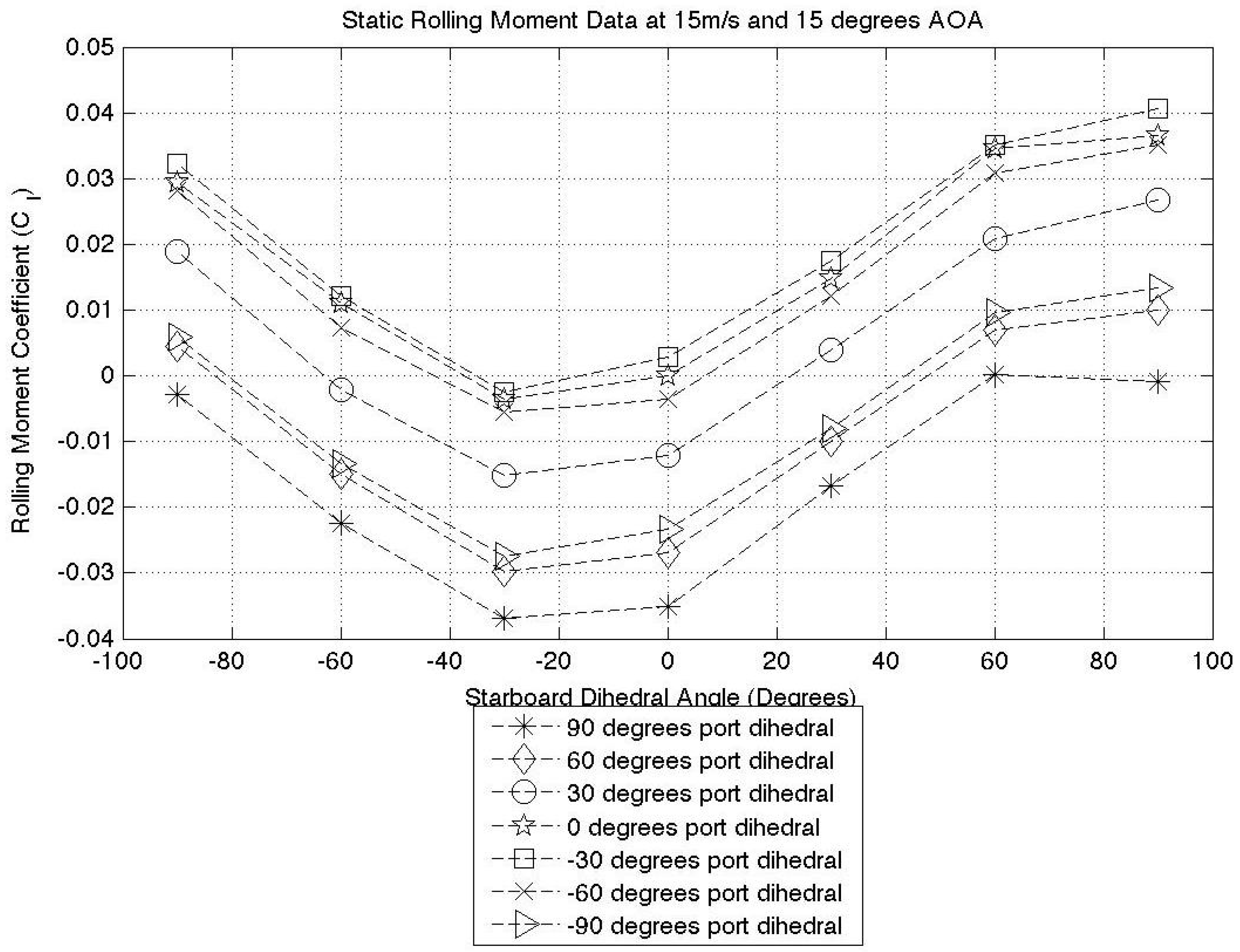
Figure 19.
Fifteen degrees AOA static test results.
Figure 19.
Fifteen degrees AOA static test results.
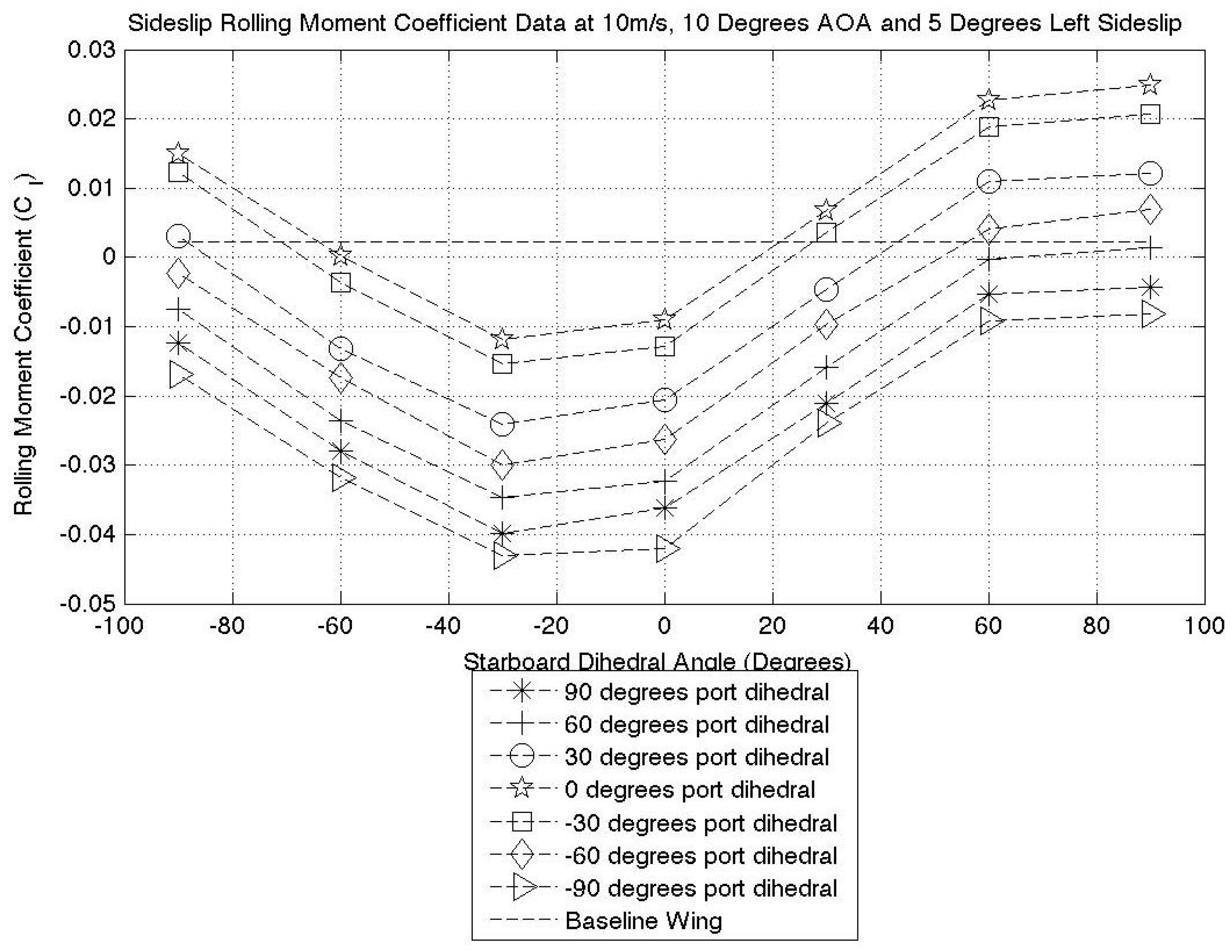
Figure 20.
Five degrees sideslip rolling moment coefficient.
Figure 20.
Five degrees sideslip rolling moment coefficient.
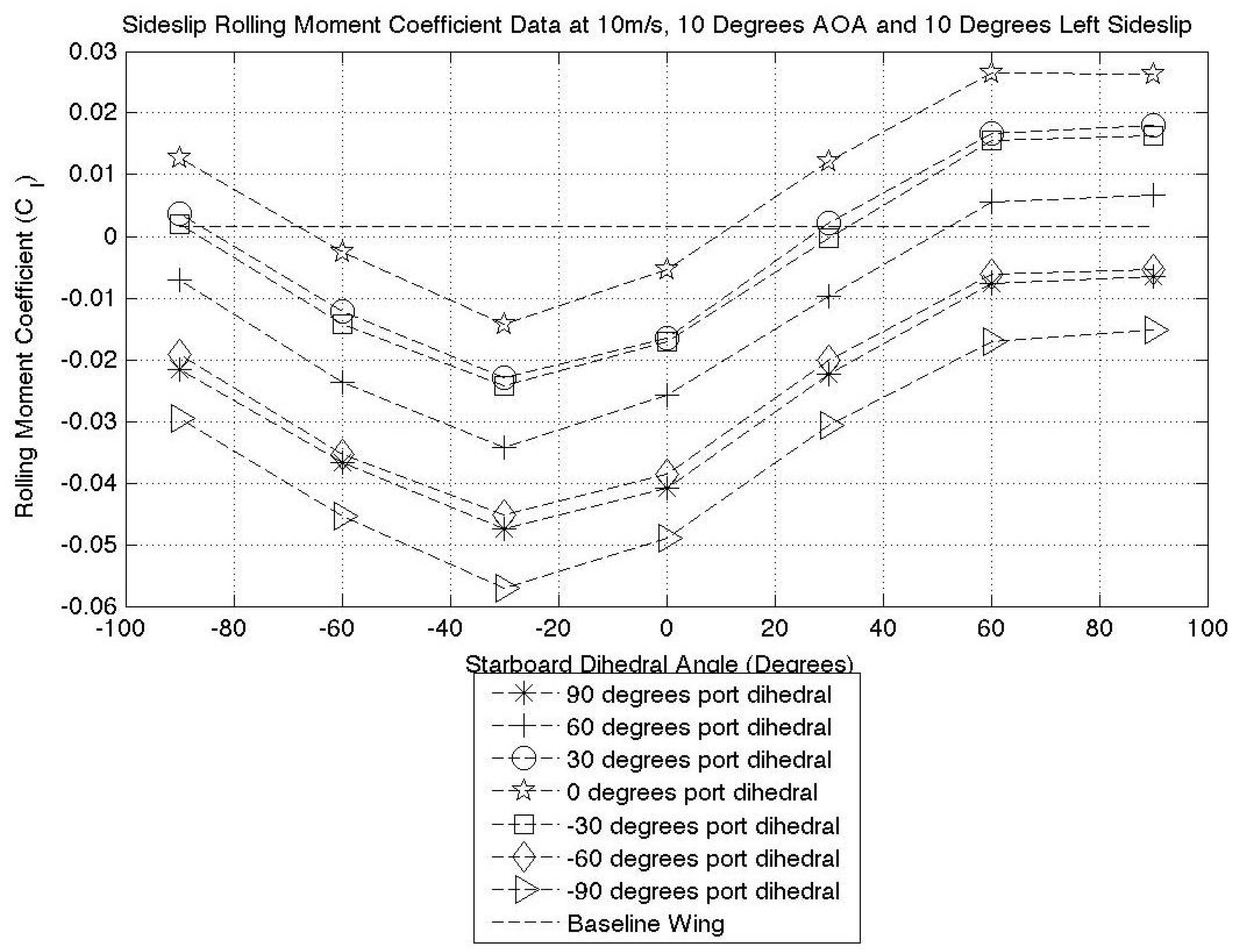
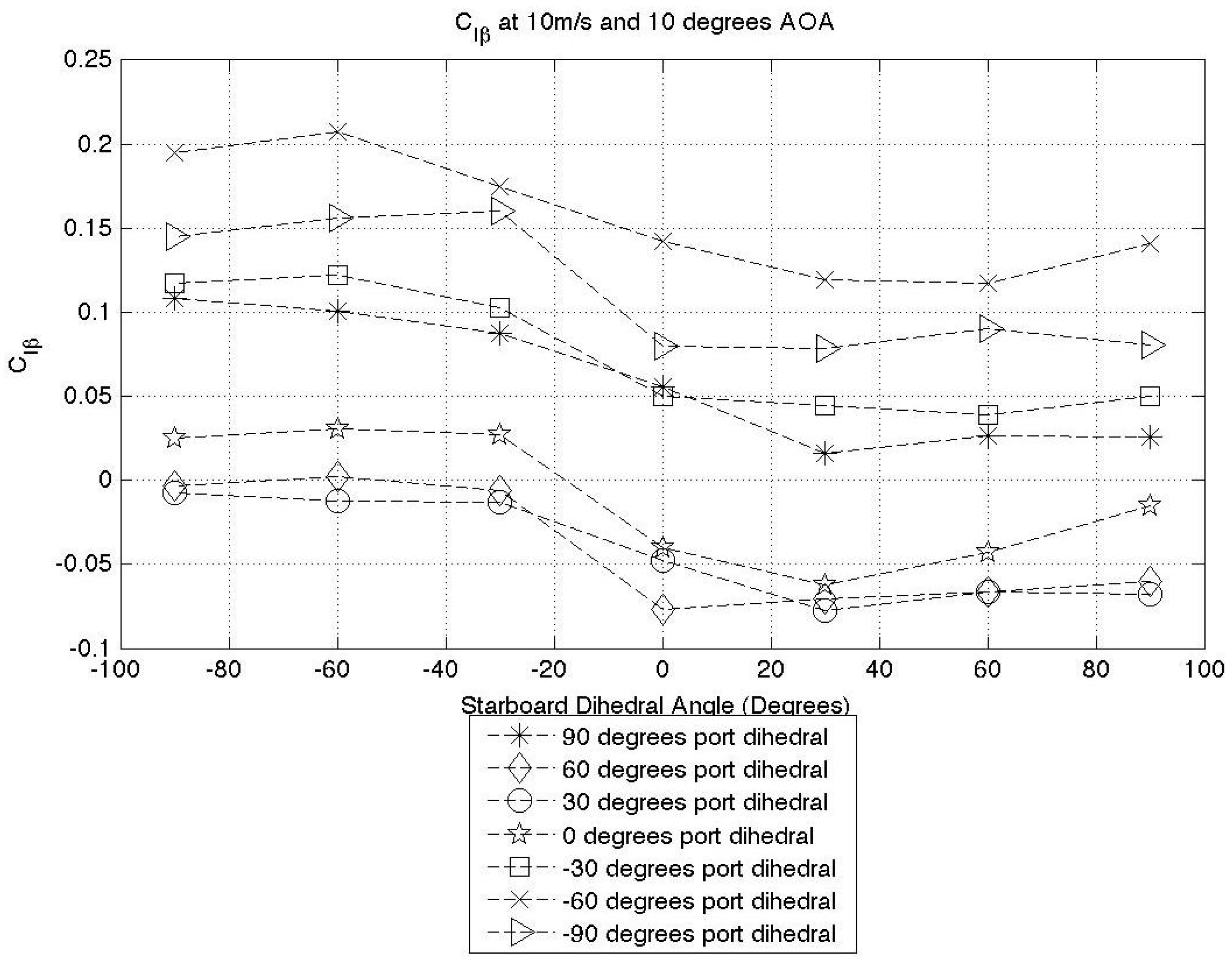
Figure 21.
Ten degrees sideslip rolling moment coefficient.
Figure 21.
Ten degrees sideslip rolling moment coefficient.
Figure 22.
Lateral stability derivative variation.
Figure 22.
Lateral stability derivative variation.
Figure 23.
Five degrees sideslip yawing moment coefficient.
Figure 23.
Five degrees sideslip yawing moment coefficient.
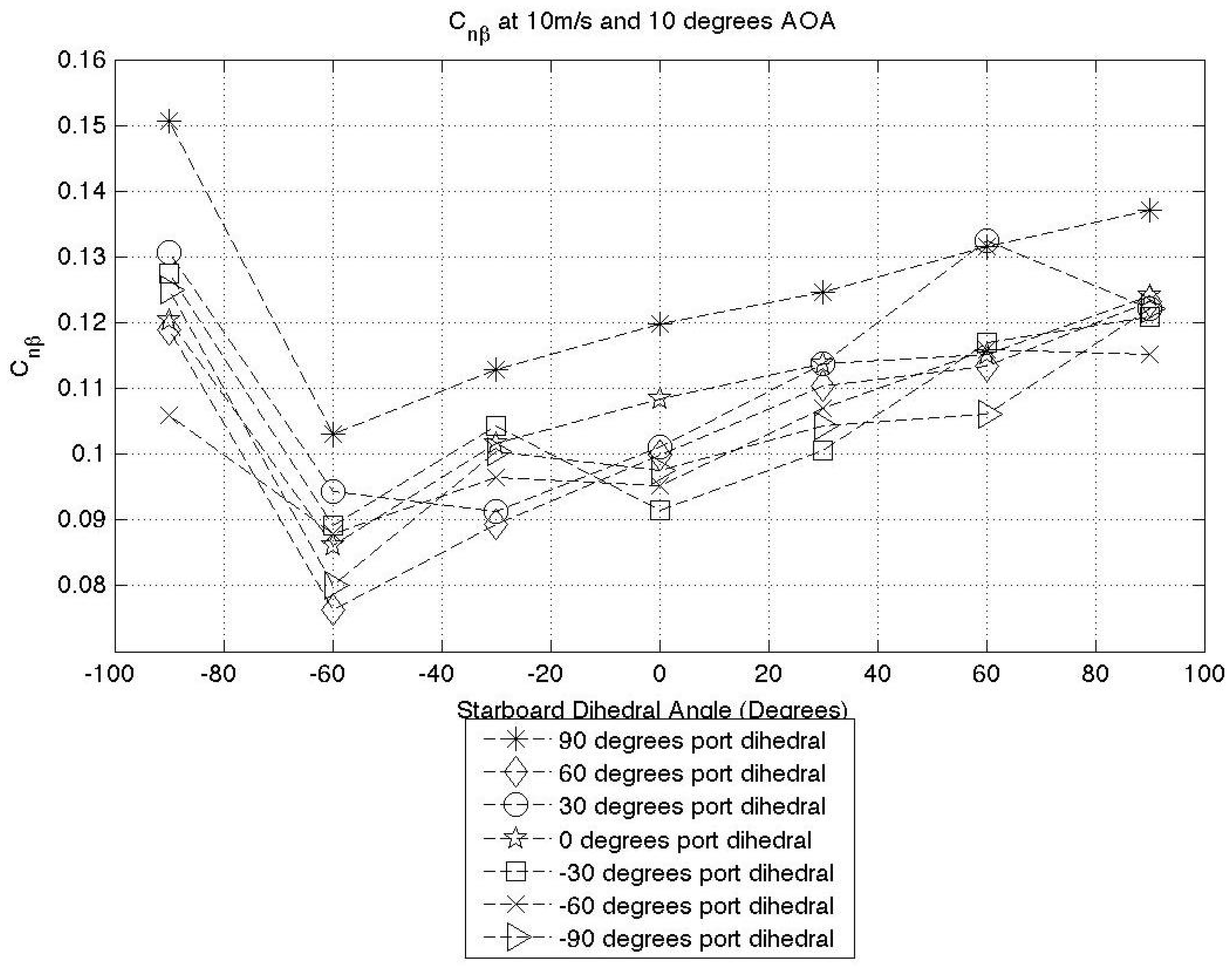
Figure 24.
Ten degrees sideslip yawing moment coefficient.
Figure 24.
Ten degrees sideslip yawing moment coefficient.
Figure 25.
Directional stability derivative variation.
Figure 25.
Directional stability derivative variation.
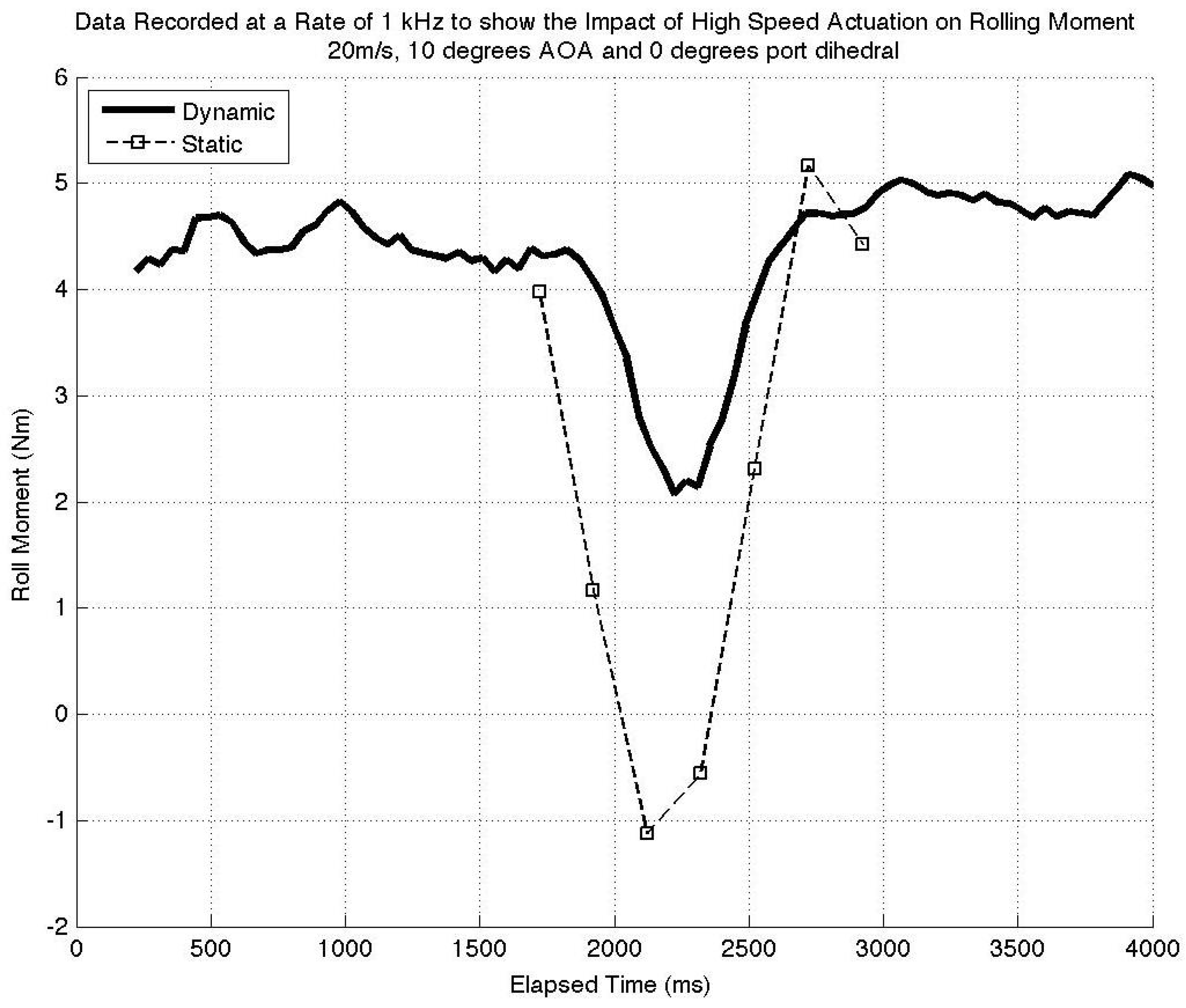
Figure 26.
Dynamic vs. static results.
Figure 26.
Dynamic vs. static results.
Table 1.
Winglet’s design parameters [
4].
Table 1.
Winglet’s design parameters [
4].
| Parameter | Limits | Units |
|---|
| Dihedral | | Degrees |
| Twist | | Degrees |
| Sweep | | Degrees |
Table 2.
Baseline wing specification and flight speed of the UAV.
Table 2.
Baseline wing specification and flight speed of the UAV.
| Parameter | Value | Units |
|---|
| Wing Span | 1.6 | m |
| Wing Area | 0.416 | m |
| Average Chord | 0.26 | m |
| Maximum Flight Speed | 20 | m/s |
Table 3.
FOLDERONS geometry.
Table 3.
FOLDERONS geometry.
| Parameter | Value | Units |
|---|
| Span | 0.26 | m |
| Root Chord | 0.26 | m |
| Tip Chord | 0.1 | m |
| Leading Edge Sweep | 38 | Degrees |
| Span of Modified Wing (with FOLDERONS in-plane) | 2.12 | m |
Table 4.
Suitability of the designs to meet the requirements.
Table 4.
Suitability of the designs to meet the requirements.
| Project Requirement | Normalised Importance of Project Requirement | Sustainability of Design 1 to Meet Project Specification | Sustainability of Design 2 to Meet Project Specification | Sustainability of Design 3 to Meet Project Specification |
|---|
| Effectiveness | 33.30% | 0.7 | 0.8 | 0.5 |
| Cost | 26.70% | 0.6 | 0.6 | 0.6 |
| Complexity | 20.00% | 0.7 | 0.8 | 0.6 |
| Robustness | 13.30% | 0.7 | 0.7 | 0.7 |
| Estimated Mass | 6.70% | 0.8 | 0.7 | 0.7 |
Table 5.
Final mapped design suitability.
Table 5.
Final mapped design suitability.
| Project Requirement | Mapped Suitability of Design 1 | Mapped Suitability of Design 2 | Mapped Suitability of Design 3 |
|---|
| Effectiveness | 0.23 | 0.27 | 0.17 |
| Cost | 0.16 | 0.16 | 0.16 |
| Complexity | 0.14 | 0.16 | 0.12 |
| Robustness | 0.09 | 0.09 | 0.09 |
| Estimated Mass | 0.05 | 0.05 | 0.05 |
| Suitability | 0.68 | 0.73 | 0.69 |
| Normalised Suitability of Design to Meet Project Needs | 34.10% | 36.50% | 29.40% |
Table 6.
Static testing parameters.
Table 6.
Static testing parameters.
| Parameter | Test Values | Units |
|---|
| Left Wingtip Dihedral | −90, −60, −30, 0, 30, 60, 90 | Degrees |
| Right Wingtip Dihedral | −90, −60, −30, 0, 30, 60, 90 | Degrees |
| Airspeed | 10, 15, 20 | m/s |
| AOA | 0, 5, 10, 15 | Degrees |
Table 7.
Sideslip testing parameters.
Table 7.
Sideslip testing parameters.
| Parameter | Test Values | Units |
|---|
| Left Wingtip | −90, −60, −30, 0, 30, 60, 90 | Degrees |
| Right Wingtip | −90, −60, −30, 0, 30, 60, 90 | Degrees |
| Airspeed | 10 | m/s |
| AOA | 0, 5, 10, 15 | Degrees |
| Sideslip | 5, 10 | Degrees |
Table 8.
Maximum and minimum rolling moment coefficients at different flight conditions.
Table 8.
Maximum and minimum rolling moment coefficients at different flight conditions.
| Airspeed (m/s) | AOA (Degrees) |
|---|
| 0 | 5 | 10 | 15 |
|---|
| 10 | −0.008:0.009 | −0.015:0.014 | −0.030:0.028 | −0.036:0.035 |
| 15 | −0.008:0.008 | −0.014:0.014 | −0.025:0.030 | −0.037:0.041 |
| 20 | −0.006:0.009 | −0.015:0.014 | −0.026:0.032 | −0.032:0.040 |