Design and Cushioning Performance Analysis of Spherical Tensegrity Structures
Abstract
1. Introduction
2. Design and Self-Equilibrium Analysis of Spherical Tensegrity Structures
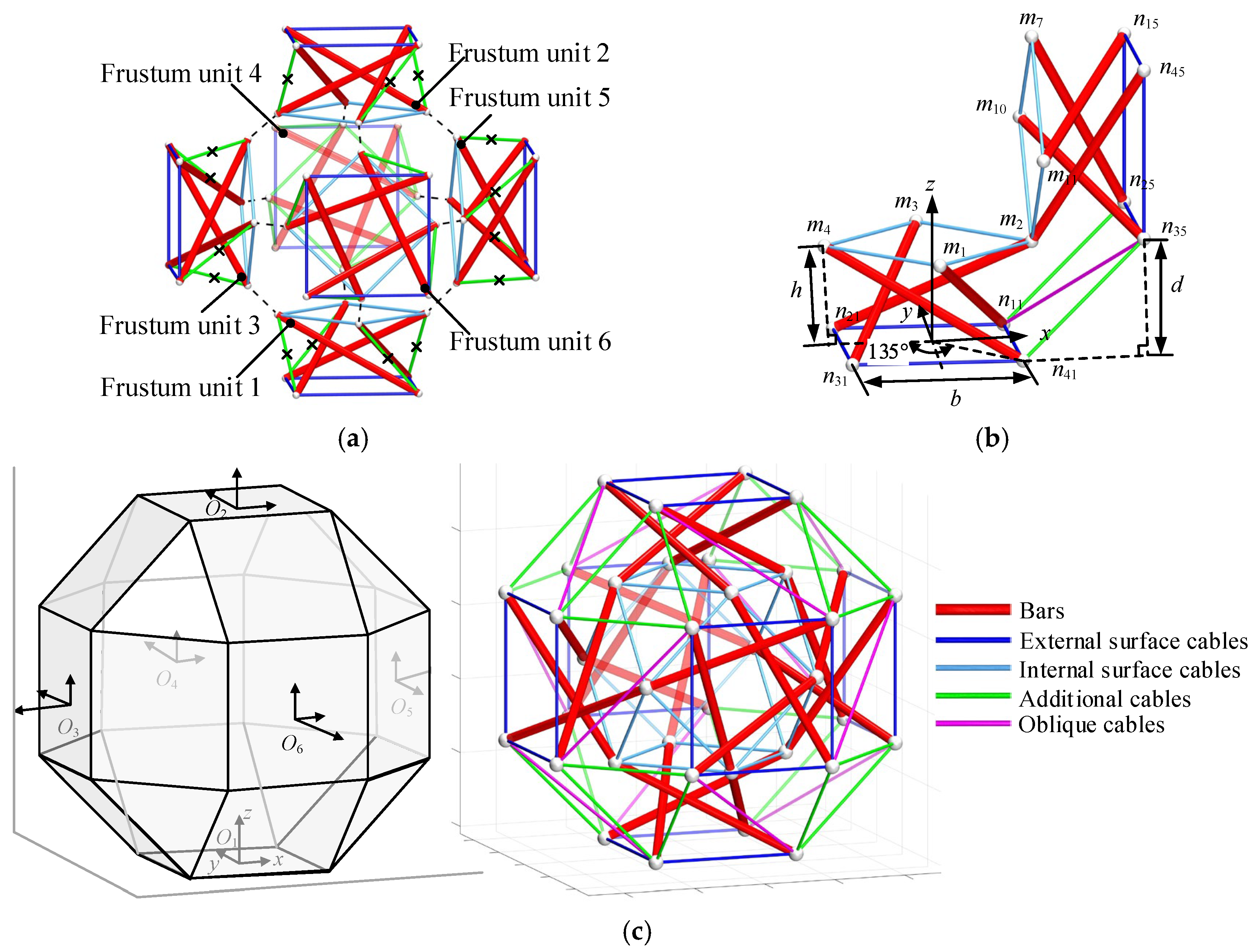
2.1. Design of the Spherical Tensegrity Structure
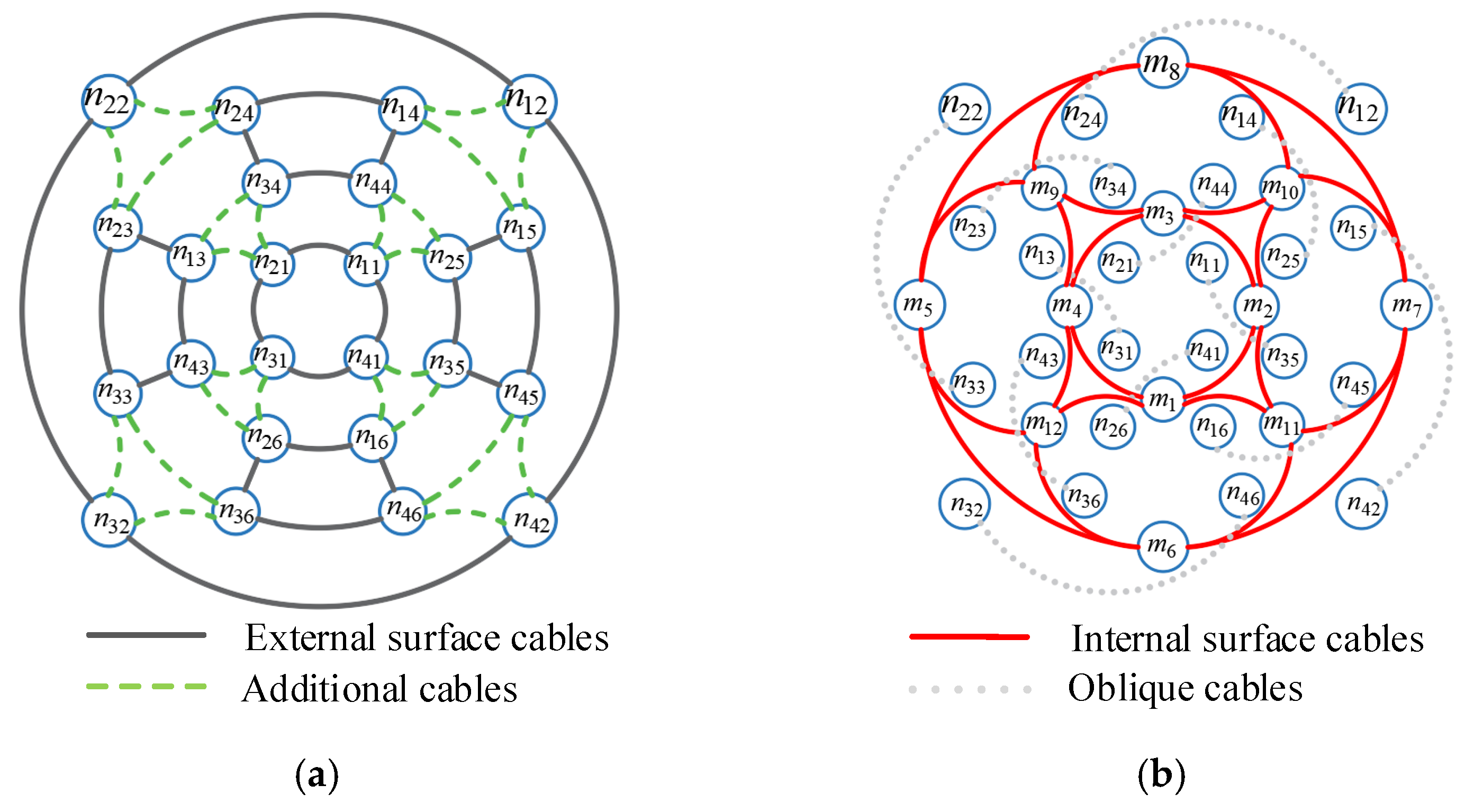
2.2. Establishment of the Geometric Model
2.3. Self-Equilibrium Analyssssis and Parametric Design of Structure
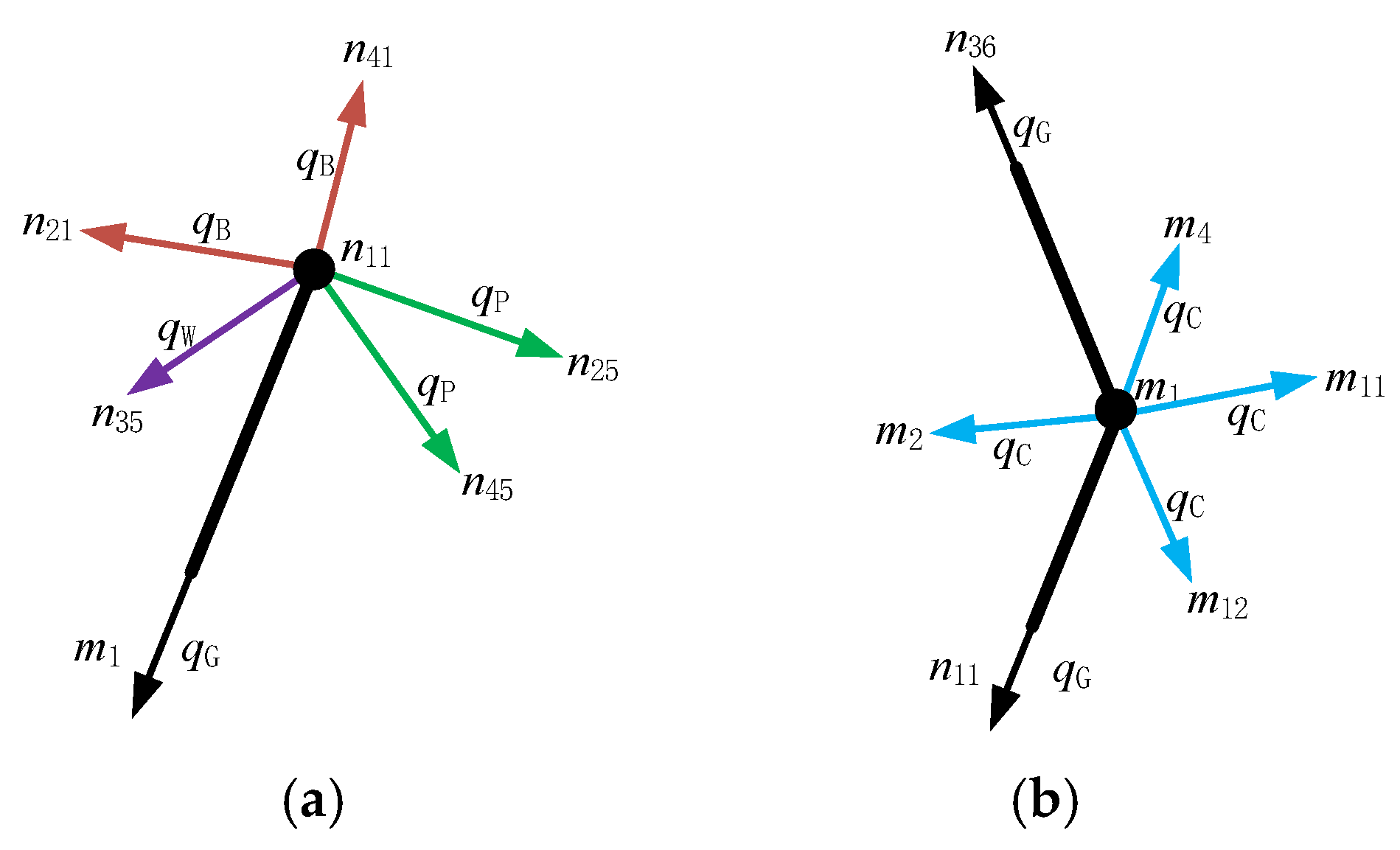
2.3.1. Establishment of Equilibrium Equations
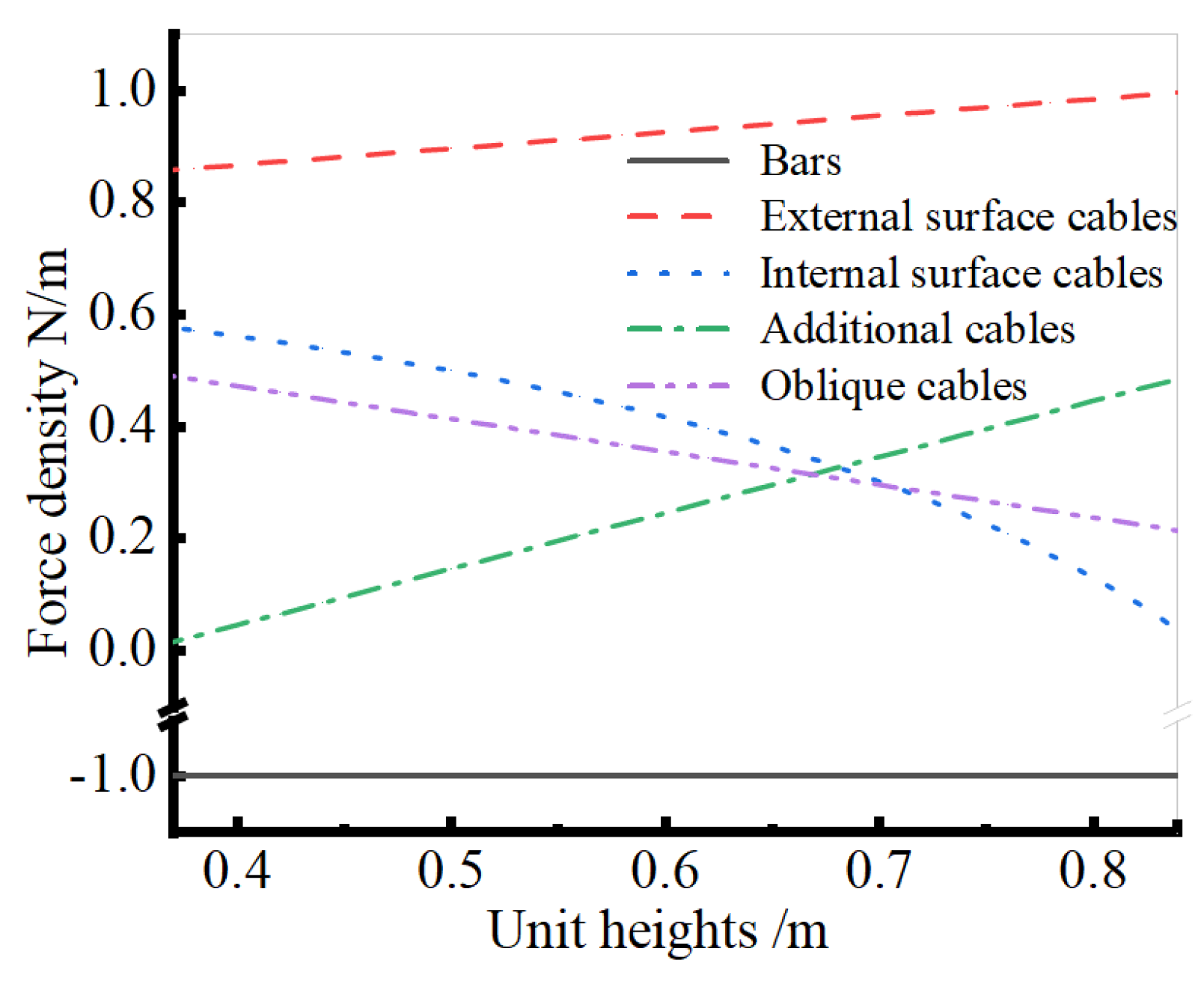
2.3.2. Self-Equilibrium Analysis
2.3.3. Self-Equilibrium Verification
2.4. Stability Determination of the Structure
2.4.1. System Determination Based on Maxwell’s Criterion
2.4.2. Structural Stability Assessment
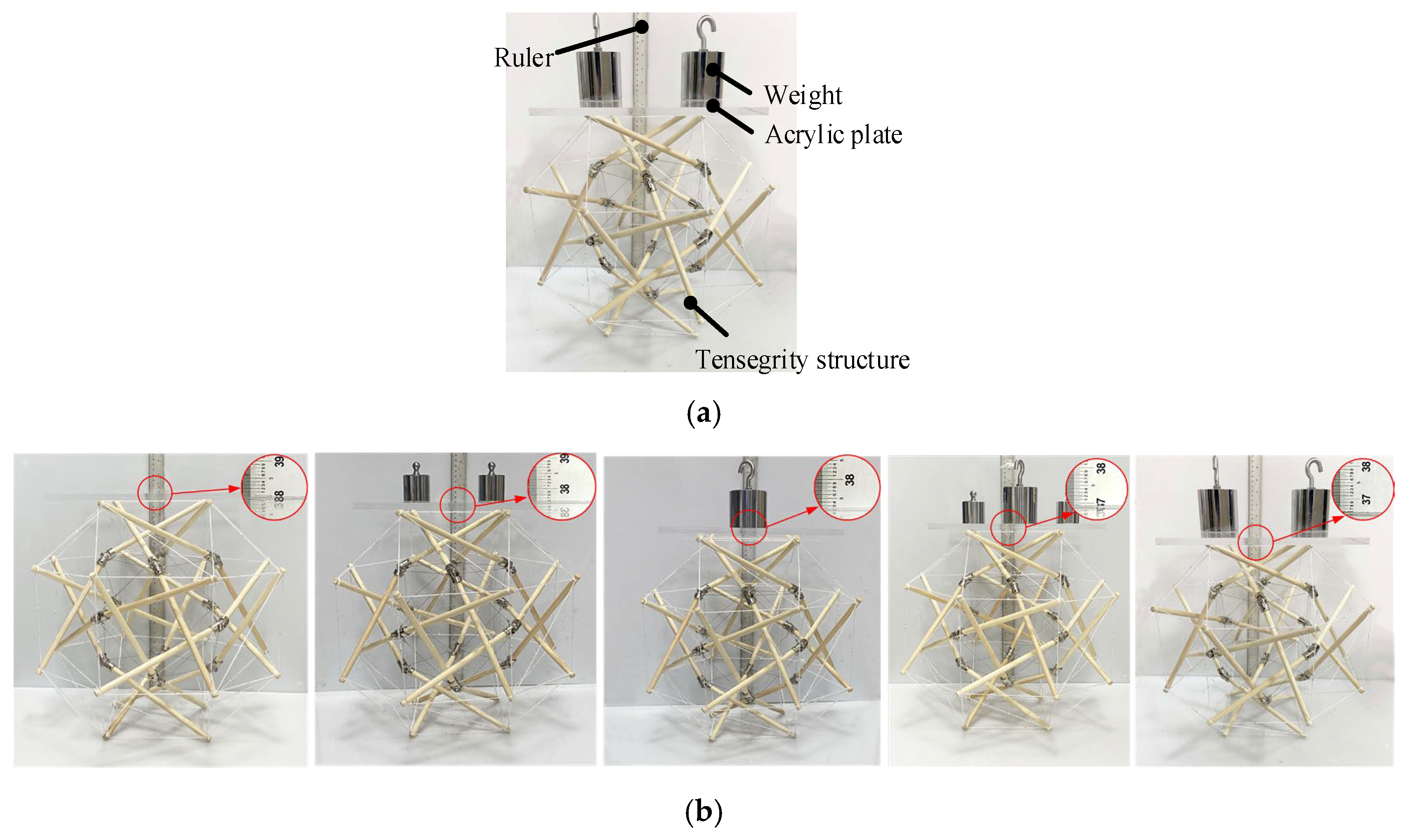
2.5. Construction of Physical Model
3. Static Characteristic Analysis
3.1. Establishment of Finite Element Model
3.2. Finite Element Simulation Analysis
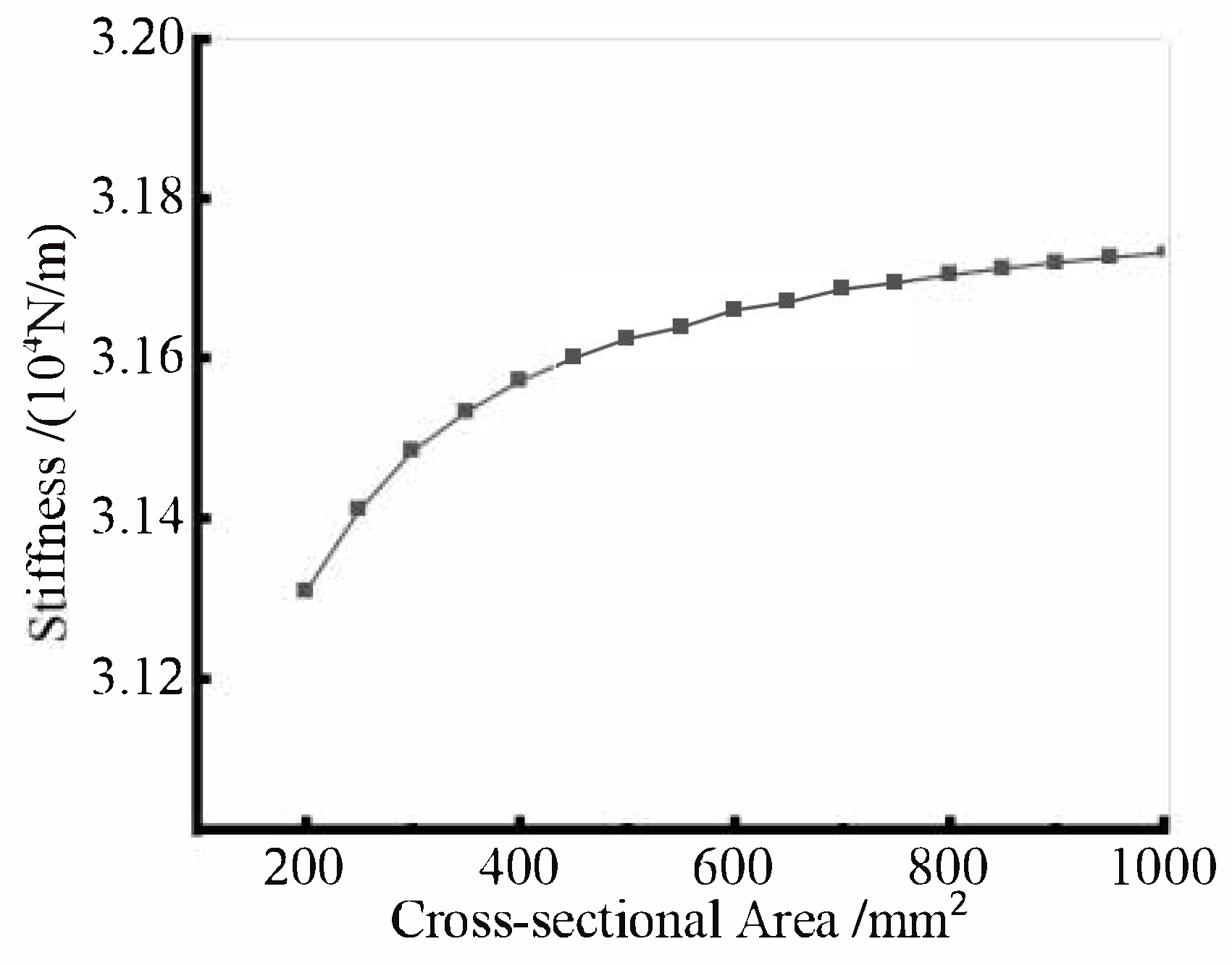
3.2.1. Analysis of the Influence of Cross-Sectional Area of Bar Members on Stiffness
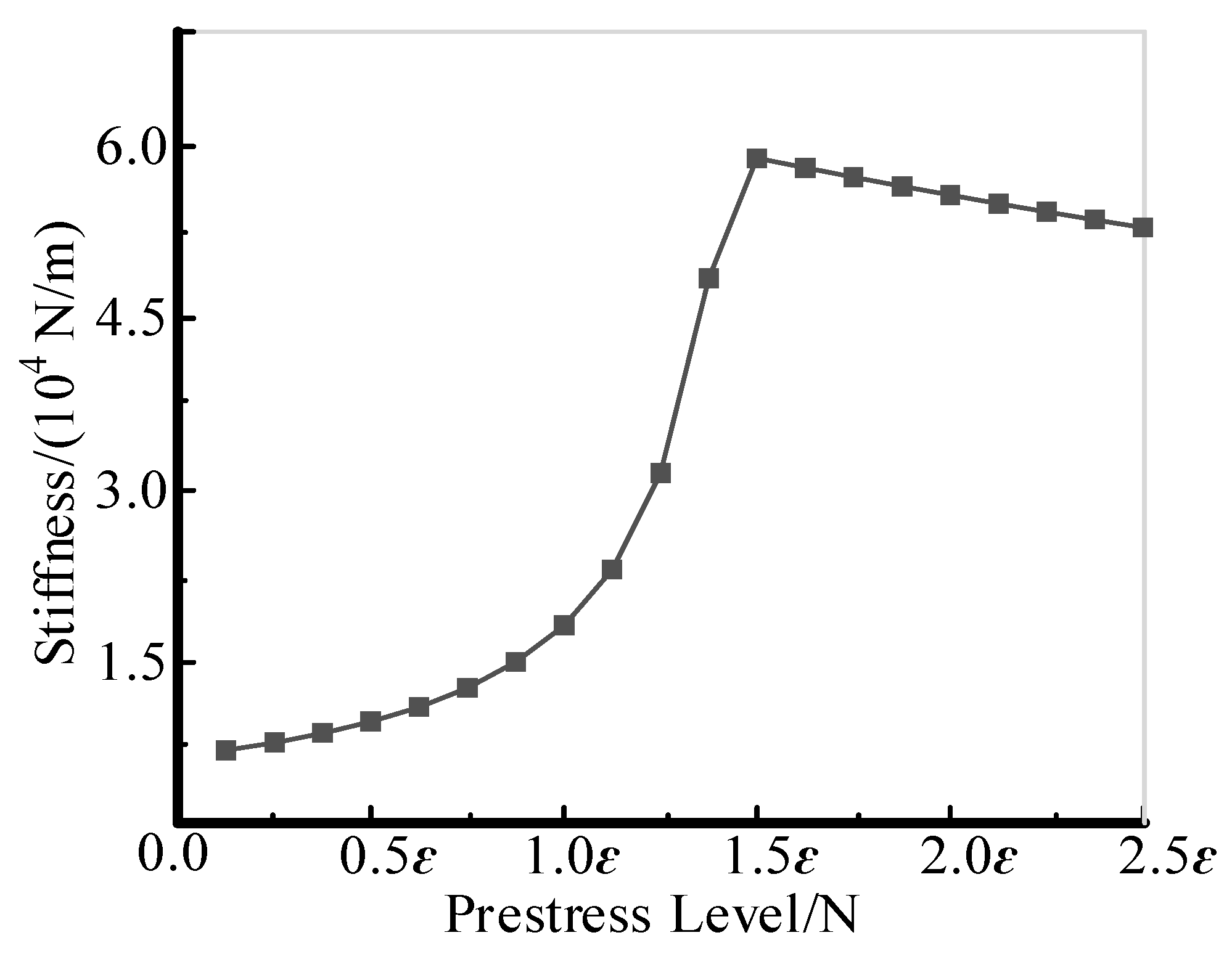
3.2.2. Analysis of the Influence of Prestress on Stiffness
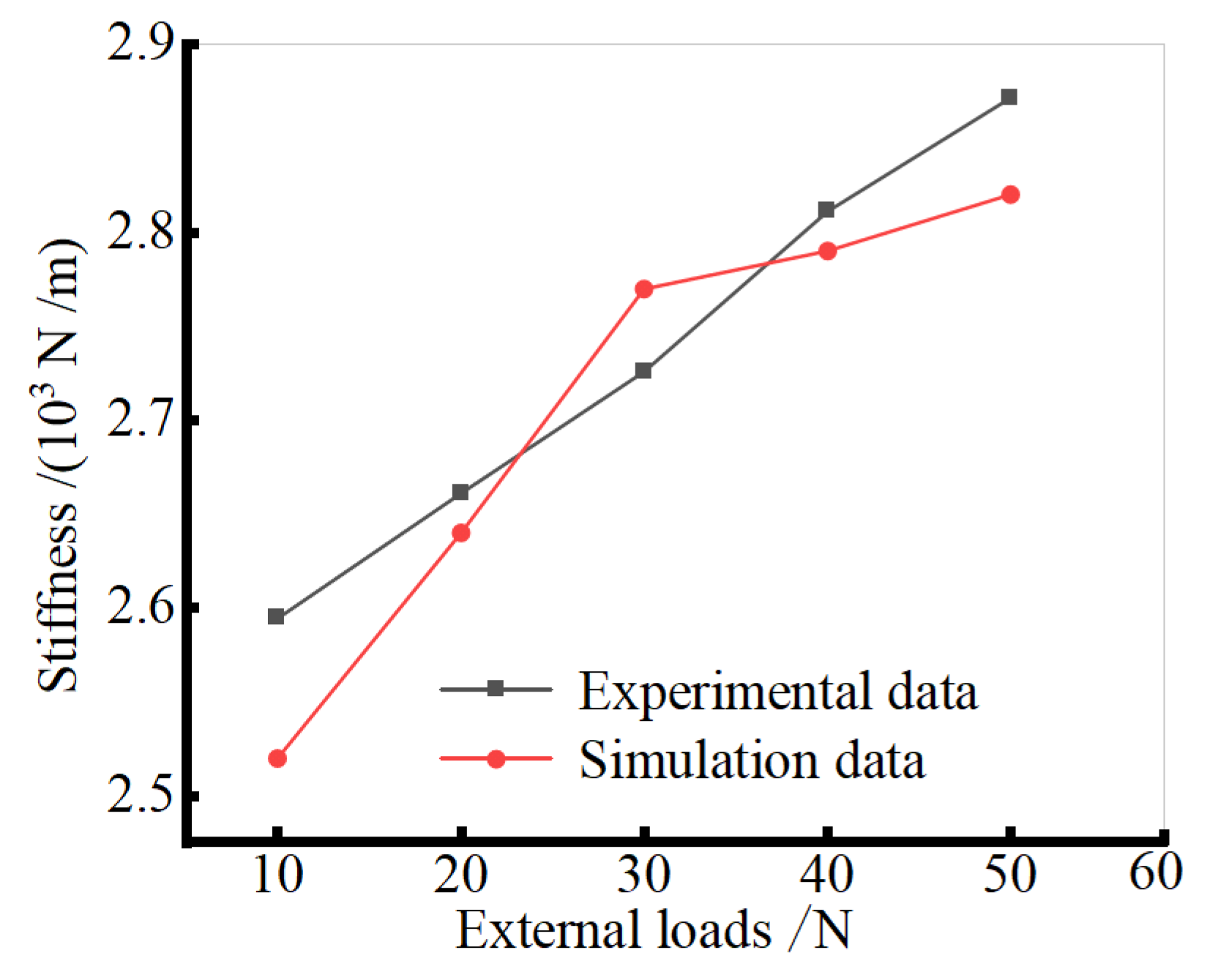
3.3. Stiffness Experiment
4. Analysis of Cushioning Performance Based on Collision Simulation
4.1. Dynamic Collision Simulation
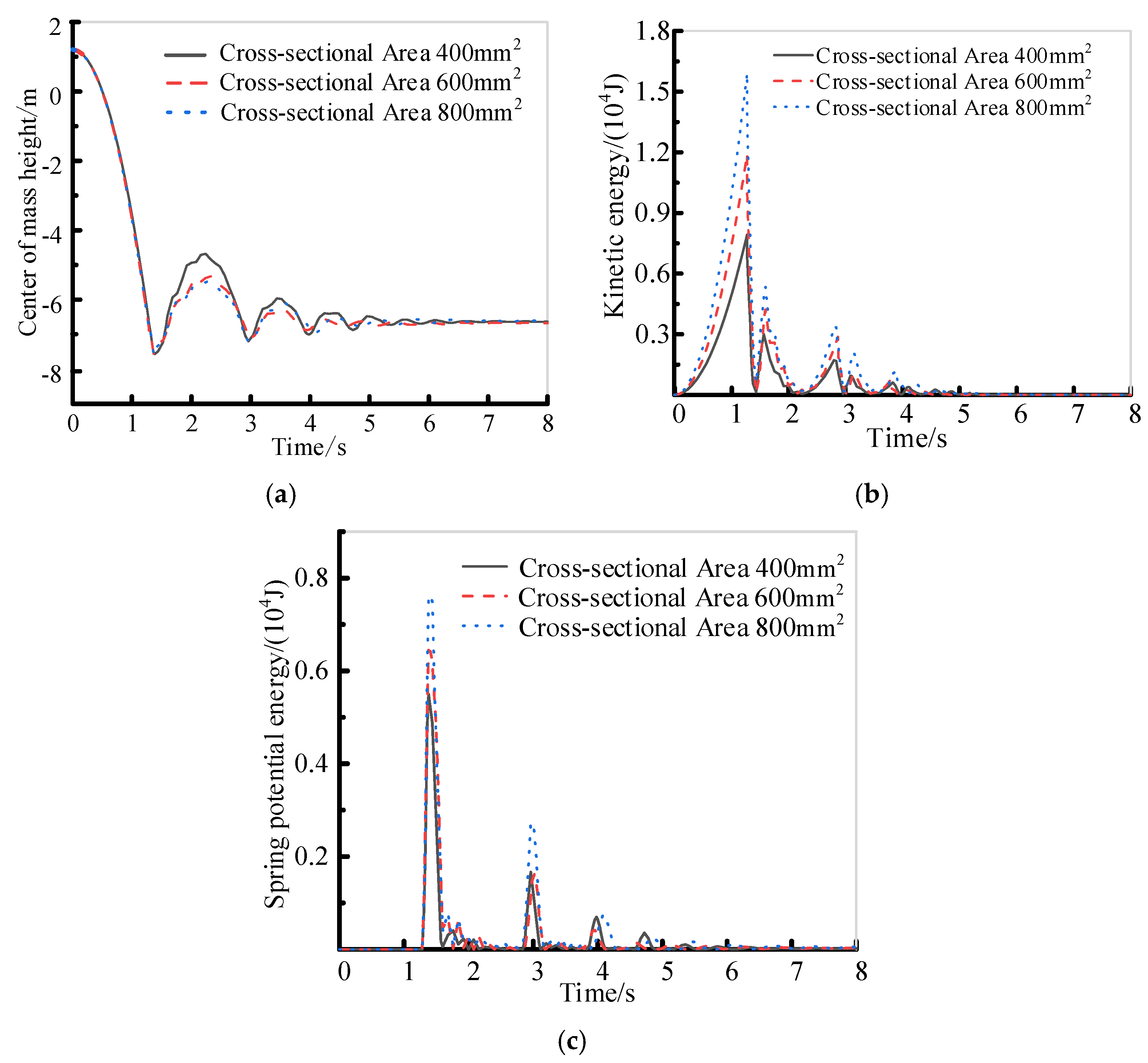
4.2. Analysis of the Impact of Bar Component Cross-Sectional Area on Cushioning Performance
4.3. Analysis of the Impact of Spring Prestress on Cushioning Performance
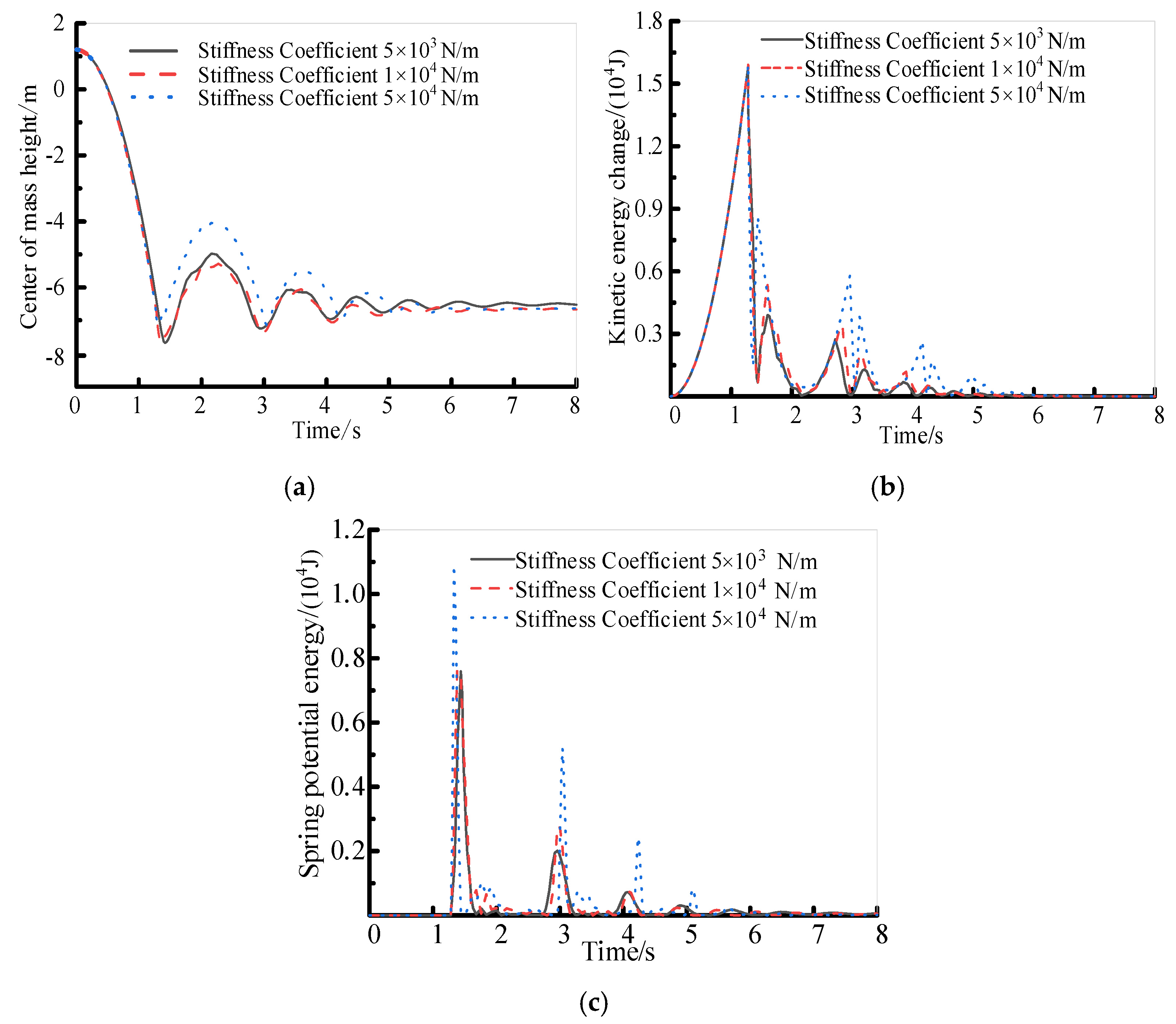
4.4. Analysis of the Impact of Spring Tension and Compression Stiffness Coefficient on Cushioning Performance
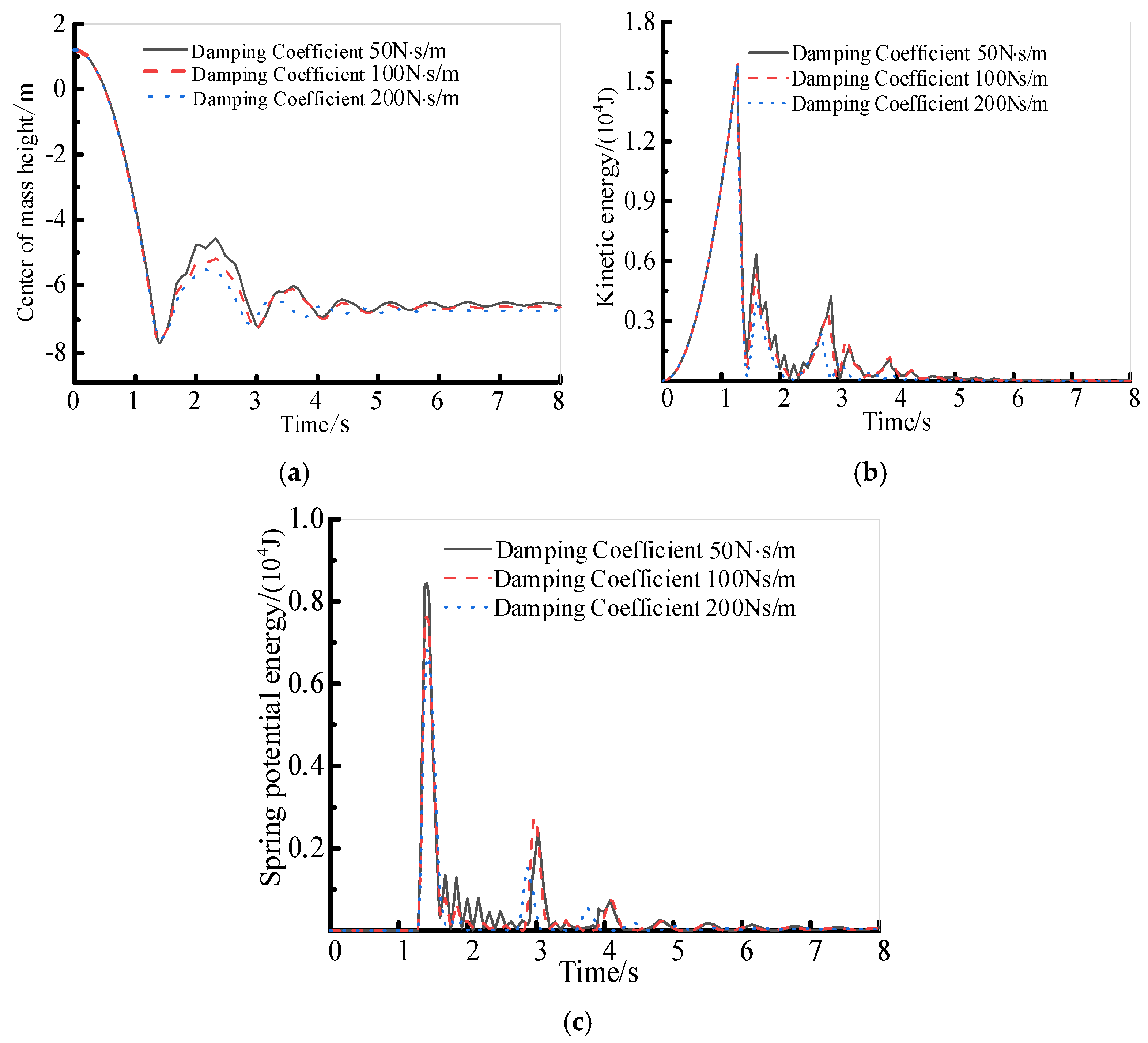
4.5. Analysis of the Impact of Spring Damping Coefficient on Cushioning Performance
4.6. Collision Experiment
5. Conclusions
- (1)
- Based on the concept of circumferential assembling in tensegrity structures, a spherical tensegrity structure was designed by connecting six four−bar truncated pyramid units using type II assembly. Based on the established geometric model of the structure, the internal forces of the components were calculated, which verify the self−balance of the structure. The stability of the structure was confirmed through analysis of the positive definiteness of its tangent stiffness matrix. Finally, the feasibility of the structure was validated through a physical experiment.
- (2)
- The finite element model was established in ANSYS APDL, and key factors affecting structural compressive stiffness were analyzed through finite element simulation. Results indicate that the structure’s compressive stiffness increases with larger bar cross−sectional areas, though the rate of increase diminishes when the area exceeds 600 mm2. Additionally, cable prestress significantly influences compressive stiffness. As prestress level increases, compressive stiffness initially rises, then declines, peaking at a prestress level of 1.5ε.
- (3)
- The collision simulations demonstrated that the structure’s cushioning performance is related to multiple structural parameters. A moderate increase in the bar component’s cross−sectional area can enhance the structure’s cushioning performance. Then the effect will be weakened by the offsetting effect of the increase in structural mass. Increasing the cable component prestress can improve the structure’s cushioning performance, but excessive prestress will lead to the instability of the bars. In addition, selecting an appropriate spring stiffness can improve the structure’s energy absorption efficiency without making it overly rigid, which would reduce its overall cushioning performance. Adjusting the spring damping coefficient in the structure can effectively optimize the energy management and dispersion mechanism after collision, enhancing the safety and reliability of the structure during the collision process.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Lu, J.; Dong, X.; Zhao, X.; Wu, X.; Shu, G. Form−finding analysis for a new type of cable–strut tensile structures generated by semi−regular tensegrity. Adv. Struct. Eng. 2017, 20, 772–783. [Google Scholar] [CrossRef]
- Wang, X.; Ling, Z.; Qiu, C.; Song, Z.; Kang, R. A four−prism tensegrity robot using a rolling gait for locomotion. Mech. Mach. Theory 2022, 172, 104828. [Google Scholar] [CrossRef]
- Gómez-Jauregui, V.; Carrillo-Rodríguez, Á.; Manchado, C.; Lastra-González, P. Tensegrity Applications to Architecture, Engineering and Robotics: A Review. Appl. Sci. 2023, 13, 8669. [Google Scholar] [CrossRef]
- Da Silva, A.R.; de Souza, L.C.G.; Schäfer, B. Joint dynamics modeling and parameter identification for space robot applications. Math. Probl. Eng. 2007, 2007, 012361. [Google Scholar] [CrossRef]
- Agogino, A.K.; SunSpiral, V.; Atkinson, D. Super Ball Bot−Structures for Planetary Landing and Exploration; Technical Report; NASA: Washington, DC, USA, 2018.
- Zhang, P.; Zhou, J.; Chen, J. Form−finding of complex tensegrity structures using constrained optimization method. Compos. Struct. 2021, 268, 113971. [Google Scholar] [CrossRef]
- Liu, Y.; Bi, Q.; Yue, X.; Wu, J.; Yang, B.; Li, Y. A review on tensegrity structures−based robots. Mech. Mach. Theory 2022, 168, 104571. [Google Scholar] [CrossRef]
- Friesen, J.M.; Glick, P.; Fanton, M.; Manovi, P.; Xydes, A.; Bewley, T.; Sunspiral, V. The second generation prototype of a Duct Climbing Tensegrity robot, DuCTTv2. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation (ICRA), Stockholm, Sweden, 16–21 May 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 2123–2128. [Google Scholar]
- Booth, J.W.; Cyr-Choiniere, O.; Case, J.C.; Shah, D.; Yuen, M.C.; Kramer-Bottiglio, R. Surface actuation and sensing of a tensegrity structure using robotic skins. Soft Robot. 2021, 8, 531–541. [Google Scholar] [CrossRef] [PubMed]
- He, Z.; Han, L.; Zhang, X.; Zhang, L. Study on Folding and Rolling Performance of a Six−strut Spherical Tensegrity. Aerosp. Shanghai 2022, 39, 59–65. [Google Scholar]
- Ma, S.; Chen, M.; Skelton, R.E. Tensegrity system dynamics based on finite element method. Compos. Struct. 2022, 280, 114838. [Google Scholar] [CrossRef]
- Goyal, R.; Peraza Hernandez, E.A.; Majji, M.; Skelton, R.E. Design of Tensegrity Structures with Static and Dynamic Modal Requirements. In Earth and Space 2021; American Society of Civil Engineers: Reston, VA, USA, 2021. [Google Scholar]
- Chen, M.; Goyal, R.; Majji, M.; Skelton, R.E. Deployable Tensegrity Lunar Tower. In Earth and Space; American Society of Civil Engineers: Reston, VA, USA, 2021; pp. 1079–1092. [Google Scholar]
- Saha, D.; Goyal, R.; Skelton, R.E. Tensegrity Lander Architectures for Planetary Explorations. In Earth and Space; American Society of Civil Engineers: Reston, VA, USA, 2021; pp. 1251–1262. [Google Scholar]
- Shibata, M.; Saijyo, F.; Hirai, S. Crawling by body deformation of tensegrity structure robots. In Proceedings of the 2009 IEEE International Conference on Robotics and Automation, Kobe, Japan, 12–17 May 2009; IEEE: Piscataway, NJ, USA, 2009; pp. 4375–4380. [Google Scholar]
- Koizumi, Y.; Shibata, M.; Hirai, S. Rolling tensegrity driven by pneumatic soft actuators. In Proceedings of the 2012 IEEE International Conference on Robotics and Automation, Saint Paul, MN, USA, 14–18 May 2012; IEEE: Piscataway, NJ, USA, 2012; pp. 1988–1993. [Google Scholar]
- Iscen, A.; Agogino, A.; SunSpiral, V.; Tumer, K. Learning to control complex tensegrity robots. In Proceedings of the 2013 International Conference on Autonomous Agents and Multi−Agent Systems, Saint Paul, MN, USA, 6–10 May 2013; pp. 1193–1194. [Google Scholar]
- Hirai, S.; Imuta, R. Dynamic simulation of six−strut tensegrity robot rolling. In Proceedings of the 2012 IEEE International Conference on Robotics and Biomimetics (ROBIO), Guangzhou, China, 11–14 December 2012; IEEE: Piscataway, NJ, USA, 2012; pp. 198–204. [Google Scholar]
- Sabelhaus, A.P.; Bruce, J.; Caluwaerts, K.; Manovi, P.; Firoozi, R.F.; Dobi, S.; Agogino, A.M.; SunSpiral, V. System design and locomotion of SUPERball, an untethered tensegrity robot. In Proceedings of the 2015 IEEE International Conference on Robotics and Automation (ICRA), Seattle, WA, USA, 26–30 May 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 2867–2873. [Google Scholar]
- Burms, J.; Caluwaerts, K.; Dambre, J. Online unsupervised terrain classification for a compliant tensegrity robot using a mixture of echo state networks. In Proceedings of the 2015 IEEE International Conference on Robotics and Automation (ICRA), Seattle, WA, USA, 26–30 May 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 4252–4257. [Google Scholar]
- Vespignani, M.; Friesen, J.M.; SunSpiral, V.; Bruce, J. Design of SUPERball v2, a compliant tensegrity robot for absorbing large impacts. In Proceedings of the 2018 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Madrid, Spain, 1–5 October 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 2865–2871. [Google Scholar]
- Bohm, V.; Kaufhold, T.; Schale, F.; Zimmermann, K. Spherical Mobile Robot Based on a Tensegrity Structure with Curved Compressed Members. In Proceedings of the 2016 IEEE International Conference on Advanced Intelligent Mechatronics (AIM), Banff, AB, Canada, 12–15 July 2016; pp. 1509–1514. [Google Scholar]
- Bohm, V.; Kaufhold, T.; Zeidis, I.; Zimmermann, K. Dynamic analysis of a spherical mobile robot based on a tensegrity structure with two curved compressed members. Arch. Appl. Mech. 2017, 87, 853–864. [Google Scholar] [CrossRef]
- Kim, K.; Moon, D.; Bin, J.Y.; Agogino, A.M. Design of a spherical tensegrity robot for dynamic locomotion. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 450–455. [Google Scholar]
- Zhang, J.; Ohsaki, M. Self−equilibrium and stability of regular truncated tetrahedral tensegrity structures. J. Mech. Phys. Solids 2012, 60, 1757–1770. [Google Scholar] [CrossRef]
- Zhang, J.; Ohsaki, M.; Tsuura, F. Self−equilibrium and super−stability of truncated hexahedral and octahedral tensegrity structures. Int. J. Solids Struct. 2019, 161, 182–192. [Google Scholar] [CrossRef]
- Kim, K.; Chen, L.H.; Cera, B.; Daly, M.; Zhu, E.; Despois, J.; Agogino, A.K.; SunSpiral, V.; Agogino, A.M. Hopping and rolling locomotion with spherical tensegrity robots. In Proceedings of the 2016 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Daejeon, Republic of Korea, 9–14 October 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 4369–4376. [Google Scholar]
- Chen, L.H.; Kim, K.; Tang, E.; Li, K.; House, R.; Zhu, E.L.; Fountain, K.; Agogino, A.M.; Agogino, A.; Sunspiral, V.; et al. Soft spherical tensegrity robot design using rod−centered actuation and control. J. Mech. Robot. 2017, 9, 025001. [Google Scholar] [CrossRef]
- Schroeder, K.K. A Comprehensive Entry, Descent, Landing, and Locomotion (EDLL) Vehicle for Planetary Exploration. Ph.D. Thesis, Virginia Tech, Blacksburg, VA, USA, 2017. [Google Scholar]
- Gebara, C.A.; Carpenter, K.C.; Woodmansee, A. Tensegrity Ocean world landers. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019; p. 0868. [Google Scholar]
- Zhao, L.; Peraza Hernandez, E.A. Tensegrity structures for impact energy absorption and dissipation in planetary landers. In Earth and Space 2021; American Society of Civil Engineers: Reston, VA, USA, 2021; pp. 908–919. [Google Scholar]
- Wu, L.; de Andrade, M.J.; Brahme, T.; Tadesse, Y.; Baughman, R.H. A reconfigurable robot with tensegrity structure using nylon artificial muscle. Act. Passiv. Smart Struct. Integr. Syst. 2016, 2016, 9799. [Google Scholar]
- Zhang, J.Y.; Ohsaki, M. Tensegrity Structures: Form, Stability, and Symmetry; Springer: Tokyo, Japan, 2015. [Google Scholar]
- Mark, W. Spong. In Robot Dynamics and Control; China Machine Press: Beijing, China, 2016; pp. 27–31. [Google Scholar]
- Chen, W.F. Steel Beam−to−Column Building Connections; Elsevier Science Publishing Co., Inc.: New York, NY, USA, 1988. [Google Scholar]
- Luo, A.; Wang, L.; Liu, H.; Wang, Y.; Li, Q.; Cao, P. Analysis of configuration and structural stability of 3−bar tensegrity prism. Harbin Gongye Daxue Xuebao J. Harbin Inst. Technol. 2016, 48, 82. [Google Scholar]
- Pellegrino, S. Structural computations with the singular value decomposition of the equilibrium matrix. Int. J. Solids Structures. 1993, 30, 3025–3035. [Google Scholar] [CrossRef]
- Zhang, P.; Feng, J. Stability criterion and stiffness analysis of tensegrity structures. China Civ. Eng. J. 2013, 46, 48–57. [Google Scholar]
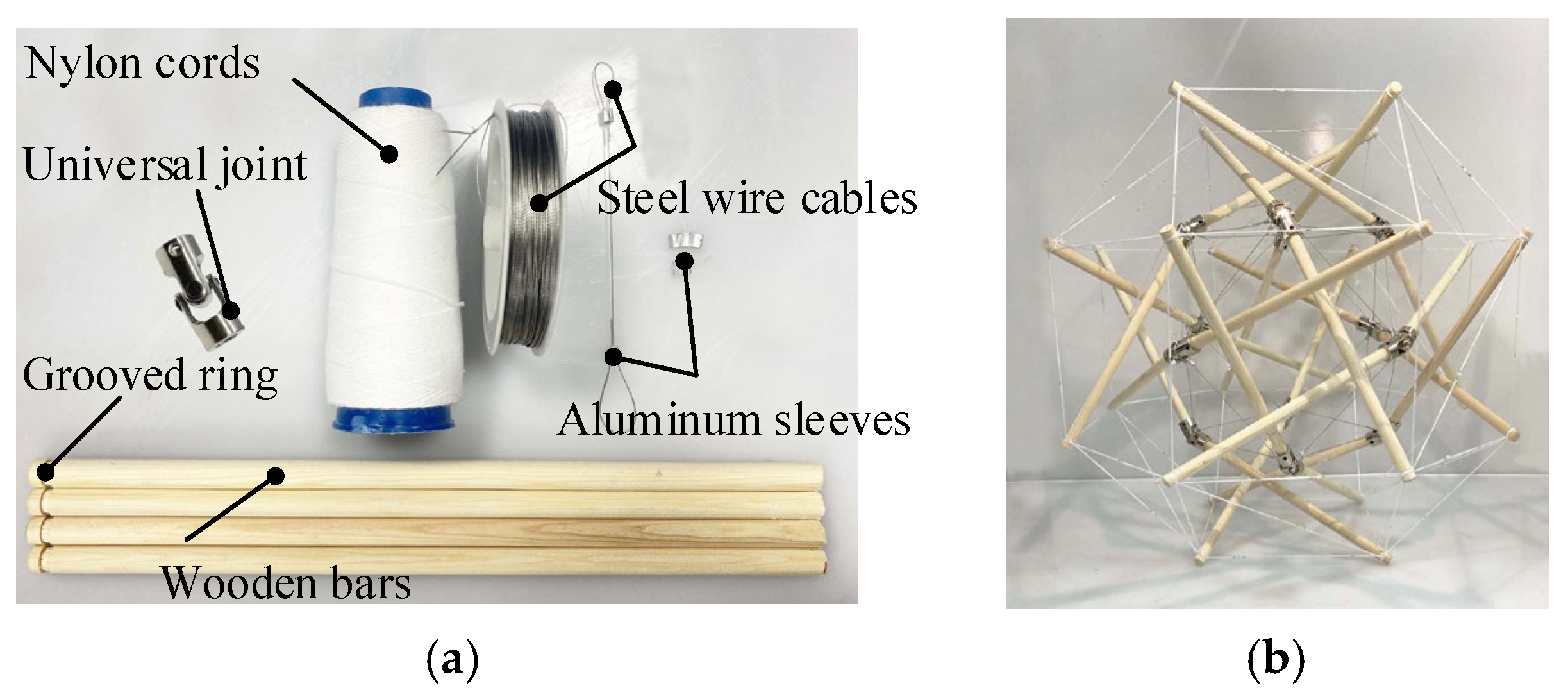
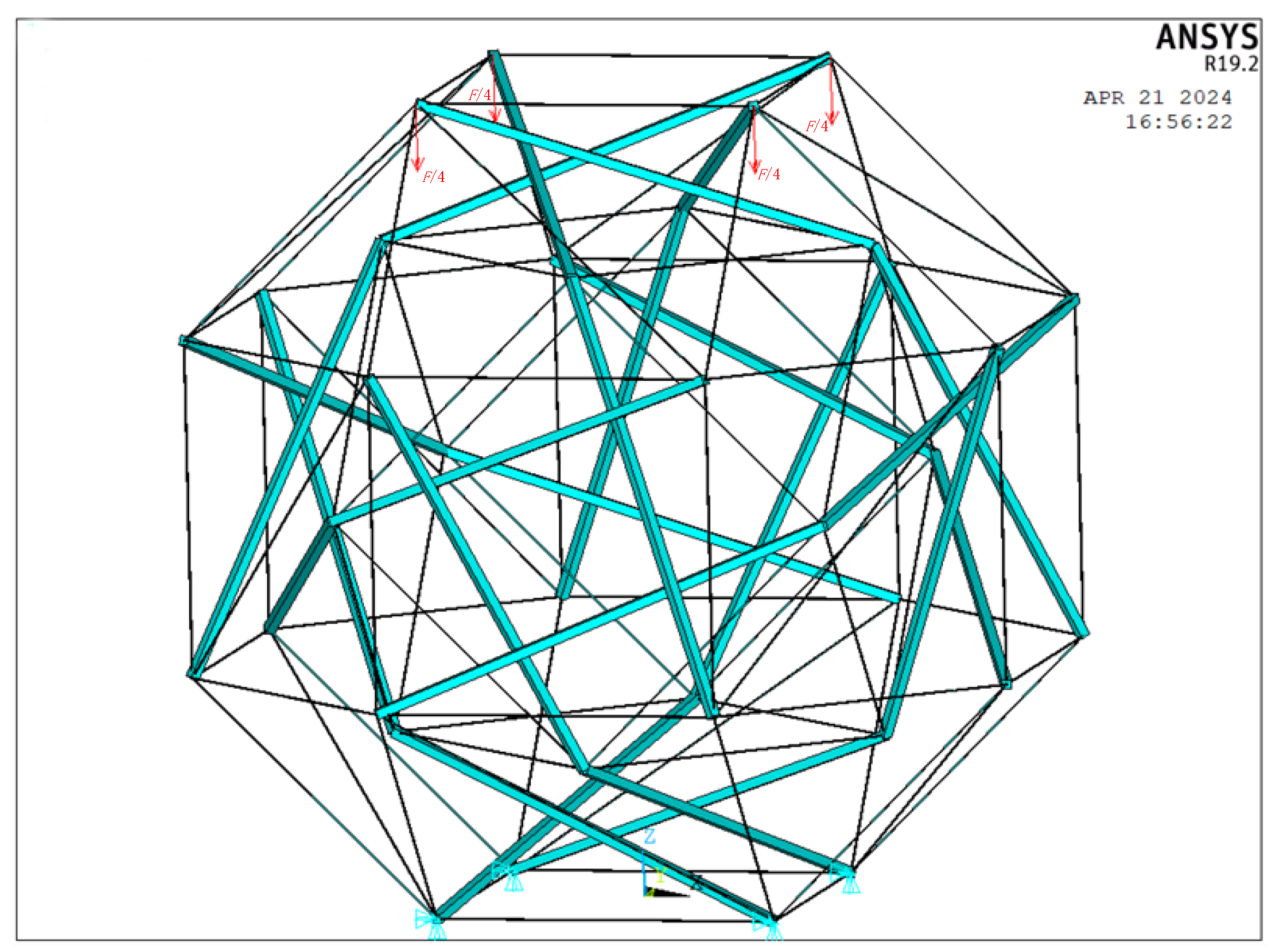



| Components | Number of Components |
|---|---|
| bars | 24 |
| external surface cables | 24 |
| internal surface cables | 24 |
| additional cables | 24 |
| oblique cables | 12 |
| external surface nodes | 24 |
| internal surface nodes | 12 |
| Component Class | Bars | Cables |
|---|---|---|
| Element type | Link180 | Link180 |
| Element attribute | Compression-only | Tension-only |
| Cross-sectional area/mm2 | 706.86 | 40.72 |
| Elasticity modulus/Gpa | 206 | 185 |
| Poisson’s ratio | 0.3 | 0.3 |
| Density/kg·m−3 | 7850 | 7850 |
| Thermal expansivity/K−1 | 10−5 | 10−5 |
| Simulation Parameters | Parameter Values |
|---|---|
| Gravity/N·kg−1 | 9.8 |
| Landing height/m | 8 |
| Elastic modulus of the bar/Gpa | 206 |
| Density of the bar/kg·m−3 | 7850 |
| Cross-sectional area of the bar/mm2 | 706.86 |
| Poisson’s ratio of the bar | 0.3 |
| Elasticity modulus of the cable/Gpa | 185 |
| Density of the cable/kg·m−3 | 7850 |
| Cross-sectional area of the cable/mm2 | 40.72 |
| Poisson’s ratio of the cable | 0.3 |
| Stiffness of the contact surface/N·m−1 | 104 |
| Damping coefficient of the contact surface/N·s·m−1 | 10 |
| Invasion depth/mm | 0.1 |
| HL/m | Experimental | Simulation | The Error of HMR | ||
|---|---|---|---|---|---|
| Whether Failed | HMR/m | FMS/N | HMR/m | ||
| 2 | No | 0.07 | 13.31 | 0.10 | 30.00% |
| 3 | No | 0.17 | 17.07 | 0.25 | 32.00% |
| 4 | No | 0.21 | 23.57 | 0.34 | 38.24% |
| 5 | No | 0.40 | 36.05 | 0.49 | 18.37% |
| 6 | Yes | —— | 50.24 | —— | —— |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Shi, C.; Geng, K.; Chen, Y.; Guo, H.; Liu, R.; Kou, Z. Design and Cushioning Performance Analysis of Spherical Tensegrity Structures. Aerospace 2025, 12, 453. https://doi.org/10.3390/aerospace12060453
Zhang J, Shi C, Geng K, Chen Y, Guo H, Liu R, Kou Z. Design and Cushioning Performance Analysis of Spherical Tensegrity Structures. Aerospace. 2025; 12(6):453. https://doi.org/10.3390/aerospace12060453
Chicago/Turabian StyleZhang, Jing, Chuang Shi, Kun Geng, Yanzheng Chen, Hongwei Guo, Rongqiang Liu, and Ziming Kou. 2025. "Design and Cushioning Performance Analysis of Spherical Tensegrity Structures" Aerospace 12, no. 6: 453. https://doi.org/10.3390/aerospace12060453
APA StyleZhang, J., Shi, C., Geng, K., Chen, Y., Guo, H., Liu, R., & Kou, Z. (2025). Design and Cushioning Performance Analysis of Spherical Tensegrity Structures. Aerospace, 12(6), 453. https://doi.org/10.3390/aerospace12060453






