Structural and Topological Optimization of a Novel Elephant Trunk Mechanism for Morphing Wing Applications
Abstract
1. Introduction
2. Materials and Methods
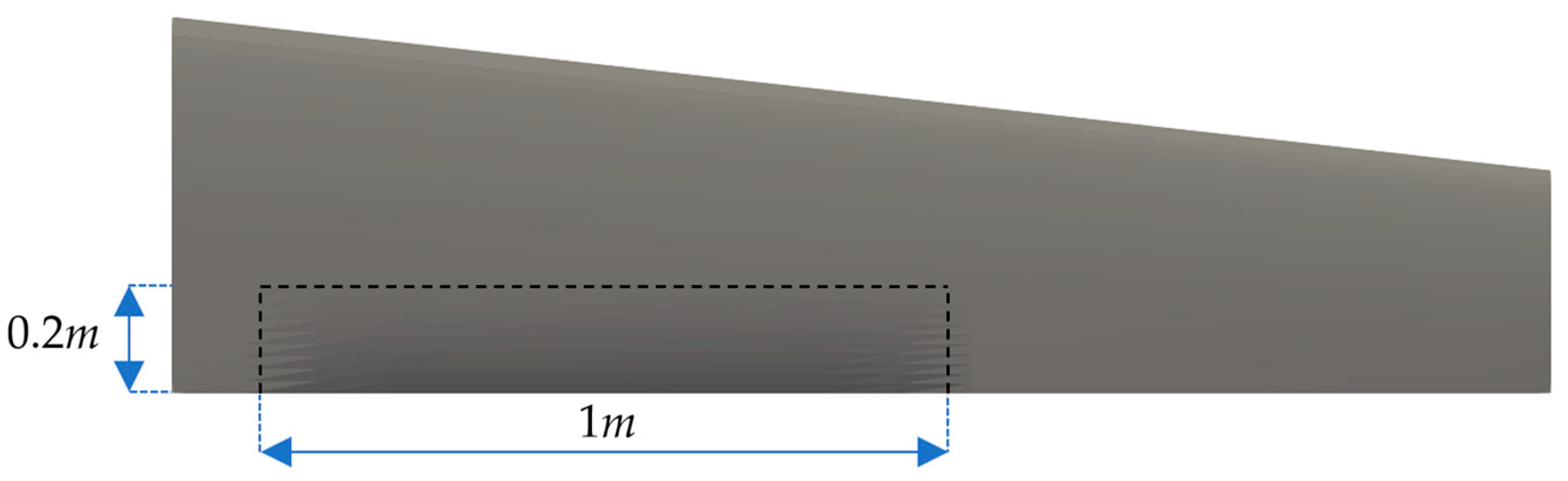
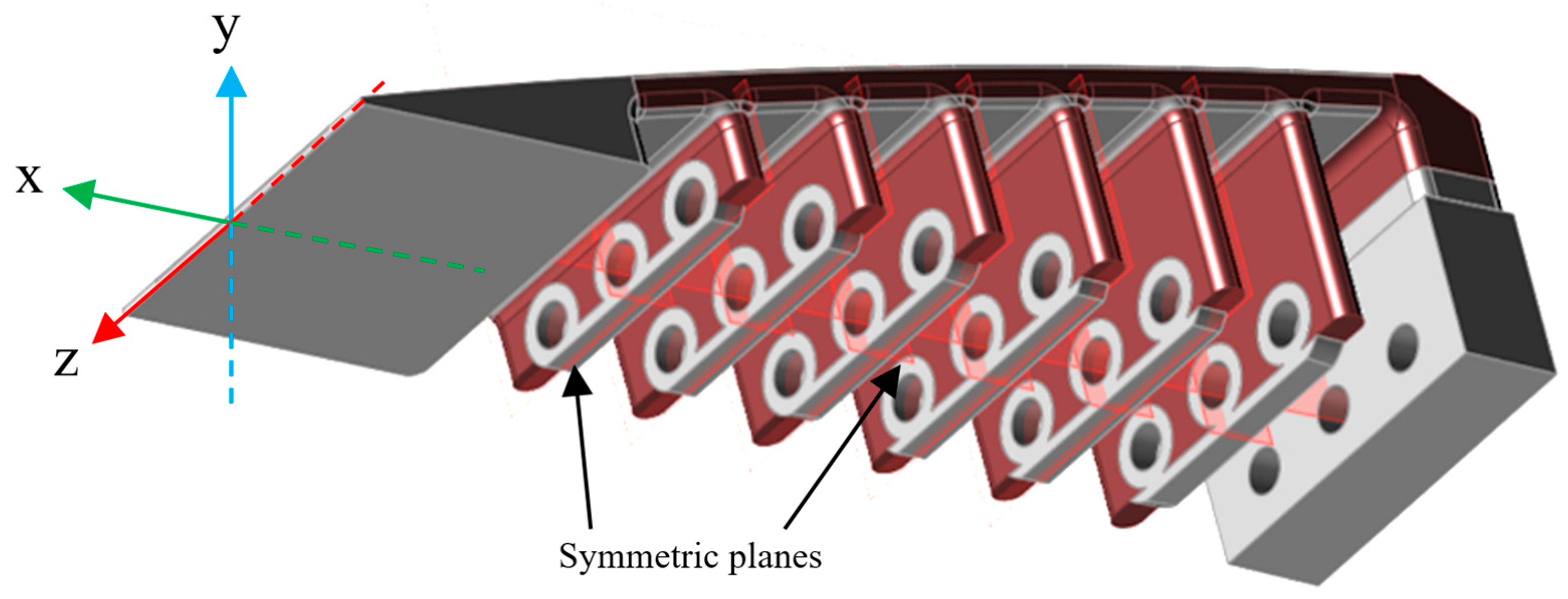
2.1. Elephant Trunk Mechanism (ETM)
2.2. Aerodynamic Characteristics of the SMTE Flap
2.3. Structural Analysis
2.4. Material Selection
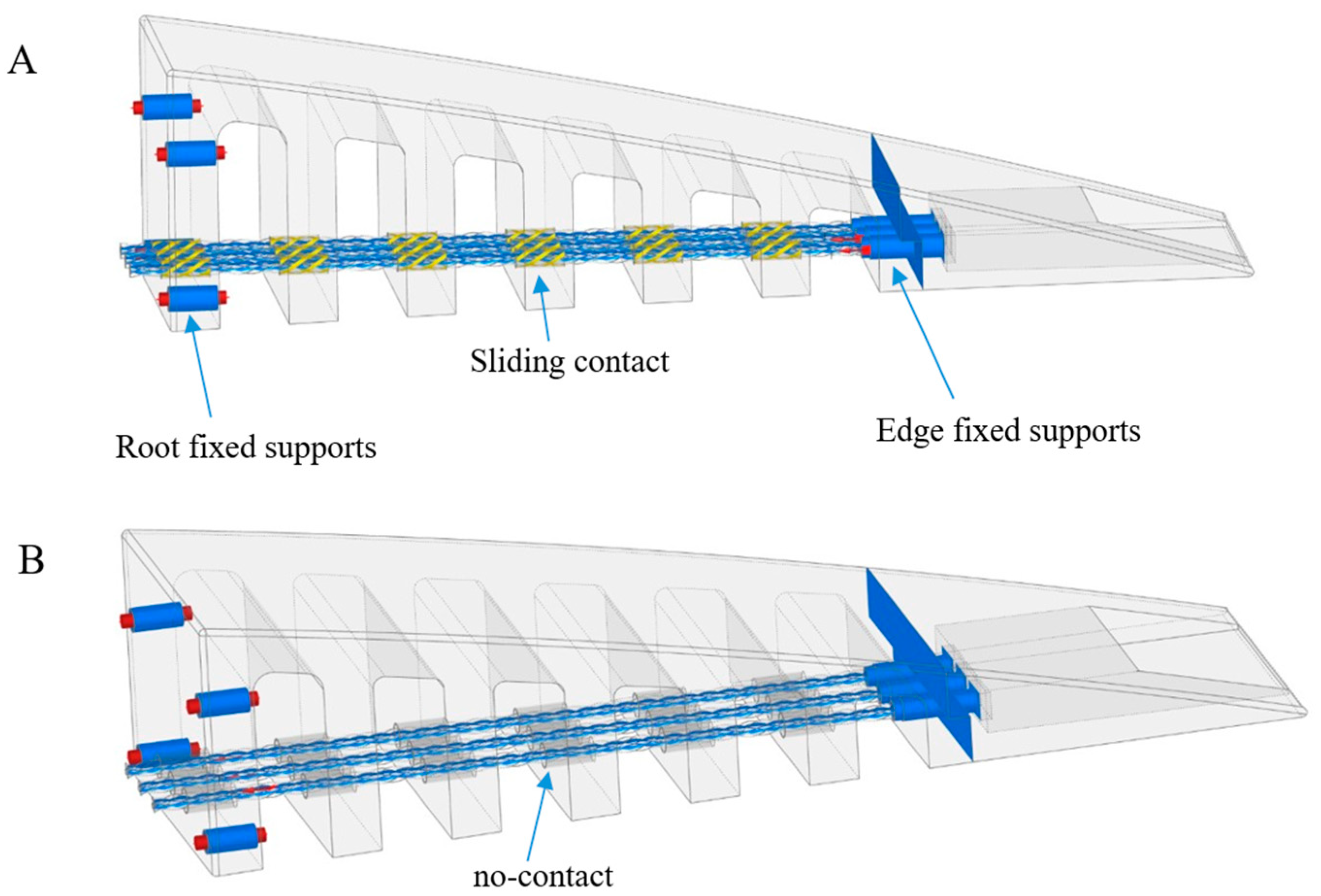
2.5. Boundary Conditions
2.5.1. Sliding Cable/Hole Configuration
2.5.2. No-Contact Cable/Hole Configuration
3. Results and Discussion
3.1. Geometrical Modifications
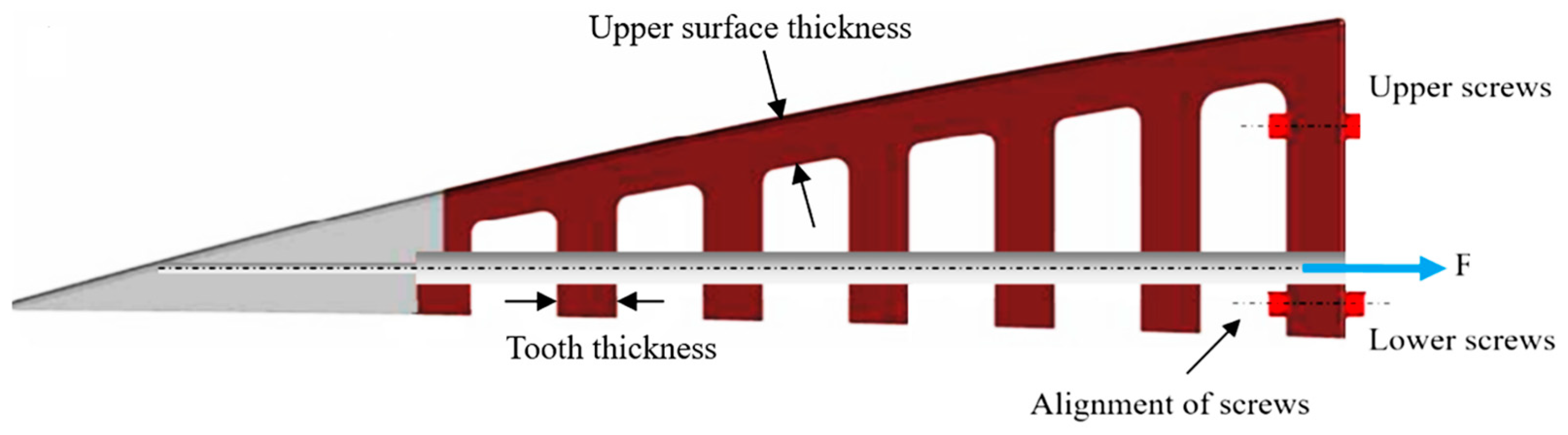
3.2. Analysis of the Teeth Number and Their Thickness
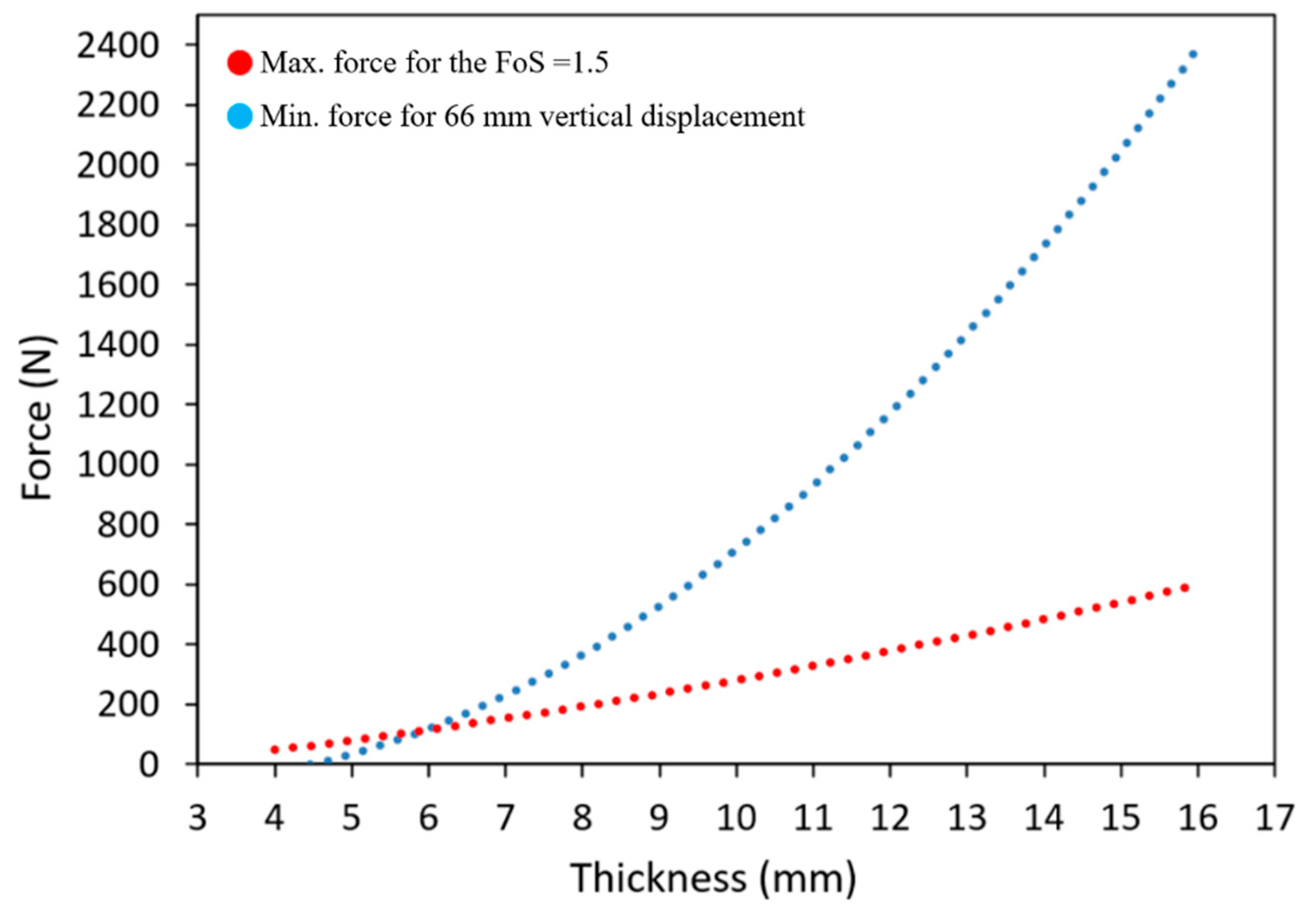
3.3. Analysis of the Upper Surface Thickness
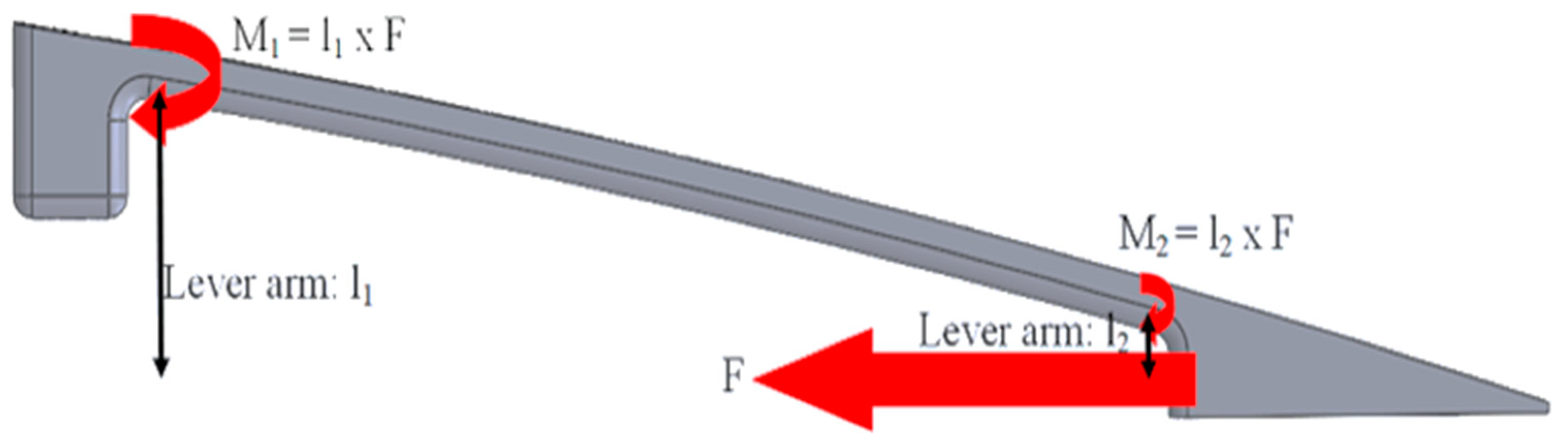
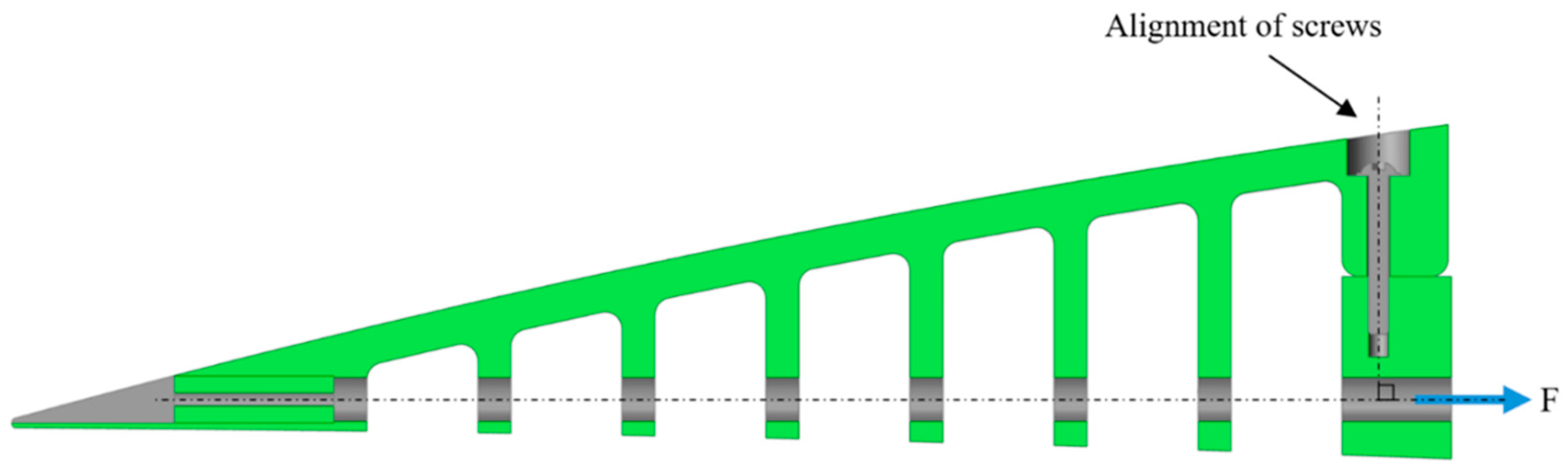
3.4. Modification of the ETM Fixation to the Wing Box
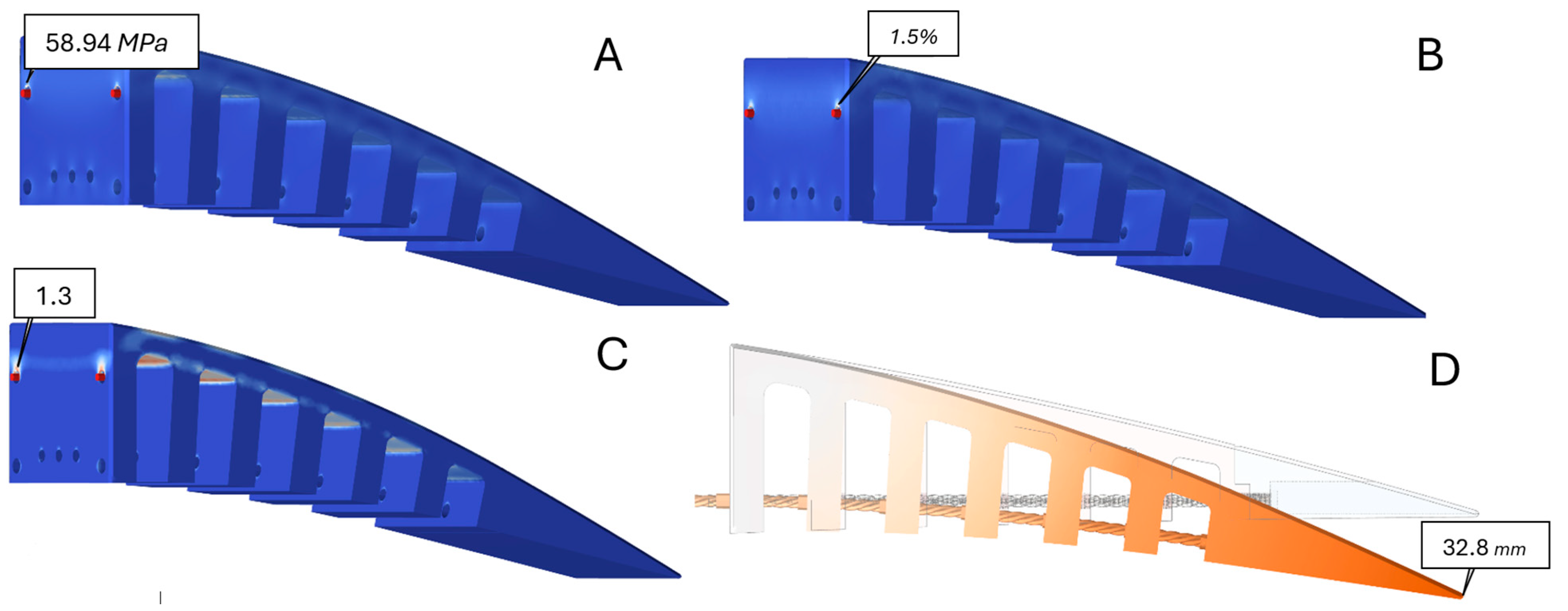
3.5. Results of the Structural Analysis
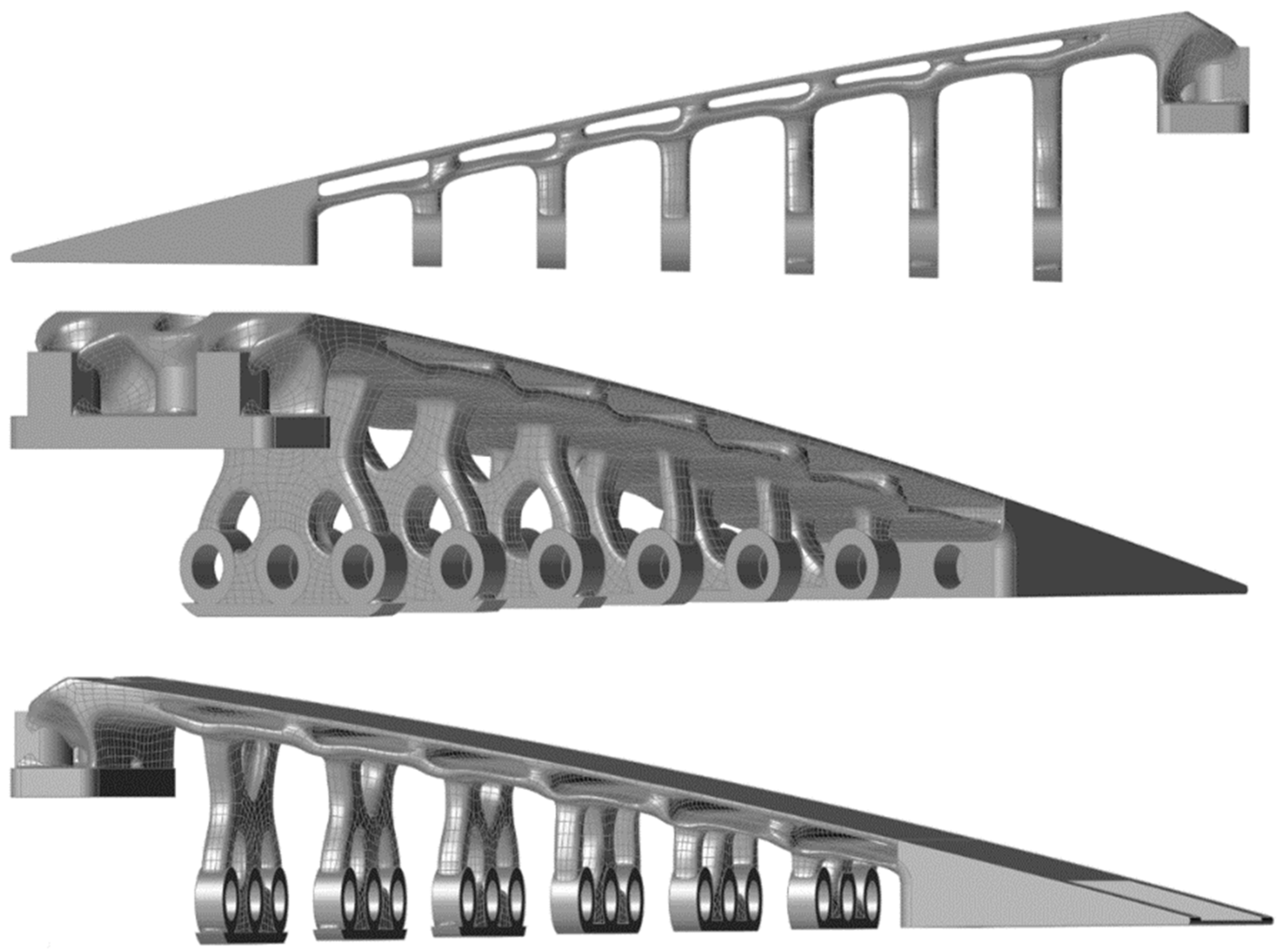
3.6. Topology Optimization
3.7. Elephant Trunk Mechanism Integration on the UAS-S45 Wing Box
3.8. Skin Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Specification | Value |
|---|---|
| Wing span | 6.11 m |
| Wing area | 2.72 m2 |
| Total length | 3.01 m |
| Mean aerodynamic chord | 0.57 m |
| Empty weight | 57 kg |
| Maximum take-off Weight | 79.6 kg |
| Loitering airspeed | 55 knots |
| Service ceiling | 17,000 ft |
| Operational range | 120 km |

Appendix B
| Element Size (mm) | Run Time (S) | Displacement (mm) | Relative Error % | Von Mises Stress MPa | Relative Error % |
|---|---|---|---|---|---|
| 6 | 32 | 59.32 | 4.87 | 43.39 | 26.85 |
| 4 | 42 | 61.56 | 1.28 | 52.27 | 11.88 |
| 2 | 116 | 62.17 | 0.30 | 58.45 | 1.47 |
| 1.5 | 218 | 62.24 | 0.19 | 57.45 | 3.15 |
| 1.25 | 368 | 62.31 | 0.08 | 58.80 | 0.88 |
| 1 | 727 | 62.36 | - | 59.32 | - |
References
- Ameduri, S.; Concilio, A. Morphing wings review: Aims, challenges, and current open issues of a technology. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2023, 237, 4112–4130. [Google Scholar] [CrossRef]
- Mrazova, M. Sustainable development-the key for green aviation. INCAS Bull. 2014, 6, 109–122. [Google Scholar]
- Khalid, M.; Juhany, K. Effects of blowing upon dynamic stability of blunt nosed re entry vehicles pitching in hypersonic flow. Aeronaut. J. 2024, 128, 547–558. [Google Scholar] [CrossRef]
- Bartley-Cho, J.D.; Wang, D.P.; Martin, C.A.; Kudva, J.N.; West, M.N. Development of high-rate, adaptive trailing edge control surface for the smart wing phase 2 wind tunnel model. J. Intell. Mater. Syst. Struct. 2004, 15, 279–291. [Google Scholar] [CrossRef]
- Kota, S.; Osborn, R.; Ervin, G.; Maric, D.; Flick, P.; Paul, D. Mission adaptive compliant wing–design, fabrication and flight test. In Proceedings of the RTO Applied Vehicle Technology Panel (AVT) Symposium, Lisbon, Portugal, 20–24 April 2009; pp. 1–18. [Google Scholar]
- Botez, R.M.; Koreanschi, A.; Gabor, O.S.; Tondji, Y.; Guezguez, M.; Kammegne, J.; Grigorie, L.; Sandu, D.; Mebarki, Y.; Mamou, M.; et al. Numerical and experimental transition results evaluation for a morphing wing and aileron system. Aeronaut. J. 2018, 122, 747–784. [Google Scholar] [CrossRef]
- Ameduri, S.; Concilio, A.; Dimino, I.; Pecora, R.; Ricci, S. AIRGREEN2—Clean Sky 2 Programme: Adaptive Wing Technology Maturation, Challenges and Perspectives. In Proceedings of the ASME 2018 Conference on Smart Materials, Adaptive Structures and Intelligent Systems, San Antonio, TX, USA, 10–12 September 2018. [Google Scholar]
- Liauzun, C.; Le Bihan, D.; David, J.-M.; Joly, D.; Paluch, B. Study of morphing winglet concepts aimed at improving load control and the aeroelastic behavior of civil transport aircraft. Aerosp. Lab 2018, 1–15. [Google Scholar] [CrossRef]
- Eguea, J.P.; Bravo-Mosquera, P.D.; Catalano, F.M. Camber morphing winglet influence on aircraft drag breakdown and tip vortex structure. Aerosp. Sci. Technol. 2021, 119, 107148. [Google Scholar] [CrossRef]
- Ajaj, R.; Friswell, M.; Saavedra Flores, E.; Little, O.; Isikveren, A. Span morphing: A conceptual design study. In Proceedings of the 53rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Honolulu, HI, USA, 23–26 April 2012; p. 1510. [Google Scholar]
- Ismail, N.; Zulkifli, A.; Abdullah, M.; Basri, M.H.; Abdullah, N.S. Optimization of aerodynamic efficiency for twist morphing MAV wing. Chin. J. Aeronaut. 2014, 27, 475–487. [Google Scholar] [CrossRef]
- Mamou, M.; Mébarki, Y.; Khalid, M.; Genest, M.; Coutu, D.; Popov, A.; Sainmont, C.; Georges, T.; Grigorie, L.; Botez, R.; et al. Aerodynamic performance optimization of a wind tunnel morphing wing model subject to various cruise flow conditions. In Proceedings of the 27th International Congress of the Aeronautical Sciences ICAS, Nice, France, 19–24 September 2010; pp. 1–25. [Google Scholar]
- Kan, Z.; Li, D.; Shen, T.; Xiang, J.; Zhang, L. Aerodynamic characteristics of morphing wing with flexible leading-edge. Chin. J. Aeronaut. 2020, 33, 2610–2619. [Google Scholar] [CrossRef]
- Fereidooni, A.; Marchwica, J.; Leung, N.; Mangione, J.; Wickramasinghe, V. Development of a hybrid (rigid-flexible) morphing leading edge equipped with bending and extending capabilities. J. Intell. Mater. Syst. Struct. 2021, 32, 1024–1037. [Google Scholar] [CrossRef]
- Woods, B.K.S.; Friswell, M.I. Preliminary investigation of a fishbone active camber concept. In Proceedings of the ASME 2012 Conference on the Smart Materials, Adaptive Structures and Intelligent Systems, Stone Mountain, GA, USA, 19–21 September 2012; pp. 555–563. [Google Scholar]
- Woods, B.K.; Bilgen, O.; Friswell, M.I. Wind tunnel testing of the fish bone active camber morphing concept. J. Intell. Mater. Syst. Struct. 2014, 25, 772–785. [Google Scholar] [CrossRef]
- Shi, X.; Yang, Y.; Wang, Z.; Zhang, S.; Sun, X.; Feng, W. Design and shape monitoring of a morphing wing trailing edge. Aerospace 2023, 10, 127. [Google Scholar] [CrossRef]
- Cheng, G.; Ma, T.; Yang, J.; Chang, N.; Zhou, X. Design and Experiment of a Seamless Morphing Trailing Edge. Aerospace 2023, 10, 282. [Google Scholar] [CrossRef]
- Dimino, I.; Flauto, D.; Diodati, G.; Concilio, A.; Pecora, R. Actuation system design for a morphing wing trailing edge. Recent Pat. Mech. Eng. 2014, 7, 138–148. [Google Scholar] [CrossRef]
- Campanile, L.; Sachau, D. The belt-rib concept: A structronic approach to variable camber. J. Intell. Mater. Syst. Struct. 2000, 11, 215–224. [Google Scholar] [CrossRef]
- Sinapius, M.; Monner, H.P.; Kintscher, M.; Riemenschneider, J. DLR’s morphing wing activities within the European network. Procedia IUTAM 2014, 10, 416–426. [Google Scholar] [CrossRef]
- Wang, Q.; Xu, Z.; Zhu, Q. Structural design of morphing trailing edge actuated by SMA. Front. Mech. Eng. 2013, 8, 268–275. [Google Scholar] [CrossRef]
- Wu, R.; Soutis, C.; Zhong, S.; Filippone, A. A morphing aerofoil with highly controllable aerodynamic performance. Aeronaut. J. 2017, 121, 54–72. [Google Scholar] [CrossRef]
- Dumont, A. Adjoint-based aerodynamic shape optimization applied to morphing technology on a regional aircraft wing. In Morphing Wing Technologies; Elsevier: Amsterdam, The Netherlands, 2018; pp. 145–174. [Google Scholar]
- Li, B.; Li, G. Analysis and optimization of a camber morphing wing model. Int. J. Adv. Robot. Syst. 2016, 13. [Google Scholar] [CrossRef]
- Nguyen, N.T.; Livne, E.; Precup, N.; Urnes, J.M.; Nelson, C.; Ting, E.; Lebofsky, S. Experimental investigation of a flexible wing with a variable camber continuous trailing edge flap design. In Proceedings of the 32nd AIAA Applied Aerodynamics Conference, Atlanta, GA, USA, 16–20 June 2014; p. 2441. [Google Scholar]
- Di Matteo, N.; Guo, S. Morphing trailing edge flap for high lift wing. In Proceedings of the 52nd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Denver, CO, USA, 4–7 April 2011; p. 2164. [Google Scholar]
- Concilio, A.; Dimino, I.; Pecora, R.; Ciminello, M. Structural design of an adaptive wing trailing edge for enhanced cruise performance. In Proceedings of the 24th AIAA/AHS Adaptive Structures Conference, San Diego, CA, USA, 4–8 January 2016. [Google Scholar]
- Takahashi, H.; Yokozeki, T.; Hirano, Y. Development of variable camber wing with morphing leading and trailing sections using corrugated structures. J. Intell. Mater. Syst. Struct. 2016, 27, 2827–2836. [Google Scholar] [CrossRef]
- Barbarino, S.; Dettmer, W.G.; Friswell, M.I. Morphing trailing edges with shape memory alloy rods. In Proceedings of the 21st International Conference on Adaptive Structures and Technologies (ICAST), University Park, PA, USA, 4–6 October 2010. [Google Scholar]
- Kota, S.; Flick, P.; Collier, F.S. Flight testing of flexfloiltm adaptive compliant trailing edge. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016; p. 0036. [Google Scholar]
- Negahban, M.H.; Bashir, M.; Priolet, C.; Botez, R.M. Novel twist morphing aileron and winglet design for UAS control and performance. Drones 2024, 8, 392. [Google Scholar] [CrossRef]
- Bourchak, M.; Ajaj, R.; Flores, E.S.; Khalid, M.; Juhany, K. Optimum design of a PID controller for the adaptive torsion wing. Aeronaut. J. 2015, 119, 871–889. [Google Scholar] [CrossRef]
- Du, S.; Ang, H. Design and feasibility analyses of morphing airfoil used to control flight attitude. Stroj. Vestn.-J. Mech. Eng. 2012, 58, 46–55. [Google Scholar] [CrossRef]
- Khorrami, M.R.; Lockard, D.P.; Moore, J.B.; Su, J.; Turner, T.L.; Lin, J.C.; Taminger, K.M.; Kahng, S.K.; Verden, S.A. Elastically Deformable Side-Edge Link for Trailing-Edge Flap Aeroacoustic Noise Reduction. U.S. Patent 8,695,925, 15 April 2014. [Google Scholar]
- Woods, B.K.; Parsons, L.; Coles, A.B.; Fincham, J.H.; Friswell, M.I. Morphing elastically lofted transition for active camber control surfaces. Aerosp. Sci. Technol. 2016, 55, 439–448. [Google Scholar] [CrossRef]
- Negahban, M.H.; Bashir, M.; Traisnel, V.; Botez, R.M. Seamless morphing trailing edge flaps for UAS-S45 using high-fidelity aerodynamic optimization. Chin. J. Aeronaut. 2024, 37, 12–29. [Google Scholar] [CrossRef]
- Hydra Technologies. UAS-S45 Baalam. Available online: https://www.hydra-technologies.com/s45 (accessed on 19 April 2025).
- Communier, D.; Botez, R.M.; Wong, T. Design and validation of a new morphing camber system by testing in the price—Païdoussis subsonic wind tunnel. Aerospace 2020, 7, 23. [Google Scholar] [CrossRef]
- Negahban, M.H.; Bashir, M.; Botez, R.M. Free-form deformation parameterization on the aerodynamic optimization of morphing trailing edge. Appl. Mech. 2023, 4, 304–316. [Google Scholar] [CrossRef]
- Negahban, M.H.; Bashir, M.; Botez, R.M. Aerodynamic optimization of a novel synthetic trailing edge and chord elongation morphing: Application to the UAS-S45 airfoil. In Proceedings of the AIAA SCITECH 2023 Forum, National Harbor, MD, USA & Online, 23–27 January 2023; p. 1582. [Google Scholar]
- Negahban, M.H.; Bashir, M.; Botez, R.M. Impact of Free-Form Deformation Control Points on the Optimization of the UAS-S45. In New Technologies and Developments in Unmanned Systems, Proceedings of the International Symposium on Unmanned Systems and the Defense Industry, Madrid, Spain, 30 May–1 June 2022; Springer: Cham, Switzerland, 2023; pp. 21–27. [Google Scholar]
- Smith, K.K.; Kier, W.M. Trunks, tongues, and tentacles: Moving with skeletons of muscle. Am. Sci. 1989, 77, 28–35. [Google Scholar]
- Liang, Y.; McMeeking, R.; Evans, A. A finite element simulation scheme for biological muscular hydrostats. J. Theor. Biol. 2006, 242, 142–150. [Google Scholar] [CrossRef]
- Yang, J.; Pitarch, E.P.; Potratz, J.; Beck, S.; Abdel-Malek, K. Synthesis and analysis of a flexible elephant trunk robot. Adv. Robot. 2006, 20, 631–659. [Google Scholar] [CrossRef]
- Available online: https://stock.adobe.com/ca/images/ct-scan-of-an-elephants-trunk-detailing-muscular-and-tissue-structure/806136007?prev_url=detail (accessed on 5 June 2024).
- He, P.; Mader, C.A.; Martins, J.R.; Maki, K.J. Dafoam: An open-source adjoint framework for multidisciplinary design optimization with openfoam. AIAA J. 2020, 58, 1304–1319. [Google Scholar] [CrossRef]
- He, P.; Mader, C.A.; Martins, J.R.; Maki, K. An object-oriented framework for rapid discrete adjoint development using OpenFOAM. In Proceedings of the AIAA Scitech 2019 Forum, San Diego, CA, USA, 7–11 January 2019; p. 1210. [Google Scholar]
- Federal Aviation Administration. 1970. Available online: https://www.ecfr.gov/current/title-14/part-25/section-25.303 (accessed on 10 August 2024).
- Beccari, C.V.; Ceruti, A.; Chudy, F. From Topological Optimization to Spline Layouts: An Approach for Industrial Real-Wise Parts. Axioms 2025, 14, 72. [Google Scholar] [CrossRef]
- Bacciaglia, A.; Ceruti, A.; Ciccone, F.; Liverani, A. Topology optimization for thin-walled structures with distributed loads. In Advances on Mechanics, Design Engineering and Manufacturing IV, Proceedings of the International Joint Conference on Mechanics, Design Engineering & Advanced Manufacturing, Ischia, Italy, 1–3 June 2022; Springer: Cham, Switzerland, 2022; pp. 1042–1054. [Google Scholar]
- Bacciaglia, A.; Ceruti, A.; Liverani, A. Surface smoothing for topological optimized 3D models. Struct. Multidiscip. Optim. 2021, 64, 3453–3472. [Google Scholar] [CrossRef]
- Daynes, S. High stiffness topology optimised lattice structures with increased toughness by porosity constraints. Mater. Des. 2023, 232, 112183. [Google Scholar] [CrossRef]







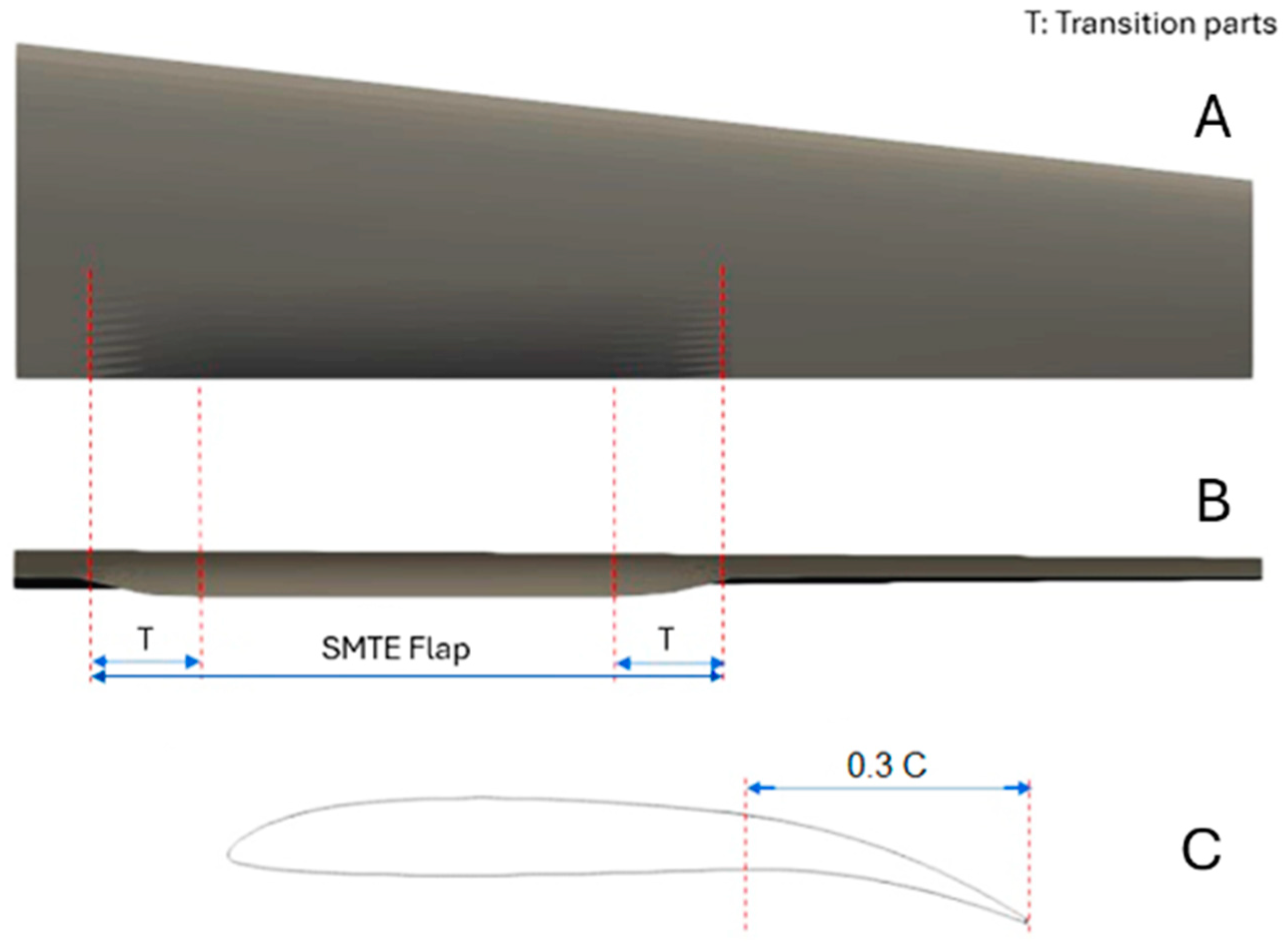


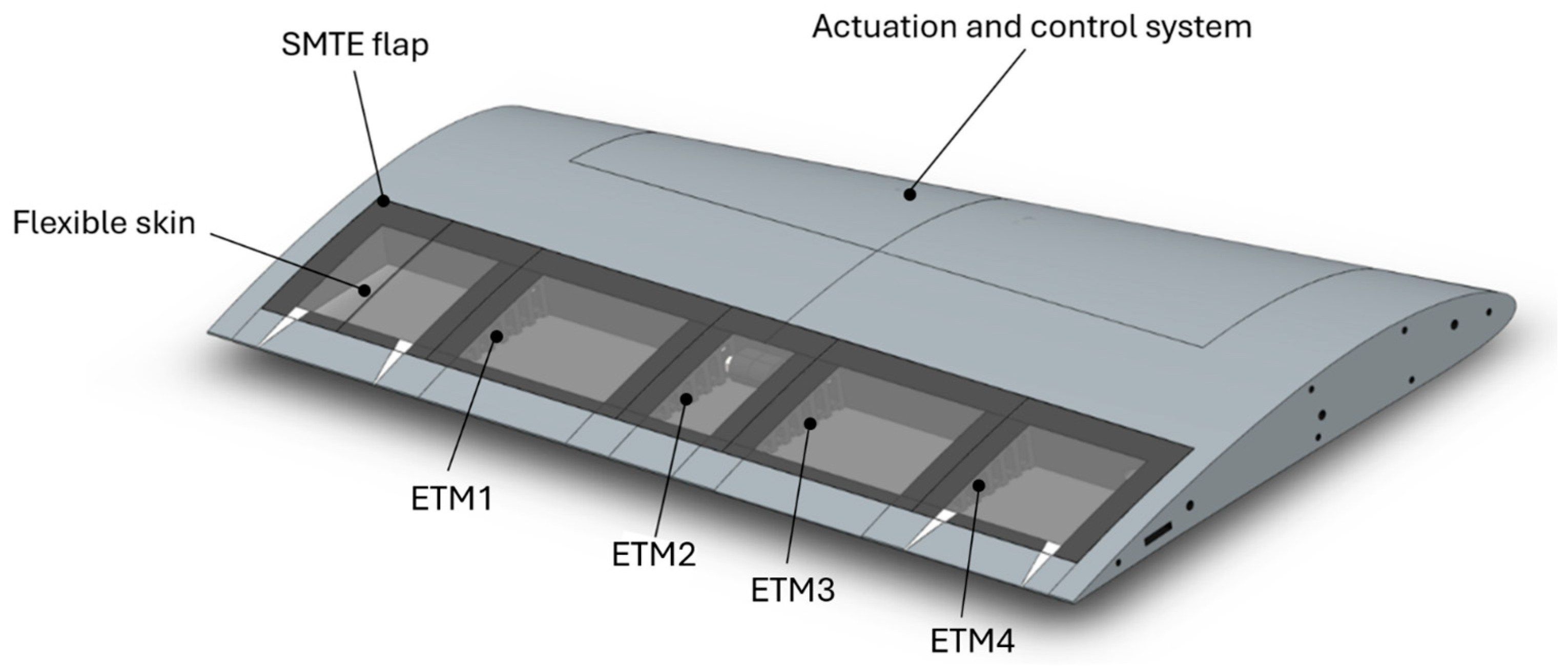
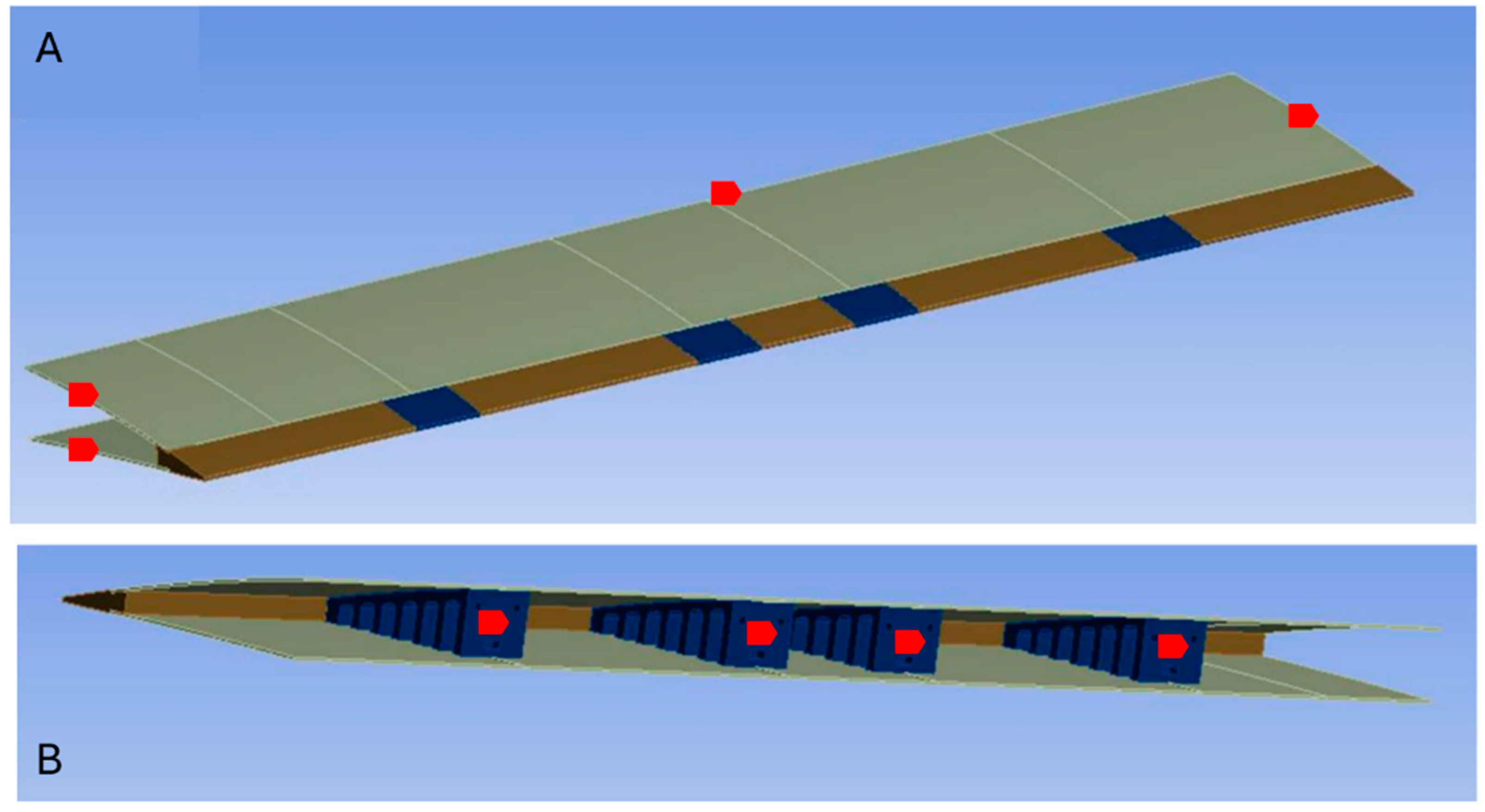

| Part | Material | Young’s Modulus (MPa) | Poisson’s Ratio | Density (kg/m3) | Yield Strength (MPa) |
|---|---|---|---|---|---|
| Elastic tooth-like part | Nylon | 2960 | 0.41 | 1140 | 75 |
| Solid edge | Aluminium | 70,000 | 0.33 | 2700 | 40 |
| Cables | High Carbon Steel | 200,000 | 0.29 | 7870 | 375 |
| Cable/Hole Contact Type | Max. Von Mises Stress (MPa) | Max. Strain % | Min. FoS | Max. Displacement (mm) |
|---|---|---|---|---|
| Sliding | 58.94 | 1.5 | 1.3 | 32.8 |
| No contact | 105.3 | 1.6 | 1.2 | 39.53 |
| Contact Type | Advantages | Disadvantages |
|---|---|---|
| Sliding |
|
|
| No-contact |
|
|
| Number of Teeth | Maximum Force with the Factor of Safety of 1.5 (N) | ||
|---|---|---|---|
| Tooth Thickness (mm) | |||
| 5 | 6 | 8 | |
| 3 | 100.92 | 106.80 | 105.11 |
| 4 | 101.55 | 107.40 | 106.87 |
| 5 | 104.84 | 108.34 | 109.75 |
| 6 | 106.23 | 108.36 | 108.25 |
| 7 | 106.24 | 110.54 | 109.40 |
| 8 | 105.87 | 108.40 | 110.26 |
| 9 | 106.21 | 109.54 | 111.27 |
| 10 | 107.66 | 112.10 | 109.94 |
| Tooth Thickness (mm) | Weighting Factor | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 5 | 6 | 8 | ||||||||
| Number of Teeth | 6 | 7 | 10 | 6 | 7 | 10 | 6 | 7 | 10 | |
| Structural Weight (g) | 150 | 158 | 184 | 170 | 191 | 201 | 208 | 222 | 235 | 3 |
| Score | 27 | 24 | 18 | 21 | 15 | 12 | 9 | 6 | 3 | |
| Vertical Displacement (mm) | 51.4 | 50.1 | 47 | 50 | 46.4 | 45.9 | 43.5 | 41.8 | 39.3 | 2 |
| Score | 18 | 16 | 12 | 14 | 10 | 8 | 6 | 4 | 2 | |
| Total score | 45 | 40 | 30 | 35 | 25 | 20 | 15 | 10 | 5 | |
| Ranking | 1 | 2 | 4 | 3 | 5 | 6 | 7 | 8 | 9 | |
| Thickness (mm) | Force (N) | Displacement (mm) | FoS | Von Mises Stress (MPa) | Weight (g) |
|---|---|---|---|---|---|
| 4 | 35 | 62.49 | 2.2 | 33.6 | 81 |
| 28 | 50.35 | 2.8 | 27.1 | ||
| 21 | 38.21 | 3.6 | 20.6 | ||
| 14 | 26.07 | 5.3 | 14.1 | ||
| 7 | 13.93 | 9.8 | 7.6 | ||
| 8 | 300 | 62.39 | 0.9 | 80.1 | 122 |
| 240 | 51.57 | 1.2 | 64.1 | ||
| 180 | 38.75 | 1.6 | 48.2 | ||
| 120 | 25.93 | 2.3 | 32.3 | ||
| 60 | 13.11 | 4.6 | 16.3 | ||
| 12 | 1000 | 62.94 | 0.6 | 128.4 | 162 |
| 800 | 50.37 | 0.7 | 102.8 | ||
| 600 | 37.81 | 1.0 | 77.1 | ||
| 400 | 25.24 | 1.5 | 51.5 | ||
| 200 | 12.68 | 2.9 | 25.9 | ||
| 16 | 2500 | 63.48 | 0.4 | 208.2 | 201 |
| 2000 | 54.00 | 0.5 | 166.6 | ||
| 1500 | 40.52 | 0.6 | 125.1 | ||
| 1000 | 27.03 | 0.9 | 83.5 | ||
| 500 | 13.55 | 1.8 | 42.0 |
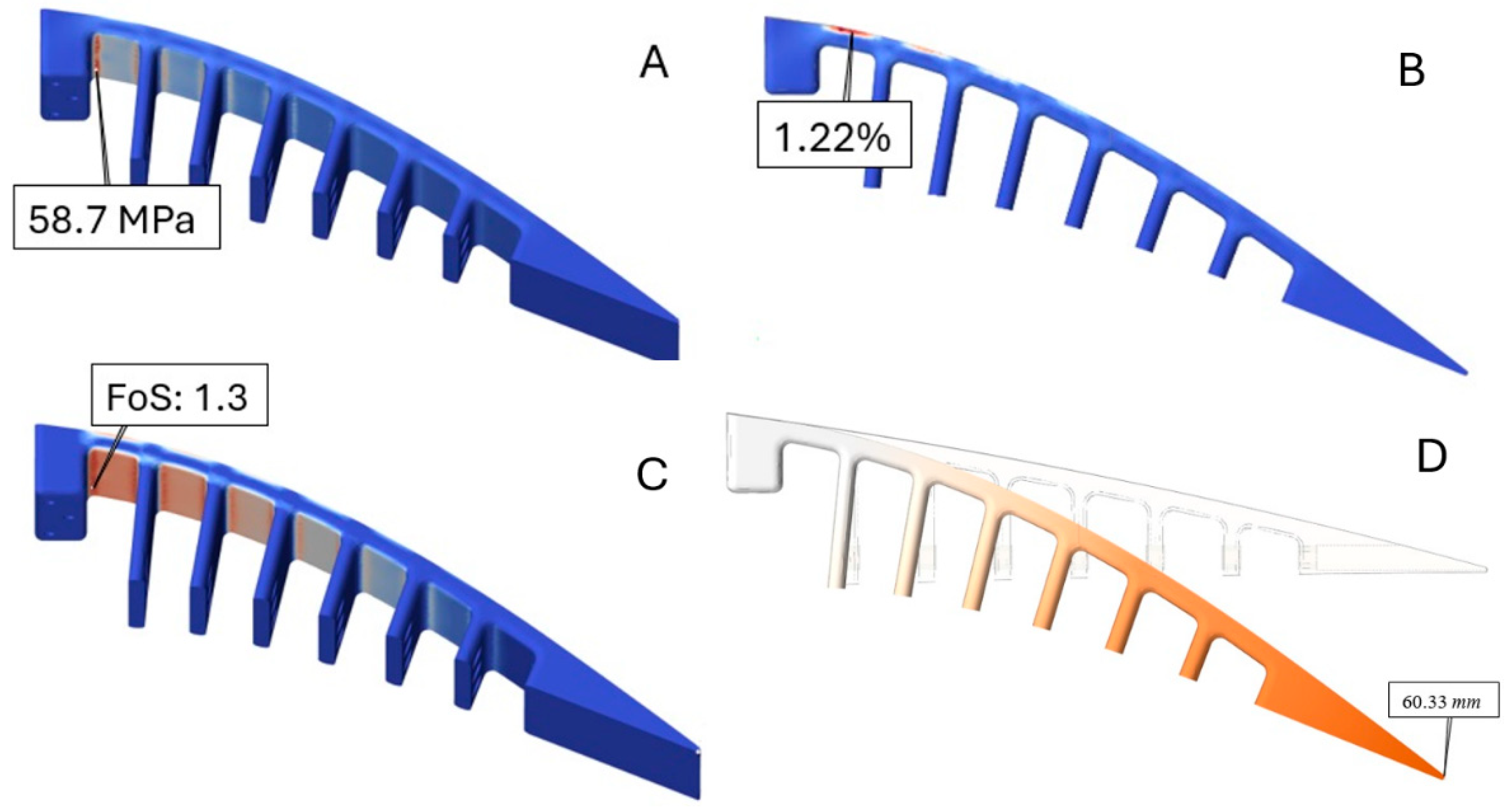
| Max. Von-Mise Stress (MPa) | Max. Strain% | Min. Factor of Safety | Max. Displacement (mm) | |
|---|---|---|---|---|
| Initial model | 58.94 | 1.51 | 1.3 | 32.8 |
| Modified model | 58.7 | 1.22 | 1.3 | 60.33 |
| Function/Variable | Description |
|---|---|
| Objective functions | |
| Min. Min. | minimization of structural compliance minimization of weight |
| Subject to: | |
| Equilibrium constraint function | |
| Material distribution constraint | |
| Volume constraint | |
| 0 | Density constraint |
| Symmetry constraint | |
| Stiffness constraint |
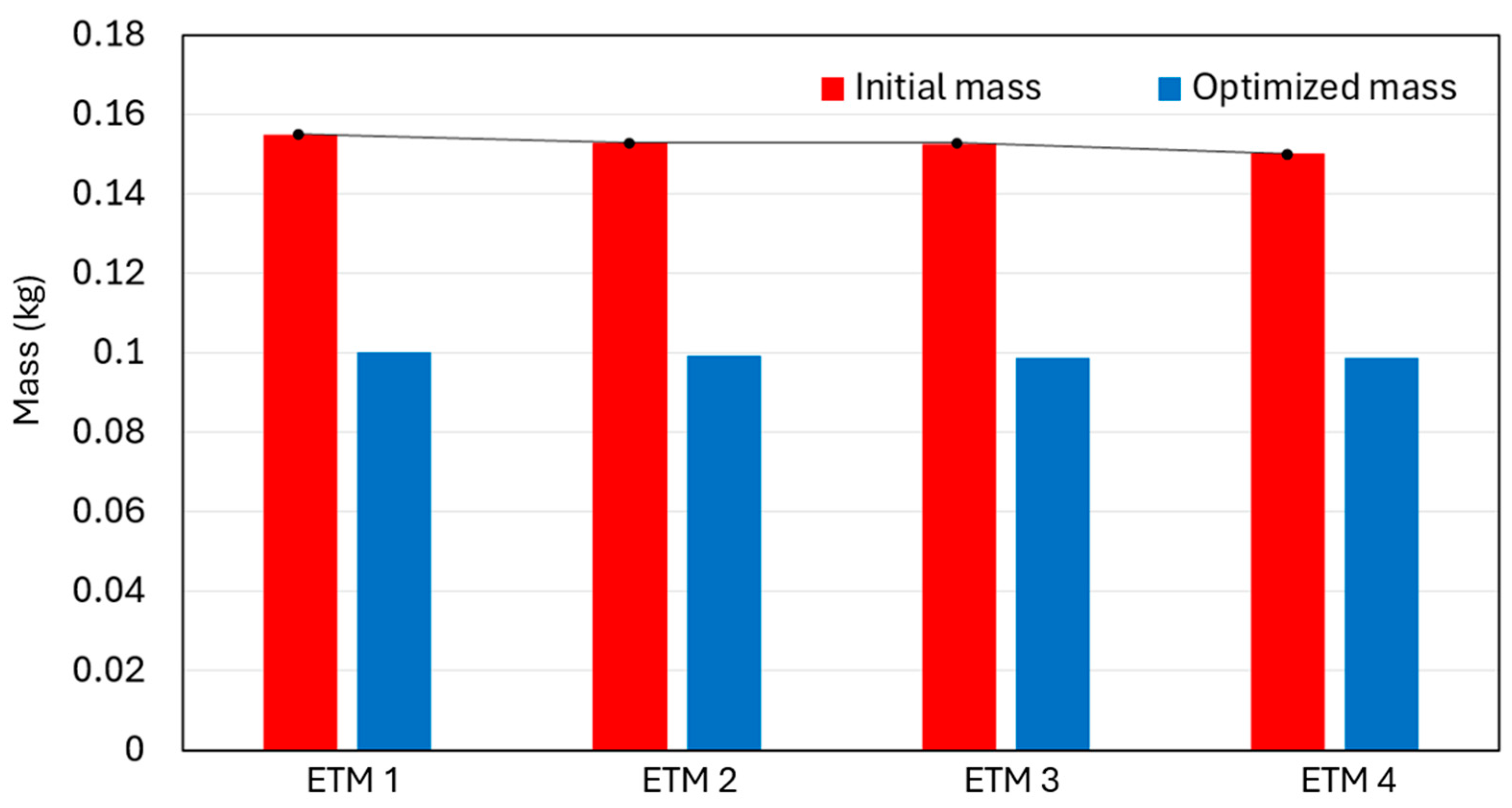
| Max. Von Mises Stress (MPa) | Max. Strain% | Min. FoS | Max. Vertical Displacement (mm) | Mass (kg) | |
|---|---|---|---|---|---|
| Initial ETM | 58.7 | 1.2 | 1.3 | 60.33 | 0.155 |
| Optimized ETM | 52.58 | 1.63 | 1.5 | 66.02 | 0.100 |
| Young’s Modulus (MPa) | Poisson Ratio | Tensile Yield Strength (MPa) | Density (kg/m3) |
|---|---|---|---|
| 17 | 0.5 | 10.4 | 1230 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Negahban, M.H.; Hallonet, A.; Noupoussi Woumeni, M.; Nguyen, C.; Botez, R.M. Structural and Topological Optimization of a Novel Elephant Trunk Mechanism for Morphing Wing Applications. Aerospace 2025, 12, 381. https://doi.org/10.3390/aerospace12050381
Negahban MH, Hallonet A, Noupoussi Woumeni M, Nguyen C, Botez RM. Structural and Topological Optimization of a Novel Elephant Trunk Mechanism for Morphing Wing Applications. Aerospace. 2025; 12(5):381. https://doi.org/10.3390/aerospace12050381
Chicago/Turabian StyleNegahban, Mir Hossein, Alexandre Hallonet, Marie Noupoussi Woumeni, Constance Nguyen, and Ruxandra Mihaela Botez. 2025. "Structural and Topological Optimization of a Novel Elephant Trunk Mechanism for Morphing Wing Applications" Aerospace 12, no. 5: 381. https://doi.org/10.3390/aerospace12050381
APA StyleNegahban, M. H., Hallonet, A., Noupoussi Woumeni, M., Nguyen, C., & Botez, R. M. (2025). Structural and Topological Optimization of a Novel Elephant Trunk Mechanism for Morphing Wing Applications. Aerospace, 12(5), 381. https://doi.org/10.3390/aerospace12050381







