Non-Linear and Quasi-Linear Models for the Large-Amplitude Static Aeroelastic Response of Very-Flexible Slender Wings in Subsonic Flow at Low Speed
Abstract
1. Introduction
2. Aeroelastic Problem Formulation
3. Continuous Non-Linear and Quasi-Linear Models
3.1. Semi-Analytical Solution for a Uniform Beam-like Wing Structure
3.1.1. Open-Loop Aero-Structural Response
3.1.2. Closed-Loop Aeroelastic Response
4. Discrete Non-Linear Model
5. Results and Discussion
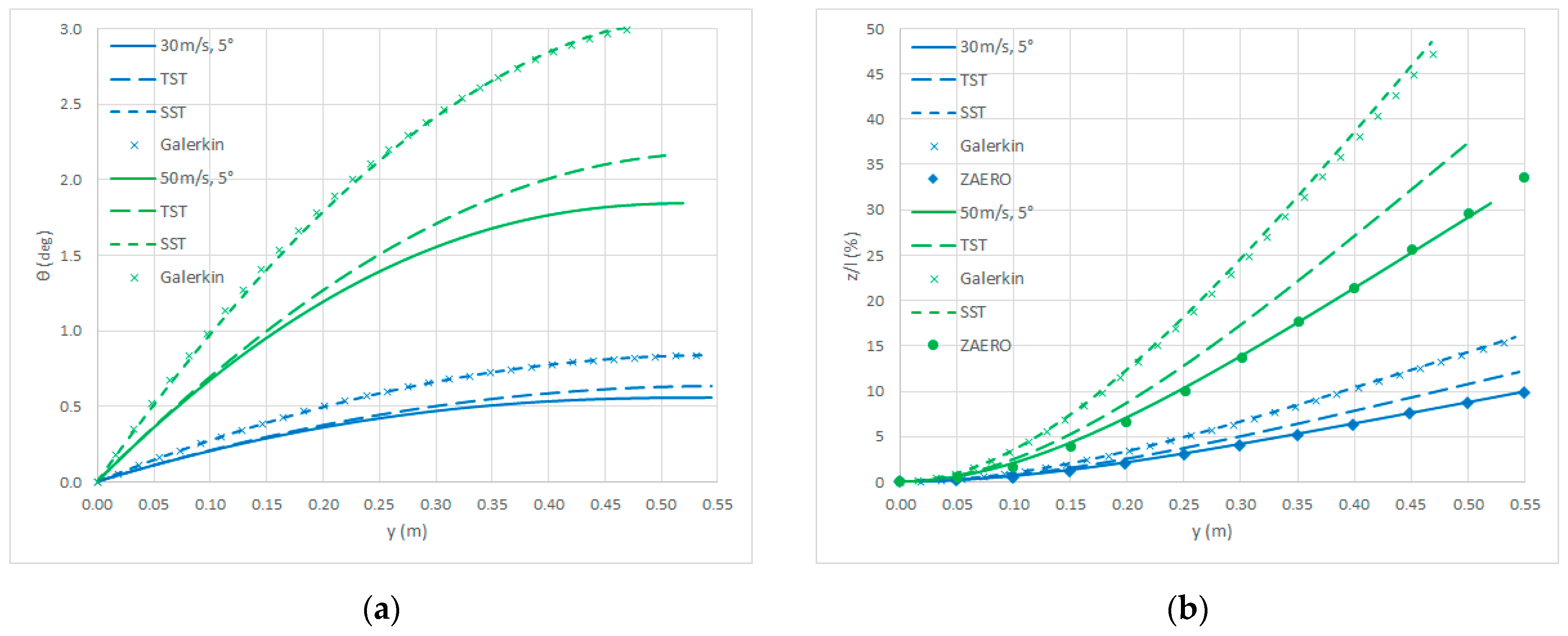
5.1. Open-Loop Aero-Structural Response
5.2. Closed-Loop Aeroelastic Response
6. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| CFD | computational fluid dynamics |
| DLM | doublet lattice method |
| FEM | finite element method |
| HALE | High-Altitude Long-Endurance |
| HBCM | Hencky’s bar-chain model |
| LLT | lifting-line theory |
| MDO | Multidisciplinary Design and Optimisation |
| MRM | modal rotation method |
| MST | modified strip theory |
| POD | proper orthogonal decomposition |
| ROM | reduced-order model |
| SST | standard strip theory |
| TST | tuned strip theory |
| VLM | vortex lattice method |
| WLM | Weissinger’s line method |
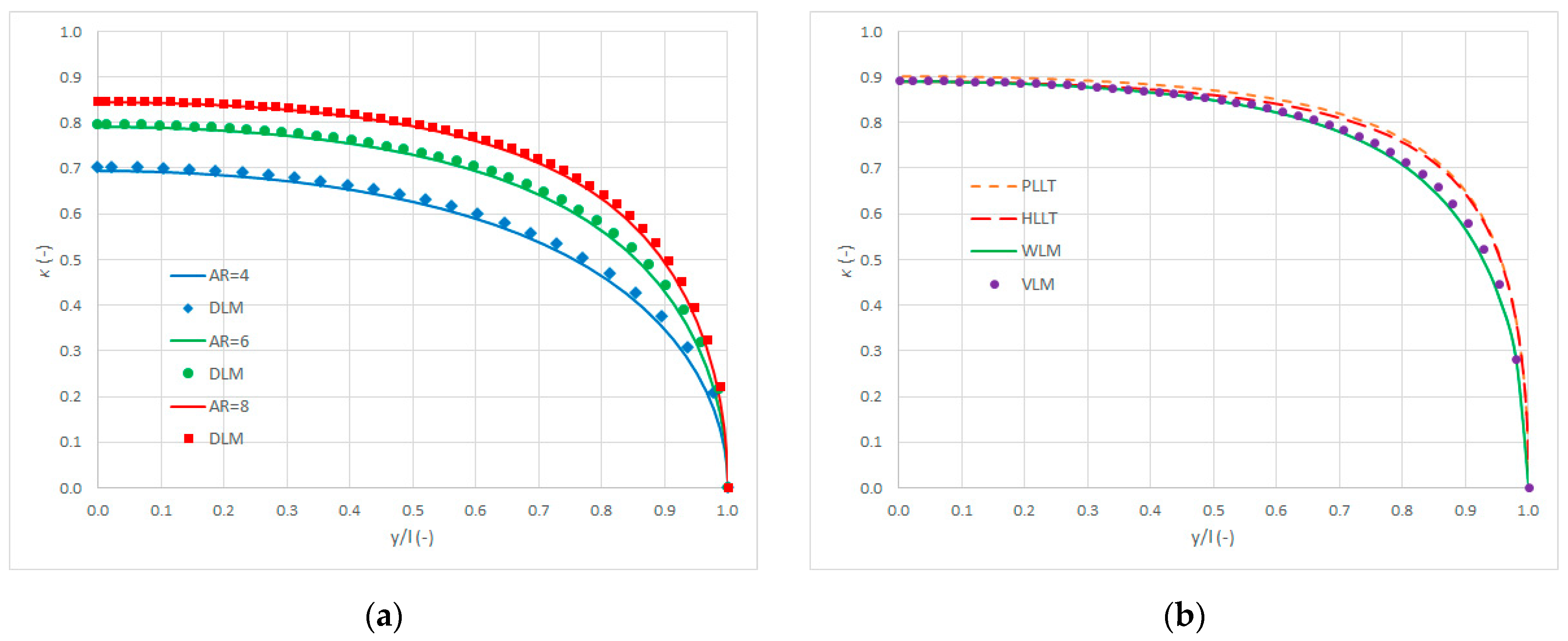
Appendix A. Weissinger’s Line Method
Appendix B. Structural Open-Loop Response to Tip Weight
Appendix C. Quasi-Aeroelastic Model and Closed-Loop Response

References
- Tang, D.; Dowell, E.H. Experimental and Theoretical Study on Aeroelastic Response of High-Aspect-Ratio Wings. AIAA J. 2001, 39, 1430–1441. [Google Scholar] [CrossRef]
- Riso, C.; Cesnik, C. Geometrically Nonlinear Effects in Wing Aeroelastic Dynamics at Large Deflections. J. Fluids Struct. 2023, 120, 103897. [Google Scholar] [CrossRef]
- Livne, E. The Future of Aircraft Aeroelasticity. J. Aircr. 2003, 40, 1066–1092. [Google Scholar] [CrossRef]
- Martins, J.R.; Lambe, A.B. Multidisciplinary Design Optimization: A Survey of Architectures. AIAA J. 2013, 51, 2049–2075. [Google Scholar] [CrossRef]
- Quarteroni, A.; Rozza, G. Reduced Order Methods for Modeling and Computational Reduction; Springer International Publishing: Cham, Switzerland, 2014. [Google Scholar]
- Qu, Z.Q. Model Order Reduction Techniques with Applications in Finite Element Analysis; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Fossati, M. Evaluation of Aerodynamic Loads via Reduced-Order Methodology. AIAA J. 2015, 53, 2389–2405. [Google Scholar] [CrossRef]
- Vanderplaats, G.N. Numerical Optimization Techniques for Engineering Design: With Applications; McGraw Hill: New York, NY, USA, 1984. [Google Scholar]
- Berci, M.; Torrigiani, F. Multifidelity Sensitivity Study of Subsonic Wing Flutter for Hybrid Approaches in Aircraft Multidisciplinary Design and Optimisation. Aerospace 2020, 7, 161. [Google Scholar] [CrossRef]
- Berci, M. Semi-Analytical Static Aeroelastic Analysis and Response of Flexible Subsonic Wings. Appl. Math. Comput. 2015, 267, 148–169. [Google Scholar] [CrossRef]
- Berci, M.; Cavallaro, R. A Hybrid Reduced-Order Model for the Aeroelastic Analysis of Flexible Subsonic Wings—A Parametric Assessment. Aerospace 2018, 5, 76. [Google Scholar] [CrossRef]
- Vindigni, C.R.; Mantegna, G.; Orlando, C.; Alaimo, A.; Berci, M. A Refined Aeroelastic Beam Finite Element for the Stability Analysis of Flexible Subsonic Wings. Comput. Struct. 2025, 307, 107618. [Google Scholar] [CrossRef]
- Bungartz, H.J.; Schafer, M. Fluid-Structure Interaction: Modelling, Simulation, Optimization; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Anderson, J.D. Fundamentals of Aerodynamics; McGraw-Hill: New York, NY, USA, 2017. [Google Scholar]
- Heil, M.; Haze, A.; Boyle, J. Solvers for Large-Displacement Fluid–Structure Interaction Problems: Segregated Versus Monolithic Approaches. Comput. Mech. 2008, 43, 91–101. [Google Scholar] [CrossRef]
- Pike, E.C. Manual on Aeroelasticity; AGARD 578; AGARD: London, UK, 1971. [Google Scholar]
- Wright, J.R.; Cooper, J.E. Introduction to Aircraft Aeroelasticity and Loads; Wiley: Hoboken, NY, USA, 2014. [Google Scholar]
- Dhatt, G.; Lefrançois, E.; Touzot, G. Finite Element Method; Wiley: Hoboken, NY, USA, 2013. [Google Scholar]
- Rodden, W.P. Theoretical and Computational Aeroelasticity; Crest Publishing: Fort Worth, TX, USA, 2011. [Google Scholar]
- Demasi, L. Introduction to Unsteady Aerodynamics and Dynamic Aeroelasticity; Springer: Berlin/Heidelberg, Germany, 2024. [Google Scholar]
- Katz, J.; Plotkin, A. Low-Speed Aerodynamics; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Dimitriadis, G. Unsteady Aerodynamics: Potential and Vortex Methods; Wiley: Hoboken, NY, USA, 2023. [Google Scholar]
- Chung, T.J. Computational Fluid Dynamics; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Avin, O.; Raveh, D.; Drachinsky, A.; Ben-Shmuel, Y.; Tur, M. Experimental Aeroelastic Benchmark of a Very Flexible Wing. AIAA J. 2022, 60, 1745–1768. [Google Scholar]
- Drachinsky, A.; Raveh, D. Nonlinear Aeroelastic Analysis of Highly Flexible Wings Using the Modal Rotation Method. AIAA J. 2022, 60, 3122–3134. [Google Scholar]
- Hilger, J.; Ritter, M. Nonlinear Aeroelastic Simulations and Stability Analysis of the Pazy Wing Aeroelastic Benchmark. Aerospace 2021, 8, 308. [Google Scholar] [CrossRef]
- Riso, C.; Cesnik, C. Impact of Low-Order Modeling on Aeroelastic Predictions for Very Flexible Wings. J. Aircr. 2023, 60, 662–687. [Google Scholar]
- Dowell, E.H. A Modern Course in Aeroelasticity; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Levy, R.; Spillers, W.R. Analysis of Geometrically Nonlinear Structures; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Dimitriadis, G. Introduction to Nonlinear Aeroelasticity; Wiley: Hoboken, NY, USA, 2017. [Google Scholar]
- Berci, M.; Gaskell, P.; Hewson, R.; Toropov, V. Multifidelity Metamodel Building as a Route to Aeroelastic Optimization of Flexible Wings. J. Mech. Eng. Sci. 2011, 225, 2115–2137. [Google Scholar]
- Berci, M.; Toropov, V.; Hewson, R.; Gaskell, P. Multidisciplinary Multifidelity Optimisation of a Flexible Wing Aerofoil with Reference to a Small UAV. Struct. Multidiscip. Optim. 2014, 50, 683–699. [Google Scholar]
- Sheldon, J.; Miller, S.; Pitt, J. Methodology for Comparing Coupling Algorithms for Fluid-Structure Interaction Problems. World J. Mech. 2014, 4, 54–70. [Google Scholar]
- Sucipto, T.; Berci, M.; Krier, J. Gust Response of a Flexible Typical Section via High- and (Tuned) Low-Fidelity Simulations. Comput. Struct. 2013, 122, 202–216. [Google Scholar]
- Berci, M.; Mascetti, S.; Incognito, A.; Gaskell, P.; Toropov, V. Dynamic Response of Typical Section Using Variable-Fidelity Fluid Dynamics and Gust-Modeling Approaches—With Correction Methods. J. Aerosp. Eng. 2014, 27, 1–20. [Google Scholar]
- Forrester, A.J.; Keane, A.J. Engineering Design via Surrogate Modelling: A Practical Guide; Wiley: Hoboken, NY, USA, 2008. [Google Scholar]
- Fung, Y.C. An Introduction to the Theory of Aeroelasticity; Dover: Mineola, NY, USA, 1993. [Google Scholar]
- Hodges, D.; Pierce, G. Introduction to Structural Dynamics and Aeroelasticity; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Weisshaar, T.A. Static and Dynamic Aeroelasticity; Wiley: Hoboken, NY, USA, 2010. [Google Scholar]
- Bisplinghoff, R.L.; Ashley, H.; Halfman, R.L. Aeroelasticity; Dover: Mineola, NY, USA, 1996. [Google Scholar]
- Bisplinghoff, R.L.; Ashley, H. Principles of Aeroelasticity; Dover: Mineola, NY, USA, 2013. [Google Scholar]
- Cavagna, L.; Ricci, S.; Travaglini, L. NeoCASS: An Integrated Tool for Structural Sizing, Aeroelastic Analysis and MDO at Conceptual Design Level. Prog. Aerosp. Sci. 2011, 47, 621–635. [Google Scholar]
- Sotoudeh, Z.; Hodges, D.; Chang, C.-S. Validation Studies for Aeroelastic Trim and Stability Analysis of Highly Flexible Aircraft. J. Aircr. 2010, 47, 1240–1247. [Google Scholar] [CrossRef]
- Murua, J.; Palacios, R.; Graham, J. Applications of the unsteady vortex-lattice method in aircraft aeroelasticity and flight dynamics. Prog. Aerosp. Sci. 2012, 55, 46–72. [Google Scholar] [CrossRef]
- Hencky, H. Über die Angenäherte Lösung von Stabilitätsproblemen im Raum Mittels der Elastischen Gelenkkette. Der Eisenbau 1920, 11, 437–452. [Google Scholar]
- Wang, C.; Zhang, H.; Challamel, N.; Pan, W. Hencky Bar-Chain/Net for Structural Analysis; World Scientific Publishing: Singapore, 2020. [Google Scholar]
- Le, V.; Nguyen, A.; Dang, N. Multibody-Dynamics Approach to Study the Deformation and Aerodynamics of an Insect Wing. AIAA J. 2023, 61, 2500–2516. [Google Scholar] [CrossRef]
- Young, W.C.; Budynas, R.G. Roark’s Formulas for Stress and Strain; McGraw-Hill: New York, NY, USA, 2011. [Google Scholar]
- Megson, T.H.G. Aircraft Structures for Engineering Students; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Amabili, M. Nonlinear Vibrations and Stability of Shells and Plates; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Swischuk, R.; Mainini, L.; Peherstorfer, B.; Willcox, K. Projection-Based Model Reduction: Formulations for Physics-Based Machine Learning. Comput. Fluids 2019, 179, 704–717. [Google Scholar] [CrossRef]
- Farhat, C.; Lesoinne, M.; LeTallec, P. Load and Motion Transfer Algorithms for Fluid/Structure Interaction Problems with Non-Matching Discrete Interfaces: Momentum and Energy Conservation, Optimal Discretization and Application to Aeroelasticity. Comput. Methods Appl. Mech. Eng. 1998, 157, 95–114. [Google Scholar]
- Yang, B. Strain, Stress and Structural Dynamics; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Hampton, R.; Leamy, M.; Bryant, P.; Quraishi, N. Deformation and Flexibility Equations for Curved, End-Loaded, Planar Elastica. AIAA J. 2008, 46, 356–363. [Google Scholar]
- Parnes, R. On the Accuracy of Linear Beam Theory. AIAA J. 1979, 17, 219–220. [Google Scholar] [CrossRef]
- Peters, D.A. Two-Dimensional Incompressible Unsteady Airfoil Theory–A Review. J. Fluids Struct. 2008, 24, 295–312. [Google Scholar] [CrossRef]
- Glauert, H. The Elements of Aerofoil and Airscrew Theory; Cambridge University Press: Cambridge, UK, 1983. [Google Scholar]
- Thwapiah, G.; Campanile, L.F. Nonlinear Aeroelastic Behavior of Compliant Aerofoils. Smart Mater. Struct. 2010, 19, 035020. [Google Scholar]
- Gal-Rom, Y.C.; Raveh, D. Simplified Aerostructural Static Model for Inflated Wings. AIAA J. 2011, 49, 1180–1190. [Google Scholar]
- Prandtl, L. Applications of Modern Hydrodynamics to Aeronautics; NACA-TR-116; NACA: Boston, MA, USA, 1921. [Google Scholar]
- Holmes, R.B. A Course on Optimization and Best Approximation; Springer: Berlin/Heidelberg, Germany, 1972. [Google Scholar]
- Berci, M. On the Incipient Indicial Lift of Thin Wings in Subsonic Flow: Acoustic Wave Theory with Unsteady Three-Dimensional Effects. Acoustics 2022, 4, 26–52. [Google Scholar] [CrossRef]
- Quarteroni, A.; Sacco, R.; Saleri, F. Numerical Mathematics; Springer-Verlag: Berlin, Germany, 2000. [Google Scholar]
- Antipov, Y. Nonlinear Bending Models of Beams and Plates. Proc. R. Soc.—A 2014, 470, 20140064. [Google Scholar] [PubMed]
- Belendez, T.; Neipp, C.; Belendez, A. Numerical and Experimental Analysis of a Cantilever Beam: A Laboratory Project to Introduce Geometric Nonlinearity in Mechanics of Materials. Int. J. Eng. Educ. 2003, 19, 885–892. [Google Scholar]
- Shvartsman, B.S. Large Deflections of a Cantilever Beam Subjected to a Follower Force. J. Sound Vib. 2007, 304, 969–973. [Google Scholar]
- Kierzenka, J.; Shampine, L.F. A BVP Solver that Controls Residual and Error. J. Numer. Anal. Appl. Math. 2008, 3, 27–41. [Google Scholar]
- Gürgöze, M. A Note on the Vibrations of Restrained Beams and Rods with Point Masses. J. Sound Vib. 1984, 96, 461–468. [Google Scholar]
- Belendez, T.; Neipp, C.; Belendez, A. Large and Small Deflections of a Cantilever Beam. Eur. J. Phys. 2002, 23, 371–379. [Google Scholar]
- Weissinger, J. The Lift Distribution of Swept-Back Wings; NACA TM 1120; NACA: Boston, MA, USA, 1947. [Google Scholar]
- Duncan, W.J. Galerkin’s Method in Mechanics and Differential Equations; ARC RM 1798; ARC: London, UK, 1937. [Google Scholar]
- Reddy, J.N. Energy Principles and Variational Methods in Applied Mechanics; Wiley: Hoboken, NY, USA, 2002. [Google Scholar]
- Flax, A.H. The Influence of Structural Deformation on Airplane Characteristics. J. Aeronaut. Sci. 1945, 12, 94–102. [Google Scholar]
- Pugsley, A.G. Influence of Wing Elasticity upon the Longitudinal Stability of an Aeroplane; ARC RM 1548; ARC: London, UK, 1933. [Google Scholar]
- Seifert, G. A Third Order Boundary Value Problem Arising in Aeroelastic Wing Theory. Q. Appl. Math. 1951, 9, 210–218. [Google Scholar]
- Ghiringhelli, G.; Masarati, P.; Mantegazza, P. Multibody Implementation of Finite Volume C0 Beams. AIAA J. 2000, 38, 131–138. [Google Scholar]
- Wang, Y.; Huston, R.L. A Lumped Parameter Method in the Nonlinear Analysis of Flexible Multibody Systems. Comput. Struct. 1994, 50, 421–432. [Google Scholar] [CrossRef]
- Duncan, W.J. A Critical Examination of the Representation of Massive and Elastic Bodies by Systems of Rigid Masses Elastically Connected. Q. J. Mech. Appl. Math. 1952, 5, 97–108. [Google Scholar]
- Leckie, F.A.; Lindberg, G.M. The Effect of Lumped Parameters on Beam Frequencies. Aeronaut. Q. 1963, 14, 224–240. [Google Scholar] [CrossRef]
- Rubinstein, D. Dynamics of a Flexible Beam and a System of Rigid Rods, with Fully Inverse (One-Sided) Boundary Conditions. Comput. Methods Appl. Mech. Eng. 1999, 175, 87–97. [Google Scholar]
- Giorgio, I.; Del Vescovo, D. Non-Linear Lumped-Parameter Modeling of Planar Multi-Link Manipulators with Highly Flexible Arms. Robotics 2018, 7, 60. [Google Scholar] [CrossRef]
- Turco, E. Discrete is it Enough? The Revival of Piola-Hencky Keynotes to Analyze Three-Dimensional Elastica. Contin. Mech. Thermodyn. 2018, 30, 1039–1057. [Google Scholar]
- Baroudi, D.; Giorgio, I.; Battista, A.; Turco, E.; Igumnov, L. Nonlinear Dynamics of Uniformly Loaded Elastica: Experimental and Numerical Evidence of Motion around Curled Stable Equilibrium Configurations. ZAMM J. Appl. Math. Mech. 2019, 99, e201800121. [Google Scholar]
- Turco, E.; Barchiesi, E.; Giorgio, I.; dell’Isola, F. A Lagrangian Hencky-Type Non-Linear Model Suitable for Metamaterials Design of Shearable and Extensible Slender Deformable Bodies Alternative to Timoshenko Theory. Int. J. Non-Linear Mech. 2020, 123, 103481. [Google Scholar]
- Coleman, T.F.; Li, Y. On the Convergence of Reflective Newton Methods for Large-Scale Nonlinear Minimization Subject to Bounds. Math. Program. 1994, 67, 189–224. [Google Scholar]
- Moré, J.J.; Sorensen, D.C. Computing a Trust Region Step. SIAM J. Sci. Stat. Comput. 1983, 3, 553–572. [Google Scholar] [CrossRef]
- Coleman, T.F.; Li, Y. An Interior, Trust Region Approach for Nonlinear Minimization Subject to Bounds. SIAM J. Optim. 1996, 6, 418–445. [Google Scholar] [CrossRef]
- Gilbert, J.R.; Peierls, T. Sparse Partial Pivoting in Time Proportional to Arithmetic Operations. SIAM J. Sci. Stat. Comput. 1988, 9, 862–874. [Google Scholar] [CrossRef]
- Coleman, T.F.; Verma, A. A Preconditioned Conjugate Gradient Approach to Linear Equality Constrained Minimization. Comput. Optim. Appl. 2001, 20, 61–72. [Google Scholar] [CrossRef]
- Huang, Y.; Chen, J.; Ma, Q. Geometrically Nonlinear Static Analysis of High-Aspect-Ratio Truss-Braced Wing. Eng. Proc. 2024, 80, 18. [Google Scholar]
- Wu, X.; Zhou, Z.; Wang, Z. Effects of Propeller Distribution on the Aeroelastic Characteristics of Large-Deformation Wings. Aerospace 2024, 11, 971. [Google Scholar] [CrossRef]
- dell’Isola, F.; Giorgio, I.; Pawlikowski, M.; Rizzi, N. Large Deformations of Planar Extensible Beams and Pantographic Lattices: Heuristic Homogenization, Experimental and Numerical Examples of Equilibrium. Proc. R. Soc.—A 2016, 472, 20150790. [Google Scholar] [CrossRef]
- Pan, W.; Wang, C.; Zang, H. Hencky Bar-Chain Model for Buckling Analysis of Non-Symmetric Portal Frames. Eng. Struct. 2019, 182, 391–402. [Google Scholar] [CrossRef]
- Salinic, S. An Improved Variant of Hencky Bar-Chain Model for Buckling and Bending Vibration of Beams with End Masses and Springs. Mech. Syst. Signal Process. 2017, 90, 30–43. [Google Scholar] [CrossRef]
- Giorgio, I. A Discrete Formulation of Kirchhoff Rods in Large-Motion Dynamics. Math. Mech. Solids 2020, 25, 1081–1100. [Google Scholar] [CrossRef]
- Peters, D.; Hsieh, M.; Torrero, A. A State-Space Airloads Theory for Flexible Airfoils. J. Am. Helicopter Soc. 2007, 52, 329–342. [Google Scholar] [CrossRef]
- Berci, M.; Gaskell, P.; Hewson, R.; Toropov, V. A Semi-Analytical Model for the Combined Aeroelastic Behaviour and Gust Response of a Flexible Aerofoil. J. Fluids Struct. 2013, 38, 3–21. [Google Scholar] [CrossRef]
- Meirovitch, L. Analytical Methods in Vibrations; Macmillan: New York, NY, USA, 1967. [Google Scholar]
- Savu, D.C.; Higgins, A.J. Structural Stability of a Lightsail for Laser-Driven Interstellar Flight. Acta Astronaut. 2022, 201, 376–393. [Google Scholar]
- Harder, R.L.; Desmarais, R.N. Interpolation Using Surface Splines. J. Aircr. 1972, 9, 189–191. [Google Scholar]
- del Carre, A.; Munoz-Simon, A.; Goizueta, N.; Palacios, R. SHARPy: A Dynamic Aeroelastic Simulation Toolbox for Very Flexible Aircraft and Wind Turbines. J. Open Source Softw. 2019, 4, 1885. [Google Scholar]
- Biot, M.A.; Arnold, L. Low-Speed Flutter and its Physical Interpretation. J. Aeronaut. Sci. 1948, 15, 232–236. [Google Scholar]
- Karamcheti, K. Principles of Ideal-Fluid Aerodynamics; Wiley: Hoboken, NY, USA, 1967. [Google Scholar]
- Kutta, W.M. Auftriebskräfte in Strömenden Flüssigkeiten. Illus. Aeronaut. Mitteilungen 1902, 6, 133–135. [Google Scholar]
- Joukowski, N.E. Sur les Tourbillons Adjionts. Traraux Sect. Phys. Soc. Imp. Amis Sci. Nat. 1906, 13, 261–284. [Google Scholar]
- DeYoung, J. Theoretical Additional Span Loading Characteristics of Wings with Arbitrary Sweep, Aspect Ratio, and Taper Ratio; NACA TN 1491; NACA: Boston, MA, USA, 1947. [Google Scholar]
- Jones, R.T. Correction of the Lifting-Line Theory for the Effect of the Chord; NACA-TN-817; NACA: Boston, MA, USA, 1941. [Google Scholar]
- Helmbold, H.B. Der Unverwundene Ellipsenflügel als Tragende Fläche. Jahrb. Der Dtsch. Luftfahrtforsch. 1942, I, 111–113. [Google Scholar]
- Diederich, F.W. A Plan-Form Parameter for Correlating Certain Aerodynamic Characteristics of Swept Wings; NACA TN 2335; NACA: Boston, MA, USA, 1951. [Google Scholar]
- Diederich, F.W. Approximate Aerodynamic Influence Coefficients for Wings of Arbitrary Plan Form in Subsonic Flow; NACA TN 2092; NACA: Boston, MA, USA, 1950. [Google Scholar]
- Diederich, F.W.; Zlotnick, M. Calculated Spanwise Lift Distributions, Influence Functions and Influence Coefficients for Unswept Wings in Subsonic Flow; NACA 1228; NACA: Boston, MA, USA, 1955. [Google Scholar]
- Multhopp, H. Methods for Calculating the Lift Distribution of Wings (Subsonic Lifting-Surface Theory); ARC R&M 2884; ARC: London, UK, 1955. [Google Scholar]
- Simpson, R.W. An Extension of Multhopp’s Lifting Surface Theory; CoA Report 132; Cranfield University: Cranfield, UK, 1960. [Google Scholar]
- Hildebrand, F.B. A Least-Squares Procedure for the Solution of the Lifting-Line Integral Equation; NACA TN 925; NACA: Boston, MA, USA, 1944. [Google Scholar]
- Lee, K. Large Defections of Cantilever Beams of Non-Linear Elastic Material under a Combined Loading. Int. J. Non-Linear Mech. 2002, 37, 439–443. [Google Scholar]

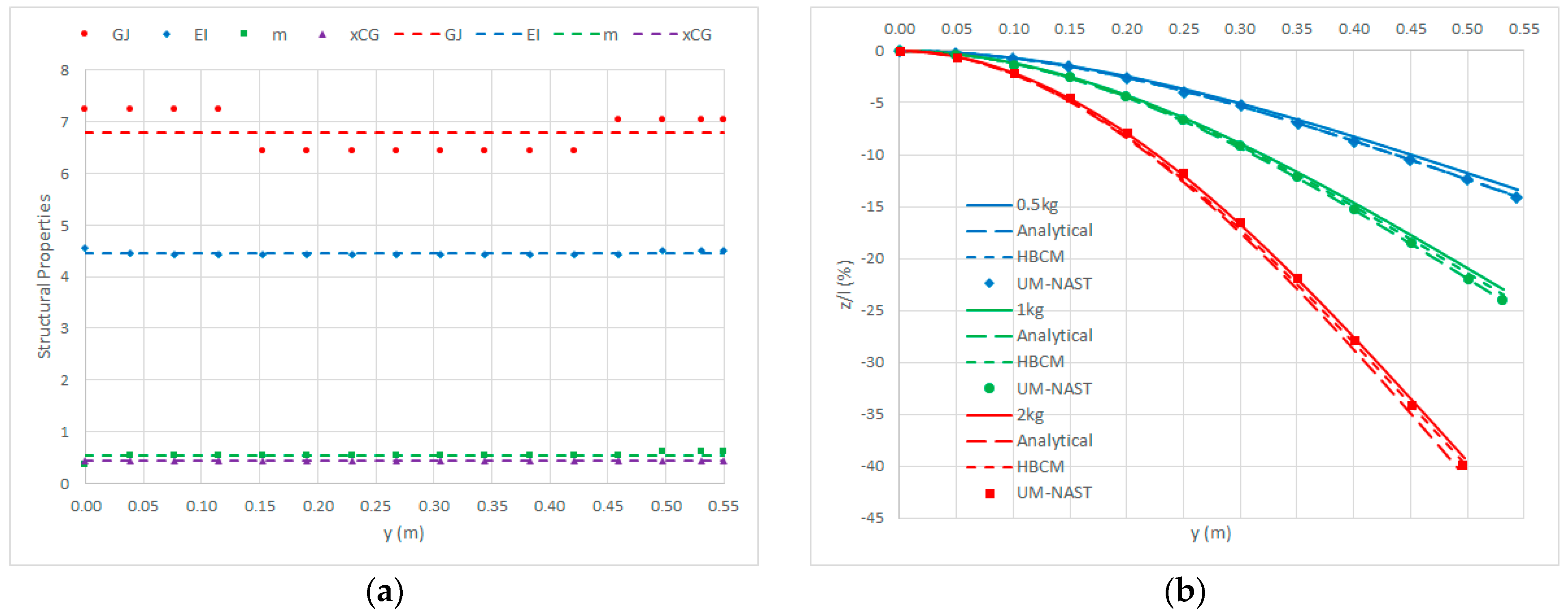
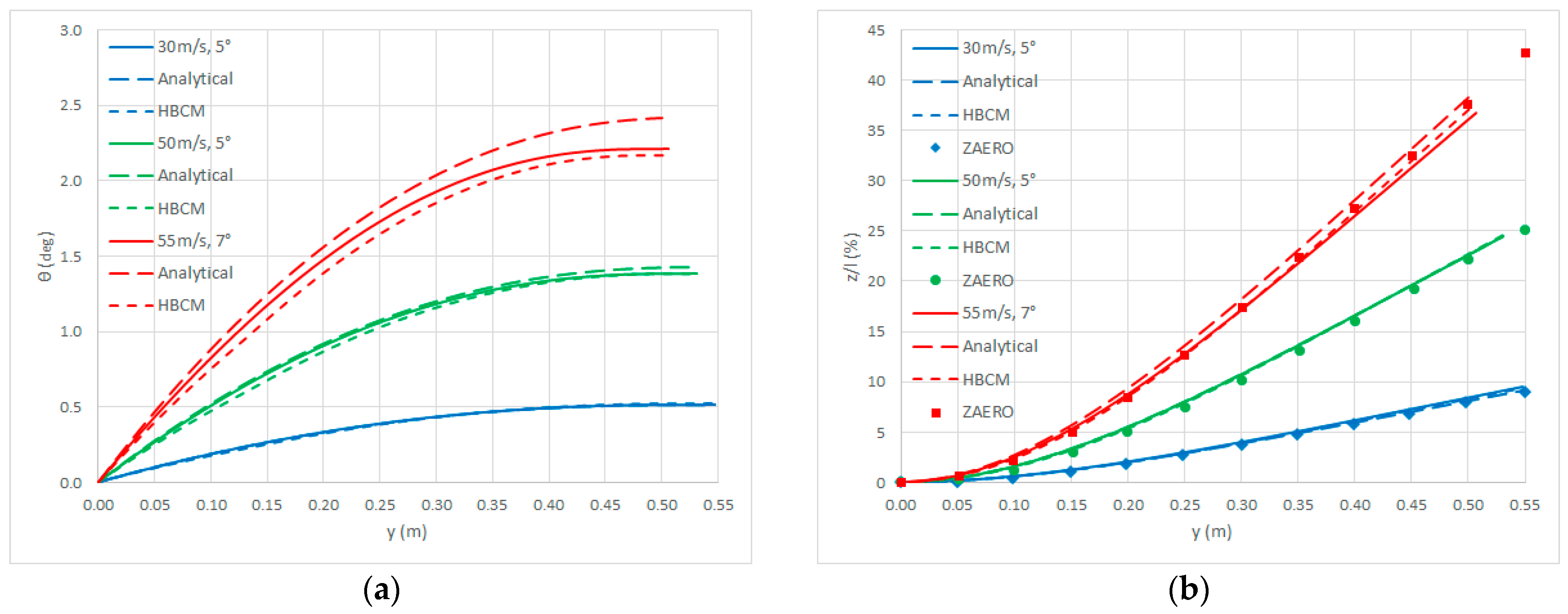
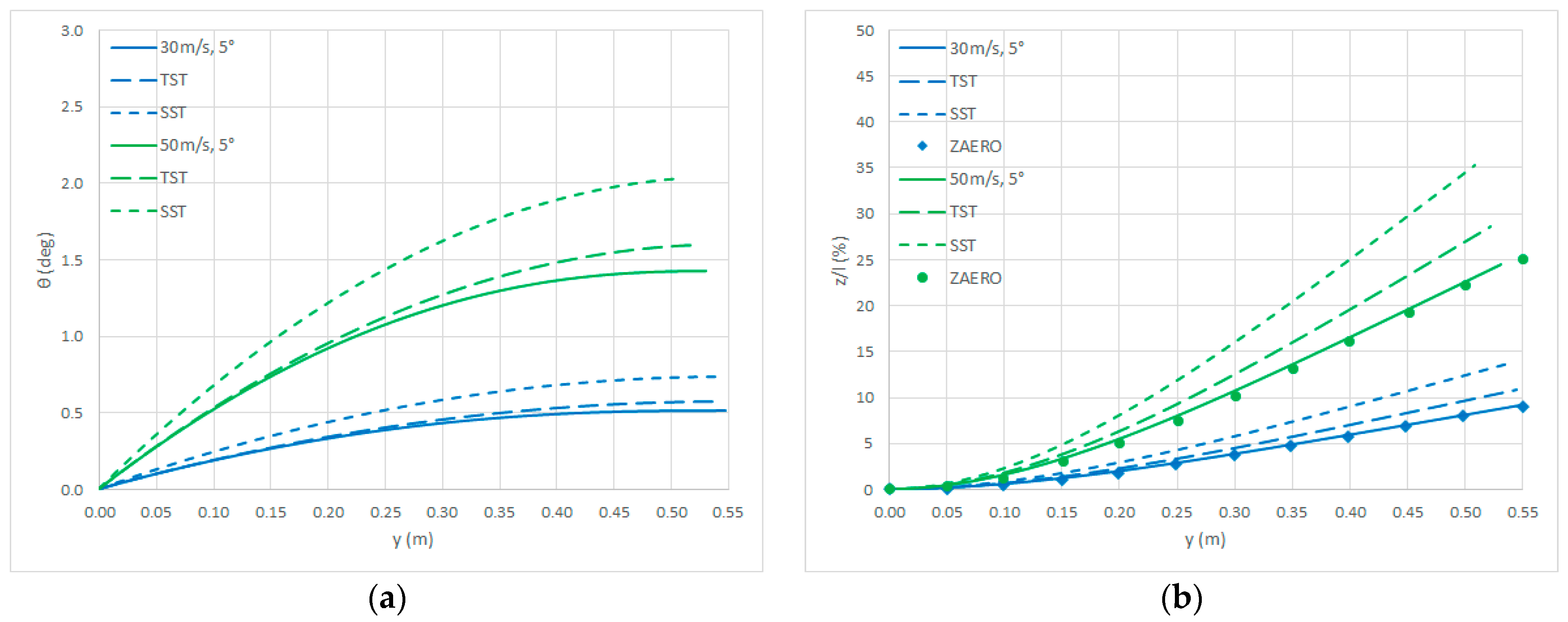
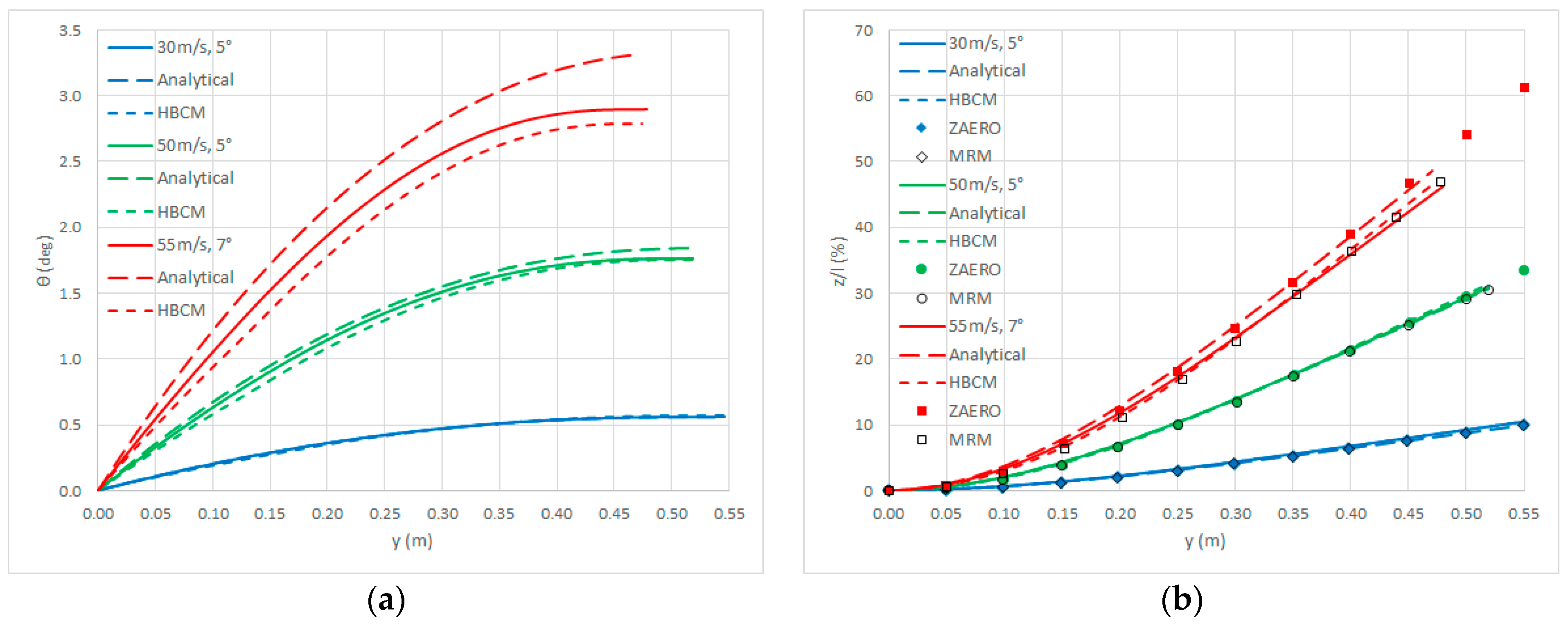
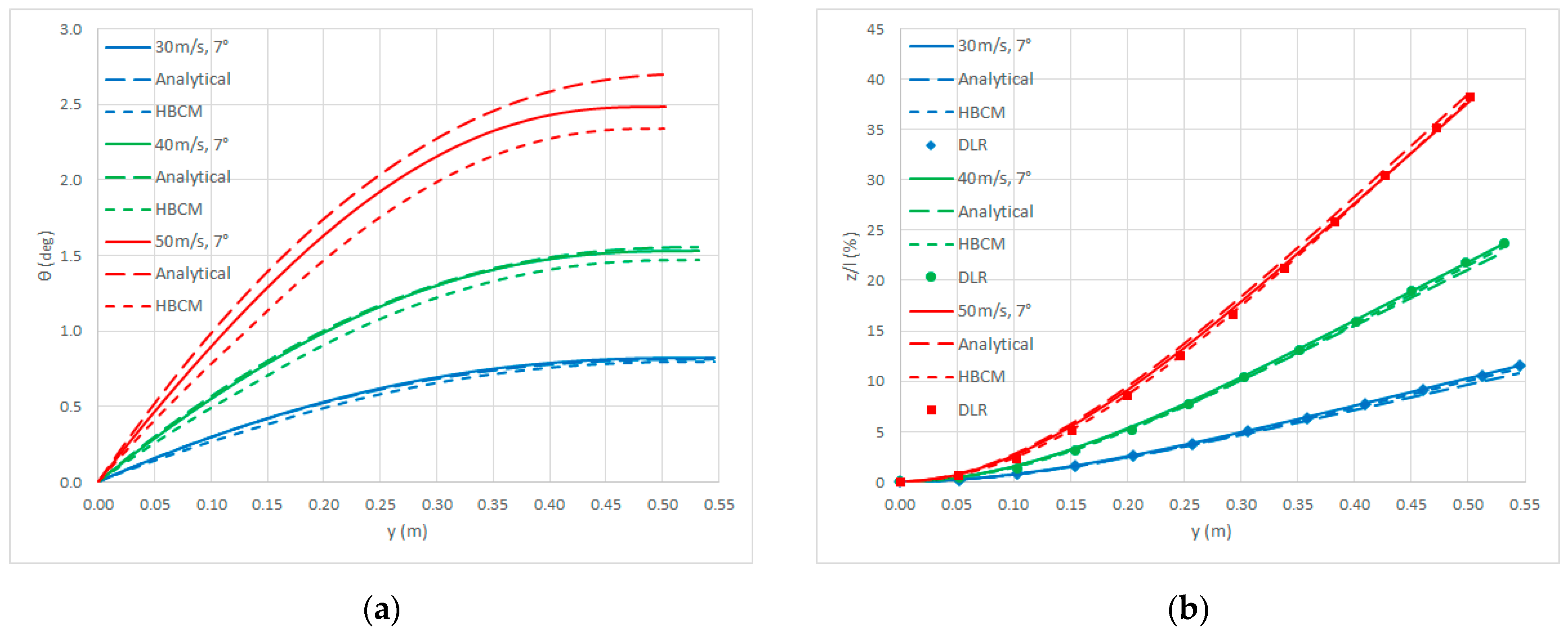
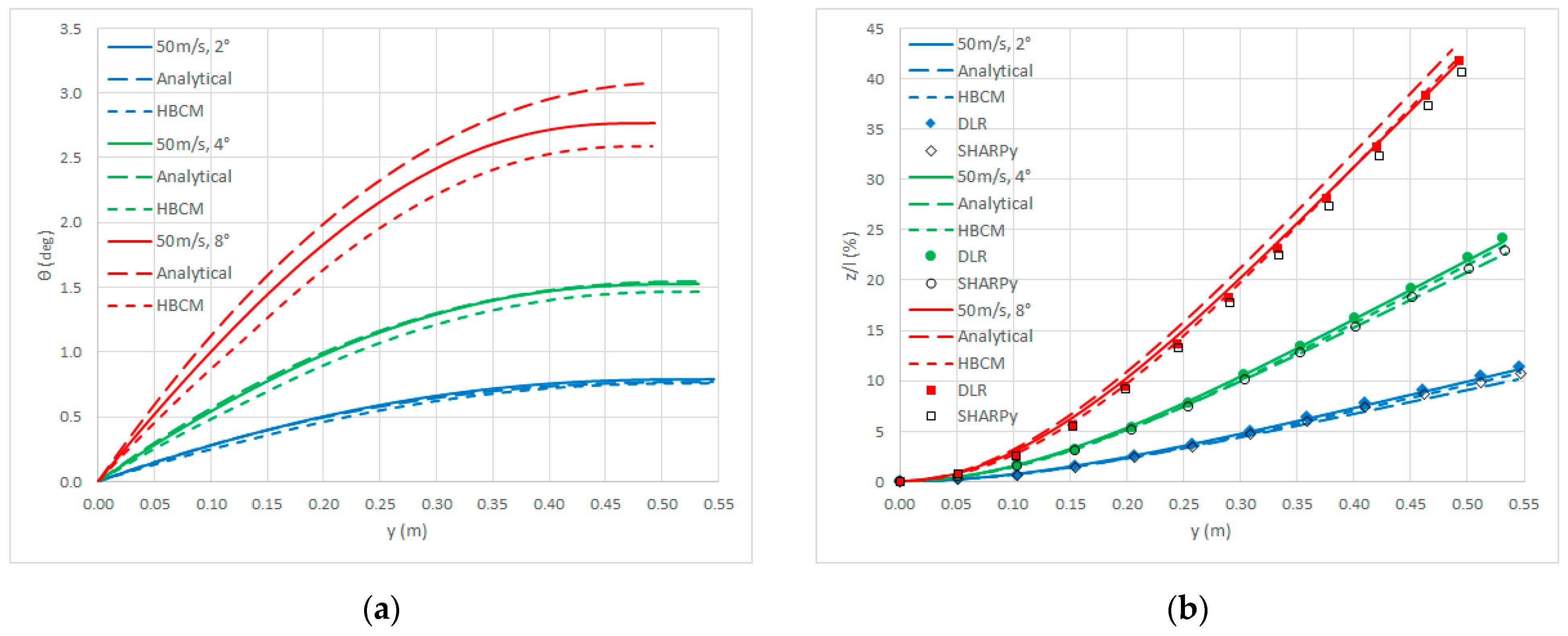
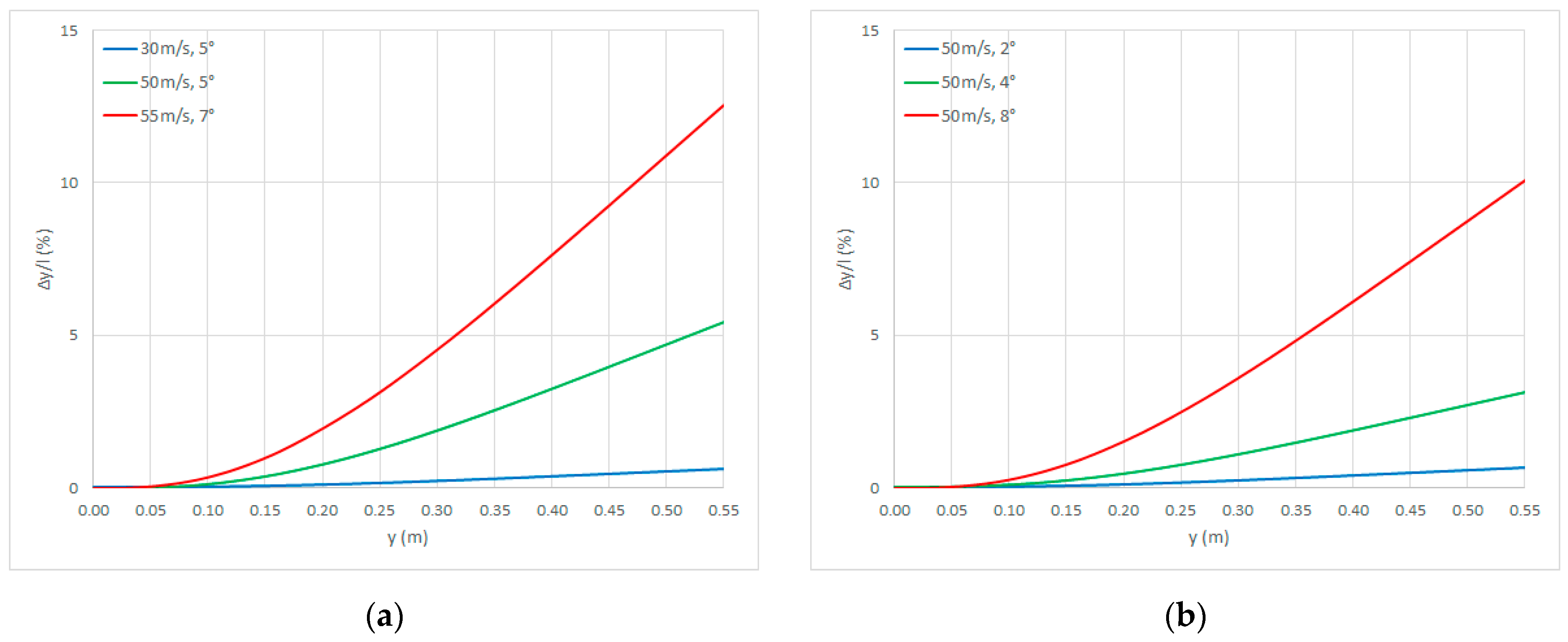
| m [kg/m] | P [kg] | EI [N∙m2] | GJ [N∙m2] | ωϚ [Hz] | ωθ [Hz] | c [m] | l [m] | σ [-] | ε [-] | κTST [-] |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.545 | 0.029 | 4.45 | 6.80 | 4.33 | 41.5 | 0.10 | 0.55 | 0.891 | 8.183 | 0.782 |
| Model | Type | Load | MST | Kinematics | Equations | Solution |
|---|---|---|---|---|---|---|
| Quasi-linear | Continuous | Linear | WLM | Nonlinear | Differential | Semi-Analytical |
| Nonlinear | Continuous | Nonlinear | VLM | Nonlinear | Differential | Numerical |
| HBCM | Discrete | Nonlinear | WLM | Nonlinear | Algebraic | Numerical |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berci, M. Non-Linear and Quasi-Linear Models for the Large-Amplitude Static Aeroelastic Response of Very-Flexible Slender Wings in Subsonic Flow at Low Speed. Aerospace 2025, 12, 294. https://doi.org/10.3390/aerospace12040294
Berci M. Non-Linear and Quasi-Linear Models for the Large-Amplitude Static Aeroelastic Response of Very-Flexible Slender Wings in Subsonic Flow at Low Speed. Aerospace. 2025; 12(4):294. https://doi.org/10.3390/aerospace12040294
Chicago/Turabian StyleBerci, Marco. 2025. "Non-Linear and Quasi-Linear Models for the Large-Amplitude Static Aeroelastic Response of Very-Flexible Slender Wings in Subsonic Flow at Low Speed" Aerospace 12, no. 4: 294. https://doi.org/10.3390/aerospace12040294
APA StyleBerci, M. (2025). Non-Linear and Quasi-Linear Models for the Large-Amplitude Static Aeroelastic Response of Very-Flexible Slender Wings in Subsonic Flow at Low Speed. Aerospace, 12(4), 294. https://doi.org/10.3390/aerospace12040294





