Spin Period Evolution of Decommissioned GLONASS Satellites
Abstract
1. Introduction
2. GLONASS Satellites
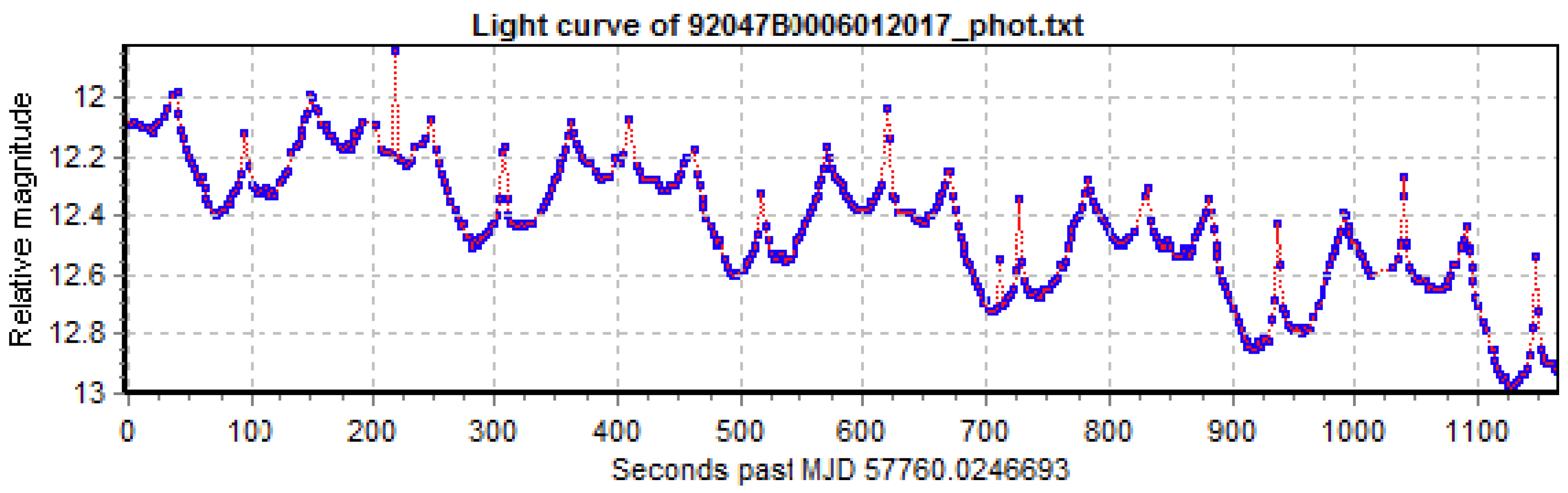
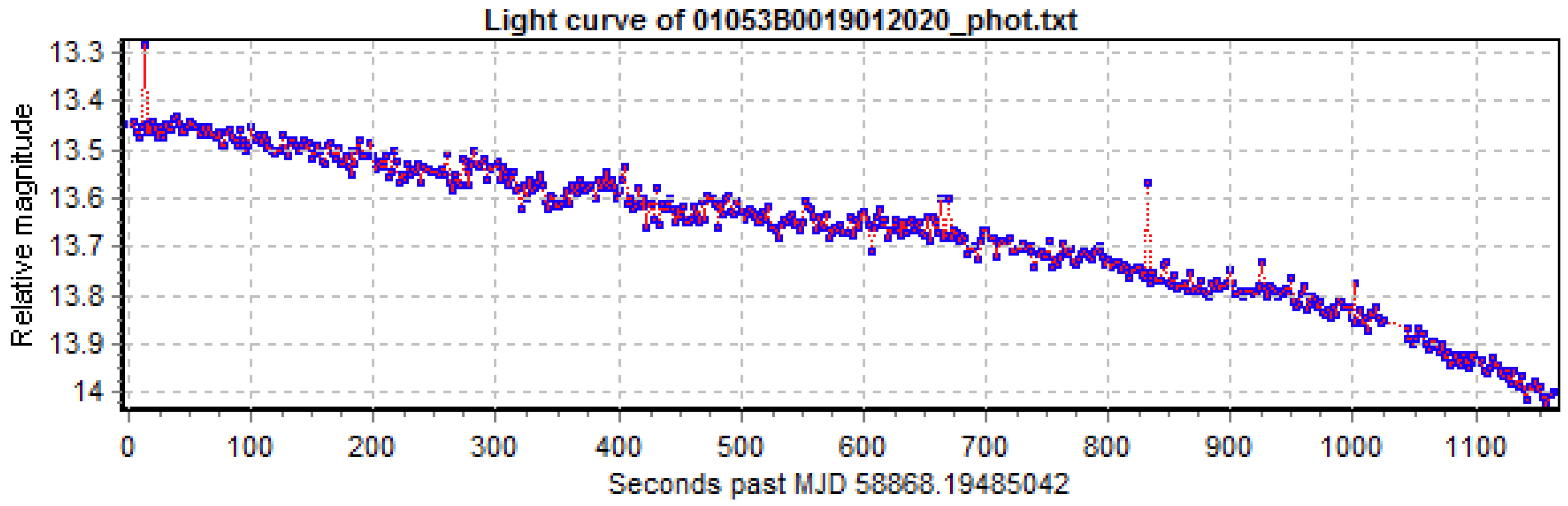
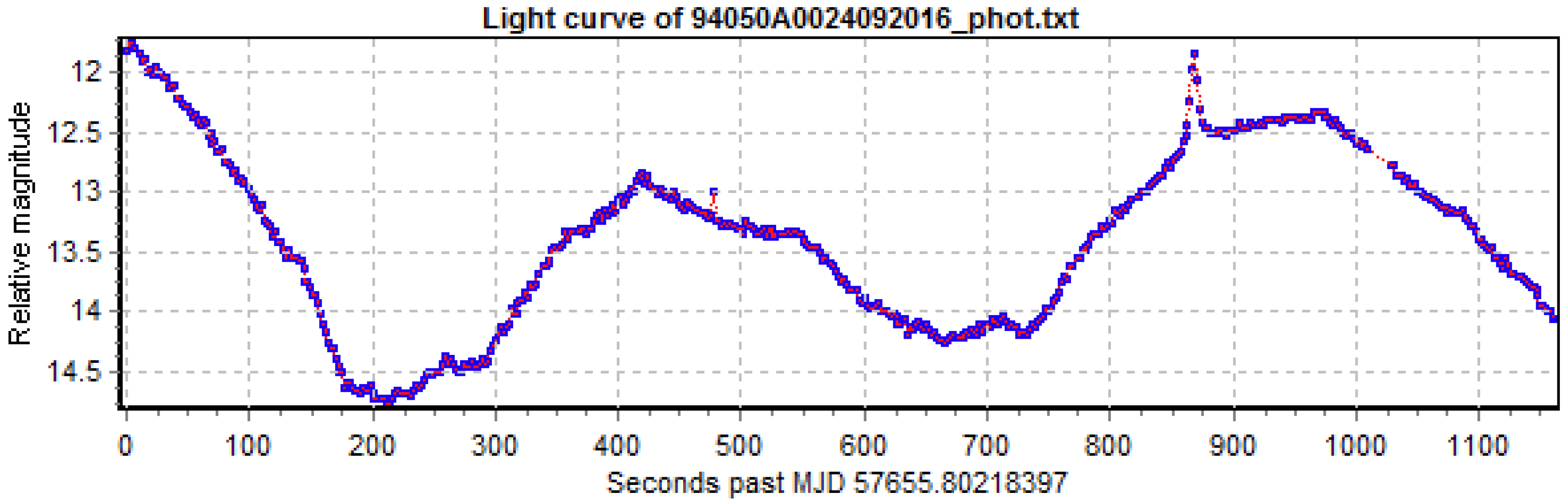
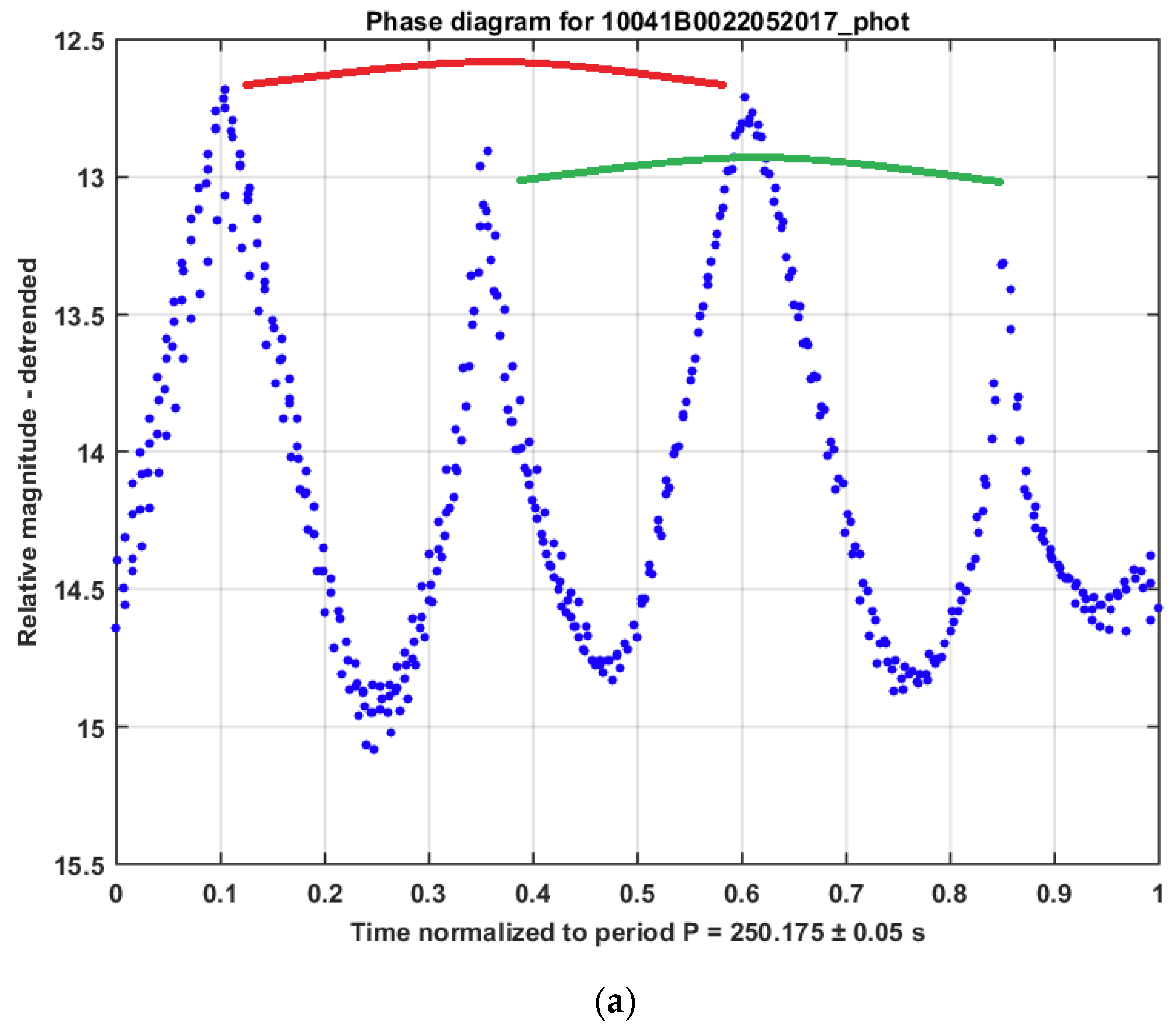
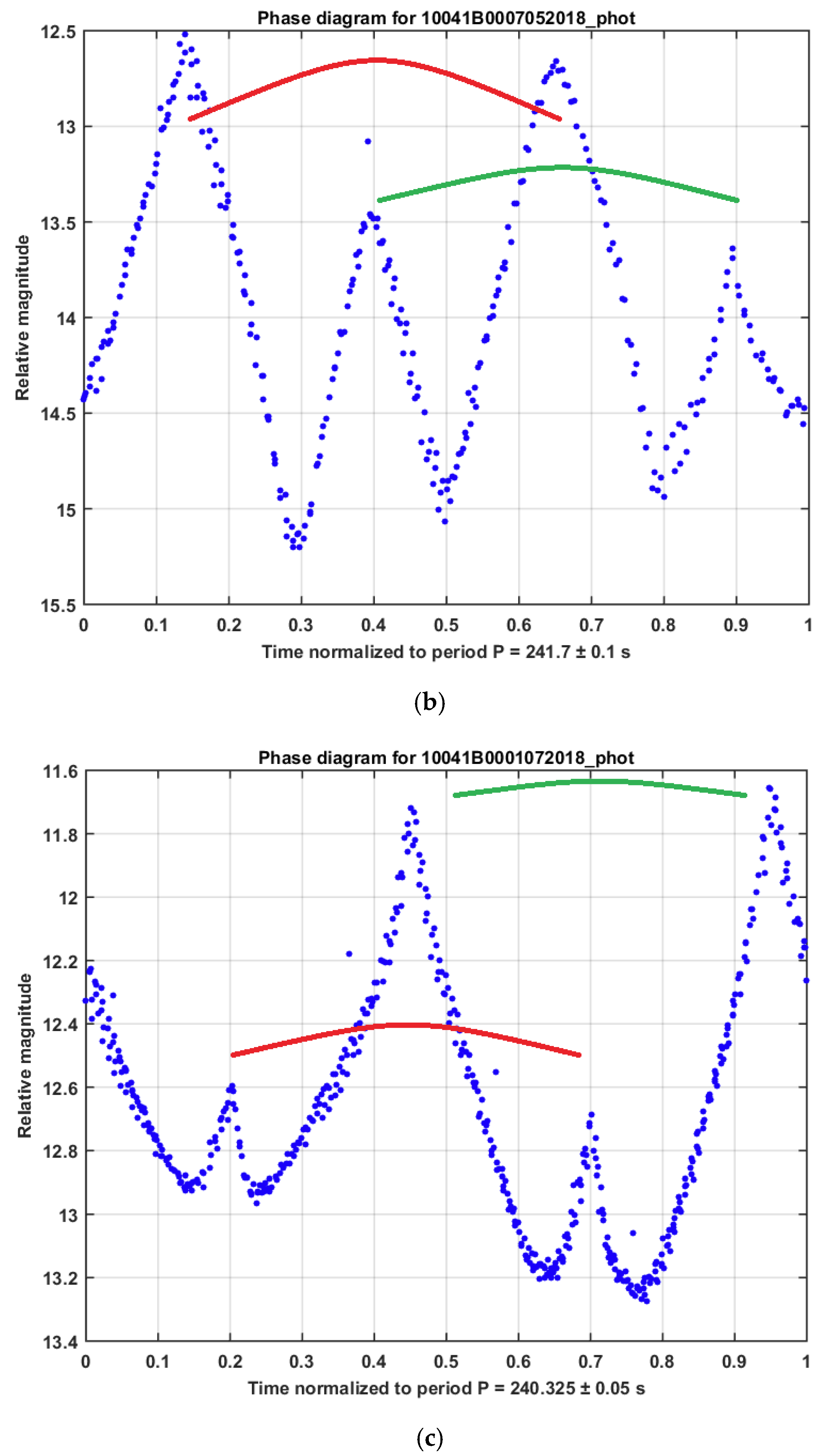
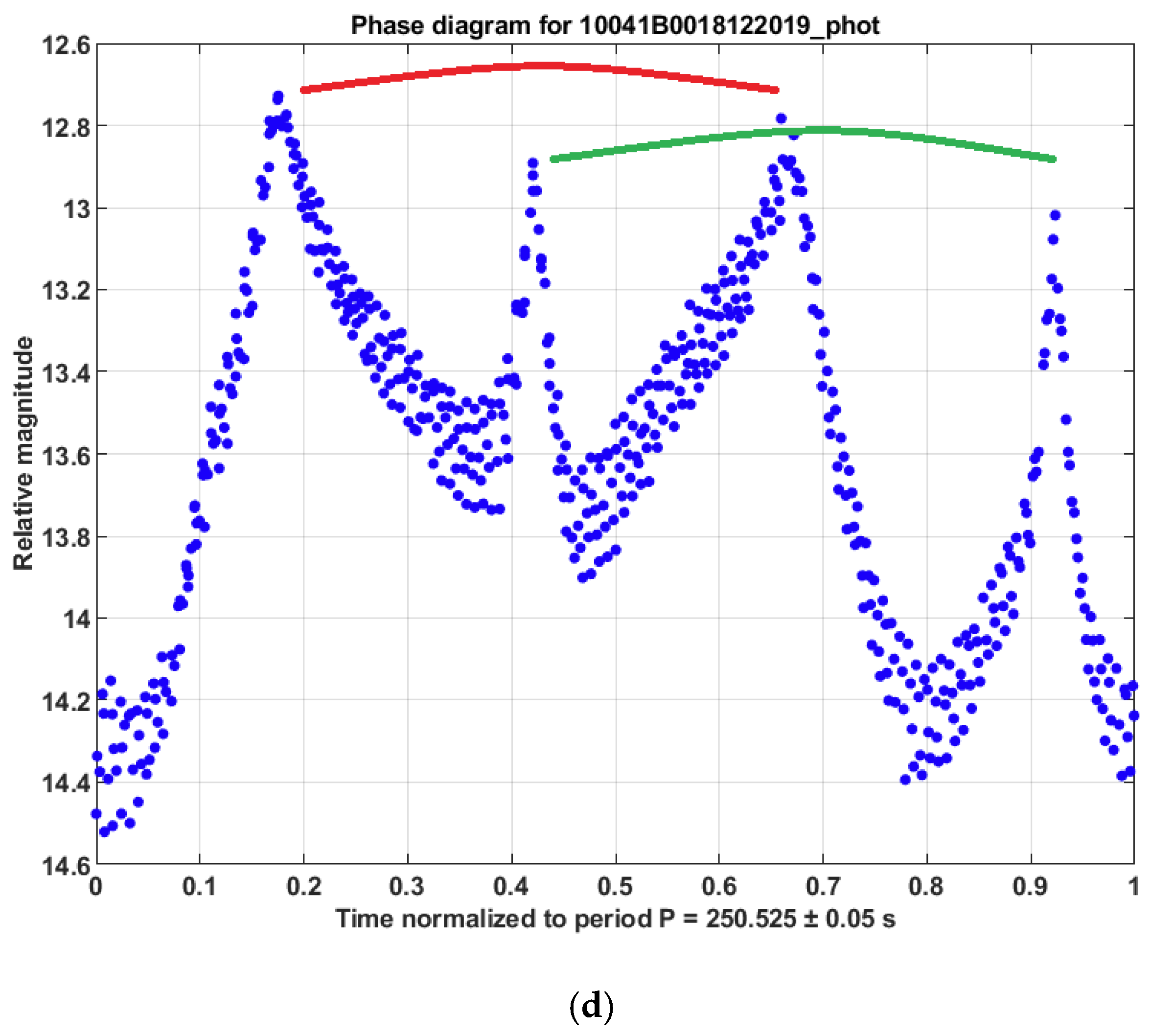
3. Light Curves of GLONASS Satellites
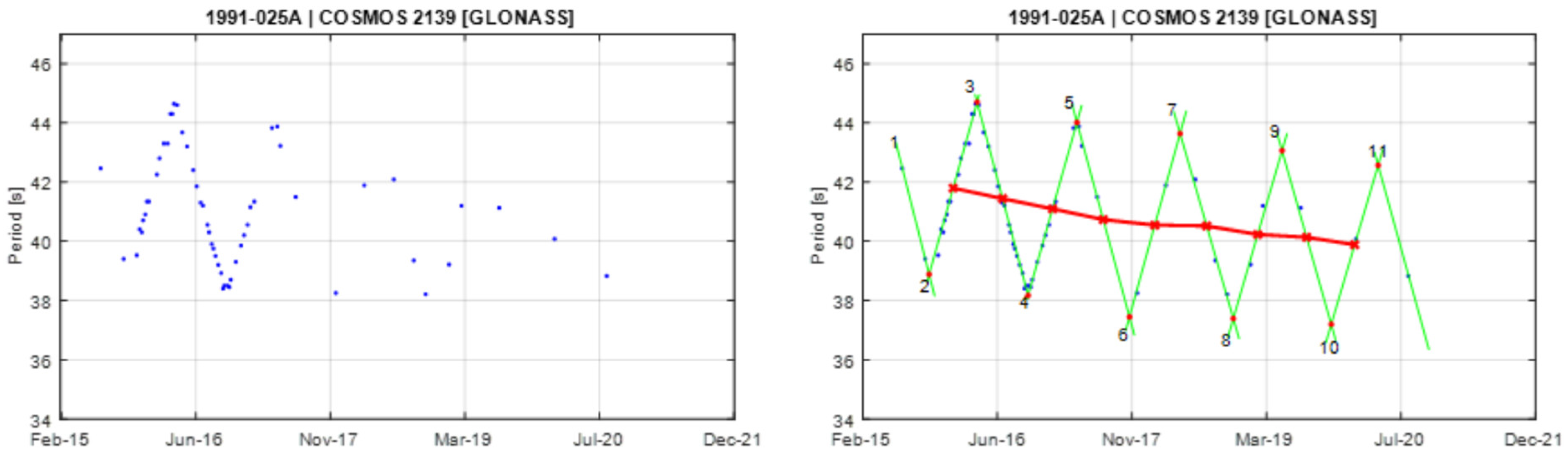
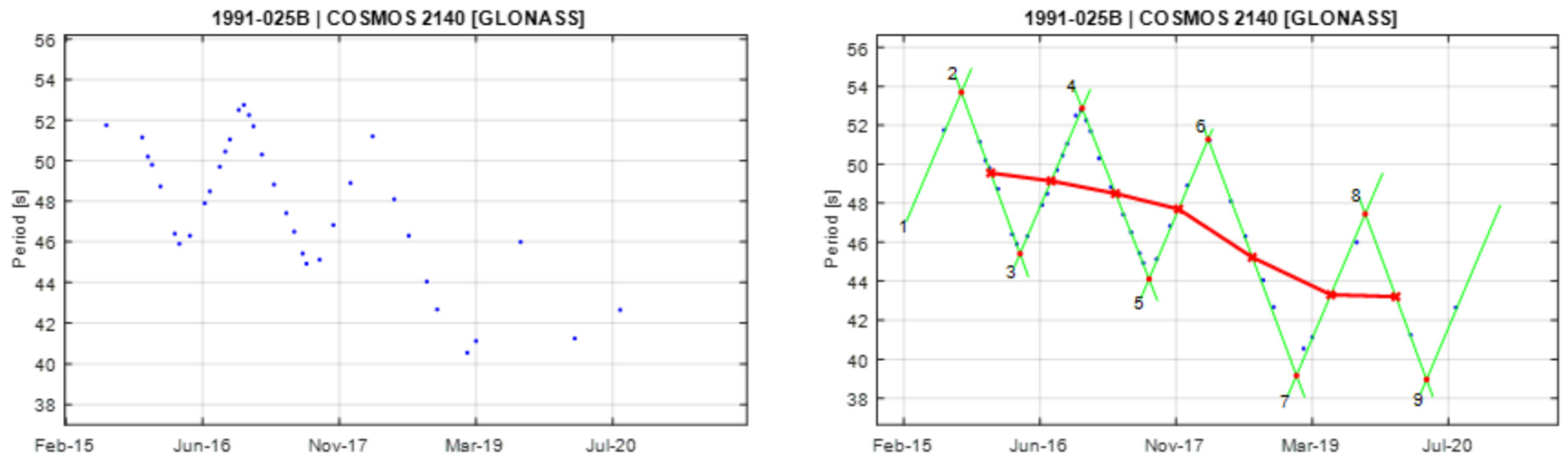
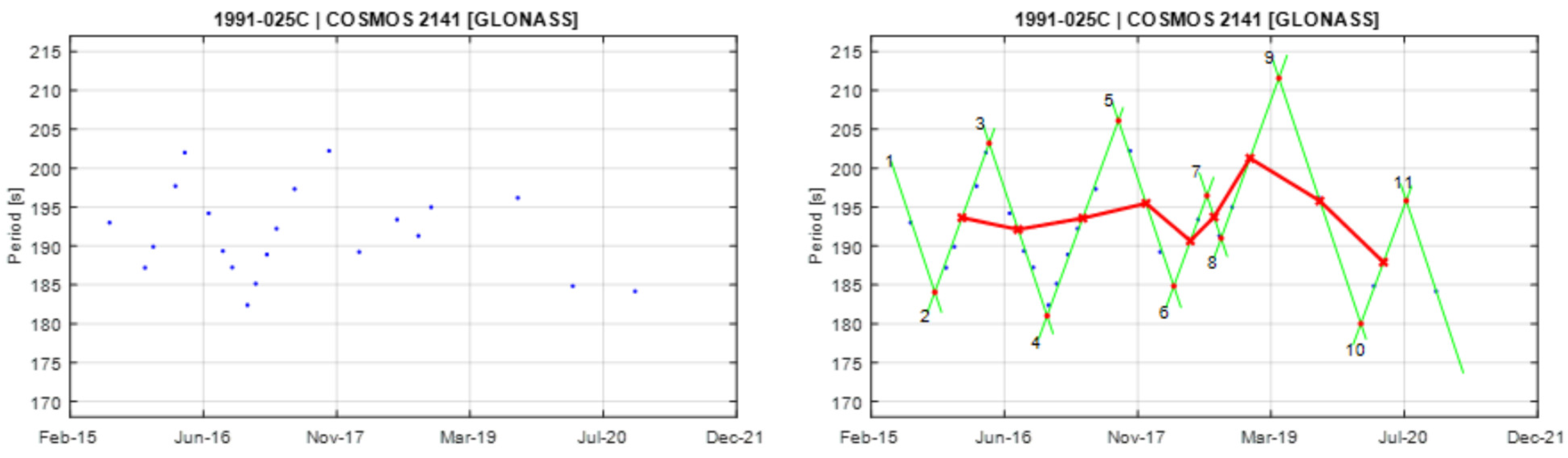
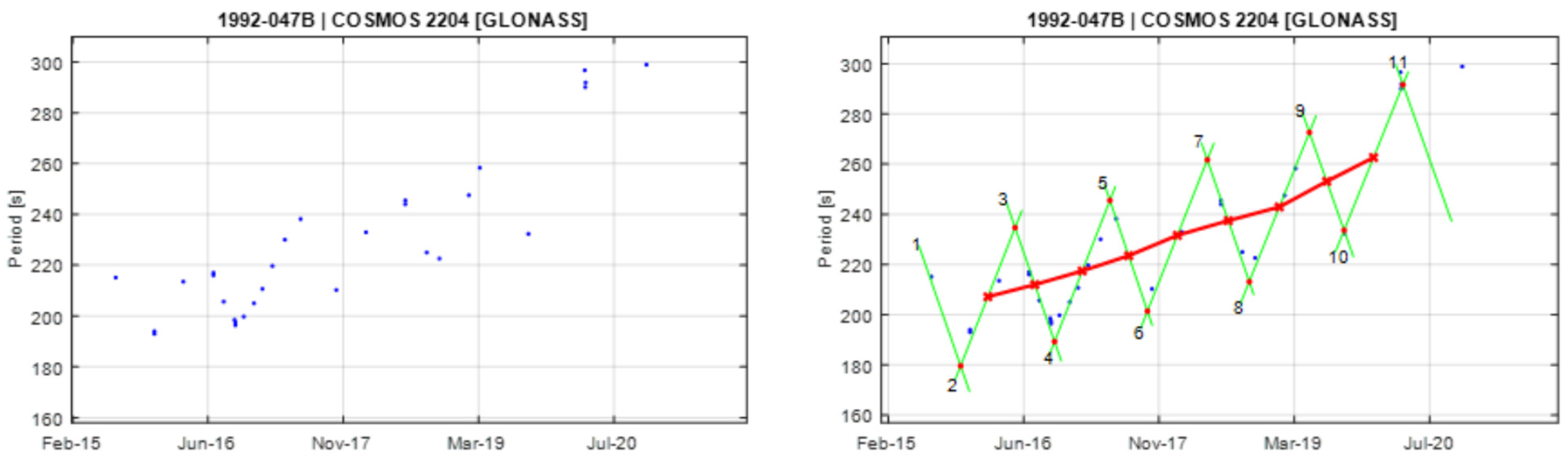
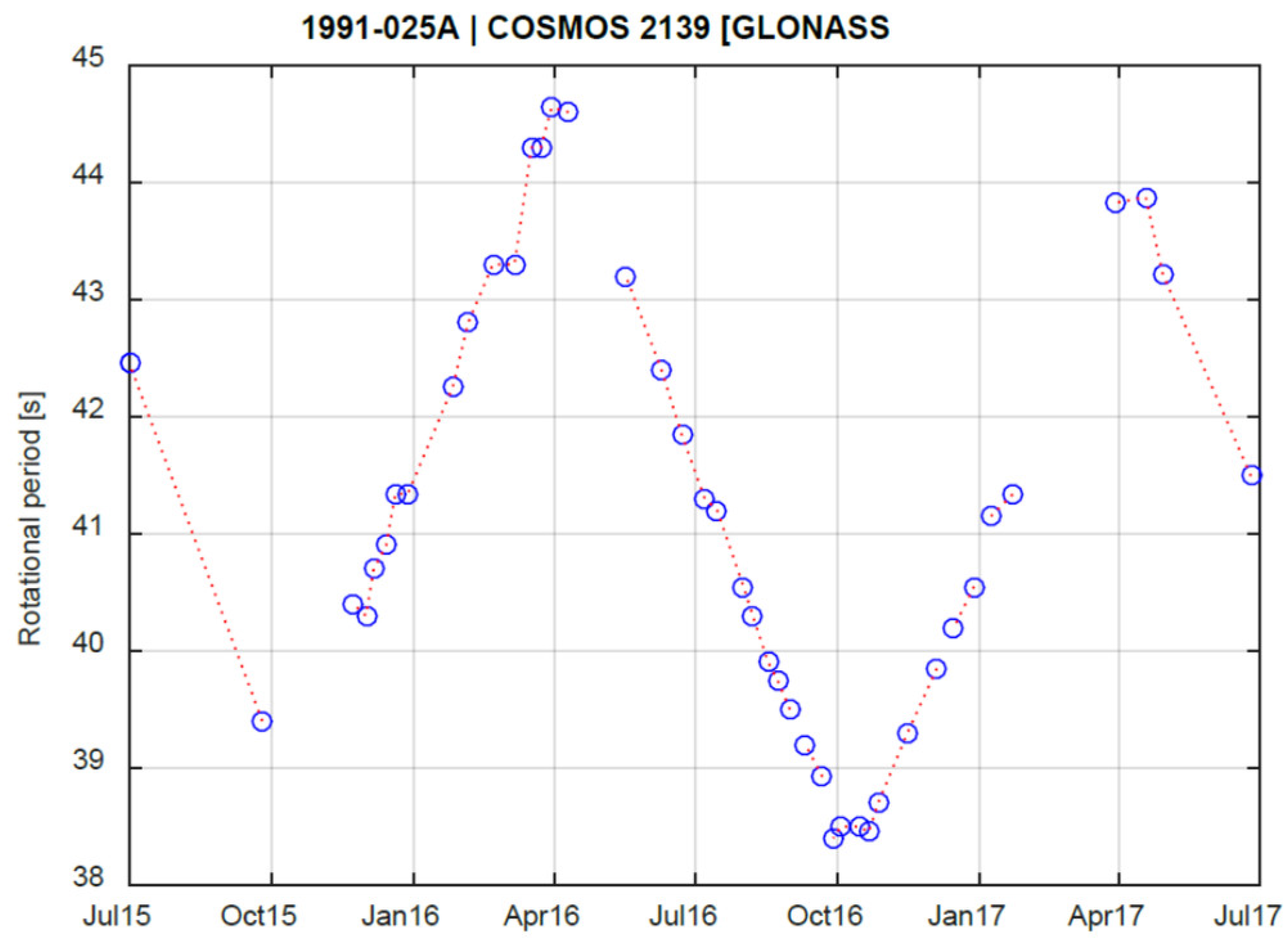
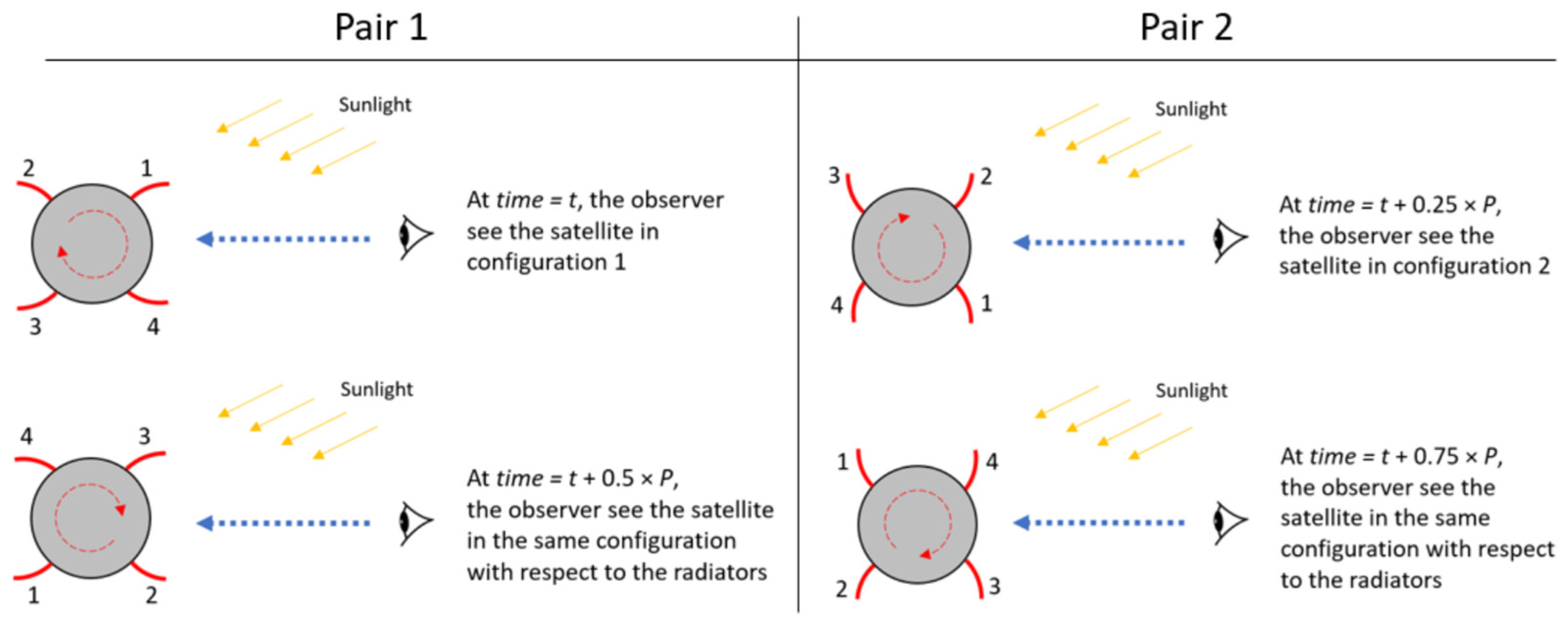
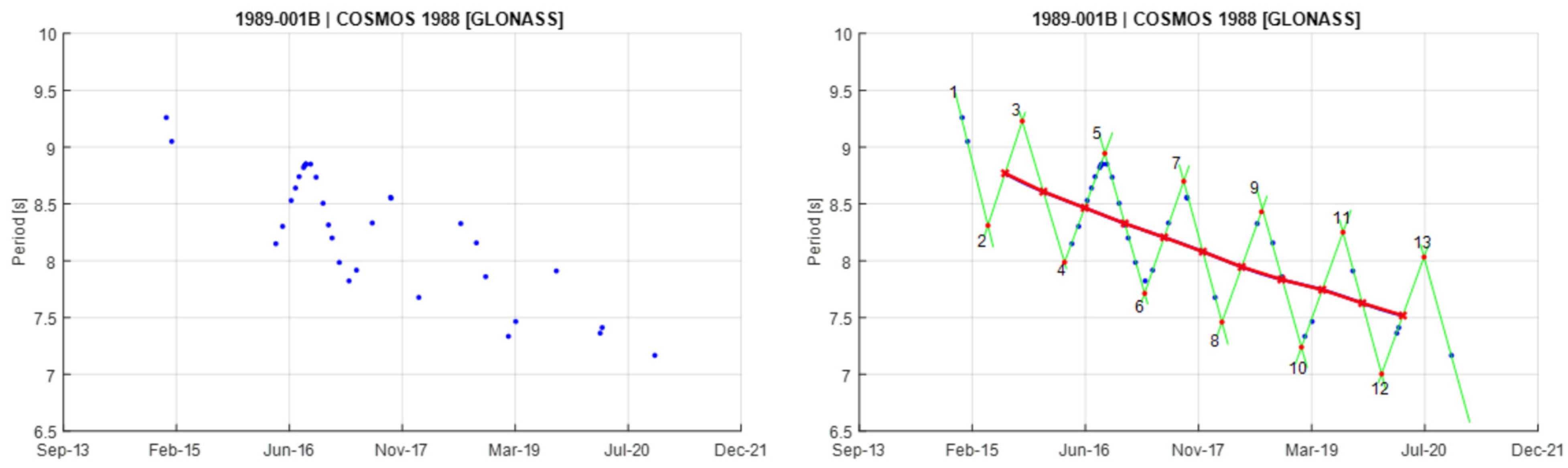
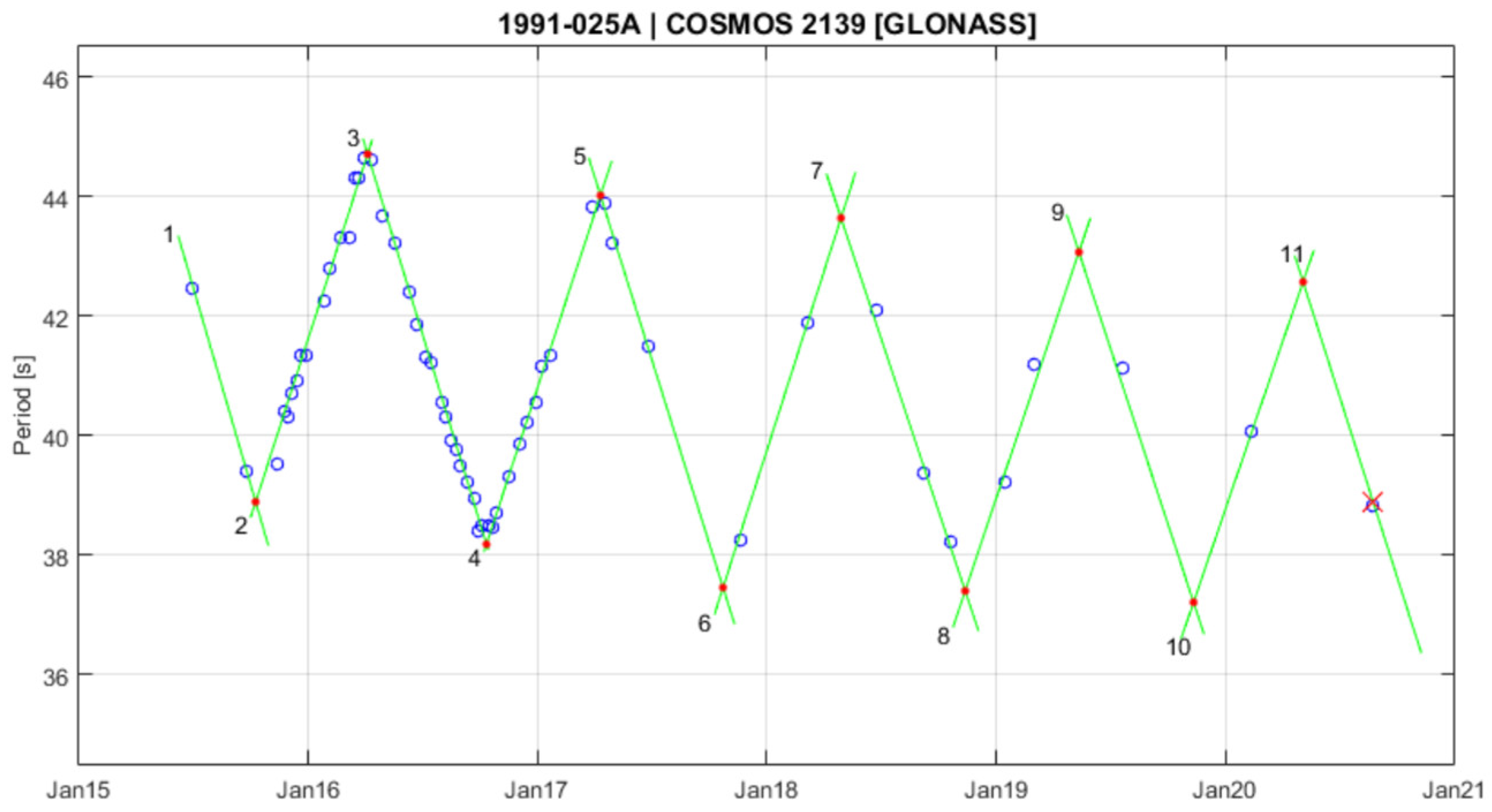
3.1. Cyclic Spin Period
3.2. Cases Without Cyclic Pattern
3.3. Light Curve Characteristic
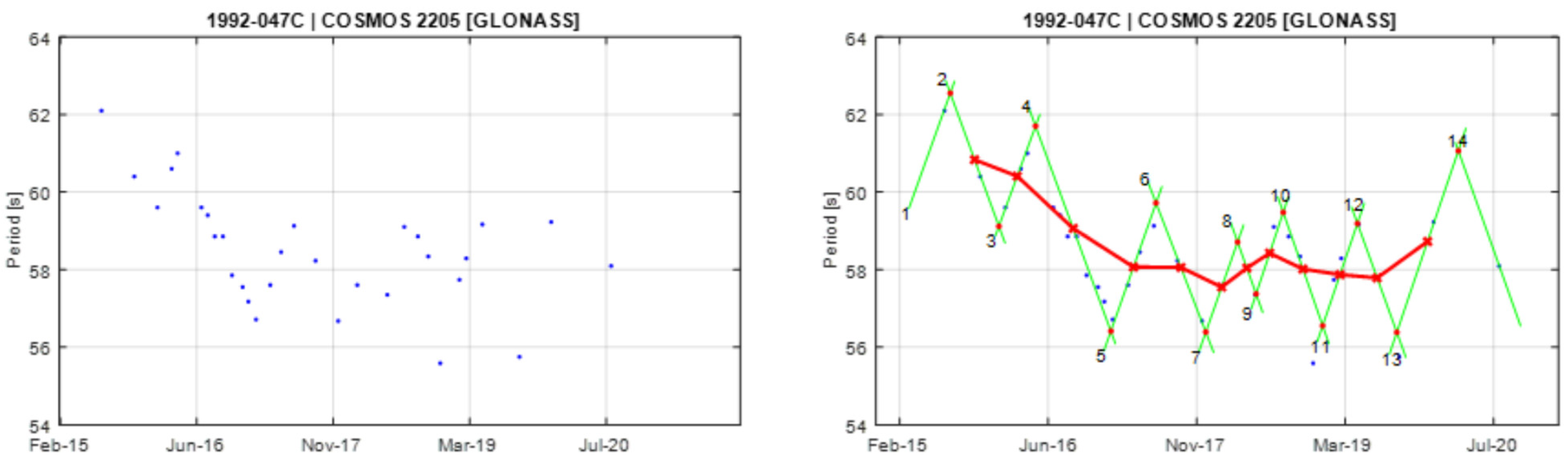
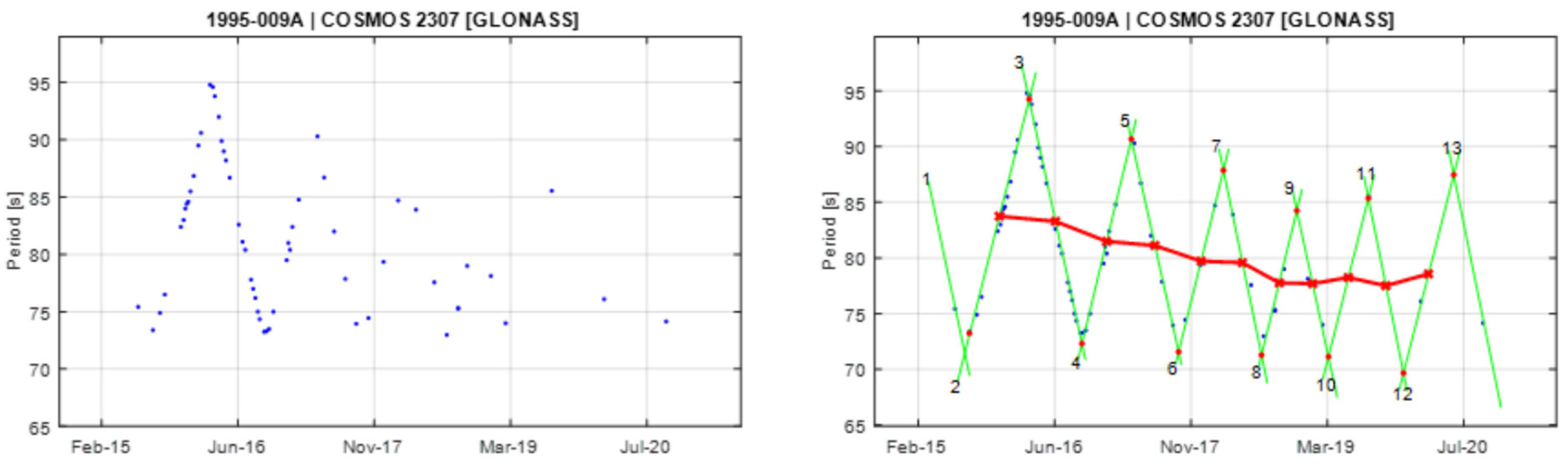
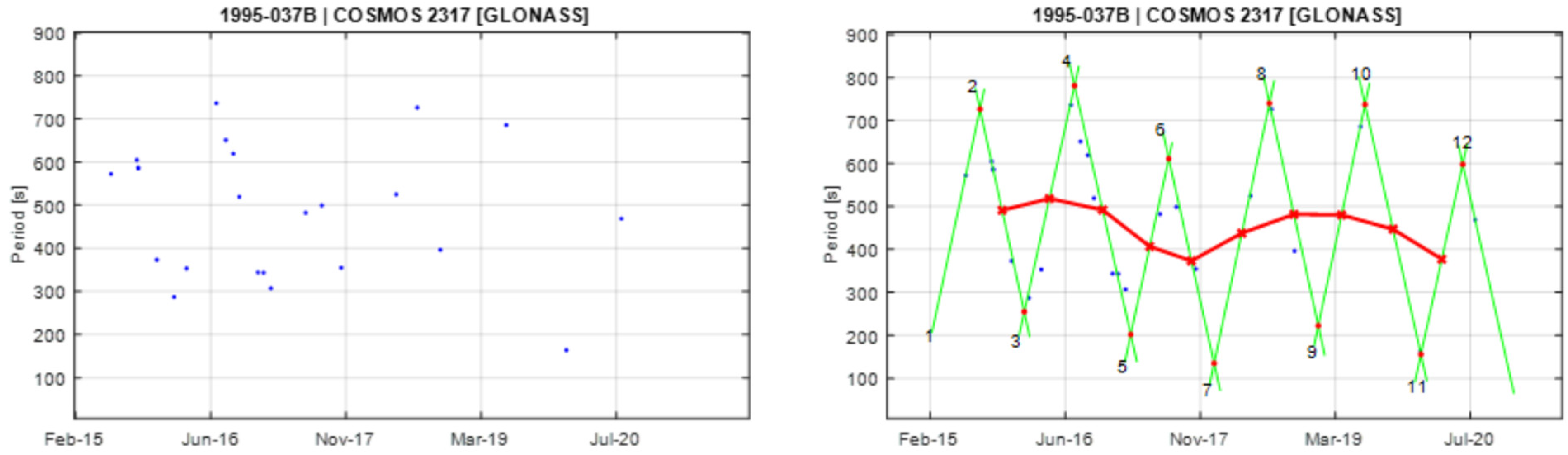
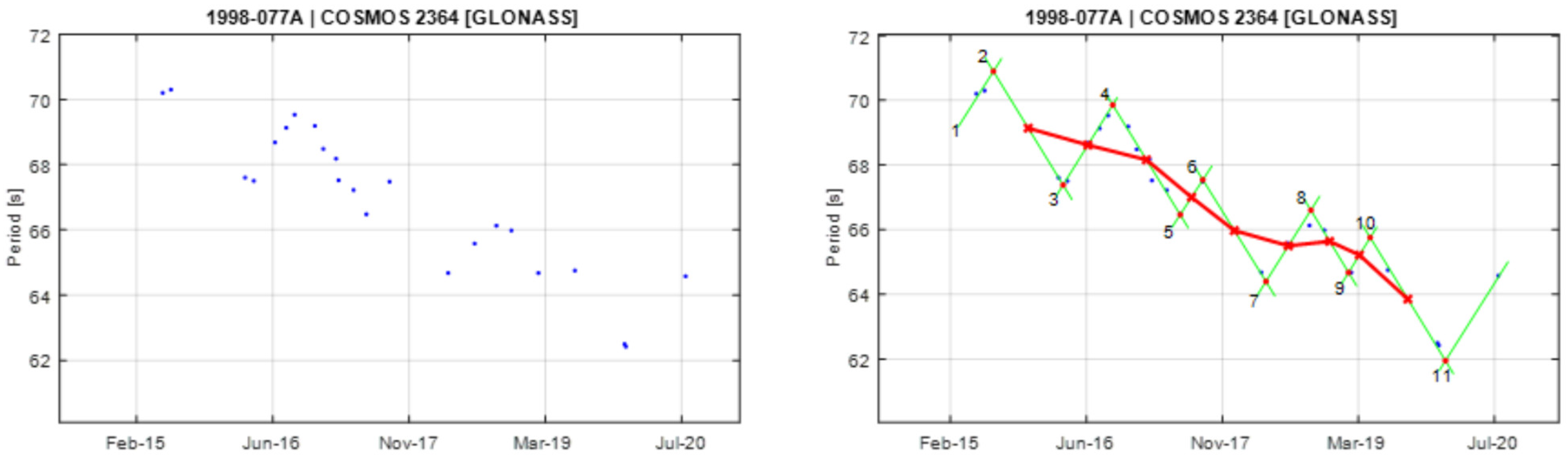
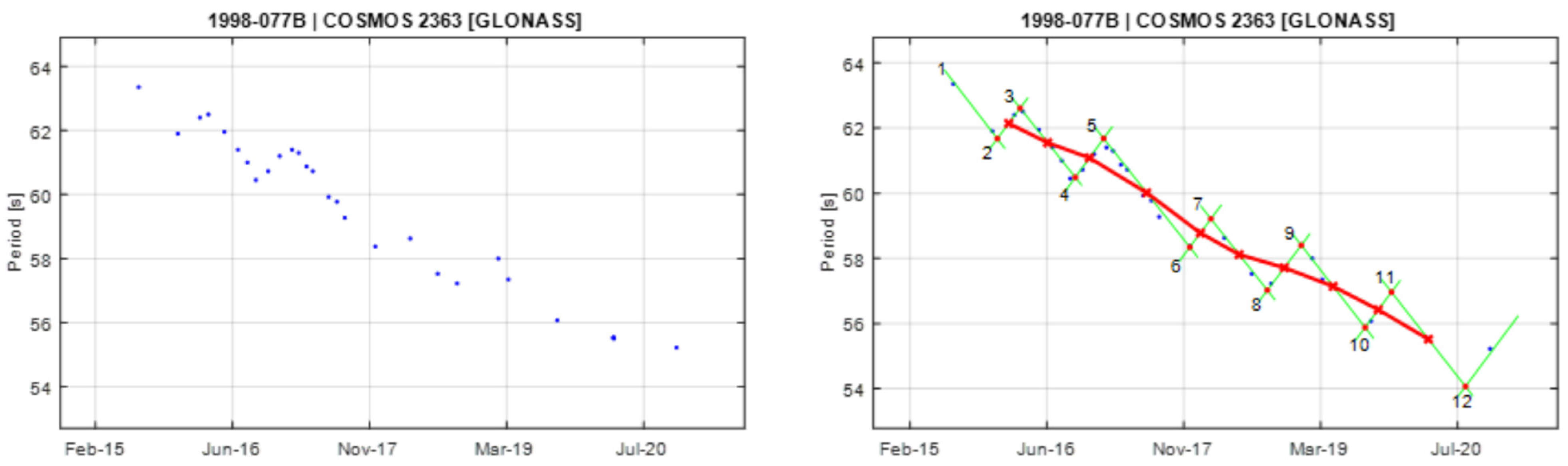
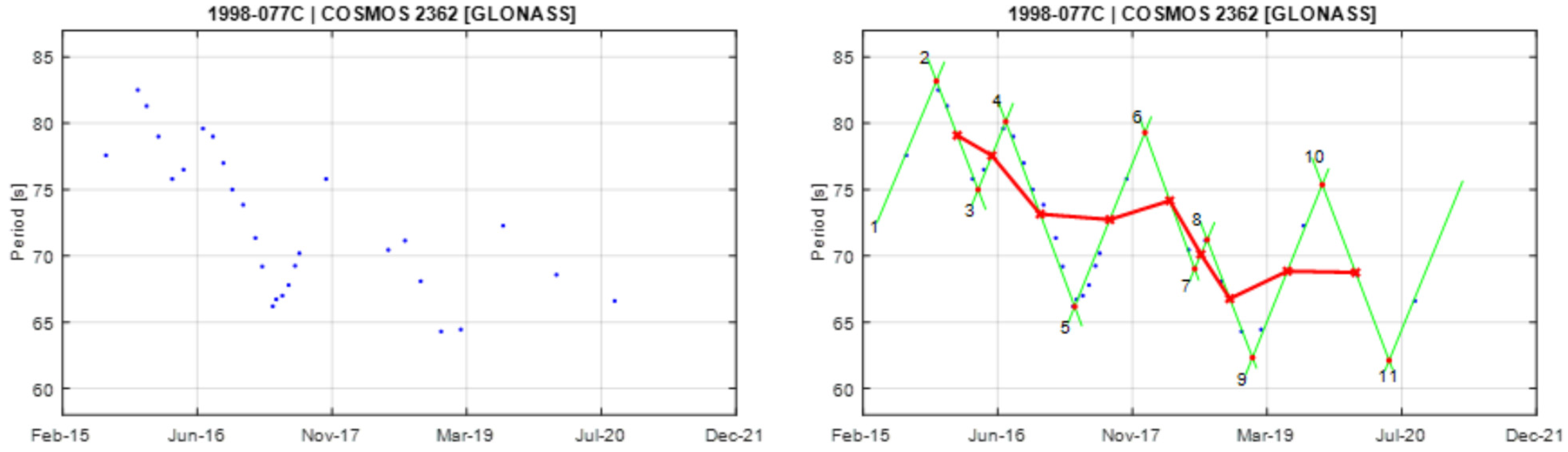
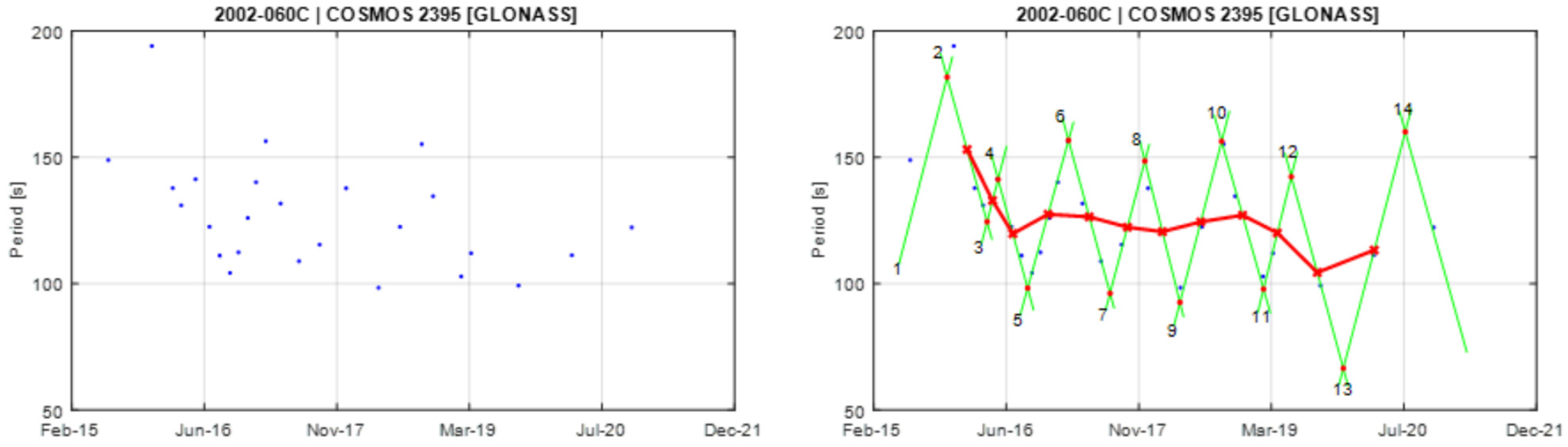
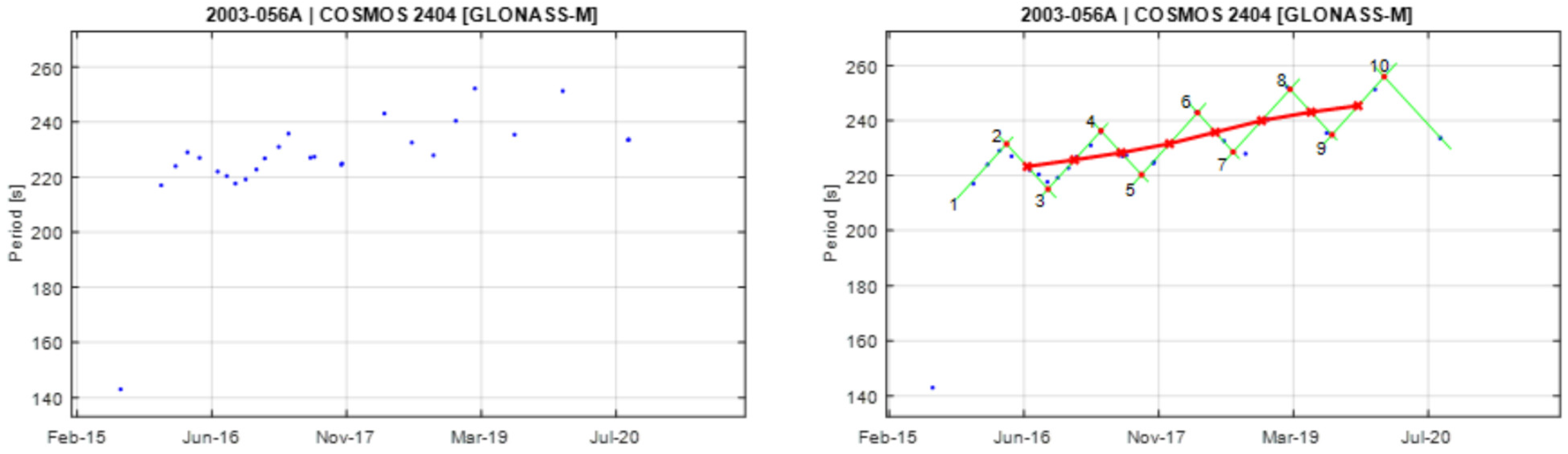
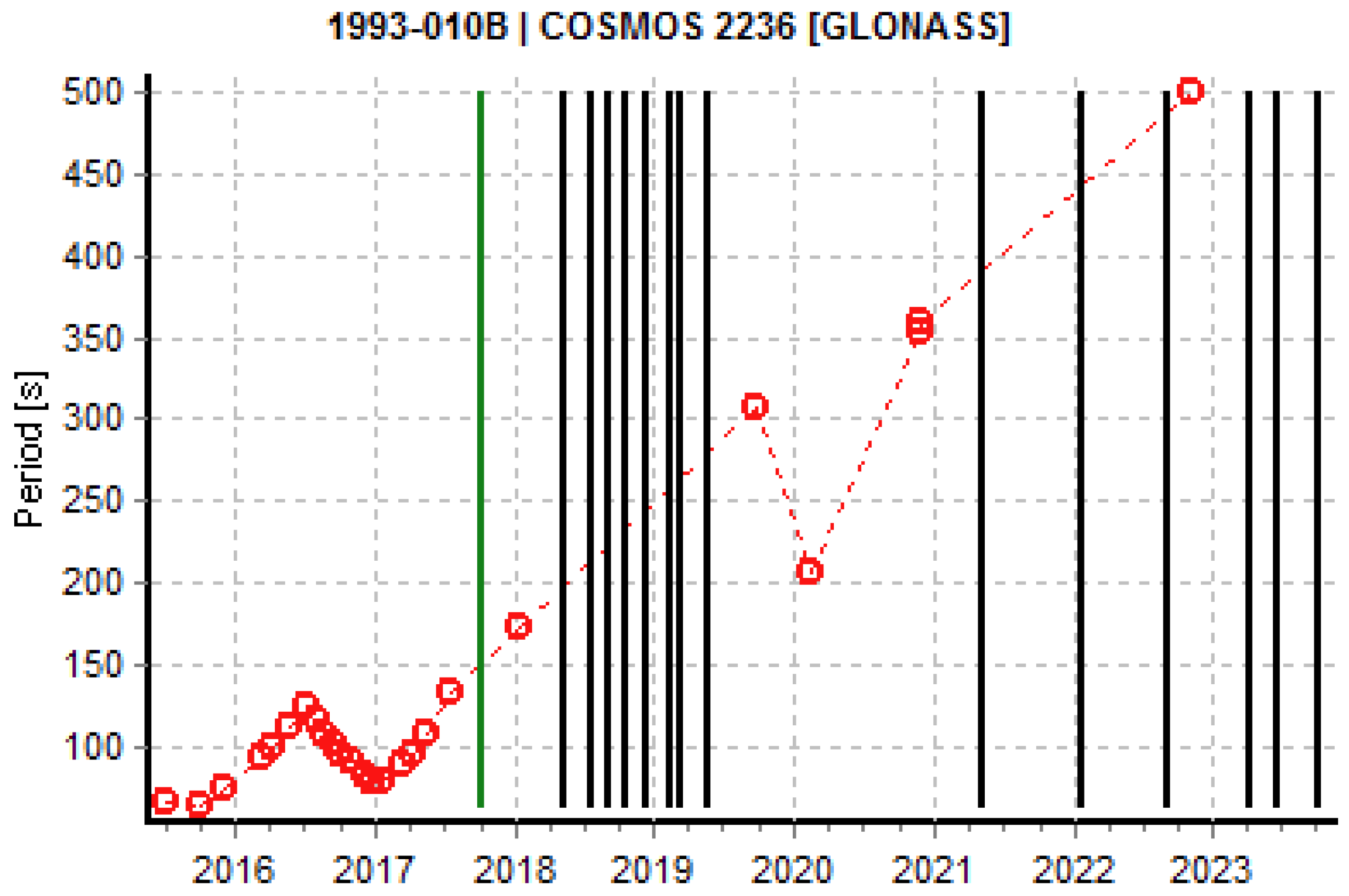
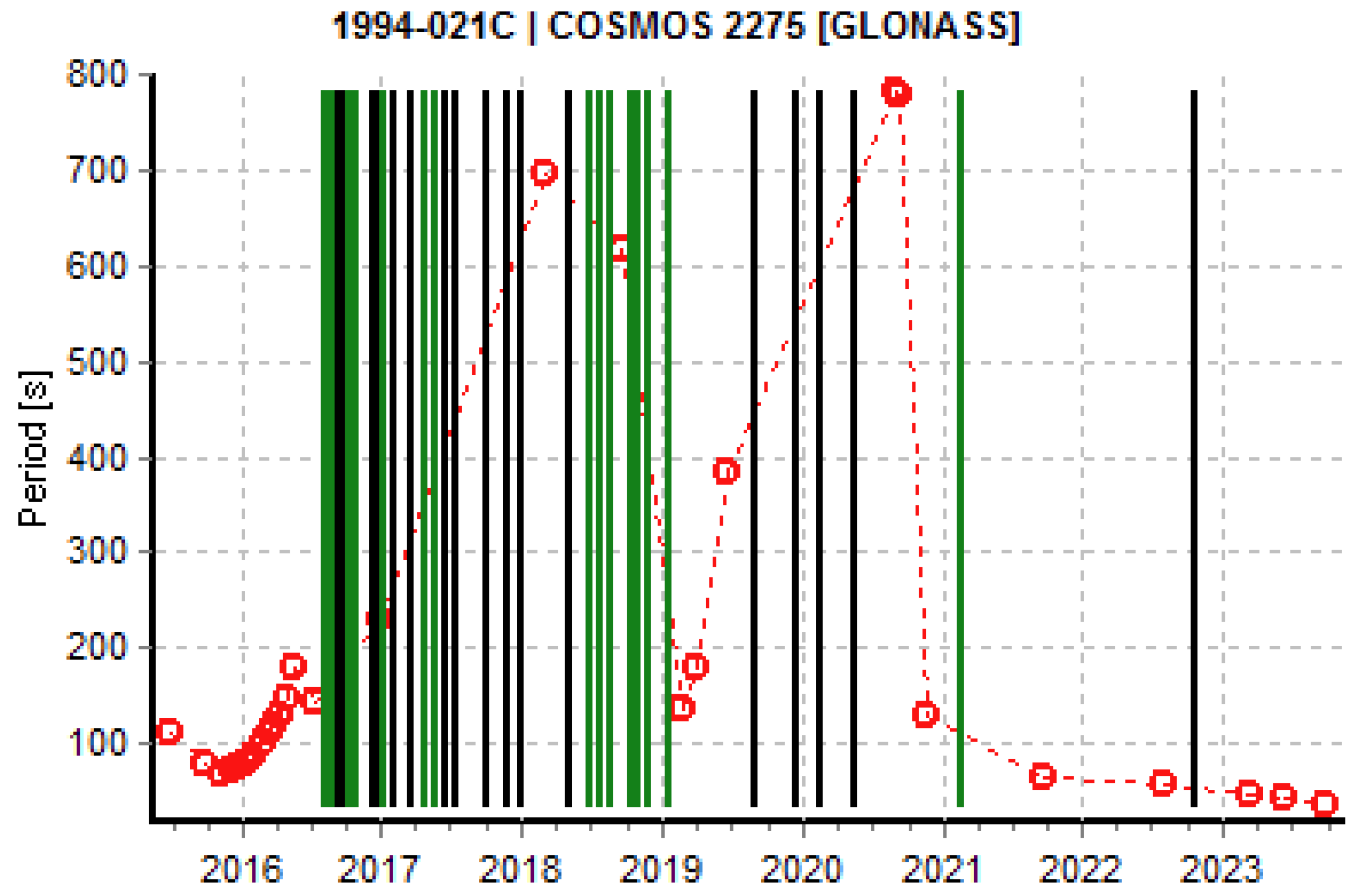
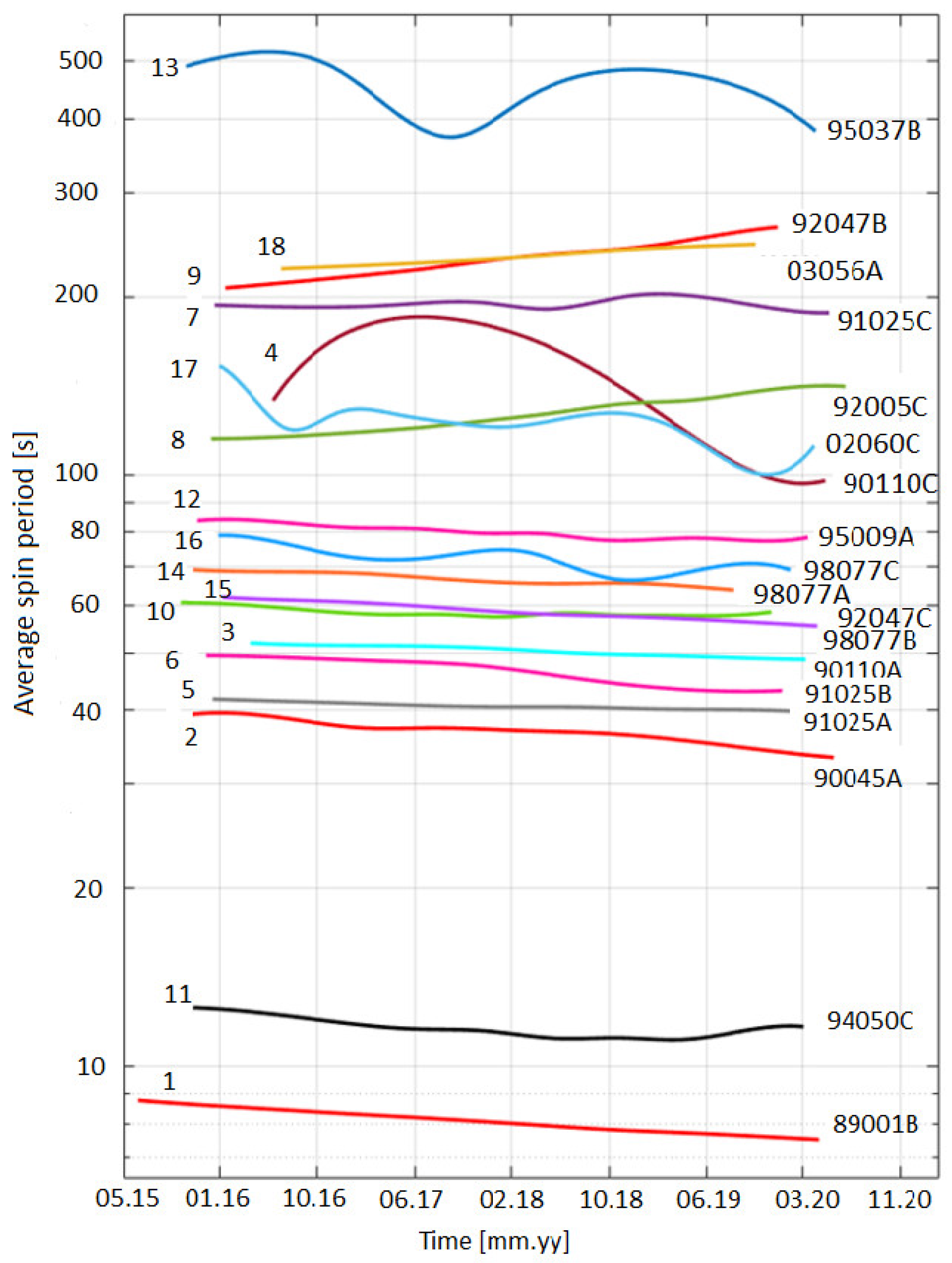
4. Spin Period Evolution
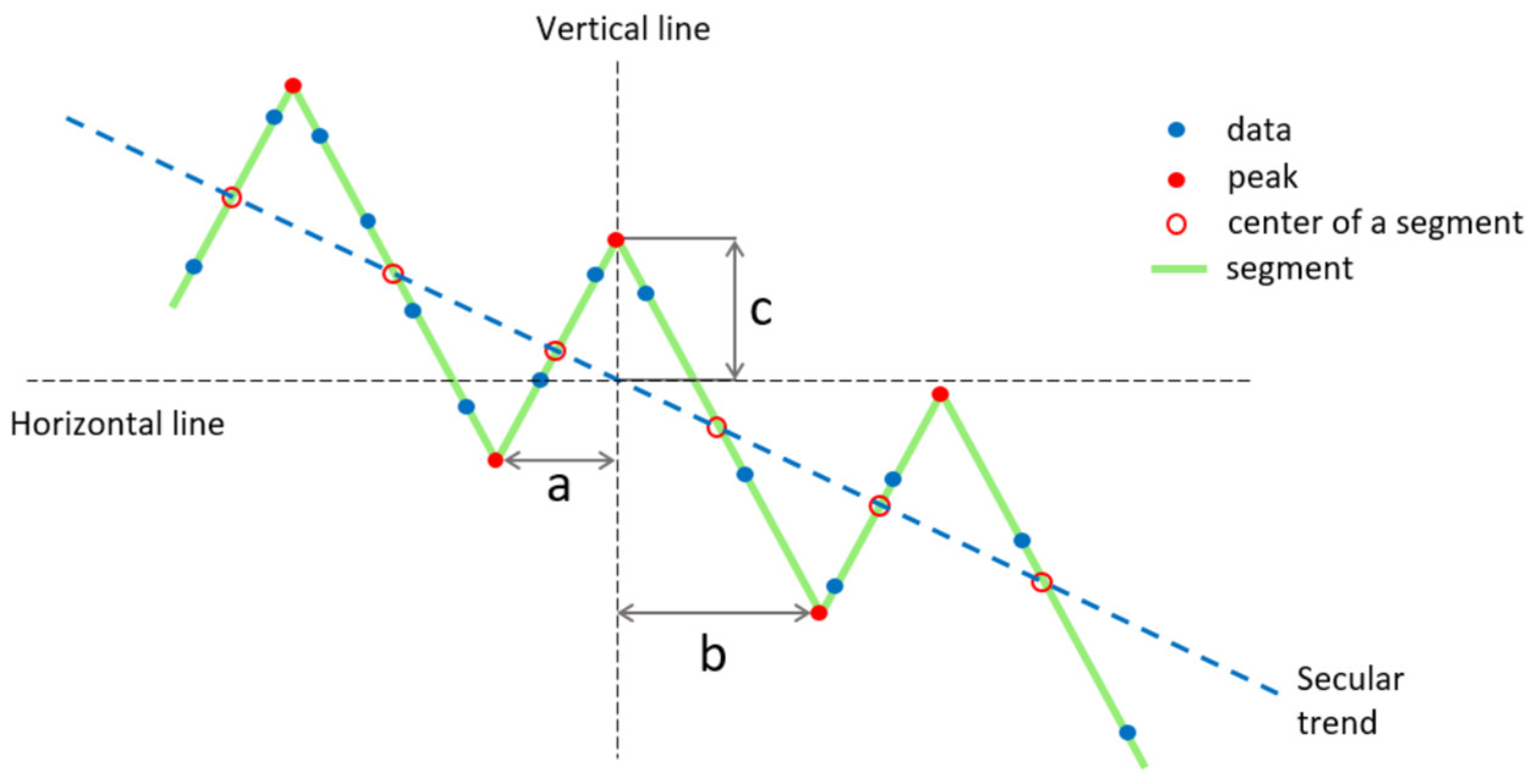
4.1. Spin Period Prediction
4.2. Statistics of Spin Period Evolution
5. Simulations of the Spin Period Evolution
5.1. Simulation Setup
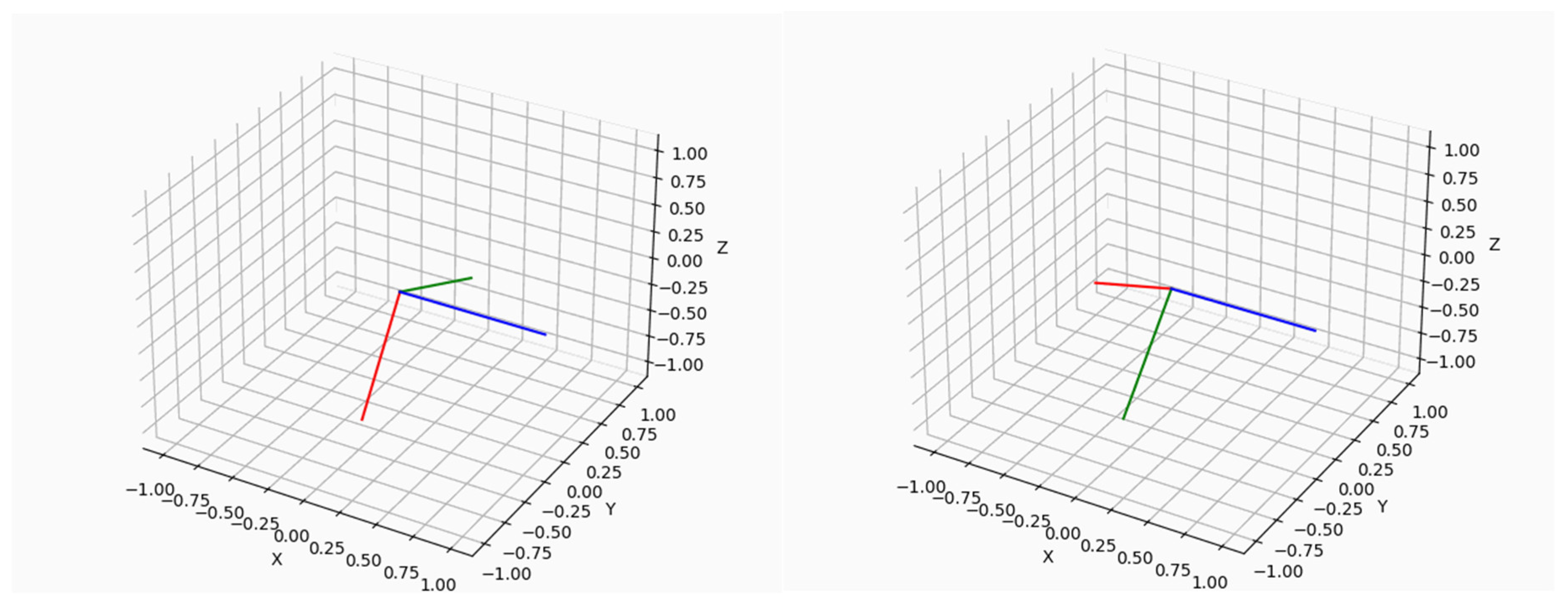
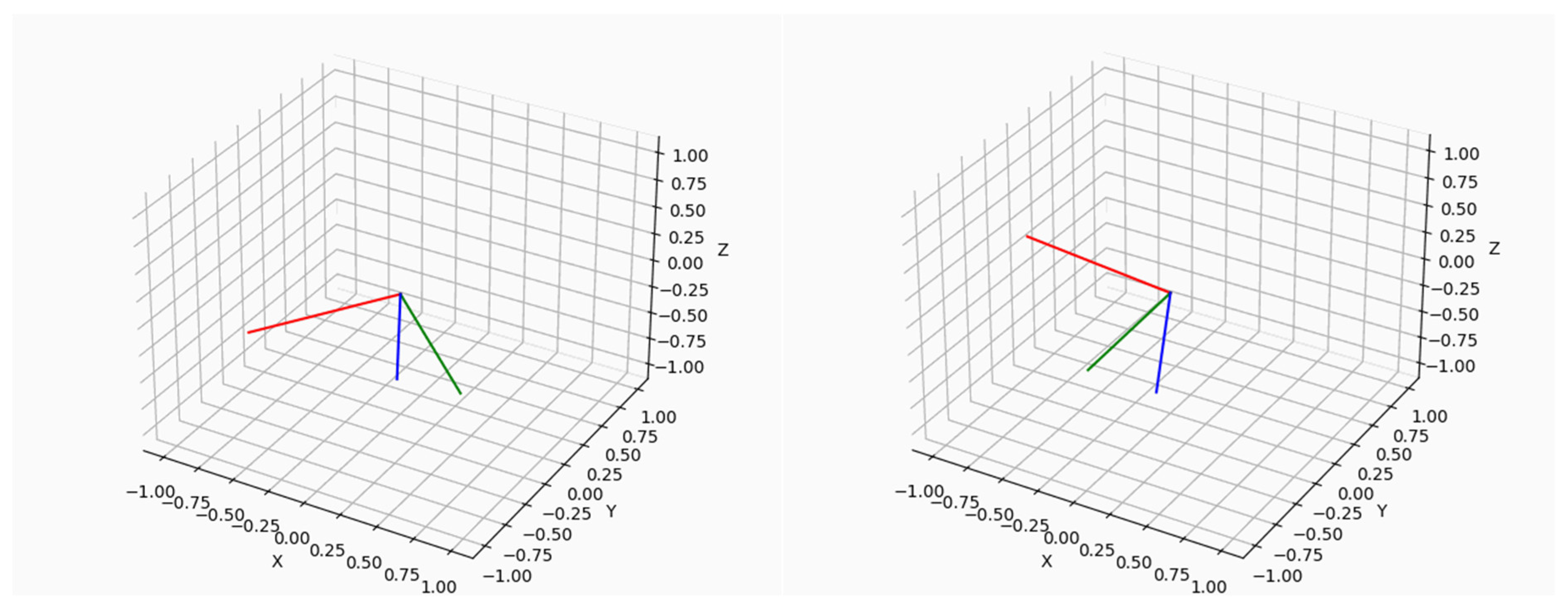
- The orientation of the solar panels with respect to the body frame and their canting angle;
- The initial orientation of the satellite with respect to the orbital frame;
- The initial angular velocity vector of the rotation.
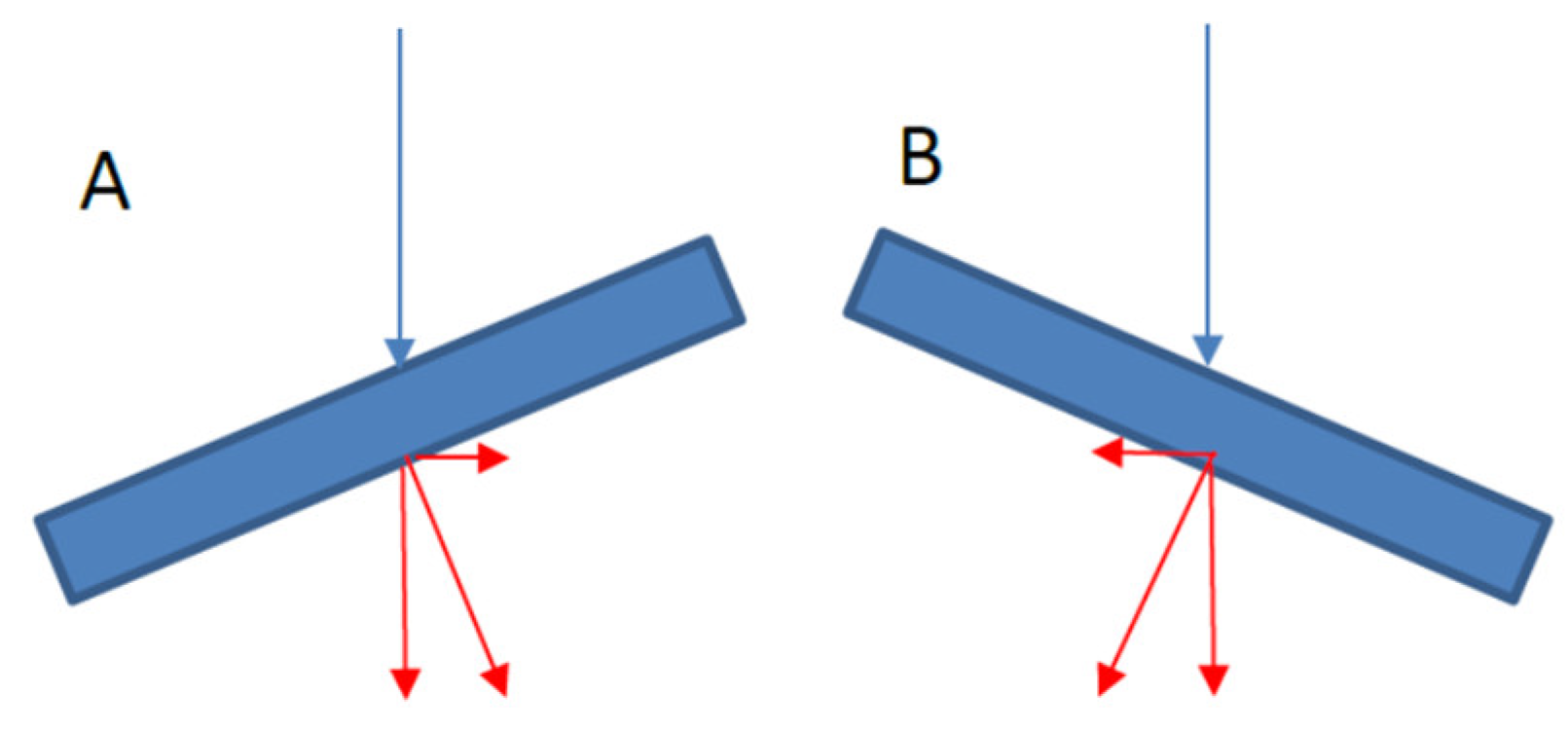
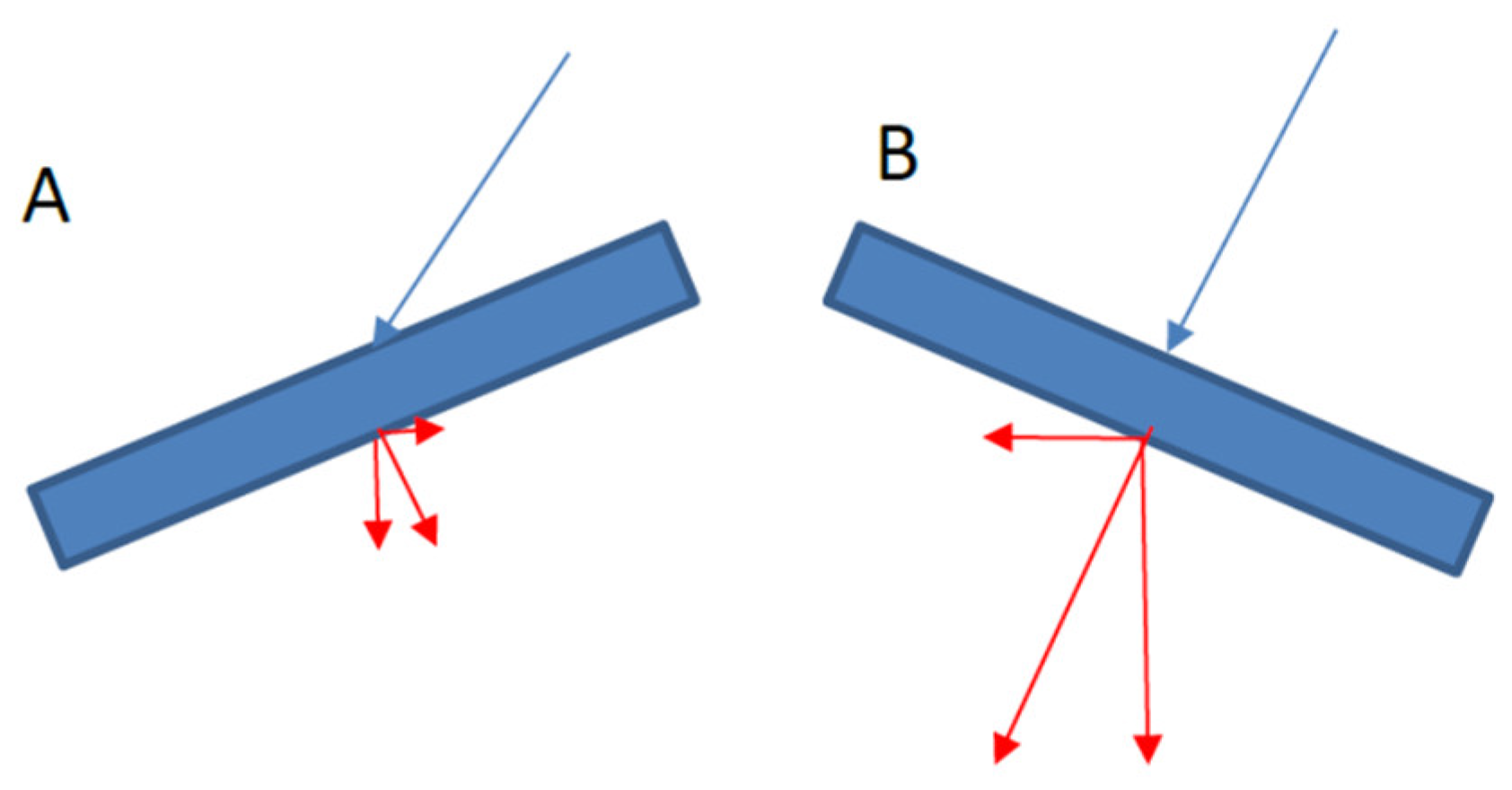
5.2. Wind Wheel Model
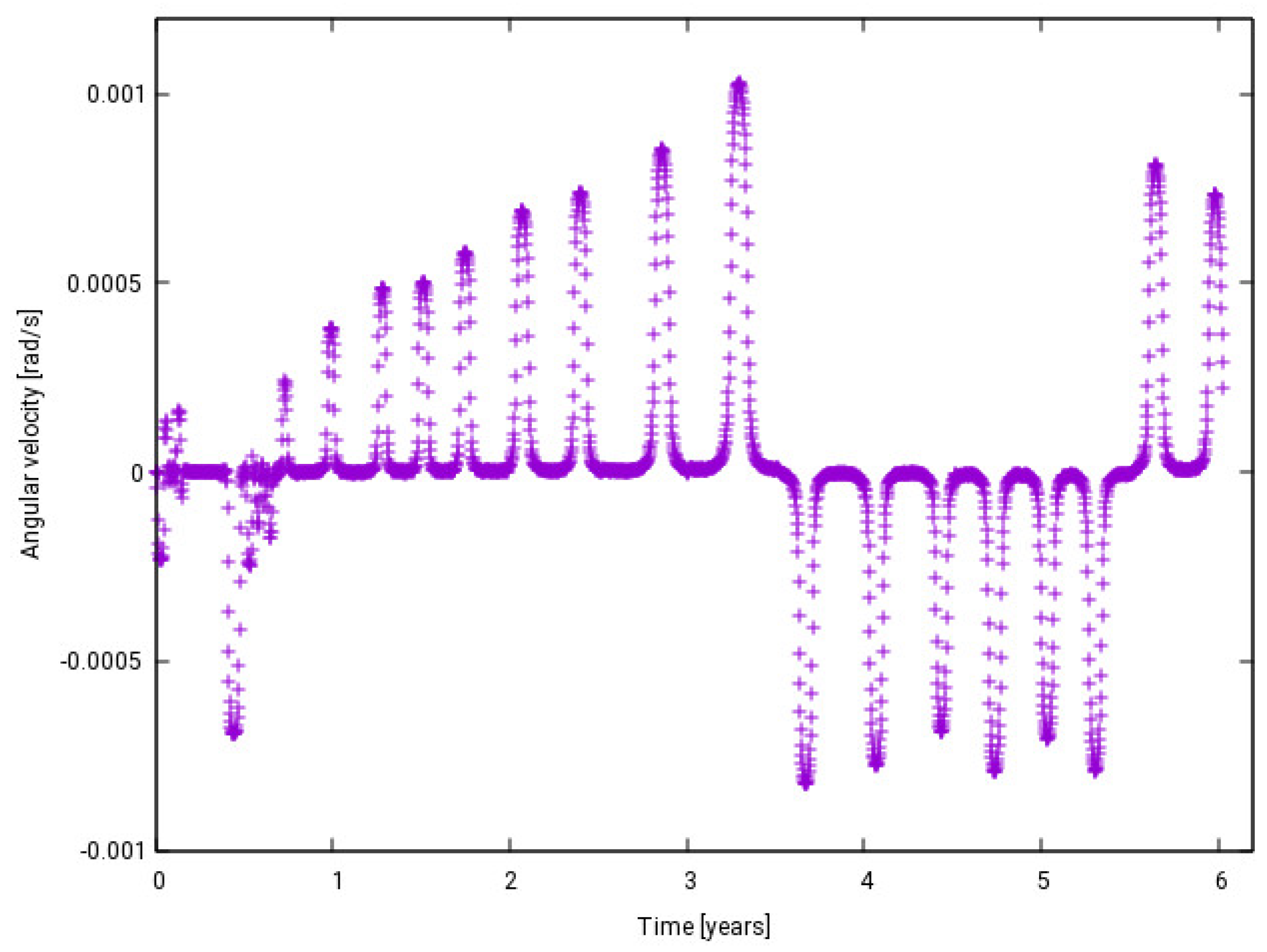
- The initial velocity brings stability in the evolution and the rotation axis keeps its orientation. Simulations with no initial velocity show strong dependence on initial conditions.
- Through the wind wheel effect, the canting of the panel(s) with a constant orientation of the rotation (wind wheel) axis introduces a periodical variation in the angular velocity related to the position of the Sun during the year.
- The amplitude of the periodical variation is determined by the inclination of the wind wheel axis with respect to the ecliptic. If the axis lies in the ecliptic, the maximal amplitude is obtained. Conversely, the minimum is reached with a perpendicular axis.
- The above amplitude also changes with different canting angles, reflection coefficients, or moments of inertia.
- The phase of the periodical variation depends on the orientation of the wind wheel axis with respect to the Sun’s direction. The maximum and minimum of the periodic curve are reached during the year when the axis is perpendicular to the Sun’s direction.
- Different reflection coefficients for the front/back side of the panels introduce a secular trend in the evolution.
5.3. Simulation of Real Case
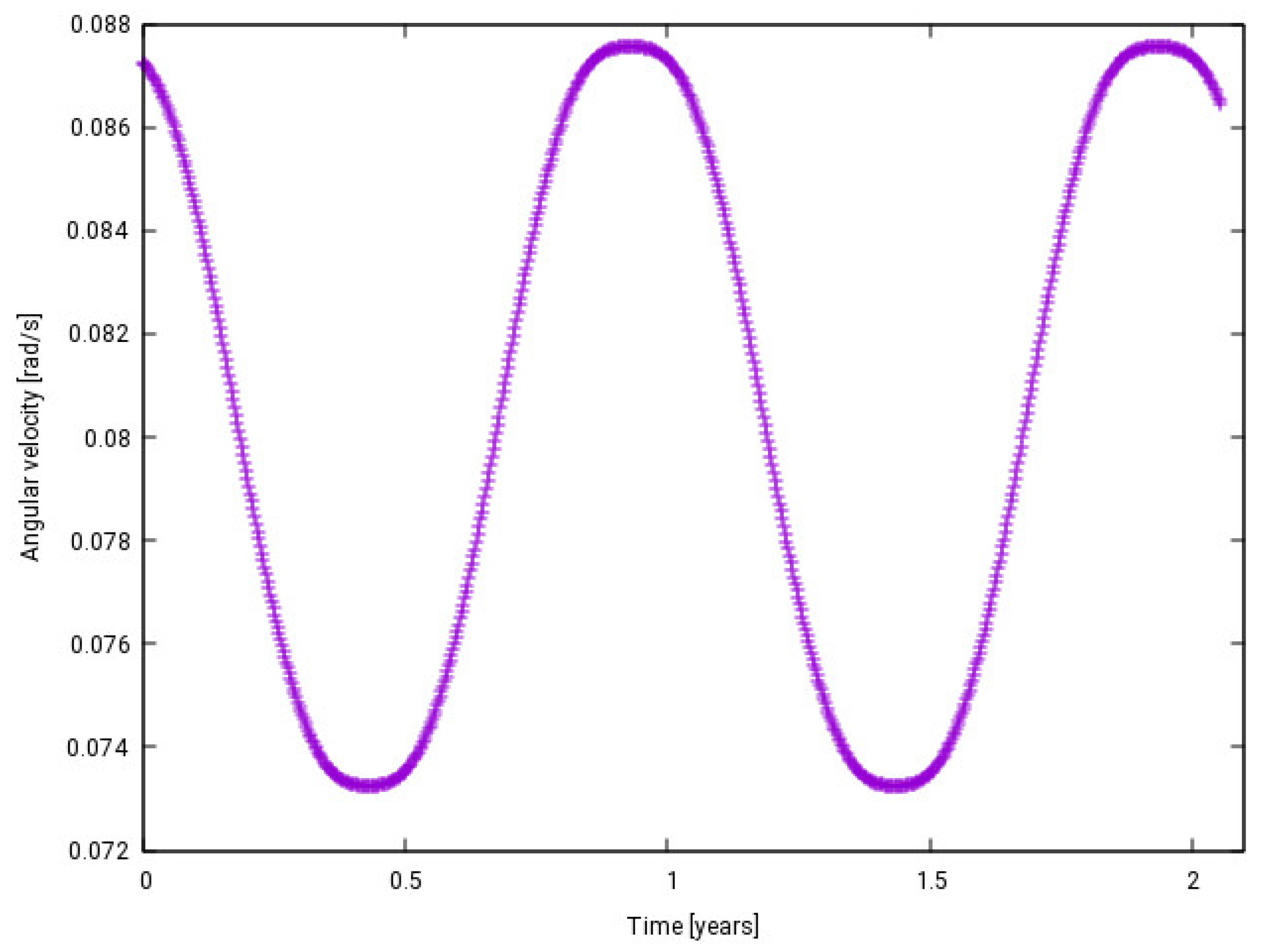
- The initial velocity shifts the whole plot up or down.
- The direction of the z axis in the ecliptic plane shifts the whole plot left or right (phase of the periodic pattern).
- The value of the specular reflection coefficients determines the amplitude of the plot. In particular, the ratio of the coefficients for the front and back sides of the panels determines the secular slope.
- The canting angle also determines the amplitude in the plot (alternative to the change in reflection coefficients).
- Initial velocity: 8.5°/s;
- Direction of z axis in ecliptic plane: 119.3° ecliptic longitude;
- Reflection coefficients, front: 0.5, back: 0.4;
- Canting angle: 5°.
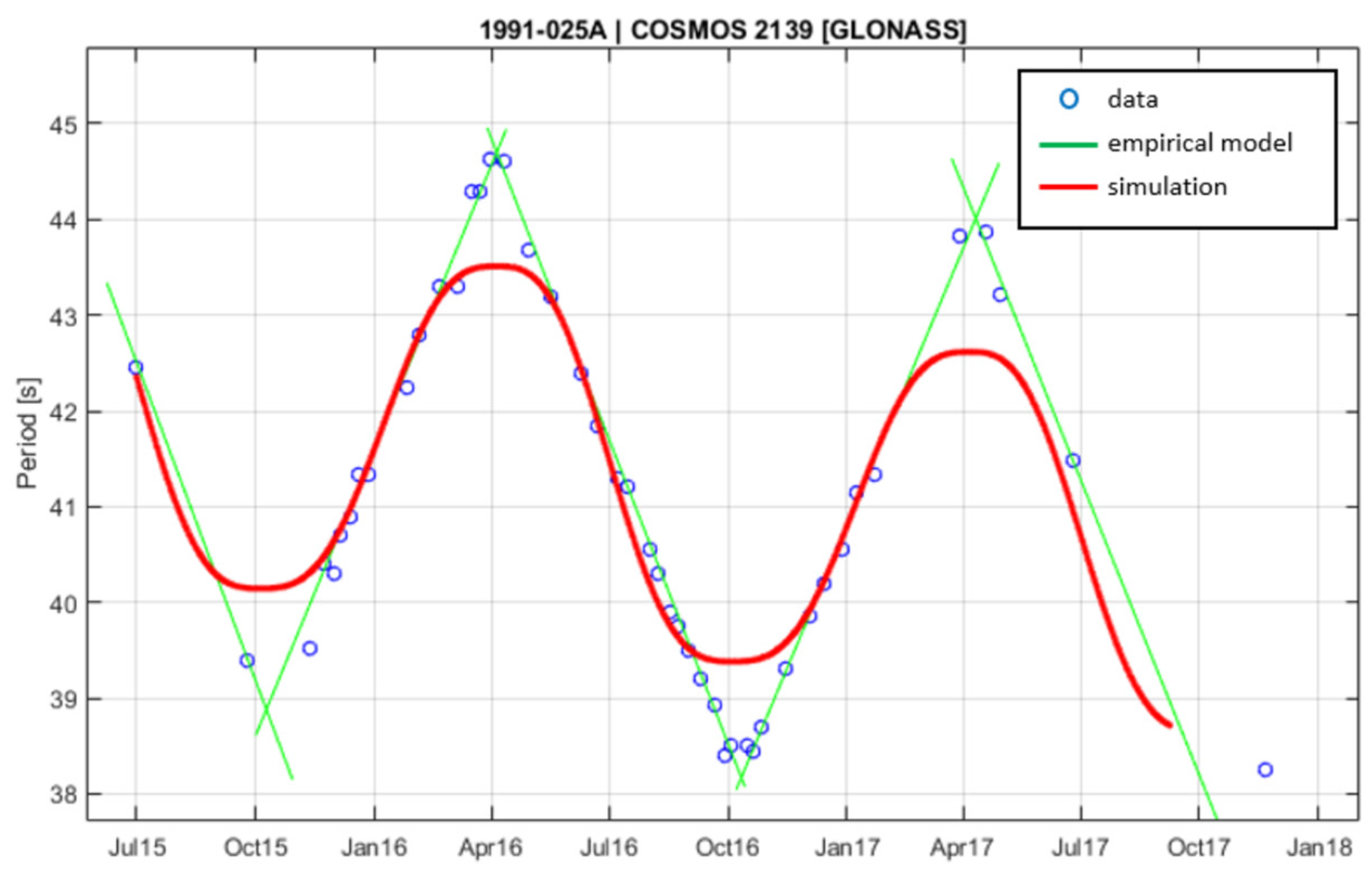
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
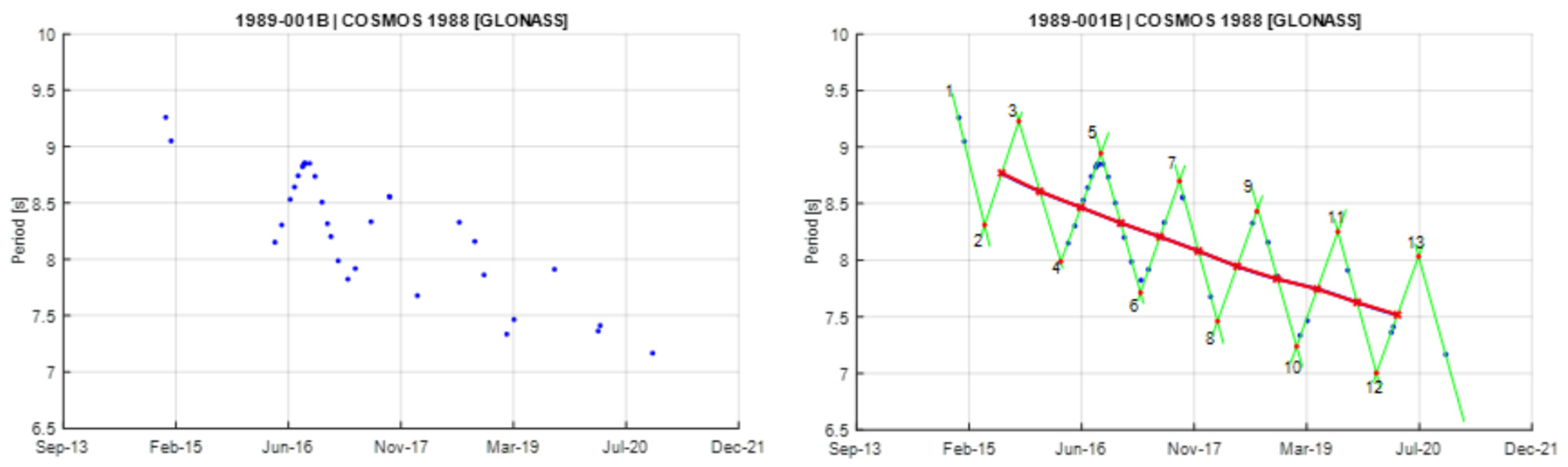

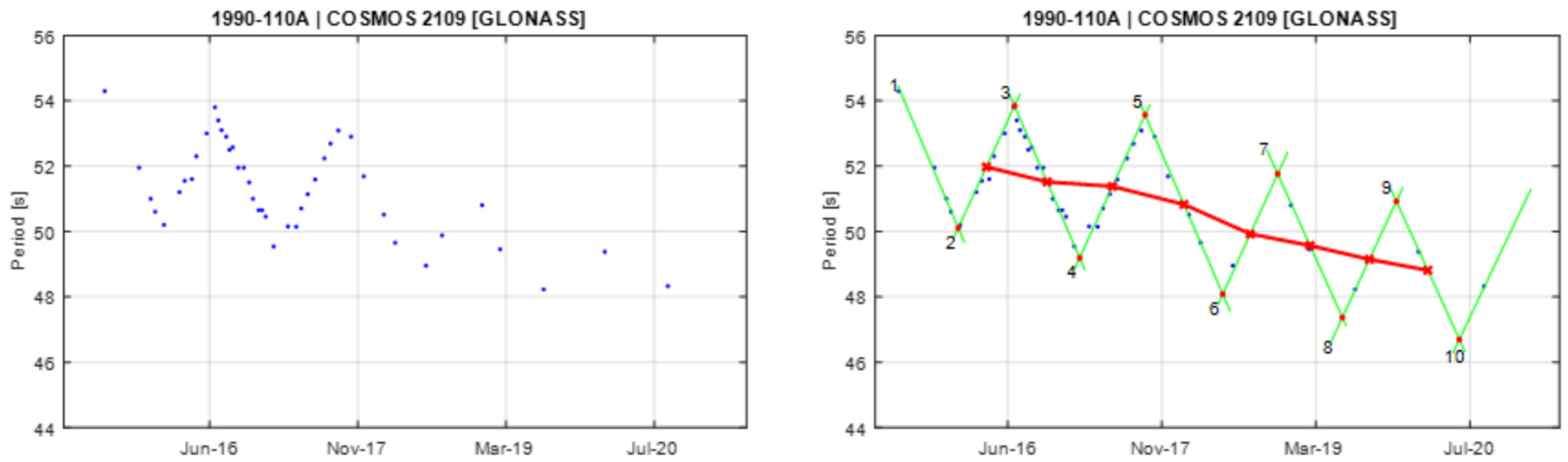
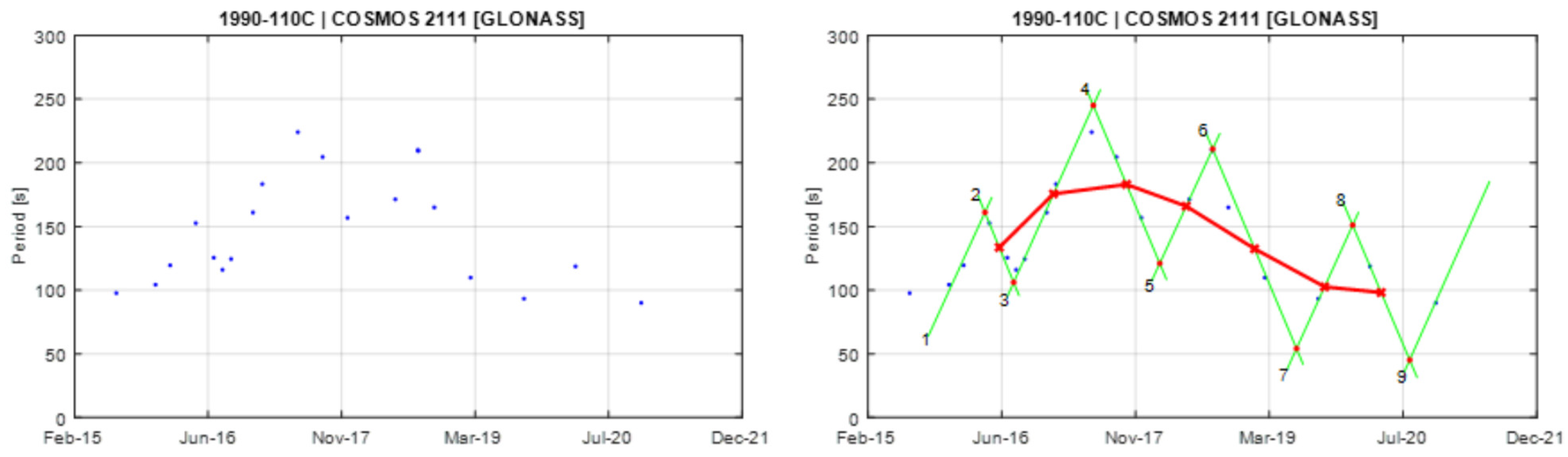


References
- Earl, M.A.; Wade, G.A. Observations and analysis of the apparent spin period variations of inactive boxwing geosynchronous resident space objects. In Proceedings of the 65th International Astronautical Congress, Toronto, ON, Canada, 29 September–3 October 2014. [Google Scholar]
- Silha, J.; Pittet, J.N.; Hamara, M.; Schildknecht, T. Apparent rotation properties of space debris extracted from photometric measurements. Adv. Space Res. 2018, 61, 844–861. [Google Scholar]
- Silha, J.; Krajcovic, S.; Zigo, M.; Toth, J.; Zilkova, D.; Zigo, P.; Kornos, L.; Simon, J.; Schildknecht, T.; Cordelli, E.; et al. Space debris observations with the Slovak AGO70 telescope: Astrometry and light curves. Adv. Space Res. 2020, 65, 2018–2035. [Google Scholar]
- Santoni, F.; Cordelli, E.; Piergentili, F. Determination of disposed-upper-stage attitude motion by ground-based optical observations. J. Spacecr. Rocket. 2013, 50, 701–708. [Google Scholar] [CrossRef]
- Yanagisawa, T.; Kurosaki, H. Shape and motion estimate of LEO debris using light curves. Adv. Space Res. 2012, 50, 136–145. [Google Scholar] [CrossRef]
- Zhao, S.; Steindorfer, M.; Kirchner, G.; Zheng, Y.; Koidl, F.; Wang, P.; Shang, W.; Zhang, J.; Li, T. Attitude analysis of space debris using SLR and light curve data measured with single-photon detector. Adv. Space Res. 2020, 65, 1518–1527. [Google Scholar] [CrossRef]
- Vananti, A.; Lu, Y.; Schildknecht, T. Attitude estimation of H2A rocket body from light curve measurements. Int. J. Astrophys. Space Sci. 2023, 11, 15–22. [Google Scholar] [CrossRef]
- Nussbaum, M.; Schafer, E.; Yoon, Z.; Keil, D.; Stoll, E. Spectral light curve simulation for parameter estimation from space debris. Aerospace 2022, 9, 403. [Google Scholar] [CrossRef]
- Vasile, M.; Walker, L.; Dunphy, R.D.; Zabalza, J.; Murray, P.; Marshall, S.; Savitski, V. Intelligent characterisation of space objects with hyperspectral imaging. Acta Astronaut. 2023, 203, 510–534. [Google Scholar]
- Rachman, A.; Schildknecht, T.; Silha, J.; Pittet, J.N.; Vananti, A. Attitude state evolution of space debris determined from optical light curve observations. In Proceedings of the 68th International Astronautical Congress, Adelaide, Australia, 25–29 September 2017. [Google Scholar]
- Rachman, A.; Schildknecht, T.; Vananti, A. Analysis of temporal evolution of debris objects’ rotation rates inside AIUB light curve database. In Proceedings of the 69th International Astronautical Congress, Bremen, Germany, 1–5 October 2018. [Google Scholar]
- Lee, J.; Park, E.; Choi, M.S.; Kucharski, D.; Yi, Y.; Park, J.U. Spin axis determination of defunct GLONASS satellites using photometry observation. J. Astron. Space Sci. 2021, 38, 45–53. [Google Scholar]
- Albuja, A.; Scheeres, D.J. Defunct satellites, rotation rates and the YORP effect. In Proceedings of the 14th AMOS Technical Conference, Maui, HI, USA, 10–13 September 2013. [Google Scholar]
- Earl, M.A. Photometric analysis and attitude estimation of inactive boxwing geosynchronous satellites. Ph.D. Thesis, Royal Military College of Canada, Kingston, ON, Canada, 2017. [Google Scholar]
- Benson, C.; Scheeres, D.J.; Ryan, W.H.; Ryan, E.V.; Moskovitz, N. Rotation state evolution of retired geosynchronous satellites. In Proceedings of the 18th AMOS Technical Conference, Maui, HI, USA, 19–22 September 2017. [Google Scholar]
- Pittet, J.N.; Šilha, J.; Schildknecht, T. Spin motion determination of the Envisat satellite through laser ranging measurements from a single pass measured by a single station. Adv. Space Res. 2018, 61, 1121–1131. [Google Scholar] [CrossRef]
- Kirchner, G.; Steindorfer, M.; Wang, P.; Koidl, F.; Kucharski, D.; Silha, J.; Schildknecht, T.; Krag, H.; Flohrer, T. Determination of attitude and attitude motion of space debris, using laser ranging and single-photon light curve data. In Proceedings of the 7th European Conference on Space Debris, Darmstadt, Germany, 18–21 April 2017. [Google Scholar]
- Silha, J.; Schildknecht, T.; Pittet, J.N.; Kirchner, G.; Steindorfer, M.; Kucharski, D.; Cerutti-Maori, D.; Rosebrock, J.; Sommer, S.; Leushacke, L.; et al. Debris attitude motion measurements and modelling by combining different observation techniques. J. Br. Interplanet. Soc. 2017, 70, 52–62. [Google Scholar]
- Pontieu, B.D. Database of photometric periods of artificial satellites. Adv. Space Res. 1997, 19, 229–232. [Google Scholar] [CrossRef]
- Albuja, A.A.; Scheeres, D.J.; McMahon, J.W. Evolution of angular velocity for defunct satellites as a result of YORP: An initial study. Adv. Space Res. 2015, 56, 237–251. [Google Scholar] [CrossRef]
- Ojakangas, G.W.; Hill, N. Toward realistic dynamics of rotating orbital debris and implications for light curve. In Proceedings of the 12th AMOS Technical Conference, Maui, HI, USA, 13–16 September 2011. [Google Scholar]
- Albuja, A.A.; Scheeres, D.J.; Cognion, R.L.; Ryan, W.; Ryan, E.V. The YORP effect on the GOES 8 and GOES 10 satellites: A case study. Adv. Space Res. 2018, 61, 122–144. [Google Scholar] [CrossRef]
- Benson, C.J.; Scheeres, D.J.; Ryan, W.H.; Ryan, E.V. Cyclic complex spin state evolution of defunct GEO satellites. In Proceedings of the 19th AMOS Technical Conference, Maui, HI, USA, 11–14 September 2018. [Google Scholar]
- Benson, C.J.; Scheeres, D.J.; Ryan, W.H.; Ryan, E.V.; Moskovitz, N.A. GOES spin state diversity and the implications for GEO debris mitigation. Acta Astronaut. 2020, 167, 212–221. [Google Scholar] [CrossRef]
- Revnivykh, S.; Bolkunov, A.; Serdyukov, A.; Montenbruck, O. GLONASS. In Springer Handbook of Global Navigation Satellite Systems; Teunissen, P.J.G., Montenbruck, O., Eds.; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Linder, E.; Silha, J.; Schildknecht, T.; Hager, M. Extraction of spin periods of space debris from optical light curves. In Proceedings of the 66th International Astronautical Congress, Jerusalem, Israel, 12–16 October 2015. [Google Scholar]
- Earl, M.A.; Somers, P.W.; Kabin, K.; Bedard, D.; Wade, G.A. Estimating the spin axis orientation of the Echostar-2 box-wing geosynchronous satellite. Adv. Space Res. 2018, 61, 2135–2146. [Google Scholar] [CrossRef]
- Kanzler, R.; Silha, J.; Schildknecht, T.; Fritsche, B.; Lips, T.; Krag, H. Space debris attitude simulation-iOTA (In-Orbit Tumbling Analysis). In Proceedings of the 15th AMOS Technical Conference, Maui, HI, USA, 9–12 September 2014. [Google Scholar]
- Sagnieres, L.B.M.; Sharf, I.; Deleflie, F. Simulation of long-term rotational dynamics of large space debris: A TOPEX/Poseidon case study. Adv. Space Res. 2020, 65, 1182–1195. [Google Scholar]
- Rachman, A.; Vananti, A.; Schildknecht, T. Understanding the oscillating pattern in the rotational period evolution of several GLONASS satellites. In Proceedings of the 21st AMOS Technical Conference, Maui, HI, USA, 14–18 September 2020. [Google Scholar]
- Wertz, J.R. Spacecraft Attitude Determination and Control; Astrophysics and Space Science Library; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1978; Volume 73. [Google Scholar]
- Lips, T.; Kanzler, R.; Breslau, A.; Kärräng, P.; Silha, J.; Schildknecht, T.; Kucharski, D.; Kirchner, G.; Rosebrock, J.; Cerutti-Maori, D.; et al. Debris attitude motion measurements and modelling observation vs. simulation. In Proceedings of the 18th AMOS Technical Conference, Maui, HI, USA, 19–22 September 2017. [Google Scholar]






| No | Satellite | Cospar ID | Type | Launch Date | Decommissioning Date | First Observation Date | Debris Age [yr] |
|---|---|---|---|---|---|---|---|
| 1 | COSMOS 1988 | 1989-001B | IIv | 1989-01-10 | 1992-02-16 | 2014-12-22 | 22.85 |
| 2 | COSMOS 2079 | 1990-045A | IIv | 1990-05-19 | 1994-04-23 | 2015-07-06 | 21.20 |
| 3 | COSMOS 2109 | 1990-110A | IIv | 1990-12-08 | 1994-03-17 | 2015-07-01 | 21.29 |
| 4 | COSMOS 2111 | 1990-110C | IIv | 1990-12-08 | 1996-06-09 | 2015-07-11 | 19.09 |
| 5 | COSMOS 2139 | 1991-025A | IIv | 1991-04-04 | 1994-09-29 | 2015-07-01 | 20.75 |
| 6 | COSMOS 2140 | 1991-025B | IIv | 1991-04-04 | 1992-01-06 | 2015-07-03 | 23.49 |
| 7 | COSMOS 2141 | 1991-025C | IIv | 1991-04-04 | 1992-02-26 | 2015-07-03 | 23.35 |
| 8 | COSMOS 2179 | 1992-005C | IIv | 1992-01-29 | 1996-10-25 | 2015-07-06 | 18.69 |
| 9 | COSMOS 2204 | 1992-047B | IIv | 1992-07-30 | 1997-06-27 | 2015-07-14 | 18.05 |
| 10 | COSMOS 2205 | 1992-047C | IIv | 1992-07-30 | 1994-06-29 | 2015-07-06 | 21.02 |
| 11 | COSMOS 2288 | 1994-050C | IIv | 1994-08-11 | 1999-08-24 | 2015-06-28 | 15.84 |
| 12 | COSMOS 2307 | 1995-009A | IIv | 1995-03-07 | 1999-09-10 | 2015-06-19 | 15.77 |
| 13 | COSMOS 2317 | 1995-037B | IIv | 1995-07-24 | 2001-01-24 | 2015-06-17 | 14.39 |
| 14 | COSMOS 2364 | 1998-077A | IIv | 1998-12-30 | 2002-07-08 | 2015-05-12 | 12.84 |
| 15 | COSMOS 2363 | 1998-077B | IIv | 1998-12-30 | 2003-12-19 | 2015-07-13 | 11.56 |
| 16 | COSMOS 2362 | 1998-077C | IIv | 1998-12-30 | 2003-10-20 | 2015-07-16 | 11.74 |
| 17 | COSMOS 2395 | 2002-060C | M | 2002-12-25 | 2008-01-12 | 2015-06-23 | 7.44 |
| 18 | COSMOS 2404 | 2003-056A | M | 2003-12-10 | 2009-06-18 | 2015-07-16 | 6.08 |
| No | Cospar ID | Average Spin Period [s] | Average Velocity [°/s] | Average Cycle Amplitude [s] | Average Cycle Period [yr] | Secular Trend Change of ω Per Year [°/(s·yr)] | Average Segment Change in ω Per Month [°/(s·mo)] |
|---|---|---|---|---|---|---|---|
| 1 | 1989-001B | 8.11 | 44.68 | 1.10 | 0.95 | 1.408 | 1.040 |
| 2 | 1990-045A | 37.00 | 9.77 | 2.71 | 1.13 | 0.340 | 0.111 |
| 3 | 1990-110A | 50.17 | 7.19 | 4.26 | 1.11 | 0.120 | 0.089 |
| 4 | 1990-110C | 136.81 | 3.59 | 108.43 | 1.25 | 0.359 | 0.394 |
| 5 | 1991-025A | 40.71 | 8.89 | 6.05 | 1.02 | 0.095 | 0.212 |
| 6 | 1991-025B | 46.62 | 7.83 | 8.56 | 1.35 | 0.300 | 0.179 |
| 7 | 1991-025C | 193.41 | 1.86 | 18.06 | 1.09 | 0.001 | 0.028 |
| 8 | 1992-005C | 127.80 | 2.84 | 12.95 | 0.90 | −0.143 | 0.056 |
| 9 | 1992-047B | 232.31 | 1.58 | 49.73 | 0.97 | −0.093 | 0.060 |
| 10 | 1992-047C | 58.82 | 6.13 | 2.84 | 0.78 | 0.059 | 0.068 |
| 11 | 1994-050C | 11.94 | 32.05 | 5.52 | 0.96 | 0.634 | 2.680 |
| 12 | 1995-009A | 79.92 | 4.56 | 16.74 | 0.87 | 0.083 | 0.182 |
| 13 | 1995-037B | 469.58 | 1.17 | 513.24 | 0.98 | 0.028 | 0.243 |
| 14 | 1998-077A | 66.55 | 5.42 | 2.10 | 0.95 | 0.109 | 0.033 |
| 15 | 1998-077B | 58.76 | 6.14 | 1.51 | 0.94 | 0.165 | 0.034 |
| 16 | 1998-077C | 72.38 | 5.02 | 8.76 | 0.98 | 0.181 | 0.109 |
| 17 | 2002-060C | 127.90 | 3.05 | 52.56 | 0.79 | 0.140 | 0.304 |
| 18 | 2003-056A | 235.17 | 1.53 | 18.32 | 0.96 | −0.046 | 0.021 |
| Statistics | Average Spin Period [s] | Average Velocity [°/s] | Average Cycle Amplitude [s] | Average Cycle Period [yr] | Absolute Value of Secular Trend Change in ω Per Year [°/(s·yr)] | Average Segment Change in ω Per Month [°/(s·mo)] |
|---|---|---|---|---|---|---|
| Maximum | 469.58 | 44.68 | 513.24 | 1.35 | 1.408 | 2.680 |
| Minimum | 8.11 | 1.17 | 1.10 | 0.78 | 0.001 | 0.021 |
| Mean | 132.82 | 8.13 | 70.88 | 1.01 | 0.227 | 0.395 |
| Median | 69.47 | 5.22 | 8.66 | 0.96 | 0.130 | 0.110 |
| Property | Value |
|---|---|
| Mass | 2110 kg |
| Bus length | 5 m |
| Bus diameter | 2 m |
| Solar panel length (one side) | 5.45 m |
| Solar panel width (one side) | 3.73 m |
| Solar panel depth | 5 cm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rachman, A.; Vananti, A.; Schildknecht, T. Spin Period Evolution of Decommissioned GLONASS Satellites. Aerospace 2025, 12, 283. https://doi.org/10.3390/aerospace12040283
Rachman A, Vananti A, Schildknecht T. Spin Period Evolution of Decommissioned GLONASS Satellites. Aerospace. 2025; 12(4):283. https://doi.org/10.3390/aerospace12040283
Chicago/Turabian StyleRachman, Abdul, Alessandro Vananti, and Thomas Schildknecht. 2025. "Spin Period Evolution of Decommissioned GLONASS Satellites" Aerospace 12, no. 4: 283. https://doi.org/10.3390/aerospace12040283
APA StyleRachman, A., Vananti, A., & Schildknecht, T. (2025). Spin Period Evolution of Decommissioned GLONASS Satellites. Aerospace, 12(4), 283. https://doi.org/10.3390/aerospace12040283






