Analysis of Flutter Characteristics for Composite Laminates in Hypersonic Yawed Flow
Abstract
1. Introduction
2. Methodology
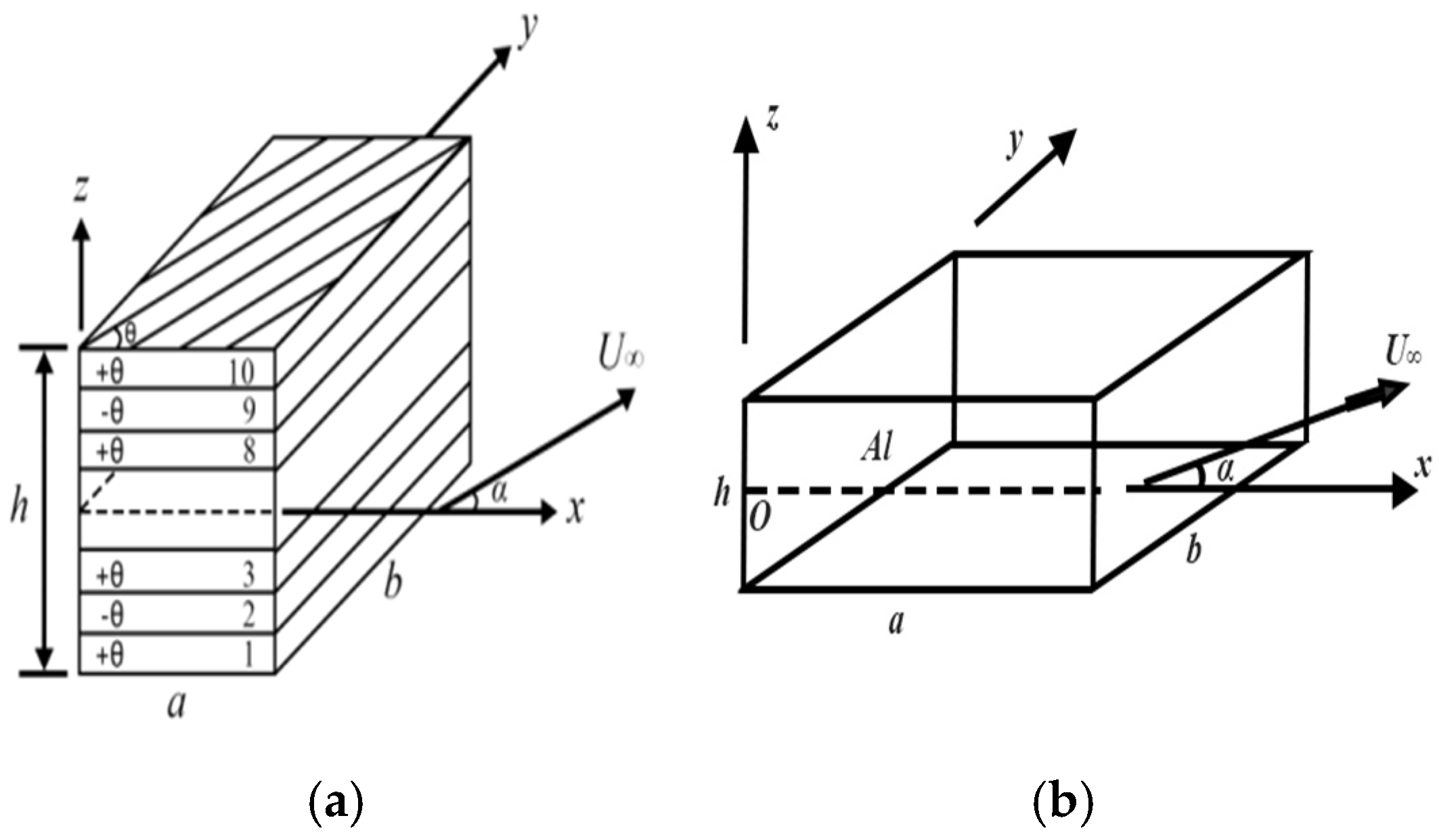
2.1. Constitutive Relation
2.2. Governing Equation
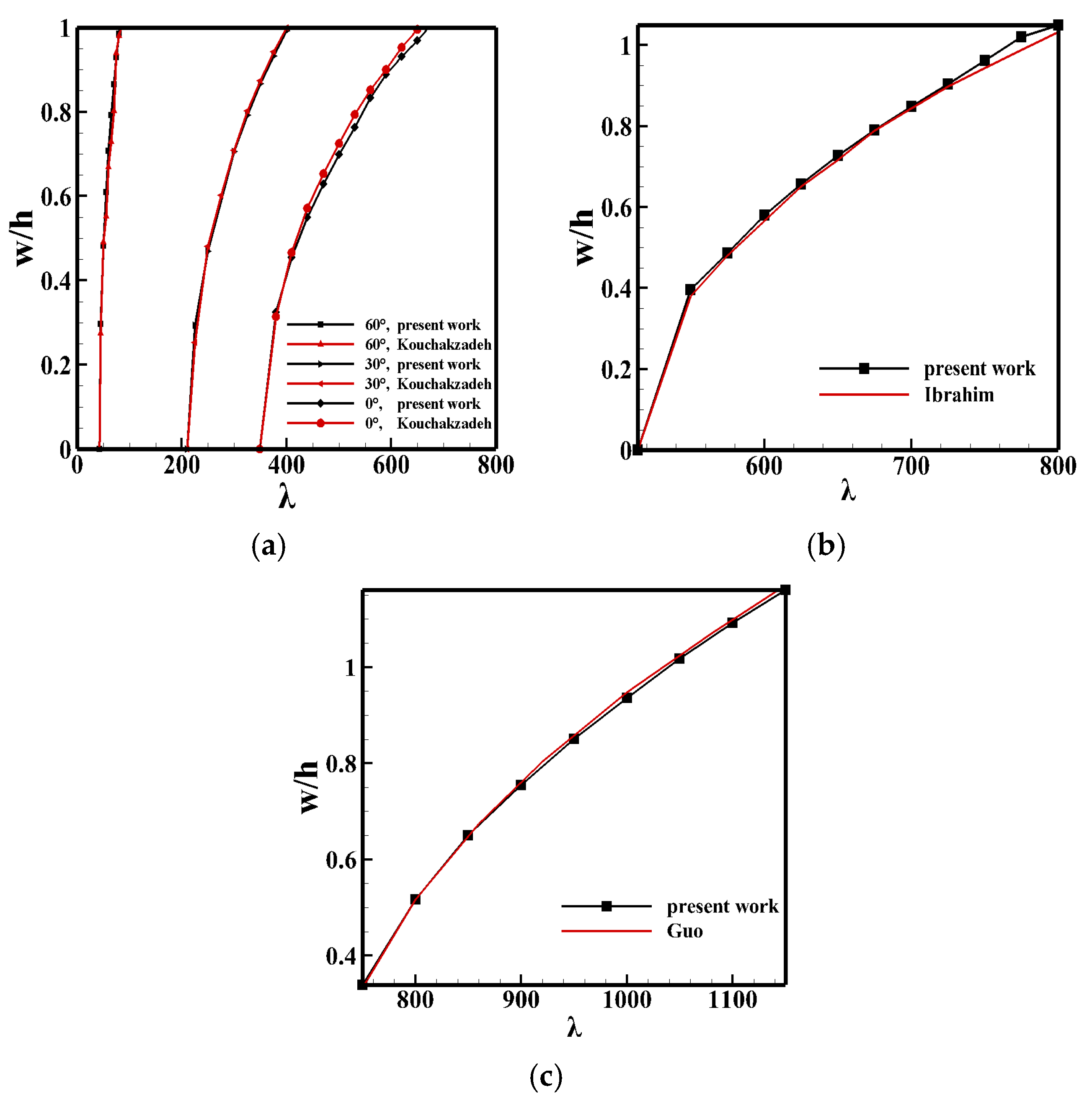
2.3. Code Validation
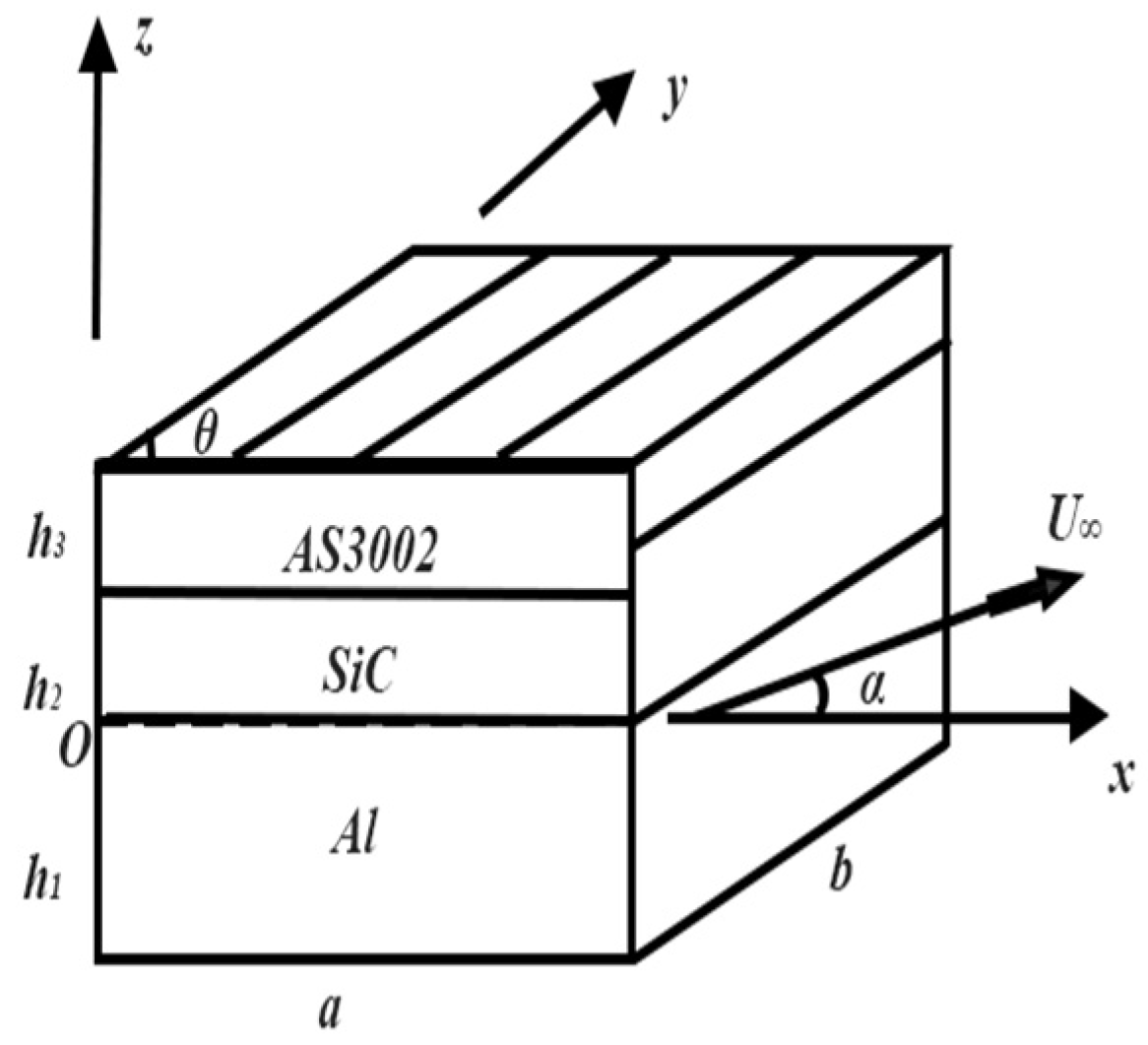
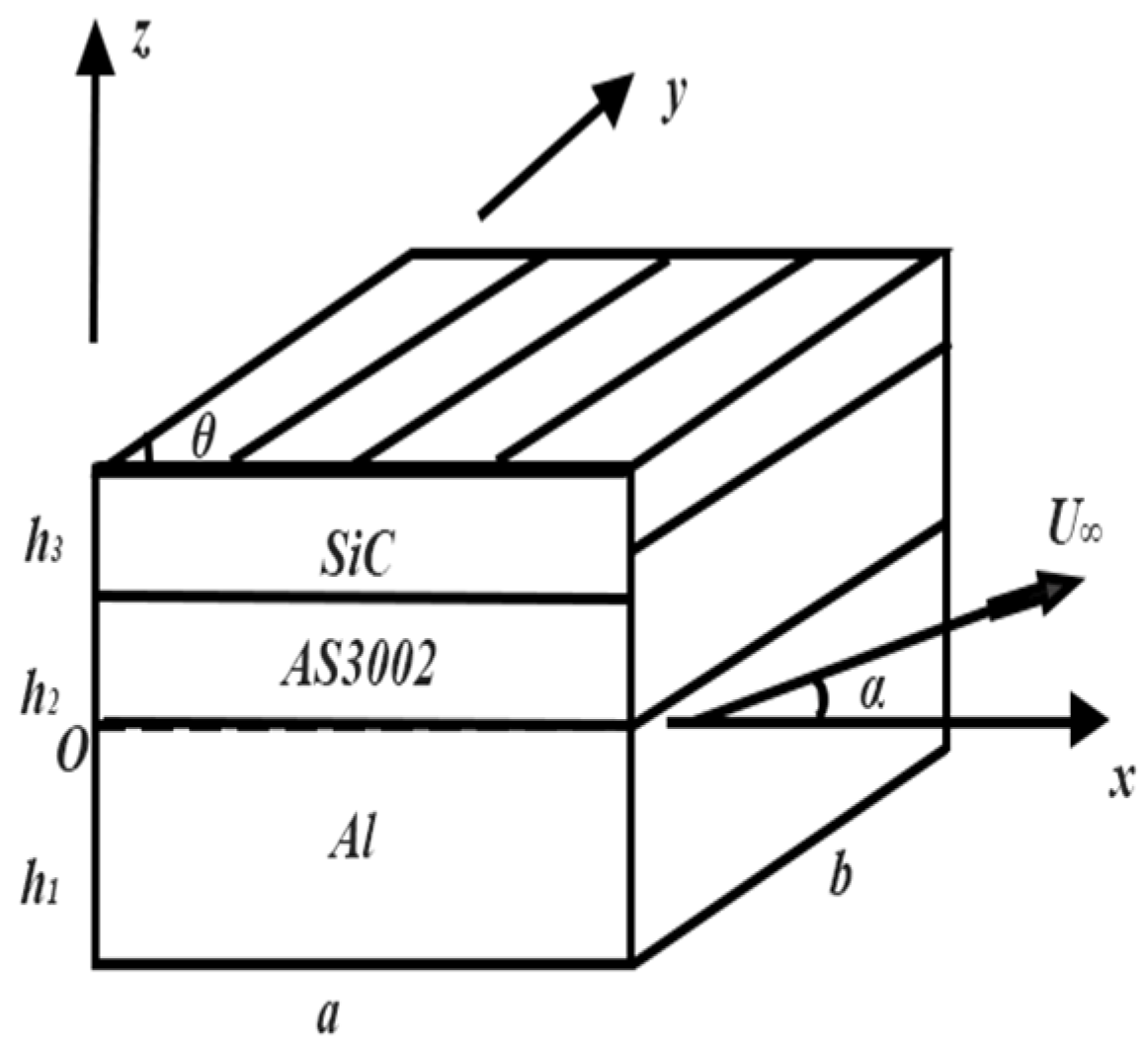
3. Numerical Results and Discussion
3.1. Effect of Stacking Sequence
3.2. Effect of Thickness Ratio
3.3. Effect of the Fiber Orientation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Meng, X.; Zhao, R.; Wang, Q.; Zhang, Z.; Wang, J. Fluid–Structure Interactions between Oblique Shock Trains and Thin-Walled Structures in Isolators. Aerospace 2024, 11, 482. [Google Scholar] [CrossRef]
- Wang, Y.-R.; Ma, Y.-H. Application of Deep Learning Models to Predict Panel Flutter in Aerospace Structures. Aerospace 2024, 11, 677. [Google Scholar] [CrossRef]
- Hasheminejad, S.M.; Aghayi Motaaleghi, M. Aeroelastic analysis and active flutter suppression of an electro-rheological sandwich cylindrical panel under yawed supersonic flow. Aerosp. Sci. Technol. 2015, 42, 118–127. [Google Scholar] [CrossRef]
- Li, J.; Narita, Y. Analysis and optimal design for supersonic composite laminated plate. Compos. Struct. 2013, 101, 35–46. [Google Scholar] [CrossRef]
- Shao, C.; Cao, D.; Xu, Y.; Zhao, H. Flutter and Thermal Buckling Analysis for Composite Laminated Panel Embedded with Shape Memory Alloy Wires in Supersonic Flow. Int. J. Aerosp. Eng. 2016, 2016, 8562716. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, M.; Yang, Z.; Kłosowski, P. Nonlinear Flutter Response of Heated Curved Composite Panels with Embedded Macrofiber Composite Actuators. Adv. Mater. Sci. Eng. 2018, 2018, 3103250. [Google Scholar] [CrossRef]
- Qi, W.; Wang, M.; Tian, S. Effects of Mass Attachments on Flutter Characteristics of Thin-Walled Panels. Aerospace 2022, 9, 748. [Google Scholar] [CrossRef]
- Dowell, E.H. Nonlinear oscillations of a fluttering plate. AIAA J. 1966, 4, 1267–1275. [Google Scholar] [CrossRef]
- Dowell, E.H. Nonlinear oscillations of a fluttering plate. II. AIAA J. 1967, 5, 1856–1862. [Google Scholar] [CrossRef]
- Ventres, C.S.; Dowell, E.H. Comparison of theory and experiment for nonlinear flutter of loaded plates. AIAA J. 1970, 8, 2022–2030. [Google Scholar] [CrossRef]
- Kouchakzadeh, M.A.; Rasekh, M.; Haddadpour, H. Panel flutter analysis of general laminated composite plates. Compos. Struct. 2010, 92, 2906–2915. [Google Scholar] [CrossRef]
- Shiau, L.-C.; Lu, L.-T. Nonlinear flutter of two-dimensional simply supported symmetric composite laminated plates. J. Aircr. 1992, 29, 140–145. [Google Scholar] [CrossRef]
- Ibrahim, H.H.; Yoo, H.H.; Lee, K.-S. Supersonic Flutter of Functionally Grated Panels Subject to Acoustic and Thermal Loads. J. Aircr. 2009, 46, 593–600. [Google Scholar] [CrossRef]
- Xie, D.; Xu, M.; Dowell, E.H. Proper Orthogonal Decomposition Reduced-Order Model for Nonlinear Aeroelastic Oscillations. AIAA J. 2014, 52, 229–241. [Google Scholar] [CrossRef]
- Zhou, K. Nonlinear dynamic analysis and vibration suppression on the composite laminated plates with general boundary conditions in supersonic airflow. Thin-Walled Struct. 2023, 190, 110956. [Google Scholar] [CrossRef]
- Amirzadegan, S.; Dowell, E.H. Correlation of Experimental and Computational Results for Flutter of Streamwise Curved Plate. AIAA J. 2019, 57, 3556–3561. [Google Scholar] [CrossRef]
- Arani, A.G.; Kiani, F.; Afshari, H. Aeroelastic Analysis of Laminated FG-CNTRC Cylindrical Panels Under Yawed Supersonic Flow. Int. J. Appl. Mech. 2019, 11, 1950052. [Google Scholar] [CrossRef]
- Singha, M.K.; Mandal, M. Supersonic flutter characteristics of composite cylindrical panels. Compos. Struct. 2008, 82, 295–301. [Google Scholar] [CrossRef]
- Lin, H.; Cao, D.; Xu, Y. Vibration characteristics and flutter analysis of a composite laminated plate with a store. Appl. Math. Mech. 2017, 39, 241–260. [Google Scholar] [CrossRef]
- Zhou, K.; Huang, X.; Tian, J.; Hua, H. Vibration and flutter analysis of supersonic porous functionally graded material plates with temperature gradient and resting on elastic foundation. Compos. Struct. 2018, 204, 63–79. [Google Scholar] [CrossRef]
- Chai, Y.; Li, F.; Song, Z. Nonlinear vibrations, bifurcations and chaos of lattice sandwich composite panels on Winkler–Pasternak elastic foundations with thermal effects in supersonic airflow. Meccanica 2019, 54, 919–944. [Google Scholar] [CrossRef]
- Meng, X.; Ye, Z.; Ye, K.; Liu, C. Analysis on location of maximum vibration amplitude in panel flutter. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 234, 457–469. [Google Scholar] [CrossRef]
- Abdukhakimov, F.A.; Vedeneev, V.V. Effect of yaw angle on flutter of rectangular plates at low supersonic speeds. AIAA J. 2022, 60, 4256–4266. [Google Scholar] [CrossRef]
- Moreira, J.A.; Moleiro, F.; Araújo, A.L.; Pagani, A. Analytical modeling of panel flutter and active control in supersonic variable stiffness composite laminates. Mech. Adv. Mater. Struct. 2022, 30, 930–944. [Google Scholar] [CrossRef]
- Mozaffari-Jovin, S.; Firouz-Abadi, R.D.; Roshanian, J. Flutter of wings involving a locally distributed flexible control surface. J. Sound Vib. 2015, 357, 377–408. [Google Scholar] [CrossRef]
- Vindigni, C.R.; Mantegna, G.; Orlando, C.; Alaimo, A.; Berci, M. A refined aeroelastic beam finite element for the stability analysis of flexible subsonic wings. Comput. Struct. 2025, 307, 107618. [Google Scholar] [CrossRef]
- Jansson, S.; Deve, H.E.; Evans, A.G. The anisotropic mechanical-properties of a TI matrix composite reinforced with SiC fibers. Metall. Trans. A-Phys. Metall. Mater. Sci. 1991, 22, 2975–2984. [Google Scholar] [CrossRef]
- Paley, M.; Aboudi, J. Viscoplastic bifurcation buckling of plates. AIAA J. 1991, 29, 627–632. [Google Scholar] [CrossRef]
- Muc, A.; Flis, J. Free vibrations and supersonic flutter of multilayered laminated cylindrical panels. Compos. Struct. 2020, 246, 112400. [Google Scholar] [CrossRef]
- Guo, X.; Mei, C. Using Aeroelastic Modes for Nonlinear Panel Flutter at Arbitrary Supersonic Yawed Angle. AIAA J. 2003, 41, 272–279. [Google Scholar] [CrossRef]
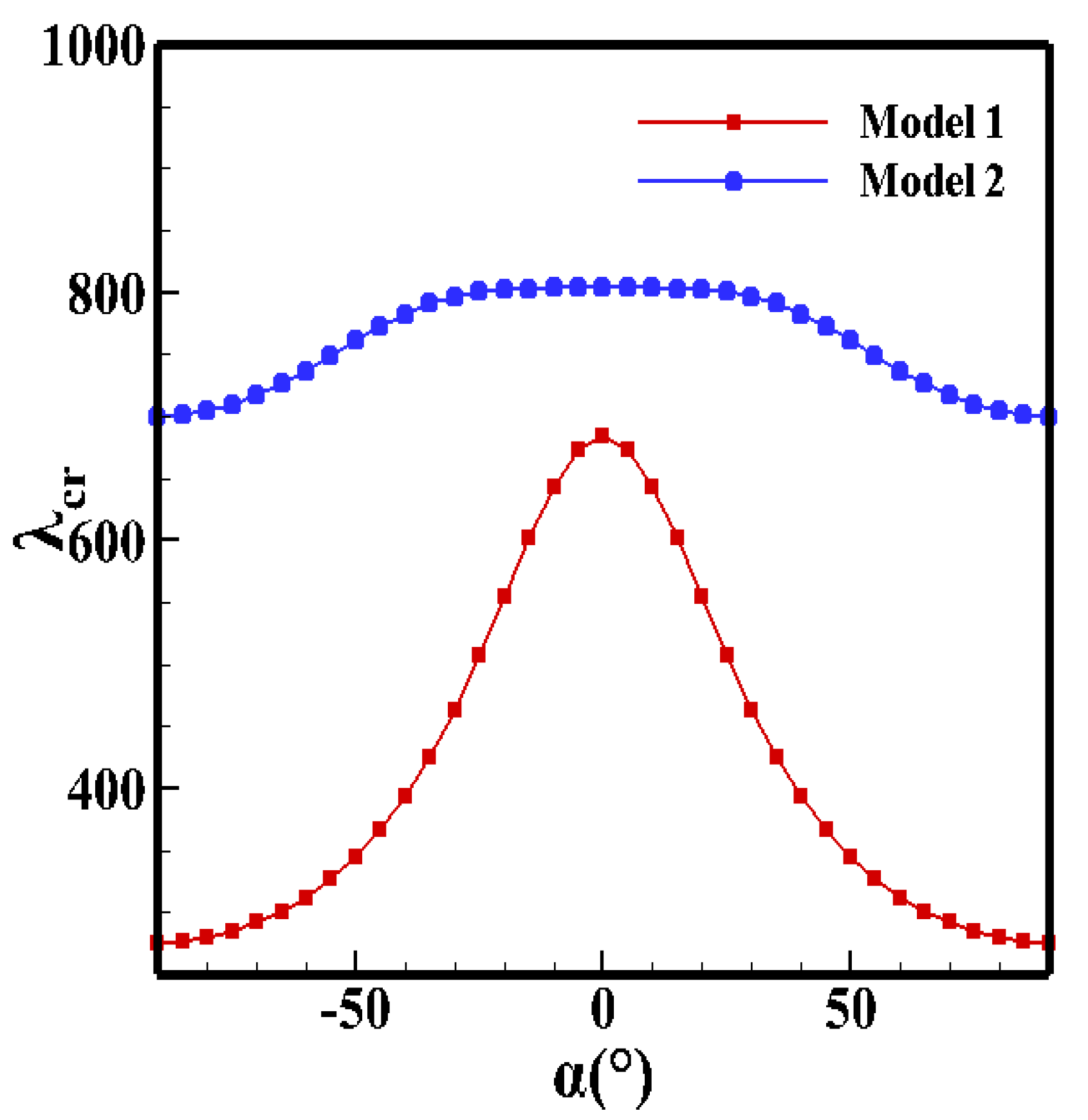

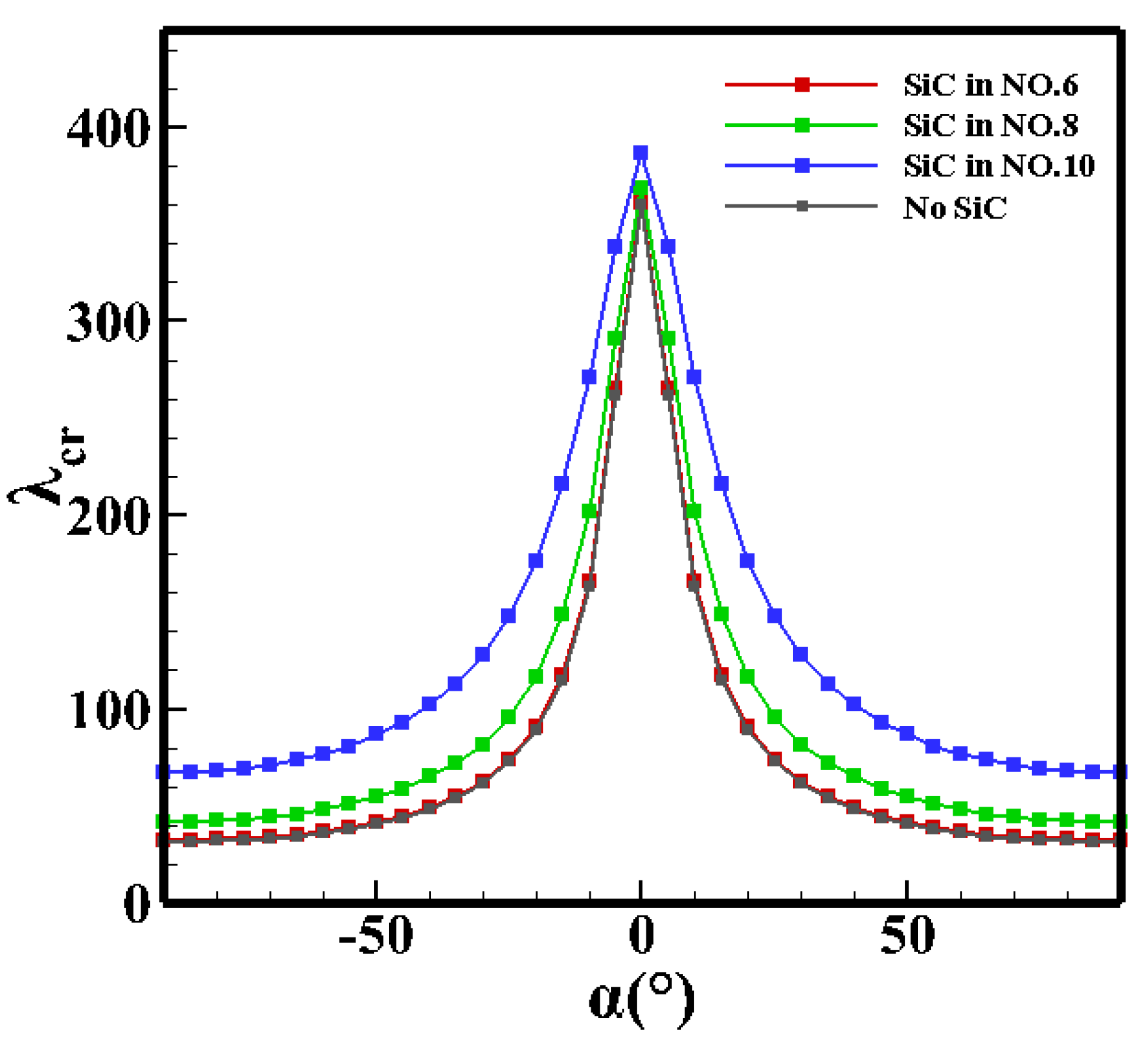
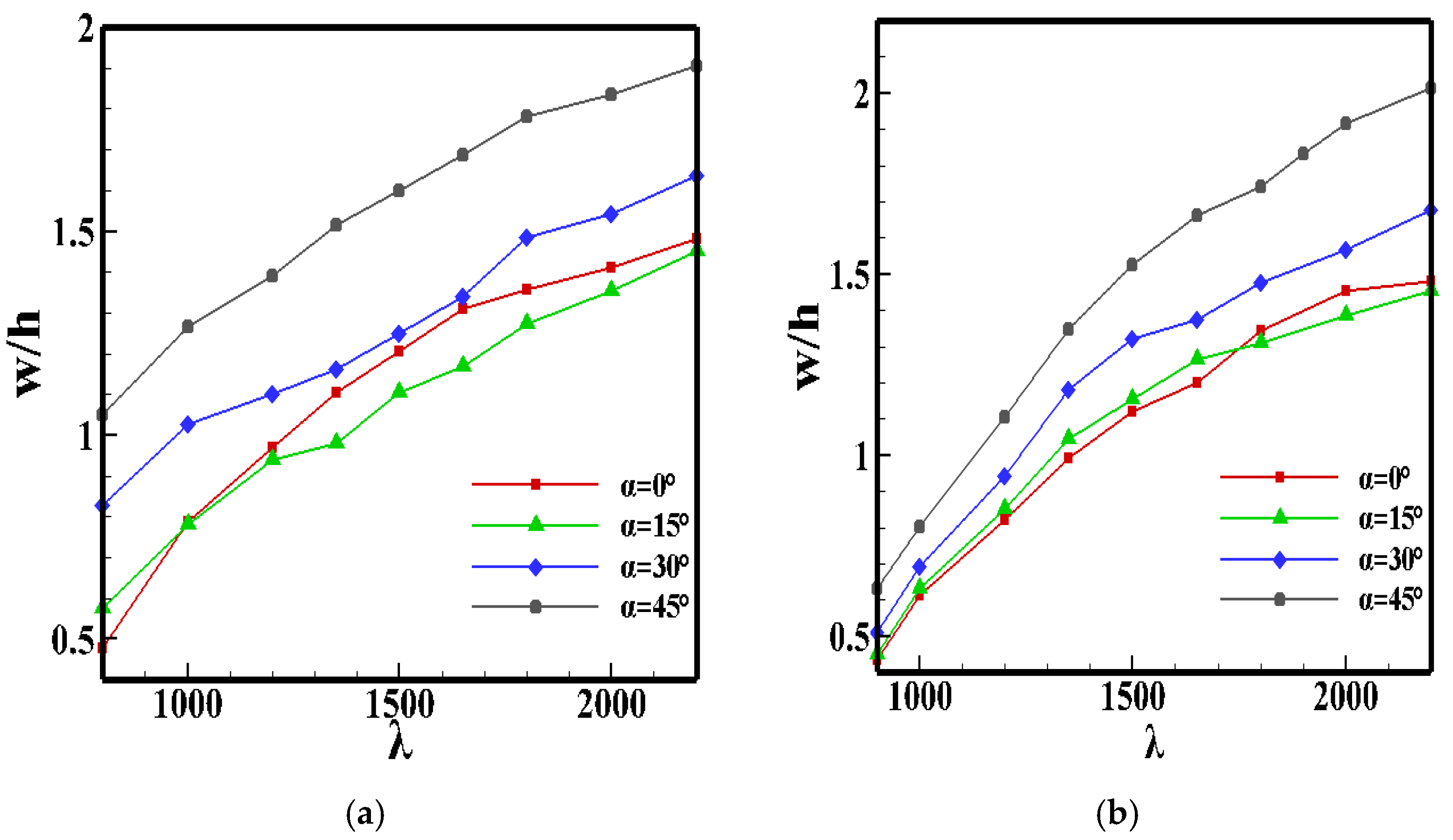




| Yaw Angle (deg) | 0 | 15 | 30 | 45 |
|---|---|---|---|---|
| Monitor point | (0.75 , 0.5 ) | (0.74 , 0.56 ) | (0.72 , 0.63 ) | (0.70, 0.70 ) |
| Material | ||||
|---|---|---|---|---|
| Al | 1.000 | 26.900 | 0.300 | 2700 |
| Graphite–Epoxy AS-3002 | 26.500 | 8.936 | 0.210 | 1600 |
| SiC | 1.169 | 62.900 | 0.270 | 3200 |
| Mode/Layer | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Al | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| SiC | 0 | 15 | −15 | 15 | 30 | −30 | 30 | 45 | −45 | 45 | 58 | 20 | −40 | 7 |
| AS3002 | 0 | 15 | 15 | −15 | 30 | 30 | −30 | 45 | 45 | −45 | 24 | −39 | −57 | 46 |
| Mode/Layer | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 15 | −15 | 15 | 30 | −30 | 30 | 45 | −45 | 45 | 59 | −3 | −42 | −19 |
| 2 | 0 | 15 | 15 | −15 | 30 | 30 | −30 | 45 | 45 | −45 | −56 | −53 | 19 | 55 |
| 3 | 0 | 15 | −15 | 15 | 30 | −30 | 30 | 45 | −45 | 45 | 47 | 22 | 2 | 51 |
| 4 | 0 | 15 | 15 | −15 | 30 | 30 | −30 | 45 | 45 | −45 | 50 | −55 | 57 | −54 |
| 5 | 0 | 15 | −15 | 15 | 30 | −30 | 30 | 45 | −45 | 45 | 36 | −52 | 18 | 29 |
| 6 | 0 | 15 | 15 | −15 | 30 | 30 | −30 | 45 | 45 | −45 | −49 | 3 | 36 | −28 |
| 7 | 0 | 15 | −15 | 15 | 30 | −30 | 30 | 45 | −45 | 45 | −29 | −49 | −6 | −9 |
| 8 | 0 | 15 | 15 | −15 | 30 | 30 | −30 | 45 | 45 | −45 | −20 | 38 | −8 | 6 |
| 9 | 0 | 15 | −15 | 15 | 30 | −30 | 30 | 45 | −45 | 45 | 22 | 18 | 39 | 54 |
| 10 | 0 | 15 | 15 | −15 | 30 | 30 | −30 | 45 | 45 | −45 | −44 | 27 | −50 | −10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, S.; Guo, T.; Wu, J.; Zhou, D.; Shen, E. Analysis of Flutter Characteristics for Composite Laminates in Hypersonic Yawed Flow. Aerospace 2025, 12, 174. https://doi.org/10.3390/aerospace12030174
Cao S, Guo T, Wu J, Zhou D, Shen E. Analysis of Flutter Characteristics for Composite Laminates in Hypersonic Yawed Flow. Aerospace. 2025; 12(3):174. https://doi.org/10.3390/aerospace12030174
Chicago/Turabian StyleCao, Shuang, Tongqing Guo, Jiangpeng Wu, Di Zhou, and Ennan Shen. 2025. "Analysis of Flutter Characteristics for Composite Laminates in Hypersonic Yawed Flow" Aerospace 12, no. 3: 174. https://doi.org/10.3390/aerospace12030174
APA StyleCao, S., Guo, T., Wu, J., Zhou, D., & Shen, E. (2025). Analysis of Flutter Characteristics for Composite Laminates in Hypersonic Yawed Flow. Aerospace, 12(3), 174. https://doi.org/10.3390/aerospace12030174






