An Evaluation of Passive Wall Treatment with Circumferential Grooves at the Casing of the First and Second Blade Rotor Rows of a High-Performance Multi-Stage Axial Compressor
Abstract
1. Introduction
2. Description of the Casing Treatment
2.1. Circumferential Grooves at the Casing of the First Blade Rotor Row
2.2. Circumferential Grooves at the Casing of the Second Blade Rotor Row
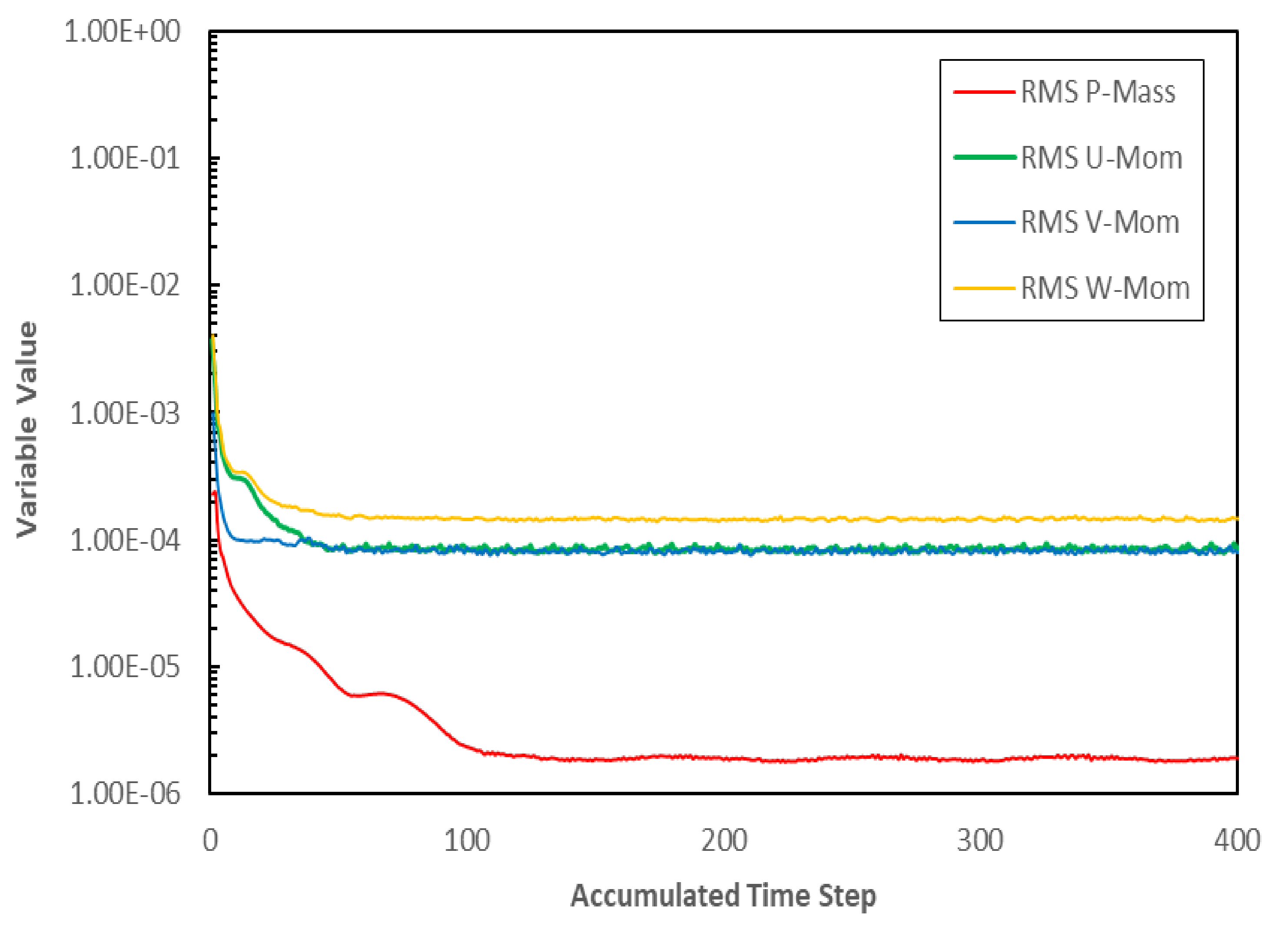
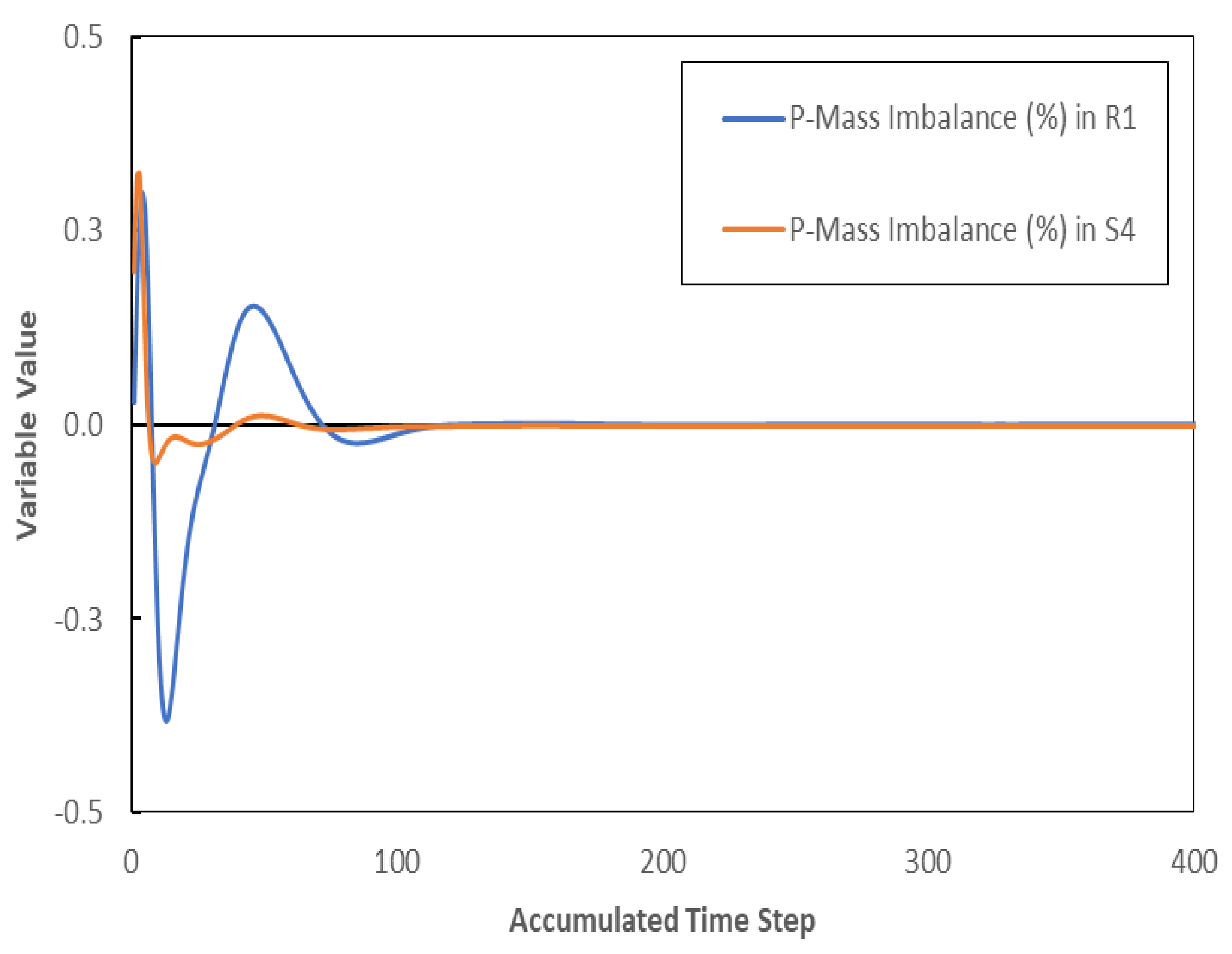
3. Numerical 3D Flow Calculations
3.1. Numerical Scheme Settings
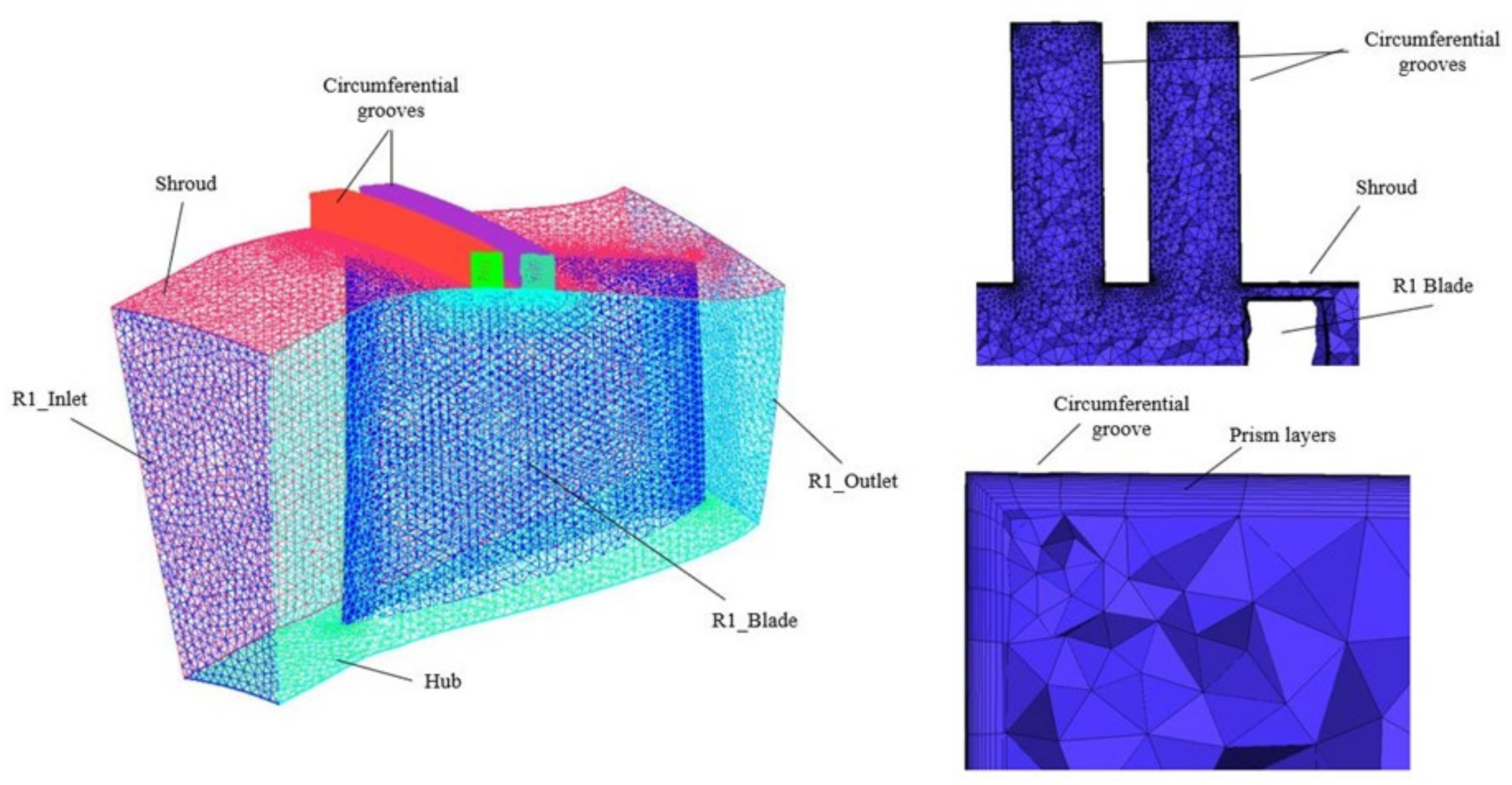
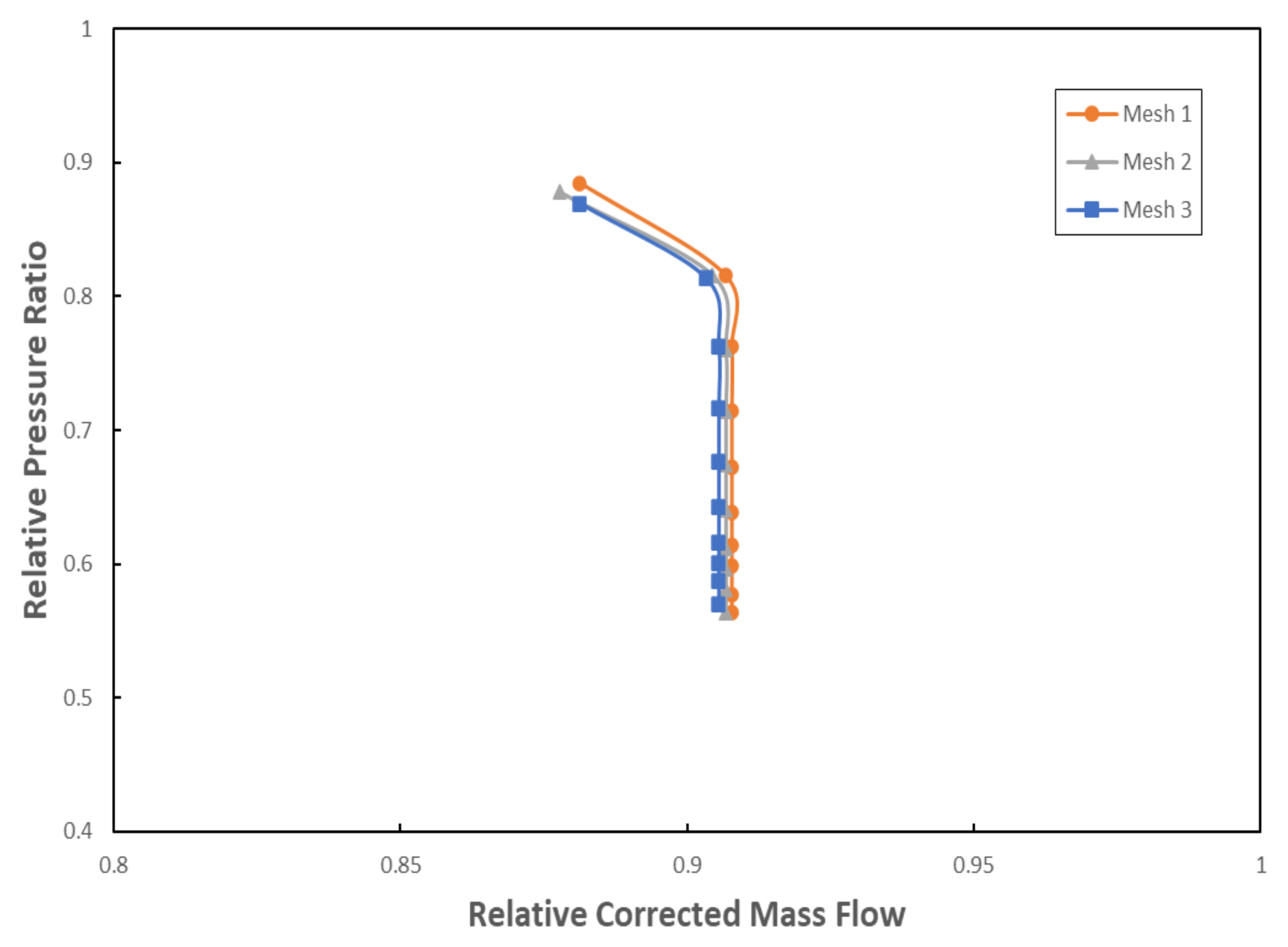
3.2. Mesh Generation and Its Evaluation
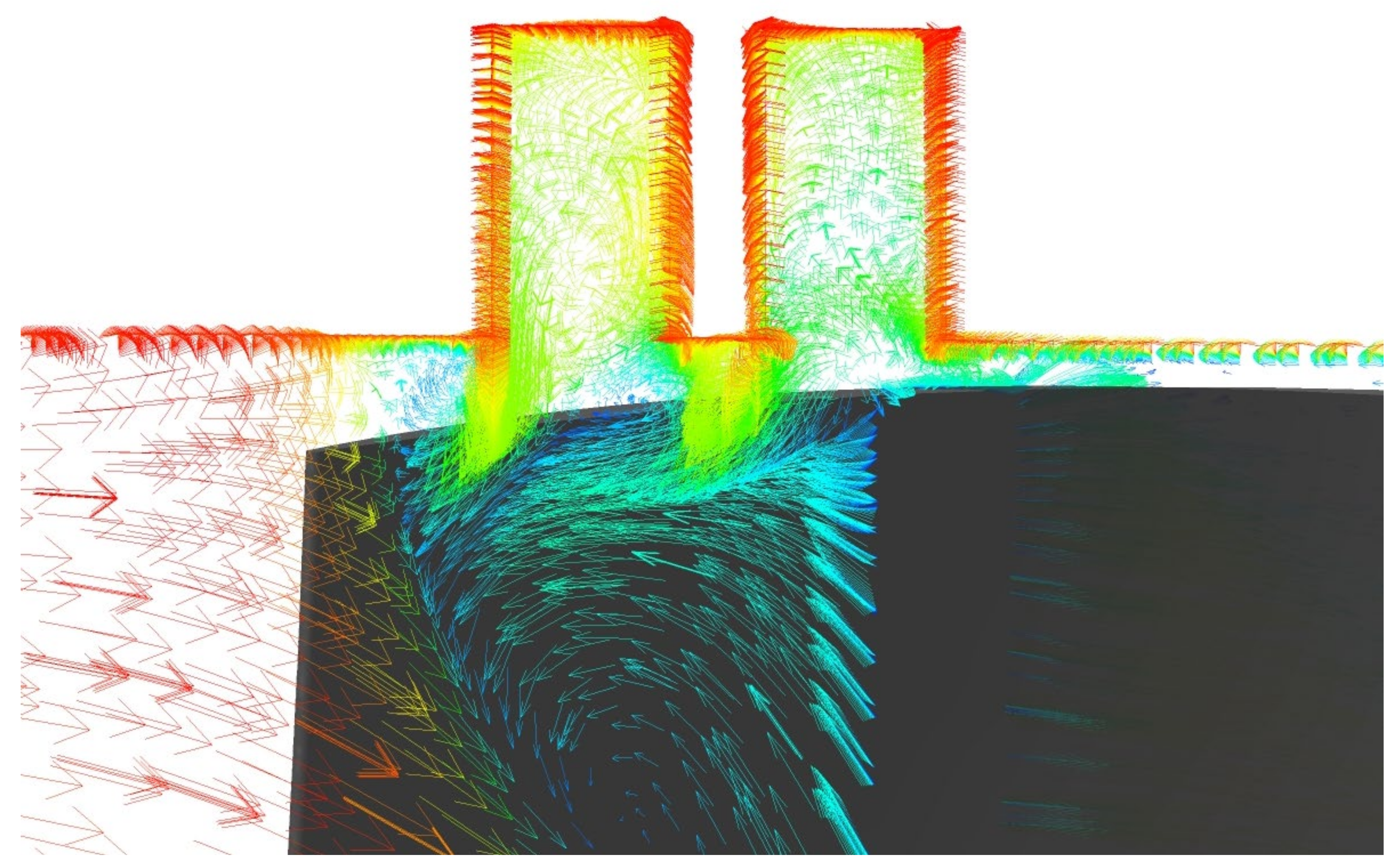
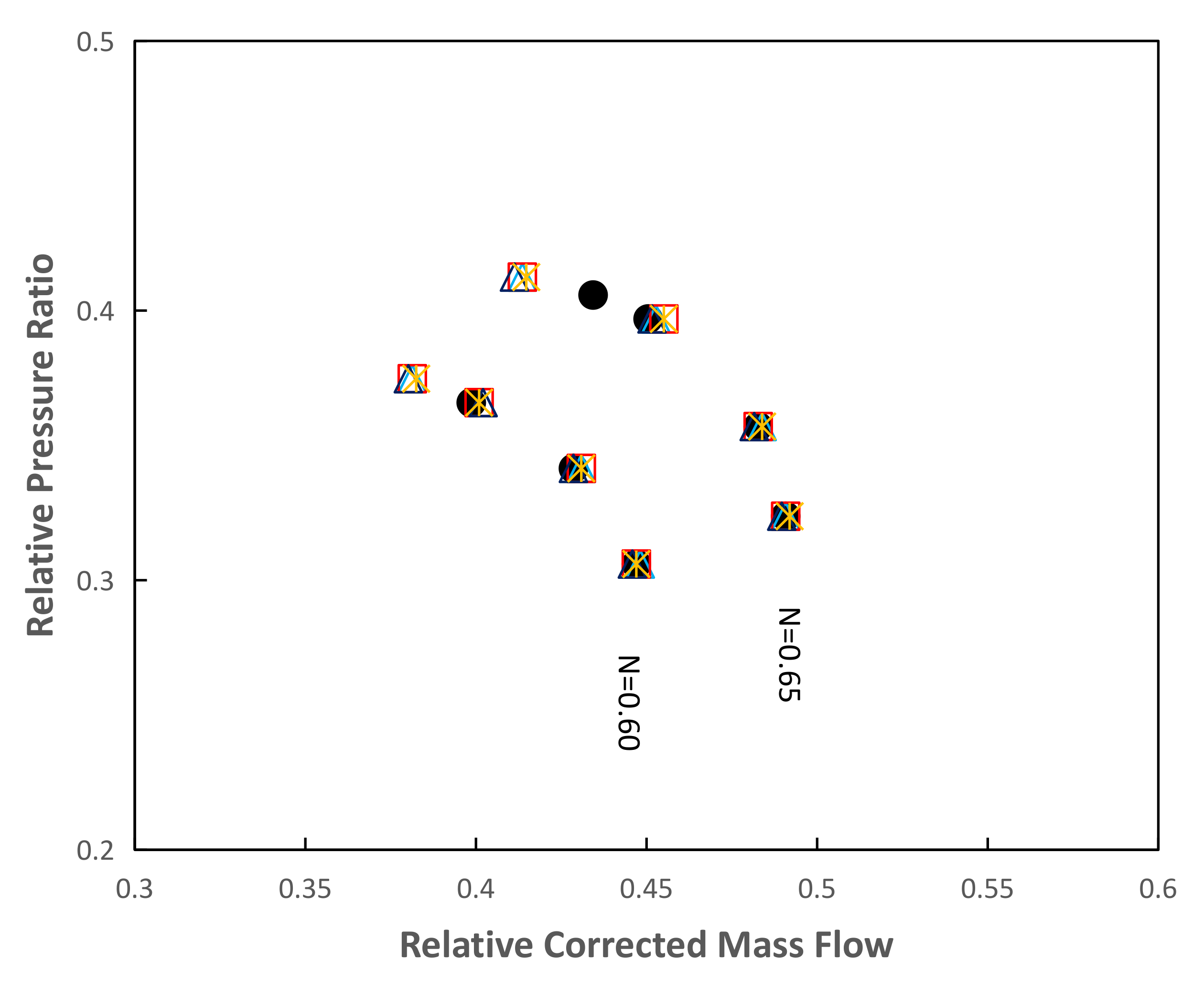
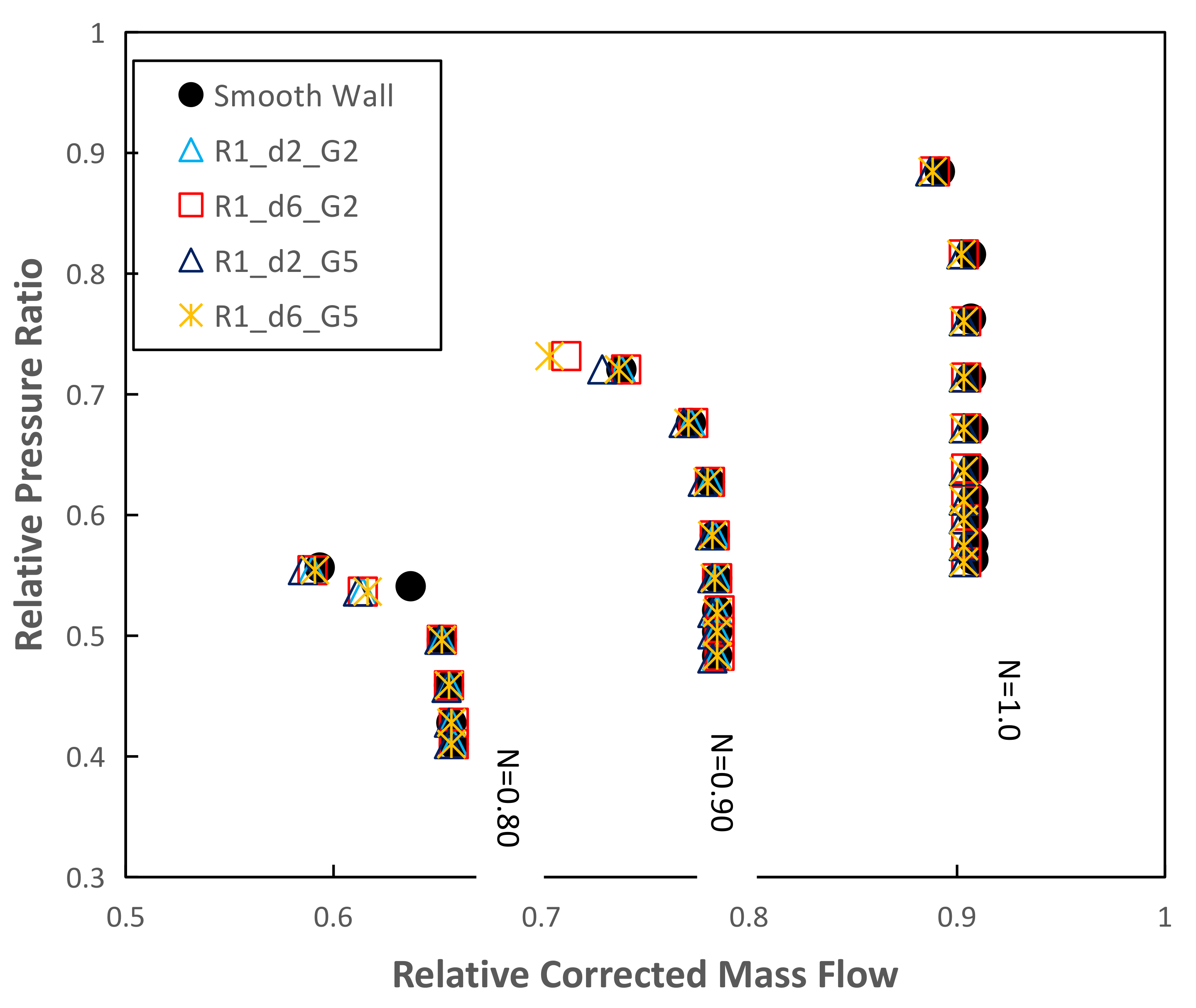
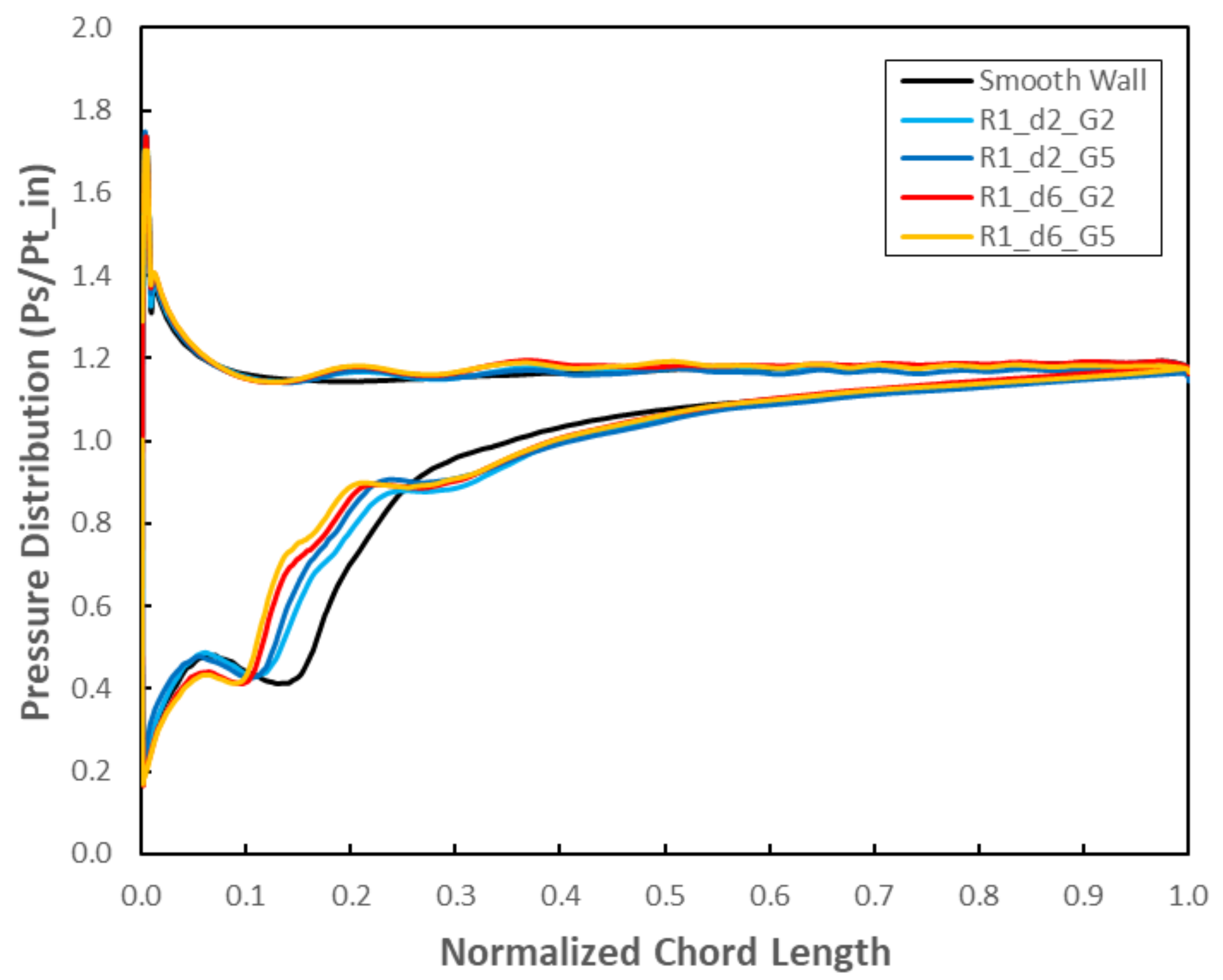
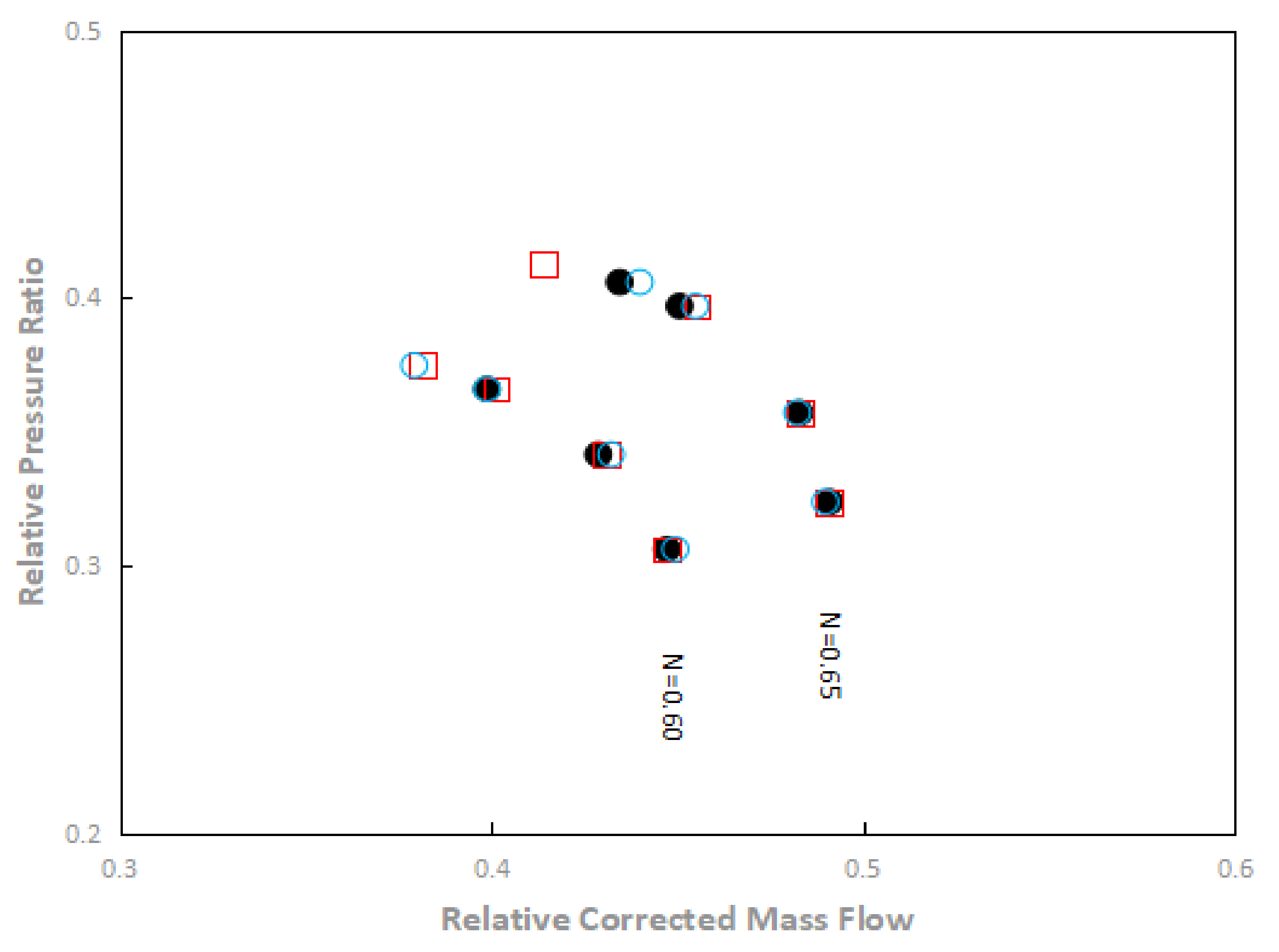
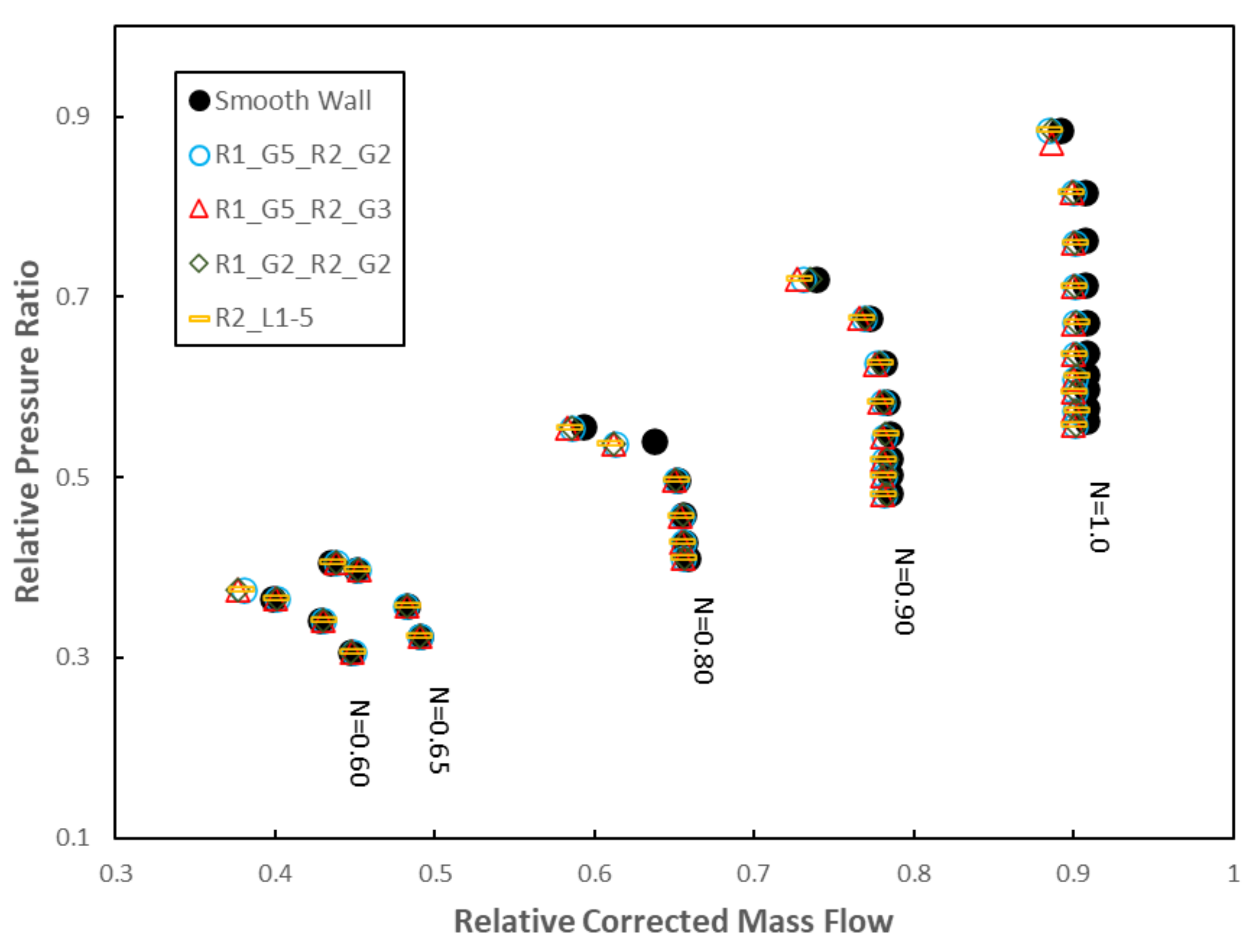
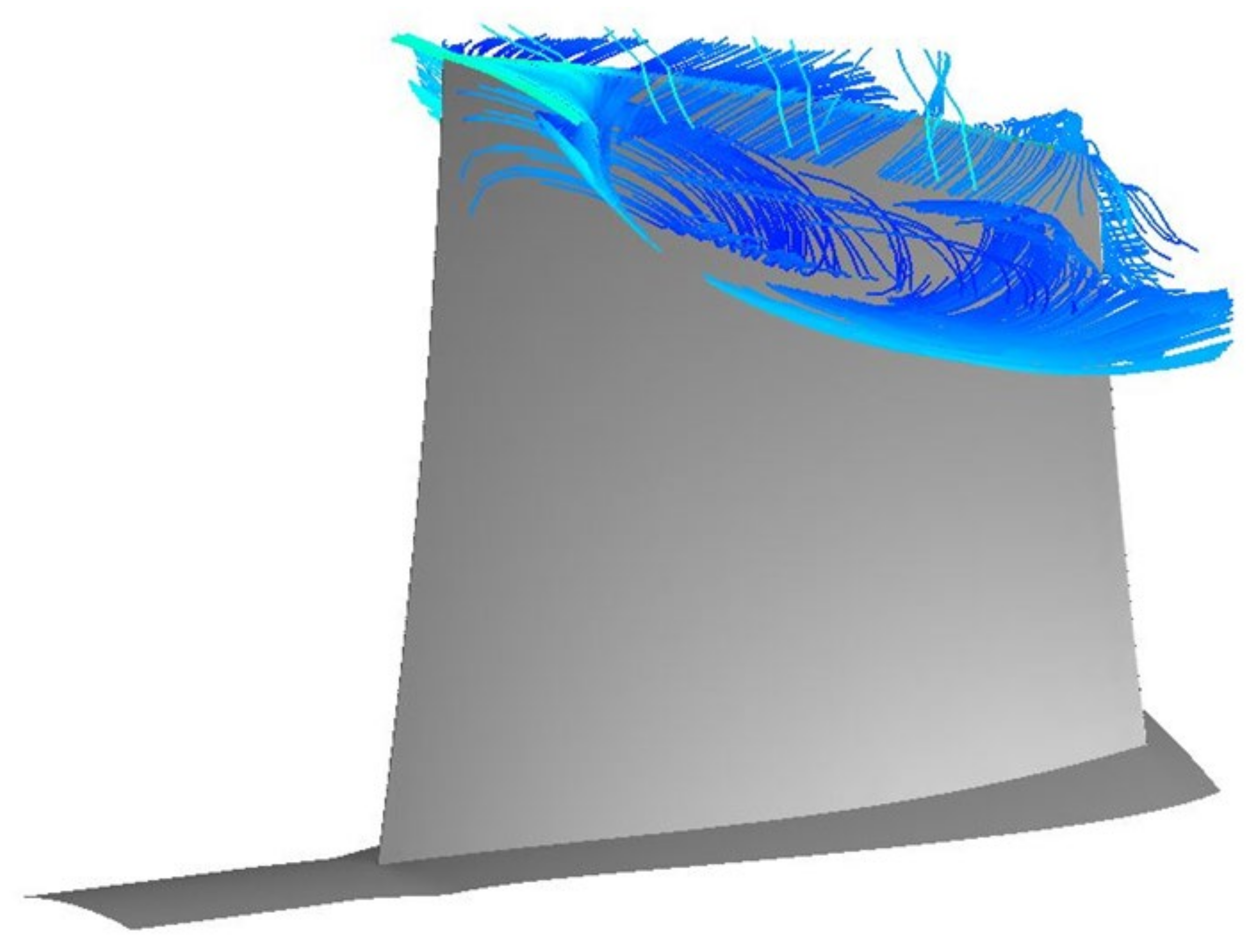
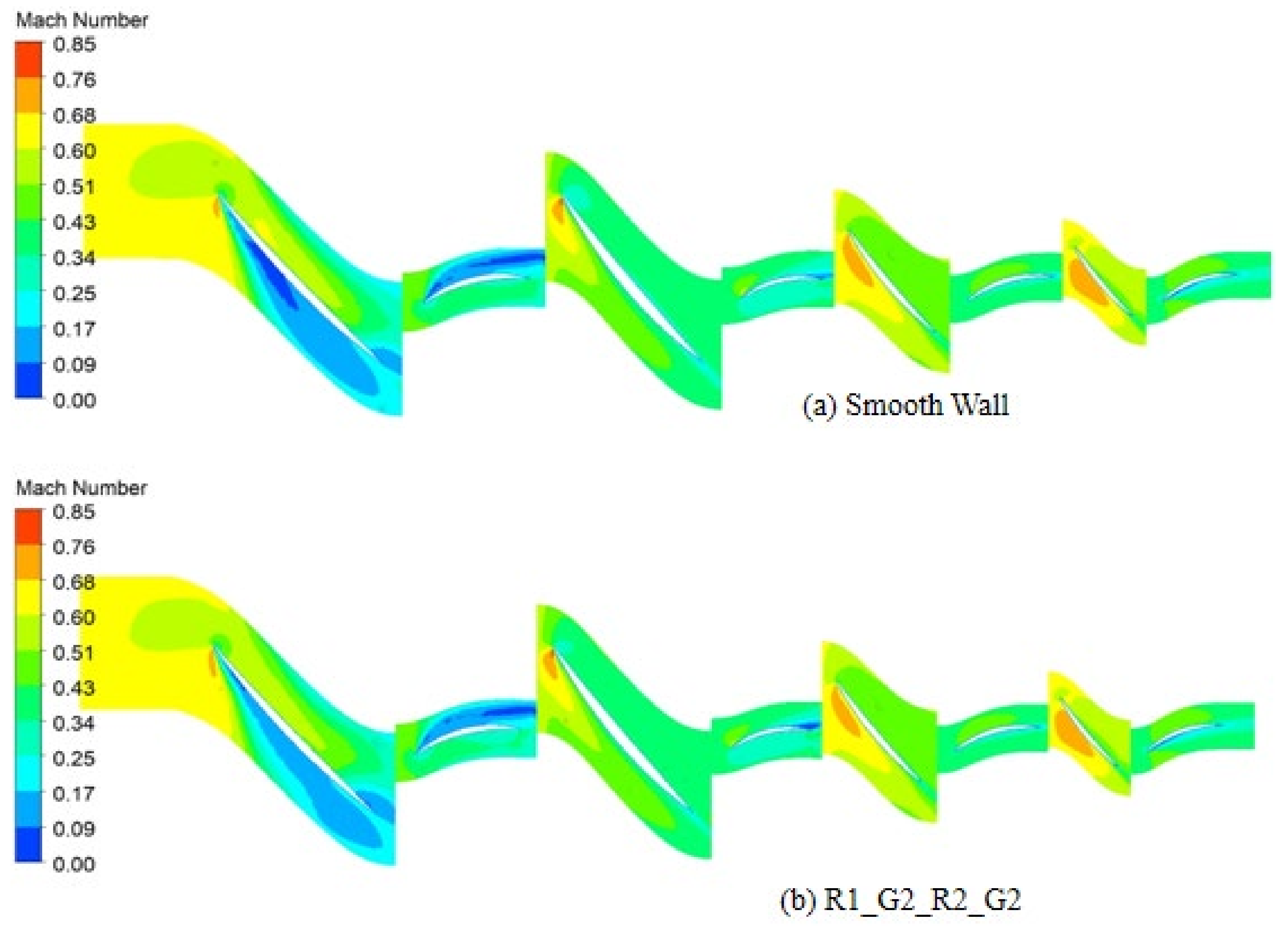
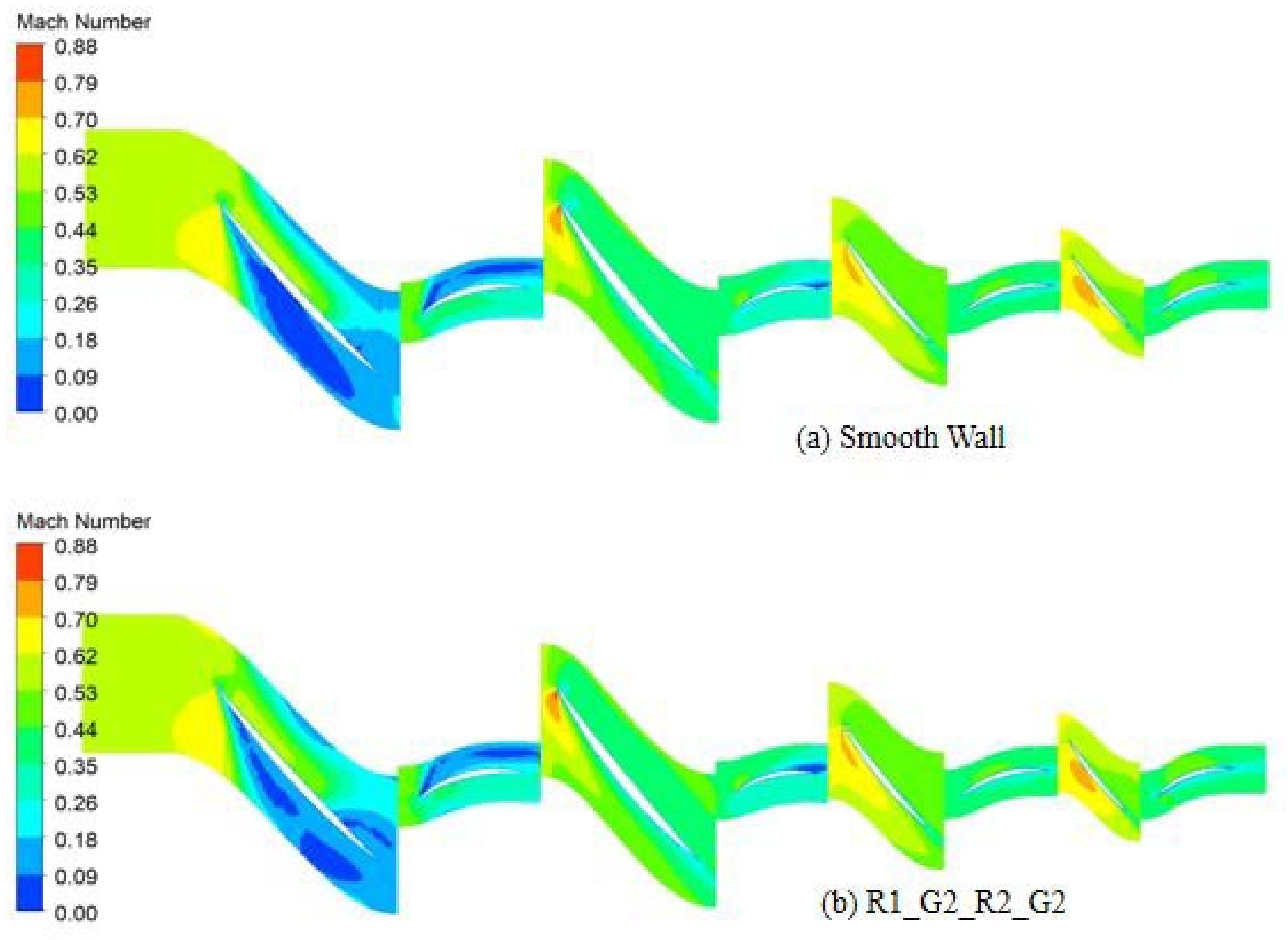
4. Results
4.1. Circumferential Grooves Located at the Casing of the First Blade Rotor Row
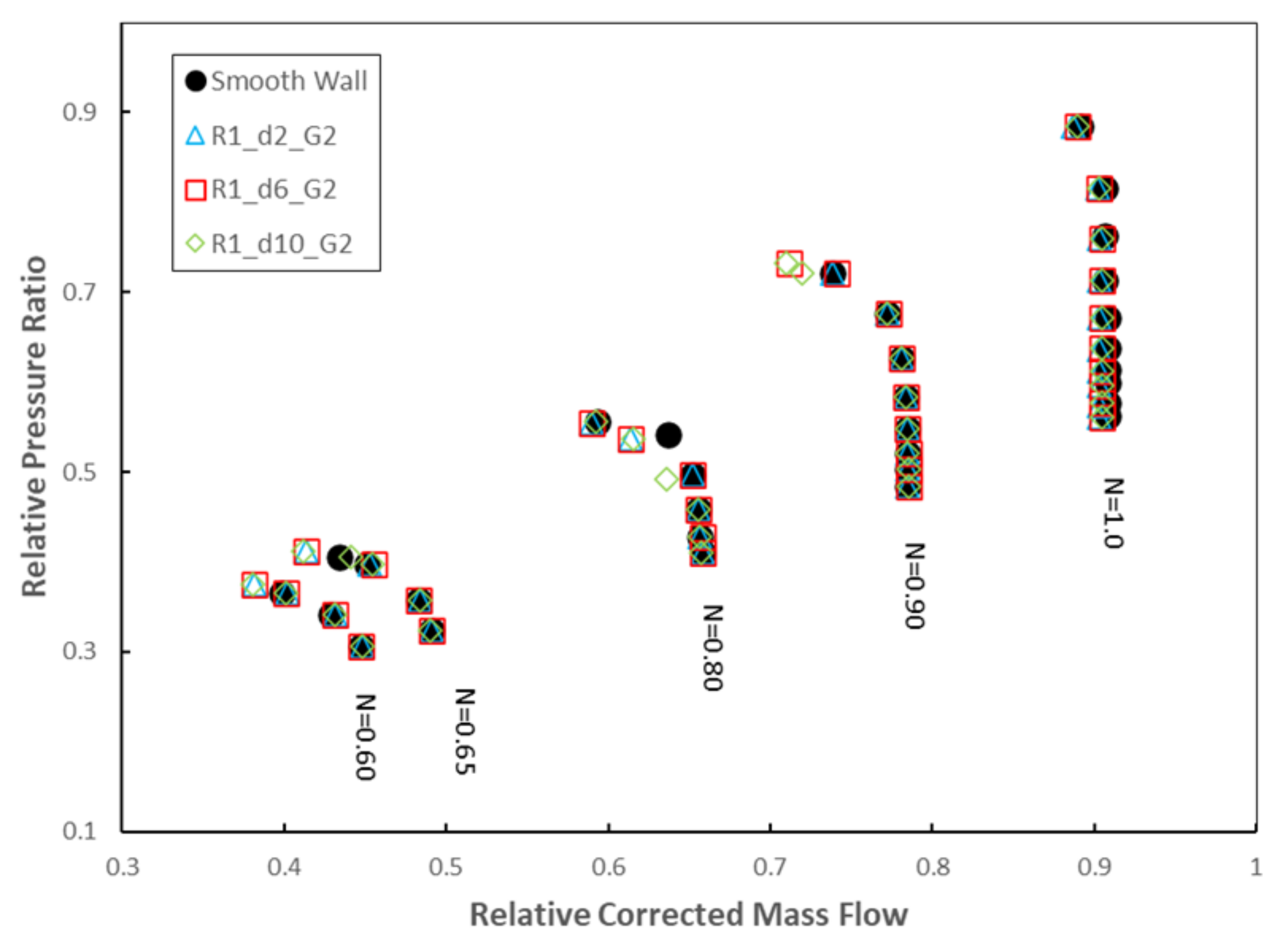
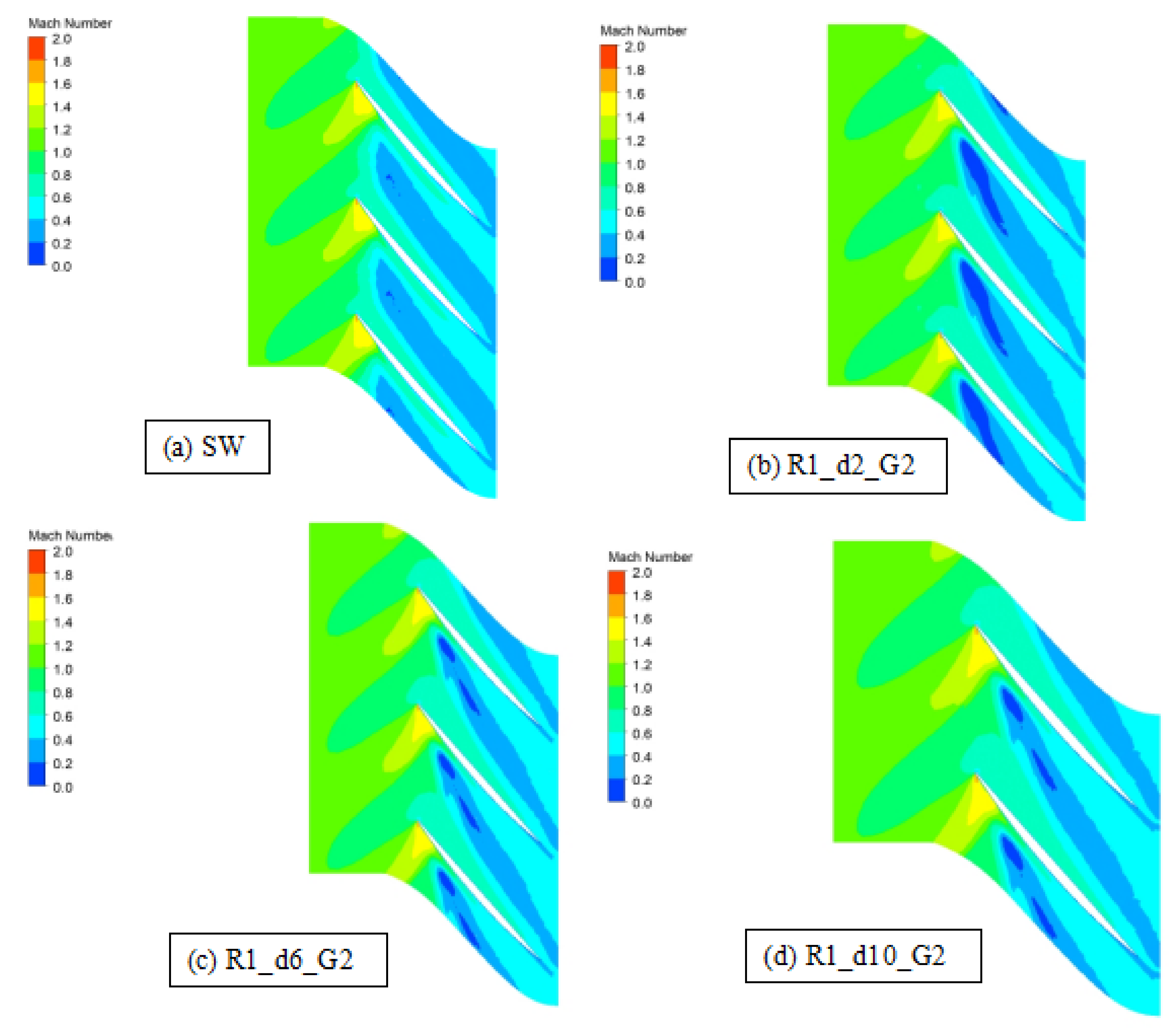
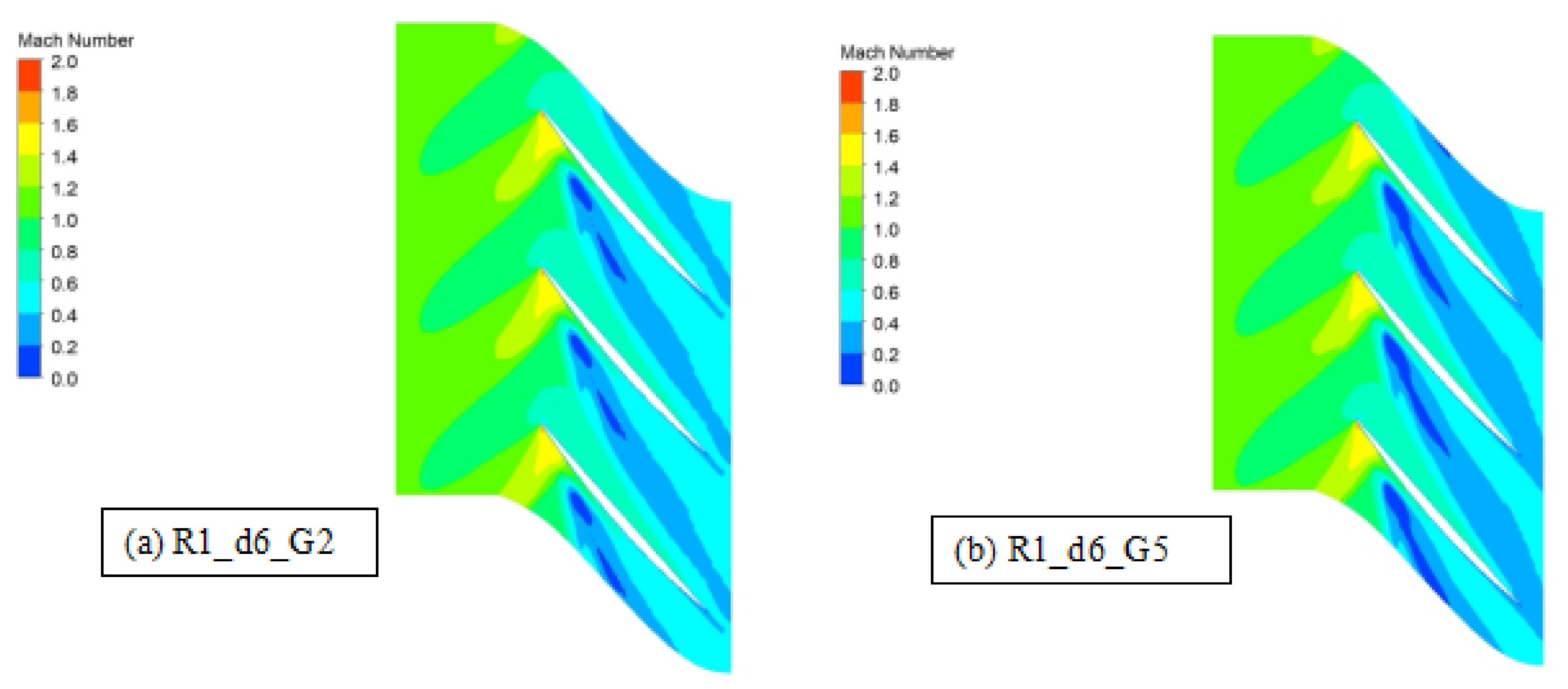
4.1.1. Analysis of the Circumferential Groove Depth
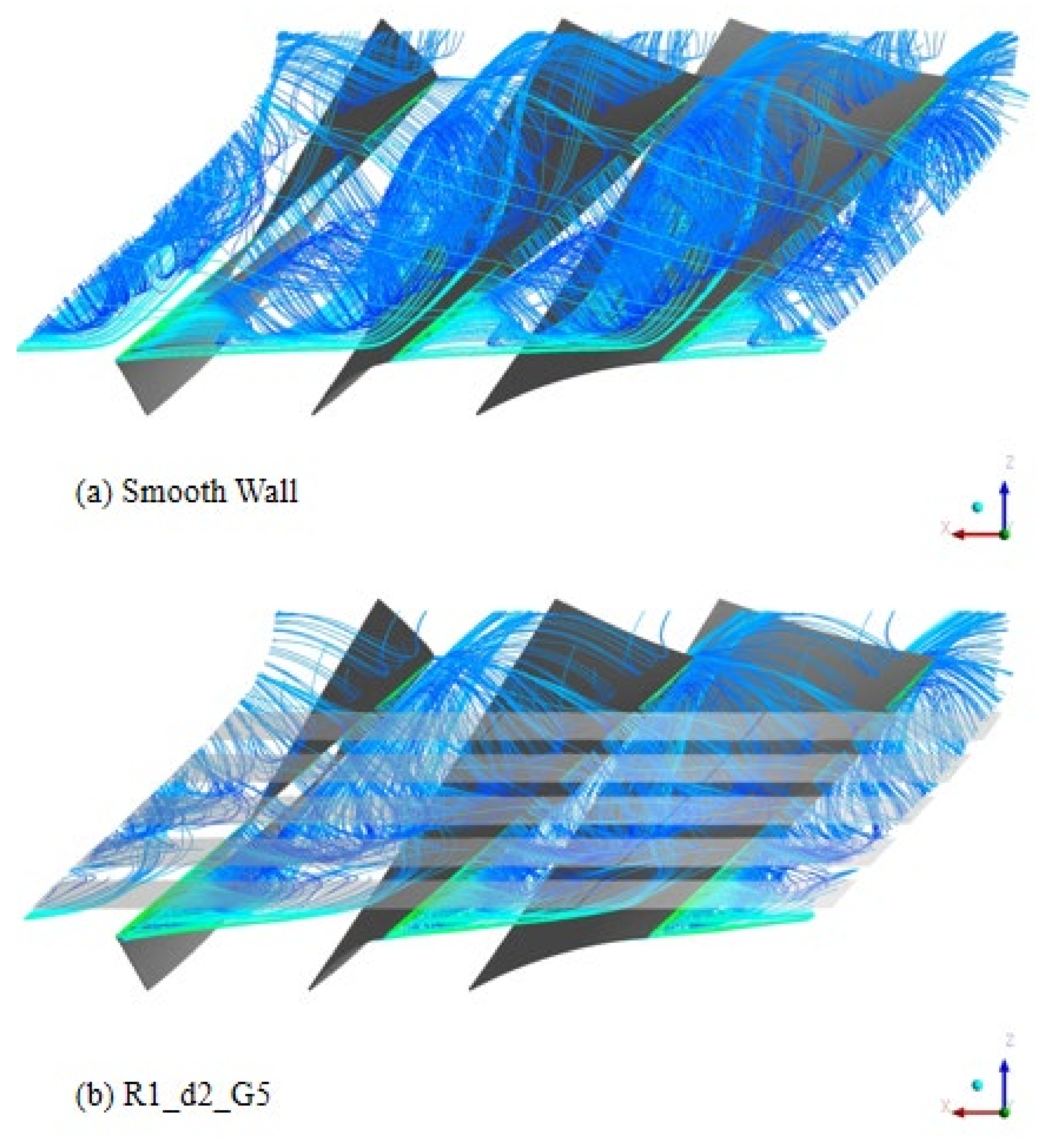
4.1.2. Number of Circumferential Groove Analysis
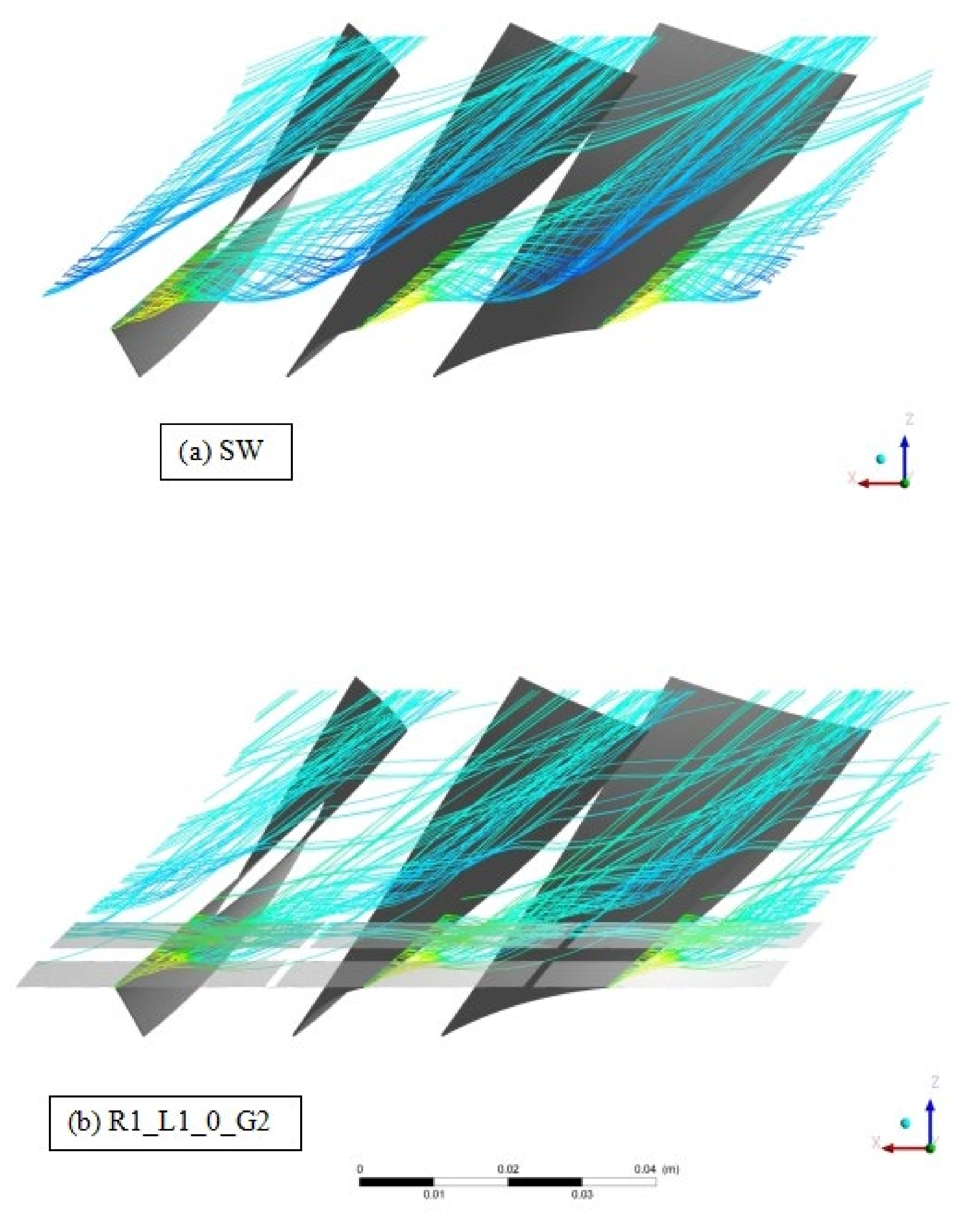
4.1.3. First Circumferential Groove Location Analysis
4.2. Circumferential Grooves Located at the Casing of the Second Blade Rotor Row
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CFD | Computational Fluid Dynamics |
| CT | Casing treatment |
| N | Rotational speed |
| PR | Pressure ratio |
| RANS | Reynolds-Averaged Navier–Stokes |
| RMS | Root Mean Square |
| SM | Stall margin |
| SST | Shear Stress Transport |
| SW | Smooth wall |
| Nomenclature | |
| Cw | Tangential velocity |
| cx | Blade tip axial chord |
| k | Turbulent kinetic energy |
| Stall mass flow | |
| ηis,peak | Isentropic efficiency at peak condition |
| ρ | Density |
| p | Pressure |
| r | Radius |
| ω | Turbulent dissipation rate |
| y+ | Non-dimensional wall distance |
References
- Zhong, J.; Han, S.; Lu, H.; Kan, X. Effect of tip geometry and tip clearance on aerodynamic performance of a linear compressor cascade. Chin. J. Aeronaut. 2013, 26, 583–593. [Google Scholar] [CrossRef]
- Berdanier, R.A.; Smith, N.R.; Young, A.M.; Key, N.L. Effects of Tip Clearance on Stall Inception in a Multistage Compressor. J. Propuls. Power 2018, 34, 308–317. [Google Scholar] [CrossRef]
- Gourdain, N.; Leboeuf, F. Unsteady Simulation of an Axial Compressor Stage with Casing and Blade Passive Treatments. ASME J. Turbomach. 2009, 131, 021013. [Google Scholar] [CrossRef]
- Yoon, S.; Cargill, P. Casing Treatment: Its Potential and Limitations. ASME J. Turbomach. 2023, 145, 041011. [Google Scholar] [CrossRef]
- Booth, T.C.; Dodge, P.R.; Hepworth, H.K. Rotor-Tip Leakage: Part I—Basic Methodology. J. Eng. Power 1982, 104, 154–161. [Google Scholar] [CrossRef]
- Wadia, A.R.; Booth, T.C. Rotor-Tip Leakage: Part II—Design Optimization Through Viscous Analysis and Experiment. J. Eng. Power 1982, 104, 162–169. [Google Scholar] [CrossRef]
- Adamczyk, J.J.; Celestina, M.L.; Greitzer, E.M. The Role of Tip Clearance in High-Speed Fan Stall. J. Turbomach 1993, 115, 28–38. [Google Scholar] [CrossRef]
- Furukawa, M.; Inoue, M.; Saiki, K.; Yamada, K. The Role of Tip Leakage Vortex Breakdown in Compressor Rotor Aerodynamics. J. Turbomach 1999, 121, 469–480. [Google Scholar] [CrossRef]
- Chen, H.; Huang, X.; Shi, K.; Fu, S.; Ross, M.; Bennington, M.A.; Cameron, J.D.; Morris, S.C.; McNulty, S.; Wadia, A. A Computational Fluid Dynamics Study of Circumferential Groove Casing Treatment in a Transonic Axial Compressor. J. Turbomach. 2014, 136, 031003. [Google Scholar] [CrossRef]
- Kau, H.-P.; Brignole, G.; Wilke, I. Designing a Casing Treatment for a Transonic Axal Compressor; Lecture Series; von Karman Institute for Fluid Dynamics: Sint-Genesius-Rode, Belgium, 2006. [Google Scholar]
- Wang, W.; Chu, W.; Zhang, H.; Kuang, H. Experimental and numerical study of tip injection in a subsonic axial flow compressor. Chin. J. Aeronaut. 2017, 30, 907–917. [Google Scholar] [CrossRef]
- Li, J.; Liu, Y.; Du, J.; Zhang, H.; Nie, C. Implementation of stability-enhancement with tip air injection in a multi-stage axial flow compressor. Aerosp. Sci. Technol. 2021, 113, 106646. [Google Scholar] [CrossRef]
- Yoon, S.; Cargill, P. Casing Treatment: Its Potential and Limitations. In Volume 10A: Turbomachinery—Axial Flow Fan and Compressor Aerodynamics, Proceedings of ASME Turbo Expo 2022: Turbomachinery Technical Conference and Exposition, Rotterdam, The Netherlands, 13–17 June 2022; American Society of Mechanical Engineers: New York, NY, USA, 2022. [Google Scholar] [CrossRef]
- Alone, D.B.; Kumar, S.S.; Shobhavathy, M.; Mudipalli, J.R.R.; Pradeep, A.; Ramamurthy, S.; Iyengar, V.S. Experimental assessment on effect of lower porosities of bend skewed casing treatment on the performance of high speed compressor stage with tip critical rotor characteristics. Aerosp. Sci. Technol. 2017, 60, 193–202. [Google Scholar] [CrossRef]
- Lu, B.; Zhu, M.; Teng, J.; Qiang, X. Design optimization of axial slot casing treatment in a highly-loaded mixed-flow compressor. Aerosp. Sci. Technol. 2020, 107, 106262. [Google Scholar] [CrossRef]
- Lu, B.; Zhu, M.; Teng, J.; Qiang, X. Design strategy of axial slot casing treatment for a transonic compressor rotor based on parametric analysis. Aerosp. Sci. Technol. 2021, 119, 107142. [Google Scholar] [CrossRef]
- Du, J.; Qiu, J.; Zhang, Q.; Ba, D.; Maroldt, N.; Seume, J.R. Unsteady Interaction Mechanisms of Axial-Slot Casing Treatment with Tip Region Flow in a Highly-Loaded Mixed-Flow Compressor. J. Eng. Gas. Turbine Power 2022, 144, 091001. [Google Scholar] [CrossRef]
- Legras, G.; Gourdain, N.; Sicot, F.; Roumeas, M. Time Spectral Calculation of a Casing Treatment Configuration for a High Pressure Compressor. In Proceedings of the 8th European Turbomachinery Conference, Graz, Austria, 23–27 March 2009. [Google Scholar]
- Mao, X.; Liu, B.; Zhao, H. Numerical analysis of the circumferential grooves casing treatment in a counter-rotating axial flow compressor. Appl. Therm. Eng. 2018, 130, 29–39. [Google Scholar] [CrossRef]
- Rolfes, M.; Lange, M.; Vogeler, K.; Mailach, R. Experimental and Numerical Investigation of a Circumferential Groove Casing Treatment in a Low-Speed Axial Research Compressor at Different Tip Clearances. J. Turbomach. 2017, 139, 121009. [Google Scholar] [CrossRef]
- Smith, G.D.J.; Cumpsty, N.A. Flow Phenomena in Compressor Casing Treatment. J. Eng. Gas. Turbine Power 1984, 106, 532–541. [Google Scholar] [CrossRef]
- Legras, G.; Gourdain, N.; Trebinjac, I. Numerical analysis of the tip leakage flow field in a transonic axial compressor with circumferential casing treatment. J. Therm. Sci. 2010, 19, 198–205. [Google Scholar] [CrossRef]
- Rabe, D.C.; Hah, C. Application of Casing Circumferential Grooves for Improved Stall Margin in a Transonic Axial Compressor. In Proceedings of the ASME Turbo Expo 2002: Power for Land, Sea, and Air, Amsterdam, The Netherlands, 3–6 June 2002; American Society of Mechanical Engineers Digital Collection: New York, NY, USA, 2002; pp. 1141–1153. [Google Scholar] [CrossRef]
- Lu, X.; Chu, W.; Zhu, J.; Wu, Y. Mechanism of the interaction between casing treatment and tip leakage flow in a subsonic axial compressor. In Proceedings of the ASME Turbo Expo 2006: Power for Land, Sea, and Air, Barcelona, Spain, 8–11 May 2006; American Society of Mechanical Engineers Digital Collection: New York, NY, USA, 2006; pp. 79–90. [Google Scholar] [CrossRef]
- Sakuma, Y.; Watanabe, T.; Himeno, T.; Kato, D.; Murooka, T.; Shuto, Y. Numerical Analysis of Flow in a Transonic Compressor with a Single Circumferential Casing Groove: Influence of Groove Location and Depth on Flow Instability. J. Turbomach. 2014, 136, 031017. [Google Scholar] [CrossRef]
- Mirzabozorg, M.A.S.; Bazazzadeh, M.; Hamzezade, M. Numerical Study on the Effect of Single Shallow Circumferential Groove Casing Treatment on the Flow Field and the Stability of a Transonic Compressor. J. Appl. Fluid. Mech. 2017, 10, 257–265. [Google Scholar] [CrossRef]
- Lin, W.; Sreenivas, K.; Webster, R.S.; Hyams, D.G. Effect of Casing Groove Locations on the Performance of an Axial Flow Stage. In Proceedings of the 49th AIAA/ASME/SAE/ASEE Joint Propulsion Conference, San Jose, CA, USA, 14–17 July 2013; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2013. [Google Scholar] [CrossRef]
- Shabbir, A.; Adamczyk, J.J. Flow Mechanism for Stall Margin Improvement due to Circumferential Casing Grooves on Axial Compressors. J. Turbomach. 2005, 127, 708–717. [Google Scholar] [CrossRef]
- Hathaway, M.D. Passive Endwall Treatments for Enhancing Stability; NASA/TM-2007-214409, ARL-TR-3878; NASA: Washington, DC, USA, 2007.
- Silva, L.M.; Tomita, J.T.; Bringhenti, C.; Whitacker, L.H.L.; Almeida, L.E.N.; Cavalca, D.F. A Small Jet Engine Preliminary Design and its Performance Calculations. In Proceedings of the 24th ISABE Conference, Canberra, Australia, 22–27 September 2019. [Google Scholar]
- Diaz, R.B.; Tomita, J.T.; Bringhenti, C.; Cavalca, D.F. Improvements in a Multistage Axial-Flow Compressor Design and its Operation on a Small Gas Turbine Performance. In Proceedings of the 25th ISABE Conference, Ottawa, ON, Canada, 25–30 September 2022. [Google Scholar]
- Qiang, X.-Q.; Zhu, M.-M.; Teng, J.-F. Effect of circumferential grooves casing treatment on tip leakage flow and loss in a transonic mixed-flow compressor. J. Theor. Appl. Mech. 2013, 51, 903–913. [Google Scholar]
- Rhie, C.M.; Chow, W.L. Numerical study of the turbulent flow past an airfoil with trailing edge separation. AIAA J. 1983, 21, 1525–1532. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
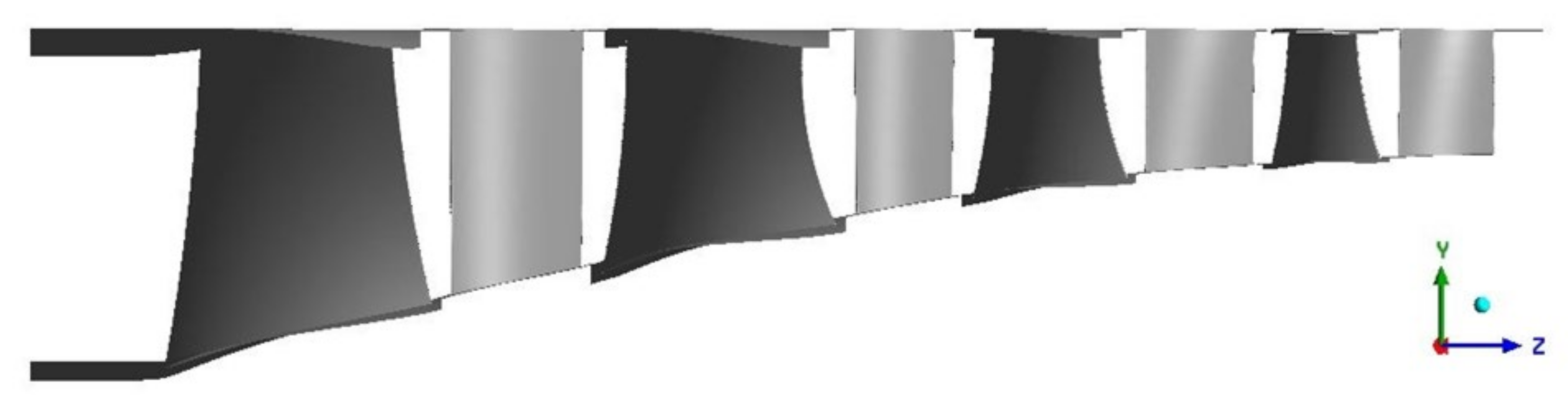

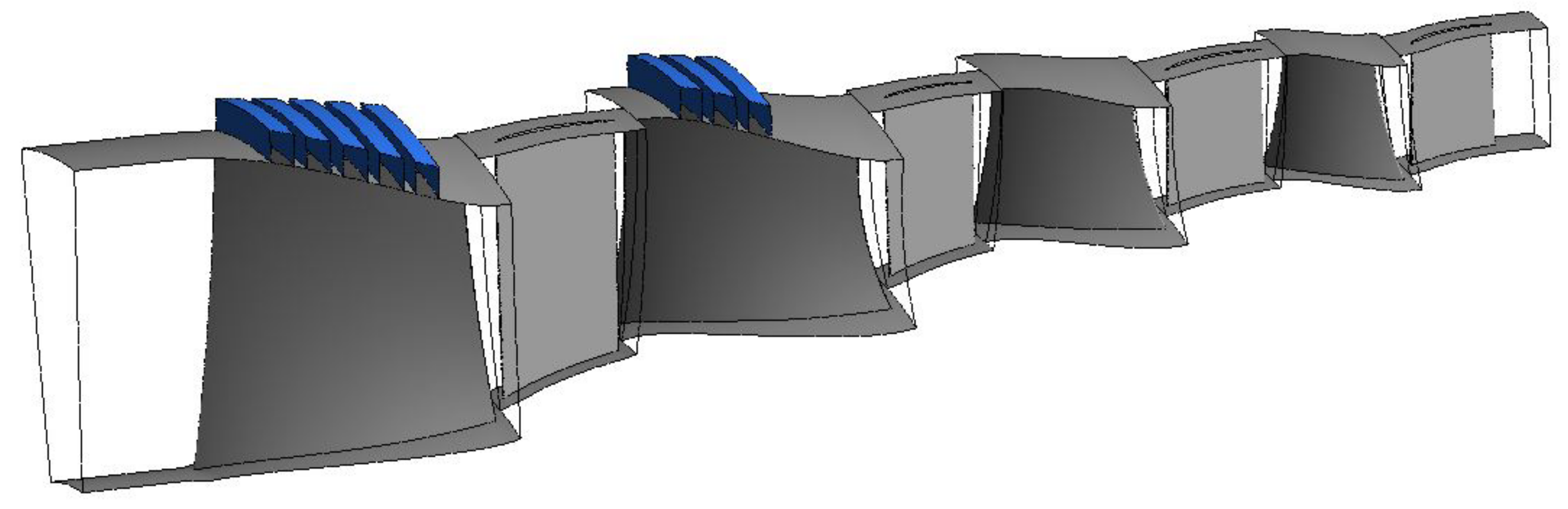










| R1_d2_G2 | R1_d2_G5 | R1_d6_G2 | R1_d6_G5 | R1_d10_G2 | R1_d6_G2_L1_0 | |
|---|---|---|---|---|---|---|
| d | 2 mm | 2 mm | 6 mm | 6 mm | 10 mm | 6 mm |
| w | 10% cx | 10% cx | 10% cx | 10% cx | 10% cx | 10% cx |
| s | 5% cx | 5% cx | 5% cx | 5% cx | 5% cx | 5% cx |
| L1 | 10% cx | 10% cx | 10% cx | 10% cx | 10% cx | 0% cx |
| Number of grooves | 2 | 5 | 2 | 5 | 2 | 2 |
| R1_d6_G2_ R2_d6_G2 | R1_d6_G5_ R2_d6_G2 | R1_d6_G5_ R2_d6_G3 | R1_d6_G2_ R2_L1_-5 | |
|---|---|---|---|---|
| d | 6 mm | 6 mm | 6 mm | 6 mm |
| w | 10% cx | 10% cx | 10% cx | 10% cx |
| s | 5% cx | 5% cx | 5% cx | 5% cx |
| L1 in R2 | 10% cx | 10% cx | 10% cx | −5% cx |
| Number of grooves in R1 | 2 | 5 | 5 | 5 |
| Number of grooves in R2 | 2 | 2 | 3 | 2 |
| Number of Elements | Number of Prism Layers | Initial Height (m) | y+ | |
|---|---|---|---|---|
| Mesh 1 | 13.0 M | 20 | 1 × 10−6 | <2 |
| Mesh 2 | 16.4 M | 30 | 1 × 10−6 | <2 |
| Mesh 3 | 29.2 M | 30 | 5 × 10−7 | <1 |
| R1_d2_G2 | R1_d6_G2 | R1_d10_G2 | ||||
|---|---|---|---|---|---|---|
| ΔSM | Δηpeak | ΔSM | Δηpeak | ΔSM | Δηpeak | |
| N = 0.60 | +7.07% | +0.32% | +7.07% | −0.08% | +7.39% | −0.21% |
| N = 0.65 | +6.74% | +0.08% | +6.74% | −0.04% | +7.15% | +0.40% |
| N = 0.80 | +0.19% | −1.03% | +0.19% | −1.05% | +0.13% | −0.86% |
| N = 0.90 | +0.16% | −0.12% | +5.48% | −0.12% | +5.82% | −0.14% |
| N = 1.0 | +0.52% | −0.32% | +0.26% | −0.14% | +0.26% | −0.04% |
| R1_d2_G2 | R1_d2_G5 | |||
|---|---|---|---|---|
| ΔSM | Δηpeak | ΔSM | Δηpeak | |
| N = 0.60 | +7.07% | +0.32% | +7.39% | −0.01% |
| N = 0.65 | +6.74% | +0.08% | +7.33% | −0.22% |
| N = 0.80 | +0.19% | −1.03% | +0.97% | −1.21% |
| N = 0.90 | +0.16% | −0.12% | +1.42% | −0.32% |
| N = 1.0 | +0.52% | −0.32% | +0.52% | −0.32% |
| R1_d6_G2 | R1_d6_G5 | |||
|---|---|---|---|---|
| ΔSM | Δηpeak | ΔSM | Δηpeak | |
| N = 0.60 | +7.07% | −0.08% | +6.74% | −0.24% |
| N = 0.65 | +6.74% | −0.04% | +6.44% | −0.38% |
| N = 0.80 | +0.19% | −1.05% | −0.01% | −1.03% |
| N = 0.90 | +5.48% | −0.12% | +6.69% | −0.24% |
| N = 1.0 | +0.26% | −0.14% | +0.39% | −0.22% |
| R1_d6_G2 | R1_L1_0_G2 | |||
|---|---|---|---|---|
| ΔSM | Δηpeak | ΔSM | Δηpeak | |
| N = 0.60 | +7.07% | −0.08% | +7.72% | −0.07% |
| N = 0.65 | +6.74% | −0.04% | +6.72% | −0.05% |
| N = 0.80 | +0.19% | −1.05% | +0.58% | −1.09% |
| N = 0.90 | +5.48% | −0.12% | +4.47% | −0.14% |
| N = 1.0 | +0.26% | −0.14% | +0.51% | −0.27% |
| R1_G5_R2_G2 | R1_G5_R2_G3 | |||
|---|---|---|---|---|
| ΔSM | Δηpeak | ΔSM | Δηpeak | |
| N = 0.60 | +7.39% | +0.17% | +8.38% | −0.29% |
| N = 0.65 | −0.79% | +0.04% | −0.79% | −0.12% |
| N = 0.80 | +0.97% | −1.34% | +1.37% | −1.46% |
| N = 0.90 | +1.26% | −0.62% | +1.74% | −0.69% |
| N = 1.0 | +0.78% | −0.62% | −0.86% | −0.72% |
| R1_G2_R2_G2 | R2_L1-5 | |||
|---|---|---|---|---|
| ΔSM | Δηpeak | ΔSM | Δηpeak | |
| N = 0.60 | +8.38% | −0.60% | +7.83% | −0.36% |
| N = 0.65 | −0.79% | −0.29% | −0.21% | −0.38% |
| N = 0.80 | +0.97% | −1.37% | +0.97% | −1.26% |
| N = 0.90 | +0.63% | −0.48% | +1.62% | −0.74% |
| N = 1.0 | +0.65% | −0.56% | +0.78% | −0.62% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Diaz, R.B.; Tomita, J.T.; Bringhenti, C.; Silva, F.J.d.S.; Cavalca, D.F. An Evaluation of Passive Wall Treatment with Circumferential Grooves at the Casing of the First and Second Blade Rotor Rows of a High-Performance Multi-Stage Axial Compressor. Aerospace 2024, 11, 662. https://doi.org/10.3390/aerospace11080662
Diaz RB, Tomita JT, Bringhenti C, Silva FJdS, Cavalca DF. An Evaluation of Passive Wall Treatment with Circumferential Grooves at the Casing of the First and Second Blade Rotor Rows of a High-Performance Multi-Stage Axial Compressor. Aerospace. 2024; 11(8):662. https://doi.org/10.3390/aerospace11080662
Chicago/Turabian StyleDiaz, Ruben Bruno, Jesuino Takachi Tomita, Cleverson Bringhenti, Franco Jefferds dos Santos Silva, and Diogo Ferraz Cavalca. 2024. "An Evaluation of Passive Wall Treatment with Circumferential Grooves at the Casing of the First and Second Blade Rotor Rows of a High-Performance Multi-Stage Axial Compressor" Aerospace 11, no. 8: 662. https://doi.org/10.3390/aerospace11080662
APA StyleDiaz, R. B., Tomita, J. T., Bringhenti, C., Silva, F. J. d. S., & Cavalca, D. F. (2024). An Evaluation of Passive Wall Treatment with Circumferential Grooves at the Casing of the First and Second Blade Rotor Rows of a High-Performance Multi-Stage Axial Compressor. Aerospace, 11(8), 662. https://doi.org/10.3390/aerospace11080662






