Experimental and Numerical Simulation of Ejecta Size and Velocity of Hypervelocity Impact Rubble-Pile Asteroid
Abstract
1. Introduction
2. Experimental Materials and Methods
2.1. Projectile and Target Materials
2.2. Experiment Setup and Apparatuses
2.3. Experimental Results and Discussion
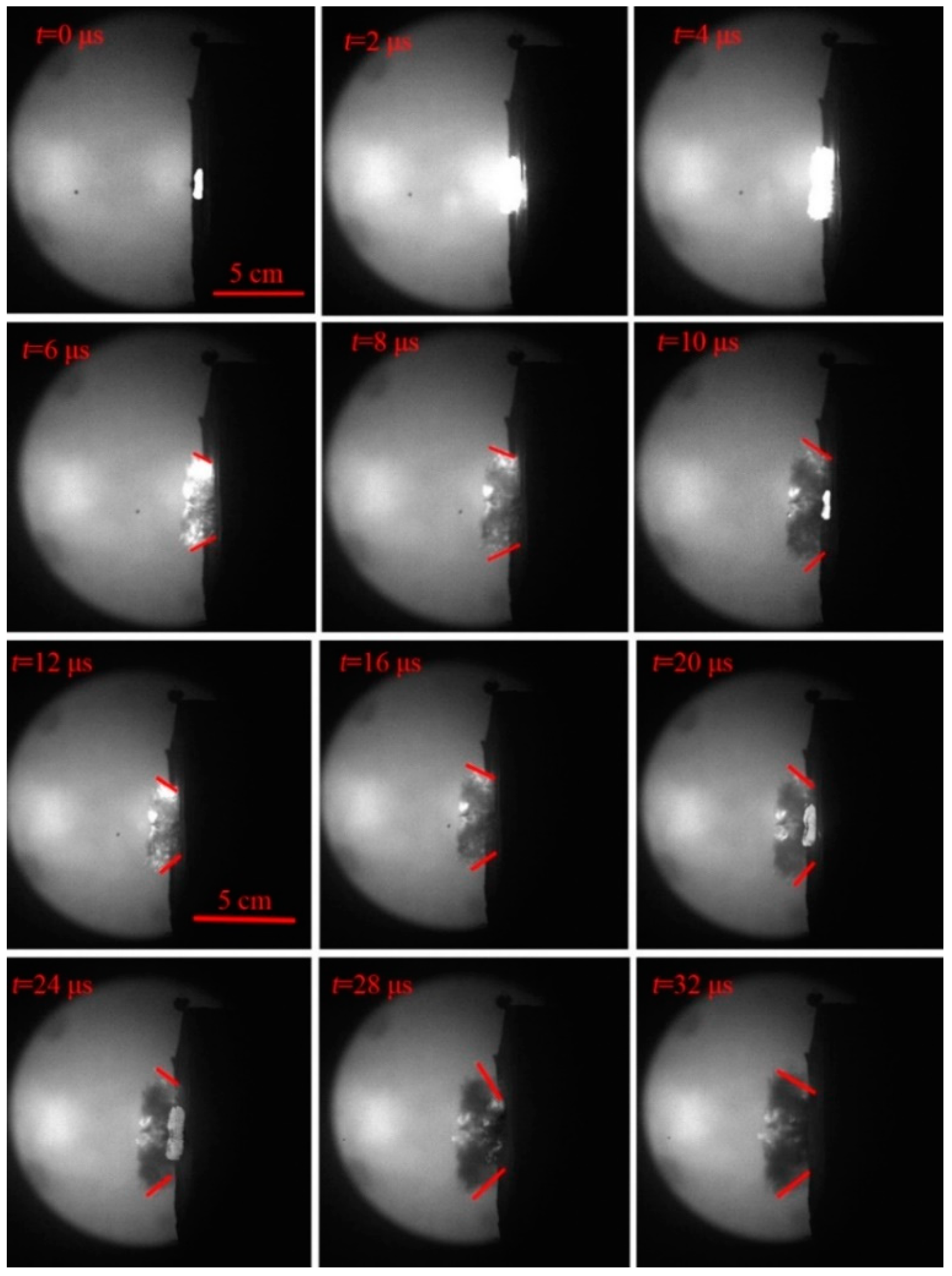
2.3.1. Crater Morphologies
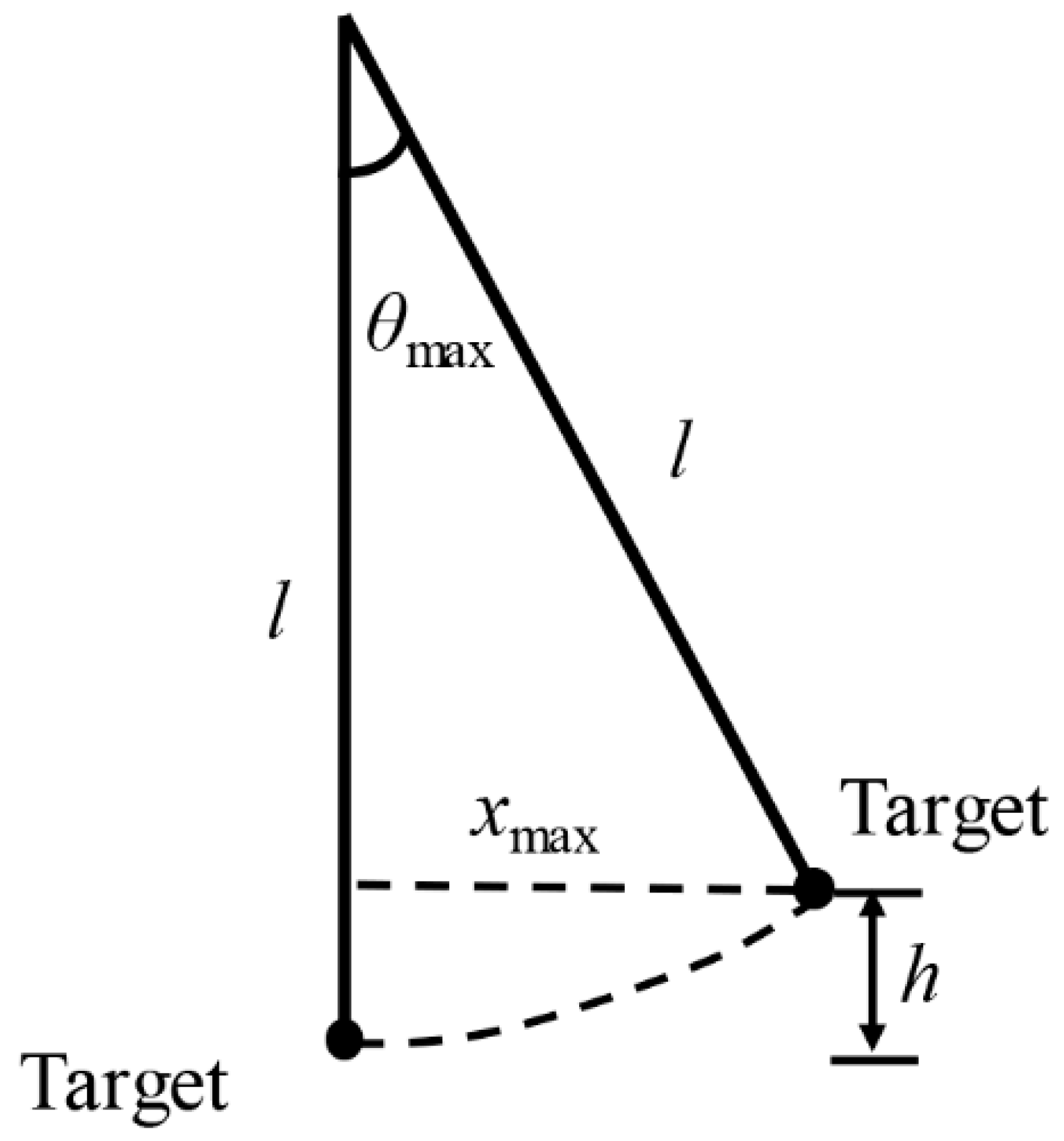
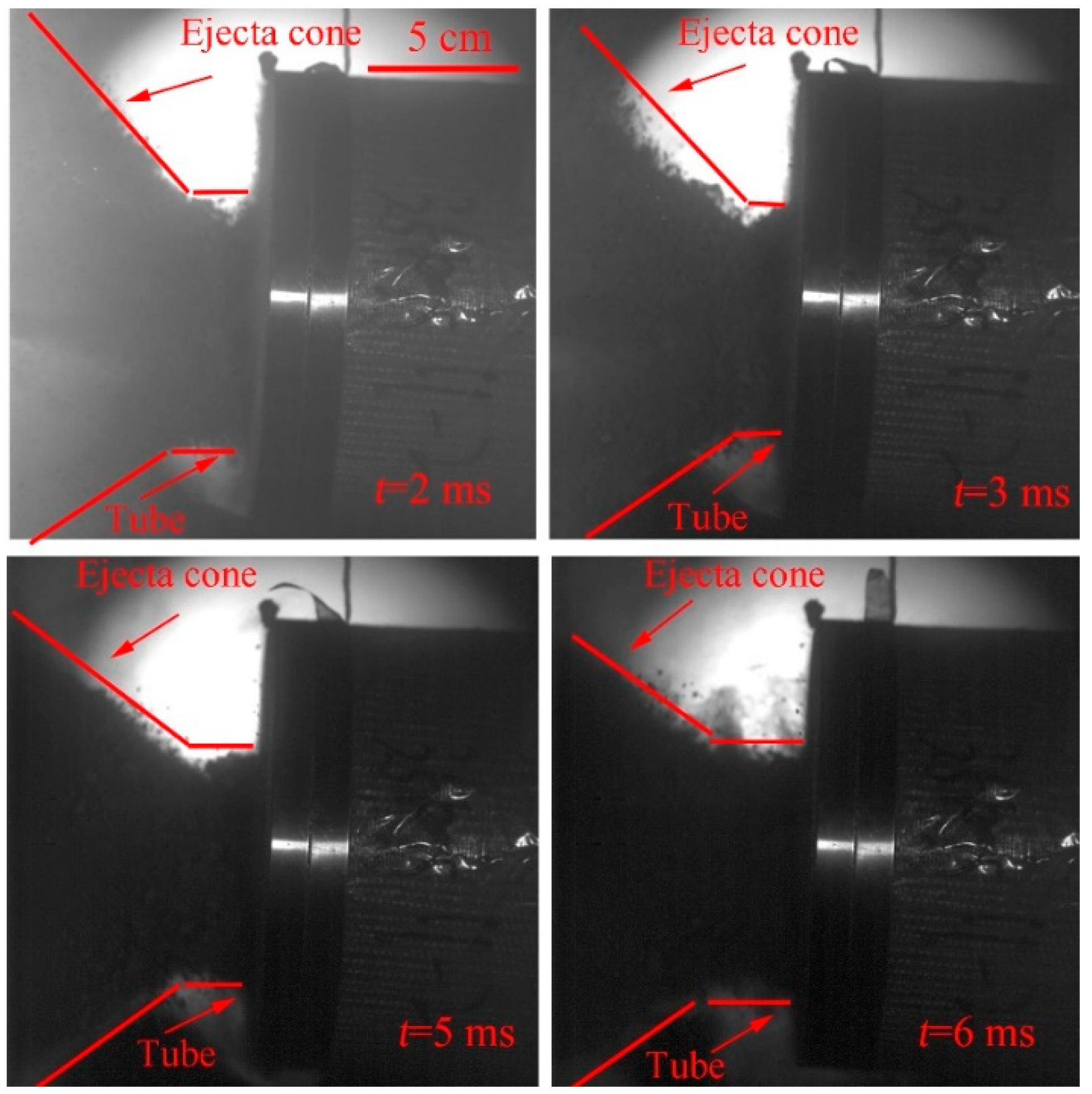
2.3.2. Ejecta
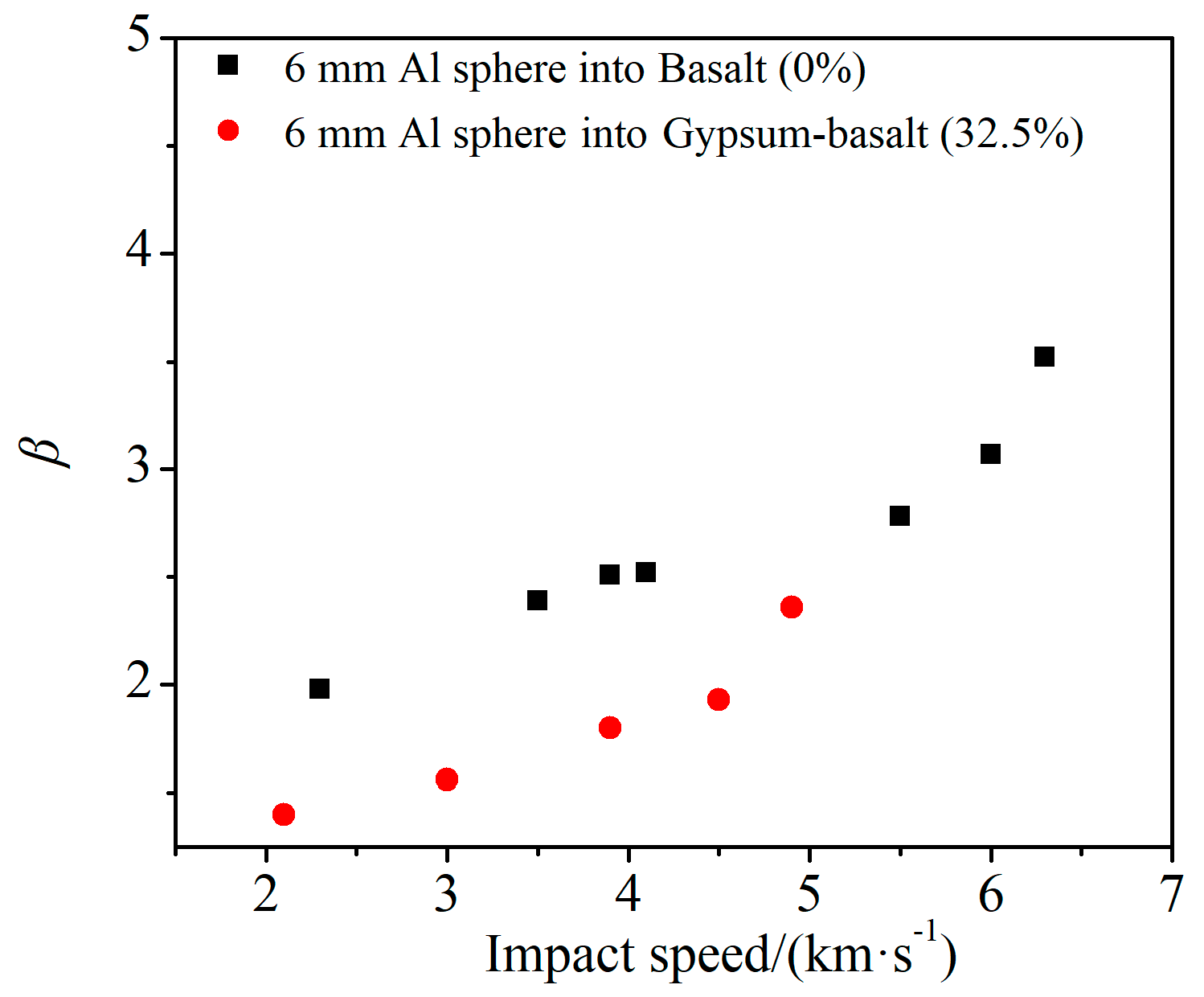
2.3.3. Momentum Enhancement
3. Numerical Model Set-Up
3.1. Two-Dimensional FEM Model
3.2. Three-Dimensional Rubble-Pile Asteroids FEM Model
3.3. Material Parameters
3.3.1. P-alpha Model
3.3.2. EOS Parameters
3.3.3. Strength Model
3.4. Simulation Results and Discussion
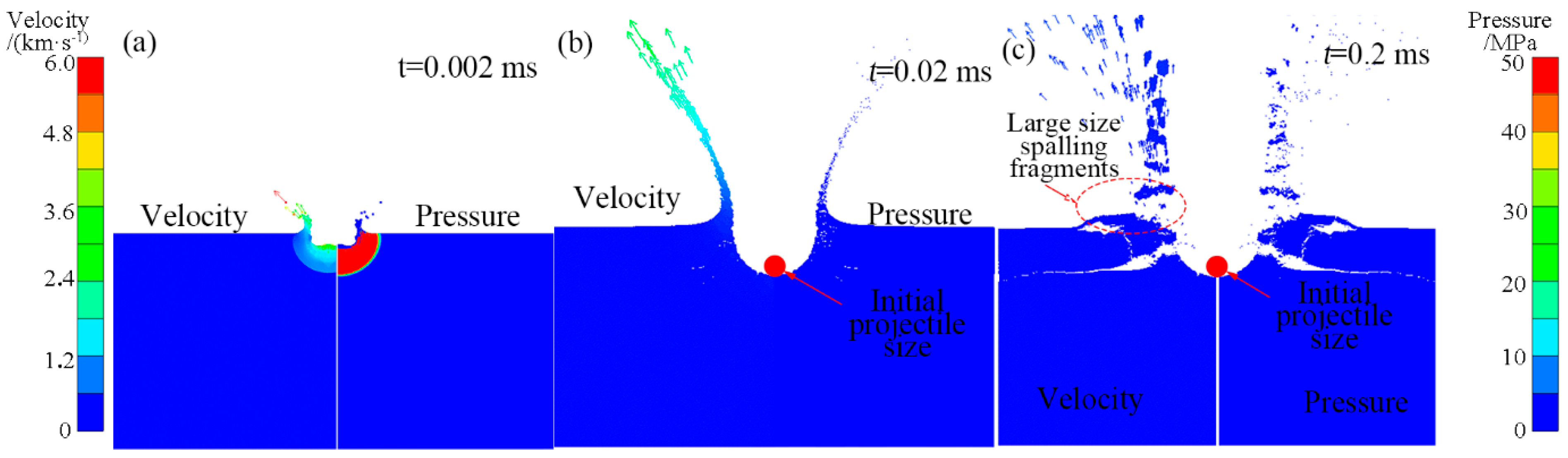
3.4.1. The Formation Process of Ejecta from AUTODYN-2D Simulations
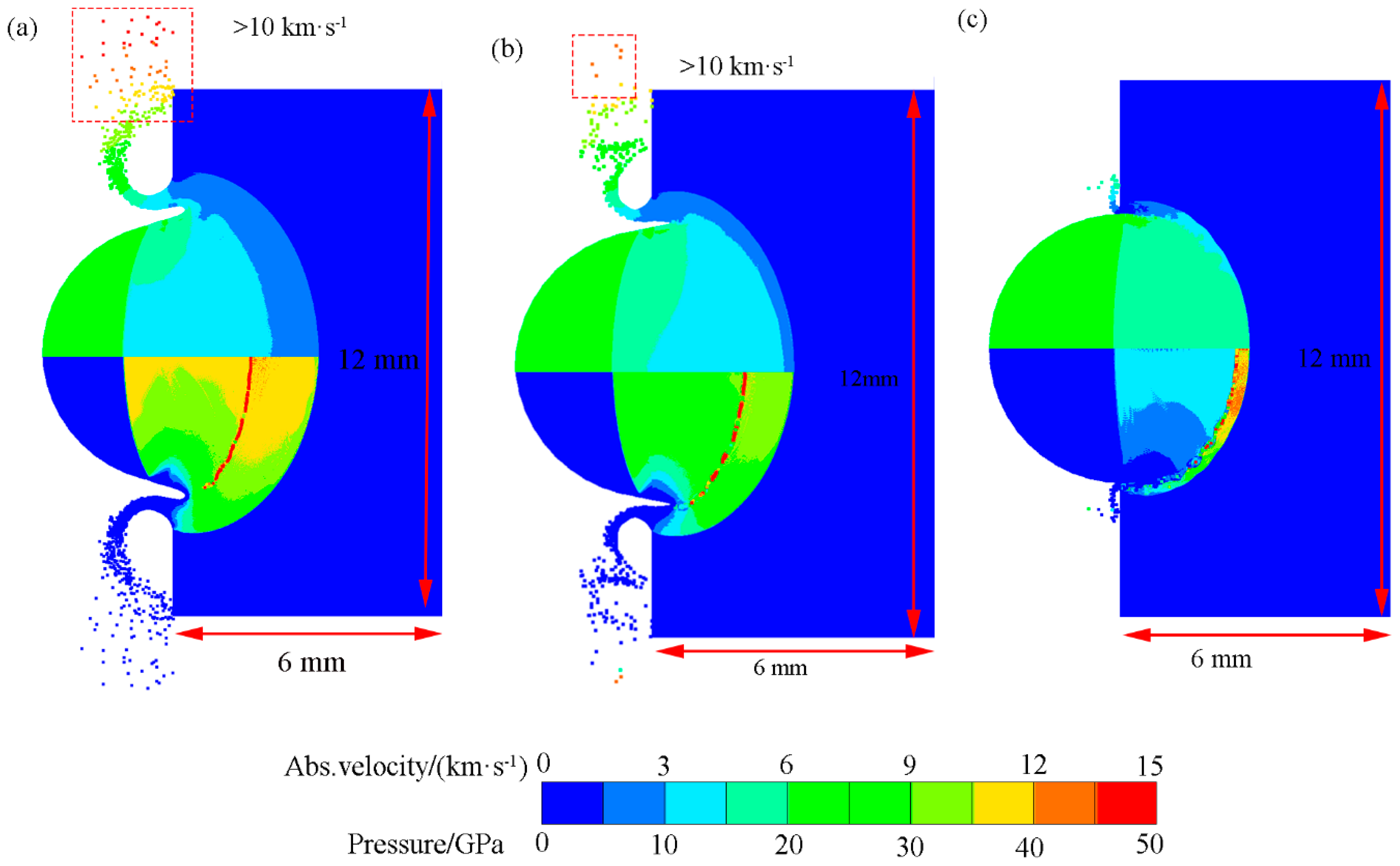
3.4.2. Jet Formation from AUTODYN-2D Simulations
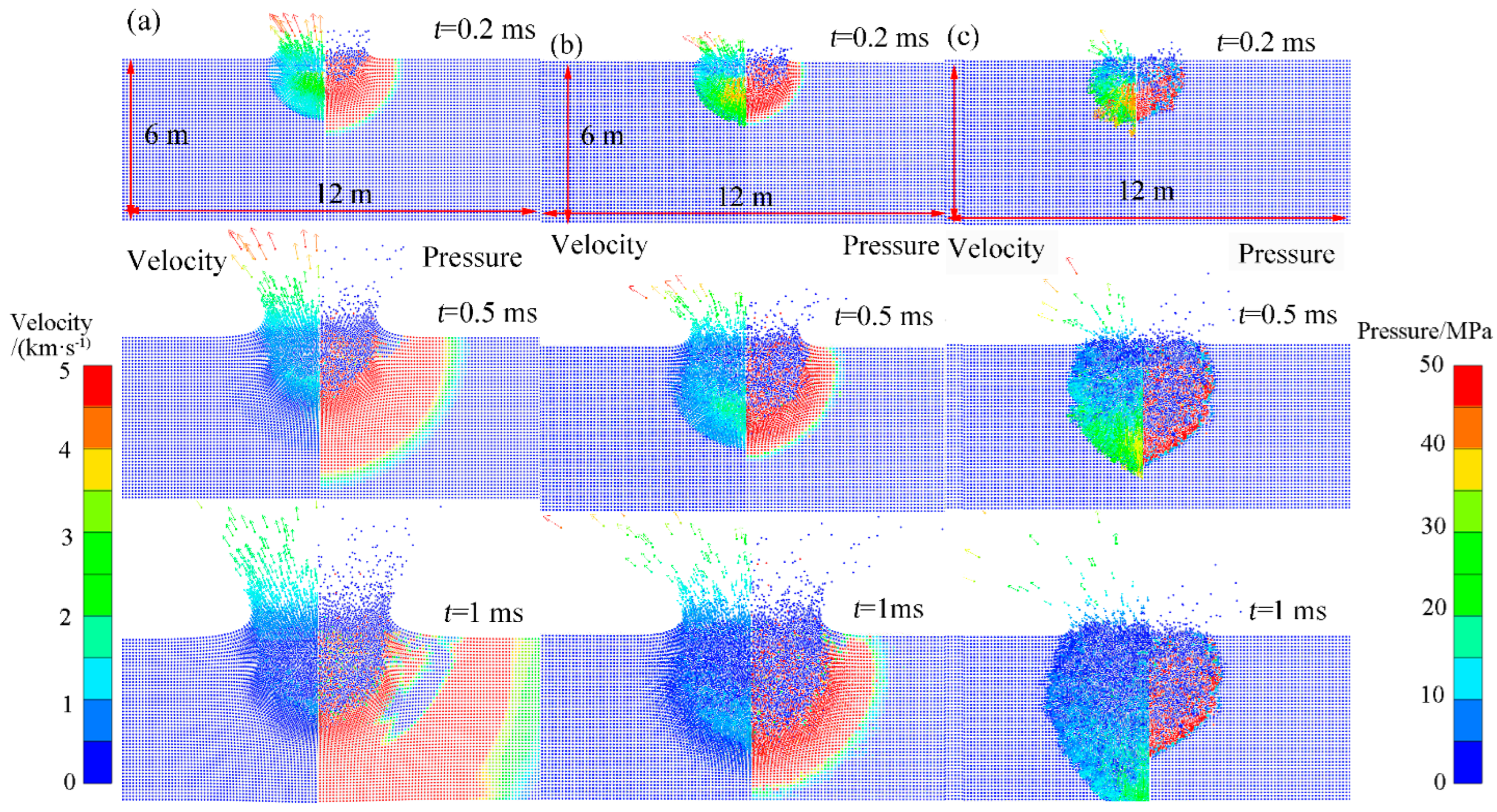
3.4.3. Pressure and Velocity Distribution from AUTODYN-3D Simulations
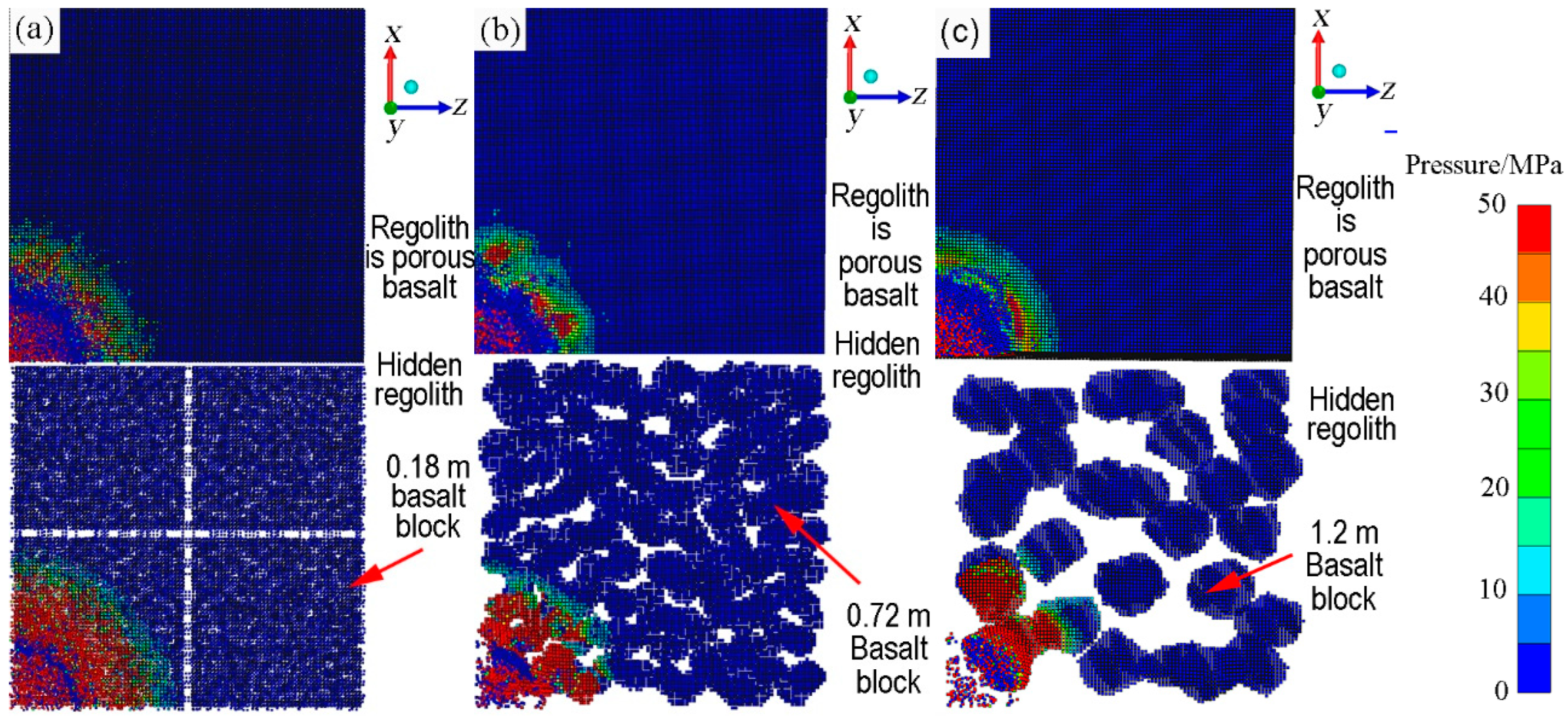
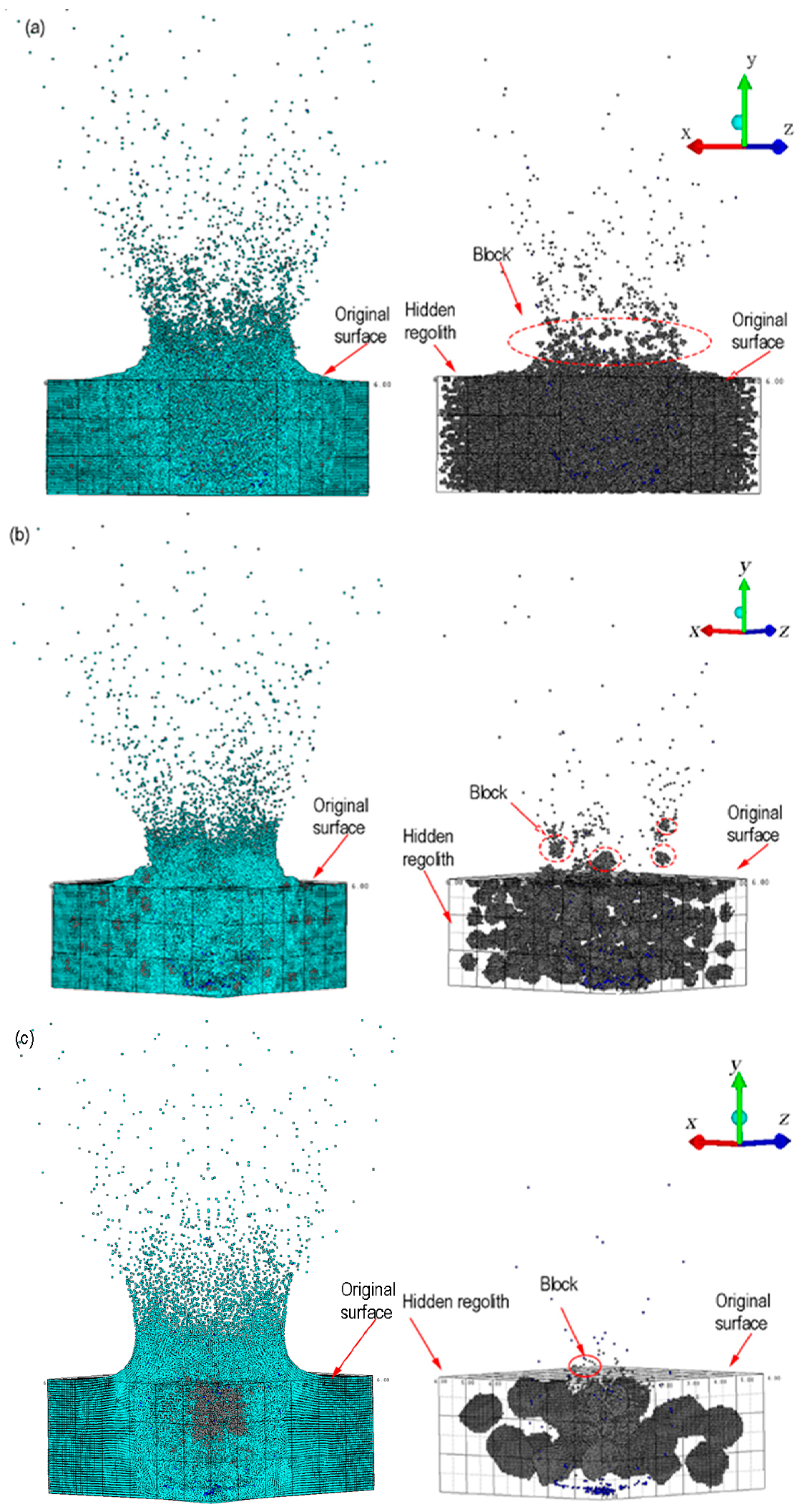
3.4.4. Influence of Boulder Size on Ejecta Size from AUTODYN-3D Simulations
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Buys, M.; Squirini, R.M.; Wilson, C.M.; Gabriel, J.L.; Salehuddin, H.; Zimmerman, G.K.; Barbee, B.W. THEO & MUFN: Defending Earth against the 2023 PDC hypothetical asteroid impact. Acta Astronaut. 2024, 215, 217–233. [Google Scholar]
- Pelton, J.N.; Allahdadi, F. Handbook of Cosmic Hazards and Planetary Defense; Springer: London, UK, 2015; pp. 5–13. [Google Scholar]
- Board, S.S. Defending Planet Earth: Near-Earth-Object Surveys and Hazard Mitigation Strategies; The National Academies Press: Washington, DC, USA, 2010; pp. 1–134. [Google Scholar]
- Gallant, J.; Gladman, B.; Ćuk, M. Current bombardment of the Earth–Moon system: Emphasis on cratering asymmetries. Icarus 2009, 202, 371–382. [Google Scholar] [CrossRef]
- Kereszturi, Á.; Barta, V.; Bondár, I.; Czanik, C.; Igaz, A.; Mónus, P.; Rezes, D.; Szabados, L.; Pál, B.D. Review of synergic meteor observations: Linking the results from cameras, ionosondes, infrasound and seismic detectors. Mon. Not. R. Astron. Soc. 2021, 506, 3629–3640. [Google Scholar] [CrossRef]
- Brown, P.G.; Assink, J.D.; Astiz, L.; Blaauw, R.; Boslough, M.B.; Borovička, J.; Brachet, N.; Brown, D.; Campbell-Brown, M.; Ceranna, L.; et al. A 500-kiloton airburst over Chelyabinsk and an enhanced hazard from small impactors. Nature 2013, 503, 238–241. [Google Scholar] [CrossRef]
- Wang, K.D.; Zhou, Q.; Liu, Y.D.; Li, M.; Wang, Y. Research on space-based kinetic impactor disrupting small-sized asteroids under short warning time conditions. Acta Astronaut. 2024, 219, 291–299. [Google Scholar] [CrossRef]
- Bowles, N.E.; Snodgrass, C.; Gibbings, A.; Sanchez, J.P.; Arnold, J.A.; Eccleston, P.; Andert, T.; Probst, A.; Naletto, G.; Vandaele, A.C. CASTAway: An asteroid main belt tour and survey. Adv. Space Res. 2018, 62, 1998–2025. [Google Scholar] [CrossRef]
- Lay, O.; Masiero, J.; Grav, T.; Mainzer, A.; Masci, F.; Wright, E. Asteroid impact hazard warning from the Near-Earth Object surveyor mission. Planet. Sci. J. 2024, 5, 149. [Google Scholar] [CrossRef]
- Schmidt, N. Planetary defense governance: Thirty years of development and the multilateral future. Acta Astronaut. 2024, 214, 343–355. [Google Scholar] [CrossRef]
- Barbee, B.W.; Syal, M.B.; Dearborn, D.; Gisler, G.; Greenaugh, K.; Howley, K.M.; Leung, R.; Lyzhoft, J.; Miller, P.L.; Nuth, J.A.; et al. Options and uncertainties in planetary defense: Mission planning and vehicle design for flexible response. Acta Astronaut. 2018, 143, 37–61. [Google Scholar] [CrossRef]
- Daly, R.T.; Ernst, C.M.; Barnouin, O.S.; Chabot, N.L.; Rivkin, A.S.; Cheng, A.F.; Adams, E.Y.; Agrusa, H.F.; Abel, E.D.; Alford, A.L.; et al. Successful kinetic impact into an asteroid for planetary defense. Nature 2023, 616, 443–447. [Google Scholar] [CrossRef]
- Thomas, C.A.; Naidu, S.P.; Scheirich, P.; Moskovitz, N.A.; Pravec, P.; Chesley, S.R.; Rivkin, A.S.; Osip, D.J.; Lister, T.A.; Benner, L.A.; et al. Orbital period change of dimorphos due to the DART Kinetic Impact. Nature 2023, 616, 448–451. [Google Scholar] [CrossRef]
- Wang, K.D.; Li, M.T.; Zhou, Q.; Wang, Y. Targets selection and mission optimization of kinetic impact deflection test mission for small size asteroids. Adv. Space Res. 2023, 72, 3477–3498. [Google Scholar] [CrossRef]
- Ormö, J.; Raducan, S.D.; Jutzi, M.; Herreros, M.I.; Luther, R.; Collins, G.S.; Wünnemann, K.; Mora-Rueda, M.; Hamann, C. Boulder exhumation and segregation by impacts on rubble-pile asteroids. Earth Planet. Sci. Lett. 2022, 594, 117713. [Google Scholar] [CrossRef]
- Popova, O.; Borovcka, J.; Hartmann, W.K.; Spurný, P.; Gnos, E.; Nemtchinov, I.; Trigo-Rodríguez, J.M. Very low strengths of interplanetary meteoroids and small asteroids. Meteorit. Planet. Sci. 2011, 46, 1525–1550. [Google Scholar] [CrossRef]
- Graninger, D.; Stickle, A.; Owen, J.M.; Syal, M. Simulating hypervelocity impacts into rubble pile structures for planetary defense. Int. J. Impact Eng. 2023, 180, 104670. [Google Scholar] [CrossRef]
- Tatsumi, E.; Sugita, S. Cratering efficiency on coarse-grain targets: Implications for the dynamical evolution of asteroid 25143 Itokawa. Icarus 2018, 300, 227–248. [Google Scholar] [CrossRef]
- Leliwa-Kopystynski, J.; Arakawa, M. Impacts experiments onto heterogeneous targets simulating impact breccia: Implications for impact strength of asteroids and formation of the asteroid families. Icarus 2014, 235, 147–155. [Google Scholar] [CrossRef]
- Rainey ES, G.; Stickle, A.M.; Cheng, A.F.; Rivkin, A.S.; Chabot, N.L.; Barnouin, O.S.; Ernst, C.M. AIDA/DART Impact Simulation Working Group Impact modeling for the Double Asteroid Redirection Test (DART) mission. Int. J. Impact Eng. 2020, 142, 103528. [Google Scholar] [CrossRef]
- Stickle, A.M.; Syal, M.B.; Cheng, A.F.; Collins, G.S.; Davison, T.M.; Gisler, G.; Güldemeister, N.; Heberling, T.; Luther, R.; Michel, P.; et al. Benchmarking impact hydrocodes in the strength regime: Implications for modeling deflection by a kinetic impactor. Icarus 2020, 338, 113446. [Google Scholar] [CrossRef]
- Yasui, M.; Arakawa, M.; Okawa, H.; Hasegawa, S. Cratering experiments on granular targets with a variety of particle sizes: Implications for craters on rubble-pile asteroids. J. Geophys. Res. Planets 2022, 127, 1–15. [Google Scholar] [CrossRef]
- Walker, J.D.; Chocron, S.; Grosch, D.J.; Marchi, S.; Alexander, A.M. Momentum enhancement from a 3 cm diameter aluminum sphere striking a small boulder assembly at 5.4 km s−1. Planet. Sci. J. 2022, 3, 215. [Google Scholar] [CrossRef]
- Güldemeister, N.; Moreau, J.; Kohout, T.; Luther, R.; Wünnemann, K. Insight into the distribution of high-pressure shock metamorphism in Rubble-pile asteroids. Planet. Sci. J. 2022, 3, 198. [Google Scholar] [CrossRef]
- Raducan, S.D.; Davison, T.M.; Collins, G.S. Ejecta distribution and momentum transfer from oblique impacts on asteroid surfaces. Icarus 2022, 374, 114793. [Google Scholar] [CrossRef]
- Raducan, S.D.; Jutzi, M.; Davison, T.M.; DeCoster, M.E.; Graninger, D.M.; Owen, J.M.; Stickle, A.M.; Collins, G.S. Influence of the projectile geometry on the momentum transfer from a kinetic impactor and implications for the DART mission. Int. J. Impact Eng. 2022, 162, 104147. [Google Scholar] [CrossRef]
- Raducan, S.D.; Davison, T.M.; Luther, R.; Collins, G.S. The role of asteroid strength, porosity and internal friction in impact momentum transfer. Icarus 2019, 329, 282–295. [Google Scholar] [CrossRef]
- Luther, R.; Zhu, M.; Collins, G.; Wünnemann, K. Effect of target properties and impact velocity on ejection dynamics and ejecta deposition. Planet. Space Sci. 2018, 53, 1705–1732. [Google Scholar] [CrossRef]
- Pierazzo, E.; Artemieva, N.; Asphaug, E.; Baldwin, E.C.; Cazamias, J.; Coker, R.; Collins, G.S.; Crawford, D.A.; Davison, T.; Elbeshausen, D.; et al. Validation of numerical codes for impact and explosion cratering: Impacts on strengthless and metal targets. Meteorit. Planet. Sci. 2008, 43, 1917–1938. [Google Scholar] [CrossRef]
- Libersky, L.D.; Petschek, A.G.; Carney, T.C.; Hipp, J.R.; Allahdadi, F.A. High Strain Lagrangian Hydrodynamics: A Three-Dimensional SPH Code for Dynamic Material Response. J. Comput. Phys. 1993, 109, 67–75. [Google Scholar] [CrossRef]
- Liu, W.; Zhang, Q.; Long, R.; Gong, Z.; Jiankang, R.; Hu, X.; Ren, S.; Wu, Q.; Song, G. Effects of projectile parameters on the momentum transfer and projectile melting during hypervelocity impact. Def. Technol. 2024, 32, 89–103. [Google Scholar] [CrossRef]
- Raducan, S.D.; Jutzi, M.; Cheng, A.F.; Zhang, Y.; Barnouin, O.; Collins, G.S.; Daly, R.T.; Davison, T.M.; Ernst, C.M.; Farnham, T.L.; et al. Physical properties of asteroid Dimorphos as derived from the DART impact. Nat. Astron. 2024, 8, 445–455. [Google Scholar] [CrossRef]
- Jiao, Y.; Yan, X.; Cheng, B.; Baoyin, H. SPH–DEM modelling of hypervelocity impacts on rubble-pile asteroids. Mon. Not. R. Astron. Soc. 2024, 527, 10348–10357. [Google Scholar] [CrossRef]
- Kereszturi, A. Surface processes in microgravity for landing and sampling site selection of asteroid missions—Suggestions for MarcoPolo-R. Planet. Space Sci. 2014, 101, 65–76. [Google Scholar] [CrossRef]
- Fujii, Y.; Nakamura, A.M. Compaction and fragmentation of porous gypsum targets from low-velocity impacts. Icarus 2009, 201, 795–801. [Google Scholar] [CrossRef]
- Nakamura, A.M.; Hiraoka, K.; Yamashita, Y.; Machii, N. Collisional disruption experiments of porous targets. Planet. Space Sci. 2009, 57, 111–118. [Google Scholar] [CrossRef][Green Version]
- Spray, J.G.; Gores PA, S.; Kenkmann, T.; Poelchau, M.H. Experimental hypervelocity impact of sedimentary and metamorphic rocks: Reconstruction of crater shapes and volumes. Int. J. Impact Eng. 2024, 190, 104965. [Google Scholar] [CrossRef]
- Kenkmann, T.; Deutsch, A.; Thoma, K.; Ebert, M.; Poelchau, M.H.; Buhl, E.; Carl, E.R.; Danilewsky, A.N.; Dresen, G.; Dufresne, A.; et al. Experimental impact cratering: A summary of the major results of the MEMIN research unit. Meteorit. Planet. Sci. 2018, 53, 1543–1568. [Google Scholar] [CrossRef]
- Li, J.; Hirabayashi, M.; Farnham, T.L.; Sunshine, J.M.; Knight, M.M.; Tancredi, G.; Moreno, F.; Murphy, B.; Opitom, C.; Chesley, S.; et al. Ejecta from the DART-produced active asteroid Dimorphos. Nature 2023, 616, 452–456. [Google Scholar] [CrossRef]
- Rivkin, A.S.; Cheng, A.F. Planetary defense with the Double Asteroid Redirection Test (DART) mission and prospects. Nat. Commun. 2023, 14, 1003. [Google Scholar] [CrossRef]
- Melosh, H.J. Impact Cratering: A Geologic Process; Oxford University Press: New York, NY, USA, 1989; pp. 1–240. [Google Scholar]
- Yasui, M.; Arakawa, M. Impact experiments of porous gypsum-glass bead mixtures simulating parent bodies of ordinary chondrites: Implications for re-accumulation processes related to rubble-pile formation. Icarus 2011, 214, 754–765. [Google Scholar] [CrossRef]
- Flynn, G.J.; Durda, D.D.; Molesky, M.J.; May, B.A.; Congram, S.N.; Loftus, C.L.; Reagan, J.R.; Strait, M.M.; Macke, R.J. Momentum transfer in hypervelocity cratering of meteorites and meteorite analogs: Implications for orbital evolution and kinetic impact deflection of asteroids. Int. J. Impact Eng. 2020, 136, 103437. [Google Scholar] [CrossRef]
- Gault, D.E.; Shoemaker, M.E.; Moore, H.J. Spray Ejected from the Lunar Surface by Meteoroid Impact; National Aeronautics and Space Administration: Washington, DC, USA, 1963. [Google Scholar]
- Saito, T.; Kaiho, K.; Abe, A.; Katayama, M.; Takayama, K. Hypervelocity impact of asteroid/comet on the oceanic crust of the earth. Int. J. Impact Eng. 2008, 35, 1770–1777. [Google Scholar] [CrossRef]
- Saito, T.; Kaiho, K.; Abe, A.; Katayama, M.; Takayama, K. Numerical simulations of hypervelocity impact of asteroid/comet on the Earth. Int. J. Impact Eng. 2006, 33, 713–722. [Google Scholar] [CrossRef]
- Wakatsuki, Y.; Akahoshi, Y.; Koura, T.; Takahara, H. Study of the effects of projectile strength and density in the asteroid deflection by hypervelocity impact. Int. J. Impact Eng. 2023, 180, 104710. [Google Scholar] [CrossRef]
- Wen, K.; Chen, X. Failure evolution in hypervelocity impact of Al spheres onto thin Al plates. Int. J. Impact Eng. 2021, 147, 103727. [Google Scholar] [CrossRef]
- Wang, Z.L.; Huang, Y.P.; Li, S.Y.; Xiong, F. SPH-FEM coupling simulation of rock blast damage based on the determination and optimization of the RHT model parameters. IOP conference series. Earth Environ. Sci. 2020, 570, 42035. [Google Scholar]
- Johnson, B.C.; Bowling, T.J.; Melosh, H.J. Jetting during vertical impacts of spherical projectiles. Icarus 2014, 238, 13–22. [Google Scholar] [CrossRef]
- Fang, Q.; Zhang, J. 3D numerical modeling of projectile penetration into rock-rubble overlays accounting for random distribution of rock-rubble. Int. J. Impact Eng. 2014, 63, 118–128. [Google Scholar] [CrossRef]
- Herrmann, W. Constitutive equation for the dynamic compaction of ductile porous materials. J. Appl. Phys. 1969, 40, 2490–2499. [Google Scholar] [CrossRef]
- Jutzi, M.; Benz, W.; Michel, P. Numerical simulations of impacts involving porous bodies I. Implementing sub-resolution porosity in a 3D SPH hydrocode. Icarus 2008, 198, 242–255. [Google Scholar] [CrossRef]
- Carroll, M.M.; Holt, A.C. Suggested modification of the P–α model for porous materials. J. Appl. Phys. 1972, 43, 759–761. [Google Scholar] [CrossRef]
- Jutzi, M.; Michel, P.; Hiraoka, K.; Nakamura, A.M.; Benz, W. Numerical simulations of impacts involving porous bodies II. Comparison with laboratory experiments. Icarus 2009, 201, 802–813. [Google Scholar] [CrossRef]
- Arthur, L.R. Linear shock-velocity-particle-velocity relationship. J. Appl. Phys. 1967, 38, 4976–4980. [Google Scholar]
- Ahrens, T.J.; Johnson, M.L. Shock Wave Data for Rocks; American Geophysical Union: Washington, DC, USA, 1995; p. 35. [Google Scholar]
- Stanley, P.M. LASL Shock Hugoniot Data; University of California Press: Berkley, CA, USA, 1980; pp. 1–653. [Google Scholar]
- Holsapple, K.A. On the “strength” of the small bodies of the solar system: A review of strength theories and their implementation for analyses of impact disruptions. Planet. Space Sci. 2009, 57, 127–141. [Google Scholar] [CrossRef]
- Steinberg, D.J.; Cochran, S.G.; Guinan, M.W. A constitutive model for metals applicable at high-strain rate. J. Appl. Phys. 1980, 51, 1498–1504. [Google Scholar] [CrossRef]
- Dotto, E.; Zinzi, A. Impact observations of asteroid Dimorphos via Light Italian CubeSat for imaging of asteroids (LICIACube). Nat. Commun. 2023, 14, 3055. [Google Scholar] [CrossRef] [PubMed]
- Graykowski, A.; Lambert, R.A.; Marchis, F.; Cazeneuve, D.; Dalba, P.A.; Esposito, T.M.; O’Conner Peluso, D.; Sgro, L.A.; Blaclard, G.; Borot, A.; et al. Light curves and colors of the ejecta from dimorphos after the DART impact. Nature 2023, 616, 461–464. [Google Scholar] [CrossRef] [PubMed]
- Cheng, A.F.; Agrusa, H.F.; Barbee, B.W.; Meyer, A.J.; Farnham, T.L.; Raducan, S.D.; Richardson, D.C.; Dotto, E.; Zinzi, A.; Della Corte, V.; et al. Momentum transfer from the DART mission kinetic impact on asteroid dimorphos. Nature 2023, 616, 457–460. [Google Scholar] [CrossRef] [PubMed]
- A’Hearn, M.F.; Belton, M.; Delamere, W.A.; Kissel, J.; Klaasen, K.; McFadden, L.A.; Meech, K.J.; Melosh, H.J.; Schultz, P.H.; Sunshine, J.M.; et al. Deep impact: Excavating Comet Tempel 1. Science 2005, 310, 258–264. [Google Scholar] [CrossRef]
- Walsh, J.M.; Shreffler, R.G.; Willig, F.J. Limiting conditions for jet formation in high velocity collisions. J. Appl. Phys. 1953, 24, 349–359. [Google Scholar] [CrossRef]
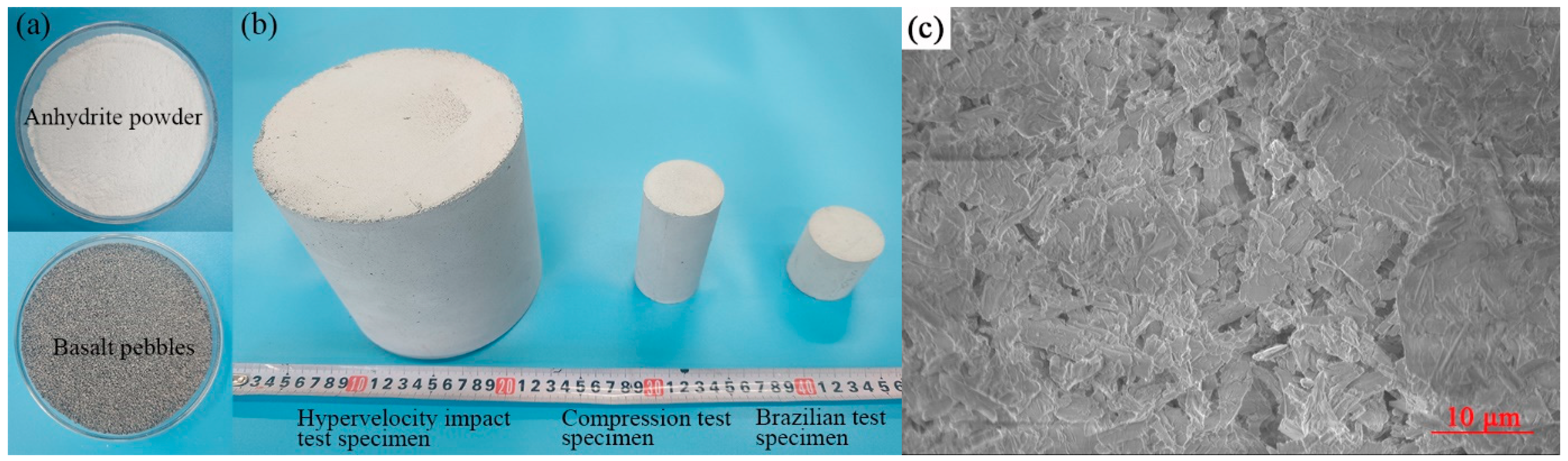
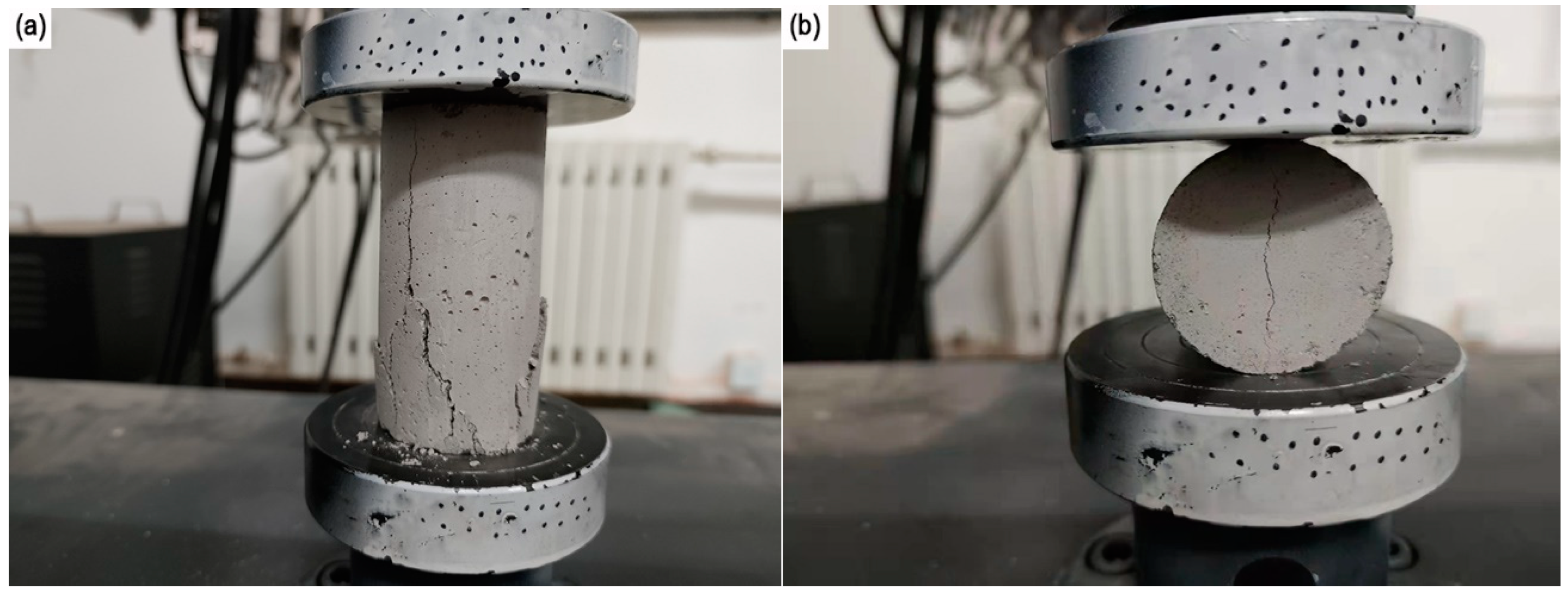
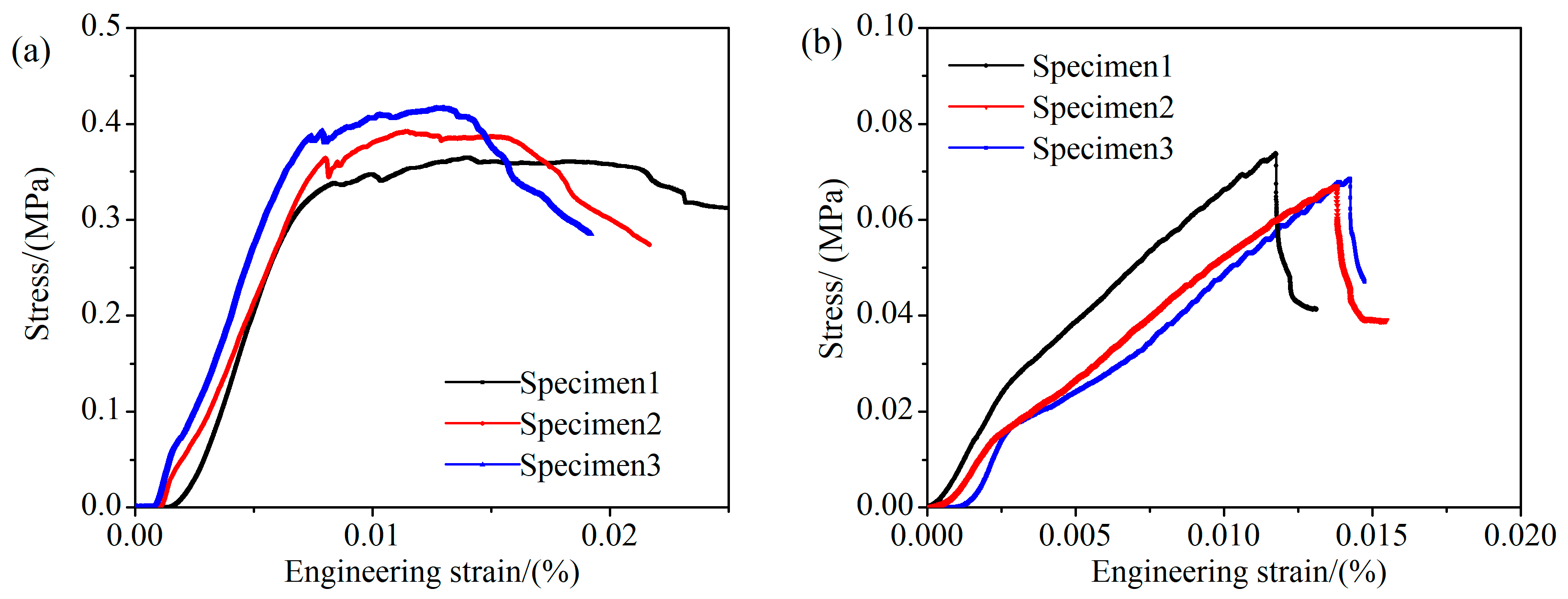
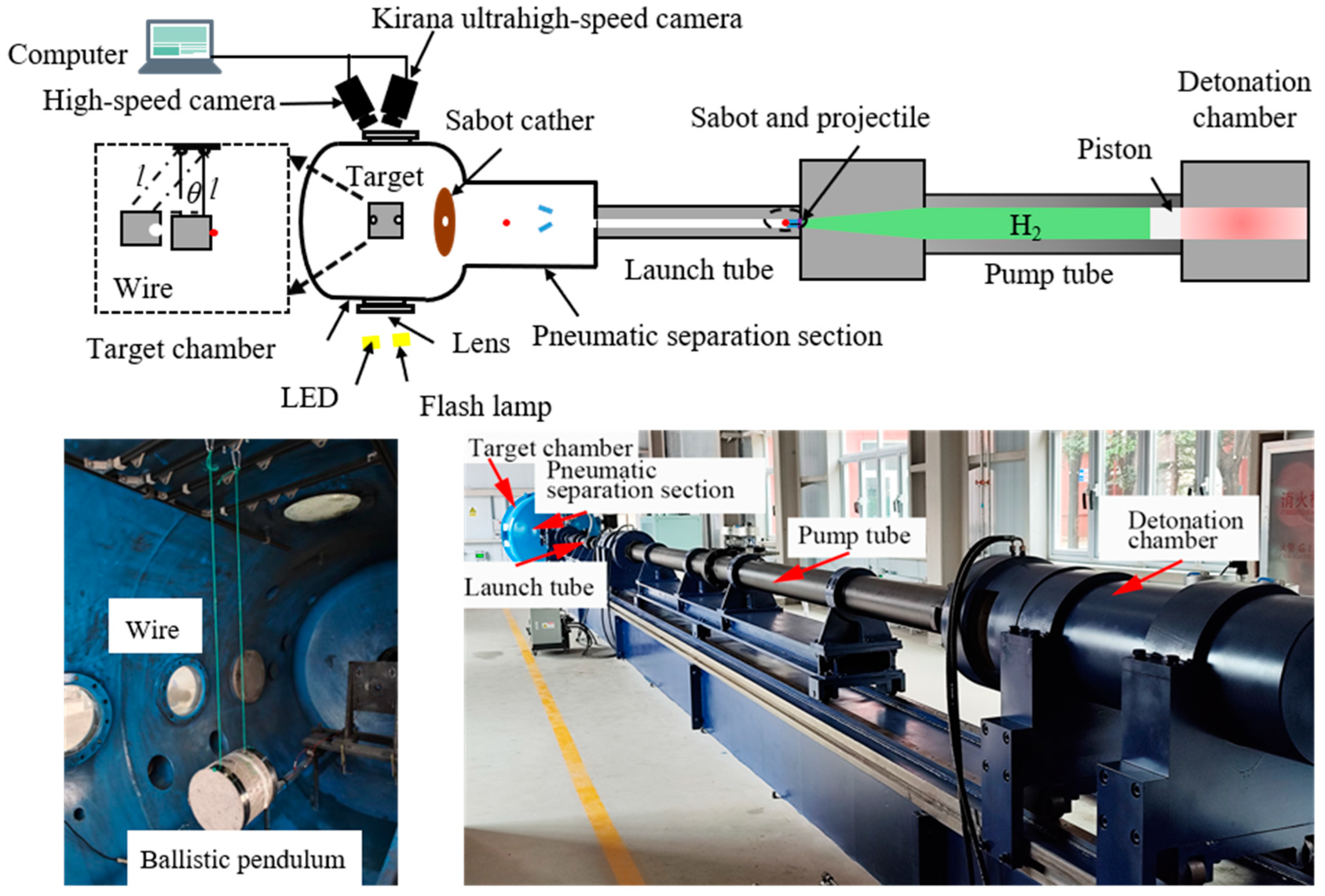




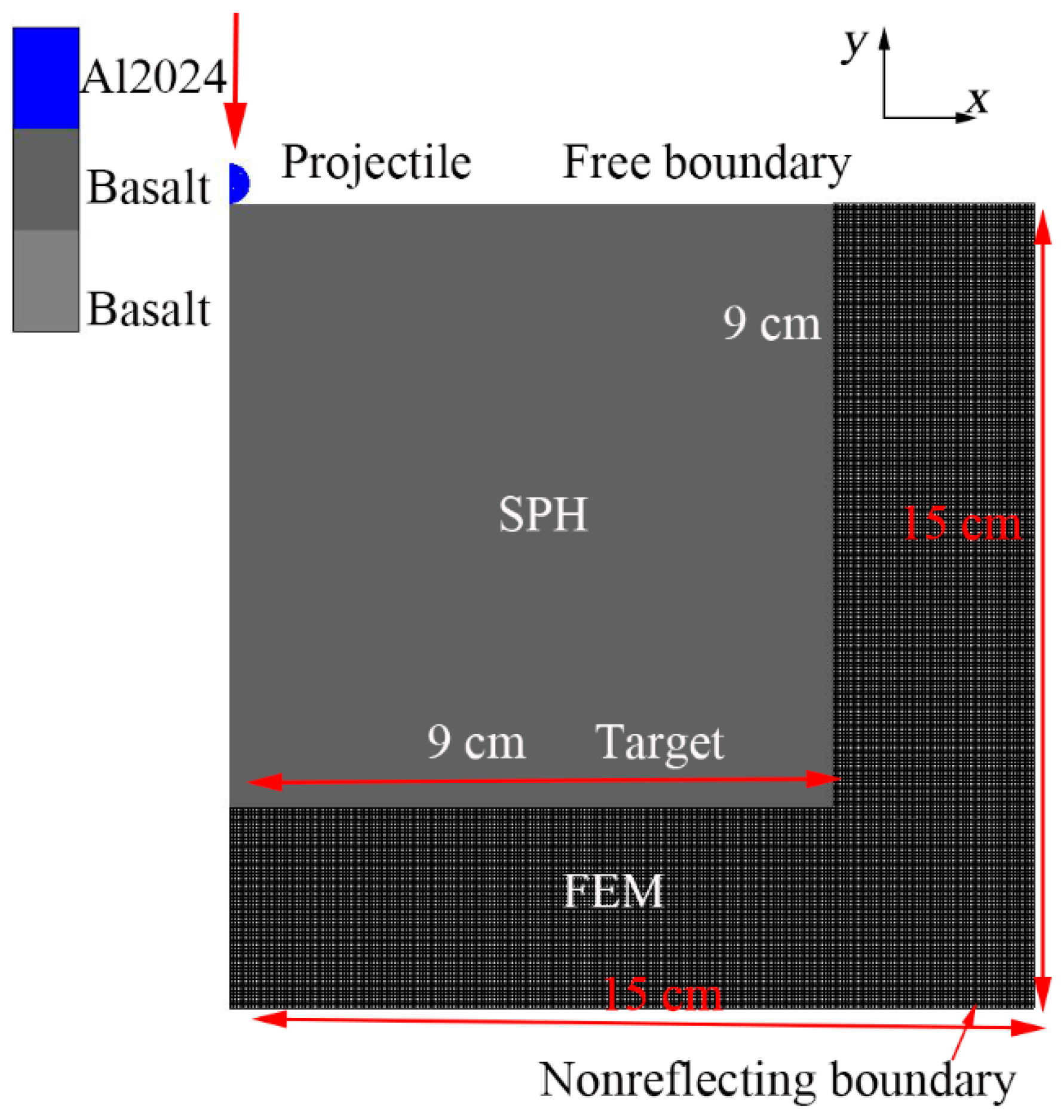
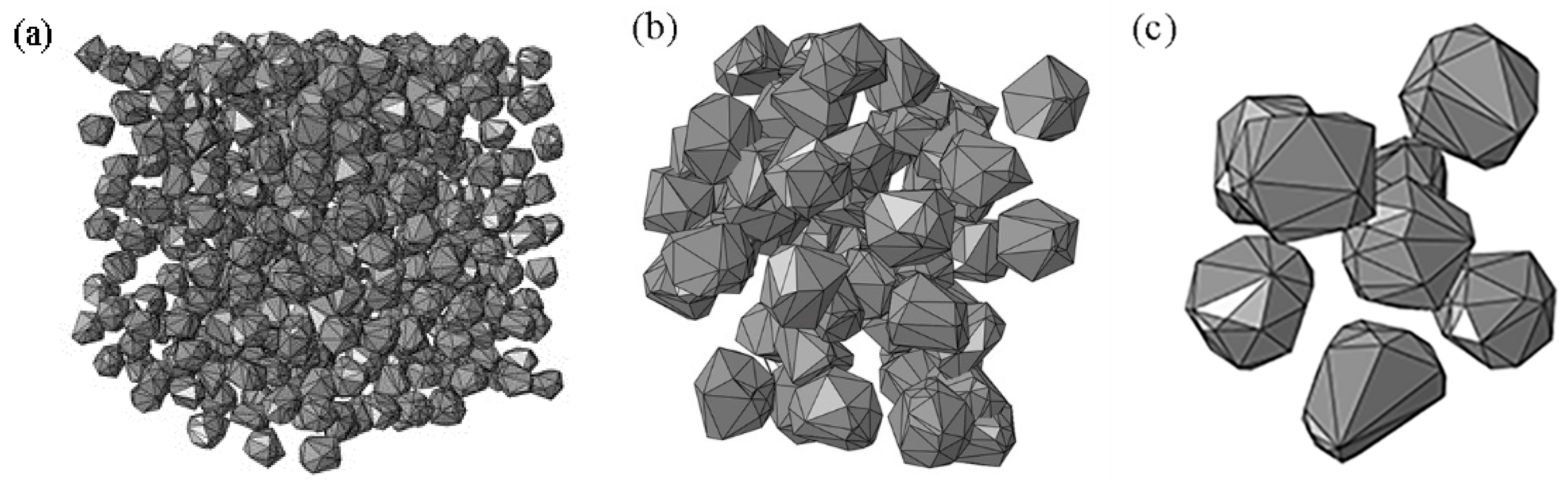
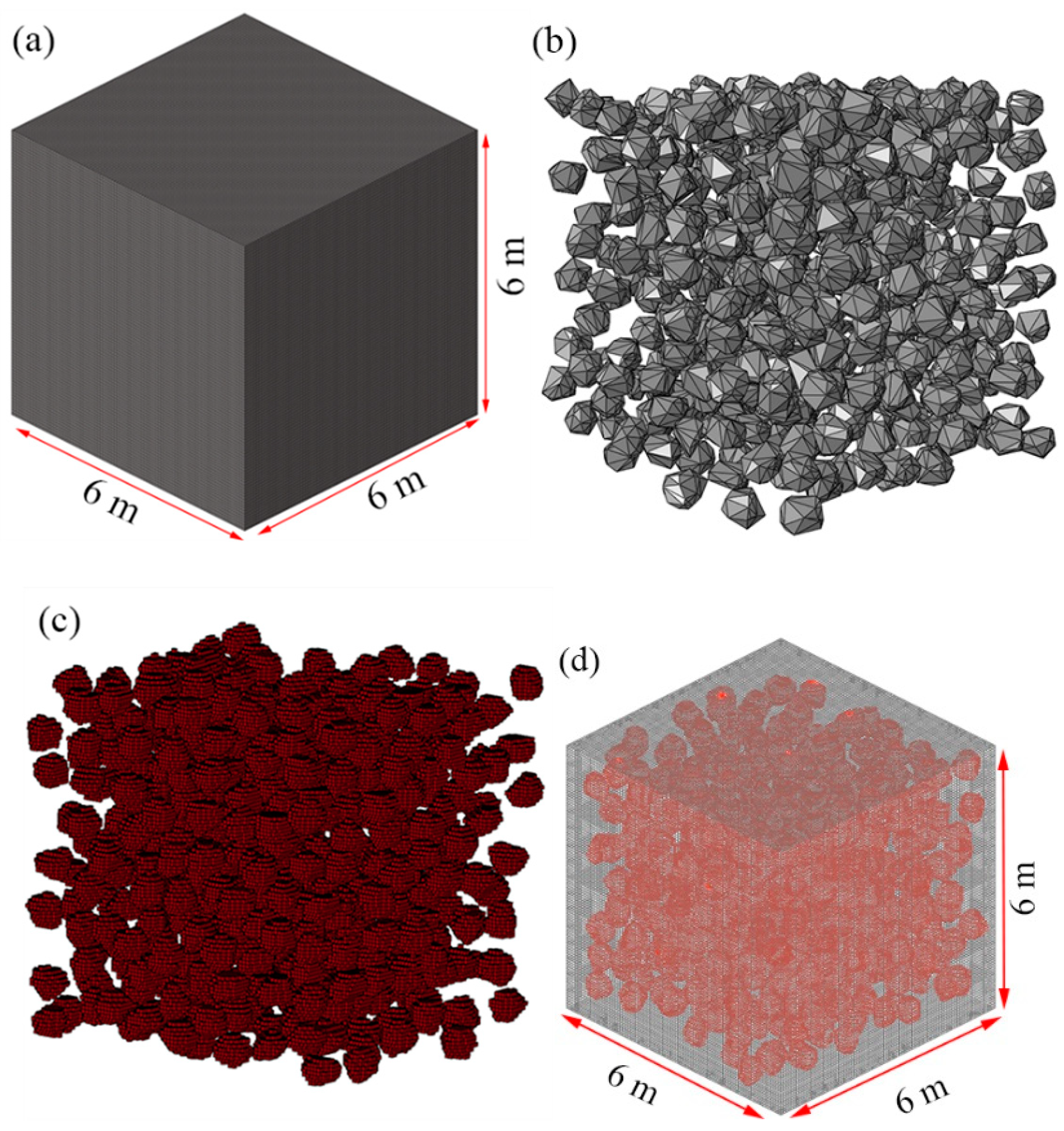
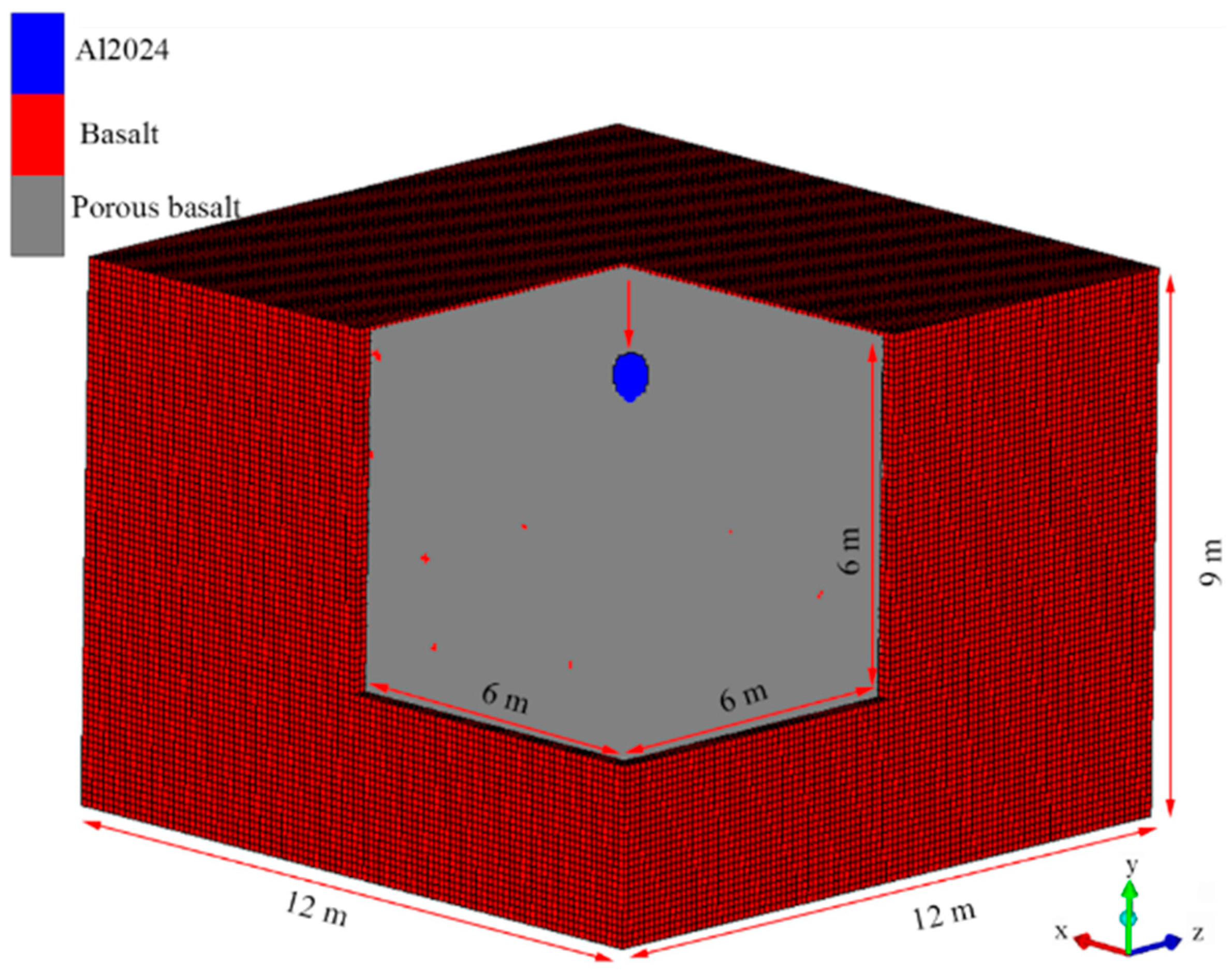
| No. | Projectile | /(km·s−1) | Target | Pendulum | |||
|---|---|---|---|---|---|---|---|
| /g | Diameter/mm | Materials | Shape | Post-Test Weight/g | |||
| 1 | 0.31 | 6 | 2.1 | GB | Cylinder | 4100.4 | 1.40 |
| 2 | 0.31 | 6 | 3.0 | GB | Cylinder | 4101.5 | 1.56 |
| 3 | 0.31 | 6 | 3.9 | GB | Cylinder | 3910.8 | 1.80 |
| 4 | 0.31 | 6 | 4.5 | GB | Cylinder | 4009.1 | 1.93 |
| 5 | 0.31 | 6 | 4.9 | GB | Cylinder | 3973.4 | 2.36 |
| Test No. | Impactor | Target | S/(cm2) | D/cm | p/cm | p/D | |
|---|---|---|---|---|---|---|---|
| /g | /(km·s−1) | ||||||
| 1 | 0.31 | 2.1 | GB | 13.9 | 4.2 | 1.3 | 0.31 |
| 2 | 0.31 | 3.1 | GB | 20.4 | 5.1 | 2.8 | 0.55 |
| 3 | 0.31 | 3.9 | GB | 30.2 | 6.2 | 3.2 | 0.52 |
| 4 | 0.31 | 4.5 | GB | 56.4 | 8.5 | 4.4 | 0.52 |
| 5 | 0.31 | 4.9 | GB | 72.3 | 9.6 | 5.0 | 0.52 |
| Materials | /(kg·m−3) | /(kg·m−3) | /(m·s−1) | /(MPa) | /(MPa) | n | |
|---|---|---|---|---|---|---|---|
| Pumice | 550 | 2237 | 4.23 | 100 | 1 | 21.3 | 3 |
| Porous basalt | 2013 | 2876 | 1.43 | 1680 | 1 | 1000 | 3 |
| Materials | ρ/(g·cm−3) | /(km·s−1) | s | γ |
|---|---|---|---|---|
| Al2024 | 2.785 | 5.35 | 1.34 | 2 |
| Basalt | 2.876 | 2.4 | 1.6 | 1 |
| Pumice | 0.55 | 0.54 | 1.347 | 1 |
| Materials | G0/GPa | Y0/GPa | Ymax/GPa | n | /(MPa·K−1) | /K | |||
|---|---|---|---|---|---|---|---|---|---|
| Al2024 | 28.6 | 0.26 | 0.76 | 310 | 0.185 | 1.8647 | −17.62 | 0.01695 | 1220 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, W.; Zhang, Q.; Long, R.; Ren, J.; Li, J.; Gong, Z.; Wu, Q.; Ren, S. Experimental and Numerical Simulation of Ejecta Size and Velocity of Hypervelocity Impact Rubble-Pile Asteroid. Aerospace 2024, 11, 621. https://doi.org/10.3390/aerospace11080621
Liu W, Zhang Q, Long R, Ren J, Li J, Gong Z, Wu Q, Ren S. Experimental and Numerical Simulation of Ejecta Size and Velocity of Hypervelocity Impact Rubble-Pile Asteroid. Aerospace. 2024; 11(8):621. https://doi.org/10.3390/aerospace11080621
Chicago/Turabian StyleLiu, Wenjin, Qingming Zhang, Renrong Long, Jiankang Ren, Juncheng Li, Zizheng Gong, Qiang Wu, and Siyuan Ren. 2024. "Experimental and Numerical Simulation of Ejecta Size and Velocity of Hypervelocity Impact Rubble-Pile Asteroid" Aerospace 11, no. 8: 621. https://doi.org/10.3390/aerospace11080621
APA StyleLiu, W., Zhang, Q., Long, R., Ren, J., Li, J., Gong, Z., Wu, Q., & Ren, S. (2024). Experimental and Numerical Simulation of Ejecta Size and Velocity of Hypervelocity Impact Rubble-Pile Asteroid. Aerospace, 11(8), 621. https://doi.org/10.3390/aerospace11080621





