Long-Duration Dynamic Numerical Simulation of Combustion and Flow in Hybrid Rocket Motors Considering Nozzle Erosion
Abstract
1. Introduction
2. Transient Simulation Models
2.1. Gas Governing Equation
2.2. Turbulent Model
2.3. Gas–Solid Coupling Model
2.4. Solid Fuel Pyrolysis Model
2.5. Chemical Reaction Model
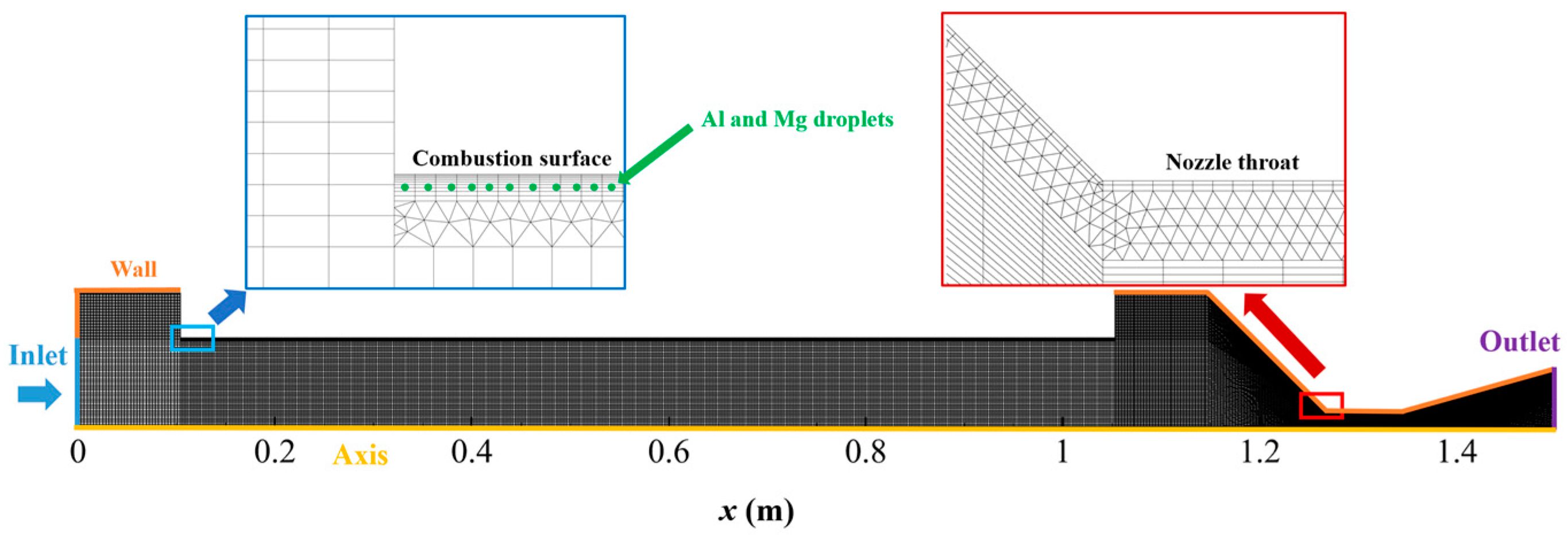
2.6. Discrete Phase Model
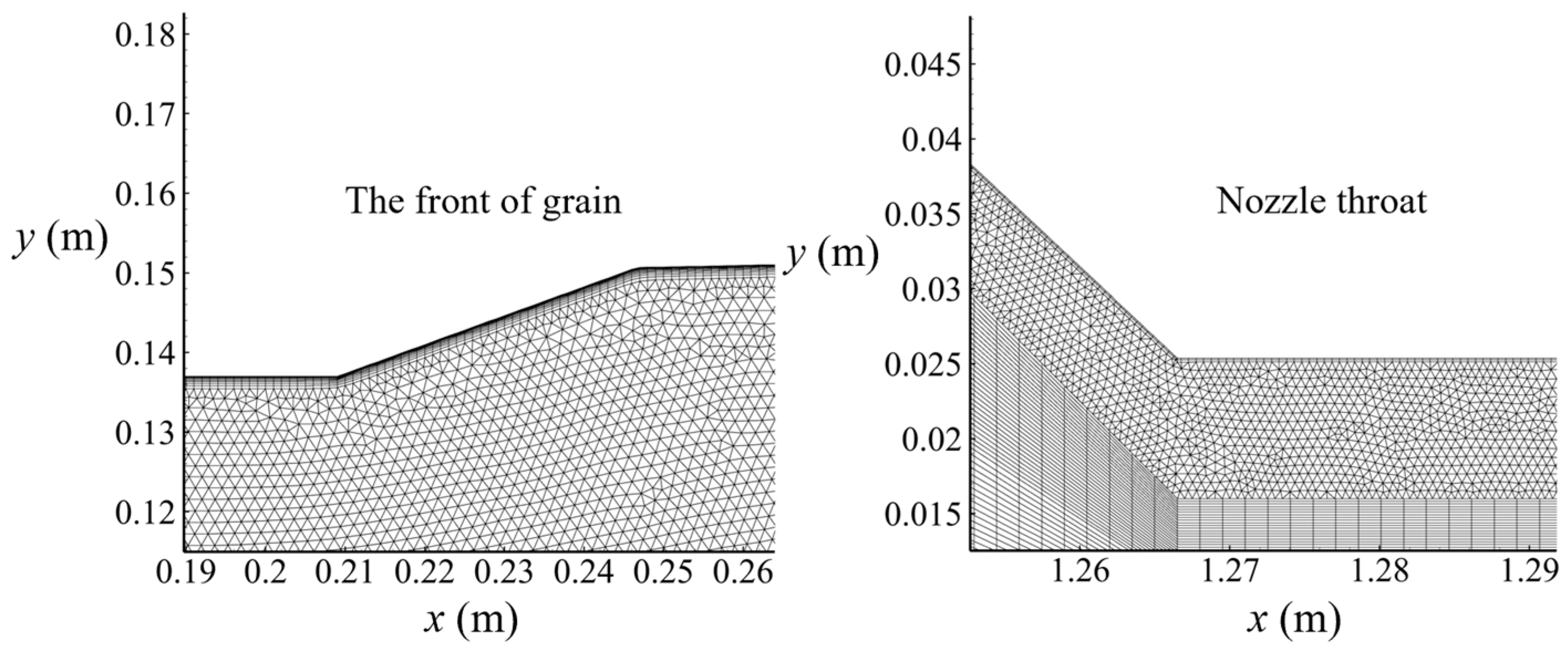
2.7. Mesh Grid
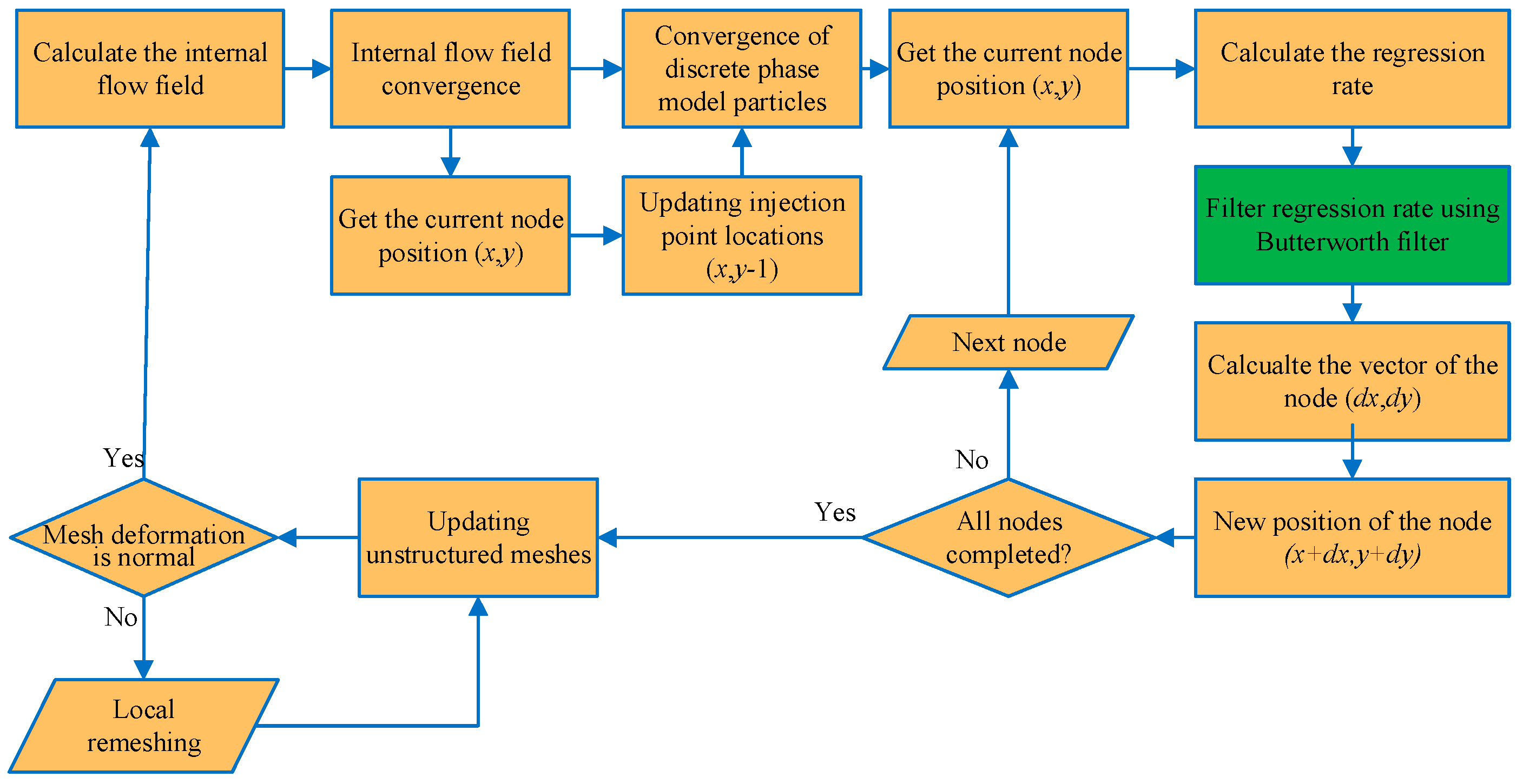
2.8. Dynamic Mesh Model
2.9. Boundary Conditions
3. Experimental System
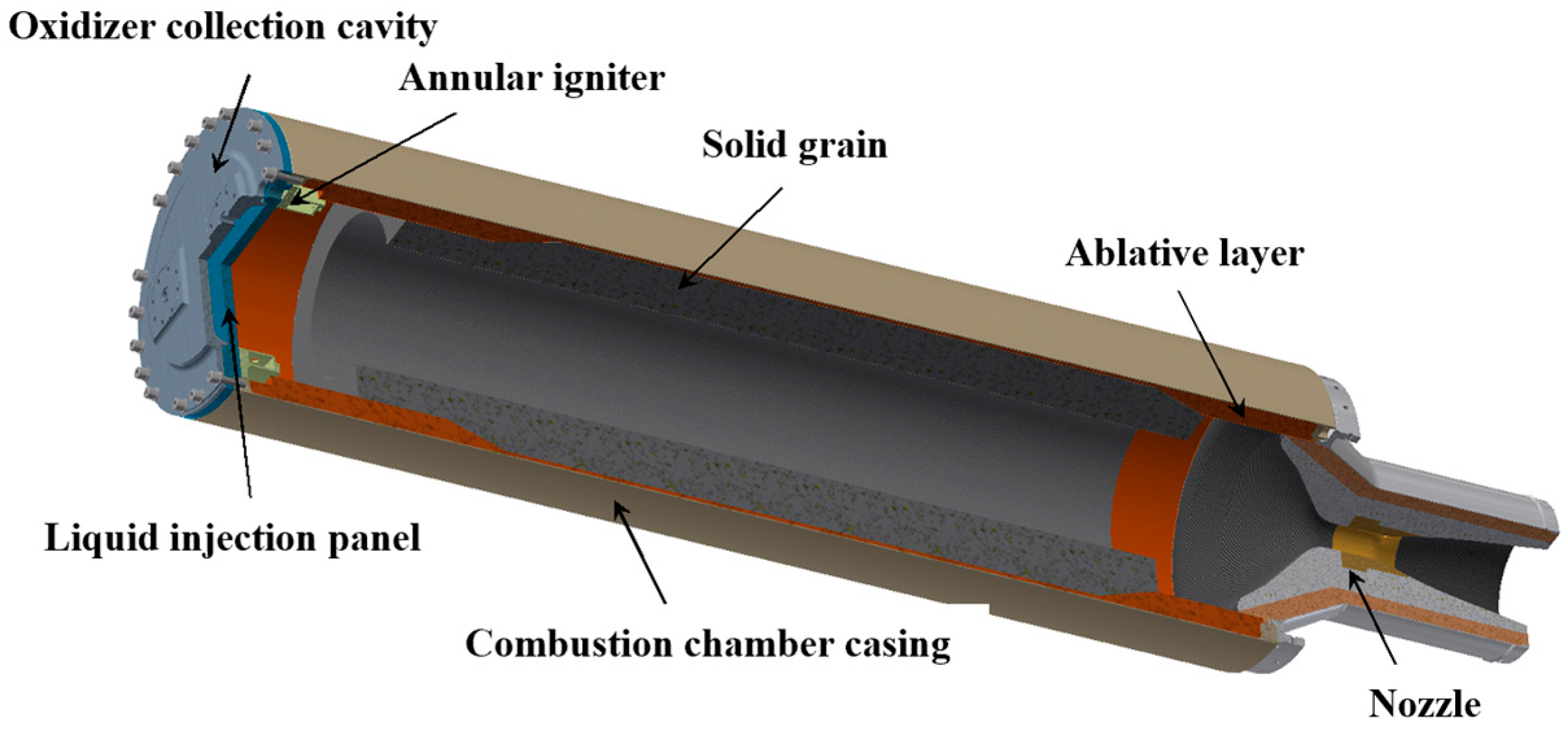
3.1. Hybrid Rocket Motor
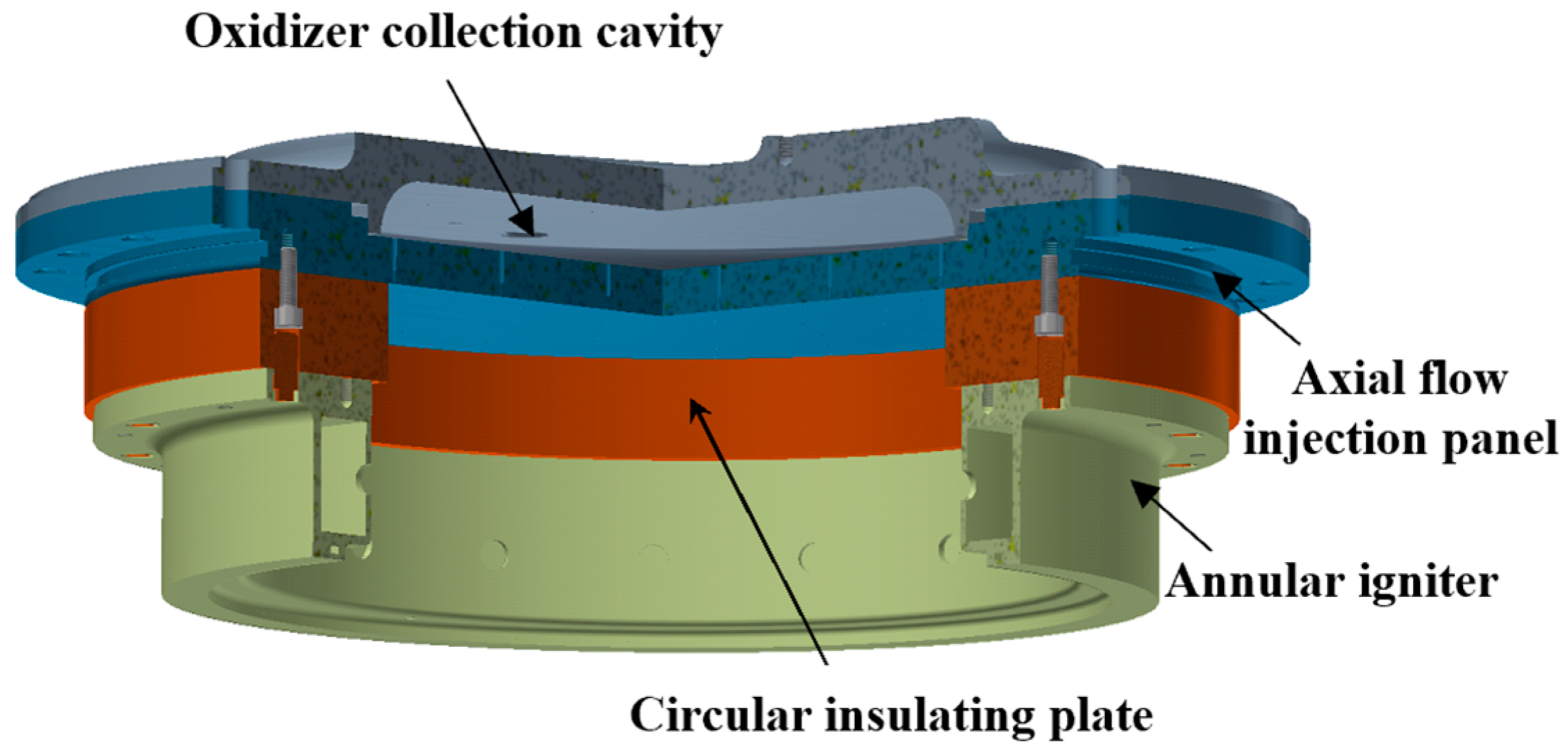
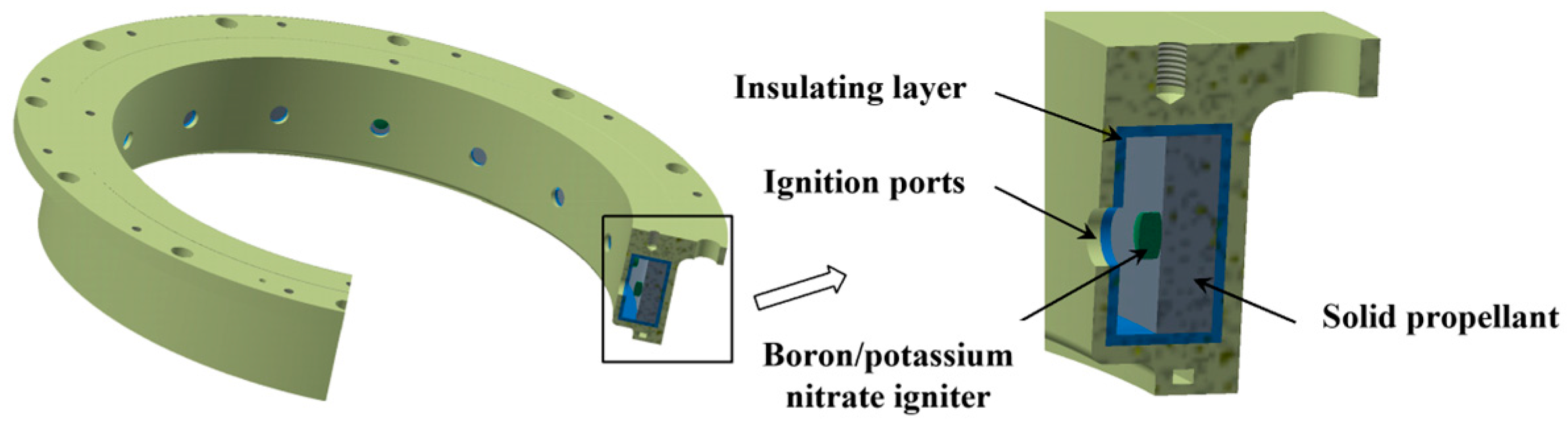
3.1.1. Motor Head Structure
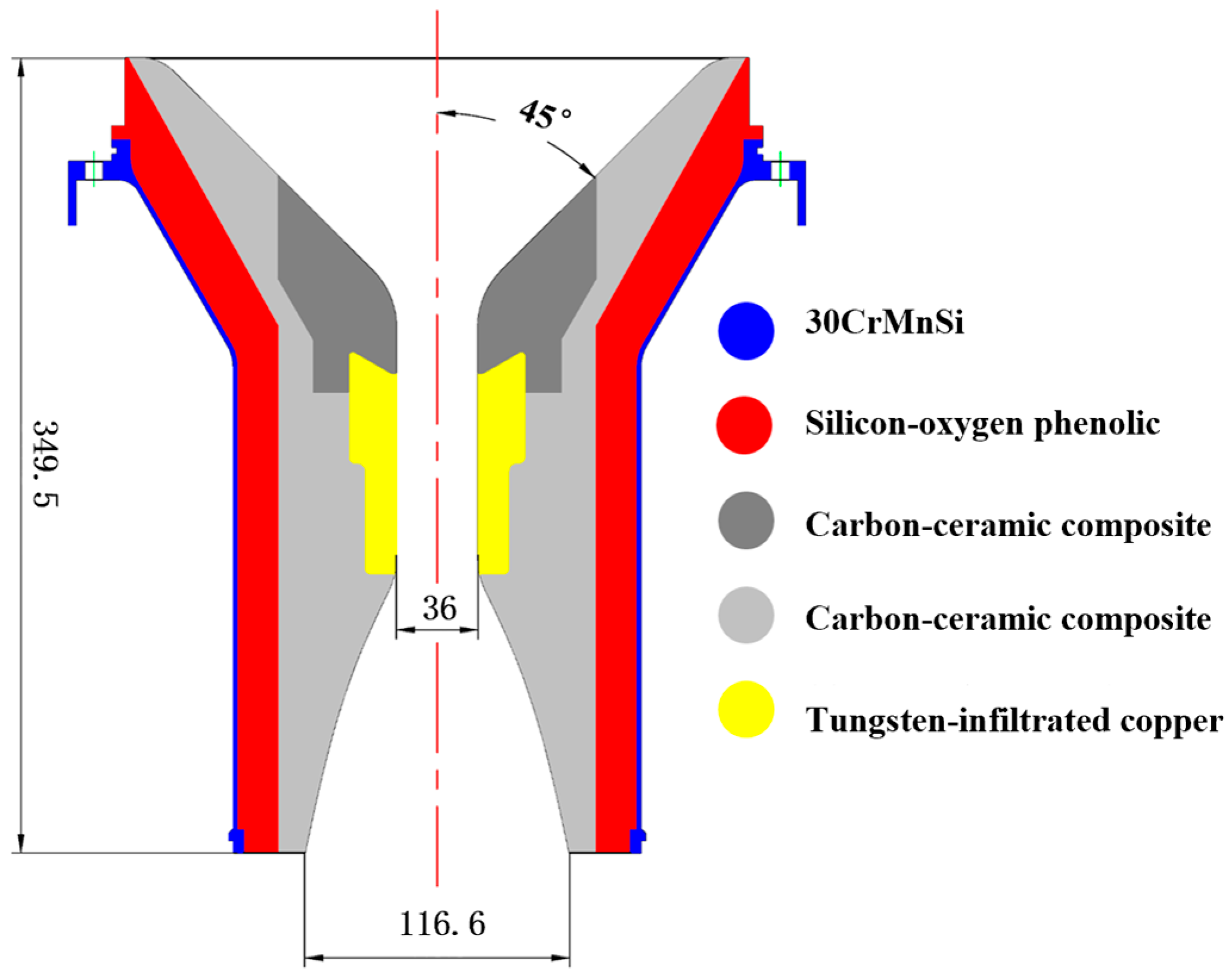
3.1.2. Motor Nozzle
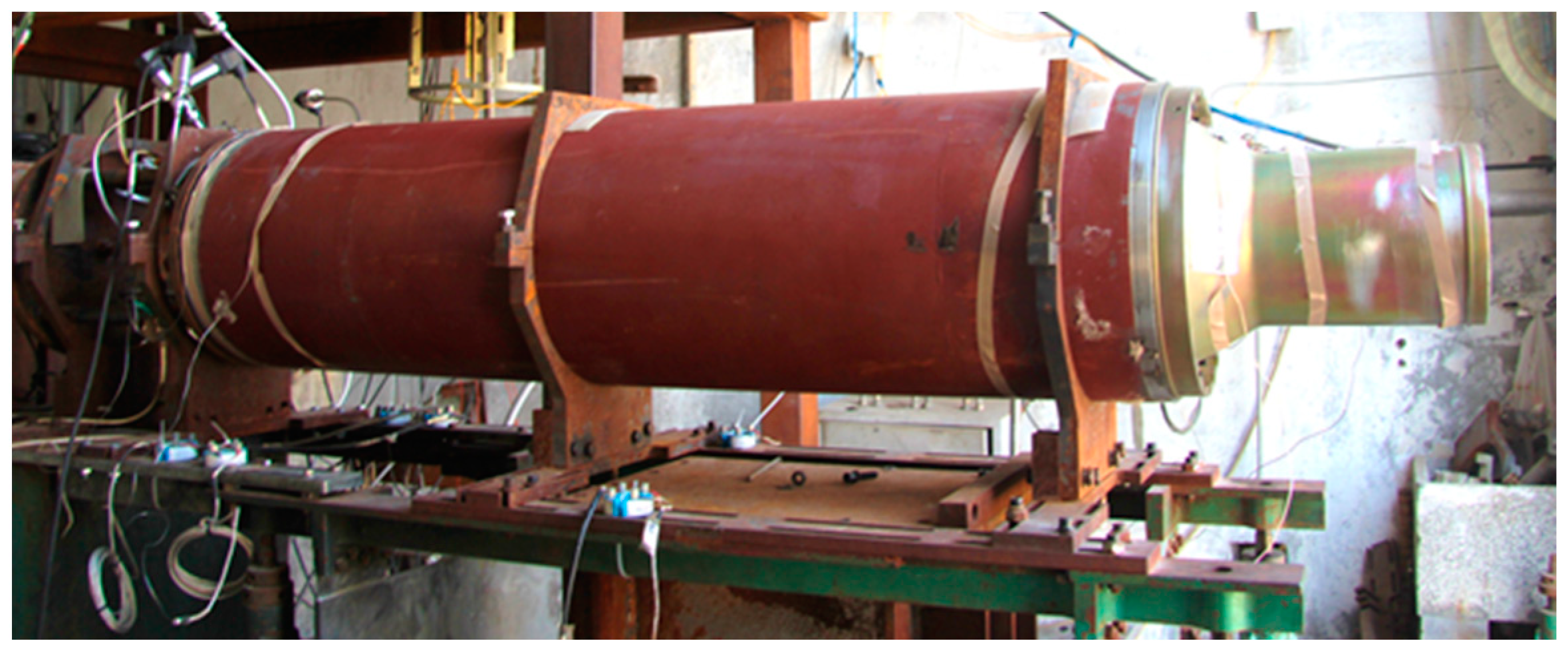
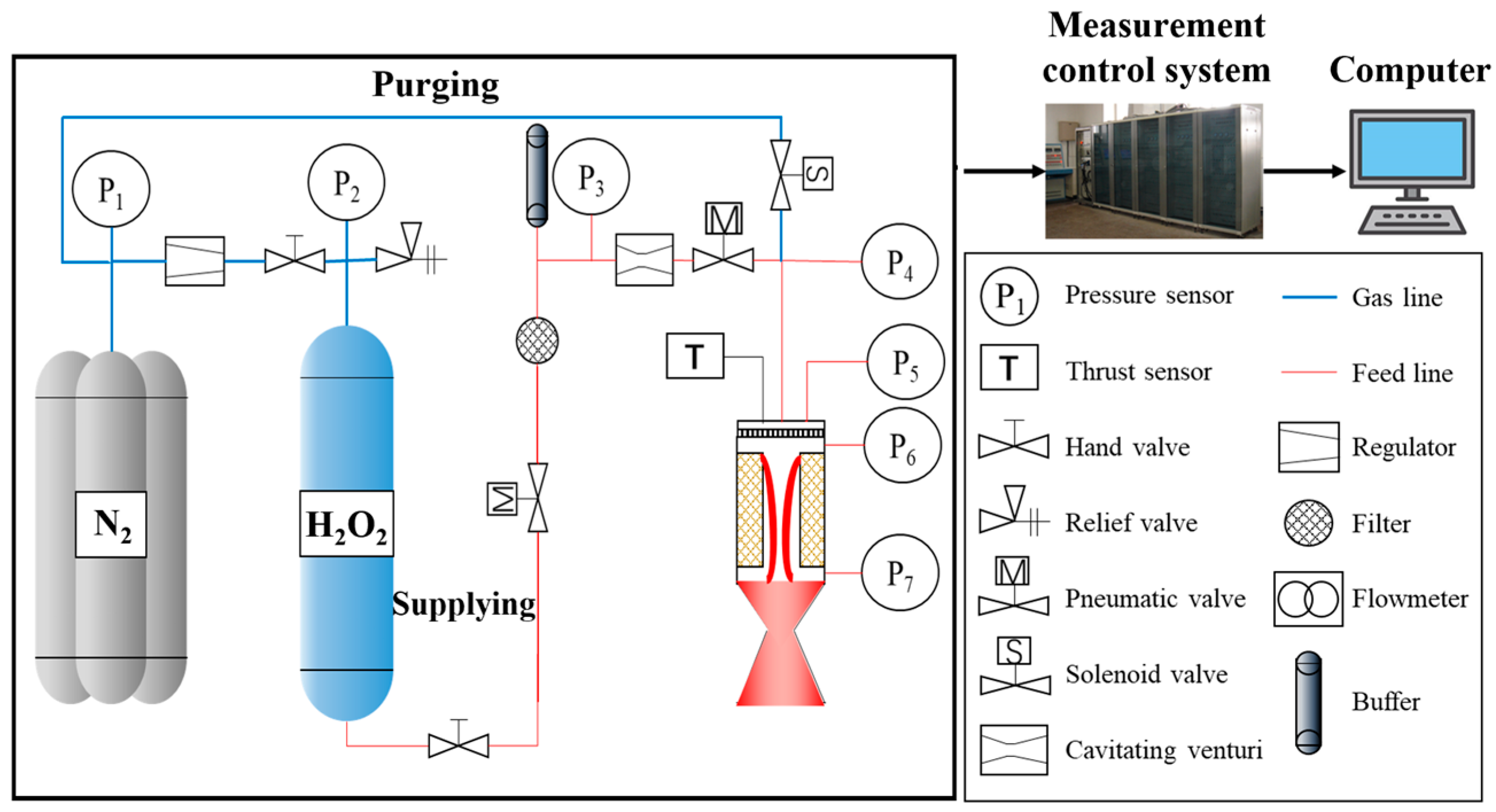
3.2. Ground Test System
4. Results and Discussions
4.1. Grid and Time Step Independence Verification
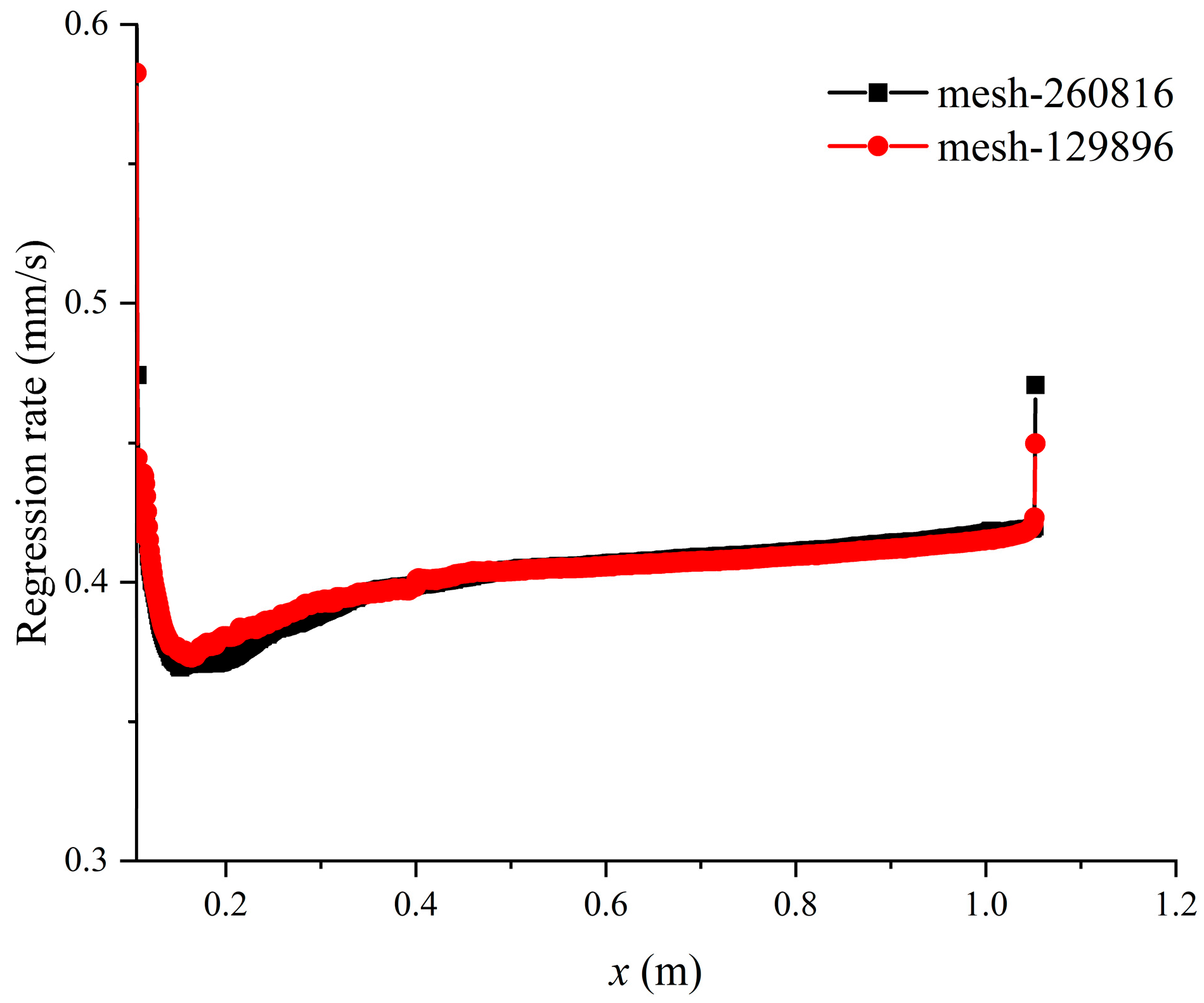
4.1.1. Grid Independence Verification
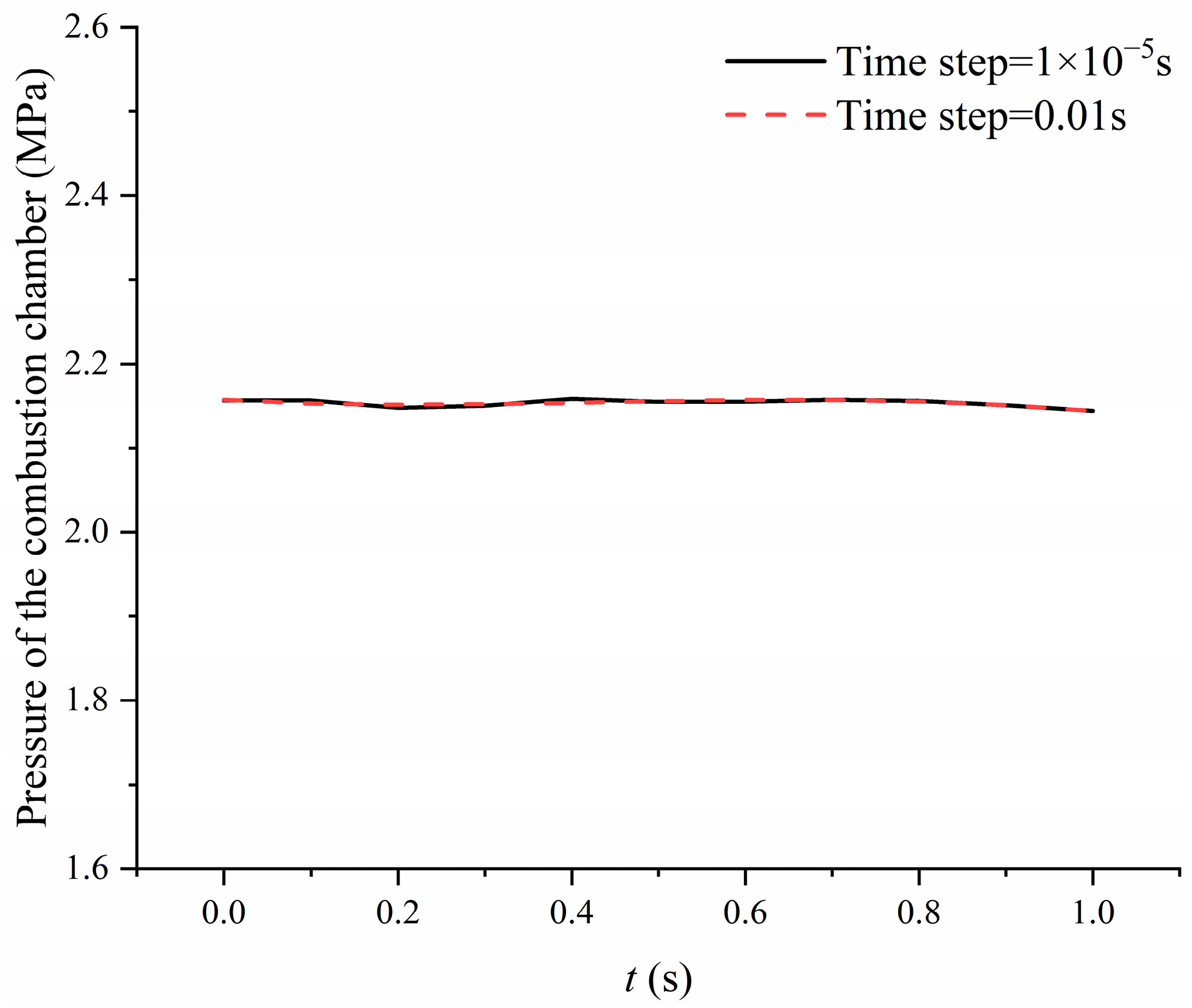
4.1.2. Time Step Independence Verification
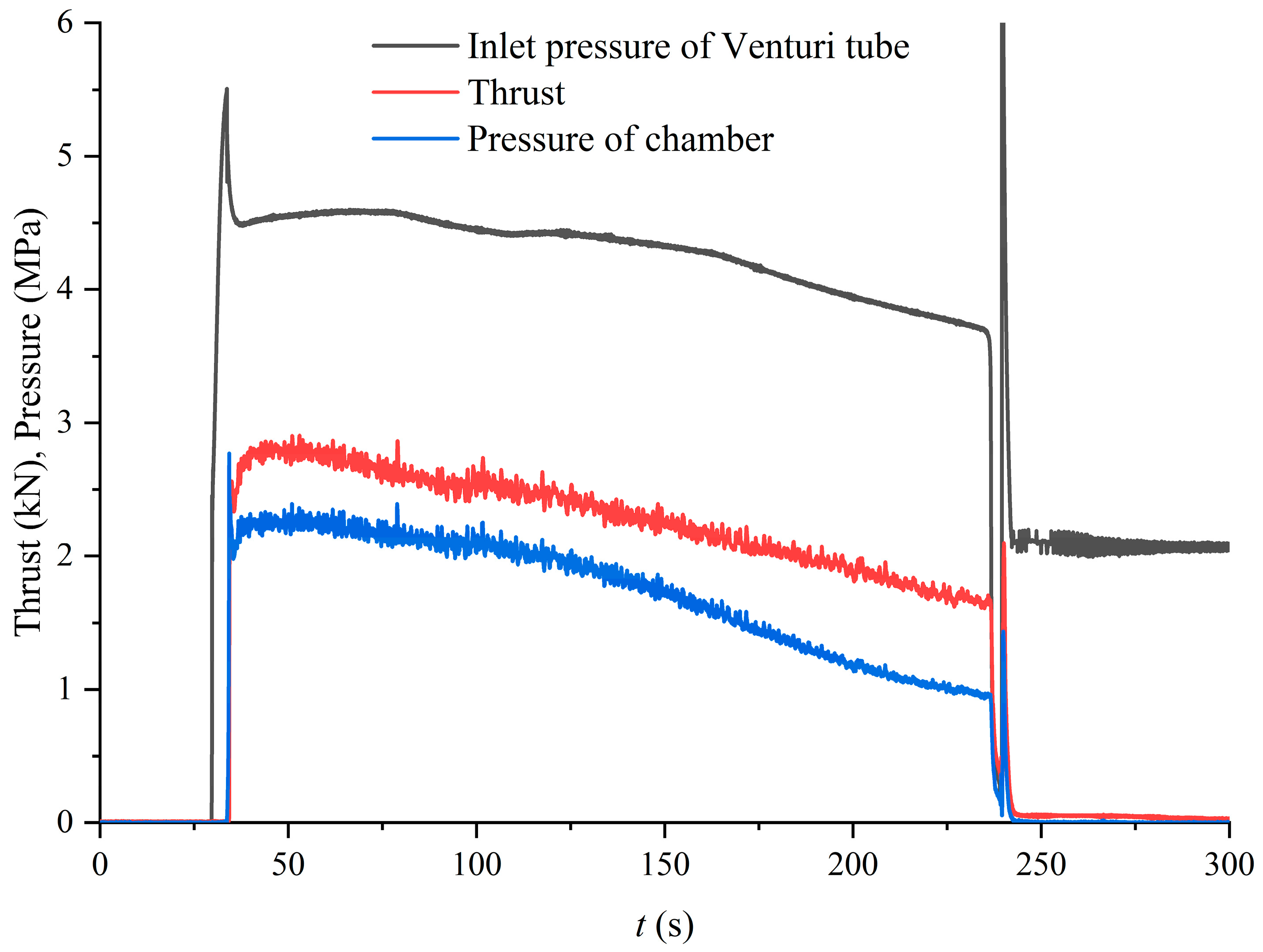
4.2. Experimental Results
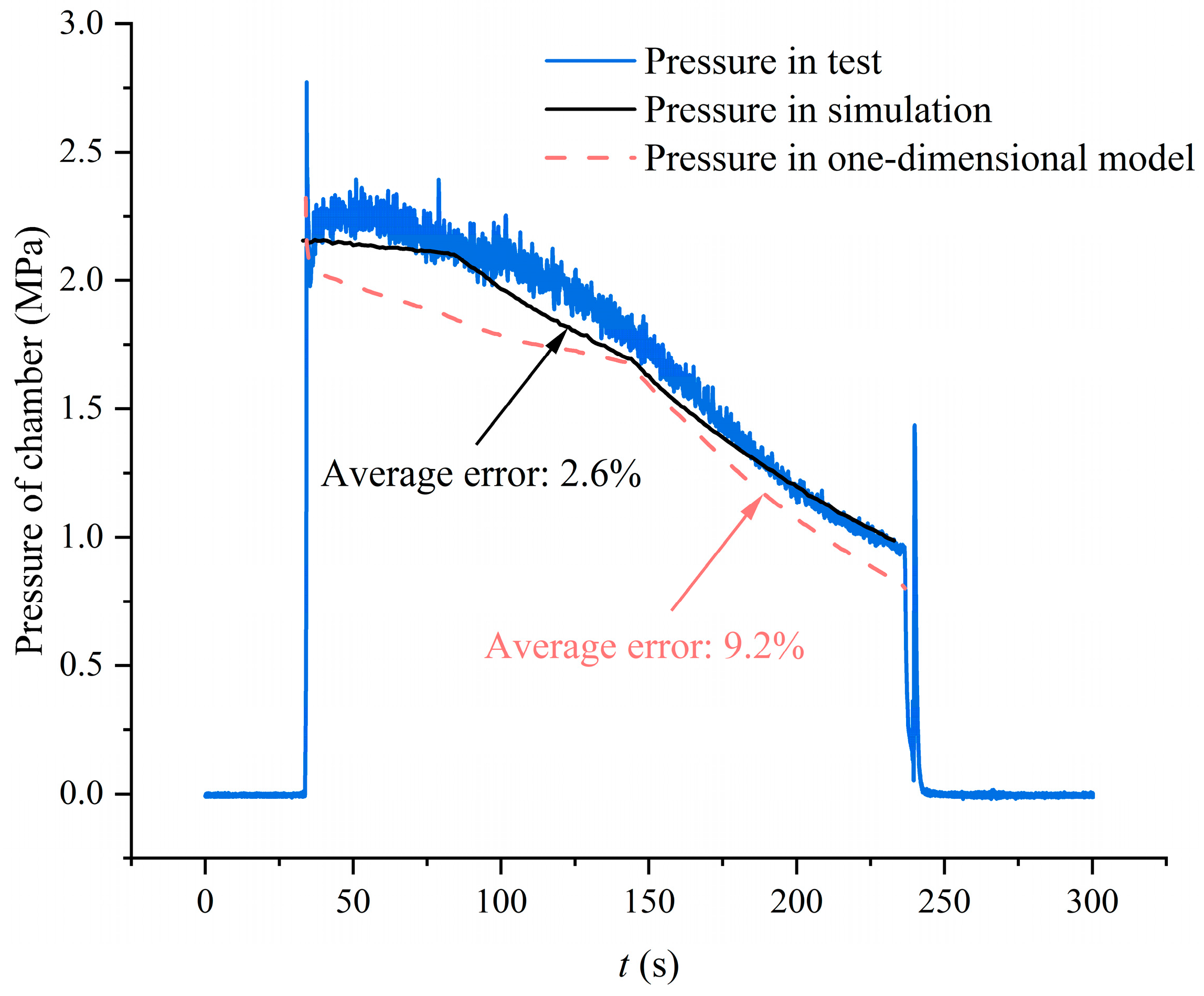
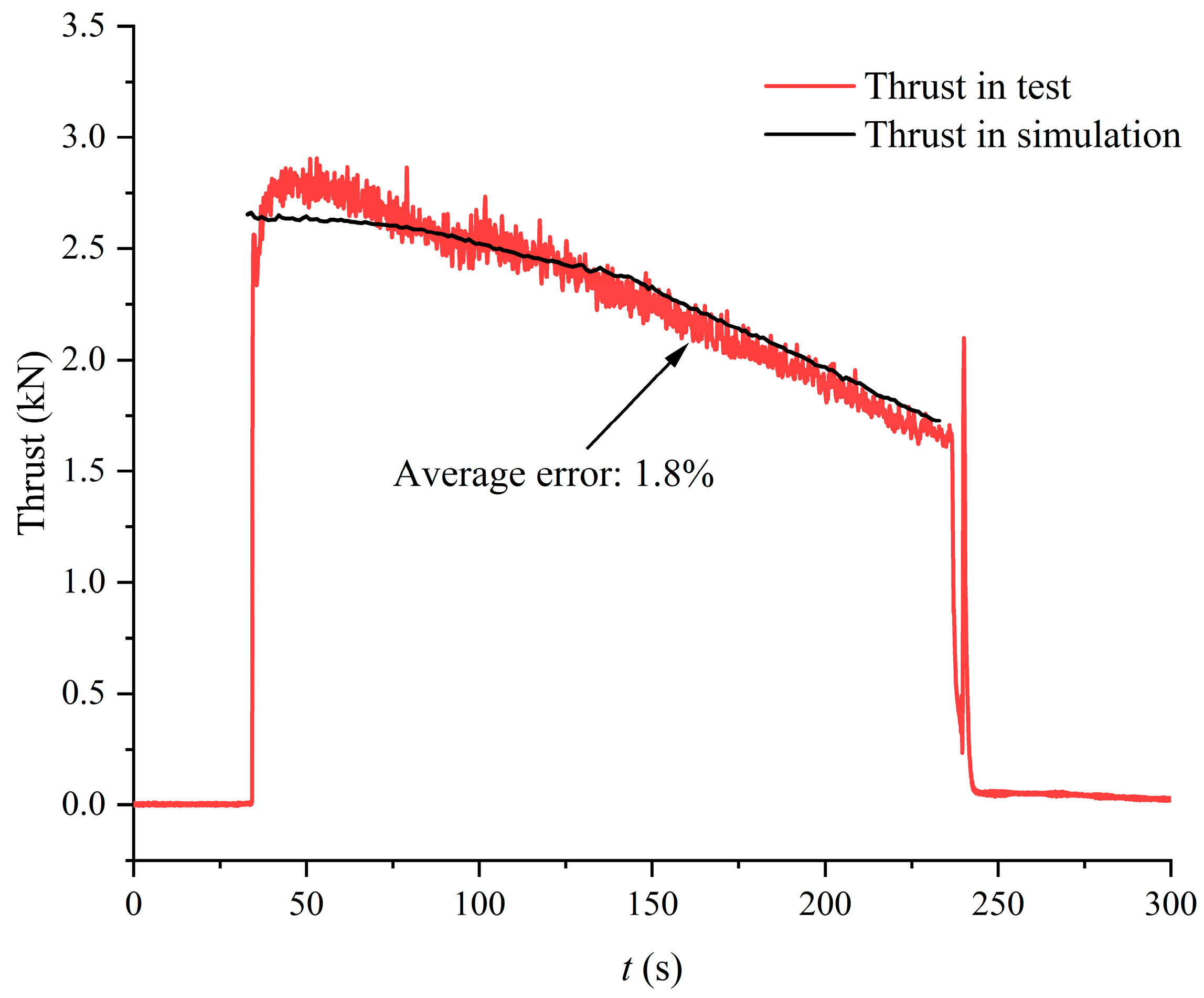
4.3. Comparison Analysis of Long-Term Simulation and Experimental Data
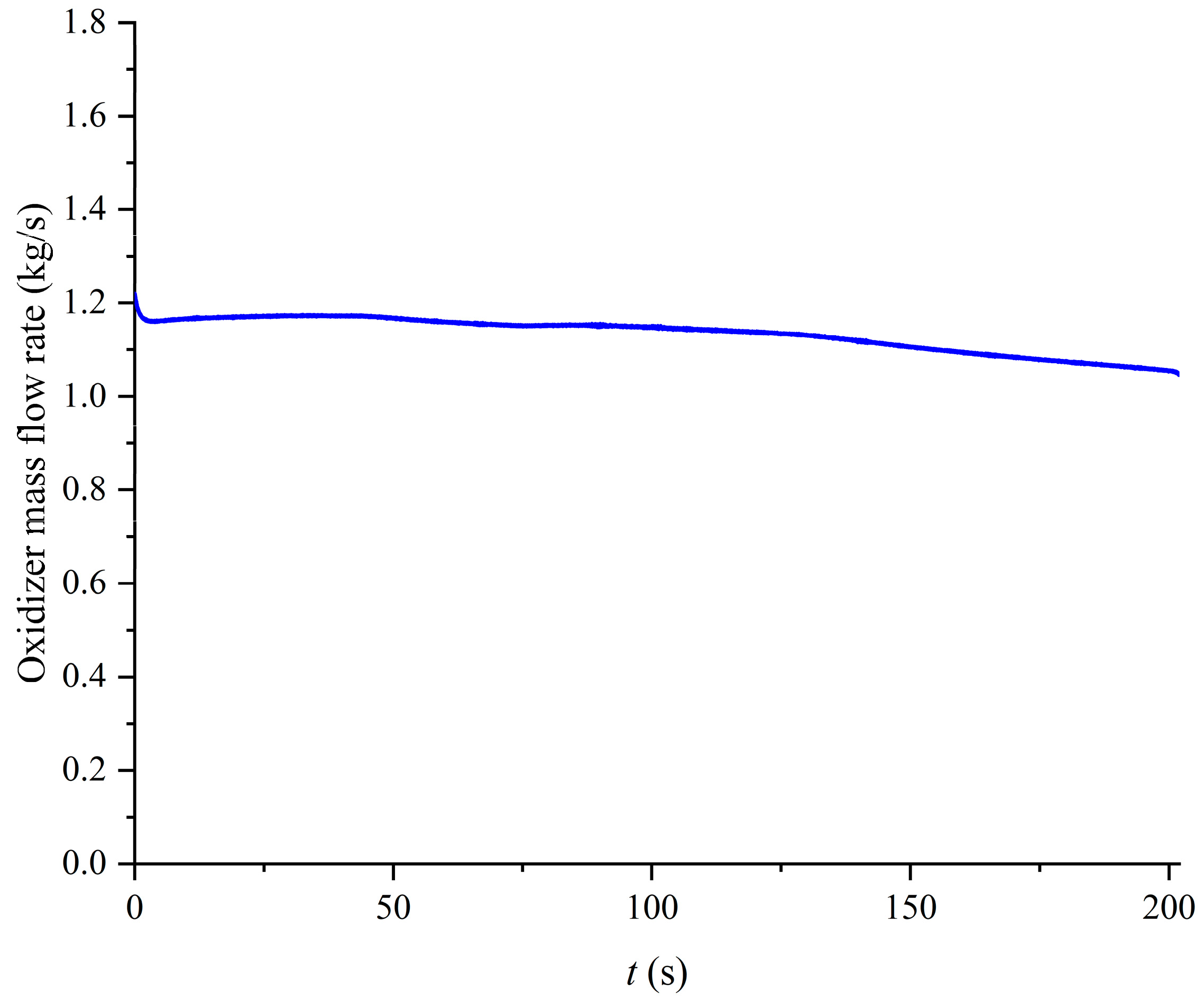
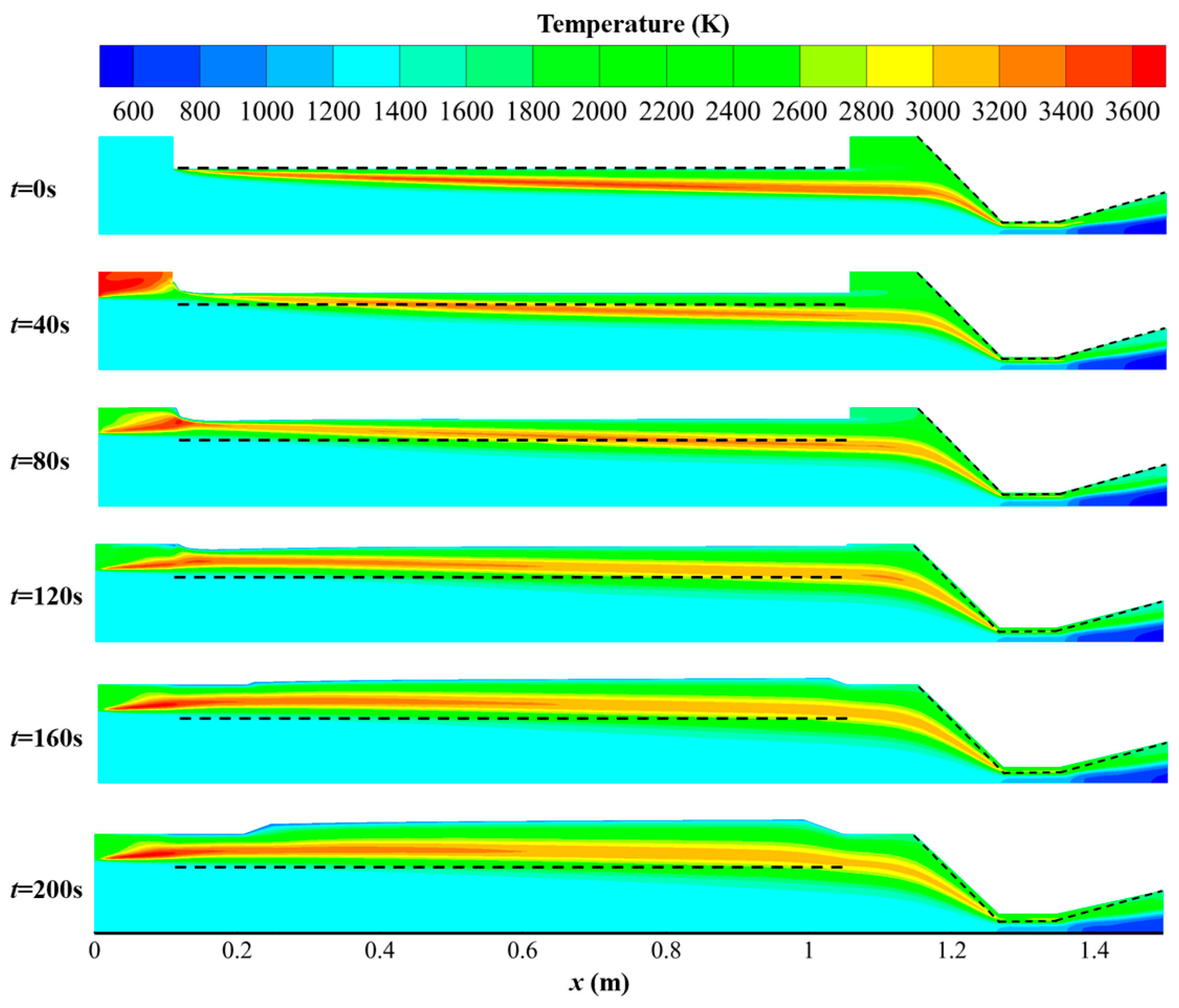
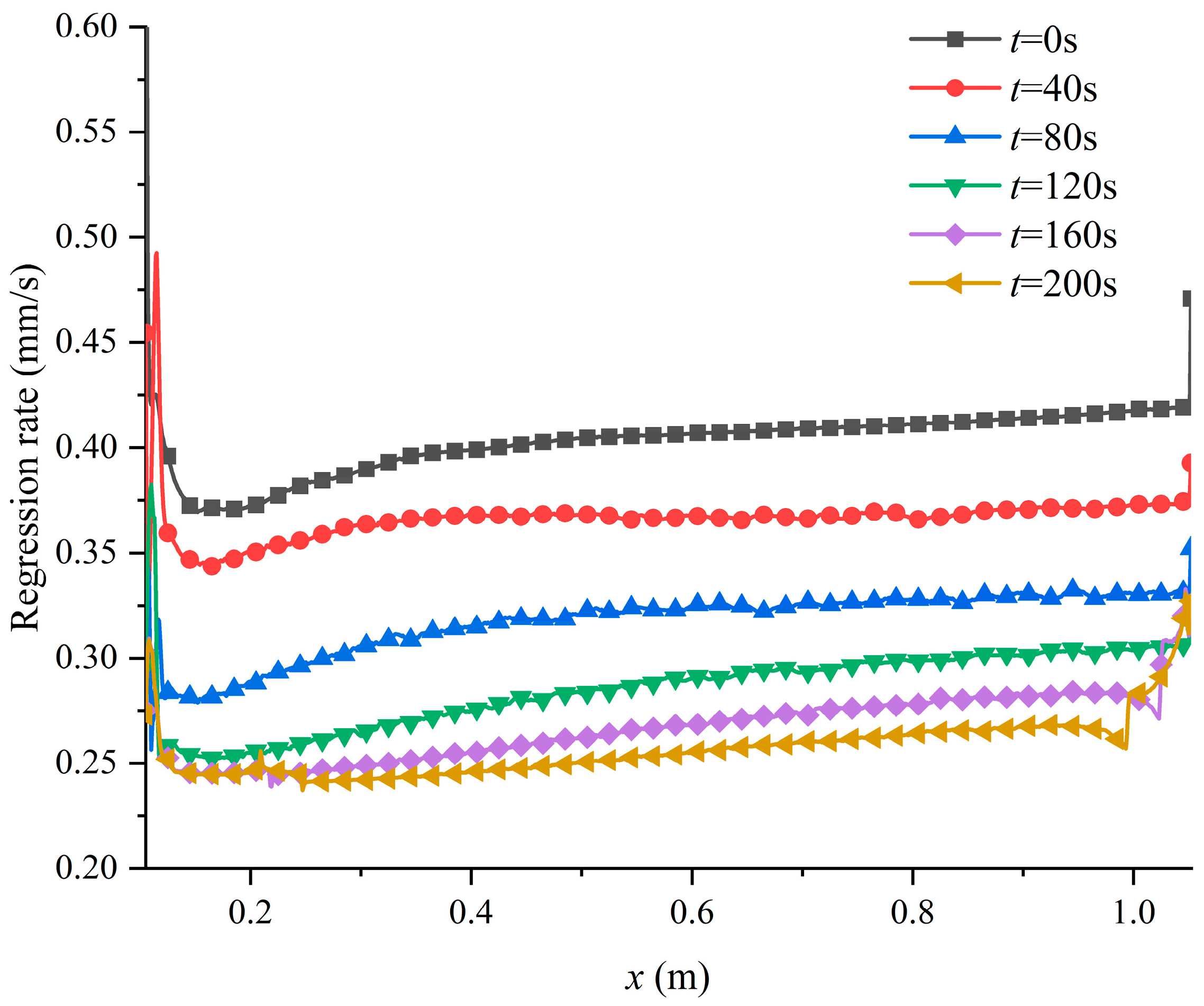
4.4. Flow Field Parameter Analysis in Long-Term Dynamic Numerical Simulation
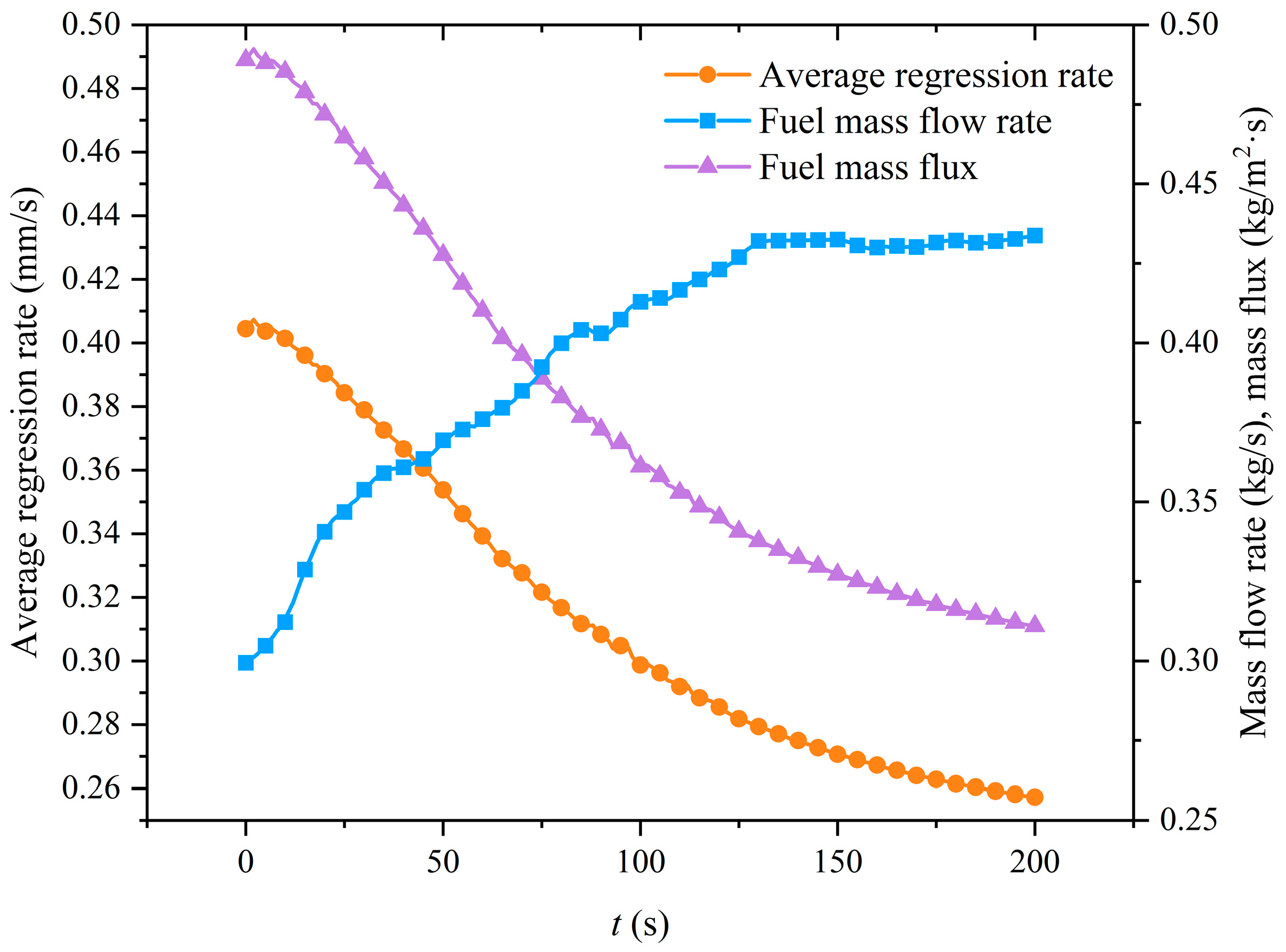
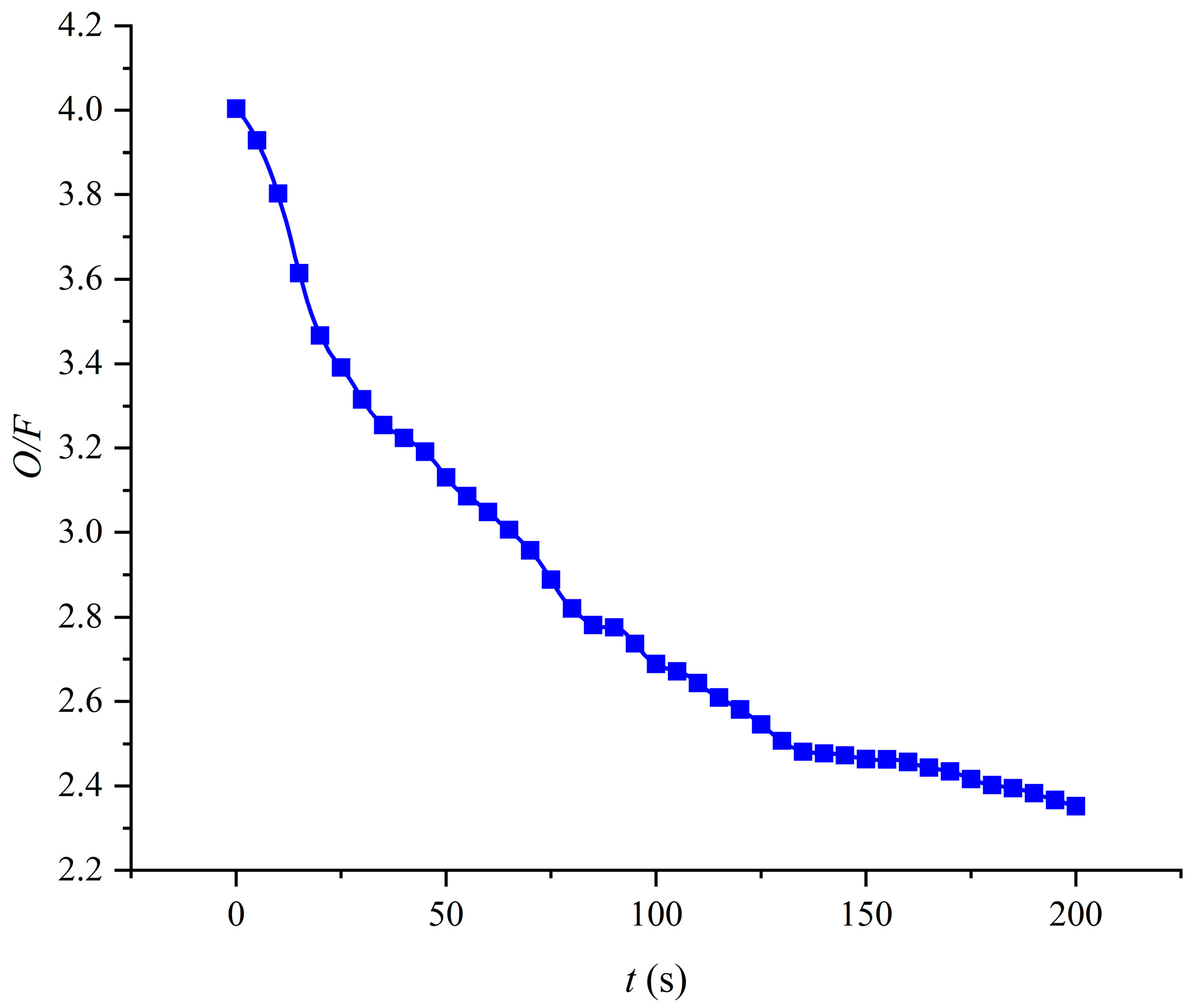
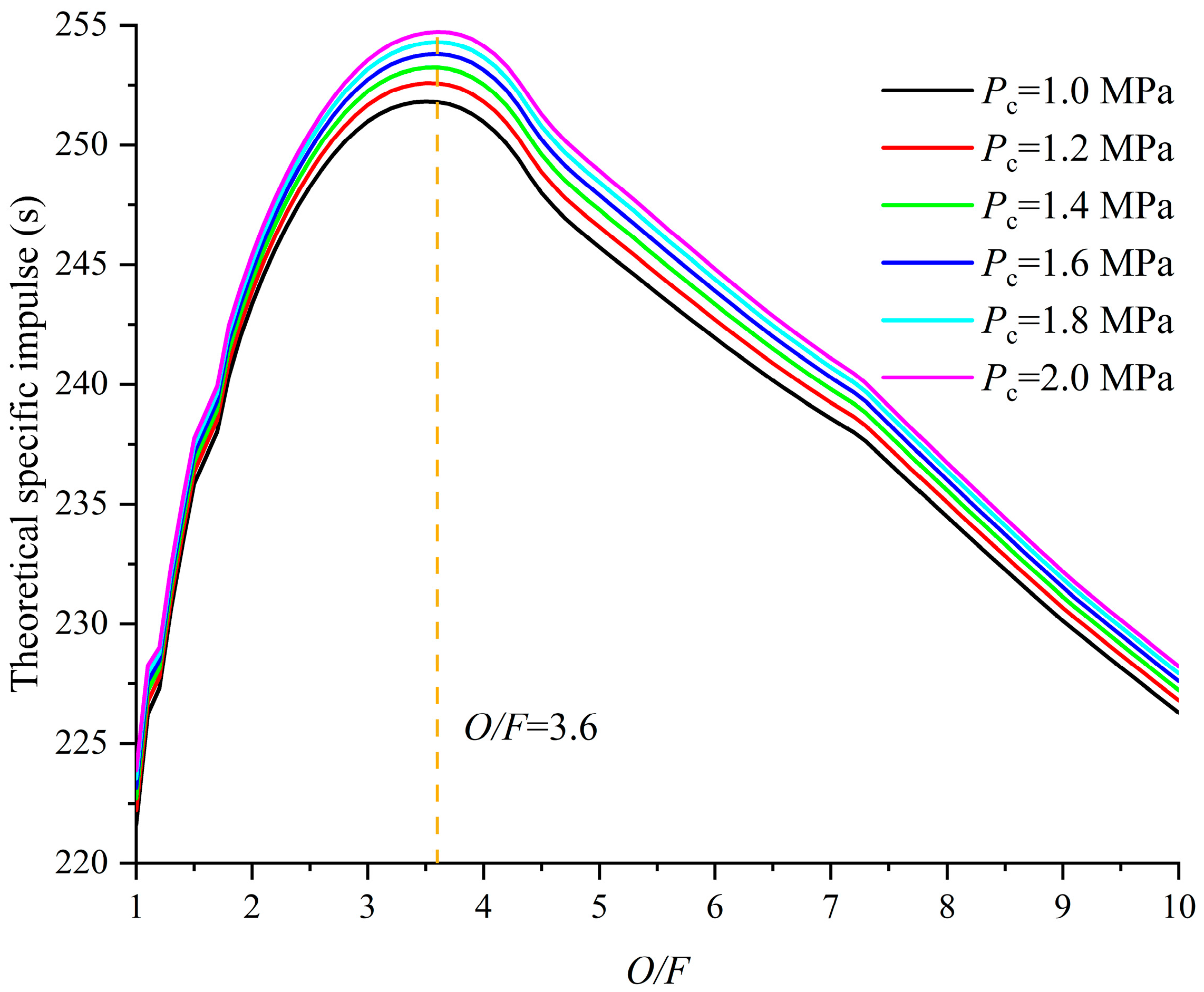
4.5. Performance Parameter Analysis in Long-Term Dynamic Numerical Simulation
5. Conclusions
- (1)
- The numerical simulation model provides insight into the distribution changes of internal flow field parameters during long-duration dynamic numerical simulations, guiding the hybrid rocket motor design. For example, the high-temperature region in the pre-combustion chamber poses a significant challenge to thermal protection, leading to the incorporation of a ring-shaped insulating plate at the head.
- (2)
- The behavior of Al droplets and Mg droplets in the flow field differs significantly over the long-term simulation, with Al droplets unable to fully vaporize in the combustion chamber due to higher boiling points and vaporization heat. In contrast, Mg droplets, with lower boiling points and vaporization heat, can completely vaporize in the combustion chamber. The longest residence time of Al and Mg droplets in the flow field differs by approximately one order of magnitude.
- (3)
- The dynamic numerical simulation study, considering nozzle erosion, significantly improves performance prediction accuracy. The average error in motor thrust is less than 1.8%, and the average error in combustion chamber pressure is less than 2.6% during the 200-s long-duration simulation, demonstrating the accuracy of the numerical simulation model, which lays a good foundation for accurate performance prediction of long-duration hybrid rocket motors.
- (4)
- Both simulation and experimental results indicated a gradual decrease in specific impulse and characteristic velocity over the extended operation period. The main reason for this is the gradual deviation of the oxygen-fuel ratio from the optimum ratio during the operation. The model can guide the design of hybrid rocket motors and optimize their design parameters. At the same time, this study can also lay the foundation for the trajectory prediction of long-duration flight, which is not available in the short-duration simulation.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Meng, X.; Tian, H.; Zhu, H.; Wang, Z.; Yu, R.; Guo, Z.; Cai, G. Effects of Aluminum and Aluminum Hydride Additives on the Performance of Hybrid Rocket Motors Based on 95% Hydrogen Peroxide. Aerosp. Sci. Technol. 2022, 130, 107914. [Google Scholar] [CrossRef]
- Hyun, W.; Lee, C. Ignition Delay and Low-Frequency Coupling in Hybrid Rocket Combustion. Acta Astronaut. 2024, 214, 83–94. [Google Scholar] [CrossRef]
- Gallo, G.; Kamps, L.; Hirai, S.; Carmicino, C.; Nagata, H. One-Dimensional Modelling of the Nozzle Cooling with Cryogenic Oxygen Flowing through Helical Channels in a Hybrid Rocket. Acta Astronaut. 2023, 210, 176–196. [Google Scholar] [CrossRef]
- Okninski, A.; Kopacz, W.; Kaniewski, D.; Sobczak, K. Hybrid Rocket Propulsion Technology for Space Transportation Revisited-Propellant Solutions and Challenges. FirePhysChem 2021, 1, 260–271. [Google Scholar] [CrossRef]
- Mangeot, A.; William-Louis, M.; Gillard, P. Static and Moving Solid/Gas Interface Modeling in a Hybrid Rocket Engine. Acta Astronaut. 2018, 148, 89–98. [Google Scholar] [CrossRef]
- Zhang, S.; Hu, F.; Wang, D.; Okolo, N.P.; Zhang, W. Numerical Simulations on Unsteady Operation Processes of N2O/HTPB Hybrid Rocket Motor with/without Diaphragm. Acta Astronaut. 2017, 136, 115–124. [Google Scholar] [CrossRef]
- Di Martino, G.D.; Carmicino, C.; Mungiguerra, S.; Savino, R. The Application of Computational Thermo-Fluid-Dynamics to the Simulation of Hybrid Rocket Internal Ballistics with Classical or Liquefying Fuels: A Review. Aerospace 2019, 6, 56. [Google Scholar] [CrossRef]
- Tian, H.; Li, Y.; Zeng, P. Transient Simulation of Regression Rate on Thrust Regulation Process in Hybrid Rocket Motor. Chin. J. Aeronaut. 2014, 27, 1343–1351. [Google Scholar] [CrossRef]
- Meng, X.; Tian, H.; Chen, X.; Jiang, X.; Wang, P.; Wei, T.; Cai, G. Numerical Simulation of Combustion Surface Regression Based on Butterworth Filter in Hybrid Rocket Motor. Acta Astronaut. 2023, 202, 400–410. [Google Scholar] [CrossRef]
- Tian, H.; Meng, X.; Zhu, H.; Li, C.; Yu, R.; Zhang, Y.; Cai, G. Dynamic Characteristics Study of Regression Rate in Variable Thrust Hybrid Rocket Motor. Acta Astronaut. 2022, 193, 221–229. [Google Scholar] [CrossRef]
- Thakre, P.; Yang, V. Chemical Erosion of Refractory-Metal Nozzle Inserts in Solid-Propellant Rocket Motors. J. Propuls. Power 2009, 25, 40–50. [Google Scholar] [CrossRef]
- D’Elia, R.; Bernhart, G.; Hijlkema, J.; Cutard, T. Experimental Analysis of SiC-Based Refractory Concrete in Hybrid Rocket Nozzles. Acta Astronaut. 2016, 126, 168–177. [Google Scholar] [CrossRef]
- Fouladi, N.; Farahani, M. Numerical Investigation of Second Throat Exhaust Diffuser Performance with Thrust Optimized Parabolic Nozzles. Aerosp. Sci. Technol. 2020, 105, 106020. [Google Scholar] [CrossRef]
- Yan, D.; Wei, Z.; Xie, K.; Wang, N. Simulation of Thrust Control by Fluidic Injection and Pintle in a Solid Rocket Motor. Aerosp. Sci. Technol. 2020, 99, 105711. [Google Scholar] [CrossRef]
- Kamps, L.; Saito, Y.; Kawabata, R.; Wakita, M.; Totani, T.; Takahashi, Y.; Nagata, H. Method for Determining Nozzle-Throat-Erosion History in Hybrid Rockets. J. Propuls. Power 2017, 33, 1369–1377. [Google Scholar] [CrossRef]
- Tian, H.; Yu, R.; Li, C.; Zhao, S.; Zhu, H. Numerical and Experimental Study of the Thermochemical Erosion of a Graphite Nozzle in a Hybrid Rocket Motor with a Star Grain. Acta Astronaut. 2019, 155, 10–22. [Google Scholar] [CrossRef]
- Kamps, L.; Hirai, S.; Sakurai, K.; Viscor, T.; Saito, Y.; Guan, R. Investigation of Graphite Nozzle Erosion in Hybrid Rockets Using Oxygen/High-Density Polyethylene. J. Propuls. Power 2020, 36, 423–434. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Z.; Wang, R.; Lu, C.; Yu, R.; Tian, H. Numerical Simulation of Chemical Ablation and Mechanical Erosion in Hybrid Rocket Nozzle. Acta Astronaut. 2022, 192, 82–96. [Google Scholar] [CrossRef]
- Kim, K.-S.; Lee, S.-H.; Nguyen, V.Q.; Yun, Y.; Kwon, S. Ablation Characteristics of Rocket Nozzle Using HfC-SiC Refractory Ceramic Composite. Acta Astronaut. 2020, 173, 31–44. [Google Scholar] [CrossRef]
- Karabeyoglu, A.; Zilliac, G.; Cantwell, B.J.; Dezilwa, S.; Castellucci, P. Scale-up Tests of High Regression Rate Liquefying Hybrid Rocket Fuels. J. Propuls. Power 2004, 20, 1037–1045. [Google Scholar] [CrossRef]
- Sun, X.; Tian, H.; Li, Y.; Yu, N.; Cai, G. Regression Rate Behaviors of HTPB-Based Propellant Combinations for Hybrid Rocket Motor. Acta Astronaut. 2016, 119, 137–146. [Google Scholar] [CrossRef]
- Tian, H.; He, L.; Yu, R.; Zhao, S.; Wang, P.; Cai, G.; Zhang, Y. Transient Investigation of Nozzle Erosion in a Long-Time Working Hybrid Rocket Motor. Aerosp. Sci. Technol. 2021, 118, 106978. [Google Scholar] [CrossRef]
- Cai, G.; Zhao, Z.; Zhao, B.; Liu, Y.; Yu, N. Regression Rate and Combustion Performance Investigation on Hybrid Rocket Motor with Head-End Swirl Injection under High Geometric Swirl Number. Aerosp. Sci. Technol. 2020, 103, 105922. [Google Scholar] [CrossRef]
- Xintian, L.I.; Hui, T.; Guobiao, C. Numerical Analysis of Fuel Regression Rate Distribution Characteristics in Hybrid Rocket Motors with Different Fuel Types. Sci. China Technol. Sci. 2013, 56, 1807–1817. [Google Scholar]
- Li, C.; Cai, G.; Tian, H. Numerical Analysis of Combustion Characteristics of Hybrid Rocket Motor with Multi-Section Swirl Injection. Acta Astronaut. 2016, 123, 26–36. [Google Scholar] [CrossRef]
- Tian, H.; Duan, Y.; Zhu, H. Three-Dimensional Numerical Analysis on Combustion Performance and Flow of Hybrid Rocket Motor with Multi-Segmented Grain. Chin. J. Aeronaut. 2020, 33, 1181–1191. [Google Scholar] [CrossRef]
- Sun, X.; Tian, H.; Yu, N.; Cai, G. Regression Rate and Combustion Performance Investigation of Aluminum Metallized HTPB/98HP Hybrid Rocket Motor with Numerical Simulation. Aerosp. Sci. Technol. 2015, 42, 287–296. [Google Scholar] [CrossRef]
- Guo, Z.; Tian, H.; Wang, Z.; Meng, X.; Cai, G. Numerical and Experimental Study on 95% Hydrogen Peroxide Catalytic Ignition of Hybrid Rocket Motors with HTPB-Based Aluminum Additive Fuel. Acta Astronaut. 2022, 195, 98–108. [Google Scholar] [CrossRef]
- Tian, H.; Yu, R.; Zhu, H.; Wu, J.; Cai, G. Three-Dimensional Numerical and Experimental Studies on Transient Ignition of Hybrid Rocket Motor. Acta Astronaut. 2017, 140, 247–254. [Google Scholar] [CrossRef]
- Bianchi, D.; Betti, B.; Nasuti, F.; Carmicino, C. Simulation of Gaseous Oxygen/Hydroxyl-Terminated Polybutadiene Hybrid Rocket Flowfields and Comparison with Experiments. J. Propuls. Power 2015, 31, 919–929. [Google Scholar] [CrossRef]
- Chiaverini, M.J.; Kuo, K.K.; Peretz, A.; Harting, G.C. Regression-Rate and Heat-Transfer Correlations for Hybrid Rocket Combustion. J. Propuls. Power 2001, 17, 99–110. [Google Scholar] [CrossRef]
- Rocket Propulsion Software+Engineering UG. Available online: https://www.rocket-propulsion.com/index.htm (accessed on 17 March 2024).
- Meng, X.; Gao, J.; Tian, H.; Niu, X.; Chen, R.; Cai, G. Study on the Dynamic Numerical Simulation of Flow and Combustion in Hybrid Rocket Motors Based on a Discrete Phase Model. Acta Astronaut. 2024, 215, 156–167. [Google Scholar] [CrossRef]
- Cheng, G.; Farmer, R.; Jones, H.; McFarlane, J. Numerical Simulation of the Internal Ballistics of a Hybrid Rocket Motor. In 32nd Aerospace Sciences Meeting and Exhibit; Aerospace Sciences Meetings; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1994. [Google Scholar] [CrossRef]
- Li, X.T.; Zeng, P.; Tian, H.; Cai, G.B. Instantaneous Fuel Regression Rate Analysis of Hybrid Rocket Motor Experiment. Tuijin Jishu/J. Propuls. Technol. 2012, 33, 211–215. [Google Scholar]
- Bandyopadhyay, A.; Majumdar, A. Network Flow Simulation of Fluid Transients in Rocket Propulsion Systems. J. Propuls. Power 2014, 30, 1646–1653. [Google Scholar] [CrossRef]





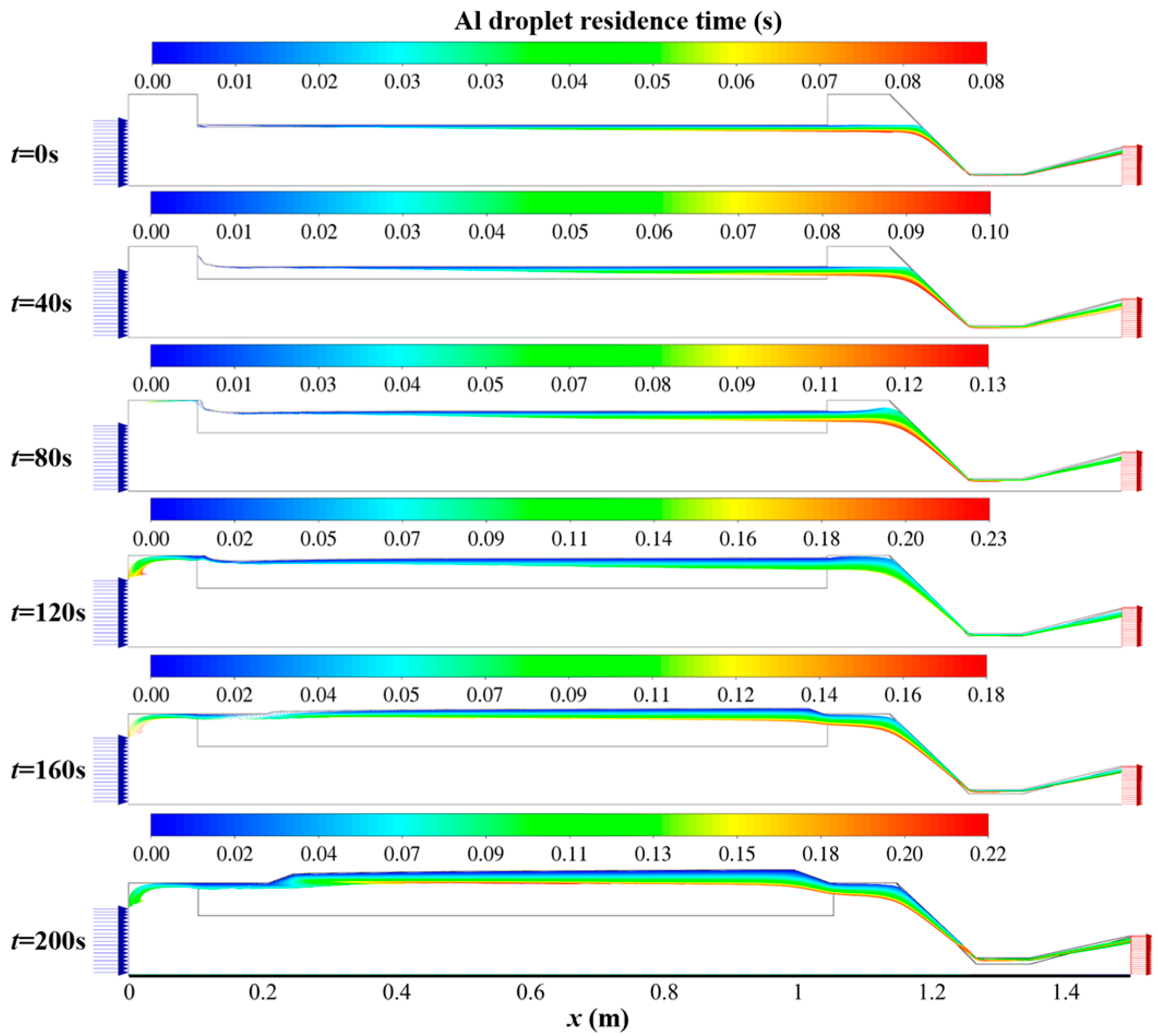
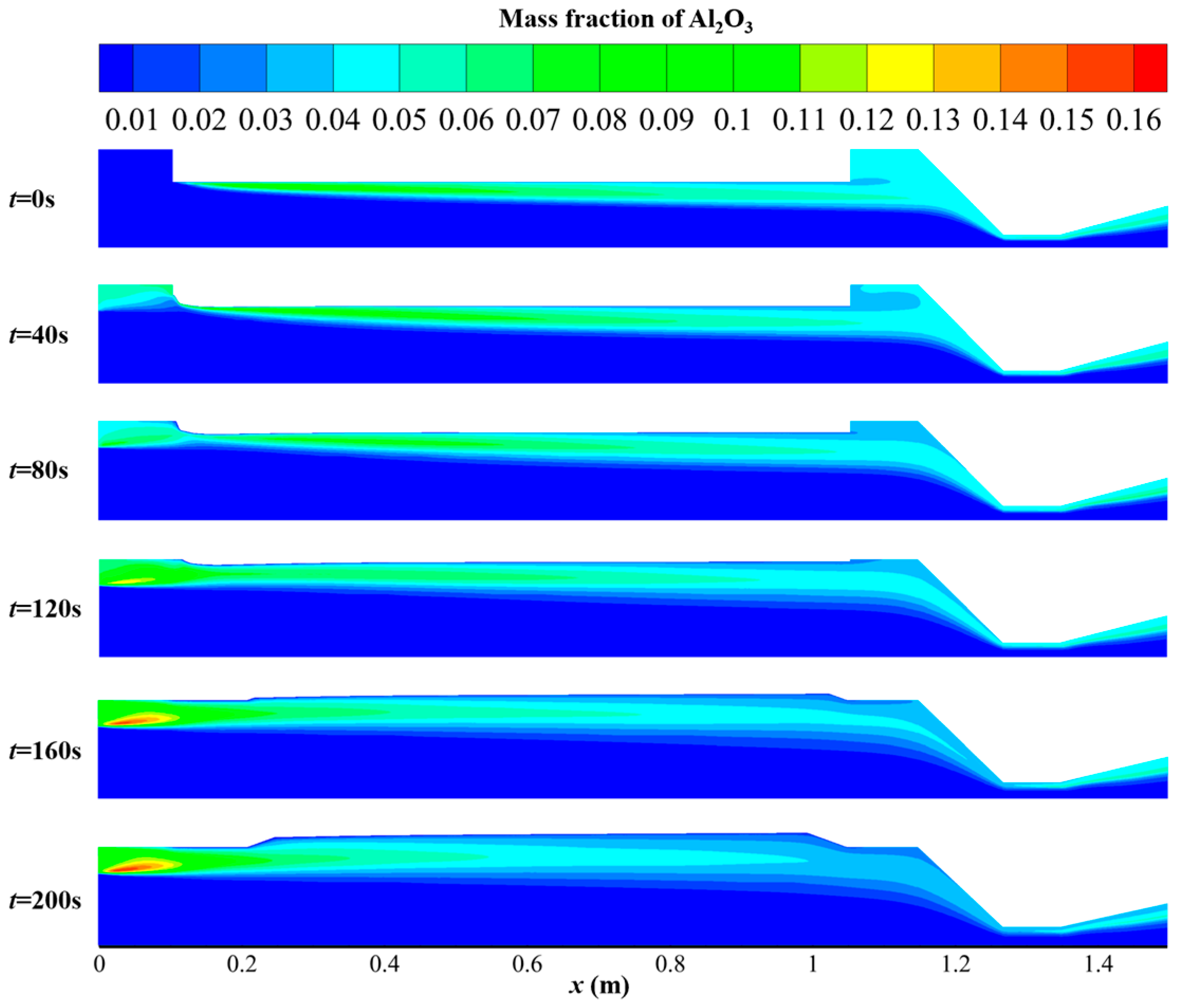
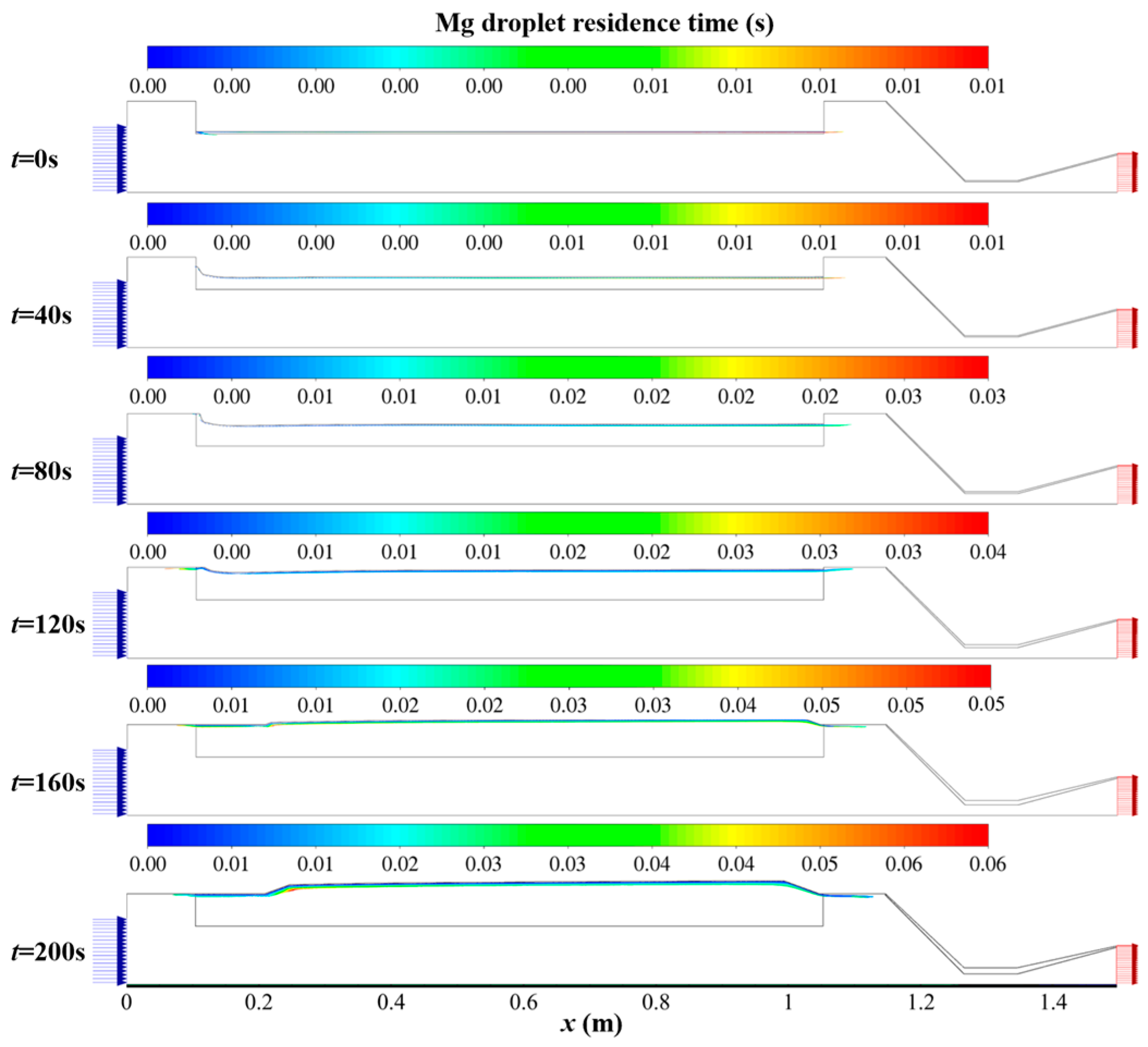
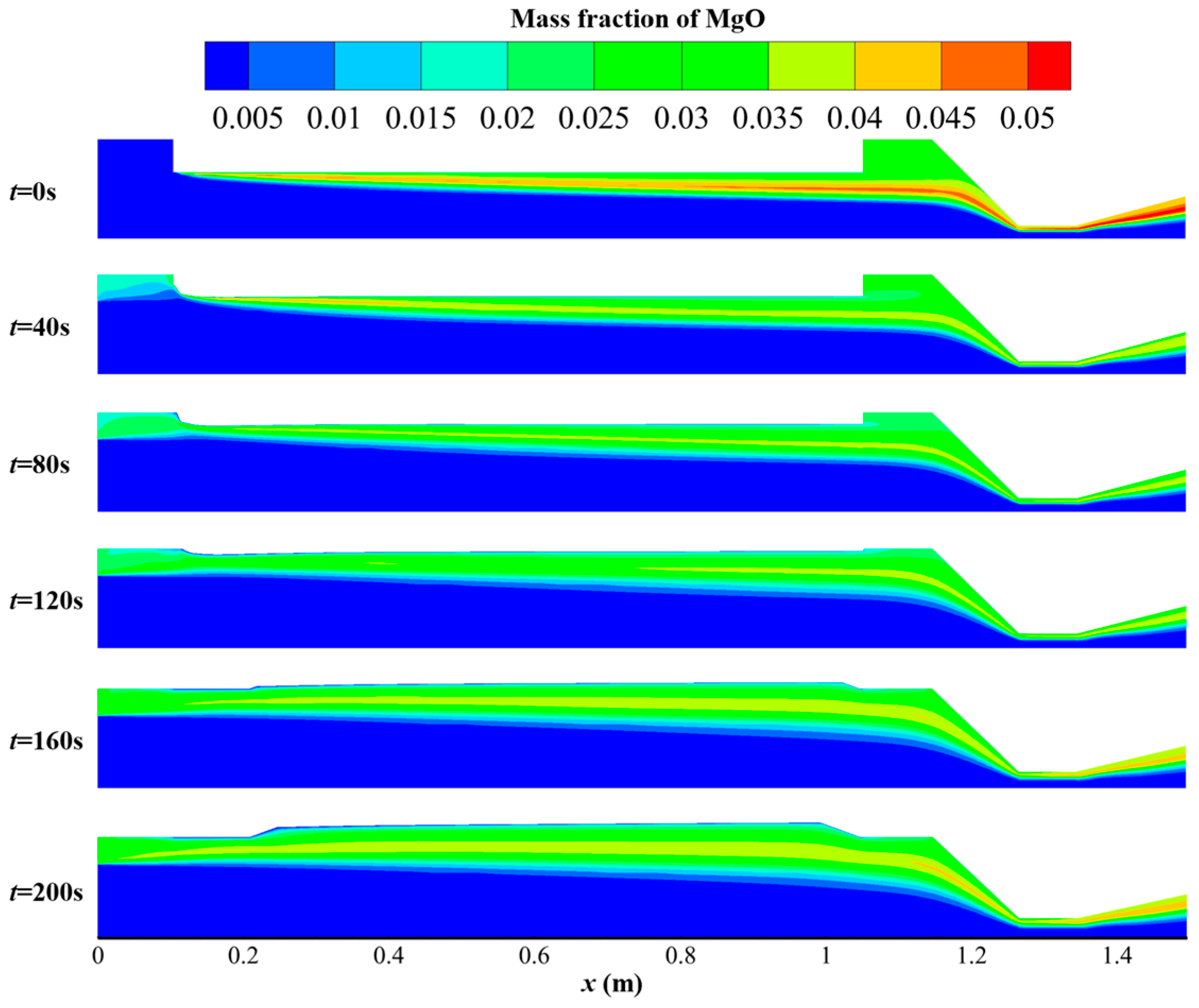


| NO. | Reaction Model | Arection | Brection | Erection/R (K) |
|---|---|---|---|---|
| 1 | 8.80 × 1011 | 0.0 | 15,200 | |
| 2 | 1.70 × 1013 | 0.0 | 24,100 | |
| 3 | 2.19× 1013 | 0.0 | 2590 | |
| 4 | 6.02× 1012 | 0.0 | 550 | |
| 5 | 1.80 × 1010 | 1.0 | 4480 | |
| 6 | 1.22 × 1017 | −0.91 | 8370 | |
| 7 | 1.00 × 1016 | 0.0 | 0 | |
| 8 | 2.55 × 1018 | −1.0 | 59,400 | |
| 9 | 5.00 × 1015 | 0.0 | 0 | |
| 10 | 8.40 × 1021 | −2.0 | 0 | |
| 11 | 4.00 × 1012 | 0.0 | 4030 | |
| 12 | 3.00 × 1012 | 0.0 | 25,000 | |
| 13 | 6.00 × 1013 | 0.0 | 0 | |
| 14 | 9.70 × 1013 | 0.0 | 80.6 | |
| 15 | 1.00 × 1015 | 0.0 | 12,000 |
| Structural Parameters | Value |
|---|---|
| Combustion chamber outer diameter dc (mm) | 336 |
| Grain inner diameter df (mm) | 183 |
| Grain length Lf (mm) | 949 |
| Pre-combustion chamber inner diameter dpre (mm) | 274 |
| Post-combustion chamber inner diameter dpost (mm) | 274 |
| Nozzle throat diameter dt (mm) | 36 |
| Nozzle outlet diameter de (mm) | 116.6 |
| Parameters | Maximum | Minimum | Average |
|---|---|---|---|
| Pressure of chamber (Mpa) | 2.30 | 0.95 | 1.73 |
| Thrust (kN) | 2.93 | 1.69 | 2.36 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, X.; Tian, H.; Niu, X.; Zhu, H.; Gao, J.; Cai, G. Long-Duration Dynamic Numerical Simulation of Combustion and Flow in Hybrid Rocket Motors Considering Nozzle Erosion. Aerospace 2024, 11, 318. https://doi.org/10.3390/aerospace11040318
Meng X, Tian H, Niu X, Zhu H, Gao J, Cai G. Long-Duration Dynamic Numerical Simulation of Combustion and Flow in Hybrid Rocket Motors Considering Nozzle Erosion. Aerospace. 2024; 11(4):318. https://doi.org/10.3390/aerospace11040318
Chicago/Turabian StyleMeng, Xiangyu, Hui Tian, Xiaoting Niu, Hao Zhu, Jingfei Gao, and Guobiao Cai. 2024. "Long-Duration Dynamic Numerical Simulation of Combustion and Flow in Hybrid Rocket Motors Considering Nozzle Erosion" Aerospace 11, no. 4: 318. https://doi.org/10.3390/aerospace11040318
APA StyleMeng, X., Tian, H., Niu, X., Zhu, H., Gao, J., & Cai, G. (2024). Long-Duration Dynamic Numerical Simulation of Combustion and Flow in Hybrid Rocket Motors Considering Nozzle Erosion. Aerospace, 11(4), 318. https://doi.org/10.3390/aerospace11040318







