Study on the Penetration Performance of a Double-Angle Linear Shaped Charge: Performance Improvement and Miniaturization
Abstract
1. Introduction
2. Structural Design
3. Numerical Analysis Setup
3.1. Finite Element Model
3.2. Material Constitutive Model and Parameters
3.2.1. Explosive
3.2.2. Liner, Case and Target
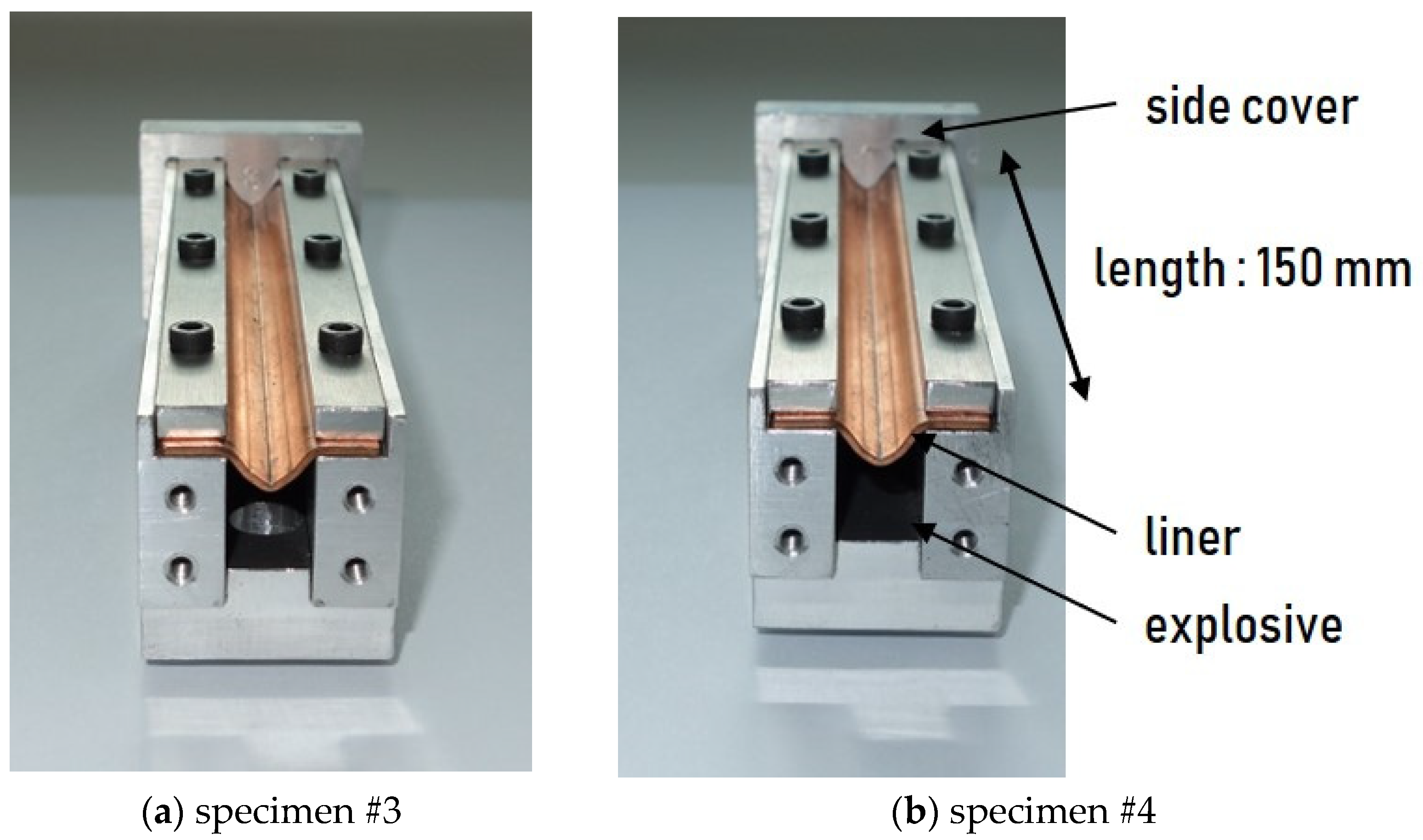
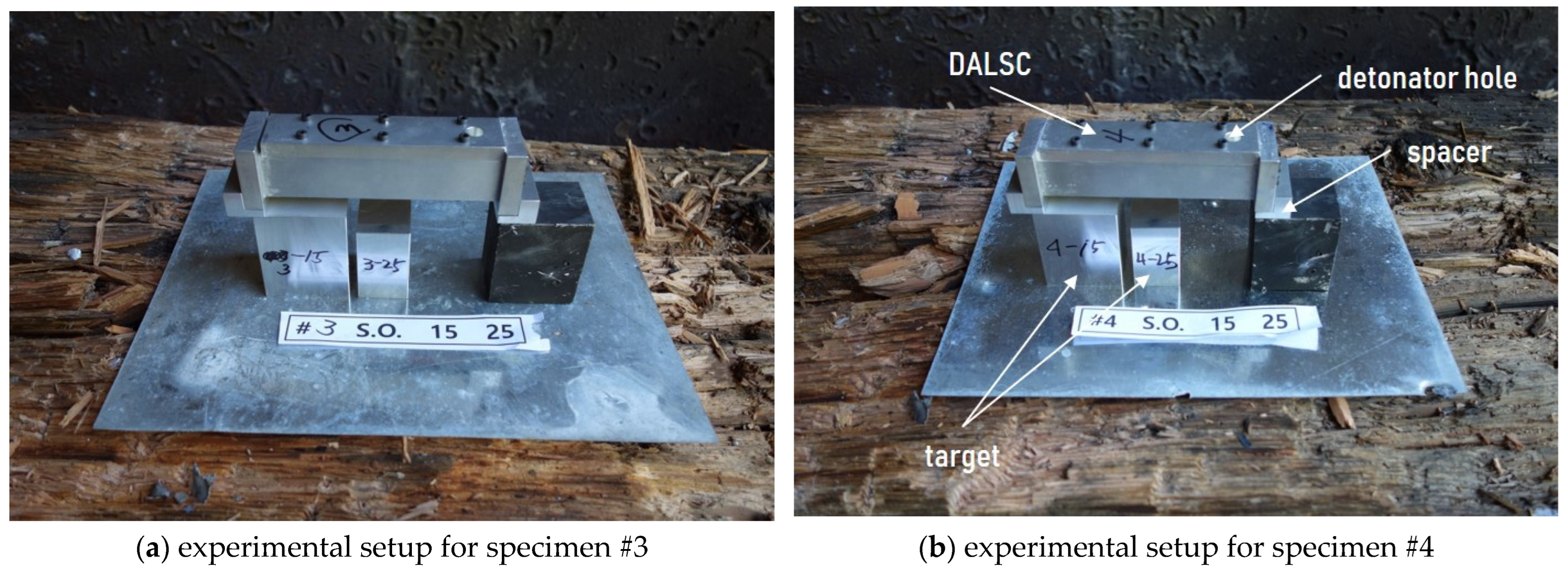
4. Experimental Setup
5. Results
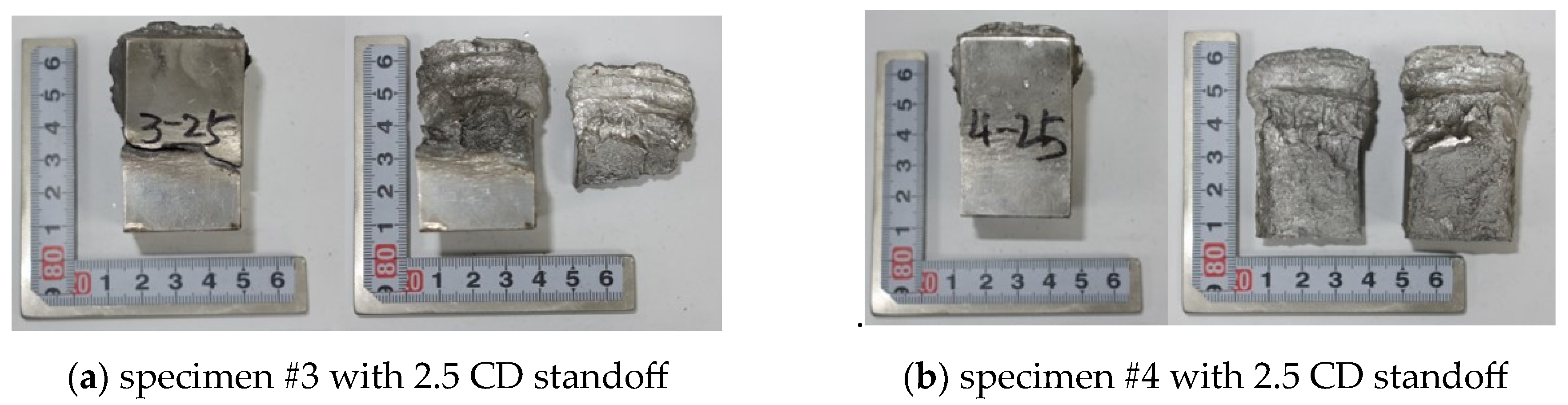
5.1. Experimental Results
- (1)
- Measure the depth from the top of the target to the end of the penetration using a Vernier caliper.
- (2)
- Measure the distance from the measurement point on the top of the target to the bottom of the target.
- (3)
- Original target height - ((2) - (1)) = penetration depth.
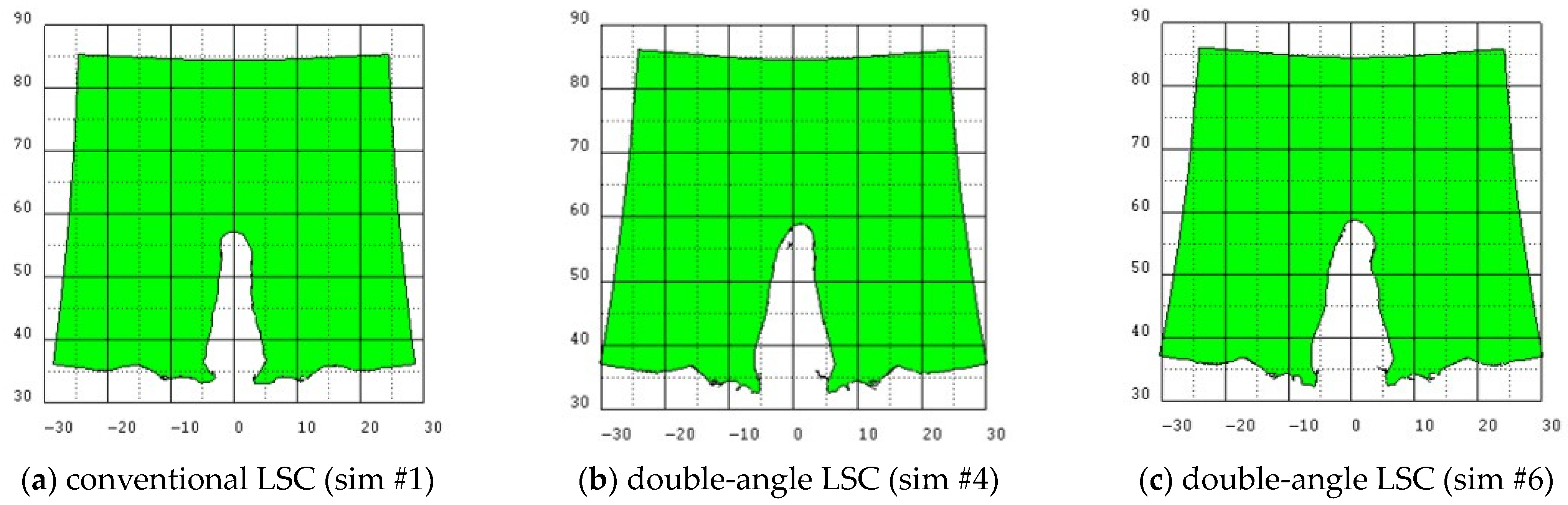
5.2. Numerical Analysis Results
5.3. Additional Numerical Analysis and Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Birkhoff, G.; MacDougall, D.P.; Pugh, E.M.; Taylor, G. Explosives with Lined Cavities. J. Appl. Phys. 1948, 19, 563–582. [Google Scholar] [CrossRef]
- Pugh, E.M.; Eichelberger, R.J.; Rostoker, N. Theory of Jet Formation by Charges with Lined Conical Cavities. J. Appl. Phys. 1952, 23, 532–536. [Google Scholar] [CrossRef]
- Walters, W.P.; Zukas, J.A. Fundamentals of Shaped Charges; A Wiley-Interscience Publication: Hoboken, NJ, USA, 1989. [Google Scholar]
- Shekhar, H. Theoretical Modelling of Shaped Charges in the Last Two Decades (1990–2010): A Review. Cent. Eur. J. Energetic Mater. 2012, 9, 155–185. [Google Scholar]
- Vigil, M.G. Design of Linear Shaped Charges Using the LESCA; Sandia Report; Sandia National Laboratories: Albuquerque, NM, USA, 1990; p. SAND90-0243.
- Vigil, M.G. Precision Linear Shaped Charge Analyses for Severance of Metal; Sandia Report; Sandia National Laboratories: Albuquerque, NM, USA, 1996; p. SAND96-2031.
- Lim, S. Steady State Equation of Motion of a Linear Shaped Charges Liner. Int. J. Impact Eng. 2012, 44, 10–16. [Google Scholar] [CrossRef]
- Lim, S. Jet Velocity Profile of Linear Shaped Charges Based on an Arced Liner Collapse. J. Energetic Mater. 2013, 31, 239–250. [Google Scholar] [CrossRef]
- Lim, S. Liner Collapse Line of Linear Shaped Charges. J. Energetic Mater. 2017, 35, 125–135. [Google Scholar] [CrossRef]
- Lim, S.; Baldovi, P.; Rood, C. Jet Grouping of Linear-Shaped Charges and Penetration Performance. Appl. Sci. 2022, 12, 12768. [Google Scholar] [CrossRef]
- Li, Y.; Zhai, Z.; Fan, X.; Han, H.; Zhao, Y.; Cao, H. Orthogonal Structure Design of the Linear Shaped Charge based on Steady State Velocity Model. J. Phys. Conf. Ser. 2023, 2478, 072057. [Google Scholar] [CrossRef]
- Bohanek, V.; Dobrilovic, M.; Skrlec, V. The Efficiency of Linear Shaped Charges. Teh. Vjesn.—Tech. Gaz. 2014, 21, 525–531. [Google Scholar]
- Sterbentz, D.M.; Jekel, C.F.; White, D.A.; Rieben, R.N.; Belof, J.L. Linear Shaped Charge Jet Optimization using Machine Learning Method. J. Appl. Phys. 2023, 134, 045102. [Google Scholar] [CrossRef]
- Cheng, X.; Huang, G.; Liu, C.; Feng, S. Design of a Novel Linear Shaped Charge and Factors Influencing its Penetration Performance. Appl. Sci. 2018, 8, 1863. [Google Scholar] [CrossRef]
- Bui, X.S.; Do, V.M.; Dang, V.L. Effect of Shaped of Double-Cone Liner on Penetration Depth of Shaped Charge without Wave Shaper. J. Sci. Tech. 2021, 16, 72–84. [Google Scholar] [CrossRef]
- El-Sayed, E.E.; Elshenawy, T.A.; Shaker, M.A. Influence of Different Shaped Charge Parameters on Jet Penetration into Metallic Target. J. Phys. Conf. Ser. 2022, 2299, 012016. [Google Scholar] [CrossRef]
- Li, H.; Li, P.; Duan, H.; Zhang, Z. Study on Jet Formation and Penetration Performance of Shaped Charge with Untypical Double-cone Titanium Liner. J. Phys. Conf. Ser. 2023, 2599, 012028. [Google Scholar] [CrossRef]
- Gürel, E. Modeling and Simulation of Shaped Charge. Master’s Thesis, Middle East Technical University, Ankara, Turkey, 2009. [Google Scholar]
- Ralph, M. JWL Equation of State; Los Alamos National Laboratory: Los Alamos, NM, USA, 2017; p. LA-UR-15-29536.
- Baudin, G.; Serradeil, R. Review of Jones-Wilkins-Lee equation of state. In Proceedings of the EPJ Web of Conferences 10, Poitiers, France, 4–9 July 2010; p. 00021. [Google Scholar]
- Heuze, O. General form of the Mie-Gruneisen equation of state. C. R. Mec. 2012, 340, 679–687. [Google Scholar] [CrossRef]
- Zocher, M.A.; Maudlin, P.J.; Chen, S.R.; Flower-Maudlin, E.C. An Evaluation of Several Hardening Models Using Taylor Cylinder Impact Data. In Proceedings of the European Congress on Computational Methods in Applied Sciences and Engineering, Barcelona, Spain, 11–14 September 2000. [Google Scholar]
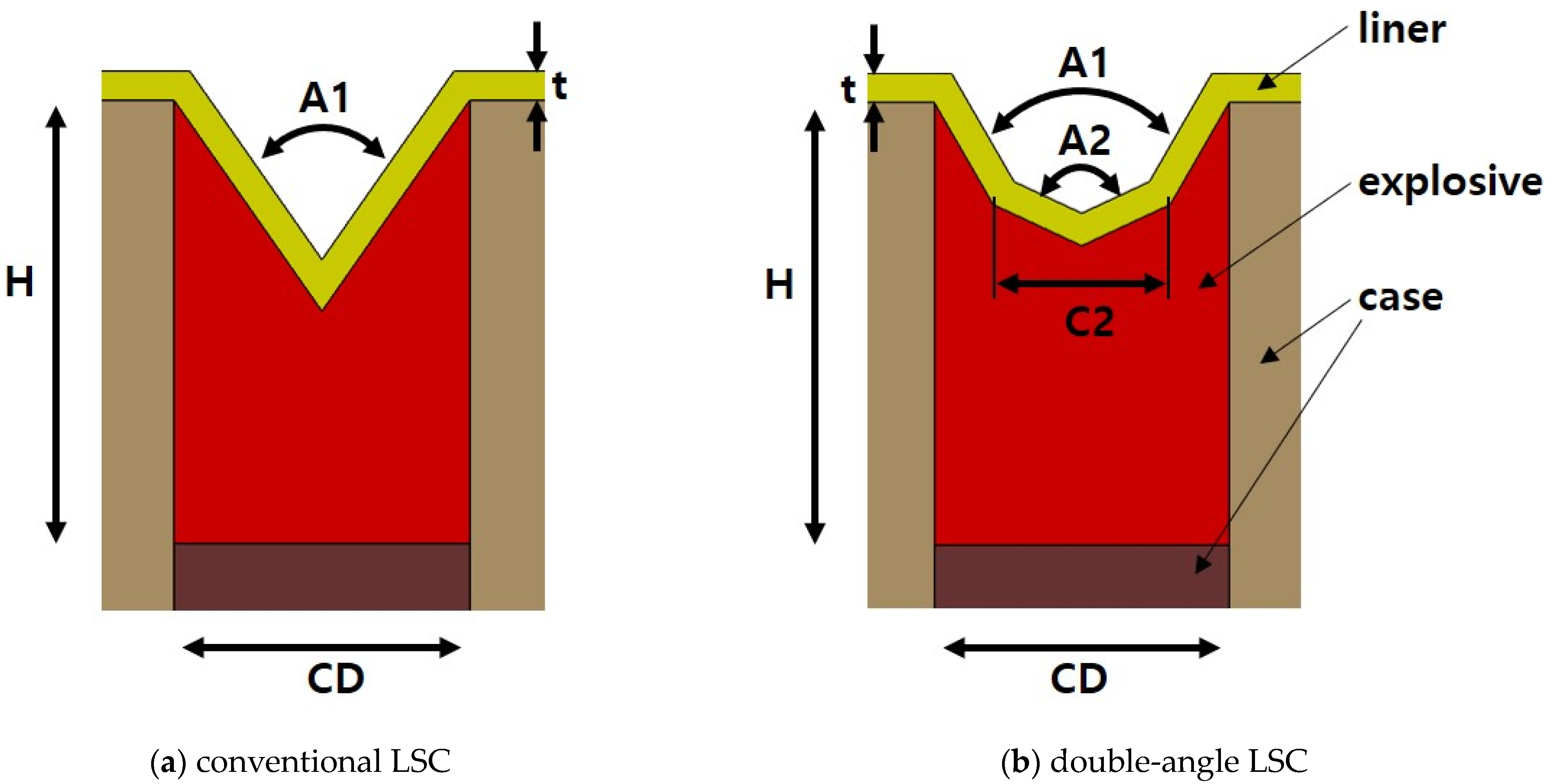
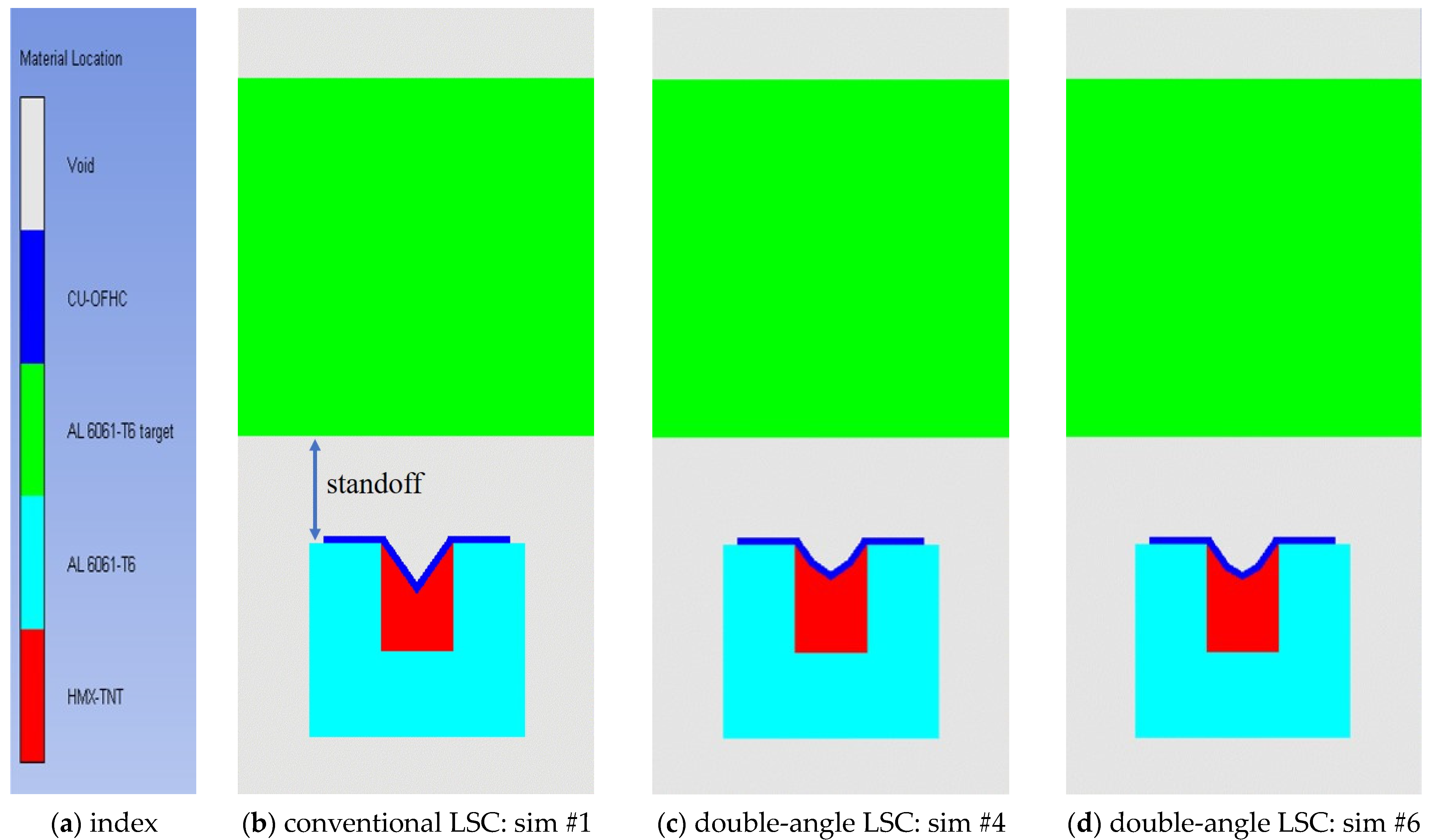





| Sim | Type | A1 (º) | A2 (º) | CD (mm) | C2 (CD) | H (CD) | t (mm) |
|---|---|---|---|---|---|---|---|
| #1 | Conventional | 70 | - | 10 | - | 1.5 | 1 |
| #2 #3 #4 | Double-angle | 70 | 110 | 10 | 0.4 0.5 0.6 | 1.5 | 1 |
| #5 #6 #7 | Double-angle | 70 | 120 | 10 | 0.4 0.5 0.6 | 1.5 | 1 |
| Part | Material | EOS | Strength Model | Erosion |
|---|---|---|---|---|
| Explosive | HMX-TNT | JWL | - | - |
| Liner | Cu-OFHC | Mie–Gruneisen shock | Steinberg–Guinan | - |
| Case | Al 6061 T6 | Mie–Gruneisen shock | Steinberg–Guinan | - |
| Target | Al 6061 T6 | Mie–Gruneisen shock | Steinberg–Guinan | Instantaneous geometric strain |
| Density (g/cc) | A (GPa) | B (GPa) | ω | C-J Detonation Velocity (m/s) | C-J Energy per Unit Volume (GJ/m3) | C-J Pressure (GPa) | ||
|---|---|---|---|---|---|---|---|---|
| 1.776 | 700.8 | 12.12 | 4.5 | 1.10 | 0.30 | 8210 | 8.9 | 31.1 |
| Material | Density (g/cc) | Γ | (m/s) | S | (K) | Specific Heat (J/kgK) |
|---|---|---|---|---|---|---|
| Cu-OFHC | 8.930 | 2.02 | 3940 | 1.489 | 300 | 383 |
| Al 6061 T6 | 2.703 | 1.97 | 5240 | 1.400 | 300 | 885 |
| Material | Shear Modulus (GPa) | Yield Stress (GPa) | Maximum Yield Stress (GPa) | Hardening Constant | Melting Temperature (K) |
|---|---|---|---|---|---|
| Cu-OFHC | 47.70 | 0.12 | 0.64 | 36 | 1790 |
| Al 6061 T6 | 27.60 | 0.29 | 0.68 | 125 | 1220 |
| Specimen | Simulation | A1 (º) | A2 (º) | CD (mm) | C2 (CD) | H (CD) | t (mm) | Standoff (CD) |
|---|---|---|---|---|---|---|---|---|
| #3 | #4 #11 | 70 | 110 | 10 | 0.6 | 1.5 | 1 | 1.5 2.5 |
| #4 | #6 #13 | 70 | 120 | 10 | 0.5 | 1.5 | 1 | 1.5 2.5 |
| DALSC # | A1 (º) | A2 (º) | C2 (CD) | Standoff (CD) | Penetration Depth (CD) |
|---|---|---|---|---|---|
| #3 | 70 | 110 | 0.6 | 1.5 | 2.37 |
| #4 | 70 | 120 | 0.5 | 1.5 | 2.43 |
| Specimen | Type | Standoff (mm) | Simulation (mm) | Experiment (mm) | Sim-Exp (mm) | Err (%) |
|---|---|---|---|---|---|---|
| #3 | DALSC | 15 | 24.3 | 23.7 | 0.6 | 2.5 |
| #4 | DALSC | 15 | 24.1 | 24.3 | −0.2 | −0.8 |
| Sim | Specimen | Type | A1 (º) | A2 (º) | C2 (CD) | Standoff (CD) | Penetration Depth (Sim) (CD) | Increase Rate (%) |
|---|---|---|---|---|---|---|---|---|
| #1 | LSC | 70 | - | - | 1.5 | 2.27 | - | |
| #2 | DALSC | 70 | 110 | 0.4 | 1.5 | 2.58 | 13.7 | |
| #3 | DALSC | 70 | 110 | 0.5 | 1.5 | 2.60 | 14.5 | |
| #4 | #3 | DALSC | 70 | 110 | 0.6 | 1.5 | 2.43 | 7.0 |
| #5 | DALSC | 70 | 120 | 0.4 | 1.5 | 2.57 | 13.2 | |
| #6 | #4 | DALSC | 70 | 120 | 0.5 | 1.5 | 2.41 | 6.2 |
| #7 | DALSC | 70 | 120 | 0.6 | 1.5 | 2.32 | 2.2 | |
| #8 | LSC | 70 | - | - | 2.5 | 2.48 | - | |
| #9 | DALSC | 70 | 110 | 0.4 | 2.5 | 2.70 | 8.9 | |
| #10 | DALSC | 70 | 110 | 0.5 | 2.5 | 2.59 | 4.4 | |
| #11 | #3 | DALSC | 70 | 110 | 0.6 | 2.5 | 2.59 | 4.4 |
| #12 | DALSC | 70 | 120 | 0.4 | 2.5 | 2.79 | 12.5 | |
| #13 | #4 | DALSC | 70 | 120 | 0.5 | 2.5 | 2.60 | 4.8 |
| #14 | DALSC | 70 | 120 | 0.6 | 2.5 | 2.45 | −1.2 |
| Sim # | Type | A1 (º) | A2 (º) | C2 (CD) | H(CD) | Standoff (CD) | Penetration (CD) | Increase Rate (%) |
|---|---|---|---|---|---|---|---|---|
| #15 | LSC | 70 | - | - | 1 | 1 | 1.51 | - |
| #16 | DALSC | 70 | 110 | 0.4 | 1 | 1 | 1.54 | 2.0 |
| #17 | DALSC | 70 | 110 | 0.5 | 1 | 1 | 1.65 | 9.3 |
| #18 | DALSC | 70 | 110 | 0.6 | 1 | 1 | 1.65 | 9.3 |
| #19 | DALSC | 70 | 120 | 0.4 | 1 | 1 | 1.73 | 14.6 |
| #20 | DALSC | 70 | 120 | 0.5 | 1 | 1 | 1.64 | 8.6 |
| #21 | DALSC | 70 | 120 | 0.6 | 1 | 1 | 1.57 | 4.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.; Kwon, S. Study on the Penetration Performance of a Double-Angle Linear Shaped Charge: Performance Improvement and Miniaturization. Aerospace 2024, 11, 310. https://doi.org/10.3390/aerospace11040310
Park J, Kwon S. Study on the Penetration Performance of a Double-Angle Linear Shaped Charge: Performance Improvement and Miniaturization. Aerospace. 2024; 11(4):310. https://doi.org/10.3390/aerospace11040310
Chicago/Turabian StylePark, Jongmin, and Sejin Kwon. 2024. "Study on the Penetration Performance of a Double-Angle Linear Shaped Charge: Performance Improvement and Miniaturization" Aerospace 11, no. 4: 310. https://doi.org/10.3390/aerospace11040310
APA StylePark, J., & Kwon, S. (2024). Study on the Penetration Performance of a Double-Angle Linear Shaped Charge: Performance Improvement and Miniaturization. Aerospace, 11(4), 310. https://doi.org/10.3390/aerospace11040310





