Tau Theory-Based Flare Control in Autonomous Helicopter Autorotation
Abstract
:1. Introduction
2. Simulation Model
| Parameter | Value | Units |
|---|---|---|
| Mass and inertia | ||
| Gross weight, W | 16,270 | lb |
| Roll-axis moment of inertia, | 5000 | sl-ft2 |
| Pitch-axis moment of inertia, | 39,000 | sl-ft2 |
| Yaw-axis moment of inertia, | 39,000 | sl-ft2 |
| Roll/yaw-axes product of inertia, | 1900 | sl-ft2 |
| Main rotor | ||
| Number of blades, | 4 | - |
| Radius, R | ft | |
| Blade chord, c | ft | |
| Blade twist, | −13 | deg |
| Flapping hinge offset | 1.25 | ft |
| Blade weight, | 256.9 | lb |
| Blade first mass moment, | 86.7 | sl-ft |
| Blade second mass moment, | 1512.6 | sl-ft2 |
| Angular speed, | 27 | rad/s |
| Tail rotor | ||
| Number of blades, | 4 | - |
| Radius, | ft | |
| Blade chord, | ft | |
| Blade twist, | −17 | deg |
| Blade second mass moment, | sl-ft | |
| Angular speed, | rad/s |
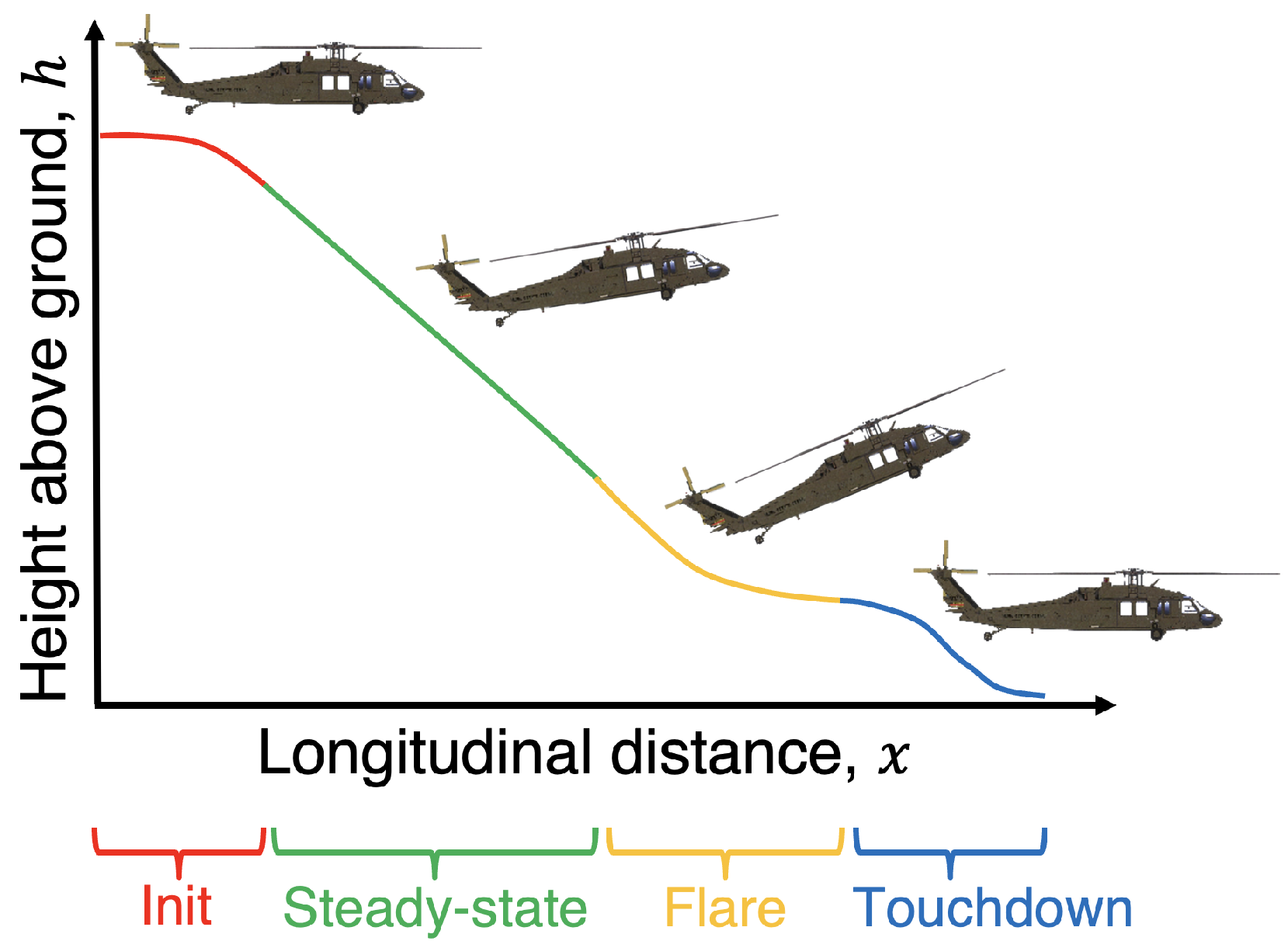
3. Trajectory Generation
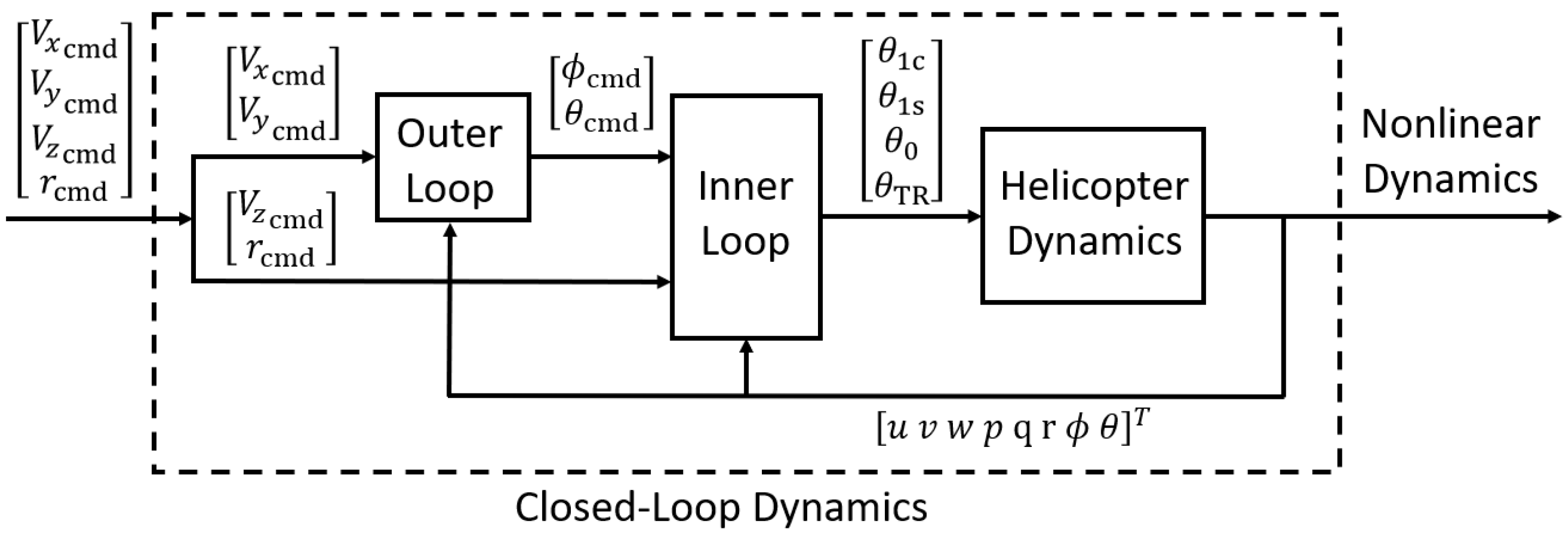
4. Autonomous Flare Control Law
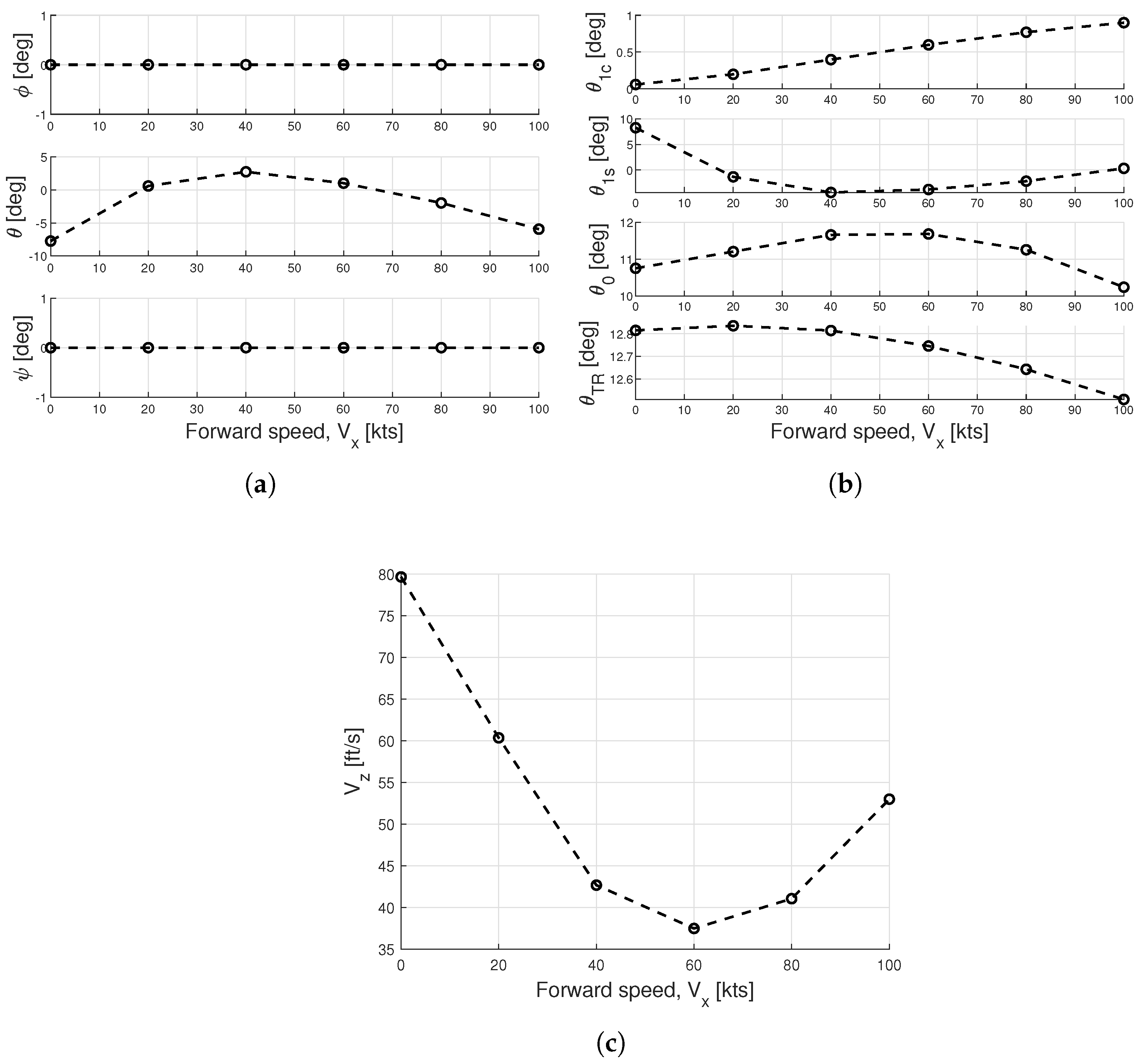
4.1. Linear Models
4.2. Inner Loop
4.3. Outer Loop
4.4. Error Dynamics
5. Results
5.1. Demonstration of Autonomous Flare Control Law
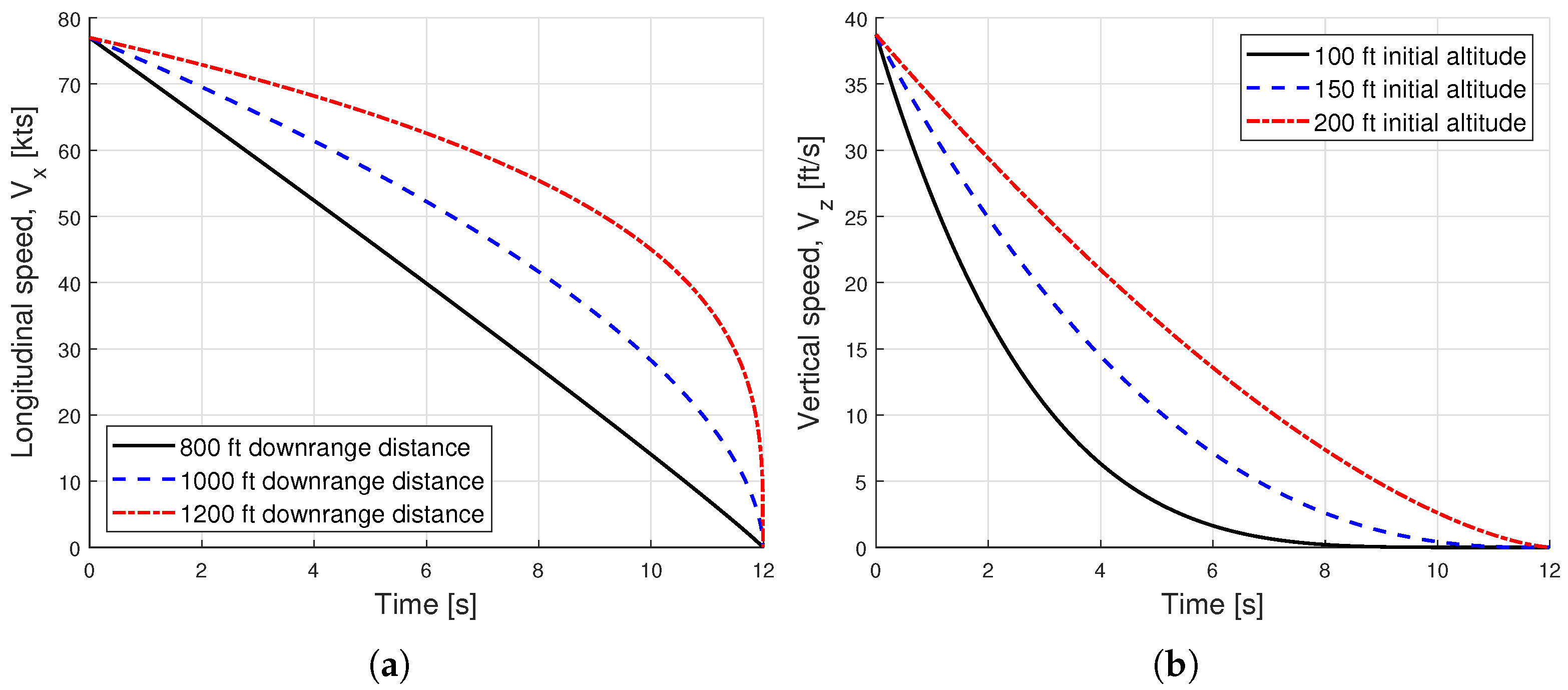
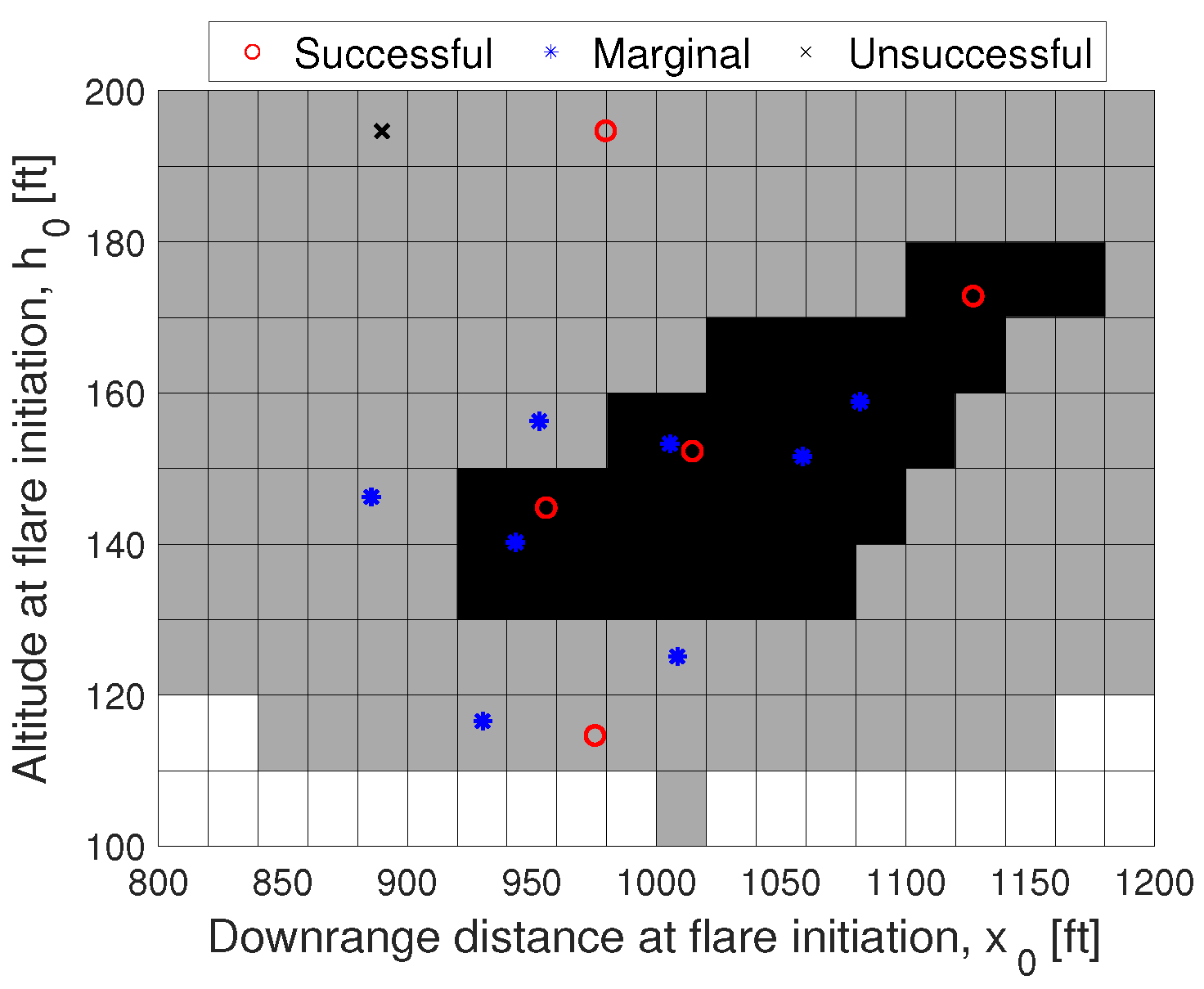
5.2. Reachability Study
6. Conclusions
- Scheduling of the NDI control law with linearized models of the rotorcraft flight dynamics in steady-state autorotation has been shown to be a successful approach for tracking flare trajectories. In addition to achieving adequate tracking of the longitudinal and vertical trajectories, the control law also showed good performance in mitigating the off-axis response.
- State histories of the autonomous flare maneuvers largely mimic those of piloted flight simulations. Noticeable differences lie in a more aggressive longitudinal deceleration in the early stages of flare, and a delayed pitchover before landing.
- The proposed method was used to predict combinations of downrange distances and altitudes at flare entry that result in desired and marginal landings. These predictions are in line with piloted flight simulation data, suggesting that the method may be used not only for real-time control, but also potentially for reachability predictions in the autorotation flare.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ACAH | Attitude Command/Attitude Hold |
| DI | Dynamic Inversion |
| EMF | Explicit Model Following |
| ETPS | Empire Test Pilot School |
| NDI | Nonlinear Dynamic Inversion |
| PI | Proportional–Integral |
| PID | Proportional–Integral–Derivative |
| RCAH | Rate Command/Attitude Hold |
| TRC | Translational Rate Command |
References
- Sunberg, Z.N.; Miller, N.R.; Rogers, J.D. A Real-Time Expert Control System For Helicopter Autorotation. J. Am. Helicopter Soc. 2015, 60, 1–15. [Google Scholar] [CrossRef]
- Bachelder, E.N.; Aponso, B.L. Using Optimal Control for Rotorcraft AutorotationTraining. In Proceedings of the 59th Annual Forum of the American Helicopter Society, Phoenix, AZ, USA, 6–8 May 2003. [Google Scholar]
- Aponso, B.L.; Lee, D.; Bachelder, E.N. Evaluation of a Rotorcraft Autorotation Training Display on a Commercial Flight Training Device. J. Am. Helicopter Soc. 2007, 52, 123–133. [Google Scholar] [CrossRef]
- Keller, J.D.; McKillip, R.; Horn, J.F.; Yomchinda, T. Active Flight Control and Appliqué Inceptor Concepts for Autorotation Performance Enhancement. In Proceedings of the 67th Annual Forum of the American Helicopter Society, Virginia Beach, VA, USA, 3–5 May 2011. [Google Scholar]
- Rogers, J.; Repola, L.; Jump, M.; Cameron, N.; Fell, T. Handling Qualities Assessment of a Pilot Cueing System for Autorotation Maneuvers. In Proceedings of the 73rd Annual Forum of the American Helicopter Society, Fort Worth, TX, USA, 9–11 May 2017. [Google Scholar]
- Yomchinda, T. Real-Time Path Planning and Autonomous Control for Helicopter Autorotation. Ph.D. Thesis, Pennsylvania State University, University Park, PA, USA, 2013. [Google Scholar]
- Yomchinda, T.; Horn, J.; Langelaan, J. Flight Path Planning for Descent-phase Helicopter Autorotation. In Proceedings of the AIAA Guidance, Navigation and Control Conference, Portland, OR, USA, 8–11 August 2011. [Google Scholar]
- Grande, N.; Tierney, S.; Horn, J.; Langelaan, J. Safe Autorotation Through Wind Shear Via Backward Reachable Sets. J. Am. Helicopter Soc. 2016, 61, 1–11. [Google Scholar] [CrossRef]
- Lee, A.Y.; Bryson, A.E.; Hindson, W.S. Optimal Landing of a Helicopter in Autorotation. J. Guid. Control. Dyn. 1988, 11, 7–12. [Google Scholar] [CrossRef]
- Lee, A.Y. Optimal Autorotational Descent of a Helicopter with Control and State Inequality Constraints. J. Guid. Control. Dyn. 1989, 13, 922–924. [Google Scholar] [CrossRef]
- Okuno, Y.; Kawachi, K.; Azuma, A. Analytical Prediction of Height-Velocity Diagram of a Helicopter Using Optimal Control Theory. J. Guid. Control. Dyn. 1991, 14, 453–459. [Google Scholar] [CrossRef]
- Saetti, U.; Enciu, J.; Horn, J.F. Flight Dynamics and Control of an eVTOL Concept Aircraft with a Propeller-Driven Rotor. J. Am. Helicopter Soc. 2022, 67, 153–166. [Google Scholar] [CrossRef]
- Eberle, B.F.; Rogers, J.D.; Alam, M.; Jump, M. Rapid Method for Computing Reachable Landing Distances in Helicopter Autorotative Descent. J. Aerosp. Inf. Syst. 2022, 19, 504–510. [Google Scholar] [CrossRef]
- Tierney, S.; Langelaan, J. Autorotation Path Planning using Backwards Reachable Set and Optimal Control. In Proceedings of the 66th Annual Forum of the American Helicopter Society, Phoenix, AZ, USA, 11–13 May 2010. [Google Scholar]
- Eberle, B.F.; Rogers, J.D. Real-Time Trajectory Generation and Reachability Determination in Autorotative Flare. J. Am. Helicopter Soc. 2020, 65, 1–17. [Google Scholar] [CrossRef]
- Lee, D.N. A Theory of Visual Control of Braking Based on Information about Time-to-Collision. Perception 1976, 5, 437–459. [Google Scholar] [CrossRef]
- Horn, J.F. Non-Linear Dynamic Inversion Control Design for Rotorcraft. Aerospace 2019, 6, 38. [Google Scholar] [CrossRef]
- Saetti, U.; Horn, J.F.; Villafana, W.; Sharma, K.; Brentner, K.S. Rotorcraft Simulations with Coupled Flight Dynamics, Free Wake, and Acoustics. In Proceedings of the 72nd Annual Forum of the American Helicopter Society, West Palm Beach, FL, USA, 16–19 May 2016. [Google Scholar]
- Saetti, U. Rotorcraft Simulations with Coupled Flight Dynamics, Free Wake, and Acoustics. Master’s Thesis, Pennsylvania State University, University Park, PA, USA, 2016. [Google Scholar] [CrossRef]
- Saetti, U.; Horn, J.F.; Lakhmani, S.; Lagoa, C.; Berger, T. Design of Dynamic Inversion and Explicit Model Following Control Laws for Quadrotor UAS. J. Am. Helicopter Soc. 2020, 65, 1–16. [Google Scholar] [CrossRef]
- Caudle, D.B. Damage Mitigation for Rotorcraft through Load Alleviating Control. Master’s Thesis, The Pennsylvania State University, University Park, PA, USA, 2014. [Google Scholar]
- Spires, J.M.; Horn, J.F. Multi-Input Multi-Output Model-Following Control Design Methods for Rotorcraft. In Proceedings of the 71st Annual Forum of the American Helicopter Society, Virginia Beach, VA, USA, 5–7 May 2015. [Google Scholar]
- Saetti, U.; Batin, B. Tiltrotor Simulations with Coupled Flight Dynamics, State-Space Aeromechanics, and Aeroacoustics. J. Am. Helicopter Soc. 2024, 69, 1–18. [Google Scholar] [CrossRef]
- Scaramal, M.; Horn, J.F.; Saetti, U. Load Alleviation Control using Dynamic inversion with Direct Load Feedback. In Proceedings of the 77th Annual Forum of the Vertical Flight Society, Virtual, 10–14 May 2021. [Google Scholar] [CrossRef]
- Berger, T.; Tischler, M.B.; Horn, J.F. Outer-Loop Control Design and Simulation Handling Qualities Assessment for a Coaxial-Compound Helicopter and Tiltrotor. In Proceedings of the 77th Annual Forum of the Vertical Flight Society, Virtual, 5–8 October 2020. [Google Scholar] [CrossRef]
- Berger, T.; Tischler, M.B.; Horn, J.F. High-Speed Rotorcraft Pitch Axis Response Type Investigation. In Proceedings of the 77th Annual Forum of the Vertical Flight Society, Virtual, 10–14 May 2021. [Google Scholar] [CrossRef]
- Padfield, G.D. Helicopter Flight Dynamics: Including a Treatment of Tiltrotor Aircraft; John Wiley & Sons: New York, NY, USA, 2018. [Google Scholar]
- Pitt, D.M.; Peters, D.A. Theoretical Prediction of Dynamic-Inflow Derivatives. Vertica 1983, 5, 21–34. [Google Scholar]
- Leishman, J.G. Principles of Helicopter Aerodynamics; Cambridge University Press: New York, NY, USA, 2006. [Google Scholar]
- Howlett, J.J. UH-60A Black Hawk Engineering Simulation Program. Volume 1: Mathematical Model; Technical Report, NASA-CR-166309; NASA: Washington, DC, USA, 1980.
- Padfield, G.D. The Tau of Flight Control. Aeronaut. J. 2011, 115, 521–556. [Google Scholar] [CrossRef]
- Jump, M.; Padfield, G. Tau Flare or not Tau Flare: That is the question: Developing Guidelines for an Approach and Landing Sky Guide. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, San Francisco, CA, USA, 15–18 August 2005. [Google Scholar] [CrossRef]
- Alam, M.; Jump, M.; Eberle, B.; Rogers, J. Flight Simulation Assessment of Autorotation Algorithms and Cues. In Proceedings of the 76th Annual Forum of the Vertical Flight Society, Virtual, 6–8 October 2020. [Google Scholar] [CrossRef]
- Houston, S.S. Analysis of Rotorcraft Flight Dynamics in Autorotation. J. Guid. Control. Dyn. 2002, 25, 33–39. [Google Scholar] [CrossRef]
- Lopez, C.A.; Wells, V.L. Dynamics and Stability of an Autorotating Rotor/Wing Unmanned Aircraft. J. Guid. Control. Dyn. 2004, 27, 258–270. [Google Scholar] [CrossRef]
- Ryu, J.H.; Park, C.S.; Tahk, M.J. Plant Inversion Control of Tail Controlled Missile. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, New Orleans, LA, USA, 11–13 August 1997. [Google Scholar] [CrossRef]
- Blakelock, J.H. Automatic Control of Aircraft and Missiles; John Wiley & Sons: New York, NY, USA, 1965. [Google Scholar] [CrossRef]
- Stevens, B.L.; Lewis, F.L. Aircraft Control and Simulation: Dynamics, Controls Design, and Autonomous Systems, 3rd ed.; John Wiley and Sons, Inc.: New York, NY, USA, 2015. [Google Scholar] [CrossRef]
- Tischler, M.B.; Berger, T.; Ivler, C.M.; Mansur, M.H.; Cheung, K.K.; Soong, J.Y. Practical Methods for Aircraft and Rotorcraft Flight Control Design: An Optimization-Based Approach; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2017. [Google Scholar] [CrossRef]
- Alam, M.; Jump, M.; Cameron, M. Can Time-To-Contact Be Used To Model A Helicopter Autorotation? In Proceedings of the 8th Asian/Australian Rotorcraft Forum, Ankara, Turkey, 30 October–2 November 2019. [Google Scholar]
- Jump, M.; Alam, M.; Rogers, J.D.; Eberle, B. Progress in the Development of a Time-To-Contact Autorotation Cueing System. In Proceedings of the 44th European Rotorcraft Forum, Delft, The Netherlands, 18–20 September 2018. [Google Scholar]
- White, M.D.; Perfect, P.; Padfield, G.D.; Gubbles, A.W.; Berryman, A.C. Acceptance Testing and Conditioning of a Flight Simulator for Rotorcraft Simulation Fidelity Research. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2013, 227, 663–686. [Google Scholar] [CrossRef]
- Du Val, R.; He, C. FLIGHTLABTMTM modeling for real-time simulation applications. Int. J. Model. Simul. Sci. Comput. 2017, 8, 98–113. [Google Scholar] [CrossRef]
- Rogers, J.D.; Eberle, B.F.; Jump, M.; Cameron, N. Time-to-Contact-Based Control Laws for Flare Trajectory Generation and Landing Point Tracking in Autorotation. In Proceedings of the 74th Annual Forum of the Vertical Flight Society, Phoenix, AZ, USA, 14–17 May 2018. [Google Scholar]
- Lopez, M.J.S.; Padthe, A.K.; Glover, E.D.; Berger, T.; Tobias, E.L. Seeking Lift Share: Design Tradeoffs for a Winged Single Main Rotor Helicopter. In Proceedings of the 78th Annual Forum of the Vertical Flight Society, Fort Worth, TX, USA, 10–12 May 2022. [Google Scholar] [CrossRef]
- Padthe, A.K.; Lopez, M.J.S.; Berger, T.; Juhasz, O.; Tobias, E.L.; Glover, E.D. Design, Modeling, and Flight Dynamics Analysis of Generic Lift-Offset Coaxial Rotor Configurations. In Proceedings of the 78th Annual Forum of the Vertical Flight Society, Fort Worth, TX, USA, 10–12 May 2022. [Google Scholar] [CrossRef]
- Berger, T.; Juhasz, O.; Lopez, M.J.S.; Tischler, M.B.; Horn, J.F. Modeling and control of lift offset coaxial and tiltrotor rotorcraft. CEAS Aeronaut. J. 2020, 11, 191–215. [Google Scholar] [CrossRef]
- Straubinger, A.; Rothfeld, R.; Shamiyeh, M.; Bütcher, K.D.; Kaiser, J.; Plötner, K.O. An overview of current research and developments in urban air mobility – Setting the scene for UAM introduction. J. Air Transp. Manag. 2020, 87, 101852. [Google Scholar] [CrossRef]
- Sliva, C.; Johnson, W.; Antcliff, K.R.; Patterson, M.D. VTOL Urban Air Mobility Concept Vehicles for Technology Development. In Proceedings of the Paper AIAA 2018-3847, Proceedings of the 2018 Aviation Technology, Integration, and Operations Conference; Atlanta, GA, USA, 25–29 June 2018. [CrossRef]
- Berger, T.; Blanken, C.; Lusardi, J.A.; Tischler, M.B.; Horn, J.F. Tiltrotor Flight Control Design and High-Speed Handling Qualities Assessment. J. Am. Helicopter Soc. 2022, 67, 114–128. [Google Scholar] [CrossRef]
- Berger, T.; Blanken, C.; Lusardi, J.A.; Tischler, M.B.; Horn, J.F. Coaxial-Compound Helicopter Flight Control Design and High-Speed Handling Qualities Assessment. J. Am. Helicopter Soc. 2022, 67, 98–113. [Google Scholar] [CrossRef]
- Theron, J.P.; Enciu, J.; Horn, J.F. Nonlinear Dynamic Inversion Control for Urban Air Mobility Aircraft with Distributed Electric Propulsion. In Proceedings of the 76th Annual Forum of the Vertical Flight Society, Virtual, 6–8 October 2020. [Google Scholar] [CrossRef]

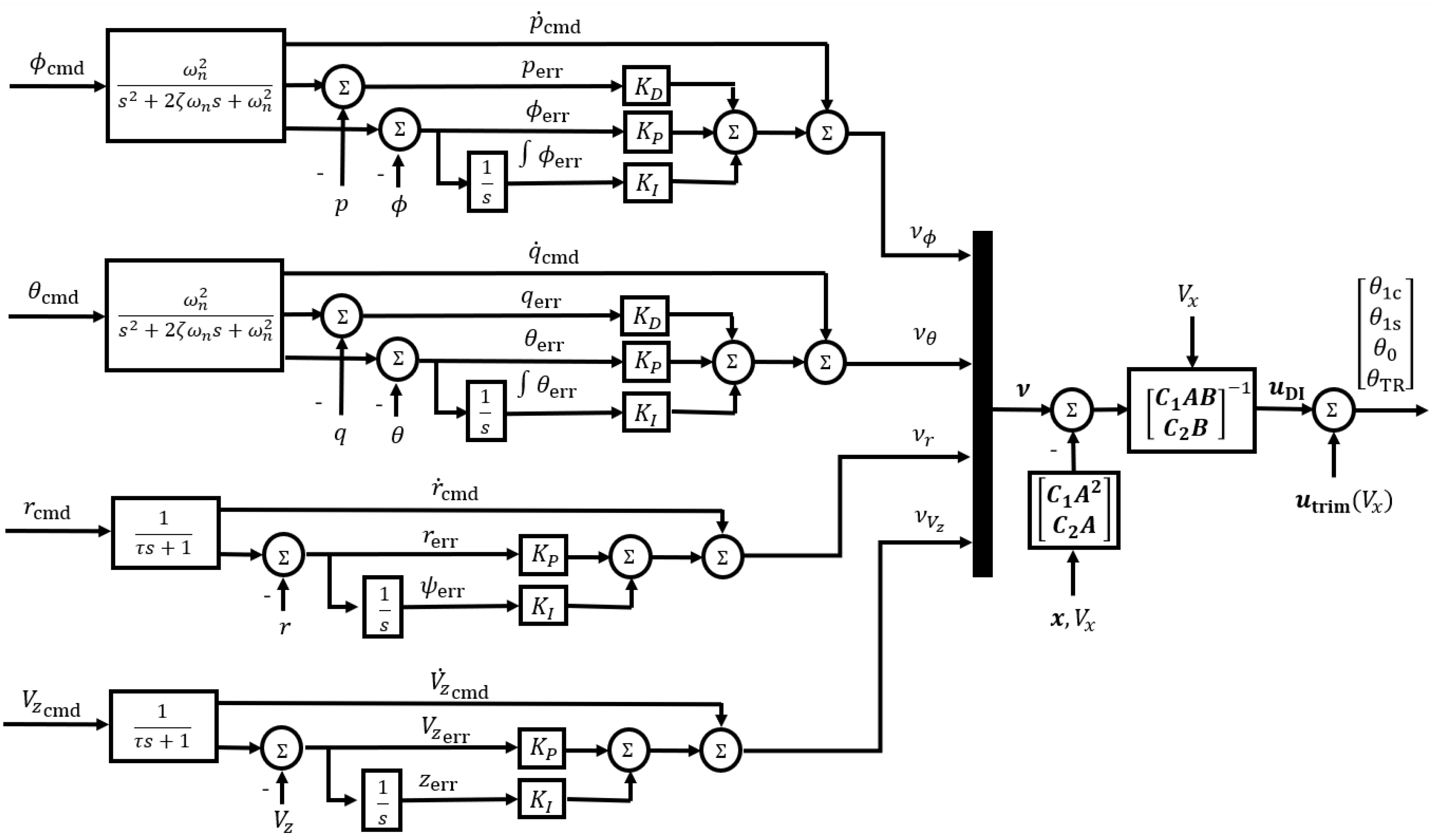




| Command | (Rad/s) | |
|---|---|---|
| Roll Attitude, | ||
| Pitch Attitude, | ||
| Yaw Rate, r | - | |
| Vertical Position, | - |
| Control Input | Min. (deg) | Max. (deg) |
|---|---|---|
| Lateral Cyclic, | −7 | 7 |
| Longitudinal Cyclic, | −12 | 12 |
| Collective, | 6.5 | 22.5 |
| Tail Rotor Collective, | −6 | 25 |
| Command | (Rad/s) | |
|---|---|---|
| Longitudinal Speed, | 1 | 0.7 |
| Lateral Speed, | 1 | 0.7 |
| (Rad/s) | p | ||
|---|---|---|---|
| 4.5 | 0.7 | 0.75 | |
| 4.5 | 0.7 | 0.75 | |
| 2 | 0.7 | - | |
| 1 | 0.7 | - |
| (Rad/s) | ||
|---|---|---|
| 1 | 0.7 | |
| 1 | 0.7 |
| 24.975 | 15.1875 | 7.05 | |
| 24.975 | 15.1875 | 7.05 | |
| 4 | 4 | 4 | |
| 2 | 1 | - |
| 1.5 | 0.5625 | |
| 1.5 | 0.5625 |
| (ft/s) | (ft/s) | (deg) | q (deg/s) | (%) | |
|---|---|---|---|---|---|
| Successful | |||||
| Marginal |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saetti, U.; Rogers, J.; Alam, M.; Jump, M. Tau Theory-Based Flare Control in Autonomous Helicopter Autorotation. Aerospace 2024, 11, 33. https://doi.org/10.3390/aerospace11010033
Saetti U, Rogers J, Alam M, Jump M. Tau Theory-Based Flare Control in Autonomous Helicopter Autorotation. Aerospace. 2024; 11(1):33. https://doi.org/10.3390/aerospace11010033
Chicago/Turabian StyleSaetti, Umberto, Jonathan Rogers, Mushfiqul Alam, and Michael Jump. 2024. "Tau Theory-Based Flare Control in Autonomous Helicopter Autorotation" Aerospace 11, no. 1: 33. https://doi.org/10.3390/aerospace11010033
APA StyleSaetti, U., Rogers, J., Alam, M., & Jump, M. (2024). Tau Theory-Based Flare Control in Autonomous Helicopter Autorotation. Aerospace, 11(1), 33. https://doi.org/10.3390/aerospace11010033







