Investigation of Vaned-Recessed Casing Treatment in a Low-Speed Axial-Flow Compressor, Part II: Unsteady Results
Abstract
:1. Introduction
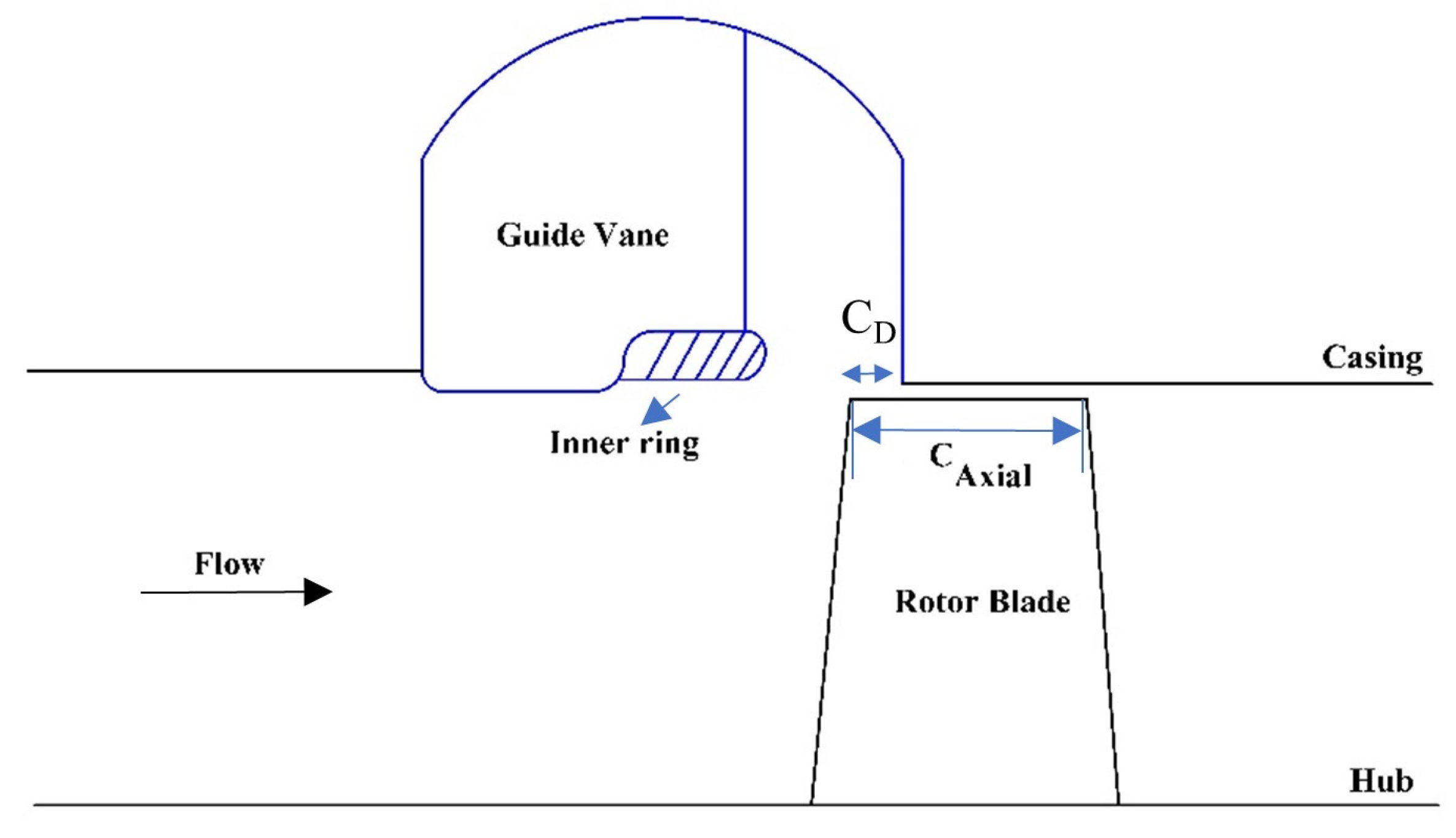
2. Investigated Compressor and Casing Treatment Configuration
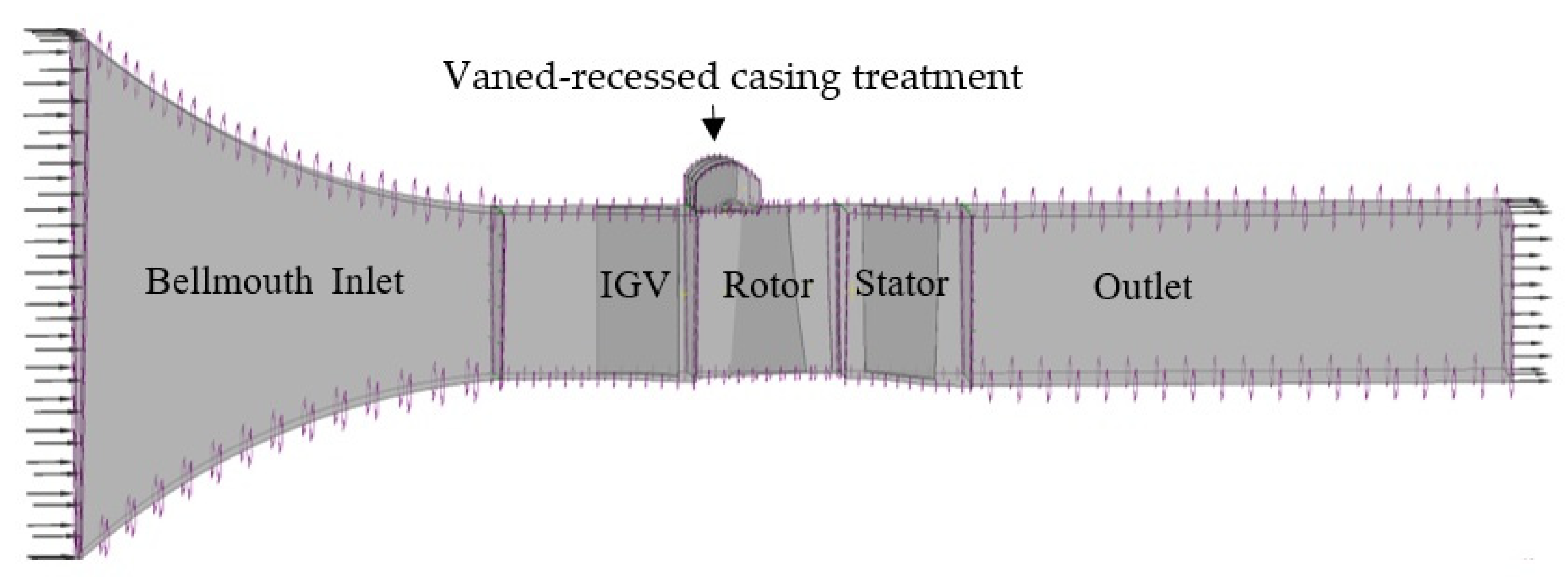
3. Numerical Details
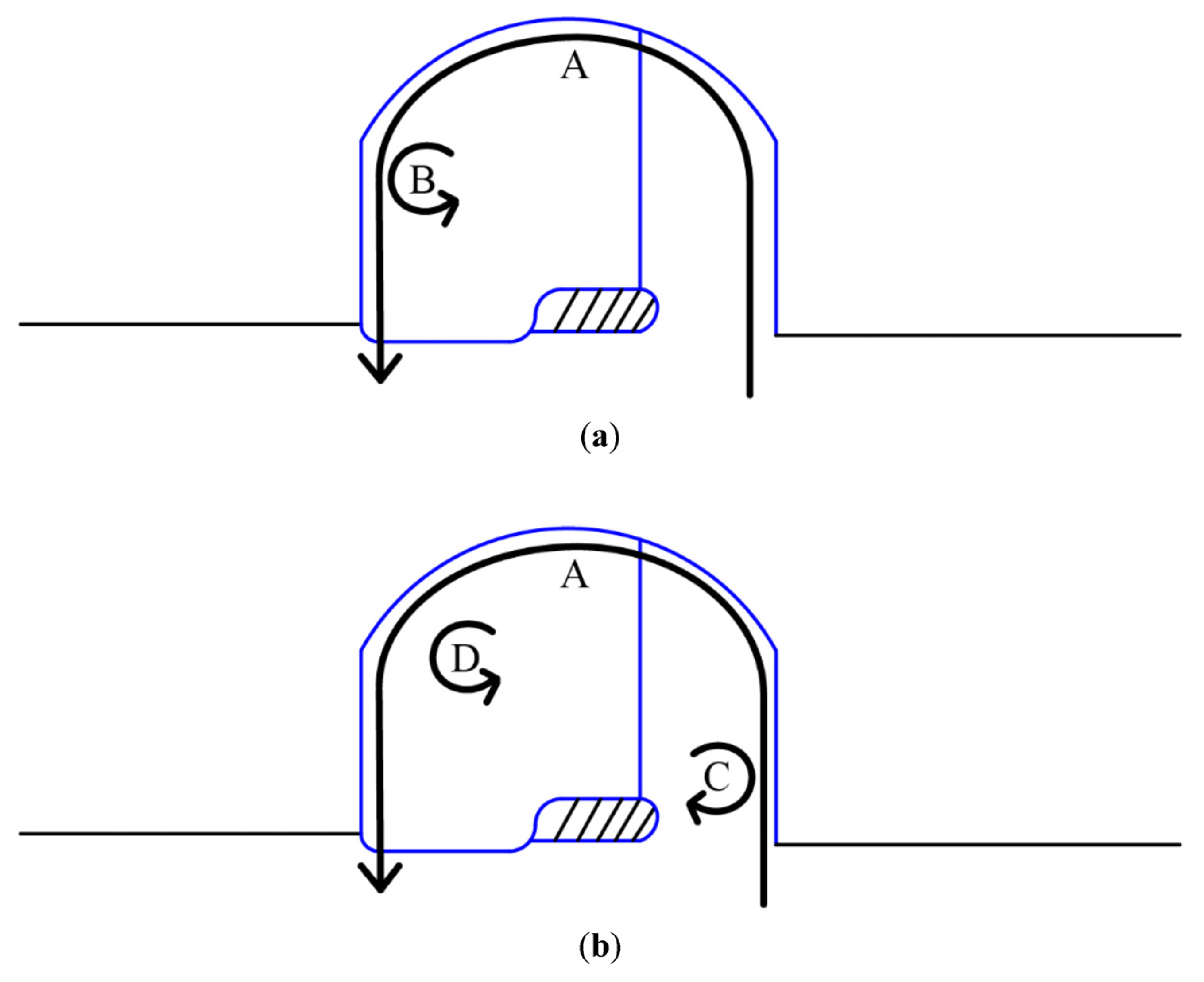
3.1. Geometry
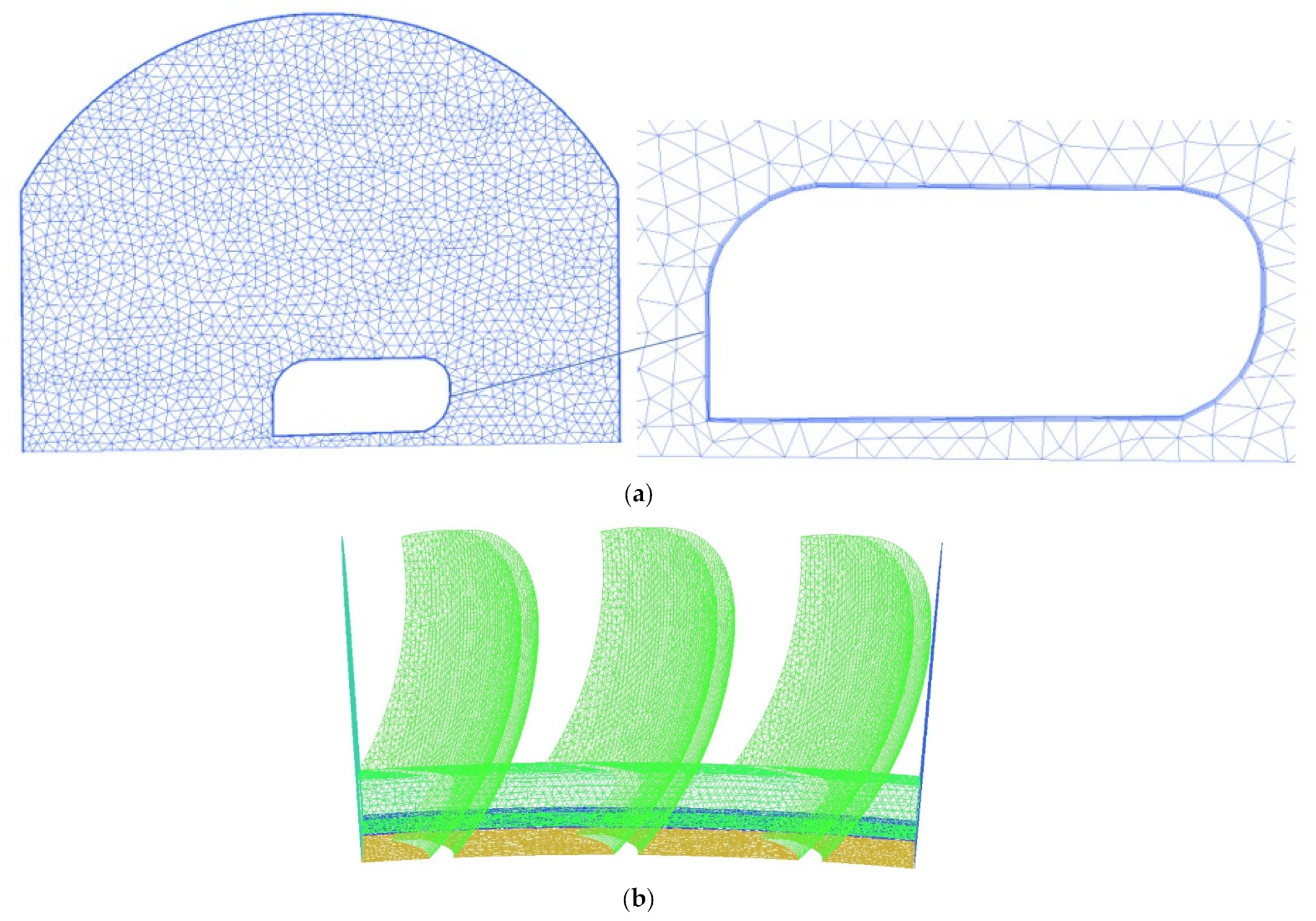
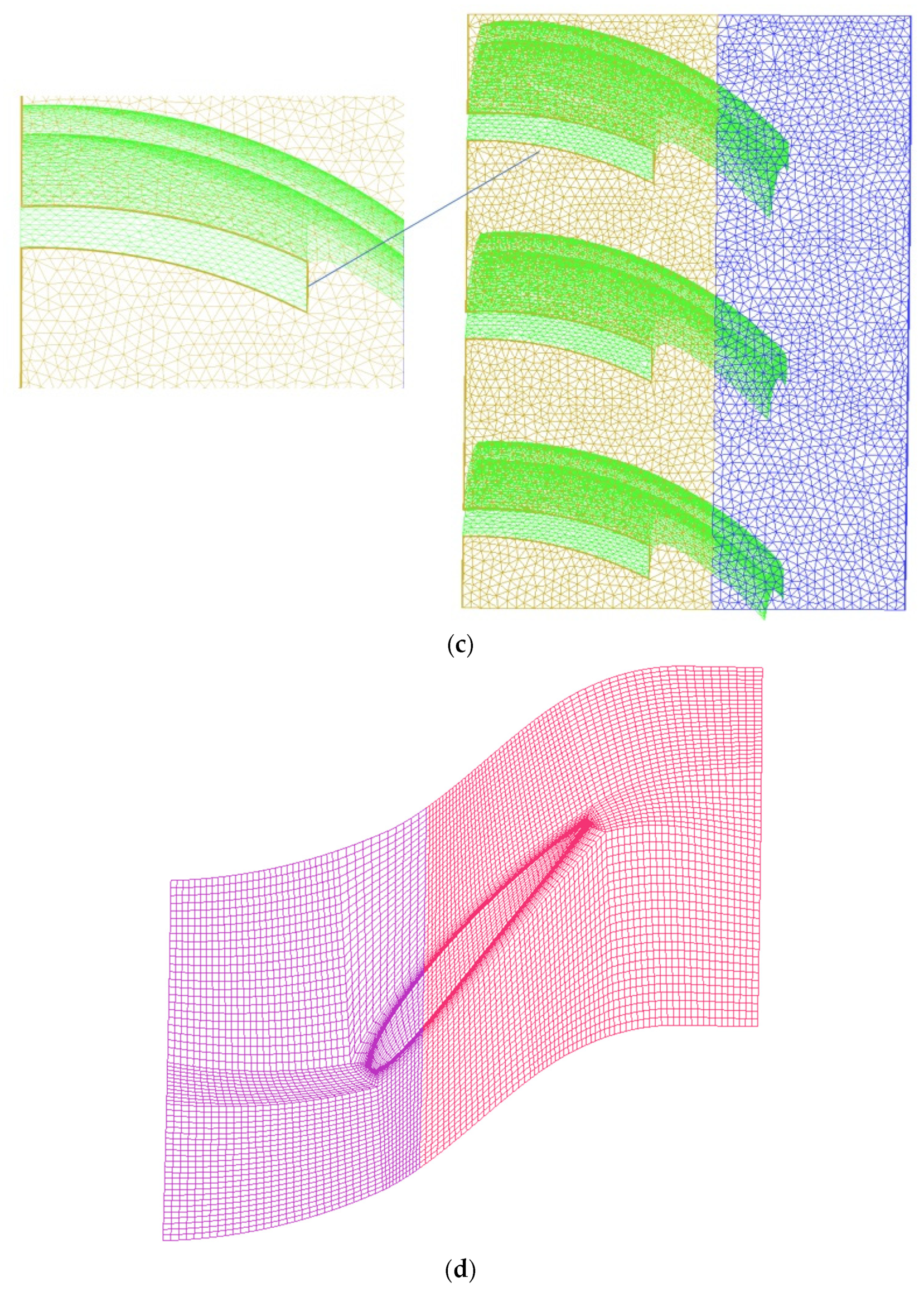
3.2. Meshing Details
3.3. Boundary Conditions
3.4. Governing and Turbulence Equations
3.5. Grid Independence Study
3.6. Convergence Assessment
3.7. Validation of Results
4. Results
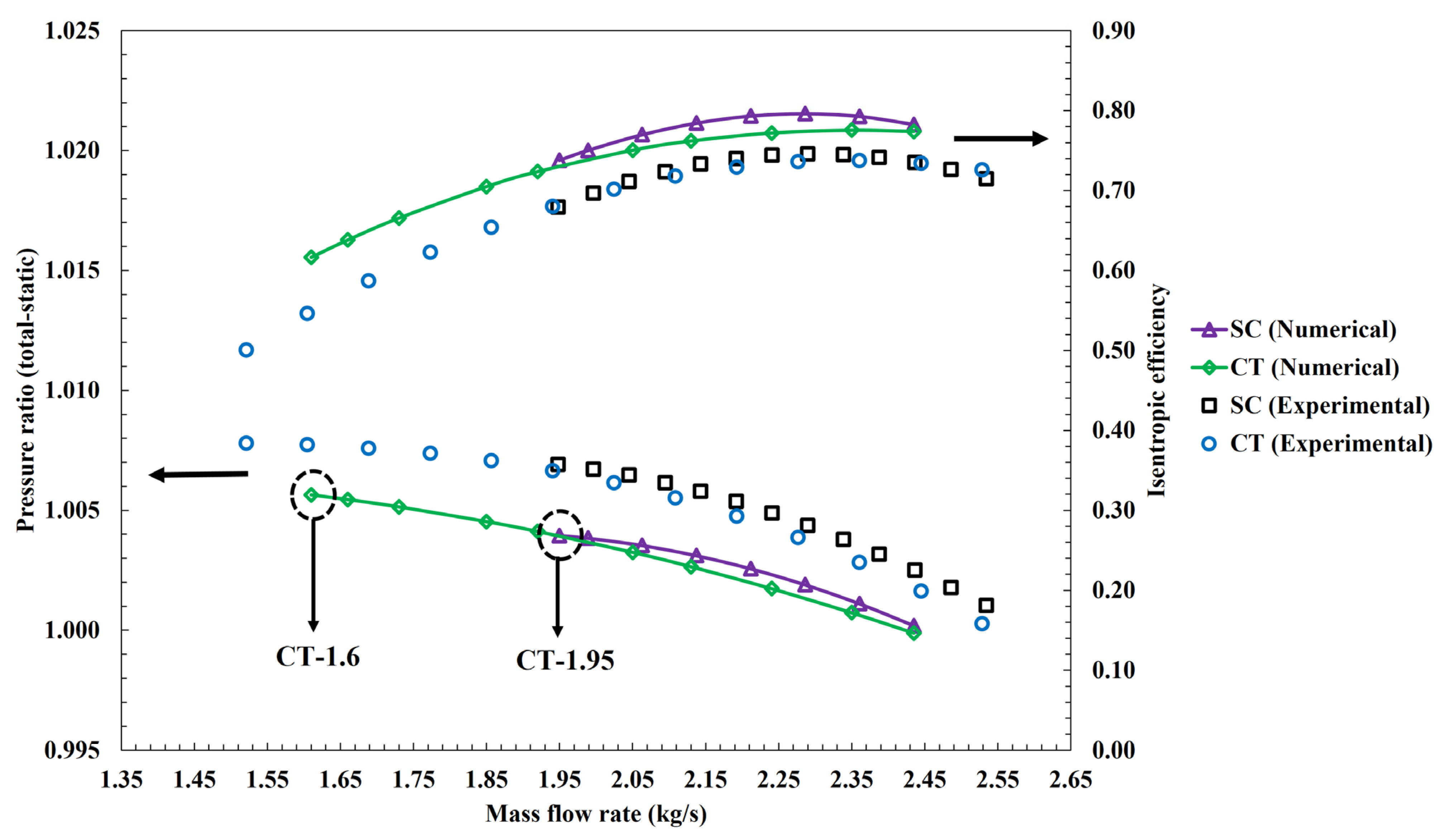
4.1. Compressor Characteristics
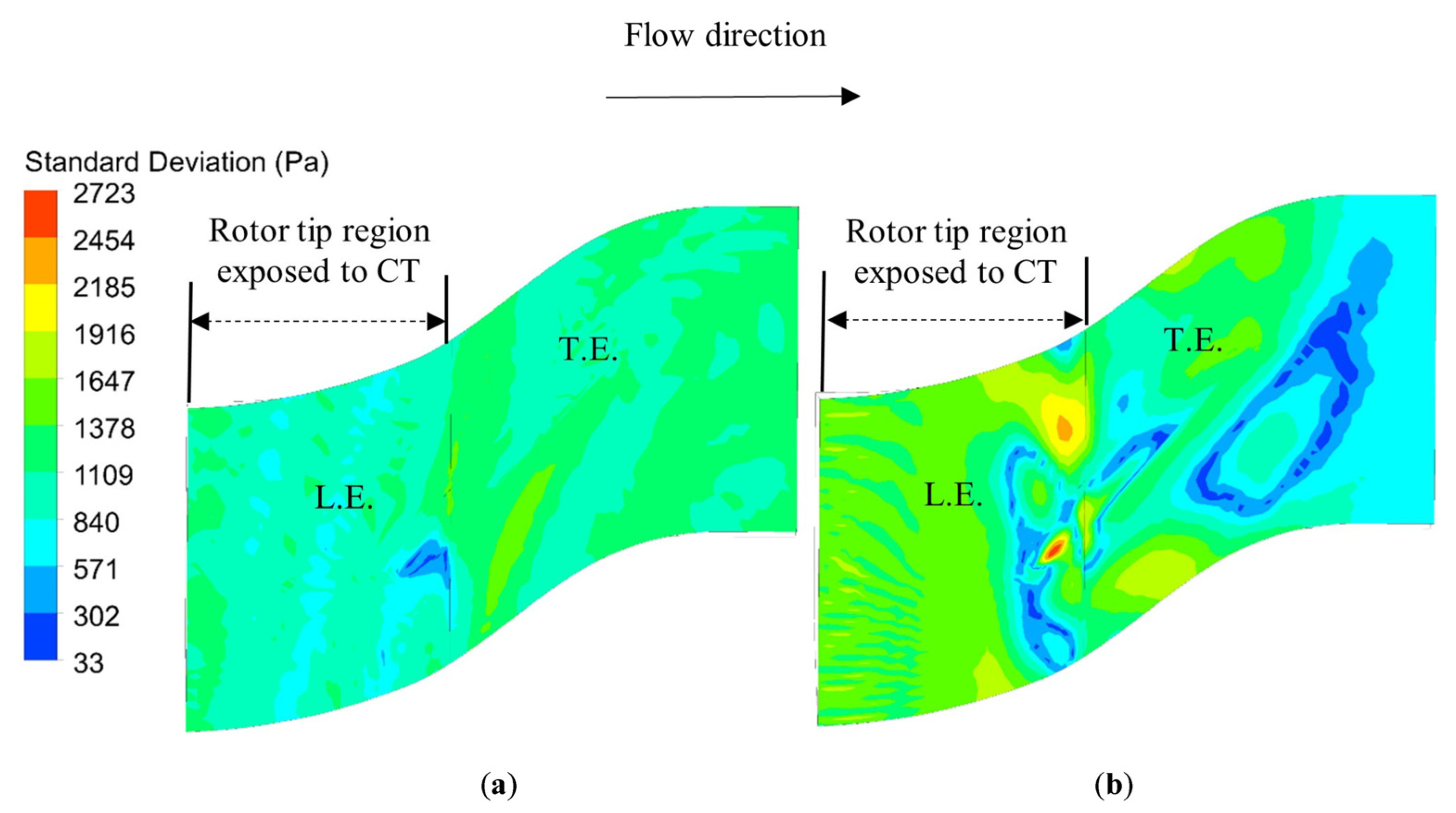
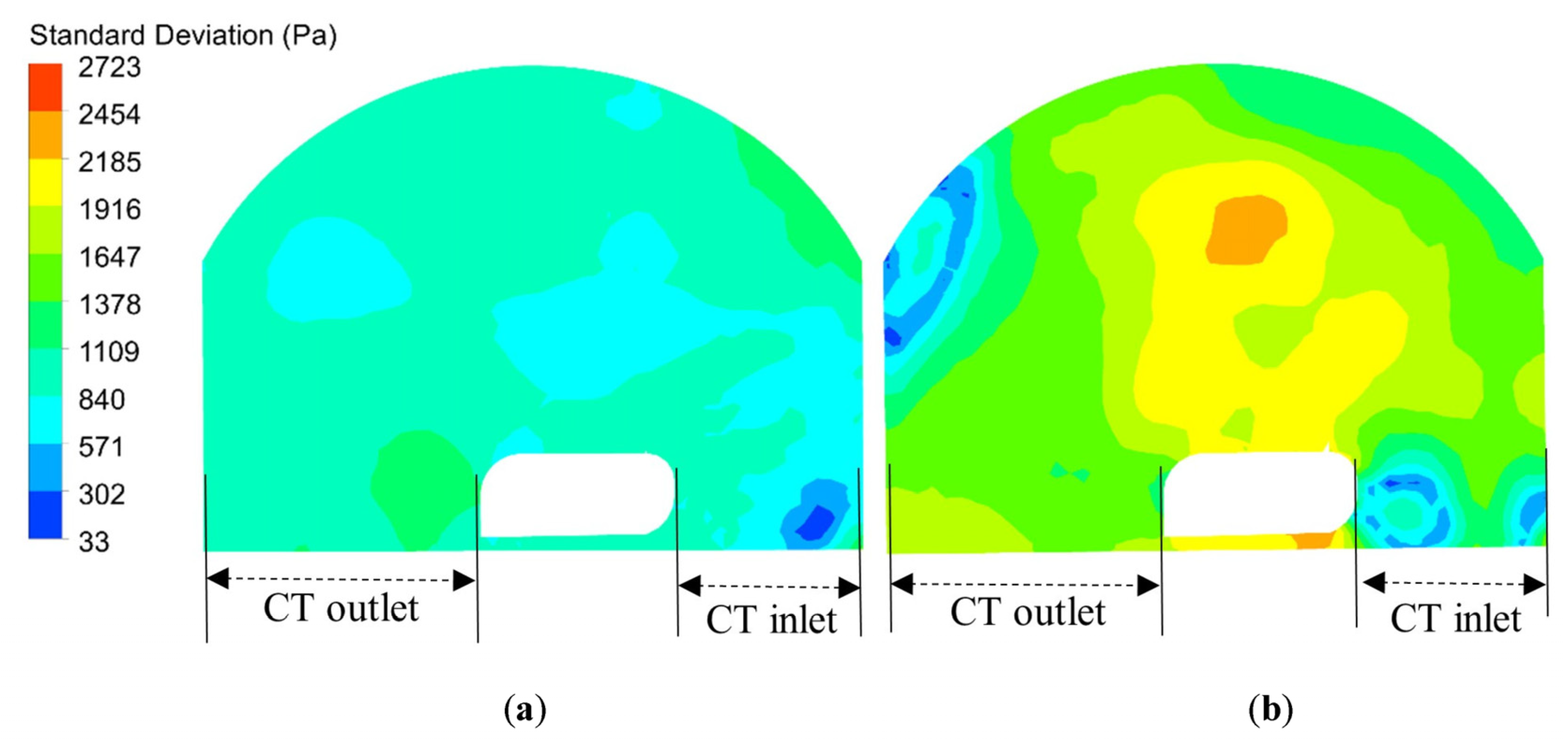
4.2. Unsteadiness of Rotor Blade Tip and Casing Treatment
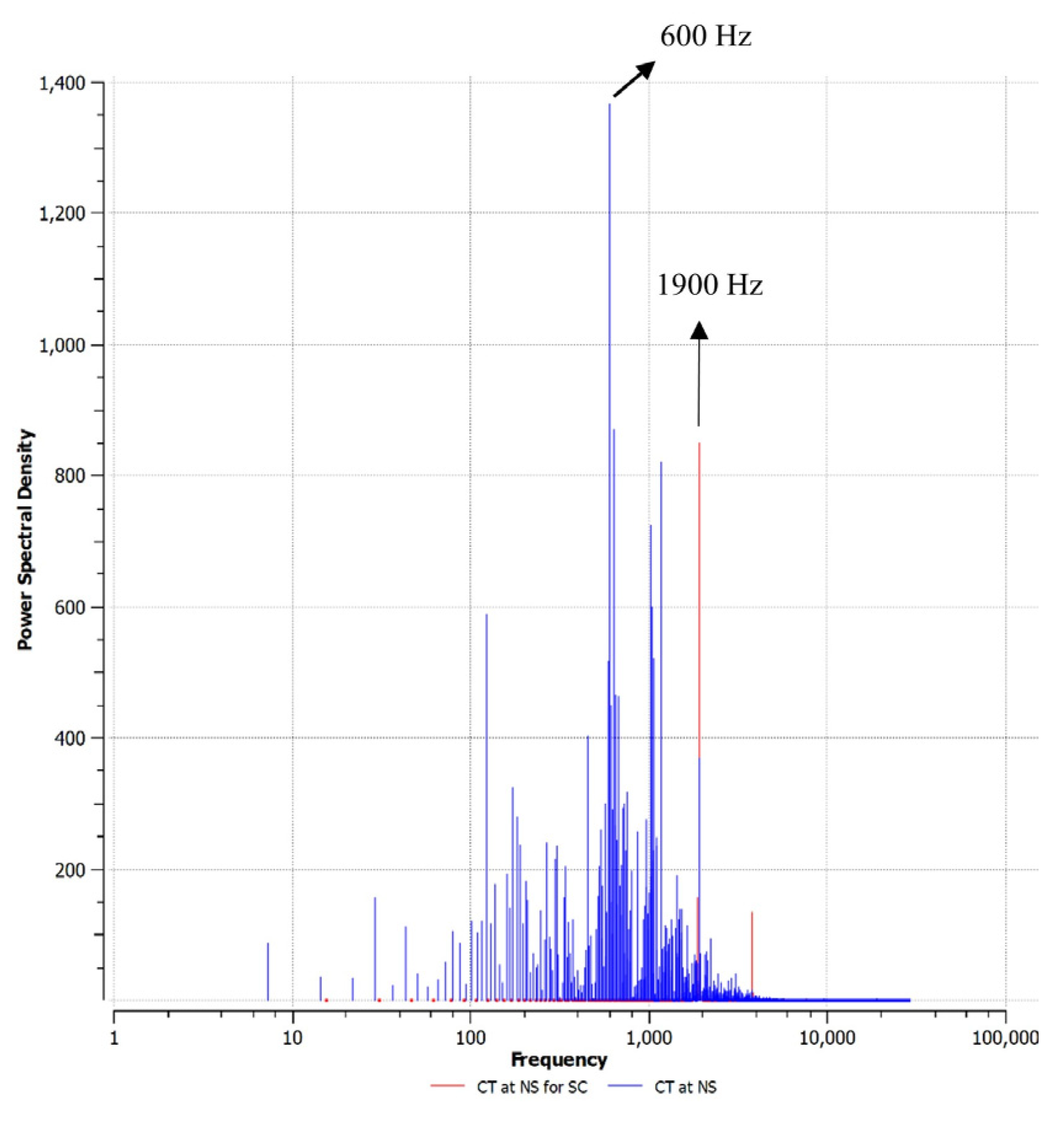
4.3. Frequency Analysis
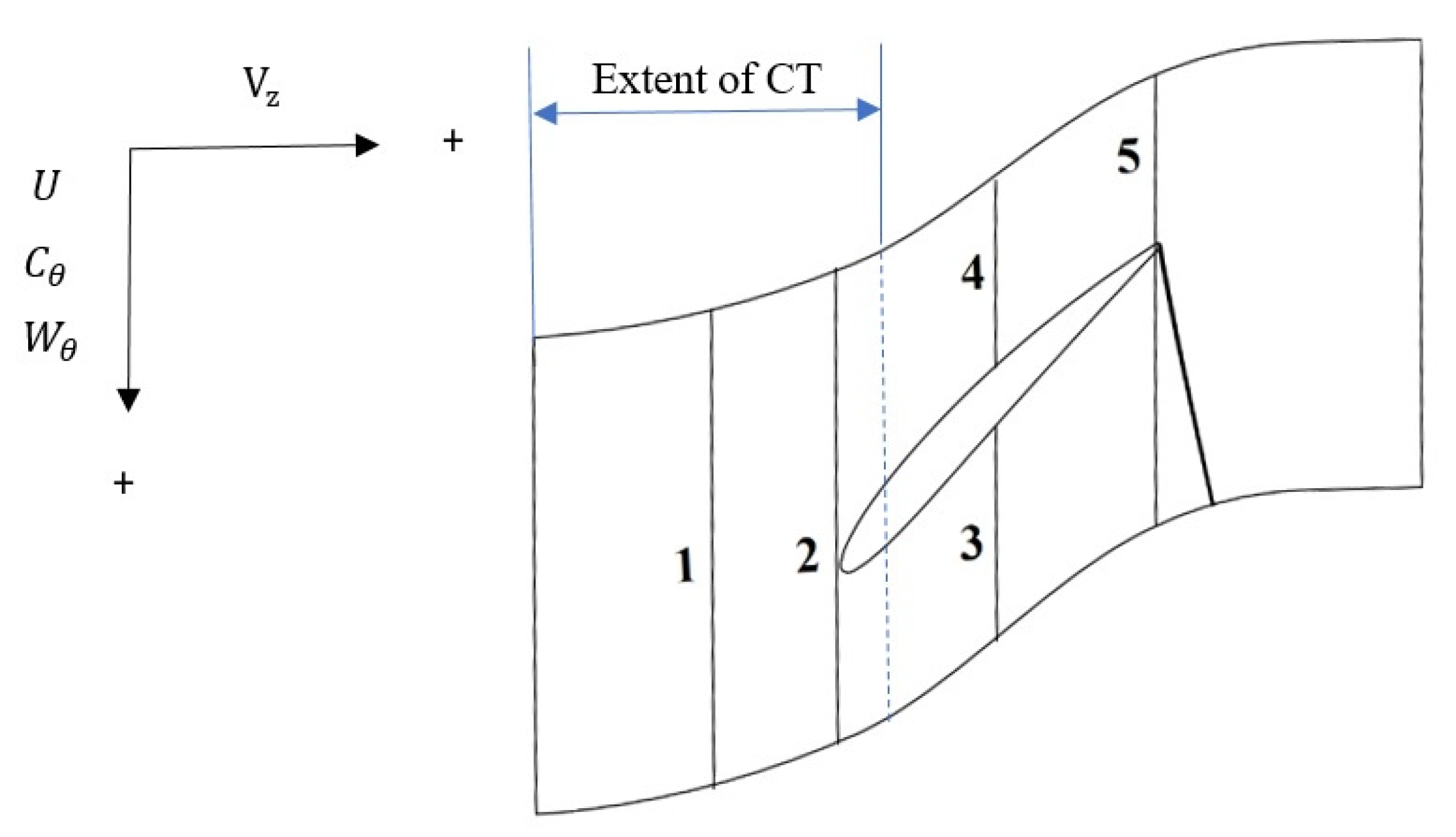
4.4. Velocity Components Analysis
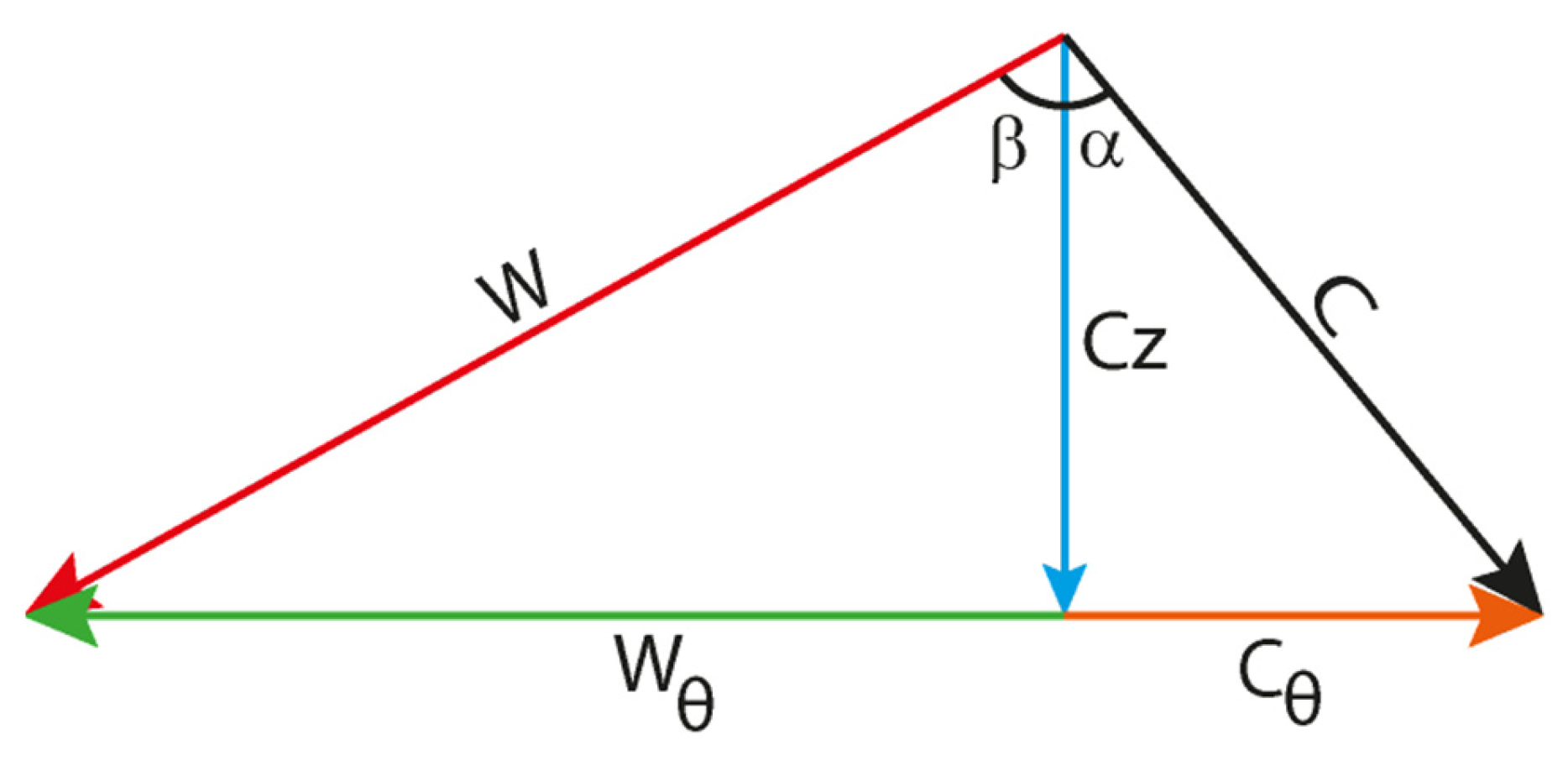
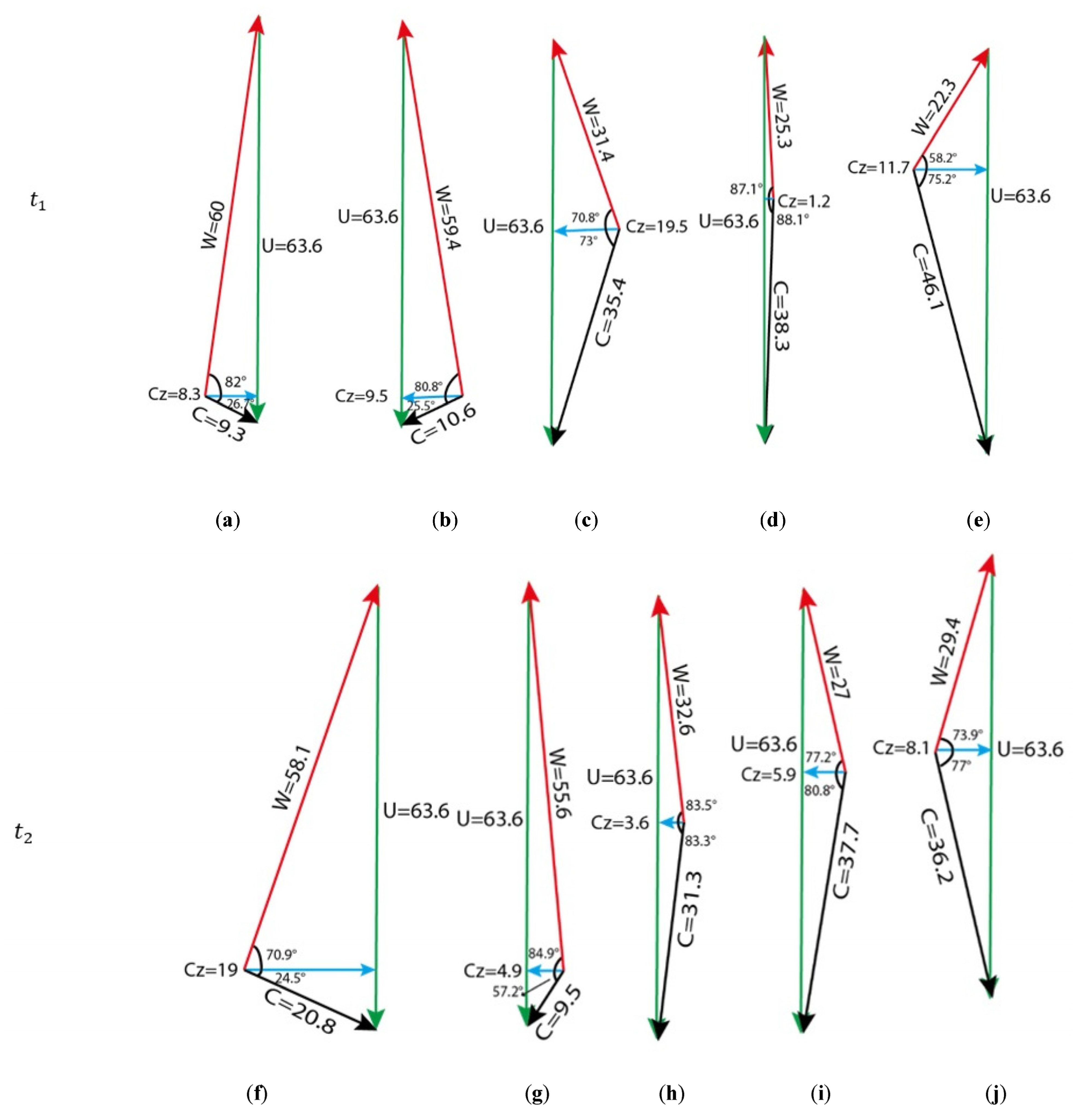
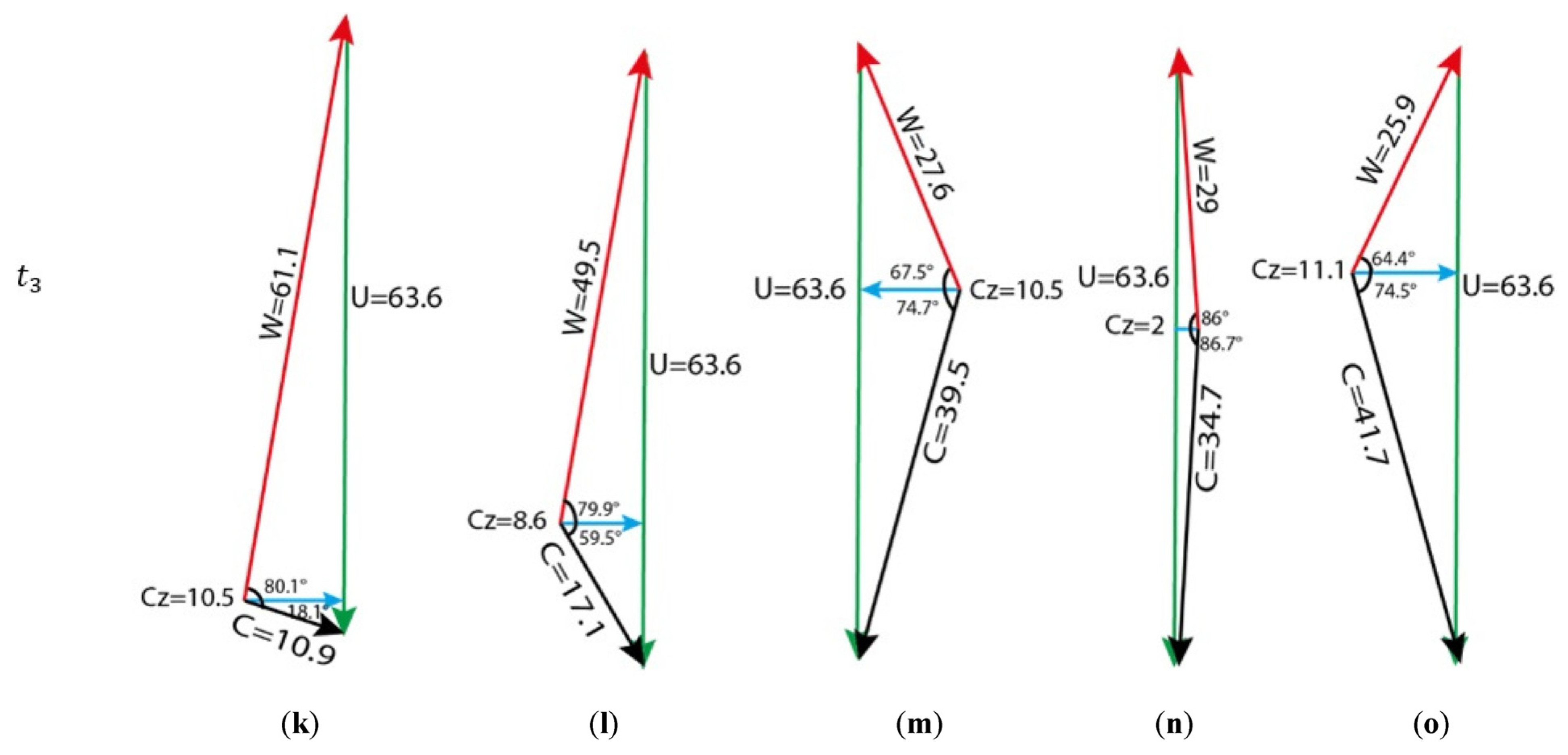
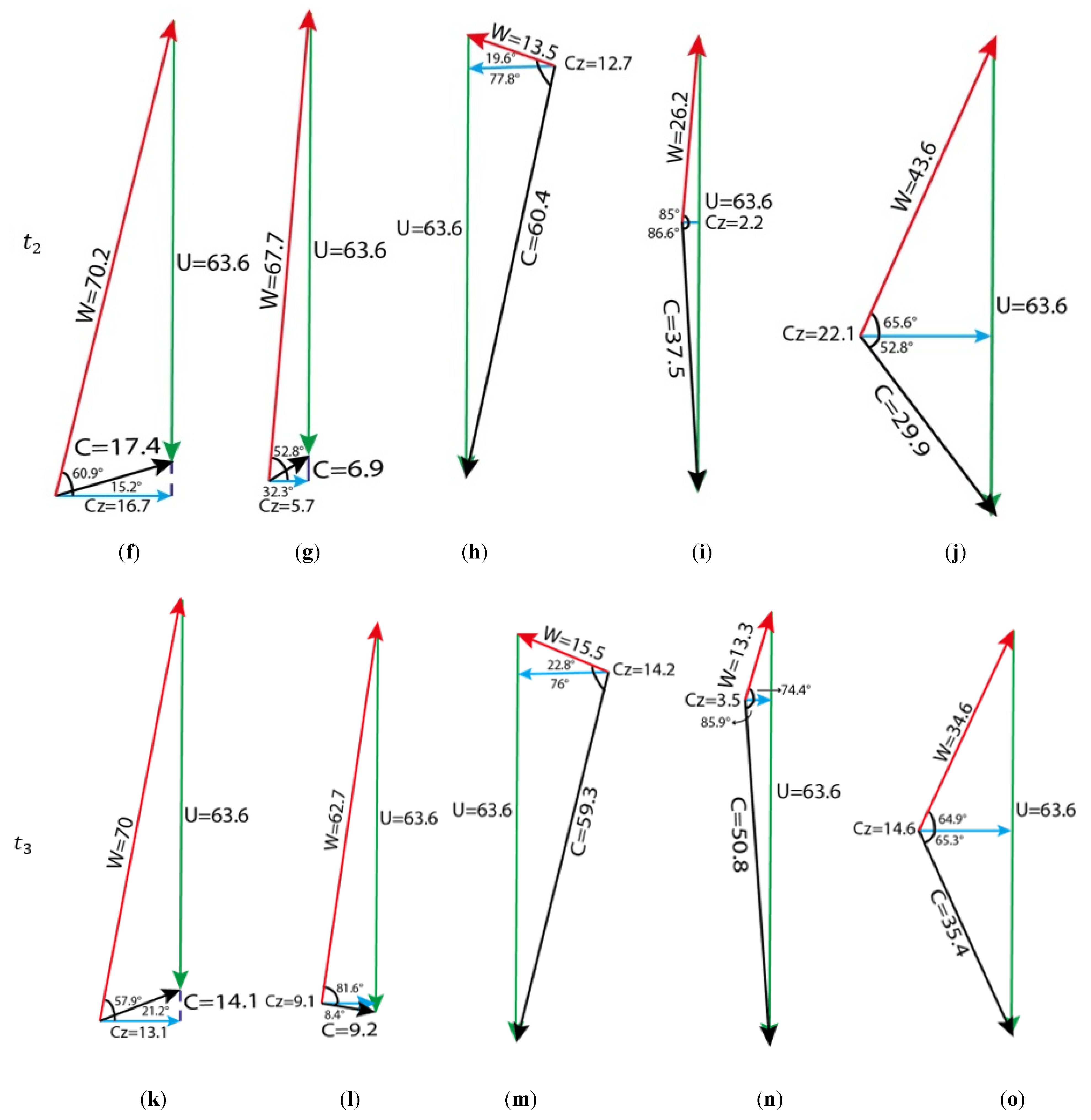
4.5. Velocity Triangles
4.6. Rotor Casing Treatment Flow-Fields
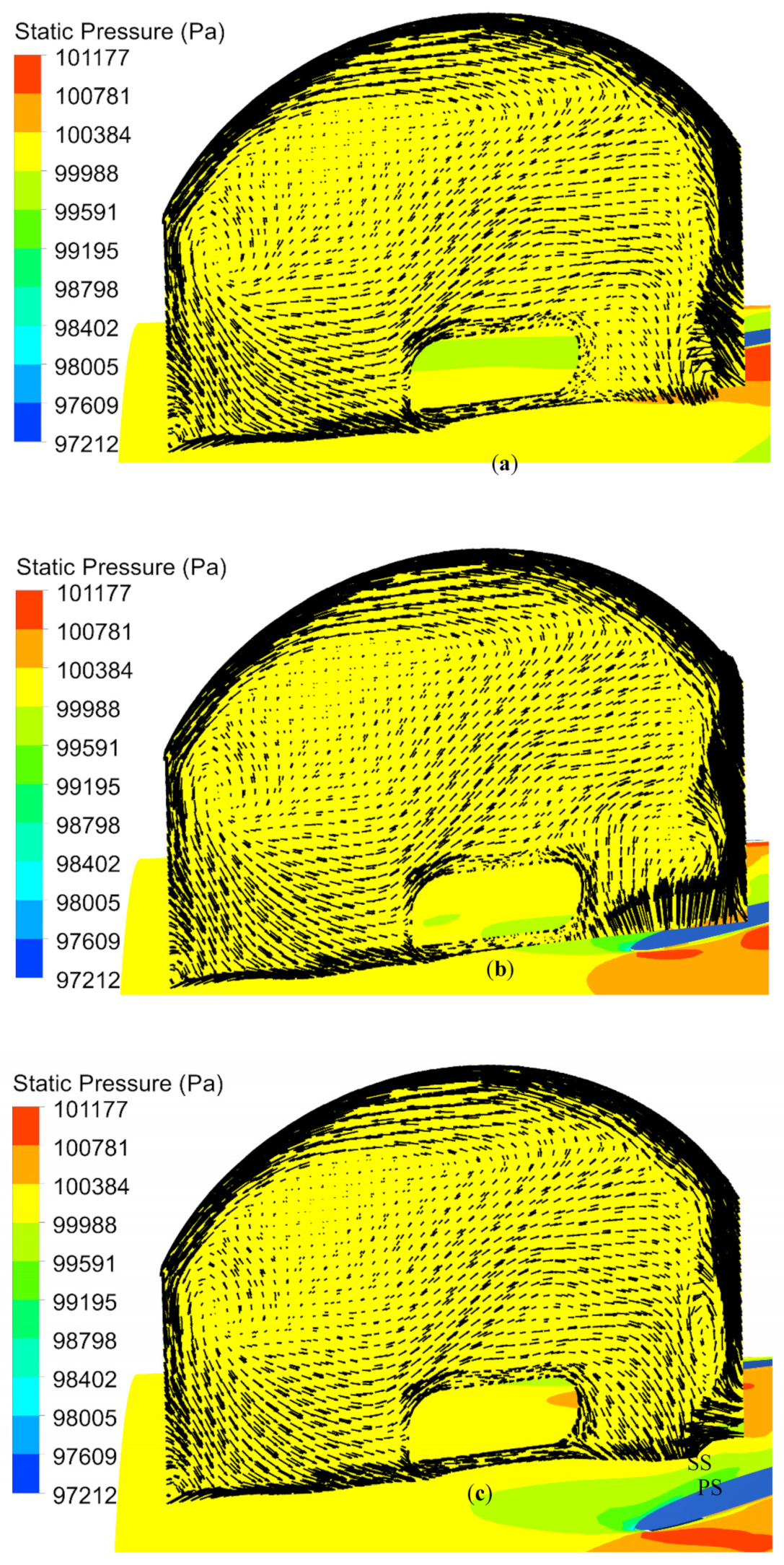
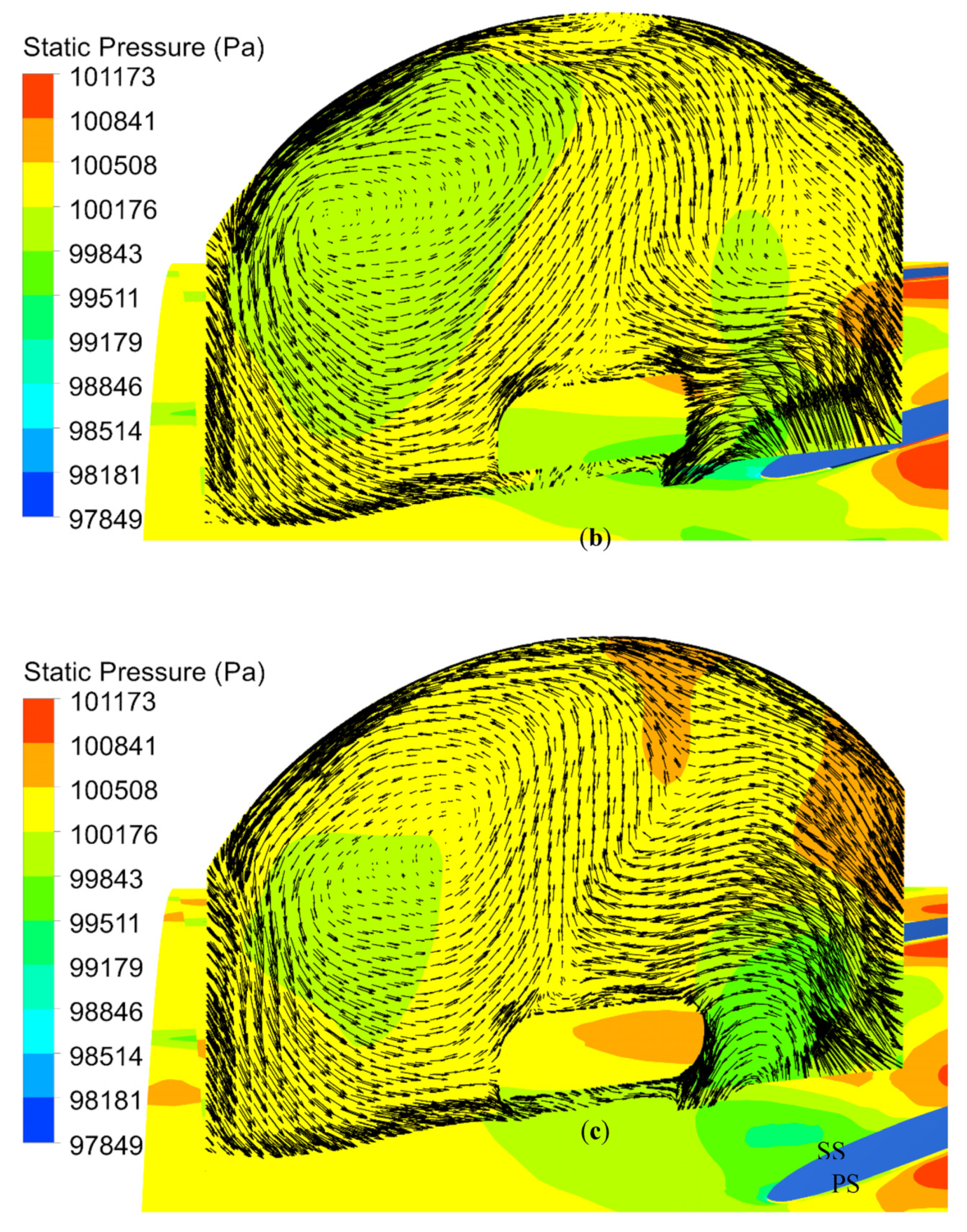
4.6.1. Flow Structure and Pressure Contours
4.6.2. Discussion of Axial Velocity Change at 1.95 and 1.6 kg/s
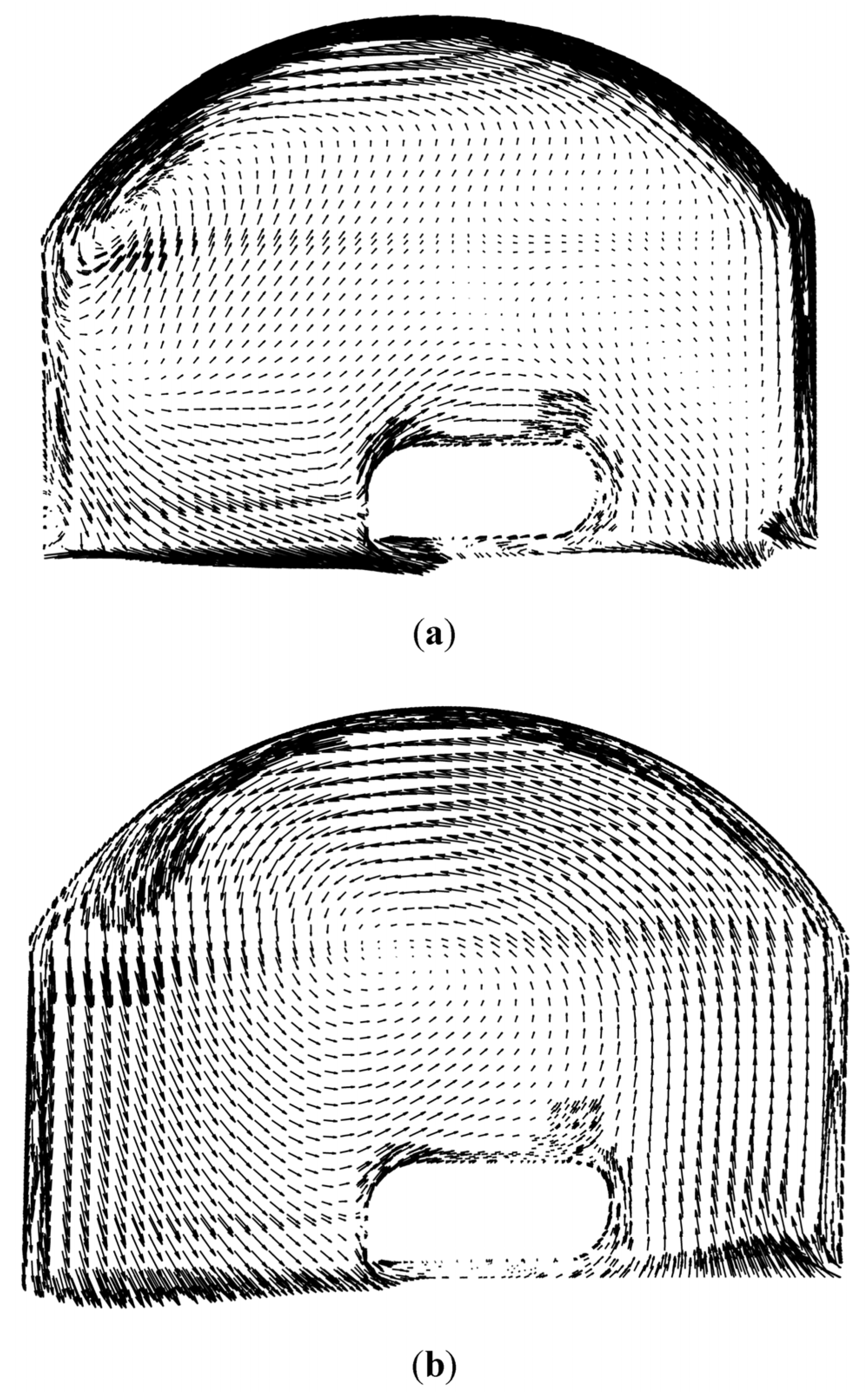
4.6.3. Summary of Flow Streamline Patterns, Fluid Trajectories, and Operating Mechanism
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| BPF | Blade passing frequency (Hz) |
| CAxial | Rotor blade tip axial chord |
| CD | Rotor blade tip axial chord exposure to the casing treatment |
| CT | Casing treatment (vaned-recessed design used in this study with 23.2% exposure) |
| CT at NS | Casing treatment at near stall condition at mass flow 1.6 kg/s |
| CT at NS for SC | Casing treatment at near stall condition for solid casing at mass flow 1.95 kg/s |
| C, W, U | Absolute, relative, blade velocity components (m/s) |
| Absolute swirl (circumferential) velocity component (m/s) | |
| Cz | Axial velocity component (m/s) |
| Mass flow rate (kg/s) | |
| NS | Near stall |
| Sample static pressure (Pa) | |
| Time-averaged static pressure (Pa) | |
| PE | Peak efficiency |
| PS | Pressure surface |
| PR | Pressure ratio |
| PT | Profile transformation |
| SC | Solid casing (no casing treatment) |
| SS | Suction surface |
| T | Rotor passing period (s) |
| t1, t2, t3 | Time (s) |
| t-s | total condition-static condition |
| U | Rotor tip tangential speed (m/s) |
| Radial velocity component (m/s) | |
| Axial velocity component (m/s) | |
| Relative swirl (circumferential) velocity component (m/s) | |
| Standard deviation (Pa) | |
| N | Number of samples |
| Relative flow angle |
References
- Rabe, D.C.; Hah, C. Application of Casing Circumferential Grooves for Improved Stall Margin in a Transonic Axial Compressor; ASME Paper No. 2002-GT-30641; ASME: New York, NY, USA, 2002. [Google Scholar]
- Wilke, I.; Kau, H.-P. A Numerical Investigation of the Flow Mechanisms in a HPC Front Stage with Axial Slots. ASME J. Turbomach. 2004, 126, 339–349. [Google Scholar] [CrossRef]
- Shabbir, A.; Adamczyk, J.D. Flow Mechanism for Stall Margin Improvement Due to Circumferential Casing Grooves on Axial Compressors. ASME J. Turbomach. 2005, 127, 708–717. [Google Scholar] [CrossRef]
- Zhu, J.; Chu, W. The Effects of Bend Skewed Groove Casing Treatment on Performance and Flow Field near Endwall of an Axial Compressor; AIAA 2005-809; AIAA: Reston, VA, USA, 2005. [Google Scholar]
- Lu, X.; Zhu, J.; Chu, W.; Wu, Y. Mechanism of the Interaction between Casing Treatment and Tip Leakage Flow in a Subsonic Compressor; ASME Paper No. GT2006-90077; ASME: New York, NY, USA, 2006. [Google Scholar]
- Lu, X.G.; Chu, W.L.; Zhang, Y.F.; Zhu, J. Experimental and numerical investigation of a subsonic compressor with bend-skewed slot casing treatment. Proc. IMechE Part C J. Mech. Eng. Sci. 2006, 220, 1785–1796. [Google Scholar] [CrossRef]
- Mueller, M.W.; Schiffer, H.P.; Hah, C. Effects of Circumferential Grooves on the Aerodynamic Performance of an Axial Single-Stage Transonic Compressor; ASME Paper No. GT-2007-27365; ASME: New York, NY, USA, 2007. [Google Scholar]
- Hathaway, M.D. Passive Endwall Treatments for Enhancing Stability; NASA TM-214409; NASA: Glenn Research Center, Cleveland, OH, USA, 2007. [Google Scholar]
- Lu, J.; Chu, W.; Wu, Y. Investigation of Skewed Slot Casing on Transonic Axial-Flow Fan Stage; ASME Paper No. GT2009-59417; ASME: New York, NY, USA, 2009. [Google Scholar]
- Houghton, T.; Day, I. Stability Enhancement by Casing Grooves: The Importance of Stall Inception Mechanism and Solidity. ASME J. Turbomach. 2010, 134, 021003. [Google Scholar] [CrossRef]
- Chen, H.; Huang, X.; Shi, K.; Fu, S.; Bennington, M.A.; Morris, S.C.; Ross, M.; McNulty, S.; Wadia, A. A CFD Study of Circumferential Groove Casing Treatments in a Transonic Axial Compressor; ASME Paper No. GT2010-23606; ASME: New York, NY, USA, 2010. [Google Scholar]
- Van de Wyer, N.; Desset, F.B.; Brouckaet, J.F.; Thomas, J.-F.; Hiernaux, S. Experimental Investigation of the Steady and Unsteady Flow Field in a Single Stage Low Pressure Axial Compressor with a Circumferential Groove Casing Treatment; ASME Paper No. GT2010-23474; ASME: New York, NY, USA, 2010. [Google Scholar]
- Zhao, S.; Lu, X. Investigation for the Effects of Circumferential Grooves on the Unsteadiness of Tip Clearance Flow to Enhance Compressor Flow Instability; ASME Paper No. GT2010-22652; ASME: New York, NY, USA, 2010. [Google Scholar]
- Houghton, T.O.; Day, I.J. Enhancing the Stability of Subsonic Compressors Using Casing Grooves. ASME J. Turbomach. 2011, 133, 021007. [Google Scholar] [CrossRef]
- Sakuma, Y.; Watanabe, T.; Himeno, T. Numerical Analysis of Flow in a Transonic Compressor with a Single Circumferential Casing Groove: Influence of Groove Location and Depth on Flow Instability; ASME Paper No. GT2013-94988; ASME: New York, NY, USA, 2013. [Google Scholar]
- Lu, X.; Chu, W.; Zhu, J.; Zhang, Y. Numerical Investigations of the Coupled Flow through a Subsonic Compressor Rotor and Axial Skewed Slot. J. Turbomach. 2009, 131, 011001. [Google Scholar] [CrossRef]
- Li, J.C.; Lin, F.; Wang, S.C.; Du, J.; Nie, C.Q.; Chen, J.Y. Extensive Experimental Study of Circumferential Single Groove in an Axial Flow Compressor; ASME Paper No. GT2014-26859; ASME: New York, NY, USA, 2014. [Google Scholar]
- Ivanov, S.K.; Dudkin, V.E.; Peredery, V.P.; Molchanov, V.N. Axial-Flow Ventilation Fan. No 08318063A, 26 March 1986. [Google Scholar]
- Bard, H. The Stabilisation of Axial Fan Performance. In Proceedings of the Institution of Mechanical Engineers (IMechE) Conference C120/84 on the Installation Effects in Ducted Fan Systems, London, UK, 1–2 May 1984; pp. 100–106. [Google Scholar]
- Miyake, Y.; Inaba, T.; Kato, T. Improvement of unstable characteristics of an axial flow fan by air-separator equipment. J. Fluids Eng. 1987, 109, 36–40. [Google Scholar] [CrossRef]
- Azimian, A.R.; Elder, R.L.; McKenzie, A.B. Application of recess vaned casing treatment to axial flow fans. ASME J. Turbomach. 1990, 112, 145–150. [Google Scholar] [CrossRef]
- Ziabasharhagh, M.; McKenzie, A.B.; Elder, R.L. Recess Vane Passive Stall Control. In Proceedings of the International Gas Turbine and Aeroangine Congress and Exposition, Cologne, Germany, 1–4 June 1992. ASME Paper 1992; 92-GT-36. [Google Scholar]
- Kang, C.S.; McKenzie, A.B.; Elder, R.L. Recessed Casing Treatment Effects on Fan Performance and Flow Field. In Proceedings of the ASME 1995 International Gas Turbine and Aeroengine Congress and Exposition, Houston, TX, USA, 5–8 June 1995. ASME Paper 1995; 95-GT-197. [Google Scholar]
- Akhlaghi, M.; Elder, R.L.; Ramsden, K.W. Effects of a Vane-Recessed Tubular-Passage Passive Stall Control Technique on a Multistage, Low-Speed, Axial-Flow Compressor: Results of Tests on the First Stage with the Rear Stages Removed. In Proceedings of the ASME Turbo Expo 2003, Collocated with the 2003 International Joint Power Generation Conference, Atlanta, GA, USA, 16–19 June 2003. ASME Paper 2003; GT2003-38301. [Google Scholar]
- Ghila, A.; Tourlidakis, A. Computational Analysis of Passive Stall Delay through Vaned Recess Treatment. In Proceedings of the ASME Turbo Expo 2001: Power for Land, Sea, and Air, New Orleans, LA, USA, 4–7 June 2001. ASME Paper 2001; GT-0342. [Google Scholar]
- Yamaguchi, N.; Ogata, M.; Kato, Y. Improvement of stalling characteristics of an axial-flow fan by radial-vaned air-separators. J. Turbomach. 2010, 132, 021015. [Google Scholar] [CrossRef]
- Yamaguchi, N.; Ogata, M.; Kato, Y. Effects of Compact Radial-Vaned Air Separators on Stalling Characteristics of an Axial-Flow Fan. J. Turbomach. 2010, 132, 021009. [Google Scholar] [CrossRef]
- Yelmar, K.; Viswanath, K. Computational Analysis of Recess Vane Geometry Modification in the Casing Treatment Approach to Enhance Stall Margin in Axial Flow Fans; ASME Paper No. IMECE2013-63096; ASME: New York, NY, USA, 2013. [Google Scholar]
- Corsini, A.; Delibra, G.; Rispoli, F.; Sheard, A.G.; Volponi, D. Investigation on Anti-Stall Ring Aerodynamic Performance in an Axial Flow Fan. In Proceedings of the ASME Turbo Expo 2014: Turbine Technical Conference and Exposition, Düsseldorf, Germany, 16–20 June 2014. ASME Paper 2014; GT2014-25794. [Google Scholar]
- Bonanni, T.; Corsini, A.; Delibra, G.; Volponi, D.; Sheard, A.G. Modelling of Axial Fan and Anti-Stall Ring on a Virtual Test Rig for Air Performance Evaluation. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, Republic of Korea, 13–17 June 2016. ASME Paper 2016; GT2016-56862. [Google Scholar]
- Chen, X.; Chu, W.; Zhang, H.; Li, J.; Lang, J. Numerical Investigation of Effect of Recess Vane Casing Treatments on an Axial Lift Fan Performance. In Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, Charlotte, NC, USA, 26–30 June 2017. ASME Paper 2017; GT2017-63767. [Google Scholar]
- Chen, X.; Chu, W.; Zhang, H.; Li, X. Numerical study on inlet angle of guide vane in recess vaned casing treatment. Aerosp. Sci. Technol. 2019, 93, 105323. [Google Scholar] [CrossRef]
- Ghila, A.; Tourlidakis, A. Unsteady Simulations of Recess Casing Treatment in Axial flow Fans. In Proceedings of the ASME Turbo Expo 2006: Power for Land, Sea, and Air, Barcelona, Spain, 8–11 May 2006. ASME Paper 2006; GT2006-90388. [Google Scholar]
- Kumar, S.S.; Alone, D.B.; Thimmaiah, S.M.; Mudipalli, J.R.R.; Kumar, L.; Ganguli, R.; Kandagal, S.B.; Jana, S. Aerodynamic behavior of a transonic axial flow compressor stage with self-recirculating casing treatment. Aerosp. Sci. Technol. 2021, 112, 106587. [Google Scholar] [CrossRef]
- Guo, Y.; Mao, X.; Gao, L. Numerical investigation on the cross-stage self-recirculating casing treatment for a counter-rotating axial compressor. Aerosp. Sci. Technol. 2023, 135, 108161. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, H.; Li, Q.; Jing, F.; Chu, W. Mechanism Underlying the Effect of Self-Circulating Casings with Different Circumferential Coverage Ratios on the Aerodynamic Performance of a Transonic Centrifugal Compressor. Aerospace 2023, 10, 312. [Google Scholar] [CrossRef]
- Vuong, T.D.; Kim, K.Y.; Dinh, C.T. Recirculation-groove coupled casing treatment for a transonic axial compressor. Aerosp. Sci. Technol. 2021, 111, 106556. [Google Scholar] [CrossRef]
- Guo, Y.; Gao, L.; Mao, X. Effect of self-recirculating casing treatment on the unsteady flow and stability of counter-rotating axial-flow compressor. Int. J. Turbo Jet-Eng. 2022, 1–13. [Google Scholar] [CrossRef]
- Ding, S.; Chen, S.; Wang, S.; Wang, Z. Flow mechanism of self-recirculating casing treatment in a low-reaction transonic compressor rotor. Aerosp. Sci. Technol. 2022, 130, 107925. [Google Scholar] [CrossRef]
- Kawase, M.; Rona, A. Effect of a Recirculating Type Casing Treatment on a Highly Loaded Axial Compressor Rotor. Int. J. Turbomach. Propuls. Power 2019, 4, 5. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, C.; Dong, F.; Zhong, X.; Chu, W. Effect of Different Radial Inclined Angles of Self-circulation Casing Treatment on the Transonic Axial Flow Compressor Performance. J. Appl. Fluid Mech. 2022, 16, 533–547. [Google Scholar]
- Akhlaghi, M. Application of a Vaned-Recessed Tubular-Passage Casing Treatment to a Multistage Axial-Flow Compressor. Ph.D. Thesis, University of Cranfield, Cranfield, UK, 2001. [Google Scholar]
- Zori, L.; Galpin, P.; Campregher, R.; Morales, J.C. Time-transformation simulation of a 1.5 stage transonic compressor. ASME J. Turbomach. 2017, 139, 071001. [Google Scholar] [CrossRef]
- Cornelius, C.; Biesinger, T.; Galpin, P.; Braune, A. Experimental and computational analysis of a multistage axial compressor including stall prediction by steady and transient CFD methods. ASME J. Turbomach. 2014, 136, 061013. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Akhlaghi, M.; Azizi, Y.; Nouri, N.M. Estimations of compressor stall and surge using passage stall behaviors. Machines 2022, 10, 706. [Google Scholar] [CrossRef]


| Parameter | Value |
|---|---|
| Number of IGV blades | 34 |
| Number of Rotor blades | 38 |
| Number of Stator blades | 37 |
| Rotor blade tip diameter | 405 mm |
| Rotor blade hub diameter | 284.4 mm |
| Tip clearance | 0.7 mm |
| Hub to tip ratio | 0.7 |
| Rotor blade chord | 30.5 mm |
| Rotor blade aspect ratio | 2.0 |
| Parameter | Value | ||||
|---|---|---|---|---|---|
| Total number of nodes | 791,713 | 1,101,803 | 2,224,443 | 5,085,835 | 10,565,038 |
| Time-averaged pressure at monitor point 1 (Pa) | 100,860 | 100,859 | 100,851 | 100,855 | 100,856 |
| Time-averaged pressure at monitor point 2 (Pa) | 101,444 | 101,453 | 101,459 | 101,471 | 101,472 |
| Time-averaged pressure at monitor point 3 (Pa) | 101,879 | 101,898 | 101,947 | 101,973 | 102,002 |
| Time-averaged pressure ratio | 1.003 | 1.003 | 1.004 | 1.004 | 1.004 |
| Parameter | Experimental | Numerical | Error (%) | |
|---|---|---|---|---|
| Total pressure at inlet | 101,628 | 101,595 | 0.032 | |
| Static pressure at outlet | 101,981 | 101,848 | 0.130 | |
| Total pressure at outlet | 102,722 | 102,578 | 0.140 | |
| Pressure ratio (t-s) | 1.003 | 1.002 | 0.051 | |
| Total pressure at inlet | 101,556 | 101,595 | 0.038 | |
| Static pressure at outlet | 102,157 | 102,001 | 0.152 | |
| Total pressure at outlet | 102,777 | 102,651 | 0.122 | |
| Pressure ratio (t-s) | 1.006 | 1.004 | 0.199 | |
| Total pressure at inlet | 101,512 | 101,595 | 0.082 | |
| Static pressure at outlet | 102,206 | 102,103 | 0.101 | |
| Total pressure at outlet | 102,724 | 102,619 | 0.102 | |
| Pressure ratio (t-s) | 1.007 | 1.005 | 0.199 |
| Parameter | Experimental | Numerical | Error (%) | |
|---|---|---|---|---|
| Total pressure at inlet | 100,281 | 100,570 | 0.288 | |
| Static pressure at outlet | 100,980 | 101,096 | 0.115 | |
| Total pressure at outlet | 101,479 | 101,686 | 0.204 | |
| Pressure ratio (t-s) | 1.007 | 1.005 | 0.176 | |
| Total pressure at inlet | 100,293 | 100,540 | 0.246 | |
| Static pressure at outlet | 101,091 | 101,193 | 0.101 | |
| Total pressure at outlet | 101,451 | 101,734 | 0.279 | |
| Pressure ratio (t-s) | 1.008 | 1.006 | 0.149 |
| Timestep | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Velocity Components (m/s) | ||||||||||
| Section | 1 | −0.4 | −58.5 | 13.3 | −0.6 | −58.3 | 13.1 | −0.8 | −59.7 | 13.4 |
| 2 | 0.5 | −58.2 | 11.6 | 0.1 | −58.1 | 10.8 | 0.6 | −59.2 | 11 | |
| 3 | 2.2 | −23 | −7.5 | 2 | −24 | −7.5 | 2.3 | −21.8 | −8.8 | |
| 4 | −0.3 | −28.2 | 0.9 | 1 | −28 | 0.5 | 0.4 | −28.9 | −1.9 | |
| 5 | 0.6 | −18.8 | 2.9 | 0.5 | −17.5 | 3.2 | 0.6 | −18 | 3 | |
| Timestep | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Velocity Components (m/s) | |||||||||
| Inflow of the casing treatment | 2.2 | 3.6 | 4.9 | 3 | 5.2 | 5 | 3.6 | 4.3 | 5.9 |
| Outflow of the casing treatment | −1.4 | 0.1 | 4.5 | −1.9 | −0.9 | 3.8 | −1.7 | 0.5 | 3.9 |
| Timestep | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Velocity Components (m/s) | ||||||||||
| Section | 1 | −3.4 | −69.3 | 10.9 | −3 | −69.5 | 12 | −2.7 | −69 | 12.5 |
| 2 | 3.5 | −53.3 | 5.7 | 5.2 | −50.4 | 5.5 | 7.4 | −50.9 | 4.4 | |
| 3 | 1.4 | −8.7 | −8.5 | 1.5 | −3.5 | −9.9 | 0.6 | −4.8 | −10.5 | |
| 4 | −0.5 | −22.3 | −0.8 | −0.7 | −25.5 | 1.9 | 0.7 | −15.9 | 8.2 | |
| 5 | 0.6 | −12.7 | 8.7 | 0.4 | −14.8 | 6.7 | 0.1 | −15.1 | 4.8 | |
| Timestep | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Velocity Components (m/s) | |||||||||
| Inflow of the casing treatment | 10.9 | 21.2 | −0.7 | 10.5 | 20.8 | −0.1 | 9.7 | 19.6 | −0.3 |
| Outflow of the casing treatment | −6.7 | −5.3 | 6.1 | −7.2 | −5.3 | 6.1 | −7.3 | −6.3 | 6.3 |
| Time (s) | |||
|---|---|---|---|
| Normalized bleeding/injecting mass flow at CT-1.95 (%) | 2.18 | 1.61 | 2.21 |
| Normalized bleeding/injecting mass flow at CT-1.6 (%) | 7.10 | 7.92 | 8.23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akhlaghi, M.; Azizi, Y. Investigation of Vaned-Recessed Casing Treatment in a Low-Speed Axial-Flow Compressor, Part II: Unsteady Results. Aerospace 2023, 10, 763. https://doi.org/10.3390/aerospace10090763
Akhlaghi M, Azizi Y. Investigation of Vaned-Recessed Casing Treatment in a Low-Speed Axial-Flow Compressor, Part II: Unsteady Results. Aerospace. 2023; 10(9):763. https://doi.org/10.3390/aerospace10090763
Chicago/Turabian StyleAkhlaghi, Mohammad, and Yahya Azizi. 2023. "Investigation of Vaned-Recessed Casing Treatment in a Low-Speed Axial-Flow Compressor, Part II: Unsteady Results" Aerospace 10, no. 9: 763. https://doi.org/10.3390/aerospace10090763
APA StyleAkhlaghi, M., & Azizi, Y. (2023). Investigation of Vaned-Recessed Casing Treatment in a Low-Speed Axial-Flow Compressor, Part II: Unsteady Results. Aerospace, 10(9), 763. https://doi.org/10.3390/aerospace10090763





