Potential Propulsive and Aerodynamic Benefits of a New Aircraft Concept: A Low-Speed Experimental Study
Abstract
1. Introduction
2. Outline of the Project
3. Experimental Setup
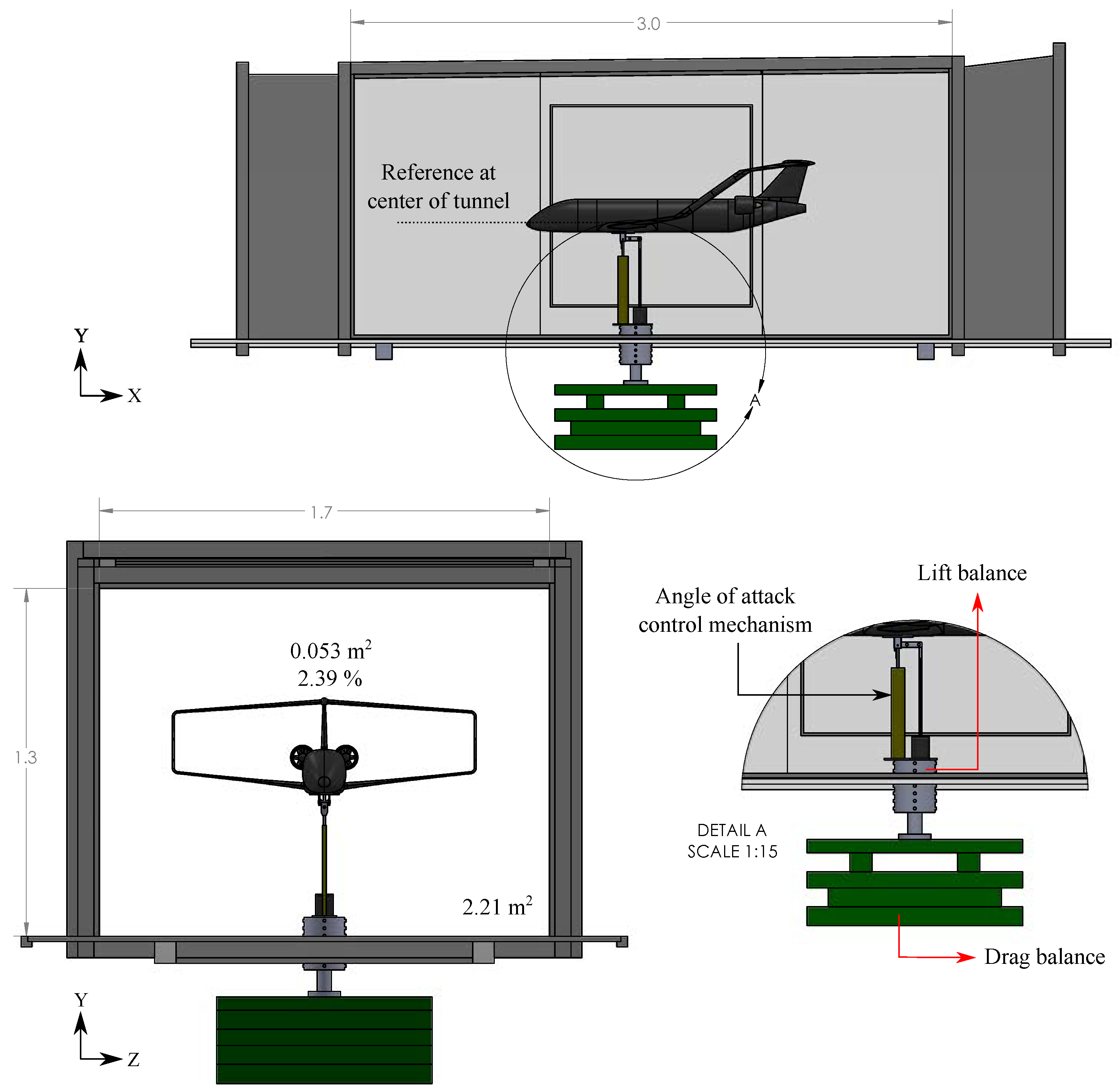
3.1. Wind-Tunnel Facility
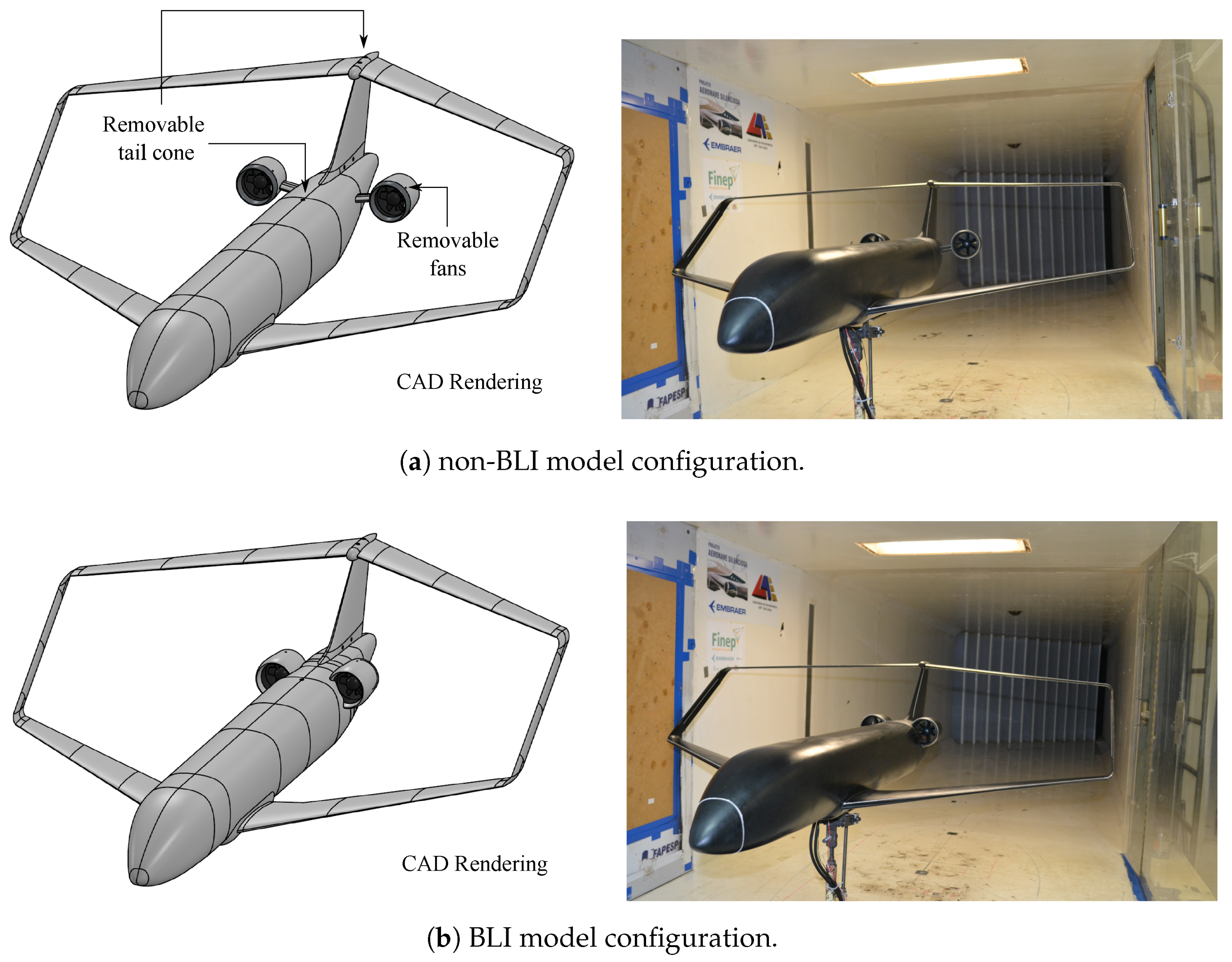
3.2. Tested Configurations
3.3. Test Conditions
3.4. Data Collection
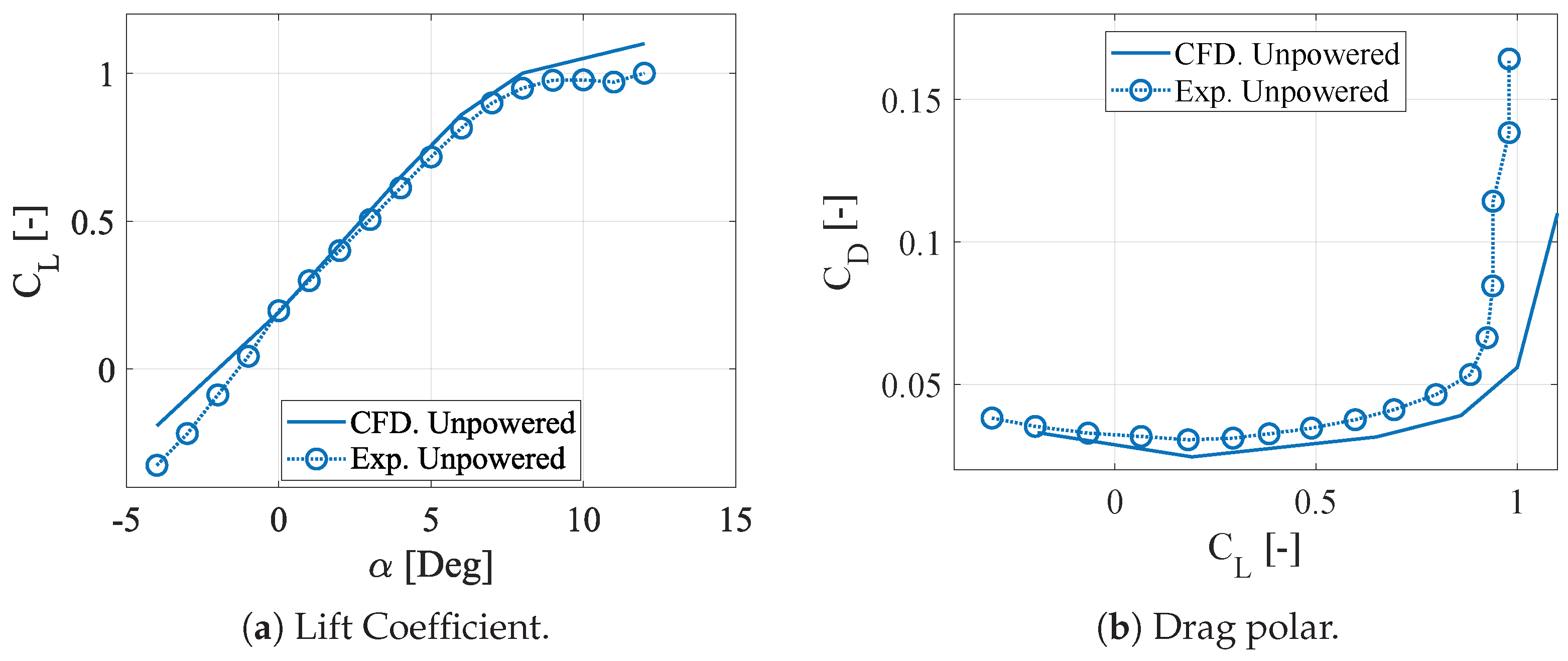
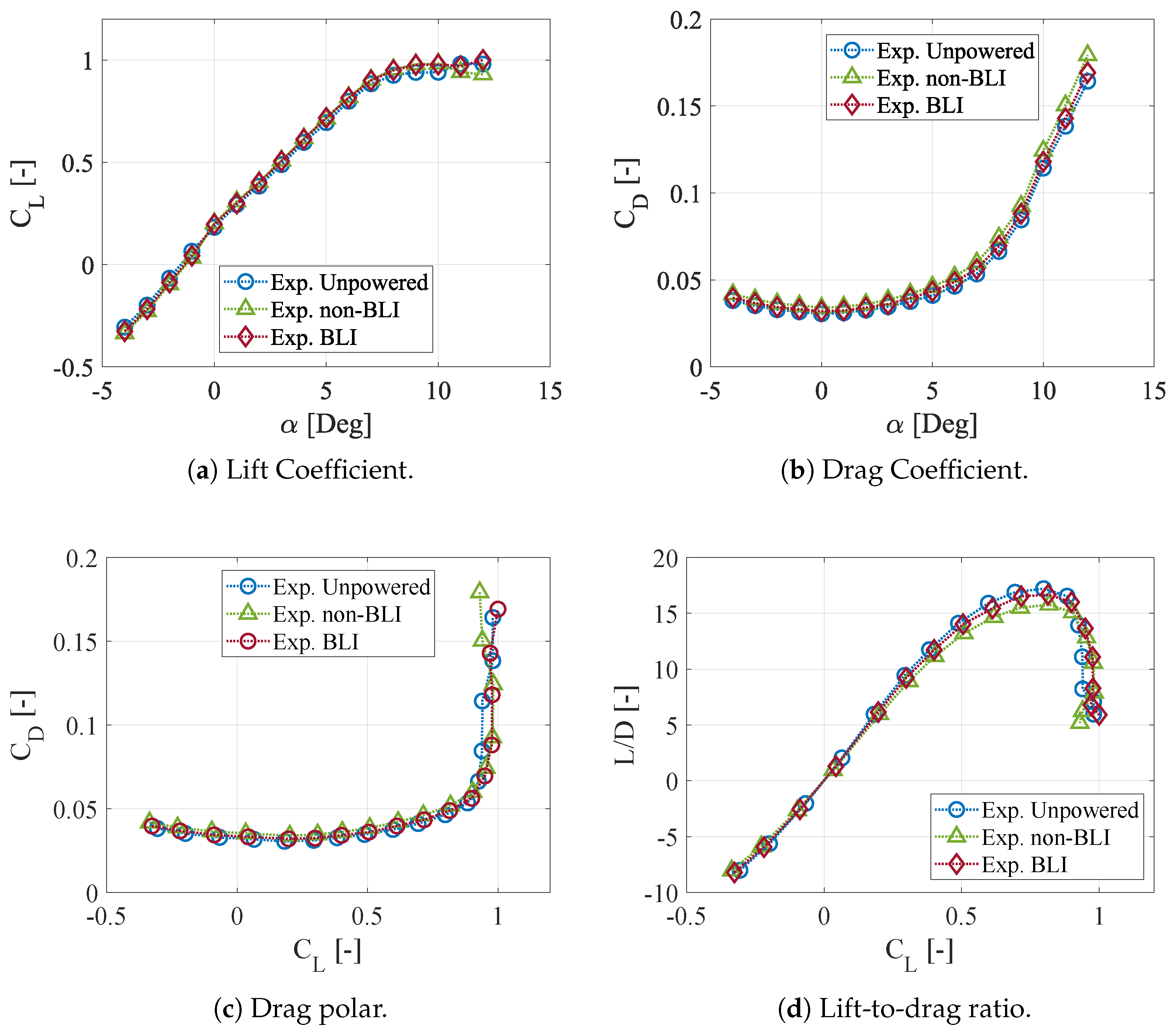
- Aerodynamic force measurements, including lift and drag, were conducted on the unpowered, non-BLI, and BLI configurations to assess their aerodynamic characteristics at various angles of attack (). To isolate the effects of geometry, the engines were easily removed from the nacelle, thus creating conditions simulating through-flow nacelles. This approach enabled the analysis of the pure geometric influences on the aerodynamics. For the unpowered configuration, the wind-tunnel results were compared to CFDs simulations to provide a form of experimental validation. The angles of attack tested ranged from to in increments of .
- Measurements of the electrical power () were conducted on the non-BLI and BLI models. The objective of this experiment was to determine the electrical power coefficient and the net stream-wise force () for a range of fan wheel speeds, with a fixed angle of attack and tunnel velocity. A set of pre-determined fan wheel speeds was defined, and force and power readings were collected. The product between the voltage input to the ESC (v) and the current from the power supply (i) determined the electrical power supplied to the propulsors ().
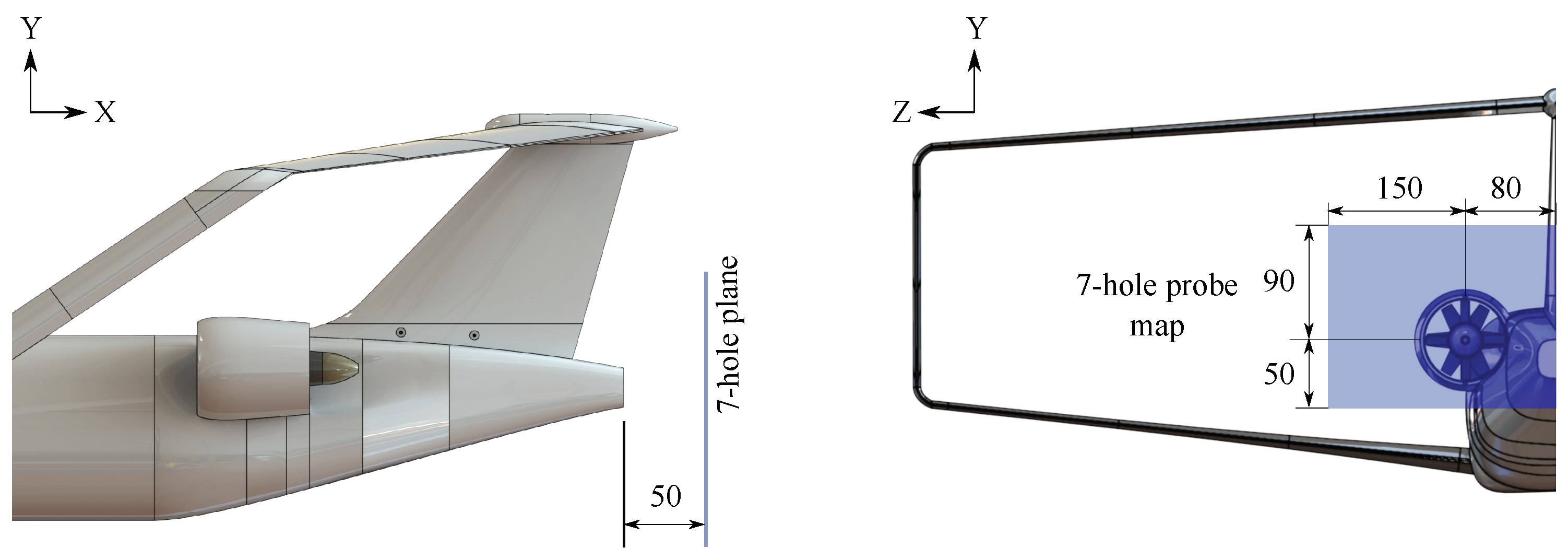
- Flow field measurements were performed on both the powered and unpowered models. The objective was to analyze the variation of the axial flow velocity () across different configurations in a transversal plane. To achieve this, the aerodynamic measurements focused on presenting the stream-wise velocity contours and flow mapping at selected fan wheel speeds, with a fixed angle of attack and tunnel velocity. In the case of the powered configurations, the flow surveys were conducted at power levels that encompassed the range of zero net stream-wise force. This allowed for a comprehensive understanding of the flow characteristics and their relationship to the propulsive performance of the models.
- Measurements of the inlet pressure distortion were conducted on the models without the fan installed to assess the influence of the airframe on the distortion levels across different points in the flight envelope. The objective was to compare the distortion levels between a non-BLI configuration and a BLI configuration. To achieve this, total pressure rake surveys were performed at a fixed tunnel velocity while varying the angle of attack within the range of to in increments. This allowed for a comprehensive analysis of the dependence of the distortion on the airframe and provided insights into the differences between the non-BLI and BLI configurations.
3.5. Measurement Techniques
3.5.1. Aerodynamic Forces
3.5.2. Application of the Power Balance Equation
3.5.3. Flow Mapping
3.5.4. Steady Total Pressure Distribution and Distortion Analysis
4. Results and Discussions
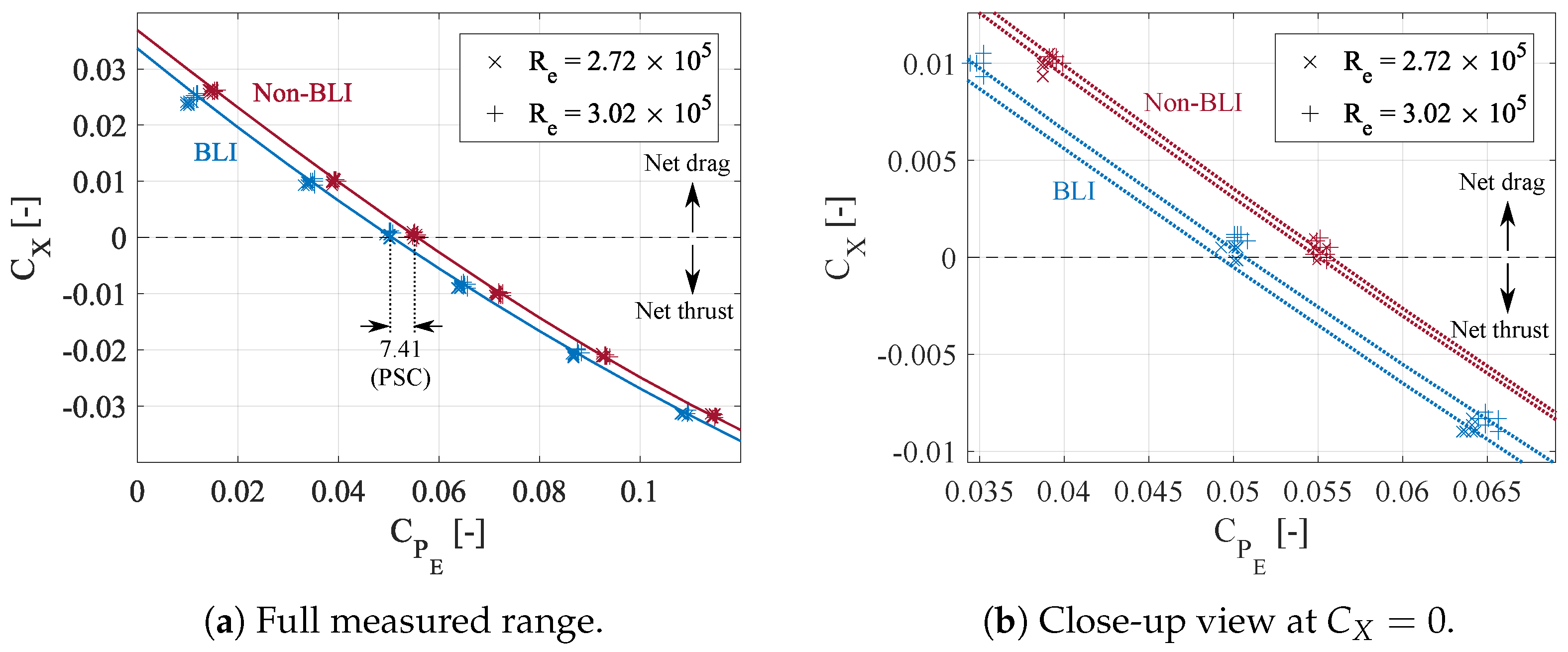
4.1. Power Balance and BLI Benefit
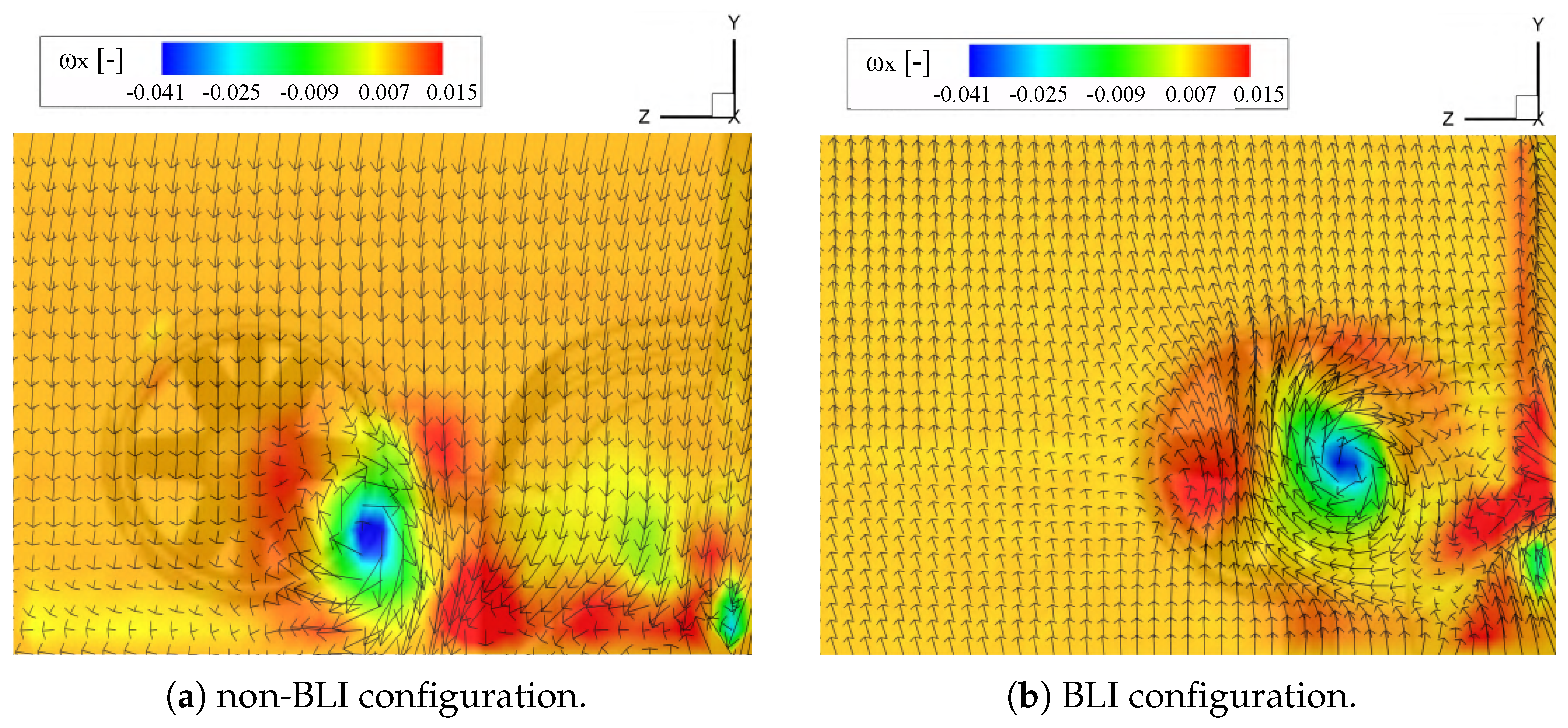
4.2. Seven-Hole Probe Measurements
4.3. Inlet Efficiency
5. Conclusions
- The analysis revealed a clear correlation between jet velocity and the power-saving coefficient due to BLI. The utilization of BLI enabled a lower jet velocity by ingesting slower flow, thus resulting in reduced momentum flow through the propulsor and more efficient power usage. The measurements demonstrated a minimum power saving of 7.41% ± 2.5% compared to conventional freestream flow ingesting configurations, with a 99% confidence interval. However, due to scale model limitations, the BLI benefit was quantified using electrical power instead of mechanical flow power measurements. Subsequent experiments will address this by converting the electrical power into mechanical flow power, thereby incorporating shaft and fan efficiencies.
- While the analysis did not explicitly evaluate the BLI benefit for an actual transonic transport aircraft, it did establish the processes necessary for evaluating the BLI’s potential on real aircraft geometries. This enables the integration of novel propulsion technologies with the airframe. The experiment’s results align closely with steady CFD-RANS simulations of the aircraft at actual scale and flight conditions, with a margin of error of ±2.5% due to aerodynamic modeling uncertainties.
- These results contribute to our understanding of BLI aerodynamics for several reasons. Firstly, the fan was appropriately scaled to match the full-scale fuselage boundary layer. Secondly, the utilized power balance method does not account for differences in the Reynolds and Mach numbers, and the BLI benefits primarily stemmed from a lower jet-to-freestream velocity ratio (reduction of approximately 4.63%) and reduced external losses due to a smaller nacelle wetted area (reduction of around 5.62%) compared to the non-BLI configuration. Thirdly, previous research suggests that compressibility effects have a minimal impact on the fuselage boundary layer. This demonstrates the efficacy of the current aerodynamic model experiment in assessing the aero-propulsive efficiency of a BLI aircraft configuration.
- The aerodynamic flow measurements confirmed the presence of flow distortion, which restricts the aerodynamic performance of the BLI configuration. Further investigations should focus on understanding the specific response of the fan to this distortion, thus considering material limitations and potential issues related to the noise and vibration caused by nonuniform incoming flow.
- Flow measurements were taken using a seven-hole probe. The probe’s interference with the flow warrants additional investigation, and the use of non-intrusive flow measurement techniques such as particle image velocimetry (PIV) can provide a comprehensive assessment of the flow field. While this research provides direct evidence of the benefits of boundary-layer ingestion, further studies are necessary to fully comprehend its influence on aircraft performance during detailed design phases.
- It is important to note that the experiments were conducted at lower Reynolds numbers and subsonic conditions when compared to actual flight. Therefore, it is essential to consider that the performance coefficients may differ in a full-scale application. More detailed research focusing on flight conditions will enable a more accurate comparison of different configurations.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Calibration and Correction of Aerodynamic Forces
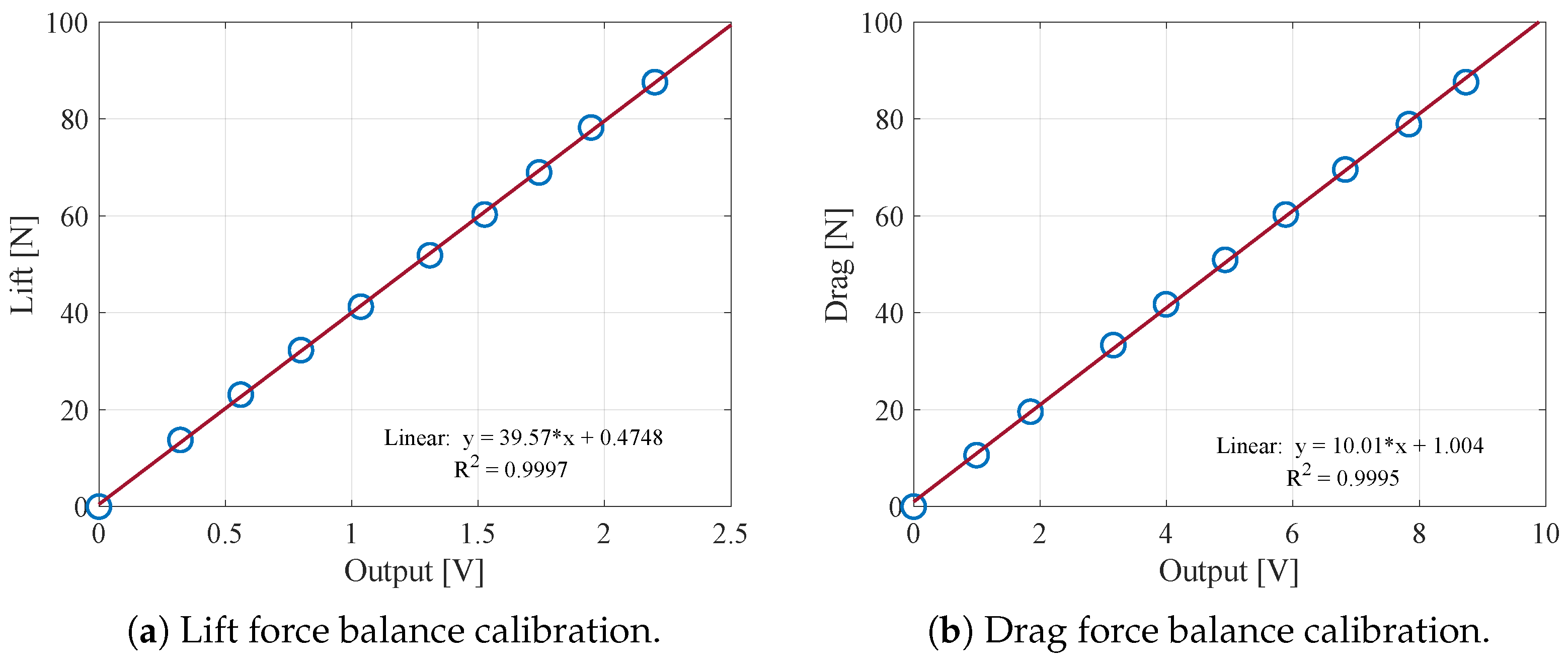
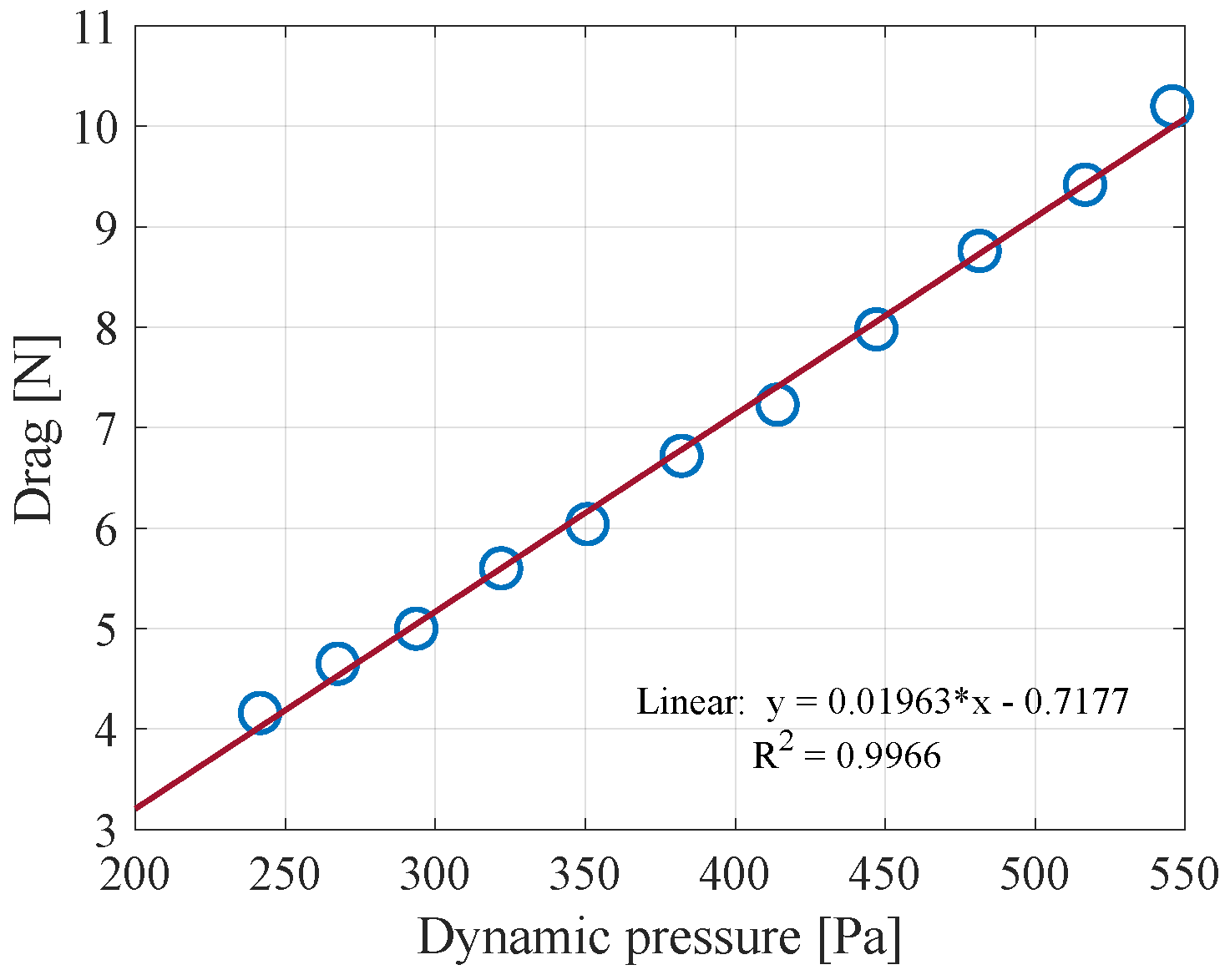
Appendix B. Measurement Uncertainty and Repeatability
| Uncertainty in | Value |
|---|---|
| Atmospheric pressure | |
| Dynamic pressure | |
| Temperature |
References
- Bravo-Mosquera, P.D.; Catalano, F.M.; Zingg, D.W. Unconventional aircraft for civil aviation: A review of concepts and design methodologies. Prog. Aerosp. Sci. 2022, 131, 100813. [Google Scholar] [CrossRef]
- Moirou, N.G.; Sanders, D.S.; Laskaridis, P. Advancements and prospects of boundary layer ingestion propulsion concepts. Prog. Aerosp. Sci. 2023, 138, 100897. [Google Scholar] [CrossRef]
- Brelje, B.J.; Martins, J.R. Electric, hybrid, and turboelectric fixed-wing aircraft: A review of concepts, models, and design approaches. Prog. Aerosp. Sci. 2019, 104, 1–19. [Google Scholar] [CrossRef]
- Braga, D.F.; Tavares, S.; Da Silva, L.F.; Moreira, P.; De Castro, P.M. Advanced design for lightweight structures: Review and prospects. Prog. Aerosp. Sci. 2014, 69, 29–39. [Google Scholar] [CrossRef]
- Liebeck, R.H. Design of the blended wing body subsonic transport. J. Aircr. 2004, 41, 10–25. [Google Scholar] [CrossRef]
- Okonkwo, P.; Smith, H. Review of evolving trends in blended wing body aircraft design. Prog. Aerosp. Sci. 2016, 82, 1–23. [Google Scholar] [CrossRef]
- Cavallaro, R.; Demasi, L. Challenges, ideas, and innovations of joined-wing configurations: A concept from the past, an opportunity for the future. Prog. Aerosp. Sci. 2016, 87, 1–93. [Google Scholar] [CrossRef]
- Abu Salem, K.; Cipolla, V.; Palaia, G.; Binante, V.; Zanetti, D. A physics-based multidisciplinary approach for the preliminary design and performance analysis of a medium range aircraft with box-wing architecture. Aerospace 2021, 8, 292. [Google Scholar] [CrossRef]
- Gur, O.; Bhatia, M.; Schetz, J.A.; Mason, W.H.; Kapania, R.K.; Mavris, D.N. Design optimization of a truss-braced-wing transonic transport aircraft. J. Aircr. 2010, 47, 1907–1917. [Google Scholar] [CrossRef]
- Bradley, M.K.; Droney, C.K.; Allen, T.J. Subsonic Ultra Green Aircraft Research: Phase II. Volume 1; Truss Braced Wing Design Exploration; Technical Report; National Aeronautics and Space Admin Langley Research Center: Hampton, VA, USA, 2015.
- Drela, M. Development of the D8 transport configuration. In Proceedings of the 29th AIAA Applied Aerodynamics Conference, Honolulu, HI, USA, 27–30 June 2011; p. 3970. [Google Scholar]
- Uranga, A.; Drela, M.; Greitzer, E.M.; Hall, D.K.; Titchener, N.A.; Lieu, M.K.; Siu, N.M.; Casses, C.; Huang, A.C.; Gatlin, G.M.; et al. Boundary layer ingestion benefit of the D8 transport aircraft. AIAA J. 2017, 55, 3693–3708. [Google Scholar] [CrossRef]
- Menegozzo, L.; Benini, E. Boundary layer ingestion propulsion: A review on numerical modeling. J. Eng. Gas Turbines Power 2020, 142, 120801. [Google Scholar] [CrossRef]
- Seitz, A.; Habermann, A.L.; Peter, F.; Troeltsch, F.; Castillo Pardo, A.; Della Corte, B.; Van Sluis, M.; Goraj, Z.; Kowalski, M.; Zhao, X.; et al. Proof of concept study for fuselage boundary layer ingesting propulsion. Aerospace 2021, 8, 16. [Google Scholar] [CrossRef]
- Carter, M.B.; Campbell, R.L.; Pendergraft, O.C., Jr.; Friedman, D.M.; Serrano, L. Designing and testing a blended wing body with boundary-layer ingestion nacelles. J. Aircr. 2006, 43, 1479–1489. [Google Scholar] [CrossRef]
- Drela, M. Power balance in aerodynamic flows. AIAA J. 2009, 47, 1761–1771. [Google Scholar] [CrossRef]
- Hall, D.K.; Huang, A.C.; Uranga, A.; Greitzer, E.M.; Drela, M.; Sato, S. Boundary layer ingestion propulsion benefit for transport aircraft. J. Propuls. Power 2017, 33, 1118–1129. [Google Scholar] [CrossRef]
- Uranga, A.; Drela, M.; Hall, D.K.; Greitzer, E.M. Analysis of the Aerodynamic Benefit from Boundary Layer Ingestion for Transport Aircraft. AIAA J. 2018, 56, 4271–4281. [Google Scholar] [CrossRef]
- Della Corte, B.; van Sluis, M.; Veldhuis, L.L.; Gangoli Rao, A. Power Balance Analysis Experiments on an Axisymmetric Fuselage with an Integrated Boundary-Layer-Ingesting Fan. AIAA J. 2021, 59, 5211–5224. [Google Scholar] [CrossRef]
- Della Corte, B.; van Sluis, M.; Gangoli Rao, A.; Veldhuis, L.L. Aerodynamic Performance of an Aircraft with Aft-Fuselage Boundary Layer Ingestion Propulsion. In Proceedings of the AIAA AVIATION 2021 FORUM, Virtual, 2–6 August 2021; p. 2467. [Google Scholar]
- A320—Aircraft Characteristics Airport and Maintenance Planning. 2005. Available online: https://www.airbus.com/sites/g/files/jlcbta136/files/2021-11/Airbus-Commercial-Aircraft-AC-A320.pdf (accessed on 5 March 2021).
- Bravo-Mosquera, P.D.; Cerón-Muñoz, H.D.; Catalano, F.M. Design, aerodynamic analysis and optimization of a next-generation commercial airliner. J. Braz. Soc. Mech. Sci. Eng. 2022, 44, 609. [Google Scholar] [CrossRef]
- Bravo-Mosquera, P.D.; Chau, T.; Catalano, F.M.; Zingg, D.W. Exploration of box-wing aircraft concept using high-fidelity aerodynamic shape optimization. In Proceedings of the 33th Congress of the International Council of the Aeronautical Sciences, Stockholm, Sweden, 4–9 September 2022. [Google Scholar]
- Catalano, F. The new closed circuit wind tunnel of the Aircraft Laboratory of University of São Paulo, Brazil. In Proceedings of the 24th International Congress of the Aeronautical Sciencies ICAS, Yokohama, Japan, 29 August–3 September 2004. [Google Scholar]
- Santana, L.D.; Carmo, M.; Catalano, F.M.; Medeiros, M.A. The update of an aerodynamic wind-tunnel for aeroacoustics testing. J. Aerosp. Technol. Manag. 2014, 6, 111–118. [Google Scholar] [CrossRef]
- de Almeida, O.; Catalano, F.M.; Pereira, L.T. Improvements of a Hard-Wall Closed Test-Section of a Subsonic Wind Tunnel for Aeroacoustic Testing. Int. J. Acoust. Vib. 2021, 26, 248–258. [Google Scholar] [CrossRef]
- Bravo-Mosquera, P.D. Methodologies for Designing, Optimizing, and Evaluating Possible Unconventional Aircraft Configurations for Future Civil Aviation. Ph.D. Thesis, São Carlos School of Engineering, University of São Paulo, São Carlos, Brazil, 2022. [Google Scholar]
- Hantrais-Gervois, J.L.; Piat, J.F.; Hantrais, J.L. A Methodology to Derive Wind Tunnel Wall Corrections from RANS Simulations; Integration: London, ON, Canada, 2012. [Google Scholar]
- Barlow, J.B.; Rae, W.H.; Pope, A. Low-Speed Wind Tunnel Testing; John Wiley & Sons: New York, NY, USA, 1999. [Google Scholar]
- Cerón-Muñoz, H. Estudo da Interferência Aerodinâmica do Sistema Motopropulsor em uma Aeronave do Tipo Blended Wing Body. Ph.D. Thesis, São Carlos School of Engineering, University of São Paulo, São Carlos, Brazil, 2009. [Google Scholar]
- Ceron-Muñoz, H.; Catalano, F. Aerodynamic interference of power-plant system on a Blended Wing Body. In Proceedings of the 27th International Congress of the Aeronautical Sciences, Nice, France, 19–24 September 2010. [Google Scholar]
- Cerón-Muñoz, H.; Diaz-Izquierdo, D.; Bravo-Mosquera, P.; Catalano, F.; De Santana, L. Experimental analyses of droop, wingtips and fences on a BWB model. In Proceedings of the 30th Congress of the International Council of the Aeronautical Sciences (ICAS 2016), Daejeon, Republic of Korea, 25–30 September 2016; pp. 25–30. [Google Scholar]
- Uranga, A.; Drela, M.; Greitzer, E.; Titchener, N.; Lieu, M.; Siu, N.; Huang, A.; Gatlin, G.M.; Hannon, J. Preliminary experimental assessment of the boundary layer ingestion benefit for the D8 aircraft. In Proceedings of the 52nd Aerospace Sciences Meeting, New York, NY, USA, 14 January 2014; p. 0906. [Google Scholar]
- Aeroprobe L-Shaped Probe. 2018. Available online: https://www.aeroprobe.com (accessed on 23 March 2022).
- Scanivalve Corp. Scanivalve DS4-48—Pressure Scanner Module, Instruction and Service Manual; Scanivalve Corp: Liberty Lake, WA, USA, 1975. [Google Scholar]
- Frediani, A.; Cipolla, V.; Oliviero, F. IDINTOS: The first prototype of an amphibious PrandtlPlane-shaped aircraft. Aerotec. Missili Spaz. 2015, 94, 195–209. [Google Scholar] [CrossRef]









| Freestream | Dynamic | Mach | Reynolds |
|---|---|---|---|
| Velocity [m/s] | Pressure [Pa] | Number [-] | Number [-] |
| 27.0 | 410.9 | 0.080 | |
| 30.0 | 507.3 | 0.089 |
| [RPM] | 9000 | 10,300 | 15,500 | 18,200 | 20,400 | |
|---|---|---|---|---|---|---|
| [-] at | 1.33 | 1.44 | 2.17 | 2.55 | 2.86 | |
| 1.20 | 1.30 | 1.96 | 2.30 | 2.58 |
| Configuration | Unpowered | Non-BLI | BLI | ||||
|---|---|---|---|---|---|---|---|
| Parameter | Exp | CFD | % Error | Exp | [%] | Exp | [%] |
| for zero [-] | 0.191 | 5.52 | |||||
| [-] | 0.111 | 8.82 | 0.0 | 0.0 | |||
| [-] | 10.86 | ||||||
| for zero [-] | 0.0245 | 19.40 | |||||
| [-] | 0.018 | 14.28 | |||||
| [-] | 28.12 | ||||||
| Tunnel Condition | Configuration | Curve Fits and Confidence Interval |
|---|---|---|
| non-BLI | ; | |
| non-BLI | ; | |
| BLI | ; | |
| BLI | ; |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bravo-Mosquera, P.D.; Cerón-Muñoz, H.D.; Catalano, F.M. Potential Propulsive and Aerodynamic Benefits of a New Aircraft Concept: A Low-Speed Experimental Study. Aerospace 2023, 10, 651. https://doi.org/10.3390/aerospace10070651
Bravo-Mosquera PD, Cerón-Muñoz HD, Catalano FM. Potential Propulsive and Aerodynamic Benefits of a New Aircraft Concept: A Low-Speed Experimental Study. Aerospace. 2023; 10(7):651. https://doi.org/10.3390/aerospace10070651
Chicago/Turabian StyleBravo-Mosquera, Pedro D., Hernán D. Cerón-Muñoz, and Fernando M. Catalano. 2023. "Potential Propulsive and Aerodynamic Benefits of a New Aircraft Concept: A Low-Speed Experimental Study" Aerospace 10, no. 7: 651. https://doi.org/10.3390/aerospace10070651
APA StyleBravo-Mosquera, P. D., Cerón-Muñoz, H. D., & Catalano, F. M. (2023). Potential Propulsive and Aerodynamic Benefits of a New Aircraft Concept: A Low-Speed Experimental Study. Aerospace, 10(7), 651. https://doi.org/10.3390/aerospace10070651







