Mode Switching in a Compressible Rectangular Cavity Flow
Abstract
1. Introduction
2. Experimental Setup
2.1. Transonic Wind Tunnel
2.2. Test Model
2.3. Instrumentation and Data Acquisition System
2.4. Mean and Fluctuating Pressure Coefficients
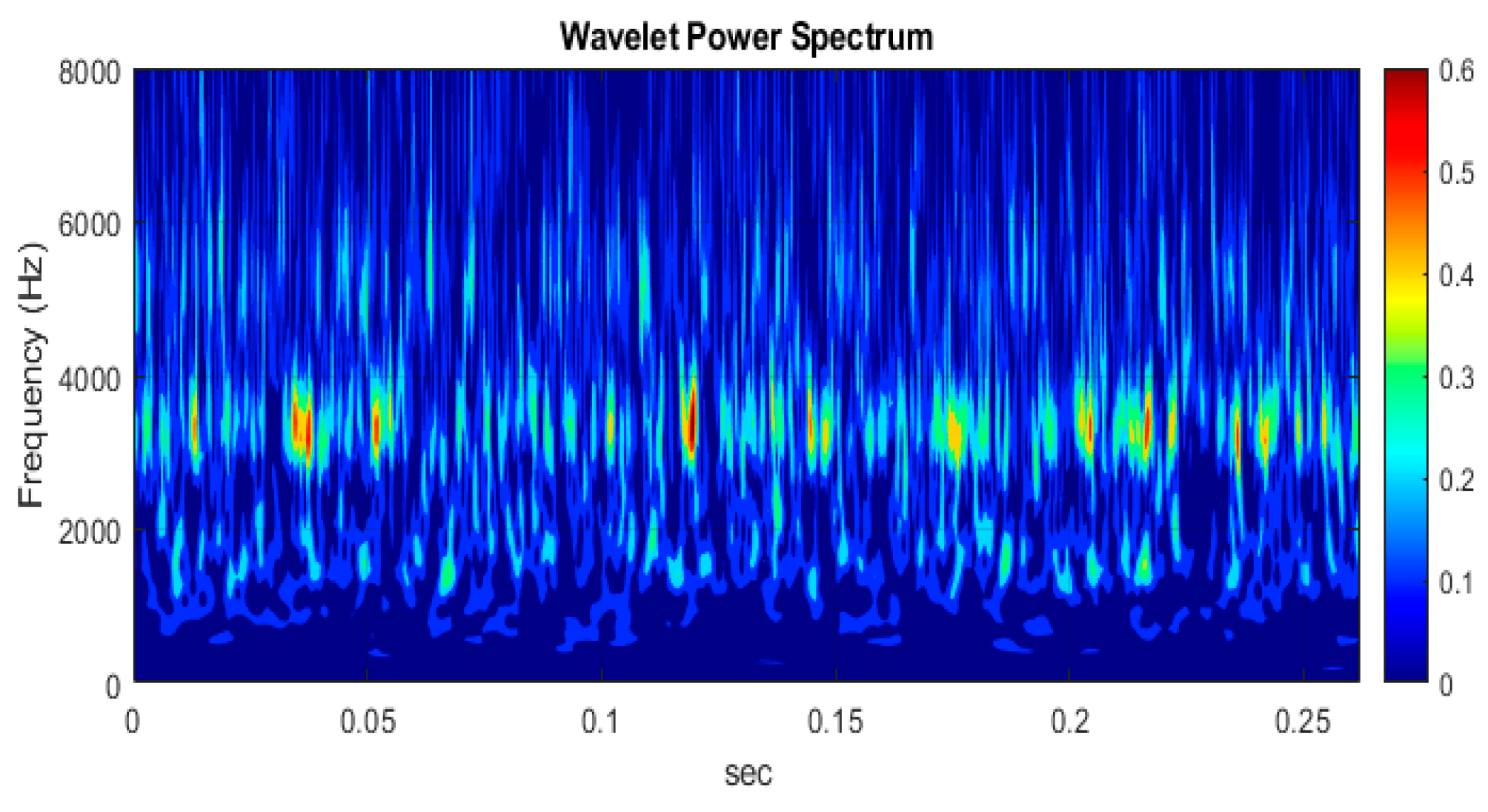
2.5. Spectral Analysis
2.6. Amplitude Demodulation Technique
3. Results and Discussion
3.1. Mean and Fluctuating Pressure
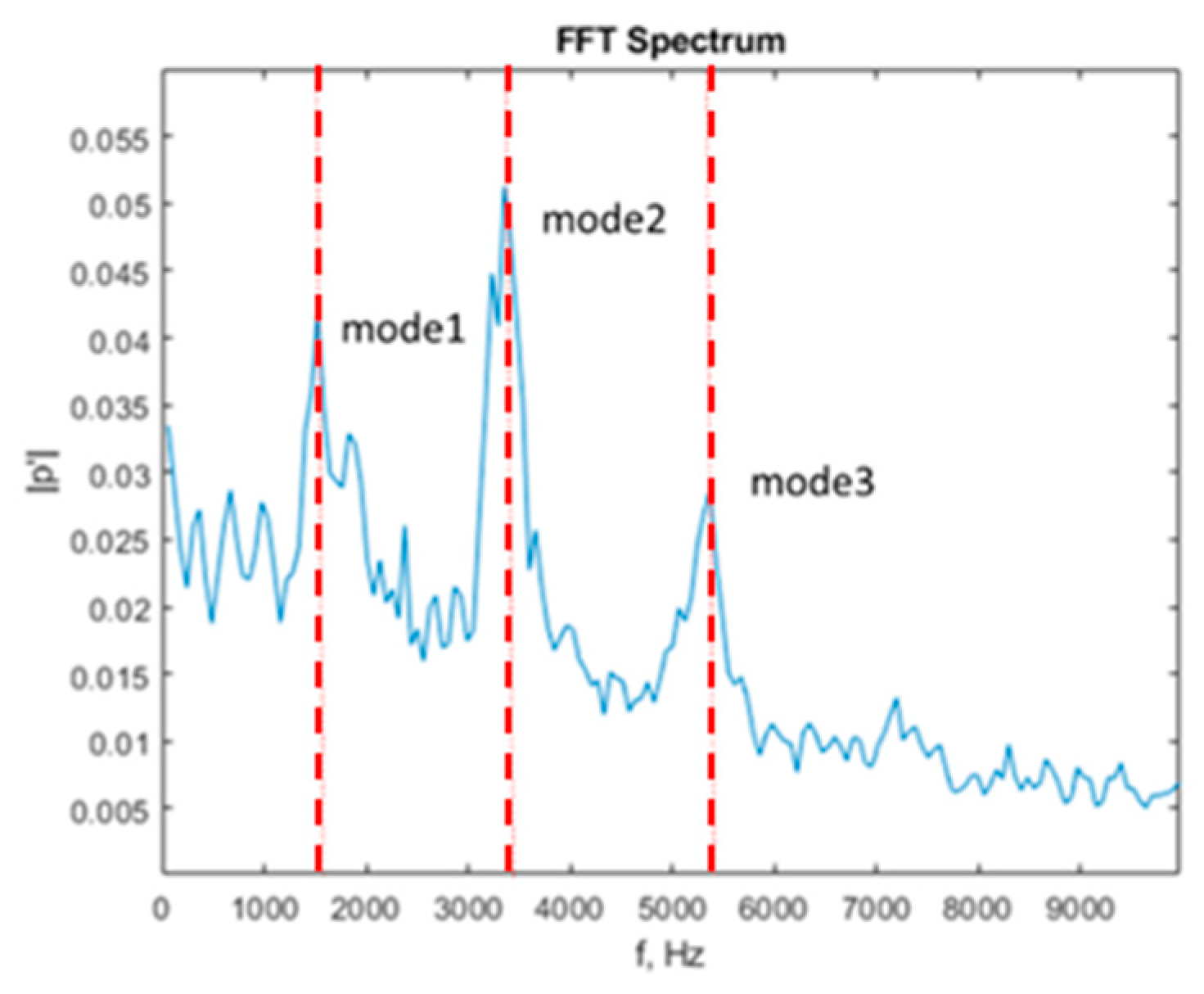
3.2. Spectral Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Cp | mean surface pressure coefficient |
| fluctuating pressure coefficient | |
| peak fluctuating pressure coefficient | |
| fi, f2, f3 | frequency of Rossiter–Heller (R–H) modes |
| h | cavity depth |
| l | cavity length |
| M | freestream Mach number |
| , pw | mean surface pressure |
| po | stagnation pressure |
| p∞ | freestream static pressure |
| pressure signal | |
| q | dynamic pressure |
| R1, R2, R3 | Rossiter–Heller modes |
| St | Strouhal number |
| U∞ | freestream velocity |
| w | cavity width |
| x | coordinate along the centerline of model surface |
| x* | normalized streamwise distance, x/δ |
| δ | incoming boundary-layer thickness |
| γ, γij | Spearman’s correlation coefficient |
| σp | fluctuating (rms) pressure |
References
- Stallings, R.L., Jr.; Wilcox, F.J., Jr. Experimental Cavity Pressure Distributions at Supersonic Speeds; NASA TP-2683; NASA: Washington, DC, USA, 1987. [Google Scholar]
- Mathias, M.S.; Medeiros, M.A.F. The effect of incoming boundary layer thickness and Mach number on linear and nonlinear Rossiter modes in open cavity flows. Theo. Comput. Fluid Dyn. 2021, 35, 495–513. [Google Scholar] [CrossRef]
- Doshi, P.S.; Ranjan, R.; Gaitonde, D.V. Global and local modal characteristics of supersonic open cavity flows. Phys. Fluids 2022, 34, 034104. [Google Scholar] [CrossRef]
- Kim, I.; Chokani, N. Navier-Stokes study of supersonic cavity flow field with passive control. J. Aircr. 1992, 29, 217–223. [Google Scholar] [CrossRef]
- Nair, K.M.; Sarkar, S. Large eddy simulation of self-sustained cavity oscillation for subsonic and supersonic flows. J. Fluids Eng. 2017, 139, 011102. [Google Scholar] [CrossRef]
- Chung, K.M. Three-dimensional effect on transonic rectangular cavity flows. Exp. Fluids 2001, 30, 531–536. [Google Scholar] [CrossRef]
- Turpin, A.M.; Speth, R.L.; Sherer, S.E.; Granlund, K.O. Low-frequency, spanwise oscillation in a finite-width cavity at Mach 1.5. Phys. Fluids 2021, 33, 076102. [Google Scholar] [CrossRef]
- Yamouni, S.; Sipp, D.; Jacquin, L. Interaction between feedback aeroacoustic and acoustic resonance mechanisms in a cavity flow: A global stability analysis. J. Fluid. Mech. 2013, 717, 134–165. [Google Scholar] [CrossRef]
- Maceda, G.Y.C.; Varon, E.; Lusseyran, F.; Noack, B.R. Stabilization of a multi-frequency open cavity flow with gradient-enriched machine learning control. J. Fluid Mech. 2023, 955, A20. [Google Scholar] [CrossRef]
- Bacci, D.; Saddington, A.J.; Bray, D. Identification of the formation of resonant tones in compressible cavity flows. Aerosp. Sci. Technol. 2018, 77, 320–331. [Google Scholar] [CrossRef]
- Rossiter, J. Wind Tunnel Experiments on the Flow over Rectangular Cavities at Subsonic and Transonic Speeds; Technical Report 64037; Royal Aircraft Establishment: Farnborough, UK, 1964. [Google Scholar]
- Heller, H.; Bliss, D. Aerodynamically Induced Pressure Oscillations in Cavities Physical Mechanisms and Suppression Concepts; Technical Report AFFDL-TR-74-133; Air Force Flight Dynamics Laboratory: Dayton, OH, USA, 1975. [Google Scholar]
- Malone, J.; Debiasi, M.; Little, J.; Samimy, M. Analysis of the spectral relationships of cavity tones in subsonic resonant cavity flows. Phys. Fluids 2009, 21, 055103. [Google Scholar] [CrossRef]
- Heller, H.; Holmes, D.G.; Covert, E.E. Flow-induced pressure oscillations in shallow cavities. J. Sound Vib. 1971, 18, 545–546. [Google Scholar] [CrossRef]
- Rowley, C.W.; Williams, D.R. Dynamics and control of high-Reynolds-number flow over open cavities. Annu. Rev. Fluid Mech. 2006, 38, 251–276. [Google Scholar] [CrossRef]
- Li, W.; Nonomura, T.; Fujii, K. On the feedback mechanism in supersonic cavity flow. Phys. Fluids 2013, 25, 056101. [Google Scholar] [CrossRef]
- Nilsson, S.; Yao, H.-D.; Karlsson, A.; Arvidson, S. Effects of aeroelastic walls on the aeroacoustics in transonic cavity flow. Aerospace 2022, 9, 716. [Google Scholar] [CrossRef]
- Wagner, J.L.; Beresh, S.J.; Casper, K.M.; DeMauro, E.P.; Arunajatesan, S. Resonance dynamics in compressible cavity flows using time-resolved velocity and surface pressure fields. J. Fluid Mech. 2017, 830, 494–527. [Google Scholar] [CrossRef]
- Kegerisea, M.A.; Spina, E.F.; Garg, S.; Cattafesta, L.N., III. Mode-switching and nonlinear effects in compressible flow over a cavity. Phys. Fluids 2004, 16, 678–687. [Google Scholar] [CrossRef]
- Pandian, S.; Desikan, S.L.N.; Niranjan, S. Nonlinear Characteristics of a Rectangular Cavity in Supersonic Flow. AIAA J. 2020, 58, 1206–1215. [Google Scholar] [CrossRef]
- Gloerfelt, X.; Bogey, C.; Bailly, C. Numerical evidence of mode switching in the flow-induced oscillations by a cavity. Aeroacoustics 2003, 2, 193–218. [Google Scholar] [CrossRef]
- Chung, K.M. Investigation on transonic convex-corner flows. J. Aircr. 2002, 39, 1014–1018. [Google Scholar] [CrossRef]
- Chung, K.M. Unsteadiness of transonic convex-corner flows. Exp. Fluids 2004, 37, 917–922. [Google Scholar] [CrossRef]
- Corcos, G.M. Resolution of pressure in turbulence. J. Acous. Soc. Am. 1963, 35, 192–199. [Google Scholar] [CrossRef]
- Seena, A.; Sung, H.J. Wavelet spatial scaling for educing dynamic structures in turbulent open cavity flows. J. Fluids Structures 2011, 27, 962–975. [Google Scholar] [CrossRef]
- Torrence, C.; Gilbert, P.; Compo, G.P. A practical guide to wavelet analysis. Bul. Am. Meteorol. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef]
- Haji, M.R.; Miksad, R.W.; Powers, E.J. Perspective: Measurements and analyses of nonlinear wave interactions with higher-order spectral moments. J. Fluids Eng. 1998, 119, 2–13. [Google Scholar] [CrossRef]
- Liu, Y. A Short Note on Spearman Correlation: Impact of Tied Observations; SSRN Tech. Report; GRIN Verlag: Munich, Germany, 2017. [Google Scholar] [CrossRef]
- Zhang, X.; Edwards, J.A. An investigation of supersonic cavity flows driven by thick shear layers. Aero. J. 1990, 1670, 355–364. [Google Scholar] [CrossRef]
- Beresh, S.J.; Wagner, J.L.; Henfling, J.F.; Spillers, R.W.; Pruett, B.O.M. Width effects in transonic flow over a rectangular cavity. AIAA J. 2015, 53, 3831–3834. [Google Scholar] [CrossRef]


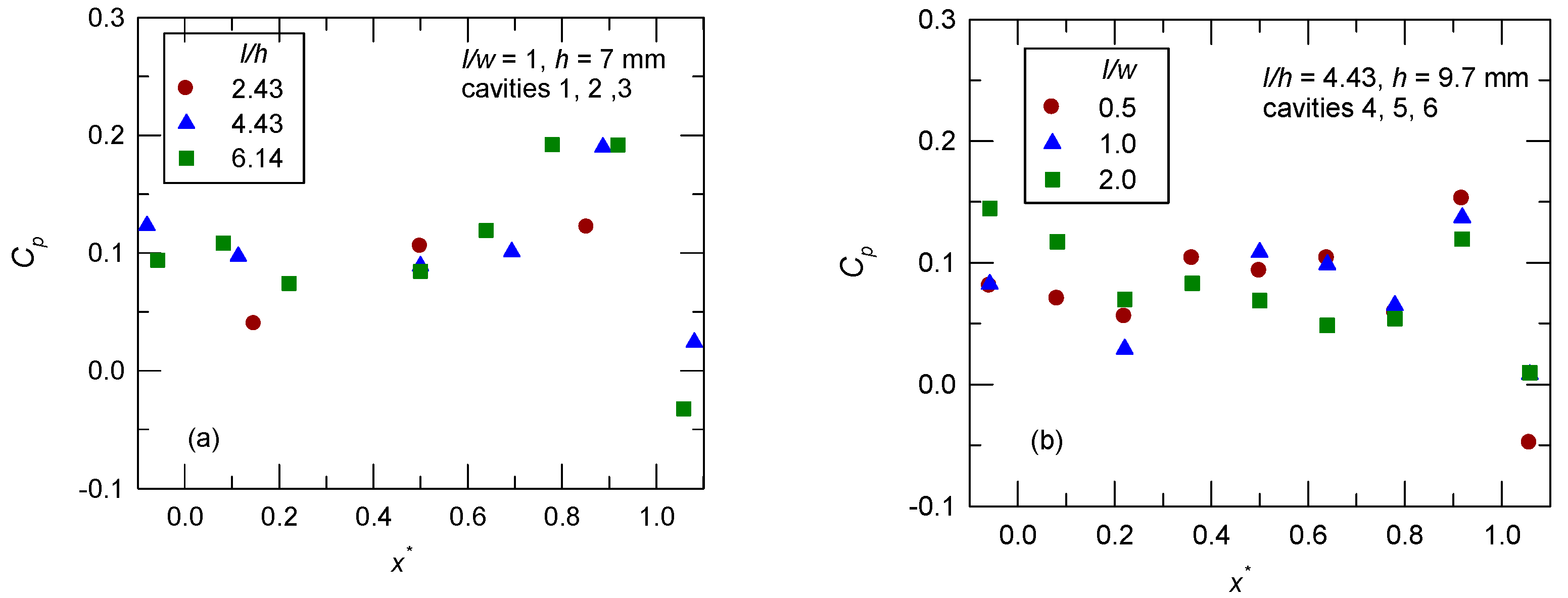
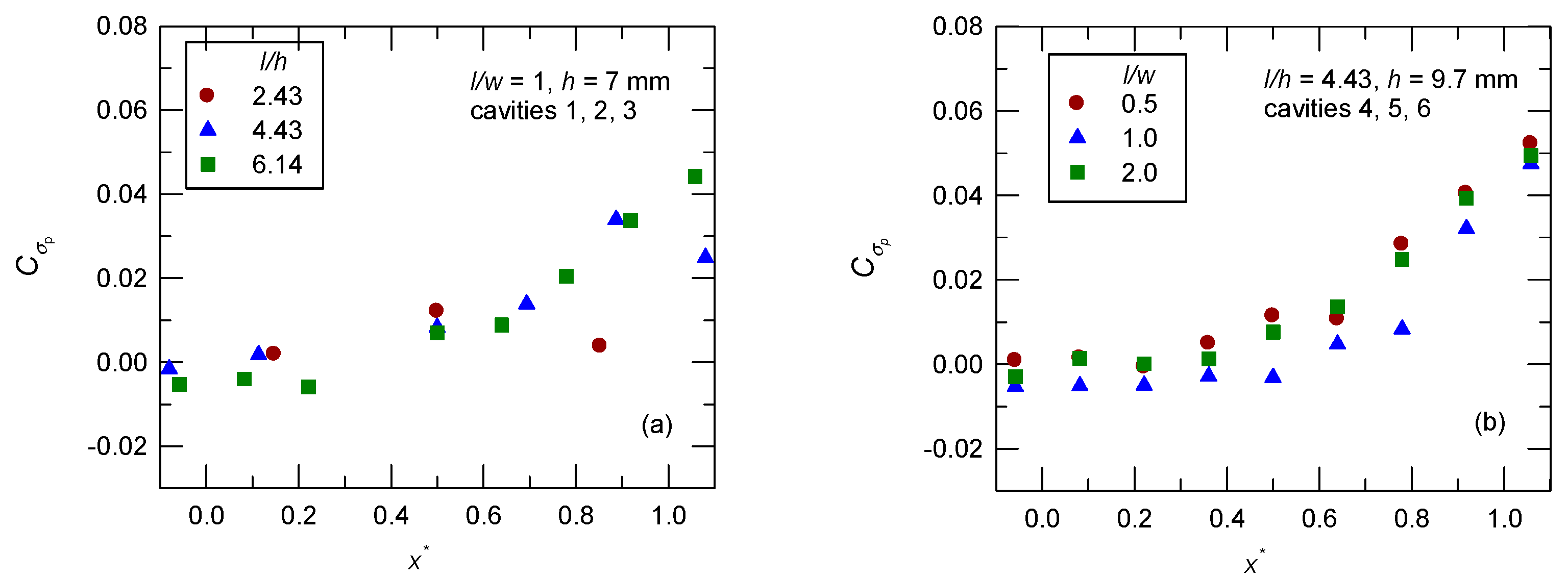
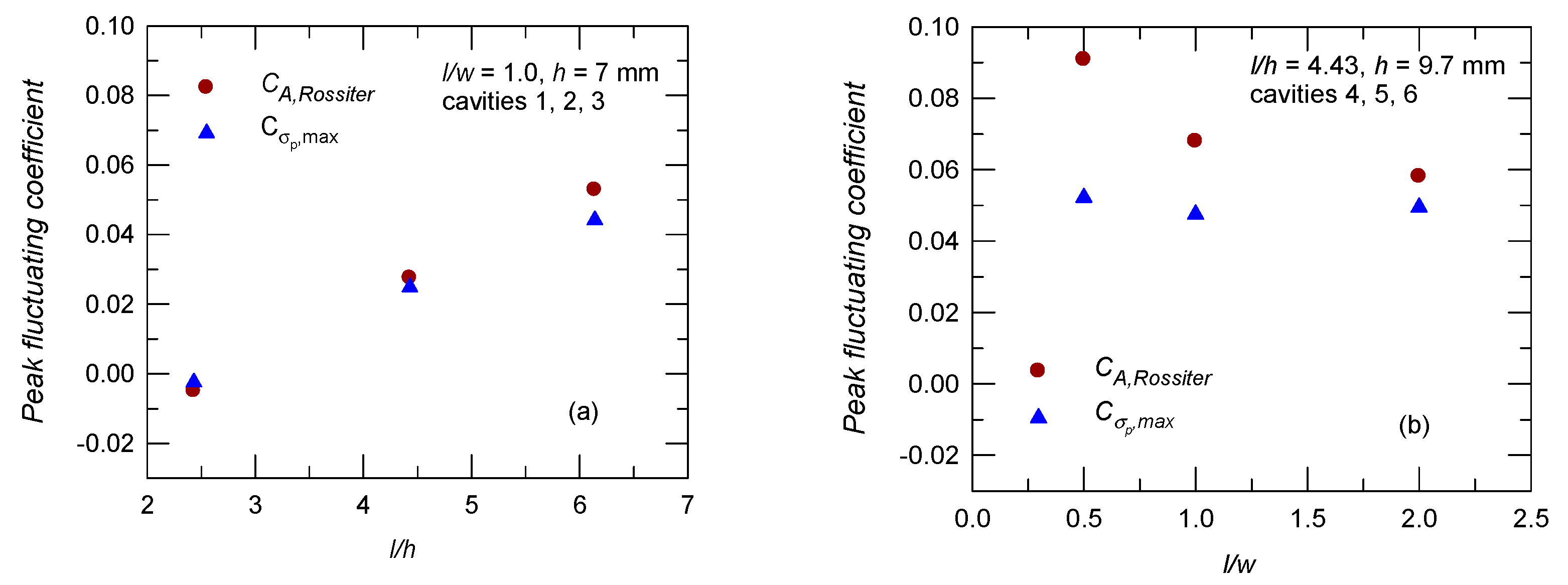

| Cavity | l | h | w | l/h | l/w |
|---|---|---|---|---|---|
| 1 | 17 | 7 | 17 | 2.43 | 1 |
| 2 | 31 | 7 | 31 | 4.43 | 1 |
| 3 | 43 | 7 | 43 | 6.14 | 1 |
| 4 | 43 | 9.7 | 86 | 4.43 | 0.5 |
| 5 | 43 | 9.7 | 43 | 4.43 | 1 |
| 6 | 43 | 9.7 | 21.5 | 4.43 | 2 |
| (a) | |||
|---|---|---|---|
| Cavity | f1, Hz | f2, Hz | f3, Hz |
| 1 | 3864 | 8983 | 14,116 |
| 2, 4–6 | 2119 | 4926 | 7741 |
| 3 | 1528 | 3551 | 5580 |
| (b) | |||
| Cavity | f1, Hz | f2, Hz | f3, Hz |
| 1 | 4339 | 8679 | 12,902 |
| 2 | 2319 | 4639 | 7446 |
| 3 | 1495 | 3326 | 5310 |
| 4 | 1635 | 3540 | 5480 |
| 5 | 1513 | 3446 | 5419 |
| 6 | 1757 | 3564 | 5297 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Y.-X.; Chung, K.-M. Mode Switching in a Compressible Rectangular Cavity Flow. Aerospace 2023, 10, 504. https://doi.org/10.3390/aerospace10060504
Huang Y-X, Chung K-M. Mode Switching in a Compressible Rectangular Cavity Flow. Aerospace. 2023; 10(6):504. https://doi.org/10.3390/aerospace10060504
Chicago/Turabian StyleHuang, Yi-Xuan, and Kung-Ming Chung. 2023. "Mode Switching in a Compressible Rectangular Cavity Flow" Aerospace 10, no. 6: 504. https://doi.org/10.3390/aerospace10060504
APA StyleHuang, Y.-X., & Chung, K.-M. (2023). Mode Switching in a Compressible Rectangular Cavity Flow. Aerospace, 10(6), 504. https://doi.org/10.3390/aerospace10060504






