High Enthalpy Non-Equilibrium Expansion Effects in Turbulent Flow of the Conical Nozzle
Abstract
1. Introduction
2. Numerical Methods
2.1. Governing Equations
2.2. Non-Equilibrium Model
2.3. Physical Model and Grid Generation
2.4. Conditions of Numerical Simulation
3. Verification of Calculation Procedure
3.1. Precision Estimates
3.2. Verification of the Calculation Method
4. Results and Discussion
4.1. Freezing Point Position
- As the MEA increases, the pressure gradient near the throat increases (see Figure 4), resulting in an increase in flow speed, as illustrated in Figure 5. As the pressure gradient increases, the pressure downstream of the throat decreases, which increases the TVERT, as deduced from Equation (25). Additionally, the increase in pressure gradient causes an increase in the flow velocity but a decrease in the flow time. Both shorten the transition from equilibrium to freezing, and the flow becomes close to the non-equilibrium flow.
- The pressure p decreases with the increase in the MEA. According to the ideal gas law p = nkT, the translational temperature is directly related to the pressure p, and the vibrational degree of freedom has no direct influence on the pressure. Therefore, the influence of the non-equilibrium on the pressure distribution is consistent with the trend of the influence on the translational temperature T.
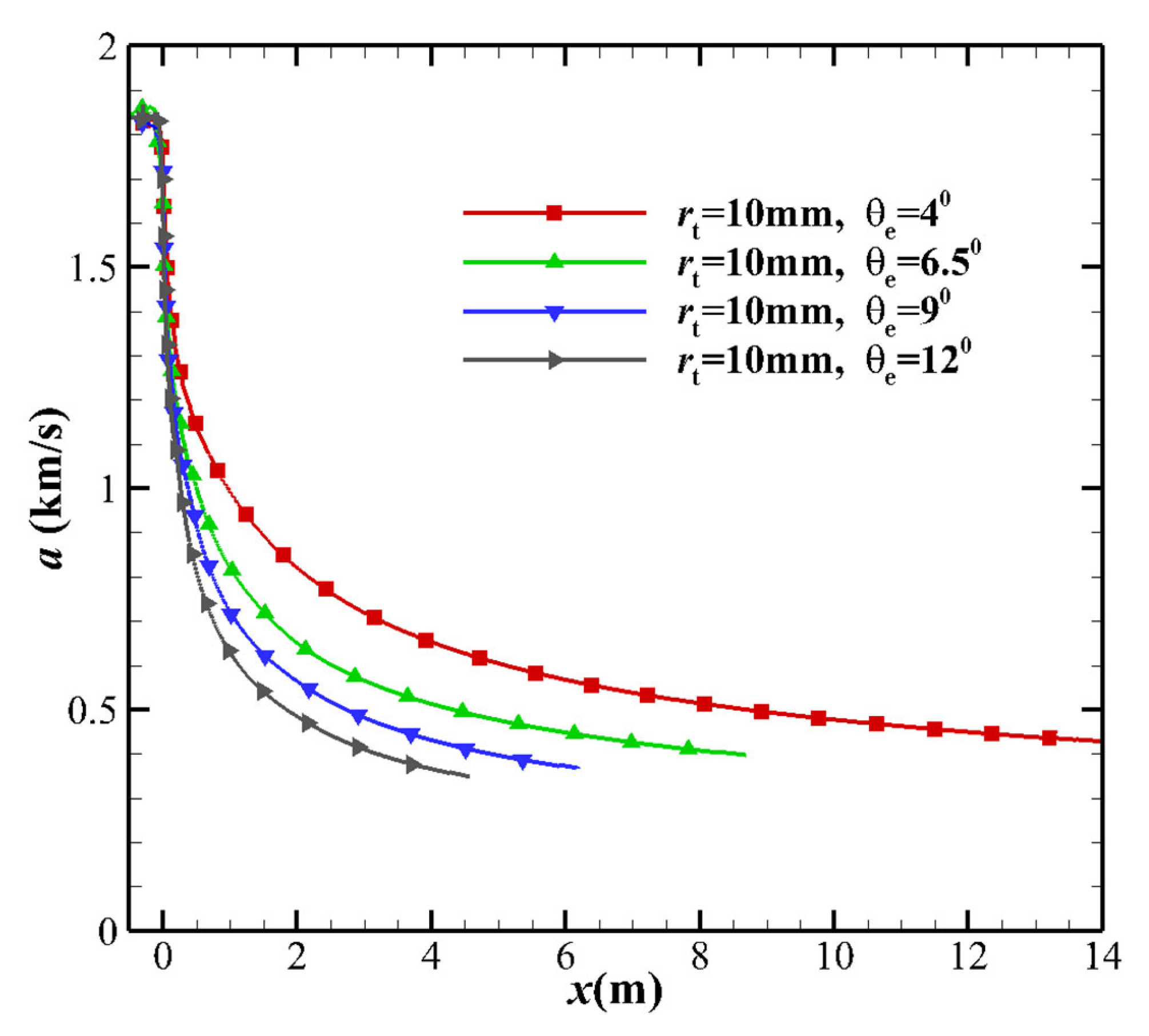
- Not far from the downstream of the nozzle throat, the larger MEAθe corresponds to a slightly larger vibrational temperature, resulting in a higher vibrational energy level. The vibrational model modifies the translational temperature, as deduced from the kinetic energy in Equation (16). Due to the freezing of vibrational energy, the increase in the kinetic energy of the airflow in the nozzle mainly originates from the translational energy, which leads to a decrease in the translational temperature T. Since the vibration energy is frozen, it can also be determined that the contribution of vibrational energy to the speed is extremely small, which means that the non-equilibrium effect has almost no effect on the velocity u of the nozzle outlet. The velocity u is almost the same, which is consistent with h ≈ u2/2, and the velocity u is only related to the total enthalpy h of the nozzle stagnation gas.
- As the TR increases, the pressure gradient near the throat decreases (see Figure 7), which raises the pressure downstream of the throat, causing a decrease in the TVERT, as deduced from Equation (26). Additionally, as the TR increases, the velocity near the throat decreases and the flow time increases. The overall effect is that the freezing point is far from the throat, and the flow state is close to the equilibrium state.
- For the equilibrium flow, the flow parameters on any cross-section are determined only by the nozzle stagnation conditions and the local area ratio. The throat at x = 0 mm and the throat upstream exhibit equilibrium flow, and the translational temperature and the vibrational temperature are not separated, as shown in Figure 7.
- The increase in the kinetic energy of the equilibrium flow originates from the translational kinetic energy and vibrational energy. Since the vibrational energy of the non-equilibrium flow freezes, the increase in the kinetic energy can only be attributed to the translational energy. As the TR increases, the velocity u at the throat decreases. The velocity u of the nozzle outlet is only related to the total enthalpy h of the nozzle stagnation gas, and the nozzle outlet velocity is almost the same (see Figure 8).
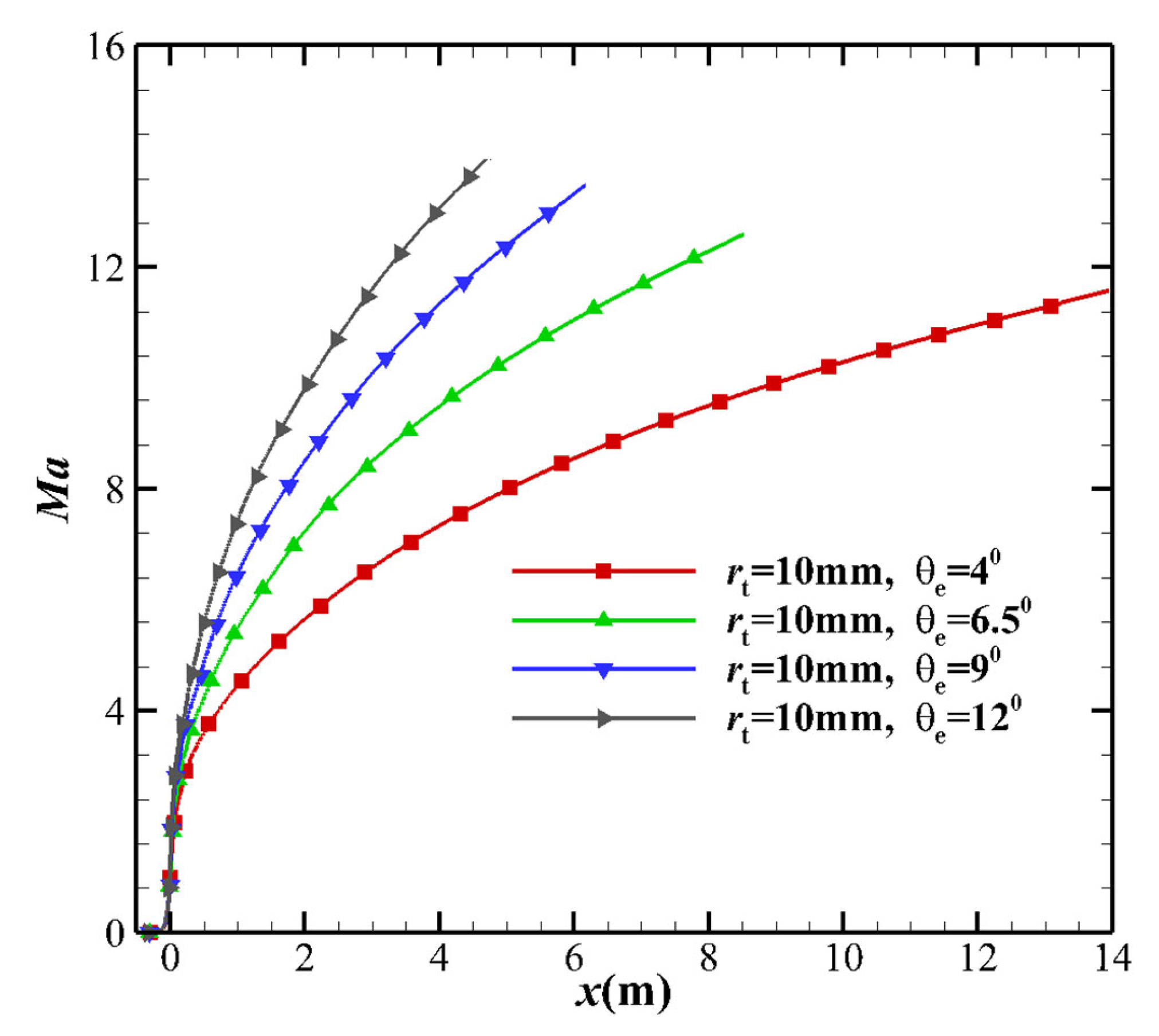
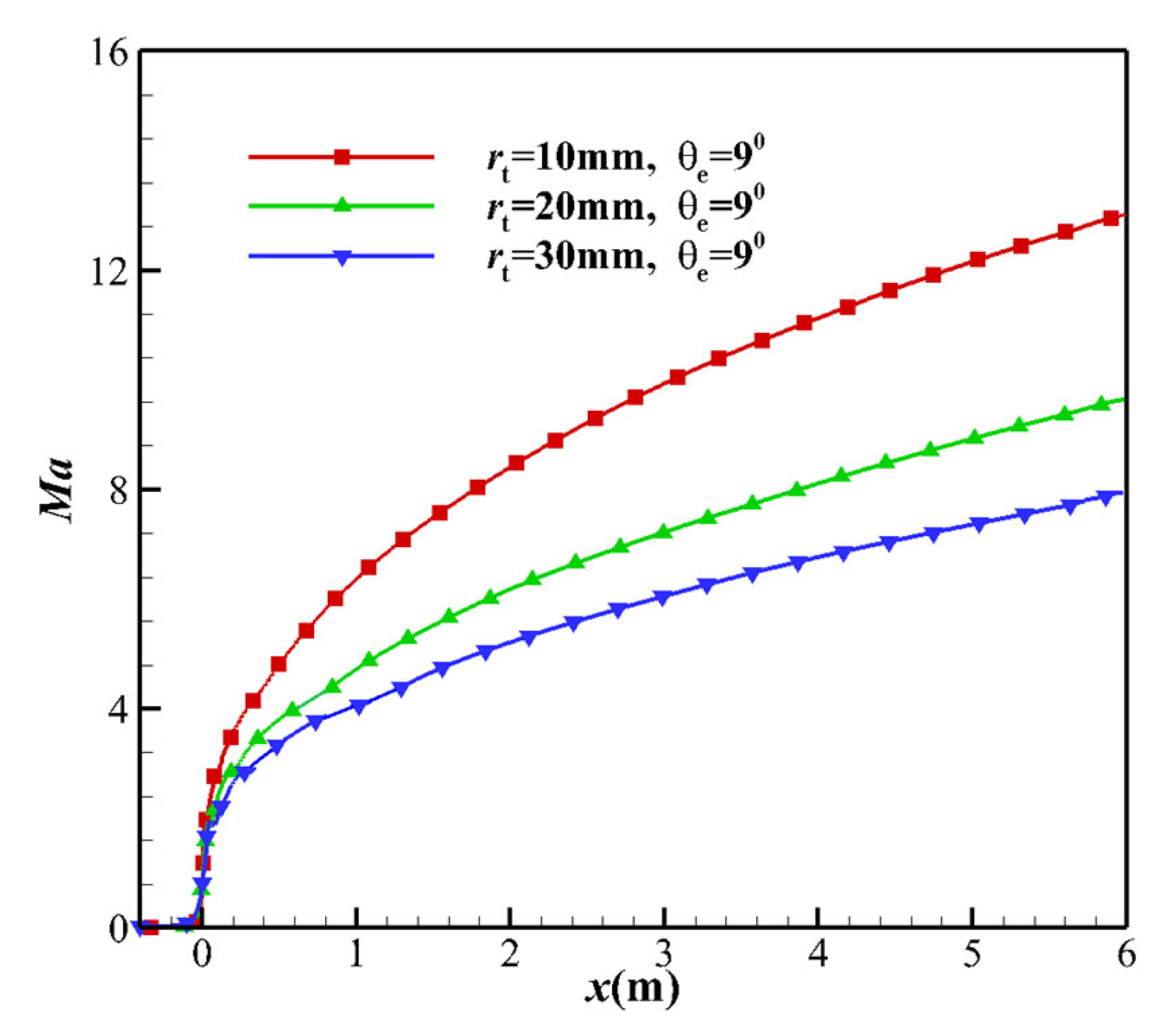
4.2. Mach Number
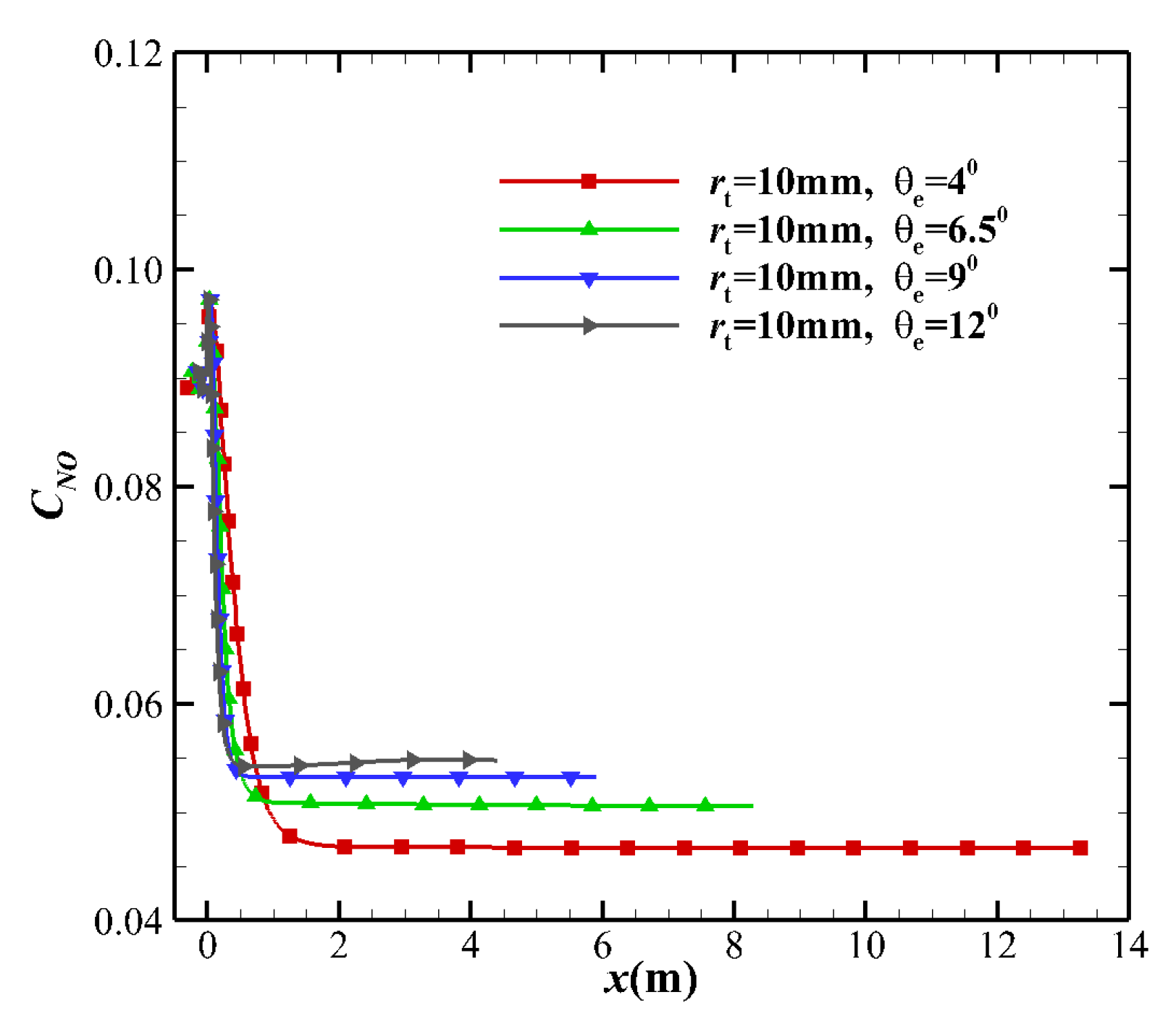
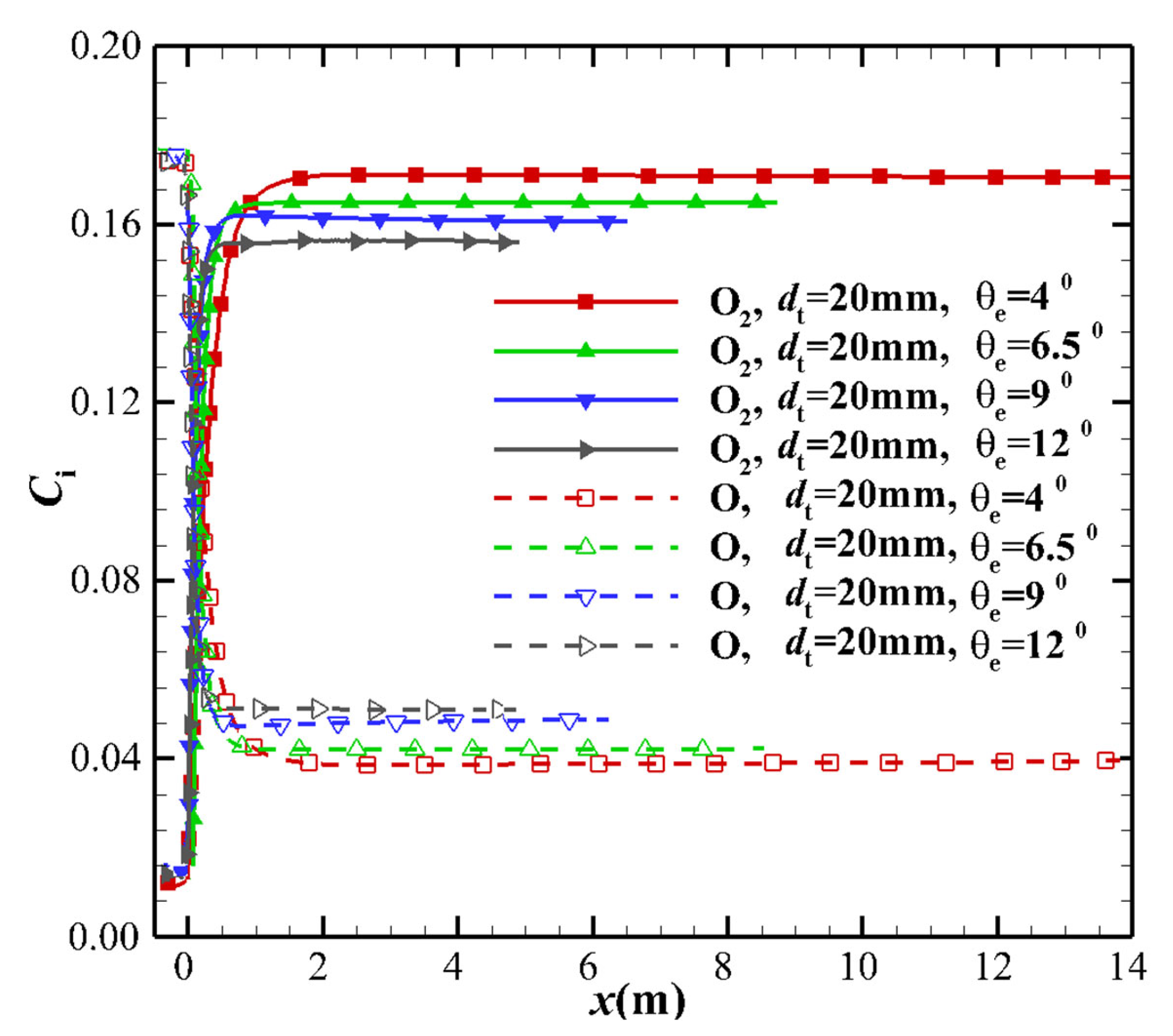
4.3. Species Mass Fraction
5. Conclusions
- As the maximum expansion angle decreases or the throat radius increases, the pressure gradient near the throat increases. The pressure downstream of the throat decreases, which increases the translational-vibrational energy relaxation time. Additionally, the increase in pressure gradient causes an increase in the flow velocity but a decrease in the flow time. The overall effect is that the freezing point is far from the throat and the flow state is close to the equilibrium state.
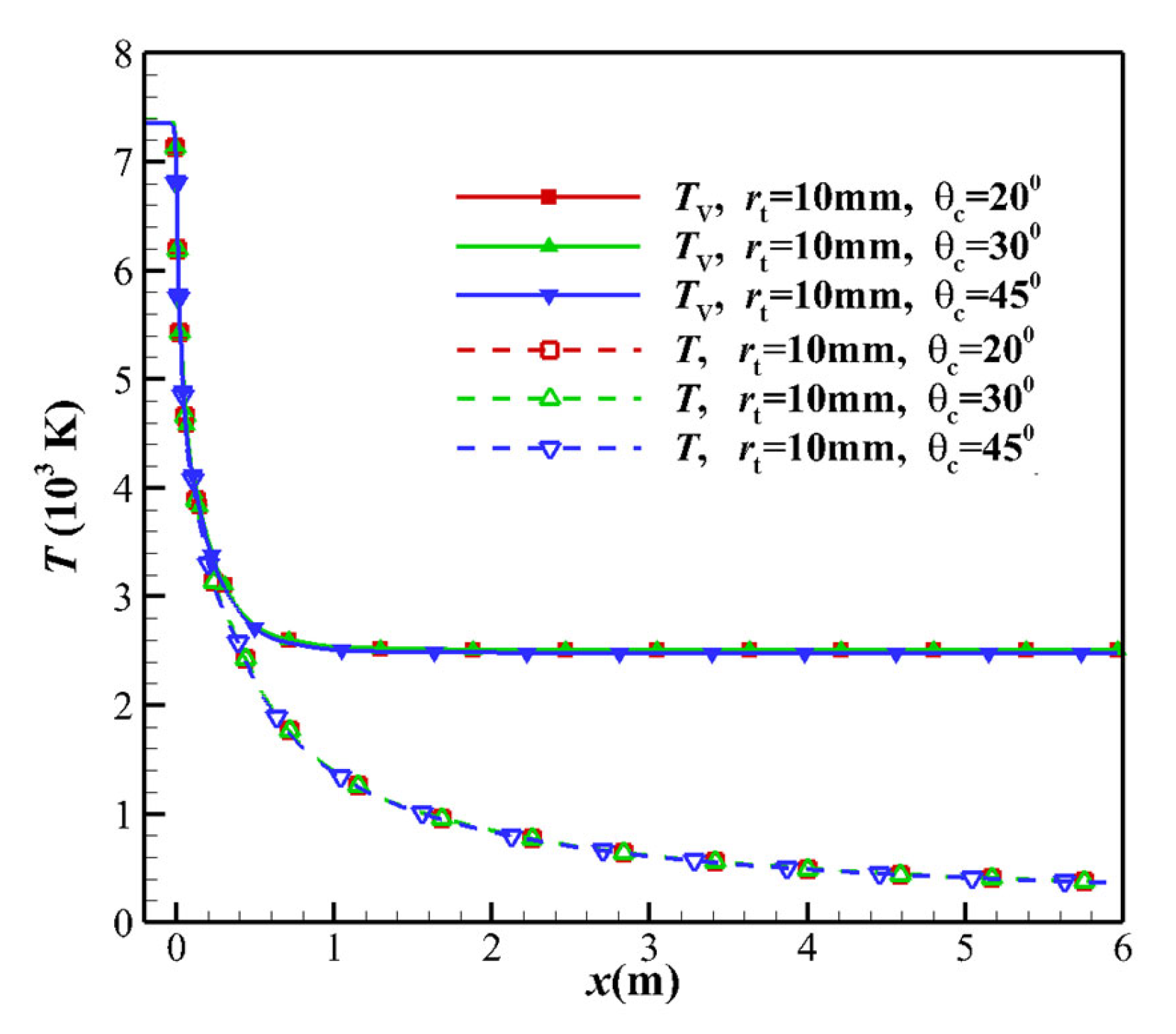
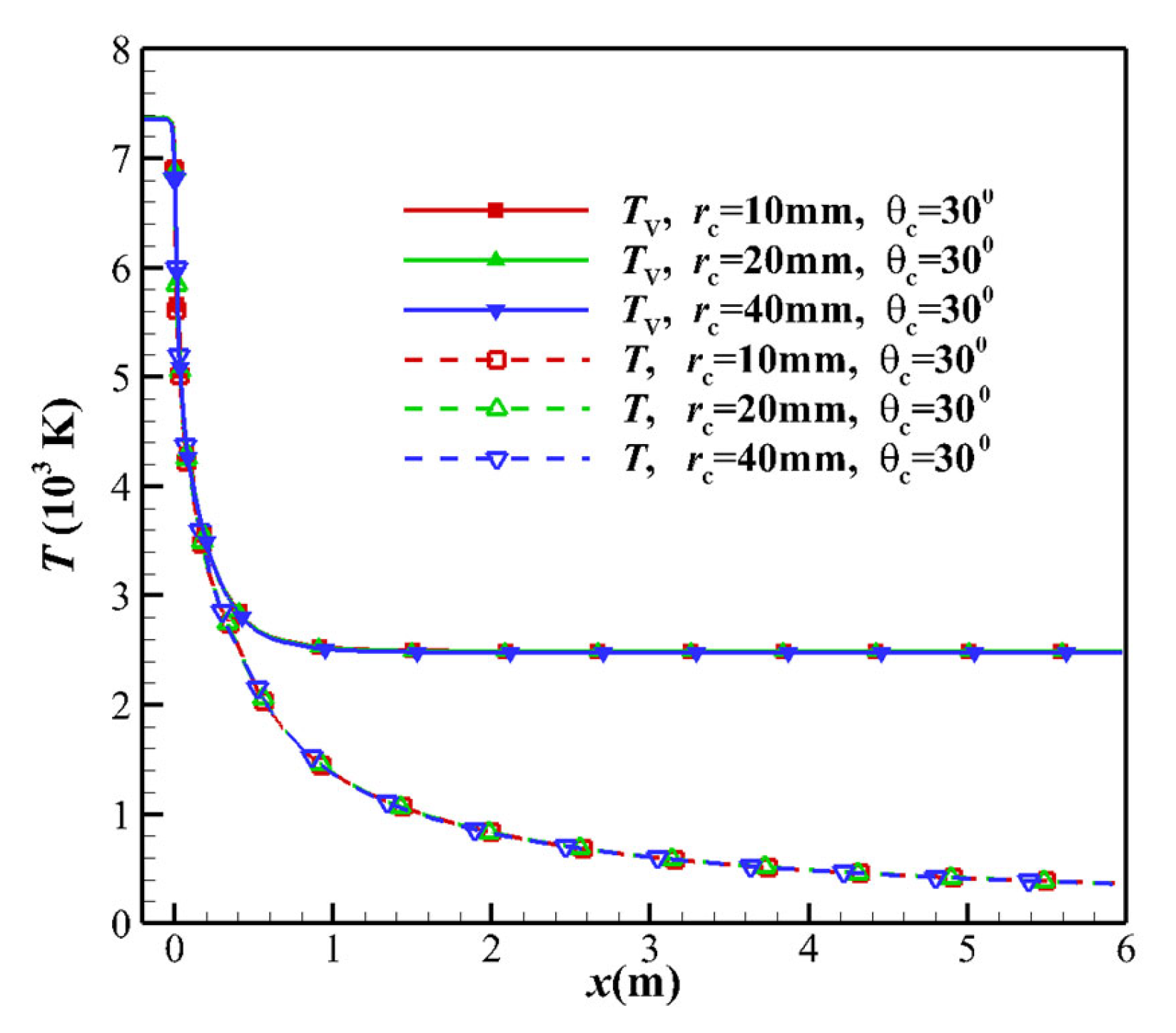
- In the region upstream of the nozzle throat, the flow is the equilibrium state. The variation of nozzle geometry in the equilibrium flow area has almost no impact on the non-equilibrium effect of the flow field, including throat curvature radius and contraction Angle.
- The thermochemical non-equilibrium expansion effects of the conical nozzle change the species mass fraction, temperature, and Mach number. When the thermochemical nonequilibrium effect is suppressed, more vibrational energy is transmitted to the translational energy, which increases the translational temperature. Under the condition that the exit velocity is basically constant, the Mach number at the nozzle exit decreases. The freezing temperature at the nozzle outlet decreases while the mass fraction of O2 and N2 species increase and the mass fraction of other species such as NO, N, and O decrease.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| 5S2T | 5-species 2-temperature model |
| 7S2T | 7-species 2-temperature model |
| CA | the convergence angle |
| ER | expansion ratio, defined as the ratio of the exit area to the throat area |
| MEA | maximum expansion angle |
| TCR | throat curvature radius |
| TR | throat radius |
| ns | the number of species, which is equal to 5 or 7 |
| nt | the number of species that remove electrons from ns |
References
- Deutsches Zentrum fur Luft-und Raumfahrt (DLR). The high enthalpy shock tunnel Gottingen of the German Aerospace Center (DLR). J. Large-Scale Res. Facil. 2018, 4, A133. [Google Scholar] [CrossRef]
- Anderson, J.D. Hypersonic and High Temperature Gas Dynamics, 2nd ed.; AIAA: Orlando, FL, USA, 2006. [Google Scholar]
- Shang, J.J.S.; Yan, H. High-enthalpy hypersonic flows. Adv. Aerodyn. 2020, 2, 19. [Google Scholar] [CrossRef]
- Jiang, H.; Liu, J.; Luo, S.; Huang, W.; Wang, J.; Liu, M. Thermochemical non-equilibrium effects on hypersonic shock wave/turbulent boundary-layer interaction. Acta Astronaut. 2022, 192, 1–14. [Google Scholar] [CrossRef]
- Gu, S.; Olivier, H. Capabilities and limitations of existing hypersonic facilities. Prog. Aerosp. Sci. 2020, 113, 100607. [Google Scholar] [CrossRef]
- Hannemann, K.; Itoh, K.; Mee, D.J.; Hornung, H.G. Free piston shock tunnels HEG, HIEST, T4 and T5. Exp. Methods Shock. Wave Res. 2016, 9, 181–264. [Google Scholar]
- Shen, J.; Lu, H.; Li, R.; Chen, X.; Ma, H. The thermochemical non-equilibrium scale effects of the high enthalpy nozzle. Adv. Aerodyn. 2020, 2, 20. [Google Scholar] [CrossRef]
- Marineau, E.C.; Hornung, H.G. High-enthalpy nonequilibrium nozzle flow: Experiments and computations. In Proceedings of the 39th AIAA Fluid Dynamics Conference and Exhibit, San Antonio, TX, USA, 22–25 June 2009. [Google Scholar]
- Wilson, Y.K.; Craddock, S.C.; Doherty, L.J. Aerodynamic design of nozzles with uniform outflow for hypervelocity ground-test facilities. J. Propuls. Power 2018, 34, 1467–1478. [Google Scholar]
- Passiatore, D.; Sciacovelli, L.; Cinnella, P.; Pascazio, G. Thermochemical non-equilibrium effects in turbulent hypersonic boundary layers. J. Fluid Mech. 2022, 941, A21. [Google Scholar] [CrossRef]
- Clarke, J.; Collen, P.L.; McGilvray, M.; di Mare, L. Numerical simulation of a shock tube in thermochemical non-equilibrium. In Proceedings of the AIAA SCITECH 2023 Forum, National Harbor, MD, USA, 23–27 January 2023. [Google Scholar]
- Mee, D.J.; Morgan, R.G.; Paull, A.; Jacobs, P.A.; Smart, M.K. The T4 Stalker Tube. In 30th International Symposium on Shock Waves 2: ISSW30-Volume 2; Springer International Publishing: Cham, Switzerland, 2017; pp. 1425–1429. [Google Scholar]
- Marineau, E.C.; Hornung, H.G. Heat flux Calibration of T5 hypervelocity shock Tunnel conical nozzle in air. In Proceedings of the 47th AIAA Aerospace Sciences Meeting Including The New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 5–8 January 2009. [Google Scholar]
- Nagayama, T.; Nagai, H.; Tanno, H.; Komuro, T. Global heat flux measurement using temperature-sensitive paint in high-enthalpy shock tunnel HIEST. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, Grapevine, TX, USA, 9–13 January 2017; p. 1682. [Google Scholar]
- Hannemann, K. High enthalpy flows in the HEG shock tunnel: Experiment and numerical rebuilding. In Proceedings of the 41st Aerospace Sciences Meeting and Exhibit 2003, Reno, Nevada, 6–9 January 2003. [Google Scholar]
- Holden, M.S.; Wadhams, T.P.; MacLean, M. Experimental studies in LENS I and X to evaluate real gas effects on hypevelocity vehicle performance. In Proceedings of the 45th AIAA Aerospace Science Meeting and Exhibit, Reno, NV, USA, 8–11 January 2007. [Google Scholar]
- Matthew, M.; Luke, J.; Doherty, R. Gildfind T6: The Oxford University Stalker Tunnel. In Proceedings of the 20th Aiaa International Space Planes and Hypersonic Systems and Technologies Conference, Glasgow, Scotland, 6–9 July 2015. [Google Scholar]
- Zhao, W.; Jiang, Z.L.; Saito, T.; Lin, J.M. Performance of a detonation driven shock tunnel. Shock Waves 2005, 14, 53–59. [Google Scholar] [CrossRef]
- Shen, J.M.; Ma, H.D.; Li, C. Initial measurements of a 2m mach10 free piston shock tunnel at CAAA. In Proceedings of the 31st International Symposium on Shock Waves, Nagoya, Japan, 9–14 July 2017. [Google Scholar]
- Shen, J.; Dong, J.; Li, R.; Zhang, J.; Chen, X.; Qin, Y.; Ma, H. Integrated supersonic wind tunnel nozzle. Chin. J. Aeronaut. 2019, 32, 2422–2432. [Google Scholar] [CrossRef]
- Park, C. Assessment of two-temperature kinetic model for ionizing air. J. Thermophys. 1989, 3, 233–252. [Google Scholar] [CrossRef]
- Campbell, N.S.; Hanquist, K.; Morin, A.; Meyers, J.; Boyd, I. Evaluation of Computational Models for Electron Transpiration Cooling. Aerospace 2021, 8, 243. [Google Scholar] [CrossRef]
- Rudinskii, A.V.; Yagodnikov, D.A.; Ryzhkov, S.V.; Onufriev, V.V. Features of Intrinsic Electric Field Formation in Low-Temperature Oxygen–Methane Plasma. Tech. Phys. Lett. 2021, 47, 520–523. [Google Scholar] [CrossRef]
- Maier, W.T.; Needels, J.T.; Garbacz, C.; Morgado, F.; Alonso, J.J.; Fossati, M. SU2-NEMO: An Open-Source Framework for High-Mach Nonequilibrium Multi-Species Flows. Aerospace 2021, 8, 193. [Google Scholar] [CrossRef]
- Gnoffo, P.A.; Gupta, R.N. Conservation Equations and Physical Models for Hypersonic Air Flows in Thermal and Chemical Nonequilibrium; Report No. TP 2867; NASA: Washington, DC, USA, 1989.
- Gupta, R.N.; Thompson, R.A. A Review of Reaction Rates and Thermodynamics and Transport Properties for an 11-Species Air Model for Chemical and Thermal Nonequilibrium Calculations to 3000k; Report No. RP-1232; NASA: Washington, DC, USA, 1990.
- Park, C. Problems of rate chemistry in the flight regimes of aeroassisted orbital transfer vehicles. In Proceedings of the 19th Thermophysics Conference, Snowmass, CO, USA, 25–28 June 1984; Volume 96, pp. 511–537. [Google Scholar]
- Grant, P.; Michael, J.W. A comparison of methods to compute high temperature gas thermal conductivity. In Proceedings of the 36th AIAA Thermophysics Conference, Orlando, FL, USA, 23–26 June 2003. [Google Scholar]
- Jameson, A. Solution of the Euler equations for complex configurations. In Proceedings of the 6th Computational Fluid Dynamics Conference, Danvers, MA, USA, 13–15 July 1983. [Google Scholar]
- Shima, E.; Kitamura, K. On new simple low-dissipation scheme of AUSM-family for all speeds. In Proceedings of the 47th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace, Orlando, FL, USA, 5–8 January 2009. [Google Scholar]
- Candler, G.V. Hypersonic nozzle analysis using an excluded volume equation of state. In Proceedings of the 38th AIAA Thermophysics Conference, Toronto, ON, USA, 6–9 June 2005. [Google Scholar]
- Wang, Y.; Hu, Z.; Lu, Y. Starting process in a large-scale shock tunnel. AIAA J. 2016, 54, 1240–1249. [Google Scholar] [CrossRef]
- Spalart, P.R.; Allmaras, S.R. A one-equation turbulence model for aerodynamic flow. In Proceedings of the 30th AIAA Aerospace Science Meeting and Exhibit, Reno, NV, USA, 6–9 January 1992. [Google Scholar]
- Tyurenkova, V.V.; Stamov, L.I. Flame propagation in weightlessness above the burning surface of material. Acta Astronaut. 2019, 159, 342–348. [Google Scholar] [CrossRef]
- Zeng, M. Numerical Rebuilding of Free-Stream Measurement and Analysis of Noequilibrium Effects in High Enhtalpy Tunnel. Ph.D. Thesis, Chinese Academy of Sciences, Beijing, China, 2007; pp. 63–64. (In Chinese). [Google Scholar]
- Smirnov, N.; Betelin, V.; Nikitin, V.; Stamov, L.; Altoukhov, D. Accumulation of errors in numerical simulations of chemically reaction gas dynamics. Acta Astronaut. 2015, 117, 338–355. [Google Scholar] [CrossRef]
- Smirnov, N.; Betelin, V.; Shagaliev, R.; Nikitin, V.; Belyakov, I.; Deryuguin, Y.; Aksenov, S.; Korchazhkin, D. Hydrogen fuel rocket engines simulation using LOGOS code. Int. J. Hydrog. Energy 2014, 39, 10748–10756. [Google Scholar] [CrossRef]
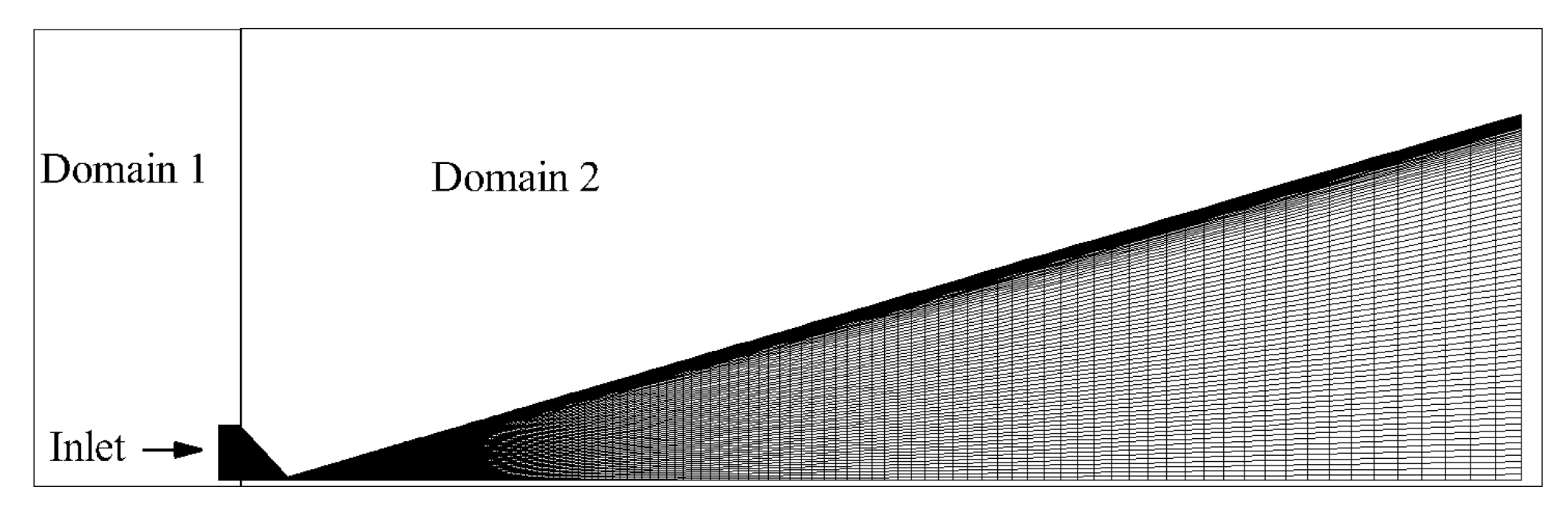
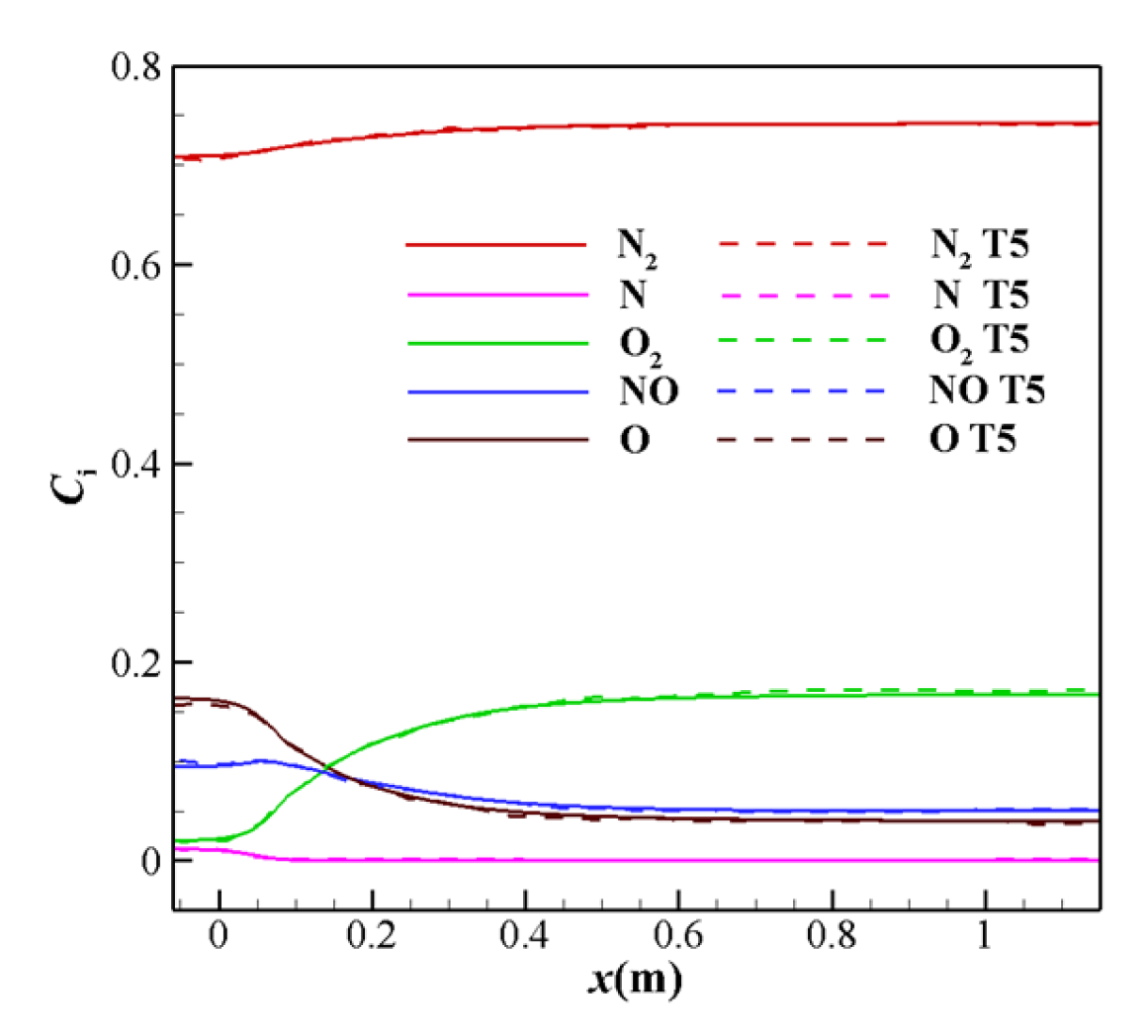

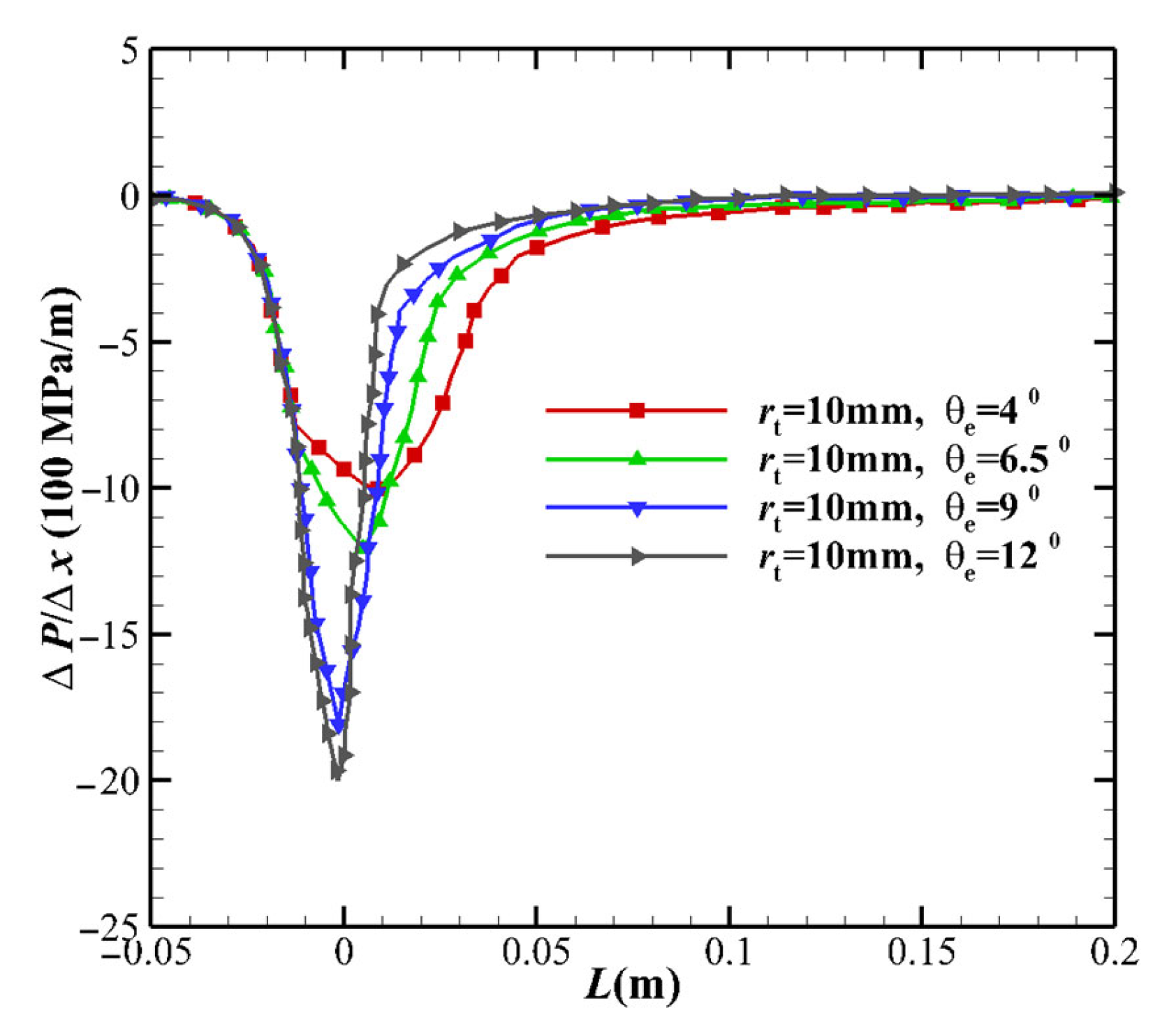



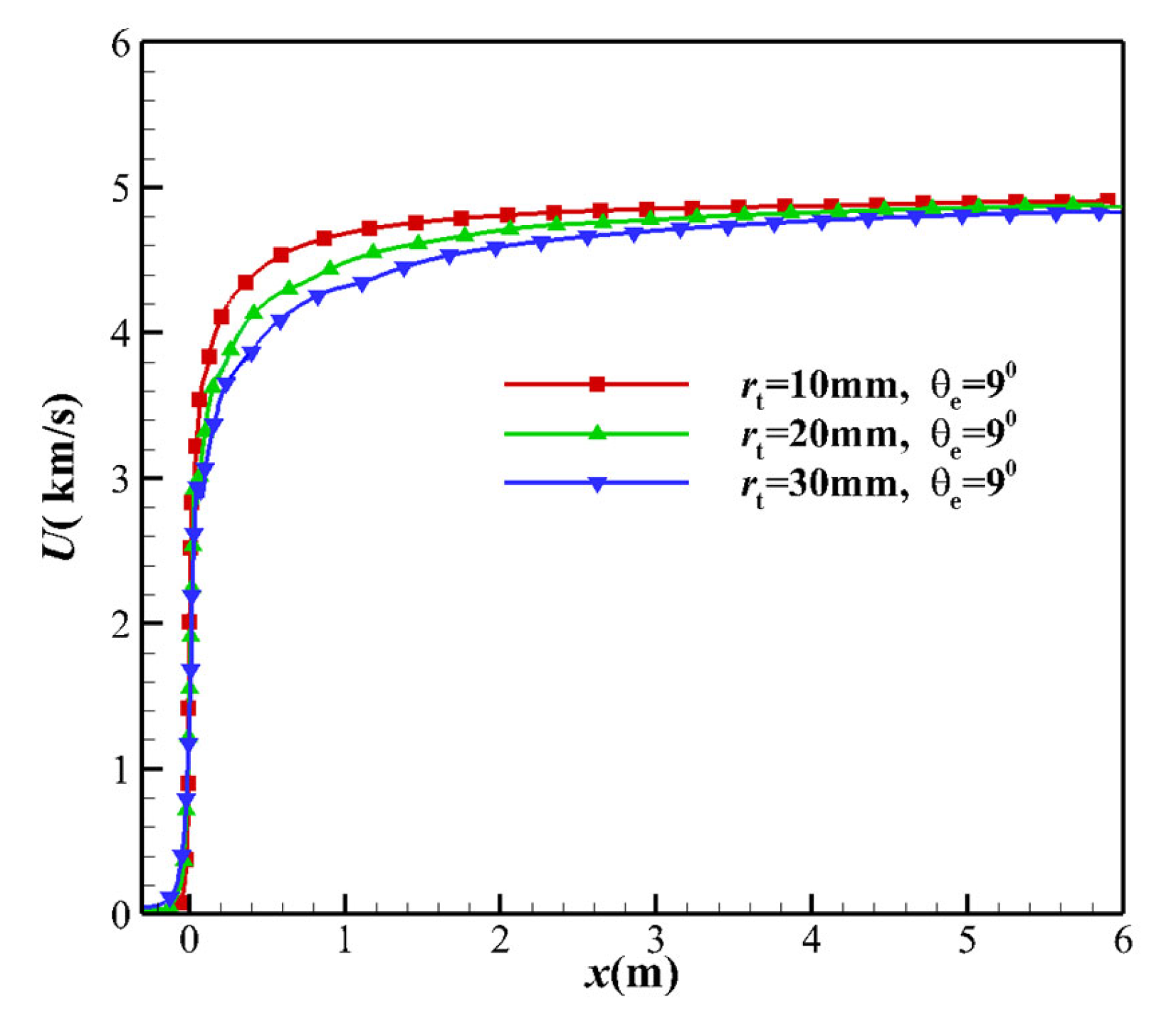
| Case | rc (mm) | θc (°) | θe (°) | rt (mm) | re (mm) | L (m) | ER |
|---|---|---|---|---|---|---|---|
| T5 | 45 | 20 | 7 | 15 | 150 | 1.2 | 100 |
| HEG | 11.0 | 30 | 6.5 | 5.5 | 440 | 3.75 | 1600 |
| Case | θc (°) | rc (mm) | θe (°) | rt (mm) | L (m) | ER |
|---|---|---|---|---|---|---|
| 1 | 30 | 20 | 4.0 | 10 | 14.43 | 10,000 |
| 2 | 30 | 20 | 6.5 | 10 | 9.01 | 10,000 |
| 3 | 30 | 20 | 9.0 | 10 | 6.52 | 10,000 |
| 4 | 30 | 20 | 12.0 | 10 | 4.91 | 10,000 |
| 5 | 30 | 20 | 9.0 | 20 | 6.32 | 2500 |
| 6 | 30 | 20 | 9.0 | 30 | 6.38 | 1111 |
| 7 | 20 | 20 | 9.0 | 10 | 6.63 | 10,000 |
| 8 | 45 | 20 | 9.0 | 10 | 6.40 | 10,000 |
| 9 | 30 | 10 | 9.0 | 10 | 6.50 | 10,000 |
| 10 | 30 | 40 | 9.0 | 10 | 6.49 | 10,000 |
| Case | P0 (MPa) | T0 (K) | h0 (MJ/kg) | CN2 (%) | CO2 (%) | CNO (%) | CN (%) | CO (%) | CNO+ (%) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 20.7 | 6359 | 11.3 | 0.7059 | 0.0234 | 0.1000 | 0.0013 | 0.1579 | 9.96E−5 |
| 2 | 48.3 | 7410 | 13.4 | 0.6872 | 0.0150 | 0.9880 | 0.3200 | 0.1670 | 0.01 |
| Case | T5 | HEG |
|---|---|---|
| Allowable error | 5% | 5% |
| Grid resolution | 1051 × 161 | 1051 × 161 |
| Physical time simulated(ms) | 4.0 | 5.0 |
| Number of time steps | 4000 | 5000 |
| Accumulated error | 2.40 × 10−7 | 2.40 × 10−7 |
| Results | P∞ (Pa) | U∞ (m/s) | CN2 | Co2 | CNO | CO | CN |
|---|---|---|---|---|---|---|---|
| T5 | 1150 | -- | 0.7414 | 0.1692 | 0.05135 | 0.0388 | 1.12 × 10−4 |
| 7S2T | 1208 | 4135 | 0.7419 | 0.1678 | 0.05015 | 0.0401 | 9.95 × 10−5 |
| 5S2T | 1250 | 4128 | 0.7411 | 0.1662 | 0.05174 | 0.0408 | 9.98 × 10−5 |
| Results | P∞ (Pa) | T∞ (K) | U∞ (m/s) | CN2 | Co2 | CNO | CO | CN |
|---|---|---|---|---|---|---|---|---|
| HEG | 713 | 694 | 4776 | 73.56 | 13.40 | 5.09 | 7.96 | 1.0 × 10−8 |
| 5S2T | 705 | 720 | 4823 | 0.73.94 | 13.48 | 5.03 | 7.83 | 1.8 × 10−8 |
| 7S2T | 701 | 723 | 4842 | 0.74.01 | 13.75 | 4.96 | 7.25 | 1.4 × 10−7 |
| Case | θe (0) | P (Pa) | T (K) | TV (K) | a (m/s) | Ma | CN2 | CO2 | CNO | CN | CO | CNO+ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 4.0 | 141 | 485 | 2095 | 435 | 11.3 | 0.7435 | 0.1706 | 0.0467 | 1.67 × 10−7 | 0.0389 | 2.61 × 10−4 |
| 2 | 6.5 | 93 | 360 | 2119 | 376 | 12.4 | 0.7422 | 0.1649 | 0.0492 | 3.32 × 10−7 | 0.0421 | 2.61 × 10−4 |
| 3 | 9.0 | 69 | 343 | 2450 | 367 | 13.2 | 0.7412 | 0.1600 | 0.0505 | 3.32 × 10−7 | 0.0482 | 2.61 × 10−4 |
| 4 | 12.0 | 61 | 323 | 2579 | 356 | 13.5 | 0.7401 | 0.1560 | 0.0548 | 6.84 × 10−7 | 0.0500 | 2.61 × 10−4 |
| Case | rt (mm) | P (Pa) | T (K) | TV (K) | a (m/s) | Ma | CN2 | CO2 | CNO | CN | CO | CNO+ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 10 | 69 | 343 | 2450 | 367 | 13.2 | 0.7412 | 0.1600 | 0.0505 | 3.32 × 10−7 | 0.0482 | 2.61 × 10−4 |
| 5 | 20 | 453 | 670 | 2194 | 511 | 9.5 | 0.7431 | 0.1684 | 0.0473 | 1.85 × 10−7 | 0.0410 | 2.61 × 10−4 |
| 6 | 30 | 1489 | 984 | 2046 | 619 | 7.8 | 0.7442 | 0.1743 | 0.0449 | 5.51 × 10−8 | 0.0364 | 2.61 × 10−4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, J.; Shao, Z.; Ji, F.; Chen, X.; Lu, H.; Ma, H. High Enthalpy Non-Equilibrium Expansion Effects in Turbulent Flow of the Conical Nozzle. Aerospace 2023, 10, 455. https://doi.org/10.3390/aerospace10050455
Shen J, Shao Z, Ji F, Chen X, Lu H, Ma H. High Enthalpy Non-Equilibrium Expansion Effects in Turbulent Flow of the Conical Nozzle. Aerospace. 2023; 10(5):455. https://doi.org/10.3390/aerospace10050455
Chicago/Turabian StyleShen, Junmou, Zongjie Shao, Feng Ji, Xing Chen, Hongbo Lu, and Handong Ma. 2023. "High Enthalpy Non-Equilibrium Expansion Effects in Turbulent Flow of the Conical Nozzle" Aerospace 10, no. 5: 455. https://doi.org/10.3390/aerospace10050455
APA StyleShen, J., Shao, Z., Ji, F., Chen, X., Lu, H., & Ma, H. (2023). High Enthalpy Non-Equilibrium Expansion Effects in Turbulent Flow of the Conical Nozzle. Aerospace, 10(5), 455. https://doi.org/10.3390/aerospace10050455





