Critical Assessment of the Bonded Single Lap Joint Exposed to Cyclic Tensile Loading
Abstract
1. Introduction and Motivation
2. Numerical Modelling
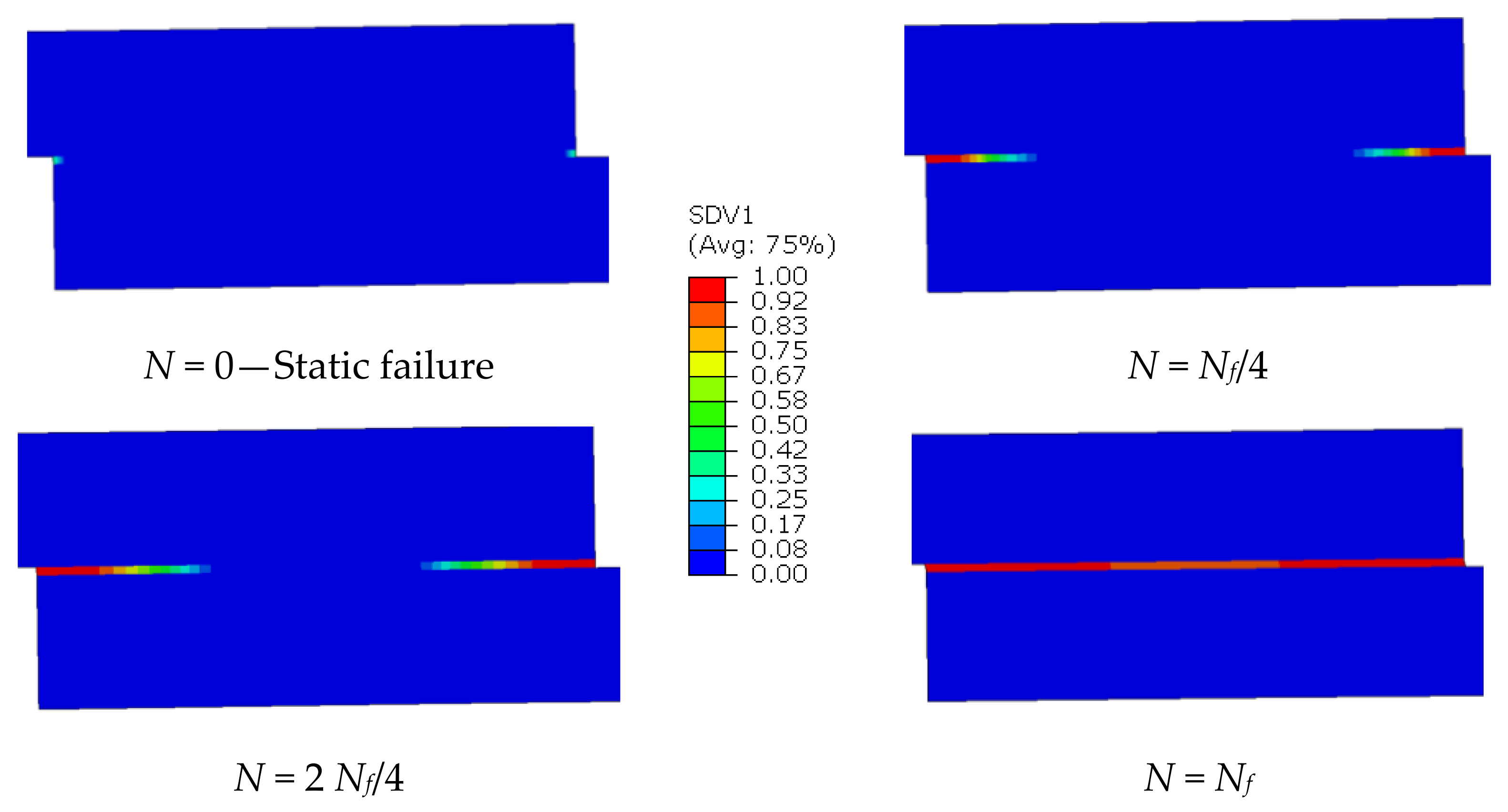
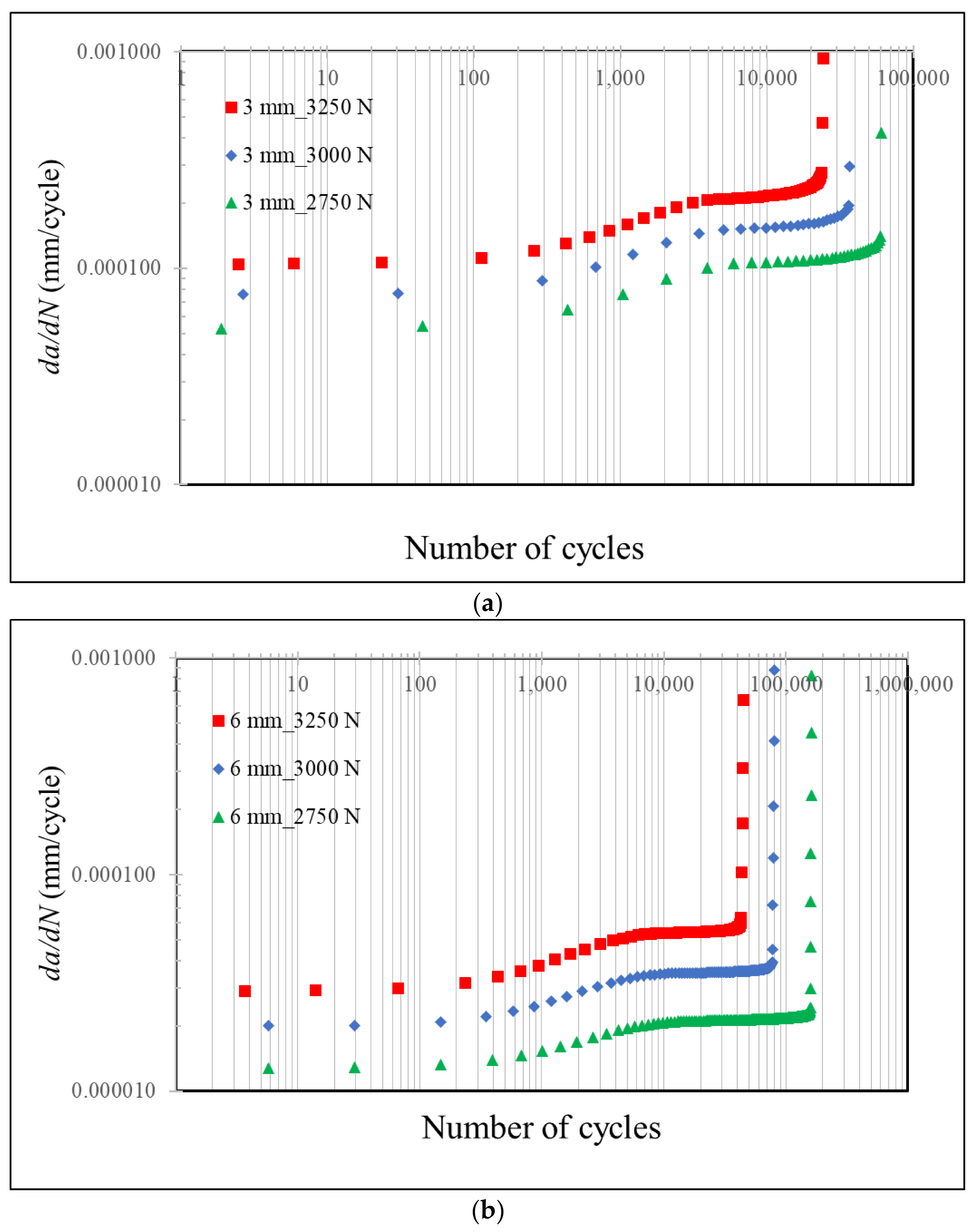
3. Result and Discussion
4. Concluding Remarks
- The SLJs with thicker adherends were observed to be more sensitive to the amount of load applied in terms of its service life.
- The life span of the SLJs changed more dramatically when subjected to a lower amount of load.
- A smaller thickness of the adherend in SLJ resulted in a decrease in the fatigue load limit, but led to an increase in the fatigue stress limit.
- When the fatigue load was increased, the crack initiation in the adhesive layer took place at a later stage when considering the failure cycle, i.e., an increased Ni/Nf with a shorter crack propagation duration was noticed.
- The crack propagation rate was not significant while the fatigue damage was still accumulating along the overlap length. Afterwards, it started increasing and reached its maximum gradually followed by a sudden jump at the final unsteady stage of the failure. The change in the crack propagation rate with a change in the amount of tensile loading applied was less for the SLJ with thinner adherends.
- It was noted that a larger share of the adhesive layer was damaged for thinner adherends for the identical fatigue loading period.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| crack length | |
| crack expansion rate | |
| local mixed-mode ratio | |
| C | constant in Paris law |
| CZ | cohesive zone |
| , | fatigue damage variables at time step and , respectively |
| , | static damage at the present and next incements, respectively |
| total damage | |
| Young’s Modulus | |
| mixed-mode Young’s Modulus | |
| FE | finite-element |
| mixed-mode fracture toughness | |
| area under the traction separation curve | |
| critical strain energy release rate for the opening mode | |
| critical strain energy release rate for the shear mode | |
| threshold value for the strain energy release rate | |
| interface stiffness for the opening mode | |
| mode dependent penalty stiffness | |
| penalty stiffness for the shear mode | |
| m | constant in Paris law |
| length of the cohesive zone | |
| length of the damaged area | |
| size of the finite element | |
| Ni | crack initiation cycle |
| Nf | failure cycle |
| n | material constant used in Benzeggagh-Kenane criterion |
| R | load ratio |
| SERR | strain energy release rate |
| SLJ | single lap joint |
| UMAT | user material available in Abaqus |
| λ | displacement jump |
| onset displacement jump | |
| critical displacement jump | |
| maximum displacement jump | |
| fatigue damage evolution variable | |
| expansion rate of the length of the damaged area | |
| skipped number of cycles | |
| selected maximum damage increase in the computations | |
| separation in Mode 1 (opening mode) | |
| separation in Mode 2 (shear mode) | |
| yield strength | |
| interfacial strength for the opening mode | |
| interfacial strength for the shear mode | |
| mixed-mode interlaminar strength | |
| Poisson’s ratio | |
| Macauley operator |
References
- Sioutis, I.; Tserpes, K. A Literature Review on Crack Arrest Features for Composite Materials and Composite Joints with a Focus on Aerospace Applications. Aerospace 2023, 10, 137. [Google Scholar] [CrossRef]
- Chowdhury, N.; WK, C.; Wang, J.; Chang, P. Static and fatigue testing thin riveted, bonded and hybrid carbon fiber double lap joints used in aircraft structures. Compos. Struct. 2015, 121, 315–323. [Google Scholar] [CrossRef]
- Adams, R.D. Strength Predictions for Lap Joints, Especially with Composite Adherends. A Review. J. Adhes. 1989, 30, 219–242. [Google Scholar] [CrossRef]
- Kadioglu, F.; Demiral, M. Failure behaviour of the single lap joints of angle-plied composites under three point bending tests. J. Adhes. Sci. Technol. 2020, 34, 531–548. [Google Scholar] [CrossRef]
- Zhao, B.; Lu, Z.-H. A Two-Dimensional Approach of Single-Lap Adhesive Bonded Joints. Mech. Adv. Mater. Struct. 2009, 16, 130–159. [Google Scholar] [CrossRef]
- Abdel Wahab, M. Fatigue in Adhesively Bonded Joints: A Review. ISRN Mater. Sci. 2012, 746308. [Google Scholar] [CrossRef]
- Khoramishad, H.; Crocombe, A.D.; Katnam, K.B.; Ashcroft, I.A. Predicting fatigue damage in adhesively bonded joints using a cohesive zone model. Int. J. Fatigue 2010, 32, 1146–1158. [Google Scholar] [CrossRef]
- Ibrahim, G.R.; Albarbar, A.; Brethee, K.F. Progressive failure mechanism of laminated composites under fatigue loading. J. Compos. Mater. 2020, 55, 137–144. [Google Scholar] [CrossRef]
- Quaresimin, M.; Ricotta, M. Life prediction of bonded joints in composite materials. Int. J. Fatigue 2006, 28, 1166–1176. [Google Scholar] [CrossRef]
- Shahani, A.R.; Pourhosseini, S.M. The effect of adherent thickness on fatigue life of adhesively bonded joints. Fatigue Fract. Eng. Mater. Struct. 2019, 42, 561–571. [Google Scholar] [CrossRef]
- Sahin, R.; Akpınar, S. The effects of adherend thickness on the fatigue strength of adhesively bonded single-lap joints. Int. J. Adhes. Adhes. 2021, 107, 102845. [Google Scholar] [CrossRef]
- Katnam, K.B.; Crocombe, A.D.; Khoramishad, H.; Ashcroft, I.A. Load Ratio Effect on the Fatigue Behaviour of Adhesively Bonded Joints: An Enhanced Damage Model. J. Adhes. 2010, 86, 257–272. [Google Scholar] [CrossRef]
- Li, W.; Zhou, S.; Shi, Z.; Wang, X.; Hu, P. Experimental and numerical analysis on fatigue durability of single-lap joints under vibration loads. J. Adhes. 2017, 93, 187–203. [Google Scholar] [CrossRef]
- Ghabezi, P.; Farahani, M. Trapezoidal traction–separation laws in mode II fracture in nano-composite and nano-adhesive joints. J. Reinf. Plast. Compos. 2018, 37, 780–794. [Google Scholar] [CrossRef]
- Sam-Daliri, O.; Faller, L.M.; Farahani, M.; Roshanghias, A.; Araee, A.; Baniassadi, M.; Oberlercher, H.; Zangl, H. Impedance analysis for condition monitoring of single lap CNT-epoxy adhesive joint. Int. J. Adhes. Adhes. 2019, 88, 59–65. [Google Scholar] [CrossRef]
- Ramalho, L.D.; Campilho, R.D.; Belinha, J.A.O.; da Silva, L.F. Static strength prediction of adhesive joints: A review. Int. J. Adhes. Adhes. 2020, 96, 102451. [Google Scholar] [CrossRef]
- Rocha, R.J.B.; Campilho, R.D.S.G. Evaluation of different modelling conditions in the cohesive zone analysis of single-lap bonded joints. J. Adhes. 2018, 94, 562–582. [Google Scholar] [CrossRef]
- Systemes, D. Abaqus 6.14 Documentation; Simulia Co.: Providence, RI, USA, 2014. [Google Scholar]
- Belnoue, J.H.; Giannis, S.; Dawson, M.; Hallett, S.R. Cohesive/adhesive failure interaction in ductile adhesive joints Part II: Quasi-static and fatigue analysis of double lap-joint specimens subjected to through-thickness compressive loading. Int. J. Adhes. Adhes. 2016, 68, 369–378. [Google Scholar] [CrossRef]
- Turon, A.; Camanho, P.; Costa, J.; Dávila, C. A damage model for the simulation of delamination in advanced composites under variable-mode loading. Mech. Mater. 2006, 38, 1072–1089. [Google Scholar] [CrossRef]
- Benzeggagh, M.L.; Kenane, M. Measurement of mixed-mode delamination fracture toughness of unidirectional glass/epoxy composites with mixed-mode bending apparatus. Compos. Sci. Technol. 1996, 56, 439–449. [Google Scholar] [CrossRef]
- Turon, A.; Camanho, P.; Costa, J.; Dávila, C. Simulation of delamination in composites under high-cycle fatigue. Compos. Part A Appl. Sci. Manuf. 2007, 38, 2270–2282. [Google Scholar] [CrossRef]
- Smeets, E. Development of a Fatigue Analysis Tool Using Cohesive Zone Modelling for Composite Specimens. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, 2019. [Google Scholar]
- Van Paepegem, W.; Degrieck, J. Fatigue degradation modelling of plain woven glass/epoxy composites. Compos. Part A Appl. Sci. Manuf. 2001, 32, 1433–1441. [Google Scholar] [CrossRef]
- Demiral, M.; Mamedov, A. Fatigue Performance of a Step-Lap Joint under Tensile Load: A Numerical Study. Polymers 2023, 15, 1949. [Google Scholar] [CrossRef] [PubMed]
- Turon, A.; González, E.; Sarrado, C.; Guillamet, G.; Maimí, P. Accurate simulation of delamination under mixed-mode loading using a cohesive model with a mode-dependent penalty stiffness. Compos. Struct. 2018, 184, 506–511. [Google Scholar] [CrossRef]
- Demiral, M.; Abbassi, F.; Muhammad, R.; Akpinar, S. Service Life Modelling of Single Lap Joint Subjected to Cyclic Bending Load. Aerospace 2023, 10, 8. [Google Scholar] [CrossRef]
- Azari, S.; Papini, M.; Spelt, J.K. Effect of adhesive thickness on fatigue and fracture of toughened epoxy joints—Part I: Experiments. Eng. Fract. Mech. 2011, 78, 153–162. [Google Scholar] [CrossRef]




| Parameter | Value | |
|---|---|---|
| Material | AA2024-T3 | DP460 |
| E | 72,400 MPa | - |
| K | - | 1014 N/mm3 |
| 0.33 | 0.38 | |
| 324 MPa | - | |
| , i = normal, shear | - | 32.6 MPa, 28.5 MPa |
| , i = normal, shear | - | 2.56 N/mm, 11.71 N/mm |
| - | 10−12 N/mm3 | |
| m | - | 2.0 |
| - | 0.005 | |
| n | - | 2.1 |
| Life to Damage Initiation/Number of Cycles to Failure (Their Ratio) | ||||||
|---|---|---|---|---|---|---|
| Load (N) | Stress for 3.0 mm (MPa) | Stress for 4.5 mm (MPa) | Stress for 6.0 mm (MPa) | 3.0 mm | 4.5 mm | 6.0 mm |
| 3250 | 43.33 | 28.89 | 21.67 | 3135/24,610 (0.127) | 5141/33,396 (0.154) | 7022/45,953 (0.153) |
| 3000 | 40.00 | 26.67 | 20.00 | 4133/37,857 (0.109) | 5862/53,712/ (0.109) | 9299/80,748/ (0.115) |
| 2750 | 36.67 | 24.44 | 18.33 | 5937/61,728 (0.096) | 8256/91,064/ (0.091) | 14,023/159,330/ (0.088) |
| 2500 | 33.33 | 22.22 | 16.67 | 6393/101,870 (0.063) | 9980/171,160/ (0.058) | 22,077/386,850/ (0.057) |
| 2250 | 30.00 | 20.00 | 15.00 | 9235/177,520 (0.052) | 13,503/378,670/ (0.036) | 1,000,000 |
| 2000 | 26.67 | 17.78 | 13.33 | 17,177/344,120 (0.050) | 1,000,000 | |
| 1750 | 23.33 | 15.56 | 11.67 | 28,994/817,550 (0.035) | ||
| 1500 | 20.00 | 13.33 | 10.00 | 1,000,000 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Demiral, M.; Abbassi, F.; Zahedi, A.; Akpinar, S. Critical Assessment of the Bonded Single Lap Joint Exposed to Cyclic Tensile Loading. Aerospace 2023, 10, 454. https://doi.org/10.3390/aerospace10050454
Demiral M, Abbassi F, Zahedi A, Akpinar S. Critical Assessment of the Bonded Single Lap Joint Exposed to Cyclic Tensile Loading. Aerospace. 2023; 10(5):454. https://doi.org/10.3390/aerospace10050454
Chicago/Turabian StyleDemiral, Murat, Fethi Abbassi, Abolfazl Zahedi, and Salih Akpinar. 2023. "Critical Assessment of the Bonded Single Lap Joint Exposed to Cyclic Tensile Loading" Aerospace 10, no. 5: 454. https://doi.org/10.3390/aerospace10050454
APA StyleDemiral, M., Abbassi, F., Zahedi, A., & Akpinar, S. (2023). Critical Assessment of the Bonded Single Lap Joint Exposed to Cyclic Tensile Loading. Aerospace, 10(5), 454. https://doi.org/10.3390/aerospace10050454









