Comparison of Doses in Lunar Habitats Located at the Surface and in Crater
Abstract
:1. Introduction
2. Materials and Methods
2.1. Simulation Code
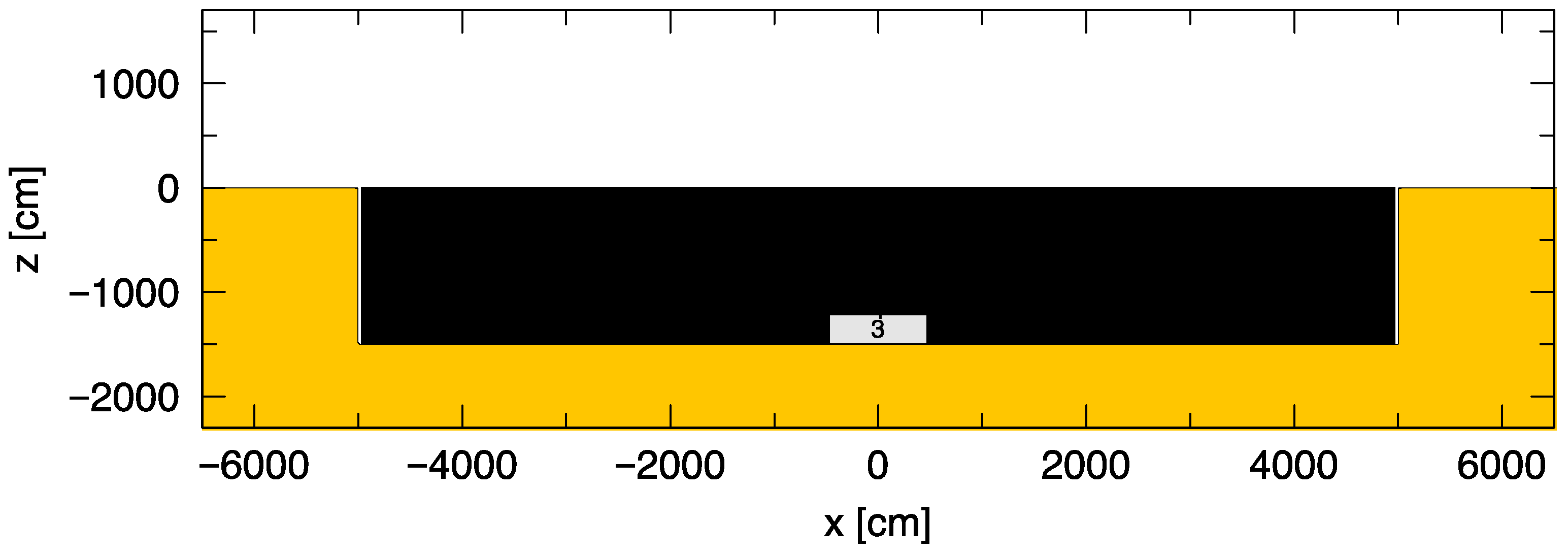
2.2. Geometry
2.3. Radiation Source
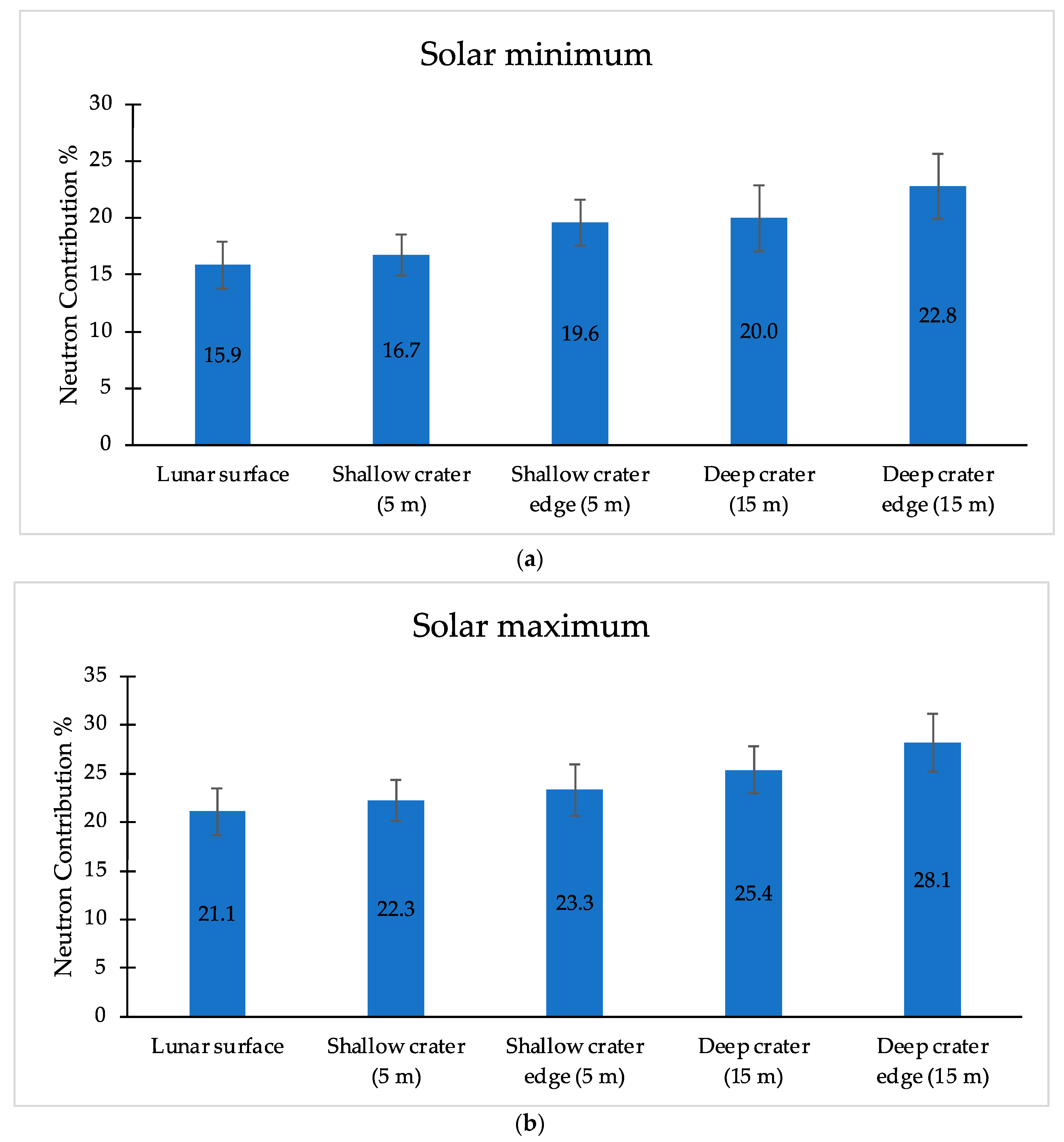
3. Results
3.1. Solar Minumum
3.2. Solar Maximum
4. Discussion
5. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- National Council on Radiation Protection and Measurement (NCRP). Guidance on Radiation Received in Space Activities; NCRP Report No. 98; NCRP: Bethesda, MD, USA, 1988. [Google Scholar]
- Simpson, J.A. Elemental and Isotopic Composition of the Galactic Cosmic Rays. Annu. Rev. Nucl. Part. Sci. 1983, 33, 323–382. [Google Scholar] [CrossRef]
- NASA. Nasa Spaceflight Human-System Standard—Volume 1: Crew Health; NASA-STD 3001, Revision C; NASA: Washington, DC, USA, 2023. [Google Scholar]
- Li, S. Design Structure Matrix Approach Applied to Lunar Habitat Design. Buildings 2023, 13, 1284. [Google Scholar] [CrossRef]
- Rueß, F.; Schänzlin, J.; Benaroya, H. Structural Design of a Lunar Habitat. J. Aerosp. Eng. 2006, 19, 133–157. [Google Scholar] [CrossRef]
- Slaba, T.C.; Bahadori, A.A.; Reddell, B.D.; Singleterry, R.C.; Clowdsley, M.S.; Blattnig, S.R. Optimal shielding thickness for galactic cosmic ray environments. Life Sci. Space Res. 2017, 12, 1–15. [Google Scholar] [CrossRef] [PubMed]
- Zaman, F.A.; Townsend, L.W.; de Wet, W.C.; Burahmah, N.T. The Lunar Radiation Environment: Comparisons between PHITS, HETC-HEDS, and the CRaTER Instrument. Aerospace 2021, 8, 182. [Google Scholar] [CrossRef]
- Zaman, F.A.; Townsend, L.W.; de Wet, W.C.; Looper, M.D.; Brittingham, J.M.; Burahmah, N.T.; Spence, H.E.; Schwadron, N.A.; Smith, S.S. Modeling the Lunar Radiation Environment: A Comparison Among FLUKA, Geant4, HETC-HEDS, MCNP6, and PHITS. Space Weather 2022, 20, e2021SW002895. [Google Scholar] [CrossRef]
- Heilbronn, L.H.; Borak, T.B.; Townsend, L.W.; Tsai, P.-E.; Burnham, C.A.; McBeth, R.A. Neutron yields and effective doses produced by Galactic Cosmic Ray interactions in shielded environments in space. Life Sci. Space Res. 2015, 7, 90–99. [Google Scholar] [CrossRef] [PubMed]
- Bahadori, A.A.; Sato, T.; Slaba, T.C.; Shavers, M.R.; Semones, E.J.; Van Baalen, M.; Bolch, W.E. A comparative study of space radiation organ doses and associated cancer risks using PHITS and HZETRN. Phys. Med. Biol. 2013, 58, 7183. [Google Scholar] [CrossRef] [PubMed]
- Sihver, L.; Sato, T.; Puchalska, M.; Reitz, G. Simulations of the MATROSHKA experiment at the international space station using PHITS. Radiat. Environ. Biophys. 2010, 49, 351–357. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.; Wimmer-Schweingruber, R.F.; Yu, J.; Wang, C.; Fu, Q.; Zou, Y.; Sun, Y.; Wang, C.; Hou, D.; Böttcher, S.I. First measurements of the radiation dose on the lunar surface. Sci. Adv. 2020, 6, eaaz1334. [Google Scholar] [CrossRef] [PubMed]
- Sato, T.; Iwamoto, Y.; Hashimoto, S.; Ogawa, T.; Furuta, T.; Abe, S.-i.; Kai, T.; Tsai, P.-E.; Matsuda, N.; Iwase, H.; et al. Features of Particle and Heavy Ion Transport code System (PHITS) version 3.02. J. Nucl. Sci. Technol. 2018, 55, 684–690. [Google Scholar] [CrossRef]
- Boudard, A.; Cugnon, J.; David, J.C.; Leray, S.; Mancusi, D. New potentialities of the Liège intranuclear cascade model for reactions induced by nucleons and light charged particles. Phys. Rev. C 2013, 87, 014606. [Google Scholar] [CrossRef]
- Nara, Y.; Otuka, N.; Ohnishi, A.; Niita, K.; Chiba, S. Relativistic nuclear collisions at 10A GeV energies from p + Be to Au+Au with the hadronic cascade model. Phys. Rev. C 1999, 61, 024901. [Google Scholar] [CrossRef]
- Furihata, S. Statistical analysis of light fragment production from medium energy proton-induced reactions. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2000, 171, 251–258. [Google Scholar] [CrossRef]
- Ogawa, T.; Sato, T.; Hashimoto, S.; Satoh, D.; Tsuda, S.; Niita, K. Energy-dependent fragmentation cross sections of relativistic 12C. Phys. Rev. C 2015, 92, 024614. [Google Scholar] [CrossRef]
- Hirayama, H.; Namito, Y.; Nelson, W.R.; Bielajew, A.F.; Wilderman, S.J. The EGS5 Code System; United States Department of Energy: Washington, DC, USA, 2005. [Google Scholar]
- Dietze, G.; Bartlett, D.T.; Cool, D.A.; Cucinotta, F.A.; Jia, X.; McAulay, I.R.; Pelliccioni, M.; Petrov, V.; Reitz, G.; Sato, T. ICRP Publication 123: Assessment of Radiation Exposure of Astronauts in Space. Ann. ICRP 2013, 42, 1–339. [Google Scholar] [CrossRef] [PubMed]
- Singleterry, R., Jr.; Blattnig, S.; Clowdsley, M.; Qualls, G.; Sandridge, C.; Simonsen, L.; Norbury, J.; Slaba, T.; Walker, S.; Badavi, F. OLTARIS: On-Line Tool for the Assessment of Radiation in Space (NASA/TP-2010-216722); National Aeronautics and Space Administration (NASA): Washington, DC, USA, 2010. [Google Scholar]
- Matthiä, D.; Berger, T.; Mrigakshi, A.I.; Reitz, G. A ready-to-use galactic cosmic ray model. Adv. Space Res. 2013, 51, 329–338. [Google Scholar] [CrossRef]
- Cucinotta, F.A. Radiation Risk Acceptability and Limitations; Space Radiation Program Element; NASA Johnson Space Center: Houston, TX, USA, 2010. [Google Scholar]
- Zaman, F.A.; Townsend, L.W.; Burahmah, N.T. Radiation Risks in a Mission to Mars for a Solar Particle Event Similar to the AD 993/4 Event. Aerospace 2021, 8, 143. [Google Scholar] [CrossRef]

| Element | Weight Fraction |
|---|---|
| O | 0.605 |
| Si | 0.154 |
| Fe | 0.0571 |
| Mg | 0.0548 |
| Al | 0.0497 |
| Ca | 0.0444 |
| Ti | 0.0279 |
| Na | 0.00286 |
| S | 0.000869 |
| Mn | 0.000785 |
| Cr | 0.00141 |
| K | 0.000386 |
| P | 0.000229 |
| Proton | Neutron | Total | ||||||
|---|---|---|---|---|---|---|---|---|
| Base Location | Solar Activity | Gender | Dose | Uncertainty | Dose | Uncertainty | Dose | Uncertainty |
| Lunar surface | minimum | Male | 230.9 | 9.6% | 120.9 | 12% | 762.1 | 4.8% |
| Female | 231 | 9.6% | 121 | 12% | 762.1 | 4.7% | ||
| maximum | Male | 61.4 | 9.6% | 55 | 10% | 260.8 | 5.6% | |
| Female | 61.4 | 9.6% | 54.7 | 10.1% | 261.1 | 5.5% | ||
| Shallow crater (5 m) | minimum | Male | 226.8 | 11% | 119 | 9.6% | 710.7 | 4.4% |
| Female | 226.4 | 11% | 118.5 | 9.6% | 711.1 | 4.4% | ||
| maximum | Male | 61.6 | 9.6% | 55 | 8.4% | 247.0 | 4.1% | |
| Female | 61.4 | 9.6% | 54.7 | 8.4% | 247.1 | 4.1% | ||
| Deep crater (15 m) | minimum | Male | 181.9 | 11% | 123.7 | 9% | 619.2 | 4.6% |
| Female | 182.8 | 11% | 122.8 | 9% | 619.7 | 4.6% | ||
| maximum | Male | 47.7 | 10.4% | 53.8 | 8.3% | 212.2 | 4.6% | |
| Female | 47.4 | 10.4% | 53.4 | 8.3% | 212.7 | 4.6% | ||
| Shallow crater edge (5 m) | minimum | Male | 230.3 | 10% | 133.3 | 14% | 680.8 | 5.6% |
| Female | 230.7 | 10% | 132.5 | 14% | 680.2 | 5.6% | ||
| maximum | Male | 60.4 | 10.2% | 53.2 | 10.2% | 228.2 | 5.2% | |
| Female | 52.8 | 10.3% | 52.8 | 10.3% | 228.4 | 5.2% | ||
| Deep crater edge (15 m) | minimum | Male | 121.6 | 14.7% | 95.7 | 10.5% | 419.8 | 6.6% |
| Female | 122.2 | 14.7% | 95 | 10.6% | 420.2 | 6.6% | ||
| maximum | Male | 33.2 | 14.1% | 42.1 | 8.6% | 149.6 | 6.1% | |
| Female | 33.2 | 14.2% | 41.6 | 8.7% | 149.6 | 6.1% | ||
| Base Location | Solar Minimum | Solar Maximum | |
|---|---|---|---|
| Percent Reduction in the Incident GCR Flux | Percent Reduction in the Dose in the Base Relative to the Surface Dose | ||
| Shallow center | 0.5% | 6.7% | 5.3% |
| Shallow crater edge | 7.3% | 10.7% | 12.5% |
| Deep center | 4.2% | 18.8% | 18.6% |
| Deep crater edge | 25.8% | 44.9% | 42.7% |
| Age at Exposure | Effective Dose Limit (mSv) | |
|---|---|---|
| Male | Female | |
| 30 | 620 | 470 |
| 35 | 720 | 550 |
| 40 | 800 | 620 |
| 45 | 950 | 750 |
| 50 | 1150 | 920 |
| 55 | 1470 | 1120 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Burahmah, N.T.; Heilbronn, L.H. Comparison of Doses in Lunar Habitats Located at the Surface and in Crater. Aerospace 2023, 10, 970. https://doi.org/10.3390/aerospace10110970
Burahmah NT, Heilbronn LH. Comparison of Doses in Lunar Habitats Located at the Surface and in Crater. Aerospace. 2023; 10(11):970. https://doi.org/10.3390/aerospace10110970
Chicago/Turabian StyleBurahmah, Naser T., and Lawrence H. Heilbronn. 2023. "Comparison of Doses in Lunar Habitats Located at the Surface and in Crater" Aerospace 10, no. 11: 970. https://doi.org/10.3390/aerospace10110970
APA StyleBurahmah, N. T., & Heilbronn, L. H. (2023). Comparison of Doses in Lunar Habitats Located at the Surface and in Crater. Aerospace, 10(11), 970. https://doi.org/10.3390/aerospace10110970






