Impacts of Climate Change on Rainfall ‘Seasonality Index’ and Its Potential Implications on Water Savings and Reliability through Household Rainwater Tanks
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area
2.2. Climate Model and Data
2.3. SI Calculation
2.4. Calculation of Water Savings and Reliability
3. Results
3.1. Results on Annual Water Savings
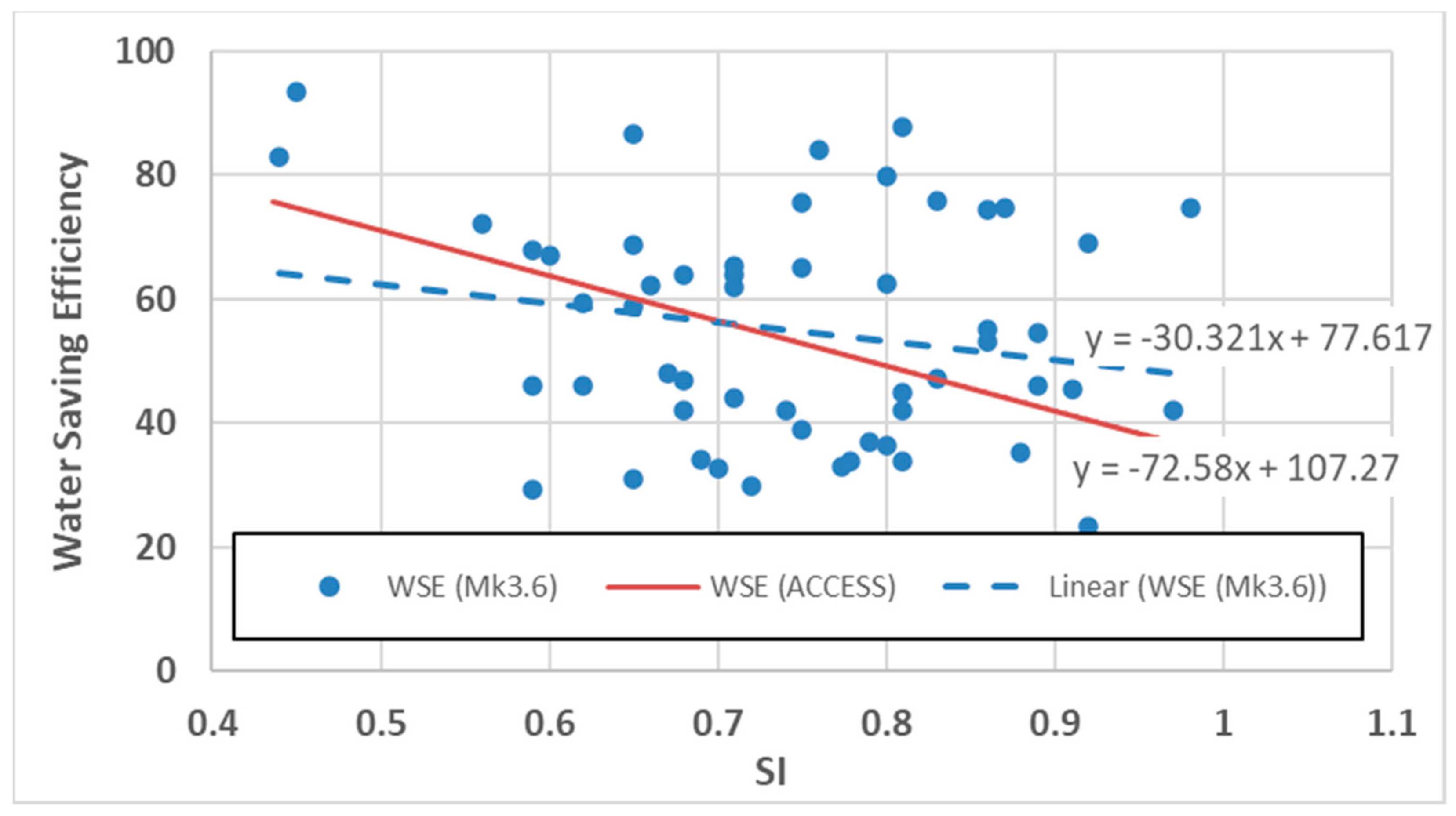
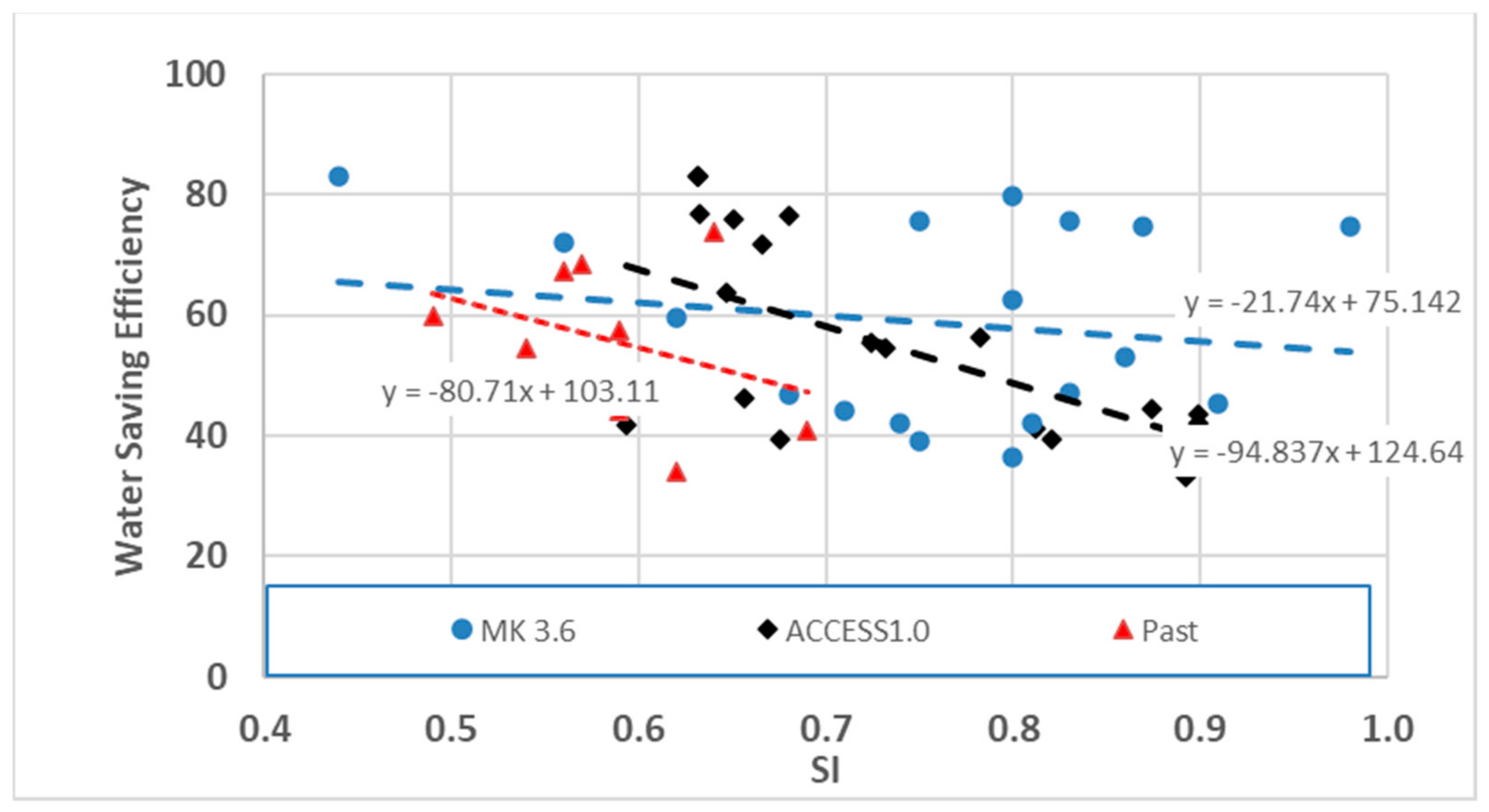
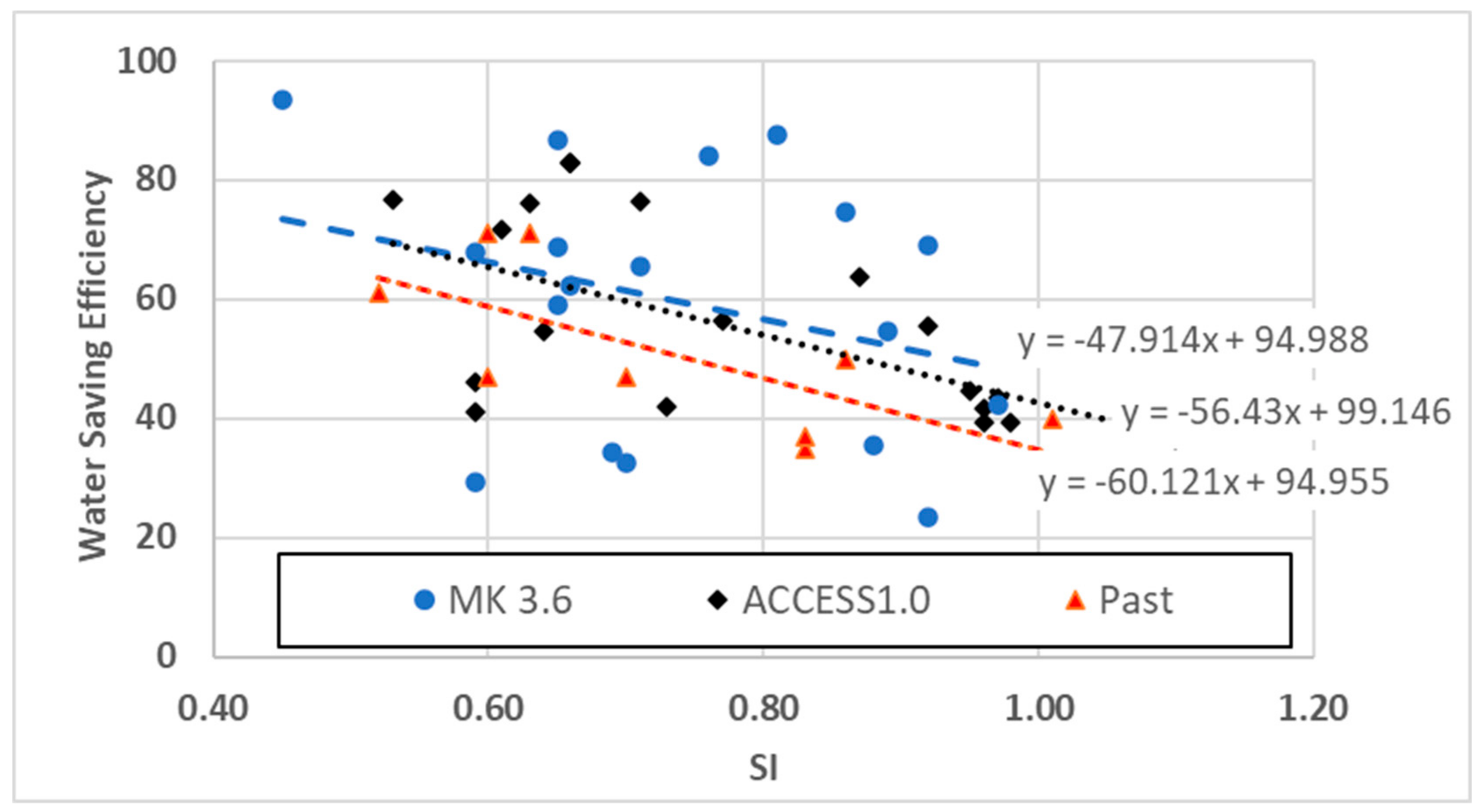
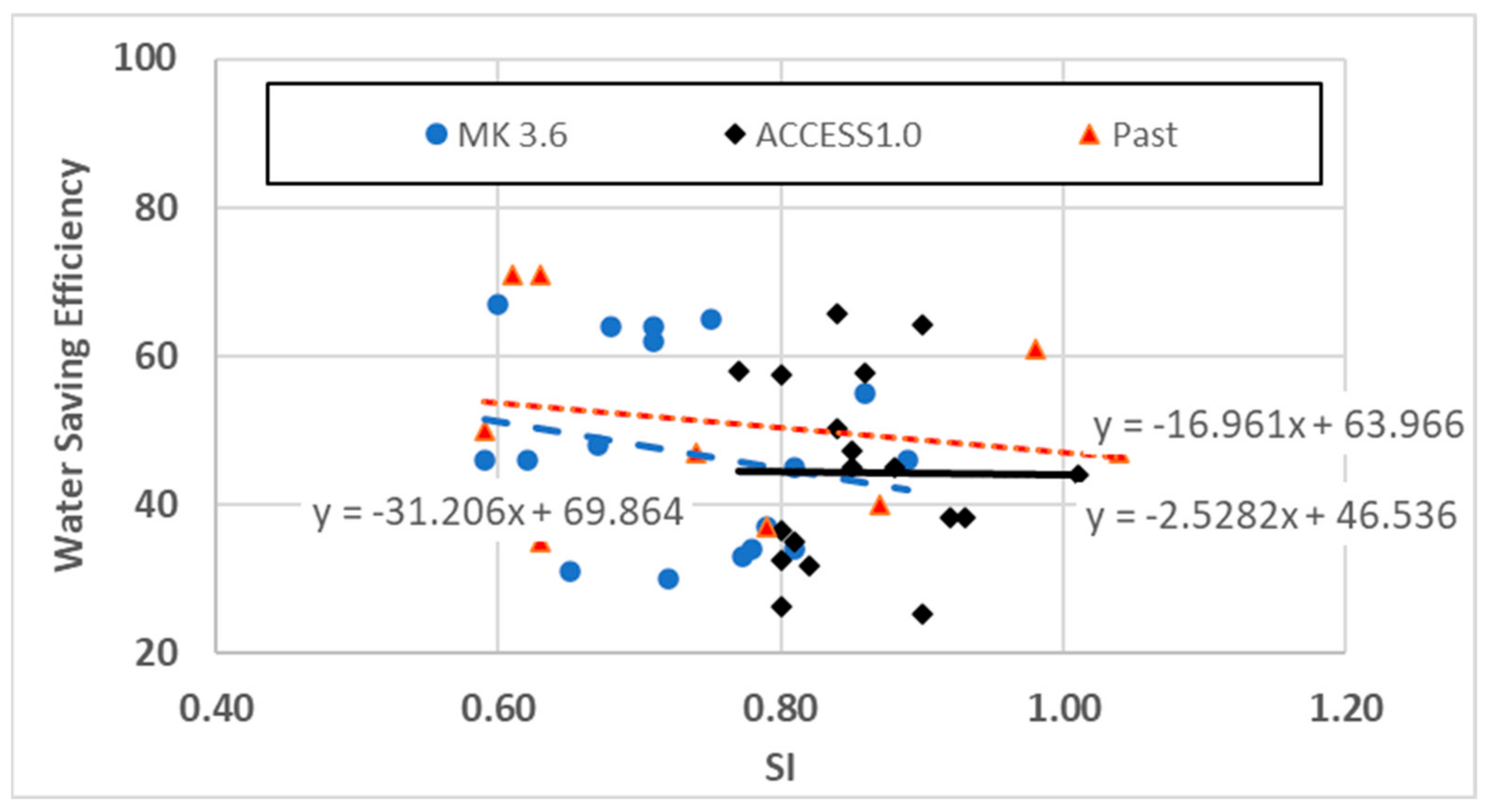
3.2. Results on Water Saving Efficiency
3.3. SI–Reliability Relationships
4. Discussions
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Special Note
Conflicts of Interest
References
- Liu, Z.; Zhang, J.; Wen, T.; Cheng, J. Uncertainty Quantification of Rainfall-runoff Simulations Using the Copula-based Bayesian Processor: Impacts of Seasonality, Copula Selection and Correlation Coefficient. Water Resour. Manag. 2022, 36, 4981–4993. [Google Scholar] [CrossRef]
- Rachid, G.; Alameddine, I.; El-Fadel, M. Management of Saltwater Intrusion in Data-scarce Coastal Aquifers: Impacts of Seasonality, Water Deficit, and Land Use. Water Resour. Manag. 2021, 35, 5139–5153. [Google Scholar] [CrossRef]
- Serrano, L.; Ribeiro, R.B.; Borges, A.C.; Pruski, F.F. Low-Flow Seasonality and Effects on Water Availability throughout the River Network. Water Resour. Manag. 2020, 34, 1289–1304. [Google Scholar] [CrossRef]
- Sumner, G.N. Precipitation: Process and Analysis; John Wiley & Sons Ltd: Chichester, UK, 1988. [Google Scholar]
- Risbey, J.S.; Pook, M.J.; McIntosh, P.C.; Wheeler, M.C.; Hendon, H.H. On the remote drivers of rainfall variability in Australia. Mon. Weather. Rev. 2009, 137, 3233–3253. [Google Scholar] [CrossRef]
- Imteaz, M.A.; Yilmaz, A.; Ahsan, A. Climatic and spatial variations in seasonality index of rainfall pattern for major Australian cities. Int. J. Hydrol. Sci. Technol. 2021, 14, 14–26. [Google Scholar] [CrossRef]
- Easterling, D.R.; Meehl, G.A.; Parmesan, C.; Changnon, S.A.; Karl, T.R.; Mearns, L.O. Climate extremes: Observations, modeling, and impacts. Science 2000, 289, 2068–2074. [Google Scholar] [CrossRef] [PubMed]
- Mikhaylov, A.; Moiseev, N.; Aleshin, K.; Burkhardt, T. Global climate change and greenhouse effect. Entrep. Sustain. Issues 2020, 7, 2897. [Google Scholar] [CrossRef] [PubMed]
- Feng, X.; Porporato, A.; Rodriguez-Iturbe, I. Changes in rainfall seasonality in the tropics. Nat. Clim. Chang. 2013, 3, 811–815. [Google Scholar] [CrossRef]
- Chowdhury, R.; Beecham, S. Australian rainfall trends and their relation to the southern oscillation index. Hydrol. Process Int. J. 2010, 24, 504–514. [Google Scholar] [CrossRef]
- Kamruzzaman, M.; Beecham, S.; Metcalfe, A. Climatic influences on rainfall and runoff variability in the southeast region of the Murray-Darling Basin. Int. J. Climatol. 2013, 33, 291–311. [Google Scholar] [CrossRef]
- King, A.D.; Pitman, A.J.; Henley, B.J.; Ukkola, A.M.; Brown, J.R. The role of climate variability in Australian drought. Nat. Clim. Chang. 2020, 10, 177–179. [Google Scholar] [CrossRef]
- Holgate, C.; Evans, J.; Taschetto, A.; Gupta, A.; Santoso, A. The Impact of Interacting Climate Modes on East Australian Precipitation Moisture Sources. J. Clim. 2022, 35, 3147–3159. [Google Scholar] [CrossRef]
- Mohamadi, S.; Khozani, Z.; Ehteram, M.; Ahmed, A.N.; El-Shafie, A. Rainfall prediction using multiple inclusive models and large climate indices. Environ. Sci. Pollut. Res. 2022, 29, 85312–85349. [Google Scholar] [CrossRef] [PubMed]
- Ehteram, M.; Afan, H.A.; Dianatikhah, M.; Ahmed, A.N.; Ming Fai, C.; Hossain, M.S.; Allawi, M.F.; Elshafie, A. Assessing the predictability of an improved ANFIS model for monthly streamflow using lagged climate indices as predictors. Water 2019, 11, 1130. [Google Scholar] [CrossRef]
- Imteaz, M.A.; Moniruzzaman, M.; Yilmaz, A. Towards developing generalised equations for calculating potential rainwater savings. In Handbook of Water Harvesting and Conservation; Eslamian, S., Ed.; John Wiley & Sons Inc: Hoboken, NJ, USA, 2021; Chapter 27; ISBN 978–1119478959. [Google Scholar]
- Pascale, S.; Lucarini, V.; Feng, X.; Porporato, A.; Hasson, S.U. Analysis of rainfall seasonality from observations and climate models. Clim. Dyn. 2015, 44, 3281–3301. [Google Scholar] [CrossRef]
- Preeti, P.; Haddad, K.; Rahman, A. Assessing the impacts of climate change on rainwater harvesting: A case study for eight Australian capital cities. Water 2022, 14, 3123. [Google Scholar] [CrossRef]
- Toosi, A.S.; Danesh, S.; Tousi, E.G.; Doulabian, S. Annual and seasonal reliability of urban rainwater harvesting system under climate change. Sustain. Cities Soc. 2020, 63, 102427. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, J.; Yue, T.; Jing, X. Impacts of climate change on urban rainwater harvesting systems. Sci. Total Environ. 2019, 665, 262–274. [Google Scholar] [CrossRef] [PubMed]
- Kisakye, V.; Van der Bruggen, B. Effects of climate change on water savings and water security from rainwater harvesting systems. Resour. Conserv. Recycl. 2018, 138, 49–63. [Google Scholar] [CrossRef]
- Jenkins, G. Use of continuous simulation for the selection of an appropriate urban rainwater tank. Australas. J. Water Resour. 2007, 11, 231–246. [Google Scholar] [CrossRef]
- Imteaz, M.A.; Hossain, I. Climate Change Impacts on ‘Seasonality Index’ and its Potential Implications on Rainwater Savings. Water Resour. Manag. 2022, 37, 2593–2606. [Google Scholar] [CrossRef]
- Imteaz, M.A.; Khan, M.S.; Yilmaz, A.G.; Shanableh, A. Climate Change Impacts on Rainwater Tank’s Potential Water Savings, Efficiency and Reliability Presenting Relationship Between ‘Seasonality Index’ and Water Savings Efficiency. Water Resour. Manag. 2023, 37, 2593–2606. [Google Scholar] [CrossRef]
- Rotstayn, L.D.; Jeffrey, S.J.; Collier, M.A.; Dravitzki, S.M.; Hirst, A.C.; Syktus, J.I.; Wong, K.K. Aerosol and greenhouse gas induced changes in summer rainfall and circulation in the Australasian region: A study using single-forcing climate simulations. Atmos. Chem. Phys. 2012, 12, 6377–6404. [Google Scholar] [CrossRef]
- Imteaz, M.A.; Karki, R.; Shamseldin, A.; Matos, C. eTank and contemporary online tools for rainwater tank outcomes analysis. Int. J. Comput. Aided Eng. Technol. 2017, 9, 372–384. [Google Scholar] [CrossRef]

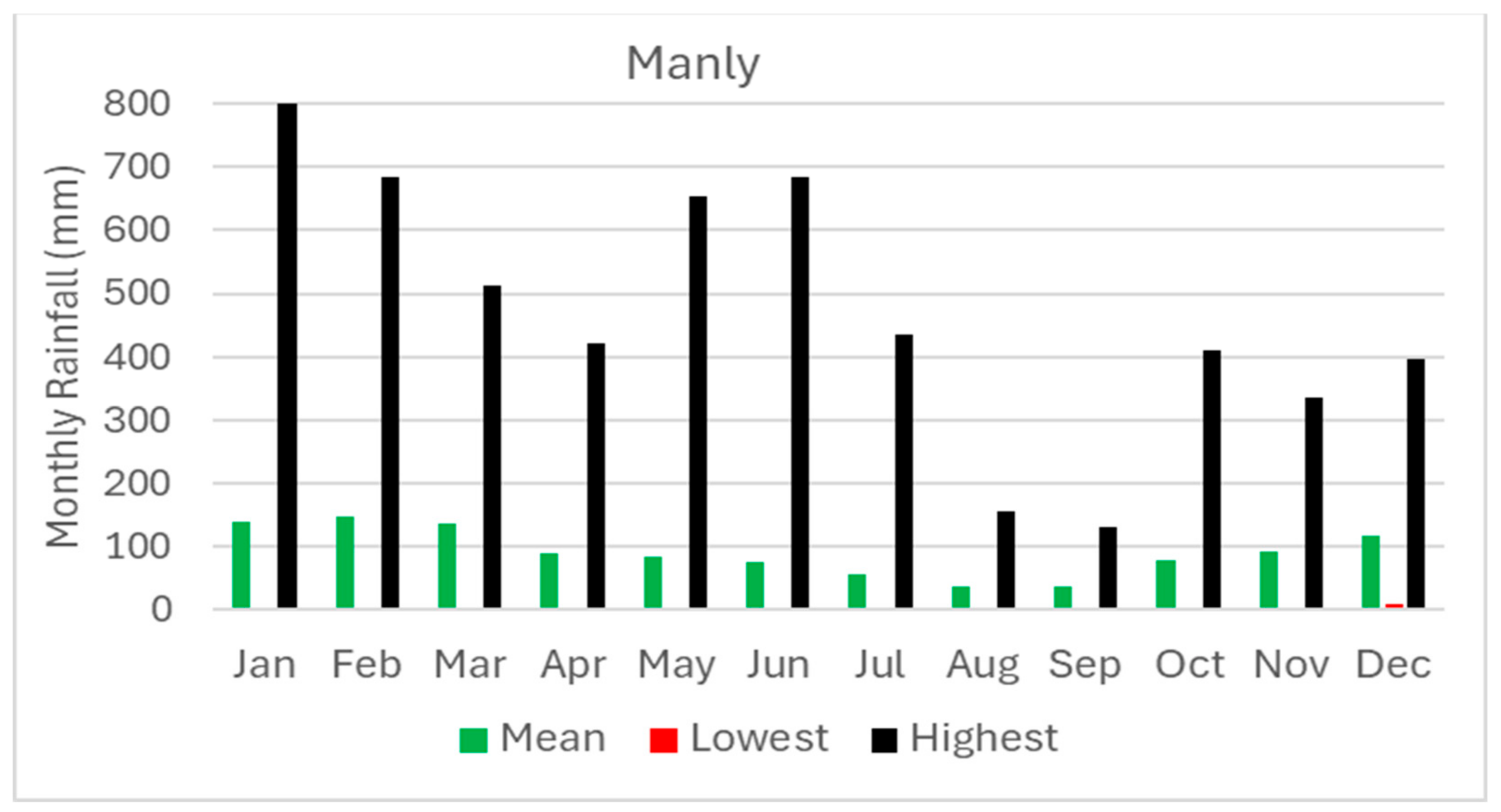
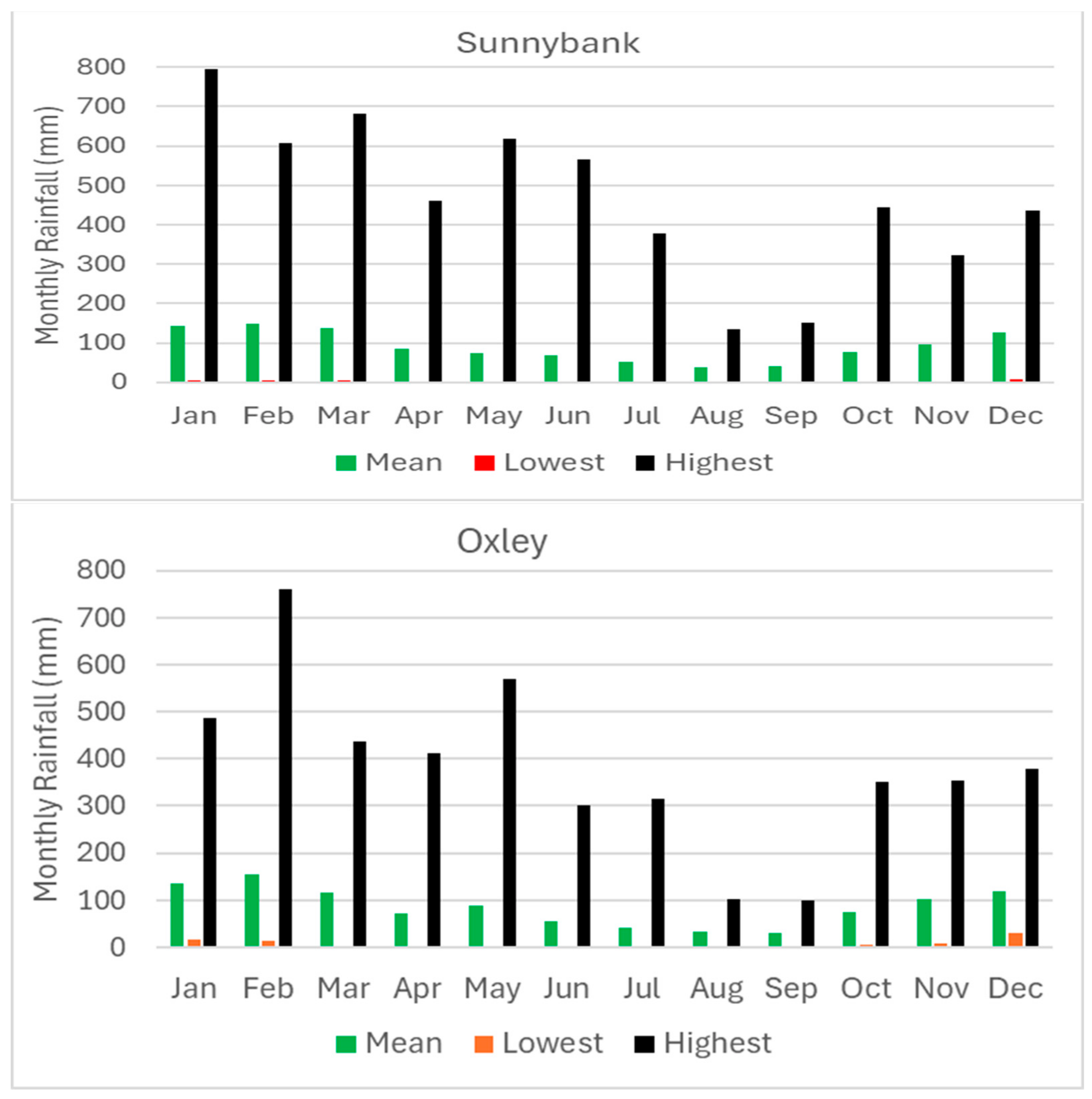


| Rainfall Station | Station Number | Elevation (m) | Location of Station |
|---|---|---|---|
| Manly | 40231 | 25 | Eastern |
| Sunnybank | 40244 | 50 | Southern |
| Oxley | 40463 | 20 | Western |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Imteaz, M.A.; Khan, M.S.; Hossain, I.; Ahsan, A. Impacts of Climate Change on Rainfall ‘Seasonality Index’ and Its Potential Implications on Water Savings and Reliability through Household Rainwater Tanks. Climate 2024, 12, 153. https://doi.org/10.3390/cli12100153
Imteaz MA, Khan MS, Hossain I, Ahsan A. Impacts of Climate Change on Rainfall ‘Seasonality Index’ and Its Potential Implications on Water Savings and Reliability through Household Rainwater Tanks. Climate. 2024; 12(10):153. https://doi.org/10.3390/cli12100153
Chicago/Turabian StyleImteaz, Monzur Alam, Mohammad S. Khan, Iqbal Hossain, and Amimul Ahsan. 2024. "Impacts of Climate Change on Rainfall ‘Seasonality Index’ and Its Potential Implications on Water Savings and Reliability through Household Rainwater Tanks" Climate 12, no. 10: 153. https://doi.org/10.3390/cli12100153
APA StyleImteaz, M. A., Khan, M. S., Hossain, I., & Ahsan, A. (2024). Impacts of Climate Change on Rainfall ‘Seasonality Index’ and Its Potential Implications on Water Savings and Reliability through Household Rainwater Tanks. Climate, 12(10), 153. https://doi.org/10.3390/cli12100153








