Decomposing the Bonferroni Inequality Index by Subgroups: Shapley Value and Balance of Inequality

Abstract

Additive decomposability is an interesting feature of inequality indices which, however, is not always fulfilled; solutions to overcome such an issue have been given by Deutsch and Silber (2007) and by Di Maio and Landoni (2017). In this paper, we apply these methods, based on the “Shapley value” and the “balance of inequality” respectively, to the Bonferroni inequality index. We also discuss a comparison with the Gini concentration index and highlight interesting properties of the Bonferroni index.

1. Introduction

Carlo Emilio Bonferroni (1930) proposed the inequality index $B$ as an alternative to the Gini index $R$, also referred to as the concentration ratio (Gini 1914). For about half a century, $B$ remained almost forgotten because it was ostracized by Corrado Gini and his followers, who tried to prevent any measures of inequality from overshadowing the concentration ratio $R$ (Giorgi 1998). De Vergottini (1950) proposed an interesting and general formula that nests Bonferroni and Gini indices as special cases.

In the last two decades, B has been revalued and studied for its interesting features. Piesch (1975) and Nygård and Sandström (1981) were the first to investigate $B$ in depth. New and interesting interpretations and extensions of $B$ have been just recently proposed: its welfare implications have been studied by Benedetti (1986), Aaberge (2000), Chakravarty (2007) and Bárcena-Martin and Silber (2013). Giorgi and Crescenzi (2001c) proposed a poverty measure based on $B$, while other socio-economic aspects have been studied by Bárcena-Martin and Imedio Olmedo (2008), Silber and Son (2010), Bárcena-Martin and Silber (2011, 2013), and Imedio Olmedo et al. (2012). The Bonferroni index has also been investigated in fuzzy and reliability frameworks (Giordani and Giorgi 2010; Giorgi and Crescenzi 2001b) and, in particular cases, a Bayesian estimation is followed (Giorgi and Crescenzi 2001a).

An important topic in the literature on inequality measures entails their decomposability. Many contributions are related to the decomposition of $R$ (for a deep investigation see, e.g., Kakwani 1980; Nygård and Sandström 1981; Giorgi 2011a). Tarsitano (1990) introduced several standard results that can be used for the decomposition of $B$, while Bárcena-Martin and Silber (2013) derived an algorithm that greatly simplifies such a decomposition.

In this field, two main lines of research can be distinguished: decomposition by income sources and by population subgroups. The former has been widely treated, while less attention has been paid to the latter (Giorgi 2011a). The reason lies in the difficulties we face when trying to additively decompose (as in the analysis of variance) inequality indices, including $R$ and $B$. To overcome such a drawback when $R$ is entailed, Deutsch and Silber (2007) used the so-called “Shapley value”, while Di Maio and Landoni (2017) suggested the “balance of inequality” ($BOI$).

In the present paper, we detail how the Bonferroni index can be decomposed using these methods. We further discuss interesting similarities and differences between $R$ and $B$ and propose a deeper investigation of some properties of $B$.

The paper is organized as follows: in Section 2, the main properties of Gini and Bonferroni indices are discussed. A brief overview on the inequality indices’ decomposition is given in Section 3, while the so-called “Shapley method” and “balance of inequality” ($BOI$) are detailed in Section 4 and Section 5, respectively. We also extend the $BOI$ to provide a decomposition of $B$. In Section 6, $R$ and $B$ are compared on income data drawn from the 2015 Italian component of the European Survey on Income and Living Conditions (It-SILC). The differences between the two decompositions and the two indices are highlighted in Section 7.

2. The Gini and the Bonferroni Inequality Index

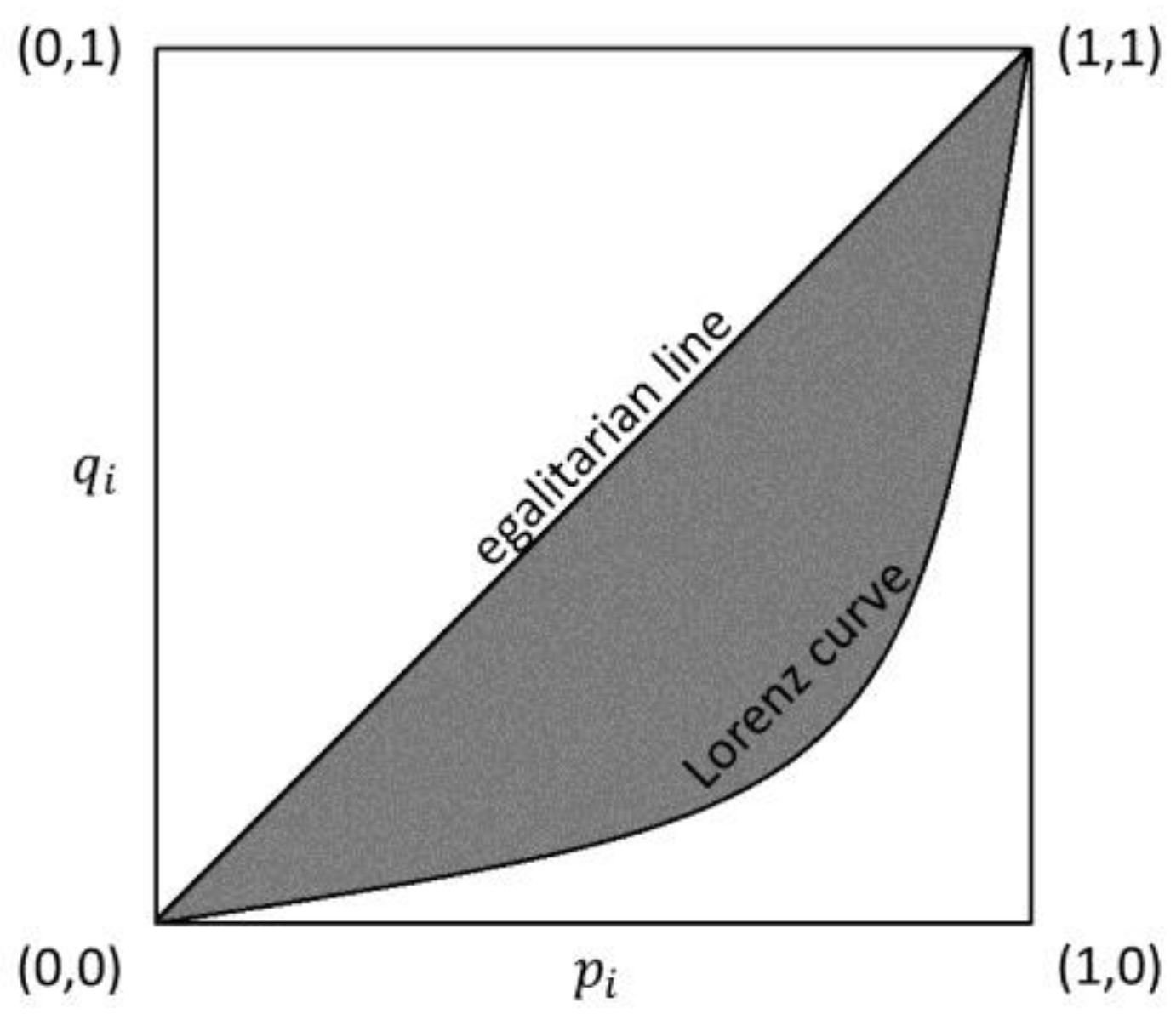
2.1. The Gini Concentration Index

The Gini concentration ratio (Gini 1914), also referred to as the Gini coefficient or the Gini index, is probably the most used index to measure inequality in income distributions. Simplicity, fulfillment of general properties, useful decompositions, the links with the Lorenz curve (Lorenz 1905) and the mean difference (Gini 1912) are just few of the reasons of its widespread use and longevity (see, e.g., Giorgi (1990, 1993, 1998, 1999, 2005, 2011b)).

Among the several ways we may use to define the Gini index (see Giorgi 1992; Yitzhaki 1998), the most useful, for the present purpose, is

$$\begin{array}{}\mathrm{(1)}& \hfill R& =\frac{2{\sum }_{i=1}^N{x}_i(i-1)}{\left(N-1\right)t_x}-1\hfill & & & & 0\le R\le 1\mathrm{(2)}& & =\frac{2{\sum }_{i=1}^{N}i x_i}{\left(N-1\right)t_x}-\frac{\left(N+1\right)}{\left(N-1\right)}\hfill \end{array}$$

where $N$ is the population size, and $i$ is the rank, within the observed population, for the generic recipient, arranged in non-decreasing income values. Furthermore, $x_i$ is the income earned by the $i$-th recipient and $t_x={\sum }_{i=1}^N{x}_i$ is the total income in the whole population.

The Gini concentration index is linked to the Lorenz curve (Figure 1). In the discrete case, the Lorenz curve is the polygonal line connecting points with coordinates given by the cumulative proportion of recipients, arranged in non-decreasing values of income, $p_i=i/N$, and the corresponding share of income, $q_i={\sum }_{j=1}^i{x}_j/t_x$. In the case of perfect equality, the Lorenz curve corresponds to the egalitarian line. In the case of maximum concentration, the Lorenz curve is defined by linking coordinate points (0,0), ($\frac{N-1}{N}$,0), (1,1).

The Gini concentration index is equal to the ratio between the Lorenz area—the area between the Lorenz curve and the egalitarian line—and the Lorenz area in case of maximum concentration—the area of the triangle defined by the points (0,0), ($\frac{N-1}{N}$,0), (1,1), (Nygård and Sandström 1981, pp. 266–71). As $N$ goes to infinity, the quantity $\frac{N-1}{N}$ goes to 1 and the Lorenz area in the case of maximum concentration approaches $1/2$. Then, $R$ is twice the area between the Lorenz curve and the egalitarian line (Nygård and Sandström 1981, p. 240).

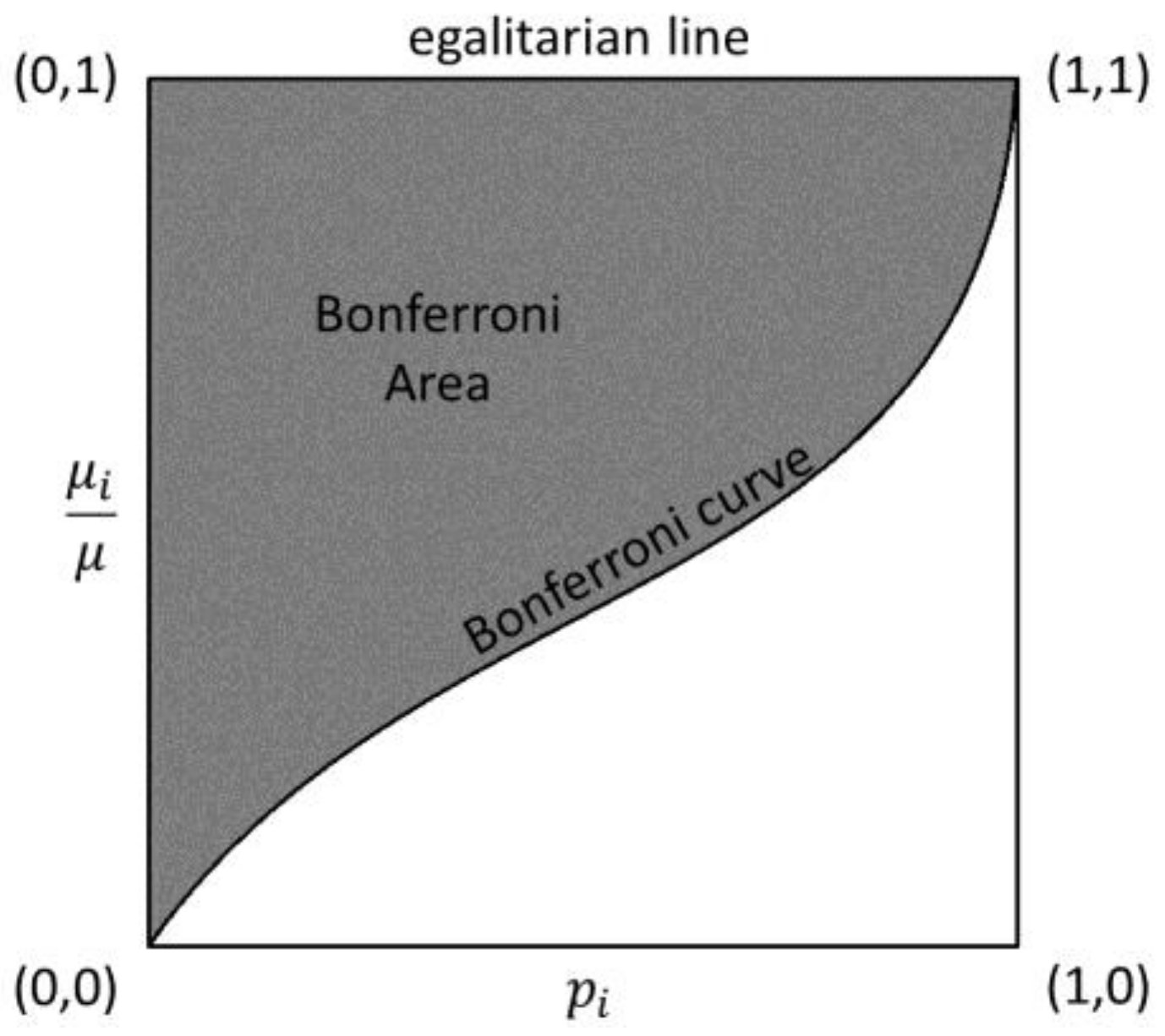
2.2. The Bonferroni Inequality Index

Bonferroni (1930) defined the inequality index as a function of partial means:

$$B=\frac{1}{N-1}{\displaystyle \sum }_{i=1}^N\frac{(\mu -{\mu }_i)}{\mu },$$

(3)

where $0\le B\le 1$, and

$$\mu =\frac{1}{N}{\displaystyle \sum }_{i=1}^N{x}_i{\mu }_i=\frac{1}{i}{\displaystyle \sum }_{j=1}^i{x}_{j}i=1,2,\dots ,N$$

denote the general and the partial means for units sorted in non-decreasing order with respect to the variable of interest, $X$.1

The $B$ index gives a higher weight to units with lower income (see, e.g., De Vergottini 1950, pp. 318–19; Pizzetti 1951, p. 302). For this reason, $B$ is more sensitive to lower levels in the distribution (see, e.g., Giorgi and Mondani 1995).

The Bonferroni index is linked to the Bonferroni curve (Figure 2) which is obtained by plotting the cumulative proportion of recipients, arranged in non-decreasing values of income, versus the corresponding ratio between partial mean and total mean (${\mu }_i/\mu$).

The polygonal line joining the points ($p_i$,${\mu }_i/\mu$) is the Bonferroni curve. If all the recipients in the population have the same income (i.e., equal to $\mu$), the Bonferroni curve coincides with the egalitarian line that joins the coordinate points (0,1), (1,$1$). If just a recipient owns the total amount of $X$, the Bonferroni curve is the broken line joining the points (0,0), ($\frac{N-1}{N}$,0), ($\frac{N-1}{N}$,1).

The value of the Bonferroni index is equal to the ratio between the Bonferroni area—the area between the Bonferroni curve and the egalitarian line—and the Bonferroni area in the case of maximum concentration—the area of the quadrangle defined by the points (0,0), ($\frac{N-1}{N}$,0), ($\frac{N-1}{N},$1), (0,1). As $N$ goes to infinity, the quantity $\frac{N-1}{N}$ goes to 1 and the Bonferroni area in case of maximum concentration is equal to $1$. Then, the value of $B$ coincides with the Bonferroni area (Giorgi and Crescenzi 2001b, pp. 572–73).

3. A Brief Overview on Inequality Index Decomposition

When we consider the decomposition of inequality indices, two main lines of research can be distinguished: decomposition by income sources and by population subgroups (for a comprehensive survey on the subject see, e.g., Giorgi 2011a).

The decomposition by income sources is based on the hypothesis that the total income is the sum of several components, such as wages, salaries, capital incomes, etc. Therefore, the contribution of each source to the overall inequality can be identified. The decomposition by income sources is appealing since the inequality indices can be exactly decomposed into separate components, each one referring to a given factor. Fields (1979a, 1979b) derived the contribution of each source to $R$ via so called Factor Inequality Weight ($FIW$). With slight changes, this method can be adapted to decompose $B$ as follows:

$$B={\displaystyle \sum }_{j=1}^k{h}_j{w}_j{B}_j$$

where ${\mu }_j$, $h_j={\mu }_j/\mu$ and $B_j$ are, respectively, the mean income, the share and the value of $B$ computed for the $j$-th factor (see, e.g., Tarsitano 1990, p. 236). The $w_j$ is the weight of the $j$-th source which can be referred to as the Bonferroni correlation. In fact, it has the same meaning of the Gini correlation in $FIW$ decomposition. The Bonferroni correlation reflects the degree of concordance between the log-rank ordering of units with respect to the $j$-th income source and the corresponding log-rank order for the total income. In other words, the overall inequality, measured through $B$, depends on the degree of inequality in the distribution of each factor ($B_j$), the importance of the factor on the total income ($h_j$) and the amount of agreement between the different rankings ($w_j$).

The decomposition by population subgroups aims at exploring the contribution of individual features such as age, sex, level of education, geographical area, etc., to total inequality (for a deeper investigation on this topic see Deutsch and Silber 1999; Mussard et al. 2006). A first attempt has been proposed by Bhattacharya and Mahalanobis (1967), who tried to decompose $R$ by subgroups via an approach based on the analysis of variance. However, $R$ cannot be additively decomposed into the sum of between and within components. Mehran (1975) showed that $R$ can be decomposed into the sum of within and across components. The difference between the across and the between components is in the interaction component; this is “a measure of the extent of income domination of one group over the other apart from the differences between their mean incomes” (see also Ferrari and Rigo 1987).

As the concentration ratio $R$, the Bonferroni index $B$ can be completely decomposed by the sum of three terms:

$$B=B_w+B_b+B_i$$

(4)

where $B_w$ is the within component, $B_b$ is the between component and $B_i$ is the interaction component that accounts for the degree of overlap between the income distributions in the different subgroups (for this reason it is also referred to as the overlapping component)2. Therefore, also $B$ cannot be additively decomposed (see, e.g., Shorrocks 1980).

4. The Shapley Decomposition

Deutsch and Silber (2007) used the Shapley decomposition introduced, in this field, by Shorrocks (1999), to solve the problem of additive decomposition of $R$ by population subgroups. They derived the impact of four components: inequality within subgroups ($w$), inequality between subgroups ($b$), ranking ($r$) and relative size in each subgroup ($n$).

The Shapley decomposition is based on the well-known concept of Shapley value in cooperative game theory (Shapley 1953). The idea of the Shapley value is to compute the value of a function considering all the possible combinations of factors. When such a decomposition is applied to inequality indices, and the factors are considered as symmetrical, it allows to derive the expected marginal contribution of each factor to inequality. Moreover, the contributions sum exactly to the amount of the inequality index considered (Shorrocks (1999, 2013)). To decompose $B$, we consider the same factors (i.e., $w$, $b$, $r$, $n$), used by Deutsch and Silber (2007) for $R$.

Let us assume that a population $P$ is partitioned into $J$ subgroups $s_j$ ($j=1,\dots ,J$) where $x_{ji}$ is the income of the $i$-th recipient ($i=1,\dots ,N_j$) in subgroup $j=1,\dots ,J$. A given inequality measure $I$ (for instance, $R$ or $B$) can be seen as a function of the observed incomes, $I=f\left(x_{11},\dots , x_{1N_1}, \dots ,x_{j1},\dots ,x_{j{N}_j},\dots ,x_{J1},\dots ,x_{J{N}_J}\right)$.

In the general case, we may consider within subgroups inequality ($x_{ji}\ne {\mu }_j$), between subgroups inequality (${\mu }_j\ne \mu$) and differences in both the size among the subgroups ($f_j\ne 1/J$, where $f_j=\frac{N_j}{N}$) and the rank of recipients $\left(r=r_{ij}\right)$. Therefore, the overall inequality can be written as a function of such factors:

$$I\left(\left(x_{ji}\ne {\mu }_j\right),\left({\mu }_j\ne \mu \right),\left(f_j\ne 1/J\right),\left(r=r_{ij}\right)\right)$$

The Shapley decomposition may help us derive the marginal impact of each factor measuring the difference in the value of the inequality index corresponding to the observed situation and the reference one, where the income does not change with the factor. Just to give an example, the impact of within subgroups inequality ($w$), is derived by comparing the situations where the incomes of recipients in a given subgroup are different ($x_{ji}\ne {\mu }_j$), to the case when all the recipients in that subgroup have the same income ($x_{ji}={\mu }_j)$. To compute the impact of inequality between subgroups $\left(b\right)$, we compare the case when the mean of incomes is different between subgroups (${\mu }_j\ne \mu$) and the case when the average income is constant across subgroups (${\mu }_j=\mu$). To obtain ${\mu }_j=\mu$ a kind of standardization is applied and $x_{ji}$ is replaced by $x_{ji}\frac{\mu }{{\mu }_j}$. To measure the effect of the differences in size ($n$), we compare the case when the subgroups have different sizes ($f_j\ne 1/J$) to the case when the sizes are equal ($f_j=1/J$). To make the subgroups have the same size, the least common multiple (lcm) is calculated for the sizes of the analyzed subgroups and the values $x_{ji}$ are repeated lcm times; this leads to equality in size between the subgroups. When applying such an approach to $B$, the objection is usually raised that $B$, as opposed to $R$, does not satisfy the Dalton (1925) principle of being replication invariant. However, using the simulation study reported in Appendix A, we may show that the effect of replications becomes negligible for $B$ when the population size is greater than 1000 units. According to this feature, $B$ can be defined as being an ‘asymptotically replication invariant’.

Finally, to derive the effect of ranking ($r$), we compare the case when the recipients are sorted by their income $\left(r=r_{ij}\right)$ to the case when we first sort the subgroups on the basis of their average income, ${\mu }_j$, and then the recipients by their income within each subgroup $\left(r=r_{ji}\right)$.

The marginal impact ($SV$) of each factor on the generic index $I$ (either $R$ or $B$) can be derived by computing the following weighted means of the index when, from time to time, the effect of components is removed.

$$\begin{array}{cc}\hfill S{V}_w=\frac{1}{4}\left(I-I_w\right)& +\frac{1}{12}\left[\left(I_b-I_{wb}\right)+\left(I_n-I_{wn}\right)+\left(I_r-I_{wr}\right)\right]\hfill \\ & +\frac{1}{12}\left[\left(I_{bn}-I_{wbn}\right)+\left(I_{br}-I_{wbr}\right)+\left(I_{rn}-I_{wrn}\right)\right]+\frac{1}{4}\left(I_{bnr}-I_{wbnr}\right)\hfill \end{array}$$

(5)

$$\begin{array}{cc}\hfill S{V}_b=\frac{1}{4}\left(I-I_b\right)& +\frac{1}{12}\left[\left(I_w-I_{wb}\right)+\left(I_n-I_{bn}\right)+\left(I_r-I_{br}\right)\right]S{V}_b\hfill \\ & +\frac{1}{12}\left[\left(I_{wn}-I_{wbn}\right)+\left(I_{wr}-I_{wbr}\right)+\left(I_{rn}-I_{bnr}\right)\right]+\frac{1}{4}\left(I_{wnr}-I_{wbnr}\right)\hfill \end{array}$$

(6)

$$\begin{array}{cc}\hfill S{V}_n=\frac{1}{4}\left(I-I_n\right)& +\frac{1}{12}\left[\left(I_w-I_{wn}\right)+\left(I_b-I_{bn}\right)+\left(I_r-I_{nr}\right)\right]S{V}_n\hfill \\ & +\frac{1}{12}\left[\left(I_{wb}-I_{wbn}\right)+\left(I_{wr}-I_{wnr}\right)+\left(I_{br}-I_{bnr}\right)\right]+\frac{1}{4}\left(I_{wbr}-I_{wbnr}\right)\hfill \end{array}$$

(7)

$$\begin{array}{cc}\hfill S{V}_r=\frac{1}{4}\left(I-I_r\right)& +\frac{1}{12}\left[\left(I_w-I_{wr}\right)+\left(I_b-I_{br}\right)+\left(I_n-I_{nr}\right)\right]S{V}_r\hfill \\ & +\frac{1}{12}\left[\left(I_{wb}-I_{wbr}\right)+\left(I_{wn}-I_{wnr}\right)+\left(I_{bn}-I_{bnr}\right)\right]+\frac{1}{4}\left(I_{wbn}-I_{wbnr}\right)\hfill \end{array}$$

(8)

In expressions (5)–(8) by the subscript of $I$ we denote the factor that has been removed. For instance, $I_w$ is the index computed when the component of within inequality ($w$) has been removed, that is $I_w=I\left(\left(x_{ji}={\mu }_j\right),\left({\mu }_j\ne \mu \right),\left(f_j\ne 1/J\right),\left(r=r_{ij}\right)\right)$. Furthermore, $I_{wbr}= I\left(\left(x_{ji}={\mu }_j\right),\left({\mu }_j=\mu \right),\left(f_j\ne 1/J\right),\left(r=r_{ji}\right)\right)$ is the index computed when component of within inequality ($w$), between inequality ($b$) have been removed and the recipients are ranked first by the average income of the subgroup they belong and then with respect to their income3.

A Numerical Illustration

To illustrate, we consider a population composed by 10 recipients with income 2, 6, 10, 18, 20, 25, 30, 50, 55, and 84. Let us assume that recipients with income 2, 6 and 25 belong to subgroup A, those with income 10, 20 and 84 to subgroup B and, last, those with income 18, 30, 50 and 55 to subgroup C.

Since this is just an illustrative example of the application of the Shapley decomposition, the replication invariance principle is overlooked. We should remark that, in some cases, when we remove $w$, the corresponding value of $B$ can be negative. It occurs when there is a negative correlation between mean income and mean rank (Frick and Jan 2007, p. 10). In fact, in these extreme cases, when sorting the income distribution in decreasing order, as shown by Rao (1969, p. 245), $R$ is equal to $-R$, and the same occurs for $B$.

Table 1. Gini concentration ratio ($R$) and Bonferroni inequality index ($B$) in different scenarios according to factors that have been removed. Illustrative example on the income of 10 recipients from three different subgroups: A = {2, 6, 25} and B = {10, 20, 84}, C = {18, 30, 50, 55}.

Removed Factor		Income Distribution	$\mathit{I}$
			$\mathit{R}$	$\mathit{B}$
1	$-$	2, 6, 10, 18, 20, 25, 30, 50, 55, 84	0.490	0.609
2	$w$	11, 11, 38, 38.25, 38, 11, 38.25, 38.25, 38.25, 38	0.151	0.252
3	$b$	5.45, 16.36, 7.89, 14.12, 15.79, 68.18, 23.53, 39.22, 43.14, 66.32	0.360	0.475
4	$n$	2, 2, 2, 2, 6, 6, 6, 6, 10, 10, 10, 10, 18, 18, 18, 20, 20, 20, 20, 25, 25, 25, 25, 30, 30, 30, 50, 50, 50, 55, 55, 55, 84, 84, 84, 84	0.474	0.612
5	$r$	2, 6, 25, 10, 20, 84, 18, 30, 50, 55	0.333	0.481
6	$wb$	30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30	0.000	0.000
7	$wn$	11, 11, 11, 11, 11, 11, 11, 11, 11, 38, 38, 38, 38.25, 38.25, 38.25 38, 38, 38, 38, 11, 11, 11, 11, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38, 38, 38	0.167	0.283
8	$wr$	11, 11, 11, 38, 38, 38, 38.25, 38.25, 38.25, 38.25	0.212	0.331
9	$bn$	5.45, 5.45, 5.45, 5.45, 16.36, 16.36, 16.36, 16.36, 27.27, 7.89, 7.89, 7.89, 14.12, 14.12, 14.12, 15.79, 15.79, 15.79, 15.79, 68.18, 68.18, 68.18, 68.18, 23.53, 23.53, 23.53, 39.22, 39.22, 39.22, 43.14, 43.14, 43.14, 65.88, 66.32, 66.32, 66.32	0.334	0.466
10	$br$	5.45, 16.36, 68.18, 7.89, 15.79, 66.32, 14.12, 23.53, 39.22, 43.14	0.128	0.233
11	$nr$	2, 2, 2, 2, 6, 6, 6, 6, 25, 25, 25, 25, 10, 10, 10, 10, 20, 20, 20, 20, 84, 84, 84, 84, 18, 18, 18, 30, 30, 30, 50, 50, 50, 55, 55, 55	0.331	0.501
12	$wbn$	30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30	0.000	0.000
13	$wbr$	30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30	0.000	0.000
14	$wnr$	11, 11, 11, 11, 11, 11, 11, 11, 11, 11, 11, 11, 38, 38, 38, 38, 38, 38, 38, 38, 38, 38, 38, 38, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25, 38.25	0.214	0.343
15	$bnr$	5.45, 5.45, 5.45, 5.45, 16.36, 16.36, 16.36, 16.36, 68.18, 68.18, 68.18, 68.18, 7.89, 7.89, 7.89, 7.89, 15.79, 15.79, 15.79, 15.79, 66.32, 66.32, 66.32, 66.32, 14.12, 14.12, 14.12, 23.53, 23.53, 23.53, 39.22, 39.22, 39.22, 43.14, 43.14, 43.14	0.127	0.259
16	$wbnr$	30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30, 30	0.000	0.000

Note: $w$ = inequality within; $b$ = inequality between; $n$ = size; $r$ = ranking.

shows all the scenarios obtained by removing factors separately, in pairs, in set of three and all together. Furthermore, the corresponding income distribution and the values of $R$ and $B$ are also presented. We report in

Table 2. Marginal impact of factors on Gini concentration ratio ($R$) and Bonferroni inequality index ($B$). Illustrative example on the income of 10 recipients from three different population subgroups: A = {2, 6, 25} and B = {10, 20, 84}, C = {18, 30, 50, 55}.

Factor	Contribution to $\mathit{R}$		Contribution to $\mathit{B}$
	$\mathit{S}\mathit{V}$	%	$\mathit{S}\mathit{V}$	%
within inequality	0.230	47.02	0.305	50.07
between inequality	0.176	35.90	0.244	40.13
size	0.005	1.00	−0.007	−1.22
ranking	0.079	16.07	0.067	11.02
Total	0.490	100.00	0.609	100.00

the marginal contributions for each factor ($SV$) derived using expressions (5)–(8).

5. The Balance of Inequality Approach

The Balance of Inequality, proposed by Di Maio and Landoni (2017), is an alternative approach that, as in the Shapley method, helps solve the problem of additive decomposition of $R$ by population subgroups. They use the center mass (or barycenter) to derive a measure of inequality, thus giving a physical interpretation to the inequality measure.

The barycenter of the income distribution, in which in abscissa is the income $x_i$ and in ordinate is the ranking minus one $\left(i-1\right)$, is defined by the following expression

$${}_R{}^{}b=\frac{{\sum }_{i=1}^N{x}_i\left(i-1\right)}{{\sum }_{i=1}^N{x}_i} .$$

It is equal to $\left(N-1\right)/2$ in the case of perfect equality, while it is equal to $N-1$ in the case of maximum inequality. Di Maio and Landoni (2017, p. 12) proceeded to normalize the barycenter and obtained, after a little algebra, the $BOI$:

$$BOI=\frac{\frac{{\sum }_{i=1}^N{x}_i\left(i-1\right)}{{\sum }_{i=1}^N{x}_i}-\frac{N-1}{2}}{\left(N-1\right)-\frac{N-1}{2}}$$

$$=\frac{2{\sum }_{i=1}^{N}i x_i}{t_x\left(N-1\right)}-\frac{N+1}{N-1}= {}_R{}^{}BOI.$$

(9)

Expression (9) corresponds to the Gini concentration index (1) and, for this reason, we will refer to expression (9) as ${}_R{}^{}BOI$ in the following. They show that ${}_R{}^{}BOI$ (9), and therefore the Gini concentration ratio, can be decomposed by considering four factors. Besides those already seen in the previous paragraph—the inequality within and between population subgroups—$BOI$ helps derive the impact on the inequality value due to asymmetry and irregularity of subgroups4. A population (or a subgroup) is symmetrical if the distribution of the analyzed variable is symmetrical with respect to its center. Furthermore, it is regular if the distance between two adjacent individuals in the population or in the subgroup is constant. A regular population (or a subgroup) is also symmetrical.

The ${}_R{}^{}BOI$ for the Gini index can therefore be decomposed as

$${}_R{}^{}BOI={\displaystyle \sum }_{j=1}^J\frac{t_x{}_j}{t_x}\left[\frac{{}_R{}^{}b{}_j^{*1}-{}_R{}^{}b{}_j^{*0}}{\frac{N-1}{2}}\right]{}_R{}^{}BO{I}_j+\left({\displaystyle \sum }_{j=1}^J\frac{t_x{}_j}{t_x}\left[\frac{{}_R{}^{}b{}_j^{*0}}{\frac{N-1}{2}}\right]\right)-1$$

$$+{\displaystyle \sum }_{j=1}^J\frac{t_x{}_j}{t_x}\left[\frac{{}_R{}^{}b{}_j^{*1}-{}_R{}^{}b{}_j^{*0}}{\frac{N-1}{2}}\right]\left({}_R{}^{}A{E}_j\right)+{\displaystyle \sum }_{j=1}^J\frac{t_x{}_j}{t_x}\left[\frac{{}_R{}^{}b{}_j^{*1}-{}_R{}^{}b{}_j^{*0}}{\frac{N-1}{2}}\right]\left({}_R{}^{}I{E}_j\right)$$

(10)

where $t_x{}_j$ is the total income in the $j$-th subgroup; equivalently, we may define the $BOI$ in the $j$-th subgroup via the following expression

$${}_R{}^{}BO{I}_j=\frac{2{\sum }_{iϵs_j}k x_i}{t_x \left(N_j-1\right)}-\frac{N_j+1}{N_j-1}$$

where $k$ is the rank of the $i$-th recipient in subgroup $j$. Furthermore, ${}_R{}^{}b{}_j^{*1}=\max i\in s_j-1$ is the barycenter of the subgroup in the population in case of perfect inequality, and ${}_R{}^{}b{}_j^{*0}=\frac{1}{N_j}{\displaystyle \sum }_{i \in s_j}^{}\left(i-1\right)$ is the barycenter of the subgroup in the population in the case of perfect equality. In this context, ${}_R{}^{}A{E}_j={}_R{}^{}BO{I}_j^{*}-{}_R{}^{}BO{I}_{j sym}^{*}$ represents the asymmetry effect and ${}_R{}^{}I{E}_j={}_R{}^{}BO{I}_{j sym}^{*}-{}_R{}^{}BO{I}_j$ the irregularity effect where

$${}_R{}^{}BO{I}_j^{*}=\frac{\frac{1}{t_{x_j}}{{\displaystyle \sum }}_{i ϵ s_j }i x_i-\frac{1}{N_j}{{\displaystyle \sum }}_{i ϵ s_j }i}{\max i\in s_j-\frac{1}{N_j}{{\displaystyle \sum }}_{i ϵ s_j }i}$$

is the ${}_R{}^{}BOI$ index for the $j$-th subgroup, while

$${}_R{}^{}BO{I}_{j sym}^{*}=\frac{2}{t_{x_j}\left(\max i\in s_j-\min i\in s_j\right)}{\displaystyle \sum }_{i ϵ s_j}\left(i-\frac{\min i\in s_j+ \max i\in s_j}{2}\right)x_i$$

is the ${}_R{}^{}BOI$ index for the $j$-th subgroup, in the case of symmetrical subgroups.

The first component in expression (10) is the weighted average of the within subgroup inequality, the second is the inequality between subgroups, the third and the fourth are the weighted average of the effects of asymmetry and irregularity of the distribution in each subgroup, respectively.5

The extension of the $BOI$ methodology to the Bonferroni inequality index ($B$) requires that we consider a different representation of the income distribution. Let us consider the couple with the income on the abscissa and $\left(\frac{1-l_i}{\mu }\right)$ on the ordinate, where $l_i={\sum }_{i=1}^N\frac{1}{i}$ and ${\sum }_{i=1}^N{l}_i=N$. The barycenter of this distribution is

$${}_B{}^{}b=\frac{{\sum }_{i=1}^N{x}_i\left(\frac{1-l_i}{\mu }\right)}{{\sum }_{i=1}^N{x}_i}.$$

It is zero in the case of perfect equality and equal to $\left(N-1\right)/{\mathrm{t}}_x$ in the case of maximum inequality. Normalizing the barycenter, as before, and using a little algebra we obtain the expression of $B$ in expression (3).

$${}_B{}^{}BOI=\frac{{{\displaystyle \sum }}_{i=1}^N{x}_i\left(1-l_i\right)}{\mu \left(N-1\right)} .$$

(11)

This enable us to apply the $BOI$ approach also to $B$. Expression (11) can be written as

$${}_B{}^{}BOI={\displaystyle \sum }_{j=1}^J\frac{t_x{}_j}{t_x}\left[\frac{{}_B{}^{}b{}_j^{*1}-{}_B{}^{}b{}_j^{*0}}{\frac{N-1}{t_x}}\right]{}_B{}^{}BO{I}_j+{\displaystyle \sum }_{j=1}^J\frac{t_x{}_j}{t_x}\left[\frac{{}_B{}^{}b{}_j^{*0}}{\frac{N-1}{t_x}}\right]$$

$$+{\displaystyle \sum }_{j=1}^J\frac{t_x{}_j}{t_x}\left[\frac{{}_B{}^{}b{}_j^{*1}-{}_B{}^{}b{}_j^{*0}}{\frac{N-1}{t_x}}\right]\left({}_B{}^{}A{E}_j\right)+{\displaystyle \sum }_{j=1}^J\frac{t_x{}_j}{t_x}\left[\frac{{}_B{}^{}b{}_j^{*1}-{}_B{}^{}b{}_j^{*0}}{\frac{N-1}{t_x}}\right]\left({}_B{}^{}I{E}_j\right) .$$

Equivalently

$${}_B{}^{}BO{I}_j=\frac{{{\displaystyle \sum }}_{i=1}^N{x}_k\left(1-l_k\right)}{{\mu }_j \left(N_j-1\right)}$$

denotes the $BOI$ for the $j$-th subgroup with size $N_j$, ${\mu }_j={\displaystyle \sum }_{i\in s_j}{x}_k/N_j$, $l_k={\displaystyle \sum }_{k=1}^{N_j}\frac{1}{k}$ and ${\displaystyle \sum }_{k=1}^{N_j}{l}_k=N_j$ where $k$ is the rank of recipients in subgroup $j$. Furthermore,

$${}_B{}^{}b{}_j^{*1}=\left(1-\begin{array}{c}\max \\ i \in s_j\end{array} l_i\right)/\mu ,$$

where $\begin{array}{c}\max \\ i \in s_j\end{array} l_i$ is the value of $l_i$ corresponding to the recipients with the highest income in subgroup $s_j$, and ${}_B{}^{}b{}_j^{*1}$ denotes the barycenter of the subgroup in the population in case of perfect inequality. The barycenter of the subgroup in the case of perfect equality is

$${}_B{}^{}b{}_j^{*0}=\left(1-\frac{{{\displaystyle \sum }}_{i \in s_j }{l}_i}{N_j}\right)/\mu .$$

As above, ${}_B{}^{}A{E}_j={}_B{}^{}BO{I}_j^{*}-{}_B{}^{}BO{I}_{jsym}^{*}$ represents the effect of asymmetry while ${}_B{}^{}I{E}_j={}_B{}^{}BO{I}_{jsym}^{*}-{}_B{}^{}BO{I}_j$ denotes the effect of irregularity on $B$. The $BOI$ index for subgroup $s_j$ is equal to

$${}_B{}^{}BO{I}_j^{*}=\frac{\frac{{{\displaystyle \sum }}_{i \in s_j }{l}_i}{N_j}-\frac{{{\displaystyle \sum }}_{i \in s_j }{x}_i l_i}{t_x{}_j}}{\frac{{{\displaystyle \sum }}_{i \in s_j }{l}_i}{N_j}-\begin{array}{c}\max \\ i \in s_j\end{array} l_i}$$

while the $BOI$ index for a symmetrical subgroup is given by

$${}_B{}^{}BO{I}_{j sym}^{*}=\frac{2}{t_x \left(\begin{array}{c}\min \\ i \in s_j\end{array} l_i-\begin{array}{c}\max \\ i \in s_j\end{array} l_i\right)}{{\displaystyle \sum }}_{i ϵ s_j}\left(\frac{\begin{array}{c}\min \\ i \in s_j\end{array} l_i+\begin{array}{c}\max \\ i \in s_j\end{array} l_i}{2}-l_i\right)x_i .$$

A Numerical Illustration

Let us consider the same population of 10 recipients we have already discussed in Section 4. We report in

Table 3. Balance of inequality decomposition for the Gini concentration ratio ($R$) and the Bonferroni inequality index ($B$). Illustrative example on the income of 10 recipients from three different population subgroups: A = {2, 6, 25} and B = {10, 20, 84}, C = {18, 30, 50, 55}.

Factor	Contribution to $\mathit{R}$		Contribution to $\mathit{B}$
	${}_{\mathit{R}}{}^{}\mathit{B}\mathit{O}\mathit{I}$	%	${}_{\mathit{B}}{}^{}\mathit{B}\mathit{O}\mathit{I}$	%
within inequality	0.256	52.38	0.365	59.95
between inequality	0.151	30.86	0.252	41.42
asymmetry	−0.007	−1.35	−0.025	−4.10
irregularity	0.010	2.05	0.017	2.73
Total	0.490	100.00	0.609	100.00

the contribution of each factor obtained via the balance inequality approach.

By looking at this illustrative example, some preliminary results can be derived. In both cases, the higher contribution to the overall inequality corresponds to the within factor, followed by the between one and other factors. However, we may observe some differences when comparing the current decomposition to the Shapley decomposition in Table 2. The impact of between inequality on $R$ is lower when measured with the $BOI$ (30.86% vs. 35.90%), while the impact of within inequality is higher (52.38% vs. 47.02%). On the other hand, when we consider $B$, the values of between inequality are very similar (41.42% vs. 40.13%), while the difference for the within inequality is substantial (59.95% vs. 50.07%). For both indices, asymmetry reduces inequality: this issue is more evident when looking at the decomposition of $R$ rather than the one of $B$.

6. An Application to the Italian Income Distribution

The Shapley decomposition of the Gini concentration ratio ($R$) and the Bonferroni index ($B$) has been applied to income data collected in 2015 by the Italian component of the European Survey on Income and Living Conditions (It-SILC, Istat 2015). The Eu-SILC is a yearly survey carried out by European countries according to the European Regulation n. 1177/2003. Its main aim is to provide data on income, poverty and social exclusion. The 2015 Italian sample is a two-stage sample of municipalities, stratified by population size, and households. The sample size is composed by 17,985 household and 36,602 individuals.

We consider the Italian households as divided into three subgroups, represented by the main geographical areas: North, Center and South.

Table 4. Some explanatory statistics on average Italian household income distribution by three subgroups (North, Center and South). Source: It-SILC, Italy 2015.

Geographical Area	Households		First Quartile	Median	Mean	Third Quartile	Fisher Asymmetry Coefficient	R	B
	Sample Size	Population Size
North	8922	12,294,699	25,809	39,180	47,621	59,749	4.273	0.346	0.439
Center	4223	5,295,623	23,114	36,459	44,626	56,524	2.379	0.360	0.457
South	4840	8,185,550	16,939	26,617	32,561	40,400	10.861	0.372	0.482
Italy	17,985	25,775,872	22,007	34,199	42,223	53,480	5.143	0.367	0.462

shows some explanatory statistics on the distribution of household income for the whole population and the subgroups.

The inequality measures have been computed on the distribution of household income. The incomes have not been equivalized to account for the different households’ size. The values of $R$ have been estimated using the expression of the sampling estimator defined by Osier (2009, p. 169), while $B$ has been estimated using the expression of the sampling estimator derived in Giorgi and Guandalini (2013, p. 154). The $BOI$ values, for $R$ and $B$, have been computed through a plug-in estimator.

Looking at Table 4, we observe that $R$= 0.367 and $B$= 0.462 for the whole population. North and Center have quite a similar situation. While in South the incomes are lower, and the inequality is higher. In the three subgroups, but also at the national level, there is a strong positive asymmetry in the income distribution. The asymmetry is greater in the South when compared to the other geographical areas.

In

Table 5. Shapley and Balance of inequality decompositions for the Gini concentration ratio ($R$) and the Bonferroni inequality index ($B$). Application to Italian household income distribution by three population subgroups (North, Center and South). Source: It-SILC, Italy 2015.

Factor	Contribution to $\mathit{R}$		Contribution to $\mathit{B}$
	Absolute Value	%	Absolute Value	%
	Shapley decomposition
within inequality	0.2348	63.99	0.3065	66.30
	[0.2281, 0.2415]	[62.16, 65.80]	[0.2956, 0.3174]	[63.94, 68.66]
between inequality	0.0530	14.44	0.0626	13.55
	[0.0439, 0.0621]	[11.95, 16.93]	[0.0522, 0.0730]	[11.28, 15.80]
size	−0.0002	−0.05	0.0090	1.95
	[−0.0037, 0.0033]	[−1.00, 0.89]	[0.0046, 0.0134]	[0.99, 2.90]
ranking	0.0793	21.62	0.0841	18.20
	[0.0700, 0.0886]	[19.08, 24.13]	[0.0757, 0.0925]	[16.37, 20.01]
Total	0.3670	100.00	0.4623	100.00
	[0.3568, 0.3772]		[0.4505, 0.4741]
	Balance of Inequality ($BOI$)
within inequality	0.3483	94.98	0.4007	81.07
	[0.3384, 0.3582]	[94.89, 95.02]	[0.3908, 0.4106]	[79.07, 83.09]
between inequality	0.0239	6.54	0.0385	7.79
	[0.0182, 0.0296]	[5.11, 7.85]	[0.0293, 0.0477]	[6.05, 9.65]
asymmetry	0.0020	0.55	0.4405	89.13
	[0.0008, 0.0032]	[0.24, 0.84]	[0.4393, 0.4417]	[90.78, 89.36]
irregularity	−0.0076	−2.07	−0.3855	−78.00
	[−0.0095, −0.0057]	[−2.65, −1.52]	[−0.3874, −0.3836]	[−80.04, −77.62]
Total	0.3668	100.00	0.4942	100.00
	[0.3566, 0.3770]		[0.3908, 0.4106]

Note: Bootstrap confidence interval at 95% in squared brackets.

, $R$ has been decomposed using the Shapley decomposition (as shown in Section 4) and the balance of inequality (as shown in Section 5). As for the Shapley decomposition, it is important to point out that the sample size for all the subgroups is larger than 4000; therefore, the Dalton principle of replication invariance can be considered as (at least approximately) satisfied also for $B$.

The impact of the different factors obtained by the decomposition methods are reported in Table 5. For each component and decomposition, the corresponding confidence interval, estimated via nonparametric bootstrap ($M$= 500 samples), are reported.

The plug-in estimators based on $BOI$ are biased. The bias is negligible for ${}_R{}^{}BOI$, while it is more evident for ${}_B{}^{}BOI$. However, this does not affect the comparison between the decomposition methods and the two indices, since, in any case, the bias does not change the balance of power between factors considered obtained via the balance of inequality.

Both decompositions identify the within inequality as a very important factor. Under the Shapley decomposition, it accounts for more than 60% of the whole inequality, both for $R$ and $B$. Ranking is more important than between inequality (20% versus 14%), since the subgroups are strongly overlapped. Finally, subgroup size plays a minor role. Under the Shapley decomposition, the magnitude of factors is similar for both the analyzed indices. However, when we consider $B$ the impact of within inequality is higher while that of ranking is lower than for $R$. The importance of differences among subgroups in size is negligible when we consider $R$, since it is population size independent, while the same is different from zero in $B$, even if not that high.

Under the $BOI$ decomposition, within inequality accounts for more than 80% of the whole inequality for both the analyzed indices, even if its role is slightly more evident in $R$. Unlike the Shapley decomposition, the two indices show a different “hierarchy” of factors when we look at the corresponding impact. When we consider $R$, the most important factor is the within inequality followed by the between inequality. The contribution of asymmetry and irregularity is almost negligible. On the contrary, when we look at $B$, the asymmetry is the most important factor followed by the within inequality and the irregularity (with a negative sign). Between inequality plays a minor role.

It is important to point out that the combined effect of asymmetry and irregularity has opposite signs on the two indices (0.55 − 2.07 = −1.52% for $R$ and 89.13 − 78.00 = 11.13% for $B$). This is probably due to the indices’ sensitivity to different levels of the income distribution. As remarked above, $B$ is more sensitive to lower values (left tail of the distribution), while $R$ is more sensitive to the central values of the distribution. Moreover, the high value for the impact of asymmetry and irregularity when we consider $B$ can be due to the asymmetry in the income distribution, as already stated, but also to an indirect effect of population size. In fact, as opposed to the numerical illustration in Section 5, the contribution of asymmetry and irregularity to $B$ is higher in the It-SILC data due to the larger population size and asymmetry.

The two decomposition methods are deeply different. The Shapley decomposition represents a more general tool which can be used to decompose not only inequality measures and not only by the four factors we have considered here. It can be modified by considering a different (lower or higher) number of factors. The $BOI$ is more similar to a standard decomposition approach, since it is less customizable. In fact it is possible to decompose the index by within inequality, between inequality, asymmetry and irregularity only.

Some Considerations on the Shapley Decomposition and the Balance of Inequality

The numerical examples and the application to real data show that the two decomposition methods point out different aspects of the inequality indices. The Shapley decomposition is more sensitive to the ranking in the income distribution, while the $BOI$ decomposition is more influenced by the shape of the distribution.

Looking at the behavior of the two indices with respect to the adopted decomposition, it is possible to draw some interesting conclusions. Since $R$ and $B$ adopt a similar ranking system, we cannot observe substantial differences when considering the Shapley decomposition; however, since the indices have different sensitivity to different portions of the distribution, asymmetry and irregularity often play a crucial role in the $BOI$ decomposition, and this may lead to different results.

Finally, as using more synthetic indices can help us highlight differences between socio-economic reality and political significance of inequality (Piketty 2014, p. 156); using more than one decomposition may help focus on different aspects and factors of inequality.

7. Conclusions and Further Research

An important topic on inequality measures is their decomposability. Two main lines of research can be identified: decomposition by income sources and by population subgroups. Some indices, such as the Gini concentration ratio $R$ (Gini 1914) and the Bonferroni inequality index $B$ (Bonferroni 1930) are not additively decomposable by population subgroups. To overcome this drawback, Deutsch and Silber (2007) proposed the so-called “Shapley value”, and Di Maio and Landoni (2017) suggested the “balance of inequality” ($BOI$) approach to decompose the Gini concentration ratio ($R$).

In this paper, we have discussed the Shapley decomposition for the Bonferroni inequality index ($B$). Furthermore, we also show how the balance of inequality can be extended to $B$. The two indices have been estimated on real data from the 2015 Italian component of the European Survey on Income and Living Conditions (It-SILC) and the two decomposition methods have been considered in this context.

The results of the application highlights that the features of each subpopulation, such as homogeneity within (denoted by the component of within inequality), and the difference in subpopulation size, have higher influence on $B$ than on $R$. Furthermore, $B$ seems to be more sensitive to asymmetry and irregularity in the observed distribution and the population size.

The two decomposition methods focus on different aspects of the distribution. The Shapley value reflects the ranking in the income distribution, while the $BOI$ is mainly influenced by the shape of the distribution. For these reasons, the two indices have a similar behavior under the Shapley decomposition, as their ranking system is similar, while they may show a completely different “hierarchy” of factors under the balance of inequality decomposition.

The results of our research also suggest the possibility of supplementing the measure of overall inequality through indices with different sensitivity to different parts of the income distribution, trying to answer, at least in part, the possible disadvantages in using a single index (Osberg 2017). This follows, in our view, the path suggested by Piketty (2014, p. 156). Piketty proposed to use different indices to account for the differences between socio-economic reality and political significance of inequality in different parts of the income distribution. In the same way, the use of different kinds of decompositions can help to focus on different aspects and factors of inequality. In this perspective, further studies could focus on the extension of the $BOI$ approach to other indices.