From the Classical Gini Index of Income Inequality to a New Zenga-Type Relative Measure of Risk: A Modeller’s Perspective
Abstract
1. Introduction
- societal references such as the population mean, median, or some population distribution-tail based measures, and
- distributions of random personal positions, or gambles, that determine person’s position on a certain population-based function.
This remark leads us towards the use of what we call relative-value functions, which, as we shall see later in this paper, offer a flexible way for coupling fundamental measures of economic inequality, or risk, with appropriate reference points, such as the mean (e.g., Equation (7) below). This is very much in the spirit of Definition 3 by Cowell (2003). We shall come back to the latter work in the second half of Section 4.In particular, Amiel and Cowell (1997, 1999) find evidence that “the appropriate inequality equivalence concept depends on the income levels at which inequality comparisons are made.” Moreover, they show that, as income increases, the equivalence concept moves from the relative attitude to the absolute one, a pattern consistent with our intuition.(Zoli 2012, p. 4)
2. The Classical Gini Index Revisited
3. From Egalitarian Lorenz to the Mean Reference
4. From the Mean to Generic Societal References
5. The Donaldson-Weymark-Kakwani Index Revisited and Extended
- (H)
- Let be any twice differentiable and convex function (i.e., for all ) that satisfies the boundary conditions and , and such that .
6. The Wang Risk Measure Revisited and Extended
- (G)
- Let be twice differentiable and concave function (i.e., for all ) that satisfies the boundary conditions and , and such that .
7. From Collective to Individual References
- The quantile is not robust with respect to realized values p of the random gamble , in the sense that the quantile may change drastically even for very small changes of p.
- For a realized value p of , the quantile is not informative about the values of for . Indeed, we may have the same value of irrespective of whether the cdf F is heavy- or light-tailed.
8. Relative Measure of Risk
- If the risk X is constant, that is, for some constant , then .
- Multiplying X by any constant does not change the relative measure of risk, that is, .
- Adding any constant to the risk X decreases the relative measure of risk, that is, .
9. Conclusions: A General Index of Inequality and Risk
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Technicalities
References
- Aaberge, Rolf. 2000. Characterizations of Lorenz curves and income distributions. Social Choice and Welfare 17: 639–53. [Google Scholar] [CrossRef]
- Alexander, Carol, Gauss M. Cordeiro, Edwin M. M. Ortega, and José María Sarabia. 2012. Generalized beta-generated distributions. Computational Statistics and Data Analysis 56: 1880–97. [Google Scholar] [CrossRef]
- Amiel, Yoram, and Frank A. Cowell. 1997. Income Transformation and Income Inequality. Discussion Paper DARP 24. London, UK: London School of Economics. [Google Scholar]
- Amiel, Yoram, and Frank A. Cowell. 1999. Thinking About Inequality. Cambridge: Cambridge University Press. [Google Scholar]
- Arcagni, Alberto, and Francesco Porro. 2013. On the parameters of Zenga distribution. Statistical Methods and Applications 22: 285–303. [Google Scholar] [CrossRef]
- Arcagni, Alberto, and Michele Zenga. 2013. Application of Zenga’s distribution to a panel survey on household incomes of European Member States. Statistica and Applicazioni 11: 79–102. [Google Scholar]
- Artzner, Philippe, Freddy Delbaen, Jean-Marc Eber, and David Heath. 1999. Coherent measures of risk. Mathematical Finance 9: 203–28. [Google Scholar] [CrossRef]
- Arnold, Barry C. 1987. Majorization and the Lorenz Order: A Brief Introduction. New York: Springer. [Google Scholar]
- Atkinson, Anthony B. 1970. On the measurement of inequality. Journal of Economic Theory 2: 244–63. [Google Scholar] [CrossRef]
- Atkinson, Anthony B., and Francois Bourguignon. 2000. Handbook of Income Distribution. Amsterdam: Elsevier, vol. 1. [Google Scholar]
- Atkinson, Anthony B., and Francois Bourguignon. 2015. Handbook of Income Distribution. Amsterdam: Elsevier, vol. 2. [Google Scholar]
- Atkinson, Anthony B., and Thomas Piketty. 2007. Top Incomes Over the Twentieth Century: A Contrast between Continental European and English-Speaking Countries. Oxford: Oxford University Press. [Google Scholar]
- Banerjee, A.V., and Esther Duflo. 2011. Poor Economics: A Radical Rethinking of the Way to Fight Global Poverty. New York: Public Affairs. [Google Scholar]
- Bennett, Christopher J., and Ricardas Zitikis. 2015. Ignorance, lotteries, and measures of economic inequality. Journal of Economic Inequality 13: 309–16. [Google Scholar] [CrossRef]
- Benedetti, C. 1986. Sulla interpretazione benesseriale di noti indici di concentrazione e di altri. Metron 44: 421–29. [Google Scholar]
- Bonferroni, C. E. 1930. Elementi di Statistica Generale. Firenze: Libreria Seeber. [Google Scholar]
- Brahimi, B., F. Meddi, and A. Necir. 2012. Bias-corrected estimation in distortion risk premiums for heavy-tailed losses. Afrika Statistika 7: 474–90. [Google Scholar] [CrossRef]
- Cannata, F., and M. Quagliariello. 2011. Basel III and Beyond. London: Risk Books. [Google Scholar]
- Ceriani, Lidia, and Paolo Verme. 2012. The origins of the Gini index: Extracts from Variabilità e Mutabilità (1912) by Corrado Gini. Journal of Economic Inequality 10: 421–43. [Google Scholar] [CrossRef]
- Chakravarty, Satya R. 1988. Extended Gini indices of inequality. International Economic Review 29: 147–56. [Google Scholar] [CrossRef]
- Chakravarty, Satya R. 2007. A deprivation-based axiomatic characterization of the absolute Bonferroni index of inequality. Journal of Economic Inequality 5: 339–51. [Google Scholar] [CrossRef]
- Chakravarty, Satya R., and Piero Muliere. 2004. Welfare indicators: A review and new perspectives. 2. Measurement of poverty. Metron 62: 247–81. [Google Scholar]
- Champernowne, D. G., and F. A. Cowell. 1998. Economic Inequality and Income Distribution. Cambridge: Cambridge University Press. [Google Scholar]
- Cobham, Alex, and Andy Sumner. 2013a. Putting the Gini Back in the Bottle? ‘The Palma’ As a Policy-Relevant Measure of Inequality. Working Paper 2013-5. London, UK: King’s College. [Google Scholar]
- Cobham, Alex, and Andy Sumner. 2013b. Is It All about the Tails? The Palma Measure of Income Inequality. Working Paper 343. Washington, DC, USA: Center for Global Development. [Google Scholar]
- Cobham, Alex, and Andy Sumner. 2014. Is inequality all about the tails?: The Palma measure of income inequality. Significance 11: 10–13. [Google Scholar] [CrossRef]
- Cowell, Frank A. 2003. Theil, Inequality and the Structure of Income Distribution. Discussion Paper DARP 67. London, UK: London School of Economics. [Google Scholar]
- Cowell, Frank A. 2011. Measuring Inequality, 3rd ed. Oxford: Oxford University Press. [Google Scholar]
- Cruz, M. 2009. The Solvency II Handbook. London: Risk Books. [Google Scholar]
- Denneberg, Dieter. 1990. Premium calculation: Why standard deviation should be replaced by absolute deviation. ASTIN Bulletin 20: 181–90. [Google Scholar] [CrossRef]
- Denuit, Michel, Jan Dhaene, Marc Goovaerts, and Rob Kaas. 2005. Actuarial Theory for Dependent Risks: Measures, Orders and Models. Chichester: Wiley. [Google Scholar]
- De Vergottini, Mario. 1940. Sul significato di alcuni indici di concentrazione. Giornale degli Economisti e Annali di Economia 11: 317–47. [Google Scholar]
- Donaldson, David, and John A. Weymark. 1980. A single-parameter generalization of the Gini indices of inequality. Journal of Economic Theory 22: 67–86. [Google Scholar] [CrossRef]
- Donaldson, David, and John A. Weymark. 1983. Ethically flexible Gini indices for income distributions in the continuum. Journal of Economic Theory 29: 353–58. [Google Scholar] [CrossRef]
- Druckman, A., and T. Jackson. 2008. Measuring resource inequalities: The concepts and methodology for an area-based Gini coefficient. Ecological Economics 65: 242–52. [Google Scholar] [CrossRef]
- Duclos, Jean-Yves. 2000. Gini indices and the redistribution of income. International Tax and Public Finance 7: 141–62. [Google Scholar] [CrossRef]
- Furman, Edward, Ruodu Wang, and Ricardas Zitikis. 2017. Gini-type measures of risk and variability: Gini shortfall, capital allocations, and heavy-tailed risks. Journal of Business and Finance 83: 70–84. [Google Scholar] [CrossRef]
- Furman, Edward, and Ricardas Zitikis. 2008. Weighted premium calculation principles. Insurance: Mathematics and Economics 42: 459–65. [Google Scholar] [CrossRef]
- Furman, Edward, and Ricardas Zitikis. 2009. Weighted pricing functionals with applications to insurance: An overview. North American Actuarial Journal 13: 483–96. [Google Scholar] [CrossRef]
- Furman, Edward, and Ricardas Zitikis. 2017. Beyond the Pearson correlation: Heavy-tailed risks, weighted Gini correlations, and a Gini-type weighted insurance pricing model. ASTIN Bulletin 47: 919–42. [Google Scholar] [CrossRef]
- Gastwirth, Joseph L. 1971. A general definition of the Lorenz curve. Econometrica 39: 1037–39. [Google Scholar] [CrossRef]
- Gastwirth, Joseph L. 2014. Median-based measures of inequality: Reassessing the increase in income inequality in the U.S. and Sweden. Journal of the IAOS 30: 311–20. [Google Scholar]
- Gini, Corrado. 1912. Variabilità e Mutabilità: Contributo allo Studio delle Distribuzioni e delle Relazioni Statistiche. Bologna: Tipografia di Paolo Cuppini. [Google Scholar]
- Gini, Corrado. 1914. On the measurement of concentration and variability of characters (English translation from Italian by Fulvio de Santis). Metron 63: 3–38. [Google Scholar]
- Gini, Corrado. 1921. Measurement of inequality of incomes. Economic Journal 31: 124–26. [Google Scholar] [CrossRef]
- Giorgi, Giovanni M. 1990. Bibliographic portrait of the Gini concentration ratio. Metron 48: 183–221. [Google Scholar]
- Giorgi, Giovanni M. 1993. A fresh look at the topical interest of the Gini concentration ratio. Metron 51: 83–98. [Google Scholar]
- Giorgi, Giovanni M. 1998. Concentration index, Bonferroni. In Encyclopedia of Statistical Sciences. Edited by S. Kotz, D. L. Banks and C. B. Read. New York: Wiley, vol. 2, pp. 141–46. [Google Scholar]
- Giorgi, Giovanni Maria, and M. Crescenzi. 2001. A look at the Bonferroni inequality measure in a reliability framework. Statistica 91: 571–83. [Google Scholar]
- Giorgi, Giovanni Maria, and Saralees Nadarajah. 2010. Bonferroni and Gini indices for various parametric families of distributions. Metron 68: 23–46. [Google Scholar] [CrossRef]
- Giovagnoli, Alessandra, and Henry P. Wynn. 2012. (U, V)-Ordering and a Duality Theorem for Risk Aversion and Lorenz-Type Orderings. LSE Philosophy Papers. London, UK: London School of Economics and Political Science. [Google Scholar]
- Greselin, Francesca. 2014. More equal and poorer, or richer but more unequal? Economic Quality Control 29: 99–117. [Google Scholar] [CrossRef]
- Greselin, Francesca, Leo Pasquazzi, and Ricardas Zitikis. 2013. Contrasting the Gini and Zenga indices of economic inequality. Journal of Applied Statistics 40: 282–97. [Google Scholar] [CrossRef]
- Greselin, Francesca, Madan L. Puri, and Ricardas Zitikis. 2009. L-functions, processes, and statistics in measuring economic inequality and actuarial risks. Statistics and Its Interface 2: 227–45. [Google Scholar] [CrossRef]
- Harsanyi, John C. 1953. Cardinal utility in welfare economics and in the theory of risk-taking. Journal of Political Economy 61: 434–35. [Google Scholar] [CrossRef]
- Imedio-Olmedo, Luis J., Elena Bárcena-Martín, and Encarnacion M. Parrado-Gallardo. 2011. A class of Bonferroni inequality indices. Journal of Public Economic Theory 13: 97–124. [Google Scholar] [CrossRef]
- Jaworski, Piotr, Fabrizio Durante, and Wolfgang Karl Härdle. 2013. Copulae in Mathematical and Quantitative Finance. Berlin: Springer. [Google Scholar]
- Jaworski, Piotr, Fabrizio Durante, Wolfgang Härdle, and Tomasz Rychlik. 2010. Copula Theory and Its Applications. Berlin: Springer. [Google Scholar]
- Jones, Bruce L., and Ricardas Zitikis. 2003. Empirical estimation of risk measures and related quantities. North American Actuarial Journal 7: 44–54. [Google Scholar] [CrossRef]
- Kakwani, Nanak C. 1980. Income Inequality and Poverty: Methods of Estimation and Policy Applications. New York: Oxford University Press. [Google Scholar]
- Kakwani, Nanak. 1980. On a class of poverty measures. Econometrica 48: 437–46. [Google Scholar] [CrossRef]
- Kakwani, N.C., and N. Podder. 1973. On the estimation of Lorenz curves from grouped observations. International Economic Review 14: 278–92. [Google Scholar] [CrossRef]
- Kenworthy, Lane, and Jonas Pontusson. 2005. Rising inequality and the politics of redistribution in affluent countries. Perspectives on Politics 3: 449–71. [Google Scholar] [CrossRef]
- Kleiber, Christian, and Samuel Kotz. 2003. Statistical Size Distributions in Economics and Actuarial Sciences. Hoboken: Wiley. [Google Scholar]
- Korpi, Walter, and Joakim Palme. 1998. The paradox of redistribution and strategies of equality: Welfare state institutions, inequality, and poverty in the Western countries. American Sociological Review 63: 661–87. [Google Scholar] [CrossRef]
- Kośny, Marek, and Gaston Yalonetzky. 2015. Relative income change and pro-poor growth. Economia Politica 32: 311–27. [Google Scholar] [CrossRef]
- Kovacevic, Milorad. 2010. Measurement of Inequality in Human Development—A Review. Human Development Research Paper 2010/35. New York, NY, USA: United Nations Development Programme. [Google Scholar]
- Lambert, Peter J. 2001. The Distribution and Redistribution of Income, 3rd ed. Manchester: Manchester University Press. [Google Scholar]
- Li, Haijun, and Xiaohu Li. 2013. Stochastic Orders in Reliability and Risk: In Honor of Professor Moshe Shaked. New York: Springer. [Google Scholar]
- Lorenz, M. O. 1905. Methods of measuring the concentration of wealth. Publications of the American Statistical Association 9: 209–19. [Google Scholar] [CrossRef]
- Machina, Mark J. 1987. Choice under uncertainty: Problems solved and unsolved. Economic Perspectives 1: 121–54. [Google Scholar] [CrossRef]
- Machina, Mark J. 2008. Non-expected utility theory. In The New Palgrave Dictionary of Economics, 2nd ed. Edited by S. N. Durlauf and L. E. Blume. New York: Palgrave Macmillan, pp. 74–84. [Google Scholar]
- McNeil, Alexander J., Rüdiger Frey, and Paul Embrechts. 2005. Quantitative Risk Management. Princeton: Princeton University Press. [Google Scholar]
- Mimoto, Nao, and Ricardas Zitikis. 2008. The Atkinson index, the Moran statistic, and testing exponentiality. Journal of the Japan Statistical Society 38: 187–205. [Google Scholar] [CrossRef]
- Meucci, Attilio. 2007. Risk and Asset Allocation. Berlin: Springer. [Google Scholar]
- Muliere, Pietro, and Marco Scarsini. 1989. A note on stochastic dominance and inequality measures. Journal of Economic Theory 49: 314–23. [Google Scholar] [CrossRef]
- Necir, Abdelhakim, and Djamel Meraghni. 2009. Empirical estimation of the proportional hazard premium for heavy-tailed claim amounts. Insurance: Mathematics and Economics 45: 49–58. [Google Scholar] [CrossRef]
- Necir, Abdelhakim, Djamel Meraghni, and Fatima Meddi. 2007. Statistical estimate of the proportional hazard premium of loss. Scandinavian Actuarial Journal 2007: 147–61. [Google Scholar] [CrossRef]
- Necir, Abdelhakim, Abdelaziz Rassoul, and Ricardas Zitikis. 2010. Estimating the conditional tail expectation in the case of heavy-tailed losses. Journal of Probability and Statistics 2010: 596839. [Google Scholar] [CrossRef]
- Nelsen, Roger B. 2006. An Introduction to Copulas, 2nd ed. New York: Springer. [Google Scholar]
- Nygård, Fredrik, and Arne Sandström. 1981. Measuring Income Inequality. Stockholm: Almqvist and Wiksell. [Google Scholar]
- Oladosu, Gbadebo, and Adam Rose. 2007. Income distribution impacts of climate change mitigation policy in the Susquehanna River Basin Economy. Energy Economics 29: 520–44. [Google Scholar] [CrossRef]
- Ostry, Jonathan D., Andrew Berg, and Charalambos G. Tsangarides. 2014. Redistribution, Inequality, and Growth. In IMF Staff Discussion Note SDN/14/02. Washington: International Monetary Fund. [Google Scholar]
- Palma, José Gabriel. 2006. Globalizing inEquality: ‘centrifugal’ and ‘centripetal’ Forces at Work. DESA Working Paper No 35. Washington, DC, USA: United Nations Department of Economics and Social Affairs. [Google Scholar]
- Pfähler, Wilhelm. 1990. Redistributive effect of income taxation: decomposing tax base and tax rates effects. Bulletin of Economic Research 42: 121–29. [Google Scholar] [CrossRef]
- Pflug, Georg Ch, and Werner Römisch. 2007. Modeling, Measuring and Managing Risk. Singapore: World Scientific. [Google Scholar]
- Pietra, Gaetano. 1915. On the relationship between variability indices (Note I). (English translation from Italian by P. Brutti and S. Gubbiotti). Metron 72: 5–16. [Google Scholar] [CrossRef]
- Piketty, Thomas. 2014. Capital in the Twenty-First Century. Cambridge: Harvard University Press. [Google Scholar]
- Puppe, Clemens. 1991. Distorted Probabilities and Choice under Risk. Berlin: Springer. [Google Scholar]
- Quiggin, John. 1982. A theory of anticipated utility. Journal of Economic Behavior and Organization 3: 323–43. [Google Scholar] [CrossRef]
- Quiggin, John. 1993. Generalized Expected Utility Theory: The Rank-Dependent Model. Dordrecht: Kluwer. [Google Scholar]
- Ramos, Hector M., Jorge Ollero, and Miguel A. Sordo. 2000. A sufficient condition for generalized Lorenz order. Journal of Economic Theory 90: 286–92. [Google Scholar] [CrossRef]
- Rassoul, Abdelaziz. 2013. Kernel-type estimator of the conditional tail expectation for a heavy-tailed distribution. Insurance: Mathematics and Economics 53: 698–703. [Google Scholar] [CrossRef]
- Rawls, John. 1971. A Theory of Justice. Cambridge: Harvard University Press. [Google Scholar]
- Roemer, John E. 2013. Economic development as opportunity equalization. World Bank Economic Review 28: 189–209. [Google Scholar] [CrossRef]
- Sadoulet, Elisabeth, and Alain de Janvry. 1995. Quantitative Development Policy Analysis. Baltimore: John Hopkins University Press. [Google Scholar]
- Sandström, Arne. 2010. Handbook of Solvency for Actuaries and Risk Managers: Theory and Practice. Boca Raton: Chapman and Hall. [Google Scholar]
- Sarabia, José María. 2008. Parametric Lorenz curves: Models and applications. In Modeling Income Distributions and Lorenz Curves. Edited by D. Chotikapanich. Berlin: Springer, pp. 167–90. [Google Scholar]
- Sarabia, José María, Faustino Prieto, and María Sarabia. 2010. Revisiting a functional form for the Lorenz curve. Economics Letters 107: 249–52. [Google Scholar] [CrossRef]
- Schmeidler, David. 1986. Integral representation without additivity. Proceedings of the American Mathematical Society 97: 255–61. [Google Scholar] [CrossRef]
- Schmeidler, David. 1989. Subjective probability and expected utility without additivity. Econometrica 57: 571–87. [Google Scholar] [CrossRef]
- Sen, Amartya. 1983. Poor, relatively speaking. Oxford Economic Papers 35: 153–69. [Google Scholar] [CrossRef]
- Sen, Amartya. 1997. On Economic Inequality (expanded Edition With a Substantial Annexe by J. E. Foster and A. Sen). Oxford: Clarendon Press. [Google Scholar]
- Sen, Amartya. 1998. Choice, Welfare and Measurement. Cambridge: Harvard University Press. [Google Scholar]
- Shaked, Moshe, and J. George Shanthikumar. 2007. Stochastic Orders. New York: Springer. [Google Scholar]
- Shorrocks, Anthony. 1978. Income inequality and income mobility. Journal of Economic Theory 19: 376–93. [Google Scholar] [CrossRef]
- Silber, Jacques. 1999. Handbook on Income Inequality Measurement. Boston: Kluwer. [Google Scholar]
- Slemrod, Joel. 1992. Taxation and inequality: A time-exposure perspective. In Tax Policy and the Economy. Edited by J. M. Poterba. Chicago: University of Chicago Press, vol. 6, pp. 105–27. [Google Scholar]
- Sordo, Miguel A., and Alfonso Suárez-Llorens. 2011. Stochastic comparisons of distorted variability measures. Insurance: Mathematics and Economics 49: 11–17. [Google Scholar] [CrossRef]
- Sordo, Miguel A., Jorge Navarro, and José María Sarabia. 2014. Distorted Lorenz curves: Models and comparisons. Social Choice and Welfare 42: 761–80. [Google Scholar] [CrossRef]
- Sriboonchita, Songsak, Wing-Keung Wong, Sompong Dhompongsa, and Hung T. Nguyen. 2010. Stochastic Dominance and Applications to Finance, Risk and Economics. Boca Raton: Chapman and Hall/CRC. [Google Scholar]
- Tarsitano, Agostino. 1990. The Bonferroni index of income inequality. In Income and Wealth Distribution, Inequality and Poverty. Edited by C. Dagum and M. Zenga. New York: Springer, pp. 228–42. [Google Scholar]
- Tarsitano, Agostino. 2004. A new class of inequality measures based on a ratio of L-statistics. Metron 62: 137–60. [Google Scholar]
- Thompson, W. A., Jr. 1976. Fisherman’s luck. Biometrics 32: 265–71. [Google Scholar] [CrossRef]
- Van De Ven, Justin, John Creedy, and Peter J. Lambert. 2001. Close equals and calculation of the vertical, horizontal and reranking effects of taxation. Oxford Bulletin of Economics and Statistics 63: 381–94. [Google Scholar] [CrossRef]
- Vergnaud, J. C. 1997. Analysis of risk in a non expected utility framework and application to the optimality of the deductible. Revue Finance 18: 155–67. [Google Scholar]
- Wang, Shaun. 1995. Insurance pricing and increased limits ratemaking by proportional hazards transforms. Insurance: Mathematics and Economics 17: 43–54. [Google Scholar] [CrossRef]
- Wang, Shaun. 1998. An actuarial index of the right-tail risk. North American Actuarial Journal 2: 88–101. [Google Scholar] [CrossRef]
- Wang, Shaun S., and Virginia R. Young. 1998. Ordering risks: Expected utility theory versus Yaari’s dual theory of risk. Insurance: Mathematics and Economics 22: 145–61. [Google Scholar] [CrossRef]
- Wang, Shaun S., Virginia R. Young, and Harry H. Panjer. 1997. Axiomatic characterization of insurance prices. Insurance: Mathematics and Economics 21: 173–83. [Google Scholar] [CrossRef]
- Weymark, John A. 1981. Generalized Gini inequality indices. Mathematical Social Sciences 1: 409–30. [Google Scholar] [CrossRef]
- Weymark, John. A. 2003. Generalized Gini indices of equality of opportunity. Journal of Economic Inequality 1: 5–24. [Google Scholar] [CrossRef]
- Wirch, Julia Lynn, and Mary R. Hardy. 1999. A synthesis of risk measures for capital adequacy. Insurance: Mathematics and Economics 25: 337–47. [Google Scholar]
- Yaari, Menahem E. 1987. The dual theory of choice under risk. Econometrica 55: 95–115. [Google Scholar] [CrossRef]
- Yitzhaki, Shlomo. 1979. Relative deprivation and the Gini coefficient. Quarterly Journal of Economics 93: 321–24. [Google Scholar] [CrossRef]
- Yitzhaki, Shlomo. 1982. Stochastic dominance, mean variance, and Gini’s mean difference. American Economic Review 72: 178–85. [Google Scholar]
- Yitzhaki, Shlomo. 1983. On an extension of the Gini inequality index. International Economic Review 24: 617–28. [Google Scholar] [CrossRef]
- Yitzhaki, Shlomo. 1994. On the progressivity of commodity taxation. In Models and Measurement of Welfare and Inequality. Edited by W. Eichhorn. Berlin: Springer, pp. 448–66. [Google Scholar]
- Yitzhaki, Shlomo. 1998. More than a dozen alternative ways of spelling Gini. Research on Economic Inequality 8: 13–30. [Google Scholar]
- Yitzhaki, Shlomo. 2003. Gini’s mean difference: A superior measure of variability for non-normal distributions. Metron 51: 285–16. [Google Scholar]
- Yitzhaki, Shlomo, and Edna Schechtman. 2013. The Gini Methodology: A Primer on a Statistical Methodology. New York: Springer. [Google Scholar]
- Zenga, Michele. 2007. Inequality curve and inequality index based on the ratios between lower and upper arithmetic means. Statistica and Applicazioni 5: 3–27. [Google Scholar]
- Zenga, Michele. 2010. Mixture of Polisicchio’s truncated Pareto distributions with beta weights. Statistica and Applicazioni 8: 3–25. [Google Scholar]
- Zenga, Michele, Leo Pasquazzi, M. Polisicchio, and Mariangela Zenga. 2011. More on M. M. Zenga’s new three-parameter distribution for non-negative variables. Statistica and Applicazioni 9: 5–33. [Google Scholar]
- Zenga, Michele, Leo Pasquazzi, and Mariangela Zenga. 2012. First applications of a new three-parameter distribution for non-negative variables. Statistica and Applicazioni 10: 131–47. [Google Scholar]
- Zitikis, Ricardas. 2002. Analysis of indices of economic inequality from a mathematical point of view. Matematika 8: 772–82. [Google Scholar]
- Zitikis, Ricardas. 2003. Asymptotic estimation of the E-Gini index. Econometric Theory 19: 587–601. [Google Scholar] [CrossRef]
- Zoli, Claudio. 1999. A generalized version of the inequality equivalence criterion: A surplus sharing characterization, complete and partial orderings. In Logic, Game Theory and Social Choice. Edited by H. C. M. de Swart. Tilburg: Tilburg University Press, pp. 427–41. [Google Scholar]
- Zoli, Claudio. 2012. Characterizing Inequality Equivalence Criteria. Working Paper 32. Verona, Italy: Department of Economics, University of Verona. [Google Scholar]

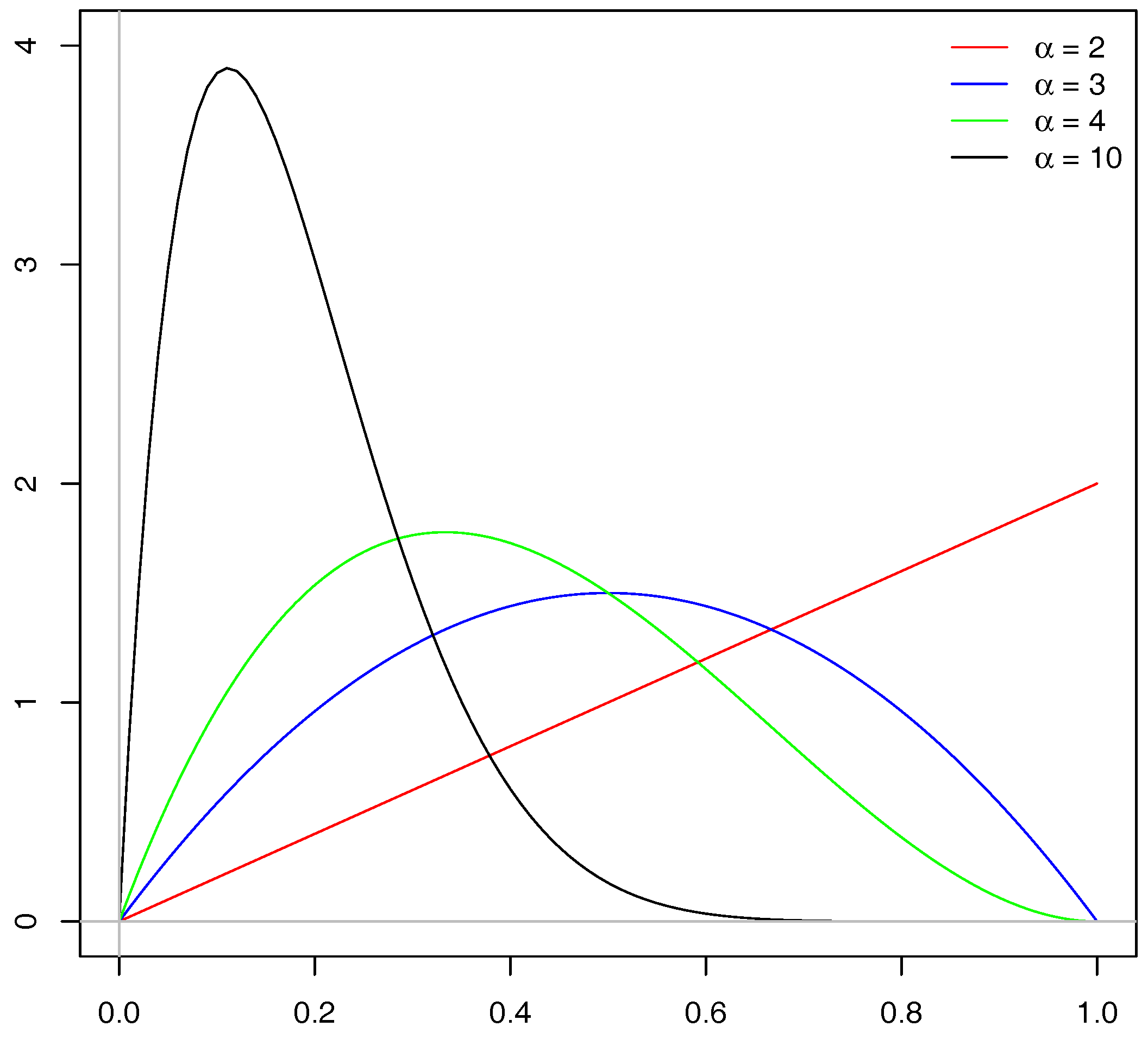
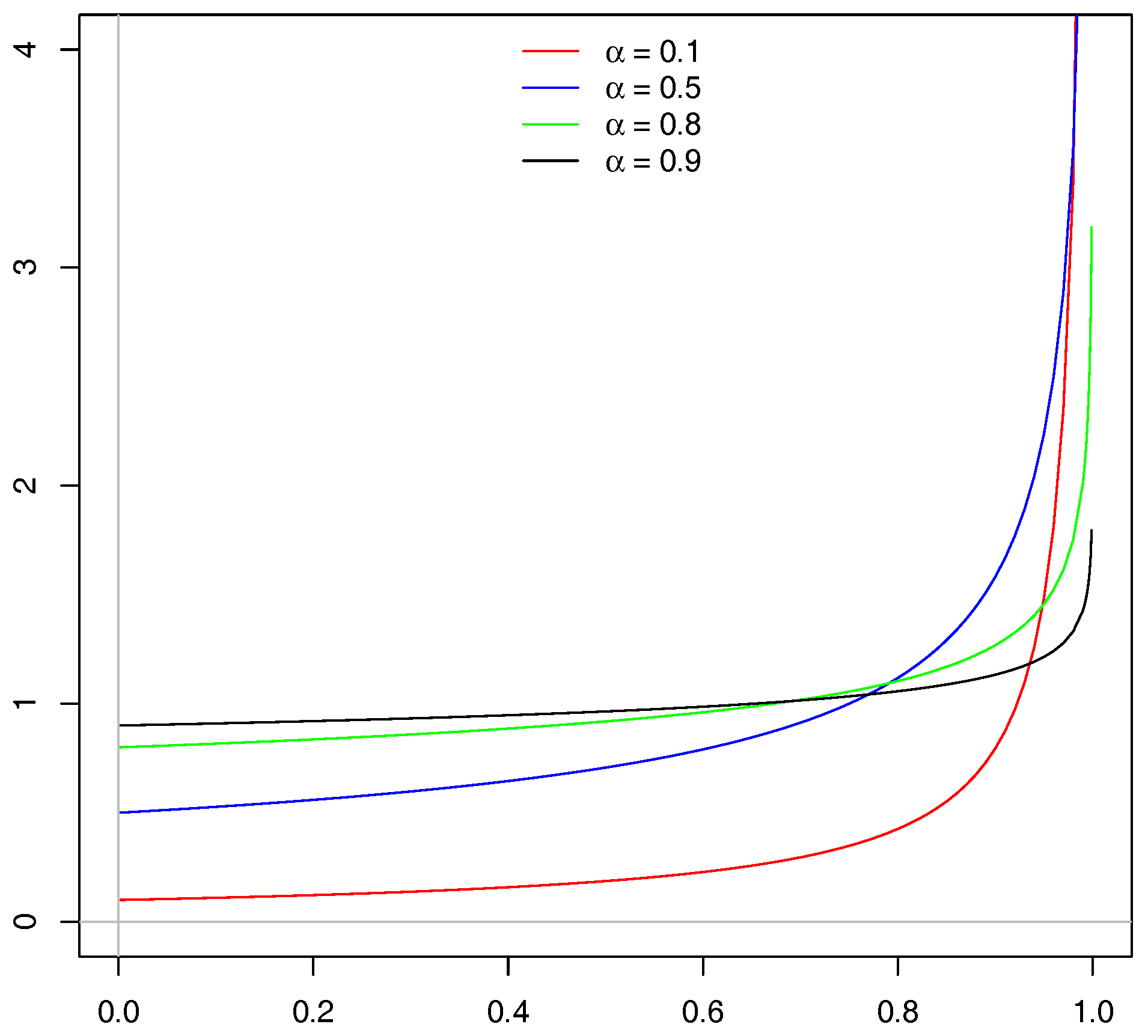
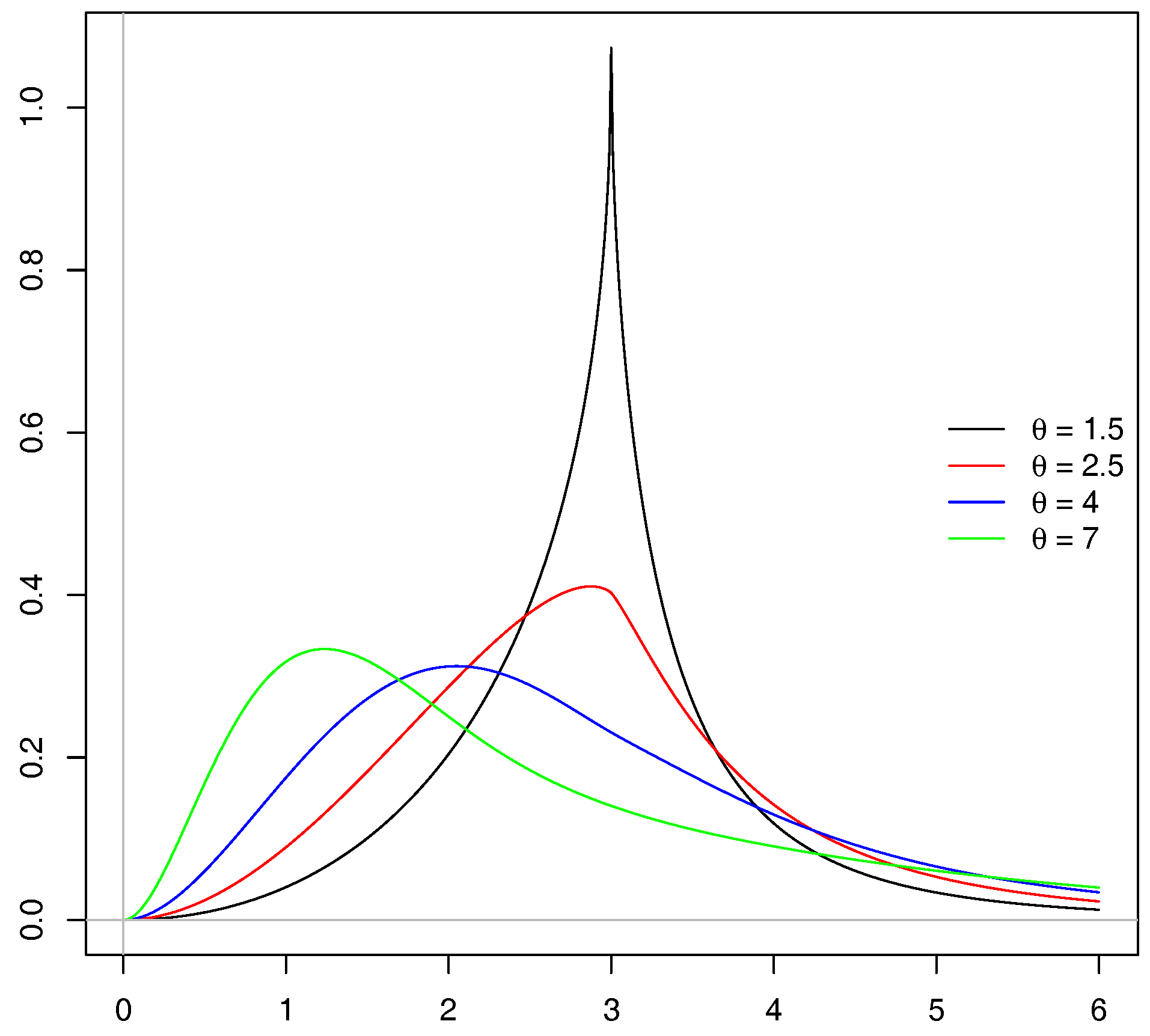
| Atkinson | 1 | 0 | |||
| Bonferroni | 0 | x | x | ||
| Chakravarty | 0 | x | |||
| Inequality index | 0 | x | |||
| Cowell | 1 | 0 | |||
| Cowell’s Generalized Entropy class | 1 | 0 | linear | ||
| Donaldson-Weymark-Kakwani | 0 | x | x | ||
| Inequality index | 0 | x | x | ||
| Gini | 0 | x | x | ||
| Palma | x | x | |||
| Risk measure | Any | x | x | ||
| Wang | 1 | x | |||
| Proportional hazards transform | 1 | x | |||
| Zenga | x | x |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Greselin, F.; Zitikis, R. From the Classical Gini Index of Income Inequality to a New Zenga-Type Relative Measure of Risk: A Modeller’s Perspective. Econometrics 2018, 6, 4. https://doi.org/10.3390/econometrics6010004
Greselin F, Zitikis R. From the Classical Gini Index of Income Inequality to a New Zenga-Type Relative Measure of Risk: A Modeller’s Perspective. Econometrics. 2018; 6(1):4. https://doi.org/10.3390/econometrics6010004
Chicago/Turabian StyleGreselin, Francesca, and Ričardas Zitikis. 2018. "From the Classical Gini Index of Income Inequality to a New Zenga-Type Relative Measure of Risk: A Modeller’s Perspective" Econometrics 6, no. 1: 4. https://doi.org/10.3390/econometrics6010004
APA StyleGreselin, F., & Zitikis, R. (2018). From the Classical Gini Index of Income Inequality to a New Zenga-Type Relative Measure of Risk: A Modeller’s Perspective. Econometrics, 6(1), 4. https://doi.org/10.3390/econometrics6010004






