Ionization Cross Sections in the Collision between Two Ground State Hydrogen Atoms at Low Energies
Abstract
1. Introduction
2. Theory
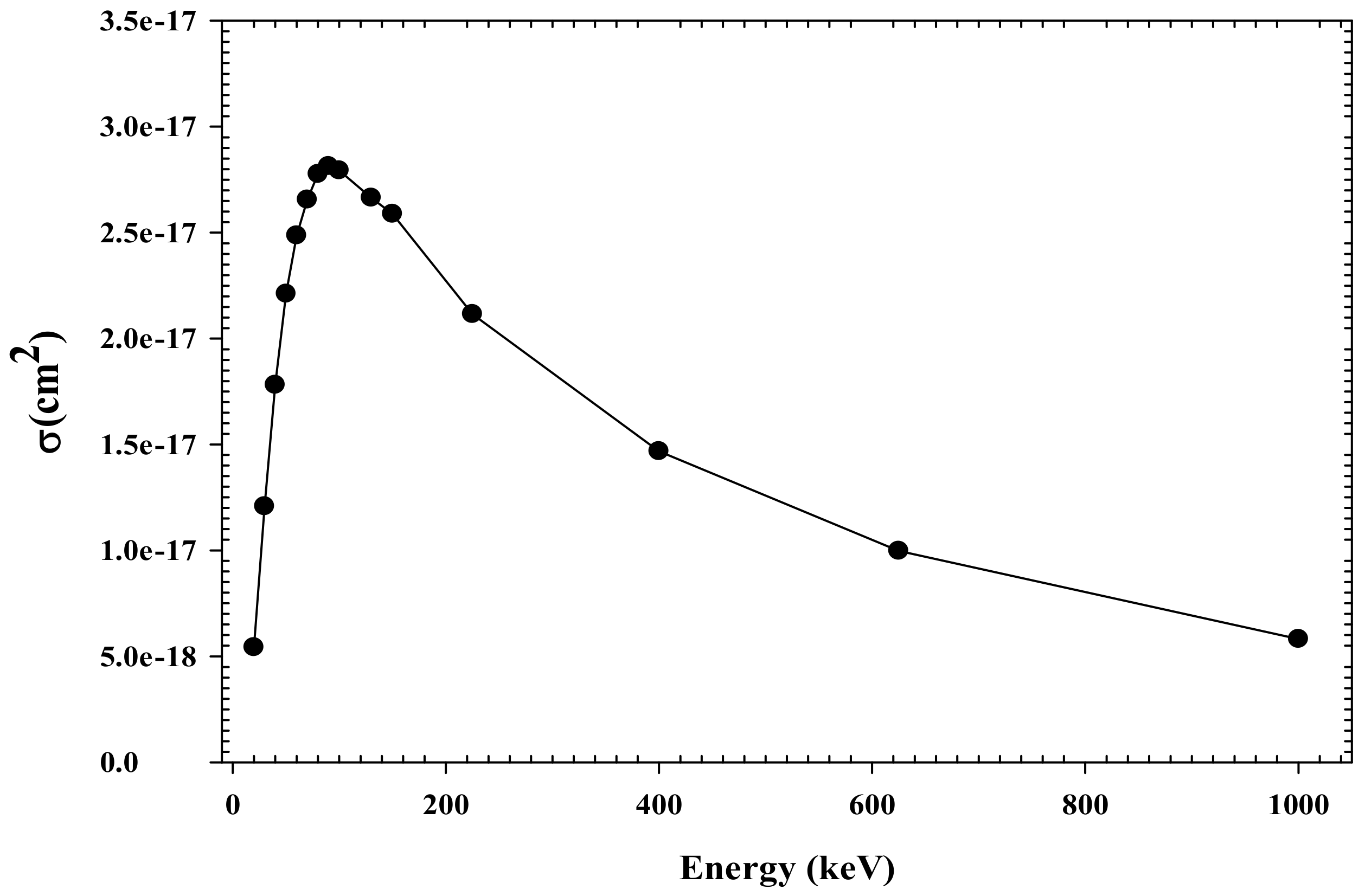
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Thomas, D.M.; McKee, G.R.; Burrell, K.H.; Levinton, F.; Foley, E.L.; Fisher, R.K. Chapter 6: Active Spectroscopy. Fusion Sci. Technol. 2008, 53, 487–527. [Google Scholar] [CrossRef]
- Guszejnov, D.; Pokol, G.I.; Pusztai, I.; Refy, D.; Zoletnik, S.; Lampert, M.; Nam, Y.U. Three-dimensional modeling of beam emission spectroscopy measurements in fusion plasmas. Rev. Sci. Instrum. 2012, 83, 113501. [Google Scholar] [CrossRef] [PubMed]
- Wolfrum, E.; Beurskens, M.; Dunne, M.G.; Frassinetti, L.; Gao, X.; Giroud, C.; Hughes, J.; Lunt, T.; Maingi, R.; Osborne, T.; et al. Impact of wall materials and seeding gases on the pedestal and on core plasma performance. Nucl. Mater. Energy 2017, 12, 18–27. [Google Scholar] [CrossRef]
- Tőkési, K.; Hock, G. Versatility of the exit channels in the three-body CTMC method. Nucl. Instrum. Methods Phys. Res. B 1994, 86, 201–204. [Google Scholar] [CrossRef]
- Reinhold, C.O.; Falcon, C.A. Classical ionization and charge-transfer cross sections for H+ + He and H+ + Li+ collisions with consideration of model interactions. Phys. Rev. A 1986, 33, 3859–3866. [Google Scholar] [CrossRef] [PubMed]
- Schultz, D.R.; Olson, R.E. Single-electron--removal processes in collisions of positrons and protons with helium at intermediate velocities. Phys. Rev. A 1988, 38, 1866–1876. [Google Scholar] [CrossRef] [PubMed]
- Schultz, D.R. Comparison of single-electron removal processes in collisions of electrons, positrons, protons, and antiprotons with hydrogen and helium. Phys. Rev. A 1989, 40, 2330–2334. [Google Scholar] [CrossRef] [PubMed]
- Schultz, D.R.; Reinhold, C.O.; Olson, R.E. Large-angle scattering in positron-helium and positron-krypton collisions. Phys. Rev. A 1989, 40, 4947–4958. [Google Scholar] [CrossRef] [PubMed]
- Schultz, D.R.; Meng, L.; Olson, R.E. Classical description and calculation of ionization in collisions of 100 eV electrons and positrons with He and H2. J. Phys. B At. Mol. Opt. Phys. 1992, 25, 4601–4618. [Google Scholar] [CrossRef]
- Acebal, E.; Otranto, S. Influence of the projectile charge sign in light particle single ionization of H2O. Eur. Phys. J. D 2019, 73, 91. [Google Scholar] [CrossRef]
- Oliveira, V.; Herbert, A.; Santos, A.; Tőkési, K. Electron capture and loss of O+ projectile in collision with water near the Bragg Peak Energies. Eur. Phys. J. D 2019, 73, 146. [Google Scholar] [CrossRef]
- Tőkési, K.; DuBois, R.D.; Mukoyama, T. Interaction of positronium with helium atoms—The classical treatment of the 5-body collision system. Eur. Phys. J. D 2014, 68, 255. [Google Scholar] [CrossRef]
- Tökési, K.; Wang, J.; Olson, R.E. Projectile ionization at forward observation angles for intermediate energy H + H collisions. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 1994, 86, 147–150. [Google Scholar] [CrossRef]
- Bailey, J.J.; Abdurakhmanov, I.B.; Kadyrov, A.S.; Bray, I.; Mukhamedzhanov, A.M. Proton-beam stopping in hydrogen. Phys. Rev. A 2019, 99, 042701. [Google Scholar] [CrossRef]
- McClure, G. Ionization and Electron Transfer in Collisions of Two H Atoms: 1.25–117 keV. Phys. Rev. 1968, 166, 22–29. [Google Scholar] [CrossRef]
- Wittkower, A.B.; Levy, G.; Gilbody, H.B. An experimental study of electron loss during the passage of fast hydrogen atoms through atomic hydrogen. Proc. Phys. Soc. 1967, 91, 306–309. [Google Scholar] [CrossRef]
- Flannery, M. Excitation and Ionization of Hydrogen by Hydrogen-Atom Impact. J. Phys. B At. Mol. Phys 1970, 3, L97–L100. [Google Scholar] [CrossRef]
- Bates, D.R.; Griffing, R.G.W. Inelastic Collisions between Heavy Particles I: Excitation and Ionization of Hydrogen Atoms in Fast Encounters with Protons and with other Hydrogen Atoms. Proc. Phys. Soc. A 1953, 67, 961–971. [Google Scholar] [CrossRef]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Atawneh, S.J.A.; Asztalos, Ö.; Szondy, B.; Pokol, G.I.; Tőkési, K. Ionization Cross Sections in the Collision between Two Ground State Hydrogen Atoms at Low Energies. Atoms 2020, 8, 31. https://doi.org/10.3390/atoms8020031
Atawneh SJA, Asztalos Ö, Szondy B, Pokol GI, Tőkési K. Ionization Cross Sections in the Collision between Two Ground State Hydrogen Atoms at Low Energies. Atoms. 2020; 8(2):31. https://doi.org/10.3390/atoms8020031
Chicago/Turabian StyleAtawneh, Saed J. Al, Örs Asztalos, Borbála Szondy, Gergő I. Pokol, and Károly Tőkési. 2020. "Ionization Cross Sections in the Collision between Two Ground State Hydrogen Atoms at Low Energies" Atoms 8, no. 2: 31. https://doi.org/10.3390/atoms8020031
APA StyleAtawneh, S. J. A., Asztalos, Ö., Szondy, B., Pokol, G. I., & Tőkési, K. (2020). Ionization Cross Sections in the Collision between Two Ground State Hydrogen Atoms at Low Energies. Atoms, 8(2), 31. https://doi.org/10.3390/atoms8020031






